Comparison of Strengthening Solutions with Optimized Passive Energy Dissipation Systems in Symmetric Buildings
Abstract
:1. Introduction
2. Description of Benchmark Investigated Buildings
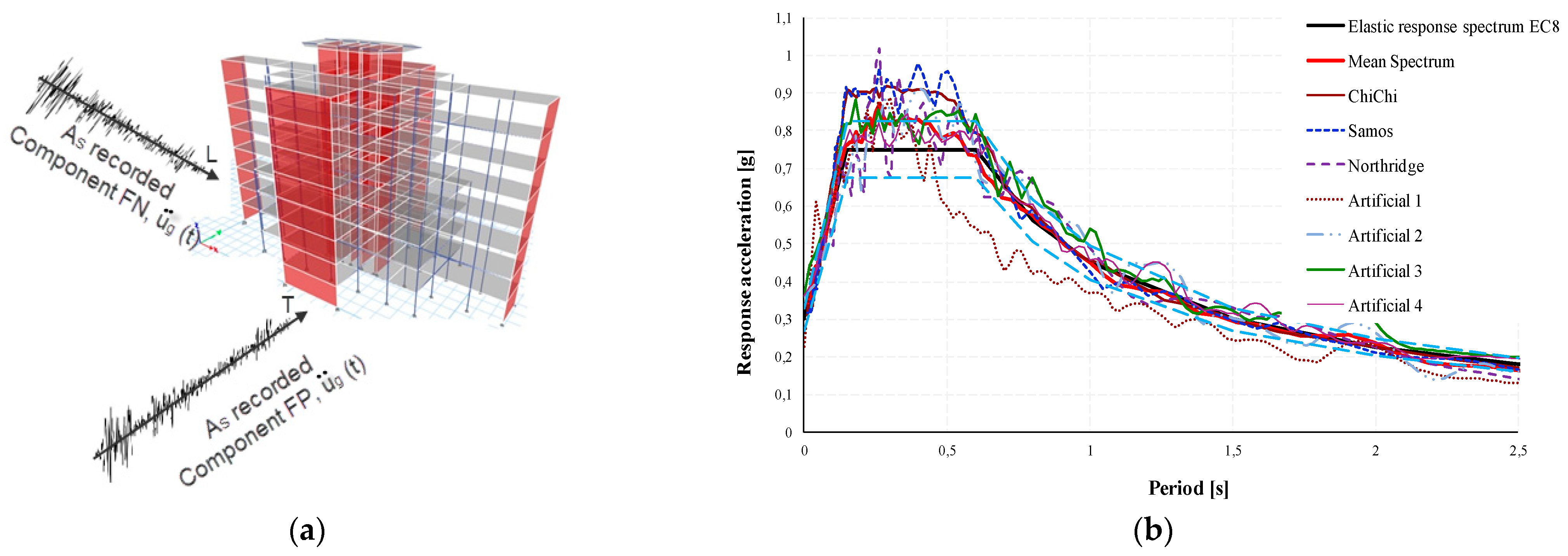
3. Building’s Modeling
4. Optimal Design of Passive Energy Dissipation Systems
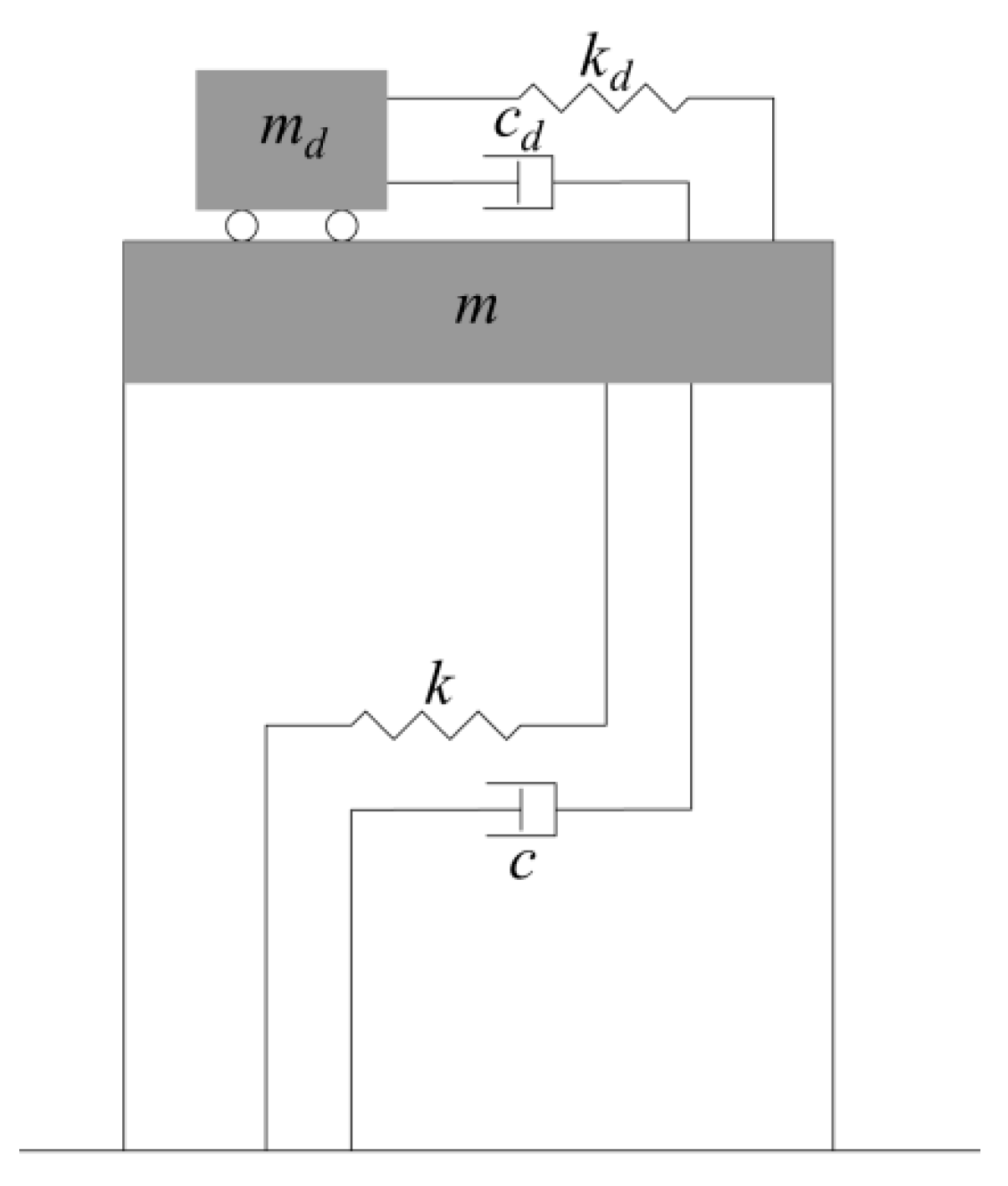
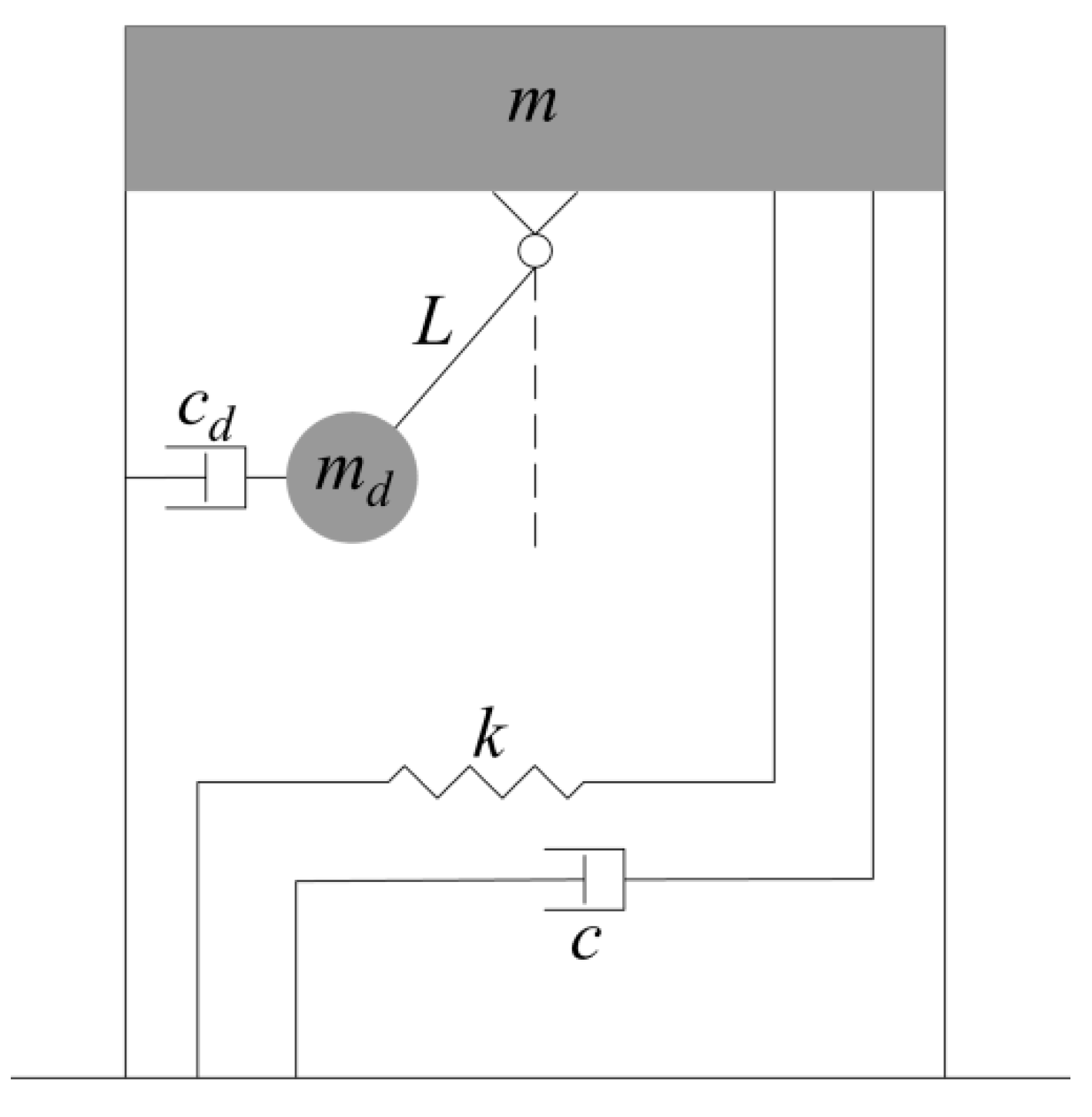
4.1. Tuned Mass Damper
4.2. Viscous Dampers (VDs)
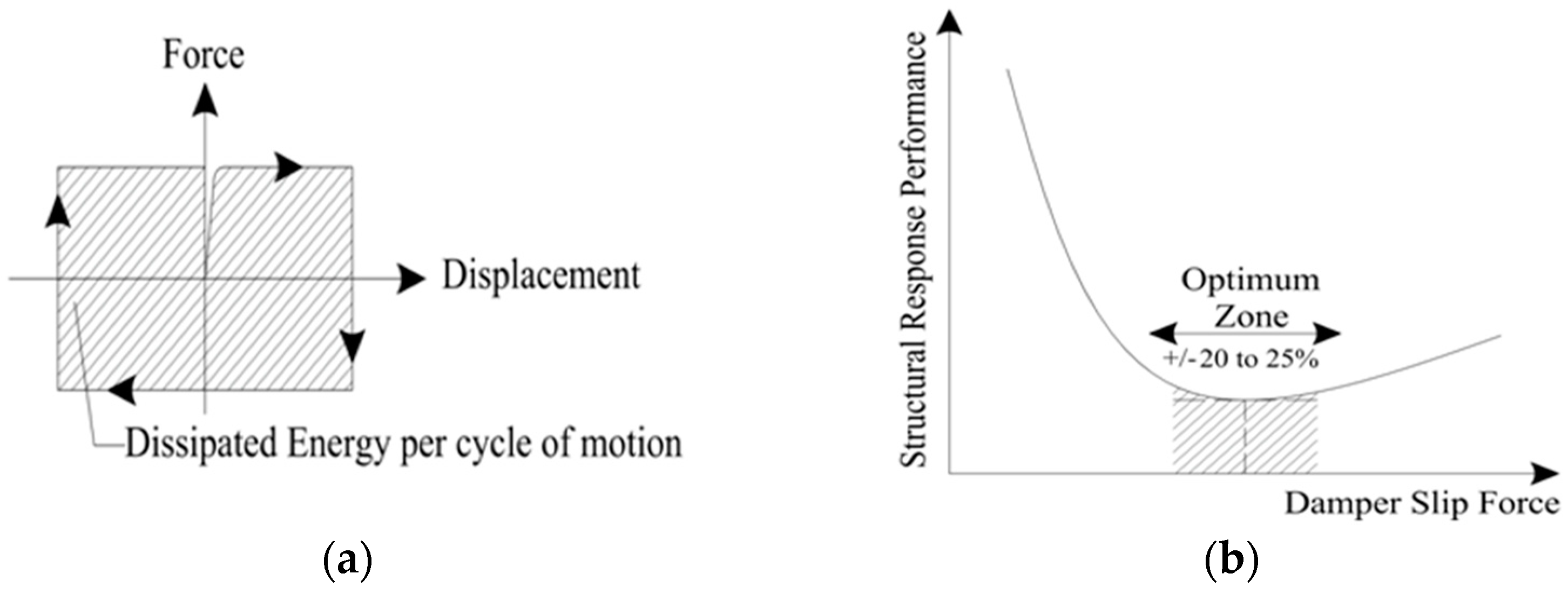
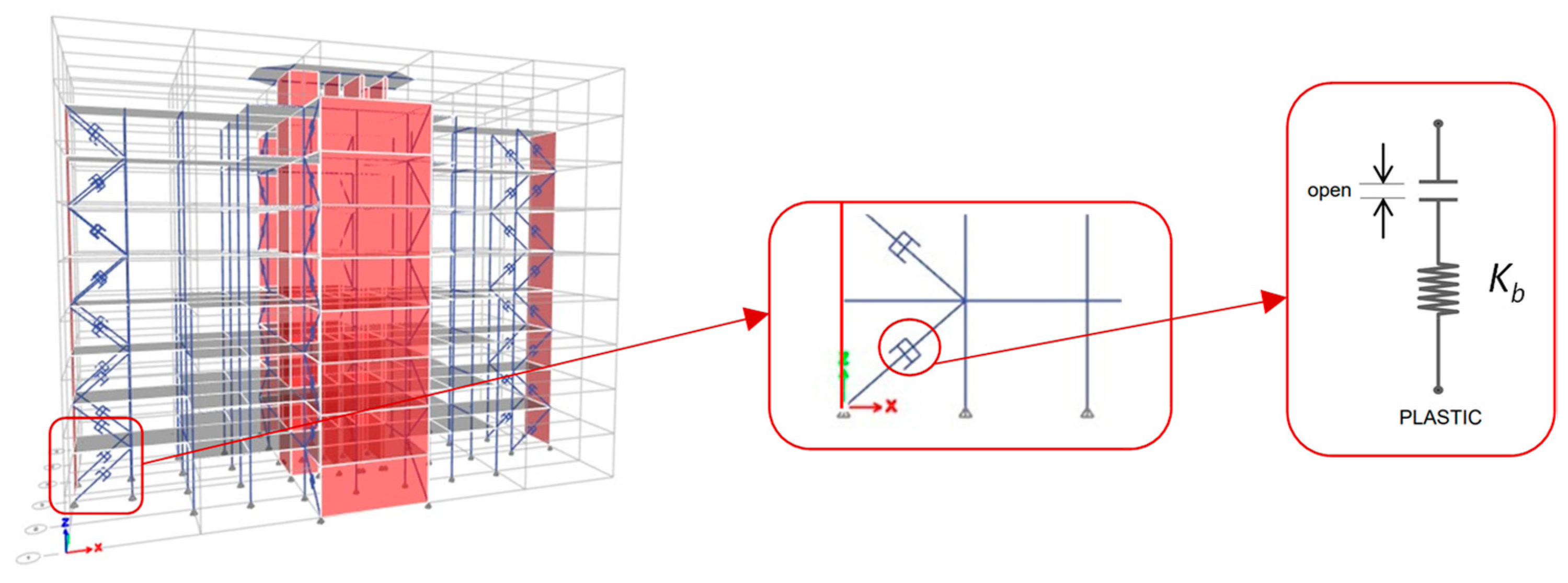
4.3. Friction Dampers (FDs)
- Force independent of the apparent contact surface
- Force proportional to the total normal force acting through the interface
- Force independent of speed even with a slip at low speed
5. Results and Discussion
5.1. Displacement at the Top of the Structures
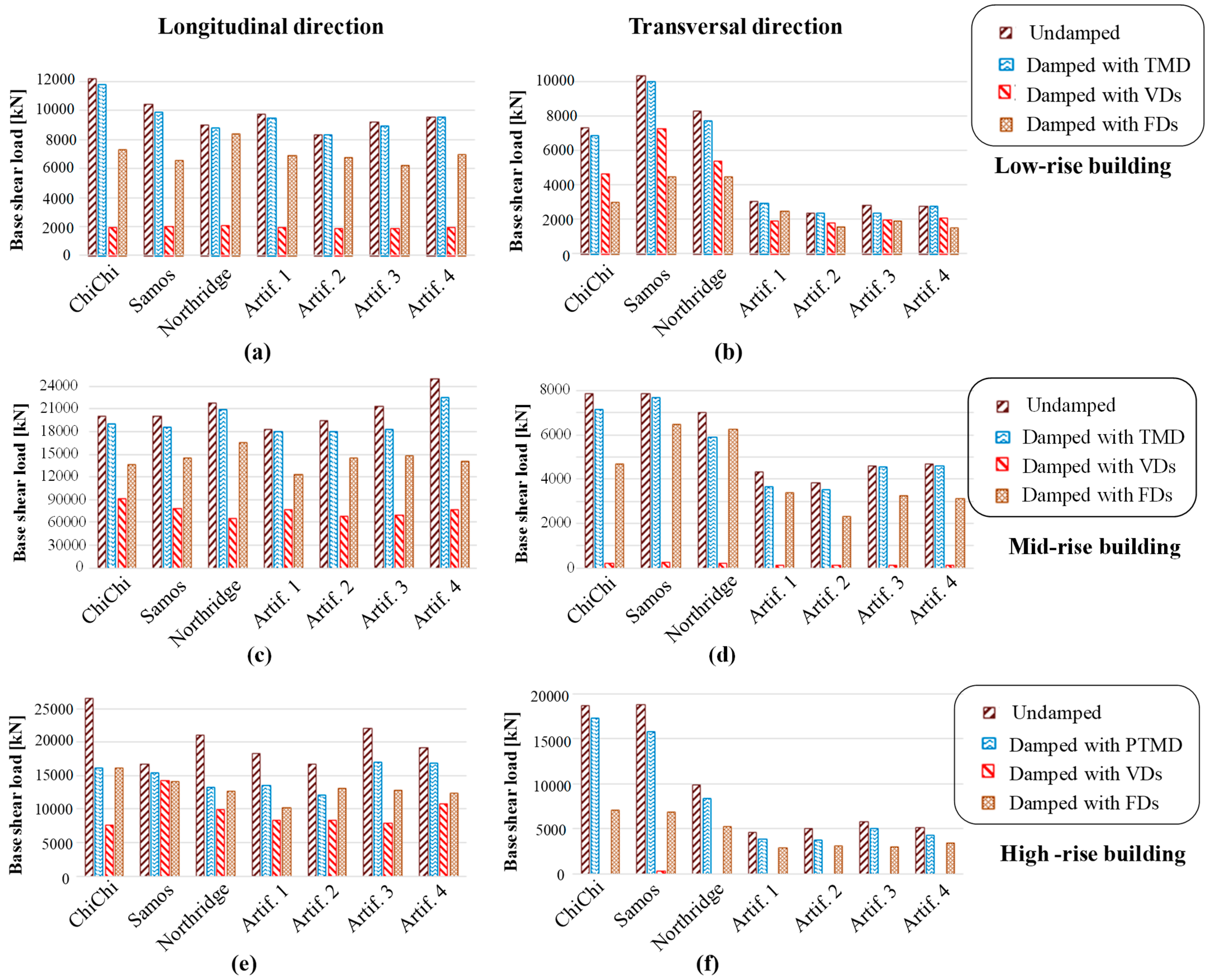
5.2. Base Shear Load
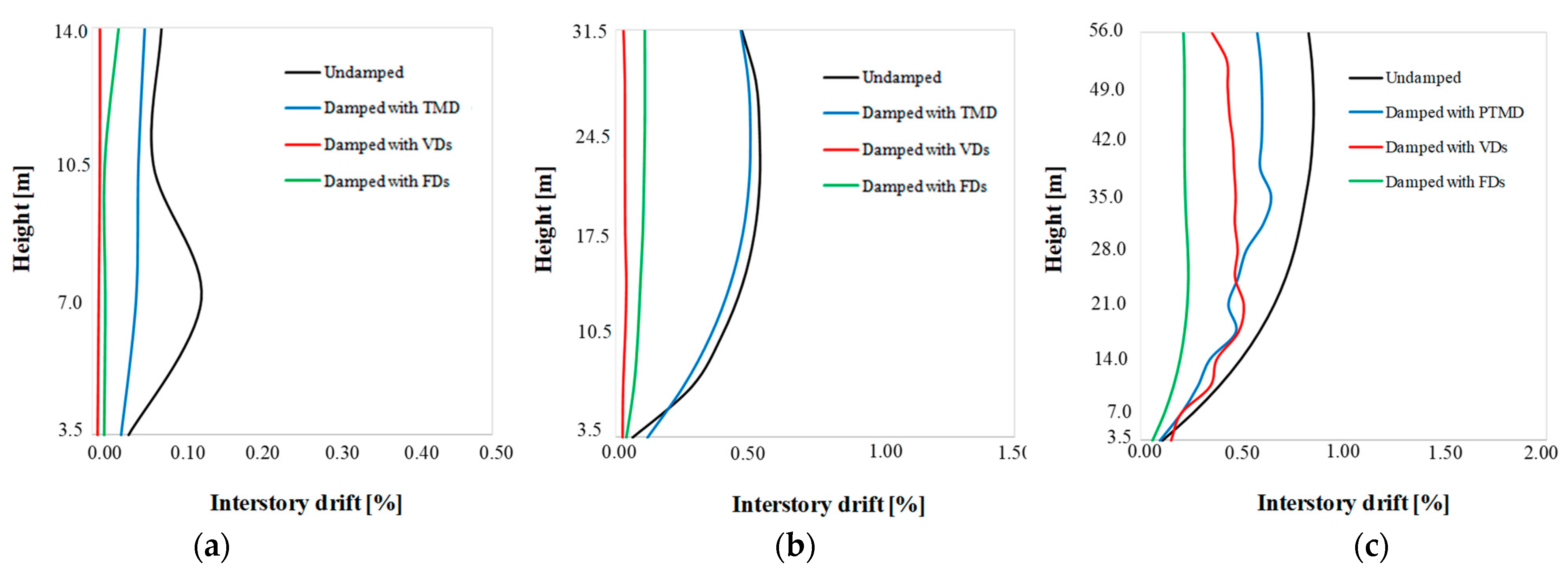
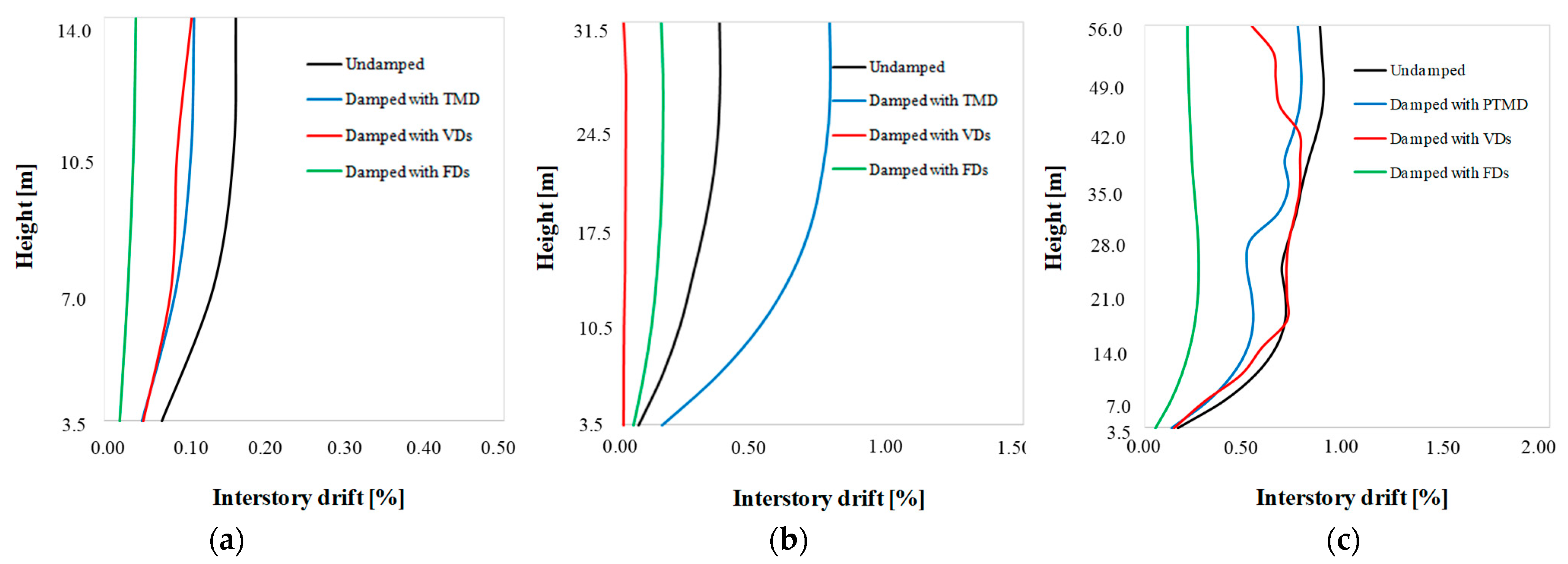
5.3. Interstory Drift
5.4. Hysteretic Loops
5.5. General Remarks
- It is well known that the structural response reduction increases as the mass of TMD increases, but this mass has a limit in practice, due to geometrical and economical constraints. That is the reason why the mass ratio is not considered as an important value to optimize, and therefore, it is hard to achieve high reduction values practically. The results show that TMD systems are not effective for low and mid-rise buildings, because both the displacement and base shear values are barely affected, unlike high-rise building values. In fact, TMD are motion-based systems that demonstrate how their effectiveness is very limited for rigid buildings. As for the high-rise building, even though damped case with PTMD provides less reduction compared to the two other damped cases, it is considered acceptable and more suitable for this kind of structure.
- Structural strengthening with viscous damper systems is defined by the desired additional damping fixed in the preliminary design. From the results obtained, it has been observed that the structural response with the viscus dampers decreases well, showing better performance in terms of the displacement and base shear. In addition, viscous dampers are velocity-dependent systems, where its effectiveness increases with high velocities, usually for flexible buildings. Even though these systems are considered effective for the three studied buildings, they are considered more suitable for mid-rise buildings.
- Friction dampers’ incorporation into the structures reduces considerably the building’s response after optimizing dampers slip forces, their numbers, and locations under all earthquakes and types of buildings considered. It can be seen from the results obtained that the friction dampers are effective for both rigid and flexible buildings.
6. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Constantinou, M.C.; Soong, T.T.; Dargush, G.F. Passive Energy Dissipation Systems for Structural Design and Retrofit; MCEER Monograph Series, No; Multidisciplinary center for Earthquake Engineering Research: Buffalo, NY, USA, 1998. [Google Scholar]
- Soong, T.T.; Spencer, B.F., Jr. Supplemental energy dissipation: State-of-the-art and state-of-the-practice. Eng. Struct. 2002, 24, 243–259. [Google Scholar] [CrossRef]
- Symans, M.D.; Charney, F.A.; Whittaker, A.S.; Constantinou, M.C.; Kircher, C.A.; Johnson, M.W.; McNamara, R.J. Energy dissipation system for seismic applications: Current practice and recent developments. J. Struct. Eng. 2008, 134, 3–21. [Google Scholar] [CrossRef] [Green Version]
- Frahm, H. Device for Damping Vibrations of Bodies. U.S. Patent No. 989,958, 30 October 1909. [Google Scholar]
- Ormondroyd, J.; Den Hartog, J.P. The theory of the dynamic vibration absorber. ASME J. Appl. Mech. 1928, 50, 9–22. [Google Scholar]
- Den Hartog, J.P. Mechanical Vibrations, 2nd ed.; McGraw-Hill: London, UK, 1940. [Google Scholar]
- Kwok, K.C.S.; Macdonald, P.A. Full-scale measurements of wind-induced acceleration response of Sydney tower. Eng. Struct. 1990, 12, 153–162. [Google Scholar] [CrossRef]
- McNamara, R.J. Tuned mass dampers for buildings. J. Struct. Div. 1977, 103, 1785–1798. [Google Scholar] [CrossRef]
- Khan, F.R. 100 Storey John Hancock Center, Chicago: A case study of the design process. Eng. Struct. 1983, 5, 10–14. [Google Scholar] [CrossRef]
- Kawabata, S.; Ohkuma, T.; Kanda, J.; Kitamura, H.; Ohtake, K. Chiba Port Tower. Full-scale measurement of wind actions Part 2. Basic properties of fluctuating wind pressures. J. Wind Eng. Indust. Aerodyn. 1990, 33, 253–262. [Google Scholar] [CrossRef]
- Ueda, T.; Nakagaki, R.; Koshida, K. Suppression of Wind-Induced Vibration of Tower-Shaped Structures by Dynamic Dampers. Struct. Eng. Inter. 1993, 3, 50–53. [Google Scholar] [CrossRef]
- Constantinou, M.C.; Symans, M.D. Experimental study of seismic response of buildings with supplemental fluid dampers. Struct. Des. Tall Build. 1993, 2, 93–132. [Google Scholar] [CrossRef]
- Larbi, W. Numerical modeling of sound and vibration reduction using viscoelastic materials and shunted piezoelectric patches. Comput. Struct. 2020, 232, 105822. [Google Scholar] [CrossRef]
- Larbi, W.; Deü, J.-F.; Ohayon, R. Vibroacoustic analysis of double-wall sandwich panels with viscoelastic core. Comput. Struct. 2016, 174, 92–103. [Google Scholar] [CrossRef]
- Keel, C.J.; Mahmoodi, P. Designing of Viscoelastic Dampers for Columbia Center Building. In Build Motion in Wind; Isyumov, N., Tschanz, T., Eds.; ASCE: New York, NY, USA, 1986; pp. 66–82. [Google Scholar]
- Tsopelas, P.; Constantinou, M.C. Experimental and Analytical Study of a System Consisting of Sliding Bearings and Fluid Restoring Force/Damping Devices; National Center for Earthquake Engineering Research: Taipei, China, 1994. [Google Scholar]
- Moreschi, L.M.; Singh, M.P. Design of yielding metallic and friction dampers for optimal seismic performance. Earthq. Eng. Struct. Dynam. 2003, 32, 1291–1311. [Google Scholar] [CrossRef]
- Pall, A.S.; Marsh, C.; Fazio, P. Friction joints for seismic control of large panel structures. J. Prestress. Concr. Inst. 1980, 25, 38–61. [Google Scholar] [CrossRef]
- Pall, A.S.; Marsh, C. Response of friction damped braced frames. J. Struct. Div. ASCE 1982, 108, 1313–1323. [Google Scholar] [CrossRef]
- Whittaker, A.S.; Bertero, V.V.; Thompson, C.L.; Alonso, J.L. Seismic testing of steel plate energy dissipating devices. Earthq. Spectra 1991, 7, 563–604. [Google Scholar] [CrossRef]
- Grigorian, C.E.; Yang, T.S.; Popov, E.P. Slotted bolted connection energy dissipators. Earth. Spectra 1993, 9, 491–504. [Google Scholar] [CrossRef]
- Ricles, J.M.; Sause, R.; Garlock, M.M.; Zhao, C. Post-tensioned seismic-resistant connections for steel frames. J. Struct. Eng. ASCE 2001, 127, 113–121. [Google Scholar] [CrossRef]
- Christopoulos, C.; Filiatrault, A.; Uang, C.M.; Folz, B. Post tensioned energy dissipating connections for moment resisting steel frames. J. Struct. Eng. ASCE 2002, 128, 1111–1120. [Google Scholar] [CrossRef]
- Kim, H.J.; Christopoulos, C. Friction damped post-tensioned self-centering steel moment-resisting frames. J. Struct. Eng. 2008, 134, 1768–1779. [Google Scholar] [CrossRef]
- Titirla, M.; Papadopoulos, P.; Doudoumis, I. Finite Element modelling of an innovative passive energy dissipation device for seismic hazard mitigation. Eng. Struct. 2018, 68, 218–228. [Google Scholar] [CrossRef]
- Fabbrocino, F.; Titirla, M.; Amendola, A.; Benzoni, G.; Fraternali, F. Innovative devices for the base isolation of existing buildings. In Proceedings of the COMPDYN 2017, 6th ECCOMAS Thematic Conference on Computational Methods in Structural Dynamics and Earthquake Engineering, Rhodes Island, Greece, 15–17 June 2017. [Google Scholar]
- Titirla, M.; Katakalos, K.; Zuccaro, G.; Frabbrocino, F. On the mechanical response of an innovative energy dissipation device. Ing. Sismic Int. J. Earthq. Eng. 2017, 126–138. [Google Scholar]
- Pall, A.S.; Verganelakis, V.; March, C. Friction Dampers for seismic control of Concordia University Library Building. In Proceedings of the 5th Canadian Conference on Earthquake Engineering, Ottawa, ON, Canada, 6–8 July 1987. [Google Scholar]
- Μartinez-Romero, E. Experiences on the Use of Supplemental Energy Dissipators on Building Structures. Earthq. Spectra 1993, 9, 581–624. [Google Scholar] [CrossRef]
- Pasquin, C.; Pall, A.; Pall, R. High-Tech Seismic Rehabilitation of Casino de Montreal. In Proceedings of the Structures Congress XII, Atlanta GA, USA, 24–28 April 1994; Volume 2, pp. 1292–1297. [Google Scholar]
- CEN. Eurocode 8: Design of Structures for Earthquake Resistance. Part 1: General Rules, Seismic Actions and Rules for Buildings; EN 1998-1; European Committee for Standardization: Brussels, Belgium, 2004. [Google Scholar]
- Malvern, L.E. Introduction to the Mechanics of a Continuous Medium; Prentice Hall: Hoboken, NY, USA, 1969. [Google Scholar]
- National Annex to NF EN 1998-1:2005; The French Association for Standardization (AFNOR): Paris, France, 2005. (In French)
- Farghaly, A.A.; Salem Ahmed, M. Optimum Design of TMD System for Tall Buildings. Int. Sch. Res. Netw. Civ. Eng. 2012, 2012, 716469. [Google Scholar] [CrossRef] [Green Version]
- Nagase, T. Earthquake records observed in tall buildings with tuned pendulum mass damper. In Proceedings of the 12th World Conference on Earthquake Engineering, Auckland, New Zealand, 30 January–4 February 2000. [Google Scholar]
- Connor, J.J. Introduction to Structural Motion Control, 1st ed.; Prentice Hall Pearson Education, Inc.: Upper Saddle River, NJ, USA, 2003. [Google Scholar]
- Gutierrez Soto, M.; Adeli, H. Tuned mass dampers. Arch. Comp. Methods Eng. 2013, 20, 419–431. [Google Scholar] [CrossRef]
- Sadek, F.; Mohraz, B.; Taylor, A.W.; Chung, R.M. A method of estimating the parameters of tuned mass dampers for seismic application. Earthq. Eng. Struct. Dynam. 1997, 26, 617–635. [Google Scholar] [CrossRef]
- Kim, H.S.; Kang, J.W. Vibration control of smart TMD for retractable-roof spatial structure considering closed and open roof condition. Int. J. Steel. Struct. 2017, 17, 1537–1548. [Google Scholar] [CrossRef]
- Lee, Y.Y.; Kim, H.S.; Kang, J.W. Seismic response control performance evaluation of tuned mass dampers for a retractable-roof spatial structure. Int. J. Steel Struct. 2021, 21, 213–224. [Google Scholar] [CrossRef]
- Almazan, J.L.; Espinoza, G.; Aguirre, J.J. Torsional balance of asymmetric structures by means of tuned mass dampers. Eng. Struct. 2012, 42, 308–328. [Google Scholar] [CrossRef]
- Elias, S.; Matsagar, V. Seismic response control of steel benchmark building with a tuned mass damper. Asian. J. Civil. Eng. 2020, 21, 267–280. [Google Scholar] [CrossRef]
- Makris, N. Viscous heating of fluid dampers. I: Small-amplitude motions. J. Eng. Mech. 1998, 124, 1210–1216. [Google Scholar] [CrossRef]
- Makris, N.; Roussos, Y.; Whittaker, A.S.; Kelly, J.M. Viscous heating of fluid dampers. II: Large-amplitude motions. J. Eng. Mech. 1998, 124, 1217–1223. [Google Scholar] [CrossRef]
- Seleemah, A.; Constantinou, M.C. Investigation of Seismic Response of Buildings with Linear and Nonlinear Fluid Viscous Dampers; Report No. NCEER 97-0004; National Center for Earthquake Engineering Research, State University of New York at Buffalo: New York, NY, USA, 1997. [Google Scholar]
- Hwang, J.S. Seismic design of structures with viscous dampers. In Proceedings of the International Training Programs for Seismic Design of Building Structures Hosted by National Centre for Research on Earthquake Engineering Sponsored by Department of International Programs, Taipei, China, 21–25 January 2002; National Science Council: Washington, DC, USA, 2002. [Google Scholar]
- Fluid Viscous Dampers . In General Guidelines for Engineers, Including a Brief History, 3rd ed.; Taylor Devices Inc.: North Tonawanda, NY, USA, 2020.
- Hwang, J.S.; Lin, W.C.; Wu, N.J. Comparison of distribution methods for viscous damping coefficients to buildings. Struct. Infrastruct. Eng. 2013, 9, 28–41. [Google Scholar] [CrossRef]
- Whittle, J.K.; Williams, M.S.; Karavasilis, T.L.; Blakeborough, A. A comparison of viscous damper placement methods for improving seismic building design. J. Earthq. Eng. 2012, 16, 540–560. [Google Scholar] [CrossRef]
- FEMA. Commentary to NEHRP Guidelines for the Seismic Rehabilitation of Buildings; FEMA 274; Building Seismic Safety Council, Federal Emergency Management Agency: Washington, DC, USA, 1997.
- Taiyari, F.; Mazzolani, F.D.; Bagheri, S. Damaged-based optimal design of friction dampers in multistory chevron braced steel frames. Soil Dyn. Earthq. Eng. 2019, 119, 11–20. [Google Scholar] [CrossRef]
- Del Gobbo, G.M. Placement of Fluid Viscous Dampers to Reduce Total-Building Seismic Damage. Ph.D. Thesis, University of Oxford, Oxford, UK, 2017. [Google Scholar]
- Moreschi, L.M. Seismic Design of Energy Dissipation Systems for Optimal Structural Performance. Ph.D. Thesis, Virginia Polytechnic Institute and State University, Blacksburg, VA, USA, 2000. [Google Scholar]
- Pall, A.; Pall, R.T. Performance-based design using Pall Friction Dampers—An economical design solution. In Proceedings of the 13th World Conference on Earthquake Engineering, Vancouver, CB, Canada, 1–6 August 2004. [Google Scholar]
- Armali, M.; Damerji, H.; Hallal, J.; Fakih, M. Effectiveness of friction dampers on the seismic behavior of high rise building VS shear wall system. Eng. Rep. 2019, 1, e12075. [Google Scholar] [CrossRef]
- Titirla, M.; Katakalos, K. Evaluation of an innovative passive mitigation device through experimental and numerical investigation. In Proceedings of the COMPDYN 2017, 6th ECCOMAS Thematic Conference on Computational Methods in Structural Dynamics and Earthquake Engineering, Rhodes Island, Greece, 15–17 June 2017. [Google Scholar]
- Titirla, M.D.; Papadopoulos, P. Finite Element investigation of a new seismic energy absorption device through simultaneously yield and friction. In Proceedings of the COMPDYN 2015, 5th ECCOMAS Thematic Conference on Computational Methods in Structural Dynamics and Earthquake Engineering, Crete Island, Greece, 25–27 May 2015. [Google Scholar]
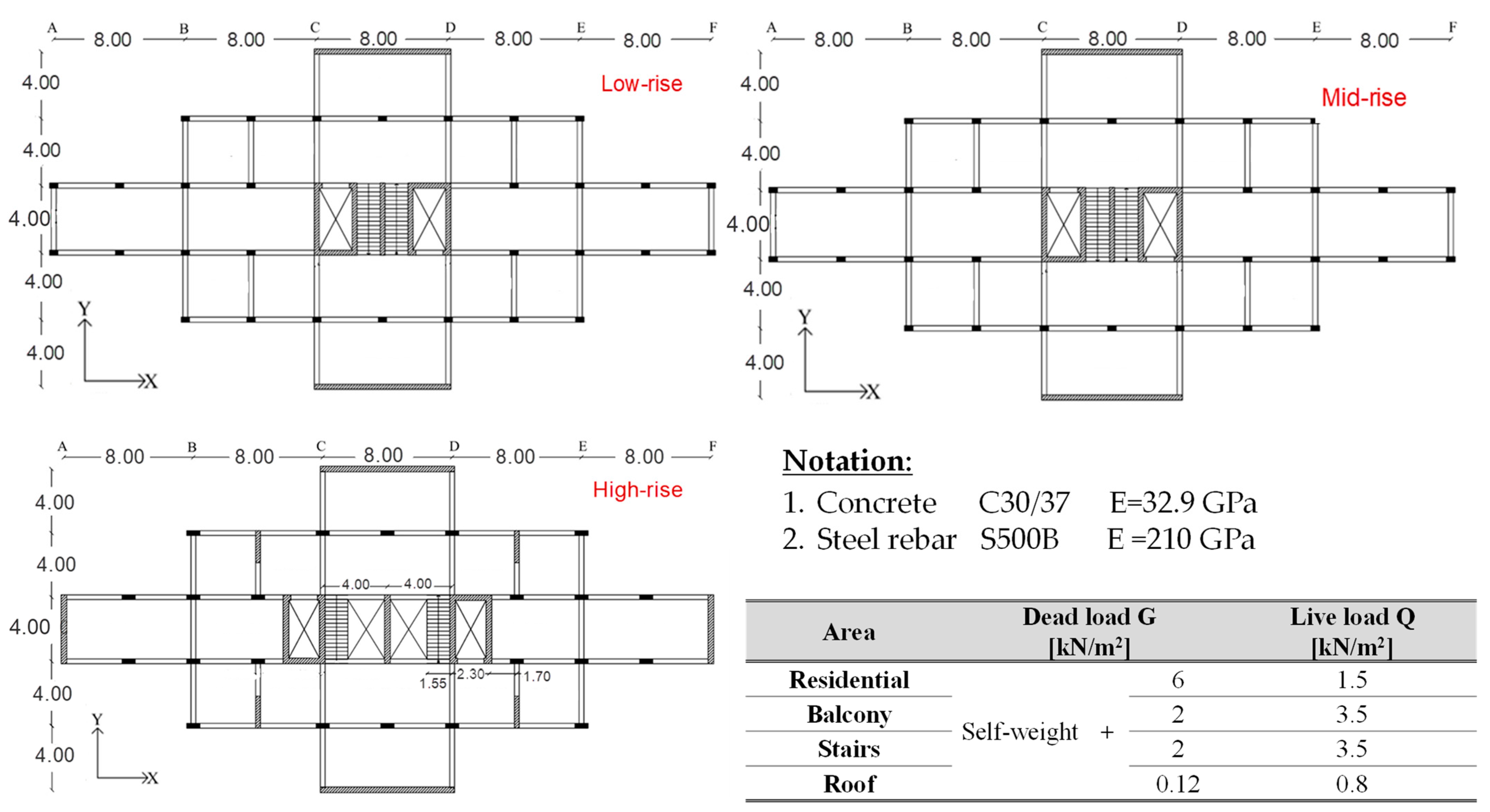

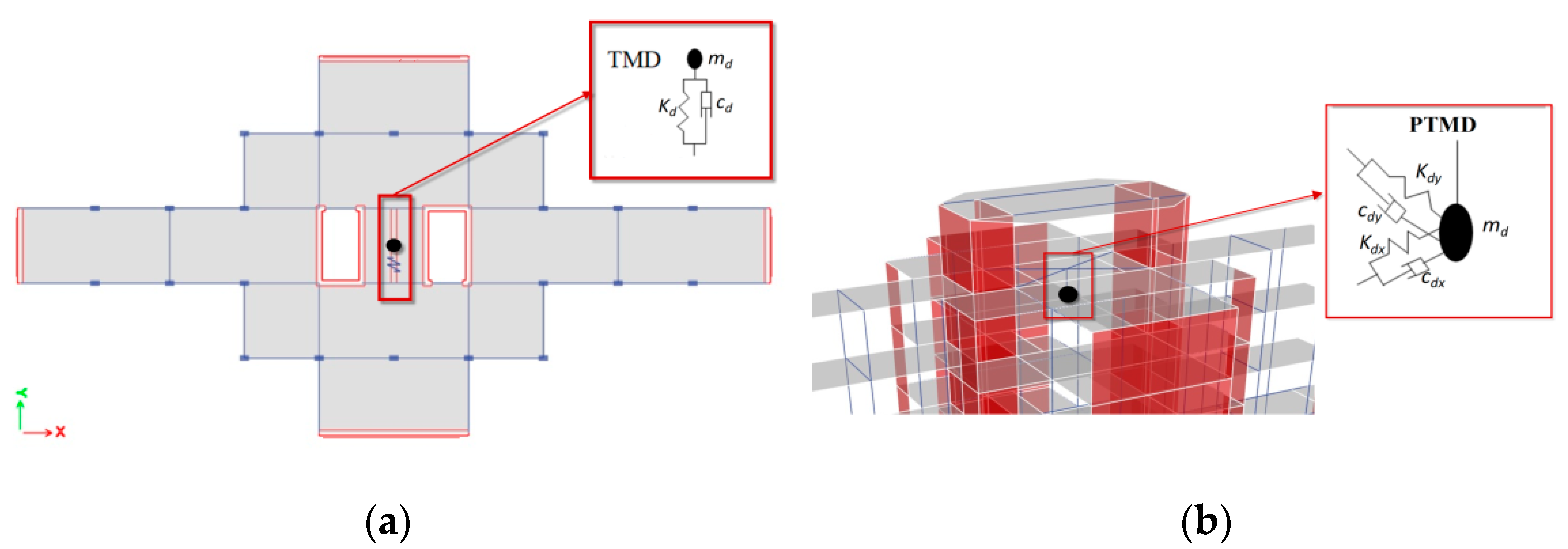
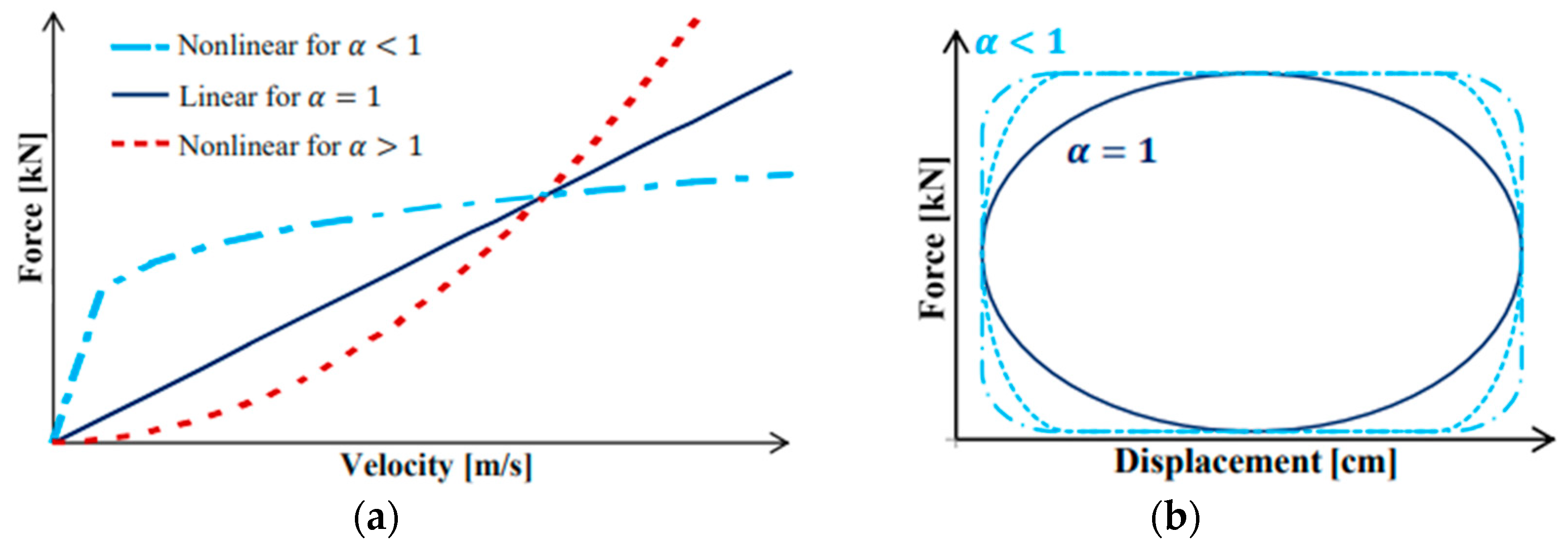
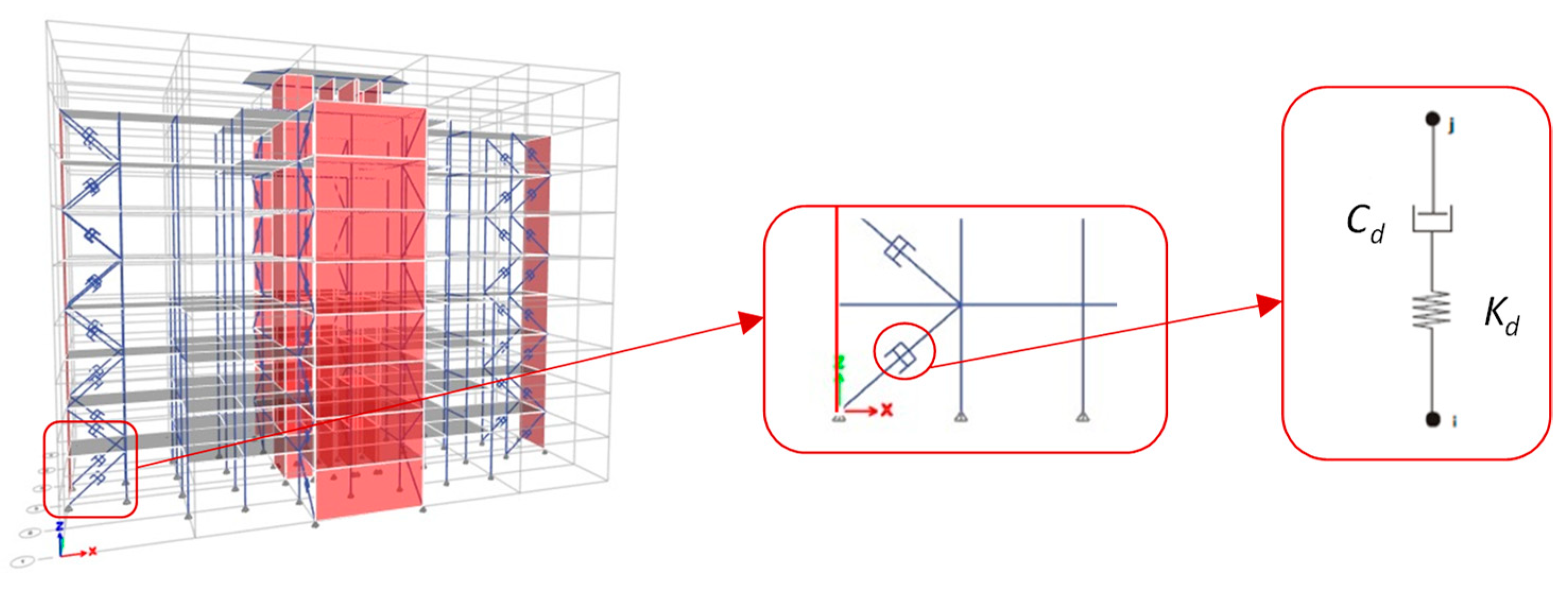


| Building Type | Low-Rise | Mid-Rise | High-Rise |
|---|---|---|---|
| Number of stories | G + 03 | G + 08 | G + 15 |
| Story height | 3.5 m | ||
| Total height including roof level | 14 m | 31.5 m | 56 m |
| Columns cross section | 30 × 50 cm | 30 × 80 cm | 30 × 80 cm |
| Beams cross section | 30 × 30 cm | ||
| Walls thickness | 30 cm | ||
| Slab thickness | 20 cm | ||
| Expressions | ||
|---|---|---|
| Optimal tuning parameters of TMD given in [38] | (2) | |
| (3) | ||
| Optimized absorber parameter | (4) | |
| (5) |
| Building Type | Structure | TMD | |||
|---|---|---|---|---|---|
| Parameter | Value | Parameter | Values | ||
| Low-rise | [s] | 0.216 | |||
| [t] | 1572.29 | ||||
| Mid-rise | [s] | 0.985 | 14.90% | ||
| [t] | 4626.92 | ||||
| High-rise | [s] | 2.202 | |||
| [t] | 8560.39 | ||||
| Building Type | md [t] | kd [kN/m] | cd [kN·s/m] | |
|---|---|---|---|---|
| Low-rise | 0.50% | 7.86 | 6561.47 | 54.63 |
| 1% | 15.72 | 12,955.58 | 134.49 | |
| Mid-rise | 1% | 46.27 | 1829.10 | 86.70 |
| 3% | 138.8 | 5238.61 | 373.86 | |
| High-rise | 2% | 171.2 | 1321.15 | 179.82 |
| 3% | 256.8 | 1937.40 | 309.24 |
| Building Type | Case | Direction | Fundamental Period | Top Roof Displacement | Base Shear | |||
|---|---|---|---|---|---|---|---|---|
| Value [s] | Margin | Value [cm] | Margin | Value [kN] | Margin | |||
| Low-rise | Undamped | Longitudinal | 0.156 | 0.8556 | 9536.64 | |||
| Transversal | 0.216 | 0.4248 | 2772.68 | |||||
| Damped with | Longitudinal | 0.158 | −1.28% | 0.8243 | 3.66% | 9530.28 | 0.07% | |
| Transversal | 0.218 | −0.93% | 0.4183 | 1.53% | 2751.67 | 0.76% | ||
| Damped with | Longitudinal | 0.157 | −0.64% | 0.8237 | 3.73% | 9287.71 | 2.61% | |
| Transversal | 0.216 | 0.00% | 0.4164 | 1.98% | 2693.44 | 2.86% | ||
| Mid-rise | Undamped | Longitudinal | 0.697 | 13.2068 | 24,870.8 | |||
| Transversal | 0.985 | 5.4141 | 4652.36 | |||||
| Damped with | Longitudinal | 0.71 | −1.87% | 12.6213 | 4.43% | 23,726.16 | 4.60% | |
| Transversal | 0.997 | −1.22% | 5.3094 | 1.93% | 4567.02 | 1.83% | ||
| Damped with | Longitudinal | 0.733 | −5.16% | 12.5036 | 5.32% | 22,507.32 | 9.50% | |
| Transversal | 1.031 | −4.67% | 4.7931 | 11.47% | 4559.55 | 1.99% | ||
| High-rise | Undamped | Longitudinal | 1.983 | 38.377 | 19,306.7 | |||
| Transversal | 2.202 | 12.5677 | 5222.92 | |||||
| Damped with | Longitudinal | 2.058 | −3.78% | 35.9845 | 6.23% | 18,077.8 | 6.37% | |
| Transversal | 2.284 | −3.72% | 10.2574 | 18.38% | 5112.19 | 2.12% | ||
| Damped with | Longitudinal | 2.091 | −5.45% | 36.3981 | 5.16% | 17,476.06 | 9.48% | |
| Transversal | 2.322 | −5.45% | 10.1373 | 19.34% | 5331.14 | −2.07% | ||
| Building Type | [m] | ||
|---|---|---|---|
| Low-rise | 0.50% | 28.893 | 0.012 |
| Mid-rise | 3.00% | 6.143 | 0.259 |
| High-rise | 3.00% | 2.747 | 1.300 |
| Case | Longitudinal | Transversal | ||||
|---|---|---|---|---|---|---|
| Undamped | With TMD | With PTMD | Undamped | With TMD | With PTMD | |
| Fundamental period [s] | 1.983 | 2.091 | 1.801 | 2.202 | 2.322 | 2.67 |
| Top roof displacement [cm] | 38.377 | 36.39 | 25.01 | 12.567 | 10.14 | 9.23 |
| Base shear [kN] | 19,306.7 | 17,476.06 | 16,974.11 | 5222.9 | 5331.14 | 4386.71 |
| Building Type | Direction | Fundamental Period [s] | Structural Rigidity Description | Suggested Velocity [m/s] | [kN·(s/m)] | |
|---|---|---|---|---|---|---|
| Low-rise | Longitudinal | 0.156 | Rigid | 0.127 | 30% | 71,537.07 |
| Transversal | 0.216 | 0.127 | 30% | 59,405.58 | ||
| Mid-rise | Longitudinal | 0.697 | Semi-rigid | 0.254 | 35% | 56,981.16 |
| Transversal | 0.985 | 0.254 | 35% | 39,325.16 | ||
| High-rise | Longitudinal | 1.983 | Flexible | 0.381 | 40% | 12,658.71 |
| Transversal | 2.202 | 0.381 | 40% | 12,095.19 |
| Building Type | Direction | Fundamental Period [s] | Top Roof Displacement [cm] | Base Shear [kN] | |||
|---|---|---|---|---|---|---|---|
| Altern. 1 | Altern. 2 | Altern. 1 | Altern. 2 | Altern. 1 | Altern. 2 | ||
| Low-rise | Longitudinal | 0.112 | 0.112 | 0.167 | 0.176 | 1973.2 | 1905.6 |
| Transversal | 0.148 | 0.147 | 0.218 | 0.345 | 2308.6 | 2070.0 | |
| Mid-rise | Longitudinal | 0.387 | 0.387 | 3.121 | 3.763 | 1888.1 | 7567.0 |
| Transversal | 0.775 | 0.775 | 2.997 | 1.760 | 4991.5 | 83.3 | |
| High-rise | Longitudinal | 0.543 | 0.543 | 5.812 | 6.821 | 10,828 | 322.5 |
| Transversal | 0.989 | 0.989 | 4.604 | 4.803 | 178.6 | 14,737.6 | |
| Building Type | Direction | Fundamental Period [s] | Top Roof Displacement [cm] | Base Shear [kN] | |||
|---|---|---|---|---|---|---|---|
| Altern. 1 | Altern. 2 | Altern. 1 | Altern. 2 | Altern. 1 | Altern. 2 | ||
| Low-rise | Longitudinal | 0.158 | 0.158 | 0.228 | 0.290 | 6971.2 | 7296.0 |
| Transversal | 0.218 | 0.218 | 0.126 | 0.057 | 1525.1 | 2000.0 | |
| Mid-rise | Longitudinal | 0.707 | 0.708 | 1.682 | 1.775 | 14,030.2 | 14,639.4 |
| Transversal | 0.998 | 0.999 | 1.124 | 0.298 | 3100.0 | 3500.0 | |
| High-rise | Longitudinal | 2.016 | 2.016 | 6.915 | 7.787 | 12,373.0 | 13,950.1 |
| Transversal | 2.239 | 2.239 | 4.349 | 2.672 | 3494.58 | 4000.0 | |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Mrad, C.; Titirla, M.D.; Larbi, W. Comparison of Strengthening Solutions with Optimized Passive Energy Dissipation Systems in Symmetric Buildings. Appl. Sci. 2021, 11, 10103. https://doi.org/10.3390/app112110103
Mrad C, Titirla MD, Larbi W. Comparison of Strengthening Solutions with Optimized Passive Energy Dissipation Systems in Symmetric Buildings. Applied Sciences. 2021; 11(21):10103. https://doi.org/10.3390/app112110103
Chicago/Turabian StyleMrad, Charbel, Magdalini D. Titirla, and Walid Larbi. 2021. "Comparison of Strengthening Solutions with Optimized Passive Energy Dissipation Systems in Symmetric Buildings" Applied Sciences 11, no. 21: 10103. https://doi.org/10.3390/app112110103
APA StyleMrad, C., Titirla, M. D., & Larbi, W. (2021). Comparison of Strengthening Solutions with Optimized Passive Energy Dissipation Systems in Symmetric Buildings. Applied Sciences, 11(21), 10103. https://doi.org/10.3390/app112110103







