The Evolutions in Time of Probability Density Functions of Polydispersed Fuel Spray—The Continuous Mathematical Model
Abstract
:1. Introduction
2. Model Description
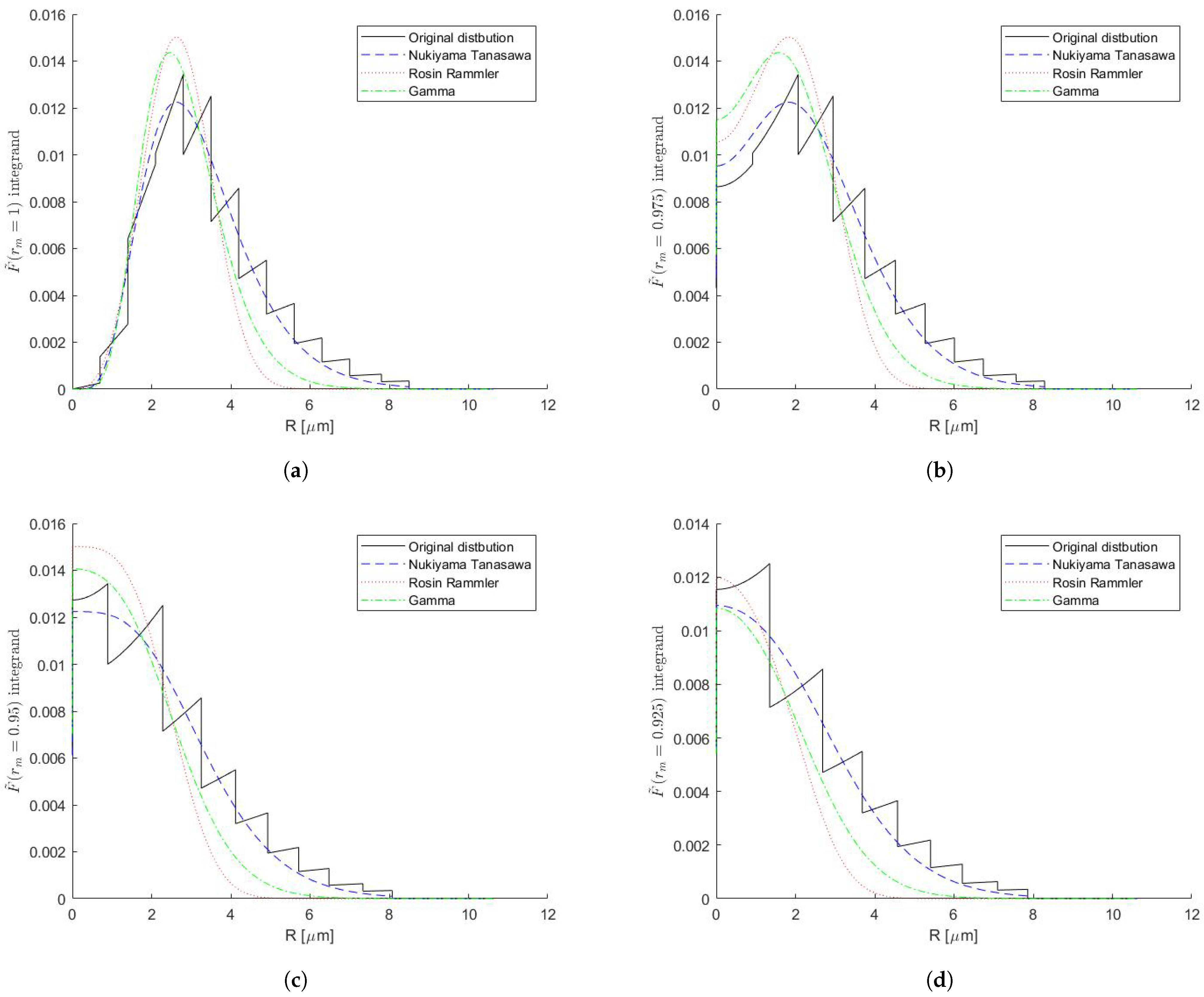
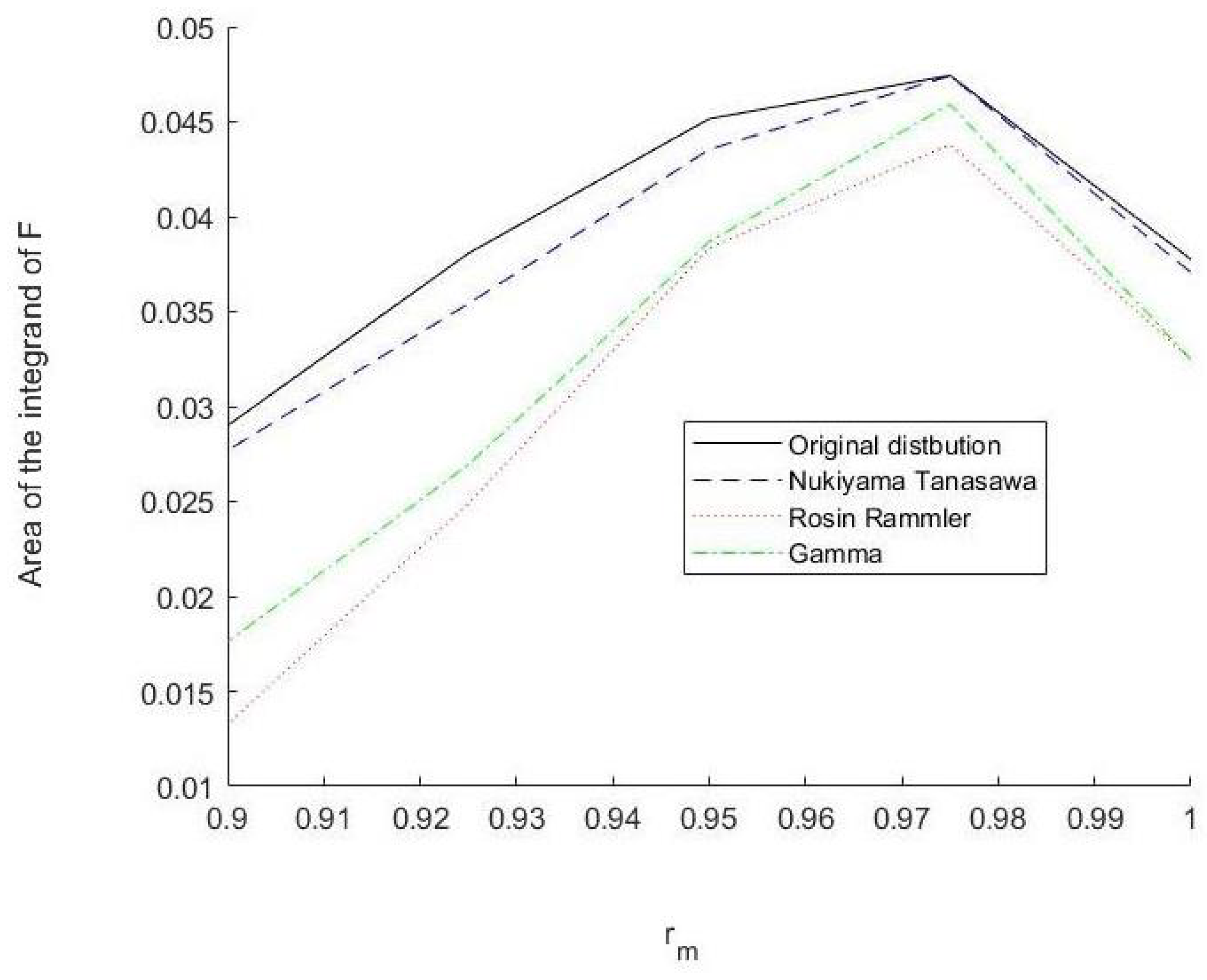
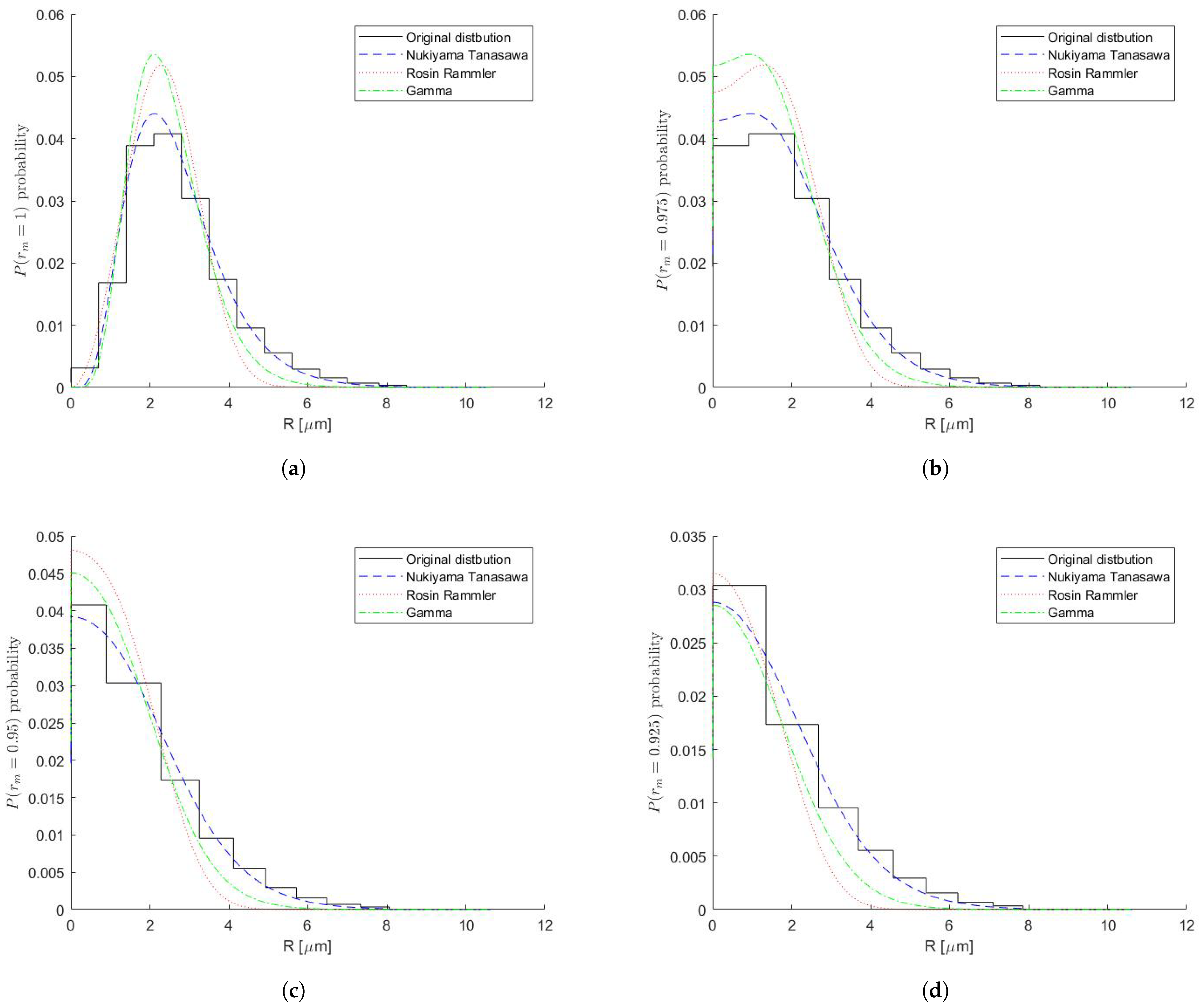
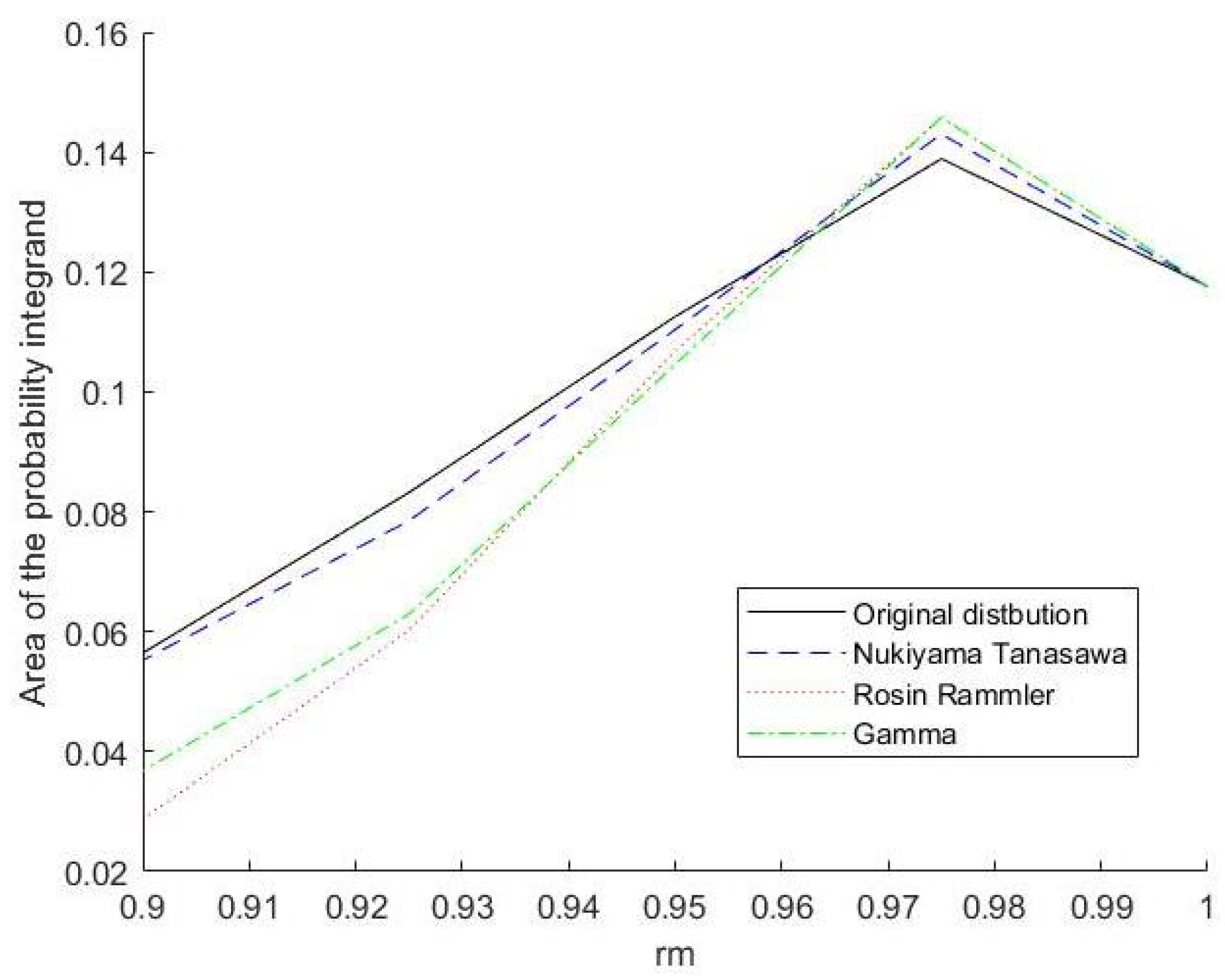
3. Results and Discussion
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
Nomenclature
| A | constant pre-exponential rate factor |
| C | molar concentration (k mol m−3) |
| c | specific heat capacity (J kg−1 K−1) |
| E | activation energy (J k mol−1) |
| L | liquid evaporation energy (i.e., latent heat of evaporation, enthalpy of evaporation) (J kg−1) |
| number of droplets of size i per unit volume (m−3) | |
| Q | combustion energy (J kg−1) |
| B | universal gas constant (J k mol−1 K−1) |
| radius of size i drops (m) | |
| maximal droplet radius at (m) | |
| T | temperature (K) |
| t | time (s) |
| (s) | |
| probability density function | |
| probability distribution function | |
| unit step function | |
| rectangle function | |
| Greek symbols and dimensionless parameters | |
| dimensionless volumetric phase content, | |
| quantity equivalent to the volumetric phase content for the continuous model | |
| dimensionless reduced initial temperature (with respect to the activation temperature ) | |
| dimensionless parameter that represents the reciprocal of the final dimensionless adiabatic temperature of the thermally insulated system after the explosion has been completed | |
| dimensionless parameters introduced in Equation (11) that describe the interaction between gaseous and liquid phases | |
| molar mass (kg kmol−1) | |
| thermal conductivity (W m−1 K−1) | |
| density (kg m−3) | |
| dimensionless time | |
| represents the internal characteristics of the fuel (the ratio of the specific combustion energy to the latent heat of evaporation) and is defined in Equation (11) (dimensionless) | |
| Dimensionless variables | |
| dimensionless fuel concentration | |
| dimensionless temperature | |
| r | dimensionless radius |
| Subscripts | |
| d | liquid fuel droplets |
| f | combustible gas component of the mixture |
| g | gas mixture |
| i | number of droplet sizes |
| L | liquid phase |
| p | under constant pressure |
| s | saturation line (surface of droplets) |
| 0 | initial state |
| m | number of droplet sizes |
| Abbreviations | |
| probability density function | |
| PSD | particle size distribution |
References
- Wirtz, J.; Cuenot, B.; Riber, E. Numerical study of a polydisperse spray counterflow diffusion flame. Proc. Combust. Inst. 2021, 38, 3175–3182. [Google Scholar] [CrossRef]
- Wang, Q.; Jaravel, T.; Ihme, M. Assessment of spray combustion models in large-eddy simulations of a polydispersed acetone spray flame. Proc. Combust. Inst. 2019, 37, 3335–3344. [Google Scholar] [CrossRef]
- Greenberg, J.B.; Katoshevski, D. Polydisperse spray diffusion flames in oscillating flow. Combust. Theory Model. 2016, 20, 349–372. [Google Scholar] [CrossRef]
- Greenberg, J.; Silverman, I.; Tambour, Y. On the origins of spray sectional conservation equations. Combust. Flame 1993, 93, 90–96. [Google Scholar] [CrossRef]
- Tambour, Y. Transient mass and heat transfer from a cloud of vaporizing droplets of various size distributions: A sectional approach. Chem. Eng. Commun. 1986, 44, 183–196. [Google Scholar] [CrossRef]
- Tambour, Y. Simulation of coalescence and vaporization of kerosene fuel sprays in a turbulent jet—A sectional approach. In Proceedings of the 21st Joint Propulsion Conference, Monterey, CA, USA, 8–10 July 1985; American Institute of Aeronautics and Astronautics: Reston, VA, USA, 1985. [Google Scholar]
- Tambour, Y. Vaporization of polydisperse fuel sprays in a laminar boundary layer flow: A sectional approach. Combust. Flame 1984, 58, 103–114. [Google Scholar] [CrossRef]
- Anidjar, F.; Tambour, Y.; Greenberg, J. Mass exchange between droplets during head-on collisions of multisize sprays. Int. J. Heat Mass Transf. 1995, 38, 3369–3383. [Google Scholar] [CrossRef]
- Silverman, I.; Greenberg, J.; Tambour, Y. Stoichiometry and polydisperse effects in premixed spray flames. Combust. Flame 1993, 93, 97–118. [Google Scholar] [CrossRef]
- Tambour, Y.; Zehavi, S. Derivation of near-field sectional equations for the dynamics of polydisperse spray flows: An analysis of the relaxation zone behind a normal shock wave. Combust. Flame 1993, 95, 383–409. [Google Scholar] [CrossRef]
- Silverman, I.; Greenberg, J.B.; Tambour, Y. Asymptotic analysis of a premixed polydisperse spray flame. SIAM J. Appl. Math. 1991, 51, 1284–1303. [Google Scholar] [CrossRef]
- Aggrawal, S.K. A review of spray ignition phenomena: Present status and future research. Prog. Energy Combust. Sci. 1998, 24, 565–600. [Google Scholar] [CrossRef]
- Stone, R. Introduction to Internal Combustion Engines; Macmillan: London, UK, 1998. [Google Scholar]
- Sazhin, E.M.; Sazhin, S.S.; Heikal, M.R.; Babushok, V.I.; Johns, R.A. A detailed modeling of the spray ignition process in diesel engines. Combust. Sci. Technol. 2000, 160, 317–344. [Google Scholar] [CrossRef]
- Greenberg, J.B. Droplet size distribution effects in an edge flame with a fuel spray. Combust. Flame 2017, 179, 228–237. [Google Scholar] [CrossRef]
- Noh, D.; Gallot-Lavallée, S.; Jones, W.P.; Navarro-Martinez, S. Comparison of droplet evaporation models for a turbulent, non-swirling jet flame with a polydisperse droplet distribution. Combust. Flame 2018, 194, 135–151. [Google Scholar] [CrossRef] [Green Version]
- Babinsky, E.; Sojka, P.E. Modeling drop size distributions. Prog. Energy Combust. Sci. 2002, 28, 303–329. [Google Scholar] [CrossRef]
- Urbán, A.; Józsa, V. Investigation of Fuel Atomization with Density Functions. Period. Polytech. Mech. Eng. 2018, 62, 33–41. [Google Scholar] [CrossRef] [Green Version]
- Warnatz, J.; Maas, U.; Dibble, W.R. Combustion: Physical and Chemical Fundamentals, Modeling and Simulation, Experiments, Pollutant Formation; Springer: Berlin/Heidelberg, Germany; New York, NY, USA, 2006. [Google Scholar]
- Kolakaluria, S.S.R.; Panchagnulab, M.V. Trends in multiphase modeling andsimulation of sprays. Int. J. Spray Combust. Dyn. 2014, 6, 317–356. [Google Scholar] [CrossRef]
- Semenov, N.N. Zur theorie des verbrennugsprozesse. Z. Phys. 1928, 48, 571–581. [Google Scholar] [CrossRef]
- Kats, G.; Greenberg, J. Forced thermal ignition of a polydisperse fuel spray. Combust. Sci. Technol. 2018, 190, 849–877. [Google Scholar] [CrossRef]
- Bykov, V.; Goldfarb, I.; Greenberg, V. Auto-ignition of a polydisperse fuel spray. Proc. Combust. Inst. 2007, 31, 2257–2264. [Google Scholar] [CrossRef]
- Nave, O.; Gol’dshtein, V.M.; Bykov, V. A probabilistic model of thermal explosion in polydisperse fuel spray. Appl. Math. Comput. 2010, 217, 2698–2709. [Google Scholar] [CrossRef]
- Sazhin, S.; Martynov, S.; Kristyadi, T.; Crua, C.; Heikal, M.R. Diesel fuel spray penetration, heating, evaporation and ignition: Modelling vs. experimentation. Int. J. Eng. Syst. Model. Simul. 2008, 1, 1–19. [Google Scholar]
- Williams, F.A. The Fundamental Theory of Chemically Reacting Flow System; Benjamin-Cummings: Menlo Park, CA, USA, 1985. [Google Scholar]
- Tyurenkova, V.V. Non-equilibrium diffusion combustion of a fuel droplet. Acta Astronaut. 2012, 75, 78–84. [Google Scholar] [CrossRef]
- Rosin, P.; Rammler, E. Laws governing the fineness of powdered coal. J. Inst. Fuel 1933, 7, 29–36. [Google Scholar]
- Nukiyama, S.; Tanasawa, Y. Experiments on the atomization of liquids in an airstream. Trans. Jpn. Soc. Mech. Eng. 1939, 5, 68–75. [Google Scholar]


Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Hareli, S.; Nave, O.; Gol’dshtein, V. The Evolutions in Time of Probability Density Functions of Polydispersed Fuel Spray—The Continuous Mathematical Model. Appl. Sci. 2021, 11, 9739. https://doi.org/10.3390/app11209739
Hareli S, Nave O, Gol’dshtein V. The Evolutions in Time of Probability Density Functions of Polydispersed Fuel Spray—The Continuous Mathematical Model. Applied Sciences. 2021; 11(20):9739. https://doi.org/10.3390/app11209739
Chicago/Turabian StyleHareli, Shlomo, Ophir Nave, and Vladimir Gol’dshtein. 2021. "The Evolutions in Time of Probability Density Functions of Polydispersed Fuel Spray—The Continuous Mathematical Model" Applied Sciences 11, no. 20: 9739. https://doi.org/10.3390/app11209739
APA StyleHareli, S., Nave, O., & Gol’dshtein, V. (2021). The Evolutions in Time of Probability Density Functions of Polydispersed Fuel Spray—The Continuous Mathematical Model. Applied Sciences, 11(20), 9739. https://doi.org/10.3390/app11209739





