Development of Hydraulic Turbodrills for Deep Well Drilling
Abstract
1. Introduction
2. Development of Mathematical Model
- —equivalent speed in the rotor, m/s,
- —equivalent speed in the stator, m/s,
- —axis velocity of the liquid, m/s,
- —district fluid velocity, m/s,
- —torque, Nm,
- —density, kg/m3,
- —drilling fluid flow rate, m3/s,
- —medium turbine diameter, m,
- —stator blade angle of inclination, n’,
- —rotor blade angle of inclination, n’,
- —rotation frequency, rev/s,
- —radial length of turbodrill blades, m.
3. Simulation of the Investigated Object
3.1. Study of the Mathematical Model
- —power, W,
- —rotation frequency, rad/s,
- —rotation frequency, rpm.
- —generalized variables of the desired values,
- —valid speed values of rotation,
- —rotation frequency function values for a set of generalized variables,
- —real space with the length of the q vector.
- h, g—restriction function,
- —positive barrier parameter, variable s is to be positive.
- e—single vector-pillar,
- S—diagonal matrix,
- —vector line components of the gradient corresponding to the restriction function.
3.2. Results of Mathematical Modeling
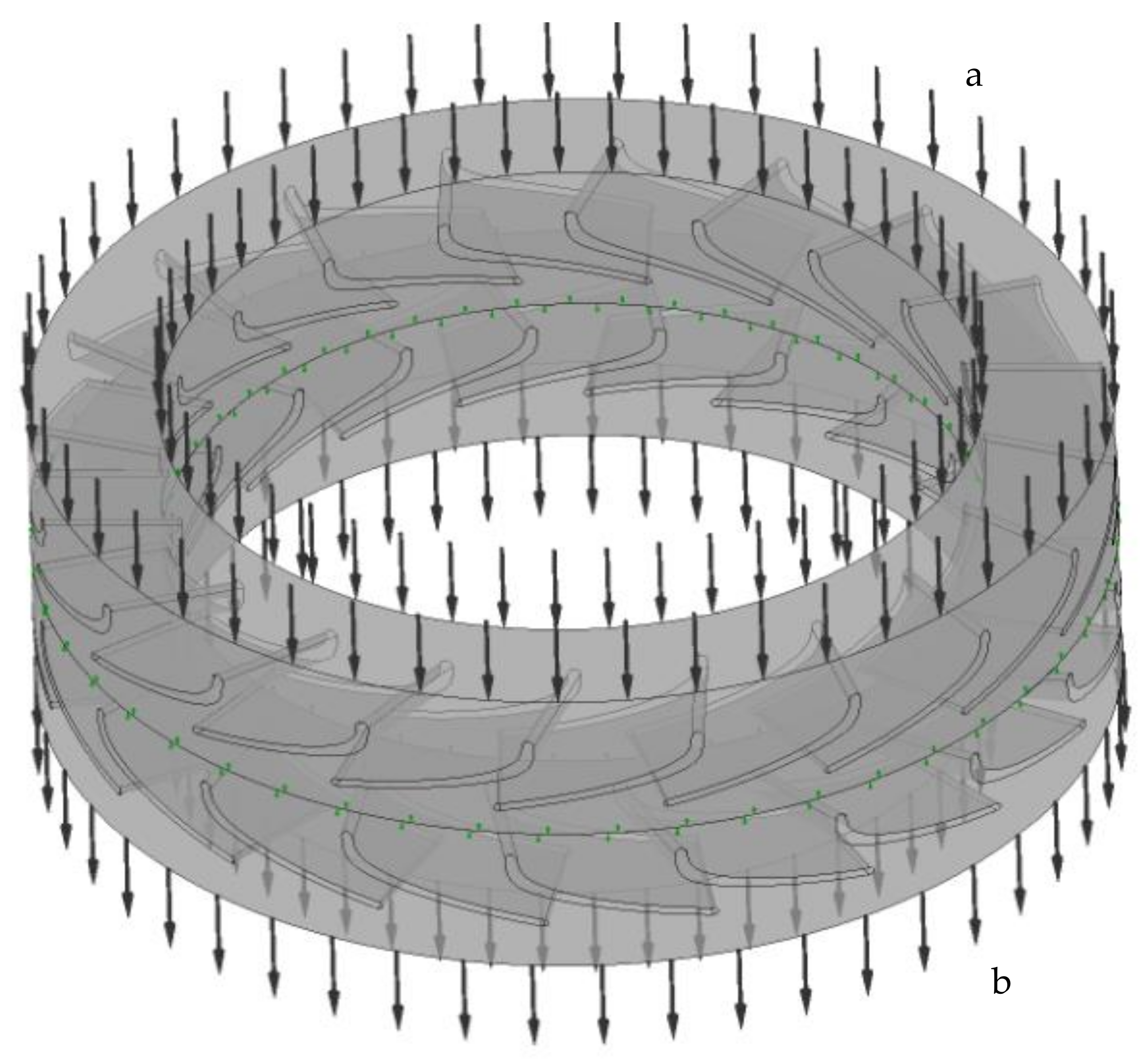
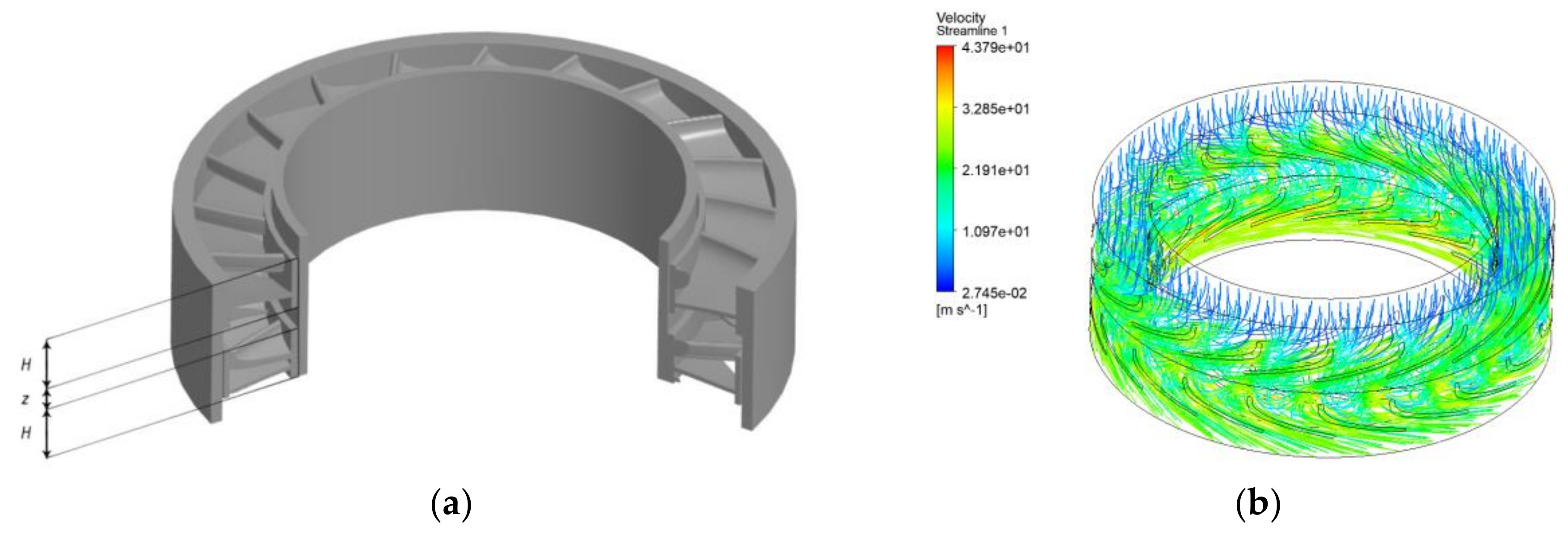
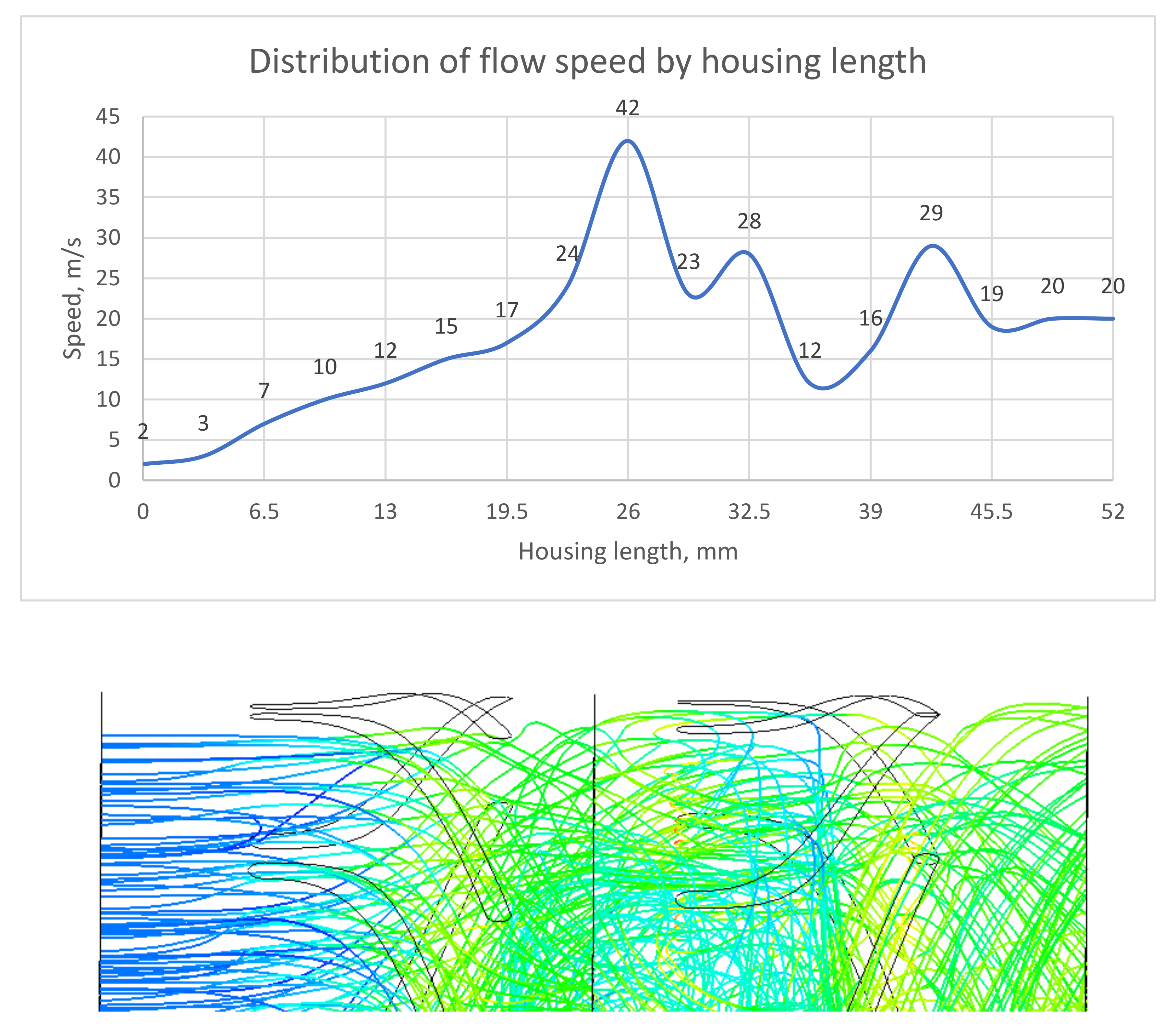
3.3. Testing the Research Results Using the Finite Element Method
4. Comparative Analysis of the Developed Turbodrill
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- How Deep can Superdeep Wells Be and What Were the USSR and the USA Looking for Inside the Earth? Available online: https://www.bbc.com/russian/vert-fut-48188978 (accessed on 5 July 2021).
- 10 Longest Wells in the World. Available online: https://www.kommersant.ru/doc/1580772 (accessed on 3 July 2021).
- Litvinenko, V.S.; Nikolaev, N.I. Process Fluids to Improve the Efficiency of Construction and Operation of Oil and Gas Wells; Notes of the Mining Institute; Saint-Petersburg Mining University: Saint-Petersburg, Russia, 2016; Volume 194, p. 84. [Google Scholar]
- Nikolaev, N.I. Development of Compositions of Drilling Fluids to Improve the Efficiency of Drilling Solid Rocks; Nikolaev, N.I., Leusheva, E.L., Eds.; Notes of the Mining Institute; Saint-Petersburg Mining University: Saint-Petersburg, Russia, 2016; Volume 219, pp. 412–420. [Google Scholar] [CrossRef]
- Nikolaev, N.I.; Ivanov, A.I. Improving the Efficiency of Drilling Oil and Gas Wells in Difficult Conditions; Notes of the Mining Institute; Saint-Petersburg Mining University: Saint-Petersburg, Russia, 2009; Volume 183, p. 308. [Google Scholar]
- Simonyants, S.L. Turbine Drilling Current Issues. Onshore and Offshore Oil and Gas Well Construction. 2006. Available online: http://ogbus.ru/files/ogbus/authors/Simonyanc/Simonyanc_1.pdf (accessed on 16 May 2021).
- Simonyants, S.L. Turbine Drilling Modernization Problem; Vector-Book: Tumen, Russia, 2003; 136p. [Google Scholar]
- Litvinenko, V.S.; Kudryashov, B.B. Modern Problems of Destruction of Rocks when Drilling Wells; Notes of the Mining Institute; Saint-Petersburg Mining University: Saint-Petersburg, Russia, 2017; Volume 148, p. 14. [Google Scholar]
- Oil and Gas Well Drilling Technology. Available online: https://portal.tpu.ru/SHARED/e/EPIKHIN/eng/Pedagogics/Tab/Lecture_TBNG_3.pdf (accessed on 23 June 2021).
- Leonov, E.G.; Simonyants, S.L. Improvement of the Technological Process of Well Deepening: Tutorial—M; RSU of Oil and Gas of the I.M. Gubkin: Moscow, Russia, 2014; 184p. [Google Scholar]
- Kamatov, K.A.; Kamatov, K.A. Solutions for drilling efficiency improvement in extreme geological conditions of Timano-Pechora region. In Proceedings of the SPE Russian Petroleum Technology Conference, Moscow, Russia, 15–17 October 2015. [Google Scholar] [CrossRef]
- Nikolaev, N.I. Theoretical and Experimental Studies of the Efficiency of Drilling Hard Rocks. In Oil and Gas and Mining; Nikolaev, N.I., Leusheva, E.L., Eds.; Bulletin PNRPU. Geology; Saint-Petersburg Mining University: Saint-Petersburg, Russia, 2015; pp. 38–47. [Google Scholar] [CrossRef]
- Simonyants, S.L.; Al Taee, M. Stimulation of the Drilling Process with the Top Driven Screw Downhole Motor. J. Min. Inst. 2019, 238, 438–442. [Google Scholar] [CrossRef]
- Dolgiy, I.E. Resistance of Rocks to Destruction When Drilling Wells; Dolgiy, I.E., Nikolaev, N.I., Eds.; Notes of the Mining Institute; Saint-Petersburg Mining University: Saint-Petersburg, Russia, 2016; Volume 221, pp. 655–660. [Google Scholar] [CrossRef]
- Zvarygin, B.I. Drilling Rigs and Well Drilling: Tutorial—Krasnoyarsk; Siberian Federal University: Krasnoyarsk, Russia, 2011; 256p. [Google Scholar]
- Superdeep Well Drilling. Available online: https://neftegaz.ru/science/booty/332315-sverkhglubokoe-burenie-skvazhin/ (accessed on 27 June 2021).
- Hlebnikov, D.; Mialitsin, N. VNIIBT-Drilling Tools. Methods for Improving the Turbodrill for Drilling in Hard Rocks. Drilling and Oil. 2013. Available online: https://burneft.ru/archive/issues/2013-06/13 (accessed on 27 April 2021).
- Bobrov, M.; Mialitsin, N.; Mingaraev, V.; Molodilo, V.; Pologeenko, V.; Asadchev, A.; Matveenko, D.; Judenko, L. Drilling by a High-Speed Turbodrill T-6 ¾ with Impregnated Bit at a Depth of 5780–6340 m. Available online: https://burneft.ru/archive/issues/2016-02/38 (accessed on 23 April 2021).
- Chudakov, G.F.; Korotaev, Y.A.; Solomatkin, A.A.; Mialitsin, N.Y. Turbine Turbodrill. Patent 2403366, 10 November 2009. [Google Scholar]
- Shumilov, V.P.; Martynov, V.N. Turbine Turbodrill. Patent 2269631, 19 August 2004. [Google Scholar]
- Neyrfor Thru-Tubing Turbodrill 2 1/8-in. Available online: https://www.slb.com/-/media/files/bdt/brochure/neyrfor-ttt-br-2.ashx (accessed on 23 April 2021).
- Neyrfor Thru-Tubing Turbodrill 2 7/8-in. Available online: https://www.slb.com/-/media/files/drilling/product-sheet/neyrfor-ttt-278-ps.ashx (accessed on 23 April 2021).
- High Temperature Drilling Operations. Available online: https://www.slb.com/-/media/files/drilling/brochure/ht-tools-br.ashx (accessed on 3 May 2021).
- Turbodrill Neyrfor and Impregnated Bits Kinetic Doubled ROP. Available online: https://www.slb.ru/library/projects/well_construction_cs/burovoe-oborudovanie-i-servisy/sistema-s-turboburom-neyrfor-i-impregnirovannymi-dolotami-kinetic-pozvolila-vdvoe-uvelichit-skorost-/ (accessed on 4 June 2021).
- Gorshkov, L.K.; Osetskiy, A.I. Development of Principles for the Design and Operation of New Diamond Rock Cutting Tools; Notes of the Mining Institute; Saint-Petersburg Mining University: Saint-Petersburg, Russia, 2012; Volume 197, p. 40. [Google Scholar]
- Mendebaev, T.N.; Smashov, N.Z.; Ismailov, H.K.; Izakov, B.K. Development of a resource-saving, small-sized downhole hydraulic machine for well drilling. East. Eur. J. Enterp. Technol. 2019, 6, 70–76. [Google Scholar] [CrossRef]
- Mialitsin, N.Y. New Solutions in the Design of Hudraulic Downhole Motors Manufactured by LLC «VNIIBT-Drilling Tools». Engineering Practice. 2012. Available online: https://oilgasconference.ru/upload/iblock/a84/20140210_122911_62376200.pdf (accessed on 18 March 2021).
- Buslaev, G.V. Development and field of the downhole multipurpose thrusting device for drilling of deep vertical and directional wells. Paper presented at the SPE Russian Petroleum Technology conference, Moscow, Russia, 26–28 October 2015. [Google Scholar] [CrossRef]
- Sazonov, Y.A.; Mokhov, M.A.; Demidova, A.A. Development of Small Hydraulic Downhole Motors for Well Drilling Applications. Am. J. Appl. Sci. 2016, 13, 1053–1059. [Google Scholar] [CrossRef][Green Version]
- Biletsky, V.; Vitryk, V.; Mishchuk, Y.; Fyk, M.; Dzhus, A.; Kovalchuk, J. Examining the current of drilling mud in a power section of the screw downhole motor. East. Eur. J. Enterp. Technol. 2018, 2, 41–47. [Google Scholar] [CrossRef][Green Version]
- Buslaev, G.V.; Ovchinnikov, V.A.; Rudnitsky, N.A. The optimization of a drill string bottom assembling of the well no 741 Kamyshinskaya. Sci. Tech. J. 2017. [Google Scholar] [CrossRef]
- Sazonov, Y.A.; Mokhov, M.A.; Frankov, M.A.; Ivanov, D.Y. The research of experimental downhole motor for well drilling using PDC type drill bits. Neftyznoe Khozyaystvo. Oil Ind. 2017, 10, 70–74. [Google Scholar] [CrossRef][Green Version]
- Petrakov, D.G.; Kupavukh, K.S.; Kupavykh, A.S. The effect on fluid saturation on the elastic plastic properties of oil reservoir rocks. Curved Layer. Struct. 2020, 7, 29–34. [Google Scholar] [CrossRef]
- Simonyants, S.L. Application of Impregnated Diamond Bits with High Power Turbodrill; Drilling Contractors Association Bulletin: Moscow, Russia, 2011; pp. 7–9. [Google Scholar]
- Simonyants, S.L. Turbodrill and Screw Motor: Development Dialectics. In Proceedings of the SPE Russian Petroleum Technology Conference and Exhibition, Moscow, Russia, 24–26 October 2016. [Google Scholar] [CrossRef]
- Nutscova, M.V. Research of oil-based drilling fluid to improve the quality of wells completion. In Proceedings of the IOP Conference Series: Materials Science and Engineering, Bangkok, Thailand, 17–19 May 2019; Volume 666, p. 12065. [Google Scholar]
- Liu, T.; Leusheva, E.L.; Morenov, V.A.; Jiang, G.; Fang, C.; Zhang, L.; Zheng, S.; Yu, Y. Influence of polymer reagents in the drilling fluids on the efficience of deviated and horizontal wells drilling. Energies 2020, 13, 4704. [Google Scholar] [CrossRef]
- Baldenko, F.D. Drilling Equipment Calculations; RSU of Oil and Gas of the I.M. Gubkin: Moscow, Russia, 2012; pp. 384–409. [Google Scholar]
- Yu, W.; Bairu, X.; Zhiqiao, W.; Liguang, W.; Qin, Z. Design and Output Perfomance of Turbodrill Blade used in a Slim Borehole. Energies 2016, 9, 1035. [Google Scholar] [CrossRef]
- Ovchinnikova, E.N. Engineering ethics and training of mining engineers. In Proceedings of the Innovation-Based Development of The Mineral resources sector: Challenges and prospects—11th conference of the Russian-German raw materials, Potsdam, Germany, 7–8 November 2018. [Google Scholar]
- Lyagov, I.A.; Baldenko, F.D.; Lyagov, A.V.; Yamaliev, V.U.; Lyagova, A.A. Methodology for calculating technical efficiency of power sections in small-sized screw downhole motors for the «Perfobur» system. J. Min. Inst. 2019, 240, 694. [Google Scholar] [CrossRef]
- Simonyants, S.L. Turbine Design of a High-Power Turbodrill for Drilling with Diamond bits. Onshore and Offshore Oil and Gas Well Construction; (co-auth. Shumilov, V.P.; Litvyak, V.A.; Mnatsakanov, I.V.); RSU of Oil and Gas of the I.M. Gubkin: Moscow, Russia, 2011; pp. 19–23. Available online: http://www.vniioeng.ru/inform/construction/sod48/ (accessed on 28 April 2021).
- Byrd, R.H. Robust trust region methods for constrained optimization. In Proceedings of the Third SIAM Conference on Optimization, Houston, TX, USA, 17–20 May 1987. [Google Scholar] [CrossRef]
- John, H. Non-Linear and Dynamic Programming; Springer: Berlin/Heidelberg, Germany, 1964; 506p. [Google Scholar]
- Byrd, R.H.; Liu, G.; Nocedal, J. On the local behavior of an interior-point algorithm for nonlinear programming. In Numerical Analysis 1997; Griffiths, D.F., Higham, D.J., Eds.; Addison–Wesley Longman: Reading, MA, USA, 1997. [Google Scholar]
- Lalee, M.; Nocedal, J.; Plantenga, T. On the implementation of an algorithm for large-scale equality constrained optimization. SIAM J. Optim. 1998, 8, 682–706. [Google Scholar] [CrossRef]
- Fletcher, R. Practical Methods of Optimization, 2nd ed.; John Wiley: New York, NY, USA, 1990. [Google Scholar]
- Wang, Y.; Yao, J.; Li, Z. Design and Development of Turbodrill Blade Used in Crystallized Section; Hindawi Publishing Corporation: London, UK, 2014. [Google Scholar] [CrossRef][Green Version]
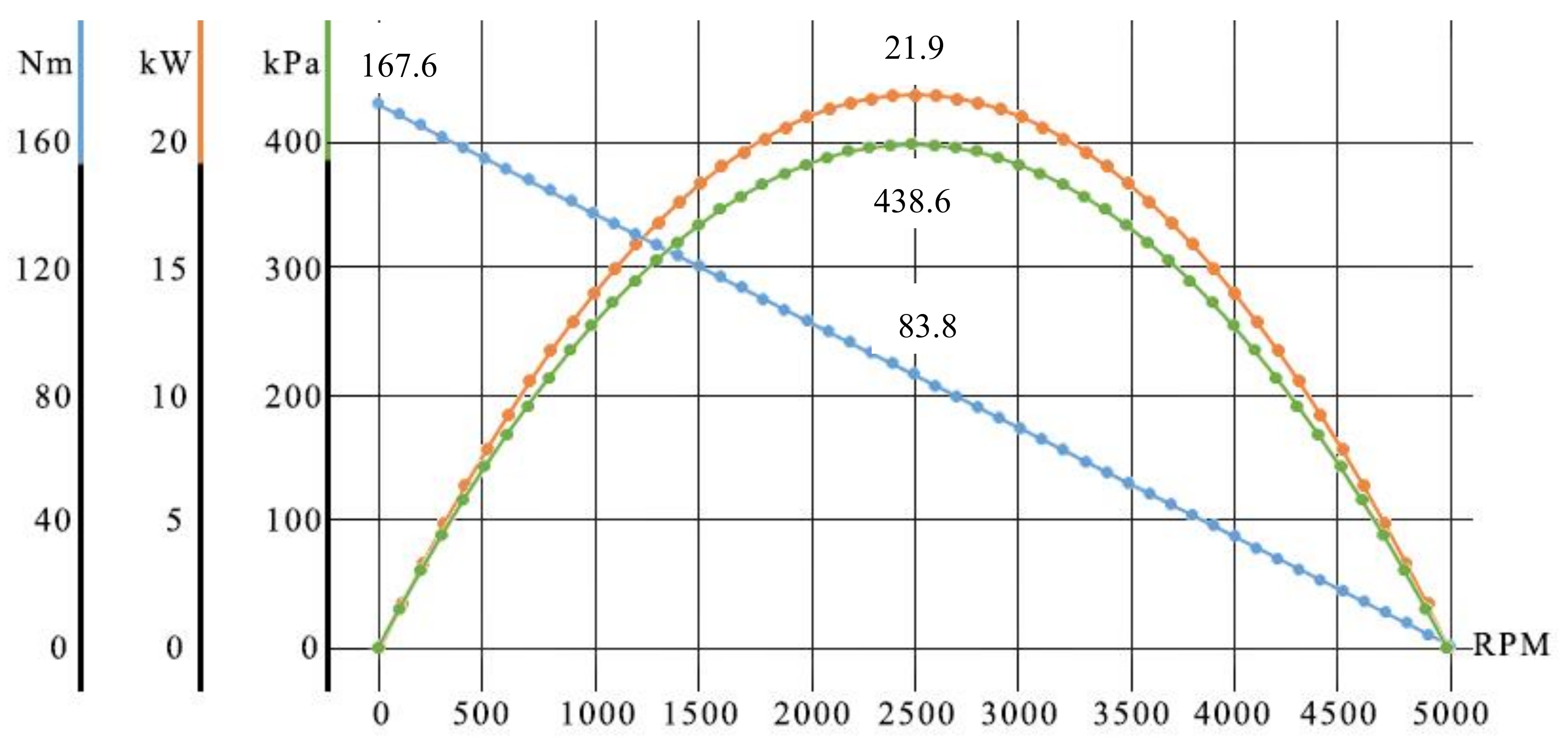
| Rotation Frequency, rpm | Torque, Nm | Power, kW | Pressure Drop, kPa |
|---|---|---|---|
| 0 | 167.6 | 0.0 | 0.0 |
| 500 | 150.8 | 7.9 | 157.9 |
| 1000 | 134.0 | 14.0 | 280.7 |
| 1500 | 117.3 | 18.4 | 368.5 |
| 2000 | 100.5 | 21.1 | 421.1 |
| 2500 | 83.8 | 21.9 | 438.6 |
| 3000 | 67.0 | 21.1 | 421.1 |
| 3500 | 50.3 | 18.4 | 368.5 |
| 4000 | 33.5 | 14.0 | 280.7 |
| 4500 | 16.8 | 7.9 | 157.9 |
| 5000 | 0.0 | 0.0 | 0.0 |
| Parameter | Value |
|---|---|
| Rotor blade angle, n’. | 12.18 |
| Stator blade angle, n’. | 12.18 |
| Flow rate, L/s | 50.00 |
| Turbine pressure drop, kPa | 438.65 |
| No. | Indicator | Model of Turbodrill | ||
|---|---|---|---|---|
| TSSh-178T | Neyrfor 2 7/8 | Developed Turbodrill | ||
| 1 | Outer diameter of the case, mm | 178 | 73 | 195 |
| 2 | Turbodrill length, mm | 7100 | 4572 | 520 |
| 3 | Drilling fluid flow rate, l/s | 28–38 | 6.3–7.5 | 45–55 |
| 4 | Drilling mud density, g/cm3 | 1.0 | Before 2.16 | 1.0 |
| 5 | Torque, Nm | 1630–3000 | Before 203 | 679–1013 |
| 6 | Operating speed, rpm | 945–1283 | 2000–2500 | 2250–2750 |
| 7 | Turbine pressure drop, MPa | 5.4–9.2 | 7.6–11.4 | 3.6–5.3 |
| 8 | Maximum power, kW | 80–201 | 24–43 | 160–292 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Dvoynikov, M.V.; Sidorkin, D.I.; Kunshin, A.A.; Kovalev, D.A. Development of Hydraulic Turbodrills for Deep Well Drilling. Appl. Sci. 2021, 11, 7517. https://doi.org/10.3390/app11167517
Dvoynikov MV, Sidorkin DI, Kunshin AA, Kovalev DA. Development of Hydraulic Turbodrills for Deep Well Drilling. Applied Sciences. 2021; 11(16):7517. https://doi.org/10.3390/app11167517
Chicago/Turabian StyleDvoynikov, Mikhail V., Dmitry I. Sidorkin, Andrey A. Kunshin, and Danil A. Kovalev. 2021. "Development of Hydraulic Turbodrills for Deep Well Drilling" Applied Sciences 11, no. 16: 7517. https://doi.org/10.3390/app11167517
APA StyleDvoynikov, M. V., Sidorkin, D. I., Kunshin, A. A., & Kovalev, D. A. (2021). Development of Hydraulic Turbodrills for Deep Well Drilling. Applied Sciences, 11(16), 7517. https://doi.org/10.3390/app11167517





