New Orthogonal Transforms for Signal and Image Processing
Abstract
1. Introduction
2. Orthogonal Generalized Transform Matrix
- .
- .
- —transpose matrix I—unit matrix.
- —inverse matrix.
- C—coefficients .
- —positive real number.
3. Exponential Form of the Transform Matrix
- a—any real number, .
- k—integer, .
- .
- , —the elements of basis sequences for matrices and , respectively.
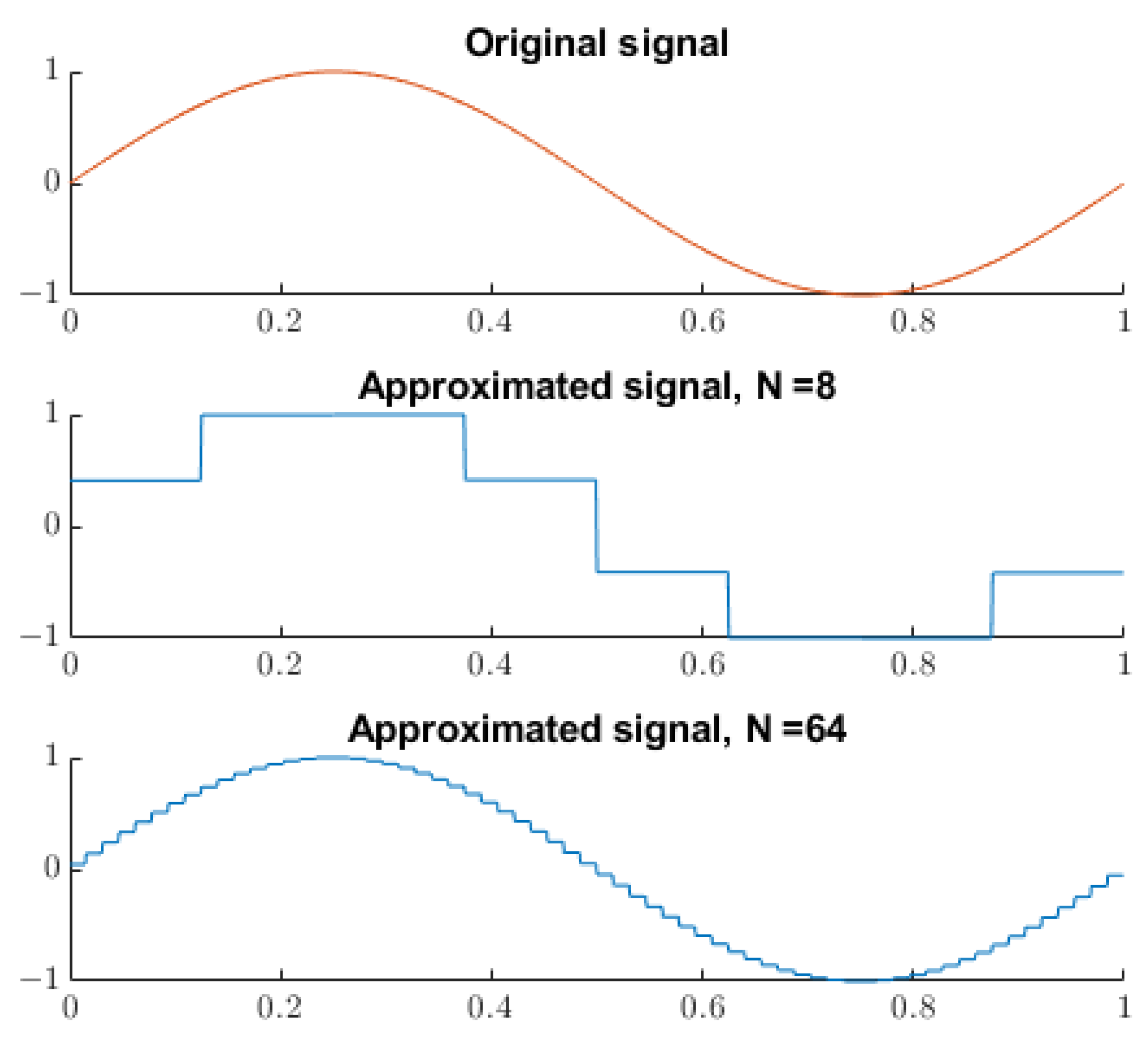
4. Signal Approximation—Orthogonal Expansion
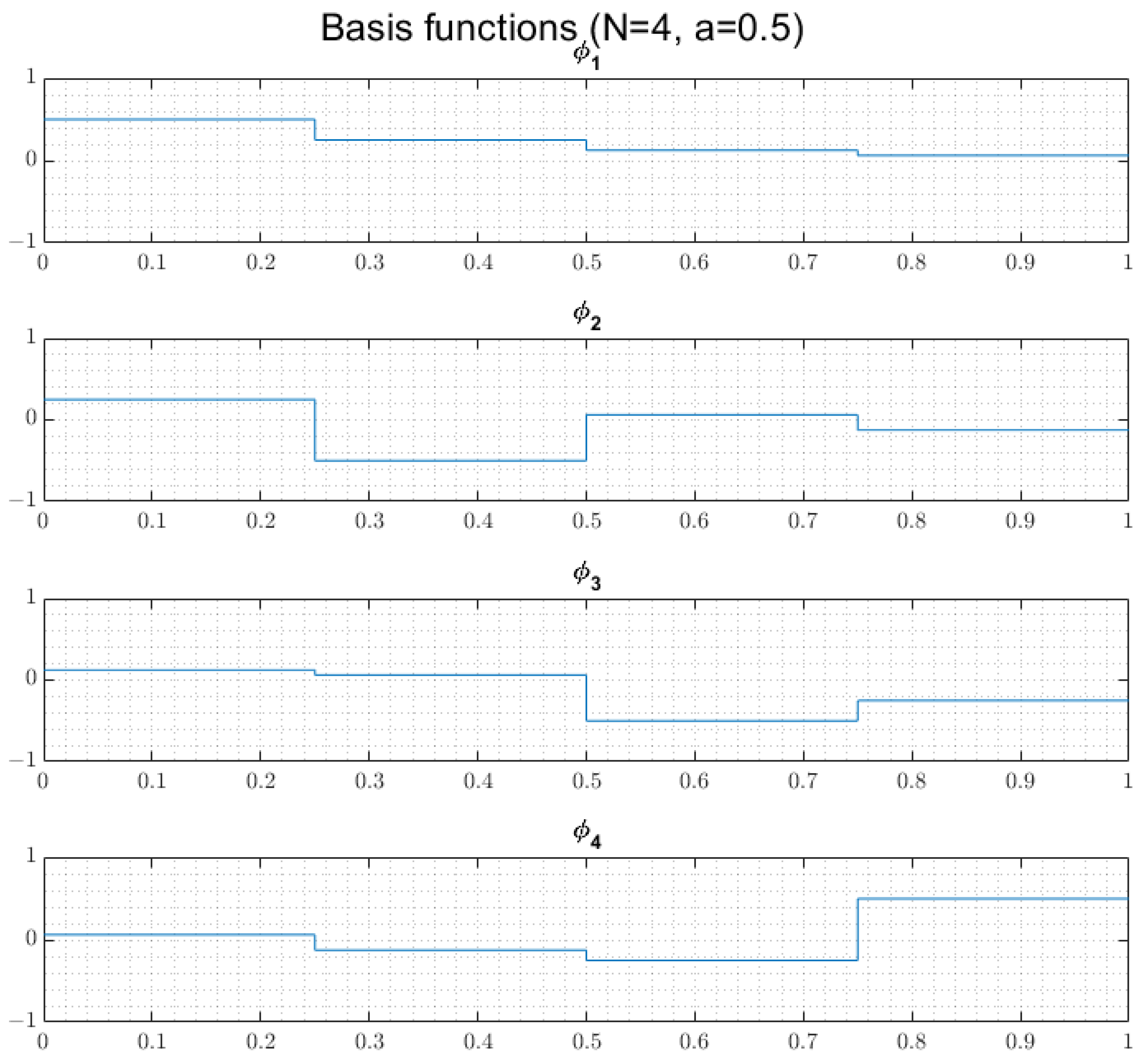
5. Proposed Orthogonal Transform and Its Modifications
5.1. One-Dimensional (1D) Transform
- —vector of spectral components.
- —vector of 1D signal.
- —transform matrix defined by Equation (15).
- C—constant defined by Equation (16).
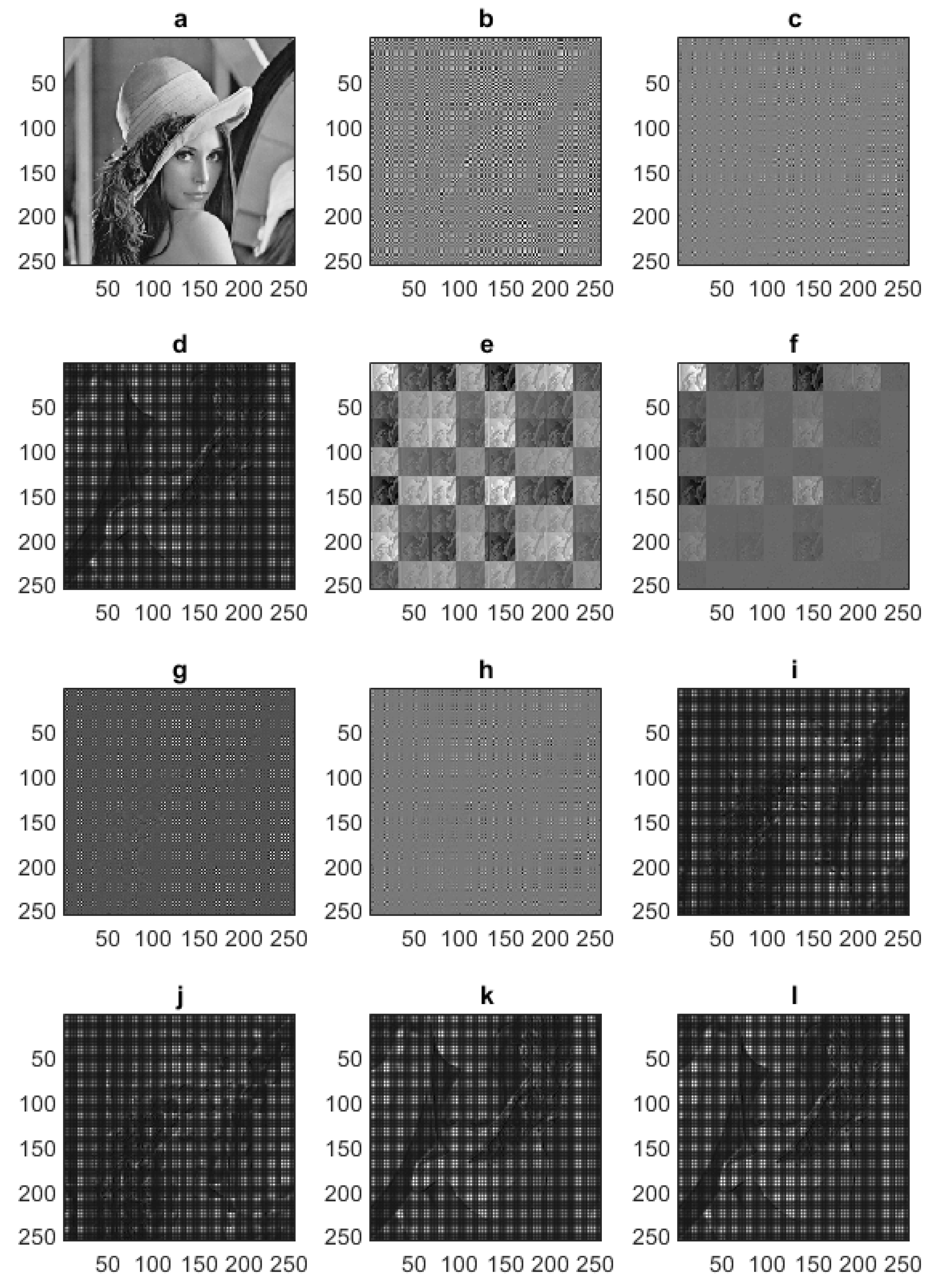
5.2. Two-Dimensional (2D) Transform
- —matrix of spectral components.
- —matrix of 2D signal.
- —transform matrix described by Equation (15).
- C—constant described by Equation (16).
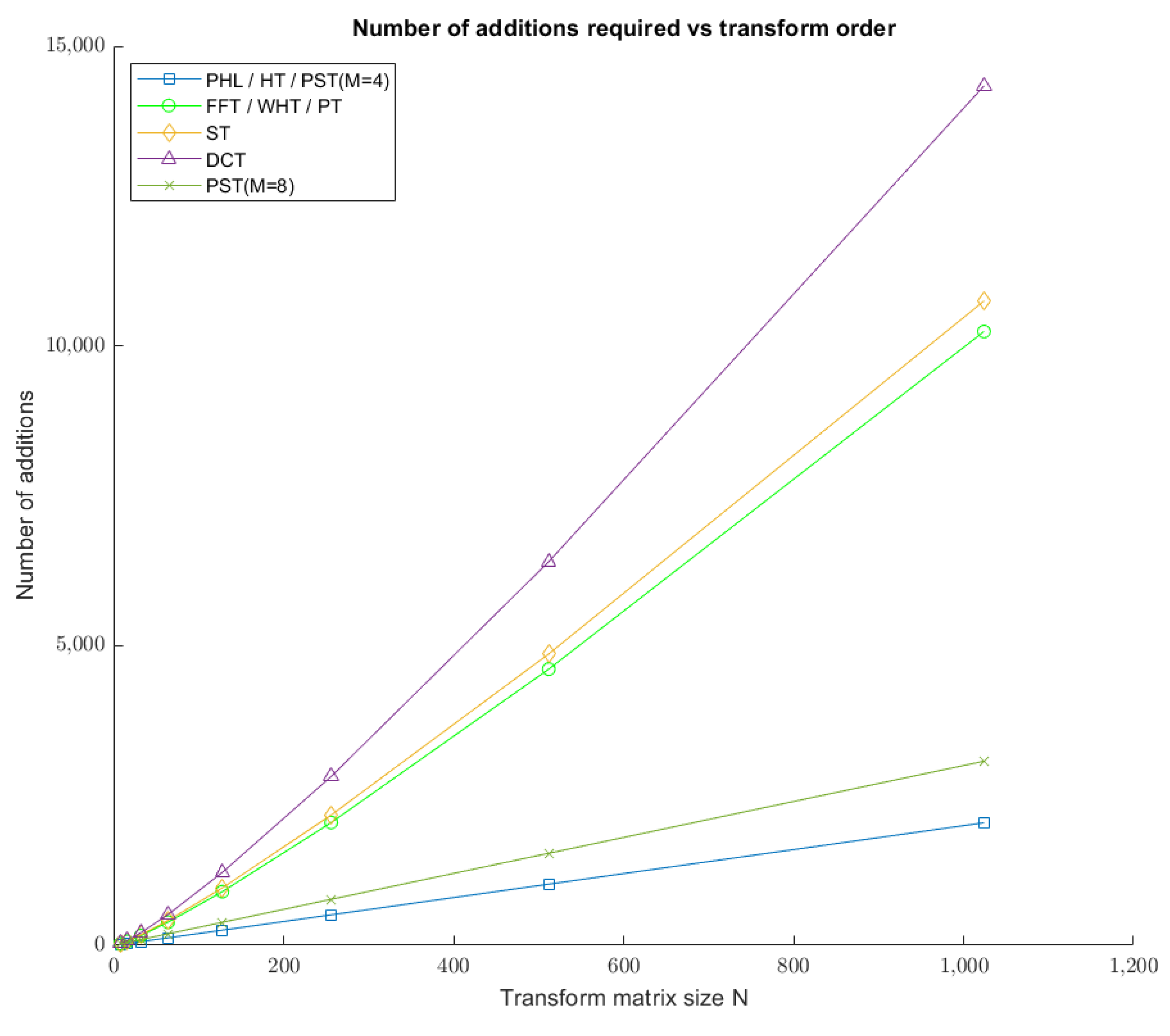
6. Fast Algorithms
7. Conclusions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Ahmed, N.; Rao, K.R. Orthogonal Transforms for Digital Signal Processing; Springer: Berlin/Heidelberg, Germany, 1975. [Google Scholar]
- Wang, R. Introduction to Orthogonal Transforms: With Applications in Data Processing and Analysis; Cambridge University Press: Cambridge, UK, 2012. [Google Scholar]
- Johnson, J.; Puschel, M. In search of the optimal Walsh–Hadamard transform. In Proceedings of the 2000 IEEE International Conference on Acoustics, Speech, and Signal Processing, Istanbul, Turkey, 5–9 June 2000; Volume 6, pp. 3347–3350. [Google Scholar]
- Gonzalez, R.; Woods, R. Digital Image Processing, 4th ed.; Pearson: London, UK, 2018. [Google Scholar]
- Madisetti, V. The Digital Signal Processing Handbook; CRC Press: Boca Raton, FL, USA, 2010. [Google Scholar]
- Ashrafi, A. Chapter One—Walsh–Hadamard Transforms: A Review; Advances in Imaging and Electron Physics; Elsevier: Amsterdam, The Netherlands, 2017. [Google Scholar]
- Sayood, K. Chapter 13—Transform Coding. Introduction to Data Compression, 5th ed.; The Morgan Kaufmann Series in Multimedia Information and Systems; Morgan Kaufmann: Burlington, MA, USA, 2018. [Google Scholar]
- Hamood, M.; Boussakta, S. Fast Walsh–Hadamard–Fourier Transform Algorithm. IEEE Trans. Signal Process. 2011, 59, 5627–5631. [Google Scholar] [CrossRef]
- Thompson, A. The Cascading Haar Wavelet Algorithm for Computing the Walsh–Hadamard Transform. IEEE Signal Process. Lett. 2017, 24, 1020–1023. [Google Scholar] [CrossRef]
- Korus, P.; Dziech, A. Efficient Method for Content Reconstruction With Self-Embedding. IEEE Trans. Image Process. 2013, 22, 1134–1147. [Google Scholar] [CrossRef] [PubMed]
- Korus, P.; Dziech, A. Adaptive Self-Embedding Scheme With Controlled Reconstruction Performance. IEEE Trans. Inf. Forensics Secur. 2014, 9, 169–181. [Google Scholar] [CrossRef]
- Kalarikkal Pullayikodi, S.; Tarhuni, N.; Ahmed, A.; Shiginah, F.B. Computationally Efficient Robust Color Image Watermarking Using Fast Walsh Hadamard Transform. J. Imaging 2017, 3, 46. [Google Scholar] [CrossRef]
- Pan, H.; Dabawi, D.; Cetin, A. Fast Walsh–Hadamard Transform and Smooth-Thresholding Based Binary Layers in Deep Neural Networks. In Proceedings of the IEEE/CVF Conference on Computer Vision and Pattern Recognition (CVPR) Workshops, Virtual Conference, 19–25 June 2021. [Google Scholar]
- Subathra, M.S.P.; Mohammed, M.A.; Maashi, M.S.; Garcia-Zapirain, B.; Sairamya, N.J.; George, S.T. Detection of Focal and Non-Focal Electroencephalogram Signals Using Fast Walsh–Hadamard Transform and Artificial Neural Network. Sensors 2020, 20, 4952. [Google Scholar]
- Wang, X.; Liang, X.; Zheng, J.; Zhou, H. Fast detection and segmentation of partial image blur based on discrete Walsh–Hadamard transform. Signal Process. Image Commun. 2019, 70, 47–56. [Google Scholar] [CrossRef]
- Andrushia, A.D.; Thangarjan, R. Saliency-Based Image Compression Using Walsh–Hadamard Transform (WHT). In Biologically Rationalized Computing Techniques for Image Processing Applications; Springer: Cham, Switzerland, 2018. [Google Scholar]
- Aristidi, E. Representation of Signals as Series of Orthogonal Functions. EAS Publ. Ser. 2016, 78–79, 99–126. [Google Scholar] [CrossRef][Green Version]
- Dziech, A.; Ślusarczyk, P.; Tibken, B. Methods of Image Compression by PHL Transform. J. Intell. Robot. Syst. 2004, 39, 447–458. [Google Scholar] [CrossRef]

Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Dziech, A. New Orthogonal Transforms for Signal and Image Processing. Appl. Sci. 2021, 11, 7433. https://doi.org/10.3390/app11167433
Dziech A. New Orthogonal Transforms for Signal and Image Processing. Applied Sciences. 2021; 11(16):7433. https://doi.org/10.3390/app11167433
Chicago/Turabian StyleDziech, Andrzej. 2021. "New Orthogonal Transforms for Signal and Image Processing" Applied Sciences 11, no. 16: 7433. https://doi.org/10.3390/app11167433
APA StyleDziech, A. (2021). New Orthogonal Transforms for Signal and Image Processing. Applied Sciences, 11(16), 7433. https://doi.org/10.3390/app11167433





