Unexpected Ferromagnetism—A Review
Abstract
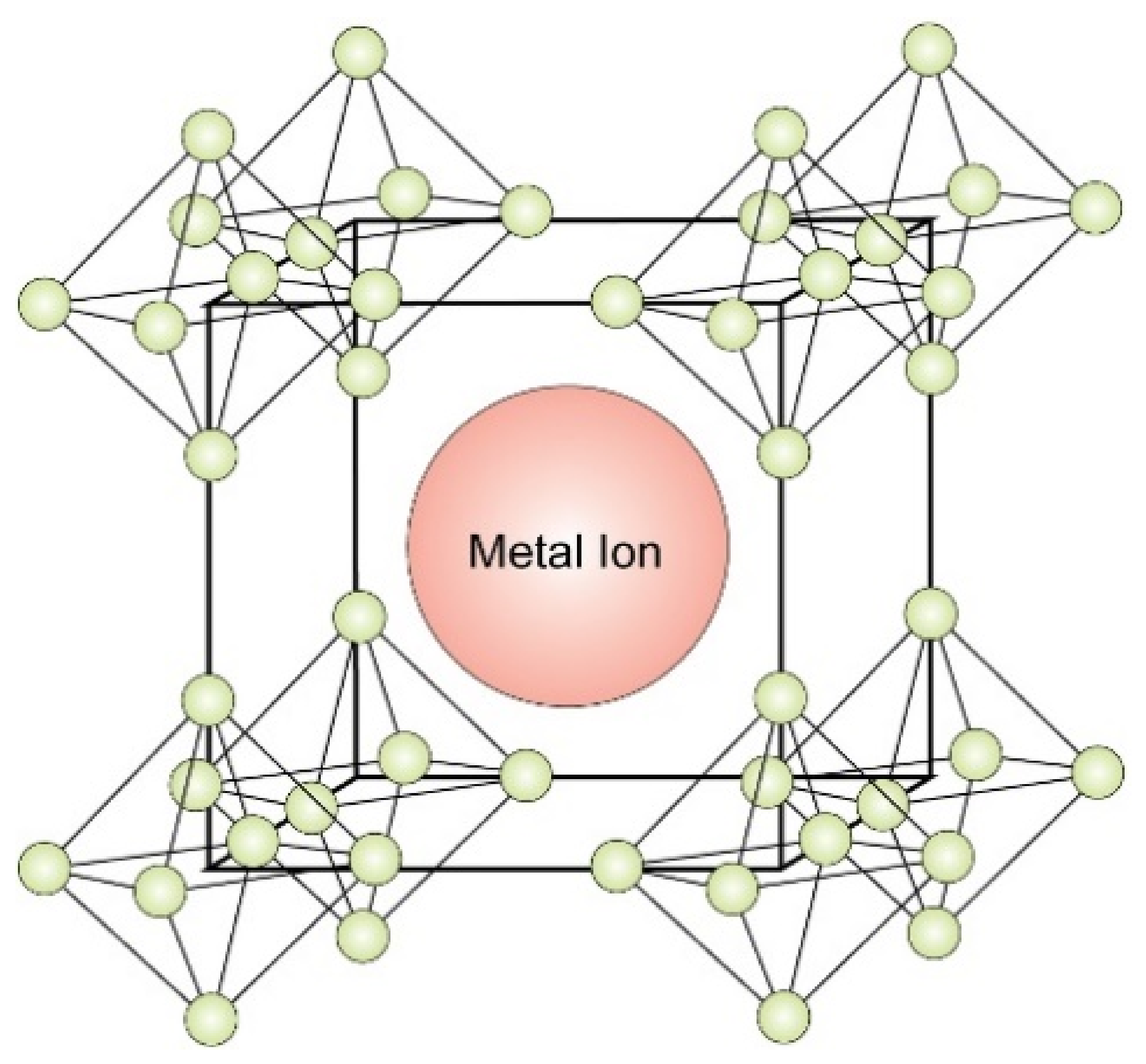
1. Conventional vs. d0 Magnetism
2. Examples of d0 Ferromagnetism
2.1. Alkaline-Earth Hexaborides
2.2. Non-Magnetic Oxides
2.2.1. HfO2
2.2.2. MgO
2.2.3. CeO2
2.2.4. ZnO
2.3. Carbon Nanostructures
2.3.1. Fullerenes
2.3.2. Graphene
2.3.3. Graphite
2.3.4. Carbon Nanotubes
3. Drawbacks of Unexpected Ferromagnetism
- -
- Material contaminations in a homogeneous manner which seems to be proportional to their weight and can be identified by careful chemical analysis; and
- -
- Specific contaminations during the procedure of measurement, which include the sample fixation in a certain geometry inside apparatus and that can produce a false signal, which do not depend on the sample mass and can be due to stainless-steel tools such as tweezers or fine blades, polyimide Kapton tape, plastic tubes (straws), gelatin capsules, cotton, silver conductive paint, and inks iron/iron oxide-contaminated or even anisotropy artifacts [114]. For example, the sticky Kapton tape, which is usually employed to attach the samples on their holders, can be simply contaminated by magnetic air dust microscopic particles and can induce ferromagnetic or paramagnetic signals between 10−4 and 10−5 emu [113].
- -
- False (spurious) ferromagnetism originating from the magnetometer magnetic field non-uniformity, resulting in errors that become important for the case of nanomaterials with a magnetic signal much lower than those of the diamagnetic signal of their substrate;
- -
- Features associated with non-intrinsic ferromagnetism (such as those derived from the asymmetry of ferromagnetic volume versus the center of the moment in the case of the uniformly magnetized diamagnetic sample);
- -
- Intrinsic-like features associated with non-intrinsic ferromagnetism (anisotropy artifacts linked to axial or radial effects).
4. Applications and Future Trends
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Singh, R. Unexpected magnetism in nanomaterials. J. Magn. Magn. Mater. 2013, 346, 58–73. [Google Scholar] [CrossRef]
- Makarova, T. Nanomagnetism in Otherwise Nonmagnetic Materials. arXiv, 2009; arXiv:0904.1550. [Google Scholar]
- Coey, J.M.D.; Venkatesan, M.; Fitzgerald, C.B. Donor impurity band exchange in dilute ferromagnetic oxides. Nat. Mater. 2005, 4, 173–179. [Google Scholar] [CrossRef] [PubMed]
- Das Sarma, S.; Hwang, E.H.; Kaminski, A. Temperature-dependent magnetization in diluted magnetic semiconductors. Phys. Rev. B 2003, 67, 155201. [Google Scholar] [CrossRef]
- Kaminski, A.; Das Sarma, S. Polaron Percolation in Diluted Magnetic Semiconductors. Phys. Rev. Lett. 2002, 88, 247202. [Google Scholar] [CrossRef]
- Coey, J.M.D.; Wongsaprom, K.; Alaria, J.; Venkatesan, M. Charge-transfer ferromagnetism in oxide nanoparticles. J. Phys. D Appl. Phys. 2008, 41, 134012. [Google Scholar] [CrossRef]
- Dietl, T.; Haury, A.; Merle d’Aubigné, Y. Free carrier-induced ferromagnetism in structures of diluted magnetic semiconductors. Phys. Rev. B 1997, 55, R3347–R3350. [Google Scholar] [CrossRef]
- Dietl, T.; Ohno, H.; Matsukura, F.; Cibert, J.; Ferrand, D. Zener Model Description of Ferromagnetism in Zinc-Blende Magnetic Semiconductors. Science 2000, 287, 1019. [Google Scholar] [CrossRef]
- Dietl, T. Origin of ferromagnetic response in diluted magnetic semiconductors and oxides. J. Phys. Condens. Matter 2007, 19, 165204. [Google Scholar] [CrossRef]
- Yokoyama, M.; Yamaguchi, H.; Ogawa, T.; Tanaka, M. Zinc-blende-type MnAs nanoclusters embedded in GaAs. J. Appl. Phys. 2005, 97, 10D317. [Google Scholar] [CrossRef]
- Karczewski, G.; Sawicki, M.; Ivanov, V.; Ruester, C.; Grabecki, G.; Matsukura, F.; Molenkamp, L.W.; Dietl, T. Ferromagnetism in (Zn,Cr)Se Layers Grown by Molecular Beam Epitaxy. J. Supercond. 2003, 16, 55–58. [Google Scholar] [CrossRef]
- Saito, H.; Zayets, V.; Yamagata, S.; Ando, K. Room-Temperature Ferromagnetism in a II-VI Diluted Magnetic Semiconductor Zn1−xCrxTe. Phys. Rev. Lett. 2003, 90, 207202. [Google Scholar] [CrossRef]
- Cahill, J.T.; Graeve, O.A. Hexaborides: A review of structure, synthesis and processing. J. Mater. Res. Technol. 2019, 8, 6321–6335. [Google Scholar] [CrossRef]
- Mohanta, S.K.; Mishra, S.N. Electronic structure and magnetic moment of dilute transition metal impurities in semi-metallic CaB6. J. Magn. Magn. Mater. 2017, 444, 349–353. [Google Scholar] [CrossRef]
- Young, D.P.; Hall, D.; Torelli, M.E.; Fisk, Z.; Sarrao, J.L.; Thompson, J.D.; Ott, H.R.; Oseroff, S.B.; Goodrich, R.G.; Zysler, R. High-temperature weak ferromagnetism in a low-density free-electron gas. Nature 1999, 397, 412–414. [Google Scholar] [CrossRef]
- Moriwaka, T.; Nishioka, T.; Sato Noriaki, K. Ferromagnetism Induced by Ca Vacancy in CaB 6. J. Phys. Soc. Japan 2001, 70, 341–344. [Google Scholar] [CrossRef]
- Lofland, S.E.; Seaman, B.; Ramanujachary, K.V.; Hur, N.; Cheong, S.W. Defect driven magnetism in calcium hexaboride. Phys. Rev. B 2003, 67, 020410. [Google Scholar] [CrossRef]
- Dorneles, L.S.; Venkatesan, M.; Moliner, M.; Lunney, J.G.; Coey, J.M.D. Magnetism in thin films of CaB6 and SrB6. Appl. Phys. Lett. 2004, 85, 6377–6379. [Google Scholar] [CrossRef]
- Ackland, K.; Venkatesan, M.; Coey, J.M.D. Magnetism of BaB6 thin films synthesized by pulsed laser deposition. J. Appl. Phys. 2012, 111, 07A322. [Google Scholar] [CrossRef]
- Bao, L.; Qi, X.; Tana; Chao, L.; Tegus, O. Synthesis, and magnetic and optical properties of nanocrystalline alkaline-earth hexaborides. CrystEngComm 2016, 18, 1223–1229. [Google Scholar] [CrossRef]
- Koch, R.; Metz, P.C.; Jaime, O.; Vargas-Consuelos, C.I.; Borja-Urby, R.; Ko, J.Y.P.; Cahill, J.T.; Edwards, D.; Vasquez, V.R.; Graeve, O.A.; et al. Nanodomains and local structure in ternary alkaline-earth hexaborides. J. Appl. Crystallogr. 2018, 51, 1445–1454. [Google Scholar] [CrossRef]
- Muñoz, M.C.; Gallego, S.; Sanchez, N. Surface ferromagnetism in non-magnetic and dilute magnetic oxides. J. Phys. Conf. Ser. 2011, 303, 012001. [Google Scholar] [CrossRef]
- Yates, K. Dilute Magnetic Oxides: Current Status and Prospects. In Nanomagnetism and Spintronics; World Scientific: Singapore, 2010; pp. 223–265. [Google Scholar]
- Venkatesan, M.; Fitzgerald, C.B.; Coey, J.M.D. Unexpected magnetism in a dielectric oxide. Nature 2004, 430, 630. [Google Scholar] [CrossRef] [PubMed]
- Xie, Q.; Wang, W.-P.; Xie, Z.; Zhan, P.; Li, Z.-C.; Zhang, Z.-J. Room temperature ferromagnetism in un-doped amorphous HfO 2 nano-helix arrays. Chin. Phys. B 2015, 24, 057503. [Google Scholar] [CrossRef]
- Zhang, Q.; Chen, G.; Yunoki, S. Surface ferromagnetism in HfO2 induced by excess oxygen. Solid State Commun. 2017, 252, 33–39. [Google Scholar] [CrossRef]
- Singh, P.J.; Chae, H.K. d° Ferromagnetism of Magnesium Oxide. Condens. Matter 2017, 2, 36. [Google Scholar] [CrossRef]
- Hassabo, A.G.; Mohamed, A.L. Novel flame retardant and antibacterial agent containing MgO NPs, phosphorus, nitrogen and silicon units for functionalise cotton fabrics. Biointerface Res. Appl. Chem. 2019, 9, 4272–4278. [Google Scholar] [CrossRef]
- Droghetti, A.; Sanvito, S. Electron doping and magnetic moment formation in N- and C-doped MgO. Appl. Phys. Lett. 2009, 94, 252505. [Google Scholar] [CrossRef]
- Araujo, C.M.; Kapilashrami, M.; Jun, X.; Jayakumar, O.D.; Nagar, S.; Wu, Y.; Århammar, C.; Johansson, B.; Belova, L.; Ahuja, R.; et al. Room temperature ferromagnetism in pristine MgO thin films. Appl. Phys. Lett. 2010, 96, 232505. [Google Scholar] [CrossRef]
- Li, J.; Jiang, Y.; Li, Y.; Yang, D.; Xu, Y.; Yan, M. Origin of room temperature ferromagnetism in MgO films. Appl. Phys. Lett. 2013, 102, 072406. [Google Scholar] [CrossRef]
- Mahadeva, S.K.; Fan, J.; Biswas, A.; Sreelatha, K.S.; Belova, L.; Rao, K.V. Magnetism of Amorphous and Nano-Crystallized Dc-Sputter-Deposited MgO Thin Films. Nanomaterials 2013, 3, 486–497. [Google Scholar] [CrossRef]
- Wu, Y.; Yang, X.; Li, J.; Rao, K.V.; Belova, L. Solution processed room temperature ferromagnetic MgO thin films printed by inkjet technique. Mater. Lett. 2017, 196, 388–391. [Google Scholar] [CrossRef]
- Khamkongkaeo, A.; Mothaneeyachart, N.; Sriwattana, P.; Boonchuduang, T.; Phetrattanarangsi, T.; Thongchai, C.; Sakkomolsri, B.; Pimsawat, A.; Daengsakul, S.; Phumying, S.; et al. Ferromagnetism and diamagnetism behaviors of MgO synthesized via thermal decomposition method. J. Alloy. Compd. 2017, 705, 668–674. [Google Scholar] [CrossRef]
- Shojaee, A.; Mostafavi, A.; Shamspur, T.; Fathirad, F. Green synthesis of cerium oxide nanoparticles: Characterization, parameters optimization and investigation of photocatalytic application. Biointerface Res. Appl. Chem. 2020, 10, 5932–5937. [Google Scholar] [CrossRef]
- Han, X.; Lee, J.; Yoo, H.-I. Oxygen-vacancy-induced ferromagnetism in CeO2 from first principles. Phys. Rev. B 2009, 79. [Google Scholar] [CrossRef]
- Sundaresan, A.; Bhargavi, R.; Rangarajan, N.; Siddesh, U.; Rao, C.N.R. Ferromagnetism as a universal feature of nanoparticles of the otherwise non-magnetic oxides. Phys. Rev. B 2006, 74, 161306. [Google Scholar] [CrossRef]
- Killivalavan, G.; Prabakar, A.C.; Naidu, K.C.B.; Sathyaseelan, B.; Rameshkumar, G.; Sivakumar, D.; Senthilnathan, K.; Baskaran, I.; Manikandan, E.; Rao, B.R. Synthesis and characterization of pure and Cu doped CeO2 nanoparticles: Photocatalytic and antibacterial activities evaluation. Biointerface Res. Appl. Chem. 2020, 10, 5306–5311. [Google Scholar] [CrossRef]
- Liu, Y.; Lockman, Z.; Aziz, A.; MacManus-Driscoll, J. Size dependent ferromagnetism in cerium oxide (CeO2) nanostructures independent of oxygen vacancies. J. Phys. Condens. Matter 2008, 20, 165201. [Google Scholar] [CrossRef]
- Fernandes, V.; Mossanek, R.J.O.; Schio, P.; Klein, J.J.; de Oliveira, A.J.A.; Ortiz, W.A.; Mattoso, N.; Varalda, J.; Schreiner, W.H.; Abbate, M.; et al. Dilute-defect magnetism: Origin of magnetism in nanocrystalline CeO2. Phys. Rev. B 2009, 80, 035202. [Google Scholar] [CrossRef]
- Ribeiro, A.; Ferreira, N. Systematic study of the physical origin of ferromagnetism in CeO 2−δ nanoparticles. Phys. Rev. B 2017, 95. [Google Scholar] [CrossRef]
- Paidi, V.K.; Brewe, D.L.; Freeland, J.W.; Roberts, C.A.; van Lierop, J. Role of Ce $4f$ hybridization in the origin of magnetism in nanoceria. Phys. Rev. B 2019, 99, 180403. [Google Scholar] [CrossRef]
- Varalda, J.; Dartora, C.A.; de Camargo, P.C.; de Oliveira, A.J.A.; Mosca, D.H. Oxygen diffusion and vacancy migration thermally-activated govern high-temperature magnetism in ceria. Sci. Rep. 2019, 9, 4708. [Google Scholar] [CrossRef] [PubMed]
- Straumal, B.B.; Protasova, S.G.; Mazilkin, A.A.; Goering, E.; Schütz, G.; Straumal, P.B.; Baretzky, B. Ferromagnetic behaviour of ZnO: The role of grain boundaries. Beilstein J. Nanotechnol. 2016, 7, 1936–1947. [Google Scholar] [CrossRef] [PubMed]
- Sato, K.; Katayama-Yoshida, H. First principles materials design for semiconductor spintronics. Semicond. Sci. Technol. 2002, 17, 367–376. [Google Scholar] [CrossRef]
- Droepenu, E.K.; Wee, B.S.; Chin, S.F.; Kok, K.Y.; Asare, E.A. Synthesis and characterization of single phase ZnO nanostructures via solvothermal method: Influence of alkaline source. Biointerface Res. Appl. Chem. 2020, 10, 5648–5655. [Google Scholar] [CrossRef]
- Lin, X.-L.; Yan, S.-S.; Zhao, M.-W.; Hu, S.-J.; Han, C.; Chen, Y.-X.; Liu, G.-L.; Dai, Y.-Y.; Mei, L.-M. Possible origin of ferromagnetism in un-doped ZnO: First-principles calculations. Phys. Lett. A 2011, 375, 638–641. [Google Scholar] [CrossRef]
- Wu, H.; Stroppa, A.; Sakong, S.; Picozzi, S.; Scheffler, M.; Kratzer, P. Magnetism in C- or N-doped MgO and ZnO: A Density-Functional Study of Impurity Pairs. Phys. Rev. Lett. 2010, 105, 267203. [Google Scholar] [CrossRef]
- Straumal, B.B.; Mazilkin, A.A.; Protasova, S.G.; Myatiev, A.A.; Straumal, P.B.; Schütz, G.; van Aken, P.A.; Goering, E.; Baretzky, B. Magnetization study of nanograined pure and Mn-doped ZnO films: Formation of a ferromagnetic grain-boundary foam. Phys. Rev. B 2009, 79, 205206. [Google Scholar] [CrossRef]
- Pazhanivelu, D.V.; Blessington, P.; Rathinasamy, M. Unexpected ferromagnetism in Ist group elements doped ZnO based DMS nanoparticles. Mater. Lett. 2015, 151. [Google Scholar] [CrossRef]
- Gao, D.; Zhang, Z.; Fu, J.; Xu, Y.; Qi, J.; Xue, D. Room temperature ferromagnetism of pure ZnO nanoparticles. J. Appl. Phys. 2009, 105, 113928. [Google Scholar] [CrossRef]
- Sun, Y.; Zong, Y.; Feng, J.; Li, X.; Yan, F.; Lan, Y.; Zhang, L.; Ren, Z.; Zheng, X. Oxygen vacancies driven size-dependent d0 room temperature ferromagnetism in well-dispersed dopant-free ZnO nanoparticles and density functional theory calculation. J. Alloy. Compd. 2018, 739, 1080–1088. [Google Scholar] [CrossRef]
- Wang, Y.; Huang, Y.; Song, Y.; Zhang, X.; Ma, Y.; Liang, J.; Chen, Y. Room-Temperature Ferromagnetism of Graphene. Nano Lett. 2009, 9, 220–224. [Google Scholar] [CrossRef] [PubMed]
- Stamenov, P.; Coey, J.M.D. Magnetic susceptibility of carbon—Experiment and theory. J. Magn. Magn. Mater. 2005, 290–291, 279–285. [Google Scholar] [CrossRef]
- Kroto, H.W.; Heath, J.R.; O’Brien, S.C.; Curl, R.F.; Smalley, R.E. C60: Buckminsterfullerene. Nature 1985, 318, 162–163. [Google Scholar] [CrossRef]
- Höhne, R.; Esquinazi, P. Can Carbon Be Ferromagnetic? Adv. Mater. 2002, 14, 753–756. [Google Scholar] [CrossRef]
- Ata, M.; Machida, M.; Watanabe, H.; Seto, J.e. Polymer-C60Composite with Ferromagnetism. Jpn. J. Appl. Phys. 1994, 33, 1865–1871. [Google Scholar] [CrossRef]
- Makarova, T.L.; Sundqvist, B. Pressure-induced ferromagnetism of fullerenes. High. Press. Res. 2003, 23, 135–141. [Google Scholar] [CrossRef]
- Makarova, T. Magnetism in Polymerized Fullerenes. In Frontiers of Multifunctional Integrated Nanosystems; Springer: Dordrecht, The Netherlands, 2005; Volume 152, p. 331. [Google Scholar]
- Wood, R.A.; Lewis, M.H.; Lees, M.R.; Bennington, S.M.; Cain, M.G.; Kitamura, N. Ferromagnetic fullerene. J. Phys. Condens. Matter 2002, 14, L385–L391. [Google Scholar] [CrossRef]
- Murakami, Y.; Suematsu, H. Magnetism of C60 induced by photo-assisted oxidation. Pure Appl. Chem. 1996, 68, 1463–1467. [Google Scholar] [CrossRef][Green Version]
- Ghosh, S.; Tongay, S.; Hebard, A.F.; Sahin, H.; Peeters, F.M. Ferromagnetism in stacked bilayers of Pd/C60. J. Magn. Magn. Mater. 2014, 349, 128–134. [Google Scholar] [CrossRef]
- Vozmediano, M.A.H.; López-Sancho, M.P.; Stauber, T.; Guinea, F. Local defects and ferromagnetism in graphene layers. Phys. Rev. B 2005, 72, 155121. [Google Scholar] [CrossRef]
- Yazyev, O.V.; Helm, L. Defect-induced magnetism in graphene. Phys. Rev. B 2007, 75, 125408. [Google Scholar] [CrossRef]
- Radovic, L.R.; Bockrath, B. On the Chemical Nature of Graphene Edges: Origin of Stability and Potential for Magnetism in Carbon Materials. J. Am. Chem. Soc. 2005, 127, 5917–5927. [Google Scholar] [CrossRef]
- Wang, W.L.; Meng, S.; Kaxiras, E. Graphene NanoFlakes with Large Spin. Nano Letters 2008, 8, 241–245. [Google Scholar] [CrossRef]
- Sahu, V.; Maurya, V.K.; Singh, G.; Patnaik, S.; Sharma, R.K. Enhanced ferromagnetism in edge enriched holey/lacey reduced graphene oxide nanoribbons. Mater. Design 2017, 132, 295–301. [Google Scholar] [CrossRef]
- Sharpe, A.L.; Fox, E.J.; Barnard, A.W.; Finney, J.; Watanabe, K.; Taniguchi, T.; Kastner, M.A.; Goldhaber-Gordon, D. Emergent ferromagnetism near three-quarters filling in twisted bilayer graphene. Science 2019, 365, 605. [Google Scholar] [CrossRef] [PubMed]
- Tuček, J.; Holá, K.; Zoppellaro, G.; Błoński, P.; Langer, R.; Medved’, M.; Susi, T.; Otyepka, M.; Zbořil, R. Zigzag sp2 Carbon Chains Passing through an sp3 Framework: A Driving Force toward Room-Temperature Ferromagnetic Graphene. ACS Nano 2018, 12, 12847–12859. [Google Scholar] [CrossRef] [PubMed]
- Badry, R.; Radwan, S.H.; Ezzat, D.; Ezzat, H.; Elhaes, H.; Ibrahim, M. Study of the Electronic Properties of Graphene Oxide/(PANi/Teflon). Biointerface Res. Appl. Chem. 2020, 10, 6926–6935. [Google Scholar] [CrossRef]
- Daoudi, K.; Gaidi, M.; Columbus, S. Silver nanoprisms/graphene oxide/silicon nanowires composites for R6G surface-enhanced Raman spectroscopy sensor. Biointerface Res. Appl. Chem. 2020, 10, 5670–5674. [Google Scholar] [CrossRef]
- Sun, X.L.; Qi, Z.; Li, Q.Q.; Zhang, X.R.; Shao, X.L.; Jin, Y.; Zhang, J.J.; Liu, Y.Y. Utilization of carbon nanotube and graphene in electrochemical CO2 reduction. Biointerface Res. Appl. Chem. 2020, 10, 5815–5827. [Google Scholar] [CrossRef]
- Swamy, B.K.; Shiprath, K.; Rakesh, G.; Ratnam, K.V.; Manjunatha, H.; Janardan, S.; Naidu, K.C.B.; Ramesh, S.; Suresh, K.; Ratnamala, A. Simultaneous detection of dopamine, tyrosine and ascorbic acid using NiO/graphene modified graphite electrode. Biointerface Res. Appl. Chem. 2020, 10, 5599–5609. [Google Scholar] [CrossRef]
- Lehtinen, P.O.; Foster, A.S.; Ma, Y.; Krasheninnikov, A.V.; Nieminen, R.M. Irradiation-induced magnetism in graphite: A density functional study. Phys. Rev. Lett. 2004, 93, 187202. [Google Scholar] [CrossRef] [PubMed]
- Mombrú, A.; Pardo, H.; Faccio, R.; Lima, O.; Leite, E.; Zanelatto, G.; Lanfredi, A.; Cardoso, C.; Araujo-Moreira, F. Multilevel ferromagnetic behavior of room-temperature bulk magnetic graphite. Phys. Rev. B 2005, 71. [Google Scholar] [CrossRef]
- Esquinazi, P.; Setzer, A.; Höhne, R.; Semmelhack, C.; Kopelevich, Y.; Spemann, D.; Butz, T.; Kohlstrunk, B.; Lösche, M. Ferromagnetism in oriented graphite samples. Phys. Rev. B 2002, 66, 024429. [Google Scholar] [CrossRef]
- He, Z.; Xia, H.; Zhou, X.; Yang, X.; Wang, T. Raman study of correlation between defects and ferromagnetism in graphite. J. Phys. D Appl. Phys. 2011, 44, 085001. [Google Scholar] [CrossRef]
- Yang, X.; Xia, H.; Qin, X.; Li, W.; Dai, Y.; Liu, X.; Zhao, M.; Xia, Y.; Yan, S.; Wang, B. Correlation between the vacancy defects and ferromagnetism in graphite. Carbon 2009, 47, 1399–1406. [Google Scholar] [CrossRef]
- Guevenilir, E.; Kincal, C.; Kamber, U.; Guerlue, O.; Yildiz, D.; Grygiel, C.; Van der Beek, C.J. Investigation of ferromagnetism on graphite due to swift heavy ion irradiation. In Verhandlungen der Deutschen Physikalischen Gesellschaft; International Atomic Energy Agency (IAEA): Dresden, Germany, 2017; Volume 1. [Google Scholar]
- Haroun, A.A.; Ahmed, H.M.; Mossa, A.T.H.; Mohafrash, S.M.; Ahmed, E.F. Production, characterization and immobilization of Aspergillus versicolor L-asparaginase onto multi-walled carbon nanotubes. Biointerface Res. Appl. Chem. 2020, 10, 5733–5740. [Google Scholar] [CrossRef]
- Céspedes, O.; Ferreira, M.S.; Sanvito, S.; Kociak, M.; Coey, J.M.D. Contact induced magnetism in carbon nanotubes. J. Phys. Condens. Matter 2004, 16, L155–L161. [Google Scholar] [CrossRef]
- Friedman, A.L.; Chun, H.; Jung, Y.J.; Heiman, D.; Glaser, E.R.; Menon, L. Possible room-temperature ferromagnetism in hydrogenated carbon nanotubes. Phys. Rev. B 2010, 81, 115461. [Google Scholar] [CrossRef]
- Fang, Z.; Zhao, H.; Xiong, L.; Zhang, F.; Fu, Q.; Ma, Z.; Xu, C.; Lin, Z.; Wang, H.; Hu, Z.; et al. Enhanced ferromagnetic properties of N2 plasma-treated carbon nanotubes. J. Mater. Sci. 2019, 54, 2307–2314. [Google Scholar] [CrossRef]
- Kim, D.W.; Lee, K.W.; Lee, C.E. Defect-induced room-temperature ferromagnetism in single-walled carbon nanotubes. J. Magn. Magn. Mater. 2018, 460, 397–400. [Google Scholar] [CrossRef]
- Singh, S.B.; Wang, Y.-F.; Shao, Y.-C.; Lai, H.-Y.; Hsieh, S.-H.; Limaye, M.V.; Chuang, C.-H.; Hsueh, H.-C.; Wang, H.; Chiou, J.-W.; et al. Observation of the origin of d0 magnetism in ZnO nanostructures using X-ray-based microscopic and spectroscopic techniques. Nanoscale 2014, 6, 9166–9176. [Google Scholar] [CrossRef] [PubMed]
- Chaboy, J.; Boada, R.; Piquer, C.; Laguna-Marco, M.A.; García-Hernández, M.; Carmona, N.; Llopis, J.; Ruíz-González, M.L.; González-Calbet, J.; Fernández, J.F.; et al. Evidence of intrinsic magnetism in capped ZnO nanoparticles. Phys. Rev. B 2010, 82, 064411. [Google Scholar] [CrossRef]
- Zippel, J.; Lorenz, M.; Setzer, A.; Wagner, G.; Sobolev, N.; Esquinazi, P.; Grundmann, M. Defect-induced ferromagnetism in undoped and Mn-doped zirconia thin films. Phys. Rev. B 2010, 82, 125209. [Google Scholar] [CrossRef]
- Zhan, P.; Xie, Z.; Li, Z.; Wang, W.; Zhang, Z.; Li, Z.; Cheng, G.; Zhang, P.; Wang, B.; Cao, X. Origin of the defects-induced ferromagnetism in un-doped ZnO single crystals. Appl. Phys. Lett. 2013, 102, 071914. [Google Scholar] [CrossRef]
- Liu, Y.; Zhou, W.; Huang, Y.; Wu, P. Unexpected ferromagnetism in n-type polycrystalline K-doped ZnO films prepared by RF-magnetron sputtering. J. Mater. Sci. Mater. Electron. 2015, 26, 8451–8455. [Google Scholar] [CrossRef]
- Naji Aljawfi, R.; Rahman, F.; Shukla, D.K. Effect of the annealing temperature on the structural and magnetic properties of ZnO nanoparticles. Mater. Lett. 2013, 99, 18–20. [Google Scholar] [CrossRef]
- Zhan, P.; Wang, W.; Liu, C.; Hu, Y.; Li, Z.; Zhang, Z.; Zhang, P.; Wang, B.; Cao, X. Oxygen vacancy–induced ferromagnetism in un-doped ZnO thin films. J. Appl. Phys. 2012, 111, 033501. [Google Scholar] [CrossRef]
- Phan, T.-L.; Zhang, Y.D.; Yang, D.S.; Nghia, N.X.; Thanh, T.D.; Yu, S.C. Defect-induced ferromagnetism in ZnO nanoparticles prepared by mechanical milling. Appl. Phys. Lett. 2013, 102, 072408. [Google Scholar] [CrossRef]
- Das, J.; Pradhan, S.K.; Mishra, D.K.; Sahu, D.R.; Sarangi, S.; Varma, S.; Nayak, B.B.; Huang, J.-L.; Roul, B.K. Unusual ferromagnetism in high purity ZnO sintered ceramics. Mater. Res. Bull. 2011, 46, 42–47. [Google Scholar] [CrossRef]
- Poornaprakash, B.; Chalapathi, U.; Babu, S.; Park, S.-H. Structural, morphological, optical, and magnetic properties of Gd-doped and (Gd, Mn) co-doped ZnO nanoparticles. Phys. E Low-Dimens. Syst. Nanostructures 2017, 93, 111–115. [Google Scholar] [CrossRef]
- Zhu, M.; Zhang, Z.; Zhong, M.; Tariq, M.; Li, Y.; Li, W.; Jin, H.; Skotnicova, K.; Li, Y. Oxygen vacancy induced ferromagnetism in Cu-doped ZnO. Ceram. Int. 2016, 43. [Google Scholar] [CrossRef]
- Rumaiz, A.K.; Ali, B.; Ceylan, A.; Boggs, M.; Beebe, T.; Ismat Shah, S. Experimental studies on vacancy induced ferromagnetism in undoped TiO2. Solid State Commun. 2007, 144, 334–338. [Google Scholar] [CrossRef]
- Kim, D.; Hong, J.; Park, Y.R.; Kim, K.J. The origin of oxygen vacancy induced ferromagnetism in undoped TiO2. J. Phys. Condens. Matter 2009, 21, 195405. [Google Scholar] [CrossRef] [PubMed]
- Singhal, R.K.; Kumar, S.; Kumari, P.; Xing, Y.T.; Saitovitch, E. Evidence of defect-induced ferromagnetism and its “switch” action in pristine bulk TiO2. Appl. Phys. Lett. 2011, 98, 092510. [Google Scholar] [CrossRef]
- Sangaletti, L.; Mozzati, M.C.; Galinetto, P.; Azzoni, C.B.; Speghini, A.; Bettinelli, M.; Calestani, G. Ferromagnetism on a paramagnetic host background: The case of rutile TM:TiO2single crystals (TM = Cr, Mn, Fe, Co, Ni, Cu). J. Phys. Condens. Matter 2006, 18, 7643–7650. [Google Scholar] [CrossRef] [PubMed]
- Singhal, R.K.; Samariya, A.; Kumar, S.; Xing, Y.T.; Jain, D.C.; Dolia, S.N.; Deshpande, U.P.; Shripathi, T.; Saitovitch, E.B. Study of defect-induced ferromagnetism in hydrogenated anatase TiO2:Co. J. Appl. Phys. 2010, 107, 113916. [Google Scholar] [CrossRef]
- Potzger, K.; Osten, J.; Levin, A.A.; Shalimov, A.; Talut, G.; Reuther, H.; Arpaci, S.; Bürger, D.; Schmidt, H.; Nestler, T.; et al. Defect-induced ferromagnetism in crystalline SrTiO3. J. Magn. Magn. Mater. 2011, 323, 1551–1562. [Google Scholar] [CrossRef]
- Shi, S.; Gao, D.; Xu, Q.; Yang, Z.; Xue, D. Singly-charged oxygen vacancy-induced ferromagnetism in mechanically milled SnO2 powders. RSC Adv. 2014, 4, 45467–45472. [Google Scholar] [CrossRef]
- Mehraj, S.; Ansari, M.S.; Al-Ghamdi, A.A.; Alimuddin. Annealing dependent oxygen vacancies in SnO2 nanoparticles: Structural, electrical and their ferromagnetic behavior. Mater. Chem. Phys. 2016, 171, 109–118. [Google Scholar] [CrossRef]
- Chang, G.S.; Forrest, J.; Kurmaev, E.Z.; Morozovska, A.N.; Glinchuk, M.D.; McLeod, J.A.; Moewes, A.; Surkova, T.P.; Hong, N.H. Oxygen-vacancy-induced ferromagnetism in undoped SnO2 thin films. Phys. Rev. B 2012, 85, 165319. [Google Scholar] [CrossRef]
- Lee, Y.F.; Wu, F.; Kumar, R.; Hunte, F.; Schwartz, J.; Narayan, J. Epitaxial integration of dilute magnetic semiconductor Sr3SnO with Si (001). Appl. Phys. Lett. 2013, 103, 112101. [Google Scholar] [CrossRef]
- Qi, L.-Q.; Han, R.-S.; Liu, L.-H.; Sun, H.-Y. Preparation and magnetic properties of DC-sputtered porous HfO2 films. Ceram. Int. 2016, 42, 18925–18930. [Google Scholar] [CrossRef]
- Zhao, G.; Zhang, L.; Hu, L.; Yu, H.; Min, G.; Yu, H. Structure and magnetic properties of nanocrystalline CaB6 films deposited by magnetron sputtering. J. Alloy. Compd. 2014, 599, 175–178. [Google Scholar] [CrossRef]
- Bennett, M.C.; van Lierop, J.; Berkeley, E.M.; Mansfield, J.F.; Henderson, C.; Aronson, M.C.; Young, D.P.; Bianchi, A.; Fisk, Z.; Balakirev, F.; et al. Weak ferromagnetism in CaB6. Phys. Rev. B 2004, 69, 132407. [Google Scholar] [CrossRef]
- Coey, J.M.D. Publisher Correction: Magnetism in d0 oxides. Nat. Mater. 2019, 18, 770. [Google Scholar] [CrossRef] [PubMed]
- Simões Valentin, C.B.; de Sousa e Silva, R.L.; Banerjee, P.; Franco, A. Investigation of Fe-doped room temperature dilute magnetic ZnO semiconductors. Mater. Sci. Semicond. Process. 2019, 96, 122–126. [Google Scholar] [CrossRef]
- Elilarassi, R.; Chandrasekaran, G. Optical, electrical and ferromagnetic studies of ZnO:Fe diluted magnetic semiconductor nanoparticles for spintronic applications. Spectrochim. Acta Part. A Mol. Biomol. Spectrosc. 2017, 186, 120–131. [Google Scholar] [CrossRef]
- Mantovan, R.; Gunnlaugsson, H.P.; Johnston, K.; Masenda, H.; Mølholt, T.E.; Naidoo, D.; Ncube, M.; Shayestehaminzadeh, S.; Bharuth-Ram, K.; Fanciulli, M.; et al. Atomic-Scale Magnetic Properties of Truly 3d-Diluted ZnO. Adv. Electron. Mater. 2015, 1, 1400039. [Google Scholar] [CrossRef]
- Qi, B.; Ólafsson, S.; Gíslason, H.P. Vacancy defect-induced d0 ferromagnetism in undoped ZnO nanostructures: Controversial origin and challenges. Prog. Mater. Sci. 2017, 90, 45–74. [Google Scholar] [CrossRef]
- Garcia, M.A.; Fernandez Pinel, E.; de la Venta, J.; Quesada, A.; Bouzas, V.; Fernández, J.F.; Romero, J.J.; Martín González, M.S.; Costa-Krämer, J.L. Sources of experimental errors in the observation of nanoscale magnetism. J. Appl. Phys. 2009, 105, 013925. [Google Scholar] [CrossRef]
- Pereira, L.M.C. Experimentally evaluating the origin of dilute magnetism in nanomaterials. J. Phys. D Appl. Phys. 2017, 50, 393002. [Google Scholar] [CrossRef]
- Song, C.; Pan, F. Chapter Seven—Transition Metal-Doped Magnetic Oxides. In Semiconductors and Semimetals; Svensson, B.G., Pearton, S.J., Jagadish, C., Eds.; Elsevier: Amsterdam, The Netherlands, 2013; Volume 88, pp. 227–259. [Google Scholar]
- Wolf, S.A.; Chtchelkanova, A.Y.; Treger, D.M. Spintronics—A retrospective and perspective. IBM J. Res. Dev. 2006, 50, 101–110. [Google Scholar] [CrossRef]
- Sinova, J.; Žutić, I. New moves of the spintronics tango. Nat. Mater. 2012, 11, 368–371. [Google Scholar] [CrossRef]
- Wolf, S.A.; Awschalom, D.D.; Buhrman, R.A.; Daughton, J.M.; von Molnár, S.; Roukes, M.L.; Chtchelkanova, A.Y.; Treger, D.M. Spintronics: A Spin-Based Electronics Vision for the Future. Science 2001, 294, 1488. [Google Scholar] [CrossRef]
- Pan, F.; Song, C.; Liu, X.J.; Yang, Y.C.; Zeng, F. Ferromagnetism and possible application in spintronics of transition-metal-doped ZnO films. Mater. Sci. Eng. R Rep. 2008, 62, 1–35. [Google Scholar] [CrossRef]
- Zhu, J.-G.; Park, C. Magnetic tunnel junctions. Mater. Today 2006, 9, 36–45. [Google Scholar] [CrossRef]
- Toyosaki, H.; Fukumura, T.; Ueno, K.; Nakano, M.; Kawasaki, M. A Ferromagnetic Oxide Semiconductor as Spin Injection Electrode in Magnetic Tunnel Junction. Jpn. J. Appl. Phys. 2005, 44, L896–L898. [Google Scholar] [CrossRef]
- Liu, X.; Zeng, F.; Pan, F. Fully epitaxial (Zn,Co)O/ZnO/(Zn,Co)O junction and its tunnel magnetoresistance. Appl. Phys. Lett. 2007, 91, 042106. [Google Scholar] [CrossRef]
- Ohno, H.; Chiba, D.; Matsukura, F.; Omiya, T.; Abe, E.; Dietl, T.; Ohno, Y.; Ohtani, K. Electric-field control of ferromagnetism. Nature 2000, 408, 944–946. [Google Scholar] [CrossRef] [PubMed]
- Chiba, D.; Sawicki, M.; Nishitani, Y.; Nakatani, Y.; Matsukura, F.; Ohno, H. Magnetization vector manipulation by electric fields. Nature 2008, 455, 515–518. [Google Scholar] [CrossRef] [PubMed]
- Zhao, T.; Shinde, S.R.; Ogale, S.B.; Zheng, H.; Venkatesan, T.; Ramesh, R.; Das Sarma, S. Electric field effect in diluted magnetic insulator anatase Co: TiO2. Phys. Rev. Lett. 2005, 94, 126601. [Google Scholar] [CrossRef] [PubMed]
| System | Ref | Saturation Magnetization (MS) | Observations | Species Responsible for Ferromagnetic Behavior |
|---|---|---|---|---|
| Non-magnetic oxides | ||||
| ZnO nanowire (NW) arrays | [85] | 2.3 emu/cm3 (0.41 emu/g) at T = 300 K | The magnetic field applied parallel to the growth direction of elongated nanostructures. |
|
| ZnO nanocactuses on ZnO NW arrays | 3.1 emu/cm3 (0.55 emu/g) at T = 300 K | |||
| Tryoctylphosphine capped-ZnO nanoparticles (NPs) | [86] | 3 memu/g at T = 5 K | The sp conduction band is where the magnetic polarization of the Zn atoms is attained. |
|
| Dodecylaminne capped-ZnO NPs | 2.5 memu/g at T = 5 K | |||
| Dodecanethiol capped-ZnO NPs | 2.6 memu/g at T = 5 K | |||
| ZrO2 Mn-doped thin films | [87] | - 20% Mn (cubic phase) 0.83–0.96 emu/cm3 (0.15–0.17 emu/g) at T = 5 K - 10% Mn (monoclinic phase) 0.36 emu/cm3 (0.06 emu/g) at T= 5 K | At T = 5 K -> Hc coercive fields = 95 and 205 Oe. |
|
| ZnO single crystals | [88] | 6.3 × 10−5 emu/g T = 300 K (untreated sample) 1.6 × 10−4 emu/g T= 300 K (treated) | Curie temperature > 300 K. |
|
| Li, Na, and K-doped ZnO NPs | [50] | ZnO: 0.129 × 10−3 emu/g Zn0.95Na0.05O: 0.094 × 10−3 emu/g Zn0.95K0.05O: 0.219 × 10−3 emu/g Zn0.95Li0.05O: very weak ferromagnetic + diamagnetic at T = 300 K | Ion doping enhances FM behavior through exchange interactions. Decreased FM behavior for Li-doped ZnO at higher field. |
|
| K-doped ZnO films | [89] | 0%K: 4.4 emu/cm3 (0.79 emu/g) 4%K: 6.1 emu/cm3 (1.09 emu/g) 6%K: 7.3 emu/cm3 (1.3 emu/g) 8%K: 10.7 emu/cm3 (1.91 emu/g) 11%K: 3.5 emu/cm3 (0.63 emu/g) at T = 300 K | Maximum MS value at 8% K content. |
|
| ZnO NPs | [90] | 250 °C annealed: 0.004 emu/g 500 °C annealed: 0.0042 emu/g 750 °C annealed: 0.0024 emu/g 1000 °C annealed: 0.0018 emu/g at T = 300 K | By increasing the annealing temperature, the MS decreases. |
|
| ZnO thin film | [91] | 150 °C annealed: 0.08 emu/g 600 °C annealed: 0.42 emu/g at T = 300 K | - induced defects by thermal annealing in argon flow |
|
| ZnO NPs | [92] | Raw NPs: diamagnetic after 50 h milling: 0.031 emu/g after 100 h milling: 0.047 emu/g after 200 h milling: 0.086 emu/g at T = 300 K | - induced defects by mechanical milling in diamagnetic ZnO powders - increased density of defects leads to increased FM order - Hc = 20–90 Oe |
|
| ZnO high pure (99.999%) sinthered bulk ceramics | [93] | 500 °C sintered: 0.0183 emu/g 850 °C sintered: 0.0190 emu/g 1300 °C sintered: 0.00188 emu/g at T = 300 K | Hc = 1300 Oe Hc = 12 Oe Hc = 34.4 Oe |
|
| ZnO Gd or Gd + Mn-doped NPs | [94] | Undoped NPs: Diamagnetic Gd-doped NPs: 0.15 memu/g Gd + Mn-doped NPs: 0.11 memu/g | The magnetization for Gd-doped NPs did not saturate at high magnetic fields. Mn ions suppressed FM behavior. |
|
| ZnO Cu-doped short facetted microrods or microtubes | [95] | Pure ZnO: Diamagnetic ZnO-Cu 0 T: 0.008 emu/g ZnO-Cu 4 T 0.012 emu/g at T = 298 K | Improved MS by applying a pulsed magnetic field at the time of synthesis. |
|
| TiO2 films on Si substrates | [96] | PO2 = 50 mtorr: diamagnetic PO2 = 0.2 mtorr Very weak ferromagnetic+ Diamagnetic ~0.02 emu/cm3 (~0.005 emu/g) PO2 = 0.02 mtorr ~0.3 emu/cm3 (~0.075 emu/g) At T = 298 K | A clear relationship between the magnetic moment and the concentration of oxygen vacancy |
|
| TiO2 films | [97] | Anatase film: 2 emu/cm3 (~0.52 emu/g) Rutile film: 6 emu/cm3 (~1.42 emu/g) | Vacuum annealing could produce a finite supply of oxygen vacancies. |
|
| TiO2 anatase H2 annealed 12 h at 600 °C | [98] | Pristine TiO2 very weak paramagnetism TiO2:H 0.066 emu/g Hc = 171 Oe TC ~ 450 K | Local 3d moments generated by hydrogenation. |
|
| TiO2 rutile single crystals doped with transitional metal ions (TM = Cr, Mn, Fe, Co, Ni, Cu) | [99] | TiO2 undoped 0.013 emu/mol (0.16 memu/g) TiO2-Cr 0.029 emu/mol (0.36 memu/g) TiO2- Mn 0.044 emu/mol (0.55 memu/g) TiO2- Fe 0.110 emu/mol (1.36 memu/g) TiO2- Co 0.017 emu/mol (0.21 memu/g) TiO2- Ni 0.069 emu/mol (0.86 memu/g) TiO2- Cu 0.012 emu/mol (0.15 memu/g) | The results suggest a precise superposition of a feromagnetic and paramagnetic behavior. |
|
| TiO2 Co-doped polycrystalline pellets | [100] | TiO2 diamagnetic Ti0.95Co0.05O2 0.07 emu/g Ti0.95Co0.05O2:H 2.35 emu/g * Ti0.95Co0.05O2:Htd 0.08 emu/g At T = 300 K *Also at T = 50 K | Co-doping generated weak ferromagnetic ordering; however, when annealed in H atmosphere, there is a significant increase in the magnetization. Furthermore, reheating in air reverses the effect to the original. |
|
| SrTiO3 single crystal | [101] | H+ ions irradiated ~1.3 × 10−3 emu/cm2 N+ ions irradiated ~4.2–6.2 × 10−4 emu/cm2 At T = 300 K | FM reduced when secondary oxygen-deficient phases increased |
|
| SnO2 high purity powders | [102] | 0 h milled 0.6 memu/g 4 milled 1.9 memu/g 12 h milled 5.5 memu/g 20 h milled * 10.5 memu/g | *20 h milled sample was then heated In air 4 h at diff temp. 0 °C 8.7 memu/g 200 °C 3.8 memu/g 400 °C 1.9 memu/g 600 °C 0.8 memu/g |
|
| SnO2 NPs | [103] | Raw powder 0.019 emu/g Annealed at 500 °C 0.015 emu/g Annealed at 700 °C 0.012 emu/g Annealed at 900 °C 0.010 emu/g Annealed at 1100 °C 0.006 emu/g Annealed at 1300 °C 0.001 emu/g Measured at T = 300 K Coercivities ~181–206 Oe | Raw powder 0.75 emu/g Annealed at 500 °C 0.54 emu/g Annealed at 700 °C 0.23 emu/g Annealed at 900 °C 0.019 emu/g Annealed at 1100 °C 0.13 emu/g Annealed at 1300 °C 0.05 emu/g Measured at T = 5 K Coercivities ~178–187 Oe |
|
| SnO2 films on LaAlO3 substrates | [104] | 10 nm film ~27 emu/cm3 (~3.94 emu/g) 220 nm raw film ~12 emu//cm3 (~1.75 emu/g) 220 nm 700 °C O2- post-annealed film for 10 h ~diamagnetic At T = 300 K | the magnetic field is applied parallel to the film’s plane |
|
| Sr3SnO film | [105] | ~7 emu/cm3 (1.48 emu/g) At T= 300 K | Hc ~75 Oe |
|
| CeO2-x films | [40] | For x = 0.03 ~1.34 emu/g For x = 0.1 ~1.02 emu/g At T = 300 K | Both Ce4+ and Ce3+ ions are present |
|
| HfO2 films | [106] | 128 emu/cm3 (~13.223 emu/g) | Vacuum and air annealing clearly affected FM |
|
| MgO films | [32] | ~2.69 emu/cm3 (~0.751 emu/g) After annealing treatment: 1.18 emu/cm3 (~0.329 emu/g) | Reduction of Ms after annealing related to a reduction of Mg vacancies. |
|
| Alkaline-earth hexaborides | ||||
| CaB6 films | [107] | Thickness: 0.5 µm 11.36 emu/cm3 (~4.63 emu/g) Thickness: 1.6 µm 1.136 emu/cm3 (~0.463 emu/g) Thickness: 2.3 µm 0.25 emu/cm3 (~0.102 emu/g) | Increasing the thickness resulted in Ms decrease. Results ruled out iron contamination. |
|
| CaB6 crystal | [108] | 0.12 emu/cm3 (~0.0489 emu/g) | FM mostly on/near the surface of the sample |
|
| BaB6 thin film | [19] | 10.7 emu/cm3 (~2.454 emu/g) at T = 450–550 °C | Results ruled out contamination. No variations due to film thickness. |
|
| Carbon nanostructures | ||||
| Highly-oriented graphite samples | [79] | AC1-HOPG 1.39 memu/g AC2-HOPG 0.33 ± 0.04 memu/g UC2-HOPG 0.83 ± 0.03 memu/g UC3-HOPG 1.1 ± 0.2 memu/g HOPG-2 0.93 ± 0.1 memu/g HOPG-3 2.5 memu/g Kish graphite 0.6 ± 0.2 memu/g At T = 300 K | Different possibilities for the FM-like behavior in the samples. |
|
| C60 | [60] | 0.045 emu/g T ~ 800 K | Pressure used 9 GPa. Results indicate a reduction in the ferromagnetic behavior after 800 K. |
|
| Graphene | [53] | Annealing temp 400 °C At 300 K—0.004 emu/g At 2 K—0.25 emu/g Annealing temp 600 °C At 300 K—0.020 emu/g At 2 K—0.90 emu/g | Graphene prepared at 800 °C showed no clear sign of FM. |
|
| Graphene nanoribbons | [67] | 1.1 emu/g | Optimization of density zig-zag edge defects. |
|
| Highly oriented pyrolytic graphite—12C+ ion implantation | [76] | 14.4 emu/g | Increase of implantation steps correlated with the increase in vacancy density. |
|
| Carbon nanotubes | [83] | 0.5227 emu/g | N2 plasma treatment. |
|
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Lungu, I.I.; Grumezescu, A.M.; Fleaca, C. Unexpected Ferromagnetism—A Review. Appl. Sci. 2021, 11, 6707. https://doi.org/10.3390/app11156707
Lungu II, Grumezescu AM, Fleaca C. Unexpected Ferromagnetism—A Review. Applied Sciences. 2021; 11(15):6707. https://doi.org/10.3390/app11156707
Chicago/Turabian StyleLungu, Iulia Ioana, Alexandru Mihai Grumezescu, and Claudiu Fleaca. 2021. "Unexpected Ferromagnetism—A Review" Applied Sciences 11, no. 15: 6707. https://doi.org/10.3390/app11156707
APA StyleLungu, I. I., Grumezescu, A. M., & Fleaca, C. (2021). Unexpected Ferromagnetism—A Review. Applied Sciences, 11(15), 6707. https://doi.org/10.3390/app11156707







