Optical Realization of Wave-Based Analog Computing with Metamaterials
Abstract
1. Introduction
2. Computational Metamaterials
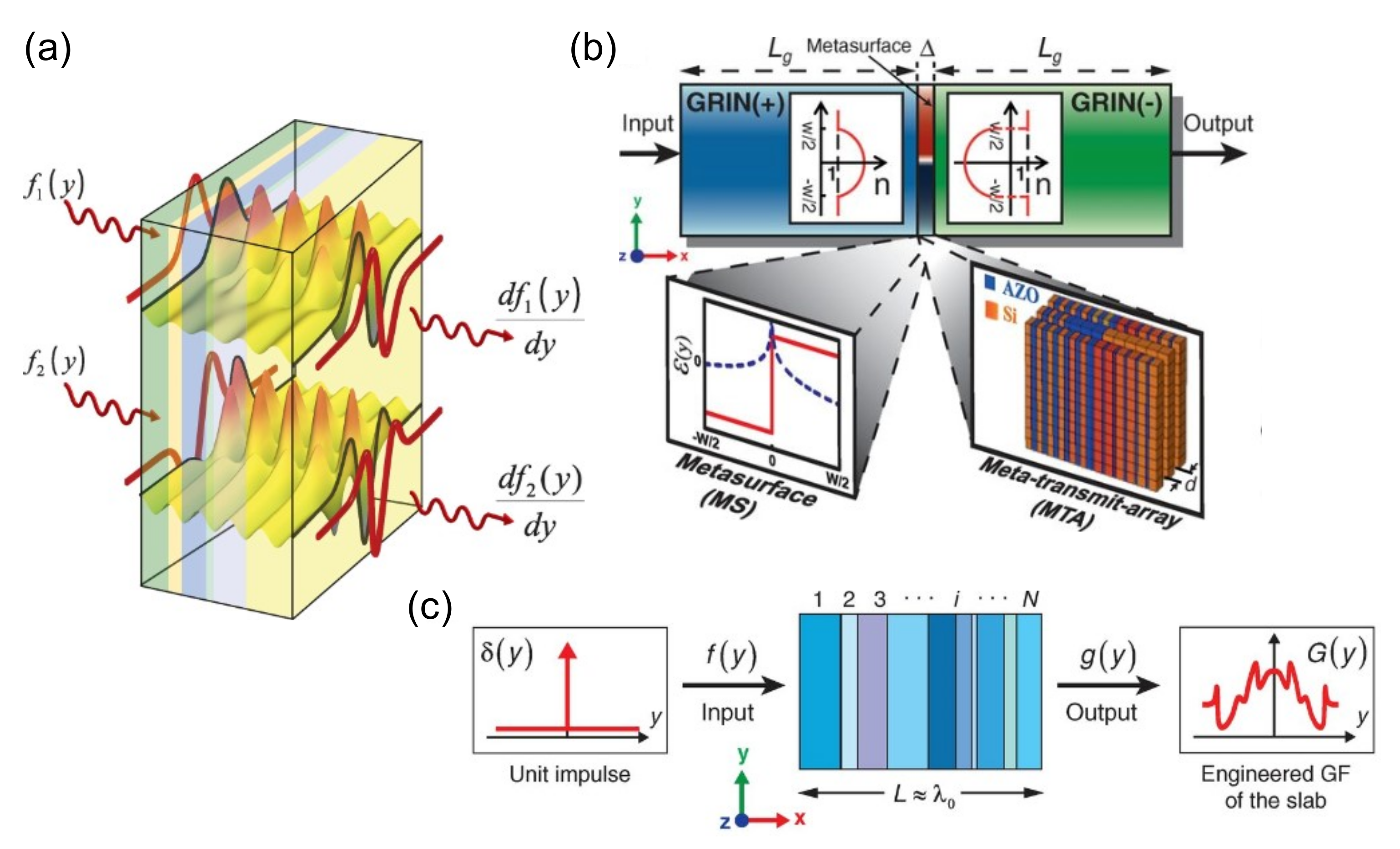
2.1. Metasurface Approach
2.1.1. Reflective Configurations of MS Approach
2.1.2. Transmittive Configurations of MS Approach
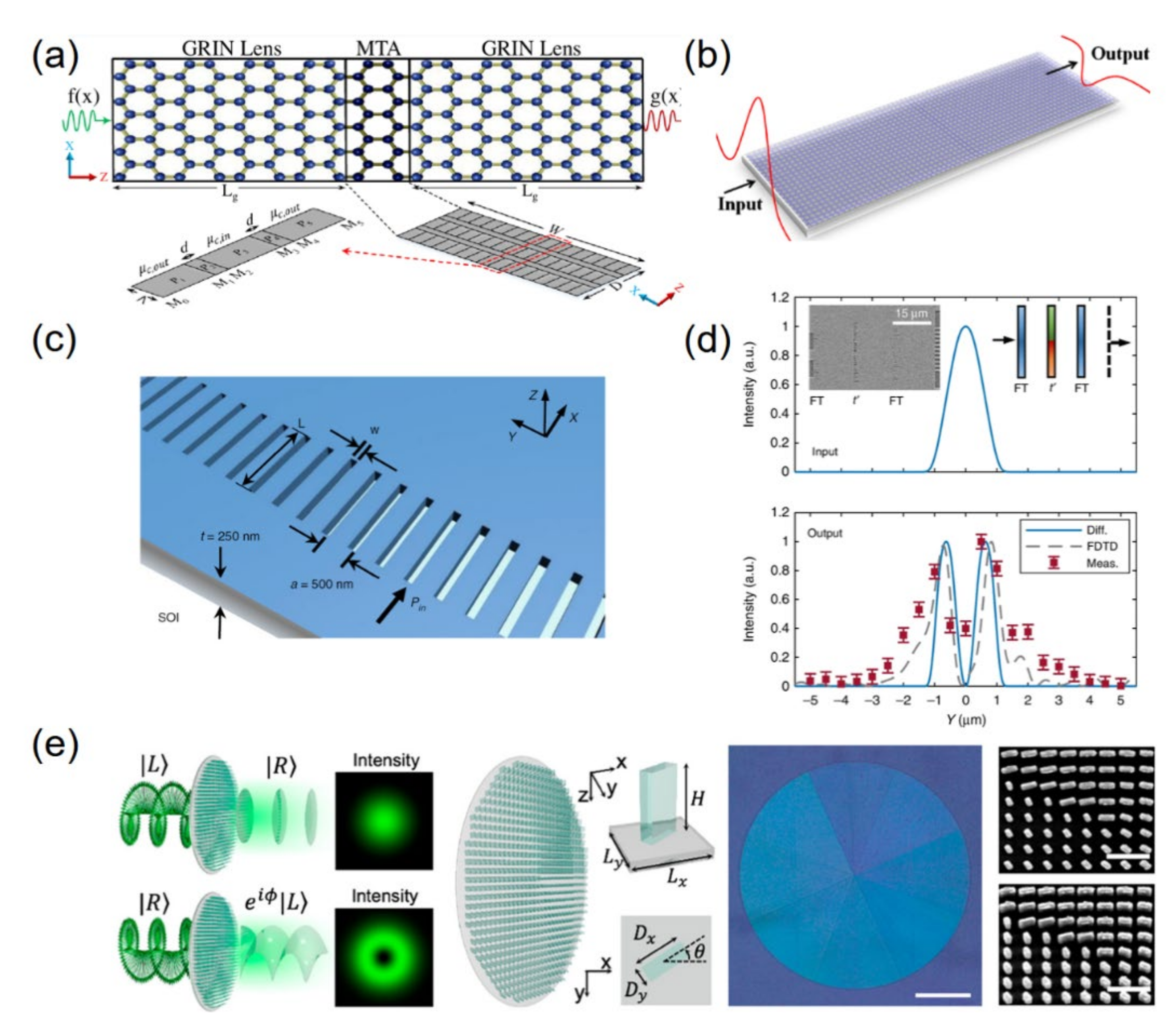
2.2. Green’s Function Approach
2.2.1. Diffraction Gratings
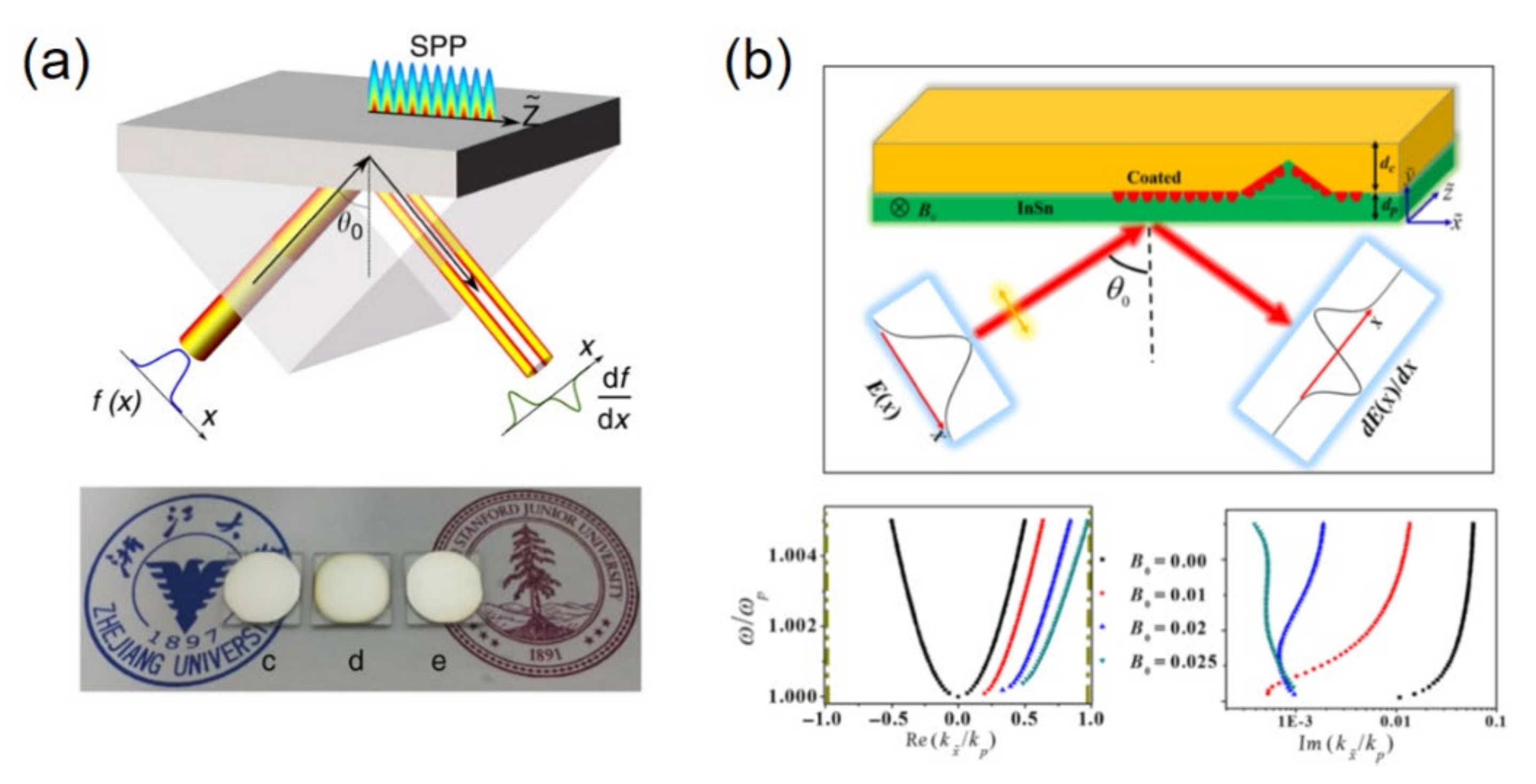
2.2.2. Plasmonic Structure
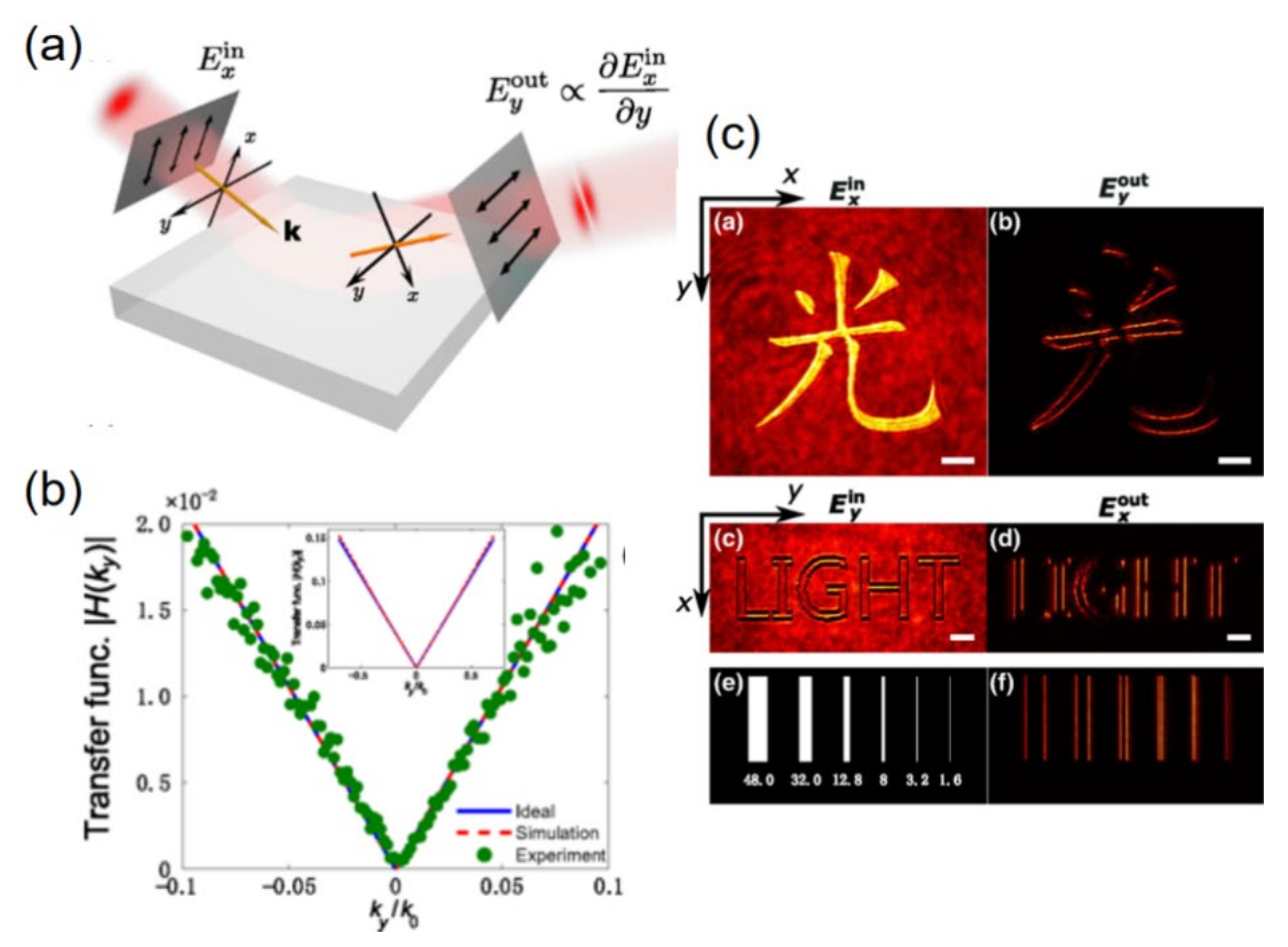
2.2.3. Two-Dimensional Dielectric Metasurfaces
3. Other Emerging Approaches
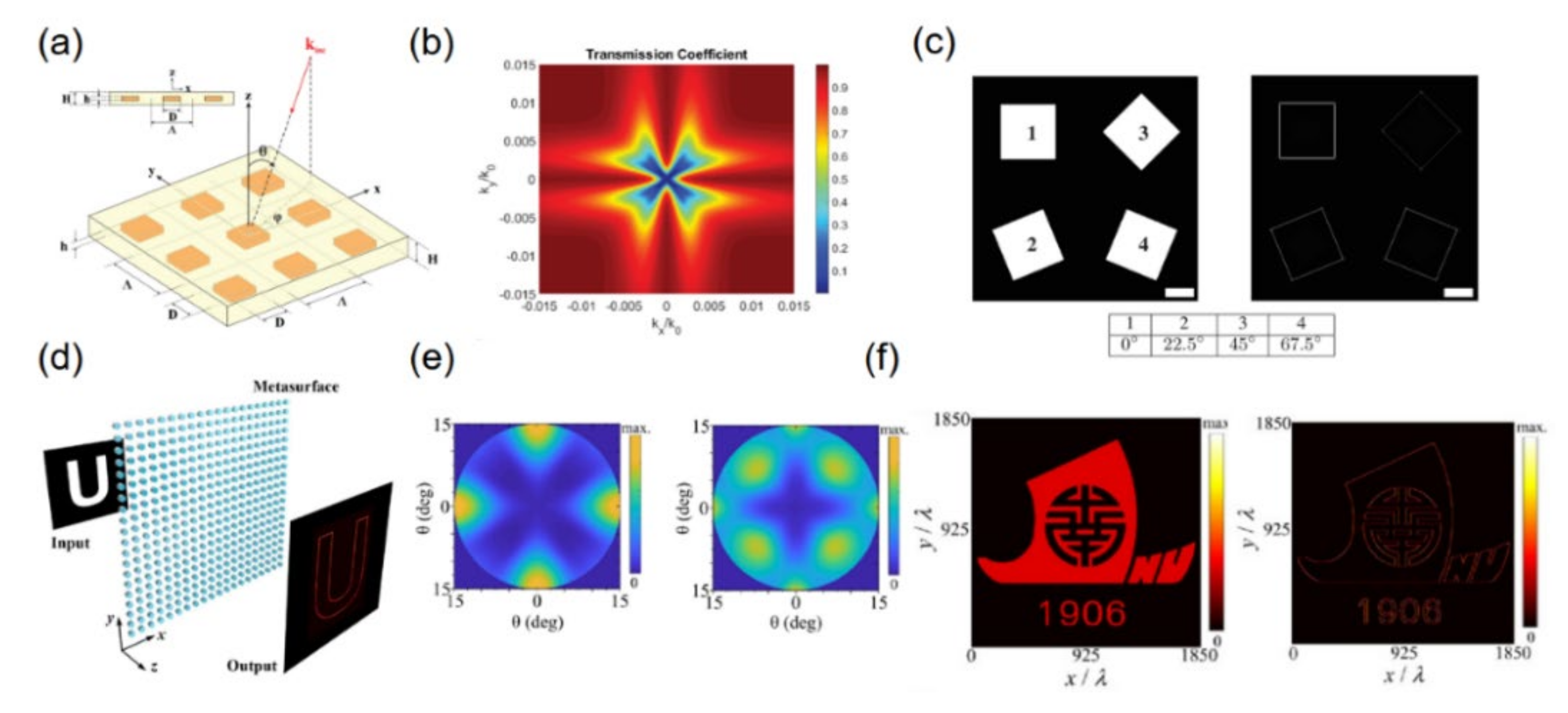
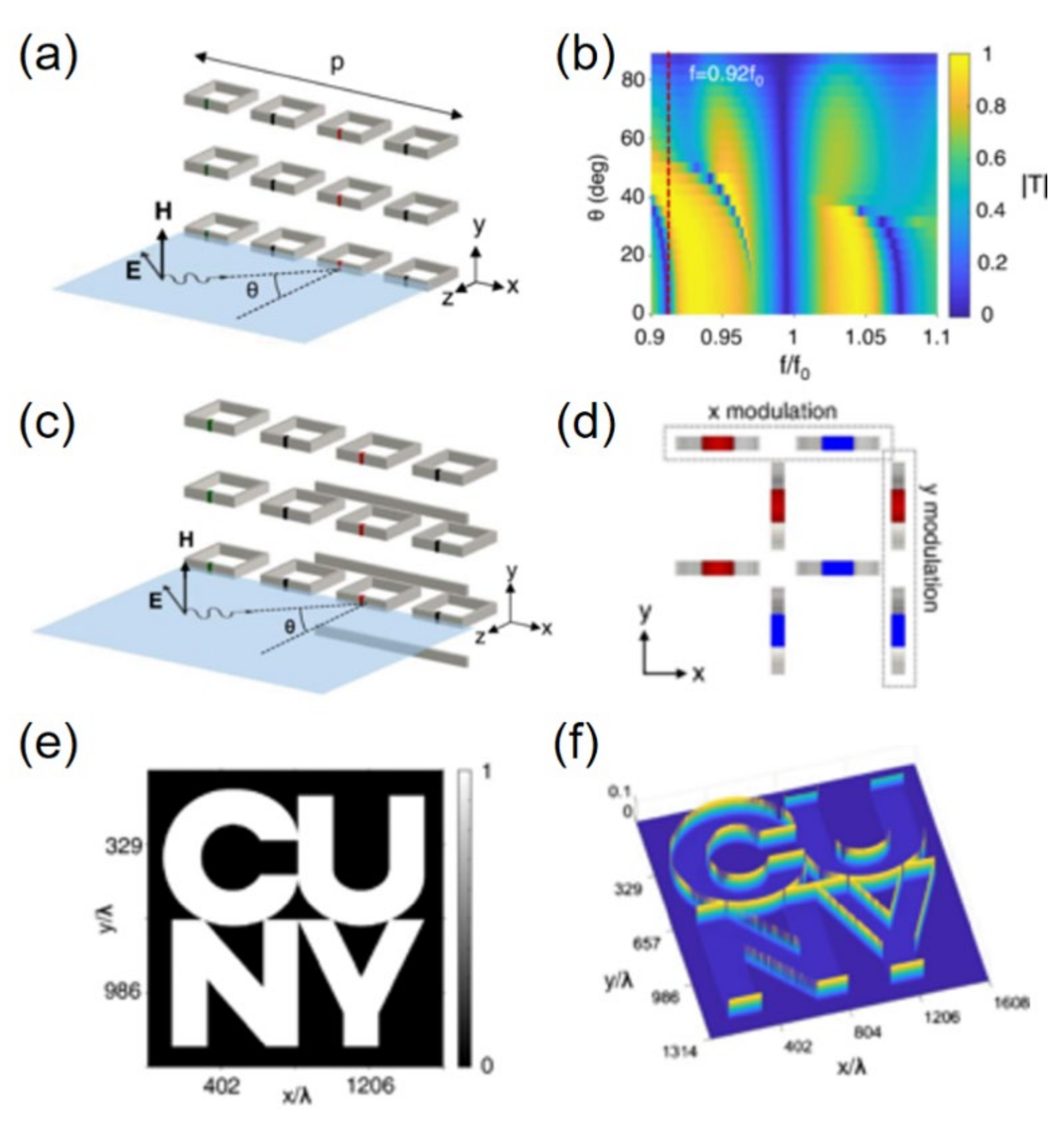
3.1. Nonlocal Metasurface
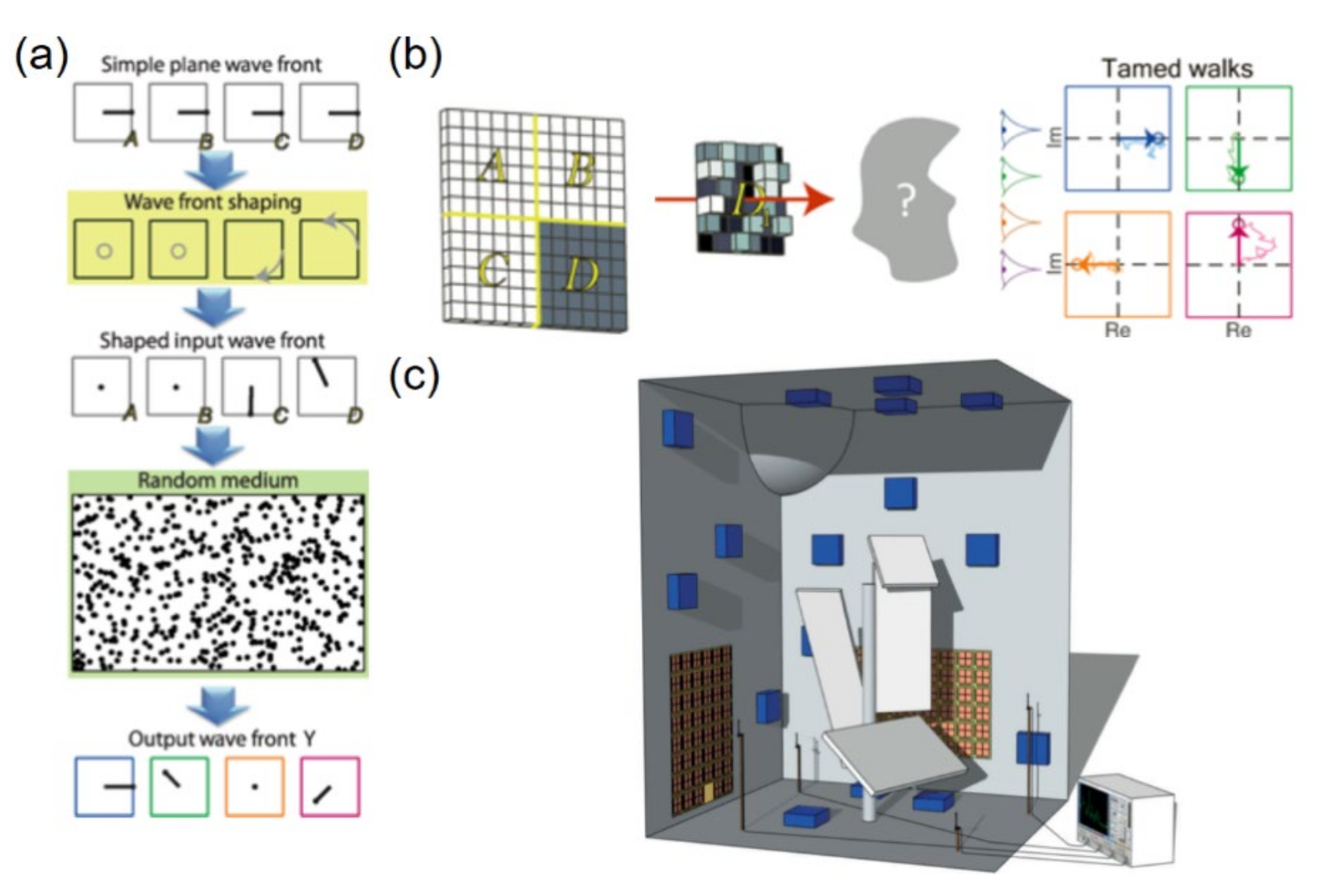
3.2. Random Medium
3.3. Inverse Design
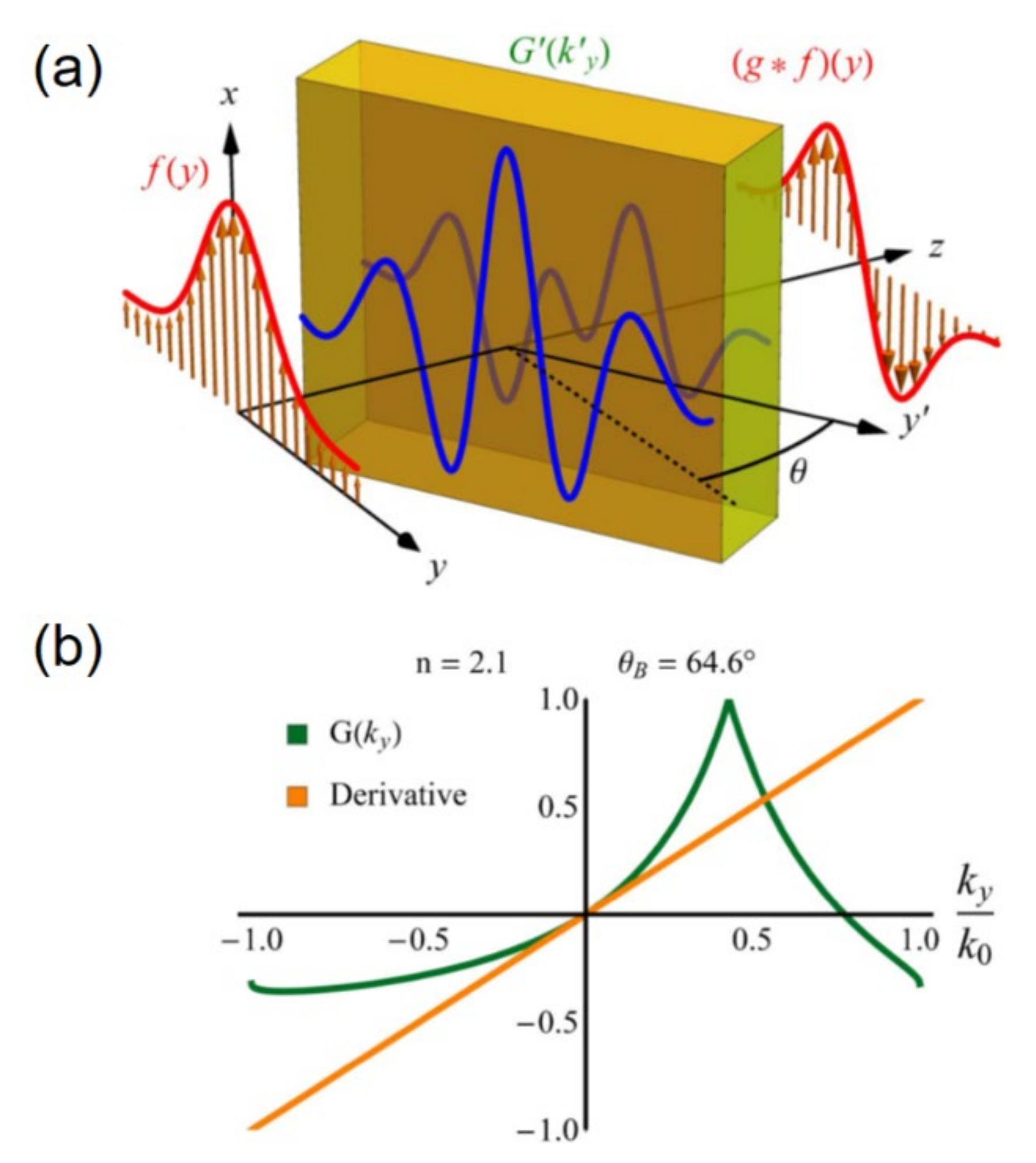
3.4. Brewster Effect
3.5. Goos-Hänchen Effect
3.6. Spin Hall Effect
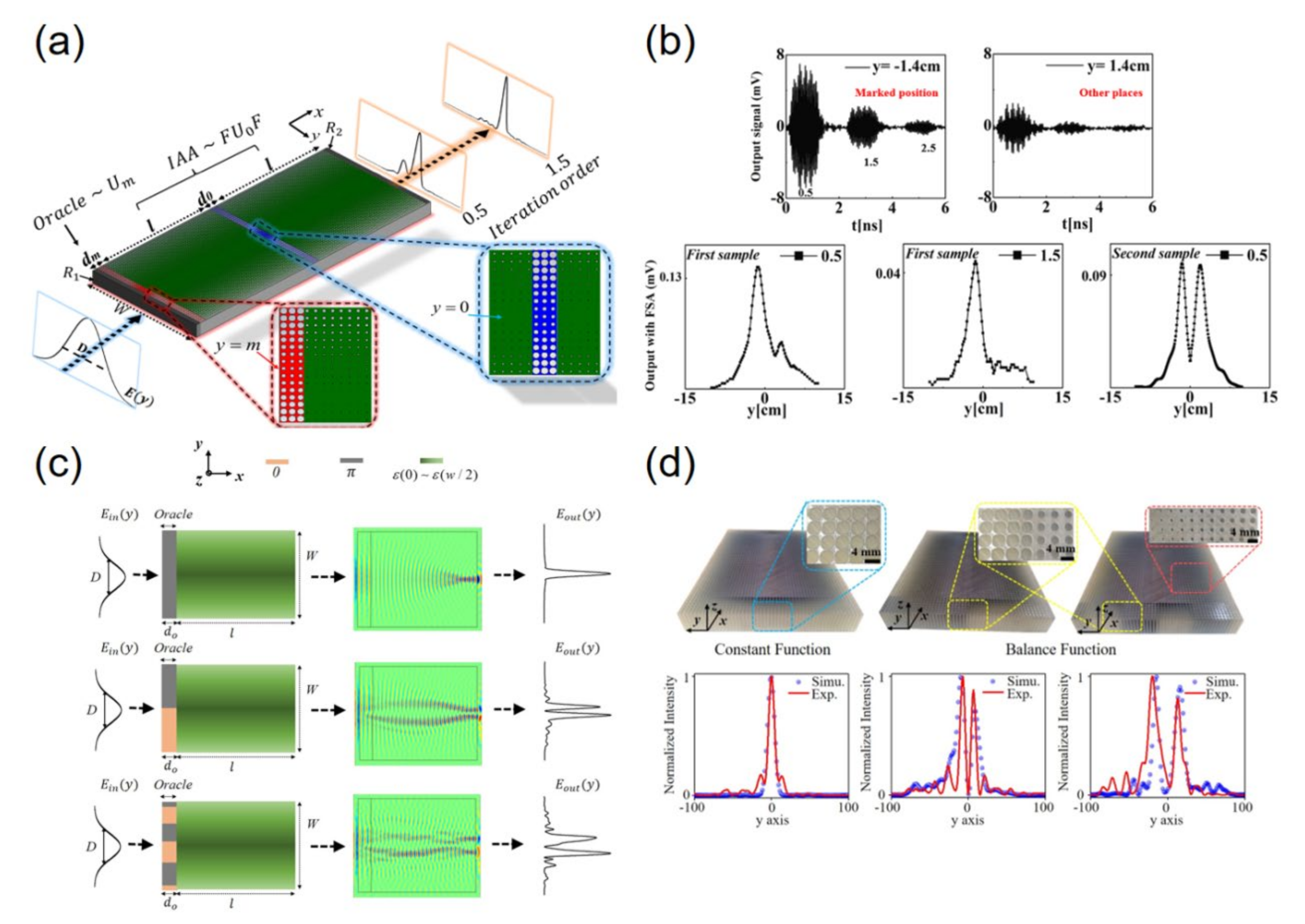
3.7. Quantum Computing with Metamaterials
4. Conclusions and Outlook
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Clymer, A.B. The mechanical analog computers of Hannibal Ford and William Newell. IEEE Ann. Hist. Comput. 1993, 15, 19–34. [Google Scholar] [CrossRef]
- Price, D.D.S. A History of Calculating Machines. IEEE Micro 1984, 4, 22–52. [Google Scholar] [CrossRef]
- Hausner, A. Analog and Analog/Hybrid Computer Programming; Prentice-Hall: Englewood Cliffs, NJ, USA, 1971. [Google Scholar] [CrossRef]
- Barrios, G.A.; Retamal, J.C.; Solano, E.; Sanz, M. Analog simulator of integro-differential equations with classical memristors. Sci. Rep. 2019, 9, 12928. [Google Scholar] [CrossRef] [PubMed]
- Tsividis, Y. Not your Father’s analog computer. IEEE Spectr. 2018, 55, 38–43. [Google Scholar] [CrossRef]
- Caulfield, H.J.; Dolev, S. Why future supercomputing requires optics. Nat. Photonics 2010, 4, 261–263. [Google Scholar] [CrossRef]
- The power of analogies. Nat. Photonics 2014, 8. [CrossRef]
- Passian, A.; Imam, N. Nanosystems, Edge Computing, and the Next Generation Computing Systems. Sensors 2019, 19, 4048. [Google Scholar] [CrossRef]
- Solli, D.R.; Jalali, B. Analog optical computing. Nat. Photonics 2015, 9, 704–706. [Google Scholar] [CrossRef]
- Karim, M.A.; Awwal, A.A. Optical Computing: An Introduction; Wiley, Inc.: New York, NY, USA, 1992. [Google Scholar]
- Herden, A.; Tschudi, T. Analog Optical Computing; Springer: Berlin/Heidelberg, Germany, 1986; pp. 369–383. [Google Scholar]
- Creasey, D.J. Digital Signal Processing: Principles, Devices and Applications. IEE Rev. 1990, 36, 275–276. [Google Scholar] [CrossRef]
- Park, Y.; Ahn, T.-J.; Dai, Y.; Yao, J.; Azaña, J. All-optical temporal integration of ultrafast pulse waveforms. Opt. Express 2008, 16, 17817–17825. [Google Scholar] [CrossRef]
- Quoc Ngo, N. Design of an optical temporal integrator based on a phase-shifted fiber Bragg grating in transmission. Opt. Lett. 2007, 32, 3020–3022. [Google Scholar] [CrossRef] [PubMed]
- Rutkowska, K.A.; Duchesne, D.; Strain, M.J.; Morandotti, R.; Sorel, M.; Azaña, J. Ultrafast all-optical temporal differentiators based on CMOS-compatible integrated-waveguide Bragg gratings. Opt. Express 2011, 19, 19514–19522. [Google Scholar] [CrossRef] [PubMed]
- Slavík, R.; Park, Y.; Kulishov, M.; Morandotti, R.; Azaña, J. Ultrafast all-optical differentiators. Opt. Express 2006, 14, 10699–10707. [Google Scholar] [CrossRef] [PubMed]
- Xu, J.; Zhang, X.; Dong, J.; Liu, D.; Huang, D. High-speed all-optical differentiator based on a semiconductor optical amplifier and an optical filter. Opt. Lett. 2007, 32, 1872–1874. [Google Scholar] [CrossRef] [PubMed]
- Ferrera, M.; Park, Y.; Razzari, L.; Little, B.E.; Chu, S.T.; Morandotti, R.; Moss, D.J.; Azaña, J. On-chip CMOS-compatible all-optical integrator. Nat. Commun. 2010, 1, 29. [Google Scholar] [CrossRef] [PubMed]
- Zangeneh-Nejad, F.; Fleury, R. Topological analog signal processing. Nat. Commun. 2019, 10, 2058. [Google Scholar] [CrossRef]
- Chen, K.; Hou, J.; Huang, Z.; Cao, T.; Zhang, J.; Yu, Y.; Zhang, X. All-optical 1st- and 2nd-order differential equation solvers with large tuning ranges using Fabry-Pérot semiconductor optical amplifiers. Opt. Express 2015, 23, 3784–3794. [Google Scholar] [CrossRef]
- Yang, T.; Dong, J.; Lu, L.; Zhou, L.; Zheng, A.; Zhang, X.; Chen, J. All-optical differential equation solver with constant-coefficient tunable based on a single microring resonator. Sci. Rep. 2014, 4, 5581. [Google Scholar] [CrossRef]
- Karimi, A.; Zarifkar, A.; Miri, M. Design of a Miniaturized Broadband Silicon Hybrid Plasmonic Temporal Integrator for Ultrafast Optical Signal Processing. J. Lightwave Technol. 2020, 38, 2346–2352. [Google Scholar] [CrossRef]
- Wu, J.; Liu, B.; Peng, J.; Mao, J.; Jiang, X.; Qiu, C.; Tremblay, C.; Su, Y. On-Chip Tunable Second-Order Differential-Equation Solver Based on a Silicon Photonic Mode-Split Microresonator. J. Lightwave Technol. 2015, 33, 3542–3549. [Google Scholar] [CrossRef]
- Zhang, W.; Yao, J. Photonic integrated field-programmable disk array signal processor. Nat. Commun. 2020, 11, 406. [Google Scholar] [CrossRef] [PubMed]
- Zhang, J.; Guzzon, R.S.; Coldren, L.A.; Yao, J. Optical dynamic memory based on an integrated active ring resonator. Opt. Lett. 2018, 43, 4687–4690. [Google Scholar] [CrossRef] [PubMed]
- Brunner, D.; Soriano, M.C.; Mirasso, C.R.; Fischer, I. Parallel photonic information processing at gigabyte per second data rates using transient states. Nat. Commun. 2013, 4, 1364. [Google Scholar] [CrossRef]
- Moughames, J.; Porte, X.; Thiel, M.; Ulliac, G.; Larger, L.; Jacquot, M.; Kadic, M.; Brunner, D. Three-dimensional waveguide interconnects for scalable integration of photonic neural networks. Optica 2020, 7, 640–646. [Google Scholar] [CrossRef]
- Solli, D.R.; Herink, G.; Jalali, B.; Ropers, C. Fluctuations and correlations in modulation instability. Nat. Photonics 2012, 6, 463–468. [Google Scholar] [CrossRef]
- Donati, S.; Mirasso, C.R. Introduction to the feature section on optical chaos and applications to cryptography. IEEE J. Quantum Electron. 2002, 38, 1138–1140. [Google Scholar] [CrossRef]
- Turitsyna, E.G.; Smirnov, S.V.; Sugavanam, S.; Tarasov, N.; Shu, X.; Babin, S.A.; Podivilov, E.V.; Churkin, D.V.; Falkovich, G.; Turitsyn, S.K. The laminar–turbulent transition in a fibre laser. Nat. Photonics 2013, 7, 783–786. [Google Scholar] [CrossRef]
- Bykov, D.A.; Doskolovich, L.L.; Bezus, E.A.; Soifer, V.A. Optical computation of the Laplace operator using phase-shifted Bragg grating. Opt. Express 2014, 22, 25084–25092. [Google Scholar] [CrossRef]
- AbdollahRamezani, S.; Arik, K.; Khavasi, A.; Kavehvash, Z. Analog computing using graphene-based metalines. Opt. Lett. 2015, 40, 5239–5242. [Google Scholar] [CrossRef]
- Zangeneh-Nejad, F.; Khavasi, A.; Rejaei, B. Analog optical computing by half-wavelength slabs. Opt. Commun. 2018, 407, 338–343. [Google Scholar] [CrossRef]
- Shor, P.W. Algorithms for quantum computation: Discrete logarithms and factoring. In Proceedings of the 35th Annual Symposium on Foundations of Computer Science, Los Alamitos, CA, USA, 20–22 November 1994. [Google Scholar]
- Grover, L.K. A fast quantum mechanical algorithm for database search. In Proceedings of the 28th Annual ACM Symposium on Theory of Computing (STOC), Philadelphia, PA, USA, 6–8 July 1996; pp. 212–219. [Google Scholar]
- Grover, L.K. Quantum Computers Can Search Arbitrarily Large Databases by a Single Query. Phys. Rev. Lett. 1997, 79, 4709–4712. [Google Scholar] [CrossRef]
- Knill, E.; Laflamme, R.; Milburn, G.J. A scheme for efficient quantum computation with linear optics. Nature 2001, 409, 46–52. [Google Scholar] [CrossRef] [PubMed]
- Nielsen, M.A.; Chuang, I.L. Quantum Computation and Quantum Information; Cambridge University Press: New York, NY, USA, 2000. [Google Scholar]
- Spreeuw, R.J.C. A Classical Analogy of Entanglement. Found. Phys. 1998, 28, 361–374. [Google Scholar] [CrossRef]
- Spreeuw, R.J.C. Classical wave-optics analogy of quantum-information processing. Phys. Rev. A 2001, 63, 062302. [Google Scholar] [CrossRef]
- Collins, D.; Kim, K.W.; Holton, W.C. Deutsch-Jozsa algorithm as a test of quantum computation. Phys. Rev. A 1998, 58, R1633–R1636. [Google Scholar] [CrossRef]
- Kwiat, P.G.; Mitchell, J.R.; Schwindt, P.D.D.; White, A.G. Grover’s search algorithm: An optical approach. J. Mod. Opt. 2000, 47, 257–266. [Google Scholar] [CrossRef]
- Bhattacharya, N.; van Linden van den Heuvell, H.B.; Spreeuw, R.J.C. Implementation of Quantum Search Algorithm using Classical Fourier Optics. Phys. Rev. Lett. 2002, 88, 137901. [Google Scholar] [CrossRef]
- Dragoman, D. n-step optical simulation of the n-qubit state: Applications in optical computing. Optik 2002, 113, 425–428. [Google Scholar] [CrossRef]
- Puentes, G.; Mela, C.L.; Ledesma, S.; Iemmi, C.; Paz, J.P.; Saraceno, M. Optical simulation of quantum algorithms using programmable liquid-crystal displays. Phys. Rev. A 2004, 69, 042319. [Google Scholar] [CrossRef]
- Hijmans, T.W.; Huussen, T.N.; Spreeuw, R.J.C. Time and frequency domain solutions in an optical analogue of Grover’s search algorithm. J. Opt. Soc. Am. B 2006, 24, 214–220. [Google Scholar] [CrossRef]
- Luis, A. Coherence, polarization, and entanglement for classical light fields. Opt. Commun. 2009, 282, 3665–3670. [Google Scholar] [CrossRef]
- Zhang, P.; Liu, R.-F.; Huang, Y.-F.; Gao, H.; Li, F.-L. Demonstration of Deutsch’s algorithm on a stable linear optical quantum computer. Phys. Rev. A 2010, 82, 064302. [Google Scholar] [CrossRef]
- Lee, S.M.; Park, H.S.; Cho, J.; Kang, Y.; Lee, J.Y.; Kim, H.; Lee, D.-H.; Choi, S.-K. Experimental realization of a four-photon seven-qubit graph state for one-way quantum computation. Opt. Express 2012, 20, 6915–6926. [Google Scholar] [CrossRef] [PubMed]
- Marques, B.; Barros, M.R.; Pimenta, W.M.; Carvalho, M.A.D.; Ferraz, J.; Drumond, R.C.; Terra Cunha, M.; Pádua, S. Double-slit implementation of the minimal Deutsch algorithm. Phys. Rev. A 2012, 86, 032306. [Google Scholar] [CrossRef]
- Rohde, P.P. Optical quantum computing with photons of arbitrarily low fidelity and purity. Phys. Rev. A 2012, 86, 052321. [Google Scholar] [CrossRef]
- Goyal, S.K.; Roux, F.S.; Forbes, A.; Konrad, T. Implementing Quantum Walks Using Orbital Angular Momentum of Classical Light. Phys. Rev. Lett. 2013, 110, 263602. [Google Scholar] [CrossRef]
- Georgescu, I.M.; Ashhab, S.; Nori, F. Quantum simulation. Rev. Mod. Phys. 2014, 86, 153–185. [Google Scholar] [CrossRef]
- Goyal, S.K.; Roux, F.S.; Forbes, A.; Konrad, T. The Scalable Implementation of Quantum Walks using Classical Light. In Proceedings of the SPIE OPTO, San Francisco, CA, USA, 1–6 February 2014; Volume 8999, p. 8. [Google Scholar]
- Böhm, J.; Bellec, M.; Mortessagne, F.; Kuhl, U.; Barkhofen, S.; Gehler, S.; Stöckmann, H.-J.; Foulger, I.; Gnutzmann, S.; Tanner, G. Microwave Experiments Simulating Quantum Search and Directed Transport in Artificial Graphene. Phys. Rev. Lett. 2015, 114, 110501. [Google Scholar] [CrossRef]
- Goyal, S.K.; Roux, F.S.; Forbes, A.; Konrad, T. Implementation of multidimensional quantum walks using linear optics and classical light. Phys. Rev. A 2015, 92, 040302. [Google Scholar] [CrossRef]
- Hor-Meyll, M.; Tasca, D.S.; Walborn, S.P.; Ribeiro, P.H.S.; Santos, M.M.; Duzzioni, E.I. Deterministic quantum computation with one photonic qubit. Phys. Rev. A 2015, 92, 012337. [Google Scholar] [CrossRef]
- Perez-Garcia, B.; Francis, J.; McLaren, M.; Hernandez-Aranda, R.I.; Forbes, A.; Konrad, T. Quantum computation with classical light: The Deutsch Algorithm. Phys. Lett. A 2015, 379, 1675–1680. [Google Scholar] [CrossRef]
- Perez-Garcia, B.; Mclaren, M.; Goyal, S.K.; Hernandez-Aranda, R.I.; Forbes, A.; Konrad, T. Quantum computation with classical light: Implementation of the Deutsch–Jozsa algorithm. Phys. Lett. A 2015, 380, 1925–1931. [Google Scholar] [CrossRef]
- Zagoskin, A.M.; Felbacq, D.; Rousseau, E. Quantum metamaterials in the microwave and optical ranges. EPJ Quantum Technol. 2016, 3, 2. [Google Scholar] [CrossRef]
- Giri, P.R.; Korepin, V.E. A review on quantum search algorithms. Quantum Inf. Process. 2017, 16, 315. [Google Scholar] [CrossRef]
- Johansson, N.; Larsson, J.-Å. Efficient classical simulation of the Deutsch–Jozsa and Simon’s algorithms. Quantum Inf. Process. 2017, 16, 233. [Google Scholar] [CrossRef]
- Wei, H.-R.; Liu, J.-Z. Deterministic implementations of single-photon multi-qubit Deutsch–Jozsa algorithms with linear optics. Annals Phys. 2017, 377, 38–47. [Google Scholar] [CrossRef]
- Flamini, F.; Spagnolo, N.; Sciarrino, F. Photonic quantum information processing: A review. Rep. Prog. Phys. 2018, 82, 016001. [Google Scholar] [CrossRef] [PubMed]
- Perez-Garcia, B.; Hernandez-Aranda, R.I.; Forbes, A.; Konrad, T. The first iteration of Grover’s algorithm using classical light with orbital angular momentum. J. Mod. Opt. 2018, 65, 1942–1948. [Google Scholar] [CrossRef]
- Vianna, Y.; Barros, M.R.; Hor-Meyll, M. Classical realization of the quantum Deutsch algorithm. Am. J. Phys. 2018, 86, 914–923. [Google Scholar] [CrossRef]
- Zhang, S.; Li, P.; Wang, B.; Zeng, Q.; Zhang, X. Implementation of quantum permutation algorithm with classical light. J. Phys. Commun. 2019, 3, 015008. [Google Scholar] [CrossRef]
- Zhang, S.; Zhang, Y.; Sun, Y.; Sun, H.; Zhang, X. Quantum-inspired microwave signal processing for implementing unitary transforms. Opt. Express 2019, 27, 436–460. [Google Scholar] [CrossRef] [PubMed]
- Smith, D.R.; Pendry, J.B.; Wiltshire, M.C.K. Metamaterials and Negative Refractive Index. Science 2004, 305, 788–792. [Google Scholar] [CrossRef] [PubMed]
- Chen, H.; Chan, C.T.; Sheng, P. Transformation optics and metamaterials. Nat. Mater. 2010, 9, 387. [Google Scholar] [CrossRef] [PubMed]
- Liu, Y.; Zhang, X. Metamaterials: A new frontier of science and technology. Chem. Soc. Rev. 2011, 40, 2494–2507. [Google Scholar] [CrossRef]
- Soukoulis, C.M.; Wegener, M. Past achievements and future challenges in the development of three-dimensional photonic metamaterials. Nat. Photonics 2011, 5, 523. [Google Scholar] [CrossRef]
- Cui, T.J.; Qi, M.Q.; Wan, X.; Zhao, J.; Cheng, Q. Coding metamaterials, digital metamaterials and programmable metamaterials. Light Sci. Appl. 2014, 3, e218. [Google Scholar] [CrossRef]
- Della Giovampaola, C.; Engheta, N. Digital metamaterials. Nat. Mater. 2014, 13, 1115. [Google Scholar] [CrossRef]
- Huo, P.; Zhang, S.; Liang, Y.; Lu, Y.; Xu, T. Hyperbolic Metamaterials and Metasurfaces: Fundamentals and Applications. Adv. Opt. Mater. 2019, 7, 1801616. [Google Scholar] [CrossRef]
- Fan, Y.; Wei, Z.; Li, H.; Chen, H.; Soukoulis, C.M. Low-loss and high-Q planar metamaterial with toroidal moment. Phys. Rev. B 2013, 87, 115417. [Google Scholar] [CrossRef]
- Chen, S.; Fan, Y.; Fu, Q.; Wu, H.; Jin, Y.; Zheng, J.; Zhang, F. A Review of Tunable Acoustic Metamaterials. Appl. Sci. 2018, 8, 1480. [Google Scholar] [CrossRef]
- Fan, Y.; Zhang, F.; Shen, N.-H.; Fu, Q.; Wei, Z.; Li, H.; Soukoulis, C.M. Achieving a high-Q response in metamaterials by manipulating the toroidal excitations. Phys. Rev. A 2018, 97, 033816. [Google Scholar] [CrossRef]
- Ziolkowski, R.W.; Engheta, N. Metamaterials: Two Decades Past and Into Their Electromagnetics Future and Beyond. IEEE Trans. Antennas Propag. 2020, 68, 1232–1237. [Google Scholar] [CrossRef]
- Yu, N.; Genevet, P.; Kats, M.A.; Aieta, F.; Tetienne, J.-P.; Capasso, F.; Gaburro, Z. Light Propagation with Phase Discontinuities: Generalized Laws of Reflection and Refraction. Science 2011, 334, 333–337. [Google Scholar] [CrossRef] [PubMed]
- Holloway, C.L.; Kuester, E.F.; Gordon, J.A.; Hara, J.O.; Booth, J.; Smith, D.R. An Overview of the Theory and Applications of Metasurfaces: The Two-Dimensional Equivalents of Metamaterials. IEEE Antennas Propag. Mag. 2012, 54, 10–35. [Google Scholar] [CrossRef]
- Kildishev, A.V.; Boltasseva, A.; Shalaev, V.M. Planar Photonics with Metasurfaces. Science 2013, 339, 1232009. [Google Scholar] [CrossRef]
- Meinzer, N.; Barnes, W.L.; Hooper, I.R. Plasmonic meta-atoms and metasurfaces. Nat. Photonics 2014, 8, 889–898. [Google Scholar] [CrossRef]
- Yu, N.; Capasso, F. Flat optics with designer metasurfaces. Nat. Mater. 2014, 13, 139. [Google Scholar] [CrossRef]
- Glybovski, S.B.; Tretyakov, S.A.; Belov, P.A.; Kivshar, Y.S.; Simovski, C.R. Metasurfaces: From microwaves to visible. Phys. Rep. 2016, 634, 1–72. [Google Scholar] [CrossRef]
- Hou-Tong, C.; Antoinette, J.T.; Nanfang, Y. A review of metasurfaces: Physics and applications. Rep. Prog. Phys. 2016, 79, 076401. [Google Scholar] [CrossRef]
- Zhang, L.; Mei, S.; Huang, K.; Qiu, C.-W. Advances in Full Control of Electromagnetic Waves with Metasurfaces. Adv. Opt. Mater. 2016, 4, 818–833. [Google Scholar] [CrossRef]
- Genevet, P.; Capasso, F.; Aieta, F.; Khorasaninejad, M.; Devlin, R. Recent advances in planar optics: From plasmonic to dielectric metasurfaces. Optica 2017, 4, 139–152. [Google Scholar] [CrossRef]
- Li, G.; Zhang, S.; Zentgraf, T. Nonlinear photonic metasurfaces. Nat. Rev. Mater. 2017, 2, 17010. [Google Scholar] [CrossRef]
- Chen, M.; Kim, M.; Wong Alex, M.H.; Eleftheriades George, V. Huygens’ metasurfaces from microwaves to optics: A review. Nanophotonics 2018, 7, 1207. [Google Scholar] [CrossRef]
- He, Q.; Sun, S.; Xiao, S.; Zhou, L. High-Efficiency Metasurfaces: Principles, Realizations, and Applications. Adv. Opt. Mater. 2018, 6, 1800415. [Google Scholar] [CrossRef]
- Hail, C.U.; Michel, A.-K.U.; Poulikakos, D.; Eghlidi, H. Optical Metasurfaces: Evolving from Passive to Adaptive. Adv. Opt. Mater. 2019, 7, 1801786. [Google Scholar] [CrossRef]
- Luo, X. Metamaterials and Metasurfaces. Adv. Opt. Mater. 2019, 7, 1900885. [Google Scholar] [CrossRef]
- Sun, S.; He, Q.; Hao, J.; Xiao, S.; Zhou, L. Electromagnetic metasurfaces: Physics and applications. Adv. Opt. Photonics 2019, 11, 380–479. [Google Scholar] [CrossRef]
- Fan, Y.; Shen, N.-H.; Zhang, F.; Zhao, Q.; Wei, Z.; Zhang, P.; Dong, J.; Fu, Q.; Li, H.; Soukoulis, C.M. Photoexcited Graphene Metasurfaces: Significantly Enhanced and Tunable Magnetic Resonances. ACS Photonics 2018, 5, 1612–1618. [Google Scholar] [CrossRef]
- Fan, Y.; Shen, N.-H.; Zhang, F.; Zhao, Q.; Wu, H.; Fu, Q.; Wei, Z.; Li, H.; Soukoulis, C.M. Graphene Plasmonics: A Platform for 2D Optics. Adv. Opt. Mater. 2018, 7, 1800537. [Google Scholar] [CrossRef]
- Cheng, K.; Wei, Z.; Fan, Y.; Zhang, X.; Wu, C.; Li, H. Realizing Broadband Transparency via Manipulating the Hybrid Coupling Modes in Metasurfaces for High-Efficiency Metalens. Adv. Opt. Mater. 2019, 7, 1900016. [Google Scholar] [CrossRef]
- Lou, J.; Liang, J.; Yu, Y.; Ma, H.; Yang, R.; Fan, Y.; Wang, G.; Cai, T. Silicon-Based Terahertz Meta-Devices for Electrical Modulation of Fano Resonance and Transmission Amplitude. Adv. Opt. Mater. 2020, 8, 2000449. [Google Scholar] [CrossRef]
- Zhu, W.; Fan, Y.; Li, C.; Yang, R.; Yan, S.; Fu, Q.; Zhang, F.; Gu, C.; Li, J. Realization of a near-infrared active Fano-resonant asymmetric metasurface by precisely controlling the phase transition of Ge2Sb2Te5. Nanoscale 2020, 12, 8758–8767. [Google Scholar] [CrossRef] [PubMed]
- Wang, Z.; Li, T.; Soman, A.; Mao, D.; Kananen, T.; Gu, T. On-chip wavefront shaping with dielectric metasurface. Nat. Commun. 2019, 10, 3547. [Google Scholar] [CrossRef] [PubMed]
- Abdollahramezani, S.; Hemmatyar, O.; Adibi, A. Meta-optics for spatial optical analog computing. Nanophotonics 2020, 9, 4075–4095. [Google Scholar] [CrossRef]
- Silva, A.; Monticone, F.; Castaldi, G.; Galdi, V.; Alù, A.; Engheta, N. Performing Mathematical Operations with Metamaterials. Science 2014, 343, 160. [Google Scholar] [CrossRef]
- Mendlovic, D.; Ozaktas, H.M. Fractional Fourier transforms and their optical implementation: I. J. Opt. Soc. Am. A 1993, 10, 1875–1881. [Google Scholar] [CrossRef]
- Ozaktas, H.M.; Mendlovic, D. Fractional Fourier transforms and their optical implementation. II. J. Opt. Soc. Am. A 1993, 10, 2522–2531. [Google Scholar] [CrossRef]
- Khorasaninejad, M.; Capasso, F. Metalenses: Versatile multifunctional photonic components. Science 2017, 358, eaam8100. [Google Scholar] [CrossRef]
- Lalanne, P.; Chavel, P. Metalenses at visible wavelengths: Past, present, perspectives. Laser Photonics Rev. 2017, 11, 1600295. [Google Scholar] [CrossRef]
- Wang, S.; Wu, P.C.; Su, V.-C.; Lai, Y.-C.; Hung Chu, C.; Chen, J.-W.; Lu, S.-H.; Chen, J.; Xu, B.; Kuan, C.-H.; et al. Broadband achromatic optical metasurface devices. Nat. Commun. 2017, 8, 187. [Google Scholar] [CrossRef]
- Yang, Q.; Gu, J.; Xu, Y.; Zhang, X.; Li, Y.; Ouyang, C.; Tian, Z.; Han, J.; Zhang, W. Broadband and Robust Metalens with Nonlinear Phase Profiles for Efficient Terahertz Wave Control. Adv. Opt. Mater. 2017, 5, 1601084. [Google Scholar] [CrossRef]
- Chen, W.T.; Zhu, A.Y.; Sanjeev, V.; Khorasaninejad, M.; Shi, Z.; Lee, E.; Capasso, F. A broadband achromatic metalens for focusing and imaging in the visible. Nat. Nanotechnol. 2018, 13, 220–226. [Google Scholar] [CrossRef] [PubMed]
- Shrestha, S.; Overvig, A.C.; Lu, M.; Stein, A.; Yu, N. Broadband achromatic dielectric metalenses. Light Sci. Appl. 2018, 7, 85. [Google Scholar] [CrossRef] [PubMed]
- Huang, L.; Chen, X.; Mühlenbernd, H.; Zhang, H.; Chen, S.; Bai, B.; Tan, Q.; Jin, G.; Cheah, K.-W.; Qiu, C.-W.; et al. Three-dimensional optical holography using a plasmonic metasurface. Nat. Commun. 2013, 4. [Google Scholar] [CrossRef]
- Li, G.; Kang, M.; Chen, S.; Zhang, S.; Pun, E.Y.-B.; Cheah, K.W.; Li, J. Spin-Enabled Plasmonic Metasurfaces for Manipulating Orbital Angular Momentum of Light. Nano Lett. 2013, 13, 4148–4151. [Google Scholar] [CrossRef]
- Lovera, A.; Gallinet, B.; Nordlander, P.; Martin, O.J.F. Mechanisms of Fano Resonances in Coupled Plasmonic Systems. ACS Nano 2013, 7, 4527–4536. [Google Scholar] [CrossRef]
- Ni, X.; Ishii, S.; Kildishev, A.V.; Shalaev, V.M. Ultra-thin, planar, Babinet-inverted plasmonic metalenses. Light Sci. Appl. 2013, 2, e72. [Google Scholar] [CrossRef]
- Pors, A.; Nielsen, M.G.; Eriksen, R.L.; Bozhevolnyi, S.I. Broadband Focusing Flat Mirrors Based on Plasmonic Gradient Metasurfaces. Nano Lett. 2013, 13, 829–834. [Google Scholar] [CrossRef]
- Chalabi, H.; Schoen, D.; Brongersma, M.L. Hot-Electron Photodetection with a Plasmonic Nanostripe Antenna. Nano Lett. 2014, 14, 1374–1380. [Google Scholar] [CrossRef]
- Saeidi, C.; van der Weide, D. Wideband plasmonic focusing metasurfaces. Appl. Phys. Lett. 2014, 105, 053107. [Google Scholar] [CrossRef]
- Qin, F.; Ding, L.; Zhang, L.; Monticone, F.; Chum, C.C.; Deng, J.; Mei, S.; Li, Y.; Teng, J.; Hong, M.; et al. Hybrid bilayer plasmonic metasurface efficiently manipulates visible light. Sci. Adv. 2016, 2. [Google Scholar] [CrossRef] [PubMed]
- Yue, F.; Wen, D.; Xin, J.; Gerardot, B.D.; Li, J.; Chen, X. Vector Vortex Beam Generation with a Single Plasmonic Metasurface. ACS Photonics 2016, 3, 1558–1563. [Google Scholar] [CrossRef]
- Pors, A.; Nielsen, M.G.; Bozhevolnyi, S.I. Analog Computing Using Reflective Plasmonic Metasurfaces. Nano Lett. 2015, 15, 791–797. [Google Scholar] [CrossRef] [PubMed]
- Chen, H.; An, D.; Li, Z.; Zhao, X. Performing differential operation with a silver dendritic metasurface at visible wavelengths. Opt. Express 2017, 25, 26417–26426. [Google Scholar] [CrossRef]
- Chizari, A.; Abdollahramezani, S.; Jamali, M.V.; Salehi, J.A. Analog optical computing based on a dielectric meta-reflect array. Opt. Lett. 2016, 41, 3451–3454. [Google Scholar] [CrossRef]
- Zhang, W.; Qu, C.; Zhang, X. Solving constant-coefficient differential equations with dielectric metamaterials. J. Opt. 2016, 18, 075102. [Google Scholar] [CrossRef]
- Wu, Y.; Zhuang, Z.; Deng, L.; Liu, Y.; Xue, Q.; Ghassemlooy, Z. Arbitrary Multi-way Parallel Mathematical Operations Based on Planar Discrete Metamaterials. Plasmonics 2018, 13, 599–607. [Google Scholar] [CrossRef]
- Huo, P.; Zhang, C.; Zhu, W.; Liu, M.; Zhang, S.; Zhang, S.; Chen, L.; Lezec, H.J.; Agrawal, A.; Lu, Y.; et al. Photonic Spin-Multiplexing Metasurface for Switchable Spiral Phase Contrast Imaging. Nano Lett. 2020, 20, 2791–2798. [Google Scholar] [CrossRef]
- Wu, W.; Jiang, W.; Yang, J.; Gong, S.; Ma, Y. Multilayered analog optical differentiating device: Performance analysis on structural parameters. Opt. Lett. 2017, 42, 5270–5273. [Google Scholar] [CrossRef]
- Zangeneh-Nejad, F.; Khavasi, A. Spatial integration by a dielectric slab and its planar graphene-based counterpart. Opt. Lett. 2017, 42, 1954–1957. [Google Scholar] [CrossRef]
- Guo, C.; Xiao, M.; Minkov, M.; Shi, Y.; Fan, S. Photonic crystal slab Laplace operator for image differentiation. Optica 2018, 5, 251–256. [Google Scholar] [CrossRef]
- Doskolovich, L.L.; Bezus, E.A.; Bykov, D.A.; Soifer, V.A. Spatial differentiation of Bloch surface wave beams using an on-chip phase-shifted Bragg grating. J. Opt. 2016, 18, 115006. [Google Scholar] [CrossRef]
- Fang, Y.; Lou, Y.; Ruan, Z. On-grating graphene surface plasmons enabling spatial differentiation in the terahertz region. Opt. Lett. 2017, 42, 3840–3843. [Google Scholar] [CrossRef]
- Bykov, D.A.; Doskolovich, L.L.; Morozov, A.A.; Podlipnov, V.V.; Bezus, E.A.; Verma, P.; Soifer, V.A. First-order optical spatial differentiator based on a guided-mode resonant grating. Opt. Express 2018, 26, 10997–11006. [Google Scholar] [CrossRef] [PubMed]
- Dong, Z.; Si, J.; Yu, X.; Deng, X. Optical spatial differentiator based on subwavelength high-contrast gratings. Appl. Phys. Lett. 2018, 112, 181102. [Google Scholar] [CrossRef]
- Yang, W.; Yu, X.; Zhang, J.; Deng, X. Plasmonic transmitted optical differentiator based on the subwavelength gold gratings. Opt. Lett. 2020, 45, 2295–2298. [Google Scholar] [CrossRef]
- Parthenopoulos, A.; Darki, A.A.; Jeppesen, B.R.; Dantan, A. Optical spatial differentiation with suspended subwavelength gratings. arXiv 2020, arXiv:2008.10945. [Google Scholar]
- Zhu, T.; Zhou, Y.; Lou, Y.; Ye, H.; Qiu, M.; Ruan, Z.; Fan, S. Plasmonic computing of spatial differentiation. Nat. Commun. 2017, 8, 15391. [Google Scholar] [CrossRef]
- Zhang, J.; Ying, Q.; Ruan, Z. Time response of plasmonic spatial differentiators. Opt. Lett. 2019, 44, 4511–4514. [Google Scholar] [CrossRef]
- Zhang, W.; Zhang, X. Backscattering-Immune Computing of Spatial Differentiation by Nonreciprocal Plasmonics. Phys. Rev. Appl. 2019, 11, 054033. [Google Scholar] [CrossRef]
- Cordaro, A.; Kwon, H.; Sounas, D.; Koenderink, A.F.; Alù, A.; Polman, A. High-Index Dielectric Metasurfaces Performing Mathematical Operations. Nano Lett. 2019, 19, 8418–8423. [Google Scholar] [CrossRef] [PubMed]
- Zhou, Y.; Wu, W.; Chen, R.; Chen, W.; Chen, R.; Ma, Y. Analog Optical Spatial Differentiators Based on Dielectric Metasurfaces. Adv. Opt. Mater. 2020, 8, 1901523. [Google Scholar] [CrossRef]
- Zhou, J.; Qian, H.; Chen, C.-F.; Zhao, J.; Li, G.; Wu, Q.; Luo, H.; Wen, S.; Liu, Z. Optical edge detection based on high-efficiency dielectric metasurface. Proc. Natl. Acad. Sci. USA 2019, 116, 11137. [Google Scholar] [CrossRef] [PubMed]
- Wan, L.; Pan, D.; Yang, S.; Zhang, W.; Potapov, A.A.; Wu, X.; Liu, W.; Feng, T.; Li, Z. Optical analog computing of spatial differentiation and edge detection with dielectric metasurfaces. Opt. Lett. 2020, 45, 2070–2073. [Google Scholar] [CrossRef] [PubMed]
- Zhou, Y.; Zheng, H.; Kravchenko, I.I.; Valentine, J. Flat optics for image differentiation. Nat. Photonics 2020, 14, 316–323. [Google Scholar] [CrossRef]
- Saba, A.; Tavakol, M.R.; Karimi-Khoozani, P.; Khavasi, A. Two-Dimensional Edge Detection by Guided Mode Resonant Metasurface. IEEE Photonics Technol. Lett. 2018, 30, 853–856. [Google Scholar] [CrossRef]
- Kwon, H.; Sounas, D.; Cordaro, A.; Polman, A.; Alù, A. Nonlocal Metasurfaces for Optical Signal Processing. Phys. Rev. Lett. 2018, 121, 173004. [Google Scholar] [CrossRef]
- Kwon, H.; Cordaro, A.; Sounas, D.; Polman, A.; Alù, A. Dual-Polarization Analog 2D Image Processing with Nonlocal Metasurfaces. ACS Photonics 2020, 7, 1799–1805. [Google Scholar] [CrossRef]
- Del Hougne, P.; Lerosey, G. Leveraging Chaos for Wave-Based Analog Computation: Demonstration with Indoor Wireless Communication Signals. Phys. Rev. X 2018, 8, 041037. [Google Scholar] [CrossRef]
- Mohammadi Estakhri, N.; Edwards, B.; Engheta, N. Inverse-designed metastructures that solve equations. Science 2019, 363, 1333. [Google Scholar] [CrossRef]
- Youssefi, A.; Zangeneh-Nejad, F.; Abdollahramezani, S.; Khavasi, A. Analog computing by Brewster effect. Opt. Lett. 2016, 41, 3467–3470. [Google Scholar] [CrossRef] [PubMed]
- Xu, D.; He, S.; Zhou, J.; Chen, S.; Wen, S.; Luo, H. Goos–Hänchen effect enabled optical differential operation and image edge detection. Appl. Phys. Lett. 2020, 116, 211103. [Google Scholar] [CrossRef]
- Zhu, T.; Lou, Y.; Zhou, Y.; Zhang, J.; Huang, J.; Li, Y.; Luo, H.; Wen, S.; Zhu, S.; Gong, Q.; et al. Generalized Spatial Differentiation from the Spin Hall Effect of Light and Its Application in Image Processing of Edge Detection. Phys. Rev. Appl. 2019, 11, 034043. [Google Scholar] [CrossRef]
- Yan, W.; Qiu, M. Two-dimensional optical edge detection. Nat. Photonics 2020, 14, 268–269. [Google Scholar] [CrossRef]
- Monz, T.; Nigg, D.; Martinez, E.A.; Brandl, M.F.; Schindler, P.; Rines, R.; Wang, S.X.; Chuang, I.L.; Blatt, R. Realization of a scalable Shor algorithm. Science 2016, 351, 1068. [Google Scholar] [CrossRef]
- Rakhmanov, A.L.; Zagoskin, A.M.; Savel’ev, S.; Nori, F. Quantum metamaterials: Electromagnetic waves in a Josephson qubit line. Phys. Rev. B 2008, 77, 144507. [Google Scholar] [CrossRef]
- Zhang, W.; Cheng, K.; Wu, C.; Wang, Y.; Li, H.; Zhang, X. Implementing Quantum Search Algorithm with Metamaterials. Adv. Mater. 2017, 3, 1703986. [Google Scholar] [CrossRef]
- Cheng, K.; Zhang, W.; Wei, Z.; Fan, Y.; Xu, C.; Wu, C.; Zhang, X.; Li, H. Simulate Deutsch-Jozsa algorithm with metamaterials. Opt. Express 2020, 28, 16230–16243. [Google Scholar] [CrossRef]
- Lloyd, S. Quantum search without entanglement. Phys. Rev. A 1999, 61, 010301. [Google Scholar] [CrossRef]
- Karimi, P.; Khavasi, A.; Mousavi Khaleghi, S.S. Fundamental limit for gain and resolution in analog optical edge detection. Opt. Express 2020, 28, 898–911. [Google Scholar] [CrossRef]
- Wang, H.; Guo, C.; Zhao, Z.; Fan, S. Compact Incoherent Image Differentiation with Nanophotonic Structures. ACS Photonics 2020, 7, 338–343. [Google Scholar] [CrossRef]




| Quantum | Classical | |
|---|---|---|
| Items in the database Probability amplitude of the equivalent quantum state | “y” | |
| “E(y)” | ||
| The maximum number of the database N | “D” | |
| Um | ||
| IAA | F | |
| F | ||
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Cheng, K.; Fan, Y.; Zhang, W.; Gong, Y.; Fei, S.; Li, H. Optical Realization of Wave-Based Analog Computing with Metamaterials. Appl. Sci. 2021, 11, 141. https://doi.org/10.3390/app11010141
Cheng K, Fan Y, Zhang W, Gong Y, Fei S, Li H. Optical Realization of Wave-Based Analog Computing with Metamaterials. Applied Sciences. 2021; 11(1):141. https://doi.org/10.3390/app11010141
Chicago/Turabian StyleCheng, Kaiyang, Yuancheng Fan, Weixuan Zhang, Yubin Gong, Shen Fei, and Hongqiang Li. 2021. "Optical Realization of Wave-Based Analog Computing with Metamaterials" Applied Sciences 11, no. 1: 141. https://doi.org/10.3390/app11010141
APA StyleCheng, K., Fan, Y., Zhang, W., Gong, Y., Fei, S., & Li, H. (2021). Optical Realization of Wave-Based Analog Computing with Metamaterials. Applied Sciences, 11(1), 141. https://doi.org/10.3390/app11010141







