Hypersensitized Metamaterials Based on a Corona-Shaped Resonator for Efficient Detection of Glucose
Abstract
1. Introduction
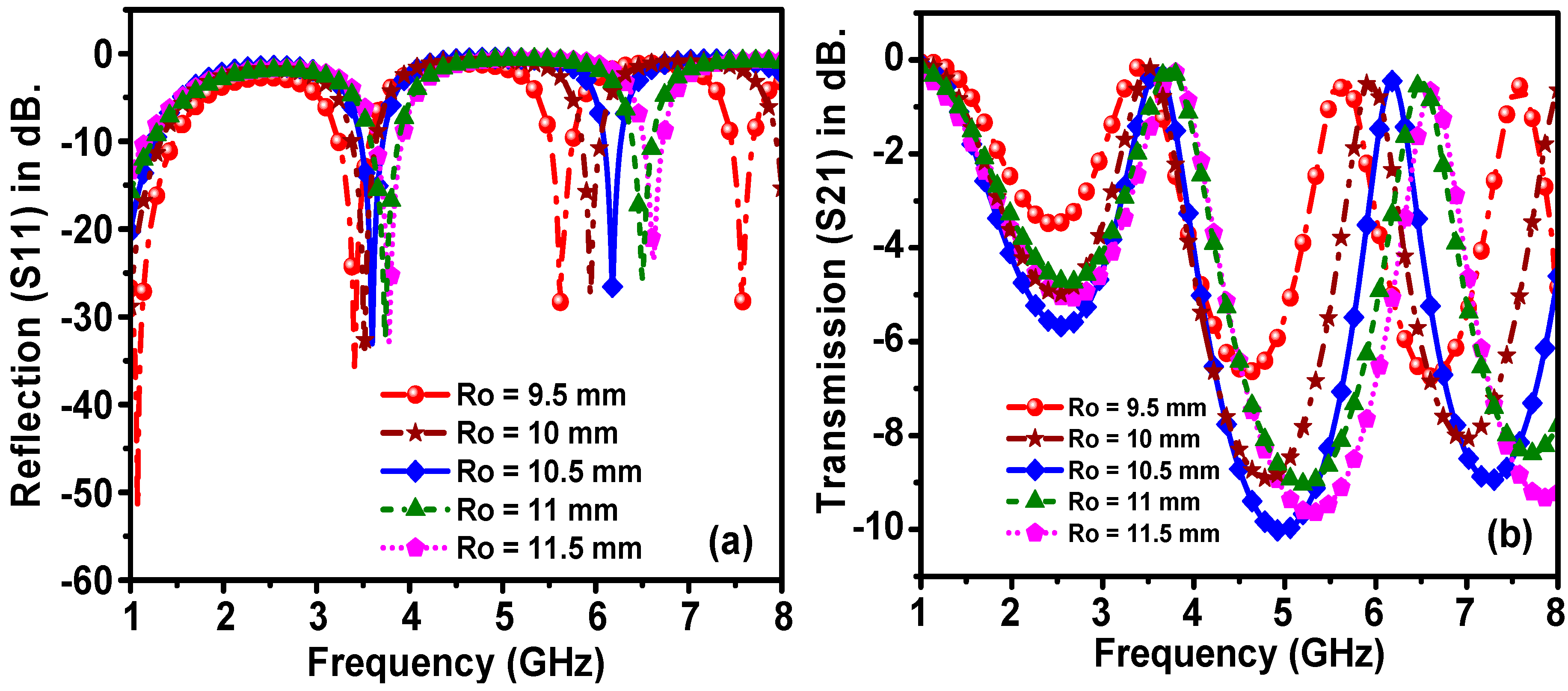
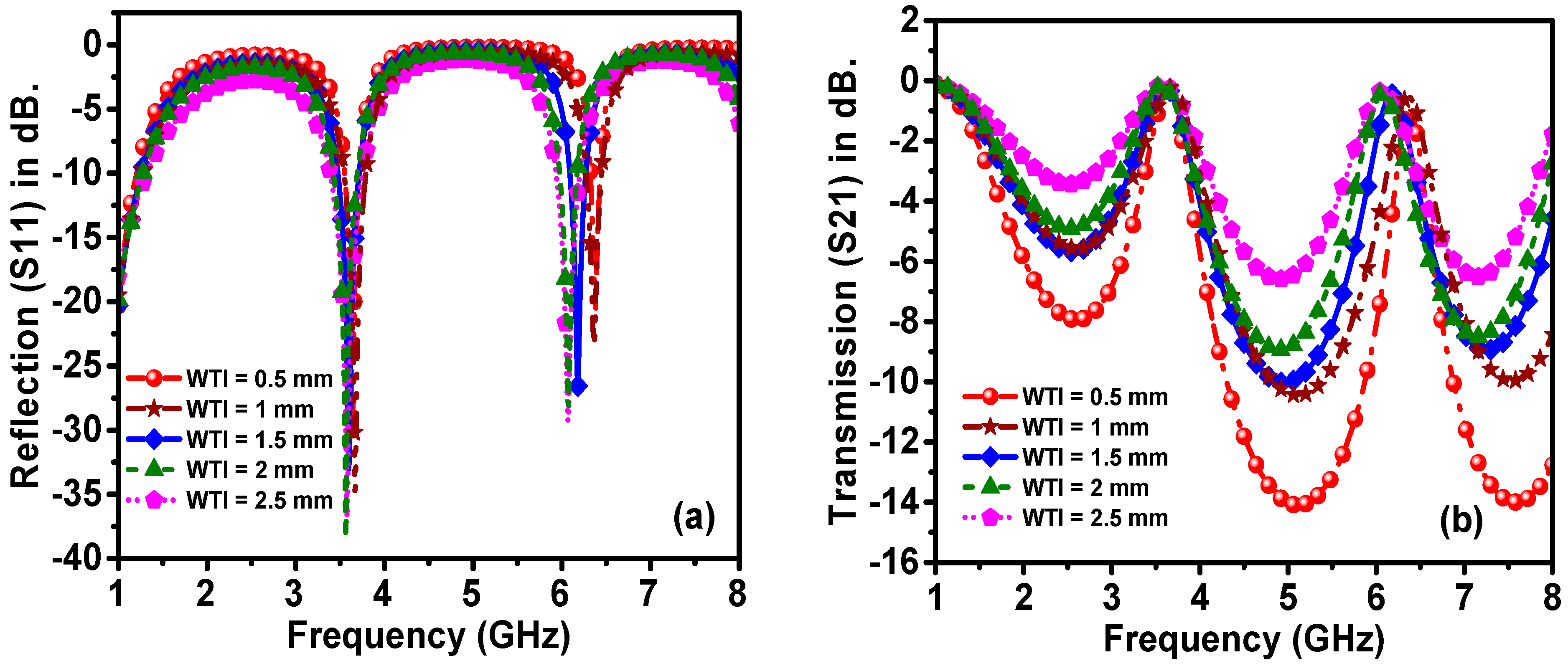
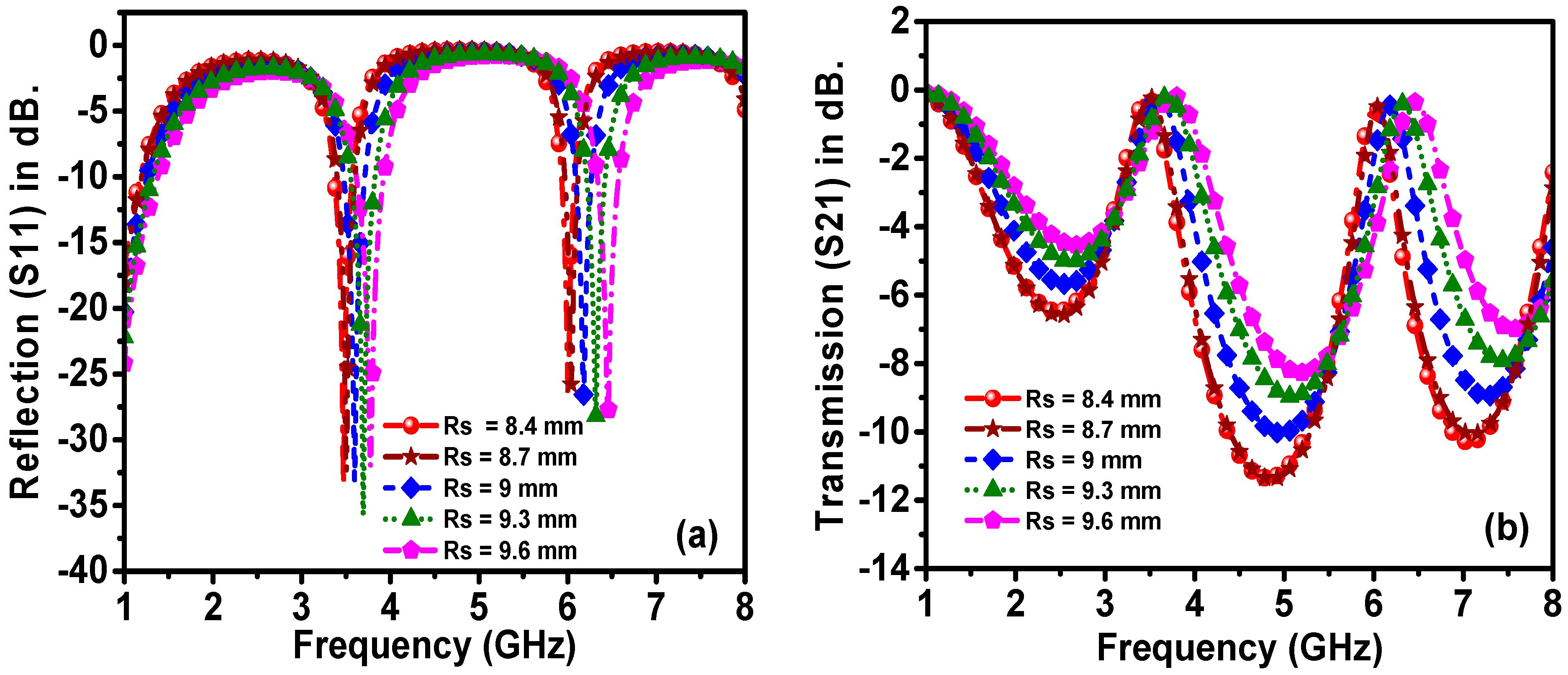
2. Structure and Design of the Metamaterial-Based Sensor
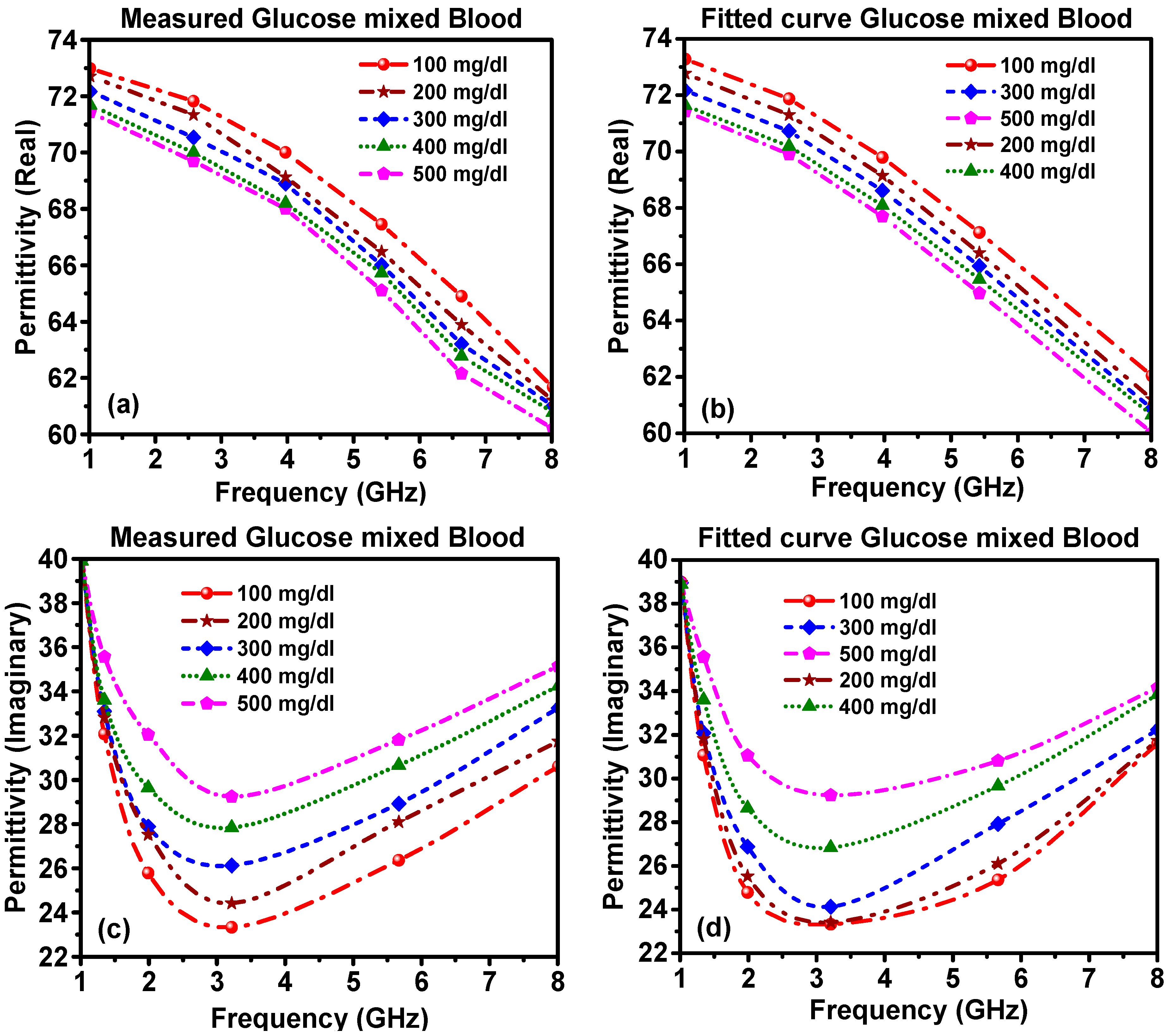
3. Dielectric Measurement of the Glucose–Water and Glucose–Blood Samples
4. Numerical and Experimental Studies of the Proposed Sensor for Glucose Detection
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Adhikari, K.K.; Chuluunbaatar, Z.; Park, H.; Jung, Y.; Cho, G.; Jo, Y.H.; Kim, S.S.; Kim, N.-Y. Flexible screen printed biosensor with high-Q microwave resonator for rapid and sensitive detection of glucose. In Proceedings of the 2014 IEEE MTT-S International Microwave Workshop Series on RF and Wireless Technologies for Biomedical and Healthcare Applications (IMWS-Bio2014), London, UK, 8–10 December 2014; pp. 1–3. [Google Scholar]
- Chou, J.-C.; Tsai, Y.-L.; Cheng, T.-Y.; Liao, Y.-H.; Ye, G.-C.; Yang, S.-Y. Fabrication of Arrayed Flexible Screen-Printed Glucose Biosensor Based on Microfluidic Framework. IEEE Sens. J. 2013, 14, 178–183. [Google Scholar] [CrossRef]
- Kim, J.; Babajanyan, A.; Hovsepyan, A.; Lee, K.; Friedman, B. Microwave dielectric resonator biosensor for aqueous glucose solution. Rev. Sci. Instrum. 2008, 79, 086107. [Google Scholar] [CrossRef]
- Amir, E.; James, S.; Kamran, G. Microwave reflective biosensor for glucose level detection in aqueous solutions. Sens. Actuators A Phys. 2020, 301, 111662. [Google Scholar] [CrossRef]
- Gonzales, W.V.; Mobashsher, A.T.; Abbosh, A.M. The Progress of Glucose Monitoring—A Review of Invasive to Minimally and Non-Invasive Techniques, Devices and Sensors. Sensors 2019, 19, 800. [Google Scholar] [CrossRef] [PubMed]
- Vélez, P.; Muñoz-Enano, J.; Grenier, K.; Mata-Contreras, J.; Dubuc, D.; Martín, F. Split ring resonator-based mi-crowave fluidic sensors for electrolyte concentration measurements. IEEE Sens. J. 2018, 19, 2562–2569. [Google Scholar] [CrossRef]
- Islam, M.T.; Hoque, A.; Almutairi, A.F.; Amin, N. Left-Handed Metamaterial-Inspired Unit Cell for S-Band Glucose Sensing Application. Sensors 2019, 19, 169. [Google Scholar] [CrossRef]
- Zhang, R.; Liu, S.; Jin, H.; Luo, Y.; Zheng, Z.; Gao, F.; Zheng, Y. Noninvasive Electromagnetic Wave Sensing of Glucose. Sensors 2019, 19, 1151. [Google Scholar] [CrossRef]
- Omer, A.E.; Safavi-Naeini, S.; Hughson, R.; Shaker, G. Blood glucose level monitoring using an FMCW millimeter-wave radar sensor. Remote Sens. 2020, 12, 385. [Google Scholar] [CrossRef]
- Omer, A.E.; Gigoyan, S.; Shaker, G.; Safavi-Naeini, S. WGM-Based Sensing of Characterized Glucose-Aqueous Solutions at mm-Waves. IEEE Access 2020, 8, 38809–38825. [Google Scholar] [CrossRef]
- Kumar, A.; Wang, C.; Meng, F.-Y.; Zhou, Z.-L.; Zhao, M.; Yan, G.; Kim, E.S.; Kim, N.-Y. High-Sensitivity, Quantified, Linear and Mediator-Free Resonator-Based Microwave Biosensor for Glucose Detection. Sensors 2020, 20, 4024. [Google Scholar] [CrossRef]
- Zarifi, M.H.; Sadabadi, H.; Hejazi, S.H.; Daneshmand, M.; Sanati-Nezhad, A. Noncontact and nonintrusive mi-crowave-microfluidic flow sensor for energy and biomedical engineering. Sci. Rep. 2018, 8, 1–10. [Google Scholar] [CrossRef] [PubMed]
- Wiltshire, B.D.; Zarifi, T.; Zarifi, M.H. Passive split ring resonator tag configuration for RFID-based wireless per-mittivity sensing. IEEE Sens. J. 2019, 20, 1904–1911. [Google Scholar] [CrossRef]
- Abdulkarim, Y.I.; Deng, L.; Karaaslan, M.; Altıntaş, O.; Awl, H.N.; Muhammadsharif, F.F.; Liao, C.; Unal, E.; Luo, H. Novel Metamaterials-Based Hypersensitized Liquid Sensor Integrating Omega-Shaped Resonator with Microstrip Transmission Line. Sensors 2020, 20, 943. [Google Scholar] [CrossRef] [PubMed]
- Lee, H.-J.; Lee, J.-H.; Moon, H.-S.; Jang, I.-S.; Choi, J.-S.; Yook, J.-G.; Jung, H.-I. A planar split-ring resonator-based microwave biosensor for label-free detection of biomolecules. Sens. Actuators B Chem. 2012, 169, 26–31. [Google Scholar] [CrossRef]
- Huang, S.Y.; Omkar; Yoshida, Y.; Inda, A.G.; Xavier, C.X.; Mu, W.C.; Meng, Y.S.; Yu, W.; Garcia, A.; Chia, X. Microstrip Line-Based Glucose Sensor for Noninvasive Continuous Monitoring Using the Main Field for Sensing and Multivariable Crosschecking. IEEE Sens. J. 2018, 19, 535–547. [Google Scholar] [CrossRef]
- Govind, G.; Akhtar, M.J. Metamaterial-Inspired Microwave Microfluidic Sensor for Glucose Monitoring in Aqueous Solutions. IEEE Sens. J. 2019, 19, 11900–11907. [Google Scholar] [CrossRef]
- Sharafadinzadeh, N.; Abdolrazzaghi, M.; Daneshmand, M. Highly sensitive microwave split ring resonator sensor using gap extension for glucose sensing. In Proceedings of the 2017 IEEE MTT-S International Microwave Workshop Series on Advanced Materials and Processes for RF and THz Applications (IMWS-AMP), Pavia, Italy, 20–22 September 2017; pp. 1–3. [Google Scholar]
- Lee, H.-J.; Choi, H.H. Microwave transmission properties of D-(+)-glucose solution with concentration variations. J. Phys. D Appl. Phys. 2019, 52, 385401. [Google Scholar] [CrossRef]
- Kumari, R.; Patel, P.N.; Yadav, R. An ENG resonator-based microwave sensor for the characterization of aqueous glucose. J. Phys. D: Appl. Phys. 2018, 51, 075601. [Google Scholar] [CrossRef]
- Hassan, R.S.; Lee, J.; Kim, S. A Minimally Invasive Implantable Sensor for Continuous Wireless Glucose Monitoring Based on a Passive Resonator. IEEE Antennas Wirel. Propag. Lett. 2020, 19, 124–128. [Google Scholar] [CrossRef]
- Buford, R.J.; Green, E.C.; McClung, M.J. A microwave frequency sensor for non-invasive blood-glucose measurement. In Proceedings of the 2008 IEEE Sensors Applications Symposium (SAS), Atlanta, GA, USA, 12–14 February 2008; pp. 4–7. [Google Scholar]
- Yilmaz, T.; Foster, R.; Hao, Y. Broadband tissue mimicking phantoms and a patch resonator for evaluating noninvasive mon-itoring of blood glucose levels. IEEE Trans. Antennas Propag. 2014, 62, 3064–3075. [Google Scholar] [CrossRef]
- Juan, C.G.; Bronchalo, E.; Potelon, B.; Quendo, C.; Ávila-Navarro, E.; Sabater-Navarro, J.M. Concentration Measurement of Microliter-Volume Water–Glucose Solutions Using Q Factor of Microwave Sensors. IEEE Trans. Instrum. Meas. 2018, 68, 2621–2634. [Google Scholar] [CrossRef]
- Choi, H.; Naylon, J.; Luzio, S.D.; Beutler, J.; Birchall, J.; Martin, C.L.; Porch, A. Design and In Vitro Interference Test of Microwave Noninvasive Blood Glucose Monitoring Sensor. IEEE Trans. Microw. Theory Tech. 2015, 63, 3016–3025. [Google Scholar] [CrossRef] [PubMed]
- Kim, N.Y.; Adhikari, K.K.; Dhakal, R.; Chuluunbaatar, Z.; Wang, C.; Kim, E.S. Rapid, Sensitive and Reusable Detection of Glucose by a Robust Radiofrequency Integrated Passive Device Biosensor Chip. Sci. Rep. 2015, 5, 7807. [Google Scholar] [CrossRef] [PubMed]
- Freer, B.; Venkataraman, J. Feasibility study for non-invasive blood glucose monitoring. In Proceedings of the 2010 IEEE Antennas and Propagation Society International Symposium 2010 (APSURSI), Toronto, ON, Canada, 11–17 July 2010; pp. 1–4. [Google Scholar]
- Yilmaz, T.; Ozturk, T.; Joof, S. A Comparative Study for Development of Microwave Glucose Sensors. In Proceedings of the 32nd URSI GASS International Union of Radio Silence, General Assembly and Scientific Symposium, Montreal, QC, Canada, 19–26 August 2017; pp. 1–4. [Google Scholar]
- Vrba, J.; Karch, J.; Vrba, D. Phantoms for development of microwave sensors for noninvasive blood glucose monitoring. Int. J. Antennas Propag. 2015, 2015, 570870. [Google Scholar] [CrossRef]
- Turgul, V.; Kale, I. Permittivity extraction of glucose solutions through artificial neural networks and non-invasive microwave glucose sensing. Sens. Actuators A Phys. 2018, 277, 65–72. [Google Scholar] [CrossRef]
- Malthesh, S.; Krishnaswamy, N. Improvement in quality factor of double microring resonator for sensing applications. J. Nanophotonics 2019, 13, 026014. [Google Scholar] [CrossRef]
- Kiani, S.; Rezaei, P.; Navaei, M.; Abrishamian, M.S. Microwave Sensor for Detection of Solid Material Permittivity in Single/Multilayer Samples with High Quality Factor. IEEE Sens. J. 2018, 18, 9971–9977. [Google Scholar] [CrossRef]
- Ramanujam, N.; El-Khozondar, H.J.; Dhasarathan, V.; Taya, S.A.; Aly, A.H. Design of one dimensional defect based photonic crystal by composited superconducting material for bio sensing applications. Phys. B Condens. Matter 2019, 572, 42–55. [Google Scholar] [CrossRef]
- Adhikari, K.K.; Kim, N.-Y. Ultrahigh-Sensitivity Mediator-Free Biosensor Based on a Microfabricated Microwave Resonator for the Detection of Micromolar Glucose Concentrations. IEEE Trans. Microw. Theory Tech. 2015, 64, 319–327. [Google Scholar] [CrossRef]
- Abdulkarim, Y.I.; Deng, L.; Luo, H.; Huang, S.; Karaaslan, M.; Altıntaş, O.; Bakır, M.; Muhammadsharif, F.F.; Awl, H.N.; Sabah, C.; et al. Design and study of a metamaterial based sensor for the application of liquid chemicals detection. J. Mater. Res. Technol. 2020, 9, 10291–10304. [Google Scholar] [CrossRef]
- Tamer, A.; Karadağ, F.; Ünal, E.; Abdulkarim, Y.I.; Deng, L.; Altintas, O.; Bakır, M.; Karaaslan, M. Metamaterial based sensor integrating transmission line for detection of branded and unbranded diesel fuel. Chem. Phys. Lett. 2020, 742, 137169. [Google Scholar] [CrossRef]
- Bakır, M.; Dalgaç, Ş.; Ünal, E.; Karadağ, F.; Demirci, M.; Köksal, A.S.; Akgöl, O.; Karaaslan, M. High Sensitive Metamaterial Sensor for Water Treatment Centres. Water Air Soil Pollut. 2019, 230, 304. [Google Scholar] [CrossRef]
- Bakır, M.; Dalgaç, Ş.; Karaaslan, M.; Karadağ, F.; Akgol, O.; Unal, E.; Depçi, T.; Sabah, C. A Comprehensive Study on Fuel Adulteration Sensing by Using Triple Ring Resonator Type Metamaterial. J. Electrochem. Soc. 2019, 166, B1044. [Google Scholar] [CrossRef]
- Abdulkarim, Y.I.; Deng, L.; Altıntaş, O.; Ünal, E.; Karaaslan, M. Metamaterial absorber sensor design by incorpo-rating swastika shaped resonator to determination of the liquid chemicals depending on electrical characteristics. Phys. E Low-Dimens. Syst. Nanostruct. 2019, 114, 113593. [Google Scholar] [CrossRef]
- Abdulkarim, Y.I.; Deng, L.; Karaaslan, M.; Unal, E. Determination of the liquid chemicals depending on the electrical characteristics by using metamaterial absorber based sensor. Chem. Phys. Lett. 2019, 732, 136655. [Google Scholar] [CrossRef]
- Erdoğan, M.; Ünal, E.; Alkurt, F.Ö.; Abdulkarim, Y.I.; Deng, L.; Karaaslan, M. Determination of frying sunflower oil usage time for local potato samples by using microwave transmission line based sensors. Measurement 2020, 163, 108040. [Google Scholar] [CrossRef]
- Yadav, R.; Patel, P.N.; Kumari, R.; Lad, V.N. Development of a Metallic Photonic Bandgap-Inspired Probe for Detection of Weak Basic Dissociation Constant Drug in Bio-Fluid. IEEE Sens. J. 2017, 17, 5410–5418. [Google Scholar] [CrossRef]
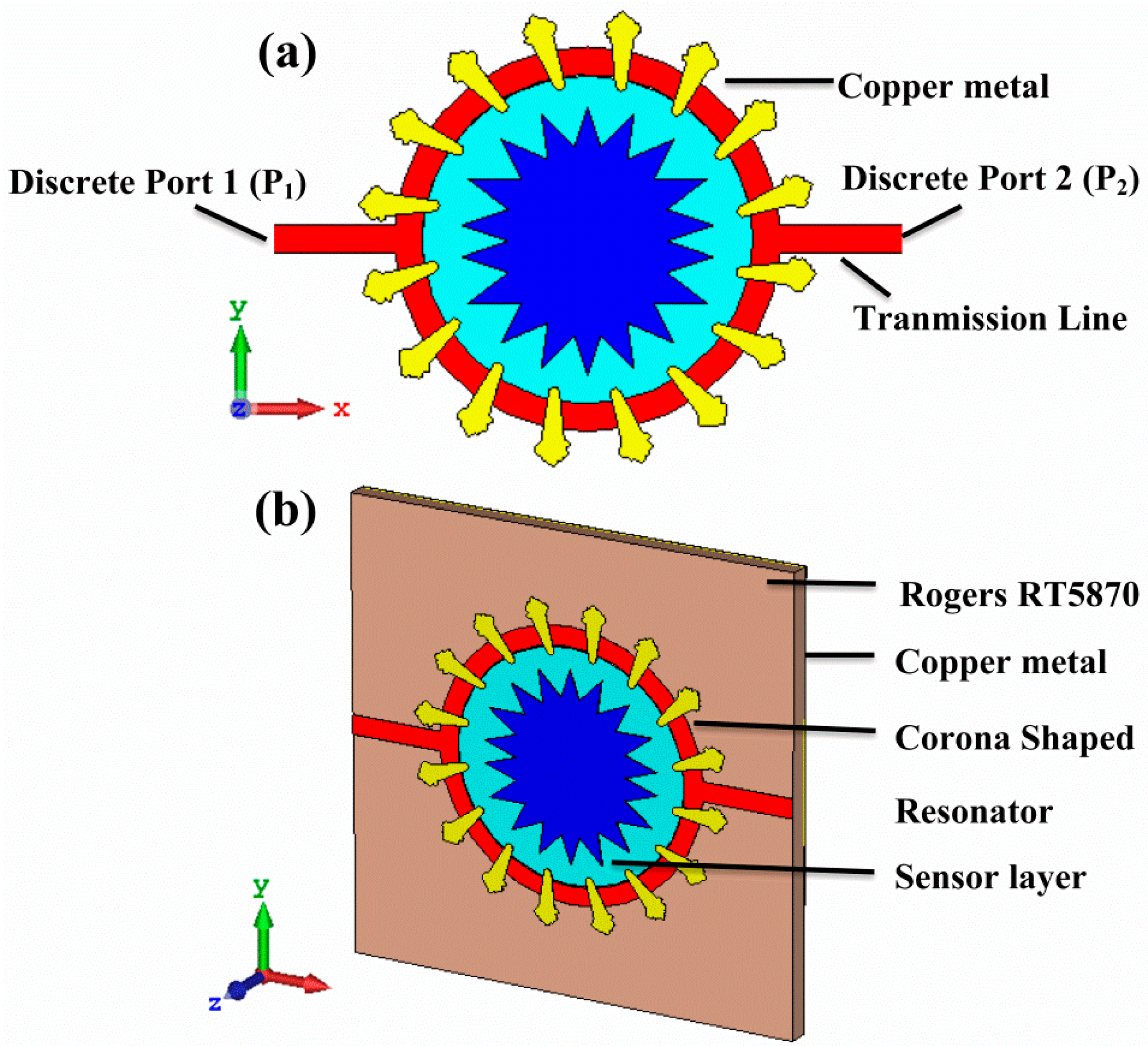
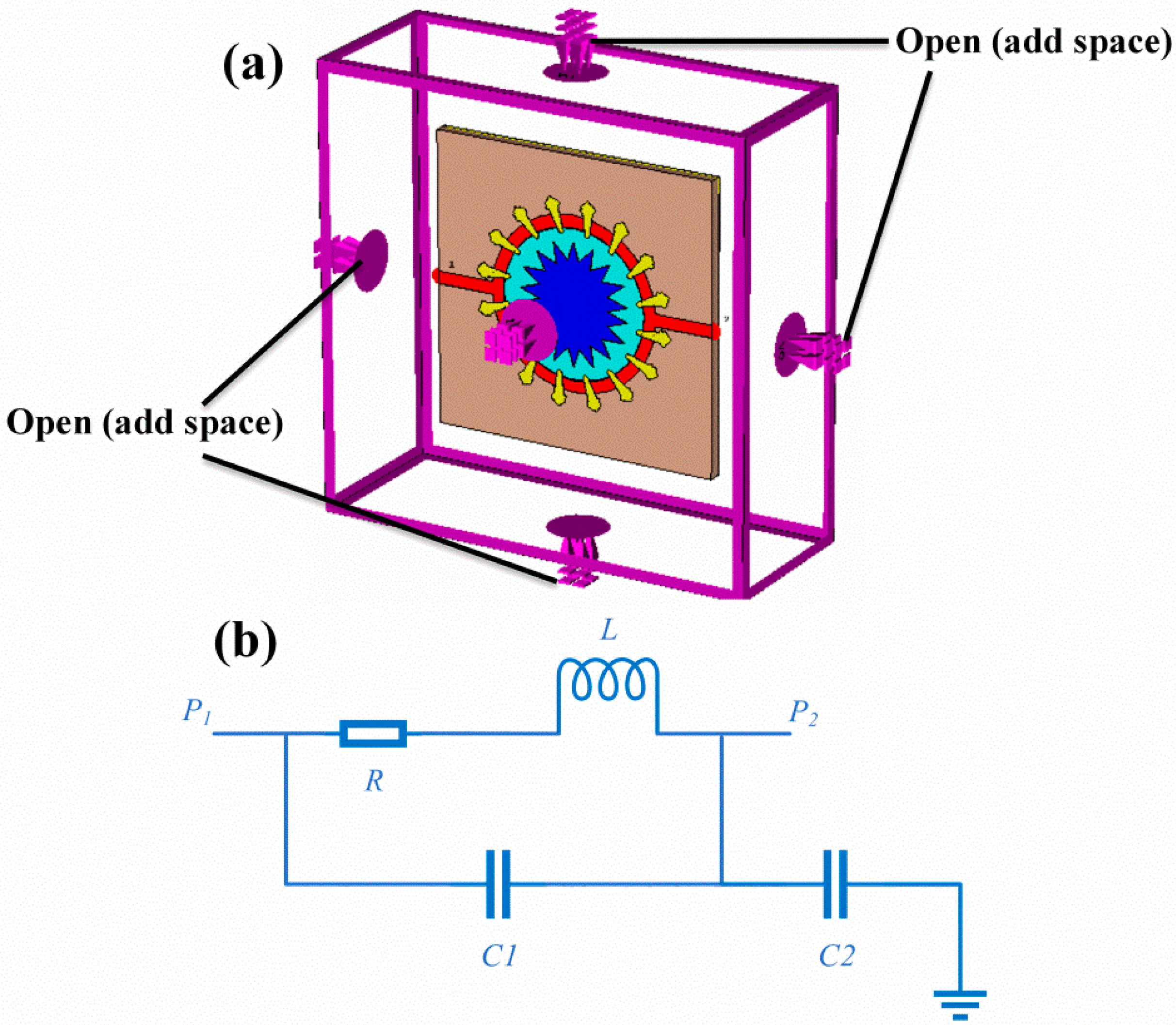
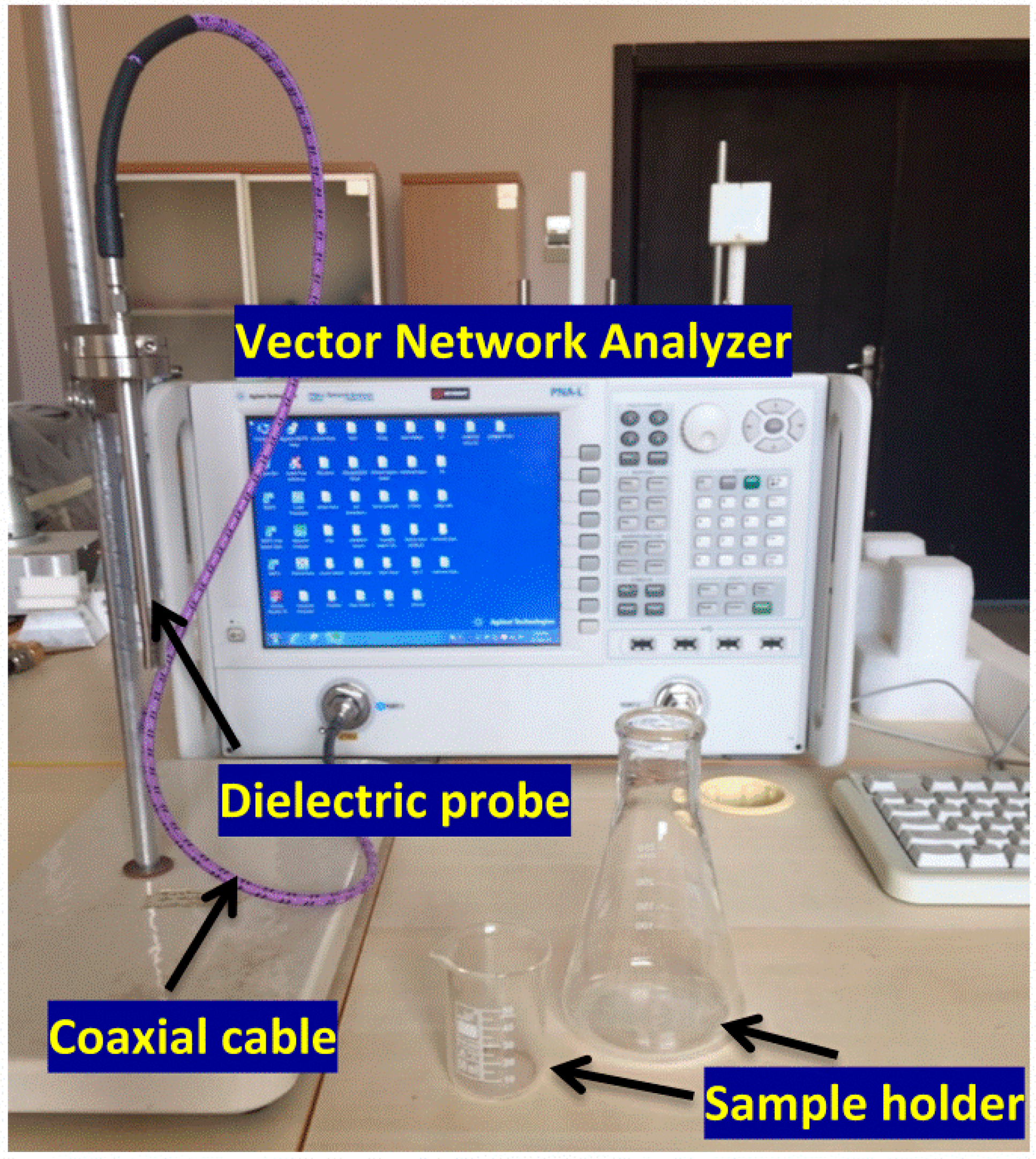
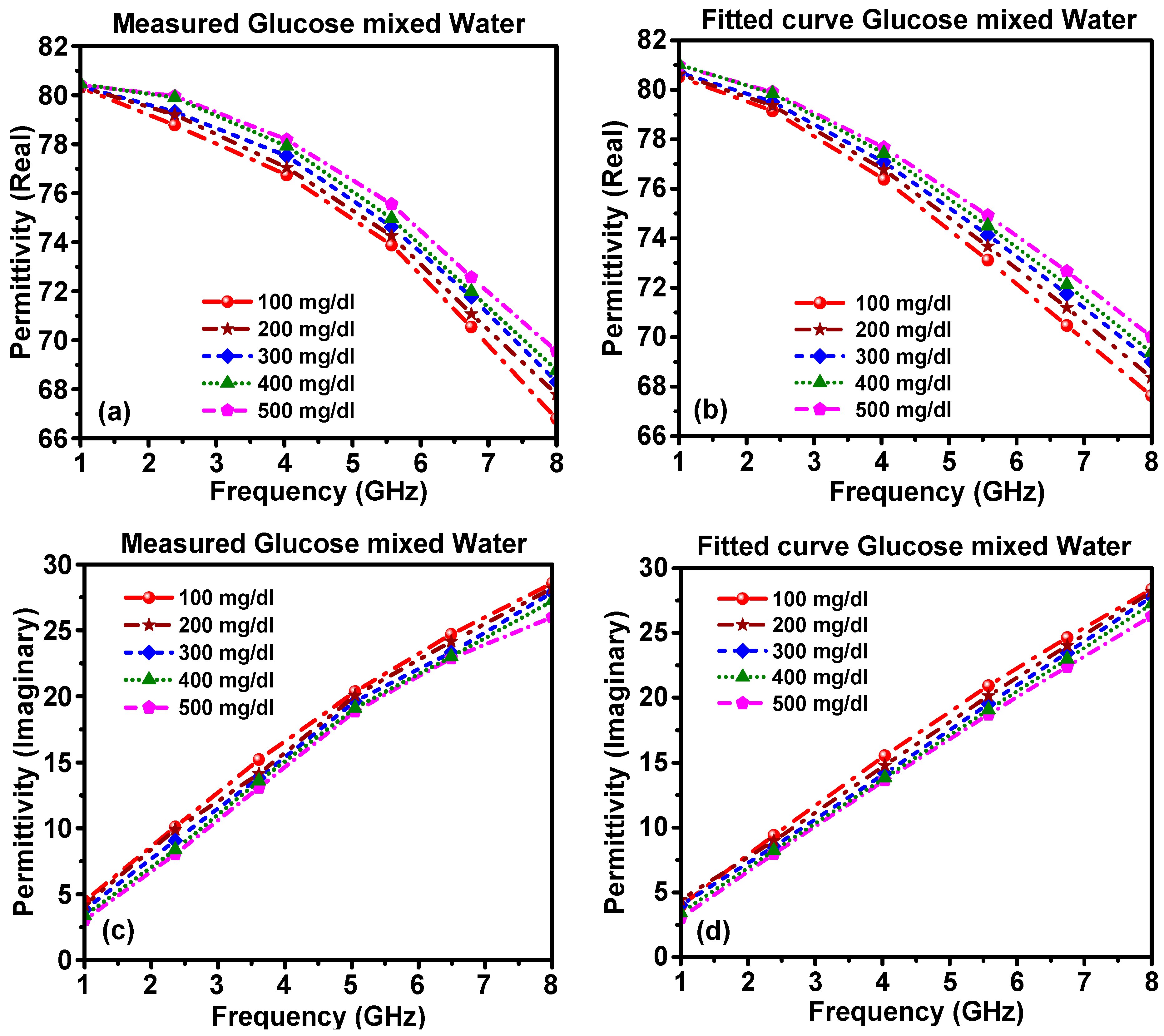




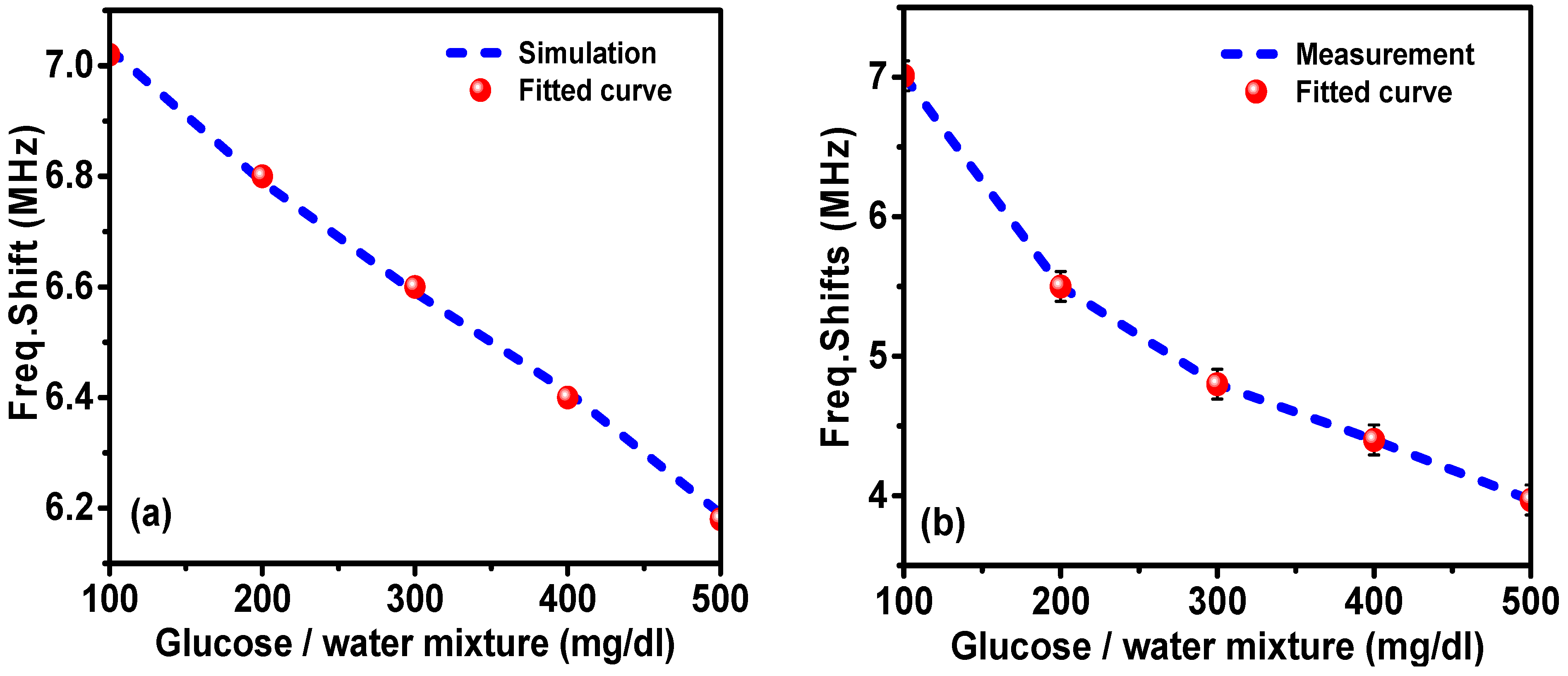
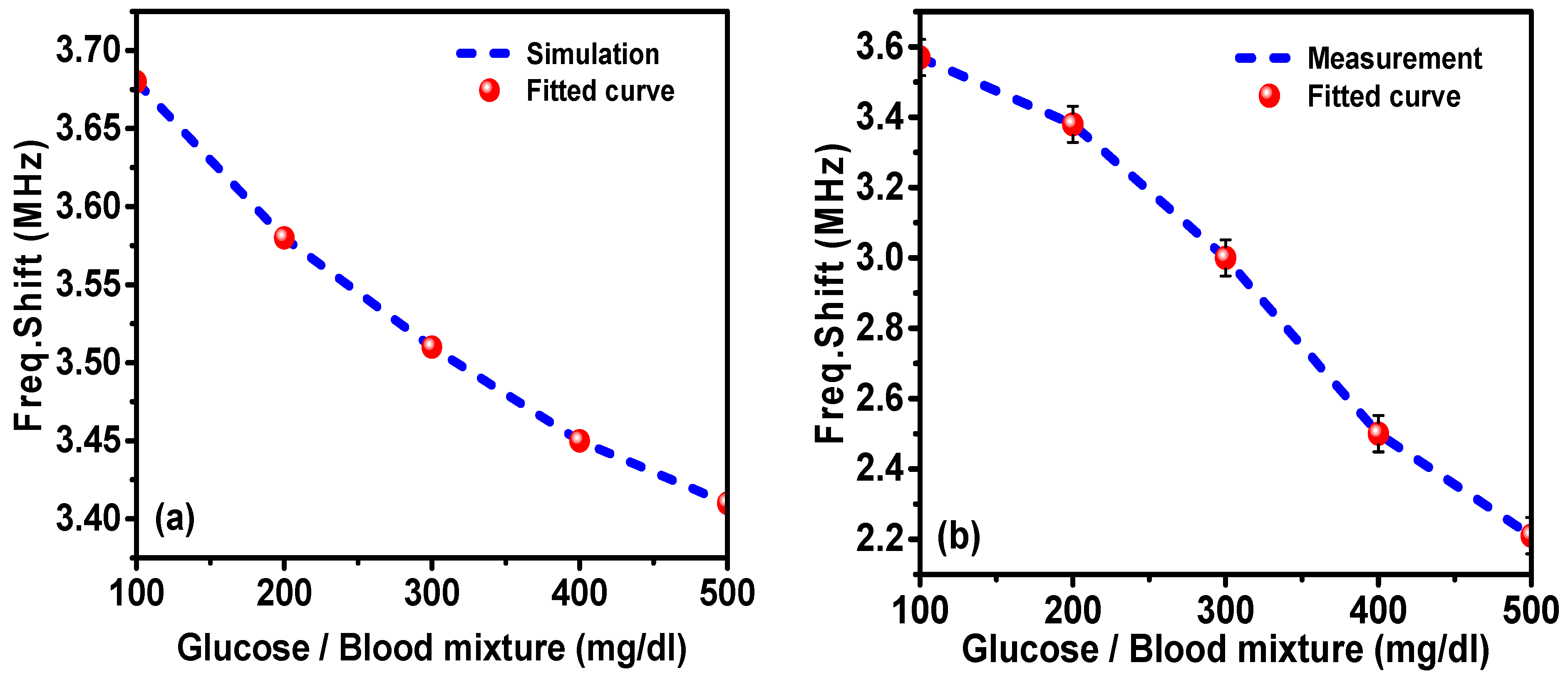
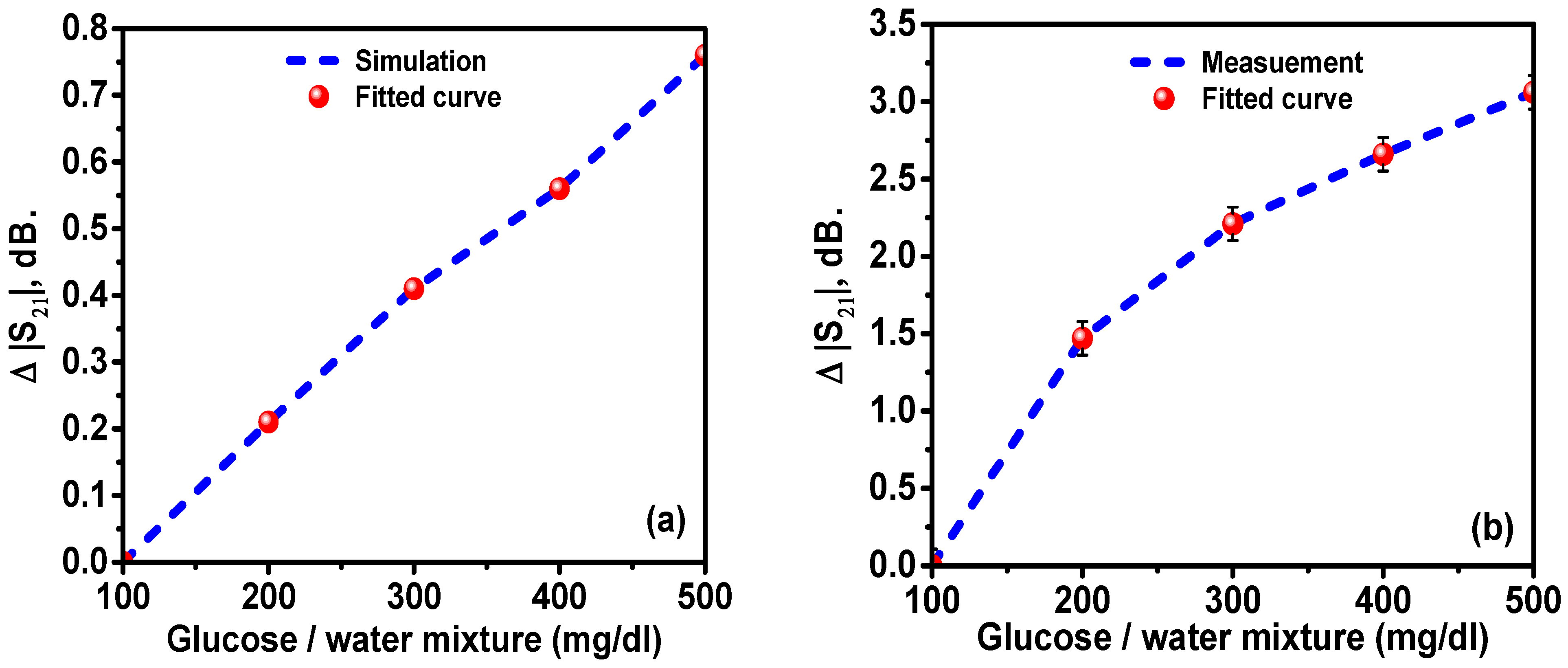



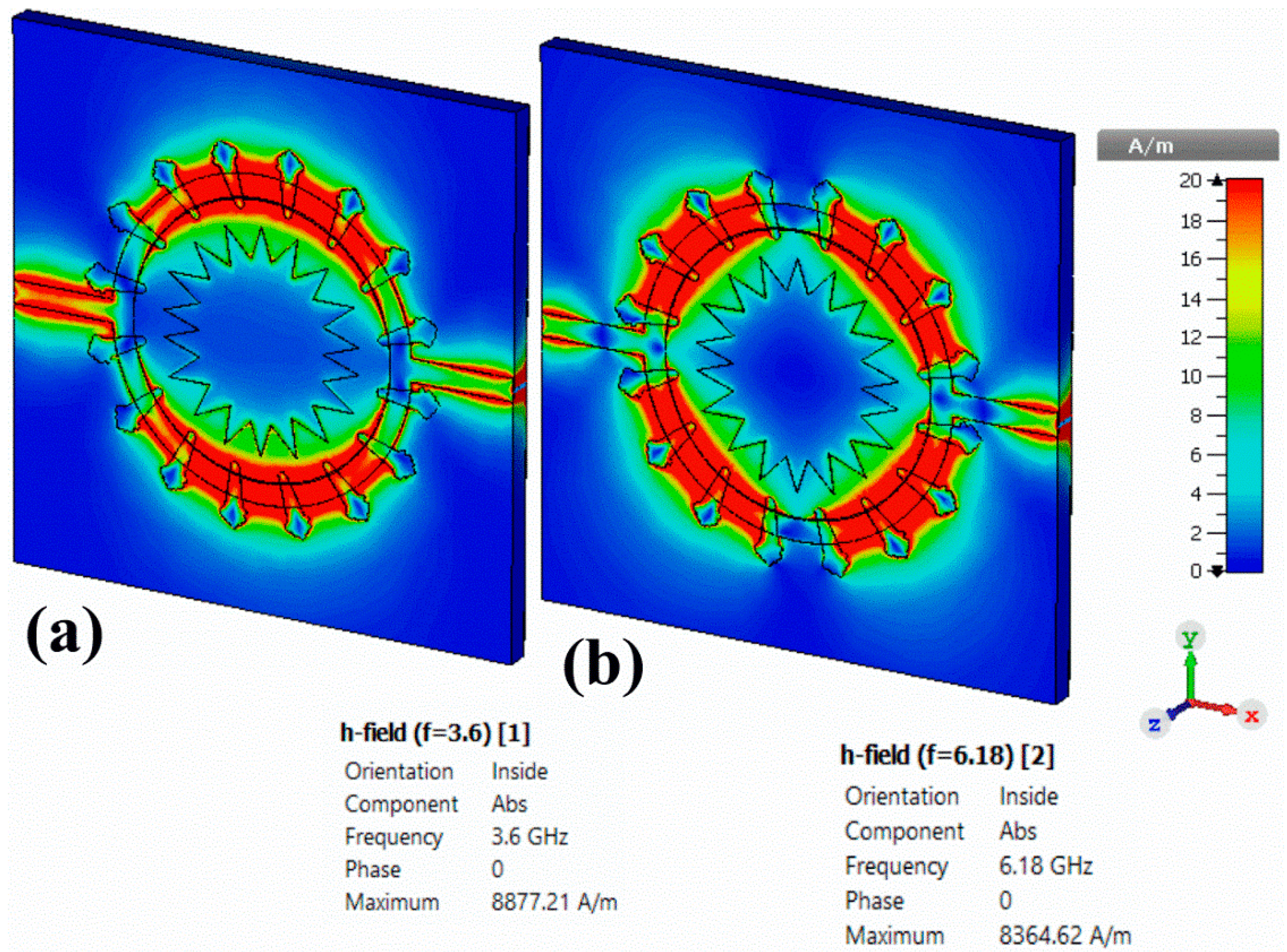
| Dimensions | Size (mm) |
|---|---|
| Radius of resonator | 9 |
| Width of resonator | 1.5 |
| Length of transmission line | 7.5 |
| Width of transmission line | 1.5 |
| Radius of star shape | 7.3 |
| Length of substrate | 35 |
| With of substrate | 35 |
| Ref. | Size (mm) | Substrate Materials | Frequency Range (GHz) | Sensitivity | Application Procedure | Remarks |
|---|---|---|---|---|---|---|
| [6] | 46 × 46 | FR-4 | 0.7–1.2 | 0.033 MHz g/L | Noninvasive | Split Ring Resonator (SRR)-based microwave fluidic sensors |
| [7] | 20 × 20 | Rogers RO4350B | 2–5 | 0.037 GHz 30 mg/dL | Noninvasive | Sensing capacity with double negative (DNG) property and minimal absorption |
| [10] | 40 × 40 | Silicon | 50–67 | Range 2.2–7.7 mg/mL | Noninvasive | Whispering Gallery Modes (WGMs) launched in a dielectric disc resonator (DDR) |
| [17] | 50 × 20 | Rogers RT6006 | 1–5 | 0.026 MHz mg/dL | Noninvasive | Metamaterial-inspired microwave microfluidic SRR |
| [18] | 20 × 15 | Rogers RT5880 | 1–2 | 1.6 MHz1–15 g/dL | Glucose-sensing | SRR resonator without metamaterials |
| [20] | 40 × 20 | FR-4 | 1–3 | Range 20–100 mg/mL | Invasive | Microwave filter as a sensor device |
| This work | 35 × 35 | Rogers RT5880 | 1–8 | 1.51 GHz 100–500 mg/dL | Glucose-sensing | Corona resonator based on metamaterials |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Abdulkarim, Y.I.; Muhammadsharif, F.F.; Bakır, M.; Awl, H.N.; Karaaslan, M.; Deng, L.; Huang, S. Hypersensitized Metamaterials Based on a Corona-Shaped Resonator for Efficient Detection of Glucose. Appl. Sci. 2021, 11, 103. https://doi.org/10.3390/app11010103
Abdulkarim YI, Muhammadsharif FF, Bakır M, Awl HN, Karaaslan M, Deng L, Huang S. Hypersensitized Metamaterials Based on a Corona-Shaped Resonator for Efficient Detection of Glucose. Applied Sciences. 2021; 11(1):103. https://doi.org/10.3390/app11010103
Chicago/Turabian StyleAbdulkarim, Yadgar I., Fahmi F. Muhammadsharif, Mehmet Bakır, Halgurd N. Awl, Muharrem Karaaslan, Lianwen Deng, and Shengxiang Huang. 2021. "Hypersensitized Metamaterials Based on a Corona-Shaped Resonator for Efficient Detection of Glucose" Applied Sciences 11, no. 1: 103. https://doi.org/10.3390/app11010103
APA StyleAbdulkarim, Y. I., Muhammadsharif, F. F., Bakır, M., Awl, H. N., Karaaslan, M., Deng, L., & Huang, S. (2021). Hypersensitized Metamaterials Based on a Corona-Shaped Resonator for Efficient Detection of Glucose. Applied Sciences, 11(1), 103. https://doi.org/10.3390/app11010103








