Modeling of the Vertical Movements of the Earth’s Crust in Poland with the Co-Kriging Method Based on Various Sources of Data
Abstract
1. Introduction
2. Materials and Methods
- (a)
- Isotropic: On isotropic surfaces, changes in attribute values are influenced by distance but not by direction; therefore, attribute values change identically in all directions.
- (b)
- Anisotropic: On anisotropic surfaces, changes in attribute values are influenced by both distance and direction; therefore, attribute values change irregularly in space and they differ in various directions.
- (a)
- Geometric anisotropy: Attribute values change similarly in all directions but vary with distance; therefore, the same variability is achieved in different directions when points are separated by a varied distance.
- (b)
- Zonal anisotropy: Variability is not regularly distributed in space; this type of anisotropy results from data trends.
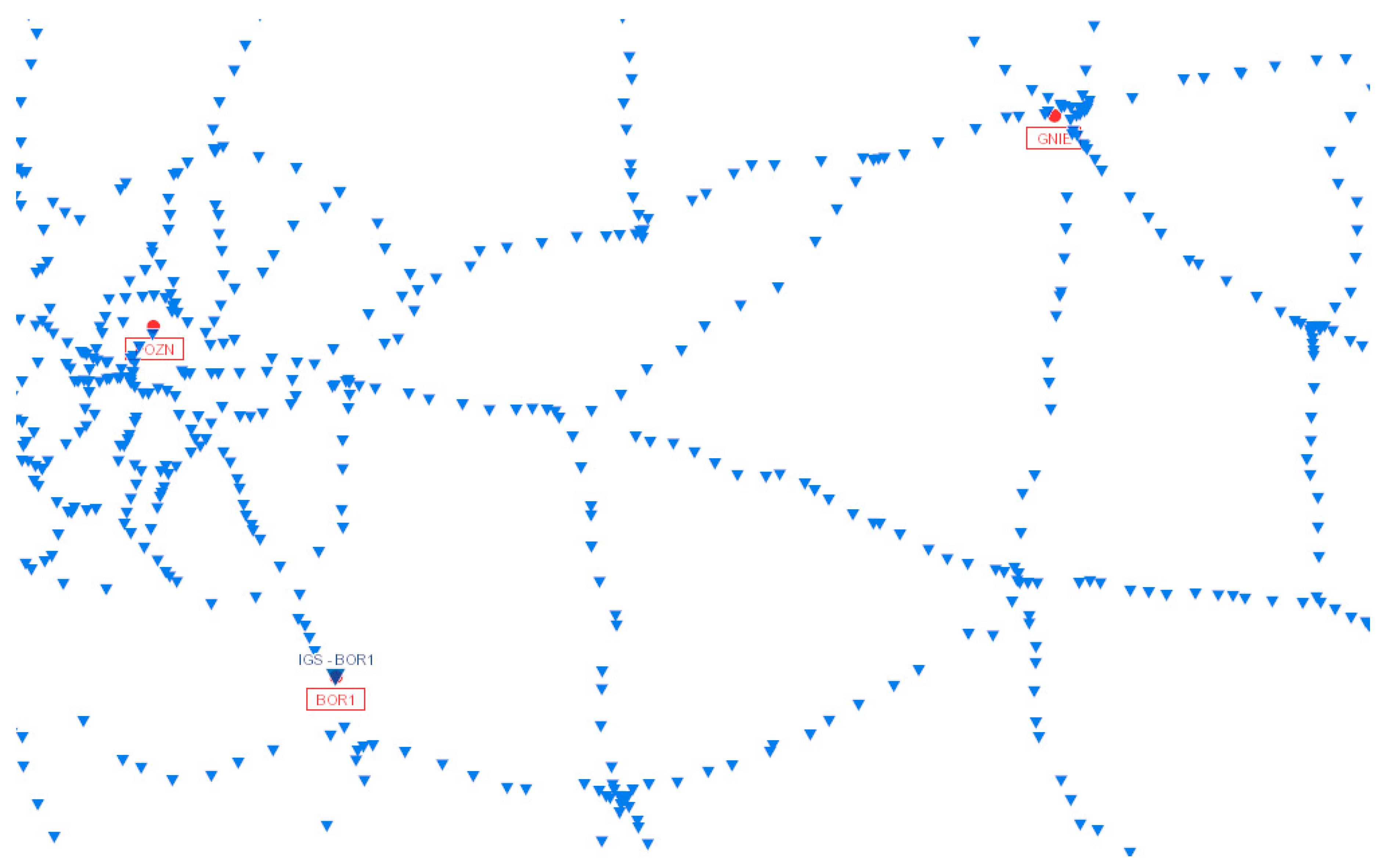
3. Calculation
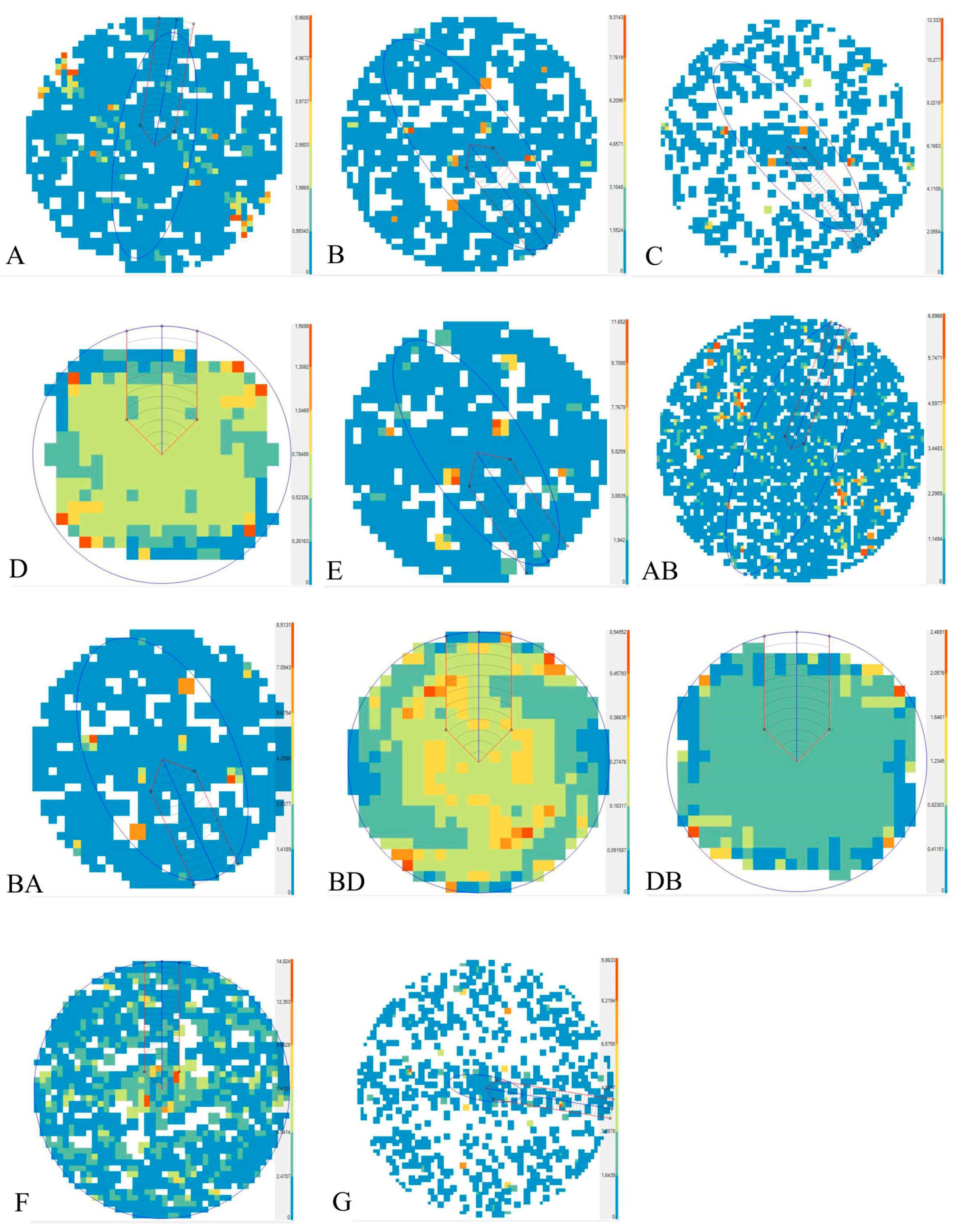
3.1. Variogram Maps for Evaluating Dataset Coherence: Anisotropy and Isotropy of Data
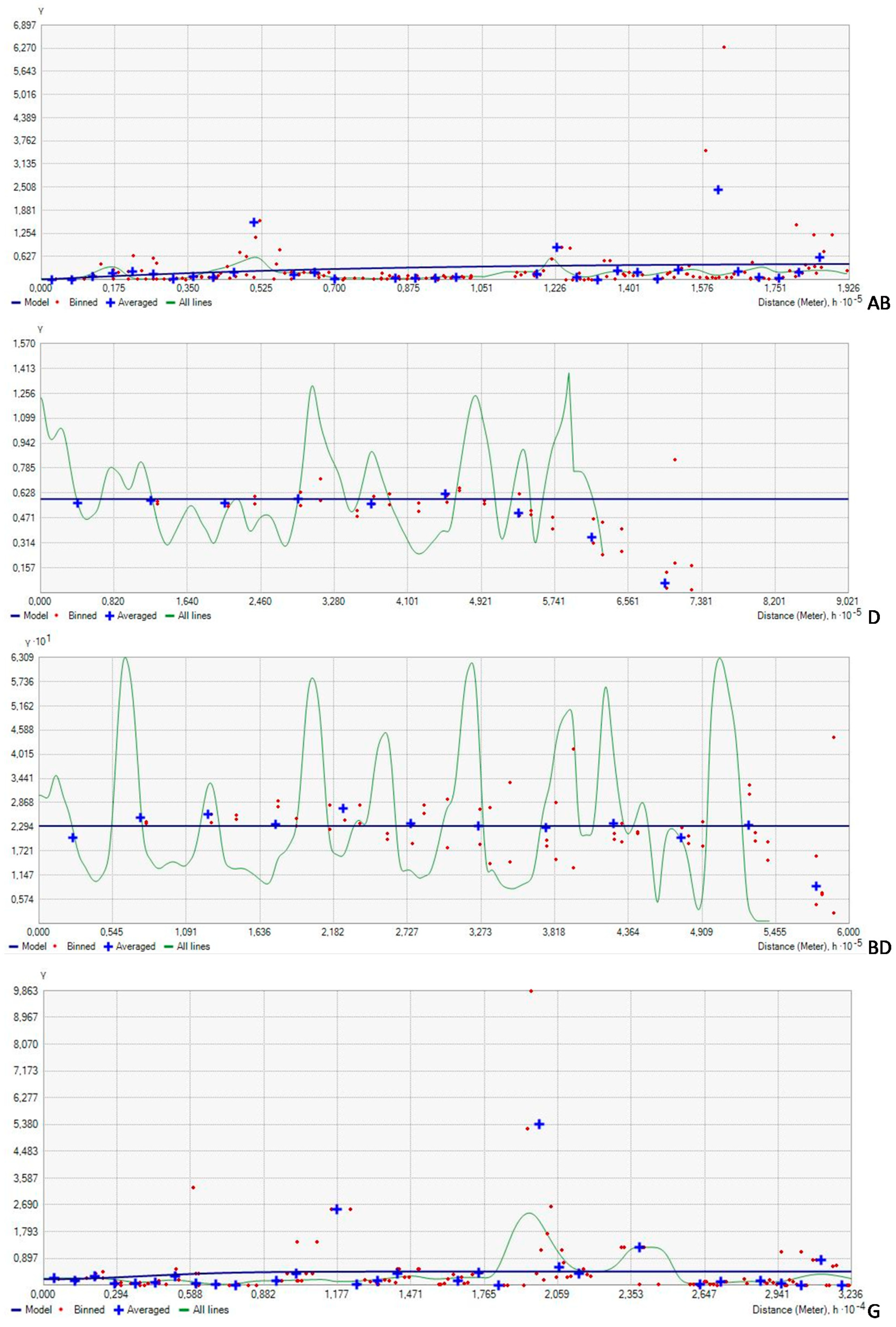
3.2. Empirical Variograms and the Selection of Theoretical Variograms
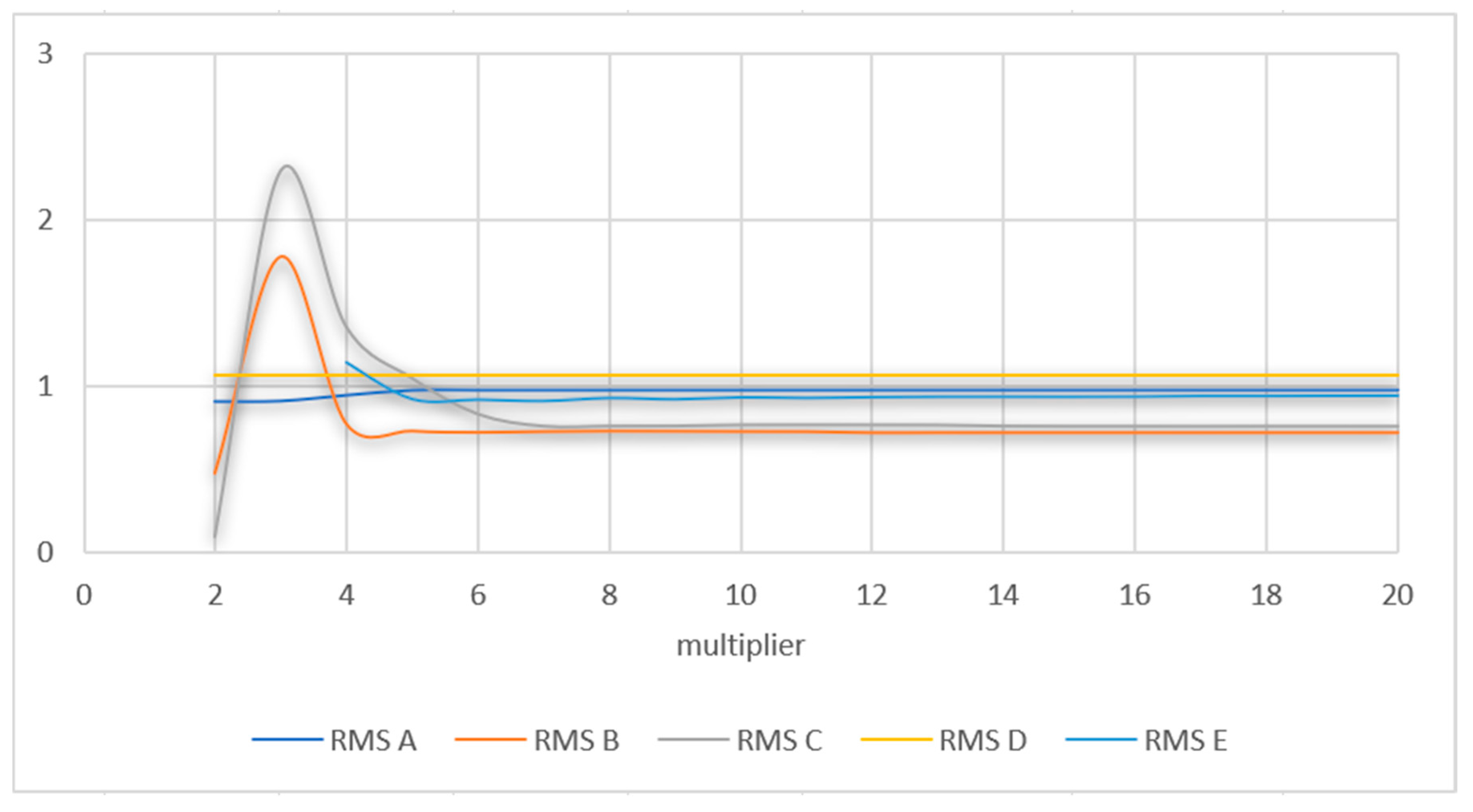
3.3. Calculation of the Interpolation Parameters
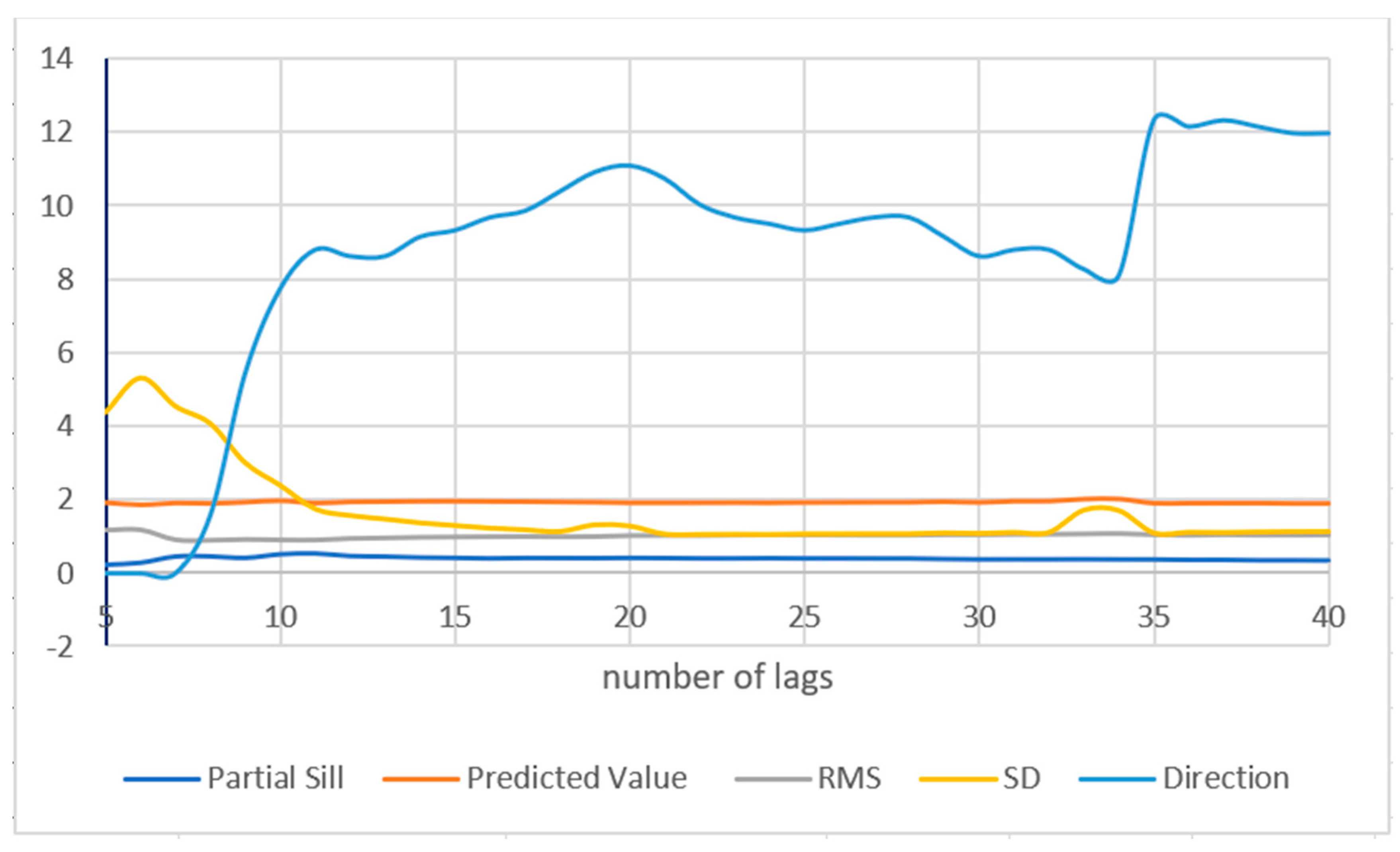
4. Results
5. Discussion
6. Conclusions
Author Contributions
Conflicts of Interest
References
- Douglas, B.C. Global sea level rise. J. Geophys. Res. 1991, 96, 6981–6992. [Google Scholar] [CrossRef]
- Billiris, H.; Paradissis, D.; Veis, G.; England, P.; Featherstone, W.; Parsons, B.; Cross, P.; Rands, P.; Rayson, M.; Sellers, P.; et al. Geodetic determination of tectonic deformation in central Greece from 1900 to 1988. Nature 1991, 350, 124–129. [Google Scholar] [CrossRef]
- Hooper, A.; Segall, P.; Zebker, H. Persistent scatterer interferometric synthetic aperture radar for crustal deformation analysis, with application to Volcán Alcedo, Galápagos. J. Geophys. Res. Solid Earth 2007, 112. [Google Scholar] [CrossRef]
- Blewitt, G.; Altamimi, Z.; Davis, J.; Gross, R.; Kuo, C.; Lemoine, F.; Moore, A.; Neilan, R.; Plag, H.; Rothacher, M.; et al. Geodetic Observations and Global Reference Frame Contributions to Understanding Sea-Level Rise and Variability. In Understanding Sea Level Rise and Variability; Church, J.A., Woodworth, P.L., Wilson, S., Aarup, T., Eds.; Wiley: Chichester, UK, 2010; pp. 256–284. [Google Scholar]
- Zhu, X.; Wang, R.; Sun, F.; Wang, J. A Unified Global Reference Frame of Vertical Crustal Movements by Satellite Laser Ranging. Sensors 2016, 16, 225. [Google Scholar] [CrossRef]
- Bogusz, J.; Kłos, A.; Grzempowski, P.; Kontny, B. Modelling the Velocity Field in a Regular Grid in the Area of Poland on the Basis of the Velocities of European Permanent Stations. Pure Appl. Geophys. 2014, 171, 809–833. [Google Scholar] [CrossRef]
- Fuhrmann, T.; Caro Cuenca, M.; Knöpfler, A.; Van Leijen, F.J.; Mayer, M.; Westerhaus, M.; Hansen, R.; Heck, B. Estimation of small surface displacements in the Upper Rhine Graben area from a combined analysis of PS-InSAR, levelling and GNSS data. Geophys. J. Int. 2015, 203, 614–631. [Google Scholar] [CrossRef]
- Hofmann-Wellenhof, B.; Lichtenegger, H.; Collins, J. Global Positioning System: Theory and Practice; Springer Science & Business Media: Wien, Austria, 2012. [Google Scholar]
- Bürgmann, R.; Rosen, P.A.; Fielding, E.J. Synthetic aperture radar interferometry to measure Earth’s surface topography and its deformation. Annu. Rev. Earth Planet. Sci. 2000, 28, 169–209. [Google Scholar] [CrossRef]
- Pearlman, M.R.; Degnan, J.J.; Bosworth, J.M. The international laser ranging service. Adv. Space Res. 2002, 30, 135–143. [Google Scholar] [CrossRef]
- Campbell, J. Very Long Baseline Interferometry—A high precision tool for geodesy and astrometry. C. R. Acad. Sci.-IV-Phys. 2000, 1, 1255–1265. [Google Scholar] [CrossRef]
- Altamimi, Z.; Sillard, P.; Boucher, C. ITRF2000 A new release of the International Terrestrial Reference Frame for earth science applications. J. Geophys. Res. 2002, 107, ETG-2. [Google Scholar] [CrossRef]
- Matsumura, S.; Murakami, M.; Imakiire, T. Concept of the New Japanese Geodetic System; Bulletin of the Geographical Survey Institute: Tokyo, Japan, 2004; Volume 51, pp. 1–9. [Google Scholar]
- Sacher, M.; Ihde, J.; Liebsch, G.; Mäkinen, J. EVRF07 as Realization of the European Vertical Reference System. Presented at the Symposium of the IAG Sub-Commission for Europe (EUREF), Brussels, Belgium, 18–21 June 2008; Volume 68, pp. 35–50. [Google Scholar]
- Kenyeres, A.; Jambor, T.; Caporali, A.; Drosč_cak, B.; Garayt, B.; Georgiev, I.; Jumare, I.; Nagl, J.; Pihlak, P.; Ryczywolski, M. Integration of the EPN and the dense national Permanent Networks. Presented at EUREF Symposium, Budapest, Hungary, 29–31 May 2013. [Google Scholar]
- Ågren, J.; Svensson, R. Postglacial Land Uplift Model and System Definition for the New Swedish Height System RH 2000; Reports in Geodesy and Geographical Information Systems; Lantmäteriverket: Gävle, Sweden, 2007.
- Vestøl, O. Determination of postglacial land uplift in Fennoscandia from leveling, tide-gauges and continuous GPS stations using least squares collocation. J. Geod. 2007, 80, 248–258. [Google Scholar] [CrossRef]
- Kowalczyk, K.; Rapiński, J. Robust network adjustment of vertical movements with GNSS data. Geofizika 2017, 34, 45–65. [Google Scholar] [CrossRef]
- Kowalczyk, K.; Bogusz, J.; Figurski, M. The analysis of the selected data from Polish Active Geodetic Network stations with the view on creating a model of vertical crustal movements. In Proceedings of the 9th International Conference “Environmental Engineering”, Vilnius, Lithuania, 22–23 May 2014; ISBN 978-609-457-640-9. [Google Scholar] [CrossRef]
- Bednarczyk, M.; Kowalczyk, K.; Kowalczyk, A.M. Identification of pseudo-nodal points on the basis of precise leveling campaigns data and GNSS. Acta Geodyn. Geomater. 2018, 15, 5–16. [Google Scholar] [CrossRef]
- Oliver, M.A.; Webster, R. Kriging: A method of interpolation for geographical information systems. Int. J. Geogr. Inf. Syst. 1990, 4, 313–332. [Google Scholar] [CrossRef]
- Li, X.; Cheng, G.; Lu, L. Comparison of spatial interpolation methods. Adv. Earth Sci. 2000, 3, 208. [Google Scholar]
- El-Sheimy, N.; Valeo, C.; Habib, A. Digital Terrain Modeling: Acquisition, Manipulation, and Applications; Artech House: Boston, MA, USA, 2005. [Google Scholar]
- Yilmaz, H.M. The effect of interpolation methods in surface definition: An experimental study. Earth Surf. Process. Landf. J. Br. Geomorphol. Res. Group 2007, 32, 1346–1361. [Google Scholar] [CrossRef]
- Wyrzykowski, T. Map of Recent Absolute Velocities of Vertical Movements the Earth’s Crust Surface on the Territory of Poland 1:2,500,000; Instytut Geodezji i Kartografii: Warszawa, Poland, 1971. [Google Scholar]
- Wyrzykowski, T. New Determination of the Speed of Modern Vertical Movements of the Earth’s Crust Surface in Poland; Instytut Geodezji i Kartografii: Warszawa, Poland, 1987; Volume 34, pp. 41–64. [Google Scholar]
- Kowalczyk, K. Determination of land uplift in the area of Poland. In Proceedings of the 6th International Conference Environmental Engineering, Vilnius, Lithuania, 26–27 May 2005; pp. 903–907. [Google Scholar]
- Kontny, B.; Bogusz, J. Models of vertical movements of the Earth crust surface in the area of Poland derived from leveling and GNSS data. Acta Geodyn. Geomater. 2012, 9, 331–337. [Google Scholar]
- Kowalczyk, K. The creation of a model of relative vertical crustal movement In the Polish territory on the basis of the data from Active Geodetic Network EUPOS (ASG EUPOS). Acta Geodyn. Geomater. 2015, 12, 215–225. [Google Scholar] [CrossRef]
- Maroufpoor, S.; Bozorg-Haddad, O.; Chu, X. Geostatistics: Principles and methods. In Handbook of Probabilistic Models; Samui, P., Bui, D.T., Chakraborty, S., Deo, R.C., Eds.; Butterworth-Heinemann: Oxford, UK, 2020; Chapter 9; pp. 229–242. [Google Scholar]
- Atkinson, P.M.; Lloyd, C.D. Geostatistical Models and Spatial Interpolation. In Handbook of Regional Science; Fischer, M.M., Nijkamp, P., Eds.; Springer: Berlin/Heidelberg, Germany, 2019; pp. 1–15. [Google Scholar] [CrossRef]
- Pajak, K.; Blaszczak-Bak, W.; Sobieraj, A. Evaluation of the semivariogram selection on the kriging interpolation. In Proceedings of the International Multidisciplinary Scientific GeoConference Surveying Geology and Mining Ecology Management, SGEM, Albena, Bulgaria, 18–24 June 2015; Volume 2, pp. 235–246. [Google Scholar]
- Urbański, J. “Gis in Enviroinmental Sciences (GIS w Badaniach Przyrodniczych)”; Centrum GIS, Uniwersity of Gdansk: Gdansk, Poland, 2008; pp. 230–232. ISBN 978-83-7326-516-5. [Google Scholar]
- Kowalczyk, K.; Rapiński, J. Evaluation of levelling data for use in vertical crustal movements model in Poland. Acta Geodyn. Geomater. 2013, 10, 401–410. [Google Scholar] [CrossRef][Green Version]
- Watson, C.; Tregoning, P.; Coleman, R. Impact of solid Earth tide models on GPS coordinate and tropospheric time series. Geophys. Res. Lett. 2006, 33. [Google Scholar] [CrossRef]




| Authors | Data | Type | Type of Data Processing | Form Maps (Models) | Interpolation | Determination of Anisotropy | Additional Isoline Corrections |
|---|---|---|---|---|---|---|---|
| [25] | Precise leveling | Point | Adjustment | Analog | Linear | No | Geological |
| [26] | Precise leveling | Point | Adjustment | Analog | Linear | No | Geological |
| [27] | Precise leveling | Point | Adjustment | Analog, numerical (grid: 20′ × 20′) | Collocation using the Hirvonen analytical function | No | No |
| [28] | Data from GNSS stations | Point | Development of time series | Analog | Kriging method with the linear semivariogram | No data | No |
| [29] | Data from GNSS stations | Point | Development of time series, adjustment | Analog | Kriging method with the linear semivariogram | No | No |
| Data Set | Leveling Data | GNSS Stations Data | Number of Nodal Points | Number of Leveling Campaing |
|---|---|---|---|---|
| A | + | − | 98 | #2, #3 |
| B | + | − | 222 | #3, #4 |
| C | + | − | 228 | #2, #3, #4 |
| D | − | + | 123 | − |
| E | + | + | 345 | #3, #4 |
| Data Collections | Nugget Efect | Anisotropy | Direction | Partial Sill | |||
|---|---|---|---|---|---|---|---|
| [1][0] | [1][1] | [1][0] | [0][1] | [1][1] | |||
| A | 0.01 | 2.44 | 10.01 | 0.39 | |||
| B | 0.05 | 1.83 | 142.73 | 0.21 | |||
| C | 0.11 | 1.65 | 139.74 | 0.17 | |||
| D | 0.59 | 1 | 0 | 0 | |||
| AB | 0 | 0.24 | 1.64 | 21.79 | 0.44 | −0.01 | 0.0003 |
| BD | 0.23 | 0.59 | 1 | 0 | 0 | 0 | 0 |
| DB | 0.60 | 0.16 | 1 | 0 | 0 | 0 | 0 |
| E | 0.26 | 2.46 | 146.60 | brak | brak | 0.28 | |
| A | B | C | D | E | F | G | AB | BA | BD | DB | |
|---|---|---|---|---|---|---|---|---|---|---|---|
| Mean standard error | 0.69 | 0.85 | 1.60 | 4.66 | 1.23 | 2.31 | 2.05 | 2.11 | 0.94 | 1.35 | 4.71 |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Kowalczyk, K.; Kowalczyk, A.M.; Chojka, A. Modeling of the Vertical Movements of the Earth’s Crust in Poland with the Co-Kriging Method Based on Various Sources of Data. Appl. Sci. 2020, 10, 3004. https://doi.org/10.3390/app10093004
Kowalczyk K, Kowalczyk AM, Chojka A. Modeling of the Vertical Movements of the Earth’s Crust in Poland with the Co-Kriging Method Based on Various Sources of Data. Applied Sciences. 2020; 10(9):3004. https://doi.org/10.3390/app10093004
Chicago/Turabian StyleKowalczyk, Kamil, Anna Maria Kowalczyk, and Agnieszka Chojka. 2020. "Modeling of the Vertical Movements of the Earth’s Crust in Poland with the Co-Kriging Method Based on Various Sources of Data" Applied Sciences 10, no. 9: 3004. https://doi.org/10.3390/app10093004
APA StyleKowalczyk, K., Kowalczyk, A. M., & Chojka, A. (2020). Modeling of the Vertical Movements of the Earth’s Crust in Poland with the Co-Kriging Method Based on Various Sources of Data. Applied Sciences, 10(9), 3004. https://doi.org/10.3390/app10093004






