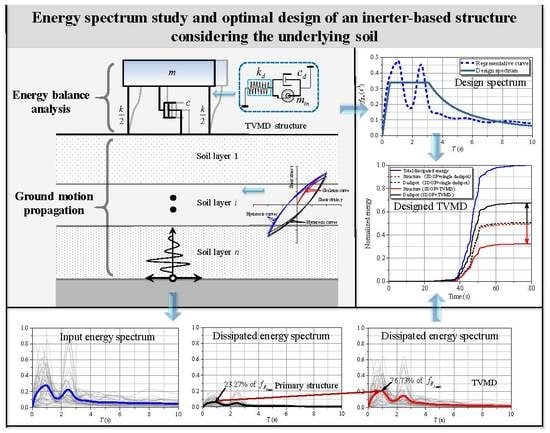
Energy Spectrum Study and Optimal Design of an Inerter-Based Structure Considering the Underlying Soil
Abstract
1. Introduction
2. Theoretical Analysis of Energy Spectrum Study
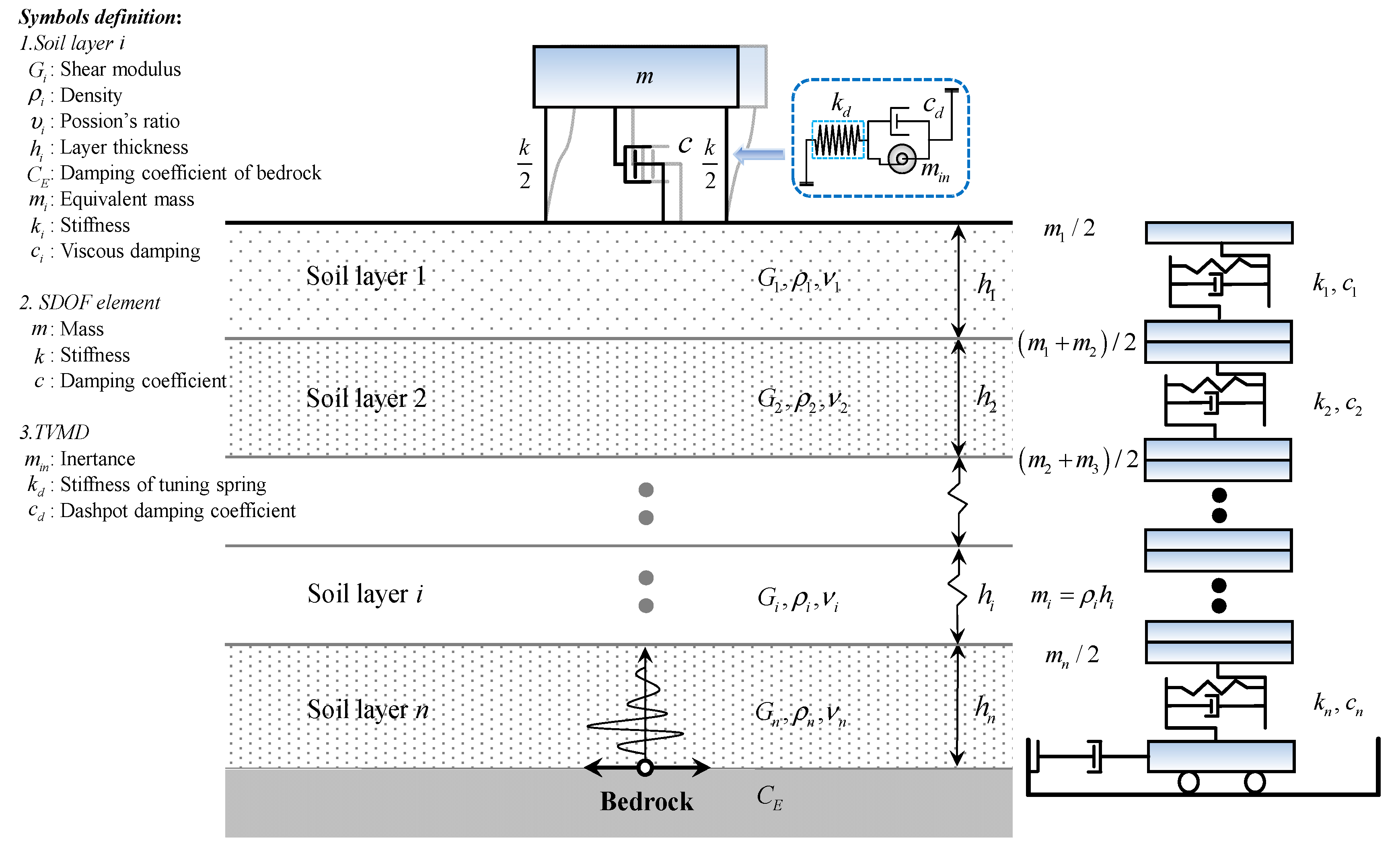
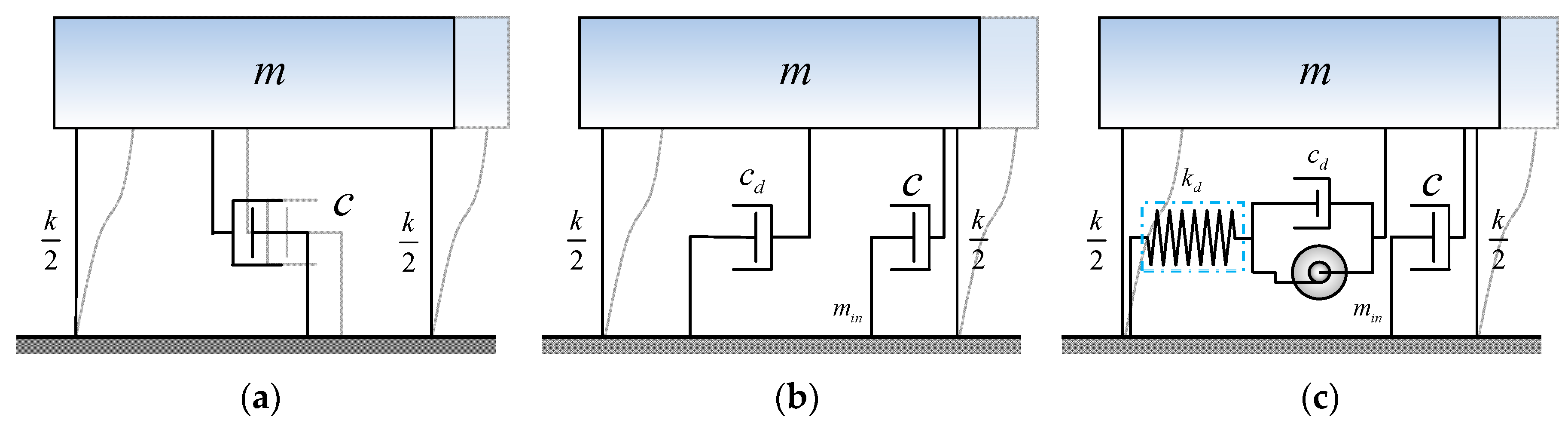
2.1. Introduction of SDOF-TVMD System and Underlying Soil
2.2. Ground Motion Propagation of the Soil
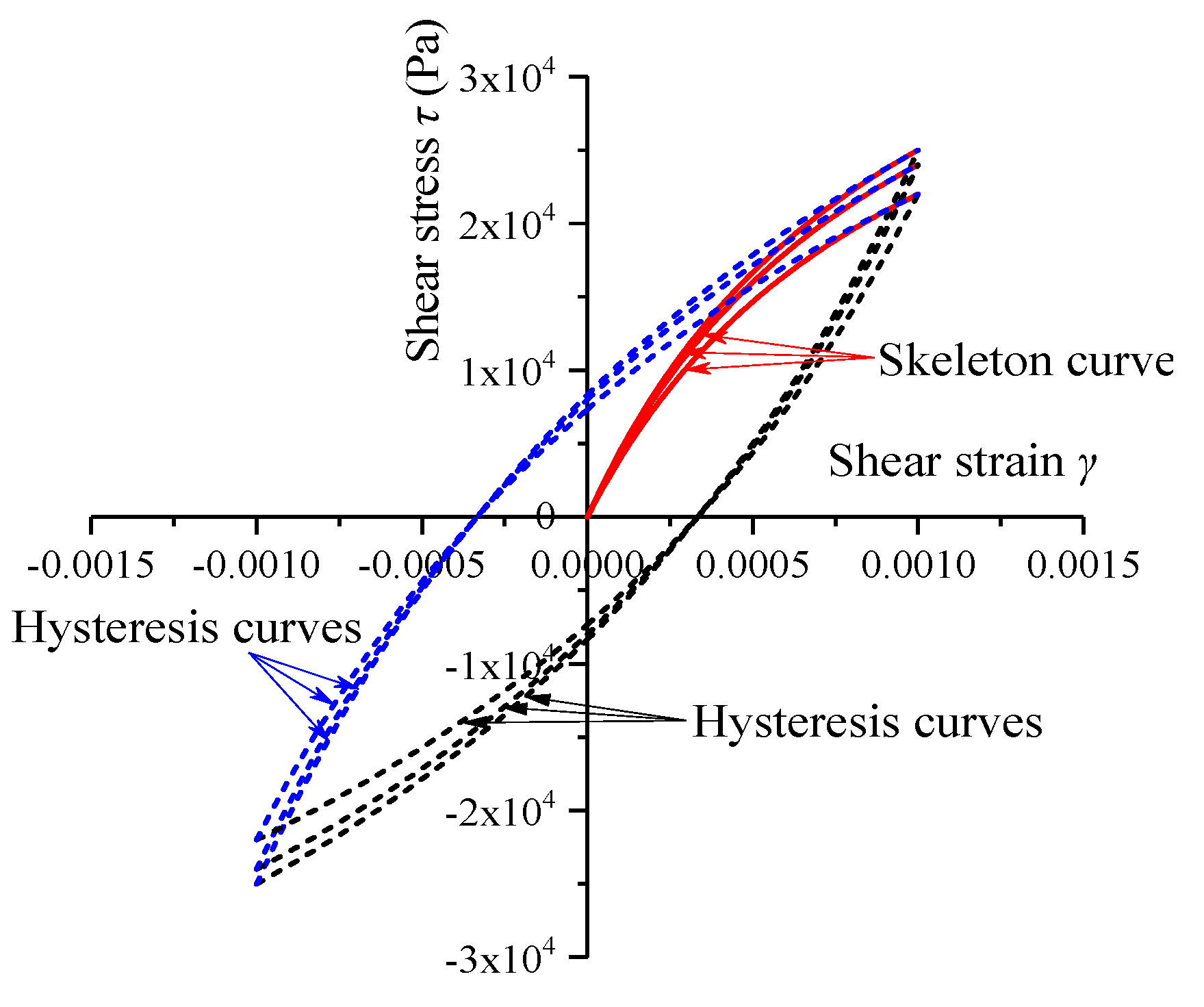
2.2.1. Davidenkov Model of Soil
2.2.2. Equation of Ground Motion Propagation
2.3. Energy Balance Analysis
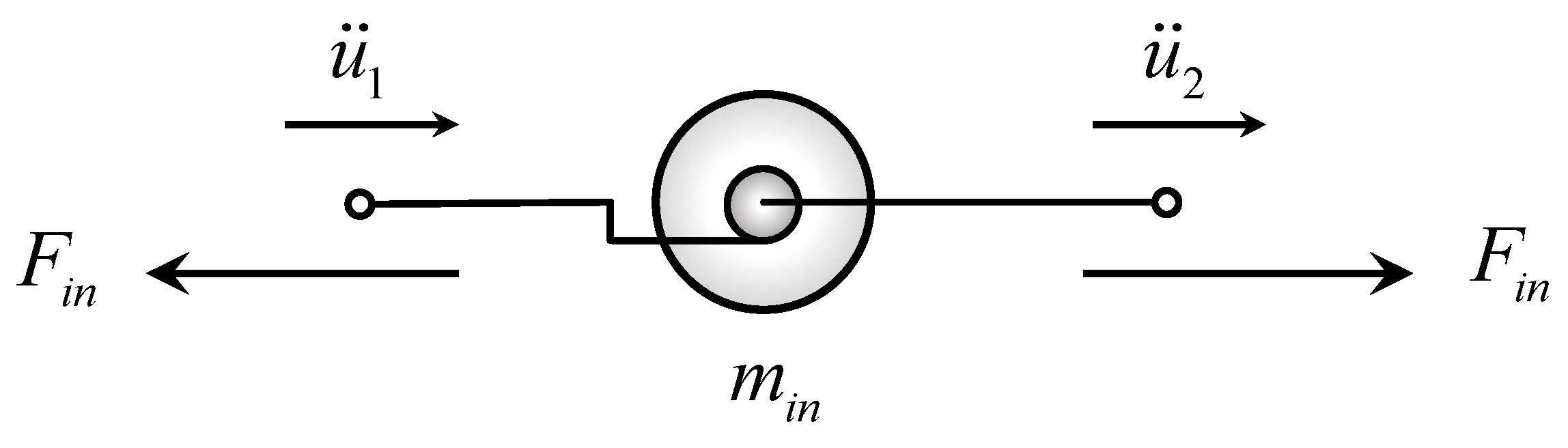
2.3.1. Introduction of the Inerter
2.3.2. Governing Equations
2.3.3. Energy Balance Analysis
3. Ground Motion Propagation of Nonlinear Soil
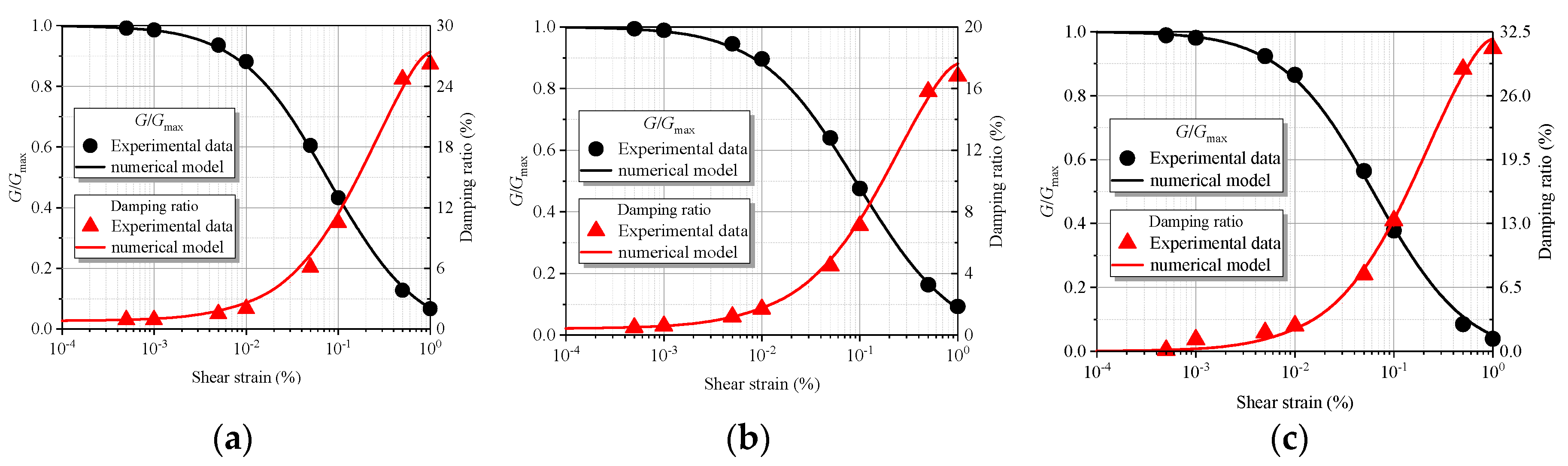
3.1. Fitting Parameters and Implementation of Davidenkov Model in ABAQUS
3.1.1. Fitting Parameters of Soil
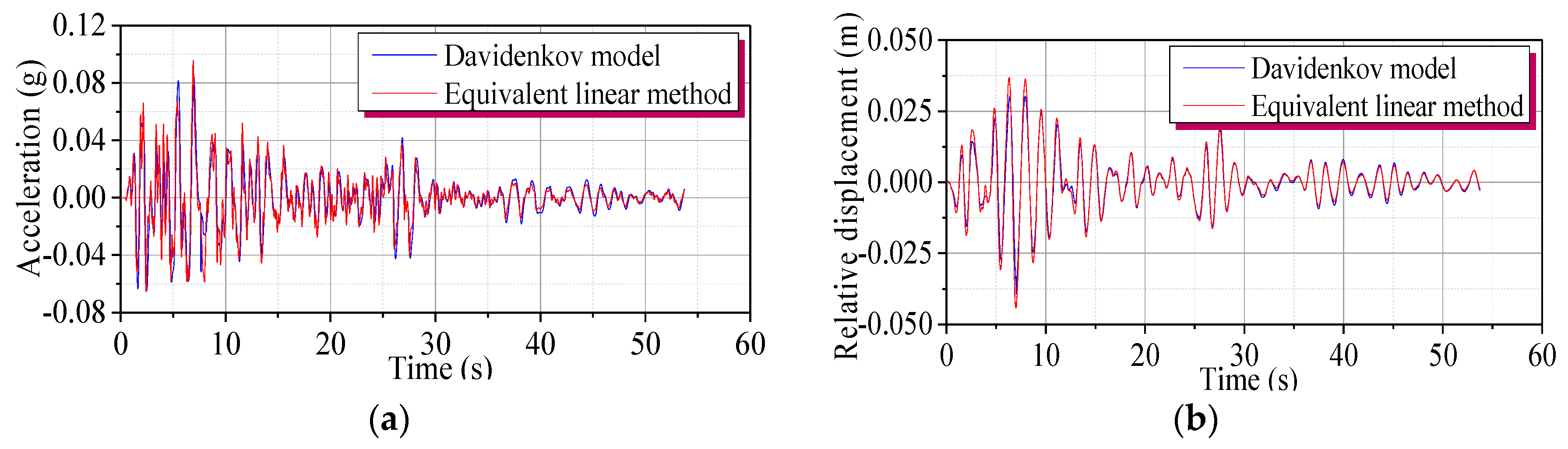
3.1.2. Justification of the Davidenkov Model in Ground Motion Propagation
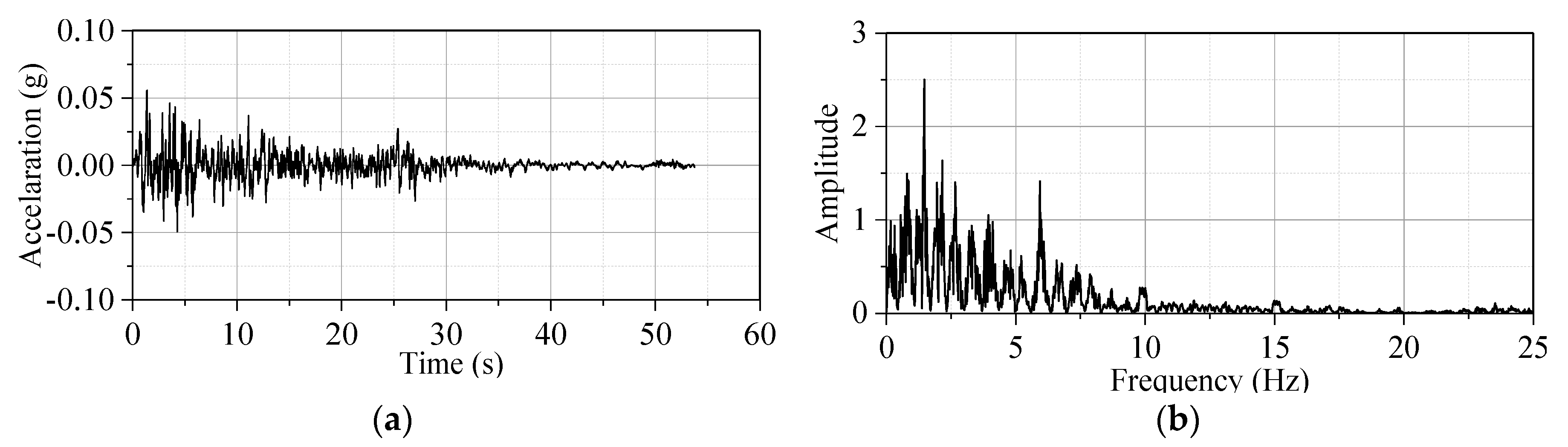
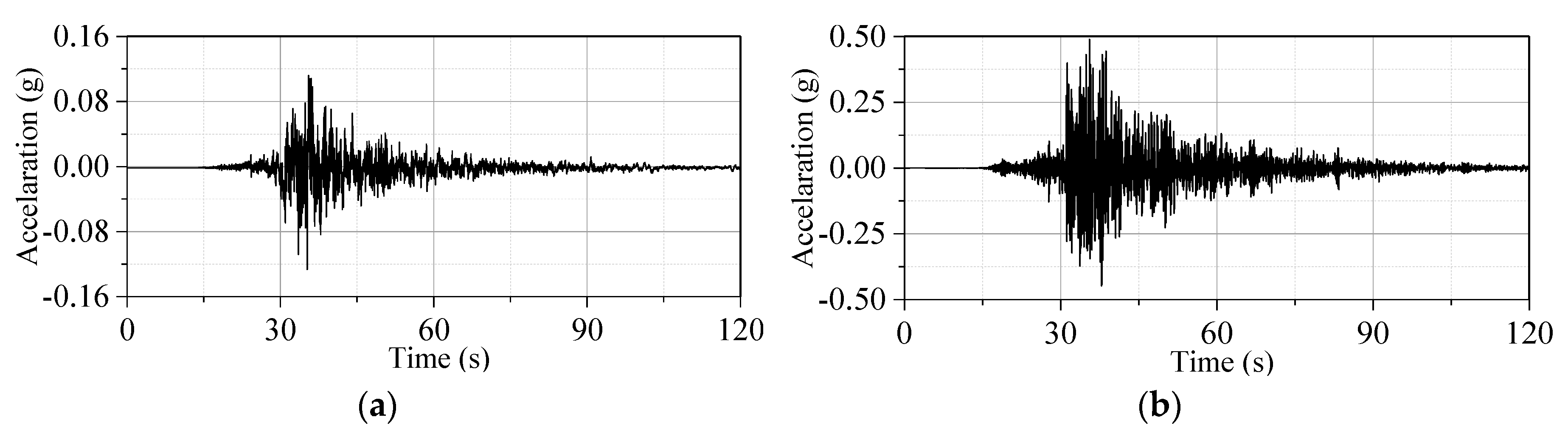
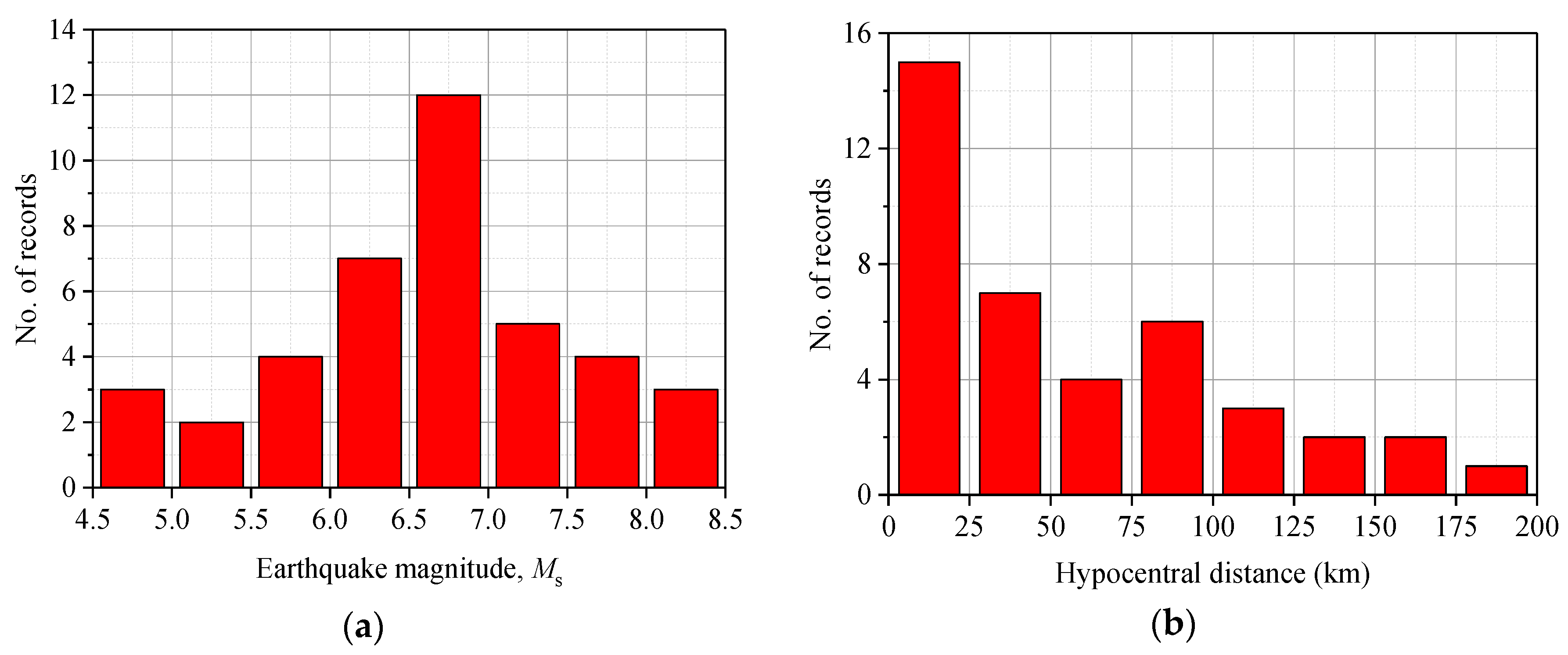
3.2. Selection of Typical Soft Deposit and Ground Motion Records
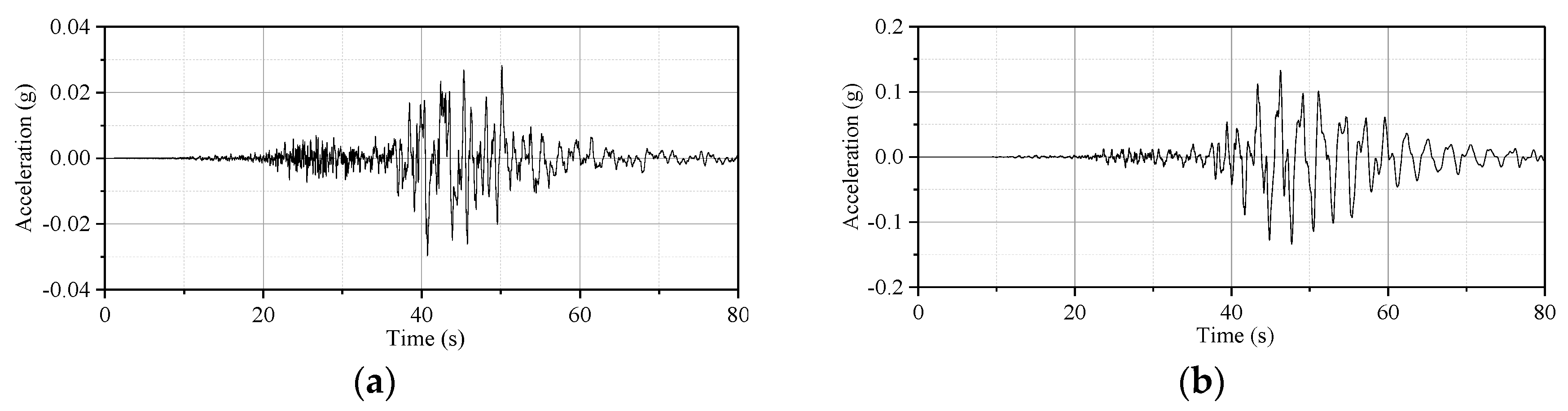
3.3. Ground Motion Propagation for Deep Cover Soft Deposit
4. Energy Spectra for Damped and Inerter-Based Structures
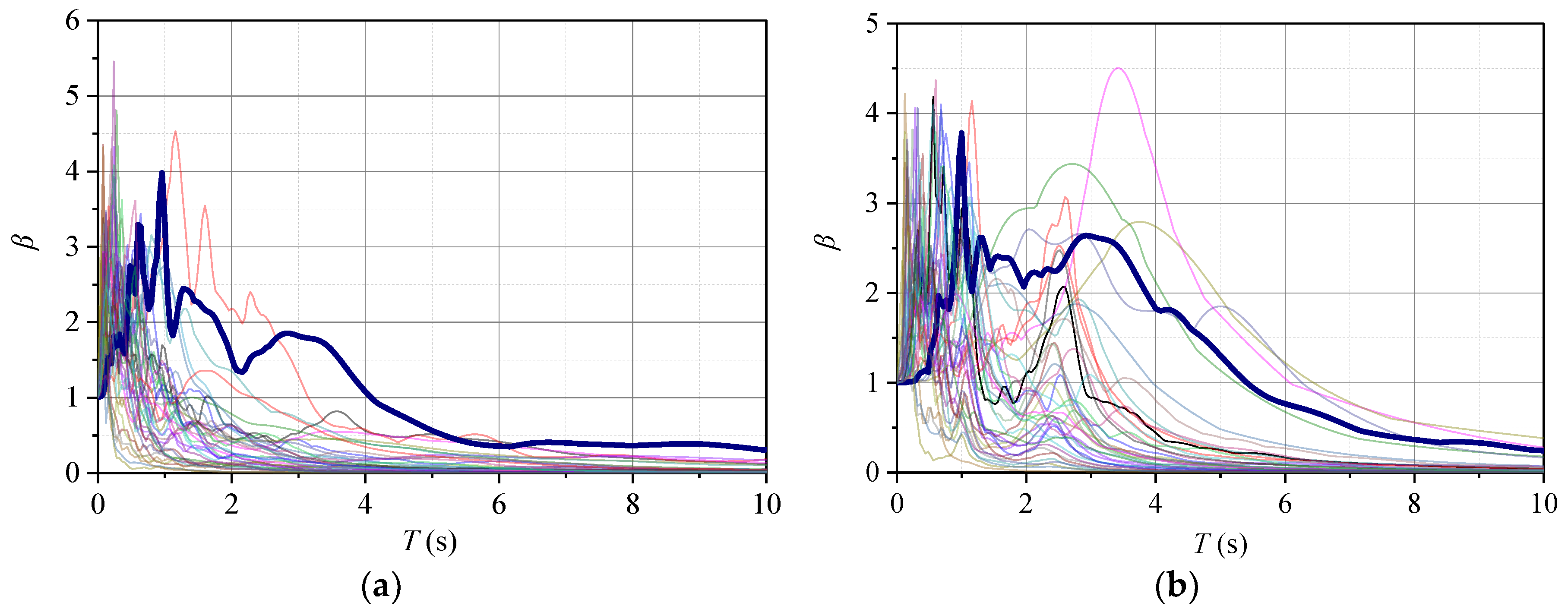
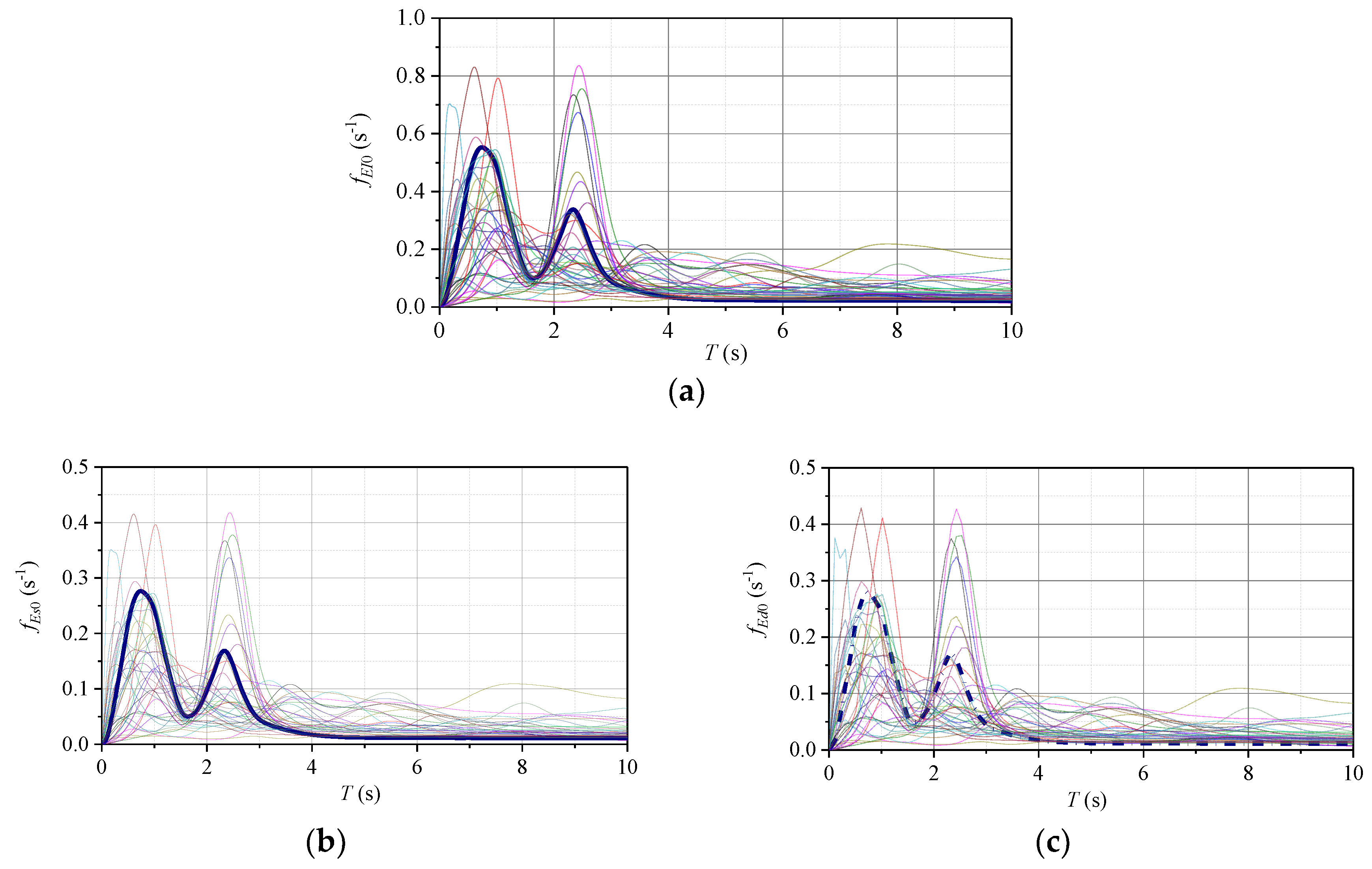
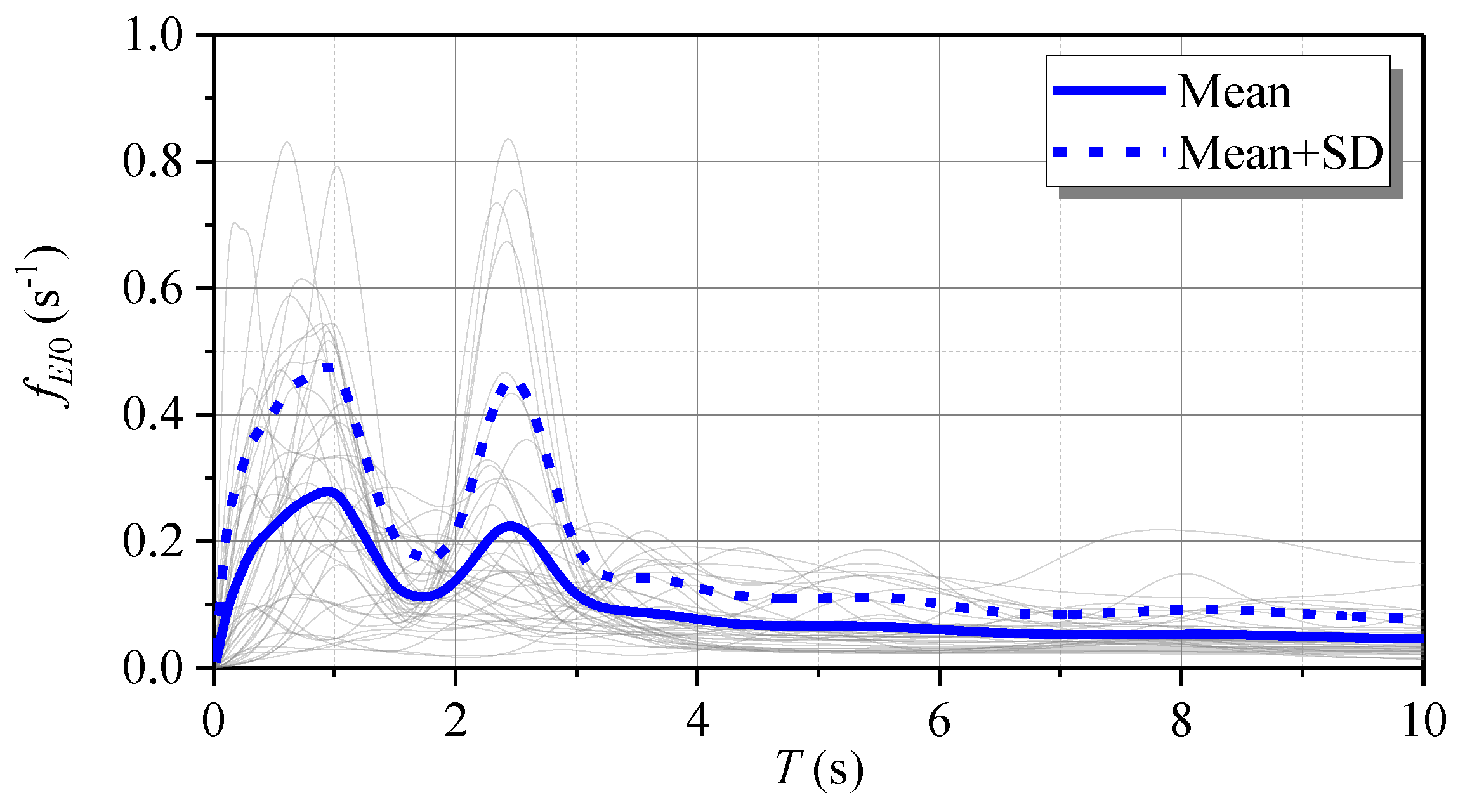
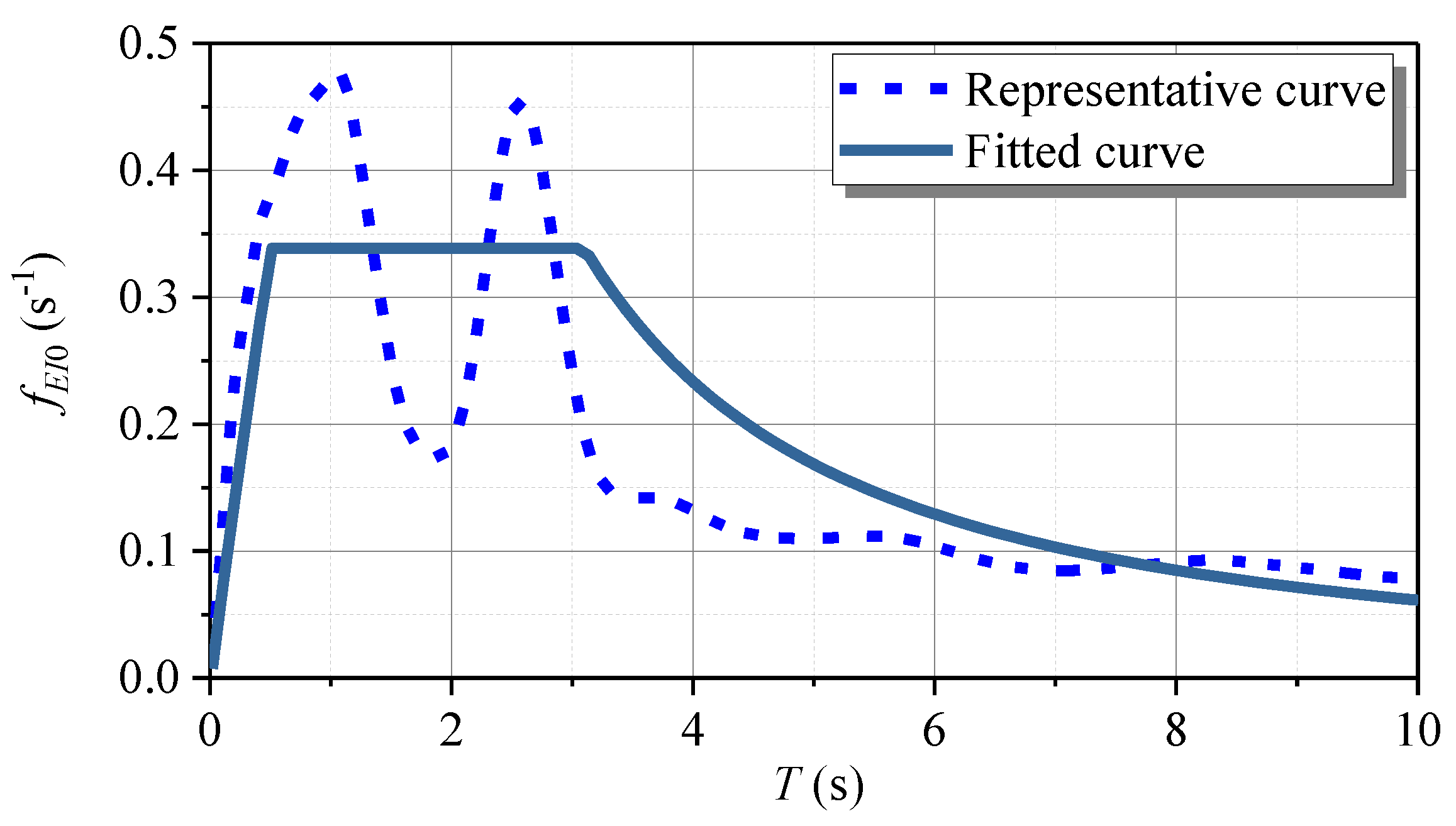
4.1. Energy Spectrum for Damped SDOF Element
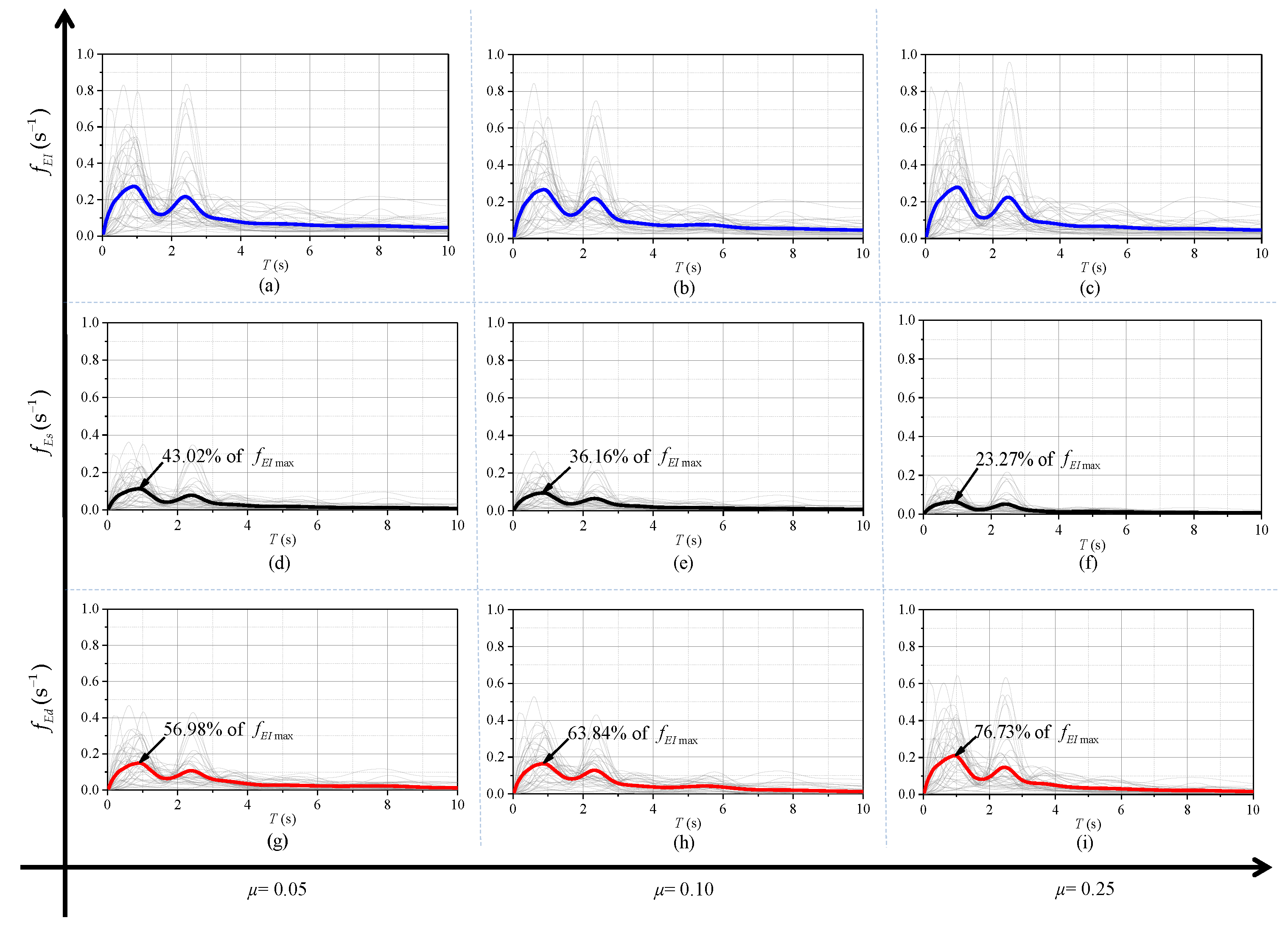
4.2. Energy Spectrum for Inerter-Based Structure
4.3. Optimal Design and Design Cases
5. Conclusions
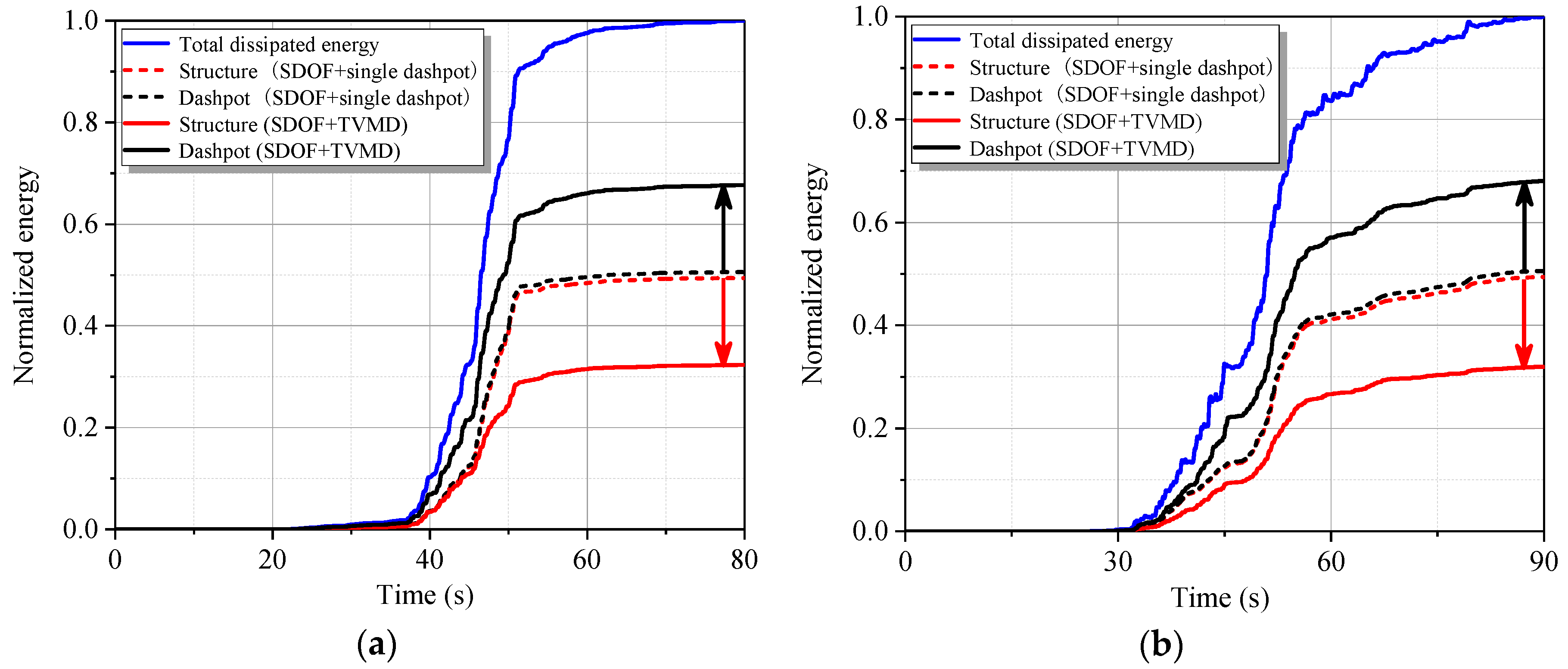
- The developed energy spectrum analysis framework effectively quantifies the degree of the energy dissipation enhancement effect of the TVMD. Compared with a single dashpot with the same damping coefficient, the TVMD exhibits improved efficiency for energy dissipation, with less energy required for the primary structure to dissipate.
- Based on the revealed energy characteristic of the inerter system, the optimal design strategy can guarantee the target demand of displacement control, which optimizes the efficiency and quantity of the TVMD for energy dissipation, reducing the energy dissipation burden on the primary structure.
- Taking the effect of the underlying soil into consideration, especially deep cover soft deposits, a fitted seismic input energy spectrum is provided for the inerter-based structure, which facilitates the optimal energy-based design of the TVMD.
- For an inerter-based structure on a deep cover soft deposit, nonlinear ground motion propagation should be quantitatively considered for energy analysis and optimal design. After considering the underlying soil, the input energy and dissipated energy responses are dominant and large for long-period inerter-based structures (), owing to the effects of the underlying soil.
Author Contributions
Funding
Conflicts of Interest
References
- Takewaki, I.; Murakami, S.; Yoshitomi, S.; Tsuji, M. Fundamental mechanism of earthquake response reduction in building structures with inertial dampers. Struct. Control Health Monit. 2012, 19, 590–608. [Google Scholar] [CrossRef]
- Zhang, R.F.; Zhao, Z.P.; Dai, K. Seismic response mitigation of a wind turbine tower using a tuned parallel inerter mass system. Eng. Struct. 2019, 180, 29–39. [Google Scholar] [CrossRef]
- Masri, S.F.; Caffrey, J.P. Transient response of a sdof system with an inerter to nonstationary stochastic excitation. J. Appl. Mech. Trans. Asme 2017, 84, 041005. [Google Scholar] [CrossRef]
- Jiang, Y.Y.; Zhao, Z.P.; Zhang, R.F.; De Domenico, D.; Pan, C. Optimal design based on analytical solution for storage tank with inerter isolation system. Soil Dyn. Earthq. Eng. 2020, 129, 105924. [Google Scholar] [CrossRef]
- Barredo, E.; Blanco, A.; Colín, J.; Penagos, V.M.; Abúndez, A.; Vela, L.G.; Meza, V.; Cruz, R.H.; Mayén, J. Closed-form solutions for the optimal design of inerter-based dynamic vibration absorbers. Int. J. Mech. Sci. 2018, 144, 41–53. [Google Scholar] [CrossRef]
- Chen, Q.J.; Zhao, Z.P.; Zhang, R.F.; Pan, C. Impact of soil–structure interaction on structures with inerter system. J. Sound Vib. 2018, 433, 1–15. [Google Scholar] [CrossRef]
- Zhang, R.F.; Zhao, Z.P.; Pan, C. Influence of mechanical layout of inerter systems on seismic mitigation of storage tanks. Soil Dyn. Earthq. Eng. 2018, 114, 639–649. [Google Scholar] [CrossRef]
- Ikago, K.; Saito, K.; Inoue, N. Seismic control of single-degree-of-freedom structure using tuned viscous mass damper. Earthq. Eng. Struct. Dyn. 2012, 41, 453–474. [Google Scholar] [CrossRef]
- Arakaki, T.; Kuroda, H.; Arima, F.; Inoue, Y.; Baba, K. Development of seismic devices applied to ball screw: Part 1 Basic performance test of RD-series. J. Archit. Build. Sci. 1999, 5, 239–244. [Google Scholar] [CrossRef]
- De Domenico, D.; Deastra, P.; Ricciardi, G.; Sims, N.D.; Wagg, D.J. Novel fluid inerter based tuned mass dampers for optimised structural control of base-isolated buildings. J. Frankl. Inst. 2019, 356, 7626–7649. [Google Scholar] [CrossRef]
- Kawamata, S. Development of a Vibration Control System of Structures by Means of Mass Pumps; Institute of Industrial Science, University of Tokyo: Tokyo, Japan, 1973. [Google Scholar]
- Smith, M.C. Synthesis of mechanical networks: The inerter. IEEE Trans. Autom. Control 2002, 47, 1648–1662. [Google Scholar] [CrossRef]
- Gonzalez-Buelga, A.; Clare, L.R.; Neild, S.A.; Jiang, J.Z.; Inman, D.J. An electromagnetic inerter-based vibration suppression device. Smart Mater. Struct. 2015, 24, 055015. [Google Scholar] [CrossRef]
- Lazar, I.F.; Neild, S.A.; Wagg, D.J. Using an inerter-based device for structural vibration suppression. Earthq. Eng. Struct. Dyn. 2014, 43, 1129–1147. [Google Scholar] [CrossRef]
- Zhao, Z.P.; Zhang, R.F.; Lu, Z. A particle inerter system for structural seismic response mitigation. J. Frankl. Inst. 2019, 356, 7669–7688. [Google Scholar] [CrossRef]
- Zhao, Z.P.; Zhang, R.F.; Jiang, Y.Y.; Pan, C. A tuned liquid inerter system for vibration control. Int. J. Mech. Sci. 2019, 164, 105171. [Google Scholar] [CrossRef]
- Chen, Q.J.; Zhao, Z.P.; Xia, Y.Y.; Pan, C.; Luo, H.; Zhang, R.F. Comfort based floor design employing tuned inerter mass system. J. Sound Vib. 2019, 458, 143–157. [Google Scholar] [CrossRef]
- Javidialesaadi, A.; Wierschem, N.E. Optimal design of rotational inertial double tuned mass dampers under random excitation. Eng. Struct. 2018, 165, 412–421. [Google Scholar] [CrossRef]
- Zhang, R.F.; Zhao, Z.P.; Pan, C.; Ikago, K.; Xue, S.T. Damping enhancement principle of inerter system. Struct. Control Health Monit. 2020. [Google Scholar] [CrossRef]
- Pan, C.; Zhang, R.F.; Luo, H.; Li, C.; Shen, H. Demand-based optimal design of oscillator with parallel-layout viscous inerter damper. Struct. Control Health Monit. 2018, 25, e2051. [Google Scholar] [CrossRef]
- Zhao, Z.P.; Zhang, R.F.; Jiang, Y.Y.; Pan, C. Seismic response mitigation of structures with a friction pendulum inerter system. Eng. Struct. 2019, 193, 110–120. [Google Scholar] [CrossRef]
- Zhao, Z.P.; Chen, Q.J.; Zhang, R.F.; Pan, C.; Jiang, Y.Y. Optimal design of an inerter isolation system considering the soil condition. Eng. Struct. 2019, 196, 109324. [Google Scholar] [CrossRef]
- Decanini, L.D.; Mollaioli, F. Formulation of elastic earthquake input energy spectra. Earthq. Eng. Struct. Dyn. 1998, 27, 1503–1522. [Google Scholar] [CrossRef]
- Bagheri, B.; Oh, S.H. Seismic design of coupled shear wall building linked by hysteretic dampers using energy based seismic design. Int. J. Steel Struct. 2018, 18, 225–253. [Google Scholar] [CrossRef]
- Benavent-Climent, A.; Lopez-Almansa, F.; Bravo-Gonzalez, D.A. Design energy input spectra for moderate-to-high seismicity regions based on Colombian earthquakes. Soil Dyn. Earthq. Eng. 2010, 30, 1129–1148. [Google Scholar] [CrossRef]
- Reggio, A.; De Angelis, M. Optimal energy-based seismic design of non-conventional Tuned Mass Damper (TMD) implemented via inter-story isolation. Earthq. Eng. Struct. Dyn. 2015, 44, 1623–1642. [Google Scholar] [CrossRef]
- Tan, J.; Jiang, J.; Liu, M.; Feng, Q.; Zhang, P.; Ho, S.C.M. Implementation of shape memory alloy sponge as energy dissipating material on pounding tuned mass damper: An experimental investigation. Appl. Sci. Basel 2019, 9, 1079. [Google Scholar] [CrossRef]
- Benavent-Climent, A.; Escobedo, A.; Donaire-Avila, J.; Oliver-Saiz, E.; Ramirez-Marquez, A.L. Assessment of expected damage on buildings subjected to Lorca earthquake through an energy-based seismic index method and nonlinear dynamic response analyses. Bull. Earthq. Eng. 2014, 12, 2049–2073. [Google Scholar] [CrossRef]
- Benavent-Climent, A.; Mota-Paez, S. Earthquake retrofitting of R/C frames with soft first story using hysteretic dampers: Energy-based design method and evaluation. Eng. Struct. 2017, 137, 19–32. [Google Scholar] [CrossRef]
- Benavent-Climent, A. An energy-based method for seismic retrofit of existing frames using hysteretic dampers. Soil Dyn. Earthq. Eng. 2011, 31, 1385–1396. [Google Scholar] [CrossRef]
- Alici, F.S.; Sucuoglu, H. Elastic and inelastic near-fault input energy spectra. Earthq. Spectra 2018, 34, 611–637. [Google Scholar] [CrossRef]
- Gullu, A.; Yuksel, E.; Yalcin, C.; Dindar, A.A.; Ozkaynak, H.; Buyukozturk, O. An improved input energy spectrum verified by the shake table tests. Earthq. Eng. Struct. Dyn. 2019, 48, 27–45. [Google Scholar] [CrossRef]
- Schnabel, P.B.; Lysmer, J.; Seed, H.B. SHAKE: A Computer Program for Earthquake Response Analysis of Horizontally Layered Sites, Report No. UCB/EERC-72/12; Earthquake Engineering Research Center, University of California: Berkeley, CA, USA, 1972. [Google Scholar]
- Wang, Z.L.; Dafalias, Y.F.; Shen, C.K. Bounding surface hypoplasticity model for sand. J. Eng. Mech. 1990, 116, 983–1001. [Google Scholar] [CrossRef]
- Martin, P.P.; Seed, H.B. One-dimensional dynamic ground response analyses. J. Geotech. Eng. Div. Asce 1982, 108, 935–952. [Google Scholar] [CrossRef]
- Srbulov, M. Geotechnical Earthquake Engineering: Simplified Analyses with Case Studies and Examples; Springer Science & Business Media: Berlin/Heidelberg, Germany, 2008. [Google Scholar]
- Jabary, R.; Madabhushi, S. Structure-soil-structure interaction effects on structures retrofitted with tuned mass dampers. Soil Dyn. Earthq. Eng. 2017, 100, 301–315. [Google Scholar] [CrossRef]
- Wu, J.; Chen, G.; Lou, M. Seismic effectiveness of tuned mass dampers considering soil–structure interaction. Earthq. Eng. Struct. Dyn. 1999, 28, 1219–1233. [Google Scholar] [CrossRef]
- Hashash, Y.M.A.; Park, D. Non-linear one-dimensional seismic ground motion propagation in the Mississippi embayment. Eng. Geol. 2001, 62, 185–206. [Google Scholar] [CrossRef]
- Ge, Q.; Xiong, F.; Xie, L.; Chen, J.; Yu, M. Dynamic interaction of soil-Structure cluster. Soil Dyn. Earthq. Eng. 2019, 123, 16–30. [Google Scholar] [CrossRef]
- Chong, S.H. Soil dynamic constitutive model for characterizing the nonlinear-hysteretic response. Appl. Sci. Basel 2017, 7, 1110. [Google Scholar] [CrossRef]
- Uang, C.M.; Bertero, V.V. Evaluation of seismic energy in structures. Earthq. Eng. Struct. Dyn. 1990, 19, 77–90. [Google Scholar] [CrossRef]
- Chapman, M.C. On the use of elastic input energy for seismic hazard analysis. Earthq. Spectra 1999, 15, 607–635. [Google Scholar] [CrossRef]
- Ordaz, M.; Huerta, B.; Reinoso, E. Exact computation of input-energy spectra from Fourier amplitude spectra. Earthq. Eng. Struct. Dyn. 2003, 32, 597–605. [Google Scholar] [CrossRef]
- Wang, Y.C.; Chen, Q.J. Parametric fitting of soft soil sites’ Davidenkov model based on PSO algorithm and its application. J. Vib. Shock 2019, 38, 8–16. [Google Scholar]
- Yang, L.D.; Wang, G.B.; Liu, Q.J.; Lei, G. A study on the dynamic properties of soft soil in shanghai. In Proceedings of the Soil and Rock Behavior and Modeling, Shanghai, China, 6 June 2006; pp. 466–473. [Google Scholar]
- Lanzo, G.; Vucetic, M. Effect of soil plasticity on damping ratio at small cyclic strains. Soils Found. 1999, 39, 131–141. [Google Scholar] [CrossRef]
- Clough, R.W.; Penzien, J. Dymnics of Structures; McCraw-Hill: New York, NY, USA, 1975. [Google Scholar]
- ABAQUS. Theory and Analysis User’s Manual Version 6.12; Dassault Systems SIMULIA Corp: Providence, RI, USA, 2012. [Google Scholar]
- Finn, W.D.; Lee, K.W.; Martin, G.R. An effective stress model for liquefaction. Am. Soc. Civ. Eng. J. Geotech. Eng. Div. 1977, 103, 517–533. [Google Scholar]
- Carlton, B.; Tokimatsu, K. Comparison of equivalent linear and nonlinear site response analysis results and model to estimate maximum shear strain. Earthq. Spectra 2016, 32, 1867–1887. [Google Scholar] [CrossRef]
- Garini, E.; Gazetas, G.; Ziotopoulou, K. Inelastic soil amplification in three sites during the Tokachi-oki M-JMA 8.0 earthquake. Soil Dyn. Earthq. Eng. 2018, 110, 300–317. [Google Scholar] [CrossRef]
- Huang, Y.; Ye, W.; Chen, Z. Seismic response analysis of the deep saturated soil deposits in Shanghai. Environ. Geol. 2009, 56, 1163–1169. [Google Scholar] [CrossRef]
- University of California. Berkeley. PEER NGA-West2 Ground Motion Database. Available online: http://ngawest2.berkeley.edu/ (accessed on 8 January 2020).
- Trifunac, M.D.; Brady, A.G. Study on duration of strong earthquake ground motion. Bull. Seismol. Soc. Am. 1975, 65, 581–626. [Google Scholar]
- Zhou, Y.; Song, G.; Huang, S.; Wu, H. Input energy spectra for self-centering SDOF systems. Soil Dyn. Earthq. Eng. 2019, 121, 293–305. [Google Scholar] [CrossRef]
- Kim, J.; Kim, S. Performance-based seismic design of staggered truss frames with friction dampers. Thin Walled Struct. 2017, 111, 197–209. [Google Scholar] [CrossRef]
- Nabid, N.; Hajirasouliha, I.; Petkovski, M. Adaptive low computational cost optimisation method for performance-based seismic design of friction dampers. Eng. Struct. 2019, 198, 109549. [Google Scholar] [CrossRef]
- MATLAB Documentation Center, Optimization Toolbox, Constrained Optimization, Fmincon. Available online: http://www.mathworks.com/help/optim/ug/fmincon.html (accessed on 8 January 2020).



| Type | |||
|---|---|---|---|
| Clay | 0.91 | 0.52 | 0.00087 |
| Muddy silty clay | 0.78 | 0.58 | 0.00082 |
| Muddy clay | 0.71 | 0.61 | 0.00096 |
| Sand | 1.08 | 0.47 | 0.00081 |
| Silty clay | 1.07 | 0.46 | 0.00071 |
| Record | Peak Ground Acceleration Response (g) | Peak Ground Relative Displacement Response (m) | ||||
|---|---|---|---|---|---|---|
| Davidenkov Model | Equivalent Linear Method | Relative Difference | Davidenkov Model | Equivalent Linear Method | Relative Difference | |
| El centro | 0.083 | 0.095 | 14.46% | 0.039 | 0.044 | 12.82% |
| Michoacan, Mexico VILE | 0.217 | 0.263 | 21.20% | 0.092 | 0.111 | 20.65% |
| Chi-Chi, Taiwan CHY102 | 0.127 | 0.148 | 16.54% | 0.059 | 0.067 | 13.56% |
| Kocaeli, Turkey Izmit | 0.168 | 0.216 | 28.57% | 0.421 | 0.544 | 29.22% |
| Iwate, Japan AKTH05 | 0.042 | 0.045 | 7.14% | 0.0041 | 0.0044 | 7.31% |
| Layer | Soil Type | Depth (m) | Thickness (m) | (kg·m−3) | ||||
|---|---|---|---|---|---|---|---|---|
| 1 | Clay | 0 | 3 | 1900 | 0.33 | 100 | 19.39 | 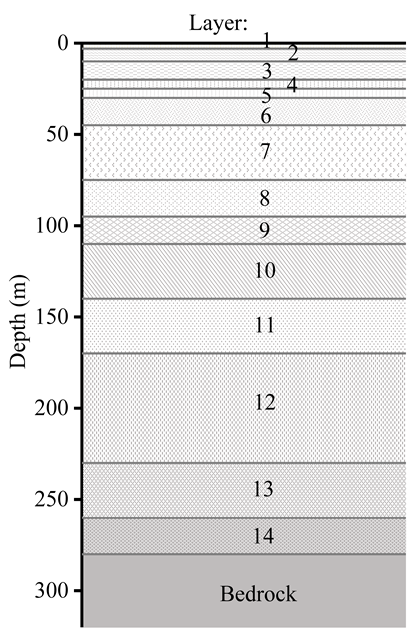 Geological profile in the urban area of Shanghai. |
| 2 | Muddy silty clay | 3 | 7 | 1750 | 0.35 | 130 | 30.18 | |
| 3 | Muddy clay | 10 | 10 | 1750 | 0.38 | 160 | 45.71 | |
| 4 | Clay | 20 | 5 | 1820 | 0.30 | 190 | 67.04 | |
| 5 | Clay | 25 | 5 | 2000 | 0.30 | 260 | 137.96 | |
| 6 | Sand | 30 | 15 | 1920 | 0.28 | 290 | 164.77 | |
| 7 | Silty clay | 45 | 30 | 1900 | 0.33 | 320 | 198.53 | |
| 8 | Sand | 75 | 20 | 1950 | 0.28 | 340 | 230.02 | |
| 9 | Clay | 95 | 15 | 2000 | 0.33 | 400 | 326.53 | |
| 10 | Sand | 110 | 30 | 2020 | 0.28 | 420 | 363.60 | |
| 11 | Clay | 140 | 30 | 2040 | 0.35 | 440 | 403.00 | |
| 12 | Sand | 170 | 60 | 2060 | 0.28 | 500 | 525.51 | |
| 13 | Clay | 230 | 30 | 2080 | 0.35 | 510 | 552.05 | |
| 14 | Sand | 260 | 20 | 2100 | 0.28 | 580 | 720.86 | |
| Bedrock | 280 | - | 2390 | 0.20 | 2000 | 9755.10 |
| No. | MS | Earthquake Events | Station | Component | PGA (g) | PGV (cm·s−1) | PGD (cm) | ||
|---|---|---|---|---|---|---|---|---|---|
| 1 | 8.1 | Michoacan, Mexico | PAPN | NS | 0.154 | 9.32 | 2.82 | 59.50 | 19.22 |
| 2 | 8.1 | Michoacan, Mexico | UNIO | EW | 0.144 | 14.01 | 4.05 | 62.74 | 26.44 |
| 3 | 8.1 | Michoacan, Mexico | VILE | EW | 0.113 | 11.38 | 3.86 | 64.00 | 42.09 |
| 4 | 7.6 | Chi-Chi, Taiwan | CHY102 | EW | 0.041 | 6.98 | 4.97 | 90.0 | 36.19 |
| 5 | 7.6 | Chi-Chi, Taiwan | KAU042 | EW | 0.011 | 3.43 | 2.23 | 67.0 | 32.83 |
| 6 | 7.6 | Chi-Chi, Taiwan | TAP077 | NS | 0.030 | 11.80 | 7.85 | 82.99 | 18.42 |
| 7 | 7.6 | Chi-Chi, Taiwan | TTN042 | NS | 0.057 | 7.73 | 3.62 | 100.0 | 34.14 |
| 8 | 7.5 | Kocaeli, Turkey | Gebze | 0° | 0.261 | 44.60 | 41.08 | 30.0 | 7.55 |
| 9 | 7.5 | Kocaeli, Turkey | Izmit | 90° | 0.230 | 38.28 | 24.30 | 30.0 | 13.27 |
| 10 | 7.4 | Tabas, Iran | Tabas | L1 | 0.854 | 98.76 | 38.04 | 32.98 | 16.54 |
| 11 | 7.3 | Landers | Lucerne | 260° | 0.725 | 97.55 | 70.50 | 48.12 | 13.15 |
| 12 | 7.2 | El Mayor-Cucapah, Mexico | El Monte County Park | 0° | 0.035 | 4.07 | 6.33 | 160.76 | 31.13 |
| 13 | 6.9 | Iwate, Japan | AKTH05 | NS | 0.010 | 0.47 | 0.98 | 262.00 | 49.01 |
| 14 | 6.9 | Iwate, Japan | MYGH03 | EW | 0.090 | 2.32 | 1.26 | 189.00 | 21.55 |
| 15 | 6.9 | Loma Prieta | Los Gatos, Lexington Dam | 0° | 0.443 | 85.69 | 17.33 | 81.91 | 4.33 |
| 16 | 6.9 | Loma Prieta | SF, Pacific Heights | 270° | 0.062 | 11.83 | 5.31 | 39.99 | 11.09 |
| 17 | 6.8 | Chuetsu-oki, Japan | NGN013 | NS | 0.016 | 1.07 | 0.48 | 84.00 | 26.55 |
| 18 | 6.7 | Northridge-01 | LA, Wonderland Ave | 185° | 0.159 | 14.64 | 2.26 | 29.98 | 6.67 |
| 19 | 6.7 | Northridge-01 | Vasquez Rocks Park | 90° | 0.139 | 11.07 | 2.88 | 39.98 | 7.34 |
| 20 | 6.6 | Niigata, Japan | TCGH17 | NS | 0.057 | 1.54 | 2.41 | 150.00 | 18.29 |
| 21 | 6.6 | San Fernando | Pasadena, Old Seismo Lab | 180° | 0.095 | 5.79 | 0.62 | 101.10 | 14.13 |
| 22 | 6.6 | Tottori, Japan | HYG007 | EW | 0.035 | 1.25 | 0.79 | 119.97 | 22.64 |
| 23 | 6.6 | Tottori, Japan | KOCH05 | EW | 0.057 | 1.54 | 0.90 | 149.99 | 24.51 |
| 24 | 6.6 | Tottori, Japan | SMNH10 | EW | 0.231 | 25.53 | 9.33 | 149.99 | 8.74 |
| 25 | 6.3 | Chi-Chi, Taiwan-06 | TAP086 | EW | 0.017 | 1.24 | 0.21 | 65.99 | 16.37 |
| 26 | 6.2 | Chi-Chi, Taiwan-04 | TTN042 | EW | 0.028 | 2.38 | 0.79 | 75.99 | 25.09 |
| 27 | 6.2 | Chi-Chi, Taiwan-04 | CHY102 | NS | 0.034 | 2.60 | 0.94 | 64.99 | 18.66 |
| 28 | 6.2 | Chi-Chi, Taiwan-05 | HWA003 | NS | 0.031 | 5.47 | 2.60 | 27.99 | 15.76 |
| 29 | 6.0 | Whittier Narrows-01 | LA, Wonderland Ave | 165° | 0.049 | 1.76 | 0.26 | 18.14 | 7.13 |
| 30 | 6.0 | Whittier Narrows-01 | Pasadena, CIT Kresge Lab | 360° | 0.095 | 3.74 | 0.33 | 39.99 | 6.25 |
| 31 | 6.0 | Parkfield-02, CA | Parkfield, Turkey Flat #1 (0M) | 270° | 0.245 | 14.61 | 1.46 | 81.92 | 8.76 |
| 32 | 5.9 | Chi-Chi, Taiwan-02 | ILA063 | NS | 0.012 | 0.80 | 0.27 | 52.99 | 23.63 |
| 33 | 5.9 | Chi-Chi, Taiwan-02 | HWA002 | NS | 0.022 | 4.19 | 0.70 | 64.99 | 22.20 |
| 34 | 5.7 | Coyote Lake | Gilroy Array #1 | 320° | 0.117 | 10.81 | 2.94 | 26.83 | 5.78 |
| 35 | 5.6 | Umbria-03, Italy | Gubbio | 90° | 0.068 | 3.55 | 0.52 | 19.36 | 6.78 |
| 36 | 5.3 | Whittier Narrows-02 | LA, Wonderland Ave | 165° | 0.016 | 0.76 | 0.05 | 12.01 | 6.13 |
| 37 | 5.1 | Hollister-03 | Gilroy Array #1 | 247° | 0.141 | 4.03 | 0.18 | 11.95 | 2.48 |
| 38 | 4.9 | Anza-02 | Idyllwild, Keenwild Fire Station | 180° | 0.033 | 0.45 | 0.05 | 45.99 | 8.07 |
| 39 | 4.9 | Anza-02 | Agave Hill | NS | 0.151 | 1.72 | 0.22 | 200.00 | 1.79 |
| 40 | 4.9 | Big Bear City | Belle Mountain Joshua Tree | EW | 0.015 | 0.54 | 0.06 | 200.00 | 9.08 |
| a | r | ||||
|---|---|---|---|---|---|
| 0.02 | 0.50 | 3.10 | 0.008 | 0.339 | 1.46 |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Chen, Q.; Wang, Y.; Zhao, Z. Energy Spectrum Study and Optimal Design of an Inerter-Based Structure Considering the Underlying Soil. Appl. Sci. 2020, 10, 2999. https://doi.org/10.3390/app10092999
Chen Q, Wang Y, Zhao Z. Energy Spectrum Study and Optimal Design of an Inerter-Based Structure Considering the Underlying Soil. Applied Sciences. 2020; 10(9):2999. https://doi.org/10.3390/app10092999
Chicago/Turabian StyleChen, Qingjun, Yanchao Wang, and Zhipeng Zhao. 2020. "Energy Spectrum Study and Optimal Design of an Inerter-Based Structure Considering the Underlying Soil" Applied Sciences 10, no. 9: 2999. https://doi.org/10.3390/app10092999
APA StyleChen, Q., Wang, Y., & Zhao, Z. (2020). Energy Spectrum Study and Optimal Design of an Inerter-Based Structure Considering the Underlying Soil. Applied Sciences, 10(9), 2999. https://doi.org/10.3390/app10092999