Fatigue Life Analysis of Ball Bearings and a Shaft System Considering the Combined Bearing Preload and Angular Misalignment
Abstract
1. Introduction
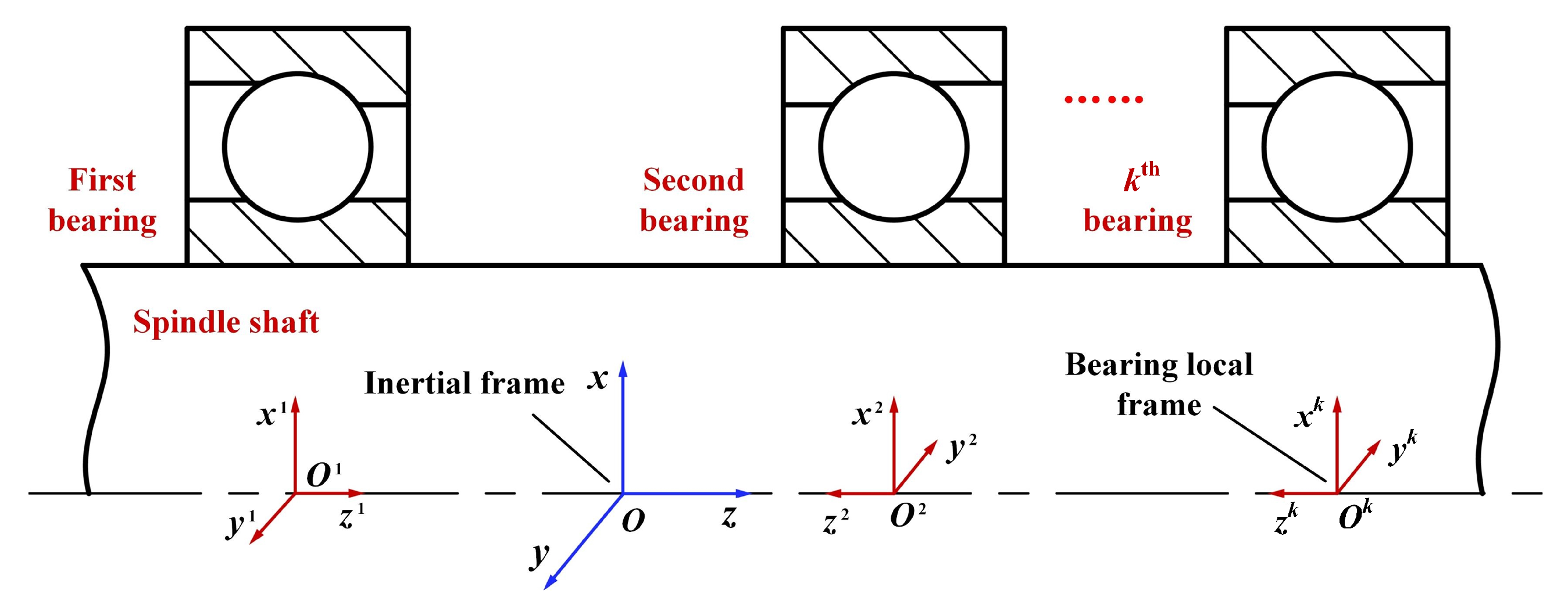
2. Shaft-Bearing System Model
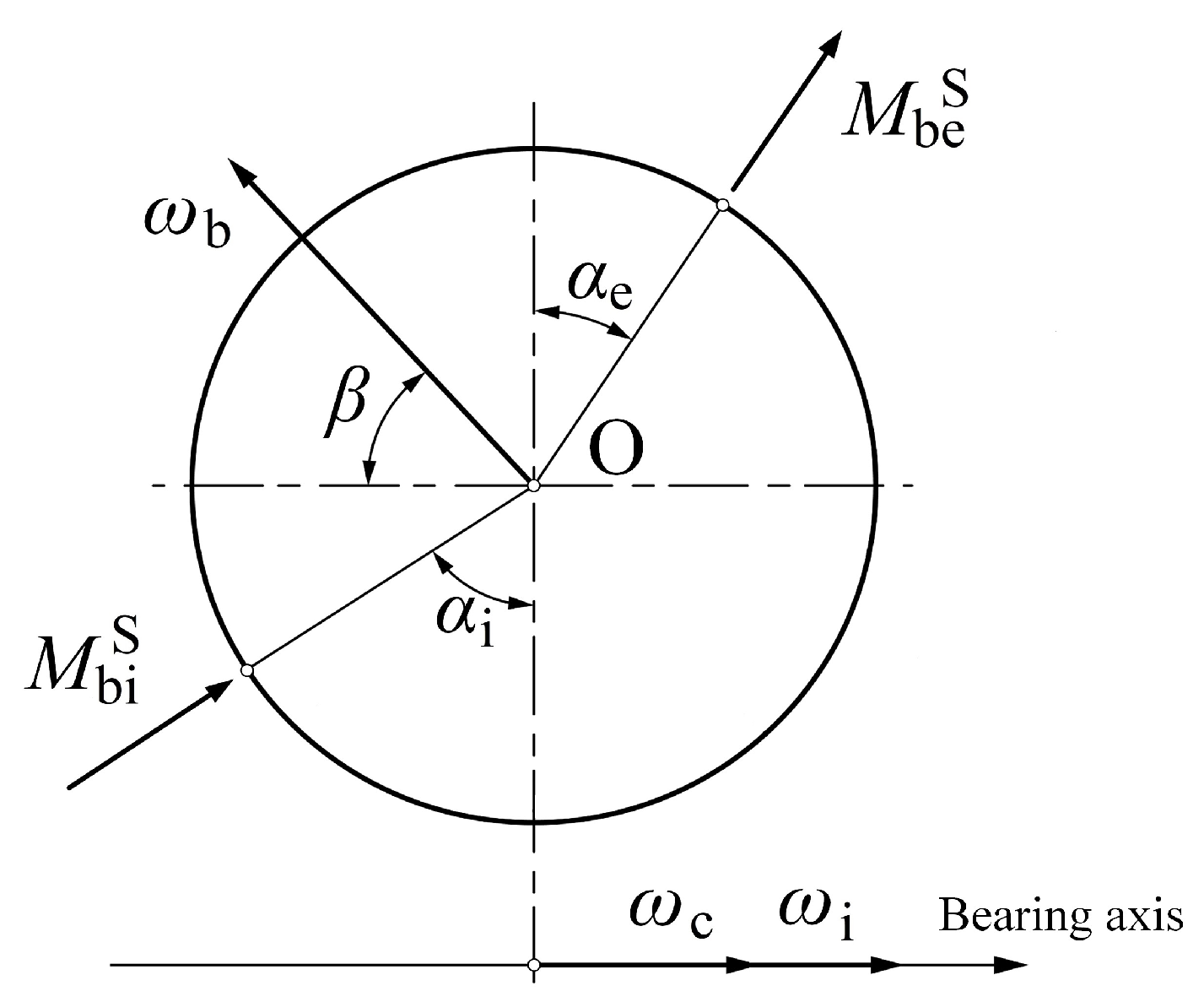
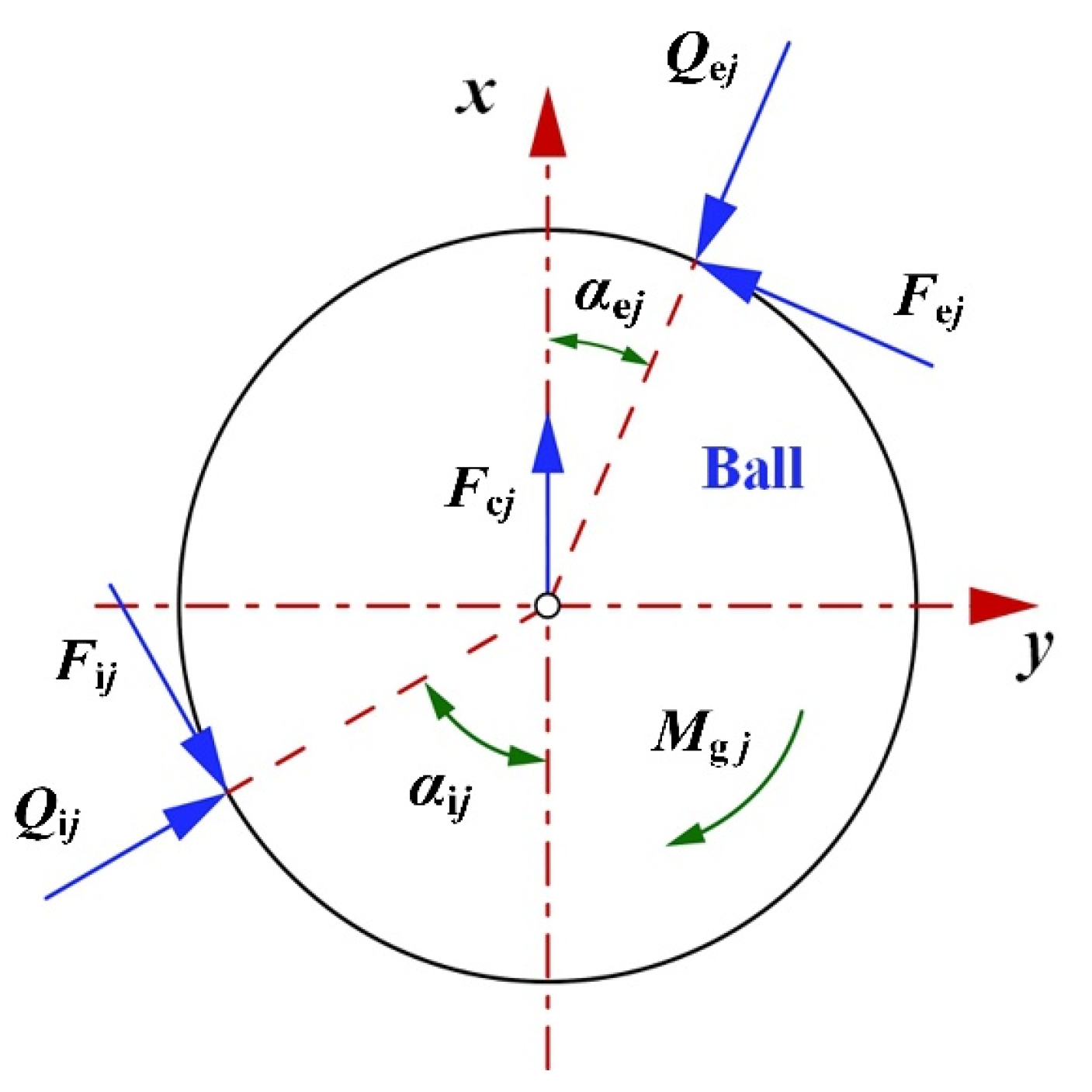
2.1. Quasi-Static Model of Angular Contact Ball Bearing
2.2. Shaft Model
2.3. Preload and Angular Misalignment Factors
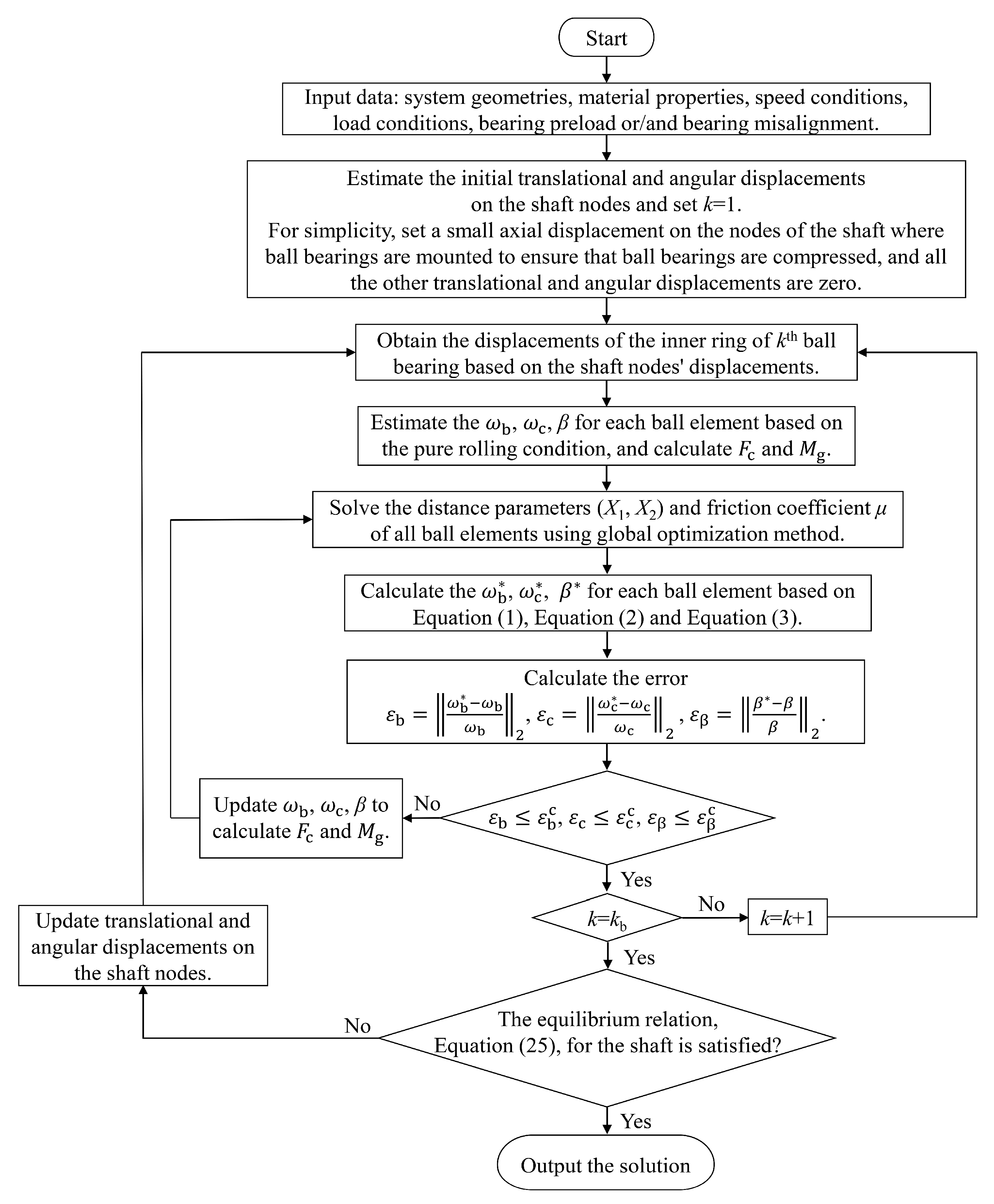
3. Numerical Solution and Model Validity
3.1. Numerical Solution of the System Model
3.2. Model Validity
4. Fatigue Life Model
5. Results and Discussion
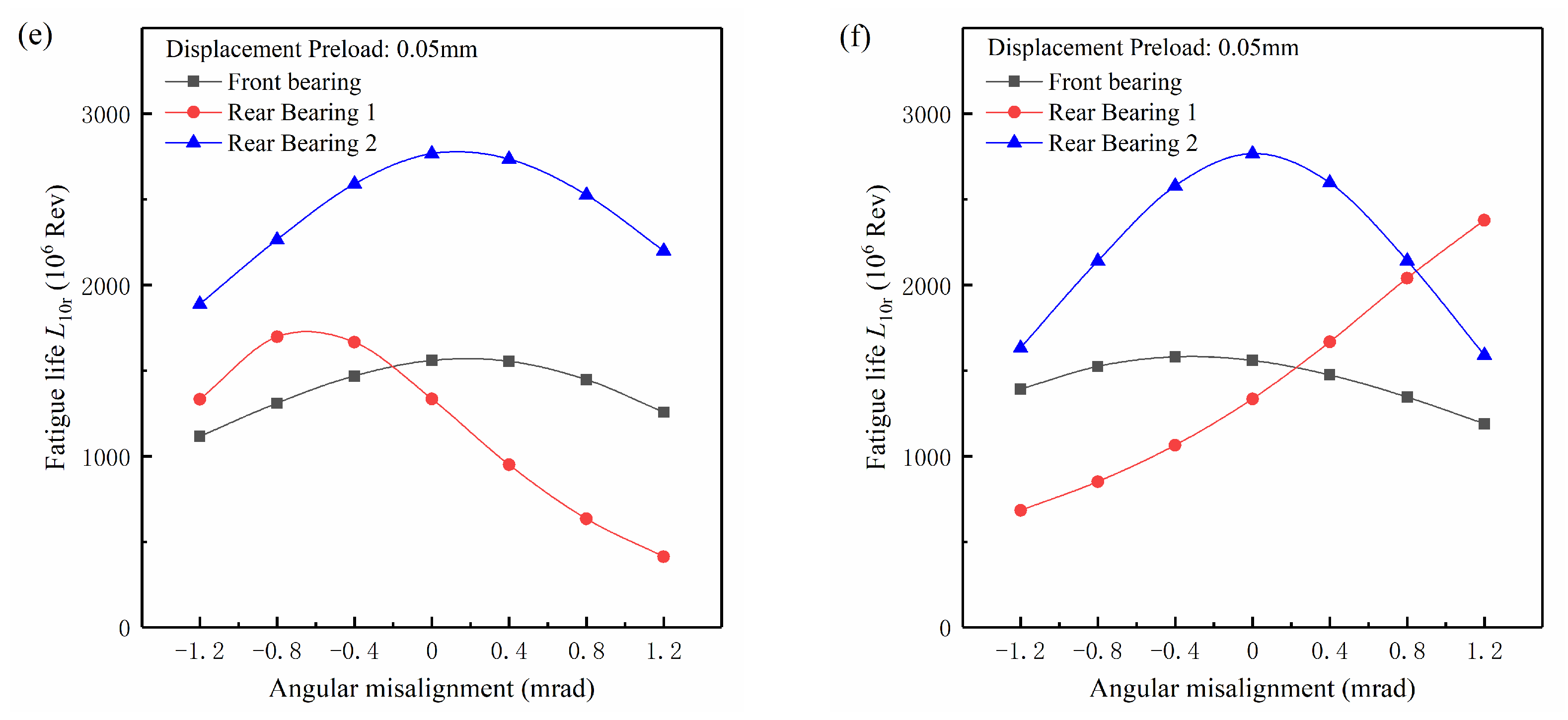
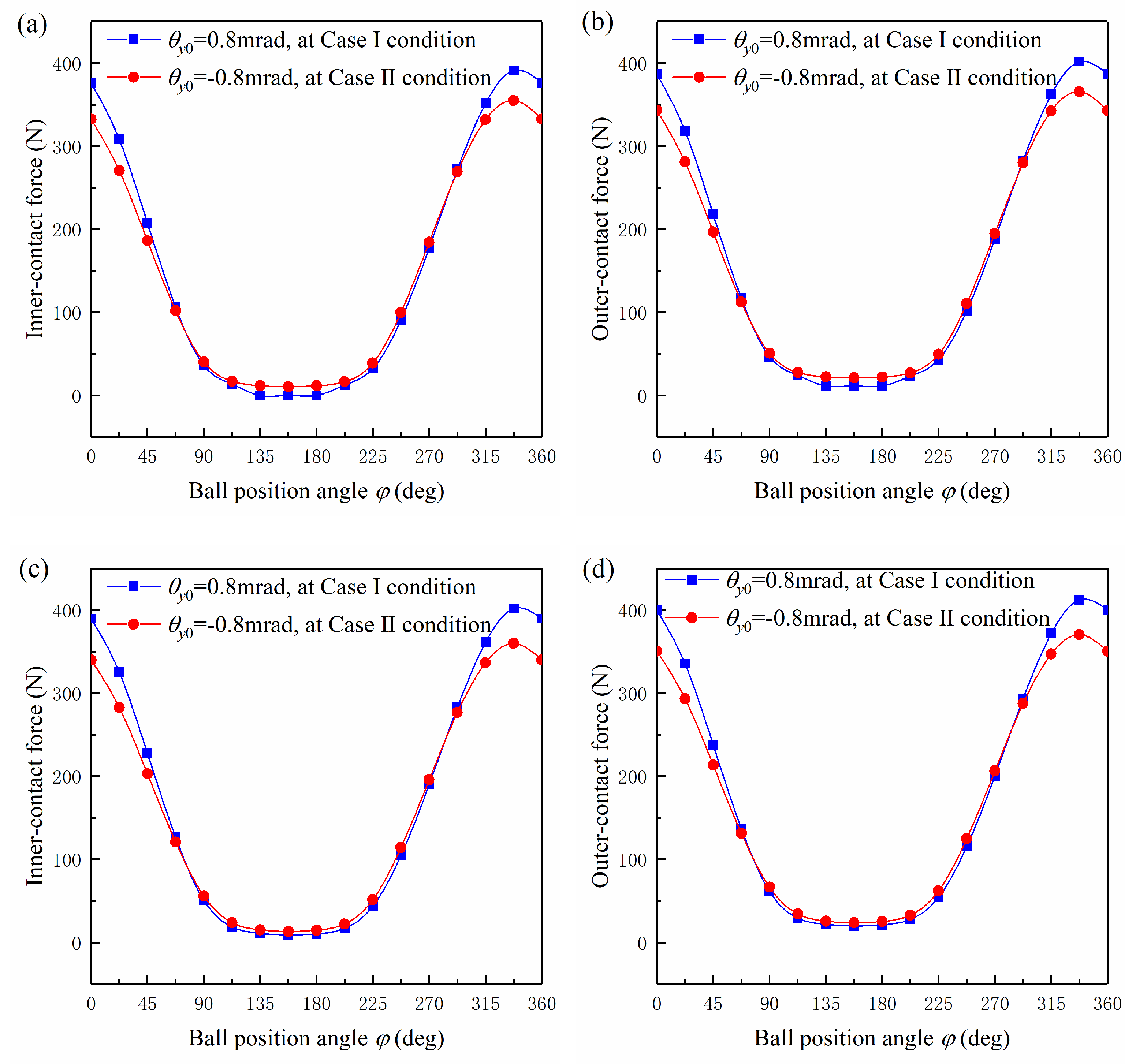
5.1. The Constant-Displacement Preload Condition
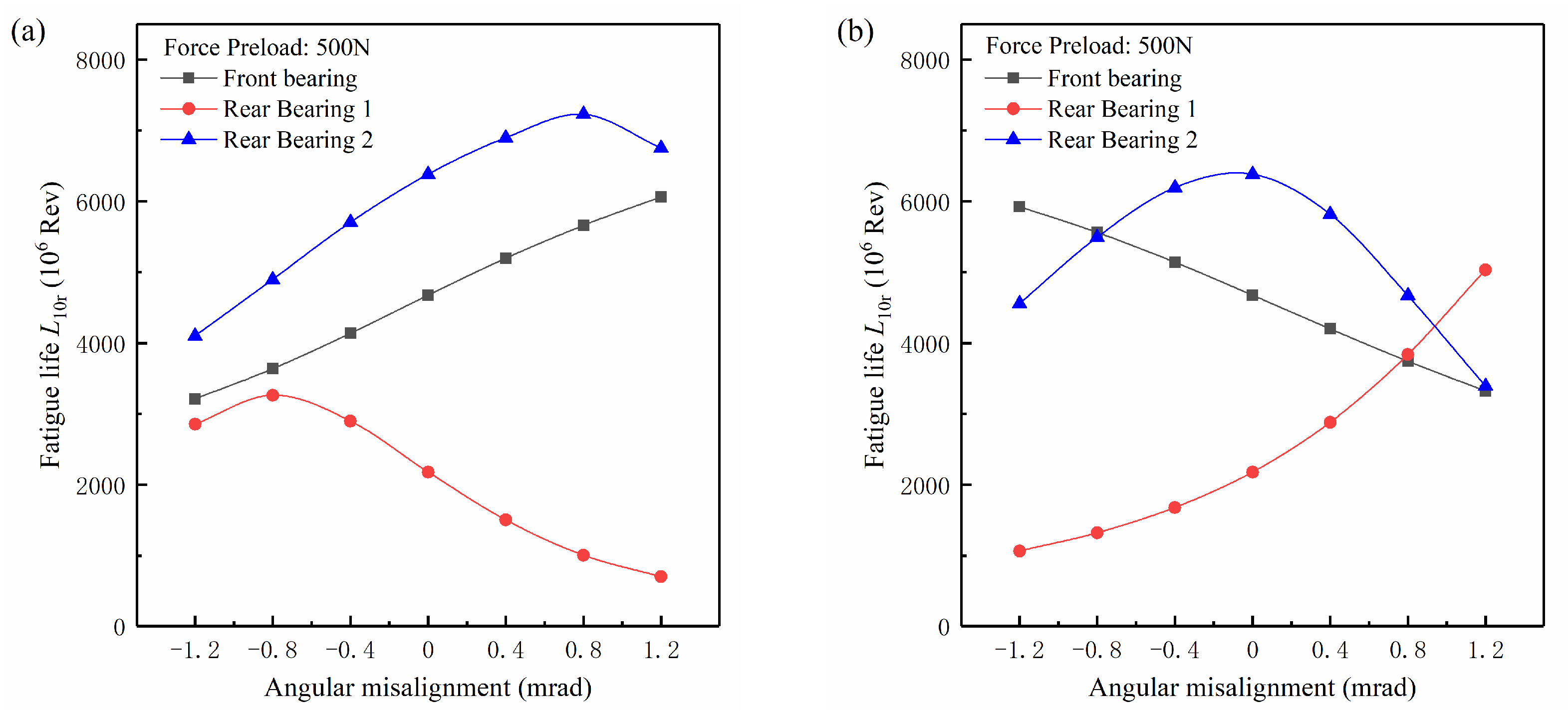
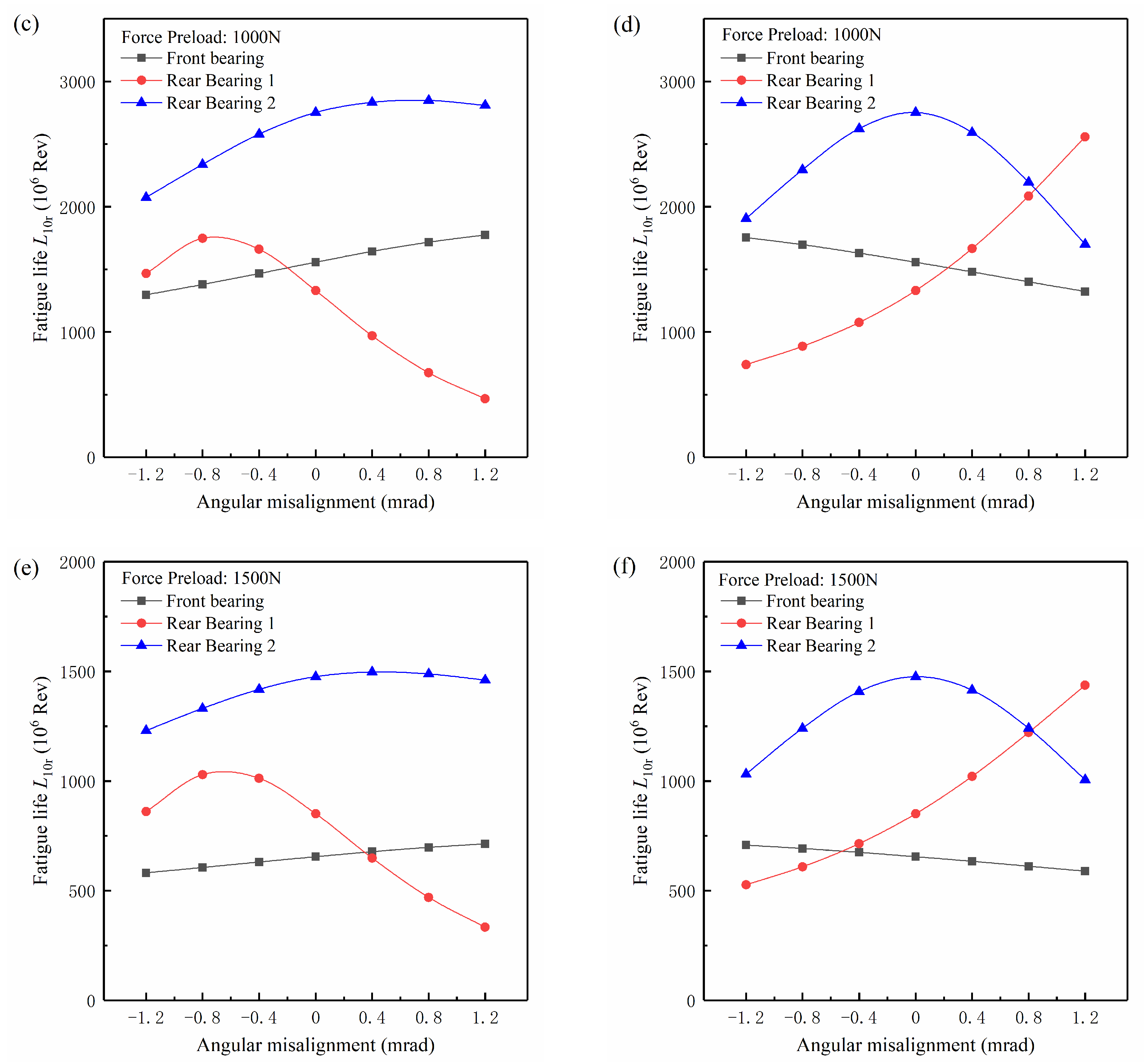
5.2. The Constant-Force Preload Condition
6. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
Nomenclature
| major semi-axis for ball-raceway contact ellipse, mm | |
| minor semi-axis for ball-raceway contact ellipse, mm | |
| ball diameter, mm | |
| bearing pitch diameter, mm | |
| inner diameter of bearing, mm | |
| outer diameter of bearing, mm | |
| thickness of bearing, mm | |
| groove curvature coefficients of outer raceway | |
| groove curvature coefficients of inner raceway | |
| mass moment of inertia, kg·mm2 | |
| density, kg/m3 | |
| ball mass, kg | |
| number of beam elements | |
| number of balls | |
| initial contact angle, rad | |
| contact angle at ball-outer raceway contact, rad | |
| contact angle at ball-inner raceway contact, rad | |
| ball pitch angle, rad | |
| angular speed of inner ring/shaft around the bearing axis, rad/s | |
| ball orbital speed around the bearing axis, rad/s | |
| ball spinning speed around its own axis, rad/s | |
| friction moment at ball-outer raceway contact due to spinning, N·mm | |
| friction moment at ball-inner raceway contact due to spinning, N·mm | |
| second kind of elliptic integral function | |
| contact force at ball-inner raceway contact, N | |
| contact force at ball-outer raceway contact, N | |
| ball centrifugal force, N | |
| gyroscopic moment, N·mm | |
| friction force at ball-inner raceway contact, N | |
| friction force at ball-outer raceway contact, N | |
| bearing force applied on the inner ring of ball bearing along x-axis of bearing local coordinate system, N | |
| bearing force applied on the inner ring of ball bearing along y-axis of bearing local coordinate system, N | |
| bearing force applied on the inner ring of ball bearing along z-axis of bearing local coordinate system, N | |
| moment applied on the inner ring of ball bearing around x-axis of bearing local coordinate system, N·mm | |
| moment applied on the inner ring of ball bearing around y-axis of bearing local coordinate system, N·mm | |
| external load vector applied on the shaft nodes, N | |
| ball-raceway friction coefficient | |
| initial position of ball center | |
| final position of ball center | |
| outer raceway groove curvature center | |
| initial position of inner raceway groove curvature center | |
| final position of inner raceway groove curvature center | |
| axial distance between ball center and outer raceway groove curvature center , mm | |
| radial distance between ball center and outer raceway groove curvature center , mm | |
| translational displacement of inner ring along x-axis of bearing local coordinate system, mm | |
| translational displacement of inner ring along y-axis of bearing local coordinate system, mm | |
| translational displacement of inner ring along z-axis of bearing local coordinate system, mm | |
| angular displacement of inner ring around x-axis of bearing local coordinate system, rad | |
| angular displacement of inner ring around y-axis of bearing local coordinate system, rad | |
| ball-outer raceway contact deformation, mm | |
| ball-inner raceway contact deformation, mm | |
| rotational speed of inner ring/shaft, rpm | |
| radius of locus of inner raceway groove curvature centers, mm | |
| ball azimuth angle, rad | |
| Poisson’s ratio | |
| deflection coefficient at ball and outer raceway contact, N/mm1.5 | |
| deflection coefficient at ball and inner raceway contact, N/mm1.5 | |
| groove curvature radius of inner raceway, mm | |
| global stiffness matrix of the shaft | |
| stiffness matrix of the beam element | |
| displacement vector of the shaft nodes, mm | |
| modulus of elasticity, GPa | |
| moment of inertia of the shaft section, mm4 | |
| length of beam element, mm | |
| total length of the shaft, mm | |
| number of angular contact ball bearings supporting the shaft | |
| calculation error of ball spinning speed | |
| calculation error of ball orbit speed | |
| calculation error of ball pitch angle | |
| axial displacement caused by constant-displacement preload or constant-force preload, mm | |
| angular misalignment of outer ring of ball bearing around x-axis of bearing local coordinate system, mrad | |
| angular misalignment of outer ring of ball bearing around y-axis of bearing local coordinate system, mrad | |
| basic reference rating life of bearing, in million revolutions | |
| basic reference rating life of inner ring, in million revolutions | |
| basic reference rating life of outer ring, in million revolutions | |
| basic dynamic load rating of inner ring, N | |
| basic dynamic load rating of outer ring, N | |
| equivalent dynamic load for rotating inner ring, N | |
| equivalent dynamic load for fixed outer ring, N |
References
- Jin, Y.; Yang, R.; Lei, H.; Chen, Y.; Zhang, Z. Experiments and Numerical Results for Varying Compliance Contact Resonance in a Rigid Rotor–Ball Bearing System. J. Tribol. 2017, 139, 041103. [Google Scholar] [CrossRef]
- Yang, Y.; Yang, W.; Jiang, D. Simulation and experimental analysis of rolling element bearing fault in rotor-bearing-casing system. Eng. Fail. Anal. 2018, 92, 205–221. [Google Scholar] [CrossRef]
- Wang, H.; Han, Q.; Zhou, D. Nonlinear dynamic modeling of rotor system supported by angular contact ball bearings. Mech. Syst. Signal Process. 2017, 85, 16–40. [Google Scholar] [CrossRef]
- Cao, H.; Niu, L.; Xi, S.; Chen, X. Mechanical model development of rolling bearing-rotor systems: A review. Mech. Syst. Signal Process. 2018, 102, 37–58. [Google Scholar] [CrossRef]
- Harris, T.A. The Effect of Misalignment on the Fatigue Life of Cylindrical Roller Bearings Having Crowned Rolling Members. J. Tribol. 1969, 91, 294–300. [Google Scholar] [CrossRef]
- Hagiu, G.; Gafitanu, M. Preload-service life correlation for ball bearings on machine tool main spindles. Wear 1994, 172, 79–83. [Google Scholar] [CrossRef]
- Hwang, Y.-K.; Lee, C.M. A review on the preload technology of the rolling bearing for the spindle of machine tools. Int. J. Precis. Eng. Manuf. 2010, 11, 491–498. [Google Scholar] [CrossRef]
- Tong, V.-C.; Hong, S.-W. Fatigue life of tapered roller bearing subject to angular misalignment. Proc. Inst. Mech. Eng. Part C J. Mech. Eng. Sci. 2015, 230, 147–158. [Google Scholar] [CrossRef]
- Yang, L.; Xu, T.; Xu, H.; Wu, Y. Mechanical behavior of double-row tapered roller bearing under combined external loads and angular misalignment. Int. J. Mech. Sci. 2018, 142, 561–574. [Google Scholar] [CrossRef]
- Warda, B.; Chudzik, A. Fatigue life prediction of the radial roller bearing with the correction of roller generators. Int. J. Mech. Sci. 2014, 89, 299–310. [Google Scholar] [CrossRef]
- Warda, B.; Chudzik, A. Effect of ring misalignment on the fatigue life of the radial cylindrical roller bearing. Int. J. Mech. Sci. 2016, 111, 1–11. [Google Scholar] [CrossRef]
- Jiang, S.; Mao, H. Investigation of variable optimum preload for a machine tool spindle. Int. J. Mach. Tools Manuf. 2010, 50, 19–28. [Google Scholar] [CrossRef]
- Xu, T.; Xu, G.; Zhang, Q.; Hua, C.; Tan, H.; Zhang, S.; Luo, A. A preload analytical method for ball bearings utilising bearing skidding criterion. Tribol. Int. 2013, 67, 44–50. [Google Scholar] [CrossRef]
- Than, V.-T.; Huang, J.H. Nonlinear thermal effects on high-speed spindle bearings subjected to preload. Tribol. Int. 2015, 96, 361–372. [Google Scholar] [CrossRef]
- Zhang, J.; Fang, B.; Hong, J.; Zhu, Y. Effect of preload on ball-raceway contact state and fatigue life of angular contact ball bearing. Tribol. Int. 2017, 114, 363–372. [Google Scholar] [CrossRef]
- Ding, C. Raceway control assumption and the determination of rolling element attitude angle. Chin. J. Mech. Eng. 2001, 37, 58–61. (In Chinese) [Google Scholar] [CrossRef]
- Harris, T.A.; Kotzalas, M.N. Rolling Bearing Analysis; Taylor & Francis: Boca Raton, FL, USA, 2007. [Google Scholar]
- Logan, D.L. A First Course in the Finite Element Method; Cengage: Boston, MA, USA, 2012. [Google Scholar]
- Glover, F. A Template for Scatter Search and Path Relinking, Lecture Notes in Computer Science 1363; Springer: Berlin/Heidelberg, Germany, 1998. [Google Scholar]
- Ugray, Z.; Lasdon, L.; Plummer, J.; Glover, F.; Kelly, J.; Martí, R. Scatter Search and Local NLP Solvers: A Multistart Framework for Global Optimization. INFORMS J. Comput. 2007, 19, 328–340. [Google Scholar] [CrossRef]
- Antoine, J.-F.; Abba, G.; Molinari, A. A New Proposal for Explicit Angle Calculation in Angular Contact Ball Bearing. J. Mech. Des. 2006, 128, 468–478. [Google Scholar] [CrossRef]
- Zhang, X.; Han, Q.; Peng, Z.-K.; Chu, F. Stability analysis of a rotor–bearing system with time-varying bearing stiffness due to finite number of balls and unbalanced force. J. Sound Vib. 2013, 332, 6768–6784. [Google Scholar] [CrossRef]
- Yang, Z.; Chen, H.; Yu, T. Effects of rolling bearing configuration on stiffness of machine tool spindle. Proc. Inst. Mech. Eng. Part C J. Mech. Eng. Sci. 2017, 232, 775–785. [Google Scholar] [CrossRef]
- Zhang, J.; Fang, B.; Zhu, Y.; Hong, J. A comparative study and stiffness analysis of angular contact ball bearings under different preload mechanisms. Mech. Mach. Theory 2017, 115, 1–17. [Google Scholar] [CrossRef]
- Tong, V.-C.; Hong, S.-W. Study on the running torque of angular contact ball bearings subjected to angular misalignment. Proc. Inst. Mech. Eng. Part J J. Eng. Tribol. 2017, 232, 890–909. [Google Scholar] [CrossRef]
- Lundberg, G.; Palmgren, A. Dynamic capacity of rolling bearings. In Acta Polytechnica, Mechanical Engineering Series I; Royal Swedish Academy of Engineering Sciences: Stockholm, Sweden, 1947; pp. 1–50. [Google Scholar]
- Li, X.; Li, H.; Hong, J.; Zhang, Y. Heat analysis of ball bearing under nonuniform preload based on five degrees of freedom quasi-static model. Proc. Inst. Mech. Eng. Part J J. Eng. Tribol. 2015, 230, 709–728. [Google Scholar] [CrossRef]
- SKF Group. SKF General Catalogue 6000/I EN; SKF Group: Gothenburg, Sweden, 2008. [Google Scholar]


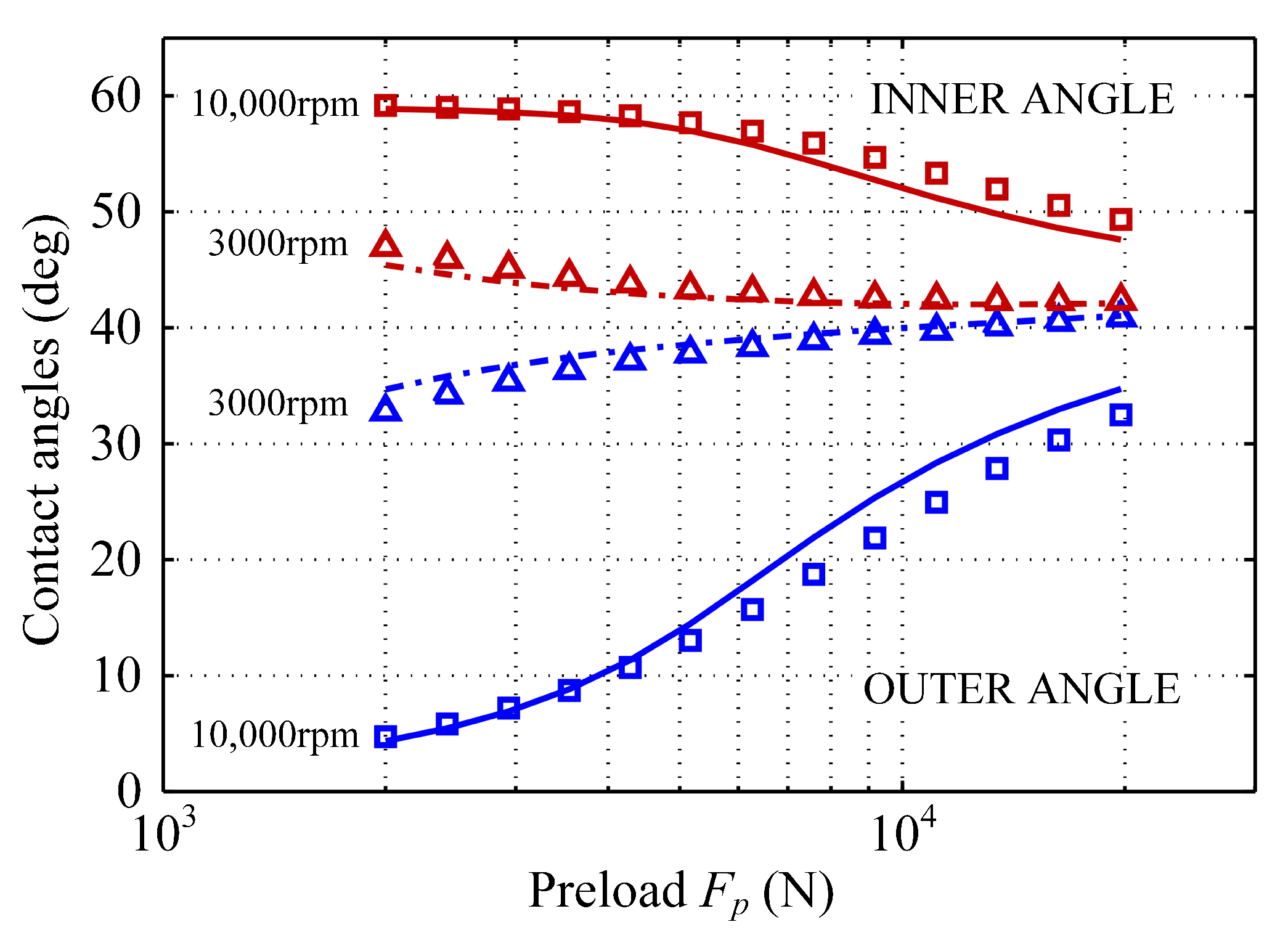
 ds = 35 mm,
ds = 35 mm,  ds = 40 mm from Tong’s model and
ds = 40 mm from Tong’s model and  ds = 35 mm,
ds = 35 mm,  ds = 40 mm from the present system model. (a) Face-to-Face arrangement; (b) Back-to-Back arrangement.
ds = 40 mm from the present system model. (a) Face-to-Face arrangement; (b) Back-to-Back arrangement.
 ds = 35 mm,
ds = 35 mm,  ds = 40 mm from Tong’s model and
ds = 40 mm from Tong’s model and  ds = 35 mm,
ds = 35 mm,  ds = 40 mm from the present system model. (a) Face-to-Face arrangement; (b) Back-to-Back arrangement.
ds = 40 mm from the present system model. (a) Face-to-Face arrangement; (b) Back-to-Back arrangement.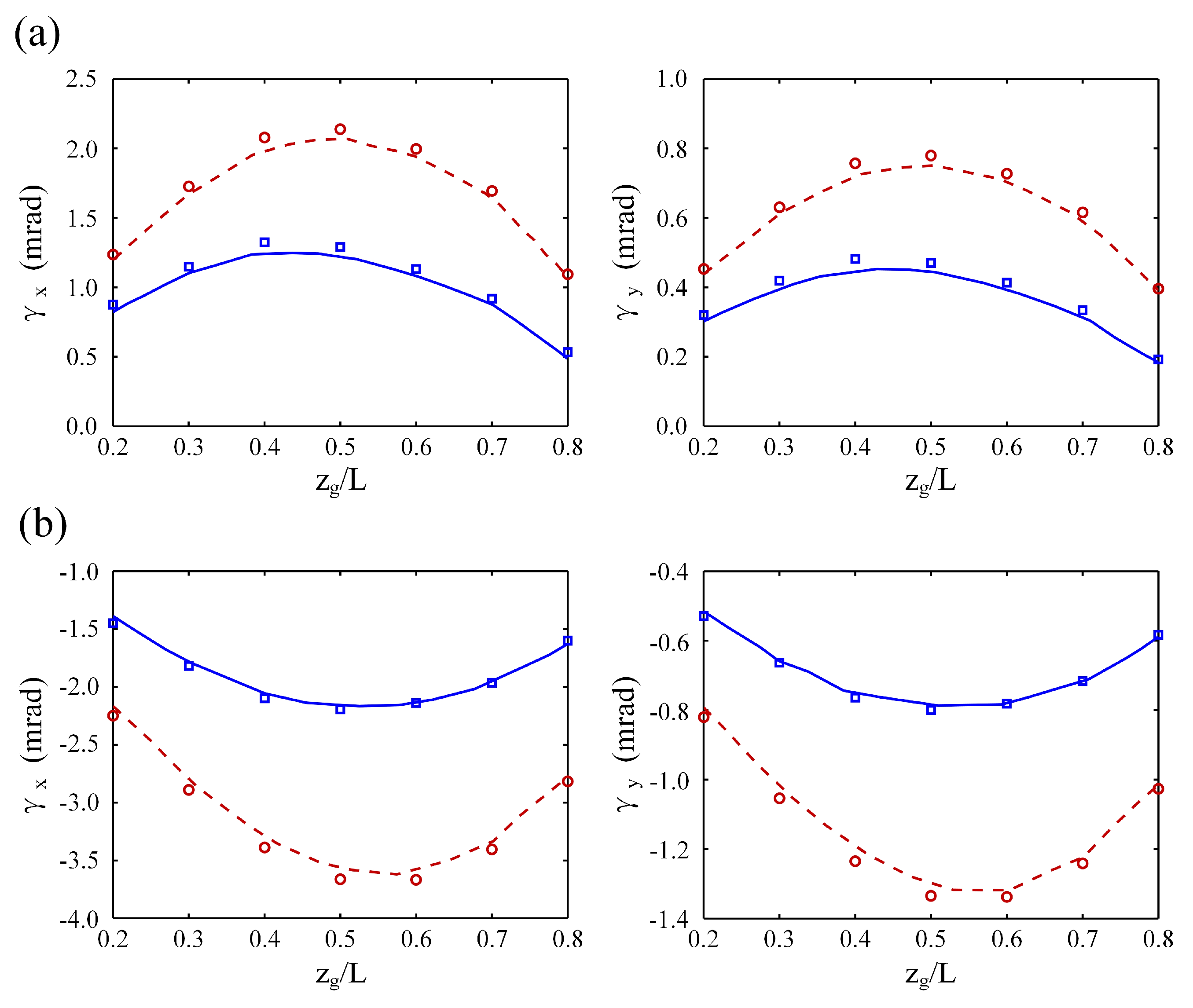
 ds = 35 mm,
ds = 35 mm,  ds = 40 mm from Tong’s model and
ds = 40 mm from Tong’s model and  ds = 35 mm,
ds = 35 mm,  ds = 40 mm from the present system model. (a) Face-to-Face arrangement; (b) Back-to-Back arrangement.
ds = 40 mm from the present system model. (a) Face-to-Face arrangement; (b) Back-to-Back arrangement.
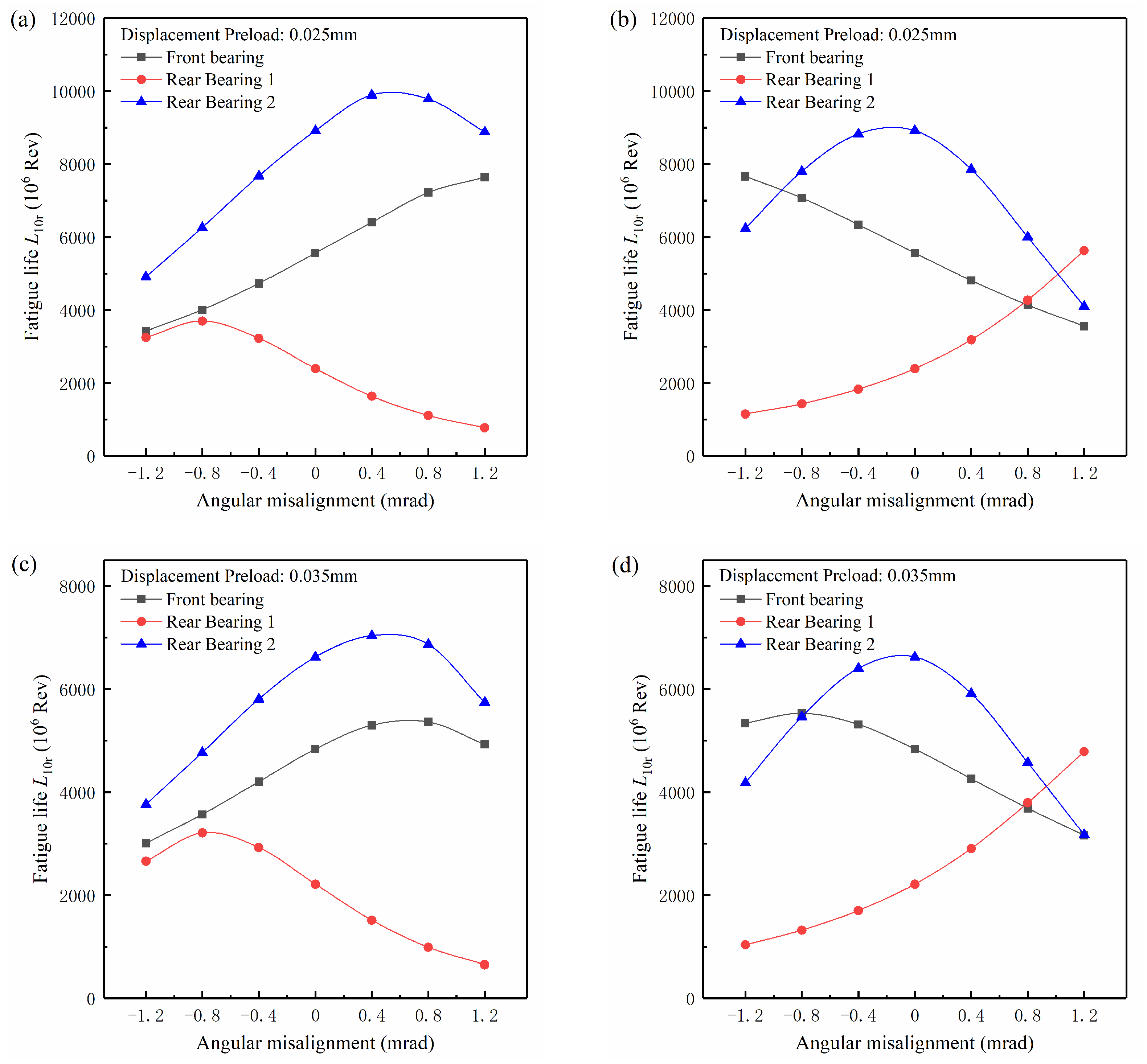
 ds = 35 mm,
ds = 35 mm,  ds = 40 mm from Tong’s model and
ds = 40 mm from Tong’s model and  ds = 35 mm,
ds = 35 mm,  ds = 40 mm from the present system model. (a) Face-to-Face arrangement; (b) Back-to-Back arrangement.
ds = 40 mm from the present system model. (a) Face-to-Face arrangement; (b) Back-to-Back arrangement.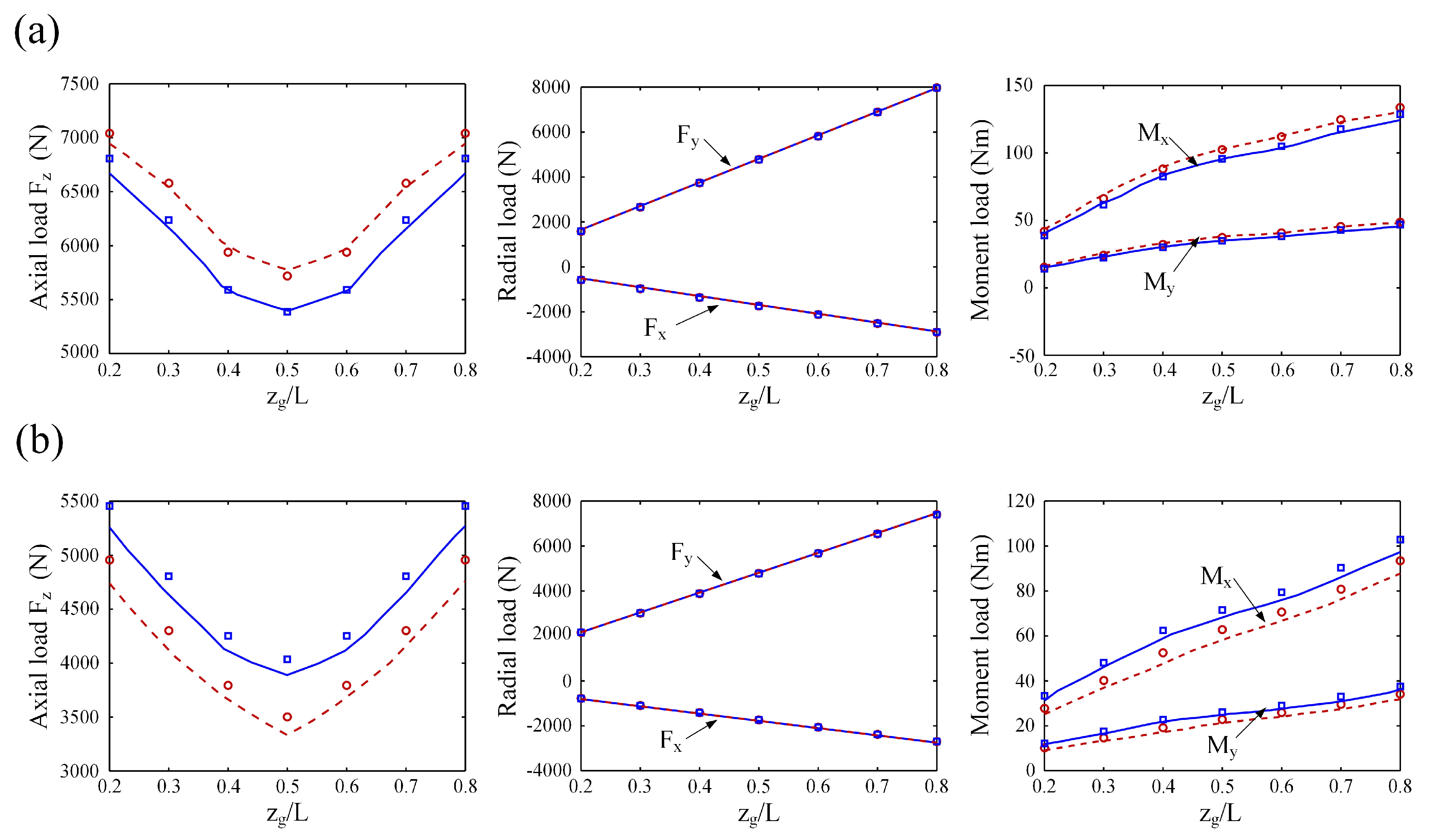

| Item | Super Light Preload | Light Preload | Medium Preload | Heavy Preload | ||||
|---|---|---|---|---|---|---|---|---|
| Force (N) | Axial Stiffness (N/μm) | Force (N) | Axial Stiffness (N/μm) | Force (N) | Axial Stiffness (N/μm) | Force (N) | Axial Stiffness (N/μm) | |
| Manual | 245 | 170 | 490 | 218 | 1080 | 293 | 2160 | 390 |
| Calculation | 245 | 164.90 | 490 | 213.48 | 1080 | 291.27 | 2160 | 389.80 |
| Error | 3.00% | 2.07% | 0.59% | 0.05% | ||||
| Parameter | Value |
|---|---|
| Inner diameter of bearing (mm) | 40 |
| Outer diameter of bearing (mm) | 68 |
| Ball diameter (mm) | 7.9 |
| Groove curvature coefficients of inner raceway | 0.52 |
| Groove curvature coefficients of outer raceway | 0.52 |
| Initial contact angle (deg) | 15 |
| Number of balls | 16 |
| Thickness (mm) | 15 |
| Modulus of elasticity (GPa) | 206 |
| Poisson’s ratio | 0.3 |
| Density (kg·m−3) | 7890 |
| Parameter | Value |
|---|---|
| (mm) | 40 |
| (mm) | 44 |
| Total length (mm) | 270 |
| Modulus of elasticity (GPa) | 206 |
| Poisson’s ratio | 0.3 |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhang, Y.; Zhang, M.; Wang, Y.; Xie, L. Fatigue Life Analysis of Ball Bearings and a Shaft System Considering the Combined Bearing Preload and Angular Misalignment. Appl. Sci. 2020, 10, 2750. https://doi.org/10.3390/app10082750
Zhang Y, Zhang M, Wang Y, Xie L. Fatigue Life Analysis of Ball Bearings and a Shaft System Considering the Combined Bearing Preload and Angular Misalignment. Applied Sciences. 2020; 10(8):2750. https://doi.org/10.3390/app10082750
Chicago/Turabian StyleZhang, Yu, Mengqi Zhang, Yawen Wang, and Liyang Xie. 2020. "Fatigue Life Analysis of Ball Bearings and a Shaft System Considering the Combined Bearing Preload and Angular Misalignment" Applied Sciences 10, no. 8: 2750. https://doi.org/10.3390/app10082750
APA StyleZhang, Y., Zhang, M., Wang, Y., & Xie, L. (2020). Fatigue Life Analysis of Ball Bearings and a Shaft System Considering the Combined Bearing Preload and Angular Misalignment. Applied Sciences, 10(8), 2750. https://doi.org/10.3390/app10082750






