Waiting Time Distributions of Transport through a Two-Channel Quantum System
Abstract
1. Introduction
2. Model and Theoretical Formalizations
2.1. Strong Coulomb Blockade Regime
2.2. Non-Interacting Dots
3. Results and Discussion
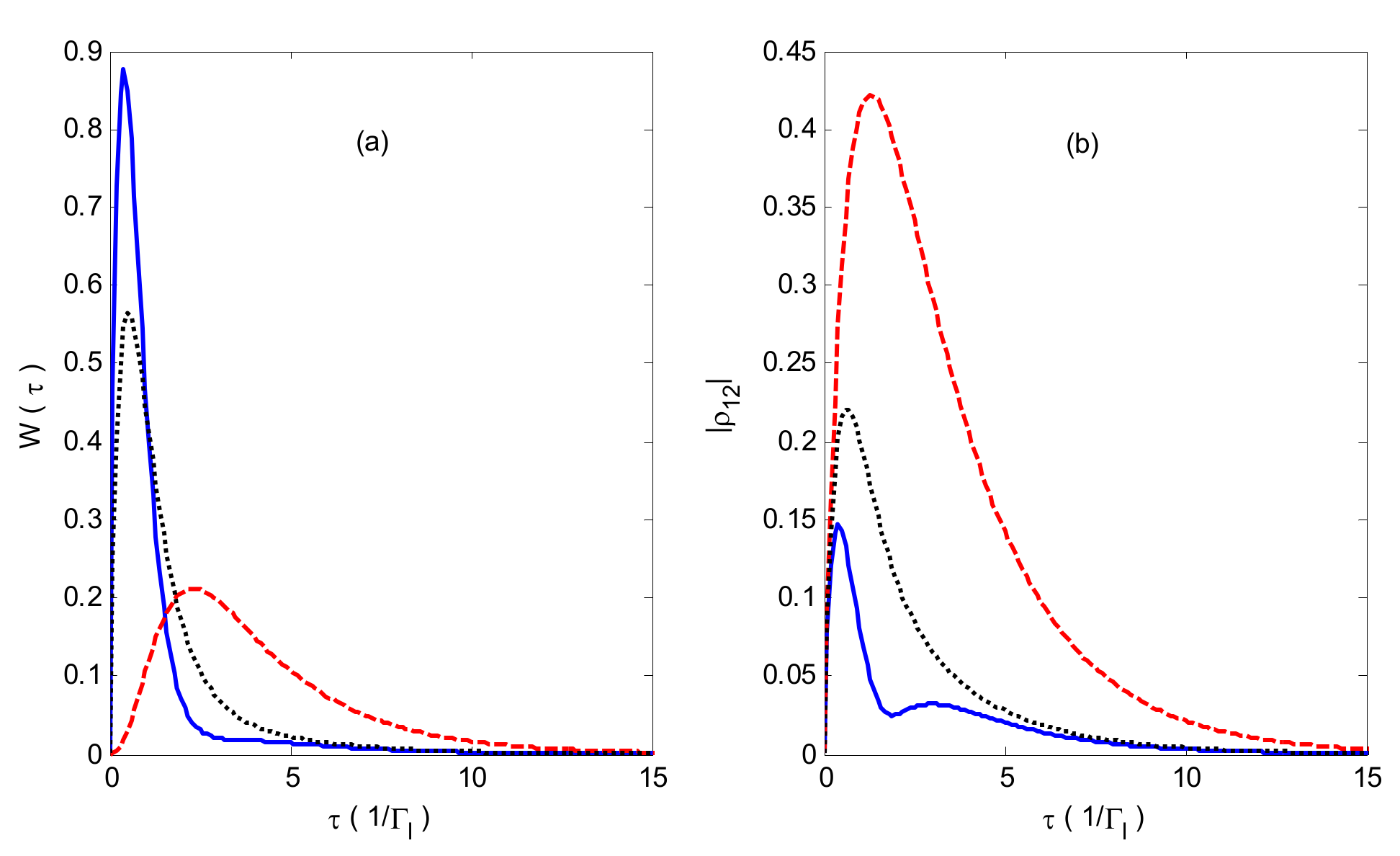
3.1. Strong Coulomb Blockade Regime
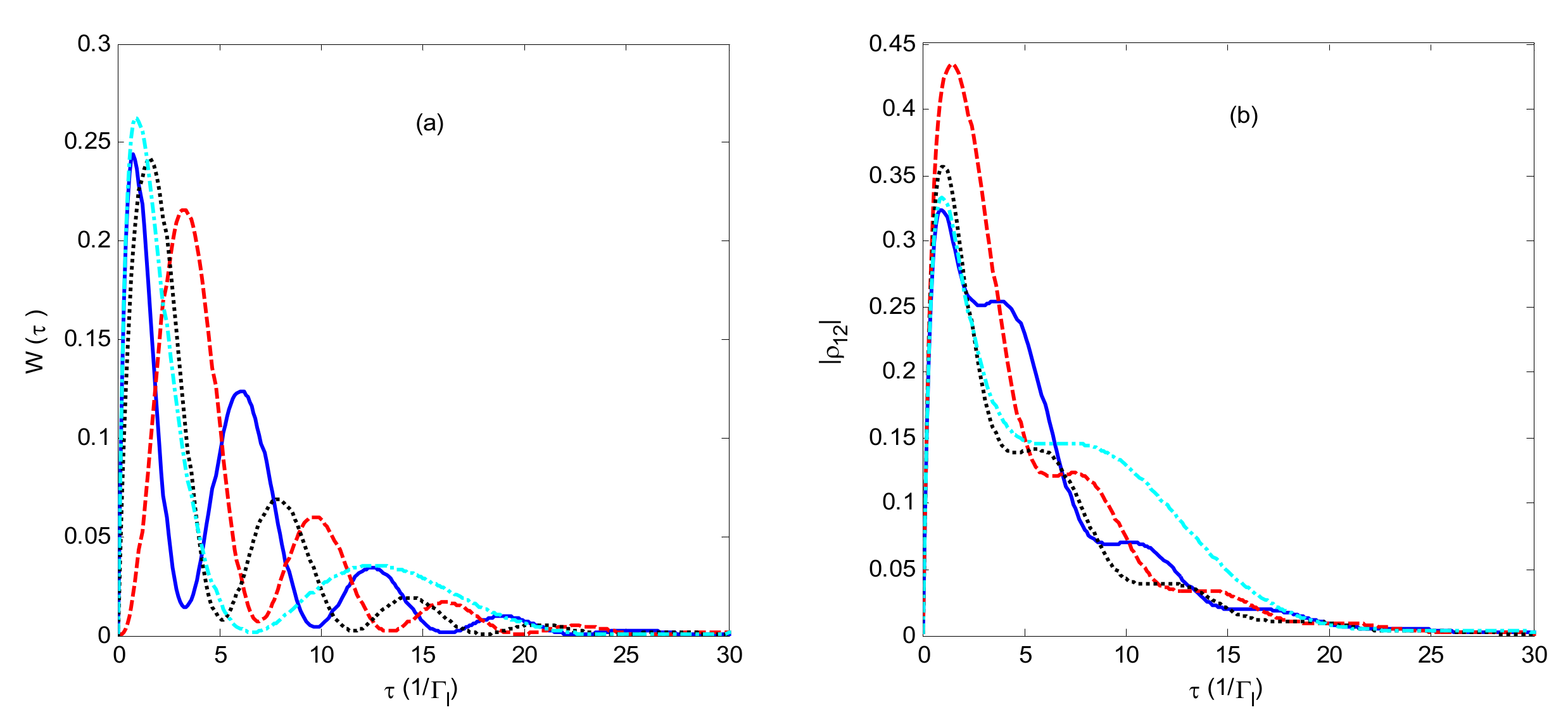
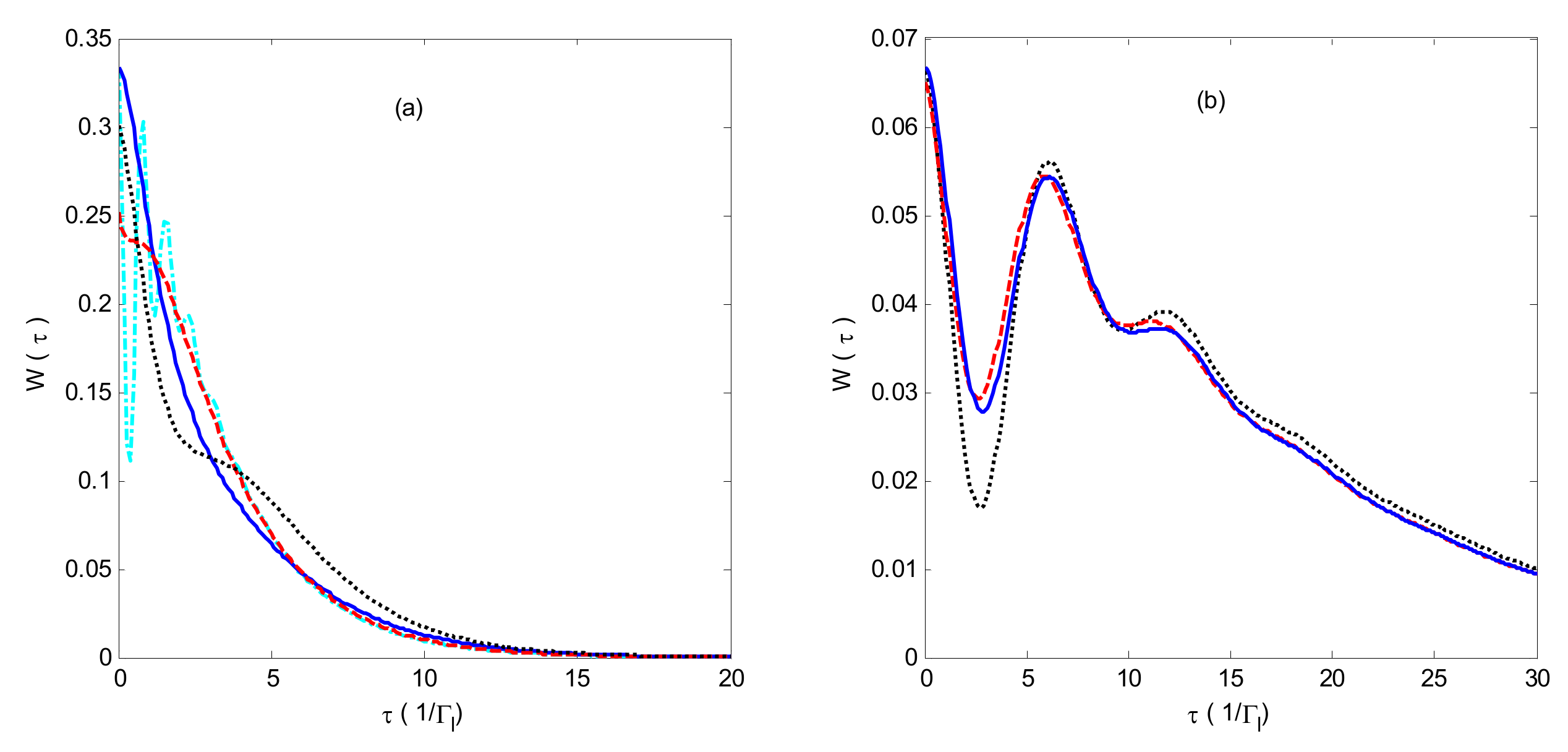
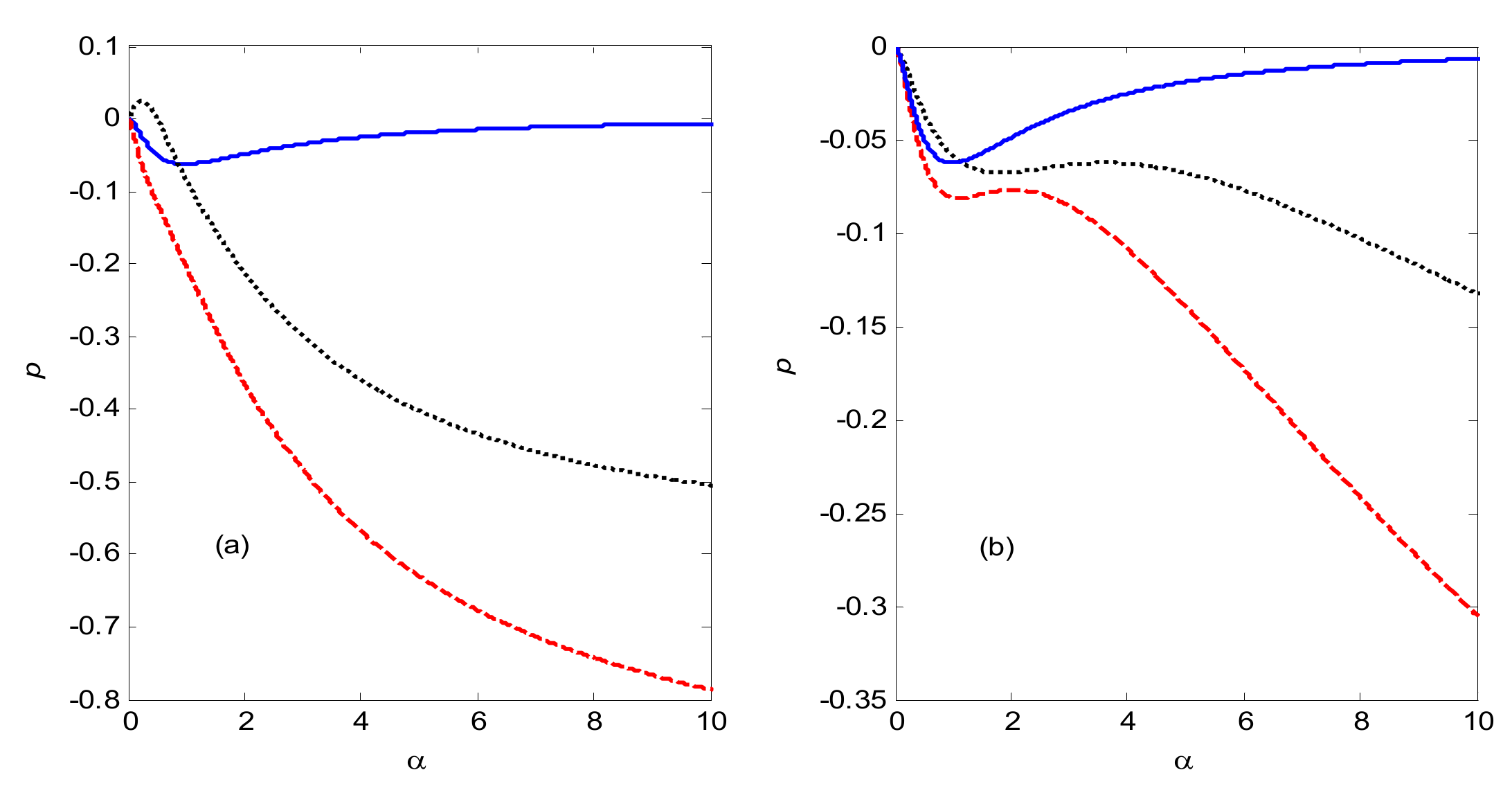
3.2. Non-Interacting Dots
4. Conclusions
Author Contributions
Funding
Conflicts of Interest
References
- Deng, G.W.; Wei, D.; Li, S.X.; Johansson, J.R.; Kong, W.C.; Li, H.O.; Jiang, H.W. Coupling two distant double quantum dots with a microwave resonator. Nano Lett. 2015, 15, 6620–6625. [Google Scholar] [CrossRef]
- Delbecq, M.R.; Schmitt, V.; Parmentier, F.D.; Roch, N.; Viennot, J.J.; Fève, G.; Huard, B.; Mora, C.; Cottet, A.; Kontos, T. Coupling a quantum dot, fermionic leads, and a microwave cavity on a chip. Phys. Rev. Lett. 2011, 107, 256804. [Google Scholar] [CrossRef] [PubMed]
- Kulkarni, M.; Cotlet, O.; Türeci, H.E. Cavity-coupled double-quantum dot at finite bias: Analogy with lasers and beyond. Phys. Rev. B 2014, 90, 125402. [Google Scholar] [CrossRef]
- Agarwalla, B.K.; Kulkarni, M.; Mukamel, S.; Segal, D. Giant photon gain in large-scale quantum dot-circuit QED systems. Phys. Rev. B 2016, 94, 121305. [Google Scholar] [CrossRef]
- Levitov, L.S.; Lee, H.; Lesovik, G.B. Electron counting statistics and coherent states of electric current. J. Math. Phys. 1996, 37, 4845. [Google Scholar] [CrossRef]
- Blanter, Y.M.; Büttiker, M. Shot noise in mesoscopic conductors. Phys. Rep. 2000, 336, 1–166. [Google Scholar] [CrossRef]
- Bennett, S.D.; Clerk, A.A. Full counting statistics and conditional evolution in a nanoelectromechanical system. Phys. Rev. B 2008, 78, 165328. [Google Scholar] [CrossRef]
- Emary, C. Counting statistics of cotunneling electrons. Phys. Rev. B 2009, 80, 235306. [Google Scholar] [CrossRef]
- Tang, G.M.; Wang, J. Full-counting statistics of charge and spin transport in the transient regime: A nonequilibrium Green’s function approach. Phys. Rev. B 2014, 90, 195422. [Google Scholar] [CrossRef]
- Tang, G.M.; Yu, Z.Z.; Wang, J. Full-counting statistics of energy transport of molecular junctions in the polaronic regime. New J. Phys. 2017, 19, 083007. [Google Scholar] [CrossRef]
- Ashida, Y.; Ueda, M. Full-counting many-particle dynamics: Nonlocal and chiral propagation of correlations. Phys. Rev. Lett. 2018, 120, 185301. [Google Scholar] [CrossRef] [PubMed]
- Fujisawa, T.; Hayashi, T.; Tomita, R.; Hirayama, Y. Bidirectional Counting of Single Electrons. Science 2006, 312, 1634–1636. [Google Scholar] [CrossRef] [PubMed]
- Albert, M.; Flindt, C.; Büttiker, M. Distributions of waiting times of dynamic single-electron emitters. Phys. Rev. Lett. 2011, 107, 086805. [Google Scholar] [CrossRef] [PubMed]
- Albert, M.; Haack, G.; Flindt, C.; Büttiker, M. Electron waiting times in mesoscopic conductors. Phys. Rev. Lett. 2012, 108, 186806. [Google Scholar] [CrossRef] [PubMed]
- Dasenbrook, D.; Hofer, P.P.; Flindt, C. Electron waiting times in coherent conductors are correlated. Phys. Rev. B 2015, 91, 195420. [Google Scholar] [CrossRef]
- Ptaszyński, K. Waiting time distribution revealing the internal spin dynamics in a double quantum dot. Phys. Rev. B 2017, 96, 035409. [Google Scholar] [CrossRef]
- Thomas, K.H.; Flindt, C. Electron waiting times in non- Markovian quantum transport. Phys. Rev. B 2013, 87, 121405. [Google Scholar] [CrossRef]
- Sothmann, B. Electronic waiting-time distribution of a quantum-dot spin valve. Phys. Rev. B 2014, 90, 155315. [Google Scholar] [CrossRef]
- Ptaszyński, K. Nonrenewal statistics in transport through quantum dots. Phys. Rev. B 2017, 95, 045306. [Google Scholar] [CrossRef]
- Hofer, P.P.; Dasenbrook, D.; Flindt, C. Electron waiting times for the mesoscopic capacitor. Physica E 2016, 82, 3–11. [Google Scholar] [CrossRef]
- Rajabi, L.; Pöltl, C.; Governale, M. Waiting Time Distributions for the Transport through a Quantum-Dot Tunnel Coupled to One Normal and One Superconducting Lead. Phys. Rev. Lett. 2013, 111, 067002. [Google Scholar] [CrossRef] [PubMed]
- Chevallier, D.; Albert, M.; Devillard, P. Probing Majorana and Andreev bound states with waiting times. Eur. Lett. 2016, 116, 27005. [Google Scholar] [CrossRef][Green Version]
- Brandes, T. Waiting times and noise in single particle transport. Ann. Der Phys. 2008, 17, 477–496. [Google Scholar] [CrossRef]
- Kosov, D.S. Waiting time distribution for electron transport in a molecular junction with electron-vibration interaction. J. Chem. Phys. 2017, 146, 074102. [Google Scholar] [CrossRef] [PubMed]
- Rudge, S.L.; Kosov, D.S. Nonrenewal statistics in quantum transport from the perspective of first-passage and waiting time distributions. Phys. Rev. B 2019, 99, 115426. [Google Scholar] [CrossRef]
- Rudge, S.L.; Kosov, D.S. Distribution of waiting times between electron cotunneling events. Phys. Rev. B 2018, 98, 245402. [Google Scholar] [CrossRef]
- Wang, S.K.; Jiao, H.; Li, F.; Li, X.Q.; Yan, Y. Full counting statistics of transport through two-channel Coulomb blockade systems. Phys. Rev. B 2007, 76, 125416. [Google Scholar] [CrossRef]
- Timm, C. Tunneling through molecules and quantum dots: Master-equation approaches. Phys. Rev. B 2008, 77, 195416. [Google Scholar] [CrossRef]
- Schuster, R.; Buks, E.; Heiblum, M.; Mahalu, D.; Umansky, V.; Shtrikman, H. Phase measurement in a quantum dot via a double-slit interference experiment. Nature 1997, 385, 417. [Google Scholar] [CrossRef]
- Entin-Wohlman, O.; Aharony, A.; Imry, Y.; Levinson, Y.; Schiller, A. Broken unitarity and phase measurements in Aharonov-Bohm interferometers. Phys. Rev. Lett. 2002, 88, 166801. [Google Scholar] [CrossRef]
- Avinun-Kalish, M.; Heiblum, M.; Zarchin, O.; Mahalu, D.; Umansky, V. Crossover from ‘mesoscopic’to ‘universal’phase for electron transmission in quantum dots. Nature 2005, 436, 529. [Google Scholar] [CrossRef] [PubMed]
- Santamore, D.H.; Neill, L.; Franco, N. Vibrationally mediated transport in molecular transistors. Phys. Rev. B 2013, 87, 075422. [Google Scholar] [CrossRef]
- Walter, S.; Trauzettel, B.; Schmidt, T.L. Transport properties of double quantum dots with electron-phonon coupling. Phys. Rev. B 2013, 88, 195425. [Google Scholar] [CrossRef]
- Brange, F.; Menczel, P.; Flindt, C. Photon counting statistics of a microwave cavity. Phys. Rev. B 2019, 99, 085418. [Google Scholar] [CrossRef]
- Woldeyohannes, M.; John, S. Coherent control of spontaneous emission near a photonic band edge. J. Opt. B 2003, 5, R43. [Google Scholar] [CrossRef]


© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Liu, W.; Wang, F.; Liang, R. Waiting Time Distributions of Transport through a Two-Channel Quantum System. Appl. Sci. 2020, 10, 1772. https://doi.org/10.3390/app10051772
Liu W, Wang F, Liang R. Waiting Time Distributions of Transport through a Two-Channel Quantum System. Applied Sciences. 2020; 10(5):1772. https://doi.org/10.3390/app10051772
Chicago/Turabian StyleLiu, Weici, Faqiang Wang, and Ruisheng Liang. 2020. "Waiting Time Distributions of Transport through a Two-Channel Quantum System" Applied Sciences 10, no. 5: 1772. https://doi.org/10.3390/app10051772
APA StyleLiu, W., Wang, F., & Liang, R. (2020). Waiting Time Distributions of Transport through a Two-Channel Quantum System. Applied Sciences, 10(5), 1772. https://doi.org/10.3390/app10051772




