Circular Dichroism in Low-Cost Plasmonics: 2D Arrays of Nanoholes in Silver
Abstract
Featured Application
Abstract
1. Introduction
2. Materials and Methods
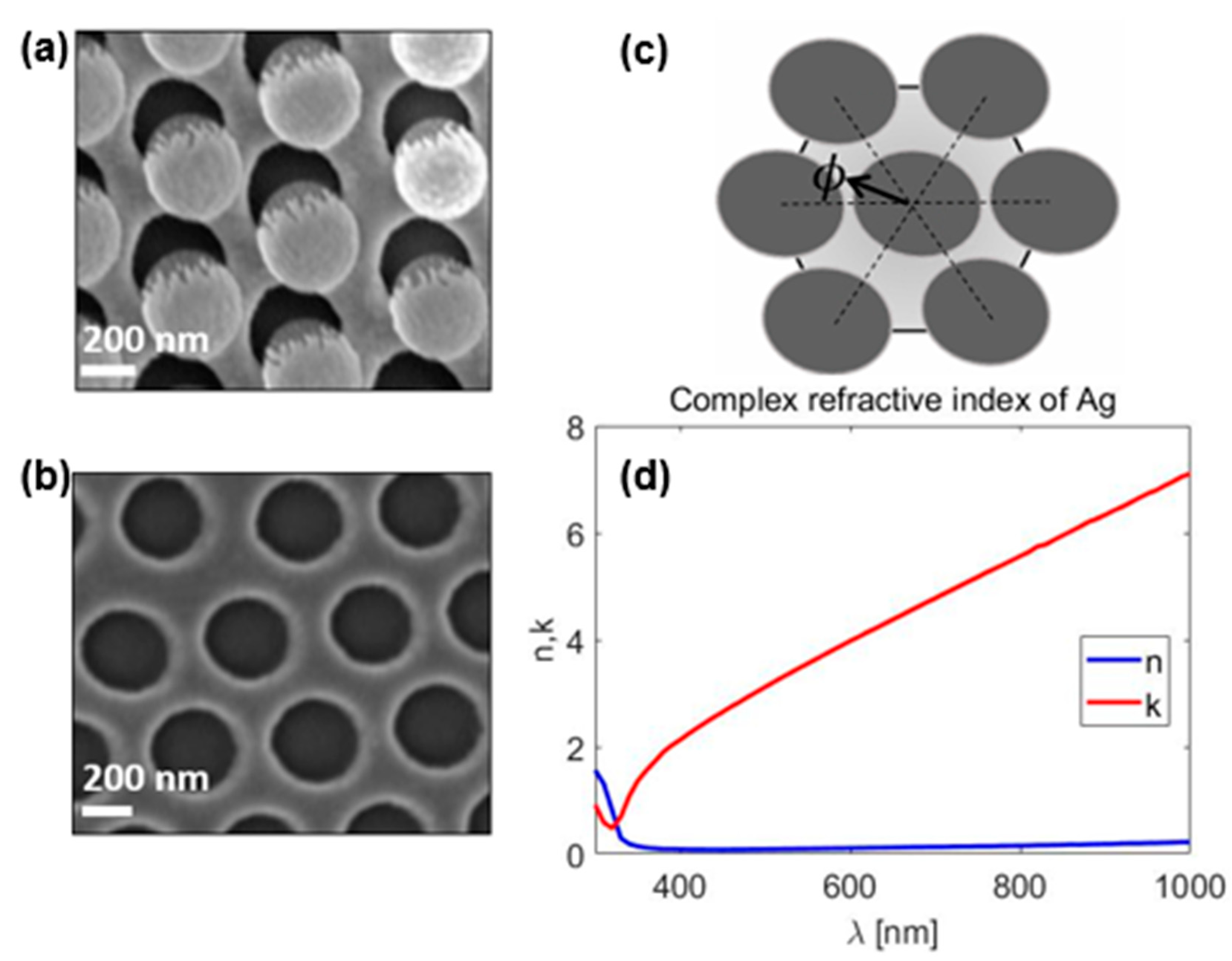
2.1. Fabrication
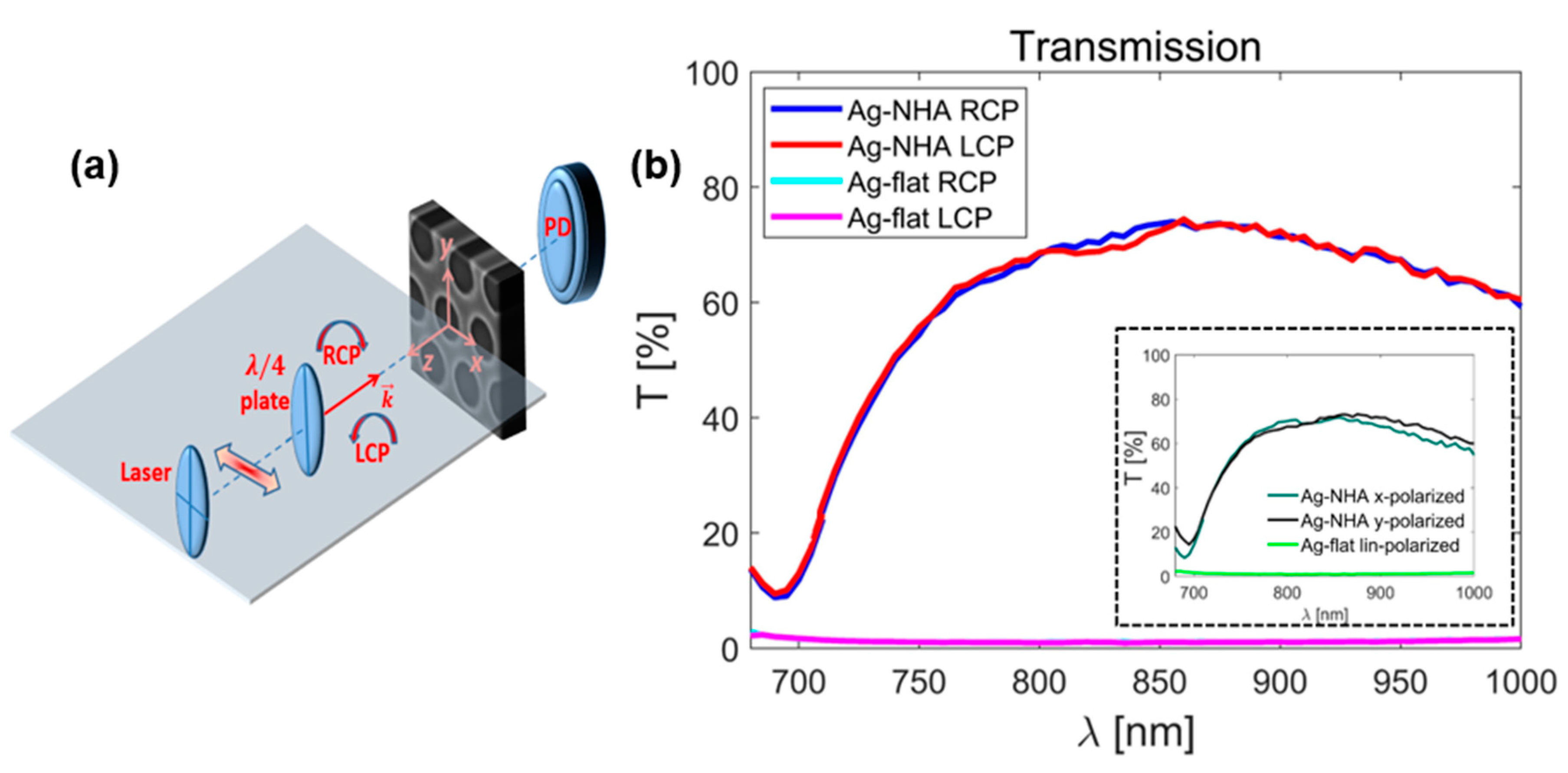
2.2. Measurements
2.3. Simulations
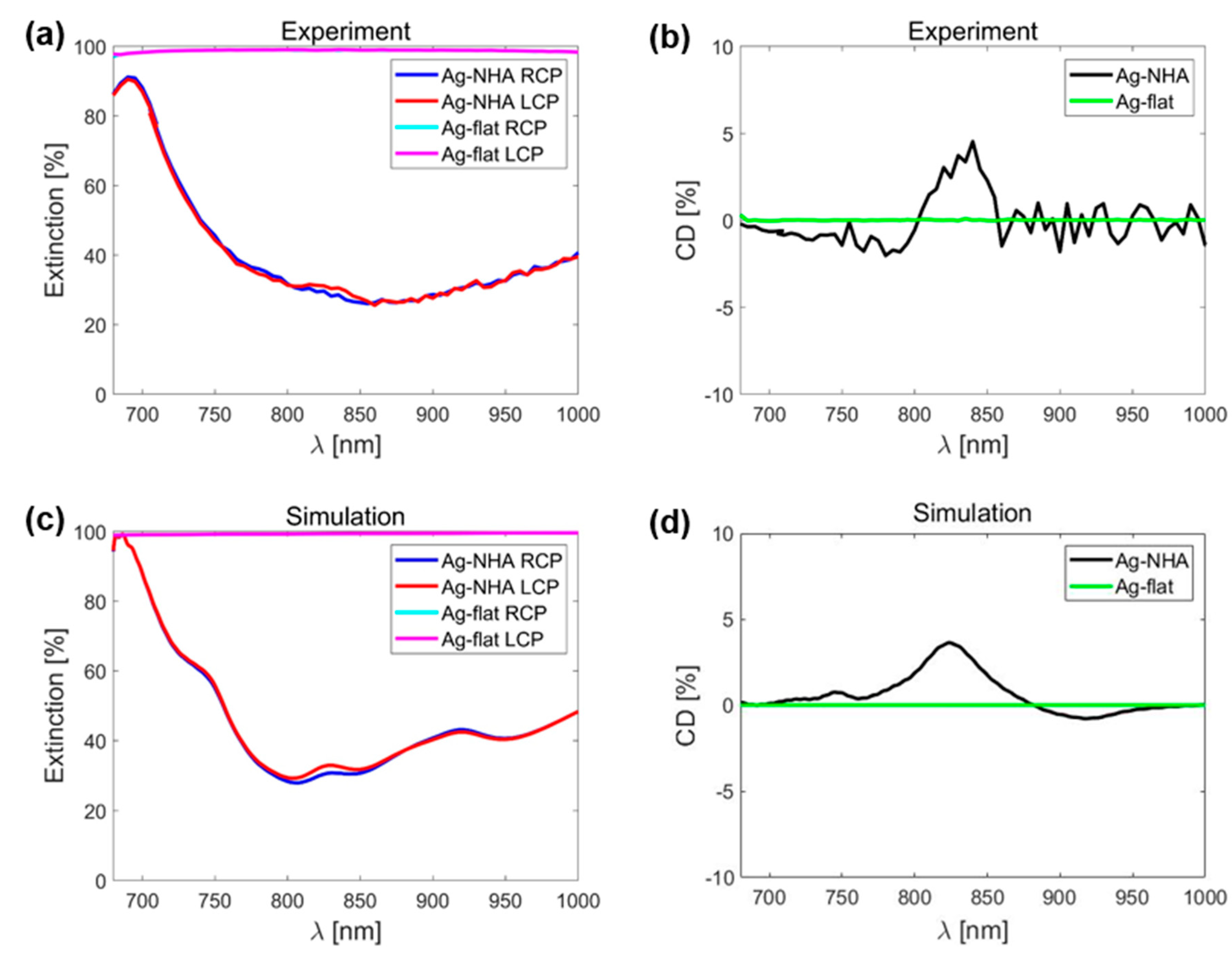
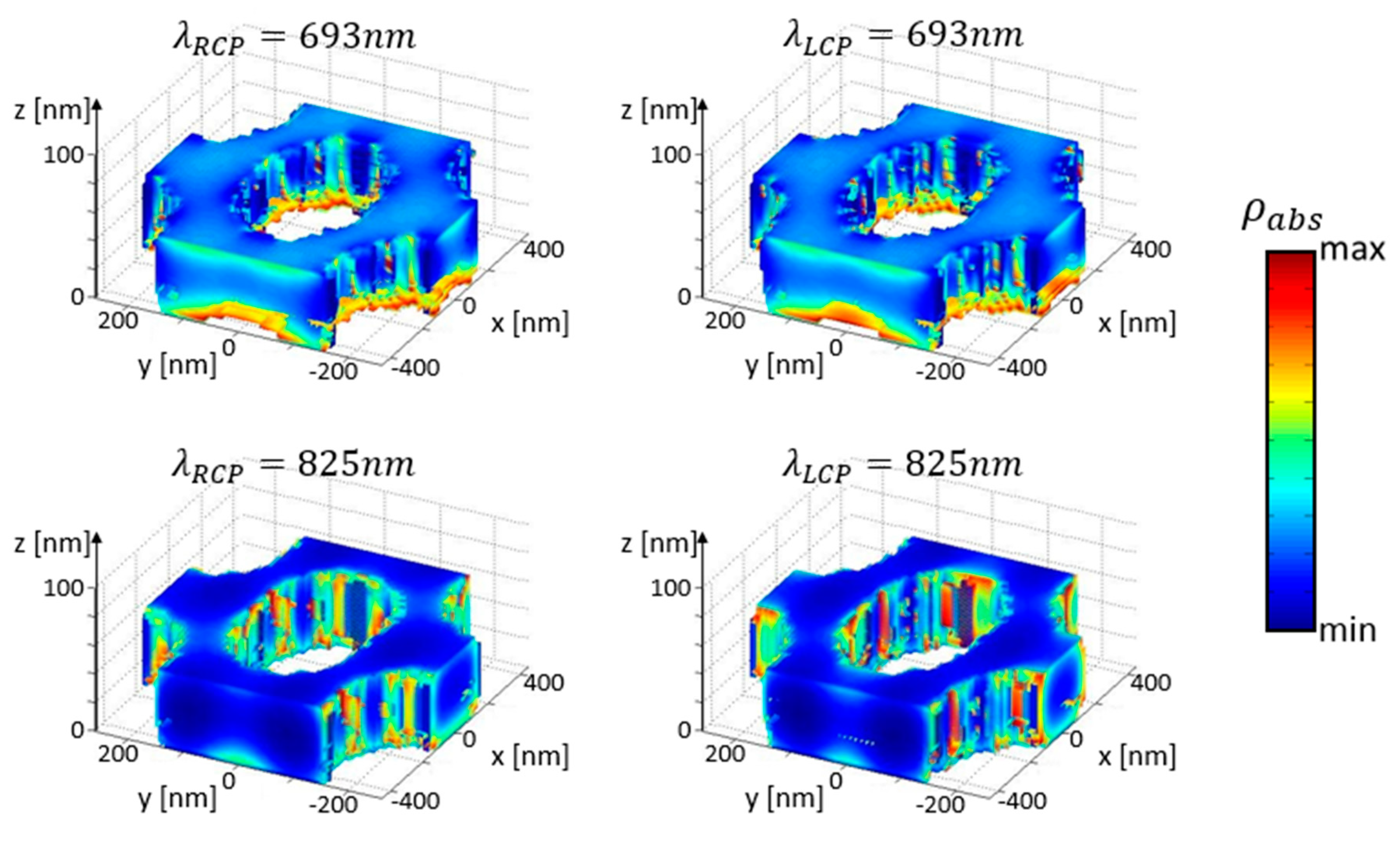
3. Results
4. Discussion
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Ebbesen, T.W.; Lezec, H.J.; Ghaemi, H.F.; Thio, T.; Wolff, P.A. Extraordinary optical transmission through sub-wavelength hole arrays. Nature 1998, 391, 667. [Google Scholar] [CrossRef]
- Maier, S.A. Plasmonics: Fundamentals and A; pplications Springer Science & Business: Media, Germany, 2007. [Google Scholar]
- Salomon, L.; Grillot, F.; Zayats, A.V.; de Fornel, F. Near-Field Distribution of Optical Transmission of Periodic Subwavelength Holes in a Metal Film. Phys. Rev. Lett. 2001, 86, 1110. [Google Scholar] [CrossRef] [PubMed]
- García de Abajo, F.J. Colloquium: Light scattering by particle and hole arrays. Rev. Mod. Phys. 2007, 79, 1267. [Google Scholar] [CrossRef]
- Barnes, W.L.; Dereux, A.; Ebbesen, T.W. Surface plasmon subwavelength optics. Nature 2003, 424, 824. [Google Scholar] [CrossRef]
- Xu, J.; Guan, P.; Kvasnička, P.; Gong, H.; Homola, J.; Yu, Q. Light Transmission and Surface-Enhanced Raman Scattering of Quasi-3D Plasmonic Nanostructure Arrays with Deep and Shallow Fabry-Pérot Nanocavities. J. Phys. Chem. C 2011, 115, 10996–11002. [Google Scholar] [CrossRef]
- Masson, J.-F.; Murray-Methota, M.-P.; Livea, L.S. Nanohole arrays in chemical analysis: manufacturing methods and applications. Analyst 2010, 135, 1483–1489. [Google Scholar] [CrossRef]
- Brolo, A.G.; Kwok, S.C.; Moffitt, M.G.; Gordon, R.; Riordon, J.; Kavanagh, K.L. Enhanced Fluorescence from Arrays of Nanoholes in a Gold Film. J. Am. Chem. Soc. 2005, 127, 14936–14941. [Google Scholar] [CrossRef]
- Michieli, N.; Kalinic, B.; Scian, C.; Cesca, T.; Mattei, G. Emission Rate Modification and Quantum Efficiency Enhancement of Er3+ Emitters by Near-Field Coupling with Nanohole Arrays. ACS Photonics 2018, 5, 2189–2199. [Google Scholar] [CrossRef]
- Cao, Z.L.; Yiu, L.Y.; Zhang, Z.Q.; Chan, C.T.; Ong, H.C. Understanding the role of surface plasmon polaritons in two-dimensional achiral nanohole arrays for polarization conversion. Phys. Rev. B 2017, 95, 155415. [Google Scholar] [CrossRef]
- Blanchard-Dionne, A.; Meunier, M. Sensing with periodic nanohole arrays. Adv. Opt. Photon. 2017, 9, 891–940. [Google Scholar] [CrossRef]
- Escobedo, C. On-chip nanohole array based sensing: a review. Lab. Chip. 2013, 13, 2445. [Google Scholar] [CrossRef] [PubMed]
- Balaşa, I.G. Nano-Hole Arrays for plasmonic biosensors. Master’s Degree Thesis, University of Padova, via Marzolo 8, I-35131 Padova, Italy, 2015. [Google Scholar]
- Couture, M.; Liang, Y.; Poirier Richard, H.-P.; Faid, R.; Peng, W.; Masson, J.-F. Tuning the 3D plasmon field of nanohole arrays. Nanoscale 2013, 5, 12399. [Google Scholar] [CrossRef] [PubMed]
- Jia, P.; Jiang, H.; Sabarinathan, J.; Yang, J. Plasmonic nanohole array sensors fabricated by template transfer with improved optical performance. Nanotechnology 2013, 24, 195501. [Google Scholar] [CrossRef] [PubMed]
- Blanchard-Dionne, A.; Meunier, M. Multiperiodic nanohole array for high precision sensing. Nanophotonics 2018, 8, 325–329. [Google Scholar] [CrossRef]
- Valsecchi, C.; Gomez Armas, L.E.; Weber de Menezes, J. Large Area Nanohole Arrays for Sensing Fabricated by Interference Lithography. Sensors 2019, 19, 2182. [Google Scholar] [CrossRef]
- Nguyen, L.A.; He, H.; Pham-Huy, C. Chiral drugs: An overview. Adv. Nat. Sci. Nanosci. Nanotechnol. 2006, 2, 85–100. [Google Scholar]
- Schäferling, M.; Dregely, D.; Hentschel, M.; Giessen, H. Tailoring enhanced optical chirality: Design principles for chiral plasmonic nanostructures. Phys. Rev. X 2012, 2, 031010. [Google Scholar] [CrossRef]
- Belardini, A.; Larciprete, M.C.; Centini, M.; Fazio, E.; Sibilia, C.; Chiappe, D.; Martella, C.; Toma, A.; Giordano, M.; Buatier de Mongeot, F. Circular dichroism in the optical second-harmonic emission of curved gold metal nanowires. Phys. Rev. Lett. 2011, 107, 257401. [Google Scholar] [CrossRef]
- Belardini, A.; Centini, M.; Leahu, G.; Hooper, D.C.; Li Voti, R.; Fazio, E.; Haus, J.W.; Sarangan, A.; Valev, V.K.; Sibilia, C. Chiral light intrinsically couples to extrinsic/pseudo-chiral metasurfaces made of tilted gold nanowires. Sci. Rep. 2016, 6, 31796. [Google Scholar] [CrossRef]
- Leahu, G.; Petronijević, E.; Belardini, A.; Centini, M.; Sibilia, C.; Hakkarainen, T.; Koivusalo, E.; Rizzo Piton, M.; Suomalainen, S.; Guina, M. Evidence of Optical Circular Dichroism in GaAs-Based Nanowires Partially Covered with Gold. Adv. Opt. Mater. 2017, 5, 1601063. [Google Scholar] [CrossRef]
- Petronijević, E.; Leahu, G.; Belardini, A.; Centini, M.; Li Voti, R.; Hakkarainen, T.; Koivusalo, E.; Rizzo Piton, M.; Suomalainen, S.; Guina, M.; et al. Photo-Acoustic Spectroscopy Reveals Extrinsic Optical Chirality in GaAs-Based Nanowires Partially Covered with Gold. Int. J. Thermophys. 2018, 39, 45. [Google Scholar] [CrossRef]
- Hakkarainen, T.; Petronijević, E.; Rizzo Piton, M.; Sibilia, C. Demonstration of extrinsic chirality of photoluminescence with semiconductor-metal hybrid nanowires. Sci. Rep. 2019, 9, 5040. [Google Scholar] [CrossRef] [PubMed]
- Tang, Y.; Cohen, A.E. Optical chirality and its interaction with matter. Phys. Rev. Lett. 2010, 104, 163901. [Google Scholar] [CrossRef]
- Tang, Y.; Cohen, A.E. Enhanced enantioselectivity in excitation of chiral molecules by superchiral light. Science 2011, 332, 333–336. [Google Scholar] [CrossRef]
- Hendry, E.; Carpy, T.; Johnston, J.; Popland, M.; Mikhaylovskiy, R.V.; Lapthorn, A.J.; Kelly, S.M.; Barron, L.D.; Gadegaard, N.; Kadodwala, M. Ultrasensitive detection and characterization of biomolecules using superchiral fields. Nat. Nanotechnol. 2010, 5, 783–787. [Google Scholar] [CrossRef] [PubMed]
- Zhao, Y.; Askarpour, A.N.; Sun, L.; Shi, J.; Li, X.; Alù, A. Chirality detection of enantiomers using twisted optical metamaterials. Nat. Commun. 2017, 8, 14180. [Google Scholar] [CrossRef]
- Petronijević, E.; Centini, M.; Belardini, A.; Leahu, G.; Hakkarainen, T.; Sibilia, C. Chiral near-field manipulation in Au-GaAs hybrid hexagonal nanowires. Opt. Express 2017, 25, 14148. [Google Scholar] [CrossRef] [PubMed]
- Leahu, G.; Petronijević, E.; Belardini, A.; Centini, M.; Li Voti, R.; Hakkarainen, T.; Koivusalo, E.; Guina, M.; Sibilia, C. Photo-acoustic spectroscopy revealing resonant absorption of self-assembled GaAs-based nanowires. Sci. Rep. 2017, 7, 2833. [Google Scholar] [CrossRef] [PubMed]
- Petronijević, E.; Sibilia, C. Enhanced Near-Field Chirality in Periodic Arrays of Si Nanowires for Chiral Sensing. Molecules 2019, 24, 853. [Google Scholar] [CrossRef]
- Petronijević, E.; Sandoval, E.M.; Ramezani, M.; Ordóňez-Romero, C.L.; Noguez, C.; Bovino, F.A.; Sibilia, C.; Pirruccio, G. Extended Chiro-optical Near-Field Response of Achiral Plasmonic Lattices. J. Phys. Chem. C 2019, 123, 38–23620. [Google Scholar] [CrossRef]
- Yao, H.; Zhong, S. Handedness-switchable chiral field in the 1D metal grooves for plasmonic circular dichroism spectroscopy. J. Opt. 2017, 19, 055001. [Google Scholar] [CrossRef]
- Maoz, B.M.; Ben Moshe, A.; Vestler, D.; Bar-Elli, O.; Markovich, G. Chiroptical Effects in Planar Achiral Plasmonic Oriented Nanohole Arrays. Nano Lett. 2012, 12, 2357–2361. [Google Scholar] [CrossRef] [PubMed]
- Gorkunov, M.V.; Ezhov, A.A.; Artemov, V.V.; Rogov, O.Y.; Yudin, S.G. Extreme optical activity and circular dichroism of chiral metal hole arrays. Appl. Phys. Lett. 2014, 104, 221102. [Google Scholar] [CrossRef]
- Gorkunov, M.V.; Dmitrienko, V.E.; Ezhov, A.A.; Artemov, V.V.; Rogov, O.Y. Implications of the causality principle for ultra chiral metamaterials. Sci. Rep. 2015, 5, 9273. [Google Scholar] [CrossRef] [PubMed]
- Kondratov, A.V.; Gorkunov, M.V.; Darinskii, A.N.; Gainutdinov, R.V.; Rogov, O.Y.; Ezhov, A.A.; Artemov, V.V. Extreme optical chirality of plasmonic nanohole arrays due to chiral Fano resonance. Phys. Rev. B 2016, 93, 195418. [Google Scholar] [CrossRef]
- Cesca, T.; Michieli, N.; Kalinic, B.; Sánchez-Espinoza, A.; Rattin, M.; Russo, V.; Mattarello, V.; Scian, C.; Mazzoldi, P.; Mattei, G. Nonlinear absorption tuning by composition control in bimetallic plasmonic nanoprism arrays. Nanoscale 2015, 7, 12411–12418. [Google Scholar] [CrossRef]
- Russo, V.; Michieli, N.; Cesca, T.; Scian, C.; Silvestri, D.; Morpurgo, M.; Mattei, G. Gold–silver alloy semi-nanoshell arrays for label-free plasmonic biosensors. Nanoscale 2017, 9, 10117–10125. [Google Scholar] [CrossRef]
- Sanchez-Esquivel, H.; Raygoza-Sanchez, K.Y.; Rangel-Rojo, R.; Gemo, E.; Michieli, N.; Kalinic, B.; Reyes-Esqueda, J.A.; Cesca, T.; Mattei, G. Spectral dependence of nonlinear absorption in ordered silver metallic nanoprism arrays. Sci. Rep. 2017, 7, 5307. [Google Scholar]
- Sanchez-Esquivel, H.; Raygoza-Sanchez, K.Y.; Rangel-Rojo, R.; Kalinic, B.; Michieli, N.; Cesca, T.; Mattei, G. Ultra-fast dynamics in the nonlinear optical response of silver nanoprisms ordered arrays. Nanoscale 2018, 10, 5182–5190. [Google Scholar] [CrossRef]
- Cesca, T.; Garcia Ramirez, E.V.; Sanchez-Esquivel, H.; Michieli, N.; Kalinic, B.; Gomez Cervantes, J.M.; Rangel-Rojo, R.; Reyes Esqueda, J.A.; Mattei, G. Dichroic nonlinear absorption response of silver nanoprism arrays. RSC Adv. 2017, 7, 17741–17747. [Google Scholar] [CrossRef]
- Petronijević, E.; Leahu, G.; Li Voti, R.; Belardini, A.; Scian, C.; Michieli, N.; Cesca, T.; Mattei, G.; Sibilia, C. Photo-acoustic detection of chirality in metal-polystyrene metasurfaces. Appl. Phys. Lett. 2019, 114, 053101. [Google Scholar] [CrossRef]
- Wang, Y.; Qi, J.; Pan, C.; Wu, Q.; Yao, J.; Chen, Z.; Chen, J.; Li, Y.; Yu, X.; Sun, Q.; et al. Giant circular dichroism of large-area extrinsic chiral metal nanocrecents. Sci. Rep. 2018, 8, 3351. [Google Scholar] [CrossRef] [PubMed]
- Lumerical Solutions, Inc. Available online: http://www.lumerical.com/tcad-products/fdtd/ (accessed on 11 January 2019).
- Huang, C.; Wang, Q.; Zhu, Y. Dual effect of surface plasmons in light transmission through perforated metal films. Phys. Rev. B 2007, 75, 245421. [Google Scholar] [CrossRef]
- Petronijević, E.; Leahu, G.; Mussi, V.; Sibilia, C.; Bovino, A.F. Photoacoustic technique for the characterization of plasmonic properties of 2D periodic arrays of gold nanoholes. AIP Adv. 2017, 7, 025210. [Google Scholar] [CrossRef]
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Petronijevic, E.; Belardini, A.; Leahu, G.; Cesca, T.; Scian, C.; Mattei, G.; Sibilia, C. Circular Dichroism in Low-Cost Plasmonics: 2D Arrays of Nanoholes in Silver. Appl. Sci. 2020, 10, 1316. https://doi.org/10.3390/app10041316
Petronijevic E, Belardini A, Leahu G, Cesca T, Scian C, Mattei G, Sibilia C. Circular Dichroism in Low-Cost Plasmonics: 2D Arrays of Nanoholes in Silver. Applied Sciences. 2020; 10(4):1316. https://doi.org/10.3390/app10041316
Chicago/Turabian StylePetronijevic, Emilija, Alessandro Belardini, Grigore Leahu, Tiziana Cesca, Carlo Scian, Giovanni Mattei, and Concita Sibilia. 2020. "Circular Dichroism in Low-Cost Plasmonics: 2D Arrays of Nanoholes in Silver" Applied Sciences 10, no. 4: 1316. https://doi.org/10.3390/app10041316
APA StylePetronijevic, E., Belardini, A., Leahu, G., Cesca, T., Scian, C., Mattei, G., & Sibilia, C. (2020). Circular Dichroism in Low-Cost Plasmonics: 2D Arrays of Nanoholes in Silver. Applied Sciences, 10(4), 1316. https://doi.org/10.3390/app10041316









