Safety Operation of n-DOF Serial Hydraulic Manipulator in Constrained Motion with Consideration of Contact-Loss Fault
Abstract
Featured Application
Abstract
1. Introduction
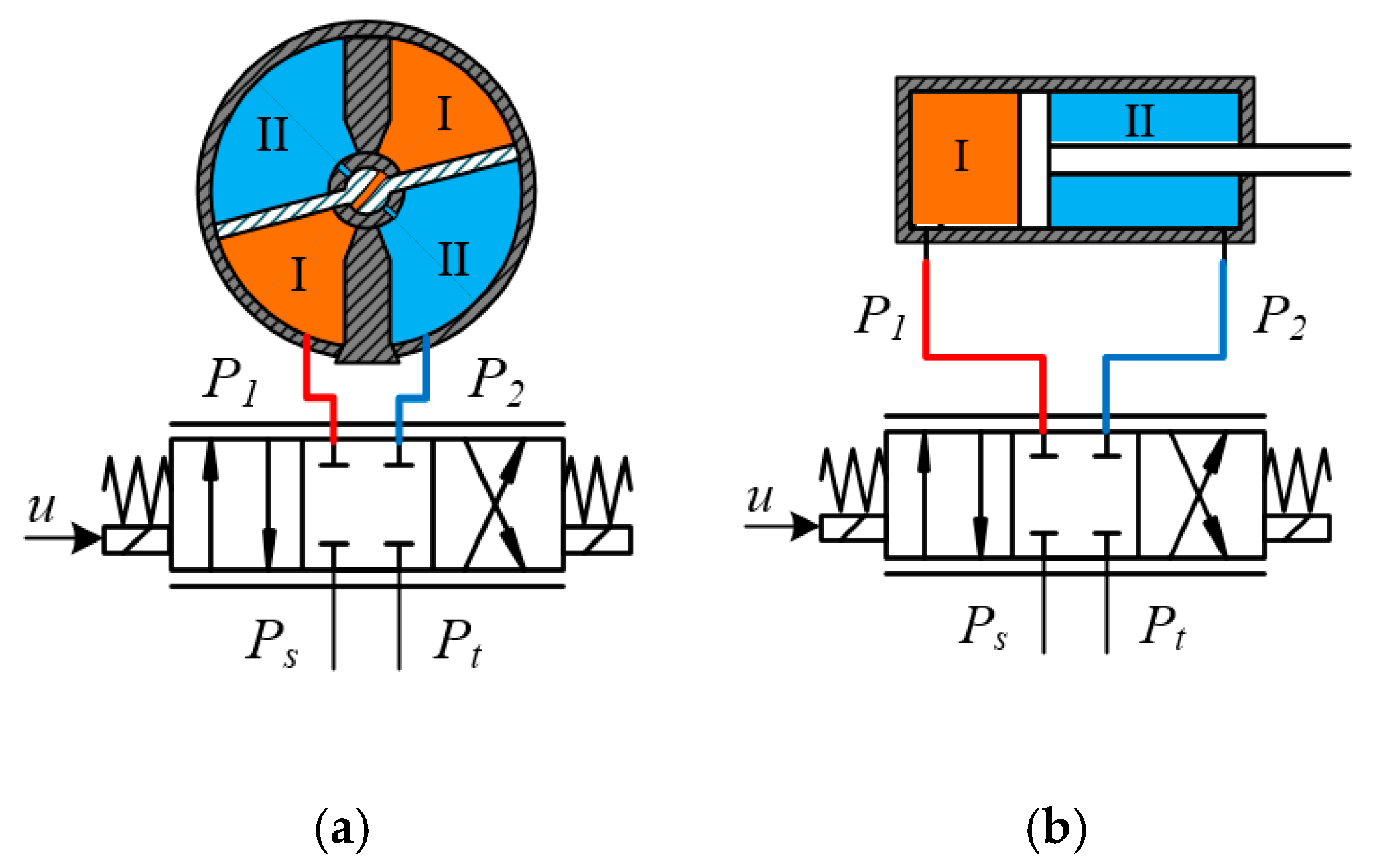
2. System Dynamics including Actuator
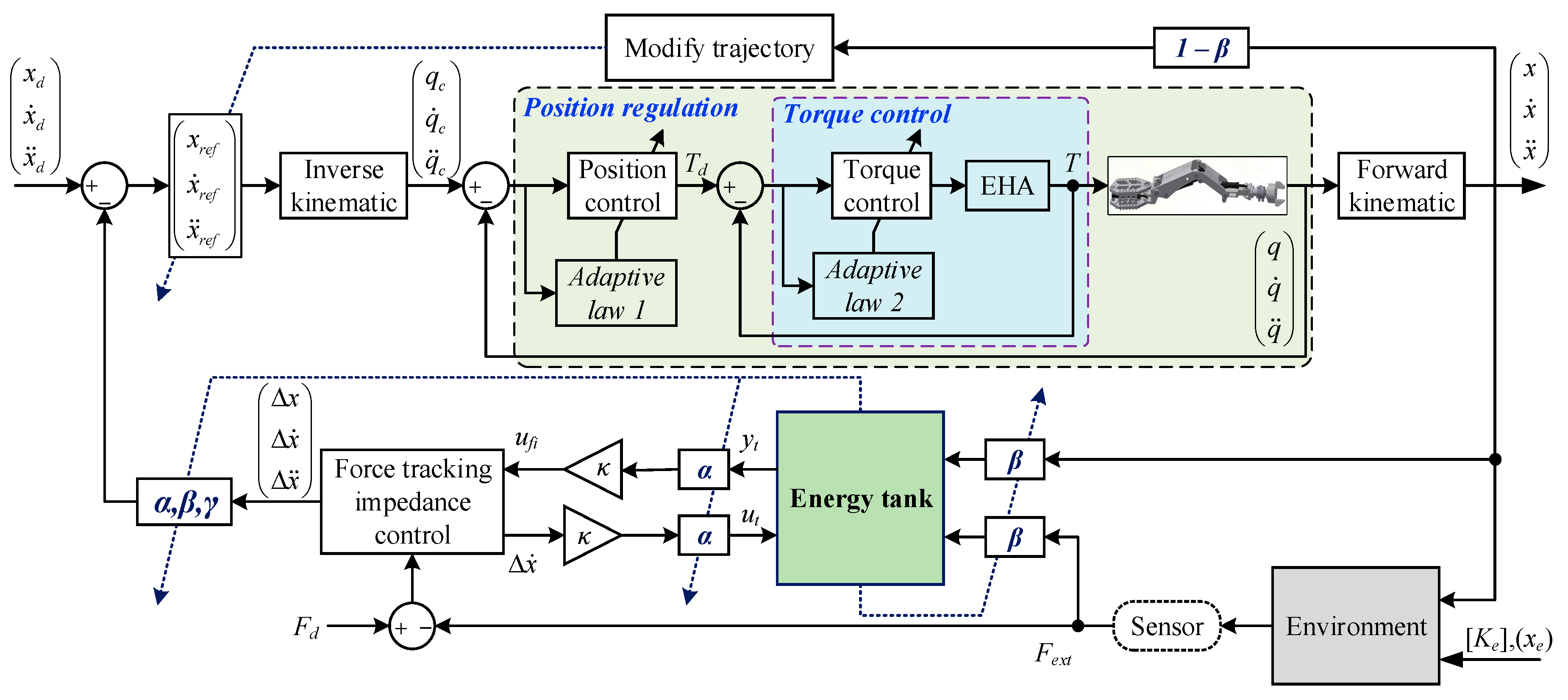
3. Proposed Control Methodology
3.1. Adaptive Backstepping Sliding Mode Algorithm
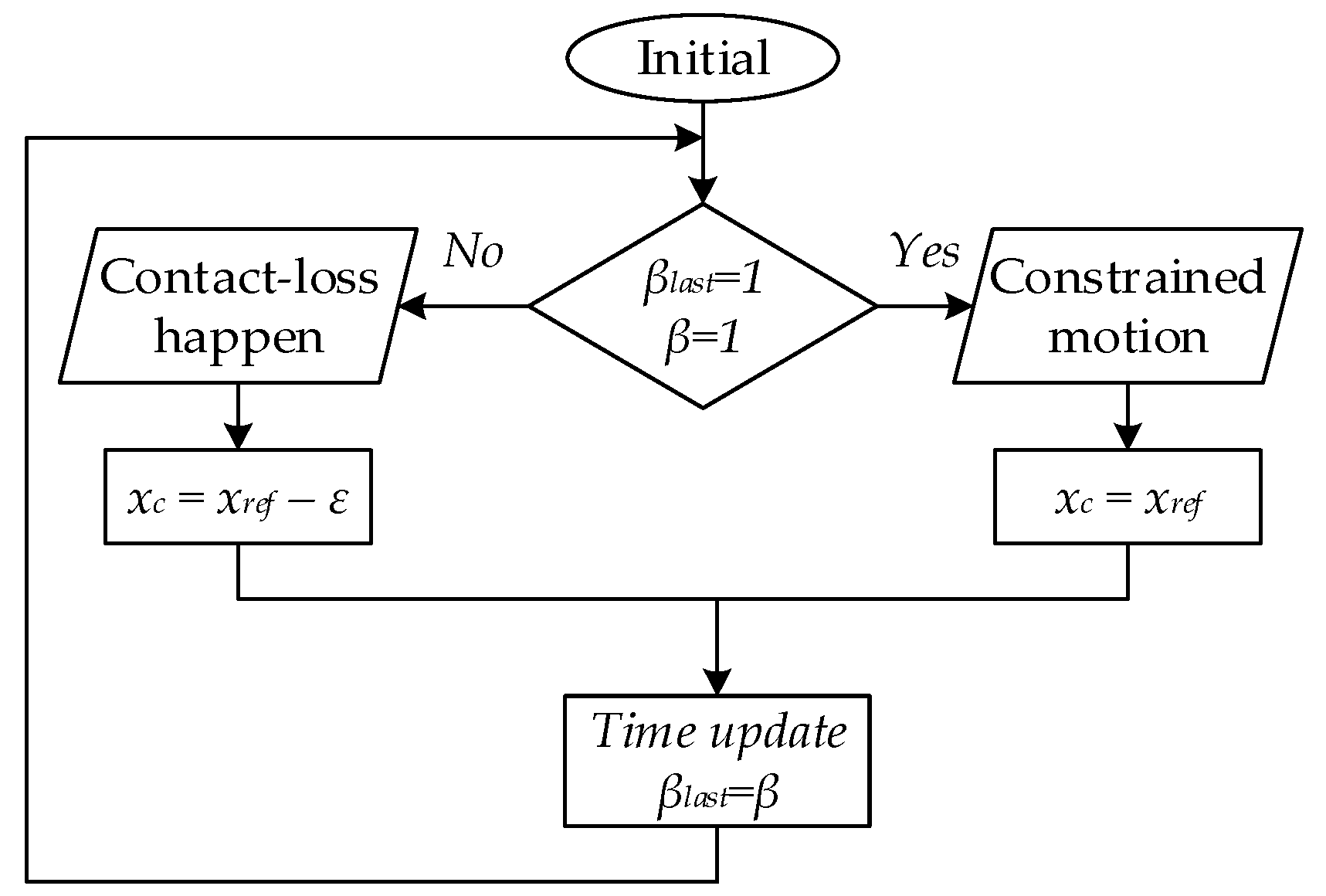
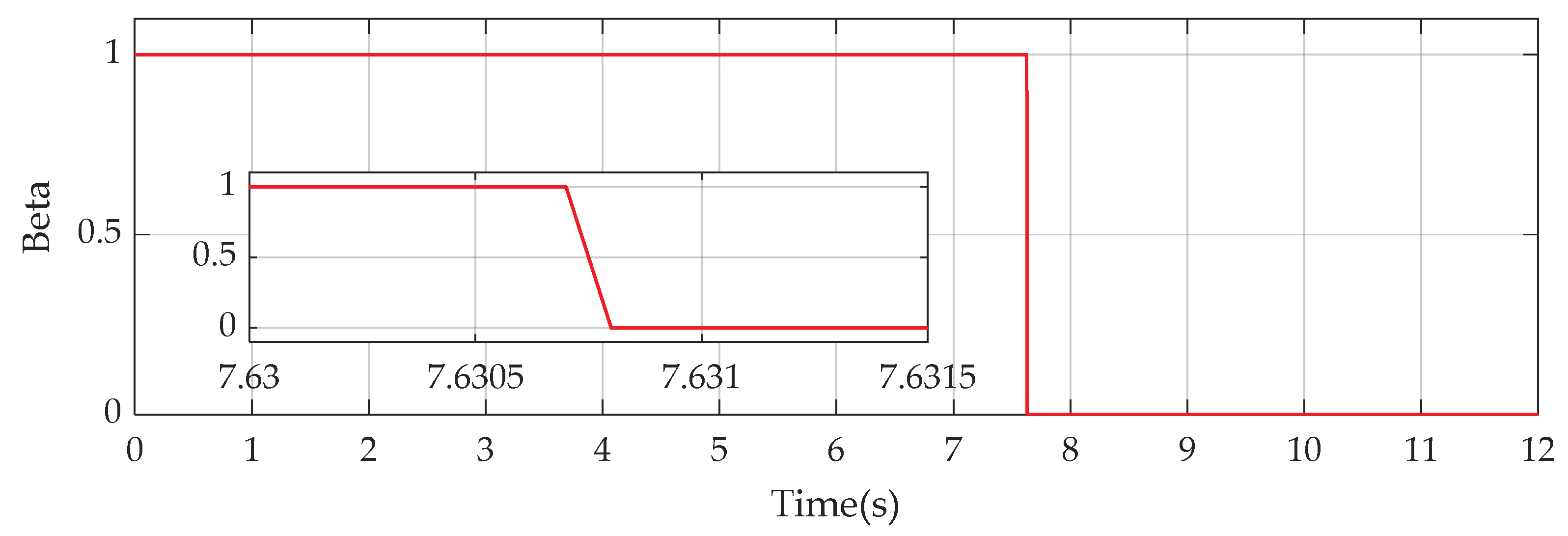
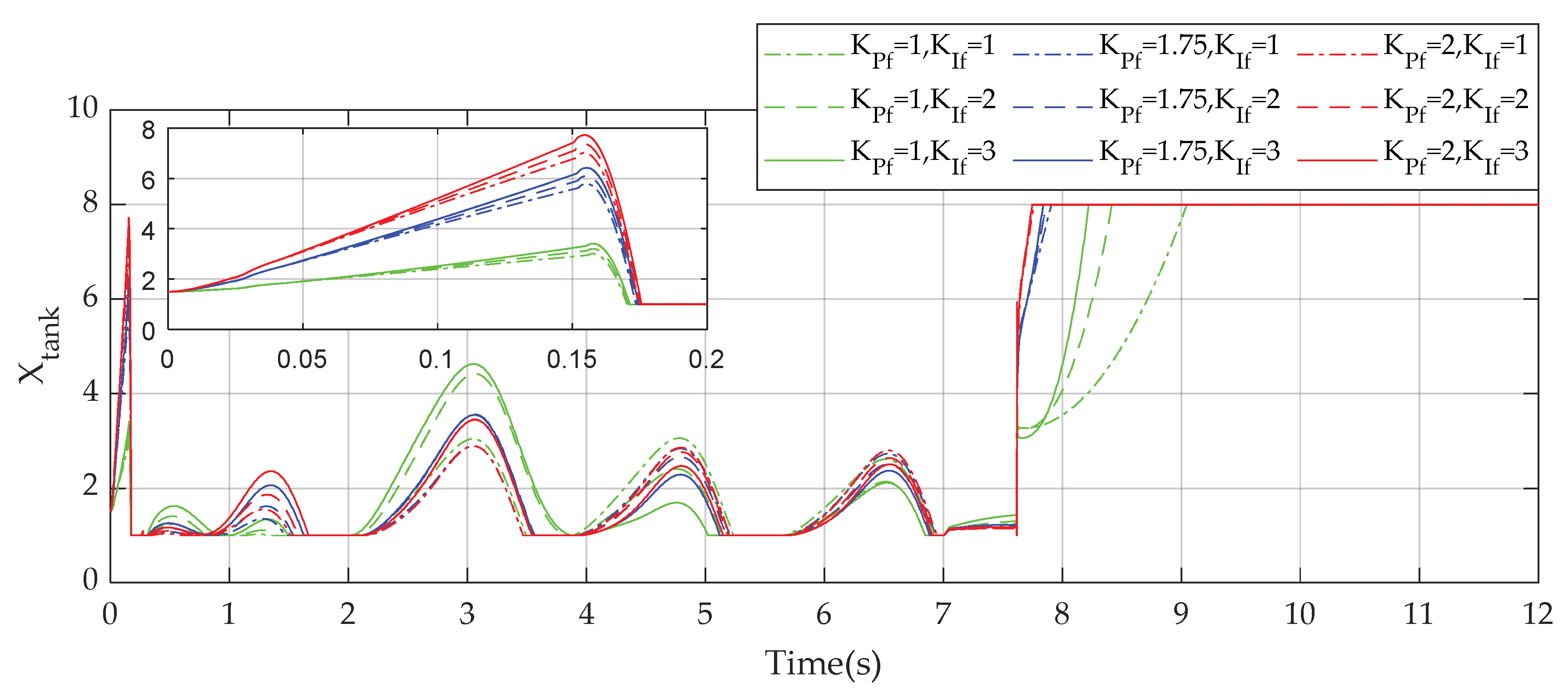
3.2. Fault Detection-Based Virtual Energy Tank
3.3. Modification of Trajectory
4. Simulations
4.1. Configuration of Testing Environments
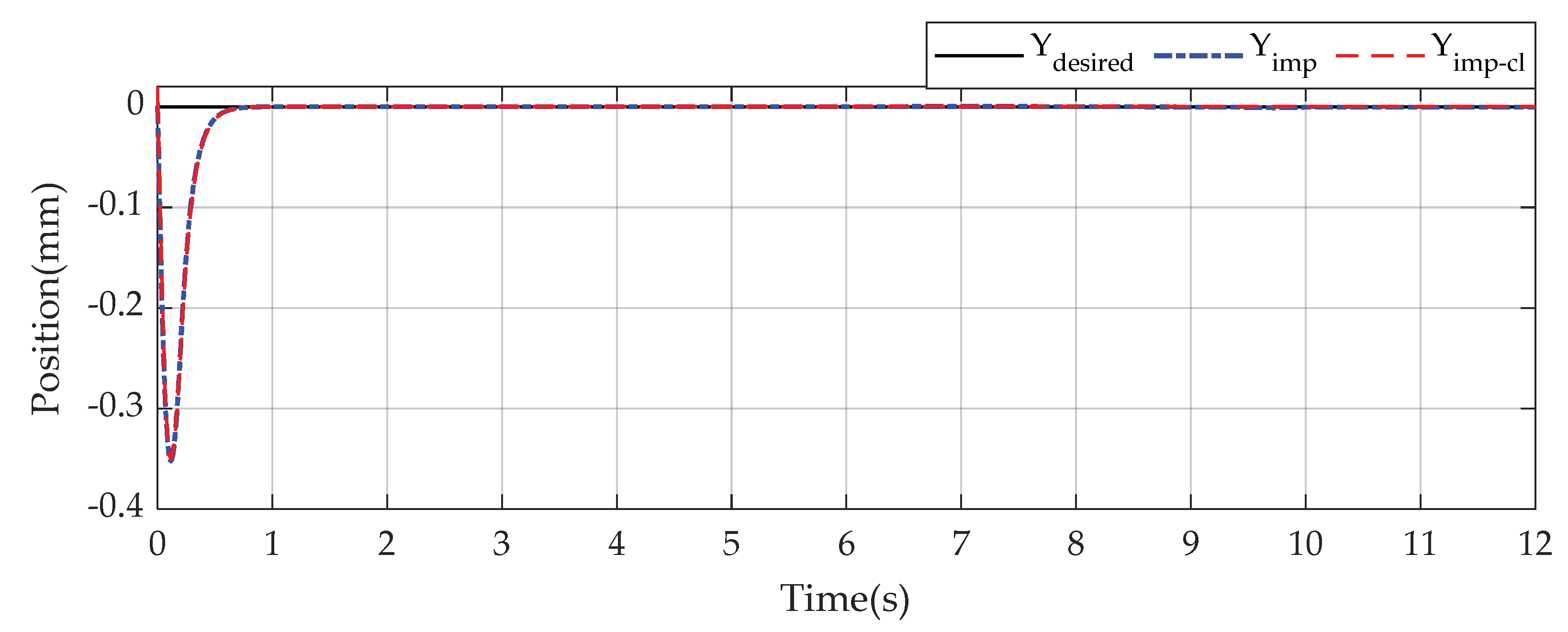
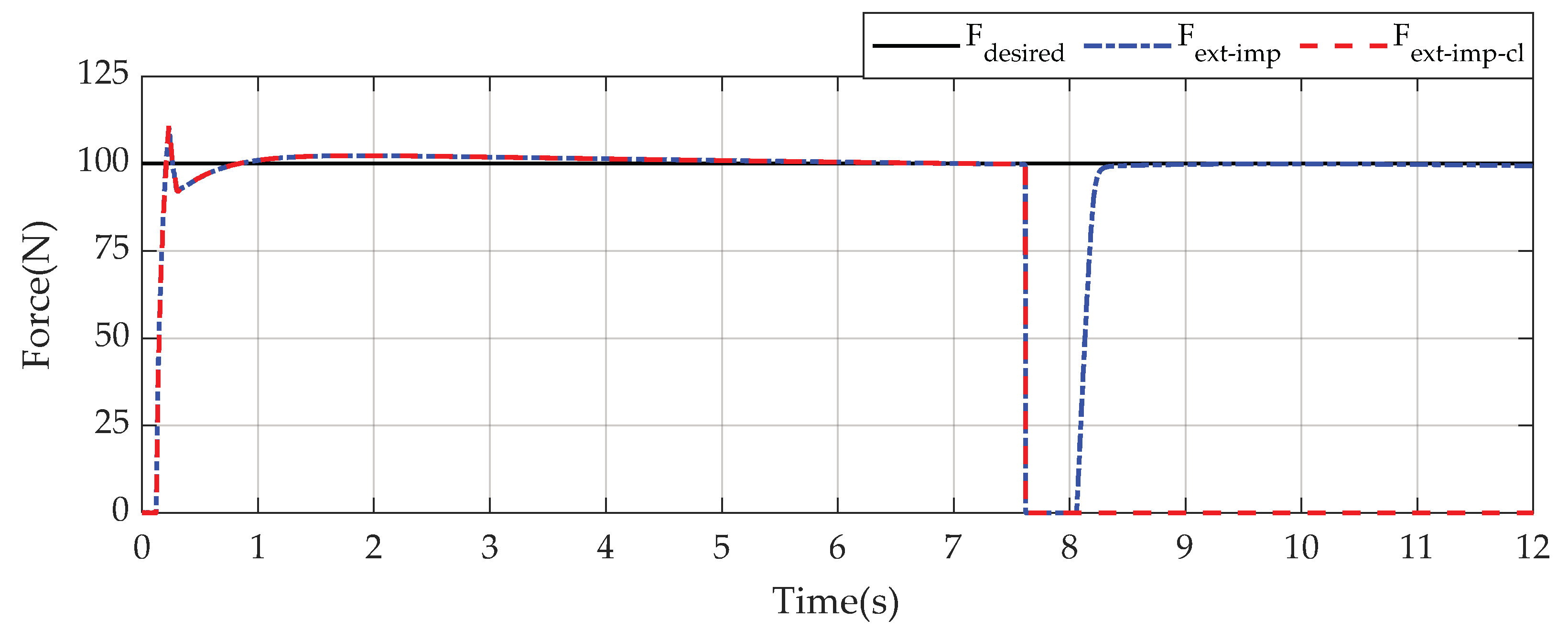
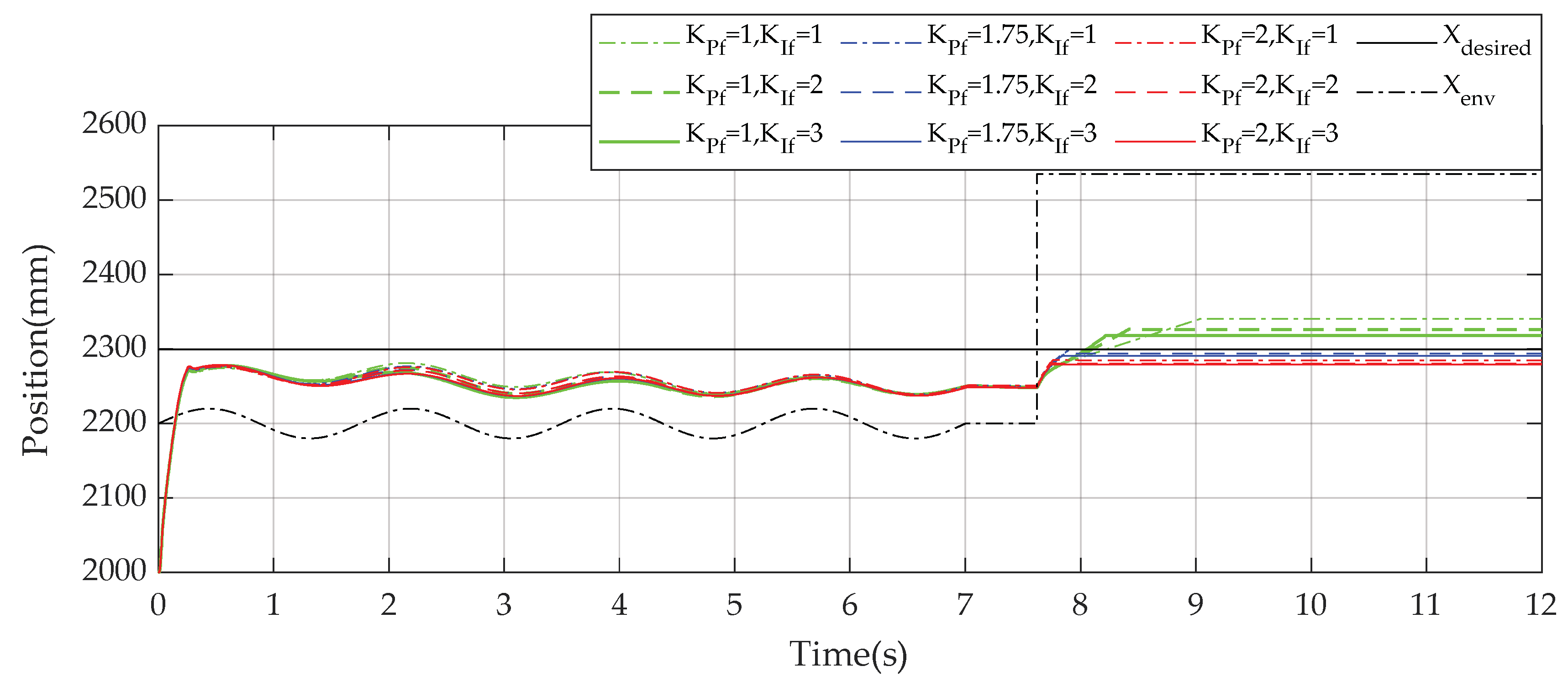
4.2. Simulation Results
5. Conclusions
Author Contributions
Funding
Conflicts of Interest
Appendix A
| ith | ||||
|---|---|---|---|---|
| 1 | 0 | 0 | 0 | |
| 2 | −90 | 200 | 0 | |
| 3 | 0 | 765 | 0 | |
| 4 | 0 | 582 | 0 | |
| 5 | 90 | 340 | 0 | |
| 6 | 90 | 0 | 400 | |
| T | 0 | 0 | 300 | 0 |
References
- Dias, L.A.; de Oliveira Silva, R.W.; da Silva Emanuel, P.C.; Filho, A.F.; Bento, R.T. Application of the Fuzzy Logic for the Development of Autonomous Robot with Obstacles Deviation. Int. J. Control Autom. Syst. 2018, 16, 823–833. [Google Scholar] [CrossRef]
- Li, Z.; Yin, Z. Zhang Dynamics based Tracking Control of Knee Exoskeleton with Time-dependent Inertial and Viscous Parameters. Int. J. Control Autom. Syst. 2018, 16, 904–911. [Google Scholar] [CrossRef]
- Jung, S. Improvement of Tracking Control of a Sliding Mode Controller for Robot Manipulators by a Neural Network. Int. J. Control Autom. Syst. 2018, 16, 937–943. [Google Scholar] [CrossRef]
- Truong, H.V.A.; Tran, D.T.; To, X.D.; Ahn, K.K.; Jin, M. Adaptive Fuzzy Backstepping Sliding Mode Control for a 3-DOF Hydraulic Manipulator with Nonlinear Disturbance Observer for Large Payload. Appl. Sci. 2019, 9, 3290. [Google Scholar] [CrossRef]
- Mahmoodabadi, M.J.; Maafi, R.A.; Taherkhorsandi, M. An optimal adaptive robust PID controller subject to fuzzy rules and sliding modes for MIMO uncertain chaotic systems. Appl. Soft Comput. 2017, 52, 1191–1199. [Google Scholar] [CrossRef]
- Boukattaya, M.; Mezghani, N.; Damak, T. Adaptive nonsingular fast terminal sliding-mode control for the tracking problem of uncertain dynamical systems. ISA Trans. 2018, 77, 1–19. [Google Scholar] [CrossRef] [PubMed]
- Yi, S.; Zhai, J. Adaptive second-order fast nonsingular terminal sliding mode control for robotic manipulators. ISA Trans. 2019, 90, 41–51. [Google Scholar] [CrossRef] [PubMed]
- Makarov, M.; Grossard, M.; Rodríguez-Ayerbe, P.; Dumur, D. Modeling and Preview H∞ Control Design for Motion Control of Elastic-Joint Robots With Uncertainties. IEEE Trans. Ind. Electron. 2016, 63, 6429–6438. [Google Scholar] [CrossRef]
- Tran, D.T.; Truong, H.V.A.; Ahn, K.K. Adaptive Backstepping Sliding Mode Control Based RBFNN for a Hydraulic Manipulator Including Actuator Dynamics. Appl. Sci. 2019, 9, 1265. [Google Scholar] [CrossRef]
- Truong, H.V.A.; Tran, D.T.; Ahn, K.K. A Neural Network Based Sliding Mode Control for Tracking Performance with Parameters Variation of a 3-DOF Manipulator. Appl. Sci. 2019, 9, 2023. [Google Scholar] [CrossRef]
- Do, T.C.; Tran, D.T.; Dinh, T.Q.; Ahn, K.K. Tracking Control for an Electro-Hydraulic Rotary Actuator Using Fractional Order Fuzzy PID Controller. Electronics 2020, 9, 926. [Google Scholar] [CrossRef]
- Shin, J.; Kim, S.; Tsourdos, A. Neural-networks-based Adaptive Control for an Uncertain Nonlinear System with Asymptotic Stability. Int. J. Control Autom. Syst. 2018, 16, 1989–2001. [Google Scholar] [CrossRef]
- Baek, J.; Kwon, W.; Kim, B.; Han, S. A Widely Adaptive Time-Delayed Control and Its Application to Robot Manipulators. IEEE Trans. Ind. Electron. 2019, 66, 5332–5342. [Google Scholar] [CrossRef]
- Lee, J.; Chang, P.H.; Jin, M. An Adaptive Gain Dynamics for Time Delay Control Improves Accuracy and Robustness to Significant Payload Changes for Robots. IEEE Trans. Ind. Electron. 2020, 67, 3076–3085. [Google Scholar] [CrossRef]
- Lee, J.; Chang, P.H.; Yu, B.; Jin, M. An Adaptive PID Control for Robot Manipulators under Substantial Payload Variations. IEEE Access 2020, 8, 162261–192270. [Google Scholar] [CrossRef]
- Tran, D.T.; Jin, M.; Ahn, K.K. Nonlinear Extended State Observer Based on Output Feedback Control for a Manipulator With Time-Varying Output Constraints and External Disturbance. IEEE Access 2019, 7, 156860–156870. [Google Scholar] [CrossRef]
- Tran, D.T.; Do, T.C.; Ahn, K.K. Extended High Gain Observer-Based Sliding Mode Control for an Electro-hydraulic System with a Variant Payload. Int. J. Precis. Eng. Manuf. 2019, 20, 2089–2100. [Google Scholar] [CrossRef]
- Tran, D.T.; Dao, H.V.; Dinh, Q.T.; Ahn, K.K. Output Feedback Control via Linear Extended State Observer for an Uncertain Manipulator with Output Constraints and Input Dead-Zone. Electronics 2020, 9, 1355. [Google Scholar] [CrossRef]
- Dang, X.B.; Dinh, Q.T.; Bae, J.; Ahn, K.K. An Effective Disturbance-Observer-Based Nonlinear Controller for a Pump-Controlled Hydraulic System. IEEE/ASME Trans. Mechatron. 2020, 25, 32–43. [Google Scholar] [CrossRef]
- Liu, P.; Yu, H.; Cang, S. Trajectory Synthesis and Optimization of an Underactuated Microrobotic System with Dynamic Constraints and Couplings. Int. J. Control Autom. Syst. 2018, 16, 2373–2383. [Google Scholar] [CrossRef]
- Yoon, H.J.; Chung, S.Y.; Hwang, M.J. Shadow Space Modeling and Task Planning for Collision-free Cooperation of Dual Manipulators for Planar Task. Int. J. Control Autom. Syst. 2019, 17, 995–1006. [Google Scholar] [CrossRef]
- Hu, J.; Xiong, R. Contact Force Estimation for Robot Manipulator Using Semiparametric Model and Disturbance Kalman Filter. IEEE Trans. Ind. Electron. 2018, 65, 3365–3375. [Google Scholar] [CrossRef]
- Chan, L.; Naghdy, F.; Stirling, D. Extended active observer for force estimation and disturbance rejection of robotic manipulators. Robot. Auton. Syst. 2013, 61, 1277–1287. [Google Scholar] [CrossRef]
- Lin, Y.; Zhao, H.; Ding, H. External Force Estimation for Industrial Robots with Flexible Joints. IEEE Robot. Autom. Lett. 2020, 5, 1311–1318. [Google Scholar] [CrossRef]
- He, W.; Dong, Y.T.; Sun, C.Y. Adaptive Neural Impedance Control of a Robotic Manipulator with Input Saturation. IEEE Trans. Syst. Man Cybern. Syst. 2016, 46, 334–344. [Google Scholar] [CrossRef]
- Ravandi, A.K.; Khanmirza, E.; Daneshjou, K. Hybrid force/position control of robotic arms manipulating in uncertain environments based on adaptive fuzzy sliding mode control. Appl. Soft Comput. 2018, 70, 864–874. [Google Scholar] [CrossRef]
- Yang, Z.; Peng, J.Z.; Liu, Y.H. Adaptive neural network force tracking impedance control for uncertain robotic manipulator based on nonlinear velocity observer. Neurocomputing 2019, 331, 263–280. [Google Scholar] [CrossRef]
- Coutinho, F.; Cortesão, R. Online stiffness estimation for robotic tasks with force observers. Control Eng. Pract. 2014, 24, 92–105. [Google Scholar] [CrossRef]
- Sariyildiz, E.; Ohnishi, K. On the Explicit Robust Force Control via Disturbance Observer. IEEE Trans. Ind. Electron. 2015, 62, 1581–1589. [Google Scholar] [CrossRef]
- Wahrburg, A.; Bös, J.; Listmann, K.D.; Dai, F.; Matthias, B.; Ding, H. Motor-Current-Based Estimation of Cartesian Contact Forces and Torques for Robotic Manipulators and Its Application to Force Control. IEEE Trans. Autom. Sci. Eng. 2018, 15, 879–886. [Google Scholar] [CrossRef]
- Ohba, Y.; Saza, M.; Ohishi, K.; Asai, T.; Majima, K.; Yoshizawa, Y.; Kageyama, K. Sensorless Force Control for Injection Molding Machine Using Reaction Torque Observer Considering Torsion Phenomenon. IEEE Trans. Ind. Electron. 2009, 56, 2955–2960. [Google Scholar] [CrossRef]
- Jung, S.; Hsia, T.C. Neural Network Impedance Force Control of Robot Manipulator Impedance. IEEE Trans. Ind. Electron. 1998, 45, 451–461. [Google Scholar] [CrossRef]
- Kronander, K.; Billard, A. Stability Considerations for Variable Impedance Control. IEEE Trans. Robot. 2016, 32, 1298–1305. [Google Scholar] [CrossRef]
- Ferraguti, F.; Secchi, C.; Fantuzzi, C. A tank-based approach to impedance control with variable stiffness. In Proceedings of the 2013 IEEE International Conference on Robotics and Automation (ICRA), Karlsruhe, Germany, 6–10 May 2013; pp. 4948–4953. [Google Scholar] [CrossRef]
- Schindlbeck, C.; Haddadin, S. Unified Passivity-Based Cartesian Force/Impedance Control for Rigid and Flexible Joint Robots via Task-Energy Tanks. In Proceedings of the 2015 IEEE International Conference on Robotics and Automation (ICRA), Seattle, WA, USA, 26–30 May 2015; pp. 440–447. [Google Scholar] [CrossRef]
- Toedtheide, A.; Shahriari, E.; Haddadin, S. Tank based unified torque/impedance control for a pneumatically actuated antagonistic robot joint. In Proceedings of the 2017 IEEE International Conference on Robotics and Automation (ICRA), Singapore, 29 May–3 June 2017; pp. 1255–1262. [Google Scholar] [CrossRef]
- Lee, D.J.; Huang, K. Passive-set-point modulation framework for interactive robotic systems. IEEE Trans. Robot. 2010, 26, 354–369. [Google Scholar]
- Franken, M.; Stramigioli, S.; Misra, S.; Secchi, C.; Macchelli, A. Bilateral telemanipulation with time delays: A two-layer approach combining passivity and transparency. IEEE Trans. Robot. 2011, 27, 741–756. [Google Scholar] [CrossRef]
- Franchi, A.; Secchi, C.; Son, H.I.; Bulthoff, H.H.; Gior-dano, P.R. Bilateral Teleoperation of Groups of Mobile Robots With Time-Varying Topology. IEEE Trans. Robot. 2012, 28, 1019–1033. [Google Scholar] [CrossRef]
- Feio, J.; Martins, J.M.; Sá da Costa, J. Variable Impedance Control of Manipulator Robots Applied to Orthopedic Surgery. In Proceedings of the 7th Workshop on Advanced Control and Diagnosis, Zielona Gra, Poland, 17–18 November 2009. [Google Scholar]
- Kordasz, M.; Kuczkowski, K.; Sauer, P. Study on Possible Control Algorithms for Lower Limb Rehabilitation System. In Proceedings of the 2011 IEEE International Conference on Rehabilitation Robotics, Zurich, Switzerland, 29 June–1 July 2011. [Google Scholar] [CrossRef]
- Ferraguti, F.; Preda, N.; Manurung, A.; Bonfè, M.; Lambercy, O.; Gassert, R.; Muradore, R.; Fiorini, P.; Secchi, C. An Energy Tank-Based Interactive Control Architecture for Autonomous and Teleoperated Robotic Surgery. IEEE Trans. Robot. 2015, 31, 1073–1088. [Google Scholar] [CrossRef]
- Tadele, T.S.; de Vries, T.J.A.; Stramigioli, S. Combining energy and power based safety metrics in controller design for domestic robots. In Proceedings of the 2014 IEEE International Conference on Robotics and Automation (ICRA), Hong Kong, China, 31 May–7 June 2014; pp. 1209–1214. [Google Scholar]
- John, J.C. Introduction to Robotics Mechanics and Control, 3rd ed.; Addison-Wisley: Reading, PA, USA; Pearson Education Inc.: Upper Saddle River, NJ, USA, 1989. [Google Scholar]
- To, X.D.; Tran, D.T.; Truong, H.V.A.; Ahn, K.K. Disturbance Observer Based Finite Time Trajectory Tracking Control for a 3 DOF Hydraulic Manipulator Including Actuator Dynamics. IEEE Access 2018, 6, 36798–36809. [Google Scholar]
- Levant, A. Robust exact differentiation via sliding mode technique. Automatica 1998, 34, 379–384. [Google Scholar] [CrossRef]
- Levant, A. Higher-order sliding modes, differentiation and output feedback control. Int. J. Control 2003, 76, 924–941. [Google Scholar] [CrossRef]
- Van, M.; Ge, S.S.; Ren, H. Finite time fault tolerant control for robot manipulators using time delay estimation and continuous nonsingular fast terminal sliding mode control. IEEE Trans. Cybern. 2017, 47, 1681–1693. [Google Scholar] [CrossRef] [PubMed]
- Duan, J.; Gan, Y.; Chen, M.; Dai, X. Adaptive variable impedance control for dynamic contact force tracking in uncertain environment. Robot. Auton. Syst. 2018, 102, 54–65. [Google Scholar] [CrossRef]
- Albu-Schaffer, A.; Ott, C.; Hirzinger, G. A unified passivity-based control framework for position, torque and impedance control of flexible joint robots. Int. J. Robot. Res. 2007, 26, 23–39. [Google Scholar] [CrossRef]
- Jung, E.J.; Kim, J.H.; Jin, M. Kinematic Analysis of Hydraulic Manipulators for a Disaster Response Robot. In Proceedings of the 14th International Conference on Ubiquitous Robots and Ambient Intelligence (URAI), Jeju, Korea, 28 June–1 July 2017; pp. 208–209. [Google Scholar]







| Symbol | Description | Value |
|---|---|---|
| β | Bulk modulus | 1.5 (Gpa) |
| Drag coefficient | 0.62 | |
| ρ | Fluid density | 861 (kg/m3) |
| Supply pressure | 200 (bar) | |
| Tank pressure | 3 (bar) | |
| ω | Valve orifice area gradient | 0.0024 |
| Valve gain |
| Actuator ith | Bore Diameter | Rod Diameter | Stroke | |
|---|---|---|---|---|
| Cylinder | 1 | 70 (mm) | 45 (mm) | 192 (mm) |
| 2 | 80 (mm) | 50 (mm) | 180 (mm) | |
| 3 | 80 (mm) | 50 (mm) | 200 (mm) | |
| 4 | 70 (mm) | 45 (mm) | 175 (mm) | |
| 5 | 63 (mm) | 40 (mm) | 100 (mm) | |
| Rotary actuator | 6 | (m3/rad) | (m3/rad) | (rad) |
| Symbols | Value | Symbols | Value |
|---|---|---|---|
| 2000 (N/m) | 32 (J) | ||
| 10−3 × eye(3) | 0.1 (J) | ||
| 0.1 × eye(3) | 0.1 (mm) | ||
| 0.2 × eye(3) | 0.01 (mm) | ||
| 2 × eye(3) | |||
| 3 × eye(3) |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Truong, H.V.A.; Trinh, H.A.; Ahn, K.K. Safety Operation of n-DOF Serial Hydraulic Manipulator in Constrained Motion with Consideration of Contact-Loss Fault. Appl. Sci. 2020, 10, 8107. https://doi.org/10.3390/app10228107
Truong HVA, Trinh HA, Ahn KK. Safety Operation of n-DOF Serial Hydraulic Manipulator in Constrained Motion with Consideration of Contact-Loss Fault. Applied Sciences. 2020; 10(22):8107. https://doi.org/10.3390/app10228107
Chicago/Turabian StyleTruong, Hoai Vu Anh, Hoai An Trinh, and Kyoung Kwan Ahn. 2020. "Safety Operation of n-DOF Serial Hydraulic Manipulator in Constrained Motion with Consideration of Contact-Loss Fault" Applied Sciences 10, no. 22: 8107. https://doi.org/10.3390/app10228107
APA StyleTruong, H. V. A., Trinh, H. A., & Ahn, K. K. (2020). Safety Operation of n-DOF Serial Hydraulic Manipulator in Constrained Motion with Consideration of Contact-Loss Fault. Applied Sciences, 10(22), 8107. https://doi.org/10.3390/app10228107






