1. Introduction
The corrosion of rebars accelerates when cracking occurs in reinforced concrete (RC) members. Cracking in RC is a phenomenon that cannot be avoided under tensile or flexural loading. Many types of research have been performed on the width of cracks in RC members [
1,
2,
3,
4,
5,
6,
7,
8,
9]. Cracking occurs because of exceeding the concrete tension strain from the maximum tension capability. Therefore, rebars endure the tension force beyond what caused the cracks [
10,
11,
12,
13,
14]. In many theories, slip is ignored at the first stage of cracking [
11,
12,
13,
14]. Therefore, the sections do not remain in place in the crack section.
Arora and Singh [
15] studied the flexural performance of a concrete beam made with 100% recycled coarse aggregate (RCA) that was subjected to fatigue failure. The results were compared with those of natural coarse aggregate (NCA) concrete beams. They revealed that using 100% RCA in concrete in the place of NCA resulted in poor fatigue performance. Choi and Yun [
16] studied the long-term deflection and flexural performance of reinforced concrete beams with RCA. The specimens were loaded for 360 days. Furthermore, the experiments were compared with ACI 318 analytical outcomes and a new model was proposed to calculate the long-term flexural deflections. Similar crack patterns were observed, regardless of the aggregate type, even though several cracks occurred in the beams made with recycled aggregate. Azad [
17] studied the flexural behaviour of reinforced concrete beams made with recycled waste materials. In this study, polyethylene terephthalate (PET) wastes were used as a recycled material. The results indicated that PET waste could be used in concrete in proportions up to 15%. Gao and Zhang [
18] investigated the flexural behaviour of steel fibres (SF) RCA concrete. The flexural strength, toughness and deflection dramatically improved by increasing the SF content. Based on previous studies, it is clear that adding SFs to RCA concrete beams is an effective method for improving their performance. Seara-Paz et al. [
19] evaluated the influence of RCA on the flexural performance of reinforced concrete beams. The specimens were subjected to four-point loading after 28 days. The cracking behaviour showed differences between RCA and conventional concrete. Therefore, it is possible that using SFs could enhance the bending performance of RCA beams. Tošic et al. [
20] compared the flexural performance of RCA-reinforced concrete with the Eurocode equations, based on 217 experimental specimens. They found that Eurocode 2 could anticipate the bending and shear resistance of RCA concrete beams without stirrups. Tarek et al. [
21] used brick as the RCA to manufacture specimens. The results were compared with the proposed ACI 318-14 model to predict the flexural performance of the concrete beams. In comparison with virgin brick aggregate, the use of RCA did not reduce the cracking moment nor the maximum load-bearing capacity of the specimens. Zaetang et al. [
22] used RCA at 0%, 20%, 40%, 60%, 80% and 100% by weight to produce concrete. Although the RCA beams were weaker than the NCA beams, improvements in the strength and abrasion resistance were obtained because of better bonding between the RCA and the cement paste due to the increased surface porosity and roughness of RCA.
Conversely, SFs offer a solution for reducing crack propagation. In some studies, the shear cracking in RC beams was investigated by using SFs only, a shear rebars ratio and the combination of stirrups and SFs [
23,
24,
25,
26]. Furthermore, the post-cracking stress transferability in concrete increases by increasing the SF content [
27,
28,
29,
30,
31,
32]. To evaluate the correct potential of the fibres, the transferred post-cracking stress through cracks must be considered by including an additional contributing term [
33]. The slip occurred in tension rebars. Therefore, the load–displacement relationship can be measured as a criterion to study cracking [
34,
35,
36,
37,
38,
39,
40]. Additionally, high SF contents improve the tensile strength of concrete members by improving the bond resistance between the concrete and rebars [
41]. Conversely, if an insufficient SF content is used, the tensile strength is not considerably enhanced [
42]. In 2008, Kim et al. [
43] investigated the performance of FRC beams with four kinds of fibres and two SF contents (0.4 and 1.2%). According to the results, high strength steel twisted fibres perform significantly better than other SF in a higher strength matrix. Altun and Aktas [
44] evaluated the influence of SF on the bending behaviour of lightweight concrete beams. Therefore, cracks propagate faster in RC members manufactured using lightweight concrete than in normal and high-strength concrete. It was shown that SFs increase the toughness capacity of prismatic concrete beams and their ductility. Yoo et al. [
45] studied the post-cracking performance of normal and high-strength SF-reinforced concrete beams. The flexural strength, post-peak ductility and deflection capacity significantly increased by using a 1% SF content.
Mertol et al. [
46] evaluated the effect of SF contents on the flexural behaviour of RC beams. For over-reinforced specimens, the post-peak stiffness of the beams was significantly lower than that of normal-weight concrete specimens. Patil and Single [
47] tested SF reinforced concrete beams under predominant torsion and bending. They showed an improvement in the torsional strength, combined torsional–shear–bending strength and crack strength of the concrete when SFs were used. Luccioni et al. [
48] investigated the static and dynamic behaviour of SF RC beams. Improvements were found in the static bending response with different fibre contents compared to those conducted under dynamic loading. Lee et al. [
49] determined the structural response of SF RC beams under different loading values. The results indicated that the use of SFs improved the ductility of the beams regardless of the strain rate. Furthermore, the shear strength of the SF-reinforced beams substantially improved. RCA generally represents a way to change a waste creation into a reserve. Consequently, utilising demolished supplies as an alternative to natural ones could be a beneficial technique to keep the environment safe. RCA has widely been utilised in non-structural concrete elements and lower feature uses, such as back-fills and lower layers of roads, and natural aggregates have been used in a wide range of structures [
50]. Alternatively, SFs not only delay the creation of cracks but also control their growth, and when combined with the post-crack bridging capability of fibres, SFs diminish the width of cracks when concrete is restrained [
51]. In the hardened state, the interaction between SFs and mortar plays a bridging role in order to delay the widening of cracks. Therefore, if sufficiently high contents of SFs are provided in the specimens, the tension resistance of the concrete matrix will substantially increase. Furthermore, the bridging role of SFs in the mortar resulted in obtaining the composite performance of concrete that causes a transition from micro-cracks to macro-cracks and prevents increasing the cracks’ width. In concrete with a low fibre content, the fibres do not meaningfully enhance the tension and bending resistance of the concrete. Alternatively, in concrete containing a high SF content, the benefits of fibre reinforcement include raising the tension resistance, the strain-hardening response before cracking and a boosted stiffness beyond crack instalment [
51]. When the member with fibres undergoes bending, their presence leads to enhancing the shear behaviour of the RC member. Furthermore, using fibres at the cracks’ location causes a postponement of the crack extension [
52].
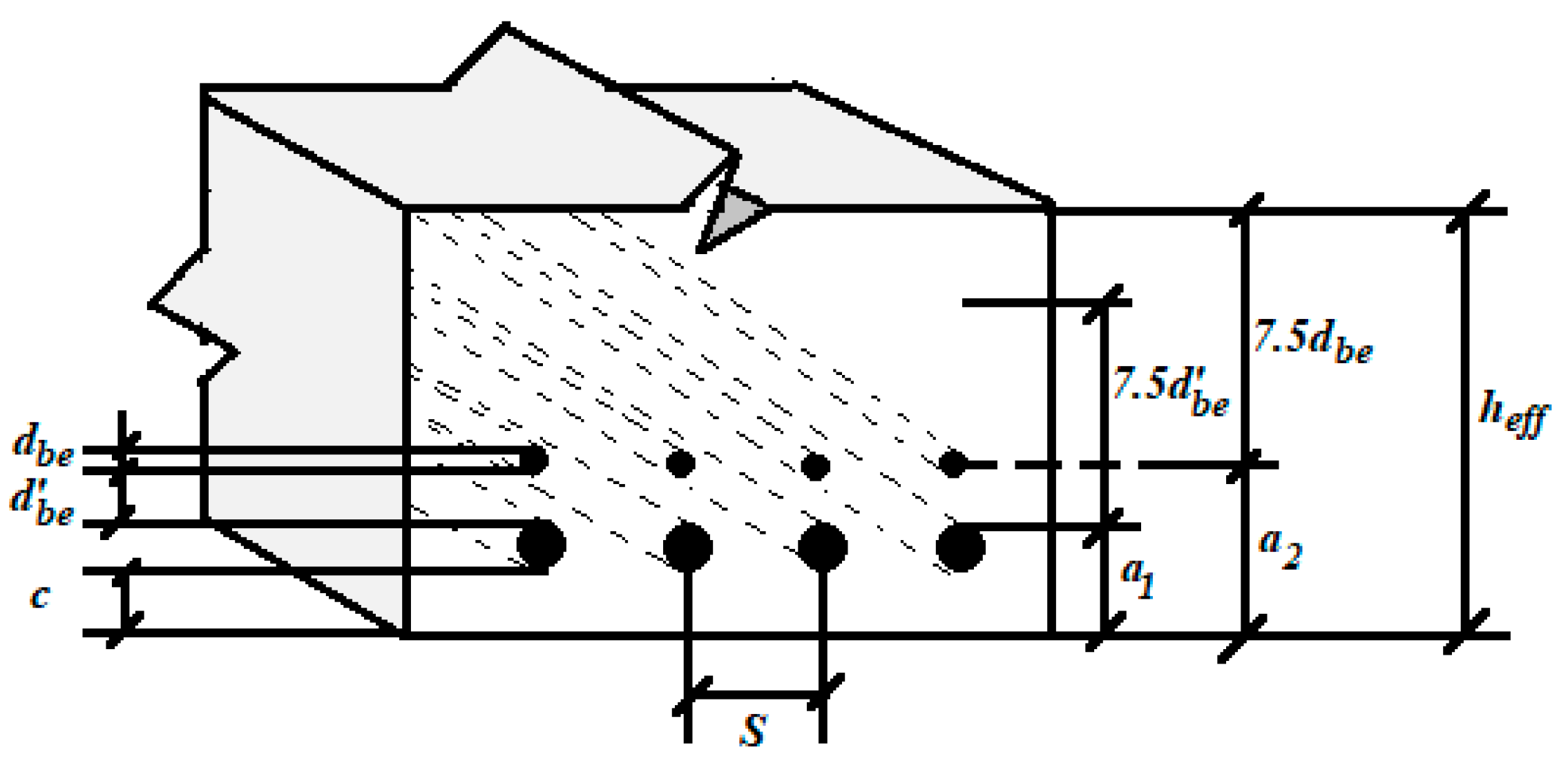
Different standards suggest different equations to predict the crack spacing and width in RC beams. According to CSA S474 [
53], the average crack distance
is calculated as follows:
where
c,
s,
b,
and
are the reinforcement cover (mm), outer rebar’s layer distance (mm), cross-section width (mm), rebar diameter (mm) and outer area of the tension rebar’s layer (mm
2), respectively. Furthermore,
refers to the participation rate and boundary condition coefficients for rebars, which are considered equal to 0.4 and 0.8 for smooth and conventional rebars, respectively;
is the strain coefficient, which is equal to
, where
and
are the highest and lowest effective strains in the tensioned area, respectively. Moreover,
is the effective tensioned depth (mm) and is considered equal to the largest amount of either
or
. Furthermore, it should not be much larger than the tensioned area or half the height of the section, according to CSA S474 [
53]. Furthermore, it should not be much larger than the area of the tension or half the thickness as shown in
Figure 1.
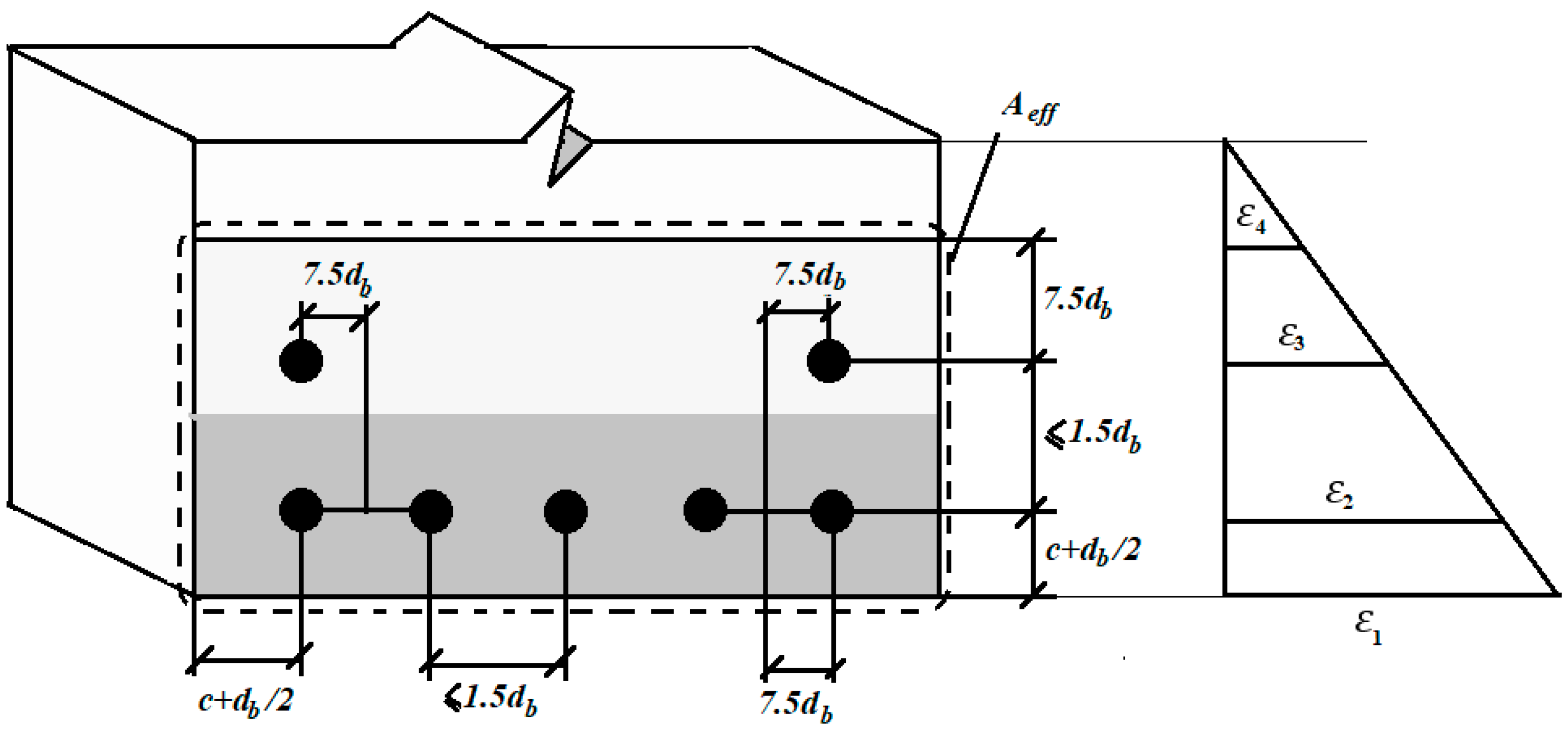
NS 3473E [
54] presents a similar equation for calculating the average crack distance, as follows:
where
c and
s are the concrete cover’s and the outer rebar layer’s distance (mm), respectively.
is an environmental factor that is based on the rebar’s corrosion, which is equal to 0.4 for ribbed rebars;
is a strain factor equal to
where
and
are the highest and the lowest effective strains in the tensioned area, respectively.
is the effective rebar’s ratio, equal to
, where
is the effective cross-section area of concrete and
is the area of the tensile rebars (
Figure 2).
CSA S474 [
53] and NS 3473E [
54] propose a similar formula to anticipate the crack spacing, with little variance in the slope of strain, which calculates the effective rebar’s section. According to NS 3473E [
54], the crack spacing is determined using the following relationship.
where the correction coefficient
r represents the plasticisers’ impact on the hardened concrete’s strain.
is the tensile strain in the outer tensile rebar’s layer, according to a non-axial stress distribution, with the main stress direction parallel to the longitudinal reinforcement,
.
,
and
are the steel rebar’s stress, the steel rebar’s modulus of elasticity and the average distance between cracks, according to Equation (2), respectively. Furthermore,
β is the conditional factor, which is defined according to the environment condition (in a natural condition with no humidity, it is considered equal to 1), and
is equal to 0.4 for ribbed rebars. CSA-S474-04 [
53] recommends calculating the average crack spacing according to average crack spacing as in Equation (1). According to EC2 [
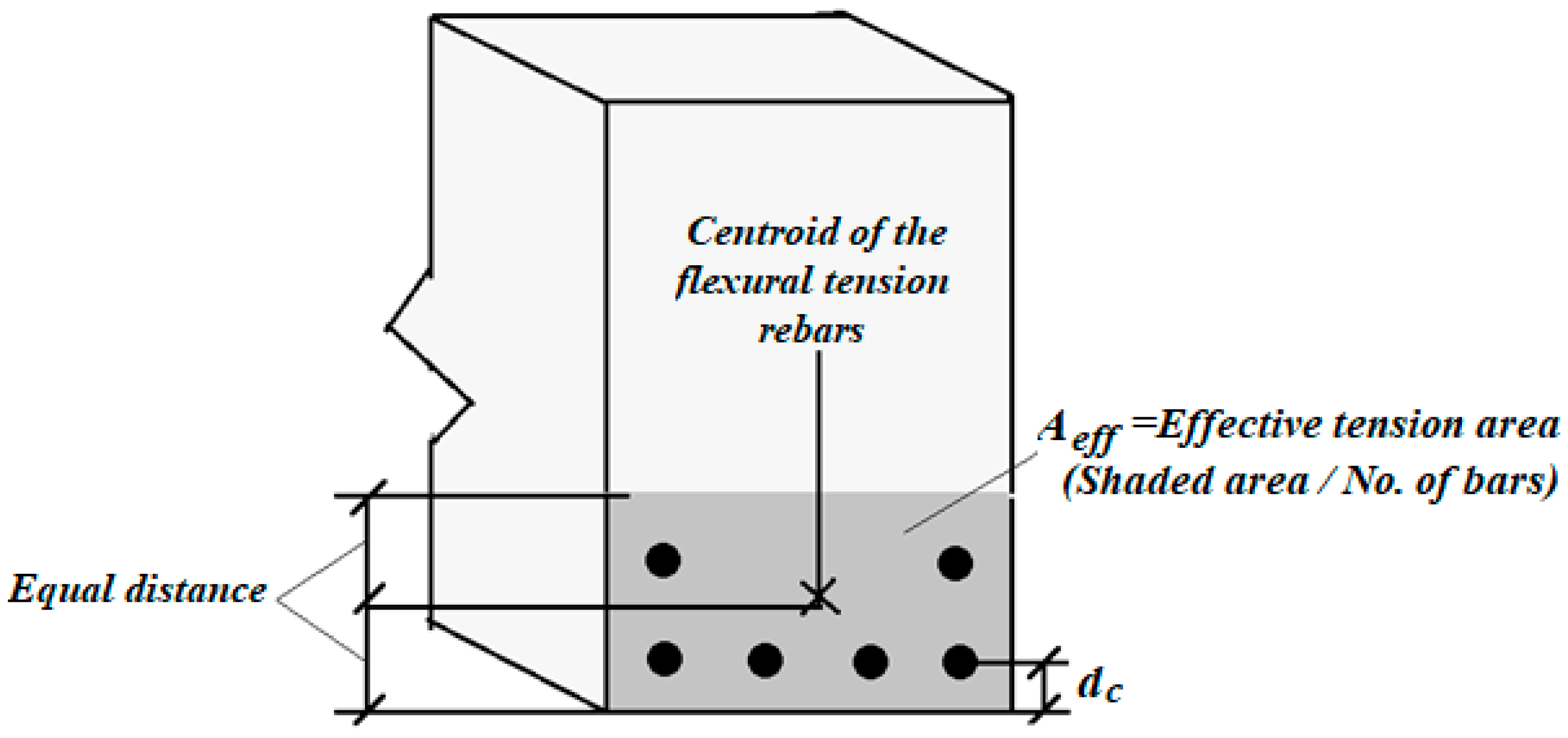
55], the concrete cover is the first parameter that affects the flexural crack’s spacing. According to this standard, the average flexural crack’s spacing can be obtained using the following formula:
where
C and
are the reinforcement cover and diameter (mm), respectively.
is the effective cross-section rebar’s ratio.
is equal to 0.8 and 1.6 for ribbed and smooth rebars, respectively.
is equal to 0.5 for members under bending and 1.0 for those under pure torsion (
Figure 3).
The crack spacing
is calculated as follows:
where
is the design crack spacing,
the crack spacing according to Equation (3) and
ξ is a dimensionless coefficient between 0 and 1.
is the average strain under external load combinations.
is the average cracking ratio for design purposes
CEB-FIP [
56] suggests a different formula for predicting the crack spacing. According to this standard, the crack spacing can be obtained utilising the formula below:
where
is the length of tension rebar’s slip, which can be calculated using Equations (8) and (9) for the complete cracking and first crack, respectively:
where
is the rebars’ tension stress at cracking (MPa);
is the rebar’s diameter (mm);
is the mean value of the boundary stress (MPa), which is equal to
, where
is the average value of concrete’s tensile strength at cracking (MPa).
is the effective rebar’s area, equal to
, where
and
are the longitudinal rebar’s area and the concrete’s effective cross-sectional area, respectively. Furthermore, to simplify Equation (9), the value of (1 +
α )can be considered equal to 1.0, where
is the ratio between the moduli of the elasticity of steel and concrete
. CEB-FIP [
56] offers the following equation to determine the flexural crack spacing:
where
is the free concrete shrinkage strain,
is the rebar’s stress due to the cracks under an equivalent load
, which can be calculated as
. Furthermore,
is a dimensionless constant and
is the steel’s strain, which can be ignored in the transition section. Using ACI 224R-14 [
57], the maximum crack spacing in the beams and slabs is calculated based on static analysis. Thus, the maximum bending crack spacing can be calculated using the following formula:
where
is the maximum crack spacing (mm),
is the steel rebar’s stress (MPa),
is the cover of the outer tensile rebar’s layer (mm) and
A the symmetrical concrete’s cross-sectional area (mm
2).
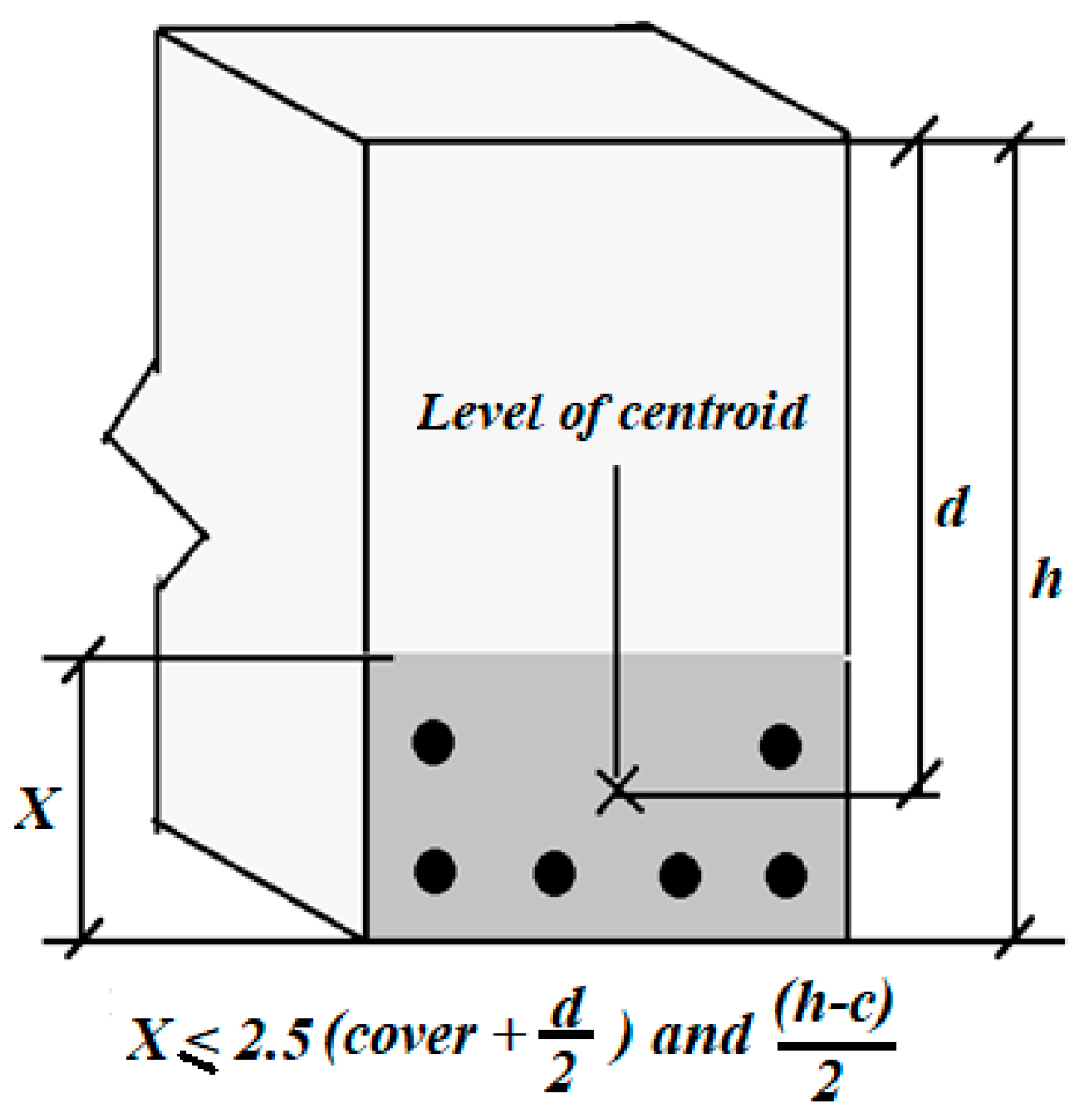
is a ratio equal to 1.2 times the space between the horizontal axis and the concrete’s outer tensile layer divided by the distance between the horizontal axis and the longitudinal rebars in the RC beam, as shown in
Figure 4.
According to ACI 224R-14 [
57], the moment crack spacing restricts the rebars stress
at the cracked section under a load of 2/3
. Furthermore, the distance between the tensile rebar’s and concrete’s outer tensile layer (
S) should not exceed the following value:
where
is the minimum distance between the surface tensile rebar’s or prestressed rebar’s and the concrete’s outer tension layer.
5. Flexural Capacity and the Load–Displacement Relationship
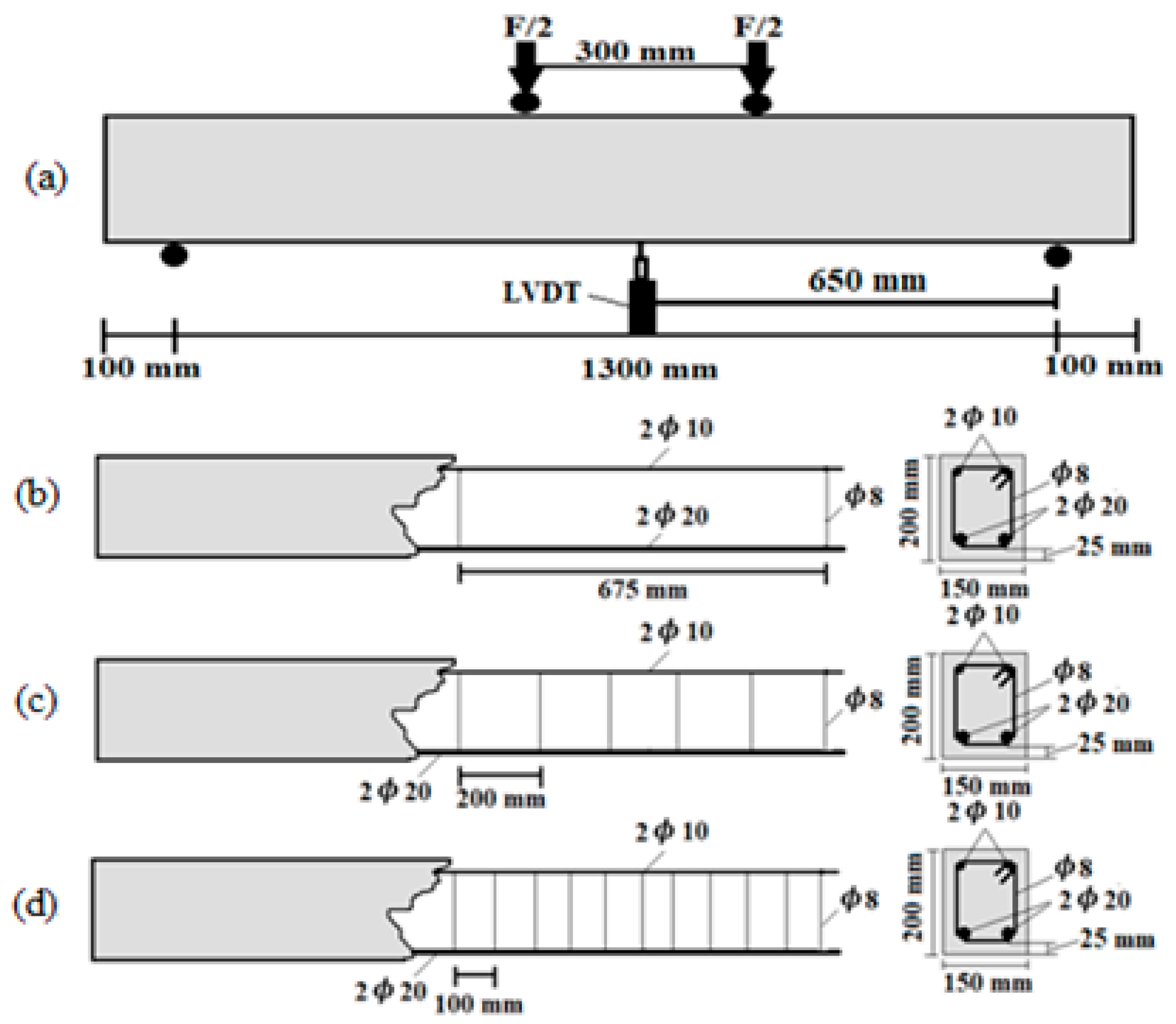
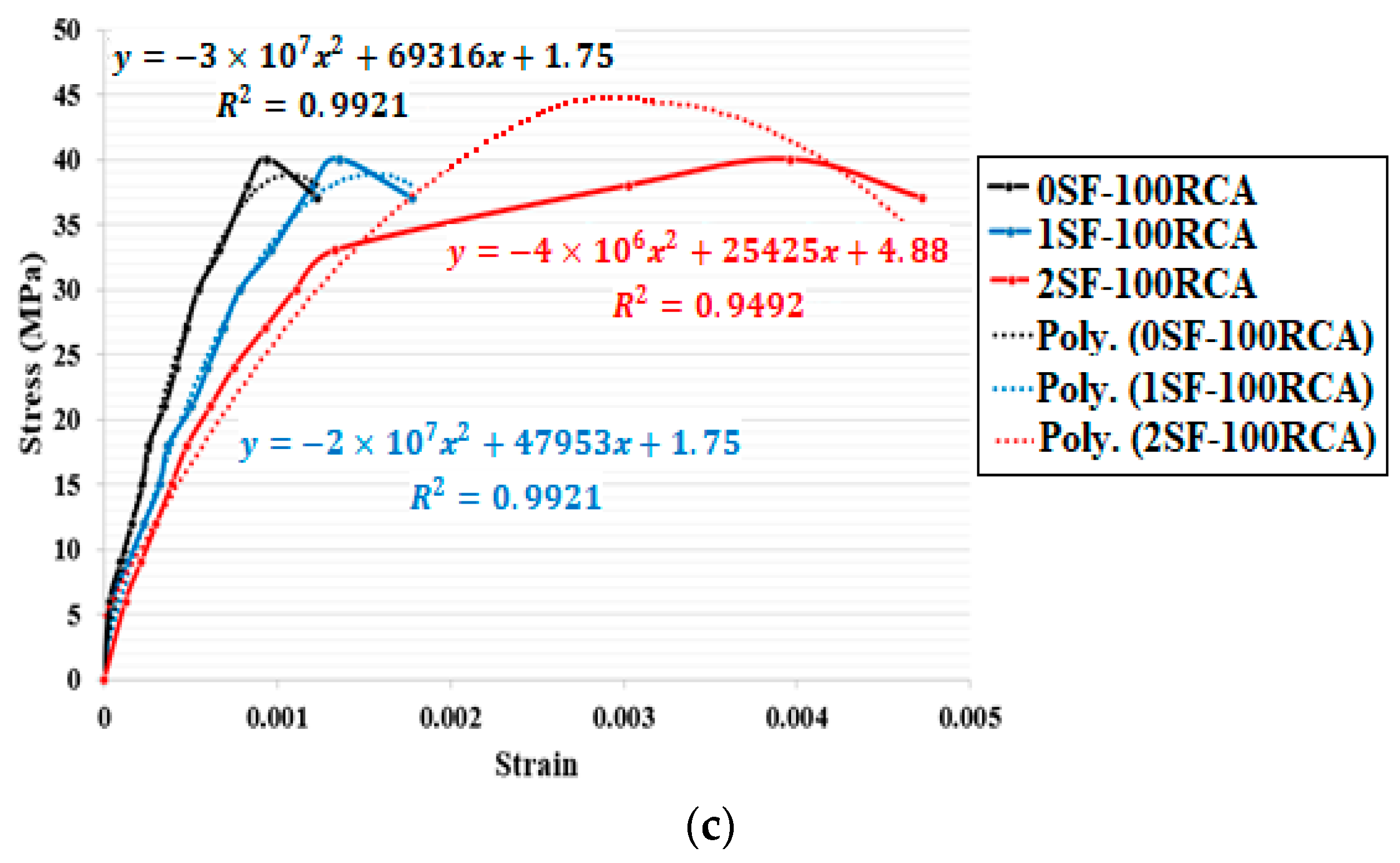
After evaluating the influence of SFs and RCA on the compressive and tensile behaviour of unreinforced concrete, 27 SF-reinforced RCARC beams were produced and measured, where the results are presented in this section. SFs were used at 0%, 1% and 2% (by volume). RCA was incorporated at 0%, 50% and 100% by weight. Furthermore, the effect of shear rebars was considered via different stirrup spacings. The load–displacement behaviour at the mid-span of the specimens, the maximum load-bearing capability and the crack widths and propagation were studied. In
Figure 14,
Figure 15,
Figure 16,
Figure 17,
Figure 18 and
Figure 19, the loading capacity and load-displacement behaviour of the specimens are represented.
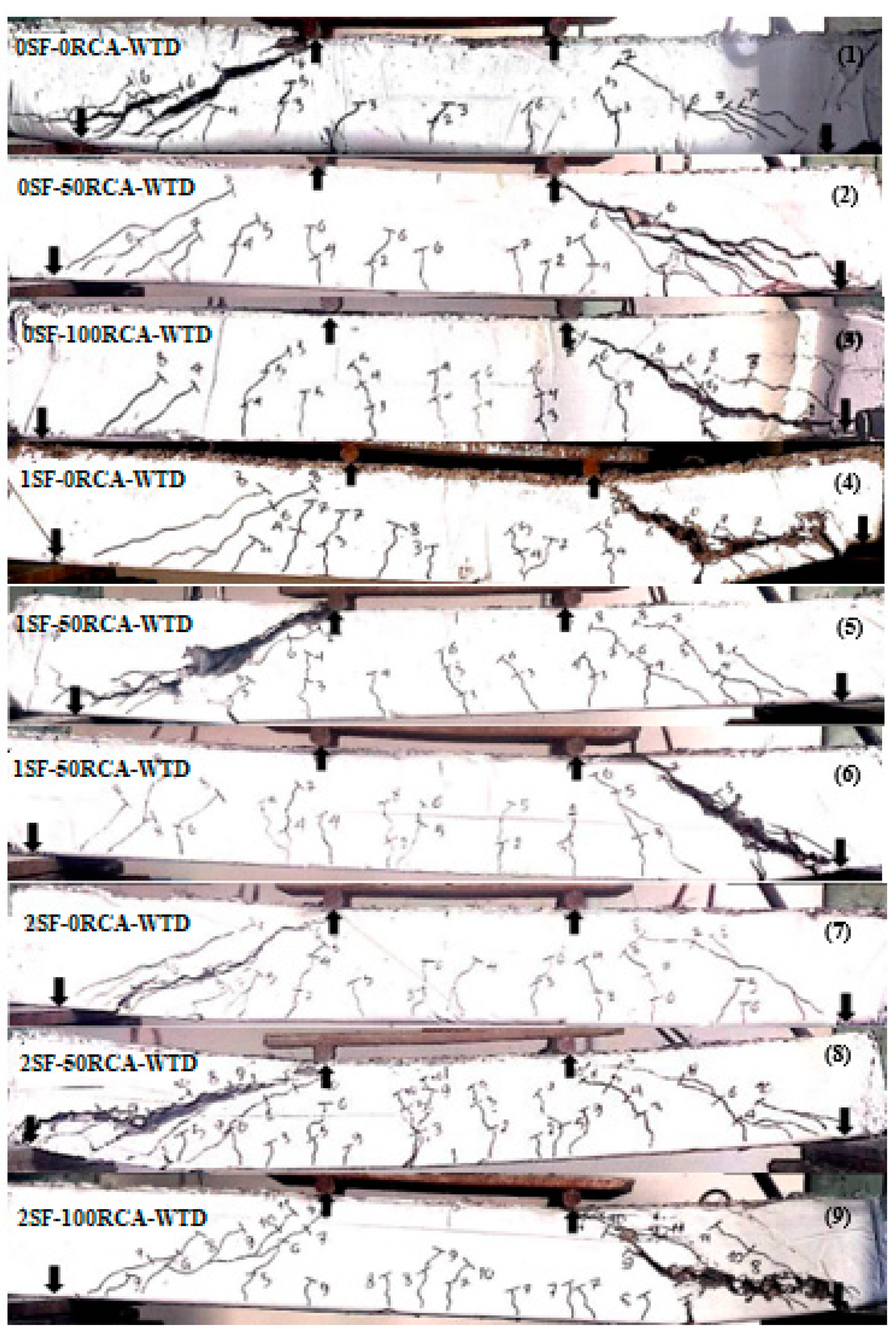
As per
Figure 14 and
Figure 15, the maximum bearing capability was substantially increased by increasing the SF content due to the effective role of SFs in improving the compressive and tensile strength of the unreinforced concrete. Furthermore, using RCA had a momentous impact on the maximum loading capacity. Furthermore, SFs enhanced the bending resistance strength of concrete due to the lack/insufficiency of shear rebars. Hence, SFs played a role as partial transverse reinforcement and improved the shear performance of the concrete beams. Therefore, the SFs reduced the inclined and shear crack widths and led to improving the first crack loading. As seen in
Figure 15, the lack of shear rebars led to shear failure of the specimens but crack propagation increased and the inclined crack widths were reduced by using RCA. Based on the models proposed by several standards, improving the compressive strength of the concrete led to improving the shear behaviour of RC beams. Therefore, according to the results of the compressive behaviour of the unreinforced concrete and the tested beams, RCA was used to produce RC beams without shear reinforcement when 2% SFs were used (
Figure 14 and
Figure 15).
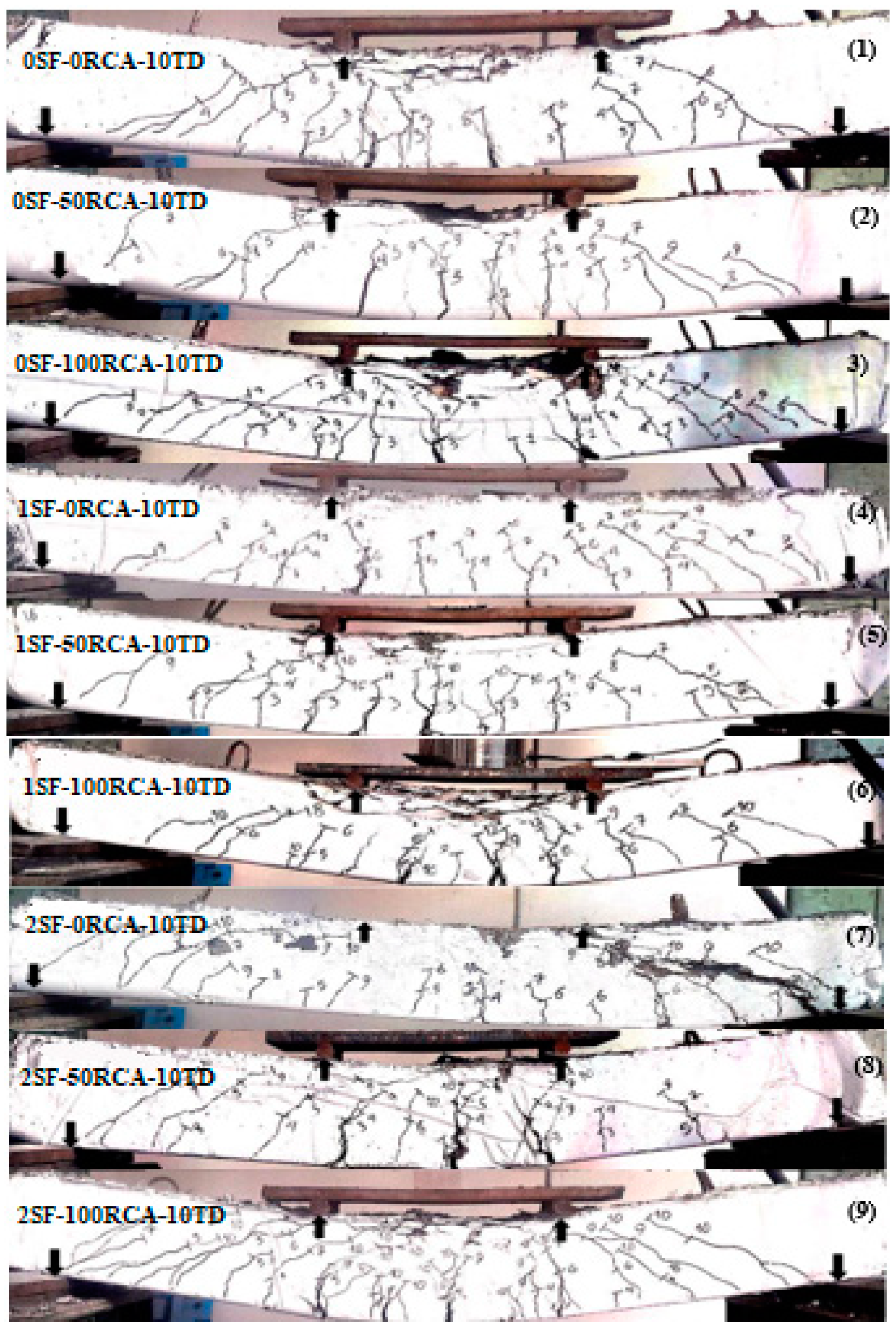
According to
Figure 16 and
Figure 17, the flexural behaviour improved by providing shear rebars spaced 200 mm apart, which was further enhanced by increasing the RCA content. Conversely, the maximum bearing resistance capacity dropped after the maximum bearing capability point when 100% RCA was used. This led to a dramatically increased crack propagation but the flexural capacity abruptly decreased after the maximum loading point in specimens without RCA. Furthermore, the flexural capacity was increased by utilising SF. Furthermore, reducing the shear rebars’ spacing was necessary. Therefore, enough stirrups should be provided and SFs should be added to the concrete mix.
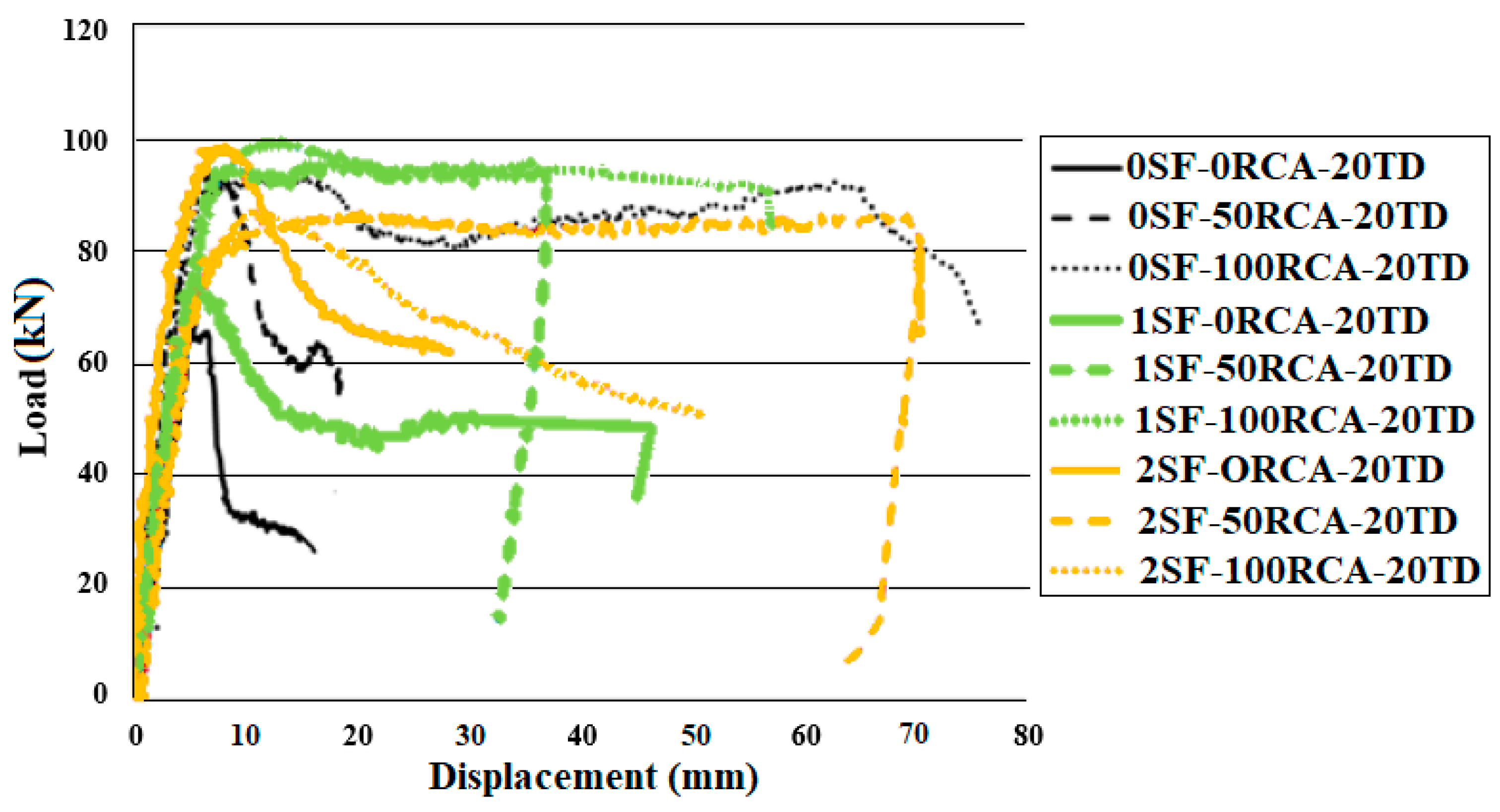
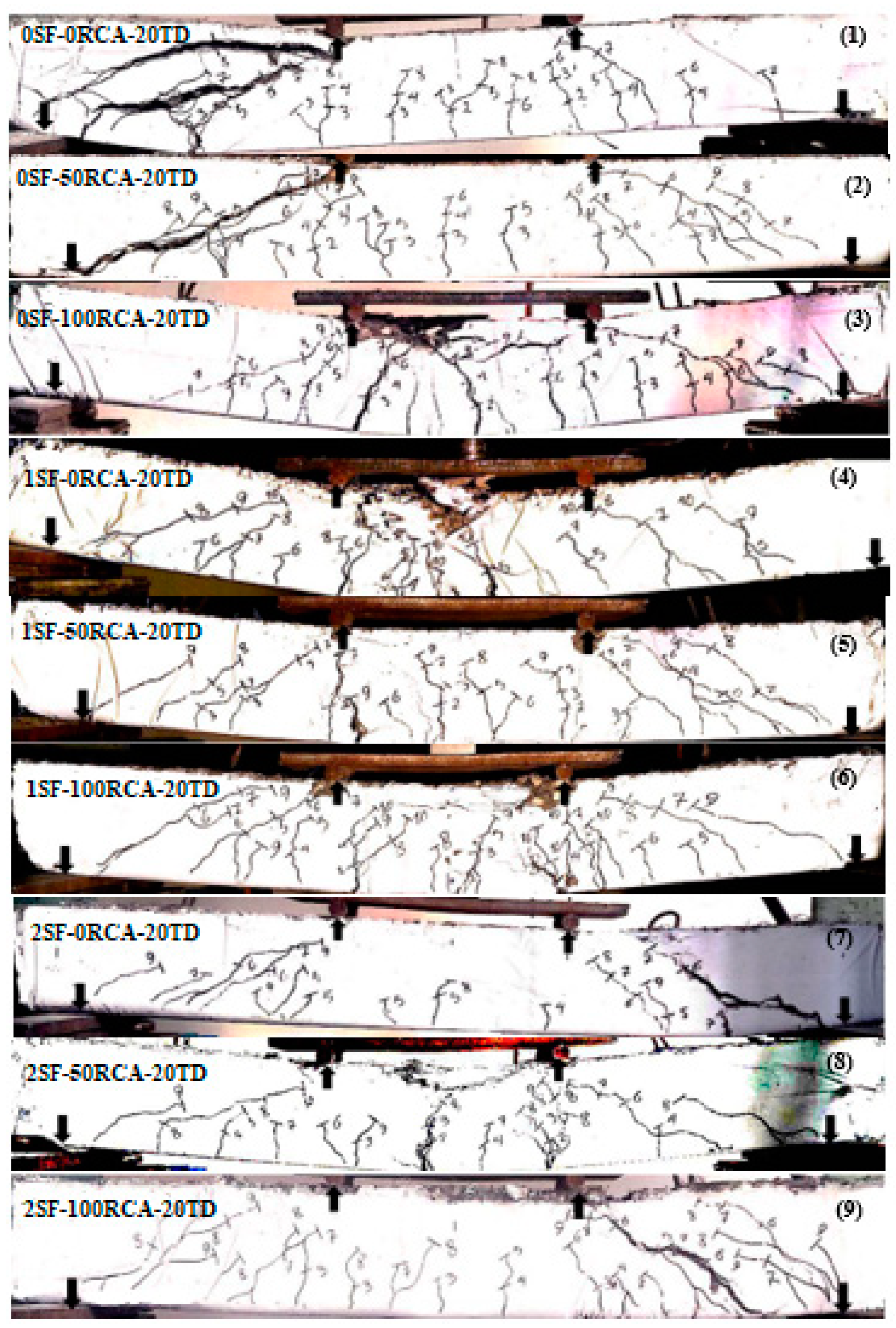
Conversely, reducing the shear rebars’ spacing to 100 mm led to an increase in the bending capability and failure cracks occurred at the middle of the specimen’s width with the maximum spacing (
Figure 18 and
Figure 19). Adding SFs to concrete improved the bending performance of the specimens as a result of improving the compressive and stress–strain behaviour of the unreinforced concrete. The minimum shear rebars’ spacing should be provided but SFs should be included as well. As it is seen in
Figure 18 and
Figure 19, NCA could be replaced with RCA at 100% if enough stirrups and SFs are provided in the specimen by comparison with the control specimen (without RCA and SF). According to
Figure 19, in addition to increasing the bond between the reinforcement and the concrete, cracks developed less when SFs were used due to the bridging role of the SFs. Therefore, specimens with 2% SFs showed greater ductility and did not fail suddenly.
According to
Figure 19, reducing shear rebars’ spacing to 100 mm led to dropping the impact of SFs on the bending performance of the RCARC beams. Furthermore, raising the RCA contents generally led to an increased deformation; however, adding SFs did not have a momentous effect on the maximum flexural capability. On the other hand, providing enough shear rebars caused an improvement in the bending performance of specimens. Therefore, crack propagation increased and the crack widths decreased. Furthermore, the first crack loading increased when increasing the RCA content. Hence, using SFs in RC beams that were manufactured with sufficient shear rebars (100 mm spacing) only reduced the crack widths and there was no significant influence of the structural performance of the RC beams. However, when insufficient shear rebars (200 mm spacing) were used, using SFs not only limited the crack widths and their propagation but also improved the structural performance of RC beams.
According to
Figure 15,
Figure 17 and
Figure 19, in addition to increasing the bonding between the rebars and the concrete, cracks developed less after adding SFs due to the bridging role of the fibres and the improvement of the bond-slip between the rebars and the concrete because, with the reduction of the slip between the concrete and rebars, the crack widths were lower. Therefore, specimens with 2% SFs deformed with more ductility and did not collapse abruptly. In specimens with no SFs, the crack propagation was much shorter than in those with SFs. In contrast, in beams with SFs, the final crack spacing corresponding to the stabilised cracking phase was on average 23% smaller than that of the specimens with no SFs. According to these outcomes, it became clear that the formed cracks were consistently much more irregular in beams with SFs than in those without. The crack spacing’s variation ratio ranged from 14 to 39% in beams with no SFs, and from 39 to 46% in specimens with SFs. The relationship between the maximum and average crack spacing was a momentous factor too. This relation was 1.60 in specimens with no SFs and 1.74 in specimens with SFs.
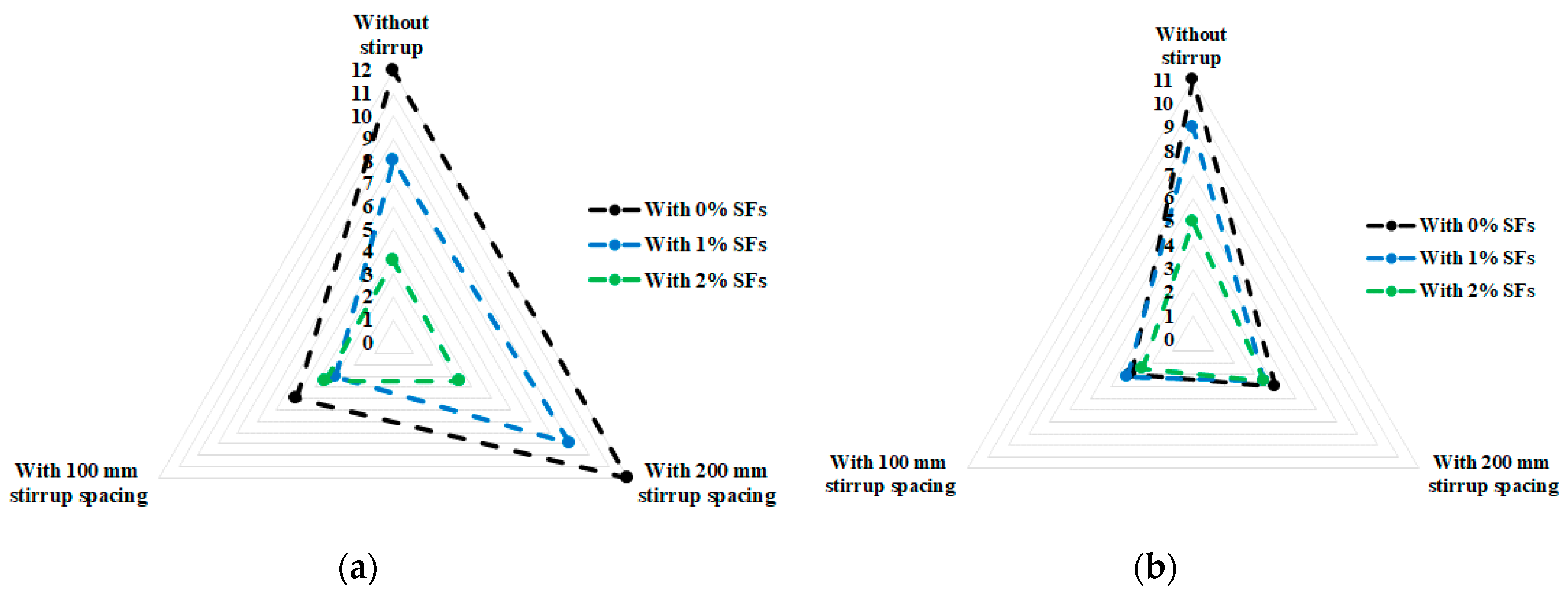
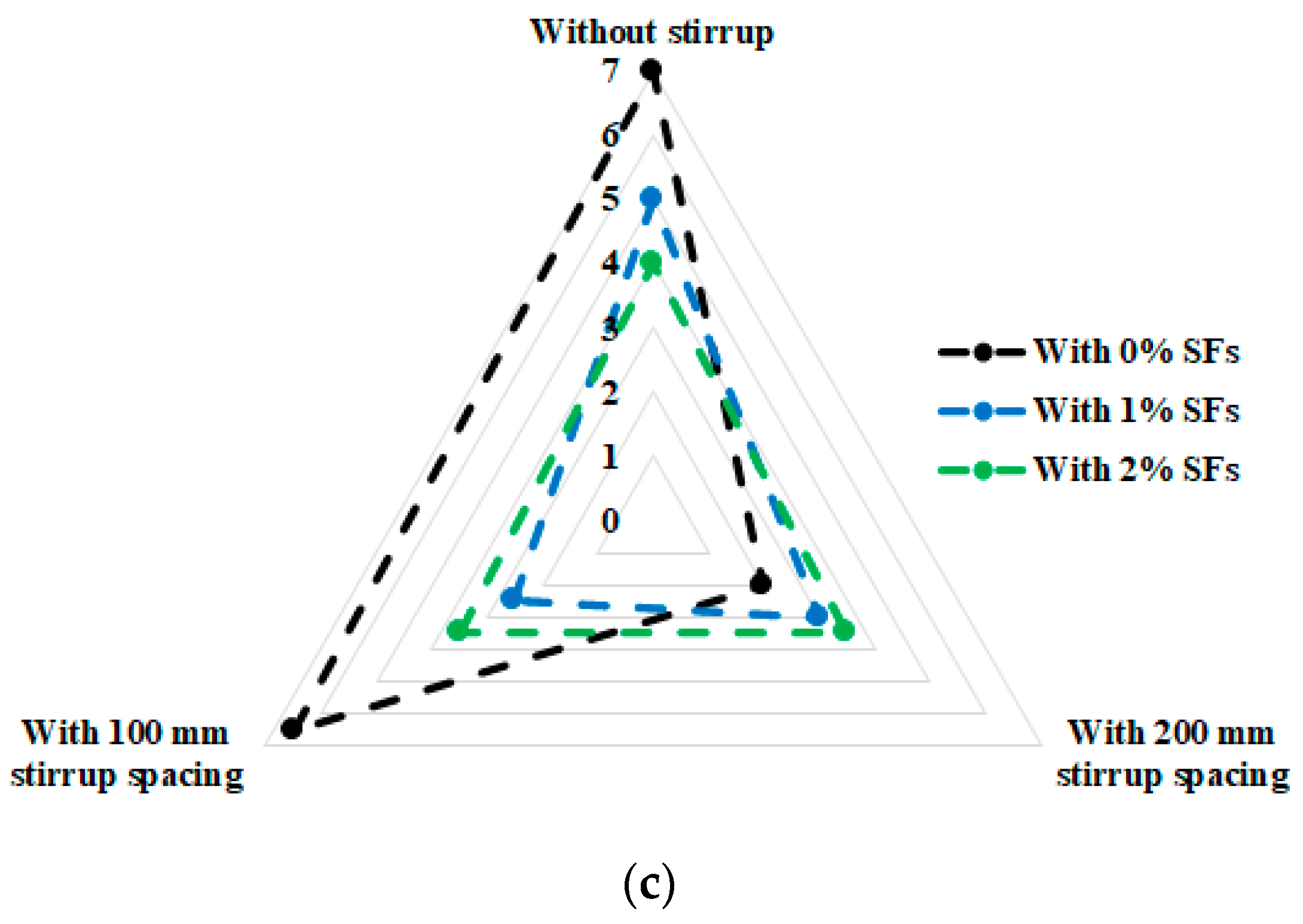
To study the effect of RCA and SF contents as a function of the shear rebars’ spacing, the crack spacing at the peak loads are presented in
Table 5 and
Figure 20. In
Figure 20, the enclosed area of the polygons indicates the influence of the variables on the crack spacing. This means that a reduction of the enclosed area shows a reduction of the crack widths and the positive influence of SFs. Furthermore, in
Table 5, the corresponding cracking loads are shown. According to
Table 5, using SFs resulted in a considerably decreased crack width at the peak load. Contrariwise, using RCA with more broken surfaces led to a better aggregate bond with cement than using NCA. Therefore, this resulted in reducing the crack widths and increasing the flexural capacity. Providing enough shear rebars along the specimens’ length was essential, especially in specimens with shear cracks, but using SFs could dramatically control the effect of the lack of shear rebars in terms of a reduction in the crack widths. Regarding
Figure 20, SFs had a substantial impact on reducing the crack widths at the peak load but there was no significant difference when 1% and 2% SFs were used (
Figure 20). Alternatively, using 1% SFs did not have an extensive impact on reducing the crack widths when 100% RCA was used (
Figure 20c), but using 2% SFs improved the crack widths in all specimens. According to
Figure 20, using 2% SFs significantly reduced the crack widths at the peak load, in contrast to using 1% SFs. Conversely, increasing the RCA content reduced the ultimate crack width reduction effect of SFs.
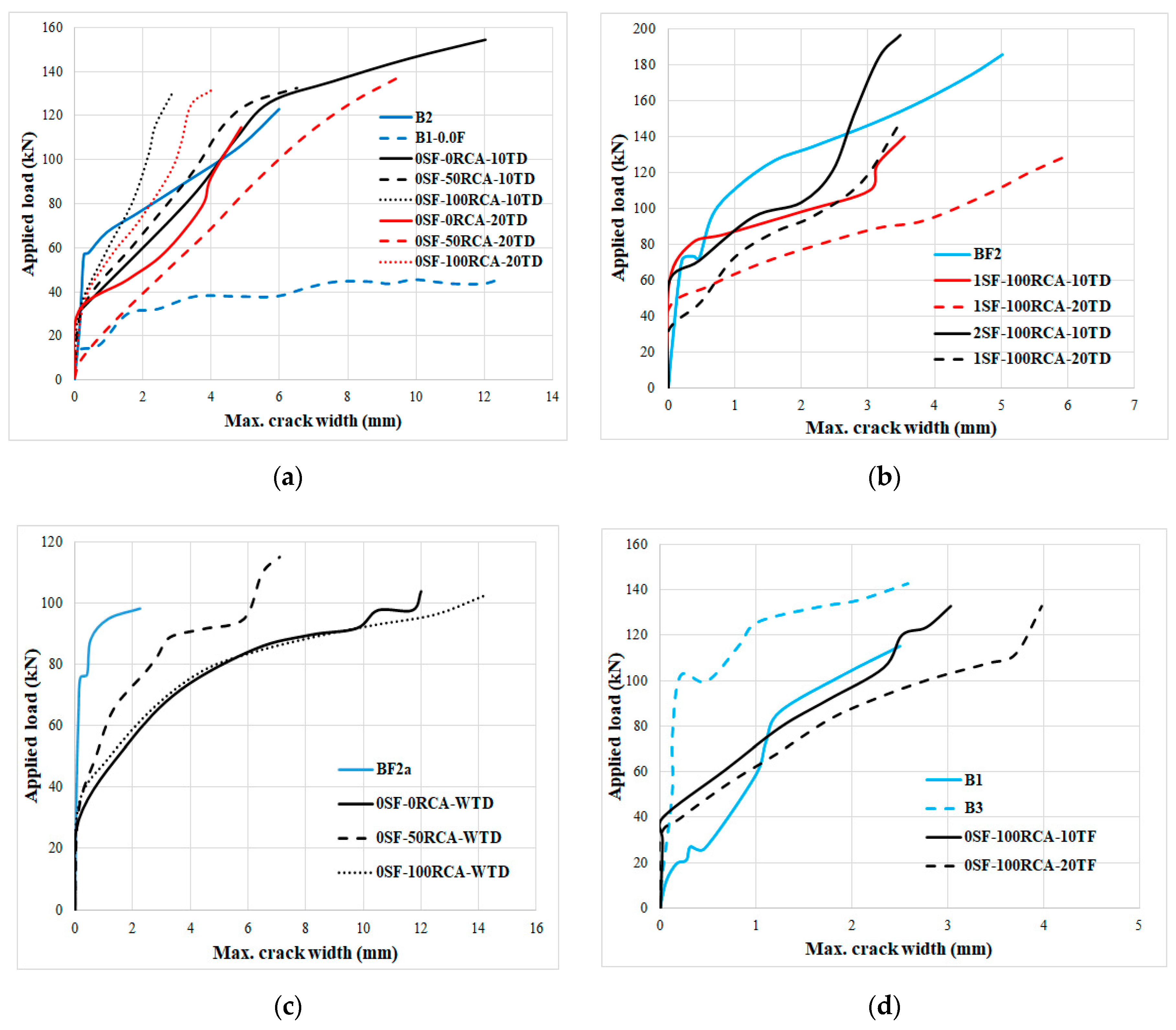
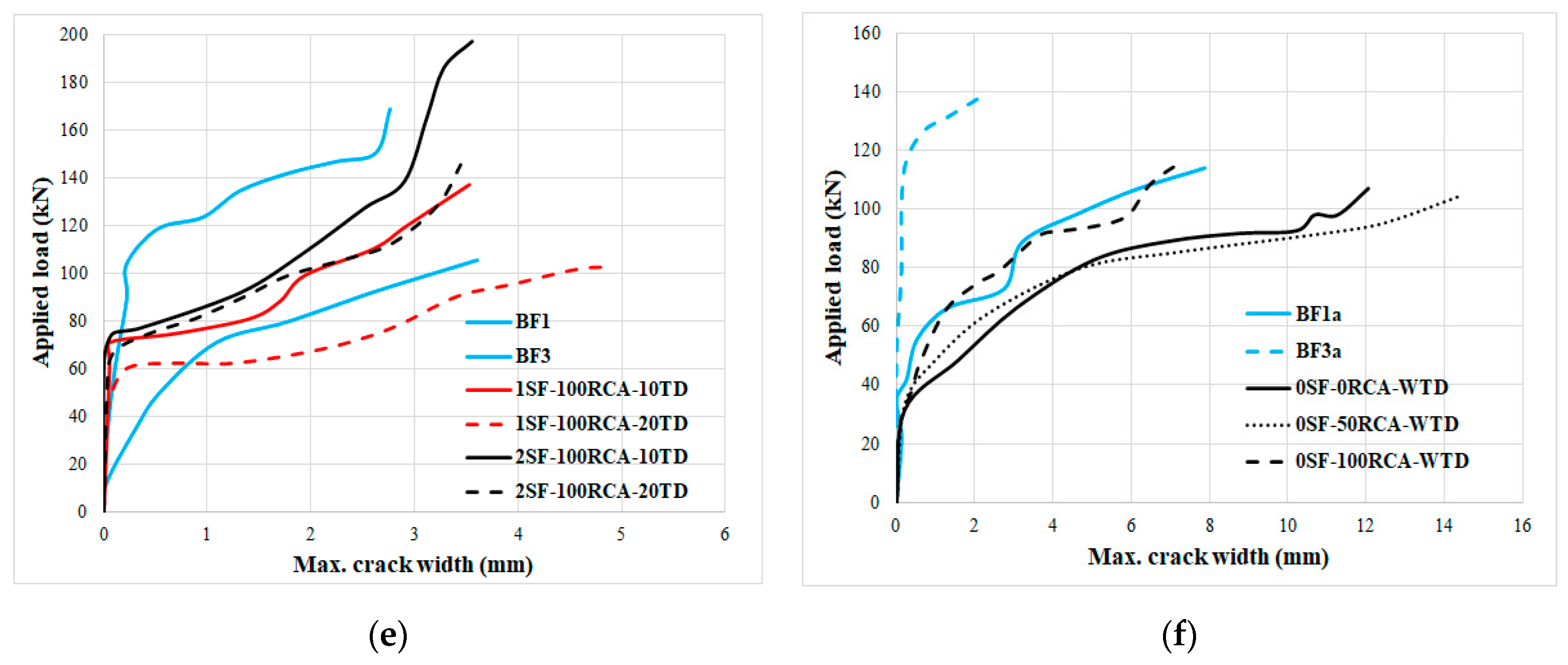
Therefore, the characteristics of these specimens are demonstrated in
Table 6. Moreover, in order to provide an accurate analysis, the results were compared with those of the literature and are presented in
Figure 21 [
59,
60].
The crack width increased under loading after increasing the shear rebars’ spacing. Furthermore, using RCA resulted in lower crack widths and a greater flexural moment resistance when cracks occurred. Using SFs led to lower flexural crack widths and the flexural strength was also significantly increased after cracks appeared (
Figure 21b,e). As seen in
Figure 20c and
Figure 21f, the flexural crack widths were increased by increasing the tensile rebars’ diameter in the RCARC beams. Furthermore,
Figure 21a–d shows that the effect of longitudinal tensile rebars was more than the effect of the shear rebars on the crack widths. Therefore, providing enough shear rebars limited the crack propagation and the crack widths decreased. Furthermore, the crack at the peak load increased due to increasing the RCA contents. Additionally, cracks developed less by adding SFs due to the bridging role of the fibres. However, in specimens with no SFs, the crack propagation was considerably lower than that with SFs. Therefore, it could be observed that the cracks were more irregular in the SF-reinforced beams relative to those of the control specimens (with no SFs or RCA).
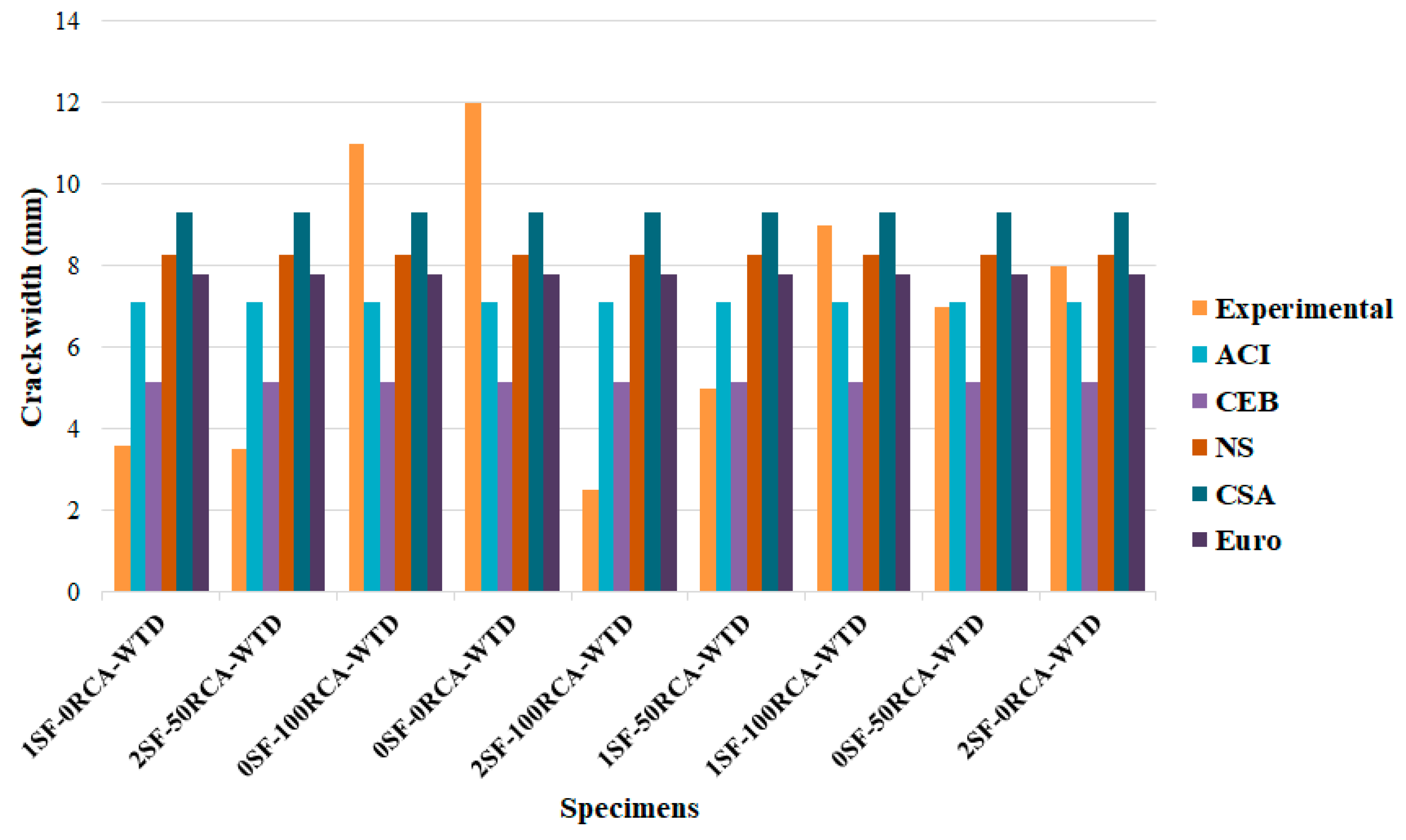
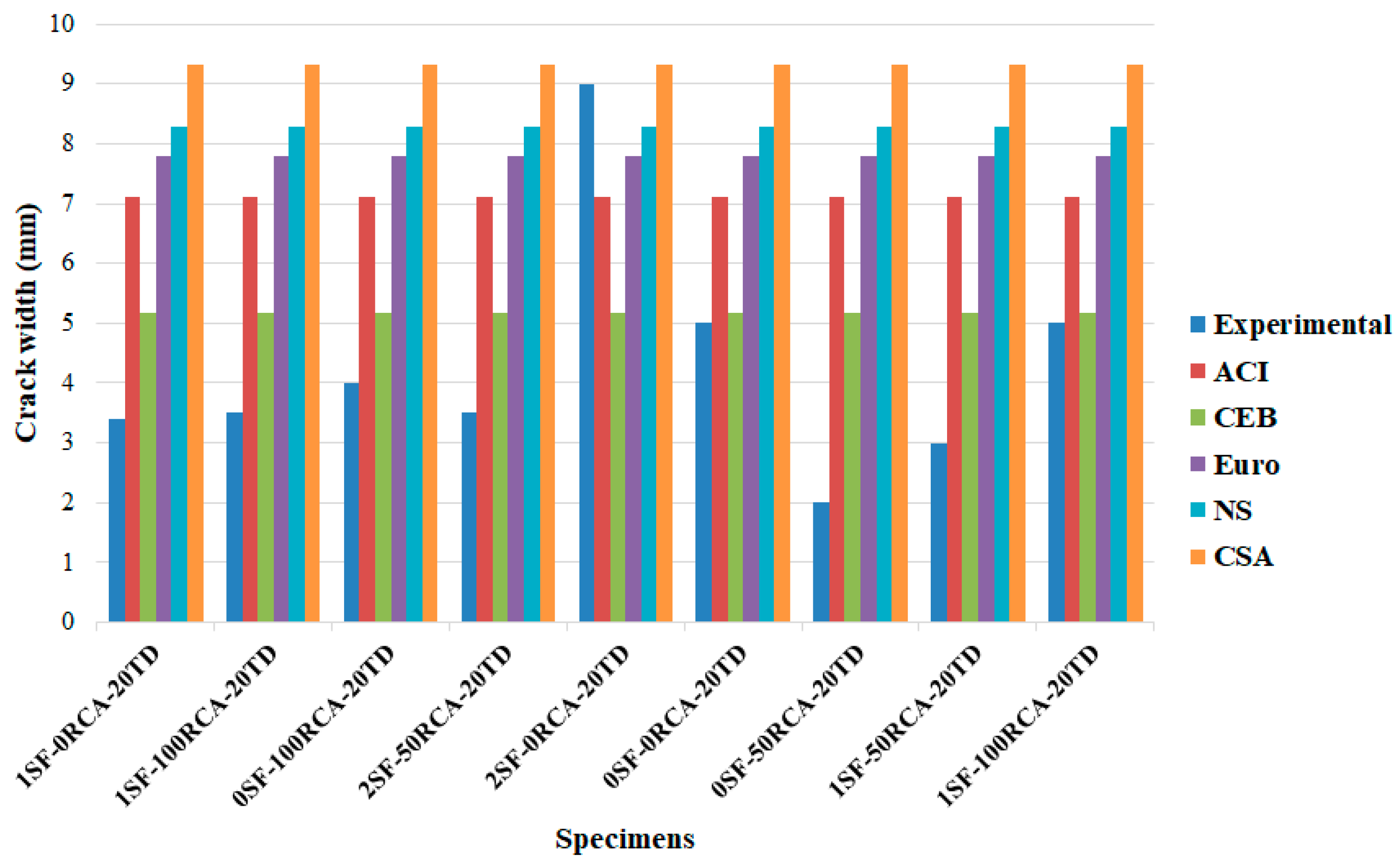
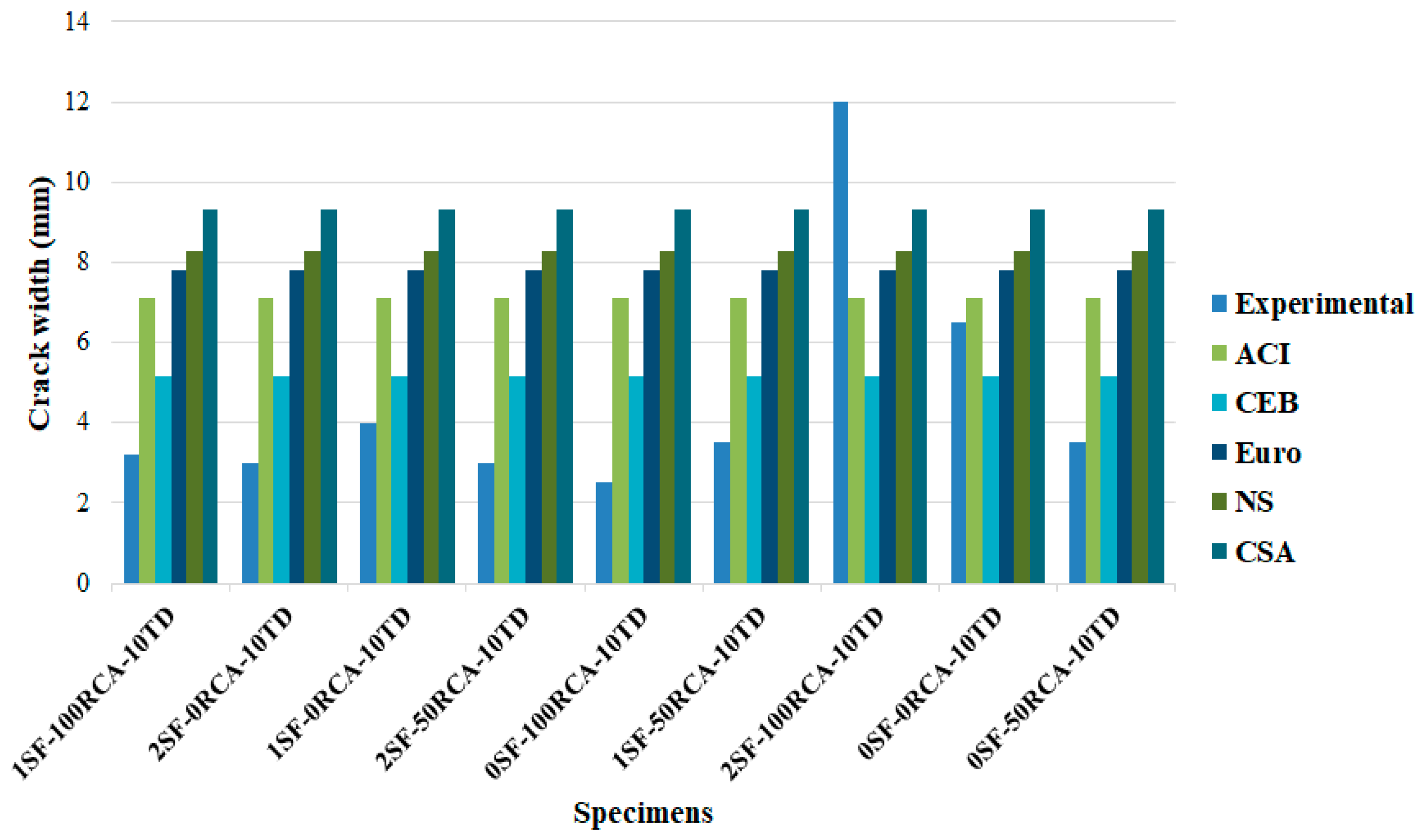
Finally, the results were compared with the requirements of CSA S474 [
53], NS 3473E [
54], EC2-04 [
55], CEB-FIP [
56] and ACI 224R-14 [
57], where the results are shown in
Figure 22,
Figure 23 and
Figure 24. In specimens with no shear rebars or SFs, the available equations predicted crack widths lower than the obtained results, where crack width was reduced by adding SFs. Furthermore, codes could not predict the crack width when NCA was replaced with RCA, especially for 100% replacement. CSA predicted the crack width properly but CEB predicted inaccurate widths when no SFs were used in the RCARC specimens (the predicted crack widths were less than that obtained numerically). Conversely, in the experimental samples with 200 mm and 100 mm shear rebar spacings, all standards presented appropriate equations regarding predicting the crack width at different SF and RCA contents (
Figure 22,
Figure 23 and
Figure 24). It should be mentioned that CEB properly predicted the crack width in specimens with 200 mm and 100 mm stirrup spacings. Additionally, the obtained experimental results were compared with those achieved based on the requirements of the codes, as illustrated in
Table 7.