Electrochemical Properties of NdCl3 and CeCl3 in Molten LiCl-KCl Eutectic Salt
Abstract
:1. Introduction
2. Experimental
2.1. Electrochemical Apparatus and Electrodes
2.2. Determination of Active Electrode Area
3. Results and Discussion
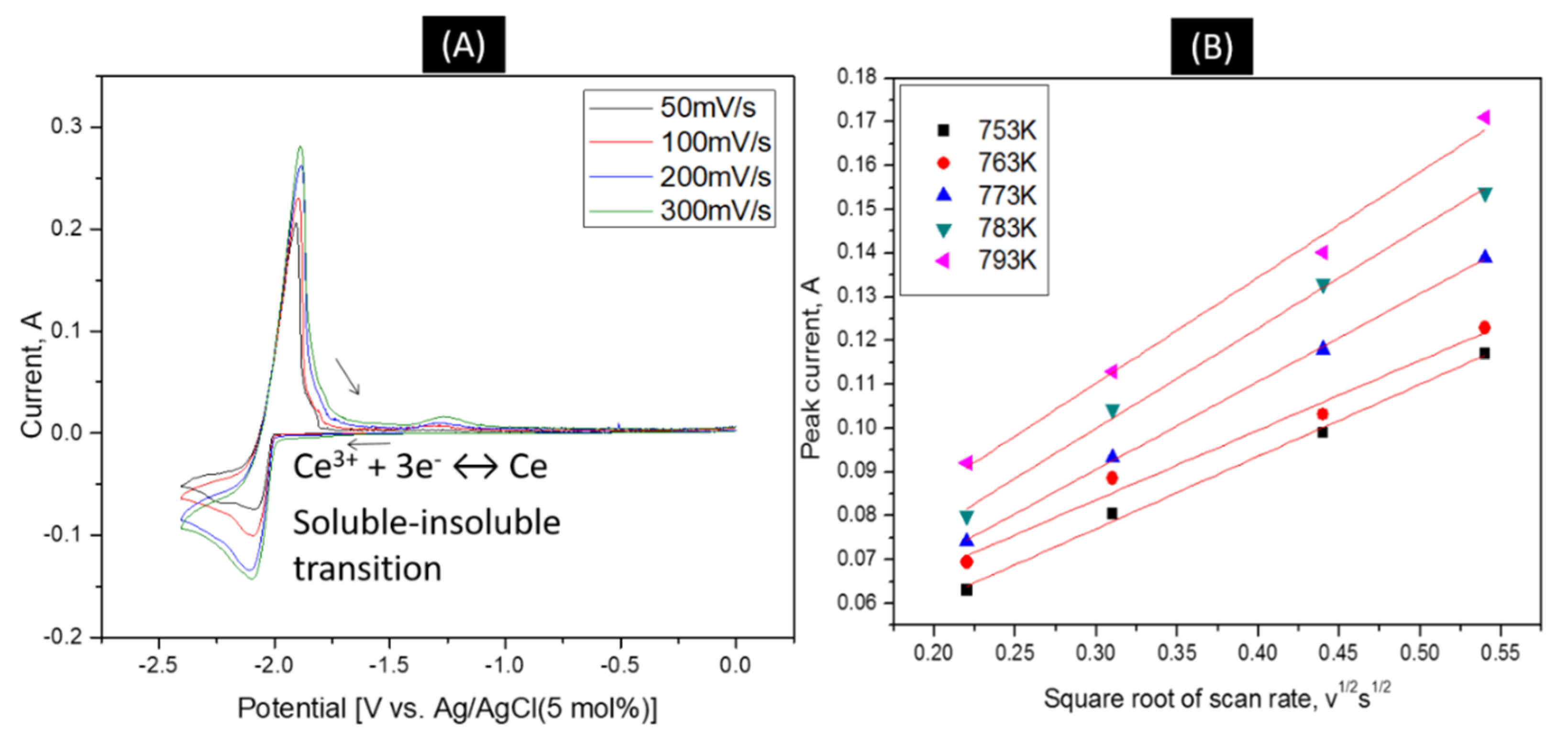
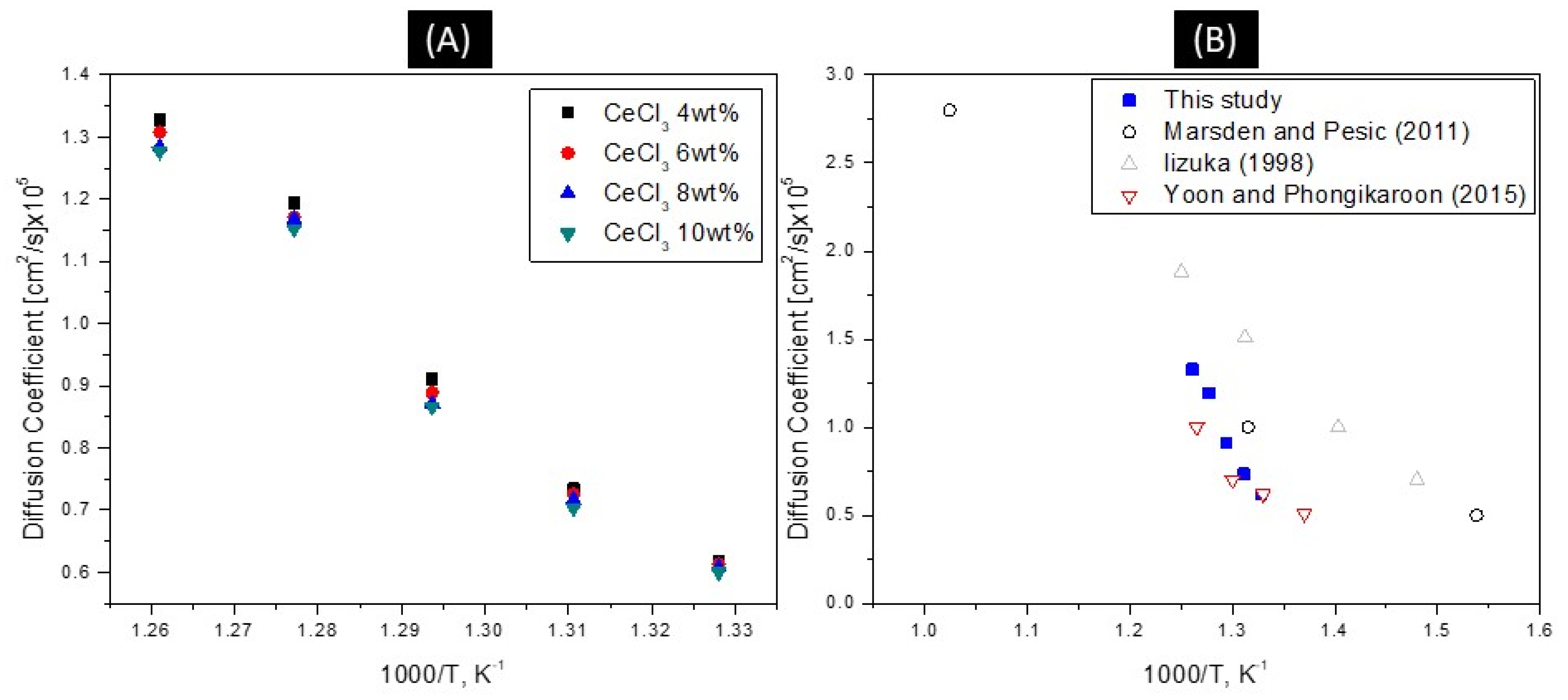
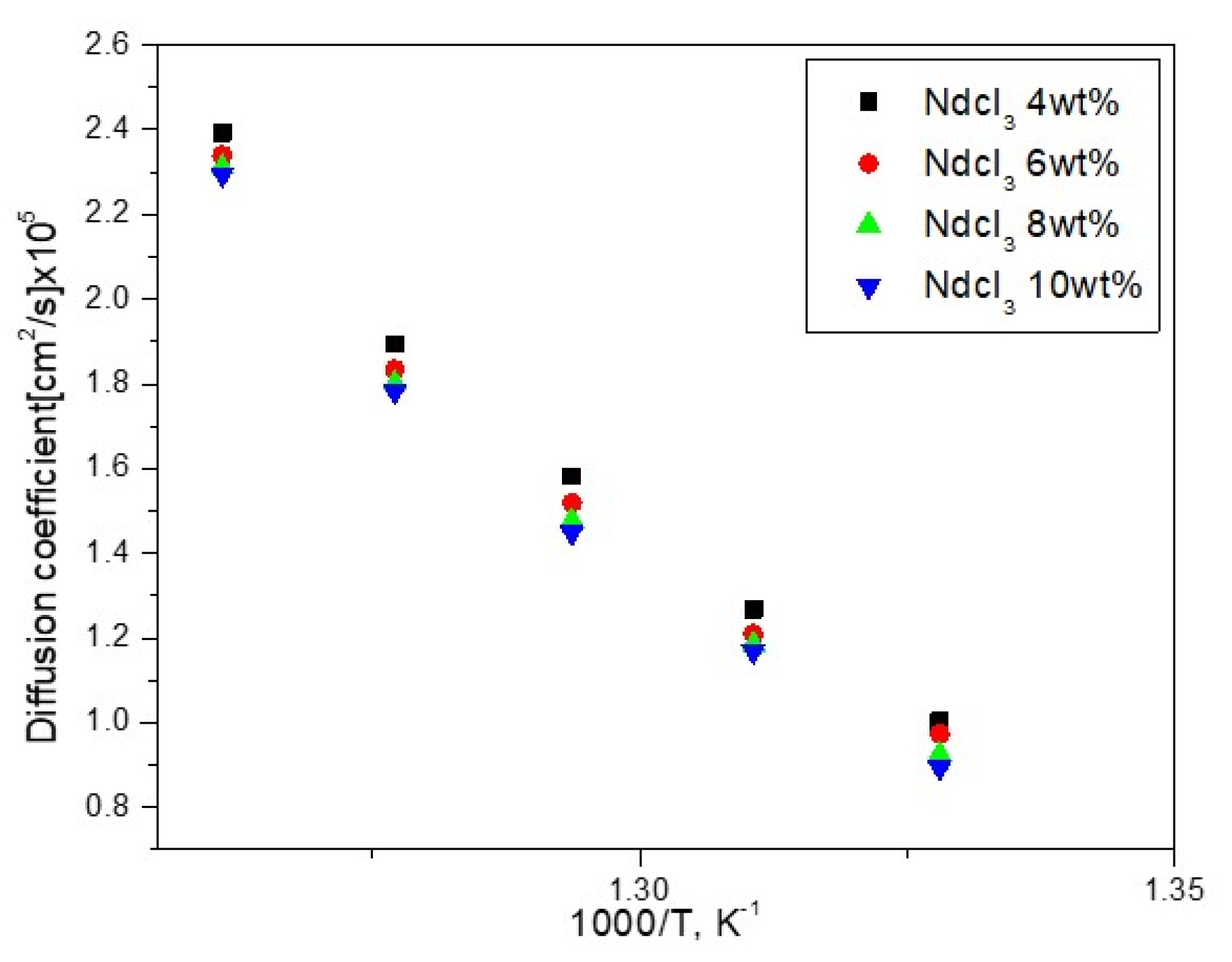
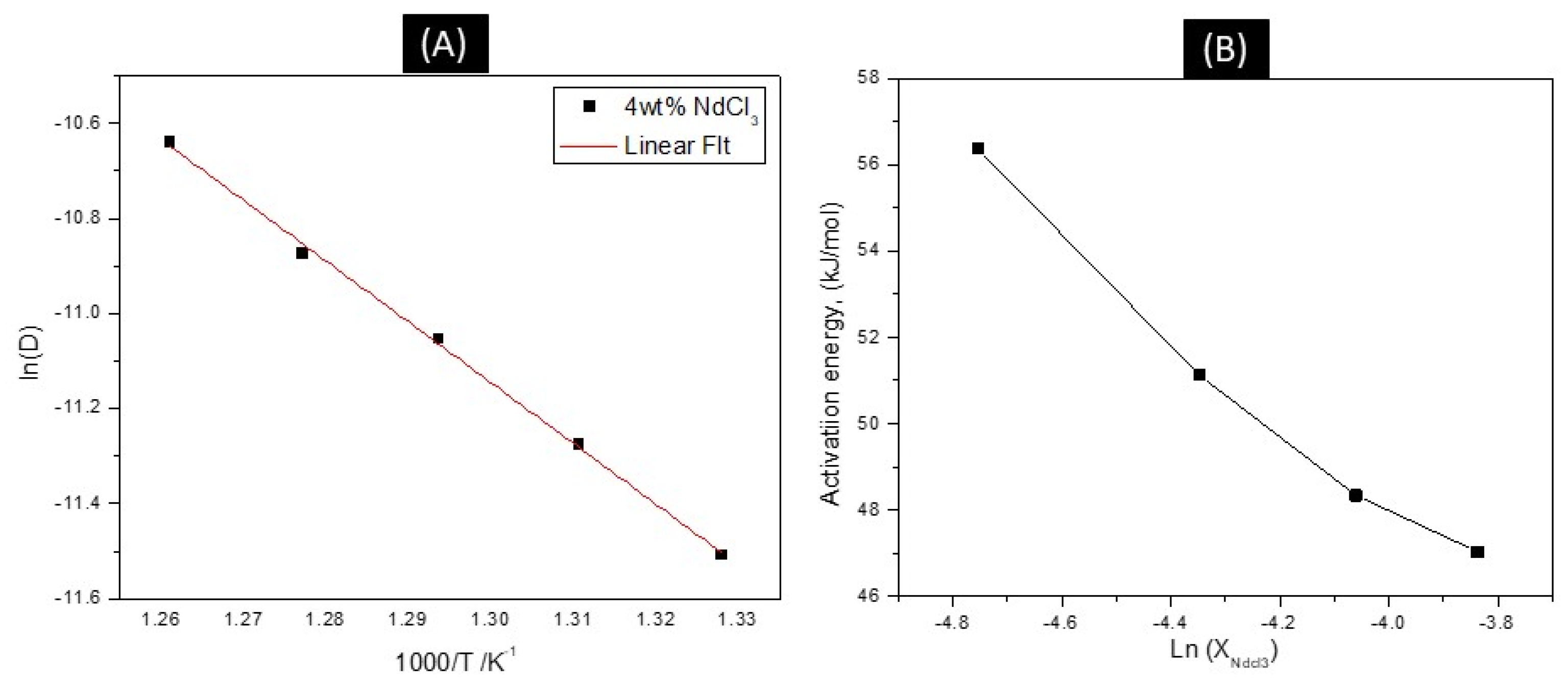
3.1. Determination of the Diffusion Coefficient of CeCl3 and NdCl3 in LiCl-KCl
3.2. Determination of the Apparent Standard Potential of CeCl3 and NdCl3 in LiCl-KCl
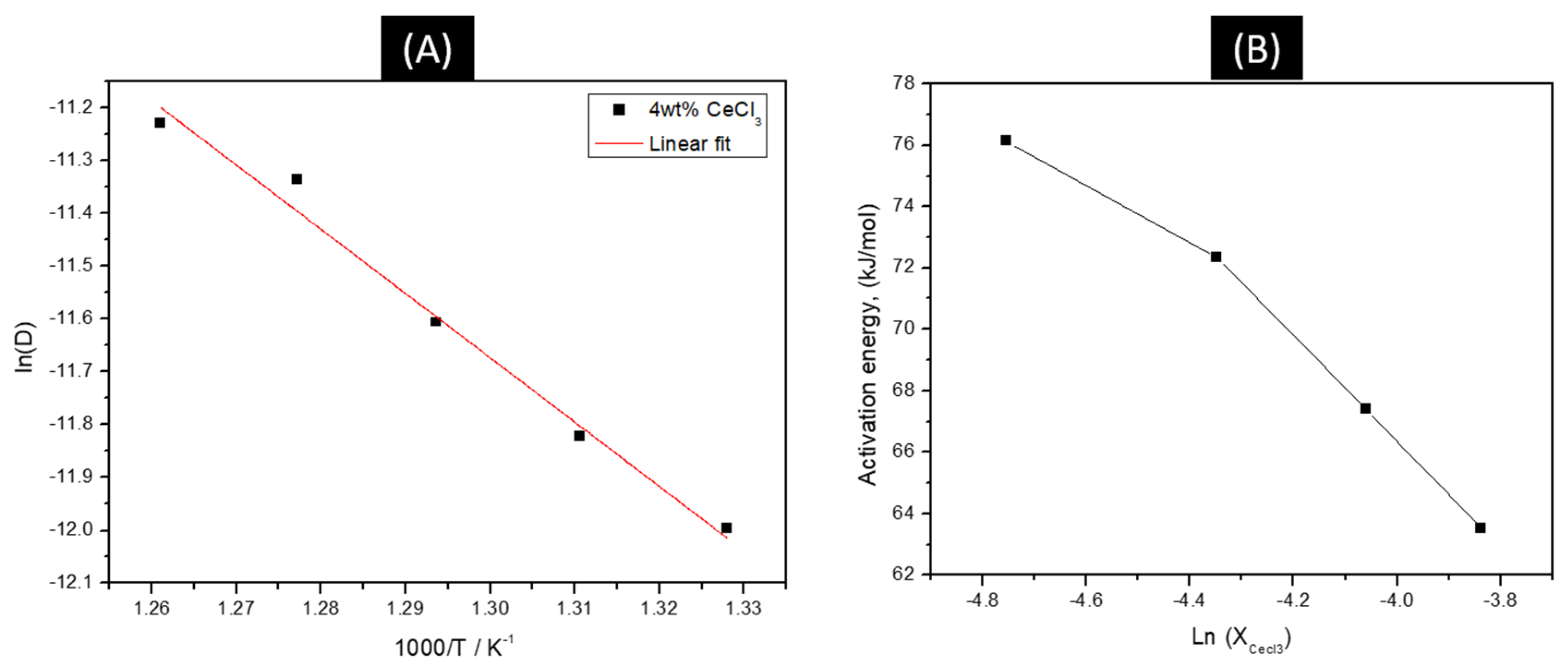
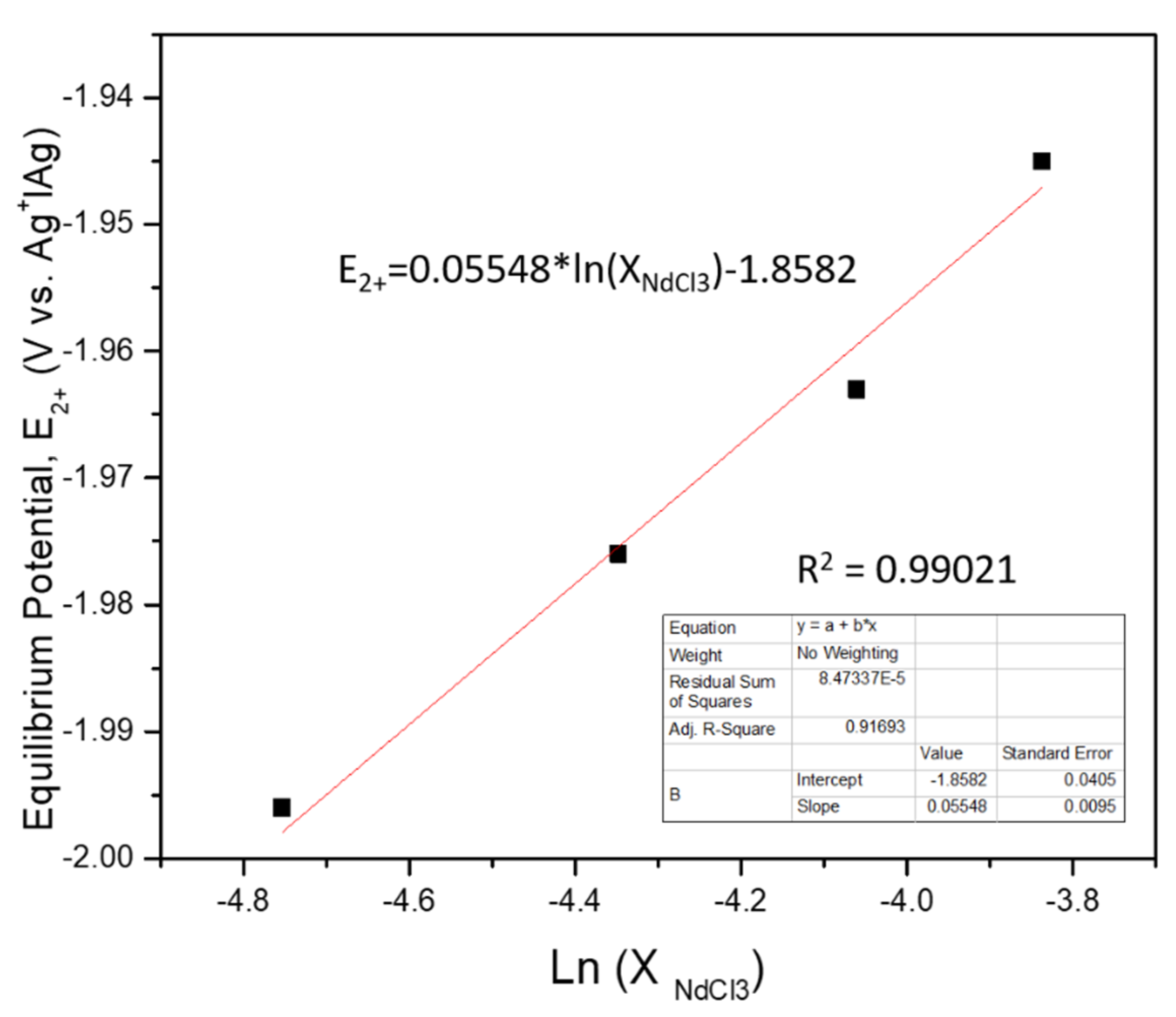
3.3. Determination of the Activity Coefficient of CeCl3 and NdCl3 in LiCl-KCl
4. Conclusions
Author Contributions
Funding
Conflicts of Interest
References
- IAEA-TECDOC-1648. Assessment of Partitioning Processes for Transmutation of Actinides; IAEA: Vienna, Austria, 2010. [Google Scholar]
- NEA. Pyrochemical Separations in Nuclear Applications, A Status Report; OECD Publications: Paris, France, 2004. [Google Scholar]
- Inoue, T.; Koch, L. Fuel cycle with pyro-process from the perspective of resisting proliferation. In Proceedings of the GLOBAL 2007, CD-ROM, Tsukuba, Ibaraki, Japan, 9–13 October 2005. [Google Scholar]
- Kim, T.J.; Ahn, D.H.; Paek, S.W.; Jung, Y. Study on electrodeposition of Ce(III) at a tungsten electrode in a LiCl-KCl molten salt solution. Int. J. Electrochem. Sci. 2013, 8, 9180–9186. [Google Scholar]
- Shirai, O.; Uehara, A.; Fujii, T.; Yamana, H. Thermochemical properties of the intermetallic compounds in the lanthanum–cadmium system. J. Nucl. Mater. 2005, 344, 142–145. [Google Scholar] [CrossRef]
- Sethi, R.S. Electrocoating from molten salts. J. Appl. Electrochem. 1979, 9, 411–426. [Google Scholar] [CrossRef]
- Castrillejo, Y.; Bermejo, M.R.; Barrado, A.I.; Pardo, R.; Barrado, E.; Martínez, A.M. Electrochemical behaviour of dysprosium in the eutectic LiCl–KCl at W and Al electrodes. Electrochim. Acta 2005, 50, 2047–2057. [Google Scholar] [CrossRef]
- Hoover, R.O.; Shaltry, M.R.; Martin, S.; Sridharan, K.; Phongikaroon, S. Electrochemical studies and analysis of 1–10wt% UCl3 concentrations in molten LiCl–KCl eutectic. J. Nucl. Mater. 2014, 452, 389–396. [Google Scholar] [CrossRef]
- Takagi, R.; Rycerz, L.; Gaune-Escard, M. Phase equilibrium in the LnCI3-mcl mixtures (Ln = Lanthanide; M = Alkali): Thermodynamics and electrical conductivity of the M3LnCI6 compounds. J. Alloys Compd. 1997, 257, 134. [Google Scholar] [CrossRef]
- Gong, W.; Gaune-Escard, M.; Rycerz, L. Thermodynamic assessment of LiCl—NdCl3 and LiCl—PrCl3 quasi-binary systems. J. Alloys Compd. 2005, 396, 92–99. [Google Scholar] [CrossRef]
- Rycerz, L.; Gaune-Escard, M. Mixing enthalpies of TbBr3-MBr liquid mixtures. Z. Nat. A 2001, 56, 859–864. [Google Scholar] [CrossRef]
- Misra, M.; Raja, K.S.; Jaques, A.V.; Baral, S. Effect of addition of multi-component lanthanides to LiCl-KCl eutectic on thermal and electrochemical properties. ECS Trans. 2010, 33, 351–360. [Google Scholar] [CrossRef]
- Iizuka, M. Diffusion coefficients of cerium and gadolinium in molten LiCl-KCl. J. Electrochem. Soc. 1998, 145, 84–88. [Google Scholar] [CrossRef]
- Marsden, K.C.; Pesic, B. Evaluation of the electrochemical behavior of CeCl3 in molten LiCl-KCl eutectic utilizing metallic ce as an anode. J. Electrochem. Soc. 2011, 158, F111–F120. [Google Scholar] [CrossRef]
- Novoselova, A.V.; Smolenskii, V.V. Electrochemical study of the properties of Nd(III) and Nd(II) ions in molten LiCl-KCl-CsCl eutectic and individual CsCl. Russ. J. Electrochem. 2013, 49, 931–937. [Google Scholar] [CrossRef]
- Kreysa, G. Electrochemical cell design and optimization procedures. J. Electroanal. Chem. Interfacial Electrochem. 1990, 283, 459–460. [Google Scholar] [CrossRef]
- Fukasawa, K.; Uehara, A.; Nagai, T.; Fujii, T.; Yamana, H. Electrochemical and spectrophotometric study on neodymium ions in molten alkali chloride mixtures. J. Alloys Compd. 2011, 509, 5112–5118. [Google Scholar] [CrossRef]
- Yu, Y.-X.; Gao, G.-H.; Li, Y.-G. Surface tension for aqueous electrolyte solutions by the modified mean spherical approximation. Fluid Phase Equilibria 2000, 173, 23–38. [Google Scholar] [CrossRef]
- Ito, H.; Hasegawa, Y.; Ito, Y. Densities of eutectic mixtures of molten alkali chlorides below 673 K. J. Chem. Eng. Data 2001, 46, 1203–1205. [Google Scholar] [CrossRef]
- Hamel, C.; Chamelot, P.; Taxil, P. Neodymium(lll) cathodic processes in molten fluorides. Electrochim. Acta 2004, 49, 4467–4476. [Google Scholar] [CrossRef]
- Gibilaro, M.; Massot, L.; Chamelot, P.; Taxil, P. Study of neodymium extraction in molten fluorides by electrochemical co-reduction with aluminium. J. Nucl. Mater. 2008, 382, 39–45. [Google Scholar] [CrossRef]
- Fusselman, S.P.; Roy, J.J.; Grimmett, D.L.; Grantham, L.F.; Krueger, C.L.; Nabelek, C.R.; Storvick, T.S.; Inoue, T.; Hijikata, T.; Kinoshita, K.; et al. Thermodynamic properties for rare earths and americium in pyropartitioning process solvents. J. Electrochem. Soc. 1999, 146, 2573–2580. [Google Scholar] [CrossRef]
- Morss, L.R.; Edelstein, N.M.; Fuger, J. The Chemistry of the Actinide and Transactinide Elements; Springer: New York, NY, USA, 2008. [Google Scholar]
- Masset, P.; Konings, R.J.; Malmbeck, R.; Serp, J.; Glatz, J.-P. Thermochemical properties of lanthanides (Ln=La,Nd) and actinides (An=U,Np,Pu,Am) in the molten LiCl–KCl eutectic. J. Nucl. Mater. 2005, 344, 173–179. [Google Scholar] [CrossRef]
- He, M.; Lu, G.; Kang, Z.; Zhang, Y. Thermodynamic assessment of the LiCl–KCl–CeCl3 system. Calphad 2015, 49, 1–7. [Google Scholar] [CrossRef]
- Toda, T.; Maruyama, T.; Moritani, K.; Moriyama, H.; Hayashi, H. Thermodynamic properties of lanthanides and actinides for reductive extraction of minor actinides. J. Nucl. Sci. Tech. 2009, 46, 18. [Google Scholar] [CrossRef]



| Elements | T/K | E0/V vs. Ag/AgCl | E0/V vs. Cl2/Cl− | /kJmol−1 [24,25,26] | Activity Coefficient |
|---|---|---|---|---|---|
| Ce3+lCe | 753 | −1.9246 | −3.0708 | −890.1 | 3.012 × 10−3 |
| 763 | −1.9183 | −3.0645 | −887.9 | 3.179 × 10−3 | |
| 773 | −1.9013 | −3.0475 | −884.2 | 3.222 × 10−3 | |
| 783 | −1.8739 | −3.0201 | −877.3 | 3.474 × 10−3 | |
| 793 | −1.8621 | −3.0083 | −871.4 | 3.7013 × 10−3 | |
| Nd2+lNd | 753 | −1.895 | −3.0412 | − | − |
| 763 | −1.882 | −3.0282 | − | − | |
| 773 | −1.858 | −3.0042 | − | − | |
| 783 | −1.851 | −2.9972 | − | − | |
| 793 | −1.843 | −2.9892 | − | − | |
| Nd3+lNd | 753 | −1.989 | −3.1352 | −923.9 | 8.973 × 10−5 |
| 763 | −1.983 | −3.1302 | −914.7 | 9.732 × 10−5 | |
| 773 | −1.978 | −3.1242 | −901.2 | 2.142 × 10−4 | |
| 783 | −1.956 | −3.1022 | −898.0 | 3.245 × 10−4 | |
| 793 | −1.945 | −3.0912 | −879.5 | 3.843 × 10−4 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Kim, S.; Lee, S.-h. Electrochemical Properties of NdCl3 and CeCl3 in Molten LiCl-KCl Eutectic Salt. Appl. Sci. 2020, 10, 7252. https://doi.org/10.3390/app10207252
Kim S, Lee S-h. Electrochemical Properties of NdCl3 and CeCl3 in Molten LiCl-KCl Eutectic Salt. Applied Sciences. 2020; 10(20):7252. https://doi.org/10.3390/app10207252
Chicago/Turabian StyleKim, Seunghyun, and Sang-hwan Lee. 2020. "Electrochemical Properties of NdCl3 and CeCl3 in Molten LiCl-KCl Eutectic Salt" Applied Sciences 10, no. 20: 7252. https://doi.org/10.3390/app10207252
APA StyleKim, S., & Lee, S.-h. (2020). Electrochemical Properties of NdCl3 and CeCl3 in Molten LiCl-KCl Eutectic Salt. Applied Sciences, 10(20), 7252. https://doi.org/10.3390/app10207252




