Nonlinear Dynamics of a Cavity Containing a Two-Mode Coherent Field Interacting with Two-Level Atomic Systems
Abstract
1. Introduction
2. The Physical Model and Its Density Matrices
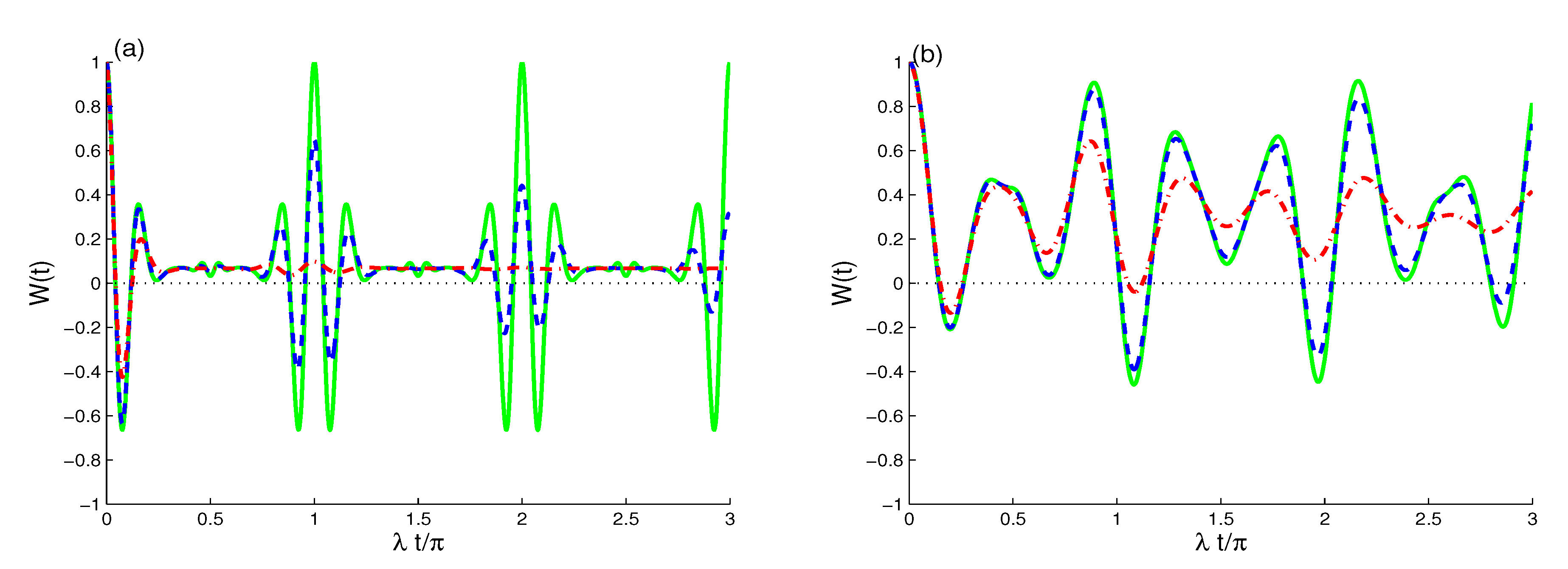
3. The Population Inversion of the Two TLAS
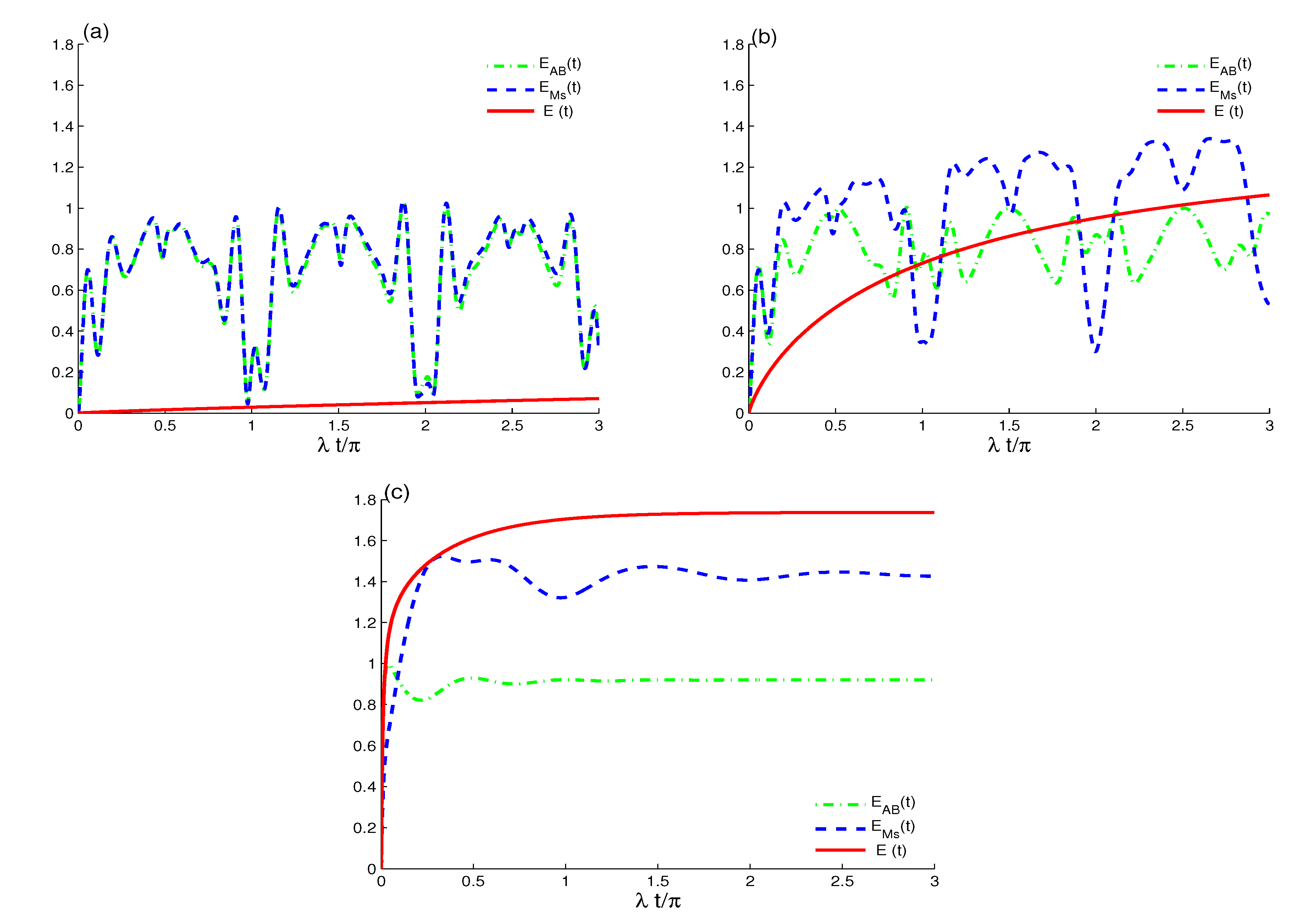
4. Coherence Loss Measures
- (1)
- The TLAS–EMF entropy is numerically calculated using the eigenvalues of the density matrix of the total system in Equation (13):
- (2)
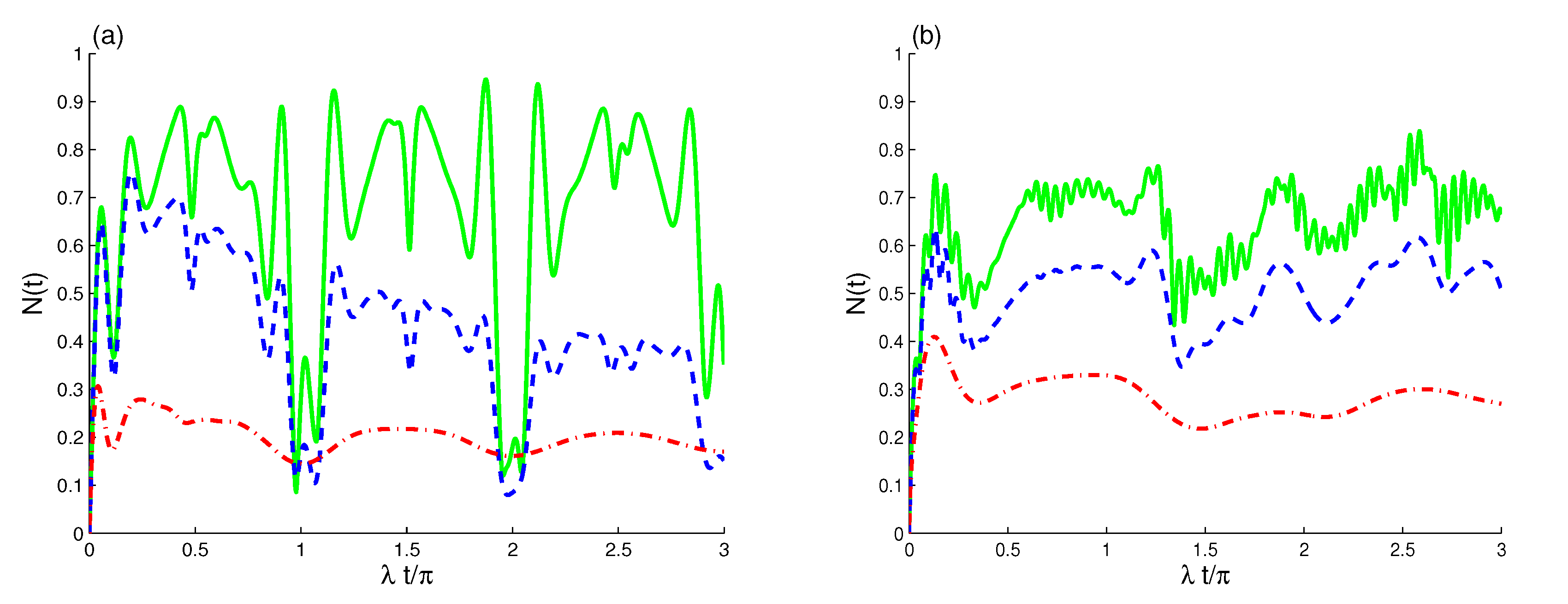
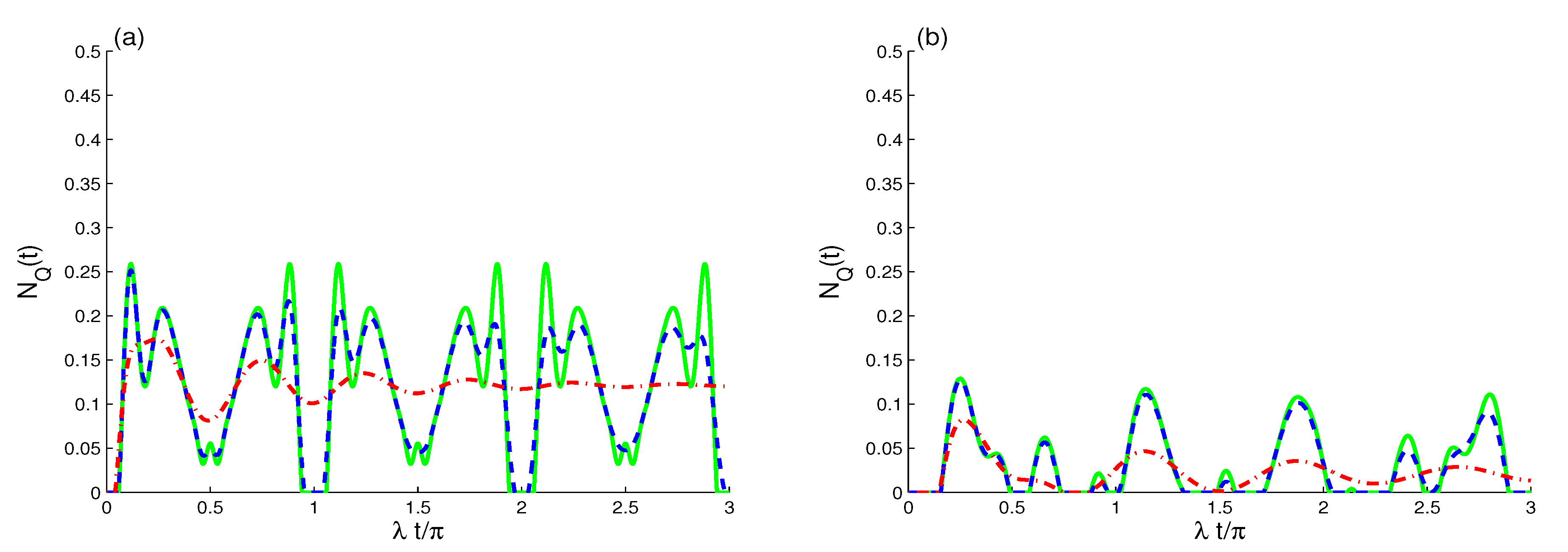
5. The TLAS–EMF System’s Negativity Entanglement
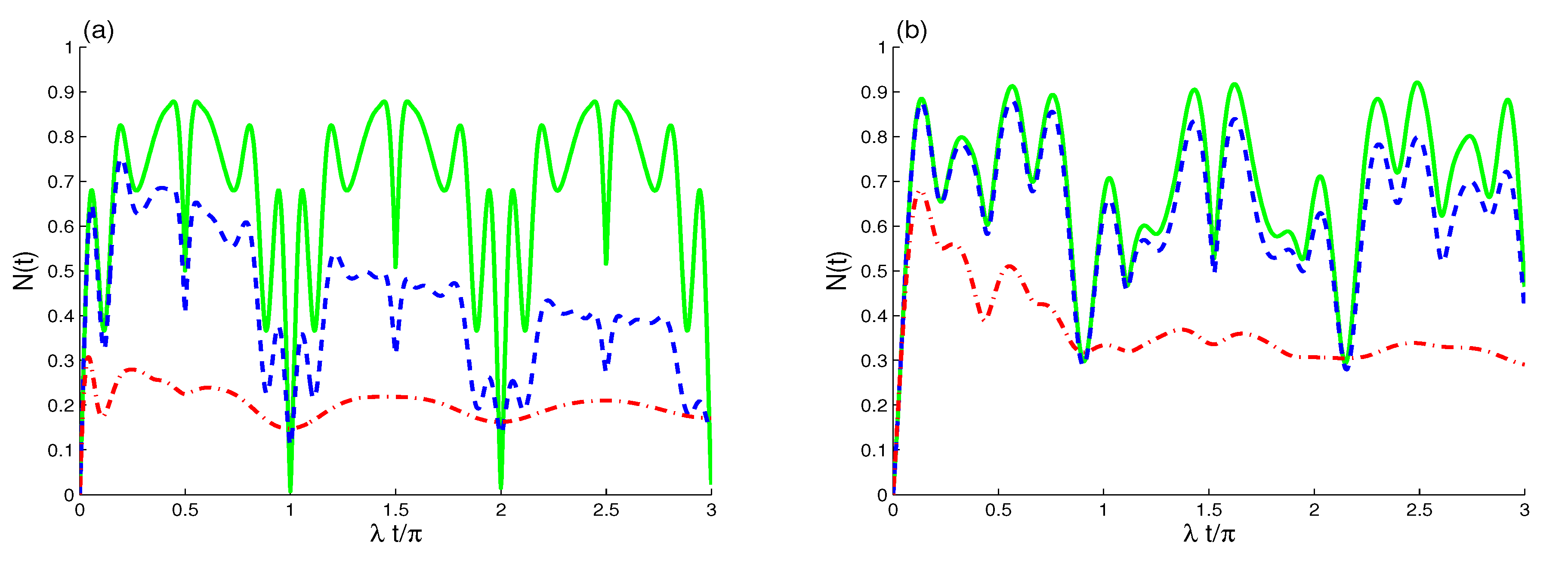
6. Two-Atom System’s Entanglement
7. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Einstein, A.; Podolsky, B.; Rosen, N. Can Quantum-Mechanical Description of Physical Reality Be Considered Complete? Phys. Rev. 1935, 47, 777. [Google Scholar] [CrossRef]
- Brunn, N.; Cavalcanti, D.; Pironio, S.; Scarani, V.; Wehner, S. Bell nonlocality. Rev. Mod. Phys. 2014, 86, 419. [Google Scholar] [CrossRef]
- Streltsov, A.; Adesso, G.; Plenio, M.B. Colloquium: Quantum coherence as a resource. Rev. Mod. Phys. 2017, 89, 041003. [Google Scholar] [CrossRef]
- Vedra, V. Quantum entanglement. Nat. Phys. 2014, 10, 256. [Google Scholar] [CrossRef]
- Franco, R.L.; Compagno, G. Indistinguishability of Elementary Systems as a Resource for Quantum Information Processing. Phys. Rev. Lett. 2018, 120, 240403. [Google Scholar] [CrossRef] [PubMed]
- Sorelli, G.; Leonhard, N.; Shatokhin, V.; Reinlein, C.; Buchleitner, A. Entanglement protection of high-dimensional states by adaptive optics. New J. Phys. 2019, 21, 023003. [Google Scholar] [CrossRef]
- Rab, A.S.; Polino, E.; Man, Z.-X.; An, N.B.; Xia, Y.-J.; Spagnolo, N.; Franco, R.L.; Sciarrino, F. Entanglement of photons in their dual waveparticle nature. Nat. Commun. 2017, 8, 915. [Google Scholar] [CrossRef] [PubMed]
- Bellomo, B.; Franco, R.L.; Compagno, G. Nidentical particles and one particle to entangle them all. Phys. Rev. A 2017, 96, 022319. [Google Scholar] [CrossRef]
- Xu, J.-S.; Sun, K.; Li, C.-F.; Xu, X.-Y.; Guo, G.-C.; Andersson, E.; Franco, R.L.; Compagno, G. Experimental recovery of quantum correlations in absence of system-environment back-action. Nat. Commun. 2013, 4, 2851. [Google Scholar] [CrossRef]
- Barenco, A.; Deutsch, D.; Ekert, A.; Jozsa, R. Conditional Quantum Dynamics and Logic Gates. Phys. Rev. Lett. 1995, 74, 4083. [Google Scholar] [CrossRef]
- Bengtsson, I.; Zyczkowski, K. Geometry of quantum states: An Introduction to Quantum Entanglement; Cambridge University Press: Cambridge, UK, 2006. [Google Scholar] [CrossRef]
- Bennett, C.H.; Brassard, G.; Crepeau, C.; Jozsa, R.; Peres, A.; Wootters, W.K. Teleporting an unknown quantum state via dual classical and Einstein-Podolsky-Rosen channels. Phys. Rev. Lett. 1993, 70, 1895. [Google Scholar] [CrossRef] [PubMed]
- Ren, J.-G.; Xu, P.; Yong, H.-L.; Zhang, L.; Liao, S.-K.; Yin, J.; Liu, W.-Y.; Cai, W.-Q.; Yang, M.; Li, L. Ground-to-satellite quantum teleportation. Nature 2017, 549, 70. [Google Scholar] [CrossRef] [PubMed]
- Krauter, H.; Salart, D.; Muschik, C.A.; Petersen, J.M.; Shen, H.; Fernholz, T.; Polzik, E.S. Deterministic quantum teleportation between distant atomic objects. Nat. Phys. 2013, 9, 400. [Google Scholar] [CrossRef]
- Takeda, S.; Mizuta, T.; Fuwa, M.; van Loock, P.; Furusawa, A. Deterministic quantum teleportation of photonic quantum bits by a hybrid technique. Nature 2013, 500, 315. [Google Scholar] [CrossRef] [PubMed]
- Li, X.; Pan, Q.; Jing, J.; Zhang, J.; Xie, C.; Peng, K. Quantum Dense Coding Exploiting a Bright Einstein-Podolsky-Rosen Beam. Phys. Rev. Lett. 2002, 88, 047904. [Google Scholar] [CrossRef] [PubMed]
- Bennett, C.H.; Wiesner, S.J. Communication via one- and two-particle operators on Einstein-Podolsky-Rosen states. Phys. Rev. Lett. 1992, 69, 2881. [Google Scholar] [CrossRef] [PubMed]
- Ekert, A.K. Quantum cryptography based on Bell’s theorem. Phys. Rev. Lett. 1991, 67, 661. [Google Scholar] [CrossRef] [PubMed]
- Bose, S.; Knight, P.L.; Plenio, M.B.; Vedral, V. Proposal for Teleportation of an Atomic State via Cavity Decay. Phys. Rev. Lett. 1999, 83, 5158. [Google Scholar] [CrossRef]
- Mohamed, A.-B.A.; Eleuch, H. Generation and robustness of bipartite non-classical correlations in two nonlinear microcavities coupled by an optical fiber. J. Opt. Soc. Am. B 2018, 35, 47. [Google Scholar] [CrossRef]
- Khalil, E.M.; Abdalla, M.S.; Obada, A.-S.F. Pair entanglement of two-level atoms in the presence of a nondegenerate parametric amplifier. J. Phys. B At. Mol. Opt. Phys. 2010, 43, 095507. [Google Scholar] [CrossRef]
- Khalil, E.M.; Abdalla, M.S.; Obada, A.-S.F.; Perina, J. Entropic uncertainty in two two-level atoms interacting with a cavity field in presence of degenerate parametric amplifier. J. Opt. Soc. Am. B 2010, 27, 266. [Google Scholar] [CrossRef]
- Phoenix, S.J.D.; Knight, P.L. Establishment of an entangled atom-field state in the Jaynes-Cummings model. Phys. Rev. A 1991, 44, 6023. [Google Scholar] [CrossRef] [PubMed]
- Obada, A.-S.F.; Mohammed, F.A.; Hessian, H.A.; Mohamed, A.-B.A. Entropies and Entanglement for Initial Mixed State in the Multi-quanta JC Model with the Stark Shift and Kerr-like Medium. Int. J. Theor. Phys. 2007, 46, 1027. [Google Scholar] [CrossRef]
- Man, Z.-X.; Xia, Y.-J.; Franco, R.L. Cavity-based architecture to preserve quantum coherence and entanglement. Sci. Rep. 2015, 5, 13843. [Google Scholar] [CrossRef] [PubMed]
- Rogers, R.; Cummings, N.; Pedrotti, L.M.; Rice, P. Atom-field entanglement in cavity QED: Nonlinearity and saturation. Phys. Rev. A 2017, 96, 052311. [Google Scholar] [CrossRef]
- Mohamed, A.-B.A. Non-local correlations via Wigner Yanase skew information in two SC-qubit having mutual interaction under phase decoherence. Eur. Phys. J. D 2017, 71, 261. [Google Scholar] [CrossRef]
- Mohamed, A.-B.A.; Eleuch, H. Coherence and information dynamics of a Λ-type three-level atom interacting with a damped cavity field. Eur. Phys. J. Plus 2017, 132, 75. [Google Scholar] [CrossRef]
- Nielsen, M.A.; Chuang, I.L. Quantum Computation and Quantum Information; Cambridge University Press: Cambridge, UK, 2000. [Google Scholar]
- Jaynes, E.T.; Cummings, F.W. Comparison of quantum and semiclassical radiation theories with application to the beam maser. Proc. IEEE 1963, 51, 89. [Google Scholar] [CrossRef]
- Mohamed, A.-B.A. Bipartite non-classical correlations for a lossy two connected qubit–cavity systems: trace distance discord and Bell’s non-locality. Quantum Inf. Process. 2018, 17, 96. [Google Scholar] [CrossRef]
- Li, S.-B.; Xu, J.-B. Entanglement, Bell violation, and phase decoherence of two atoms inside an optical cavity. Phys. Rev. A 2005, 72, 022332. [Google Scholar] [CrossRef]
- Liu, T.K.; Wang, J.S.; Feng, J.; Zhan, M.S. Entropy evolution properties in a system of two entangled atoms interacting with light field. Chin. Phys. 2005, 14, 537. [Google Scholar]
- Obada, A.-S.F.; Hessian, H.A.; Mohamed, A.-B.A. Effect of phase-damped cavity on dynamics of tangles of a nondegenerate two-photon JC model. Opt. Commun. 2008, 281, 5189–5193. [Google Scholar] [CrossRef]
- Hessian, H.A.; Mohamed, A.-B.A. Quasi-probability distribution functions for a single trapped ion interacting with a mixed laser field. Laser Phys. 2008, 18, 1217–1223. [Google Scholar] [CrossRef]
- Shi, J.-D.; Xu, S.; Ma, W.-C.; Song, X.-K.; Ye, L. Purifying two-qubit entanglement in nonidentical decoherence by employing weak measurements. Quan. Inf. Procss. 2015, 14, 1387. [Google Scholar] [CrossRef]
- Obada, A.-S.F.; Hessian, H.A.; Mohamed, A.-B.A. The effects of thermal photons on entanglement dynamics for a dispersive Jaynes-Cummings model. Phys. Lett. A 2008, 372, 3699. [Google Scholar] [CrossRef]
- Aolita, L.; de Melo, F.; Davidovich, L. Open-system dynamics of entanglement: a key issues review. Rep. Prog. Phys. 2015, 78, 042001. [Google Scholar] [CrossRef]
- Dijkstra, A.G.; Tanimura, Y. Non-Markovian Entanglement Dynamics in the Presence of System-Bath Coherence. Phys. Rev. Lett. 2010, 104, 250401. [Google Scholar] [CrossRef]
- Franco, R.L.; Bellomo, B.; Maniscalco, S.; Compagno, G. Dynamics Of Quantum Correlations In Two-Qubit Systems Within Non-Markovian Environments. Int. J. Mod. Phys. B 2013, 27, 1345053. [Google Scholar] [CrossRef]
- Milburn, G.J. Intrinsic decoherence in quantum mechanics. Phys. Rev. A 1991, 44, 5401. [Google Scholar] [CrossRef]
- Mohamed, A.-B.A. Pairwise quantum correlations of a three-qubit XY chain with phase decoherence. Quantum Inf. Process. 2013, 12, 1141. [Google Scholar] [CrossRef]
- Obada, A.-S.F.; Abdel-Hafez, A.M.; Hessian, H.A. Influence of intrinsic decoherence on nonclassical effects in the nondegenerate bimodal multiquanta Jaynes-Cummings model. J. Phys. B 1998, 31, 5085. [Google Scholar] [CrossRef]
- Raja, S.H.; Mohammadi, H.; Akhtarshenas, S.J. Geometric discord of the Jaynes-Cummings model: Pure dephasing regime. Eur. Phys. J. D 2015, 69, 14. [Google Scholar] [CrossRef]
- Mohamed, A.-B.; Farouk, A.A.; Yassen, M.F.; Eleuch, H. Quantum Correlation via Skew Information and Bell Function Beyond Entanglement in a Two-Qubit Heisenberg XYZ Model: Effect of the Phase Damping. Appl. Sci. 2020, 10, 3782. [Google Scholar] [CrossRef]
- Mohamed, A.-B.; Eleuch, A.H.; Raymond Ooi, C.H. Non-locality Correlation in Two Driven Qubits Inside an Open Coherent Cavity: Trace Norm Distance and Maximum Bell Function. Sci. Rep. 2019, 9, 19632. [Google Scholar] [CrossRef] [PubMed]
- Mohamed, A.-B.; Eleuch, A.H.; Raymond Ooi, C.H. Quantum coherence and entanglement partitions for two driven quantum dots inside a coherent micro cavity. Phys. Lett. A 2019, 383, 125905. [Google Scholar] [CrossRef]
- Giovannetti, V.; Lloyd, S.; Maccone, L. Quantum-enhanced positioning and clock synchronization. Nature 2001, 412, 417. [Google Scholar] [CrossRef] [PubMed]
- Franken, P.A.; Hill, A.E.; Peters, C.W.; Weinreich, G. Generation of Optical Harmonics. Phys. Rev. Lett. 1961, 7, 118. [Google Scholar] [CrossRef]
- Roy, A.; Devoret, M. Introduction to parametric amplification of quantum signals with Josephson circuitsIntroduction a lamplification parametrique de signaux quantiques par les circuits Josephson. Comptes Rendus Phys. 2016, 17, 740. [Google Scholar] [CrossRef]
- Rekdal, P.K.; Skagerstam, B.-S.K. Quantum Dynamics of Non-Degenerate Parametric Amplification. Phys. Scr. 2000, 61, 296. [Google Scholar] [CrossRef]
- Dehghani, A.; Braz, B.M. Minimum Uncertainty Coherent States Attached to Nondegenerate Parametric Amplifiers. Braz. J. Phys. 2015, 45, 265. [Google Scholar] [CrossRef]
- Gardiner, C.W. Quantum Noise; Springer: Berlin, Germany, 1991. [Google Scholar]
- Moya-Cessa, H.; Buzek, V.; Kim, M.S.; Knight, P.L. Intrinsic decoherence in the atom-field interaction. Phys. Rev. A 1993, 48, 3900. [Google Scholar] [CrossRef] [PubMed]
- Xu, J.-B.; Zou, X.-B. Dynamic algebraic approach to the system of a three-level atom in the Λ configuration. Phys. Rev. A 1999, 60, 4743. [Google Scholar] [CrossRef]
- de los Santos-Sánchez, O.; Gonzxaxlez-Gutiérrez, C.; Rxexcamier, J. Nonlinear Jaynes—Cummings model for two interacting two-level atoms. J. Phys. B At. Mol. Opt. Phys. 2016, 49, 165503. [Google Scholar]
- Barut, A.O.; Girardello, L. New “coherent” states associated with non-compact groups. Commun. Math. Phys. 1971, 21, 41. [Google Scholar] [CrossRef]
- Gerry, C.C.; Moyer, P.J. Squeezing and higher-order squeezing in one- and two-photon Jaynes-Cummings models. Phys. Rev. A 1988, 38, 5665. [Google Scholar] [CrossRef]
- Bennett, C.H.; Bernstein, H.J.; Popescu, S.; Schumacher, B. Concentrating partial entanglement by local operations. Phys. Rev. A 1996, 53, 2046. [Google Scholar] [CrossRef]
- Araki, H.; Lieb, E. Entropy inequalities. Commun. Math. Phys. 1970, 18, 160. [Google Scholar] [CrossRef]
- Vidal, G.; Werner, R.F. Computable measure of entanglement. Phys. Rev. A 2002, 65, 032314. [Google Scholar] [CrossRef]
- Eberly, J.H.; Yu, T. The End of an Entanglement. Science 2007, 316, 555. [Google Scholar] [CrossRef]
- Yu, T.; Eberly, J.H. Sudden Death of Entanglement. Science 2009, 323, 598. [Google Scholar] [CrossRef]
- Mohamed, A.-B.A.; Hessian, H.A.; Obada, A.-S.F. Entanglement sudden death of a SC-qubit strongly coupled with a quantized mode of a lossy cavity. Physica A 2011, 390, 519. [Google Scholar] [CrossRef]
- Xu, H.; Yong, L.; Liao, S.; Yin, J.; Cai, W.; Yang, M.; Li, L.; Yang, K.; Han, X.; Yao, Y.; et al. Ground-to-satellite quantum teleportation. Nature 2017, 549, 70. [Google Scholar]
- Chou, K.S.; Blumoff Jacob, Z.; Wang, C.S.; Reinhold, P.C.; Axline, C.J.; Gao, Y.Y.; Frunzio, L.; Devoret, M.H.; Jiang, L.; Schoelkopf, R.J. Deterministic teleportation of a quantum gate between two logical qubits. Nature 2018, 561, 368. [Google Scholar] [CrossRef] [PubMed]
- Nielsen, M.A.; Chuang, I.L. Application: Superdense Coding. Quantum Computation and Quantum Information, 10th ed.; Cambridge University Press: Cambridge, UK, 2010. [Google Scholar]
- Siomau, M.; Fritzsche, S. Quantum computing with mixed states. Eur. Phys. J. D 2011, 62, 449. [Google Scholar] [CrossRef]
- Hou, S.-Y.; Sheng, Y.-B.; Feng, G.-R.; Long, G.-L. Experimental Optimal Single Qubit Purification in an NMR Quantum Information Processor. Sci. Rep. 2015, 4, 6857. [Google Scholar] [CrossRef] [PubMed]
- Collins, D. Qubit-channel metrology with very noisy initial states. Phys. Rev. A 2019, 99, 012123. [Google Scholar] [CrossRef]




Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Khalil, E.M.; M. Alshehri, H.; Mohamed, A.-B.A.; Abdel-Khalek, S.; Obada, A.-S.F. Nonlinear Dynamics of a Cavity Containing a Two-Mode Coherent Field Interacting with Two-Level Atomic Systems. Appl. Sci. 2020, 10, 7150. https://doi.org/10.3390/app10207150
Khalil EM, M. Alshehri H, Mohamed A-BA, Abdel-Khalek S, Obada A-SF. Nonlinear Dynamics of a Cavity Containing a Two-Mode Coherent Field Interacting with Two-Level Atomic Systems. Applied Sciences. 2020; 10(20):7150. https://doi.org/10.3390/app10207150
Chicago/Turabian StyleKhalil, E. M., Hashim M. Alshehri, A.-B. A. Mohamed, S. Abdel-Khalek, and A.-S. F. Obada. 2020. "Nonlinear Dynamics of a Cavity Containing a Two-Mode Coherent Field Interacting with Two-Level Atomic Systems" Applied Sciences 10, no. 20: 7150. https://doi.org/10.3390/app10207150
APA StyleKhalil, E. M., M. Alshehri, H., Mohamed, A.-B. A., Abdel-Khalek, S., & Obada, A.-S. F. (2020). Nonlinear Dynamics of a Cavity Containing a Two-Mode Coherent Field Interacting with Two-Level Atomic Systems. Applied Sciences, 10(20), 7150. https://doi.org/10.3390/app10207150




