Numerical and Probabilistic Study on the Optimal Region for Tsunami Detection Instrument Deployment in the Eastern Sea of Korea
Abstract
1. Introduction
2. Materials and Methods
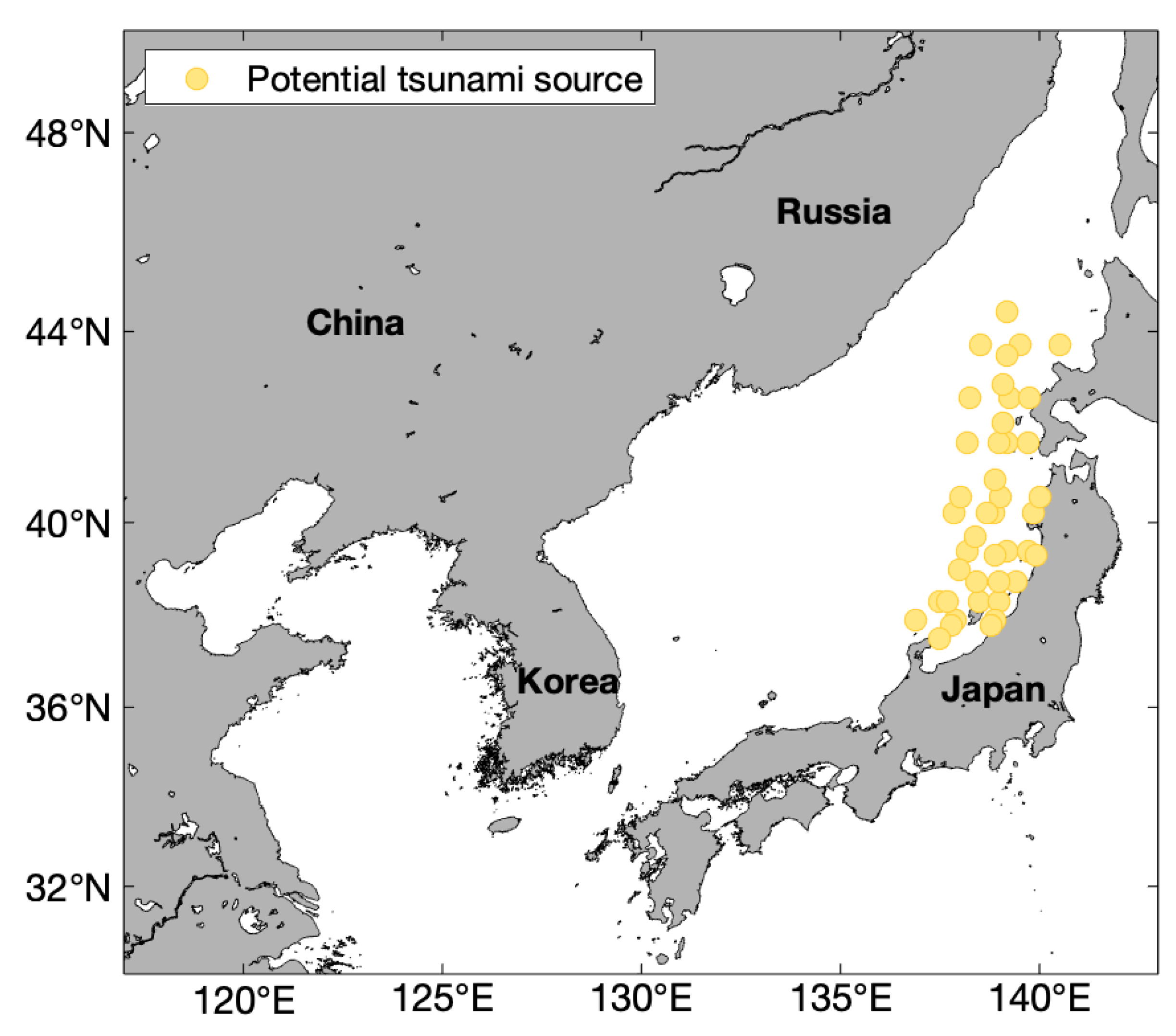
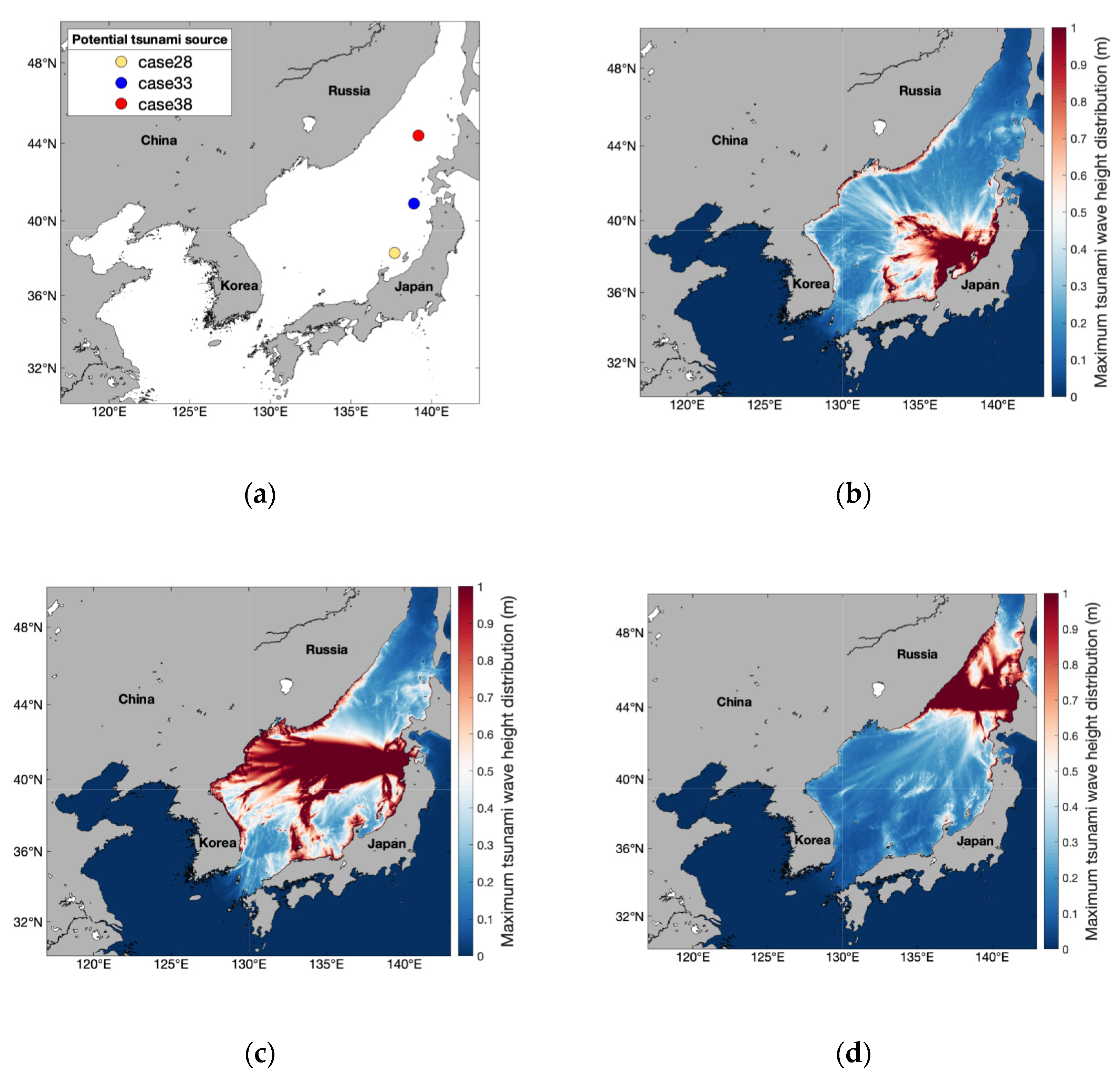
2.1. Potential Tsunami Scenario
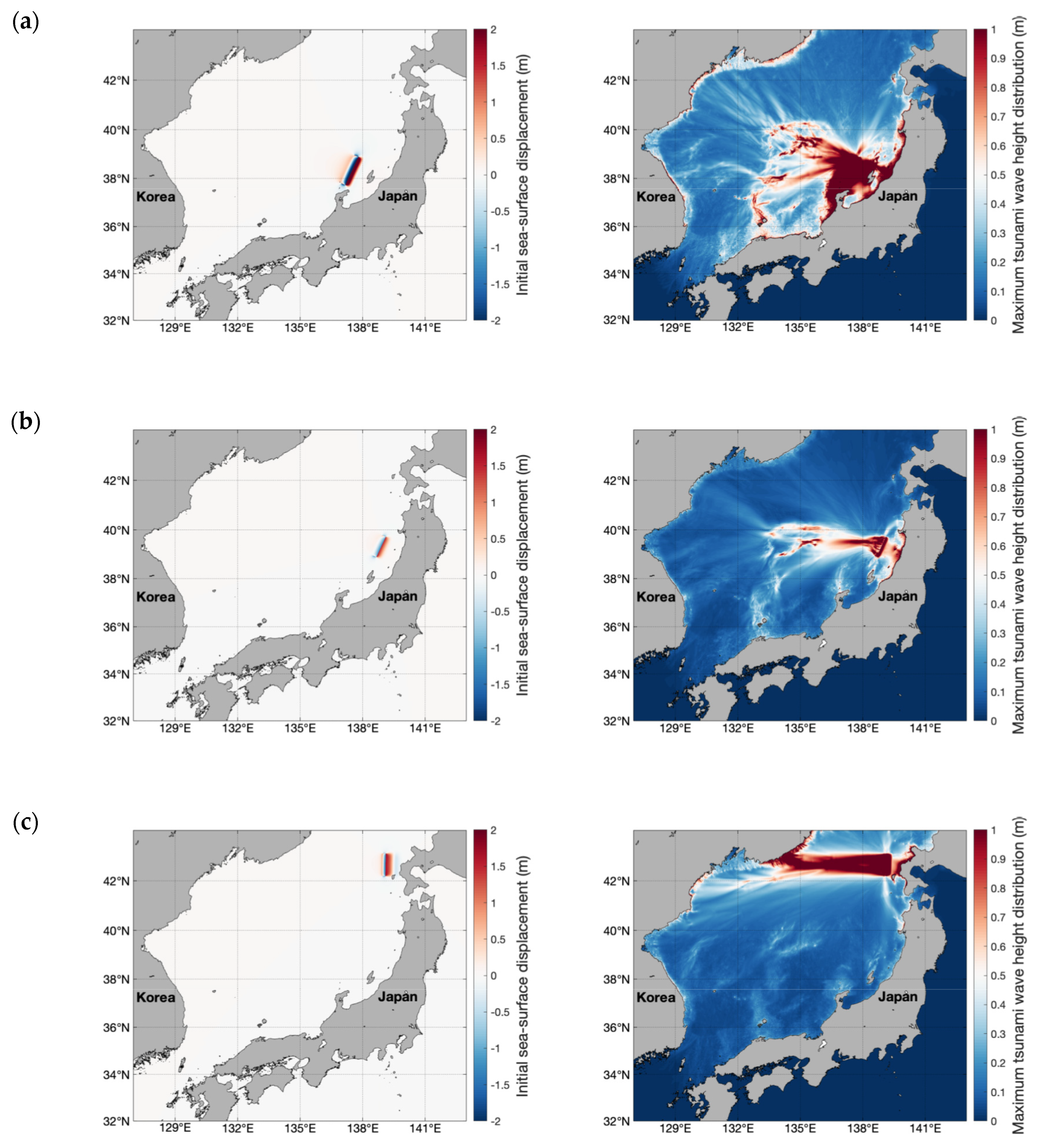
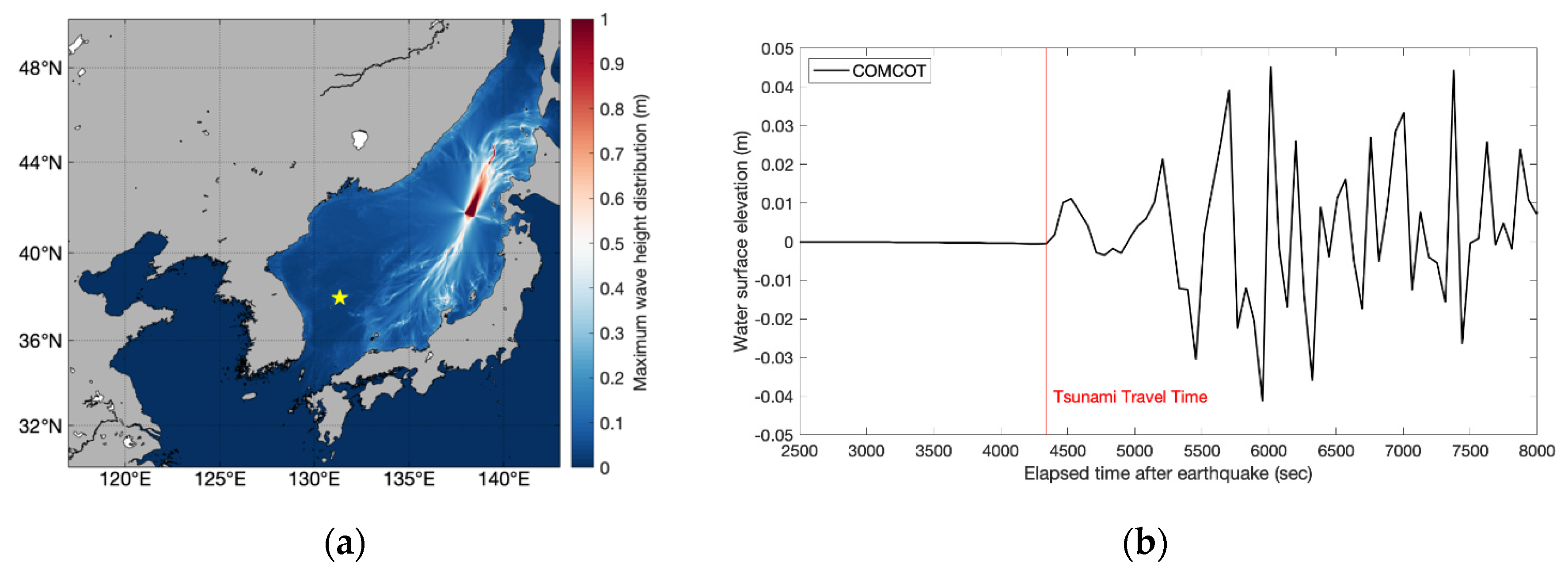
2.2. Numerical Model
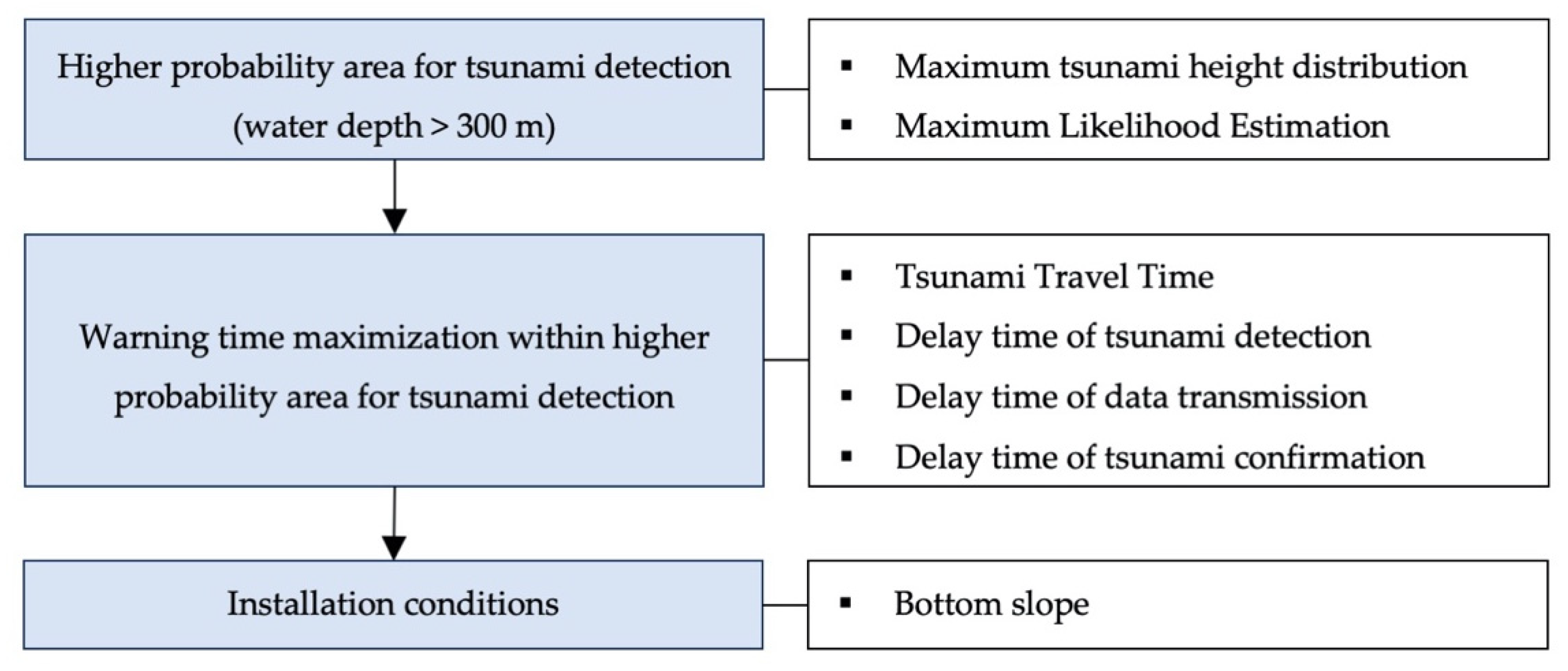
2.3. The Method to Determine Optimal Offshore Tsunami Observation Instrument Regions
3. Results
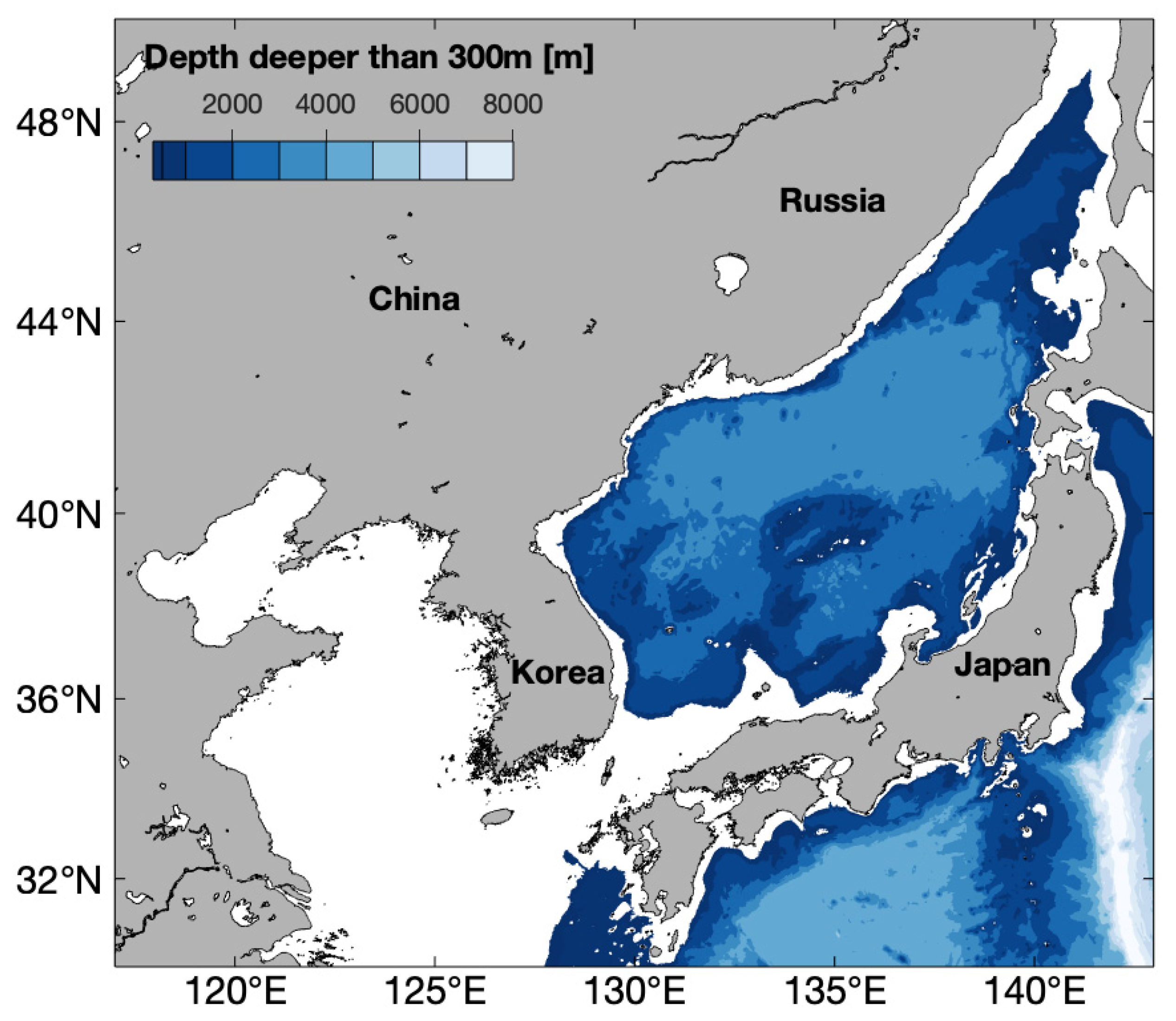
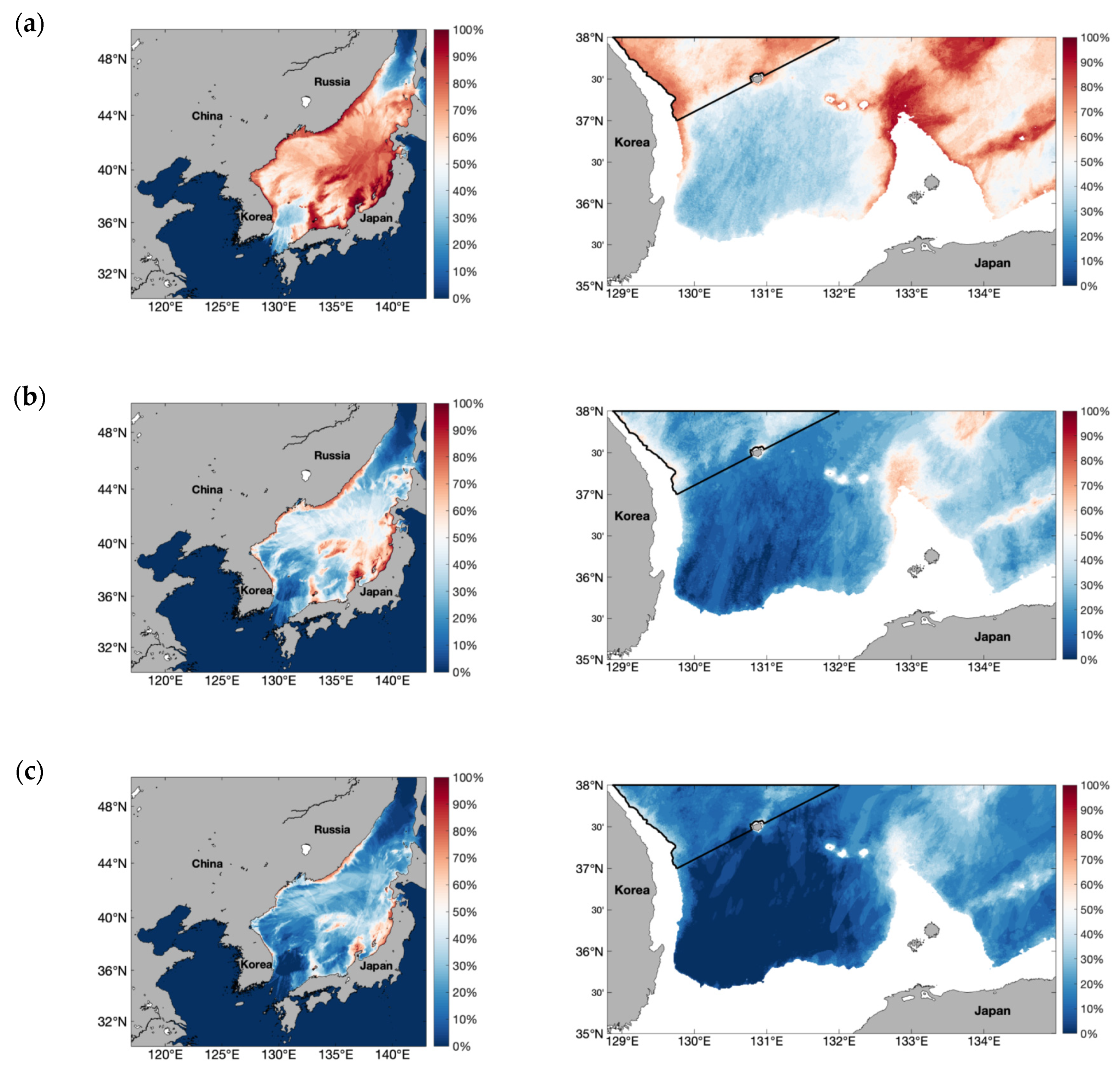
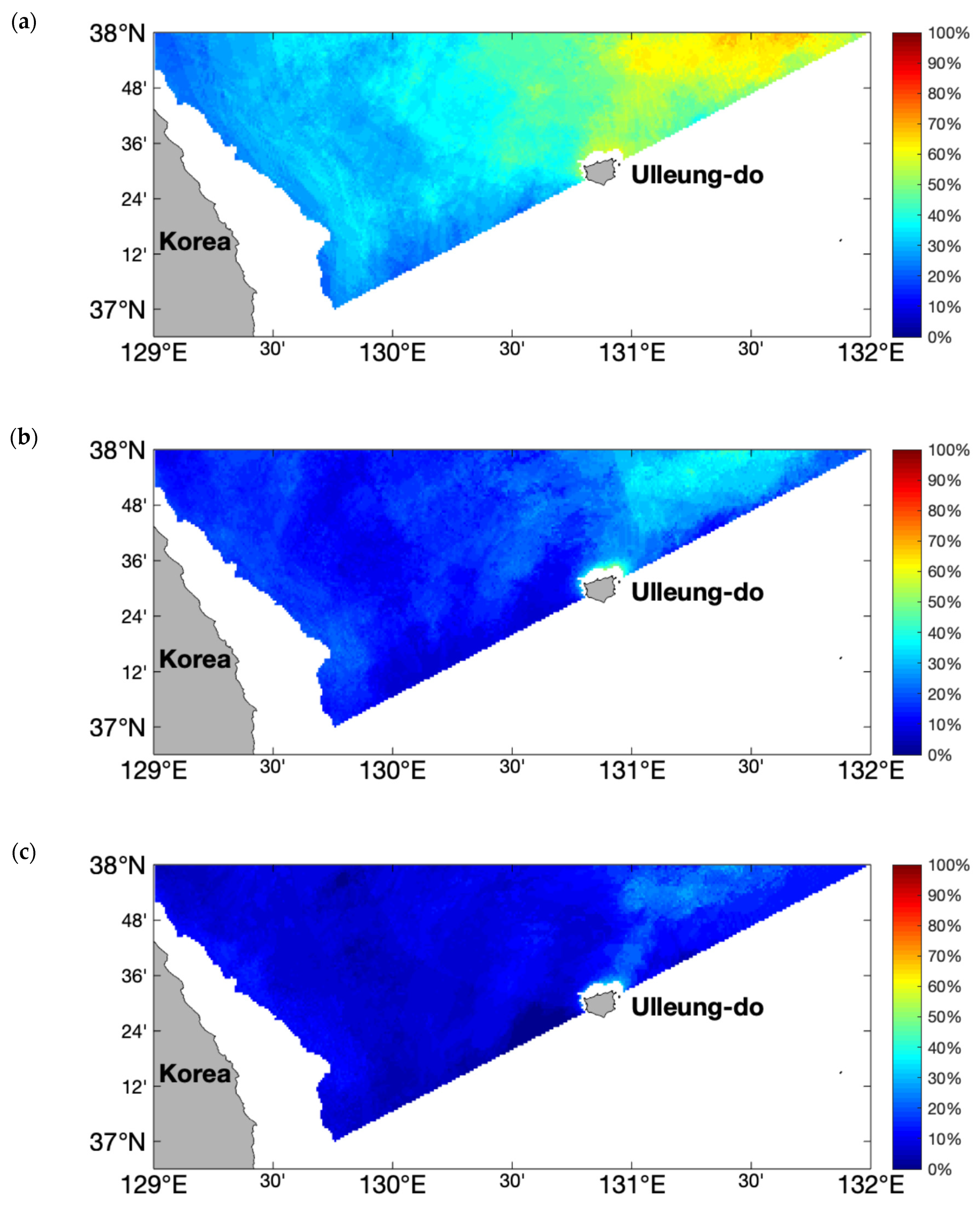
3.1. Higher Probability Area for Tsunami Detection
3.2. Warning Time Maximization
3.3. Installation Conditions: Bottom Slope
4. Discussion
5. Conclusions
- Define a high probability area for tsunami detection using numerical modeling based on 39 potential tsunami scenarios.
- Define the probability area of maximum warning time by considering tsunami travel time, the delay time of detection, data transmission time, and measurement confirmation time.
- Specify the probability map for the optimal region of offshore tsunami instrument by the product of the probability of tsunami detection and warning time.
- Consider the bottom slope required for tsunami instrument installation.
Author Contributions
Funding
Conflicts of Interest
Appendix A
| Case | Location | H (km) | θ (°) | δ (°) | λ (°) | L (km) | W (km) | D (m) | |
|---|---|---|---|---|---|---|---|---|---|
| Latitude (°N) | Longitude (°E) | ||||||||
| 1 | 41.7 | 138.2 | 3 | 110 | 45 | 100 | 45 | 25 | 2.3 |
| 2 | 41.7 | 139.7 | 3 | 110 | 45 | 100 | 45 | 25 | 2.3 |
| 3 | 38.3 | 137.5 | 1 | 23 | 35 | 90 | 140 | 50 | 5 |
| 4 | 38.3 | 138.5 | 1 | 23 | 35 | 90 | 140 | 50 | 5 |
| 5 | 38.3 | 139 | 1 | 23 | 35 | 90 | 140 | 50 | 5 |
| 6 | 39.4 | 138.2 | 1 | 105 | 45 | 90 | 100 | 50 | 4.1 |
| 7 | 39.4 | 139.2 | 1 | 105 | 45 | 90 | 100 | 50 | 4.1 |
| 8 | 39.4 | 139.7 | 1 | 105 | 45 | 90 | 100 | 50 | 4.1 |
| 9 | 39.3 | 138.9 | 1 | 23 | 45 | 75 | 100 | 50 | 2 |
| 10 | 39.3 | 139.9 | 1 | 23 | 45 | 75 | 100 | 50 | 2 |
| 11 | 37.9 | 136.9 | 1 | 15 | 20 | 90 | 70 | 40 | 3.2 |
| 12 | 37.9 | 137.9 | 1 | 15 | 20 | 90 | 70 | 40 | 3.2 |
| 13 | 37.9 | 138.9 | 1 | 15 | 20 | 90 | 70 | 40 | 3.2 |
| 14 | 37.8 | 137.8 | 1 | 190 | 55 | 90 | 60 | 20 | 1.9 |
| 15 | 37.8 | 138.8 | 1 | 190 | 55 | 90 | 60 | 20 | 1.9 |
| 16 | 43.73 | 138.53 | 1 | 347 | 40 | 90 | 100 | 35 | 5.35 |
| 17 | 43.73 | 139.53 | 1 | 347 | 40 | 90 | 100 | 35 | 5.35 |
| 18 | 43.73 | 140.53 | 1 | 347 | 40 | 90 | 100 | 35 | 5.35 |
| 19 | 38.74 | 138.42 | 1 | 189 | 56 | 90 | 80 | 30 | 7.81 |
| 20 | 38.74 | 139.42 | 1 | 189 | 56 | 90 | 80 | 30 | 7.81 |
| 21 | 38.74 | 139 | 1 | 189 | 56 | 90 | 80 | 30 | 7.81 |
| 22 | 40.21 | 137.84 | 1 | 22 | 40 | 90 | 40 | 30 | 7.6 |
| 23 | 40.21 | 138.84 | 1 | 22 | 40 | 90 | 40 | 30 | 7.6 |
| 24 | 40.21 | 139.84 | 1 | 22 | 40 | 90 | 40 | 30 | 7.6 |
| 25 | 42.63 | 138.24 | 5 | 1 | 24 | 84 | 100 | 50 | 3.7 |
| 26 | 42.63 | 139.24 | 5 | 1 | 24 | 84 | 100 | 50 | 3.7 |
| 27 | 42.63 | 139.74 | 5 | 1 | 24 | 84 | 100 | 50 | 3.7 |
| 28 | 38.3 | 137.7 | 1 | 14.5 | 40 | 90 | 125.89 | 62.95 | 6.31 |
| 29 | 37.5 | 137.5 | 1 | 0 | 40 | 90 | 125.89 | 62.95 | 6.31 |
| 30 | 39 | 138 | 1 | 27.5 | 40 | 90 | 125.89 | 62.95 | 6.31 |
| 31 | 39.7 | 138.4 | 1 | 17 | 40 | 90 | 125.89 | 62.95 | 6.31 |
| 32 | 40.2 | 138.7 | 1 | 10 | 40 | 90 | 125.89 | 62.95 | 6.31 |
| 33 | 40.9 | 138.9 | 1 | 1 | 40 | 90 | 125.89 | 62.95 | 6.31 |
| 34 | 41.7 | 139 | 1 | 1 | 40 | 90 | 125.89 | 62.95 | 6.31 |
| 35 | 42.1 | 139.1 | 1 | 4 | 40 | 90 | 125.89 | 62.95 | 6.31 |
| 36 | 42.9 | 139.1 | 1 | 2 | 40 | 90 | 125.89 | 62.95 | 6.31 |
| 37 | 43.5 | 139.2 | 1 | 2 | 40 | 90 | 125.89 | 62.95 | 6.31 |
| 38 | 44.4 | 139.2 | 1 | 3 | 40 | 90 | 125.89 | 62.95 | 6.31 |
| 39 | 38.3 | 137.7 | 1 | 14.5 | 40 | 90 | 125.9 | 62.9 | 6.3 |
References
- Pugh, D.; Woodworth, P. Sea-Level Science: Understanding Tides, Surges, Tsunamis and Mean Sea-Level Changes; Cambridge University Press: Cambridge, UK, 2014. [Google Scholar] [CrossRef]
- Mori, N.; Goda, K.; Cox, D. Recent Process in Probabilistic Tsunami Hazard Analysis (PTHA) for Mega Thrust Subduction Earthquakes. In The 2011 Japan Earthquake and Tsunami: Reconstruction and Restoration; Springer: Cham, Switzerland, 2018; pp. 469–485. [Google Scholar] [CrossRef]
- Mulia, I.E.; Gusman, A.R.; Satake, K. Optimal Design for Placements of Tsunami Observing Systems to Accurately Characterize the Inducing Earthquake. Geophys. Res. Lett. 2017, 12, 12,106–12,115. [Google Scholar] [CrossRef]
- Kawai, H.; Satoh, M.; Kawaguchi, K.; Seki, K. Characteristics of the 2011 Tohoku Tsunami Waveform Acquired Around Japan by Nowphas Equipment. Coast. Eng. J. 2018, 55, 1350008-1–1350008-27. [Google Scholar] [CrossRef]
- Percival, D.B.; Denbo, D.W.; Eblé, M.C.; Gica, E.; Mofjeld, H.O.; Spillane, M.C.; Tang, L.; Titov, V. V Extraction of tsunami source coefficients via inversion of DART buoy data. Nat. Hazards 2010, 58, 567–590. [Google Scholar] [CrossRef]
- Takagawa, T.; Tomita, T. Effects of Rupture Processes in an Inverse Analysis on the Tsunami Source of the 2011 off the Pacific Coast of Tohoku Earthquake. In Proceedings of the 22th International Society of Offshore and Polar Engineers (ISOPE), Rhodes, Greece, 17–22 June 2012. [Google Scholar]
- Japan Society of Civil Engineers. Available online: http://committees.jsce.or.jp/ceofnp/system/files/NPP_TNMT_2016_main_20170814.pdf (accessed on 15 September 2019).
- Levin, B.W.; Nosov, M. Physics of Tsunamis; Springer International Publishing: Cham, Switzerland, 2009; 237p. [Google Scholar]
- Choi, B.H.; Hong, S.J.; Pelinovsky, E. Simulation of prognostic tsunamis on the Korean Coast. Geophys. Res. Lett. 2001, 28, 2013–2016. [Google Scholar] [CrossRef]
- Kim, B.J.; Cho, Y.-S. Determination of Tsunami Height Distribution with L-moment Method. J. Korean Soc. Hazard Mitig. 2014, 14, 311–317. [Google Scholar] [CrossRef]
- Rehman, K.; Cho, Y.-S. Building Damage Assessment Using Scenario Based Tsunami Numerical Analysis and Fragility Curves. Water 2016, 8, 109. [Google Scholar] [CrossRef]
- Liu, P.L.F.; Cho, Y.S.; Yoon, S.B.; Seo, S.N. Numerical Simulations of the 1960 Chilean Tsunami Propagation and Inundation at Hilo, Hawaii. In Tsunami: Progress in Prediction, Disaster Prevention and Warning. Advances in Natural and Technological Hazards Research.; Tsuchiya, Y., Shuto, N., Eds.; Springer: Dordrecht, Switzerland, 1995; Volume 4, pp. 99–115. [Google Scholar]
- Yoon, S.B. Propagation of distant tsunamis over slowly varying topography. J. Geophys. Res. 2002, 107, 4-1–4-11. [Google Scholar] [CrossRef]
- Wang, X. Numerical Modelling of Surface and Internal Waves over Shallow and Intermediate Water. Ph.D. Dissertation, Cornell University, New York, NY, USA, August 2008. [Google Scholar]
- Wu, T.-R.; Chen, P.-F.; Tsai, W.-T.; Chen, G.-Y. Numerical Study on Tsunamis Excited by 2006 Pingtung Earthquake Doublet. Terr. Atmos. Ocean. Sci. 2008, 19, 705–715. [Google Scholar] [CrossRef]
- Lee, J.-W.; Park, E.H.; Park, S.-C.; Woo, S.-B. Development of the Global Tsunami Prediction System using the Finite Fault Model and the Cyclic Boundary Condition. J. Korean Soc. Coast. Ocean Eng. 2015, 27, 391–405. [Google Scholar] [CrossRef][Green Version]
- Mueller, C.; Power, W.; Fraser, S.; Wang, X. Effects of rupture complexity on local tsunami inundation: Implications for probabilistic tsunami hazard assessment by example. J. Geo Res. Solid Earth 2015, 120, 488–502. [Google Scholar] [CrossRef]
- Lee, E.; Jung, T.; Kim, J.-C.; Shin, S. A Study of the Optimal Deployment of Tsunami Observation Instruments in Korea. J. Ocean Eng. Technol. 2019, 33, 607–614. [Google Scholar] [CrossRef]
- Meza, J.; Catalán, P.A.; Tsushima, H. A Methodology For Optimal Designing Of Monitoring Sensor Networks For Tsunami Inversion. Nat. Hazards Earth Syst. Sci. Discuss. 2018. under review. [Google Scholar]
- Jeon, Y.J.; Lee, S.M.; Lim, C.H.; Yoon, S.B. Propagation Characteristics of 1983 Central East Sea Tsunami. In Proceedings of the Korean Society of Civil Engineers, Daegu, Korea, 11 October 2007. [Google Scholar]
- Omira, R.; Baptista, M.A.; Matias, L.; Miranda, J.M.; Catita, C.; Carrilho, F.; Toto, E. Design of a Sea-level Tsunami Detection Network for the Gulf of Cadiz. Nat. Hazards Earth Syst. Sci. 2009, 9, 1327–1338. [Google Scholar] [CrossRef]
- Schindele, F.; Loevenbruck, A.; Hebert, H. Strategy to design the sea-level monitoring networks for small tsunamigenic oceanic basins: The Western Mediterranean case. Nat. Hazards Earth Syst. Sci. 2008, 8, 1019–1027. [Google Scholar] [CrossRef][Green Version]
- Araki, E.; Kawaguchi, K.; Kaneko, S.; Kaneda, Y. Design of Deep Ocean Submarine Cable Observation Network for Earthquakes and Tsunamis. In Proceedings of the OCEANS 2008-MTS/IEEE Kobe Techno-Ocean, Kobe, Japan, 8–11 April 2008. [Google Scholar]
- Abe, I.; Imamura, F. Problems and effects of a tsunami inundation forecast system during the 2011 Tohoku earthquake. J. Jpn. Soc. Civ. Eng. 2013, 1, 516–520. [Google Scholar] [CrossRef]
- Gusman, A.R.; Murotani, S.; Satake, K.; Heidarzadeh, M.; Gunawan, E.; Watada, S.; Schurr, B. Fault slip distribution of the 2014 Iquique, Chile, earthquake estimated from ocean-wide tsunami waveforms and GPS data. Geophys. Res. Lett. 2015, 42, 1053–1060. [Google Scholar] [CrossRef]
- Miura, K. An Introduction to Maximum Likelihood Estimation and Information Geometry. Interdiscip. Inf. Sci. 2017, 17, 155–174. [Google Scholar] [CrossRef]
- Okal, E.A. The quest for wisdom: Lessons from 17 tsunamis, 2004–2014. Philos Trans. Math. Phys. Eng. Sci. 2015, 373, 20140370. [Google Scholar] [CrossRef] [PubMed]
- IOC-UNESCO. An Intra Americas Sea Tsunami Warning System Project Proposal; UNESCO Intergovernmental Oceanographic Commission: Paris, France, 2005. [Google Scholar]
- Kim, H.S.; Kim, H.S.; Kang, Y.S. The Investigation of Tsunami Occurrence and Warning System. In Proceedings of the Korean Society of Marine Engineering, Gyeongju, Korea, 26–28 June 2008. [Google Scholar]
- Outsourced Setting of Okinawa Tsunami Inundation Assumptions. Available online: https://www.pref.okinawa.jp/site/doboku/kaibo/h27tunami/documents/gaiyoubann4-1.pdf (accessed on 12 August 2019).



| Domain | Grid Size | Mesh Number | Time Step Size (Δt) | Numerical Model | ||
|---|---|---|---|---|---|---|
| Longitude | Latitude | Δx | Δy | |||
| 116.9–142.9° E | 29.9–49.9° N | 0.5 arc minute | 0.5 arc minute | 3119 × 2400 | 1 s | Linear |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Lee, E.; Jung, T.; Shin, S. Numerical and Probabilistic Study on the Optimal Region for Tsunami Detection Instrument Deployment in the Eastern Sea of Korea. Appl. Sci. 2020, 10, 6071. https://doi.org/10.3390/app10176071
Lee E, Jung T, Shin S. Numerical and Probabilistic Study on the Optimal Region for Tsunami Detection Instrument Deployment in the Eastern Sea of Korea. Applied Sciences. 2020; 10(17):6071. https://doi.org/10.3390/app10176071
Chicago/Turabian StyleLee, Eunju, Taehwa Jung, and Sungwon Shin. 2020. "Numerical and Probabilistic Study on the Optimal Region for Tsunami Detection Instrument Deployment in the Eastern Sea of Korea" Applied Sciences 10, no. 17: 6071. https://doi.org/10.3390/app10176071
APA StyleLee, E., Jung, T., & Shin, S. (2020). Numerical and Probabilistic Study on the Optimal Region for Tsunami Detection Instrument Deployment in the Eastern Sea of Korea. Applied Sciences, 10(17), 6071. https://doi.org/10.3390/app10176071






