1. Introduction
Quantum teleportation is a process of transmitting unknown quantum states between two distant nodes based on entanglement and some auxiliary classical communication. Since Bennett proposed the concept of quantum teleportation [
1], where Einstein–Podolsky–Rosen (EPR) pairs are used as an entanglement channel, quantum teleportation has been paid extensive attention in recent years. Some quantum teleportation schemes have been proposed by using Greenberger–Horne–Zeilinger (GHZ) states, mixed W states, and other entangled states as quantum channels [
2,
3,
4,
5].
To realize qubit transmission in a quantum communication network, Wang et al. [
6] proposed the idea of quantum wireless multi-hop communication based on arbitrary EPR pairs and teleportation, where simultaneous entanglement swapping is utilized to reduce the end-to-end quantum communication delay. To overcome the decoherence effects caused by the surrounding environments and take advantage of partially entangled EPR pairs efficiently, Yu et al. [
7] proposed a wireless quantum communication scheme. However, hop-by-hop qubit transmission introduces great communication delay and information leakage at the intermediate nodes. After that, Chen et al. [
8] proposed a wide area quantum communication network via partially entangled EPR states, where sequential entanglement swapping is exploited. In this scheme, the security of qubit transmission is improved, while end-to-end quantum communication delay is not reduced to a great extent. Compared to two-particle entanglement, multi-particle entangled states are equipped with better entanglement properties, and are more powerful in revealing the nonlocality of quantum physics [
9,
10]. Zhan and Zou et al. proposed multi-hop quantum teleportation schemes based on W states and GHZ–Bell channels [
11,
12]. Considering that duplex quantum communication between two arbitrary nodes is crucial to the future quantum networks, Li et al. [
13] first proposed the bidirectional controlled quantum transmission scheme using a five-qubit entangled state. Since then, some schemes for bidirectional quantum communication have been proposed [
14,
15,
16,
17,
18,
19,
20,
21].
To address all the issues mentioned above, such as quantum communication delay, information leakage, and duplex quantum communication, in this paper we investigate multi-hop bidirectional quantum communication based on GHZ states, considering that GHZ states are less prone to the decoherence effects caused by the surrounding environment [
22]. The main contribution of this work is as follows.
We propose a scheme for multi-hop bidirectional quantum communication using the composite GHZ-GHZ channel, where all the nodes perform quantum measurements in parallel. After that, they send the measurement results and the types of previously shared GHZ-GHZ states to the sender and receiver through the classical channel independently. Based on the received information, the sender and receiver perform appropriate unitary operations to recover the original qubit. Our scheme has a shorter delay for the end-to-end quantum communication and avoids information leakage at the intermediate nodes.
The rest of this paper is organized as follows. In
Section 2, one-hop bidirectional quantum communication based on a GHZ-GHZ entangled channel is discussed. In
Section 3, we investigate two-hop directional quantum communication. In
Section 4, a scheme for multi-hop bidirectional quantum communication is proposed. Finally, some discussions and conclusions are given in
Section 5.
2. One-Hop Bidirectional Quantum Communication
In this section, we investigate one-hop bidirectional quantum communication through the composite GHZ-GHZ states. Eight types of GHZ states used in quantum communication are given by
These GHZ states can be transformed into each other through unitary operations, such as
where
,
are Pauli matrices, and
is the identity matrix.
Assume that Alice intends to transmit an arbitrary qubit
to Bob, while Bob intends to transmit
to Alice at the same time,
and
are given by
where
,
,
and
are complex probability amplitudes satisfying
,
.
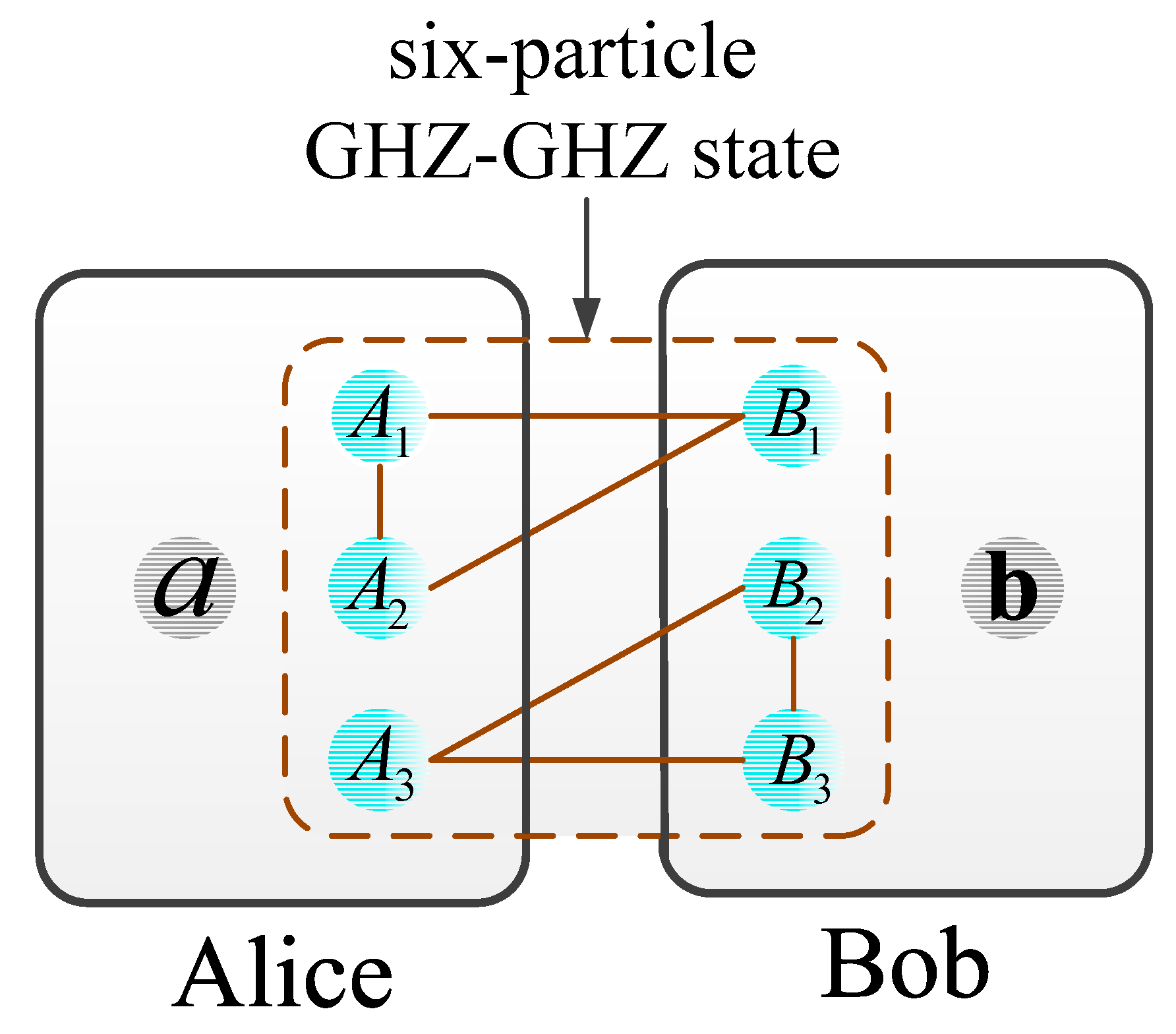
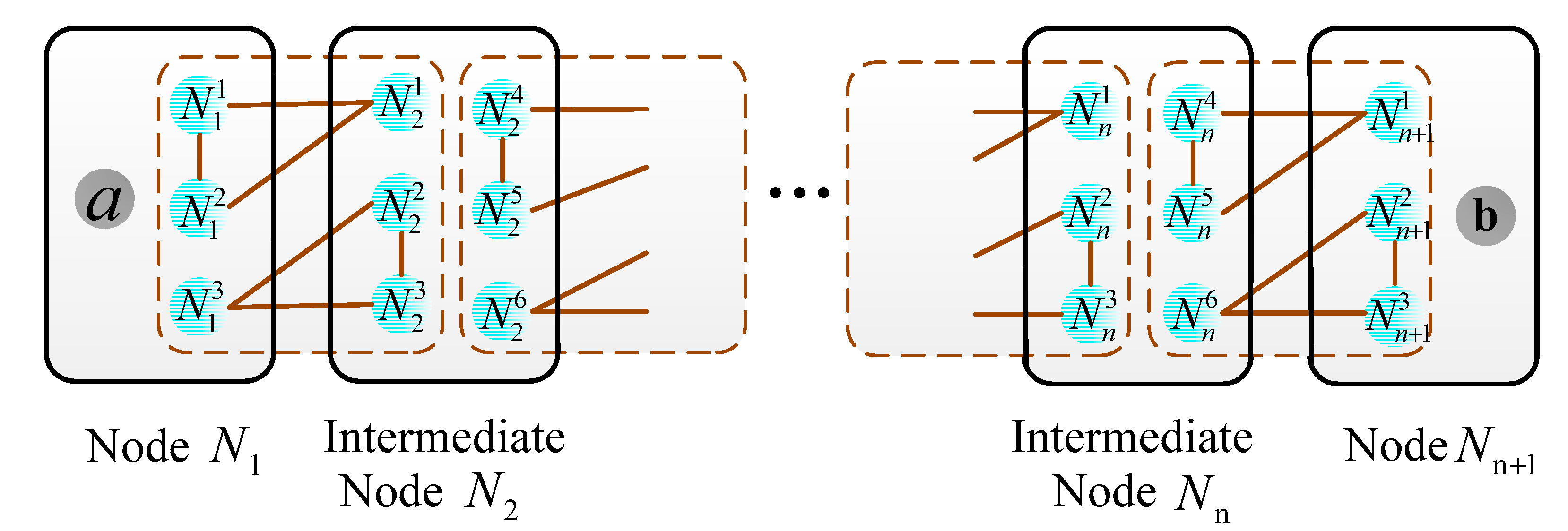
Without loss of generality, Alice and Bob previously share a six-particle GHZ-GHZ state, given by
where the particles
,
and
belong to Alice and particles
,
and
belong to Bob, as shown in
Figure 1.
The state of the initial eight-particle system
is given by
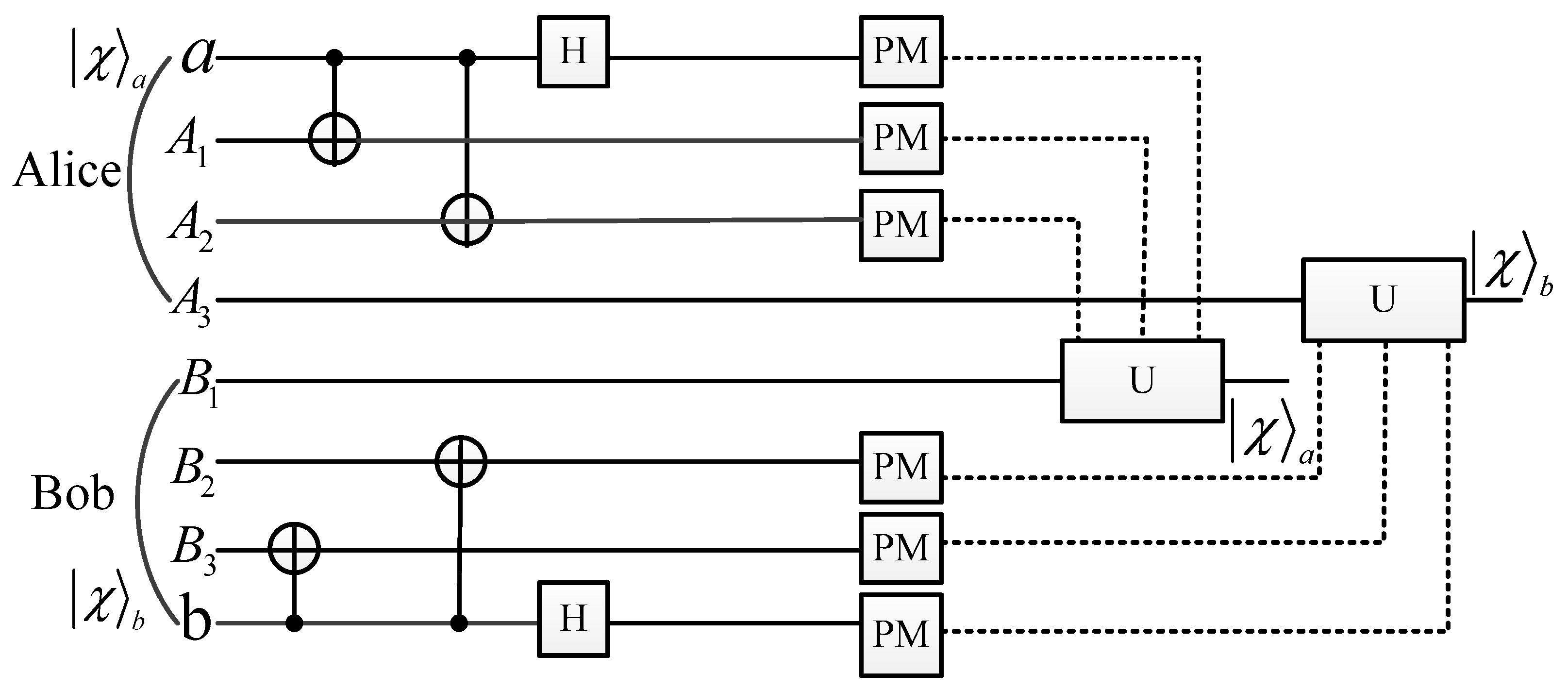
As shown in
Figure 2, to achieve bidirectional qubit transmission, Alice and Bob perform Control-NOT (CNOT) gates and Hadamard gates in parallel, and the state of the entire system becomes
After that, Alice and Bob perform quantum measurements (denoted as PM in
Figure 2) on the particles
,
,
and
,
,
under the basis
, and then inform each other of their measurement results through classical channel. According to the received measurement results, Alice and Bob perform appropriate unitary operations on the particle
and particle
to recover the original qubit. For example, when Alice’s measurement result is
and Bob’s measurement result is
, from Equation (6) we can see that the quantum state of the particles
and
would be
. Alice can retrieve the original qubit by performing the identity operation I on particle
and Bob can retrieve the original qubit by performing the Pauli-X operation on particle
, respectively.
Table 1 lists Alice’s and Bob’s unitary operations corresponding to the received measurement results.
Similarly, when other types of GHZ-GHZ states are previously shared between Alice and Bob, the unitary operations corresponding to Alice’s and Bob’s measurement results are listed in
Table 2. The first row shows the GHZ-GHZ states shared between Alice and Bob, while the first column shows Alice’s and Bob’s measurement results.
3. Two-Hop Directional Quantum Communication
Assume that Alice intends to send an arbitrary qubit
to Bob; at the same time, Bob intends to send
to Alice, where
and
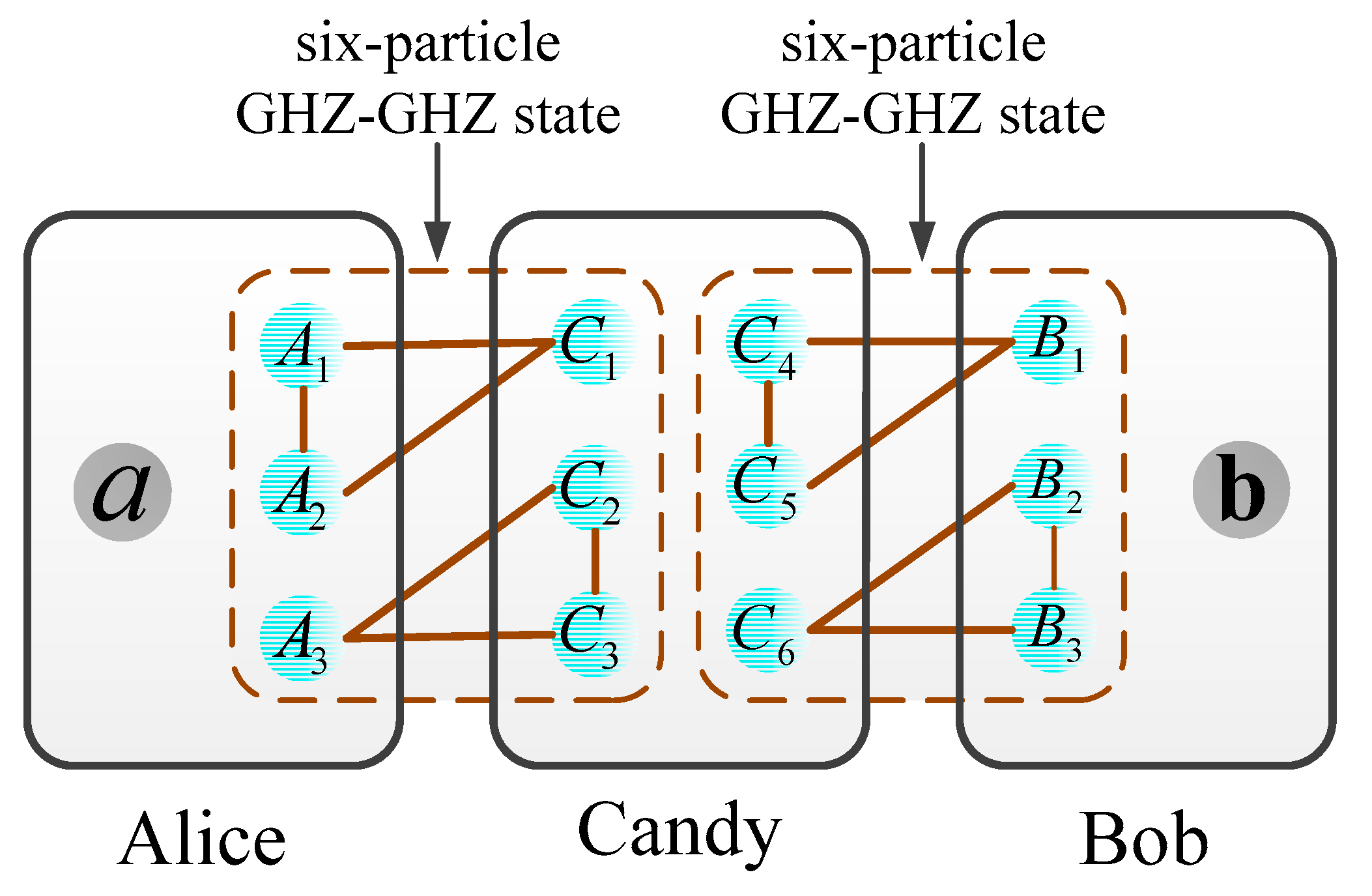
, while there is no GHZ state shared directly between Alice and Bob. Suppose that Candy previously shared a six-particle GHZ-GHZ state with Alice and Bob, respectively, as shown in
Figure 3.
Without loss of generality, assume that
was previously shared between Alice and Candy, while
was previously shared between Candy and Bob. The state of the initial fourteen-particle system
is given by
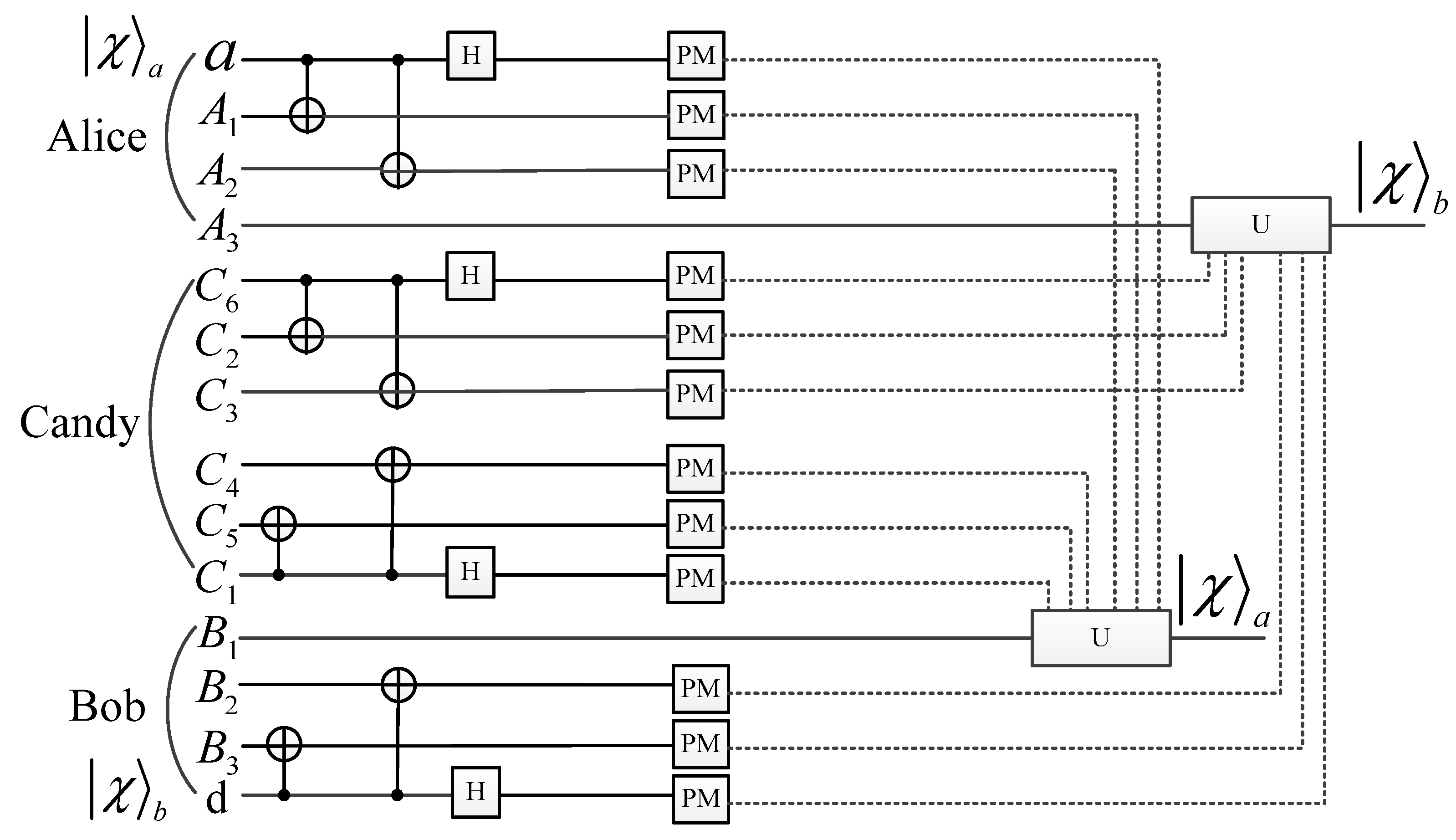
As shown in
Figure 4, to achieve bidirectional two-hop qubit transmission, Alice, Bob, and Candy perform CNOT, Hadamard operations, and quantum measurements in parallel. After that, the measurement results of Alice and Candy and the type of the composite GHZ-GHZ channel between Alice and Candy are transmitted to Bob. Similarly, the measurement results of Bob and Candy and the type of the composite GHZ-GHZ channel between Bob and Candy are transmitted to Alice. Based on the received information, Alice and Bob perform appropriate unitary operations on the particles
and
to recover the original qubit.
For example, if Bob receives measurement results and , and Alice receives measurement results and , the quantum state of the particles and would be . Alice can retrieve the original qubit by performing the Pauli-XZ operation on particle , and Bob can retrieve the original qubit by performing the Pauli-Z operation on particle .
If the above two-hop qubit transmission is realized hop-by-hop, after Alice performs CNOT, Hadamard operations, and the projective measurement, the quantum state of Candy’s particle would be
when she was informed of Alice’s measurement result of
, according to
Table 2. After Candy performs CNOT, Hadamard operations, and the projective measurement, the quantum state of Bob’s particle would be
when he was informed of Candy’s measurement result of
. Similarly, in the other direction, when Bob’s measurement result is
, the quantum state of Candy’s particle would be
. If Candy’s measurement result is
, the quantum state of Alice’s particle would be
, which is compatible with Equation (8).
5. Discussions and Conclusions
5.1. Discussions
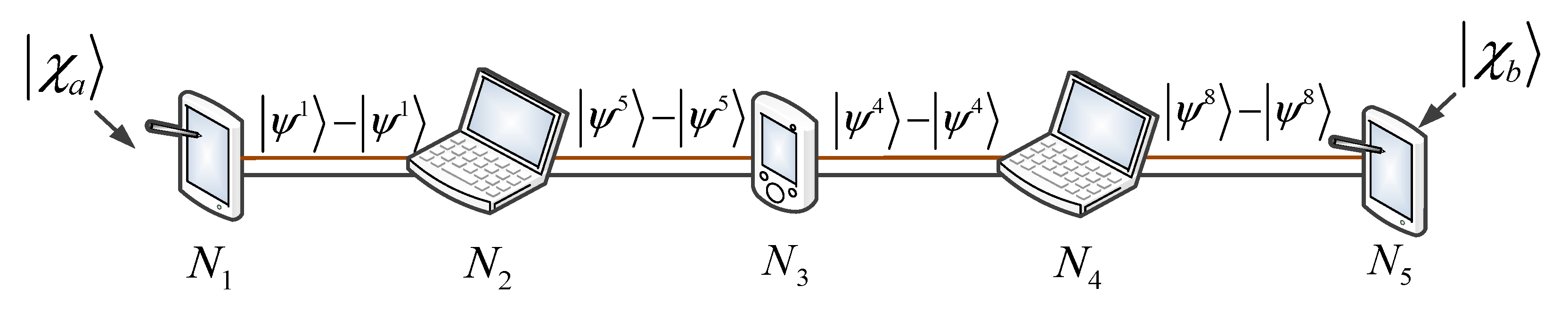
In the process of multi-hop quantum communication, the quantum communication delay is introduced mostly by quantum measurements, unitary operations, and transmission of classical information. Due to the limited decoherence time in the quantum memory and the demand for QOS (Quality of Service), a short delay is expected in quantum communication. Assume that each quantum measurement takes milliseconds, unitary operation takes milliseconds, and one-hop transmission of classic information through classical channel takes milliseconds.
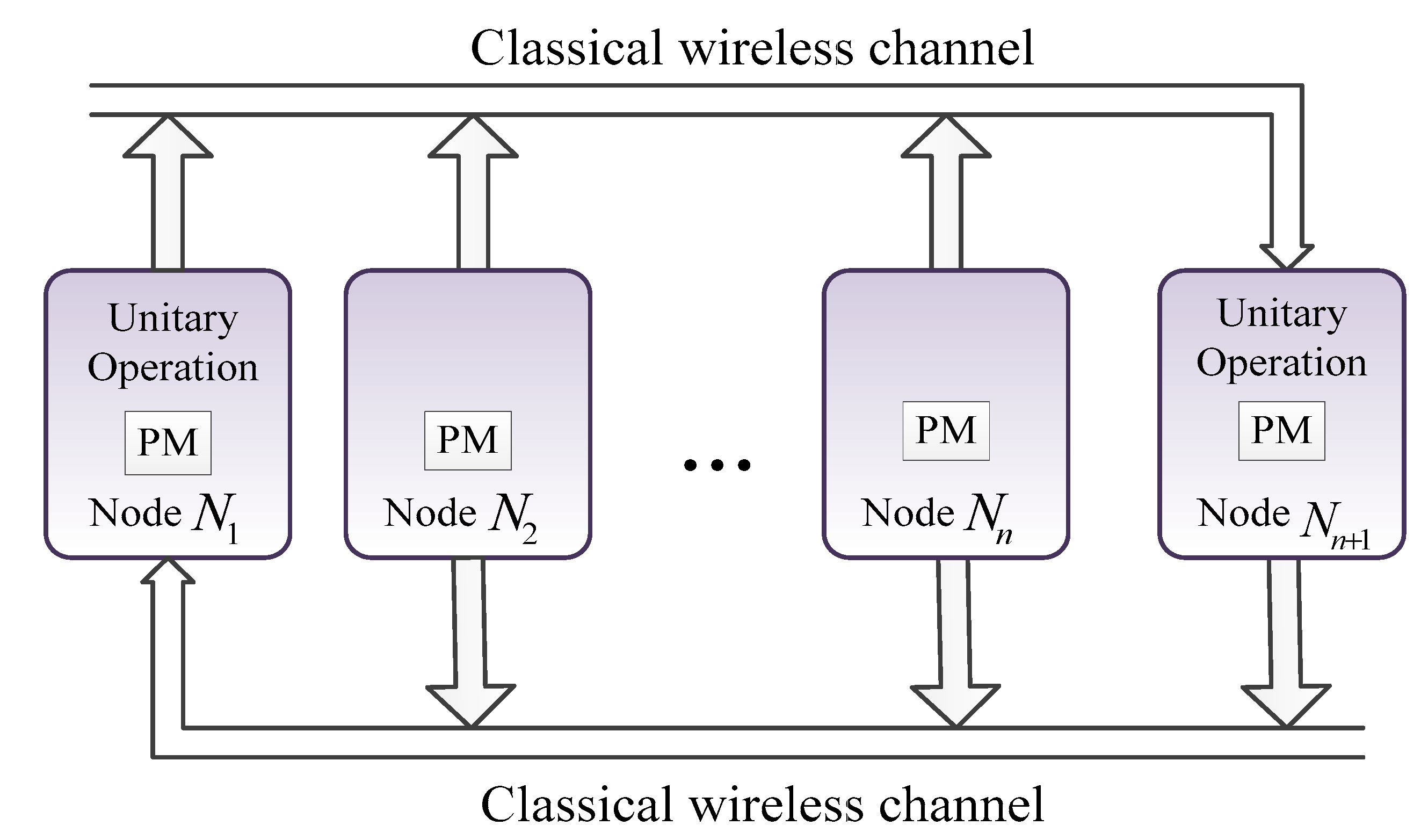
In our scheme, as shown in
Figure 7, quantum measurements (CNOT, Hadamard operations, and projective measurements are included) are performed simultaneously, and classical information is sent to the destination node independently. Taking
to denote the hop count of the classical information transmitted from the
i-th node to the destination node, therefore the total end-to-end quantum communication delay in the
n-hop case is given by
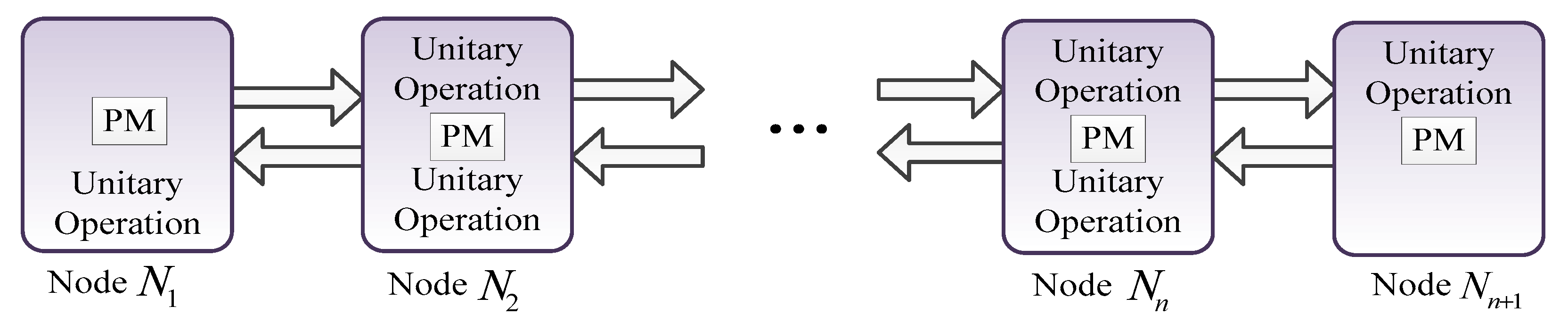
For hop-by-hop qubit transmission [
7], as shown in
Figure 8, the total end-to-end quantum communication delay is given by
Given that
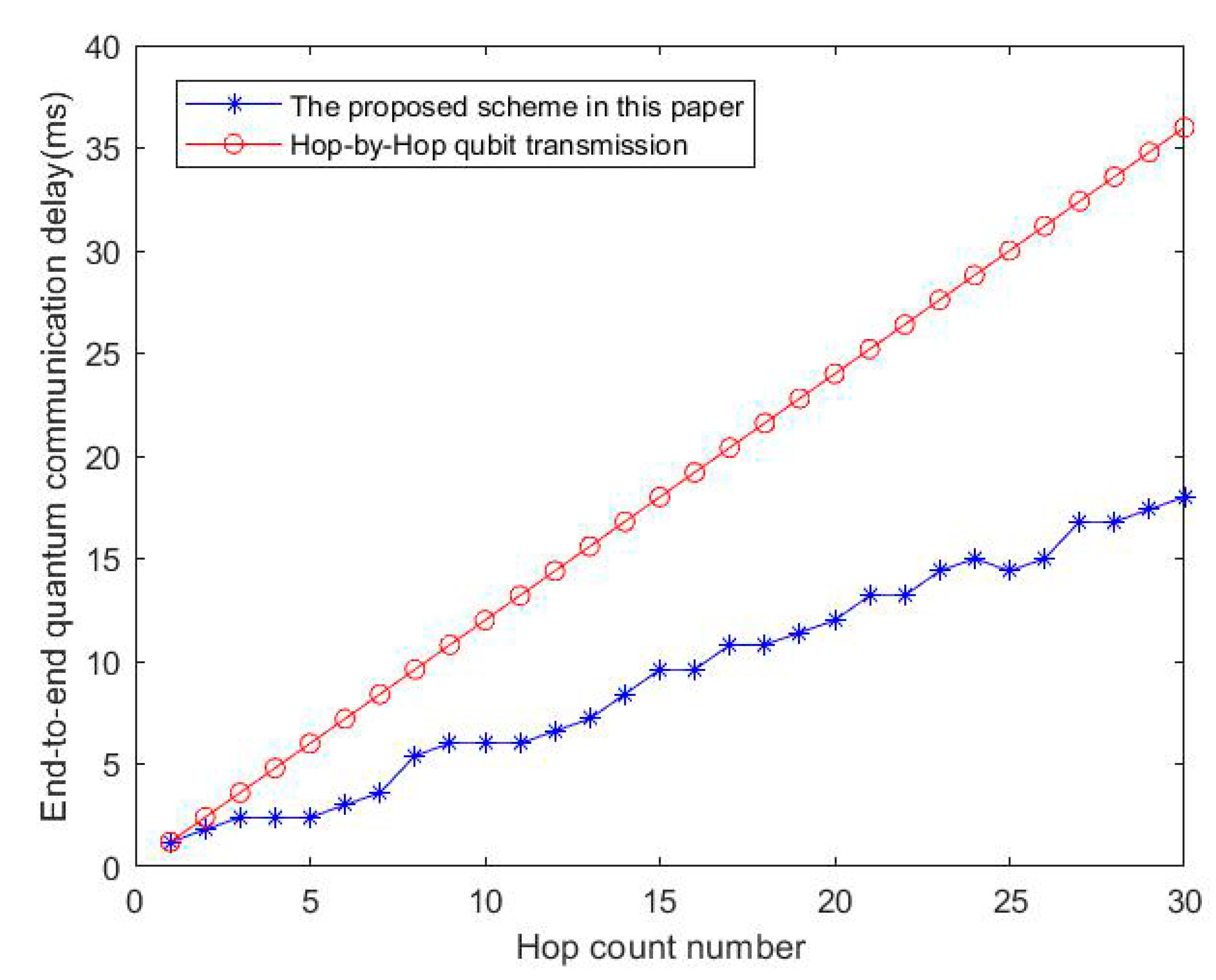
, the end-to-end quantum communication delay in our scheme and hop-by-hop qubit transmission scheme is demonstrated by
Figure 9.
From
Figure 9, it is obvious that our scheme has a shorter end-to-end quantum communication delay. In a wide-area quantum communication network where the hop count number
n is large, our scheme shows obvious advantage in the end-to-end quantum communication delay.
In addition to the shorter quantum communication delay, our scheme shows other notable advantages, such as: (i) Duplex quantum communication is available for two arbitrary nodes compared with other quantum network schemes [
6,
7,
8,
11,
12]. (ii) The composite GHZ-GHZ states are used as the quantum channel, which is less prone to the decoherence effects caused by the surrounding environment [
22]. (iii) Only quantum projective measurements and unitary operations are needed to implement qubit transmission. Auxiliary qubits are not required, in contrast to the bidirectional quantum communication scheme based on GHZ-Bell states [
20]. (iv) In our scheme, for an intermediate node, it is not necessary to recover the original qubit locally and then teleport it to its next-hop node. Therefore, information leakage can be avoided compared with the hop-by-hop qubit transmission scheme [
7].
5.2. Conclusions
In summary, we propose a bidirectional quantum communication protocol using the composite GHZ-GHZ state as quantum channel. Two arbitrary nodes in a quantum communication network can transmit qubits to each other based on quantum measurements performed in parallel by all the nodes involved. The original qubits can be retrieved locally at the destination site based on the measurement results and the types of GHZ channel. Information leakage is avoided in our scheme, and it has a shorter delay for the end-to-end quantum communication, in contrast to the hop-by-hop qubit transmission scheme.
There are many avenues for future work and extensions. One aspect is the imperfection of quantum channel, which is crucial for the efficiency and fidelity of qubit transmission. Another important aspect is the extension to multi-user quantum communication, which is a realistic scenario for future quantum networks.