Photon Subtraction-Induced Plug-and-Play Scheme for Enhancing Continuous-Variable Quantum Key Distribution with Discrete Modulation
Abstract
1. Introduction
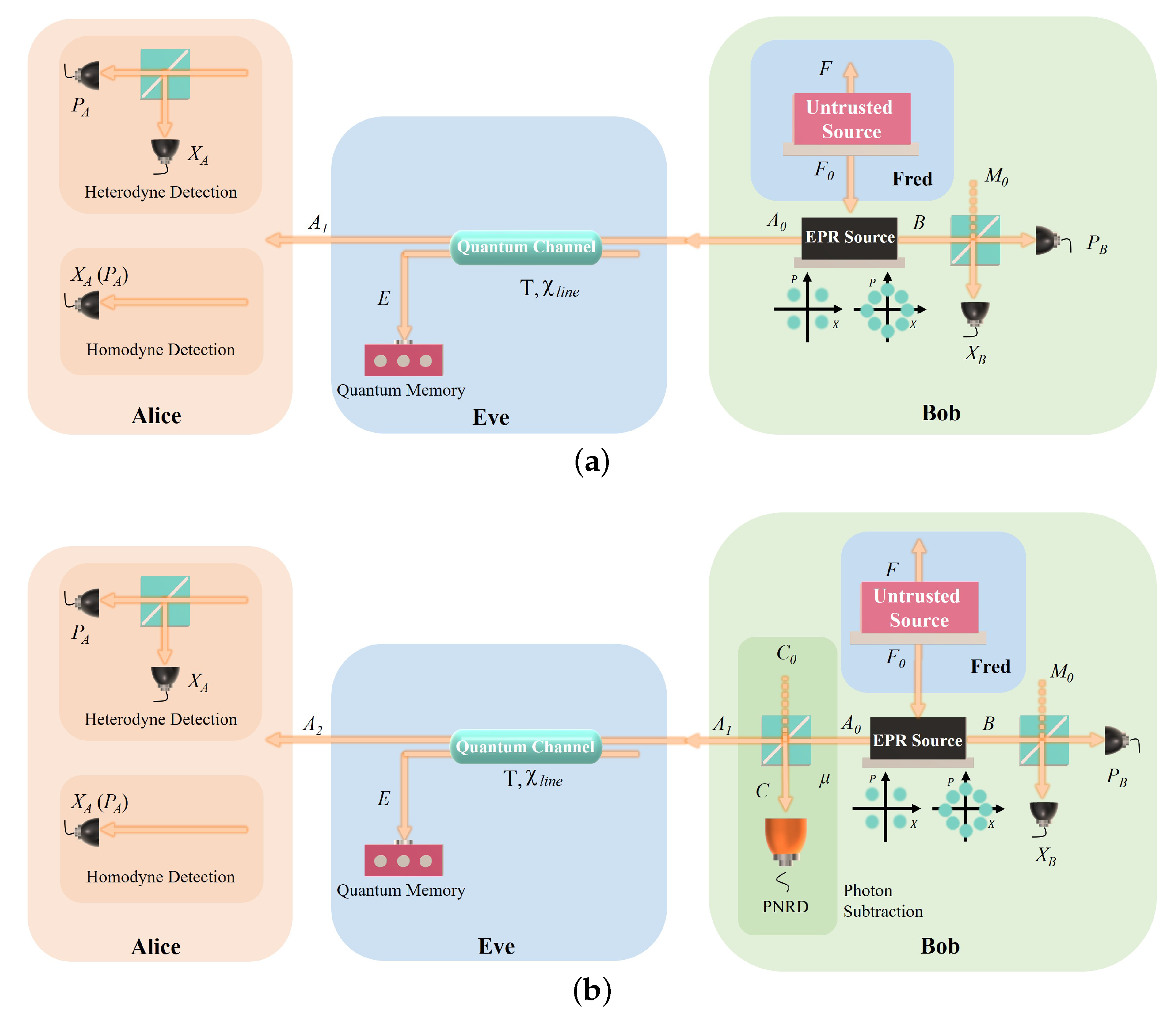
2. Photon-Subtracted Plug-and-Play DM-CVQKD
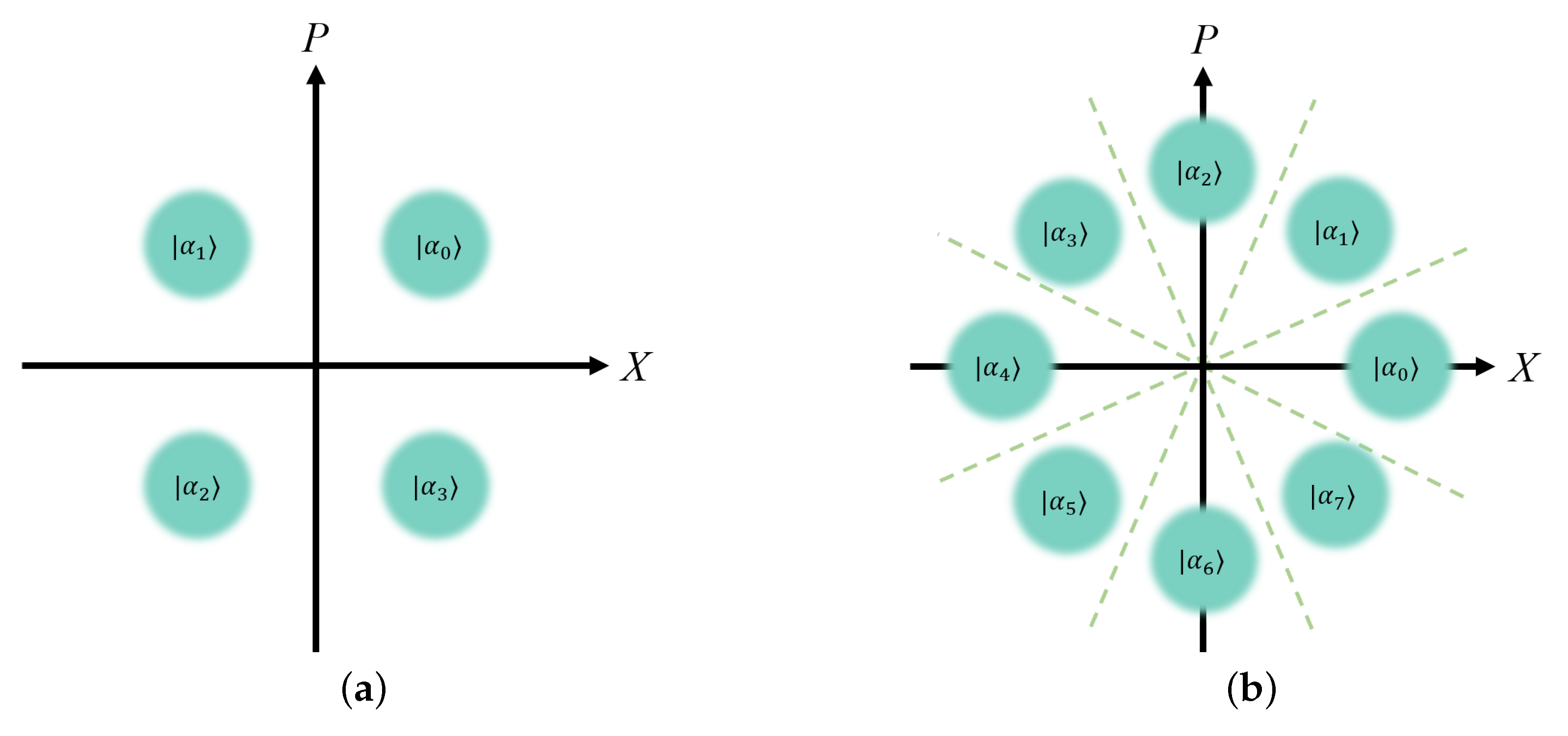
2.1. Plug-and-Play DM-CVQKD Protocol
- Fred prepares a EPR state . Fred can be a neutral party; however, to derive the tight security bound, Fred is assumed to be controlled by Eve, that is to say, Fred would introduce the untrusted source noise. Notice that the state is utilized to simulate the coherent state with extra untrusted noise , which characterized by a phase insensitive amplifier (PIA) with a gain g in the PM scheme. Namely, the state is virtually a two-mode state [43] and the calculation method of two-mode entanglement state is still suitable. The quadratures and of Bob and Alice respectively can be expressed aswhere ( with the shot noise variance 1) and . Here, is the variance of vacuum noise.
- Alice sends the mode to Bob through an untrusted channel. We assume that Eve performs the collective Gaussian attack strategy, which is proved to be an optimal attack on reverse reconciliation protocols. The transmittance of the channel represented by T and the channel-added noise is .
- Alice randomly measures one quadrature of the mode using homodyne detection or two quadratures with heterodyne detection, while Bob measures mode B with heterodyne detection. After that, Alice and Bob share two strings of correlated data as raw keys.
- Alice and Bob apply post-processing procedure, e.g., reverse reconciliation and privacy amplification to acquire secure secret key sequences.
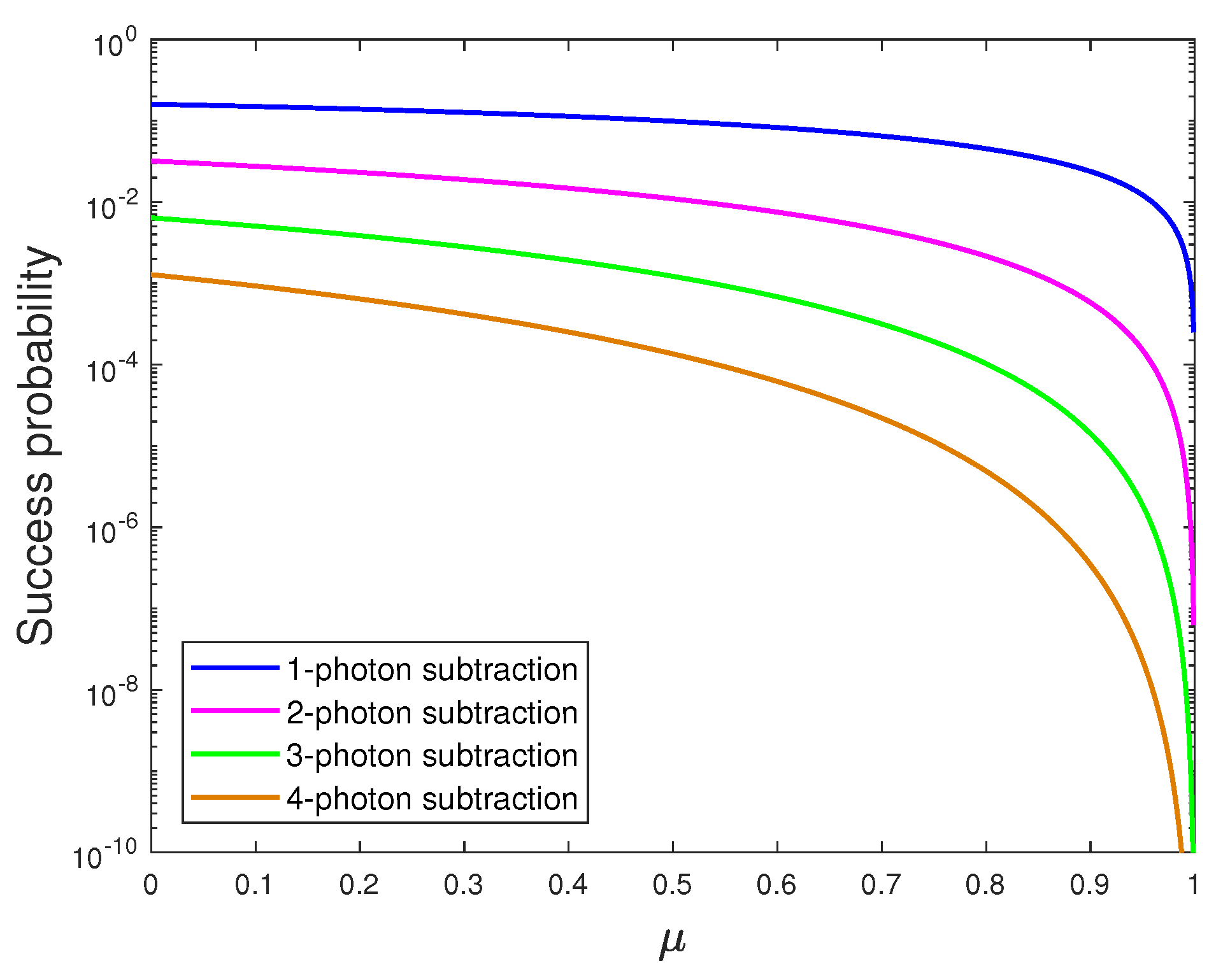
2.2. Photon-Subtracted Plug-and-Play DM-CVQKD
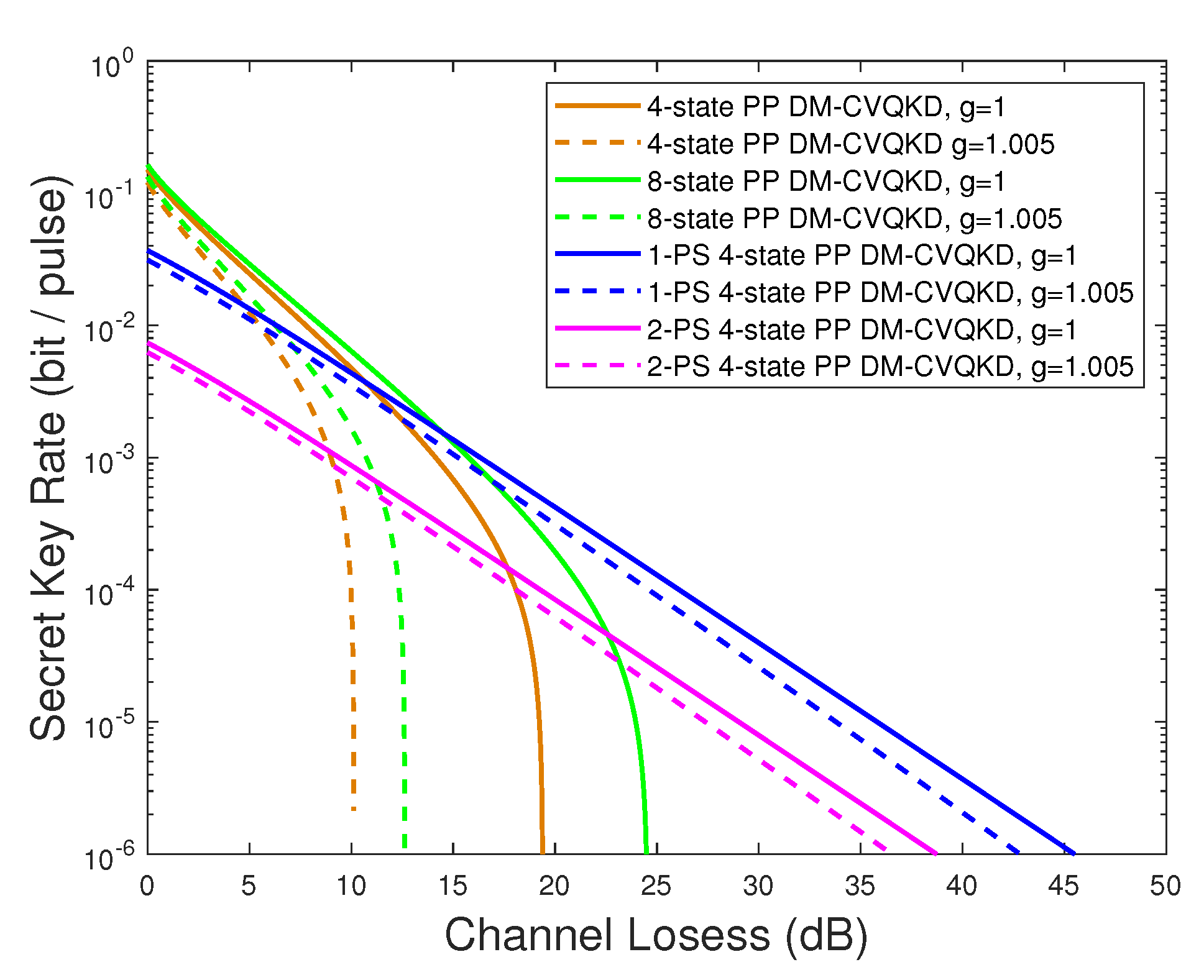
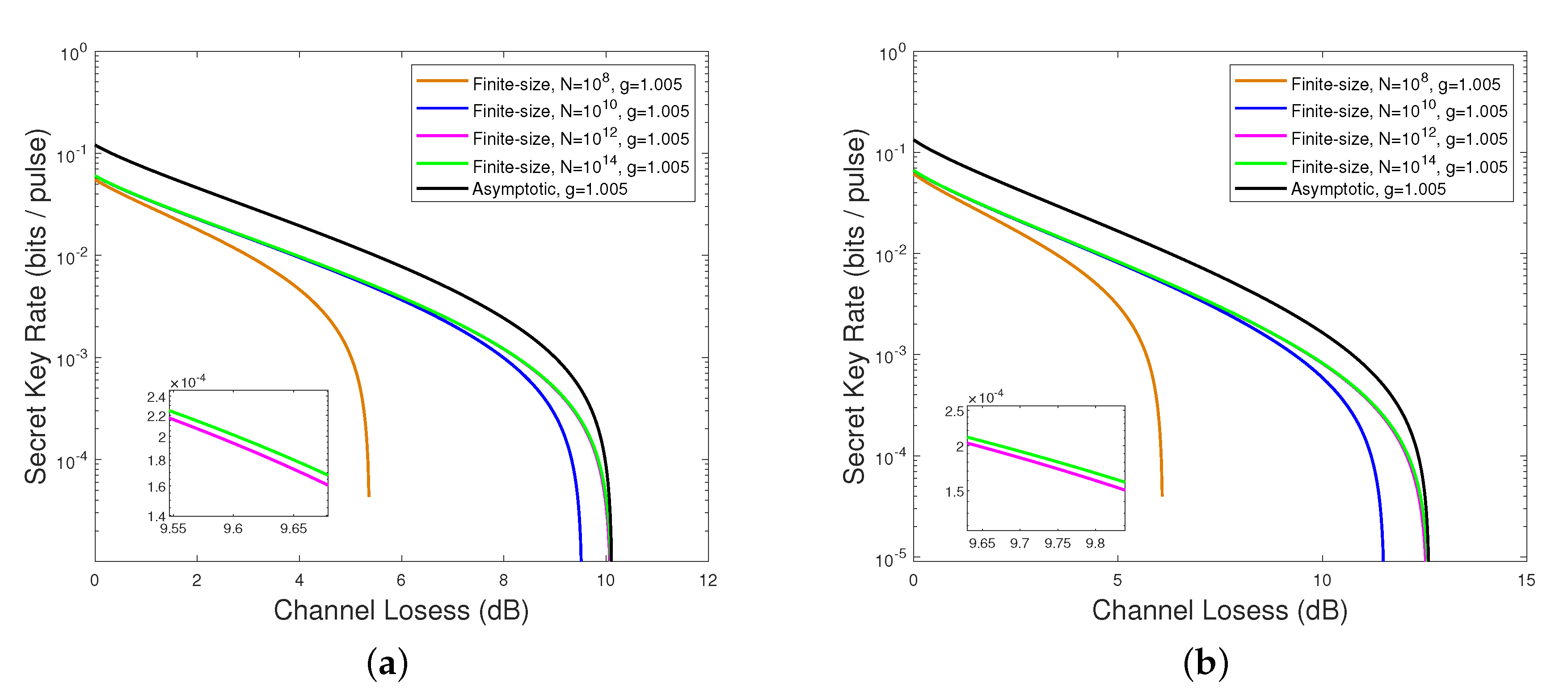
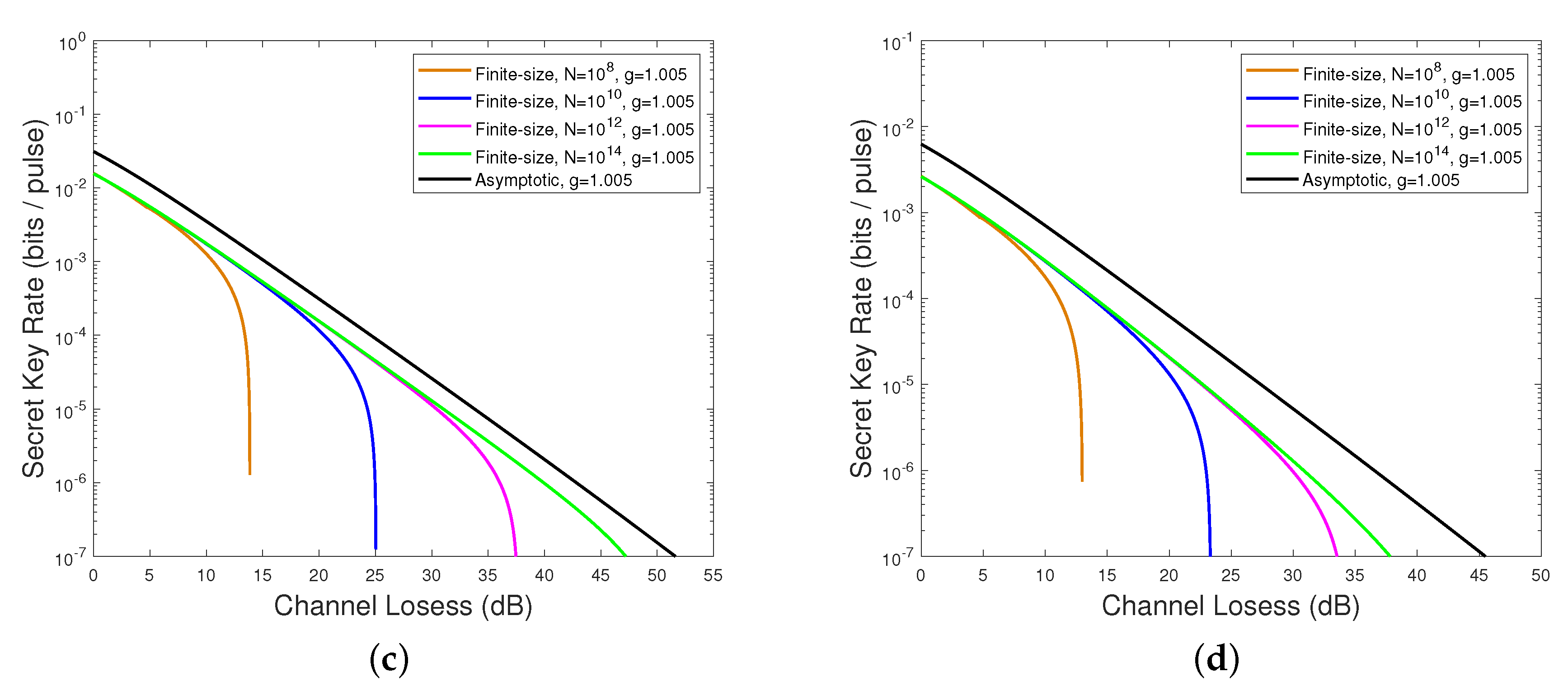
3. Security Analysis
4. Conclusions
Author Contributions
Funding
Conflicts of Interest
Abbreviations
| QKD | Quantum Key Distribution |
| CVQKD | Continuous-Variable Quantum Key Distribution |
| DVQKD | Discrete-Variable Quantum Key Distribution |
| EPR | Einstein–Podolsky–Rosen |
| PP | Plug-and-Play |
| PS | Photon Subtraction |
| EB | Entanglement-Based |
| PM | Prepare-and-Measurement |
| TMSV | Two-Mode Squeezed Vacuum |
Appendix A. Derivation of the Asymptotic Secret Key Rate
Appendix B. Derivation of Finite-Size Secret Key Rate
References
- Grosshans, F.; Grangier, P. Continuous variable quantum cryptography using coherent states. Phys. Rev. Lett. 2002, 88, 057902. [Google Scholar] [CrossRef] [PubMed]
- Grosshans, F.; Van Assche, G.; Wenger, J.; Brouri, R.; Cerf, N.J.; Grangier, P. Quantum key distribution using gaussian-modulated coherent states. Nature 2003, 421, 238–241. [Google Scholar] [CrossRef] [PubMed]
- Weedbrook, C.; Lance, A.M.; Bowen, W.P.; Symul, T.; Ralph, T.C.; Lam, P.K. Quantum cryptography without switching. Phys. Rev. Lett. 2004, 93, 170504. [Google Scholar] [CrossRef] [PubMed]
- Cerf, N.J.; Levy, M.; Van Assche, G. Quantum distribution of Gaussian keys using squeezed states. Phys. Rev. A 2001, 63, 052311. [Google Scholar] [CrossRef]
- Zhou, J.; Huang, D.; Guo, Y. Long-distance continuous-variable quantum key distribution using separable Gaussian states. Phys. Rev. A 2018, 98, 042303. [Google Scholar] [CrossRef]
- Lo, H.K.; Curty, M.; Tamaki, K. Secure quantum key distribution. Nat. Photonics 2014, 8, 595. [Google Scholar] [CrossRef]
- Vazirani, U.; Vidick, T. Fully device independent quantum key distribution. Commun. ACM 2019, 62, 133. [Google Scholar] [CrossRef]
- Li, X.H.; Deng, F.G.; Zhou, H.Y. Efficient quantum key distribution over a collective noise channel. Phys. Rev. A 2008, 78, 022321. [Google Scholar] [CrossRef]
- Pljonkin, A.; Singh, P.K. The Review of the Commercial Quantum Key Distribution System. In Proceedings of the 2018 Fifth International Conference on Parallel, Distributed and Grid Computing (PDGC), Solan, Himachal Pradesh, India, 20–22 December 2018; IEEE: Piscataway, NJ, USA, 2018; pp. 795–799. [Google Scholar]
- Pljonkin, A. Vulnerability of the Synchronization Process in the Quantum Key Distribution System. Int. J. Cloud Appl. Comput. (IJCAC) 2019, 9, 50–58. [Google Scholar] [CrossRef]
- Zhao, W.; Liao, Q.; Huang, D.; Guo, Y. Performance analysis of the satellite-to-ground continuous-variable quantum key distribution with orthogonal frequency division multiplexed modulation. Quantum Inf. Process. 2019, 18, 39. [Google Scholar] [CrossRef]
- Liao, Q.; Guo, Y.; Xie, C.; Huang, D.; Huang, P.; Zeng, G. Composable security of unidimensional continuous-variable quantum key distribution. Quantum Inf. Process. 2018, 17, 113. [Google Scholar] [CrossRef]
- Huang, P.; He, G.; Fang, J.; Zeng, G. Performance improvement of continuous-variable quantum key distribution via photon subtraction. Phys. Rev. A 2013, 87, 012317. [Google Scholar] [CrossRef]
- Wang, X.; Cao, Y.; Wang, P.; Li, Y. Advantages of the coherent state compared with squeezed state in unidimensional continuous variable quantum key distribution. Quantum Inf. Process. 2018, 17, 344. [Google Scholar] [CrossRef]
- Gong, L.H.; Song, H.C.; He, C.S.; Liu, Y.; Zhou, N.R. A continuous variable quantum deterministic key distribution based on two-mode squeezed states. Phys. Scr. 2014, 89, 035101. [Google Scholar] [CrossRef]
- Jouguet, P.; Kunz-Jacques, S.; Diamanti, E. Preventing calibration attacks on the local oscillator in continuous-variable quantum key distribution. Phys. Rev. A 2013, 87, 062313. [Google Scholar] [CrossRef]
- Liang, K.; Cao, Z.; Liu, W.; Wang, J.; Chai, G.; Zhang, T. Security analysis of continuous-variable quantum key distribution with imperfect Faraday mirror. Laser Phys. Lett. 2019, 16, 115202. [Google Scholar] [CrossRef]
- Ye, W.; Zhong, H.; Liao, Q.; Huang, D.; Hu, L.; Guo, Y. Improvement of self-referenced continuous-variable quantum key distribution with quantum photon catalysis. Opt. Express 2019, 27, 17186–17198. [Google Scholar] [CrossRef]
- Li, M.; Cvijetic, M. Continuous-variable quantum key distribution with self-reference detection and discrete modulation. IEEE J. Quantum Electron. 2018, 54, 1–8. [Google Scholar] [CrossRef]
- Gyongyosi, L.; Imre, S. Adaptive multicarrier quadrature division modulation for long-distance continuous-variable quantum key distribution. In Quantum Information and Computation XII; International Society for Optics and Photonics: Bellingham, WA, USA, 2014; Volume 9123, p. 912307. [Google Scholar]
- Zhao, W.; Guo, Y.; Zhang, L.; Huang, D. Phase noise estimation using Bayesian inference for continuous-variable quantum key distribution. Opt. Express 2019, 27, 1838–1853. [Google Scholar] [CrossRef]
- Li, Y.M.; Wang, X.Y.; Bai, Z.L.; Liu, W.Y.; Yang, S.S.; Peng, K.C. Continuous variable quantum key distribution. Chin. Phys. B 2017, 26, 040303. [Google Scholar] [CrossRef]
- Jouguet, P.; Kunz-Jacques, S.; Leverrier, A. Long-distance continuous-variable quantum key distribution with a Gaussian modulation. Phys. Rev. A 2011, 84, 062317. [Google Scholar] [CrossRef]
- Leverrier, A.; Grangier, P. Unconditional security proof of long-distance continuous-variable quantum key distribution with discrete modulation. Phys. Rev. Lett. 2009, 102, 180504. [Google Scholar] [CrossRef]
- Leverrier, A.; Grangier, P. Continuous-variable quantum key distribution protocols with a discrete modulation. arXiv 2010, arXiv:1002.4083. [Google Scholar]
- Ghorai, S.; Grangier, P.; Diamanti, E.; Leverrier, A. Asymptotic security of continuous-variable quantum key distribution with a discrete modulation. Phys. Rev. X 2019, 9, 021059. [Google Scholar] [CrossRef]
- Becir, A.; El-Orany, F.; Wahiddin, M. Continuous-variable quantum key distribution protocols with eight-state discrete modulation. Int. J. Quantum Inf. 2012, 10, 1250004. [Google Scholar] [CrossRef]
- Wang, X.-Y.; Bai, Z.-L.; Wang, S.-F.; Li, Y.-M.; Peng, K.C. Four-state modulation continuous variable quantum key distribution over a 30-km fiber and analysis of excess noise. Chin. Phys. Lett. 2013, 30, 010305. [Google Scholar] [CrossRef]
- Huang, P.; Fang, J.; Zeng, G. State-discrimination attack on discretely modulated continuous-variable quantum key distribution. Phys. Rev. A 2014, 89, 042330. [Google Scholar] [CrossRef]
- Wang, T.; Huang, P.; Zhou, Y.; Liu, W.; Zeng, G. Pilot-multiplexed continuous-variable quantum key distribution with a real local oscillator. Phys. Rev. A 2018, 97, 012310. [Google Scholar] [CrossRef]
- Huang, B.; Huang, Y.; Peng, Z. Practical security of the continuous-variable quantum key distribution with real local oscillators under phase attack. Opt. Express 2019, 27, 20621–20631. [Google Scholar] [CrossRef] [PubMed]
- Ma, X.C.; Sun, S.H.; Jiang, M.S.; Liang, L.M. Local oscillator fluctuation opens a loophole for Eve in practical continuous-variable quantum-key-distribution systems. Phys. Rev. A 2013, 88, 022339. [Google Scholar] [CrossRef]
- Huang, P.; Huang, J.; Wang, T.; Li, H.; Huang, D.; Zeng, G. Robust continuous-variable quantum key distribution against practical attacks. Phys. Rev. A 2017, 95, 052302. [Google Scholar] [CrossRef]
- Wu, X.D.; Wang, Y.J.; Huang, D.; Guo, Y. Simultaneous measurement-device-independent continuous variable quantum key distribution with realistic detector compensation. Front. Phys. 2020, 15, 1–12. [Google Scholar] [CrossRef]
- Huang, D.; Huang, P.; Lin, D.; Wang, C.; Zeng, G. High-speed continuous-variable quantum key distribution without sending a local oscillator. Opt. Lett. 2015, 40, 3695–3698. [Google Scholar] [CrossRef] [PubMed]
- Soh, D.B.; Brif, C.; Coles, P.J.; Lütkenhaus, N.; Camacho, R.M.; Urayama, J.; Sarovar, M. Self-referenced continuous-variable quantum key distribution protocol. Phys. Rev. X 2015, 5, 041010. [Google Scholar] [CrossRef]
- Kleis, S.; Rueckmann, M.; Schaeffer, C.G. Continuous variable quantum key distribution with a real local oscillator using simultaneous pilot signals. Opt. Lett. 2017, 42, 1588–1591. [Google Scholar] [CrossRef]
- Wang, T.; Huang, P.; Zhou, Y.; Liu, W.; Ma, H.; Wang, S.; Zeng, G. High key rate continuous-variable quantum key distribution with a real local oscillator. Opt. Express 2018, 26, 2794–2806. [Google Scholar] [CrossRef]
- Huang, D.; Huang, P.; Wang, T.; Li, H.; Zhou, Y.; Zeng, G. Continuous-variable quantum key distribution based on a plug-and-play dual-phase-modulated coherent-states protocol. Phys. Rev. A 2016, 94, 032305. [Google Scholar] [CrossRef]
- Valivarthi, R.; Etcheverry, S.; Aldama, J.; Zwiehoff, F.; Pruneri, V. Plug-and-play continuous-variable quantum key distribution for metropolitan networks. Opt. Express 2020, 28, 14547–14559. [Google Scholar] [CrossRef]
- Liao, Q.; Wang, Y.; Huang, D.; Guo, Y. Dual-phase-modulated plug-and-play measurement-device-independent continuous-variable quantum key distribution. Opt. Express 2018, 26, 19907–19920. [Google Scholar] [CrossRef]
- Zhang, H.; Ruan, X.; Wu, X.; Zhang, L.; Guo, Y.; Huang, D. Plug-and-play unidimensional continuous-variable quantum key distribution. Quantum Inf. Process. 2019, 18, 128. [Google Scholar] [CrossRef]
- Wu, X.D.; Wang, Y.J.; Zhong, H.; Liao, Q.; Guo, Y. Plug-and-play dual-phase-modulated continuous-variable quantum key distribution with photon subtraction. Front. Phys. 2019, 14, 41501. [Google Scholar] [CrossRef]
- Jain, N.; Anisimova, E.; Khan, I.; Makarov, V.; Marquardt, C.; Leuchs, G. Trojan-horse attacks threaten the security of practical quantum cryptography. New J. Phys. 2014, 16, 123030. [Google Scholar] [CrossRef]
- Gisin, N.; Fasel, S.; Kraus, B.; Zbinden, H.; Ribordy, G. Trojan-horse attacks on quantum-key-distribution systems. Phys. Rev. A 2006, 73, 022320. [Google Scholar] [CrossRef]
- Subacius, D.; Zavriyev, A.; Trifonov, A. Backscattering limitation for fiber-optic quantum key distribution systems. Appl. Phys. Lett. 2005, 86, 011103. [Google Scholar] [CrossRef]
- Guo, Y.; Ye, W.; Zhong, H.; Liao, Q. Continuous-variable quantum key distribution with non-Gaussian quantum catalysis. Phys. Rev. A 2019, 99, 032327. [Google Scholar] [CrossRef]
- Guo, Y.; Liao, Q.; Wang, Y.; Huang, D.; Huang, P.; Zeng, G. Performance improvement of continuous-variable quantum key distribution with an entangled source in the middle via photon subtraction. Phys. Rev. A 2017, 95, 032304. [Google Scholar] [CrossRef]
- Ma, H.X.; Huang, P.; Bai, D.Y.; Wang, S.Y.; Bao, W.S.; Zeng, G.H. Continuous-variable measurement-device-independent quantum key distribution with photon subtraction. Phys. Rev. A 2018, 97, 042329. [Google Scholar] [CrossRef]
- Liao, Q.; Guo, Y.; Huang, D.; Huang, P.; Zeng, G. Long-distance continuous-variable quantum key distribution using non-Gaussian state-discrimination detection. New J. Phys. 2018, 20, 023015. [Google Scholar] [CrossRef]
- Jouguet, P.; Kunz-Jacques, S.; Leverrier, A.; Grangier, P.; Diamanti, E. Experimental demonstration of long-distance continuous-variable quantum key distribution. Nat. Photonics 2013, 7, 378–381. [Google Scholar] [CrossRef]
- Guo, Y.; Ding, J.; Mao, Y.; Ye, W.; Liao, Q.; Huang, D. Quantum catalysis-based discrete modulation continuous variable quantum key distribution with eight states. Phys. Lett. A 2020, 384, 126340. [Google Scholar] [CrossRef]
- Wu, X.D.; Chen, F.; Wu, X.H.; Guo, Y. Controlling continuous-variable quantum key distribution with entanglement in the middle using tunable linear optics cloning machines. Int. J. Theor. Phys. 2017, 56, 415–426. [Google Scholar] [CrossRef]
- Li, Z.; Zhang, Y.; Wang, X.; Xu, B.; Peng, X.; Guo, H. Non-Gaussian postselection and virtual photon subtraction in continuous-variable quantum key distribution. Phys. Rev. A 2016, 93, 012310. [Google Scholar] [CrossRef]
- Leverrier, A.; Grosshans, F.; Grangier, P. Finite-size analysis of a continuous-variable quantum key distribution. Phys. Rev. A 2010, 81, 062343. [Google Scholar] [CrossRef]

© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Yu, C.; Zou, S.; Mao, Y.; Guo, Y. Photon Subtraction-Induced Plug-and-Play Scheme for Enhancing Continuous-Variable Quantum Key Distribution with Discrete Modulation. Appl. Sci. 2020, 10, 4175. https://doi.org/10.3390/app10124175
Yu C, Zou S, Mao Y, Guo Y. Photon Subtraction-Induced Plug-and-Play Scheme for Enhancing Continuous-Variable Quantum Key Distribution with Discrete Modulation. Applied Sciences. 2020; 10(12):4175. https://doi.org/10.3390/app10124175
Chicago/Turabian StyleYu, Chao, Shanhua Zou, Yun Mao, and Ying Guo. 2020. "Photon Subtraction-Induced Plug-and-Play Scheme for Enhancing Continuous-Variable Quantum Key Distribution with Discrete Modulation" Applied Sciences 10, no. 12: 4175. https://doi.org/10.3390/app10124175
APA StyleYu, C., Zou, S., Mao, Y., & Guo, Y. (2020). Photon Subtraction-Induced Plug-and-Play Scheme for Enhancing Continuous-Variable Quantum Key Distribution with Discrete Modulation. Applied Sciences, 10(12), 4175. https://doi.org/10.3390/app10124175




