Modeling Palletized Products: The Case of Semi-Filled Bottles under Top-Load Conditions
Abstract
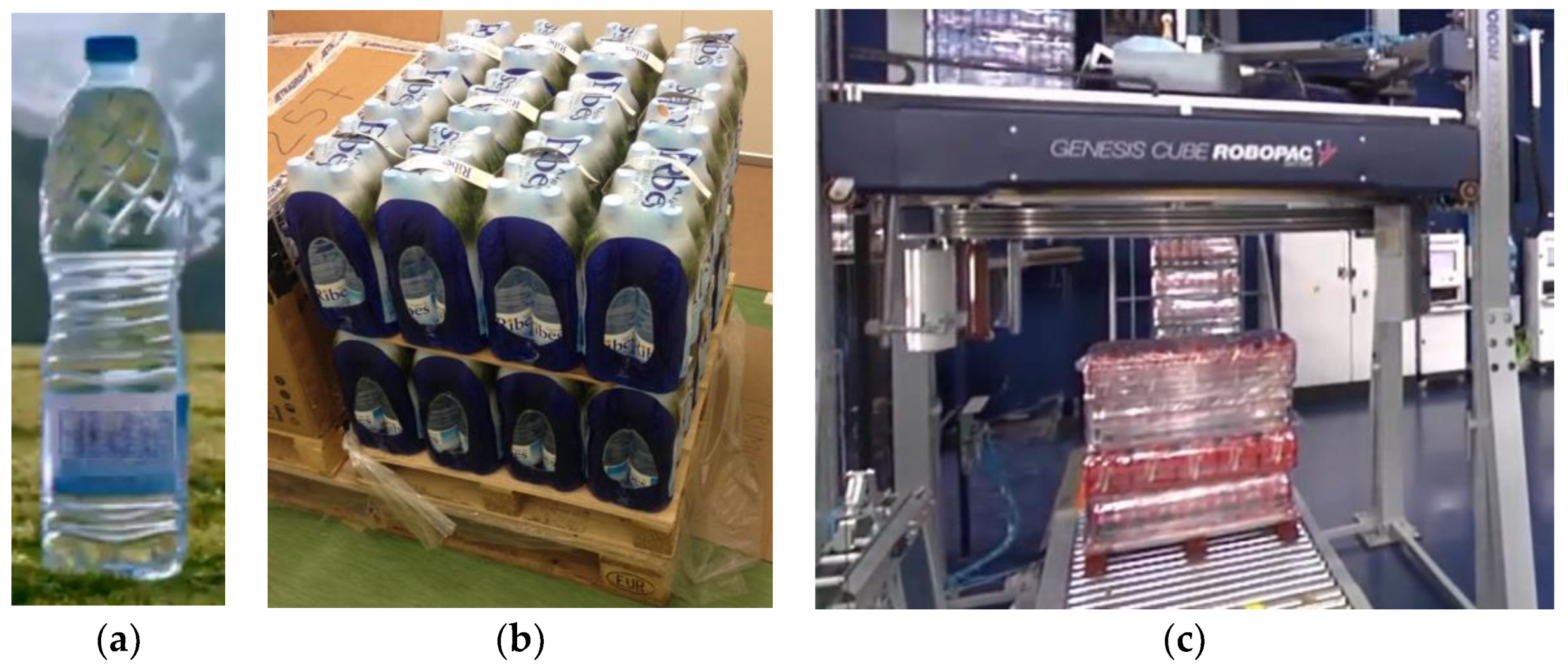
1. Introduction
- Made of Polyethylene terephthalate (PET), a material with a restricted elastic zone: by increasing the load the plastic regime is easily reached;
- Designed in complex shapes, including reinforcing and functional elements;
- Manufactured by highly efficient and standardized processes;
- Designed with minimum thicknesses to reduce weight and cost.
2. Materials and Methods
2.1. Aim and Scope
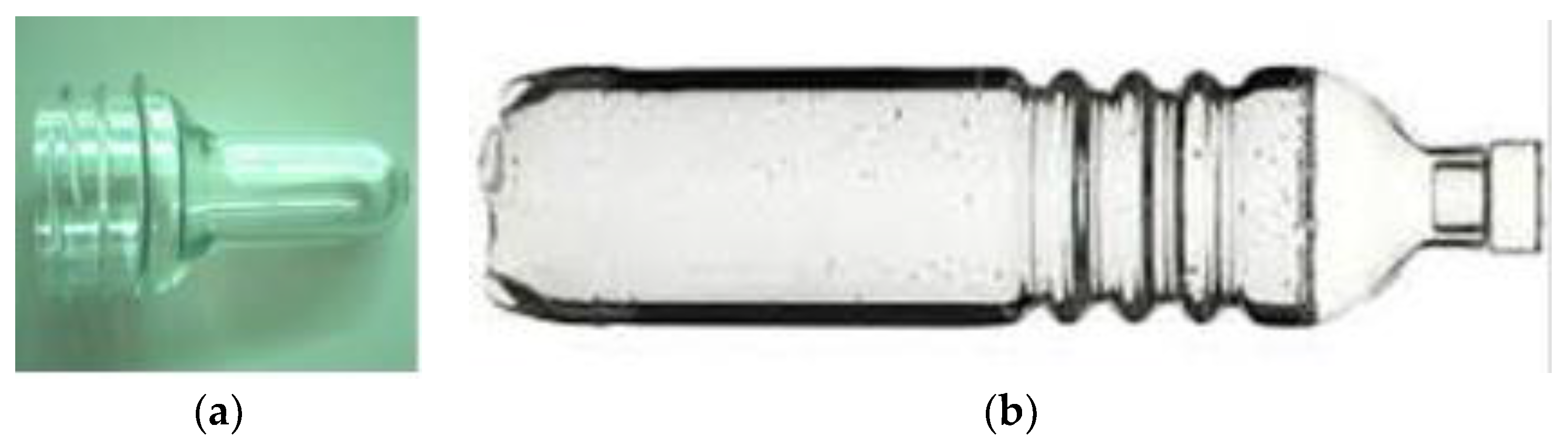
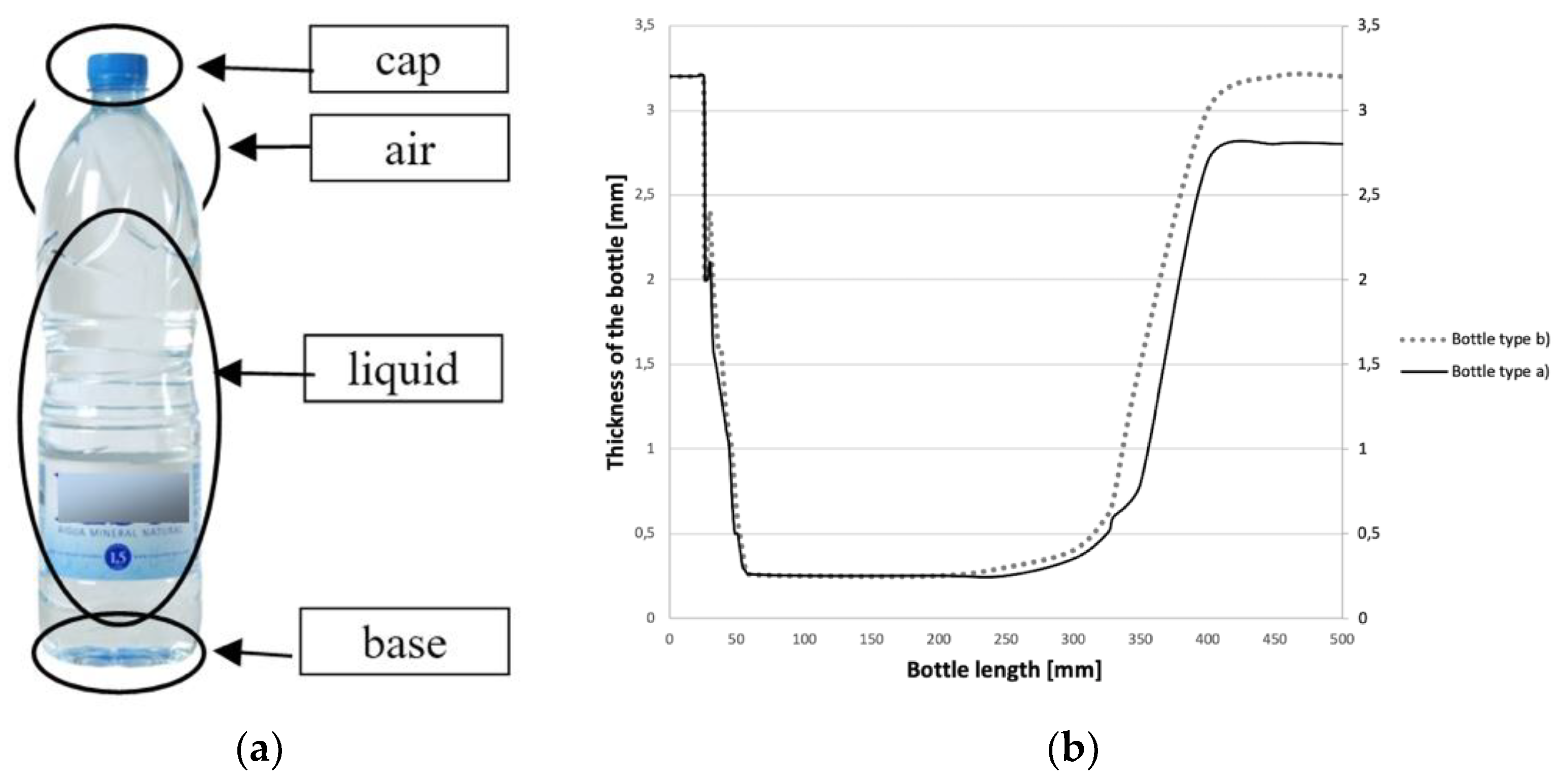
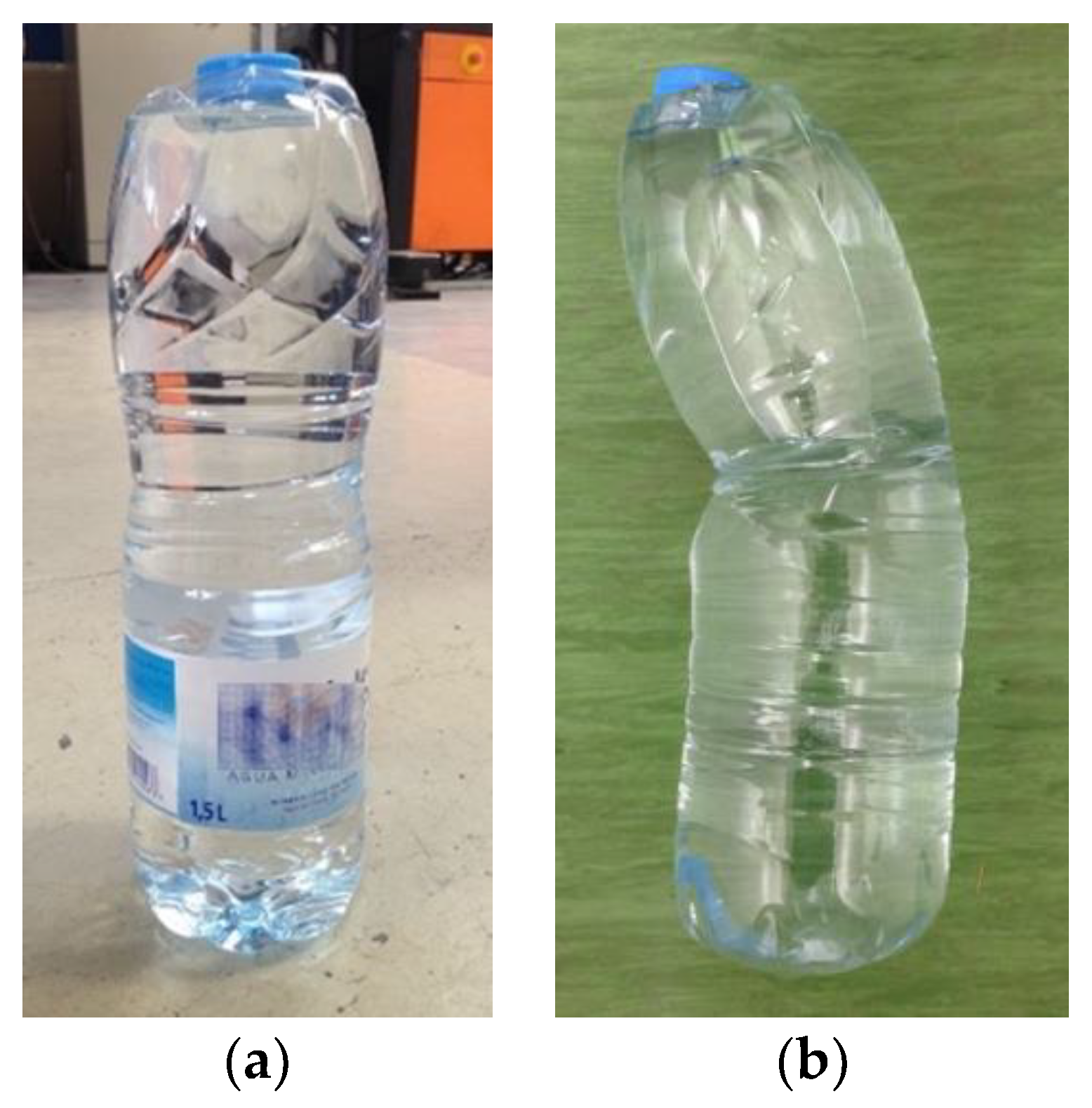
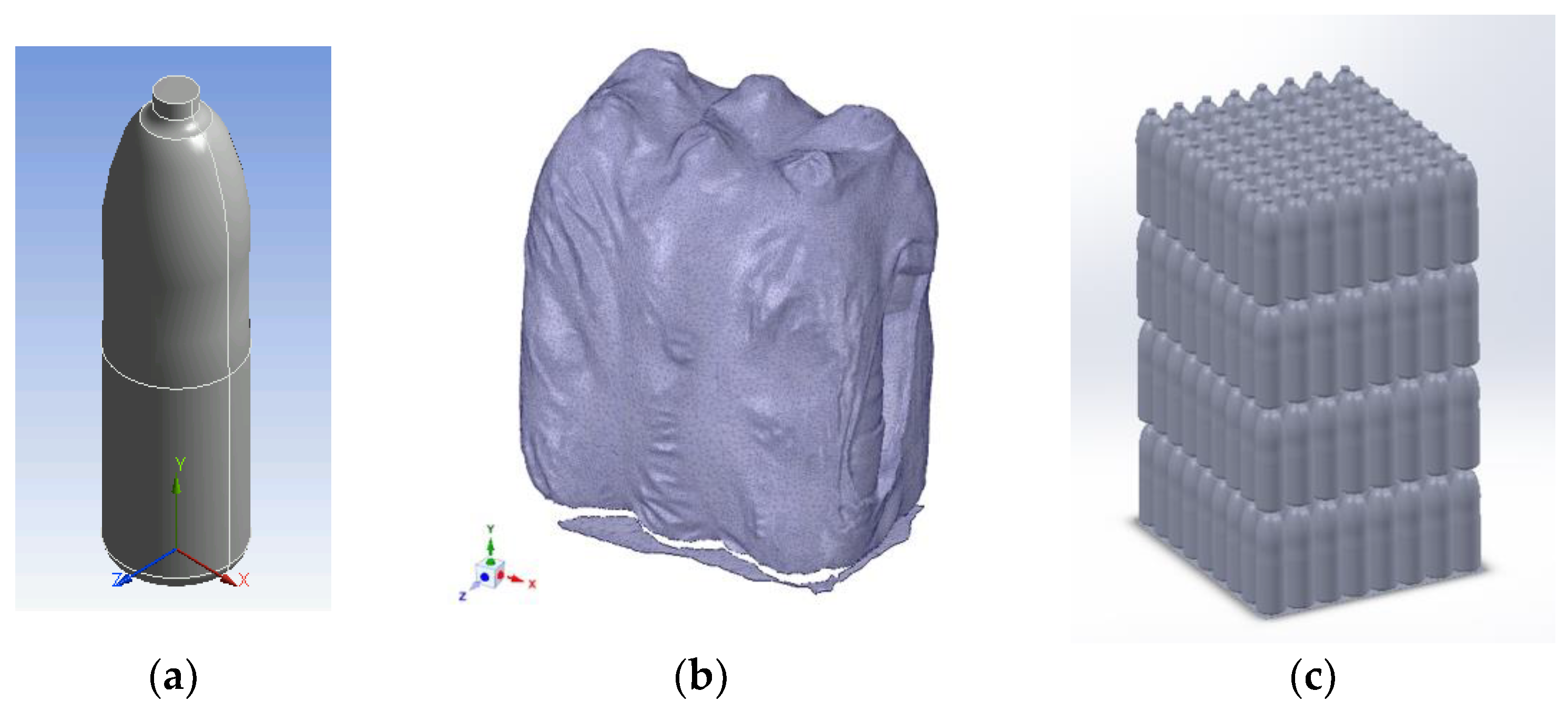
- The specific tridimensional shape of the bottle (Figure 3a);
- The variable thickness of the profile (Figure 3b);
- The orthotropic nonlinear (elastoplastic) behavior of PET;
- The cap rigidity, thicker than the profile, made of a more resistant PE;
- The column of incompressible liquid, able to transmit pressure to the inner walls of the bottle;
- The air, trapped between the free surface of the fluid and the bottle, as a compressible element.
2.2. Dual Approach
3. Experiments
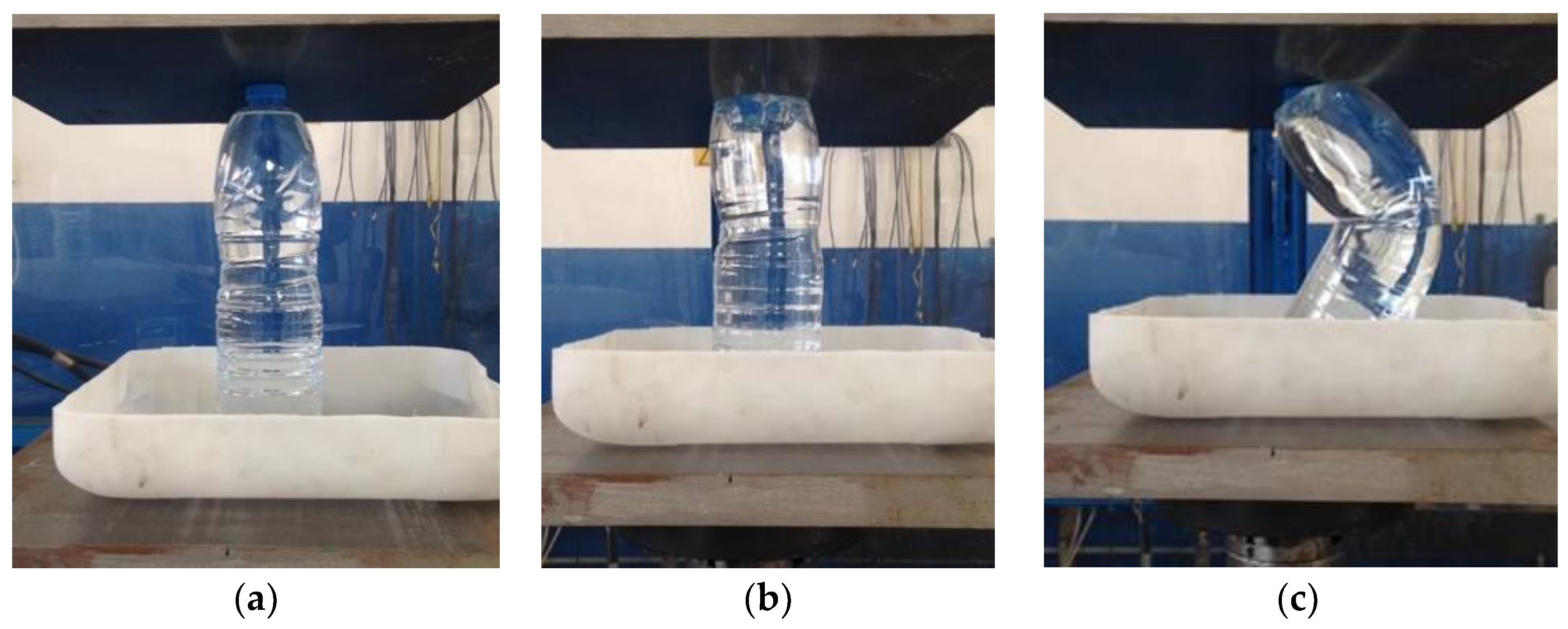
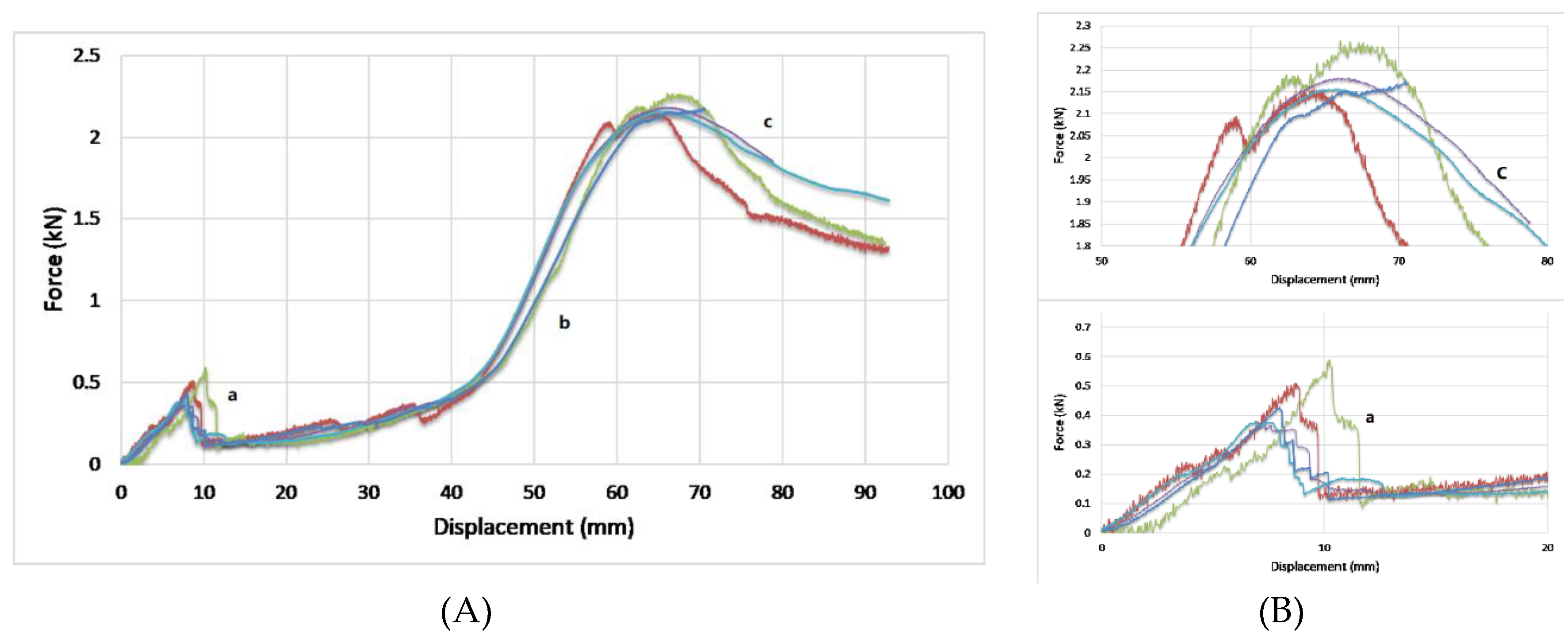
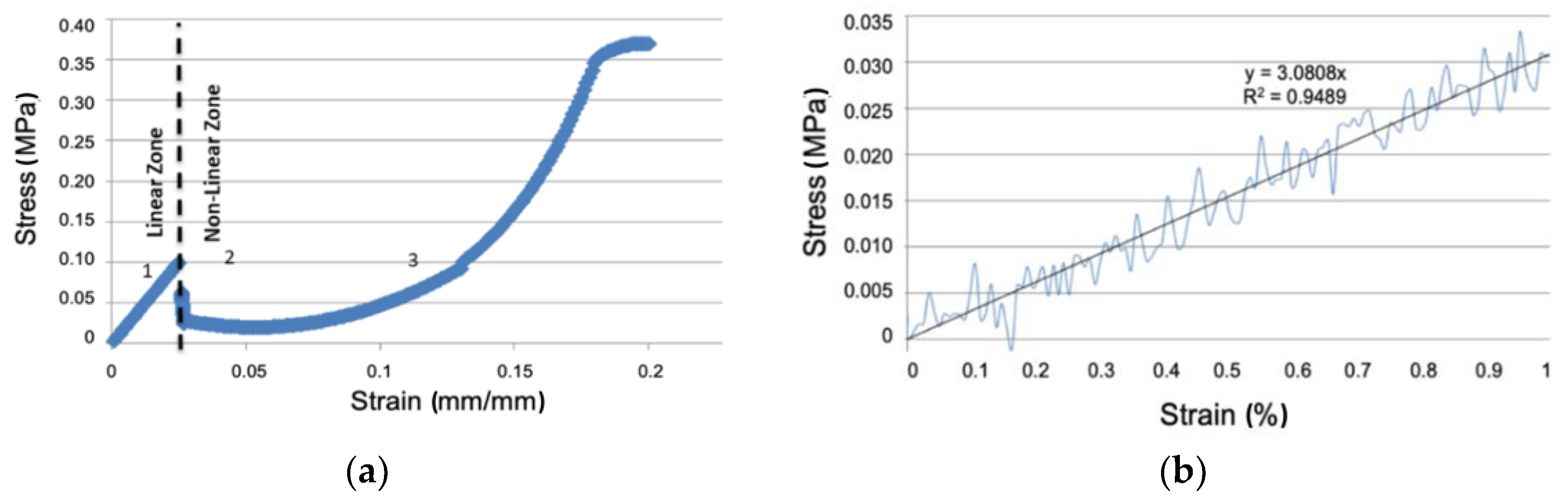
3.1. Experimental Evidence
3.2. An Interpretation
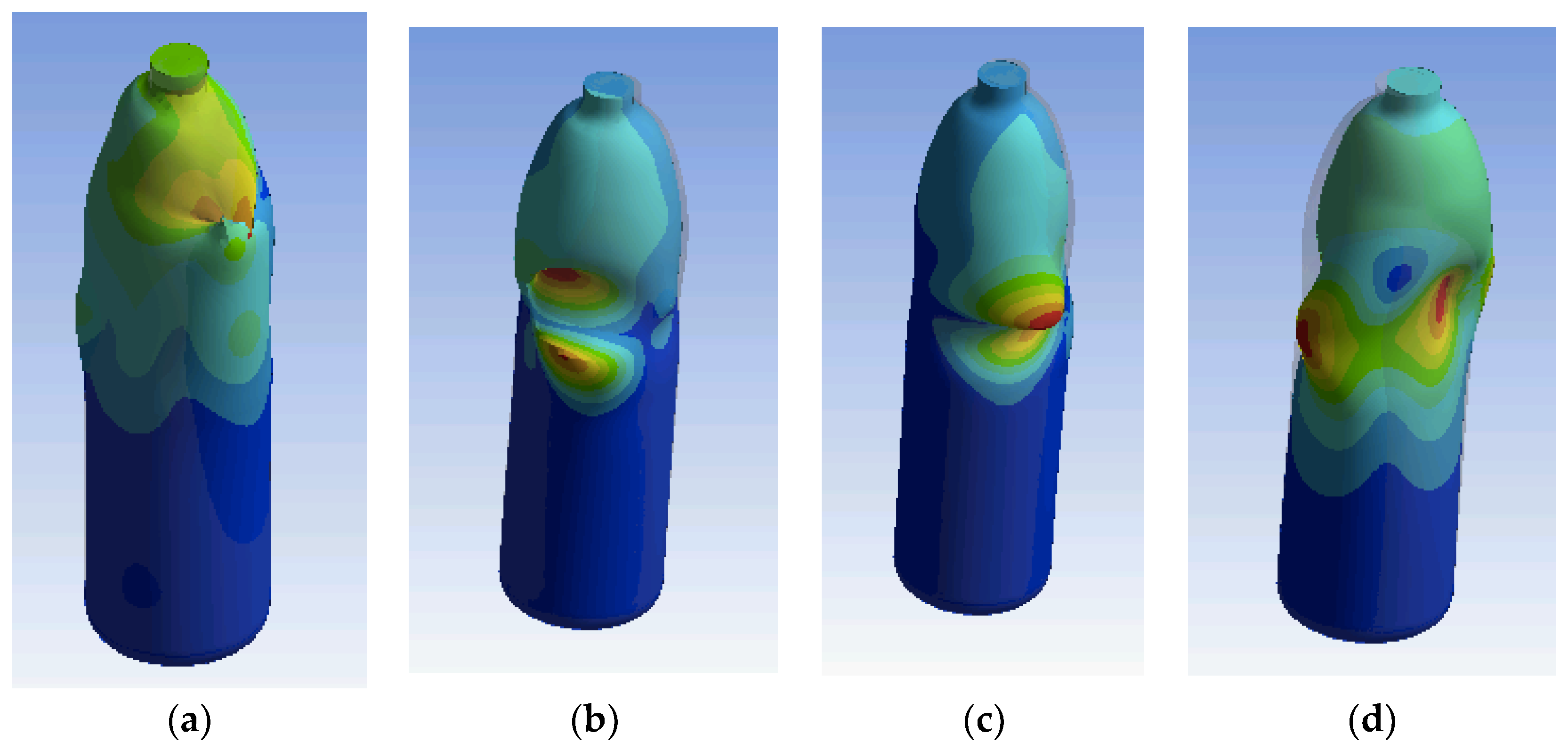
4. Numerical Models
4.1. Shell (2D) Model
4.2. Solid (3D) Model
5. Discussion
- The accurate moment of inertia is smaller because of all the tapered curves of the bottle’s shape, being clearly smaller than that of whole cylinder;
- The elastic modulus used in the theoretical calculations was obtained from experiments and has a high standard deviation due to the small sampling; being potentially smaller;
- The experimental buckling force was also provided with small sampling presenting high standard deviation; being potentially higher;
- Not only the actual moment of inertia is lower, but the tapered region in the center of the bottle acts as a massive stress concentrator, also justifying low values obtained in experiments in comparison to the theoretical model.
6. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Queiroz, J.P.T.P.; Silveira, A.C.F.; Vannucchi de Camargo, F.; Vaccari, M. A brief review on palletized products transportation technology. FME Trans. 2018, 46, 101–107. [Google Scholar]
- Vannucchi de Camargo, F.; Queiroz, J.P.T.P.; Silveira, A.C.F.; Pin, T.F.P.; Vaccari, M. Retrospect of the influence of transportation in the delivered quality of palletized product. Int. J. Qual. Res. 2018, 12, 773–788. [Google Scholar]
- Škerlič, S.; Muha, R.; Logožar, K. A decision-model for controlling logistic cost. Tech. Gaz. 2016, 23, 145–146. [Google Scholar]
- Colvile, R.N.; Hutchinson, E.J.; Mindell, J.S.; Warren, R.F. The transport sector as a source of air pollution. Atmos. Environ. 2001, 35, 1537–1565. [Google Scholar] [CrossRef]
- Càvera, L. From the market laboratory, the following packaging progresses. Leader 2015, 5, 24–25. [Google Scholar]
- Pierra, A.D.; Stewart, R.D. International Logistics, The Management of International Trade Operations, 4th ed.; Cicero Books Publisher: Berea, OH, USA, 2010. [Google Scholar]
- Mussomeli, A.; Gretchen, M.; Troy, B. Joint Industry Unsaleables Report: The Real Causes and Actionable Solutions. Available online: https://www.gmaonline.org/downloads/research-and-reports/UnsaleablesFINAL091108.pdf (accessed on 14 October 2019).
- Kandasamy, R.; Khamis, A.B. Effects of heat and mass transfer on nonlinear MHD boundary layer flow over a shrinking sheet in the presence of suction. Appl. Math. Mech. 2008, 29, 1309. [Google Scholar]
- Pagliosa, C.; Caires, F.C.; Bataglia, D.; Pelaquim, J. Strength Analysis of Thermoplastic Containers Using Numerical Simulation. In Proceedings of the Science in the Age of Experience 2016, Boston, MA, USA, 23–27 May 2016. [Google Scholar]
- Fragassa, C.; Macaluso, I.; Vaccari, M.; Lucisano, G. Measuring the mechanical and climatic conditions encountered by palletized products in handling and transport. FME Trans. 2017, 45, 382–393. [Google Scholar] [CrossRef]
- Paternoster, A.; Vanlanduit, S.; Springael, J.; Braet, J. Vibration and shock analysis of specific events during truck and train transport of food products. Food Packag. Shelf Life 2018, 15, 95–104. [Google Scholar] [CrossRef]
- Singh, S.P.; Burgess, G. Effect of internal gas pressure on the compression strength of beverage cans and plastic bottles. J. Test. Eval. 1993, 21, 129–131. [Google Scholar]
- Survanjumrat, C.; Chaichanasiri, E. Finite element method for creep testing of high density polyethylene lubricant oil bottle. Kasetsart J. 2014, 48, 487–497. [Google Scholar]
- Huang, H.H.; Chen, L.W.; Lu, W.H.; Lin, W.C.; Chen, Y.C. Design and simulation analysis of lightweight HDPE milk bottle. Polym. Polym. Compos. 2018, 26, 91–98. [Google Scholar] [CrossRef]
- Karac, A.; Ivankovic, A. Investigating the behaviour of fluid-filled polyethylene containers under base drop impact: A combined experimental/numerical approach. Int. J. Impact Eng. 2009, 36, 621–631. [Google Scholar] [CrossRef]
- Van Dijk, R.; Van Keulen, F.; Sterk, J.C. Simulation of closed thin-walled structures partially filled with fluid. Int. J. Solids Struct. 2000, 37, 6063–6083. [Google Scholar] [CrossRef]
- Vaidya, R. Structural Analysis of Poly Ethylene Terephthalate Bottles Using the Finite Element Method. Ph.D. Thesis, Oklahoma State University, Stillwater, OK, USA, 2012. [Google Scholar]
- ASTM, D2659-16. Standard Test Method for Column Crush Properties of Blown Thermoplastic Containers; ASTM Standard on Disc.: Philadelphia, PA, USA, 2005; Volume 8, Available online: https://webstore.ansi.org/standards/astm/astmd265916 (accessed on 14 October 2019).
- Rajakutty, A. Static and Dynamic Mechanical Properties of Amorphous Recycled Poly-Ethylene Terephthalate. Ph.D. Thesis, Oklahoma State University, Stillwater, OK, USA, 2012. [Google Scholar]
- Van Dijk, R.; Sterk, J.C.; Sgorbani, D.; Van Keulen, F. Lateral deformation of plastic bottles: Experiments, simulations and prevention. Packag. Technol. Sci. Int. J. 1998, 11, 91–117. [Google Scholar] [CrossRef]
- Abbès, B.; Zaki, O.; Safa, L. Experimental and numerical study of the aging effects of sorption conditions on the mechanical behavior of polypropylene bottles under columnar crush conditions. Polym. Test. 2010, 29, 902–909. [Google Scholar] [CrossRef]
- Baude, C.K. A Preliminary Analysis of PET Barrier Technologies and Mechanical Performance Related to a 3L PET Wine Bottle. Master’s Thesis, Department of Packaging Science College of Applied Science and Technology Rochester Institute of Technology Rochester, New York, NY, USA, 2008. [Google Scholar]
- Masood, S.H.; KeshavaMurthy, V. Development of collapsible PET water fountain bottles. J. Mater. Process. Technol. 2005, 162, 83–89. [Google Scholar] [CrossRef]
- Schneider, M.H. Investigation of the stability of imperfect cylinders using structural models. Eng. Struct. 1996, 18, 792–800. [Google Scholar] [CrossRef]
- Vaziri, A. Mechanics of highly deformed elastic shells. Thin-Walled Struct. 2009, 47, 692–700. [Google Scholar] [CrossRef]
- Mukherjee, S. Virtual Simulation of Top Load Performance of Plastic Bottles. In Proceedings of the 68th Annual Technical Conference of the Society of Plastics Engineers 2010 (Antec), Orlanod, FL, USA, 16–20 May 2010. [Google Scholar]
- Reed, P.E.; Breedveld, G.; Lim, B.C. Simulation of the Drop Impact for Moulded Thermoplastic Containers. Int. J. Impact Eng. 2000, 24, 133–153. [Google Scholar] [CrossRef]
- Suvanjumrat, C.; Puttapitukporn, T.; Thusneyapan, S. Comparison of Simulation for Fluid-Structure Interaction of Water Filled Plastic Bottles during Drop Test. Master’s Thesis, Kasetsart University, Bangkok, Thailand, 2007. [Google Scholar]
- Harshath, C.; Chandan, R.; Shashikantha, N.; Venkatesha, R. Buckling Evaluation of a Plastic Bottle Design. Int. Res. J. Eng. Technol. 2018, 5, 549–553. [Google Scholar]
- Karalekas, D.; Rapti, D.; Papakaliatakis, G.; Tsartolia, E. Numerical and experimental investigation of the deformational behaviour of plastic containers. Packag. Technol. Sci. Int. J. 2011, 14, 185–191. [Google Scholar] [CrossRef]
- Yuan, W.; Xie, L.H.; Zhang, G.M.; Liao, D.Z.; Lu, J.D. Structural Analysis and Design Optimization of PET Beer Bottles. Appl. Mech. Mater. 2013, 312, 21–24. [Google Scholar] [CrossRef]
- Allahkarami, M.; Ahmed, R.U.; Bandla, S. Thickness measurement methods aiding light weighting of PET bottles. In Proceedings of the 72nd Annual Technical Conference of the Society of Plastic Engineers (SPE Antec), Las Vegas, NV, USA, 28–30 April 2014. [Google Scholar]
- Fugen, D.; Bilal, D. An energy saving approach in the manufacture of carbonated soft drink bottles. Procedia Eng. 2012, 49, 280–286. [Google Scholar]
- Callister, W.D.; Rethwisch, D.G. Materials Science and Engineering: An Introduction; John Wiley & Sons: New York, NY, USA, 2007; Volume 7, pp. 665–715. [Google Scholar]





| Polyethylene Terephthalate (PET)—Orthotropic Characteristics | |||||||||
| Density | Young Modulus X dir. | Young Modulus Y dir. | Young Modulus Z dir. | Poisson’s Ratio X, Y, Z | Shear Modulus XY | Shear Modulus YZ | Shear Modulus XZ | Tensile Yield Strength | Tensile Ultimate Strength |
| (kg/m3) | (MPa) | (MPa) | (MPa) | (/) | (MPa) | (MPa) | (MPa) | (MPa) | (MPa) |
| 1335 | 1250 | 1345 | 1250 | 0.4 | 448 | 480 | 448 | 64 | 90 |
| Polyethylene (PE)—Isotropic Elastic Characteristics | |||||||||
| Density | Young Modulus | Poisson’s Ratio | Tensile Yield Strength | Tensile Ultimate Strength | |||||
| (kg/m3) | (MPa) | (/) | (MPa) | (MPa) | |||||
| 950 | 1100 | 0.42 | 250 | 330 | |||||
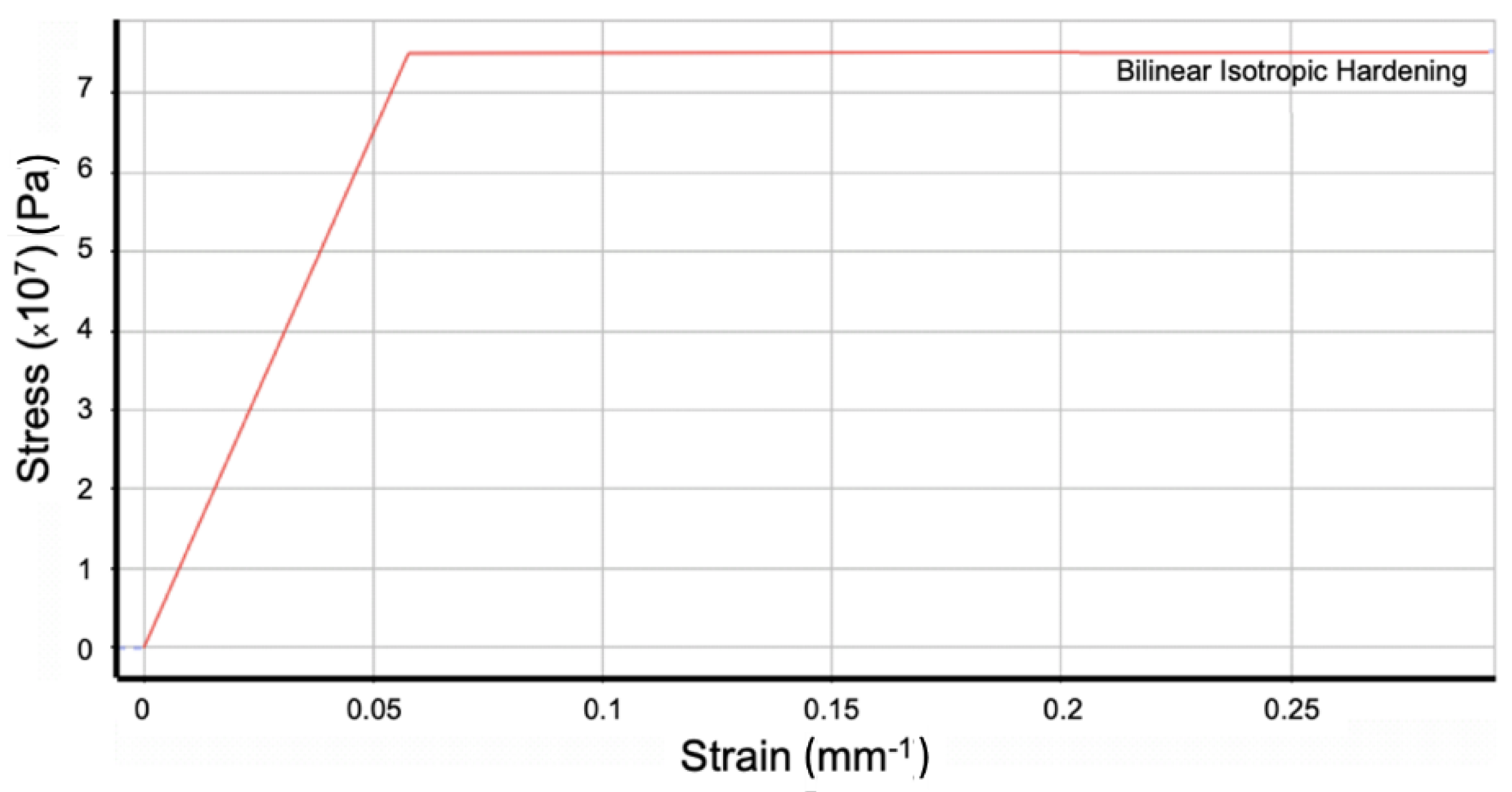
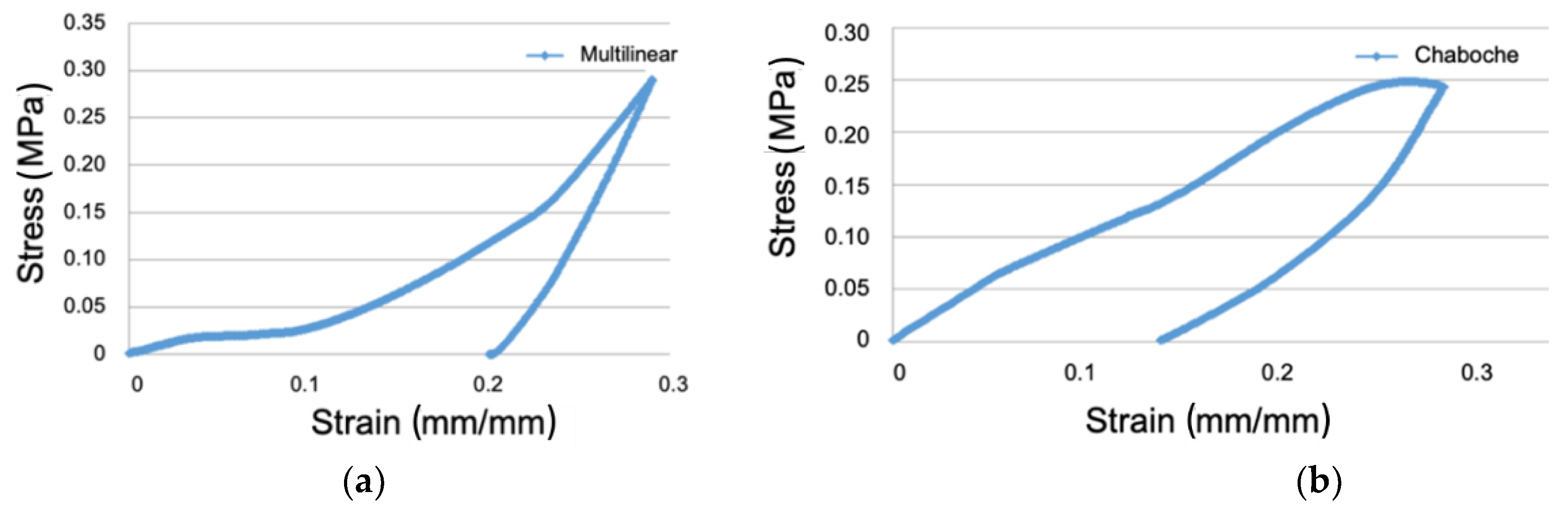
| Residual Strain | Hardening Model | |||
|---|---|---|---|---|
| Measure (εe) | Bilinear Isotropic | Multilinear Isotropic | Chaboche Kinematic | |
| mm | 46.5 | 54.9 | 61.9 | 43.2 |
| Δ | +18% | +33% | −7% | |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Pavlovic, A.; Fragassa, C.; Vegliò, L.; de Camargo, F.V.; Minak, G. Modeling Palletized Products: The Case of Semi-Filled Bottles under Top-Load Conditions. Appl. Sci. 2020, 10, 332. https://doi.org/10.3390/app10010332
Pavlovic A, Fragassa C, Vegliò L, de Camargo FV, Minak G. Modeling Palletized Products: The Case of Semi-Filled Bottles under Top-Load Conditions. Applied Sciences. 2020; 10(1):332. https://doi.org/10.3390/app10010332
Chicago/Turabian StylePavlovic, Ana, Cristiano Fragassa, Luca Vegliò, Felipe Vannucchi de Camargo, and Giangiacomo Minak. 2020. "Modeling Palletized Products: The Case of Semi-Filled Bottles under Top-Load Conditions" Applied Sciences 10, no. 1: 332. https://doi.org/10.3390/app10010332
APA StylePavlovic, A., Fragassa, C., Vegliò, L., de Camargo, F. V., & Minak, G. (2020). Modeling Palletized Products: The Case of Semi-Filled Bottles under Top-Load Conditions. Applied Sciences, 10(1), 332. https://doi.org/10.3390/app10010332









