Updated Understanding of the Ripley Landslide Kinematics Using Satellite InSAR
Abstract
:1. Introduction
1.1. Landslide Remote Sensing and Satellite InSAR
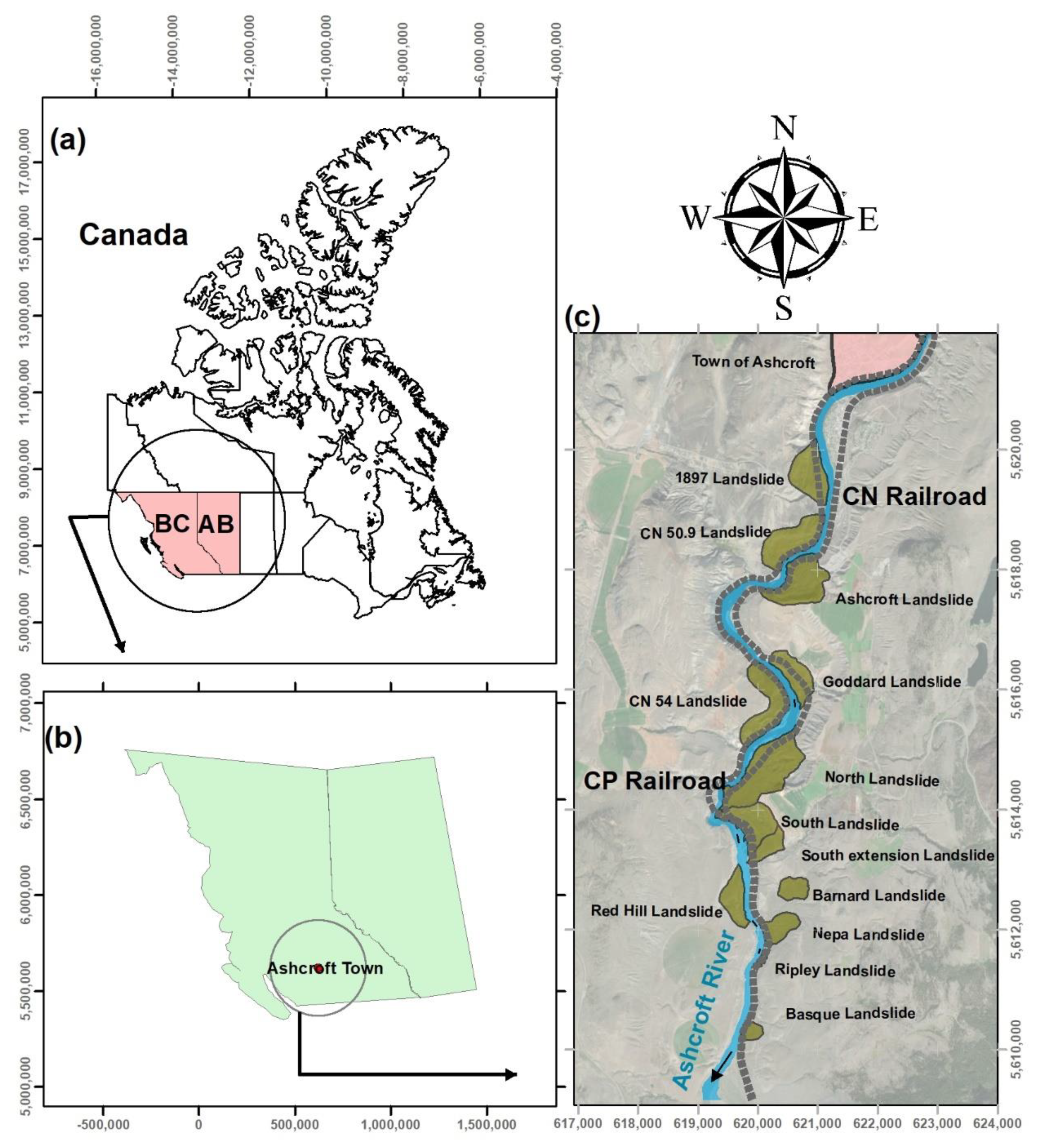
1.2. The Thompson River Valley Landslides South of Ashcroft and the Ripley Landslide
2. Materials and Methods
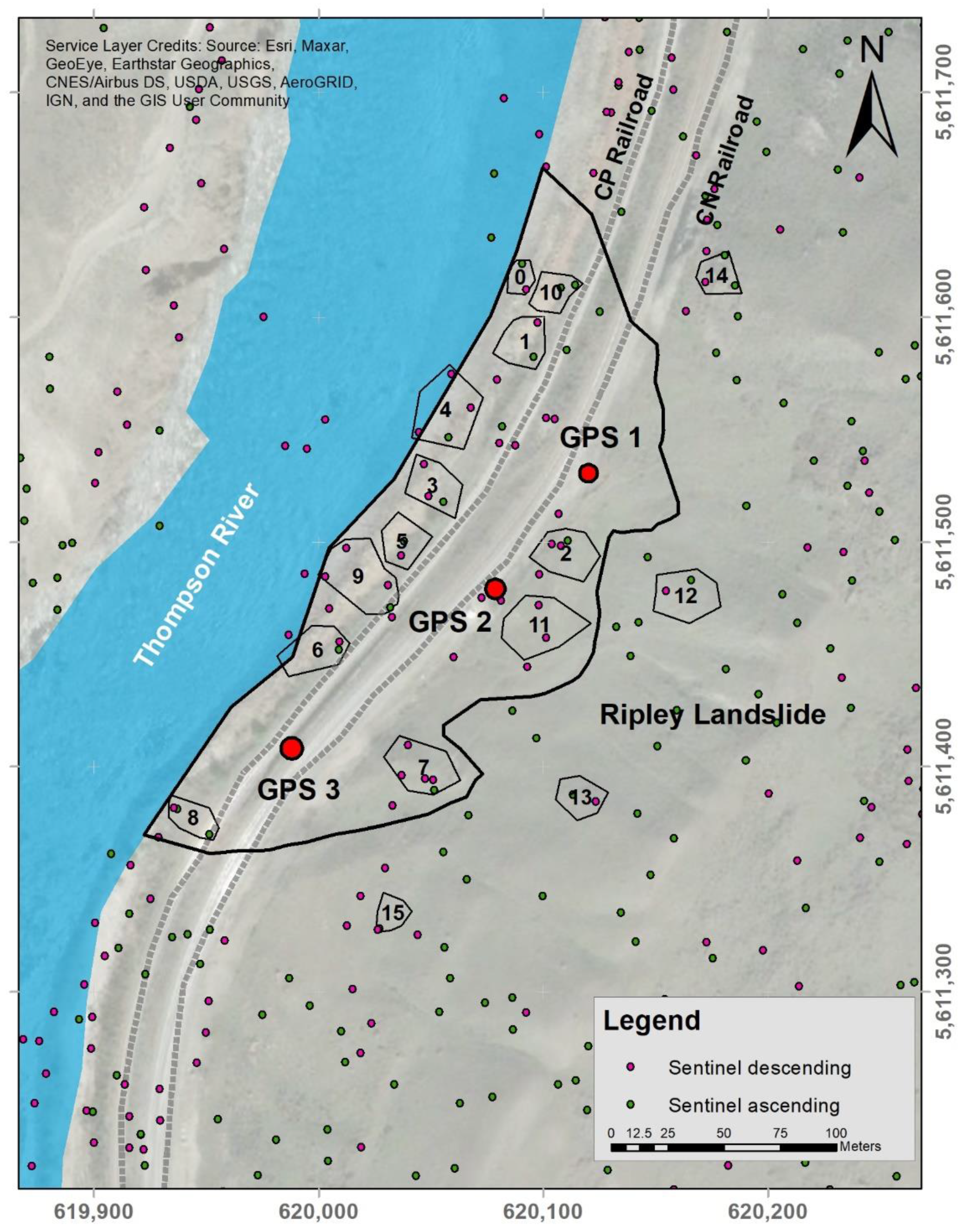
2.1. Published Displacement Monitoring Data at the Ripley Landslide
2.2. Satellite InSAR Displacement Data
Sentinel 1
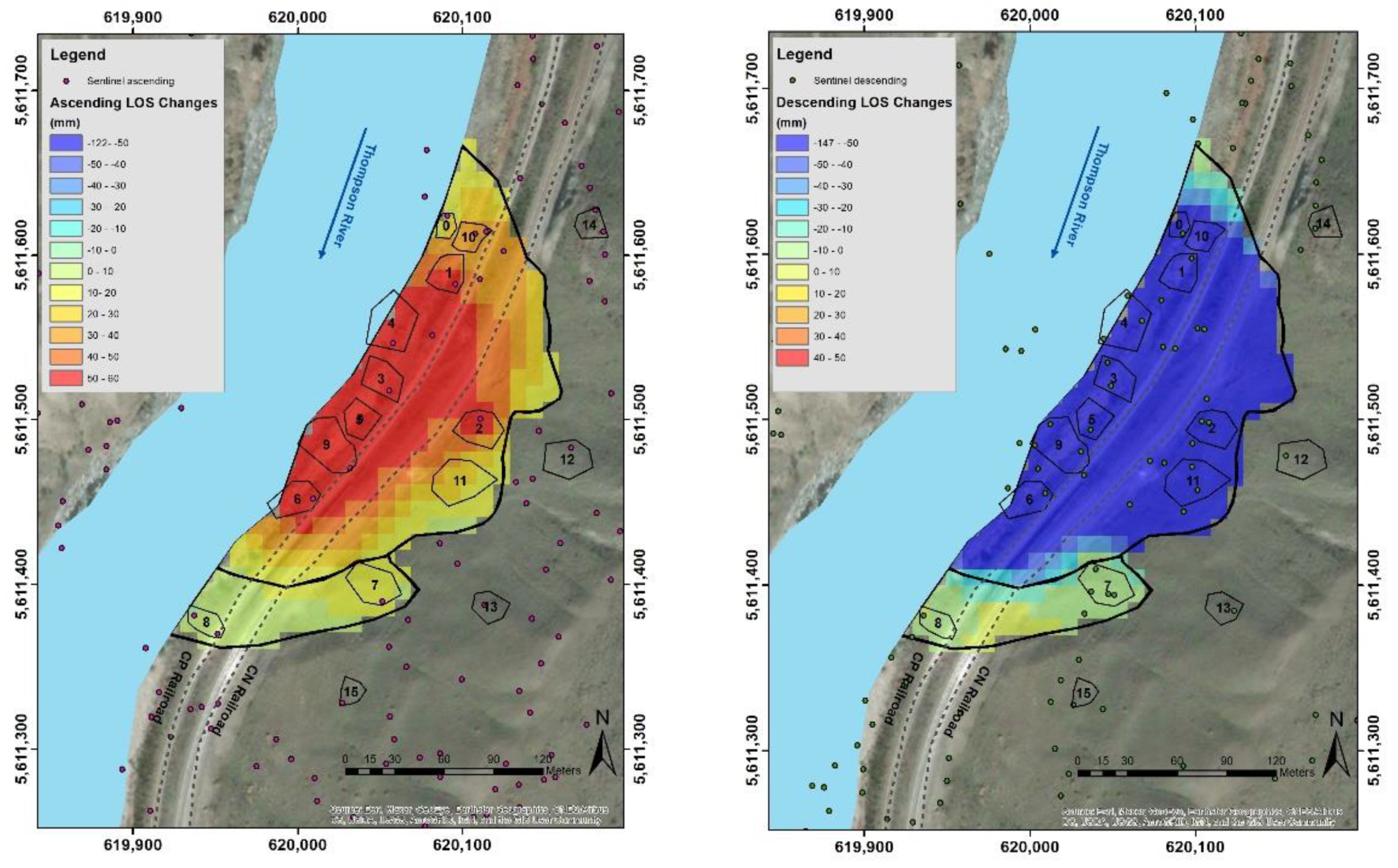
2.3. Displacement Calculation at the Surface of the Ripley Landslide Based on InSAR LOS Displacements
3. Results
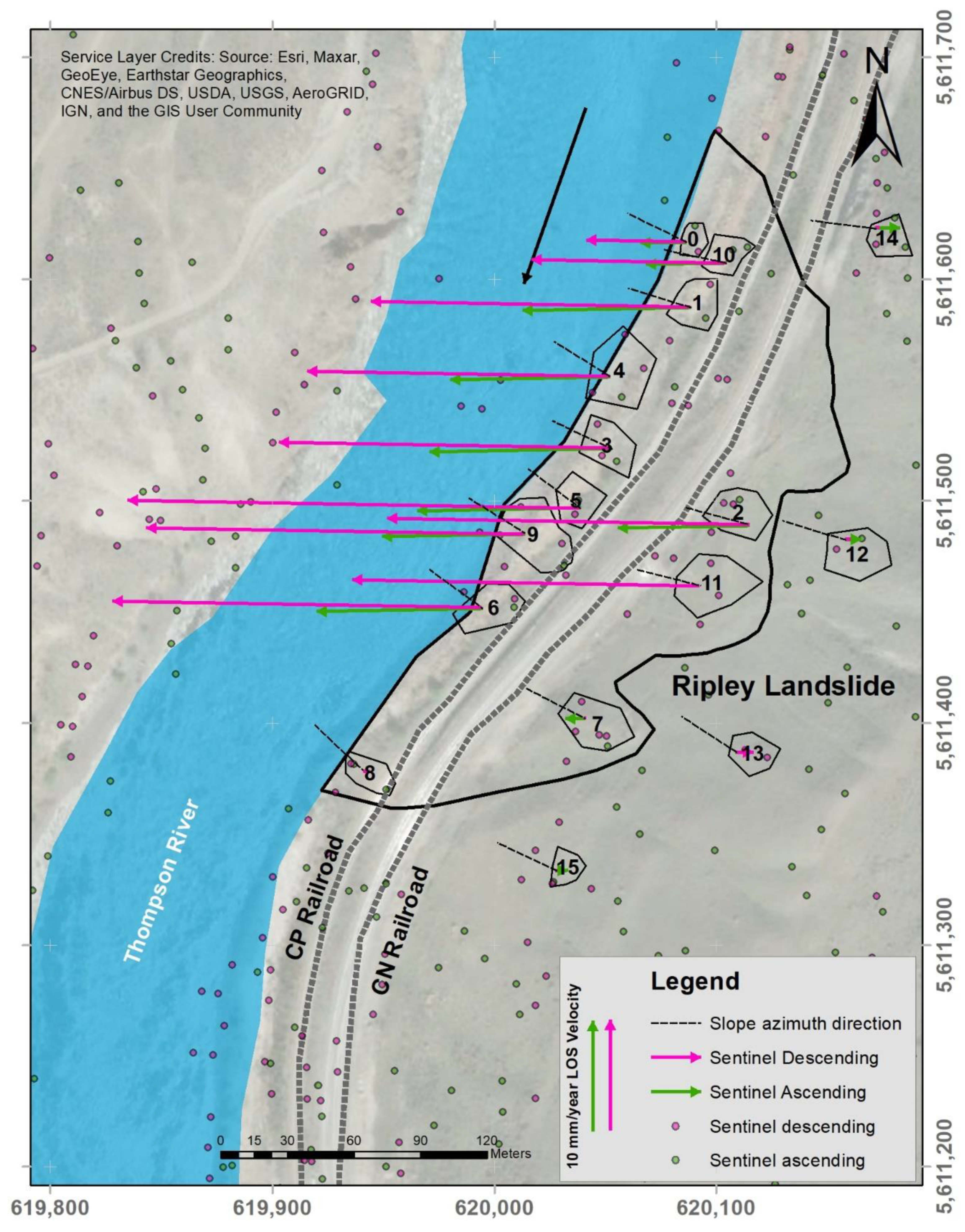
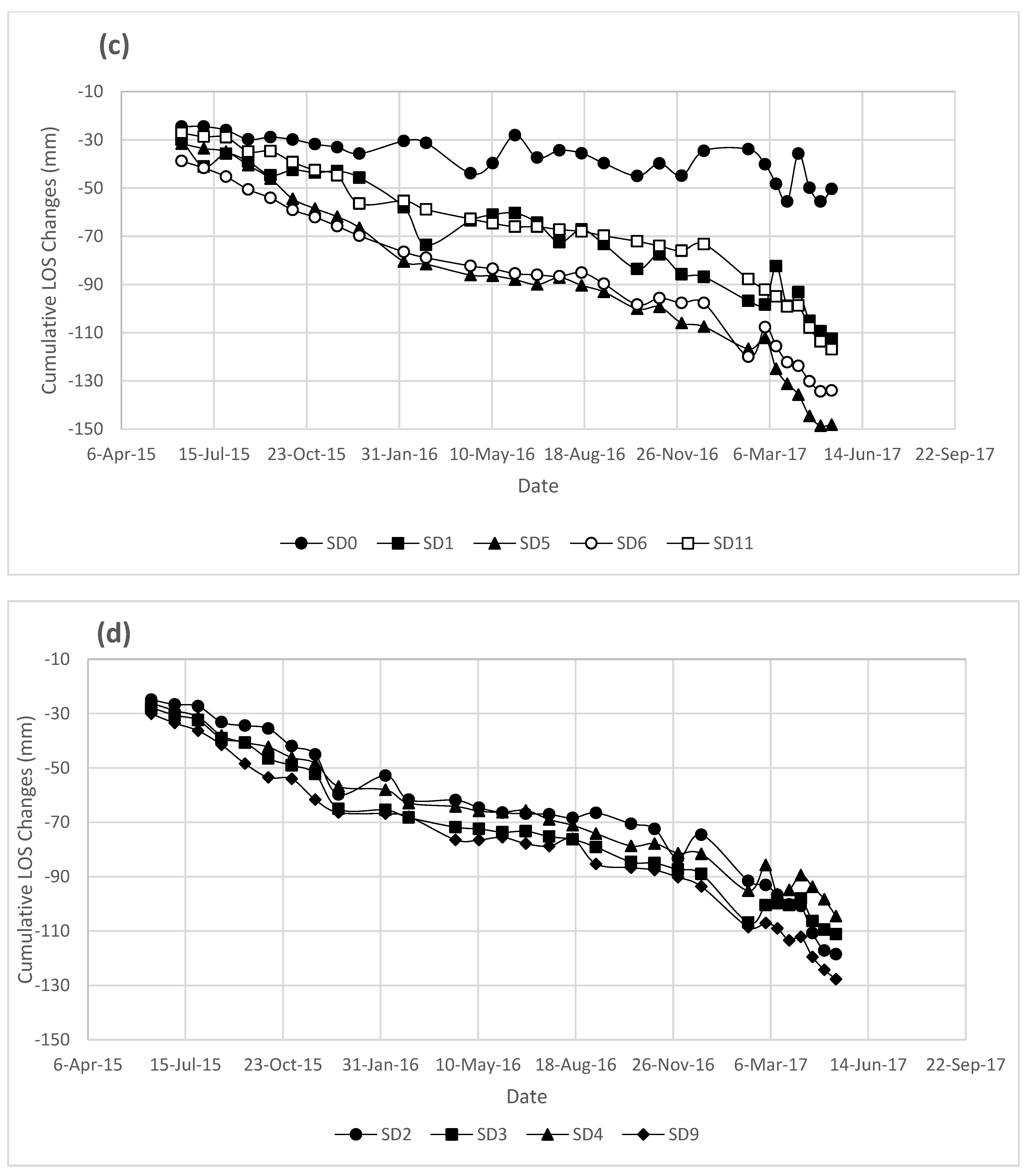
3.1. Satellite InSAR Results at the Ripley Landslide
3.2. Comparison with in-Place Displacement Monitoring Instrumentation
4. Discussion
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Charrière, M.; Humair, F.; Froese, C.; Jaboyedoff, M.; Pedrazzini, A.; Longchamp, C. From the source area to the deposit: Collapse, fragmentation, and propagation of the Frank Slide. Geol. Soc. Am. Bull. 2020, 128, 332–351. [Google Scholar] [CrossRef]
- Cruden, D.M.; Martin, C.D. Before the Frank Slide. Can. Geotech. J. 2007, 44, 765–780. [Google Scholar] [CrossRef]
- Mei, S.; Poncos, V.; Froese, C. Mapping millimetre-scale ground deformation over the underground coal mines in the Frank Slide area, Alberta, Canada, using spaceborne InSAR technology. Can. J. Remote Sens. 2008, 34, 113–134. [Google Scholar] [CrossRef]
- Vallee, M. Falling in Place: Geoscience, Disaster, and Cultural Heritage at the Frank Slide, Canada’s Deadliest Rockslide. Space Cult. 2019, 22, 66–76. [Google Scholar] [CrossRef]
- Choe, B.-H.; Blais-Stevens, A.; Samsonov, S.; Dudley, J. Sentinel-1 and RADARSAT Constellation Mission InSAR Assessment of Slope Movements in the Southern Interior of British Columbia, Canada. Remote Sens. 2021, 13, 3999. [Google Scholar] [CrossRef]
- Huntley, D.; Bobrowsky, P.; Hendry, M.; Macciotta, R.; Best, M. Multi-technique Geophysical Investigation of a Very Slow-moving Landslide near Ashcroft, British Columbia, Canada. J. Environ. Eng. Geophys. 2019, 24, 87–110. [Google Scholar] [CrossRef]
- Rodriguez, J.; Macciotta, R.; Hendry, M.T.; Roustaei, M.; Gräpel, C.; Skirrow, R. UAVs for monitoring, investigation, and mitigation design of a rock slope with multiple failure mechanisms—A case study. Landslides 2020, 17, 2027–2040. [Google Scholar] [CrossRef]
- Deane, E.; Macciotta, R.; Hendry, M.; Grapel, C.; Skirrow, R. Leveraging historical aerial photographs and digital photo-grammetry techniques for landslide investigation—A practical perspective. Landslides 2020, 17, 1989–1996. [Google Scholar] [CrossRef]
- Macciotta, R.; Gräpel, C.; Skirrow, R. Fragmented Rockfall Volume Distribution from Photogrammetry-Based Structural Mapping and Discrete Fracture Networks. Appl. Sci. 2020, 10, 6977. [Google Scholar] [CrossRef]
- Woods, A.; Macciotta, R.; Hendry, M.; Stewart, T.; Marsh, J. Updated understanding of the deformation characteristics of the Checkerboard Creek rock slope through GB-InSAR monitoring. Eng. Geol. 2020, 281, 105974. [Google Scholar] [CrossRef]
- Lan, H.; Zhao, X.; Macciotta, R.; Peng, J.; Li, L.; Wu, Y.; Zhu, Y.; Liu, X.; Zhang, N.; Liu, S.; et al. The cyclic expansion and contraction characteristics of a loess slope and implications for slope stability. Sci. Rep. 2021, 11, 2250. [Google Scholar] [CrossRef] [PubMed]
- Rodriguez, J.; Deane, E.; Hendry, M.; Macciotta, R.; Evans, T.; Gräpel, C.; Skirrow, R. Practical evaluation of single-frequency dGNSS for monitoring slow-moving landslides. Landslides 2021, 18, 3671–3684. [Google Scholar]
- Macciotta, R.; Hendry, M.T. Remote Sensing Applications for Landslide Monitoring and Investigation in Western Canada. Remote Sens. 2021, 13, 366. [Google Scholar] [CrossRef]
- Hendry, M.T.; Macciotta, R.; Martin, C.D.; Reich, B. Effect of Thompson River elevation on velocity and instability of Ripley Slide. Can. Geotech. J. 2015, 52, 257–267. [Google Scholar] [CrossRef]
- Macciotta, R.; Hendry, M.; Martin, C.D. Developing an early warning system for a very slow landslide based on displacement monitoring. Nat. Hazards 2015, 81, 887–907. [Google Scholar] [CrossRef]
- Journault, J.; Macciotta, R.; Hendry, M.T.; Charbonneau, F.; Huntley, D.; Bobrowsky, P.T. Measuring displacements of the Thompson River valley landslides, south of Ashcroft, BC, Canada, using satellite InSAR. Landslides 2018, 15, 621–636. [Google Scholar] [CrossRef]
- Huntley, D.; Bobrowsky, P.; Hendry, M.; Macciotta, R.; Elwood, D.; Sattler, K.; Best, M.; Chambers, J.; Meldrum, P. Application of multi-dimensional electrical resistivity tomography datasets to investigate a very slow-moving landslide near Ash-croft, British Columbia, Canada. Landslides 2019, 16, 1033–1042. [Google Scholar] [CrossRef]
- Schafer, M. Kinematics and Controlling Mechanics of the Slow Moving Ripley Landslide. Master’s Thesis, University of Alberta, Edmonton, AL, Canada, 2016. [Google Scholar]
- Huntley, D.; Holmes, J.; Bobrowsky, P.; Chambers, J.; Meldrum, P.; Wilkinson, P.; Donohue, S.; Elwood, D.; Sattler, K.; Hendry, M.; et al. Hydrogeological and geophysical properties of the very slow-moving Ripley Landslide, Thompson River valley, British Columbia. Can. J. Earth Sci. 2020, 57, 1371–1391. [Google Scholar] [CrossRef]
- Xu, D.; Hu, X.-Y.; Shan, C.-L.; Li, R.-H. Landslide monitoring in southwestern China via time-lapse electrical resistivity tomography. Appl. Geophys. 2016, 13, 1–12. [Google Scholar] [CrossRef]
- Herrera, G.; Gutierrez, F.; Garcia-Davalilo, J.C.; Guerrero, J.; Notti, D.; Galve, J.P.; Fernandez-Merodo, J.A.; Cooksley, G. Multi-sensor advanced DInSAR monitoring of very slow landslides: The Tena Valley case study (Central Spanish Pyrenees). Remote Sens. Environ. 2012, 381–387, 08037. [Google Scholar]
- Colesanti, C.; Wasowski, J. Investigating landslides with space-borne Synthetic Aperture Radar (SAR) interferometry. Eng. Geol. 2006, 88, 173–199. [Google Scholar] [CrossRef]
- Vadivel, S.K.P.; Kim, D.J.; Kim, Y.C. Time-series InSAR Analysis and post-processing Using ISCE-StaMPS package for Meas-uring Bridge Displacements. Korean J. Remote Sens. 2020, 36, 527–534. [Google Scholar]
- Abe, T.; Iwahana, G.; Efremov, P.V.; Desyatkin, A.R.; Kawamura, T.; Fedorov, A.; Zhegusov, Y.; Yanagiya, K.; Tadono, T. Surface displacement revealed by L-band InSAR analysis in the Mayya area, Central Yakutia, underlain by continuous permafrost. Earth Planets Space 2020, 72, 138. [Google Scholar] [CrossRef]
- Motagh, M.; Shamshiri, R.; Haghighi, M.H.; Wetzel, H.-U.; Akbari, B.; Nahavandchi, H.; Roessner, S.; Arabi, S. Quantifying groundwater exploitation induced subsidence in the Rafsanjan plain, southeastern Iran, using InSAR time-series and in situ measurements. Eng. Geol. 2017, 218, 134–151. [Google Scholar] [CrossRef]
- Hooper, A.; Bekaert, D.; Spaans, K.; Arıkan, M. Recent advances in SAR interferometry time series analysis for measuring crustal deformation. Tectonophysics 2012, 514–517, 1–13. [Google Scholar] [CrossRef]
- Chen, B.; Li, Z.; Zhang, C.; Ding, M.; Zhu, W.; Zhang, S.; Han, B.; Du, J.; Cao, Y.; Zhang, C.; et al. Wide Area Detection and Distribution Characteristics of Landslides along Sichuan Expressways. Remote Sens. 2022, 14, 3431. [Google Scholar] [CrossRef]
- Liu, Y.; Yao, X.; Gu, Z.; Zhou, Z.; Liu, X.; Chen, X.; Wei, S. Study of the Automatic Recognition of Landslides by Using InSAR Images and the Improved Mask R-CNN Model in the Eastern Tibet Plateau. Remote Sens. 2022, 14, 3362. [Google Scholar] [CrossRef]
- Mastro, P.; Masiello, G.; Serio, C.; Pepe, A. Change Detection Techniques with Synthetic Aperture Radar Images Experimens with Random Forests and Sentinel-1 Observations. Remote Sens. 2022, 14, 3323. [Google Scholar] [CrossRef]
- Abdel-Hamid, A.; Dubovyk, O.; Greve, K. The potential of sentinel-1 InSAR coherence for grasslands monitoring in Eastern Cape, South Africa. Int. J. Appl. Earth Obs. Geoinf. 2021, 98, 102306. [Google Scholar] [CrossRef]
- Dumka, K.R.; SuriBabu, D.; Malik, K.; Prajapati, S.; Narain, P. PS-InSAR derived deformation study in the Kachchh, Western India. Appl. Comput. Geosci. 2020, 8, 100041. [Google Scholar] [CrossRef]
- Castellazzi, P.; Schmid, W. Interpreting C-band InSAR ground deformation data for large-scale groundwater management in Australia. J. Hydrol. Reg. Stud. 2020, 34, 100774. [Google Scholar] [CrossRef]
- Mancini, F.; Grassi, F.; Cenni, N. A Workflow Based on SNAP–StaMPS Open-Source Tools and GNSS Data for PSI-Based Ground Deformation Using Dual-Orbit Sentinel-1 Data: Accuracy Assessment with Error Propagation Analysis. Remote Sens. 2021, 13, 753. [Google Scholar] [CrossRef]
- Huntley, D.; Rotheram-Clarke, D.; Pon, A.; Tomaszewicz, A.; Leighton, J.; Cocking, R.; Joseph, J. Benchmarked RADARSAT-2, SENTINEL-1 and RADARSAT Constellation Mission Change-Detection Monitoring at North Slide, Thompson River Valley, British Columbia: Ensuring a Landslide-Resilient National Railway Network. Can. J. Remote Sens. 2021, 47, 635–656. [Google Scholar] [CrossRef]
- Clague, J.J.; Evans, S.G. Geologic Framework of Large Historic Landslides in Thompson River Valley, British Columbia. Environ. Eng. Geosci. 2003, 9, 201–212. [Google Scholar] [CrossRef]
- Huntley, D.H.; Bobrowsky, P.T. Surficial geology and monitoring of the Ripley Slide, near Ashcroft, British Columbia, Canada. Geol. Surv. Can. 2014, 7531, 21. [Google Scholar] [CrossRef]
- Porter, M.J.; Savigny, K.W.; Keegan, T.R.; Bunce, C.M.; MacKay, C. Controls on stability of the Thompson River landslides. In Proceedings of the 55th Canadian Geotechnical Conference, Niagara Falls, ON, Canada, 20–23 October 2002. [Google Scholar]
- Eshraghian, A.; Martin, C.D.; Cruden, D.M. Complex Earth Slides in the Thompson River Valley, Ashcroft, British Columbia. Environ. Eng. Geosci. 2007, 13, 161–181. [Google Scholar] [CrossRef]
- Macciotta, R.; Hendry, M.; Martin, D.; Eldwood, E.; Lan, H.; Huntley, D.; Bobrowsky, P.; Sladen, W.; Bunce, C.; Choi, E.; et al. Monitoring of the Ripley Slide in the Thompson River Valley, BC. In Proceedings of the Geohazards 6—6th Canadian Geohazards Conference, Kingston, ON, Canada, 15–18 June 2014. [Google Scholar] [CrossRef]
- Sattler, K.; Elwood, D.; Hendry, M.T.; Huntley, D.; Holmes, J.; Wilkinson, P.B.; Chambers, J.; Donohue, S.; Meldrum, P.I.; Macciotta, R.; et al. Quantifying the contribution of matric suction on changes in stability and displacement rate of a translational landslide in glaciolacustrine clay. Landslides 2021, 18, 1675–1689. [Google Scholar] [CrossRef]
- Cruden, D.M.; Varnes, D.J. Landslide Types and Processes, Transportation Research Board; U.S. National Academy of Sciences, Special Report, 247; National Academy of Sciences: Washington, DC, USA, 1996. [Google Scholar]
- Hungr, O.; Leroueil, S.; Picarelli, L. The Varnes classification of landslide types, an update. Landslides 2014, 11, 167–194. [Google Scholar] [CrossRef]
- Huntley, D.; Rotheram-Clarke, D.; Cocking, R.; Joseph, J.; Bobrowsky, P. Understanding Plateau and Prairie Landslides: Annual Report on Landslide Research in the Thompson River Valley, British Columbia, and the Assiniboine River Valley, Manitoba-Saskatchewan 2020–2021 to 2021–2022; Geological Survey of Canada: Ottawa, ON, USA, 2021.






| Radar Data Information | ||
|---|---|---|
| Satellite | Sentinel 1 | Sentinel 1 |
| Acquisition Geometry | Ascending | Descending |
| Period Covered by Imagery | 3 November 2014 to 17 March 2018 | 6 November 2014 to 1 April 2018 |
| No. of Processed Images | 51 | 59 |
| Coordinate System | WGS 1984 | WGS 1984 |
| Area of Interest | ||
| Number of PS + DS | 194,083 (112,343 PS, 81,740 DS) | 178,396 (89,510 PS, 88,886 DS) |
| Sensor Mode | IW | IW |
| Image Resolution | 20 m × 5 m | 20 m × 5 m |
| Satellite | Orbit Geometry | Track | Sensor Mode | Symbol | Angle (Degree) |
|---|---|---|---|---|---|
| Sentinel | Ascending | 64 | IW | θ δ | 38.66 11.33 |
| Sentinel | Descending | 115 | IW | θ δ | 44.29 7.79 |
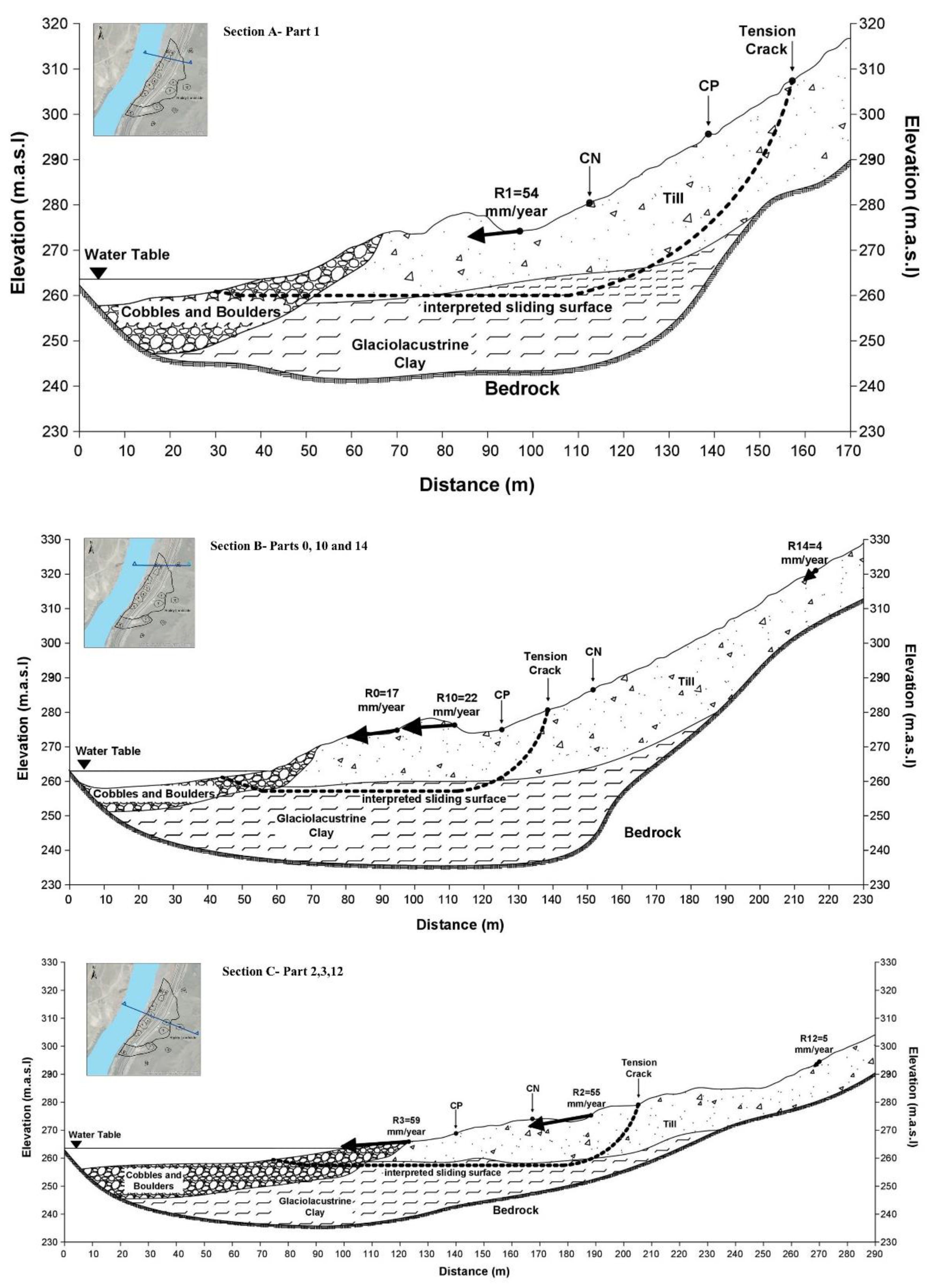
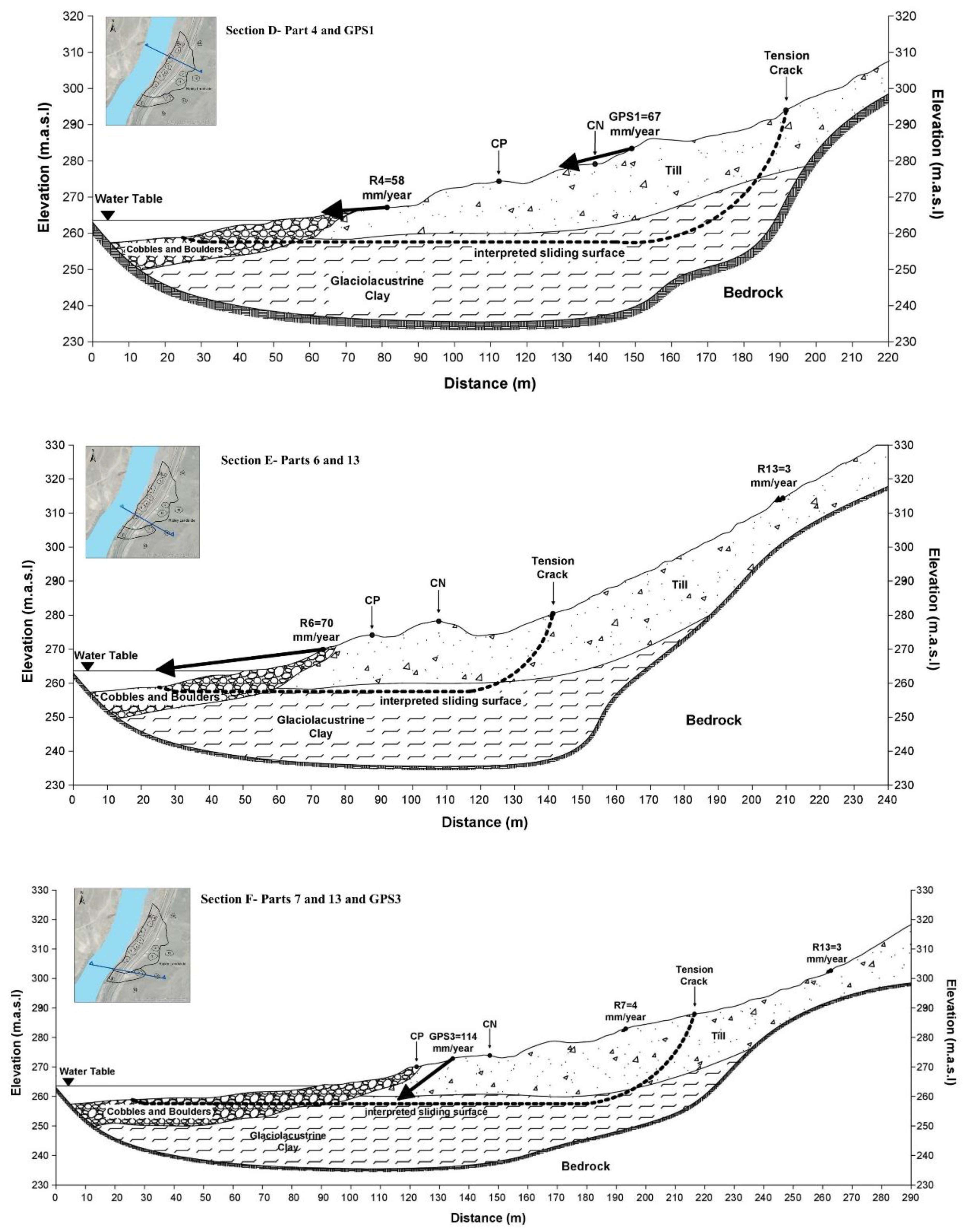
| Area | Recorded Date | Total Magnitude (mm/Year) | Horizontal Component (mm/Year) | Westward Magnitude (mm/Year) | Northward Magnitude (mm/Year) | Downward Magnitude (mm/Year) | Azimuth Angle (Degree) | Angle with Horizontal Plane (Degree) |
|---|---|---|---|---|---|---|---|---|
| R0 | May 2015 to May 2017 | 17 | 17 | 15 | 7 | 2 | 296 | 8 |
| R1 | May 2015 to May 2017 | 54 | 54 | 52 | 16 | 6 | 287 | 6 |
| R2 | May 2015 to May 2017 | 55 | 53 | 51 | 14 | 14 | 285 | 15 |
| R3 | May 2015 to May 2017 | 59 | 58 | 53 | 25 | 4 | 295 | 4 |
| R4 | May 2015 to May 2017 | 58 | 57 | 49 | 30 | 4 | 302 | 4 |
| R5 | May 2015 to May 2017 | 82 | 81 | 65 | 49 | 13 | 307 | 11 |
| R6 | May 2015 to May 2017 | 70 | 69 | 56 | 41 | 7 | 306 | 7 |
| R7 | May 2015 to May 2017 | 4 | 3 | 2 | 1 | 3 | 297 | 37 |
| R8 | May 2015 to May 2017 | 2 | 2 | 1 | 1 | 0 | 313 | 10 |
| R9 | May 2015 to May 2017 | 65 | 64 | 55 | 34 | 11 | 302 | 11 |
| R10 | May 2015 to May 2017 | 22 | 22 | 22 | 5 | 1 | 282 | 4 |
| R11 | May 2015 to May 2017 | 34 | 12 | 11 | 3 | 32 | 284 | 43 |
| R12 | May 2015 to May 2017 | 5 | 2 | 2 | 1 | 4 | 287 | 43 |
| R13 | May 2015 to May 2017 | 3 | 2 | 2 | 1 | 1 | 303 | 24 |
| R14 | May 2015 to May 2017 | 4 | 3 | 3 | 0 | 2 | 277 | 30 |
| R15 | May 2015 to May 2017 | 2 | 1 | 1 | 1 | 1 | 295 | 35 |
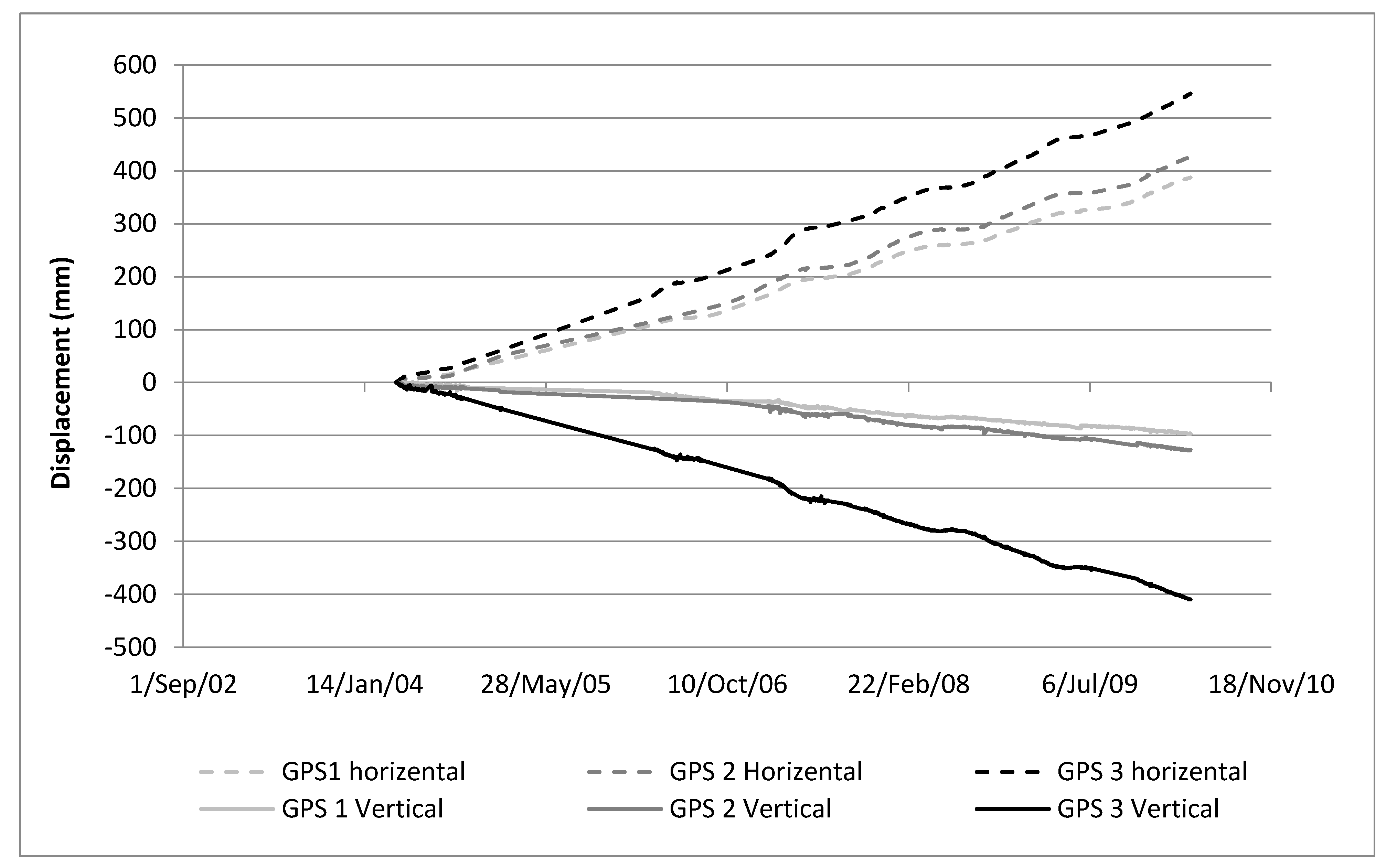
| GPS Name | Recorded Dates | Westward Movement (mm) | Northward Movement (mm) | Downward Movement (mm) | Total Movement (mm) | Horizontal Movement (mm) | Angle with Horizontal Plane (Degree) | Azimuth Angle (Degree) | Average Velocity (mm/year) |
|---|---|---|---|---|---|---|---|---|---|
| GPS1 | 11-Apr-08 to 11-Apr-14 | 355 | 154 | 97 | 399 | 387 | 14 | 293 | 67 |
| GPS2 | 11-Apr-08 to 11-Apr-14 | 377 | 199 | 127 | 445 | 426 | 17 | 298 | 74 |
| GPS3 | 11-Apr-08 to 11-Apr-14 | 443 | 319 | 410 | 683 | 546 | 37 | 306 | 114 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Soltanieh, A.; Macciotta, R. Updated Understanding of the Ripley Landslide Kinematics Using Satellite InSAR. Geosciences 2022, 12, 298. https://doi.org/10.3390/geosciences12080298
Soltanieh A, Macciotta R. Updated Understanding of the Ripley Landslide Kinematics Using Satellite InSAR. Geosciences. 2022; 12(8):298. https://doi.org/10.3390/geosciences12080298
Chicago/Turabian StyleSoltanieh, Amir, and Renato Macciotta. 2022. "Updated Understanding of the Ripley Landslide Kinematics Using Satellite InSAR" Geosciences 12, no. 8: 298. https://doi.org/10.3390/geosciences12080298
APA StyleSoltanieh, A., & Macciotta, R. (2022). Updated Understanding of the Ripley Landslide Kinematics Using Satellite InSAR. Geosciences, 12(8), 298. https://doi.org/10.3390/geosciences12080298







