1. Introduction
There is a widespread belief that it is not space but time that in the end poses the greatest challenge in science. A monograph published in 2011 [
1] reviewed the new view on time, termed natural time
(from the Greek word “
ó
” which means “time”), which was introduced by the authors in 2001 [
2,
3,
4]. In this new view, time is
not continuous, thus being in sharp contrast with the hitherto used conventional time
t which is modeled as the one-dimensional
continuum of the real numbers.
The results deduced until 2011 on the basis of this new domain revealed that novel dynamical features hidden behind time series in complex systems can emerge upon analyzing them in natural time, but cannot do so when the analysis is carried out within the frame of conventional time. It was also shown that the analysis in natural time enables the study of the dynamical evolution of a complex system and identifies when the system enters a critical stage. This reflects that natural time plays a key role in predicting impending catastrophic events in general. In several chapters of this monograph [
1] examples of data analysis in natural time were presented (that have been published mainly in Physical Review and Physical Review Letters) in diverse fields including Earth Sciences (Geophysics, Seismology), Physics (Condensed Matter, Statistical Physics, Physics of Complex Systems), Biology and Cardiology.
In [
1] (Chapter 2), it was demonstrated that natural time is optimal for enhancing the signals in time–frequency space when employing the Wigner function and measuring its localization property. In other words, natural time analysis conforms to the desire to reduce uncertainty and extract signal information as much as possible. The normalized power spectrum
introduced in natural time whose Taylor expansion leads, at low natural (cyclic) frequencies
(
), to the expression
where the coefficient
is just the variance of natural time, i.e.,
. This quantity is useful in identifying the approach to a critical point. In the natural time analysis of the seismicity, a careful inspection reveals (see [
5]; see also Chapter 6 of [
1]) that the quantity
may be considered as an order parameter of seismicity. This allows the determination of the constant
b in the Gutenberg–Richter law for earthquakes (EQs),
, by applying the Maximum Entropy Principle [
6,
7]. It leads to
, which agrees with real seismic data.
In [
1] (Chapter 3, see also [
8,
9]), the entropy
S in natural time was defined and additionally it was shown that the entropy
deduced from the natural time analysis of the time series obtained upon time reversal (i.e., reversing the time arrow) is in general different from
S, thus, the entropy in natural time does satisfy the condition to be
“causal” [
9,
10]. In addition, the physical meaning of the change
of the entropy in natural time under time reversal was discussed, which is of profound importance when studying [
11] the evolution of complex systems. Furthermore, complexity measures were introduced that quantify the fluctuations of the entropy
S and of the quantity
upon changing the length scale as well as the extent to which they are affected when shuffling randomly the consecutive events.
Since the publication of the aforementioned monograph [
1], however, i.e., in the last decade, a lot of work on the natural time analysis of seismicity has been published which has shed light on the crucial importance of the order parameter of seismicity (
) and on the entropy change
under time reversal, along with their fluctuations, in the identification of important changes before major EQs. It is the main aim of this work to review these precursory phenomena which may be of broad interest in the geosciences.
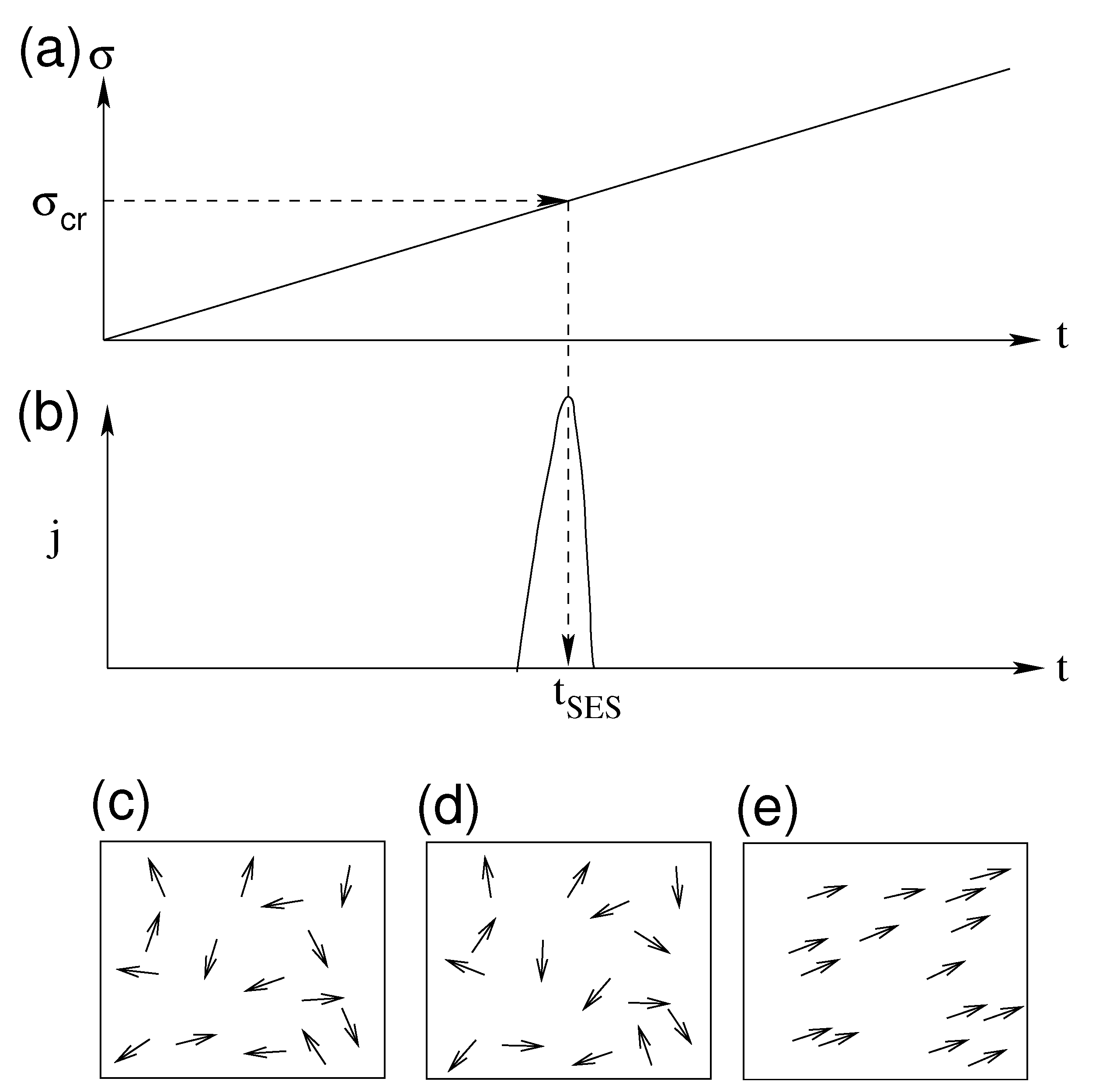
One of these phenomena is the finding that the minimum of the fluctuations of the order parameter of seismicity appears almost simultaneously with the initiation of Seismic Electric Signals (SES) activity, which is a series of transient low-frequency Earth electric field changes (whose main properties were recapitulated in the monograph [
1] in 2011) preceding major EQs (see also
Section 4). This simultaneous appearance, which was experimentally confirmed and reported in 2013, is of profound importance, because the former quantity (fluctuations of the order parameter of seismicity) comes from seismic data alone, while the second one (SES activity) solely from electric field measurements. The correlation of these two types of data has been suggested on the basis of a physical model from the 1980s in which it is underlined that SES is a critical phenomenon (cf. major EQs are also considered as critical phenomena, where a mainshock is the new phase).
In view of the above, the present feature article is structured as follows: In
Section 2, we summarize the latter physical model on the SES generation, based on the thermodynamics of point defects, a strict foundation of which was completed in the beginning of the 1980s [
12]. In the following two main parts, the precursory changes of the two quantities
and
of seismicity—along with their fluctuations—are described in the
Section 3,
Section 4,
Section 5,
Section 6,
Section 7 and
Section 8 and
Section 9,
Section 10 and
Section 11, respectively. Finally, a discussion is presented in
Section 12 and our conclusions are given in the last section.
Note that the new method for estimating the seismic risk, termed nowcasting, by Turcotte, Rundle and coworkers [
13,
14,
15,
16,
17,
18,
19], is also based on the concept of natural time.
3. Minimum of the Order Parameter Fluctuations of Seismicity before Major Earthquakes
For a time series comprising of
N EQs, we define the natural time for the occurrence of the
k-th EQ
and
is the normalized energy of the
k-th EQ of energy
calculated from seismic catalogs. The quantity
serves, as mentioned in the Introduction, as an order parameter [
1,
5,
34,
35]. We recall that
To obtain the fluctuation
of
, we need many values of
for each target EQ. For this purpose, we first take an excerpt comprising
W successive EQs just before a target EQ from the seismic catalog. The number
W is chosen to cover a period of a few months. Thus,
W is the number of EQs that on average occur during the average lead time of an SES activity that ranges from a few weeks to
months (see Chapter 7 of Ref. [
1]). For this excerpt, we form its sub-excerpts
of consecutive
N = 6 EQs (since at least six EQs are needed [
5] for obtaining reliable
) of energy
and natural time
each. Further,
, and by sliding
over the excerpt of
W EQs,
, we calculate
using Equation (
1) for each
j. We repeat this calculation for
, thus obtaining an ensemble of
values. Then, we compute the average
and the standard deviation
of thus obtained ensemble of
values. The variability
of
for this excerpt
W is defined to be:
and is assigned to the
-th EQ in the catalog, i.e., the target EQ.
The time evolution of the value can be pursued by sliding the excerpt through the EQ catalog. Namely, through the same process as above, values assigned to , EQs in the catalog can be obtained. Note that may bear a subscript to clarify the window length W used for its calculation.
Since 2013 (see [
36,
37]) the calculations have been made by taking all natural time windows comprising 6 to
W events following the procedure described above. On the other hand, before 2013 (as for example is the case in [
38]), the calculations were made as follows: First, for an event considering natural time windows from 6 to 40 events (i.e., selecting the precise value 40 as an upper limit). Second, this process was performed for all the events sliding through the whole data set (e.g., an EQ catalog).
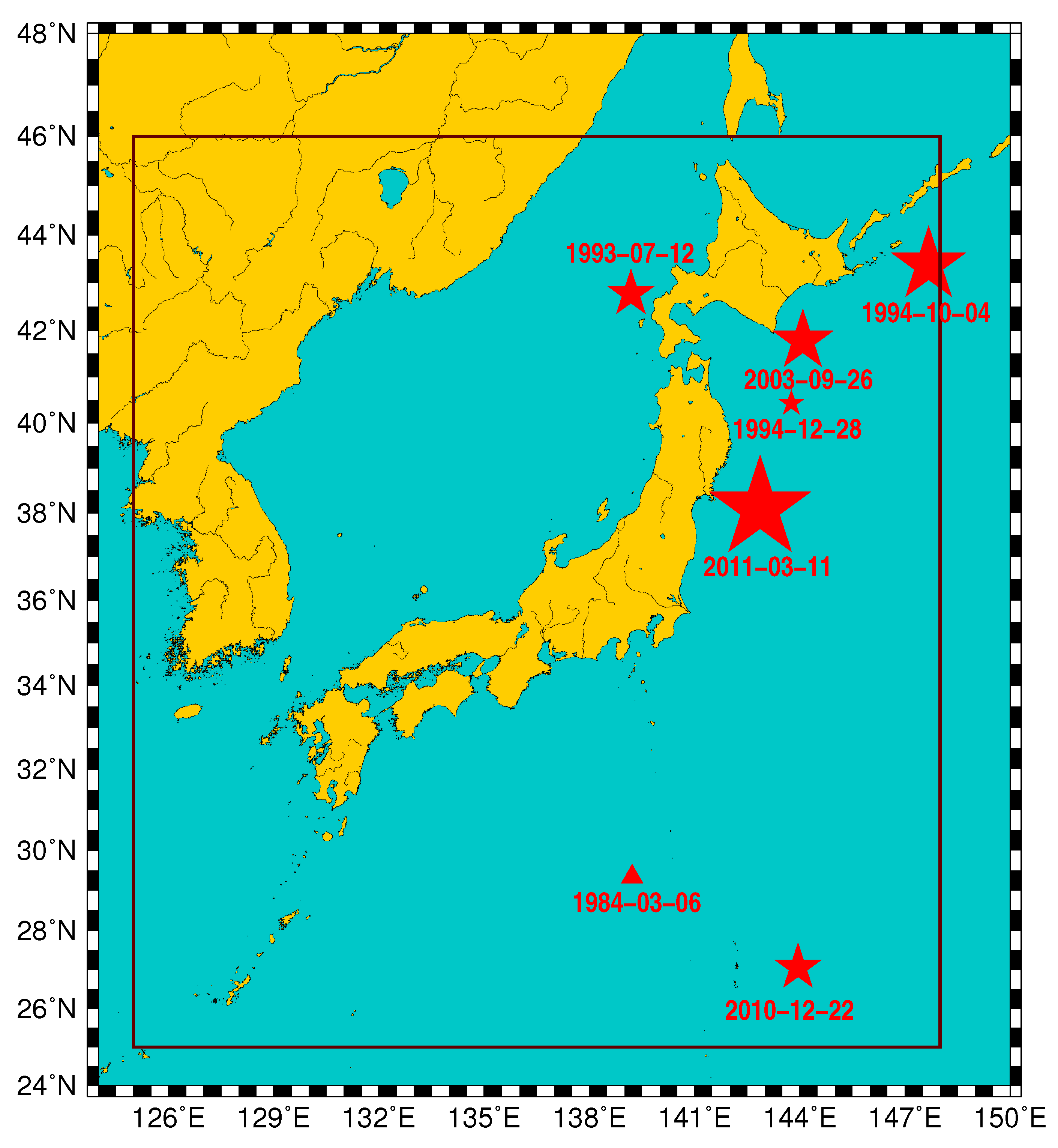
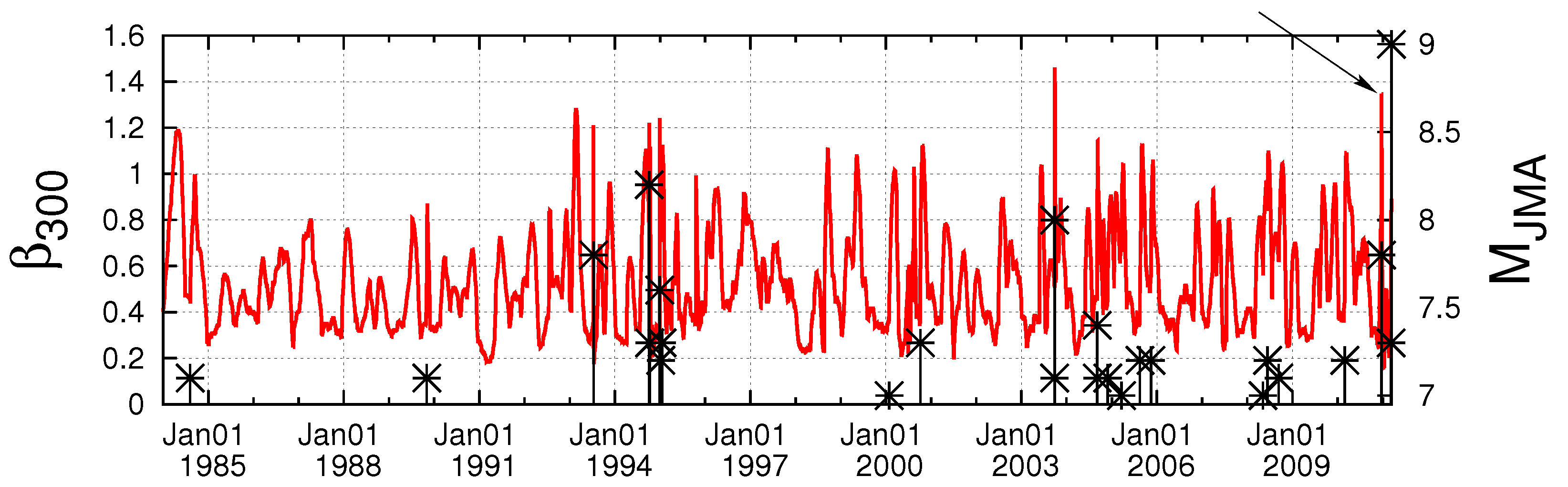
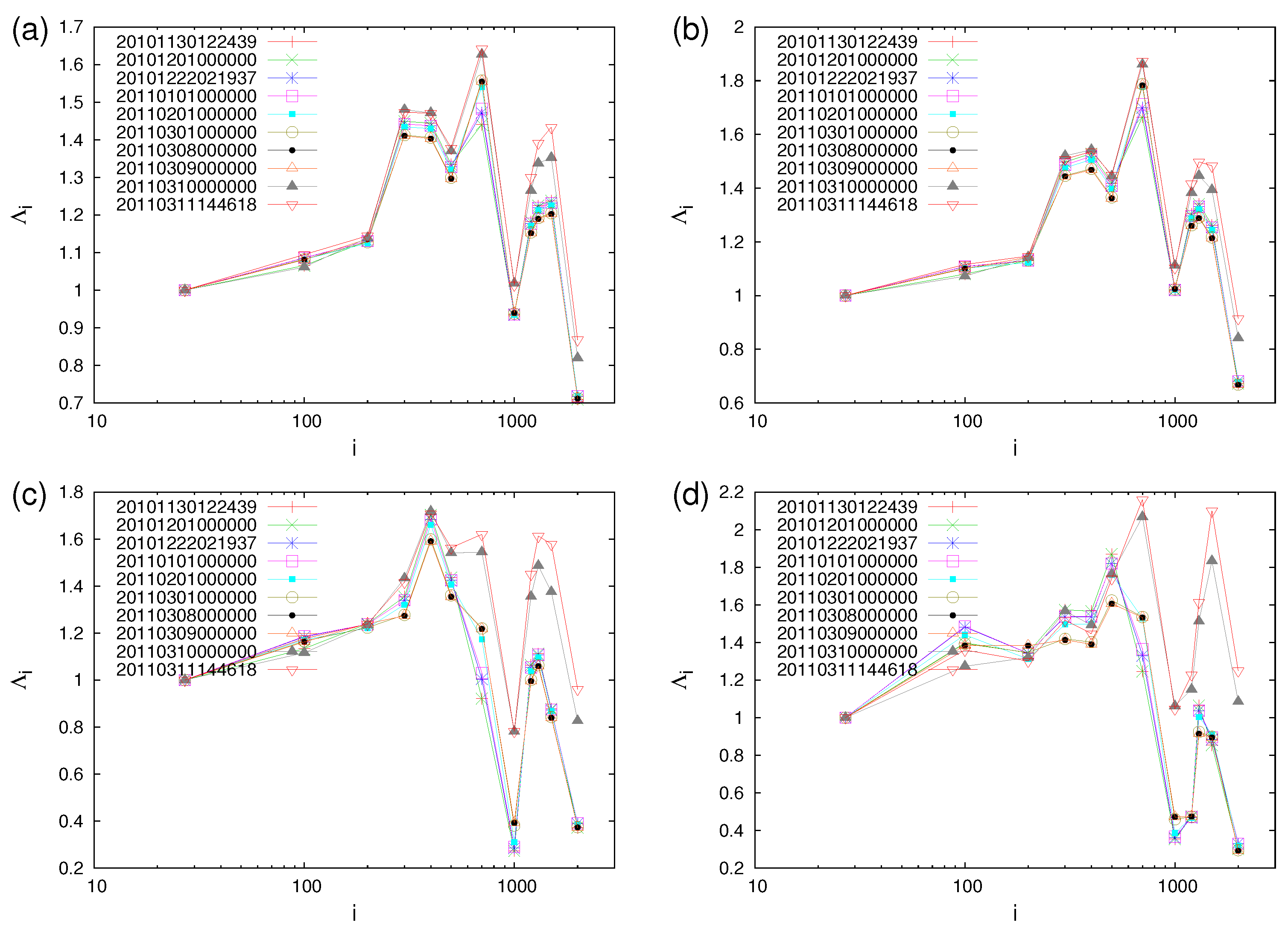
In [
36], the Japan seismic catalog was analyzed in natural time from 1 January 1984 until the occurrence time of the
M9 Tohoku EQ on 11 March 2011 considering a natural time window of fixed length comprising the number of events that would occur in a few months. It was found that the fluctuations of the order parameter of seismicity, computed by the procedure described above, exhibit minima a few months before all shallow EQs of magnitude 7.6 or greater during this 27-year period in the Japanese area (
Figure 3). Among the minima, the one before the
M9 Tohoku EQ was the deepest. In this analysis, the Japan Meteorological Agency (
JMA) seismic catalog was used by considering all EQs in the period from 1984 to the occurrence time of the
M9 Tohoku EQ, within the area
(
Figure 3). The energy of EQs was deduced from
after converting [
39] to the moment magnitude
[
40]. Considering a threshold
= 3.5 for the sake of data completeness, we are left with 47,204 EQs for the period under study, which is around 326 months. Thus, on average,
EQs occur per month. We considered the values
W = 200, 300 and 400, which occur during a period of a few months, which is comparable with the average lead time of SES activities recorded in Japan [
41] and Greece [
26,
30,
42].
We first focus on the minimum of the variability
observed before the
M9 Tohoku EQ.
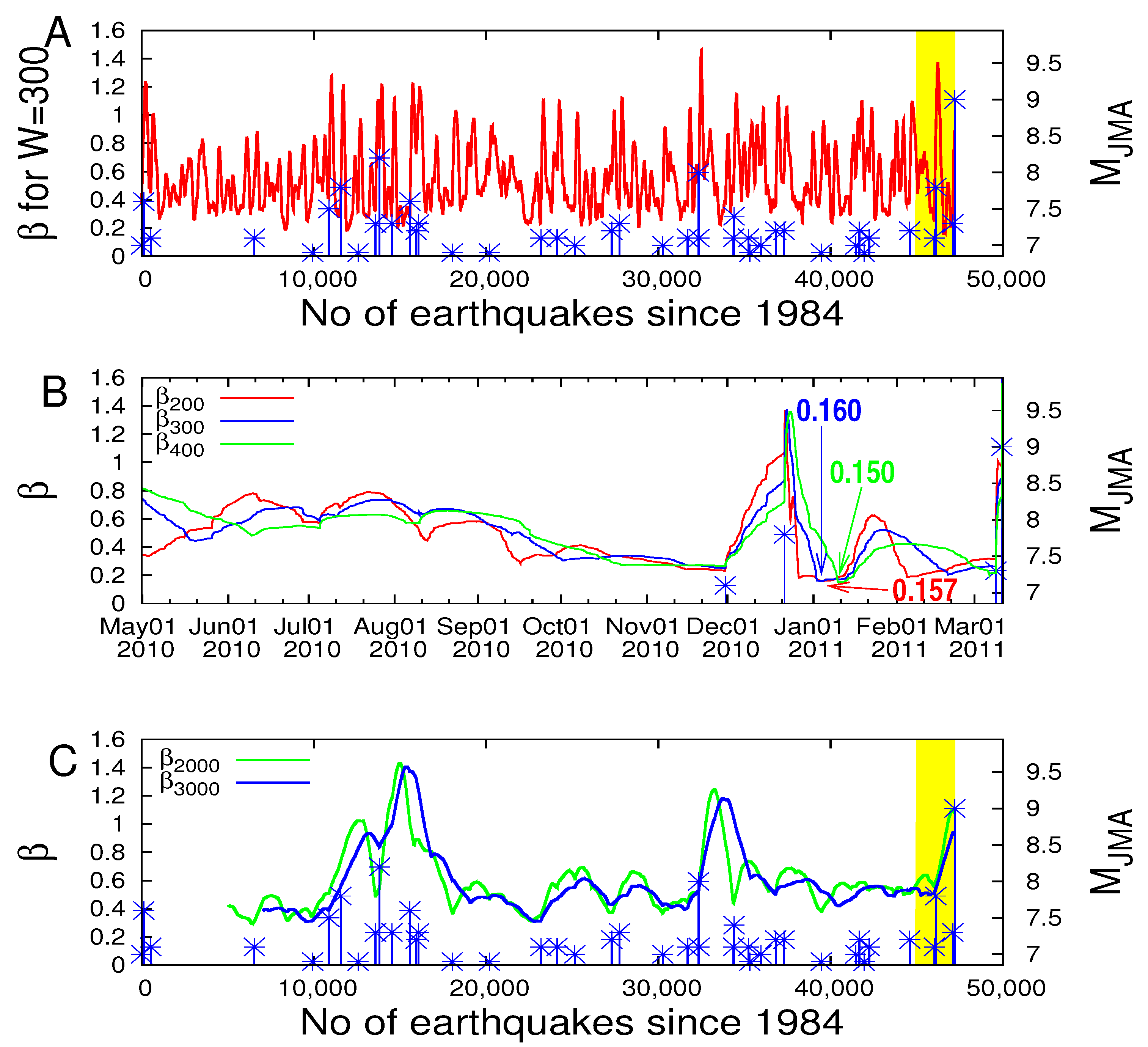
Figure 4 depicts about 47,200
-values calculated for
W = 300 versus the target EQ number from 1984 to the day of the Tohoku EQ, 11 March 2011. EQs with
(
in the right scale) are indicated by blue asterisks. The
-values show the deepest minimum (min) just before the occurrence of the Tohoku EQ (rightmost of
Figure 4A).
Figure 4B is an expanded version, in the conventional time, of the last 10-month period shown in yellow in
Figure 4A. The red, blue and green curves depict what happened to
for
W = 200, 300 and 400. Hereafter, we use the symbols
and
as needed. After around 1 September 2010, a decrease of
became evident and
fell to a minimum in early January 2011, about 2 months before the Tohoku EQ. The results of this minimum of
are summarized as follows (
Figure 4A,B): First, such a minimum of
was not observed again during the 27-year period studied. Second, the ratio
is near unity. Third, the dates of
for
W = 200, 300 and 400 are 5 January, 5 January and 10 January 2011, respectively, i.e., almost the same. Fourth, this minimum is less clear for greater
W corresponding to time intervals longer than a few months. It is almost invisible for
W = 2000 and 3000 (
Figure 4C). The same applies to all other
, as seen in
Figure 4C. In what follows, we restrict ourselves to
W = 200 and
W = 300.
We now proceed to the
minima before other major EQs in Japan during the 27-year period investigated. Six shallow EQs with
7.6 or larger (
Figure 3) occurred:
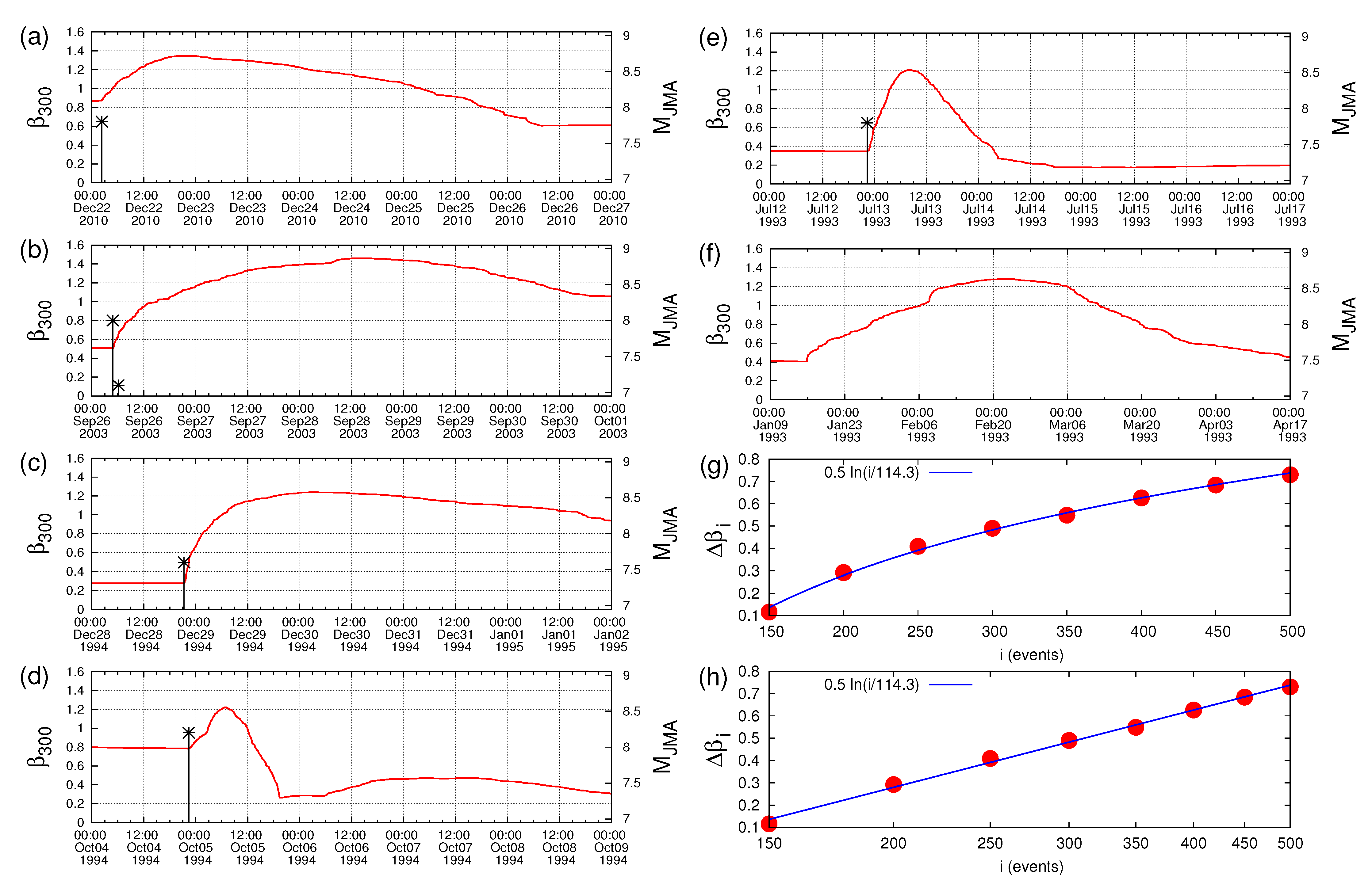
EQa 1993-07-12: 1993 Southwest-Off Hokkaido EQ ( = 7.8);
EQb 1994-10-04: 1994 East-Off Hokkaido EQ ( = 8.2);
EQc 1994-12-28: 1994 Far-Off Sanriku EQ ( = 7.6);
EQd 2003-09-26: 2003 Off Tokachi EQ ( = 8.0);
EQe 2010-12-22: 2010 Near Chichi-jima EQ ( = 7.8);
EQf 2011-03-11: 2011 Tohoku EQ ( = 9.0).
It was examined whether
minimum precede these EQs as well.
Figure 5A–C are the expanded versions in the conventional time of
Figure 4A in three 10-year periods. The EQs are marked by a–f. In view of the fact that these figures are still small, the time axis was expanded for each EQ, as shown in
Figure 6A–E, just as in
Figure 4B for the Tohoku EQ. We now see
minima within 1–3 months before every one of the six mainshocks as shown in Table 1 of [
36]. In this table, the
ratio and
of
minima before each of these EQs are very similar to those before the Tohoku EQ, e.g., the ratios lie in the range 0.95–1.08. It was then concluded that these minima are precursory to the time-correlated EQs. Beyond these
minima before the six
EQs, there were many more minima during the 27-year period, as seen in
Figure 5. We examined whether they were also followed by EQs. Along these lines, the minima were chosen deeper than the shallowest one of the six
in Table 1 of [
36], which happened before EQb, the 1994 East-Off Hokkaido EQ (
= 8.2), yielding 0.295 for
. We found that, out of the thus chosen 31 minima, 9 (labeled 1–9 in
Figure 5) also displayed
ratio (Figure 5, Table 2 of [
36]) in the range 0.95–1.08, thus almost equal to those observed before the six
EQs (Table 1 of Ref. [
36]). These nine minima have been followed by
EQs within 3 months (Figure 5 and
in Table 2 of Ref. [
36]). Such correspondences are naturally less certain, because of a larger number of EQs. In particular, there were 139
EQs during the 27-year period studied.
In summary, we conclude that analysing in natural time the seismicity of Japan from 1 January 1984 to the occurrence time of
M9 Tohoku EQ on 11 March 2011, using a natural time window comprising the number of events that would occur in a few months (this is called hereafter
crucial scale for the reasons discussed in Ref. [
43]), the following results were obtained.
Almost 2 months before the M9 Tohoku EQ, a minimum in the variability of the order parameter of seismicity is observed which is the deepest in the whole study period. Distinct minima of , but of shallower depth, were found also one month to a few months before the occurrence of all other Japanese major EQs (, depth <400 km) during 1984–2011. The minima, which seem to be precursory to sizeable EQs show the ratio in the range of 0.95–1.08.
The approximate coincidence of the minima of
with the initiation of the SES activities identified by Varotsos et al. [
38]—which will be discussed in
Section 4—strengthens the physics of both phenomena.
3.1. Statistical Significance of the Minima of the Order Parameter Fluctuations
In summary, the results obtained by Sarlis et al. [
36] are as follows: Upon analyzing the
JMA seismic catalog [
36] in natural time and considering all EQs of magnitude
from 1 January 1984 to 11 March 2011 (the time of the
M9 Tohoku EQ) within the area
, fifteen distinct minima—observed simultaneously (see, e.g., Appendix A in [
44]) at
and
with a ratio
in the range 0.95 to 1.08 and
—of the fluctuations of the order parameter of seismicity were found 1 to around 3 months before large EQs. All shallow EQs of magnitude 7.6 or larger during this 27-year period were preceded by six (out of 15) of these minima
. Remarkably, among the minima, the deepest minimum was observed around 5 January 2011, i.e., around two months before the
M9 Tohoku EQ.
In 2016, Sarlis et al. [
45] showed, using Monte Carlo calculations, that the probability to achieve the above results by chance is of the order of
. This conclusion was also strengthened in 2020 by Christopoulos et al. [
46], using the event coincidence analysis (ECA) [
47]. In addition, in 2020 Sarlis et al. [
48] found the same value (
) by means of the receiver operating characteristics (ROC) technique by focusing on the area under the ROC curve (AUC). They explained that, if this area, which is currently considered an effective way to summarize the overall diagnostic accuracy of a test, has the value 1, it corresponds to a perfectly accurate test. It was found [
48] that the AUC in these results is around 0.95, which is evaluated as outstanding. This result indicates that the study of the minima
of seismicity for an area covered by a well-developed seismological network such as Japan may be used for operational EQ forecasting.
3.2. Minimum of the Order Parameter of Seismicity Preceding the Ultra-Deep 2015 EQ () in Japan
On 30 May 2015, a powerful EQ (
7.9,
JMA reported magnitude
) struck west of Japan’s remote Ogasawara (Bonin) island chain, which lies more than 800 km south of Tokyo. It occurred at 680 km depth in an area without any known historical seismicity and caused significant shaking over a broad area of Japan at epicentral distances in the range 1000–2000 km. Specifically, the maximum shaking intensity was reached at both Hahajima Island (above the hypocenter) and Kanagawa (near Tokyo) located over 800 km from the hypocenter. It was the first EQ felt in every Japanese prefecture since intensity observations began in 1884. By applying natural time analysis, Varotsos et al. [
49] found that almost three and a half months before this powerful EQ, which is the strongest one after the
M9 Tohoku EQ on 11 March 2011, the fluctuations of the order parameter of seismicity were minimized around 17 February 2015. We have to mention that no SES has been reported for this undersea ultra-deep EQ.
6. Identification of the Occurrence Time of a Mainshock by Means of the Minimum of the Order Parameter Fluctuations
In the time series analysis using natural time, the behavior of the normalized power spectrum [
2,
3,
4]
defined by
where
stands for the angular frequency at
close to zero, is studied for capturing the dynamic evolution, because all the moments of the distribution of the
can be estimated from
at
(see p. 499 of [
52]). For this purpose, a quantity
is defined from the Taylor expansion
. The relation for the critical state that has been shown for SES activities [
1,
2]:
for
, simplifies to
which shows that the second-order Taylor expansion coefficient of
, i.e.,
, is equal to 0.070. Equation (
6) has been shown to hold also for EQ models like the Burridge–Knopoff EQ model [
53,
54] as well as in the Olami Feder Christensen (OFC) EQ model [
1,
55] when they approach the critical point.
In general, two procedures have been suggested in order to determine the occurrence time of an impending mainshock: A procedure, which solely for the sake of convenience has been called preliminary procedure, was followed for several cases (e.g., see References [
2,
5,
7,
33,
56,
57]) by starting the natural time analysis of seismicity in the candidate area
A immediately after the SES initiation, because the latter signals that the system enters the critical stage and the time variation of parameters should be traced only on the single candidate area
A. To identify, however, such a single area, one should know the properties of the preceding SES activity in general, or, in particular, the selectivity map [
30,
58] of the measuring station that recorded the SES activity. An alternative procedure, called “updated” procedure, was later developed [
42], that considers the natural time analysis of the seismicity in
all the possible subareas of
A, instead of a single area identified by the SES selectivity map. This has been inspired as follows [
42]: When area A reaches criticality, one expects in general that all its subareas have also reached criticality simultaneously. At that time, therefore, the evolution of seismicity in each of these subareas is expected to result in a
value close to 0.070. Assuming equipartition of probability among the subareas, the distribution Prob(
) of the
values of all subareas should peak at around 0.070, exhibiting also magnitude threshold invariance (for more details on how the subareas are selected, see page 300 of [
1]). This usually enables the estimation of the occurrence time of major EQs with a time window of less than a week or so.
When geoelectrical measurements are not available, we cannot identify directly the initiation time of an SES activity. Since, however, this initiation occurs almost at the time of the minimum of the fluctuations of the order parameter of seismicity in a large area, we take advantage of the occurrence time of this minimum. In addition, a spatiotemporal study of this minimum described in the preceding section enables an estimation of the epicentral area of the impending major EQ [
37]. In particular, we will present below the case of the identification of the occurrence time of the impending
M9 Tohoku mega-earthquake that occurred on 11 March 2011 in Japan.
We start the natural time analysis of seismicity at the date of 5 January 2011, at which the fluctuations of the order parameter of seismicity exhibited [
36] the deepest minimum ever observed during the period 1984–2011 in Japan. This date should almost coincide with the initiation of an SES activity [
38], as explained in
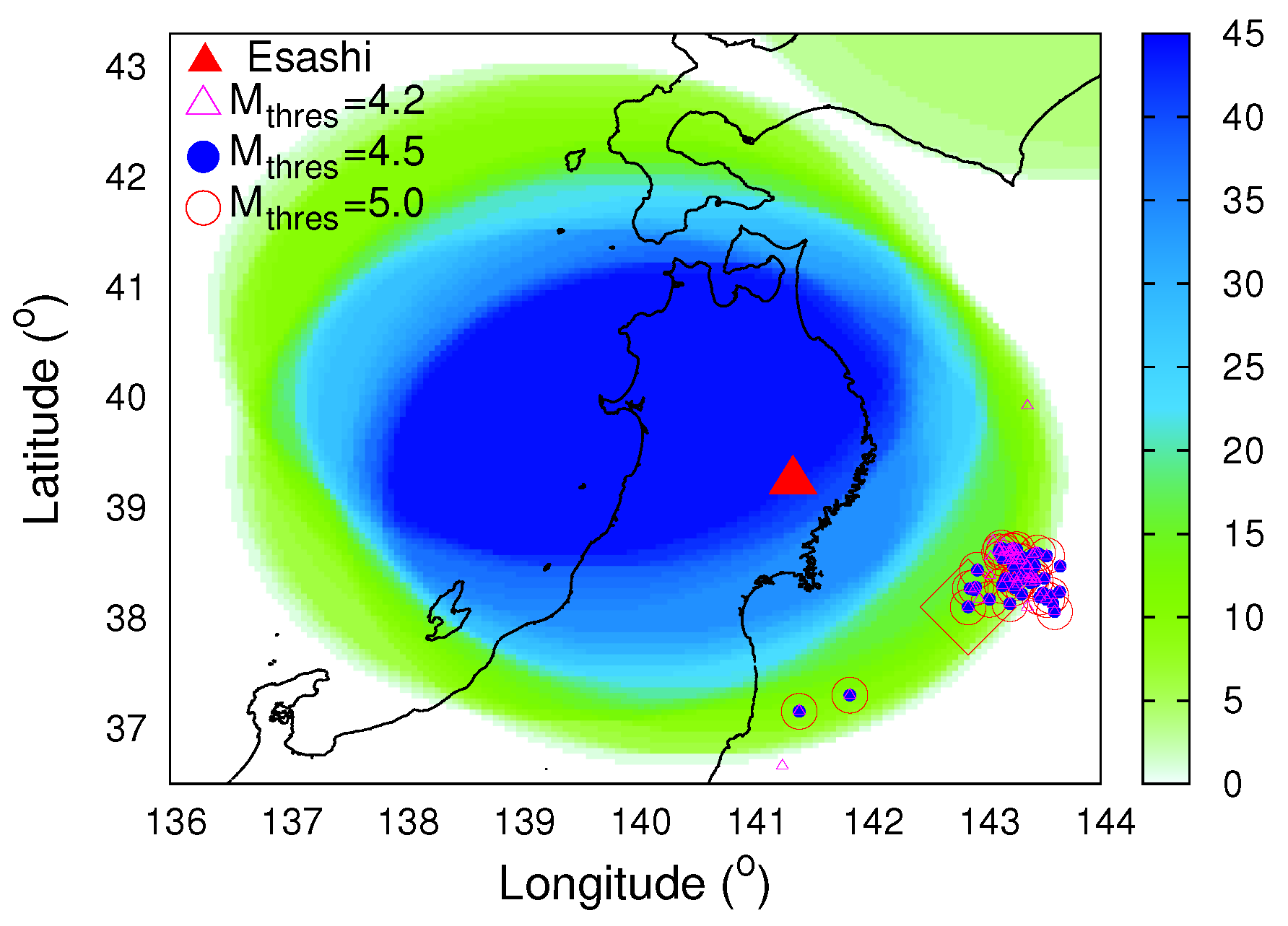
Section 4. Actually, anomalous magnetic field variations appeared during the period of 4 to 14 January 2011 on the z component at two measuring sites (Esashi and Mizusawa) lying at epicentral distances of around 130 km [
59,
60,
61,
62] (which reflect also the existence of an SES activity, see, e.g., [
32,
33]).
Since the SES selectivity maps of the aforementioned measuring stations are lacking, we proceed to the estimation of the epicentral location of the impending mainshock without making use of SES data, and follow the procedure described in
Section 5 [
37]: Dividing the entire Japanese region
(large area) into small areas, a calculation of the fluctuations of
of seismicity is carried out on them. Some small areas show a minimum of the fluctuations almost simultaneously with the minimum in the entire Japanese region (on 5 January 2011) and such small areas cluster within a few hundred km from the actual epicenter. Such a calculation results in an estimate of the candidate epicentral area shown by the blue-green area in
Figure 7.
Starting from 5 January 2011, we now compute the
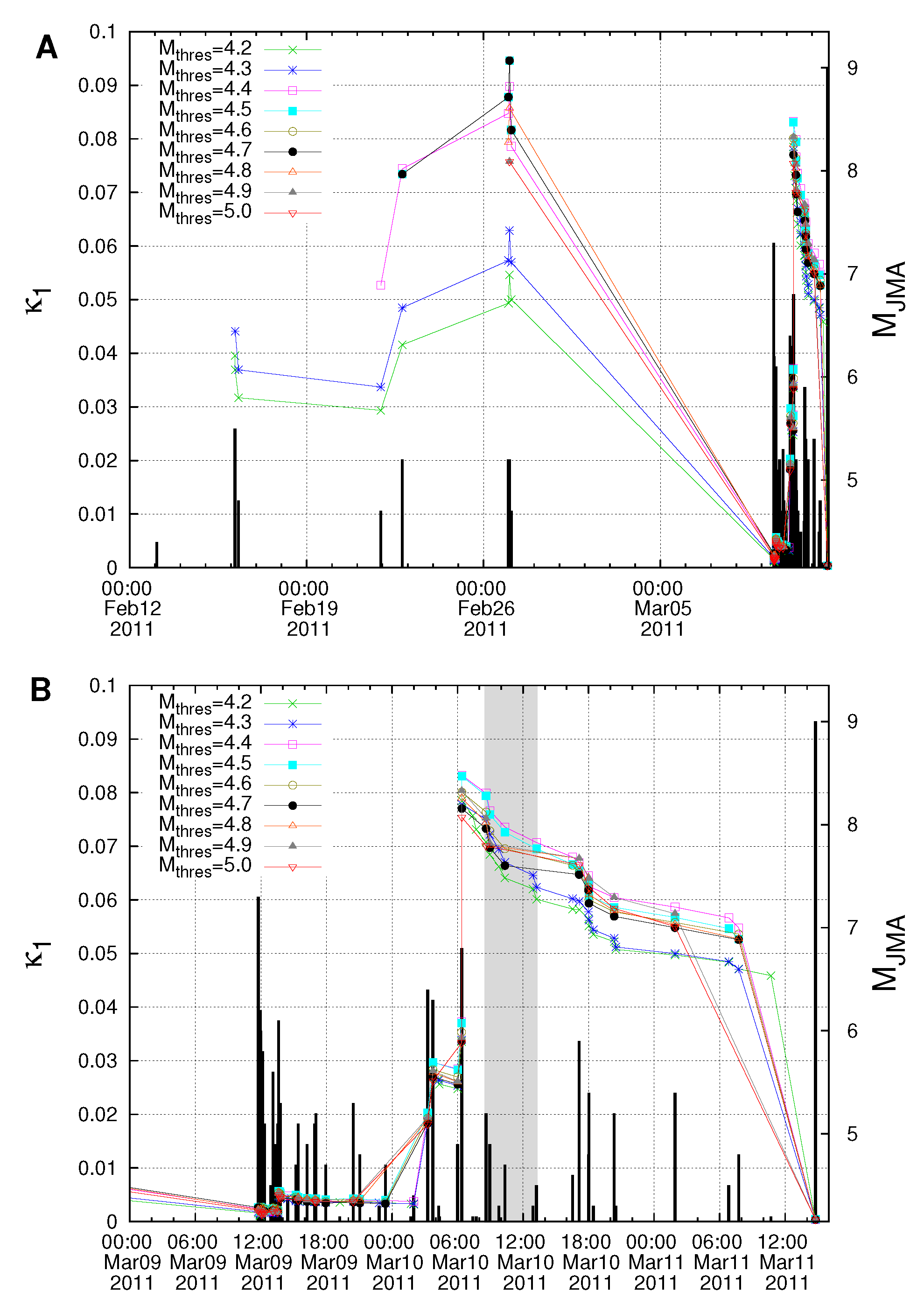
values of seismicity in the blue-green area and the results are depicted in
Figure 8 for
= 4.2 to 5.0. Considering that at least six EQs are needed [
5] for obtaining a reliable
value, which happens in the blue-green area on 16 February 2011 for
=4.2, we show the computed
values during the last four weeks before the
M9 Tohoku EQ in
Figure 8A. This figure reveals that the condition
is not satisfied for all magnitude thresholds at least until the
M7.3 EQ on 9 March 2011. Since the results afterwards cannot be seen clearly in
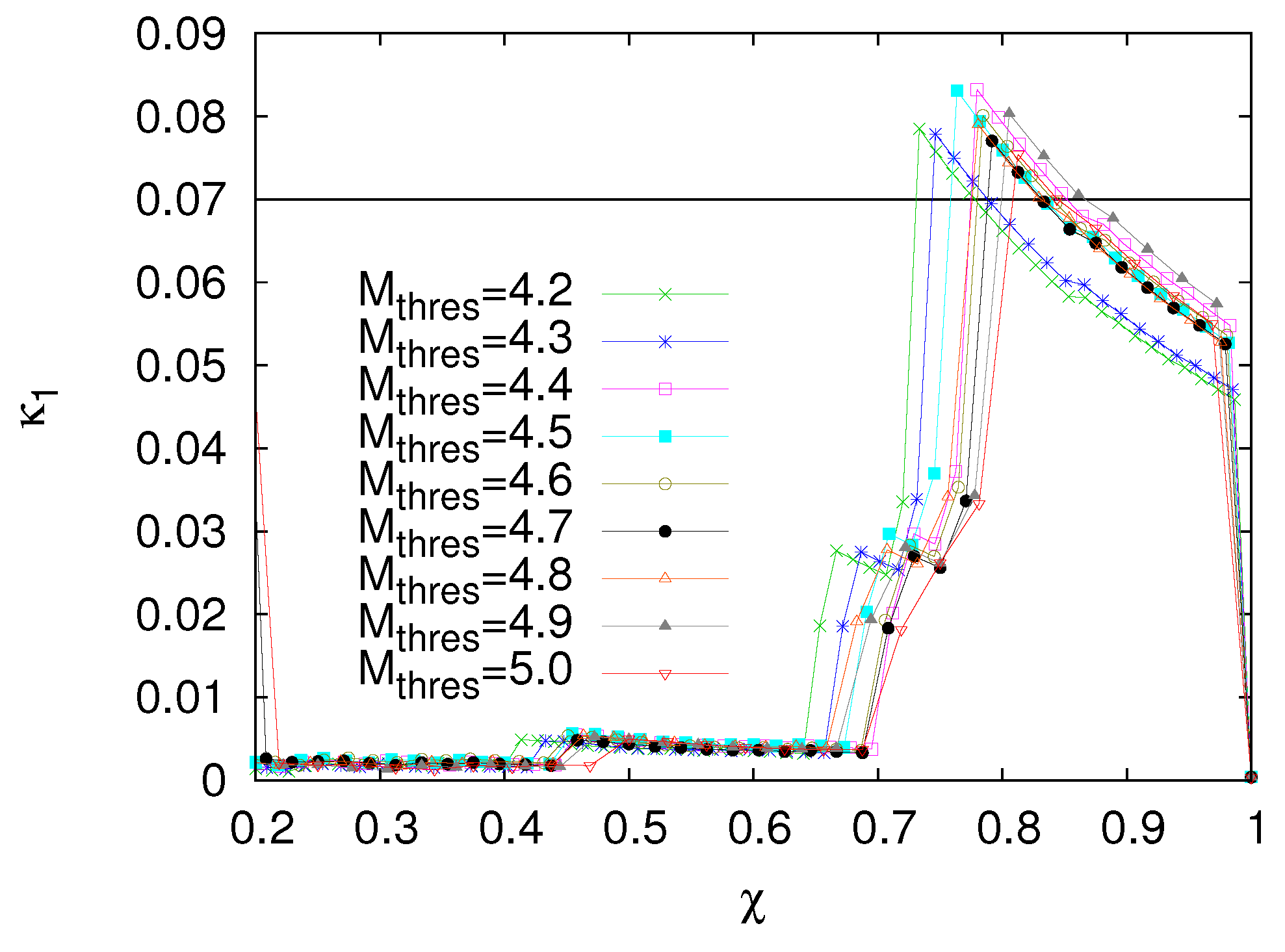
Figure 8A, we plot in expanded time scale in
Figure 8B (see also
Figure 9) the
values of seismicity from 00:00 LT on 9 March 2011 until the Tohoku EQ occurrence. This figure clearly shows that the condition
(which signals that the mainshock is going to occur within the next few days or so) is fulfilled for
= 4.2 to 5.0 in the morning of 10 March 2011 upon the occurrence of the EQs from 08:36 to 13:14 LT, i.e., almost one day before the Tohoku EQ (see the gray shaded area in
Figure 8B), thus, the
M7.3 EQ on 9 March 2011 was a foreshock.
We now return to the same example, but using the preliminary procedure according to which the criteria to assure a true coincidence of the EQ time series with that of critical state are [
1]:
The “average” distance
between the curves of
of the evolving seismicity and Equation (
5) for
should be
, for details, see [
1,
2];
The final approach of the evolving
to that of Equation (
5) must be from below. This reflects that
gradually decreases with time before strong EQs finally approaching from above that of the critical state, i.e.,
, see Figure 7.1 on page 293 of [
1];
At the coincidence, both entropies S and must be smaller than ;
Since this process (critical dynamics) is considered to be self-similar, the occurrence time of the true coincidence should not markedly vary upon changing the magnitude threshold .
In light of new advances (see
Section 9), it was shown (see, e.g., [
63]) that the second criterion for the
true coincidence starts to be fulfilled on the date at which (cf. of the seismicity in the candidate epicentral area) minimizes. In other words, the quantity
after the
minimization starts monotonically decreasing from values
and approaches finally from above the value
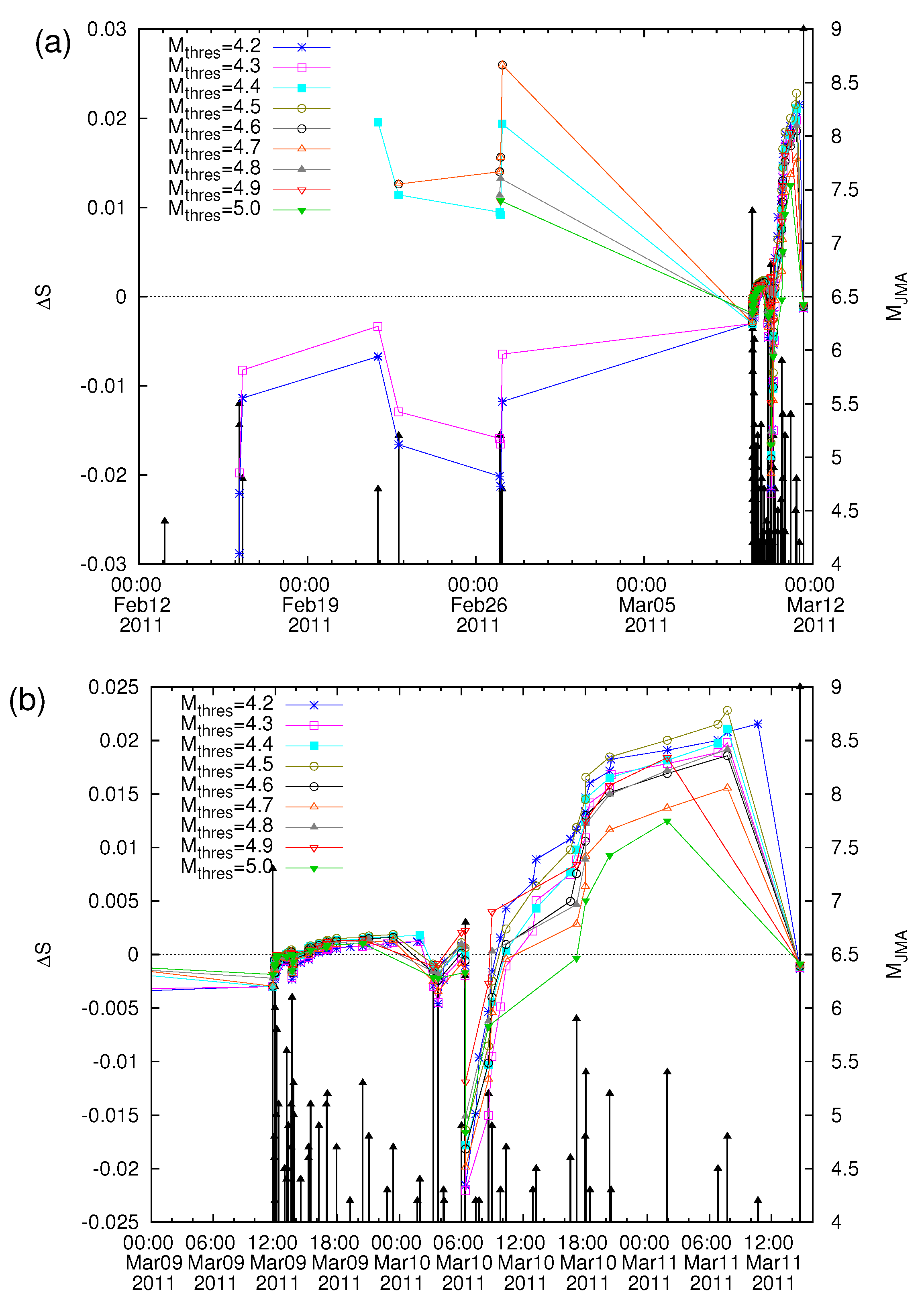
very close to the impending strong EQ occurrence date. Specifically, in
Figure 10, we show the evolution of the
values of seismicity (left scale) in the blue-green area of
Figure 7 almost a month (a) and two-and-a-half days (b) before the
M9 Tohoku EQ on 11 March 2011, i.e., the same periods as those depicted in
Figure 8 for the evolution of the
value. An inspection of this figure shows that the fulfillment of the second criterion for the true coincidence of the EQ time series with that of critical state begins around 06:24 LT on 10 March 2011, thus agreeing with the time of the minimum of
shown in
Figure 10. In other words, it is of major importance that after the appearance of the
minimum of seismicity, the order parameter begins to gradually diminish, thus approaching the critical value
, which signals that a major EQ is going to shortly occur in the blue-green area of
Figure 7.
Figure 7.
Estimating the
M9 Tohoku EQ epicenter: Color contours of the number of the small areas exhibiting the minimum of the fluctuations of
almost simultaneous with that in the entire Japanese region. The red diamond depicts the
M9 Tohoku EQ epicenter and the red triangle the location of the Esashi measuring magnetic station (the other measuring station, Mizusawa, lies only a few tens of km away from Esashi). The epicenters of the EQs that occurred within the candidate epicentral area (blue-green) after 5 January 2011 and before the
M9 Tohoku EQ for various magnitude thresholds
are also shown. Taken from Ref. [
64].
Figure 7.
Estimating the
M9 Tohoku EQ epicenter: Color contours of the number of the small areas exhibiting the minimum of the fluctuations of
almost simultaneous with that in the entire Japanese region. The red diamond depicts the
M9 Tohoku EQ epicenter and the red triangle the location of the Esashi measuring magnetic station (the other measuring station, Mizusawa, lies only a few tens of km away from Esashi). The epicenters of the EQs that occurred within the candidate epicentral area (blue-green) after 5 January 2011 and before the
M9 Tohoku EQ for various magnitude thresholds
are also shown. Taken from Ref. [
64].
Figure 8.
Determination of the occurrence time of
M9 Tohoku EQ. The evolution of the
values of seismicity (left scale) in the blue-green area of
Figure 7 along with the EQs (right scale) shown with thick black vertical bars are plotted against the conventional time: (
A) From 12 February 2011 until the
M9 Tohoku EQ occurrence on 11 March 2011. (
B) From 00:00 LT on 9 March 2011 until the Tohoku EQ occurrence. The shaded area shows the period in the morning of 10 March 2011 during which the condition
is fulfilled (see the text). Taken from Ref. [
64].
Figure 8.
Determination of the occurrence time of
M9 Tohoku EQ. The evolution of the
values of seismicity (left scale) in the blue-green area of
Figure 7 along with the EQs (right scale) shown with thick black vertical bars are plotted against the conventional time: (
A) From 12 February 2011 until the
M9 Tohoku EQ occurrence on 11 March 2011. (
B) From 00:00 LT on 9 March 2011 until the Tohoku EQ occurrence. The shaded area shows the period in the morning of 10 March 2011 during which the condition
is fulfilled (see the text). Taken from Ref. [
64].
Figure 9.
The evolution of the
values of seismicity in a similar fashion as in
Figure 8B, but plotted versus natural time
(
corresponds to the occurrence of the
M9 Tohoku EQ, while
0.2 to the
M7.3 foreshock on 9 March 2011). Taken from Ref. [
64].
Figure 9.
The evolution of the
values of seismicity in a similar fashion as in
Figure 8B, but plotted versus natural time
(
corresponds to the occurrence of the
M9 Tohoku EQ, while
0.2 to the
M7.3 foreshock on 9 March 2011). Taken from Ref. [
64].
Figure 10.
The evolution of the
values of seismicity (left scale) in the blue-green area of
Figure 7 almost a month (
a) and two-and-a-half days (
b) before the
M9 Tohoku EQ on 11 March 2011, i.e., the same periods as those depicted in
Figure 8 for the evolution of the
value. Concerning the importance of the appearance of the minimum of
, which is evident in (
b), see the fulfillment of the criteria for the approach to criticality discussed in
Section 6.
Figure 10.
The evolution of the
values of seismicity (left scale) in the blue-green area of
Figure 7 almost a month (
a) and two-and-a-half days (
b) before the
M9 Tohoku EQ on 11 March 2011, i.e., the same periods as those depicted in
Figure 8 for the evolution of the
value. Concerning the importance of the appearance of the minimum of
, which is evident in (
b), see the fulfillment of the criteria for the approach to criticality discussed in
Section 6.
In summary, the natural time analysis of seismicity results in the conclusion that the values converge to 0.070 almost one day before the Tohoku EQ occurrence, i.e., the system approaches the critical point almost one day before the mainshock.
Alternatively, one may say that natural time analysis allowed for the recognition that the approach to the critical point happened almost a day after the occurrence of the M7.3 EQ of 9 March 2011, thus recognizing that this M7.3 EQ was a foreshock.
The identification of the occurrence time of a mainshock by using the minimum of the fluctuations of the order parameter of seismicity—instead of the initiation of an SES activity—was also achieved for the
M8.2 EQ on 7 September 2017 [
65], which was the strongest EQ in Mexico for more than a century, the deadly
M7.1 EQ on 19 September 2017 in Mexico [
63], and for the Ridgecrest
M7.1 EQ on 6 July 2019 in California [
66]. At this point, we have to mention that in the latter case [
66], a natural time method introduced [
67,
68] for the estimation of the occurrence time of strong aftershocks could also provide an estimate of the occurrence time of the Ridgecrest
M7.1 EQ.
7. Temporal Correlations in the Magnitude Time Series and the Minimum of the Order Parameter Fluctuations of Seismicity
Focusing on the minima of the order parameter fluctuations of seismicity preceding all EQs of magnitude 8 (and 9) class that occurred in a Japanese area from 1 January 1984 to the
M9 Tohoku EQ occurrence on 11 March 2011 and applying Detrended Fluctuation Analysis (DFA) to the EQ magnitude time series, it was found [
44] that each of these minima is preceded as well as followed by characteristic changes of temporal correlations between EQ magnitudes. The following three main features were identified: The minima are observed during periods when long-range correlations have been developed, but they are preceded by a stage in which an evident anti-correlated behavior appears. After the minima, the long-range correlations break down to an almost random behavior turning to anti-correlation. This is strikingly similar to the findings in other complex time series as, for example, the case of electrocardiograms. In this case, the long-range temporal correlations that characterize the healthy heart rate variability break down for individuals with a high risk of sudden cardiac death and often accompanied by the emergence of uncorrelated randomness [
1,
69].
The aforementioned minima
of the order parameter fluctuations that precede
EQs can be distinguished from other minima that are either non-precursory or followed by smaller EQs on the basis of certain criteria developed in [
44] (see its Appendix). They could be summarized as follows: In order to classify a
value, it should be a minimum for at least
W values before (bef) and
W values after (aft). Further, to assure that
,
and
(where the subscript
, 250 and 300 events stands for the natural time window length used in the calculation) are precursory of the same mainshock and hence belong to the same critical process, almost all (in practice above 90% of) the events which led to
should participate in the calculation of
and
.
To distinguish the
that are precursory to EQs of magnitude
from other minima (min) which are either non-precursory or may be followed by smaller magnitude EQs, we create separate studies for the larger and the smaller area, i.e.,
and
, respectively, see
Figure 3, and the results obtained should be necessarily checked for their self-consistency. For example, a major EQ whose epicenter lies in both areas should be preceded by
identified in the separate studies of these two areas approximately (in view of their difference in seismic rates) on the same date. In particular, we work in the following way (see the Appendix of [
44]).
Let us assume that we start the investigation from the larger area where five EQs of magnitude 7.8 or larger occurred from 1 January 1984 until the
M9 Tohoku EQ in 2011 (
Table 1). We first identify the
minima that appear a few months before all these EQs and determine their
values (see
Table 2). These values are found to lie in a narrow range very close to unity [
44], i.e., in the range 0.92–1.06. (This range slightly differs from the previously reported [
36] range 0.95–1.08 mentioned in
Section 3 since in [
44], the numerical accuracy of the calculated
values for
is somewhat improved.) This is understood in the context that these values correspond to similar critical processes, thus exhibiting the same dependence of
on
W. During the whole period studied, however, beyond the above-mentioned
minima before all the
EQs, more minima exist. Among these minima, we choose those which are as deep or deeper than the shallowest one of the
values previously identified (e.g., 0.294 in
Table 2) and, in addition, they have
values lying in the narrow range determined above. Thus, we now find a list of “additional”
minima (see Table 3 of Ref. [
44]) for which is must be checked whether they are either non-precursory or may be followed by EQs of smaller magnitude.
We now repeat the whole procedure—as described above—for the determination of
minima in the smaller area. Thus, we obtain a new set of
minima (with shallowest
= 0.293 and
range 0.97–1.09, see
Table 3) that appear a few months before all the
EQs (cf. there exist four such EQs in the smaller area, see
Figure 1), as well as a new list of “additional”
minima (see Table 5 of [
44]) to be checked for whether they are non-precursory or may be followed by smaller magnitude EQs. Comparing these new
minima with the previous ones, we investigate whether they:
(1) Appear in both areas practically on the same date (differing by no more than 10 days or so);
(2) Exhibit the three main features (i.e., the sequence (A) anti-correlated behavior/(B) correlated/(C) almost random behavior) identified from the results of DFA of EQ magnitude time series discussed in the main text of [
44]. In Tables 3 and 5 of [
44], corresponding to the “additional” minima, are marked in bold the values which do not satisfy at least one of the three inequalities given below which
quantify these three main features (A), (B), and (C):
In view of the fact that considerable errors are introduced in the estimation of the
exponent when using a relatively small number of points as the one used here, i.e., 300 (for application in other seismically active areas see [
66,
70]), we adopted the value
as the maximum one in order to assure anti-correlated behavior in the period before the appearance of
minimum.
A summary of the main results obtained after carrying out this investigation is as follows:
First, the
minima identified a few months before all the
EQs with epicenters inside of both areas obey the aforementioned requirements (1) and (2), see
Table 2 and
Table 3. Remarkably, in the remaining case, i.e., the East-Off Hokkaido
M8.2 EQ labeled EQ2 in
Table 1, with an epicenter outside of the smaller area but inside the larger, we find
minimum not only in the study of the larger area (on 30 June 1994, see
Table 2), but also in the relevant study of the smaller area (see the third
minimum in Table 5 of [
44] observed on 5 July 1994). Second, all the other “additional”
minima resulting from the studies in both areas (see Tables 3 and 5 of [
44]) violate at least one of the requirements (1) and (2). In other words, only the
minima appearing a few months before the five
EQs obey all the aforementioned properties. Interestingly, this procedure could have been applied before the occurrence of the
M9 Tohoku EQ, just after the identification of the deepest
minimum on 5 January 2011 with
almost in unity, thus lying inside the narrow ranges determined from previous
EQs (see
Table 2 and
Table 3), resulting in the conclusion that a
EQ was going to occur in a few months.
We clarify that the above procedure does not preclude of course that one of the “additional” minima may be of truly precursory nature, but corresponding to an EQ of magnitude smaller than the adopted threshold. As a first example, we mention the case marked FA7 in Table 3 of [
44] referring to a
observed on 12 April 2000. It preceded the
M6.5 EQ that occurred on 1 July 2000 of the volcanic-seismic swarm activity in the Izu Island region in 2000 (which was preceded by a pronounced SES activity that started on 26 April 2000 recorded by Uyeda and coworkers, as already mentioned in
Section 4), see the
curve (red) in Figure 3C of [
44]. A second example is the case marked EQc in Table 3 of [
44], which refers to a
on 15 October 1994 that preceded the
M7.6 Far-Off Sanriku EQ on 28 December 1994 [
36].
8. On a Unique Change of the Seismicity Order Parameter Fluctuations before the M9 Tohoku Earthquake in Japan in 2011
In
Section 3, upon analyzing the Japan seismic catalog in natural time from 1 January 1984 to 11 March 2011, we computed the seismicity order parameter fluctuations
by using a sliding natural time window comprising the number
i of EQs that would occur on average within the crucial scale [
43] of a few months, or so, which is the average lead time of SES activities. We explained that these fluctuations
exhibited distinct minima
a few months before all the shallow EQs of magnitude 7.6 or larger in the Japanese area
. Among these minima, the minimum before the
M9 Tohoku EQ observed at around 5 January 2011 was the deepest. In addition to this unprecedented seismicity order parameter fluctuation decrease, almost two weeks earlier, i.e., on 22 December 2010, a unique increase of the seismicity order parameter fluctuations was observed [
71] in the following respect: Upon using a sliding natural time window comprising 300 events, which is the average number of EQs (
) that occur within ≈2 months and plotting the fluctuations
of the order parameter of seismicity in the entire Japanese region
versus the conventional time from 1 January 1984 until the Tohoku EQ occurrence on 11 March 2011 upon considering all
EQs irrespective of their depth, we find that there exist six prominent fluctuations of
(greater than 1.2) before the
M9 Tohoku EQ. These are accompanied by the following major EQs: Upon the occurrence of the
M7.5 EQ on 15 January 1993 at 42.92
N 144.35
E as well as upon the occurrences of the shallow EQs of magnitude 7.6 or larger (EQa to EQe) already listed in
Section 3 (see
Figure 11 and
Figure 12). Among these six prominent
fluctuations, the following two are the largest and were observed in 2003 and in 2010: First, the large fluctuation of
in 2003 appears upon the occurrence of the
M8 Off Tokachi EQ on 26 September 2003 (attaining its maximum value on 28 September 2003, see
Figure 12b). It is the largest one observed upon any other major EQ occurrence during the 27-year period of our study. Second, the
fluctuation increase on 22 December 2010 is observed upon the occurrence on the same day of the
M7.8 near Chichi-jima EQ. Concerning these two larger
fluctuations, the following comments apply: The one in 2010 appears almost simultaneously with the minimum
of the change
of the entropy of seismicity (cf. that of the entire Japanese region) under time reversal, which will be discussed in detail in a later part of this work, showing that such a minimum is of precursory nature signaling that a large EQ is impending according to the conclusions deduced from the natural time analysis of the OFC model summarized in [
71].
The above facts corroborate the suggestion that between the two larger
fluctuations discussed here, only the second, i.e., the one on 22 December 2010, that precedes the Tohoku
M9 EQ by almost three months, is likely to be of a precursory nature. It is very important to draw attention to the fact that the validity of this conclusion has been also checked, beyond the case of
300 events presented in
Figure 11, for several other lengths from
150 to 500 events. Such a change of the length
i also reveals the following behavior: Upon increasing
i, it is observed (see Figures 2B and 4E of Ref. [
36]) that the increase
of the
fluctuation on 22 December 2010 becomes distinctly larger, which does not happen (see Figure 4A–D of [
36]) for the
fluctuations increase upon the occurrences of all other shallow EQs in Japan of magnitude 7.6 or larger from 1 January 1984 to the time of the
M9 Tohoku EQ. Such a behavior that obeys the interrelation
, see
Figure 12g,h, has a functional form strikingly reminiscent of the one discussed by Penrose et al. [
73] in computer simulations of phase separation kinetics using the ideas of Lifshitz and Slyozov [
74], see their Equation (33) which is also based on work by Lifshitz and Slyozov (cf. the seminal work by Lifshitz and Slyozov [
74] and independently by Wagner [
75] on phase transitions, which will be explained in more detail in
Section 10). It is also very important to note that this functional form is lost in our calculation upon considering not all
EQs, but restricting ourselves to depths
h either
km or
km, see the symbols in green in
Figure 13. Hence, the
fluctuation on 22 December 2010 accompanying the minimum
is
unique. By employing the most recent method of ECA [
47], which considers a time lag
and a window
between the precursor and the event to be predicted, the profound statistical significance of this unique result can be further assured as follows: Assuming the
M9 Tohoku EQ as the event to be predicted, we estimated the probability (
p-value) that the fluctuation increase on 22 December 2010 is a chancy precursor with a lag
and a window
, i.e., the EQ occurs within the time period from
to
days after. By employing the function
CC.eca.es of the CoinCalc package [
76] for R that implements ECA, we found that the probability of this happening by chance is very small, i.e., it varies from
0.79% to 0.01% upon varying the window
varies from 78 days to 1 day, respectively.
In short, on 22 December 2010, the order parameter fluctuations (that are minimized almost two weeks later) showed a
unique increase exhibiting a functional form, which stems from the ideas of Lifshitz and Slyozov for the time growth of the characteristic size of the minority phase droplets in phase transitions. Remarkably, this unique increase occurred at the same date at which the entropy change of seismicity under time reversal was minimized as shown in [
77], which will be discussed in the next section of this work.
9. Natural Time Analysis:
Minimum of the Seismicity Entropy Change under Time Reversal before Major Earthquakes
In [
77], it was shown that upon analyzing in natural time all EQs of magnitude
M 3.5 or larger in Japan from 1 January 1984 until the occurrence of the super-giant
M9 Tohoku EQ on 11 March 2011, a pronounced minimum of the entropy change of seismicity under time reversal is observed two-and-a-half months before this
M9 EQ. Notably, a simultaneous minimum with an unusual low value (
) for the exponent
resulting from the detrended fluctuation analysis of the EQ magnitude time series indicates an evident anticorrelated behavior. These findings are consistent with an earlier result [
78] that upon analyzing the OFC model for EQs in natural time, which is the most studied non-conservative self-organized criticality model for EQs, one finds a non-zero change of the entropy upon time reversal. This reveals a breaking of the time symmetry, and hence reflects the predictability in this model. Note that OFC model originated from a simplification of the Burridge–Knopoff spring-block model [
79].
We recall from
Section 3 that for a time series comprising
N events, an index for the occurrence of the
k-th event is defined by
, which is termed natural time. We, then, study the pairs
, or the pairs
, where
is the normalized energy for the
k-th event. The entropy
S in natural time is defined [
8,
80] as
where the bracket
denotes the average value of
weighted by
, i.e.,
and
. It is
dynamic entropy depending on the sequential order of events [
81], thus changing upon the occurrence of each event. The entropy obtained by Equation (
7) upon considering [
9] the time-reversal
, i.e.,
, is labeled by
, i.e.,
(see also [
56,
57]).
The difference
labeled
may also have a subscript (
) meaning that the calculation is made (for each
S and
) with a sliding natural time window of length
i (=number of successive events), i.e., at scale
i (see also below). As for the calculation of the time series of the entropy change under time reversal, a window of length
i is sliding each time by one event, through the whole time series and the entropies
S and
(and therefrom their difference
) are calculated each time. Thus, we form a new time series comprising successive
values. It was found [
1] that
is a key measure which may identify when the system approaches the critical point (dynamic phase transition).
has been applied [
10,
82], for example, for the identification of the impending sudden cardiac death risk, which is the major cause of death in industrialized countries [
83,
84,
85]. In addition,
was employed as a useful tool [
78] (see also Section 8.3.4 of [
1]) to study the predictability of the OFC model, as mentioned above.
The analysis in natural time was conducted by using the
JMA seismic catalog, e.g., see [
36,
37] and considering all EQs of magnitude
from 1984 until the Tohoku EQ occurrence on 11 March 2011 within the area
(see the map of
Figure 3). The calculation was performed also for a second larger area
in order to avoid boundary effects and assure that the results do not depend on the selection of the area studied having on average ≈125 and ≈145 EQs per month for the smaller and larger area, respectively. The energy of EQs was obtained from the
JMA magnitude
after converting [
39] to the moment magnitude
[
40].
The time evolution of
was studied for a number of scales
i of the seismicity with
occurring in both areas, larger and smaller, by selecting proper scales
i as follows: An SES activity, whose lead time ranges from a few weeks up to around
months [
1], exhibiting critical behavior [
3,
8,
86], is observed during a period in which long-range correlations between EQ magnitudes prevail. On the other hand, before the initiation of the SES activity, and hence before
, another stage appears in which the temporal correlations between EQ magnitudes exhibit an anticorrelated behavior [
44]. Hence, there exists a significant change in the temporal correlations between EQ magnitudes when comparing the two stages that correspond to the periods before and just after the initiation of an SES activity. This change is likely to be captured by the time evolution of
, thus, our study of
started from the scale of
events, which corresponds to the number of seismic events
that occur during a period around the maximum lead time of SES activities.
Plotting the
values versus the conventional time for several scales, a careful inspection of the results depicted in Figures 2 and 4 of [
77] for the larger and smaller area, respectively, reveals the following common feature: At shorter scales, i.e., from
to
events, a number of local minima appear, but leaving aside all these changes, we find that at longer scales, i.e.,
,
and
events, a pronounced minimum is observed on 22 December 2010 (on this date, the
M7.8 Near Chichi-jima EQ occurred at
N
E [
36,
37]). It was shown that this minimum is statistically significant, for example in the larger area, by means of the following procedure: By randomly shuffling the EQ magnitude time series and assigning each magnitude to an existing EQ occurrence time, the calculations were repeated
times and investigated the resulting
time series of the longer scales, i.e.,
,
and
, for minima occurring on the same date and deeper than or equal to those depicted in the aforementioned Figure 2 of Ref. [
77]. Only three such cases were found out of the
studied. Hence, the probability of obtaining minima comparable or deeper than those shown in Figure 2 of Ref. [
77] by chance is approximately 3%, which demonstrates that our result is statistically significant.
This minimum on 22 December 2010 was found [
77] to be robust upon changing either the EQ depth (from 0–300 km) or the magnitude threshold (considering also additional thresholds
3.7 and 4.0) as well as the size of the area, i.e., beyond the two areas 21
(larger,
) and 21
(smaller,
) studied, we repeated the calculations for thirteen additional areas.
In summary, the earlier finding in [
78] that before a large avalanche in the OFC model for EQs a minimum of the entropy change of seismicity under time reversal is observed is confirmed. This is so because the natural time analysis of seismicity in Japan—during the almost 27-year period from 1 January 1984 until the occurrence of the
M9 Tohoku on 11 March 2011—revealed that for longer scales, i.e,
events, the minimum of
values was observed on 22 December 2010.
10. Fluctuations of the Entropy Change of Seismicity under Time Reversal before the M9 Tohoku Earthquake in Japan
In summary, in [
87], it was shown that almost two-and-a-half months before the
M9 Tohoku EQ that occurred in Japan on 11 March 2011, the complexity measure
associated with the entropy change of seismicity in natural time under time reversal (that will also be defined below) exhibited an abrupt increase, which conforms to the Lifshitz–Slyozov–Wagner (LSW) theory for phase transitions (mentioned in short in
Section 8). In particular,
displayed a behavior similar to that of the characteristic size of the minority phase droplets through a scaling behavior in which time growth has the form
where the prefactors
A are proportional to the scale
i, while the exponent (1/3) is independent of
i. As for the Tsallis entropic index
q [
88,
89,
90,
91,
92,
93], it exhibits a simultaneous increase which interestingly obeys the same exponent (1/3), but the prefactors
A are not proportional to the scale
i. In addition, it was found that the complexity measures
,
and
show a decrease just after the fulfillment of the criticality condition
almost one day before the Tohoku EQ occurrence.
Beyond the aforementioned
M9 Tohoku EQ in 2011, in Japan, another recent example was studied in [
94], which is the
M8.2 EQ that occurred in the Chiapas region in Mexico on 7 September 2017. This was Mexico’s largest EQ in more than a century. Upon analyzing the seismicity in natural time, it was shown that almost three months before its occurrence, the following precursory behavior was identified: The entropy change under time reversal exhibited a minimum [
94] together with increased fluctuations of the entropy change under time reversal, as well as by a simultaneous Tsallis entropic index
q increase [
95]. The variations of this index before strong EQs has been studied by several researchers [
96,
97,
98,
99,
100,
101,
102,
103]. In addition, by analyzing the seismicity during the 6-year period 2012–2017 in natural time in the Chiapas region where the
M8.2 EQ occurred, it was found [
95] that on the same date, i.e., on 14 June 2017, the complexity measure
(see below) associated with the fluctuations of the entropy change under time reversal showed an abrupt increase together with a simultaneous increase of the Tsallis entropic index
q [
88,
89,
90,
91,
92,
93].
As already explained in the preceding section, the entropy
S in natural time, which is a dynamic entropy that exhibits [
9] concavity, positivity and Lesche stability [
104,
105], is given by:
where
. Its value upon considering the time reversal
, i.e.,
, is labeled by
. The value of
differs, in general, from
S, see, e.g., [
10], (see also [
57] and references therein), and thus,
S does satisfy the conditions to be “causal”. The physical meaning of the change of entropy
in natural time under time reversal is discussed in [
1,
10,
57].
Using a window of length
i (number of consecutive events) sliding through the time series of
L consecutive EQs, the entropy in natural time has been determined for each position
of the moving window. Thus, a time series of
is obtained. By considering the standard deviation
of the time series of
, we define [
1,
95,
106], the complexity measure
when a moving natural time window comprising
i consecutive events is sliding through the time series and the denominator has been selected [
95] to correspond to the standard deviation
of the time series of
of
i = 100 events. This complexity measure quantifies how, upon changing the scale from
events to a longer scale, e.g.,
events, the statistics of the
time series changes. The calculations are performed by means of a window of length
i (=number of successive EQs) sliding, each time by one EQ. The difference
of the entropies
S and
is calculated each time, hence, a new time series comprising successive
values is formed and the complexity measure
is determined in accordance with its definition in Equation (
10).
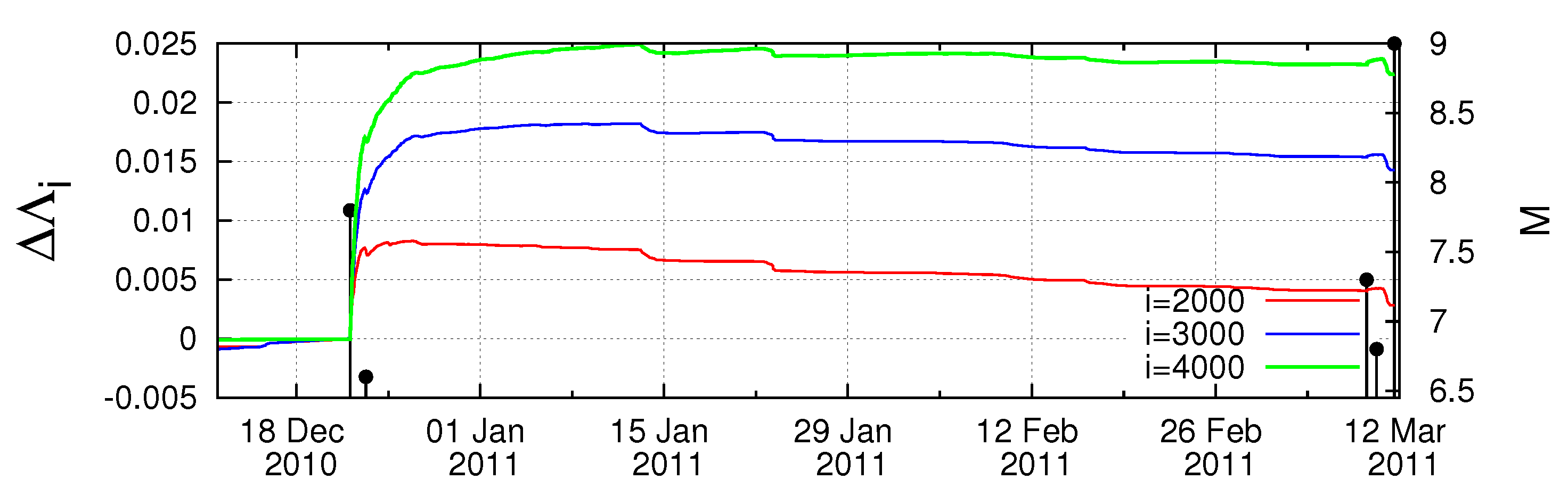
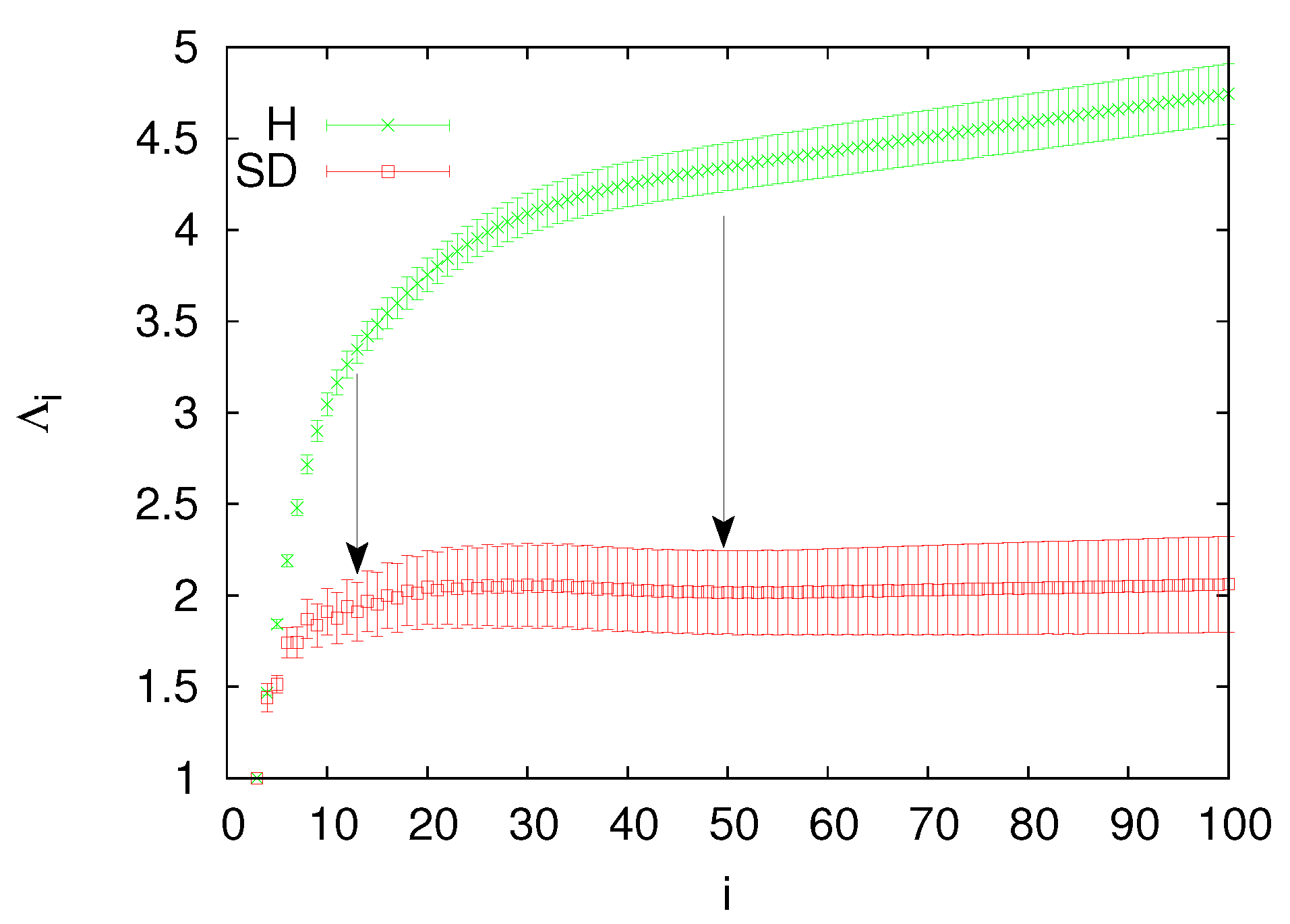
Here, in
Figure 14a–f, the
values, for example for the scales
i = 2000, 3000 and 4000 events, are plotted versus the conventional time from 1 January 1984 until the occurrence of the
M9 Tohoku EQ on 11 March 2011. Furthermore, all EQs of magnitude 7.0 or larger are also inserted in the same figure with vertical lines ending at circles, which are read in the right scale. This figure reveals that, for all the three scales studied, almost
months before the
M9 EQ, i.e., on 22 December 2010, we observe an abrupt increase of the
values. This happens upon the
M7.8 EQ occurrence with an epicenter at
N
E [
37]. This can be better seen in
Figure 15, which is a three-month excerpt of
Figure 14f in expanded horizontal scale from 10 December 2010 until 11 March 2011 and depicts for readers’ better inspection the increase
of the
values after the
M7.8 EQ on 22 December 2010 mentioned above.
Figure 15 reveals that all three complexity measures,
,
and
(i.e., the
values at the scales
i = 2000, 3000 and 4000 events, respectively), show an obvious and abrupt increase on 22 December 2010 and after the aforementioned
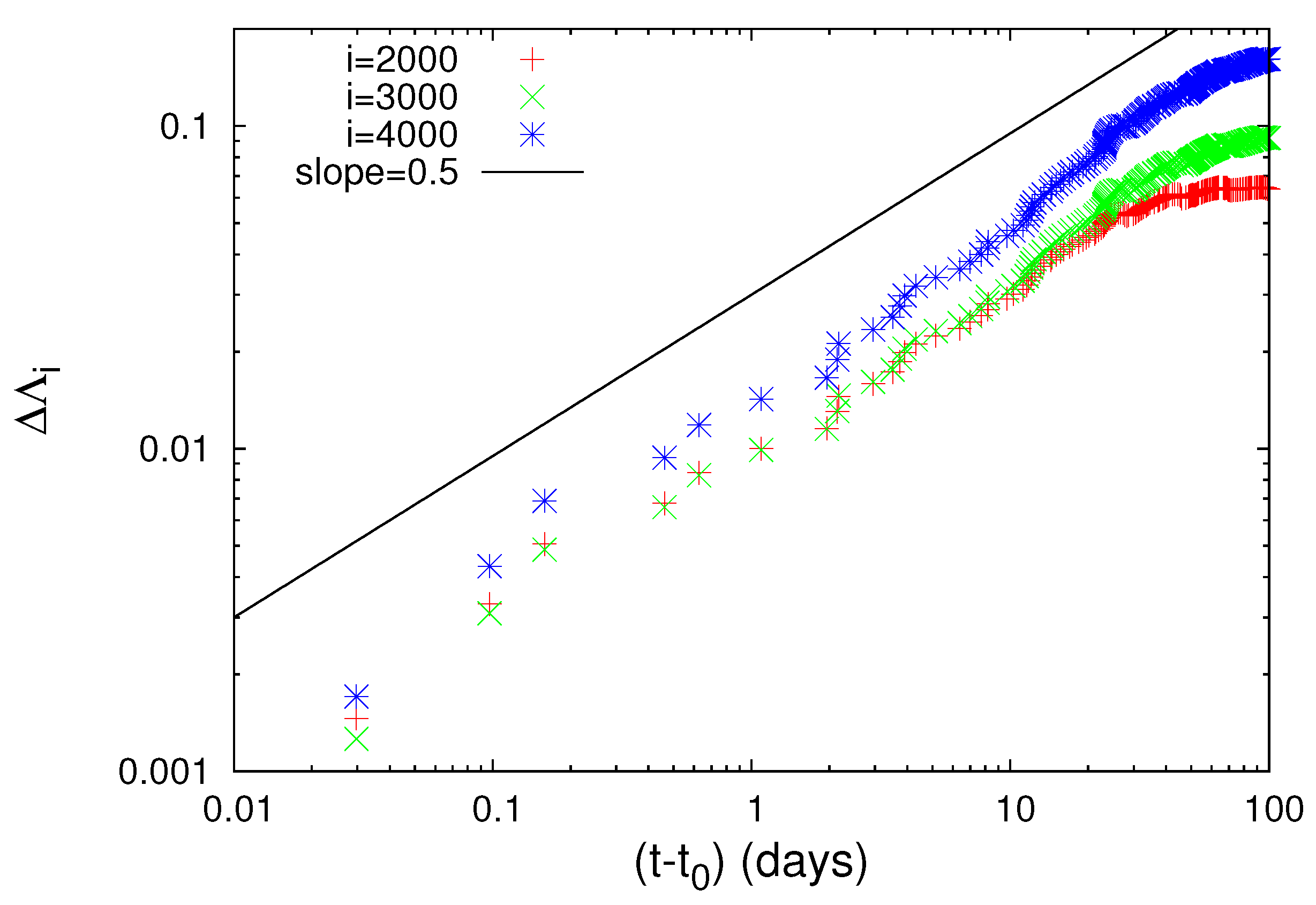
M7.8 EQ occurrence exhibit a scaling behavior of the form
where the exponent
c is approximately equal to
independently of the scale
i, while the pre-factors
A are proportional to
i (see
Figure 16) and
is almost 0.2 days after the
M7.8 EQ occurrence. Equation (
11) confirms the seminal work by Lifshitz and Slyozov [
74] and independently by Wagner [
75] on phase transitions (LSW theory), which predicts that the time growth of the characteristic size of the minority phase droplets grows with time as
.
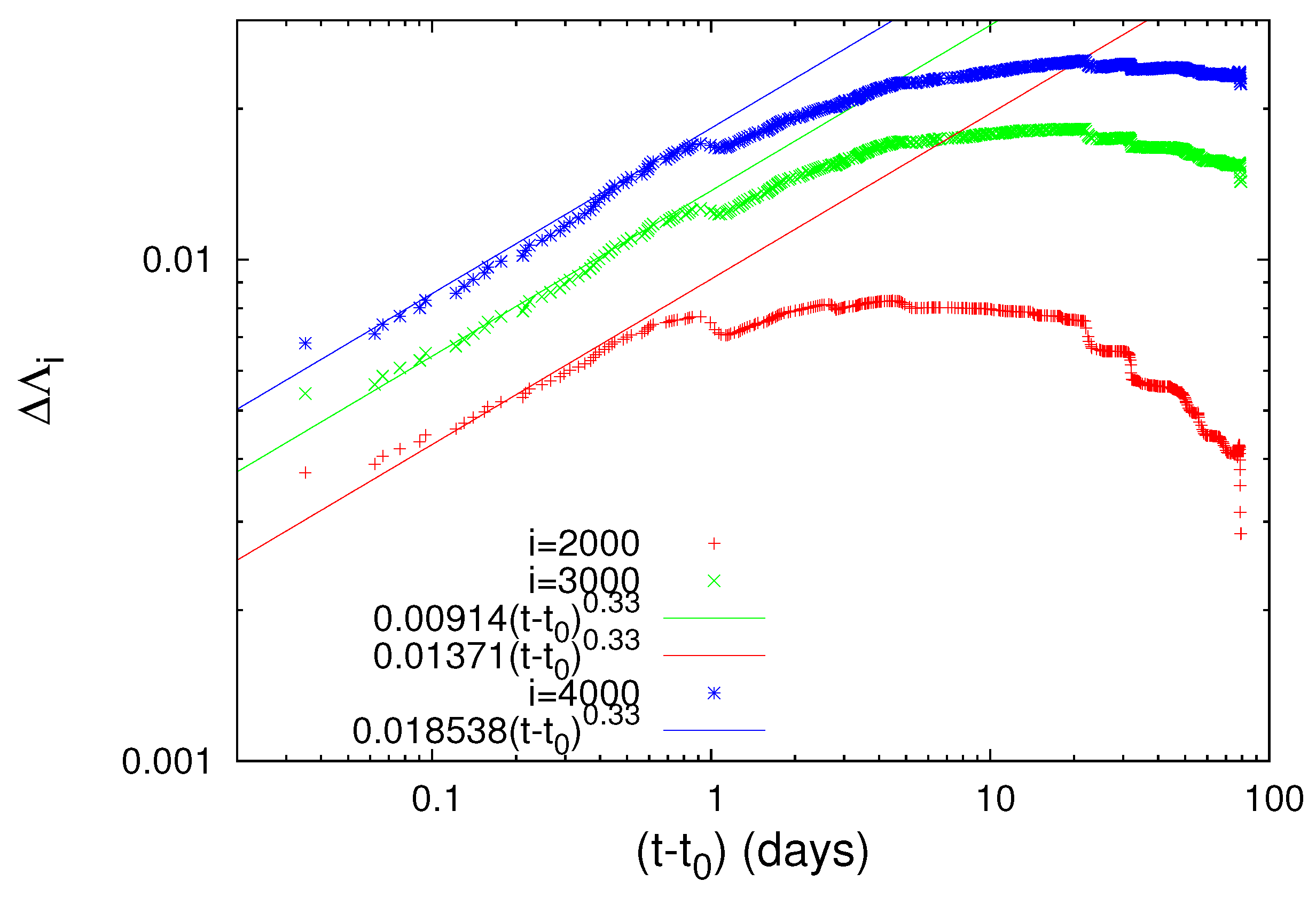
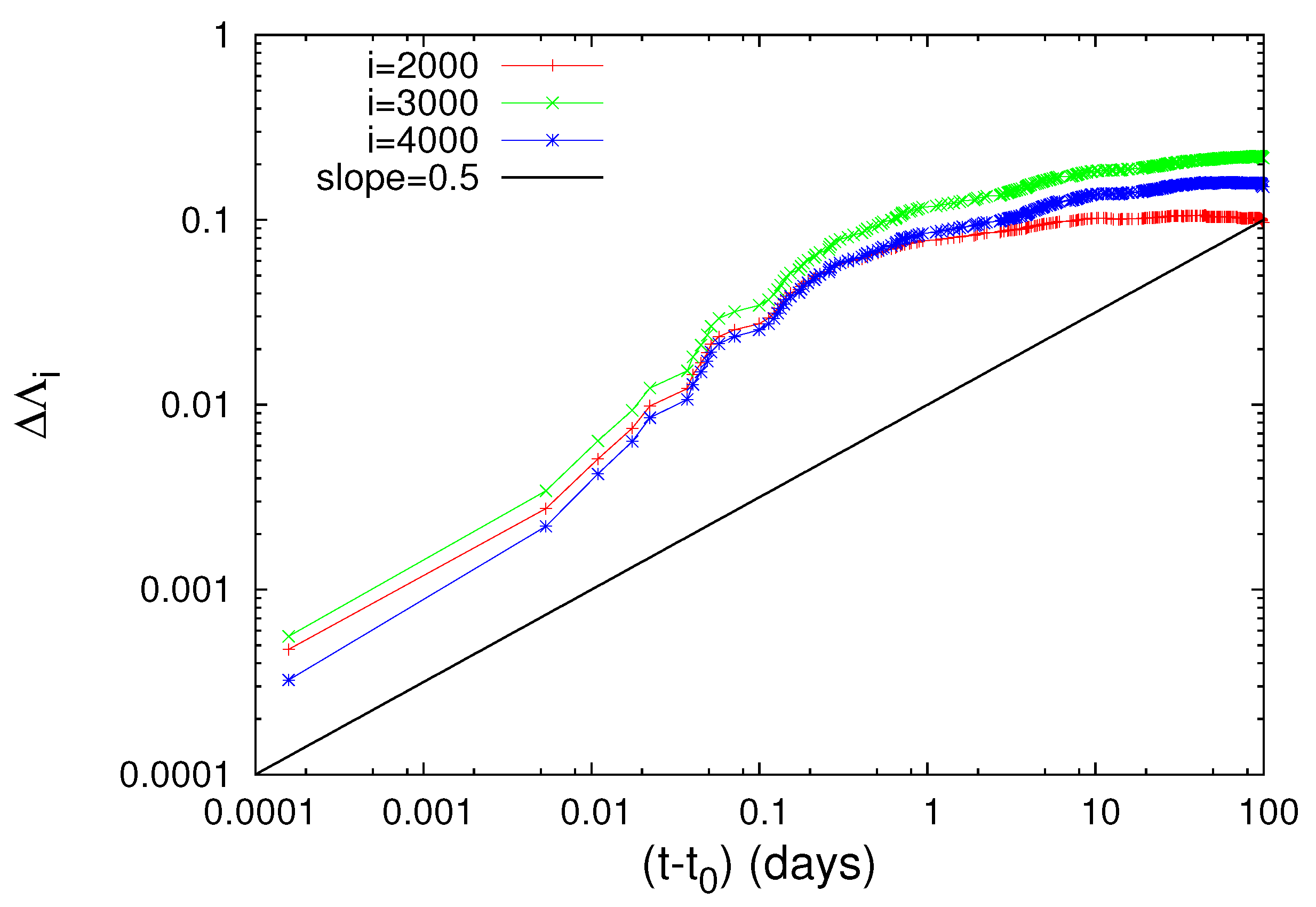
To shed more light on whether this increase of
is possibly associated with strong EQs in general, let us now further investigate the obvious increases (which are the most significant ones in
Figure 14) of
on 2 November 1989 and on 15 January 1993 that can be visualized in
Figure 14b and study whether they exhibit a scaling behavior similar to that identified above for the abrupt
increase on 22 December 2010. Concerning the
increase on 2 November 1989,
Figure 17 depicts the log–log plot of the changes
of the complexity measures
,
and
versus the elapsed time
in days from the
M7.1 EQ occurrence on the same date increased by 0.022 days. In addition, for the readers’ convenience, the straight black line with slope 0.5 is also plotted in
Figure 17 in order to easily recognize that the value of
c for the three complexity measures,
,
and
, is close to 0.5, thus differing markedly from the value
predicted by the LSW theory. Furthermore, a careful inspection of
Figure 17 reveals that the pre-factors are not proportional to
i (e.g., see that for
i = 3000 events, the green line is higher than the blue line that corresponds to
i = 4000 events). Hence, the
increase associated with the EQ on 2 November 1989 does not obey Equation (
11), thus strongly deviating from the LSW theory of phase transitions. Along the same lines, we now give in
Figure 18, as in
Figure 17, the log–log plot that corresponds to the
changes of the complexity measures
,
and
versus the elapsed time
in days from the
M7.5 EQ occurrence on 15 January 1993 increased by 0.014 days. The exponent in
Figure 18 is also close to 0.5—thus deviating from the value
of LSW theory—and the relevant prefactors
A are not proportional to the scale
i, for example, the red crosses corresponding to the scale
i = 2000 do not practically differ from the green symbols of the larger scale
i = 3000.
We now proceed to the results deduced by means of nonextensive statistical mechanics [
91], pioneered by Tsallis [
88,
93]. This has found application [
89,
90] in the physics of EQs through the Tsallis entropic index
q. On the basis of the EQ magnitude distribution, one can obtain [
92,
107] the entropic index
q and study how it varies with time as we approach a strong EQ (for a recent review, see [
103]). In
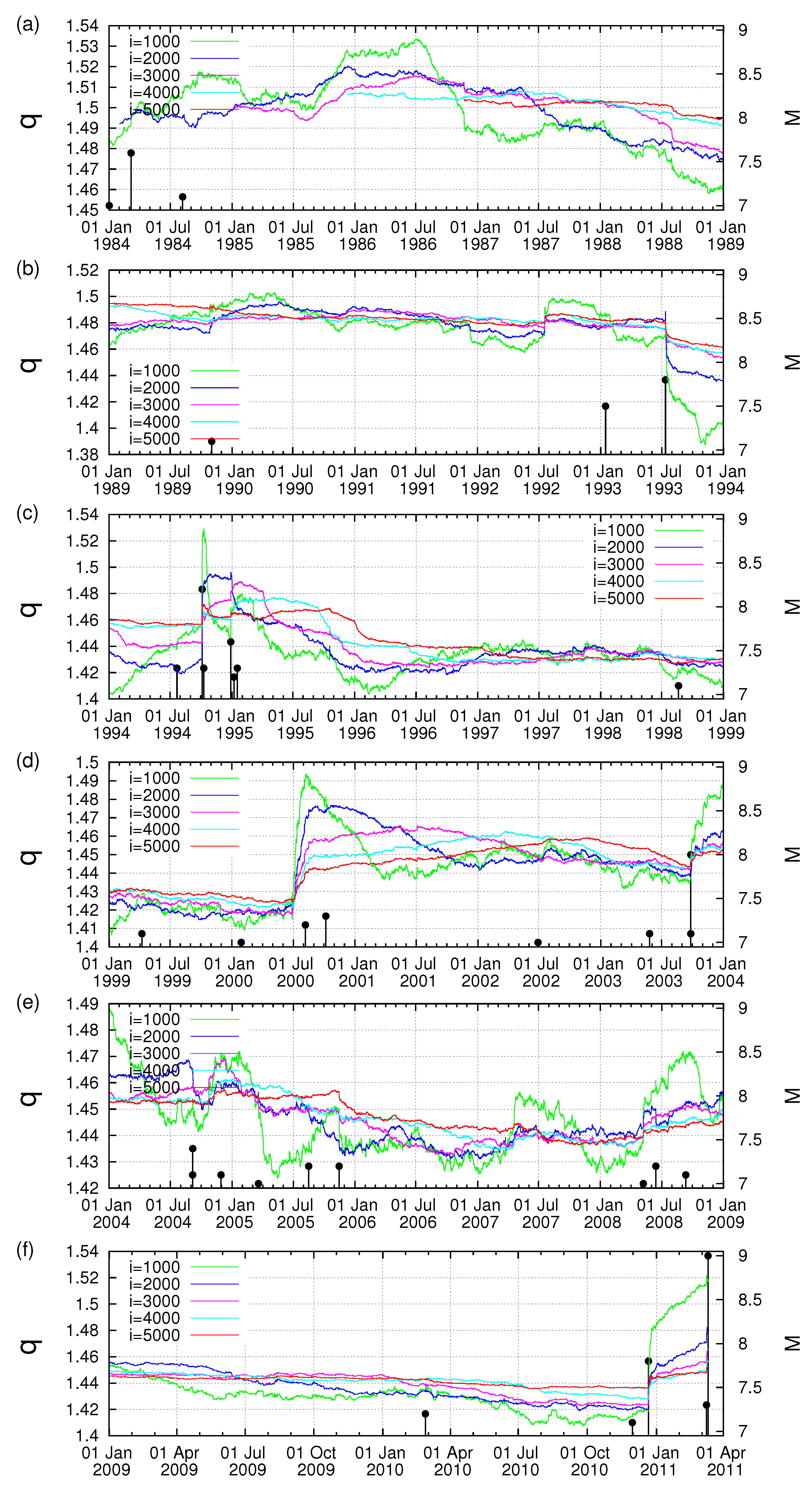
Figure 19, we present the
q-value versus conventional time during 1984–2011 as it was estimated [
107] for several sliding windows of
i = 1000, 2000, 3000, 4000 and 5000 consecutive EQs for
in the Japanese area
. We find that the
q-value shows an abrupt increase upon the
M7.8 EQ occurrence on 22 December 2010 and hence before the occurrence of the
M9 Tohoku EQ. A three-month excerpt from
Figure 19 is shown in expanded time scale in
Figure 20 from 10 December 2010 until the Tohoku EQ on 11 March 2011. We finally present in
Figure 21 as in
Figure 16,
Figure 17 and
Figure 18, a log–log plot of the changes
of the Tsallis entropic index versus the time
in days elapsed from the
M7.8 EQ occurrence on 22 December 2010 increased by approximately 0.2 days.
Figure 21 reveals that the exponent
c in Equation (
11) is around
, in accordance with the LSW theory, but the prefactors
A are not proportional to
i.
Figure 15 also shows the following very interesting feature of the complexity measure
: On 9 March 2011, a
M7.3 foreshock occurred, and the next day, on 10 March 2011, all three complexity measures,
,
and
, exhibited a simultaneous decrease. What is the origin of this
decrease since the next day, i.e., on 11 March 2011, the
M9.0 mainshock occurred? To answer this question, we recall
Figure 8 (and in particular
Figure 8B) which reveals that the condition
(which signals that the mainshock is going to occur shortly, i.e., within the next few days or so) is fulfilled for
= 4.2 to 5.0 in the morning of 10 March 2011 upon the occurrence of the EQs from 08:36 to 13:14 LT, i.e., almost one day before the Tohoku EQ. Strikingly, we now see clearly that the aforementioned decrease of
,
and
after the M7.3 EQ occurs just after the gray shaded area in
Figure 8B where the criticality condition
was obeyed, thus signaling that the mainshock was approaching.
11. Identifying the Occurrence Time of a Mainshock by Means of the Fluctuations
of the Seismicity Entropy Change under Time Reversal
It is in the scope of this section to explain a procedure to identify the approach of the critical point (mainshock) without making use of the criticality condition
for the evolution of seismicity after the initiation of the SES activity in the candidate epicentral area that was used in
Section 6. In particular, we shall show here, following [
108], that in natural time analysis, the complexity measure
that quantifies the fluctuations of the entropy change
of the seismicity in the Japanese area under time reversal plays a key role in identifying the occurrence time of the Tohoku mega-EQ that occurred on 11 March 2011 in Japan with magnitude 9.0, which is the largest-magnitude event recorded in Japan.
The main point of the physical basis for the procedure followed here is that, as mentioned in the first part of
Section 9, when analyzing in natural time (see below) the OFC model for EQs [
55],
exhibits an evident minimum [
1] (or maximum upon defining, e.g., see [
78]
instead of
) before a large avalanche, i.e., a large EQ. We also consider the following two points: First, we recall from
Section 9, that the study of the time evolution of
should be conducted at scales of the order of
events, since this scale corresponds to the number of
EQs that occur during a period of at least around the maximum lead time of SES activities (because ∼145 EQs occur per month in the entire Japanese region and consider a period of at least
months). Second, the following characteristic feature was identified in [
109], where we analyzed the seismic data (
) in the entire region of Japan in natural time and calculated the complexity measure
: When the calculation is made at scales markedly longer than 2000 events, e.g.,
3000, 4000 and 5000 events, the ending dates of the calculation show a tendency to be clearly clustered into two groups: First, the one group that comprises markedly larger
values corresponds to ending dates later than the date 22 December 2010 at which the minimum of the entropy change of seismicity under time reversal
has been observed (as discussed in
Section 9), thus being closer to the occurrence date of the Tohoku EQ. Second, the other group that comprises appreciably lower
values corresponds to earlier dates. Practically the same behavior is observed upon increasing the magnitude threshold to 4.0 and using scales that are smaller by a factor of 2.5 in view of the smaller number of EQs per month we have for this threshold (cf. Figure S6 in [
77]).
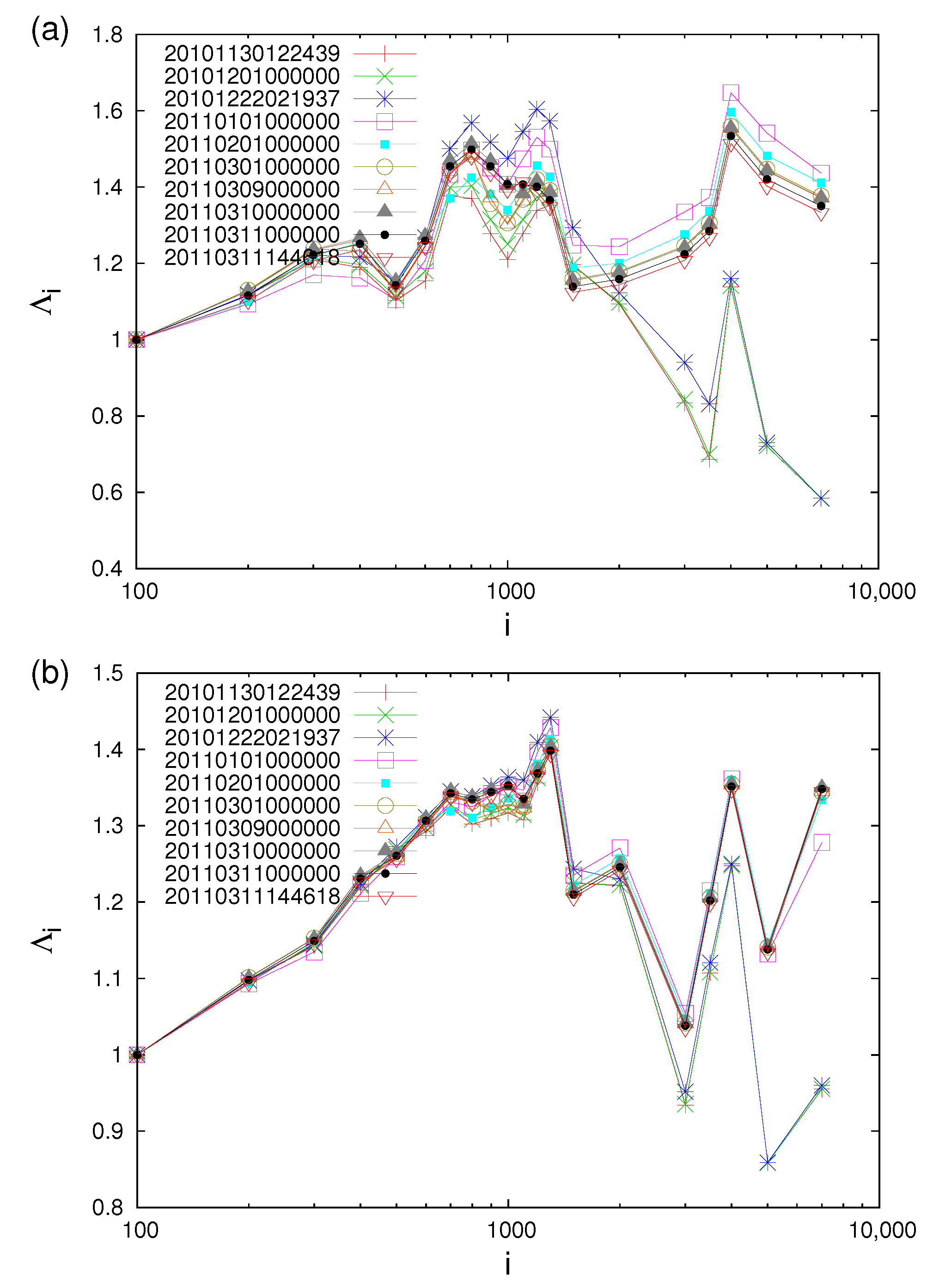
In
Figure 22, we plot the
values versus the scale
i (number of events) for all
EQs in the entire Japanese region
. They have been computed by starting the calculation on 1 January 2010 (a) and 1 January 2006 (b) until the following dates: 30 November 2010 (pluses in red, just before a
M7.1 EQ on this date), 1 December 2010 (crosses in green), 22 December 2010 (asterisks in blue, just before the
M7.8 EQ that occurred on this date), 1 January 2011 (open squares in magenta, this date is very close to the initiation of an SES activity because—as explained in
Section 6—anomalous magnetic field variations were recorded during the period of 4 to 14 January 2011 on the z-component at two measuring sites at epicentral distances ≈ 130 km), 1 February 2011 (solid circles in cyan), 1 March 2011 (open circles in olive green), 9 March 2011 (open triangles in orange, at 00:00 LT, thus almost 12 h before the
M7.3 EQ on 9 March 2011), 10 March 2011 (gray filled triangles, at 00:00 LT thus almost 12 h after the
M7.3 EQ on 9 March 2011), 11 March 2011 (solid circles in black, at 00:00 LT, thus almost 15 h before the mega-earthquake occurrence) and 11 March (inverted red triangles, almost 10 min before the
M9 Tohoku EQ).
A careful inspection of this figure reveals that, for scales longer than ≈2000 events, the
values after 1 January 2011—a date very close to the initiation of the SES activity (when long-range temporal correlations are developed, as discussed in
Section 7—maximize and later start to decrease until the mainshock occurrence (see
Figure 22a). This behavior, which is systematic (and robust since it is not affected if we change the scale in the denominator in Equation (
10) from
events to
or 200 events, see Figure S1 in the Supplementary Material of [
108]), disappears in the longer scales in
Figure 22b when for
3.5 the calculation starts on 1 January 2006 (the same feature is observed in
Figure 23a for
4.0 EQs, where the computation started on 1 January 2010, while it disappears in
Figure 23b when starting in 2006). This reflects that for each scale, when the computation starts appreciably early in the past, a smaller percentage of events close to the mainshock occurrence are included in the
calculation.
The above systematic behavior persists upon starting the calculation in 2010 even closer to Tohoku
M9 EQ, i.e., on 1 March, or 1 May, or 1 July (instead of 1 January), see
Figure 24 for M
EQs, see also Figures 2 and 3 in [
110] if we start the calculation on 1 September (a), 1 October (b) and 1 November 2010 (c). In these figures, we can see clearly that
decreases gradually when we approach the
M9 Tohoku EQ (see also
Figure 25). However, when focusing on the
values calculated by using only the EQs inside the candidate epicentral region of Figure 1 of [
109] (i.e., the blue-green area in
Figure 7 estimated, as mentioned, by the spatiotemporal study of
developed in [
37]), we find (see Figure 5 of [
109], which is also reproduced here for readers’ better information, see
Figure 26) that independently from the year at which the computation starts (i.e., 1 January 2006, 1 January 2008, 1 January 2009, and 1 January 2010, thus almost 5, 3, 2 and 1 year before the 2011
M9.0 EQ), for every scale larger than around
400–500 EQs all
values almost coincide, but after the 9 March 2011
M7.3 EQ, a monotonic increase is observed, which continues until 10 minutes before the
M9 EQ on 11 March 2011.
Let us summarize concerning the evolution of the
values obtained from the natural time analysis of the EQs inside the large area
(entire Japanese region), the following facts have been secured for (each) longer scale
i (cf. These results are consistent with the PSPC physical model for SES generation as it will become clear in the next
Section 12): The values of
start to increase after 22 December 2010, when
reached a minimum, and attain a maximum around the first days of January 2011, very close to the beginning of the strong SES activity that gave rise to the anomalous variations of the magnetic field of the Earth recorded predominantly on the vertical component (in a period when EQ magnitudes exhibit long-range correlations as evidenced by the DFA). Subsequently,
decrease gradually up to the 11 March 2011
M9 Tohoku EQ.
Second, calculated by considering the seismicity inside the candidate epicentral area behaves differently, increasing abruptly after the M7.3 EQ on 9 March 2011 until the Tohoku M9 EQ.
The above observation that, when the
M7.3 EQ occurred on 9 March 2011,
of the seismicity inside the epicentral area exhibited a clear increase, while
in the large area
continued to diminish, represents the important difference unveiled by natural time analysis leading, well in advance, to the characterization of the
M7.3 EQ as a foreshock. This characterization agrees with the conclusion drawn in
Section 6 by an independent procedure.
12. Discussion:
Compatibility of the SES Generation Model with the Experimental Results of the Recent Decade
As mentioned in the Introduction, here, we present in short the main results of the recent decade by means of natural time analysis and investigate whether the experimental results are compatible with the SES generation model presented in
Section 2. We start with the discussion of the results deduced in the preceding
Section 11: In
Figure 27a,b, we have plotted
at various ending dates close to the end of December 2010 and the beginning of January 2011 in order to make it clear that they have reached a maximum value after starting to increase from their minimum on 22 December 2010 when the entropy of the Japanese seismicity
exhibited its minimum. This behavior can be understood along the following lines.
Close to the start of 2011, in January, three phenomena appeared. First, the observation of an SES activity initiation that lasted during 4 to 14 January 2011 since Earth’s magnetic field exhibited [
59,
61,
62] anomalous variations mainly on the vertical component at two sites—Esashi (ESA) and Mizusawa (MIZ), see Figure 1 of [
109]—located at epicentral distances of around 130 km. In addition, two more phenomena appeared almost simultaneously: The establishment of long-range temporal correlations between EQ magnitudes (see
Section 7 and [
44]) and the occurrence of the deepest
(since 1 January 1984) of the seismicity order parameter fluctuations
on 5 January 2011 [
36] (see also
Section 3). The latter is in agreement with the experimental result that the seismicity order parameter
fluctuations are observed [
21,
38] to minimize when an SES activity starts. These three simultaneous phenomena around 5 January 2011 were preceded by a clear anticorrelated behavior in EQ magnitudes since, as mentioned in
Section 7, the DFA exponent of the EQ magnitudes time series becomes
upon the occurrence of a
M7.8 EQ at 27.05
N 143.94
E (Southern Japan) on 22 December 2010. Strikingly, on this date, the initially random horizontal GPS azimuths gradually started to orient toward the southern direction probably as a result of excess stress. These observations are fully compatible with the first stage (
stage A) of the SES generation model of
Section 2 (see also [
21]) according to which as a result of an excess stress disturbance (cf. this excess disturbance seems to be associated with the unique increase of the
fluctuations discussed in
Section 8),
starts to increase until it reaches
at which the prexisting electric dipoles of the future focal area cooperatively align (
stage B of the physical model, see
Section 2). Then, very interestingly, the most intense crust uplift was observed [
21]. Later than the observation of
on 5 January 2011, the GPS azimuth orientations returned [
111] to become random, being consistent with an EQ magnitudes time series DFA exponent
[
44], around 13 January 2011.
Subsequently, anti-correlation was observed around 23 January 2011 with an exponent of DFA
and a shift of EQ-related stress disturbance was identified [
111] where westward movements replaced the southward ones, in the sense that orientations of the residual displacements were re-aligned along the western direction and the crust depressed. Later, the stress disturbance gradually approached the threshold of the fault rupture, and the orientations of the residual surface displacements became random again [
111], thus agreeing with the results of DFA for the EQ magnitudes which led to
0.5 up to approximately 10 February 2011 (see Figure 5 of [
44]), indicating randomness.
We finally comment on the fact that a behavior close to random was observed before the Tohoku EQ. This is in accordance with findings in time series originating from other complex systems such as electrocardiograms (ECGs). In this case, long-range temporal correlations indicating heart rate variability of healthy subjects cease for those of high risk of sudden cardiac death. The latter is accompanied by the appearance of uncorrelated randomness [
1,
69] (cf. sudden cardiac death can be considered as a critical phenomenon, e.g., see [
10,
80,
81]).
Figure 28 shows that
values for H exceed markedly those for SD. Thus, the fact that
, for the longer scales, first reached maximum in the beginning of January 2011 and later gradually decreased until 10 minutes before the
M9 Tohoku EQ occurrence fully agrees with a similar behavior in other complex systems.
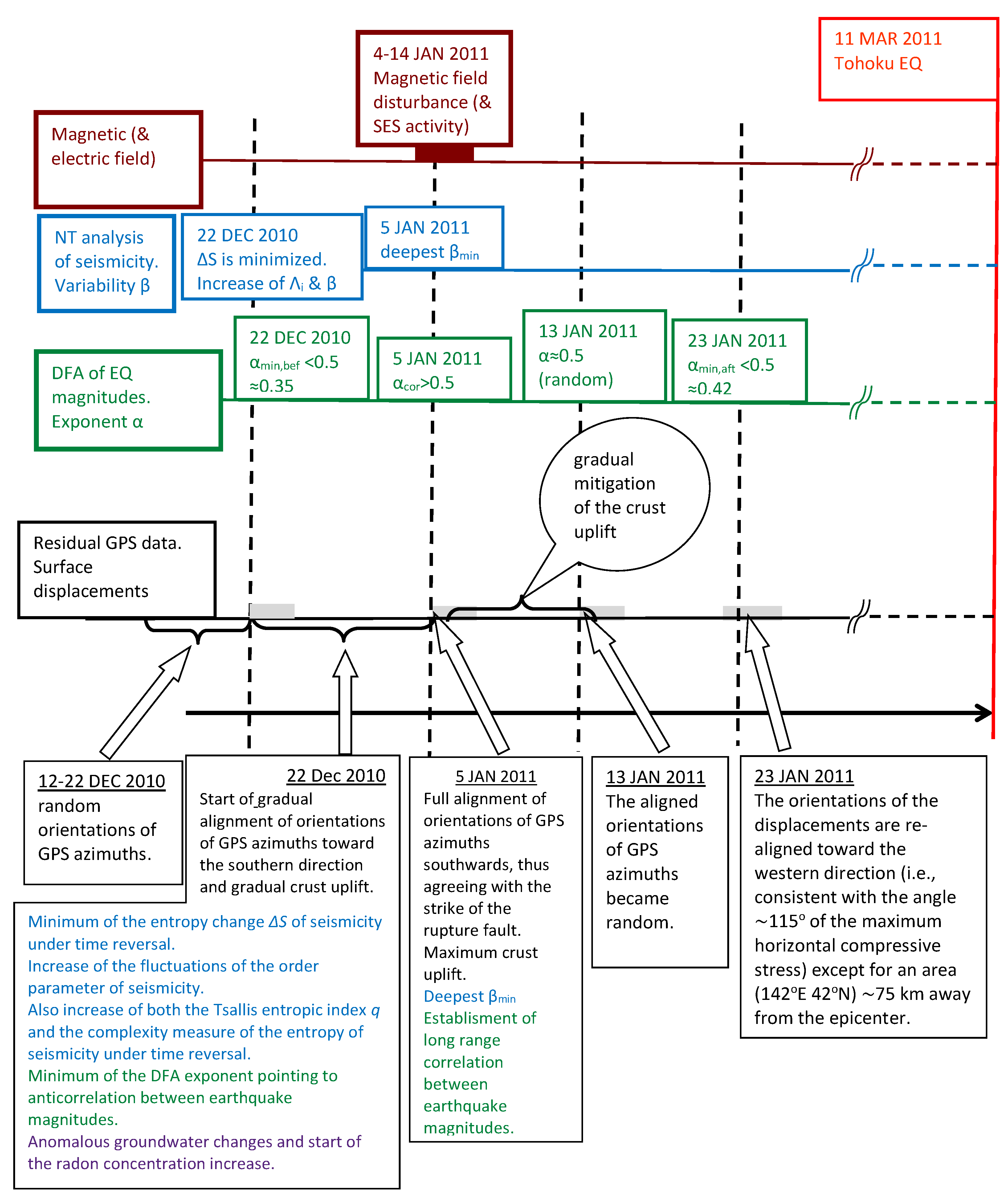
Other points explained in the frame of the SES generation physical model can be found in [
21], in which a schematic diagram is presented (see
Figure 29) that compiles the multidisciplinary changes (during the period of observations from 12 December 2010 until 23 January 2011) before the
M9 Tohoku EQ that occurred in Japan on 11 March 2011.
Last but not least, we note that in a recent perspective [
110] under the title of “Self-organized Criticality and Earthquake Predictability: A long standing question in the light of natural time analysis”, the following matter is discussed: After the Bak-Tang-Wisenfeld seminal work on self-organized criticality (SOC), the following claim appeared by other workers in the 1990s: Large earthquakes cannot be predicted, since the Earth is in a state of SOC, hence, any small EQ has some probability of cascading into a large event. In that perspective [
110], we discussed that such claims do not stand in the light shed by natural time analysis, which was shown in the beginning of 2000s to extract the maximum information possible from complex systems time series, as well as unveiling hidden properties in complex time series.
Let us summarize the most important dates mentioned in this work upon applying natural time analysis to the Japanese seismic data from 1 January 1984 until the super-giant
M9 Tohoku earthquake on 11 March 2011. We find that, leaving aside the details, precursory phenomena were mainly accumulated around two dates, i.e., 22 December 2010 and 5 January 2011, which strikingly concur with the two stages A and B of the SES generation PSPC physical model, respectively. These phenomena, accompanying other phenomena of multidisciplinary nature, include the following (see
Figure 29):
(A) Around 22 December 2010:
The entropy change of seismicity under time reversal is minimized along with increased fluctuations;
There is fluctuation increase of the order parameter of seismicity;
The DFA exponent diminished in the value 0.35, which is the lowest value observed during the period 1984–2011 of our study, pointing to an evident anti-correlated behavior in the EQ magnitude time series;
The horizontal GPS azimuths started to become gradually oriented toward the southern direction (while during the preceding period 12–22 December 2010, they had random orientations);
Anomalous changes started in the groundwater (level drop, temperature decrease, and probably increase in radon concentration);
There was an increase in the Tsallis entropic index q.
(B) Around 5 January 2011:
Unprecedented minimum of the fluctuations of the order parameter of seismicity;
Earth’s magnetic field started exhibiting anomalous variations primarily on the vertical component (which, according to Maxwell equations, should be accompanied by a strong SES activity). This agrees with the findings in Greece where EQs of magnitude
were predated by considerable SES activities with simultaneous variations of the magnetic field of the Earth [
31] predominantly observed on the vertical component [
32,
33];
Full alignment of the orientations of the GPS azimuths southwards that was accompanied by the most intense crust uplift;
Long-range temporal correlations in the earthquake magnitude time series.
All the above phenomena were observed to begin and end well before the
M9 Tohoku EQ occurrence, as schematically shown in
Figure 2a in accordance with the SES generation PSPC model (
Figure 1). This differs essentially from other proposed precursory mechanisms [
22], which suggested that the anomalous behavior becomes more intense upon approaching the EQ failure, as seen in
Figure 2b.