Sediment Bed-Load Transport: A Standardized Notation
Abstract
1. Introduction
1.1. Reason and Task
1.2. Choice of the Standardized Function Notation
2. Transcription of Some Formulas Discussed in This Paper
2.1. Meyer-Peter and Mueller (1948/1949)
2.2. Fernandez Luque and Van Beek (FlvB, 1976)
2.3. Engelund and Fredsoe (1976)
2.4. Bridge and Dominic and Bridge and Hanes
2.5. Parker, Seminara and Solari (PSS)
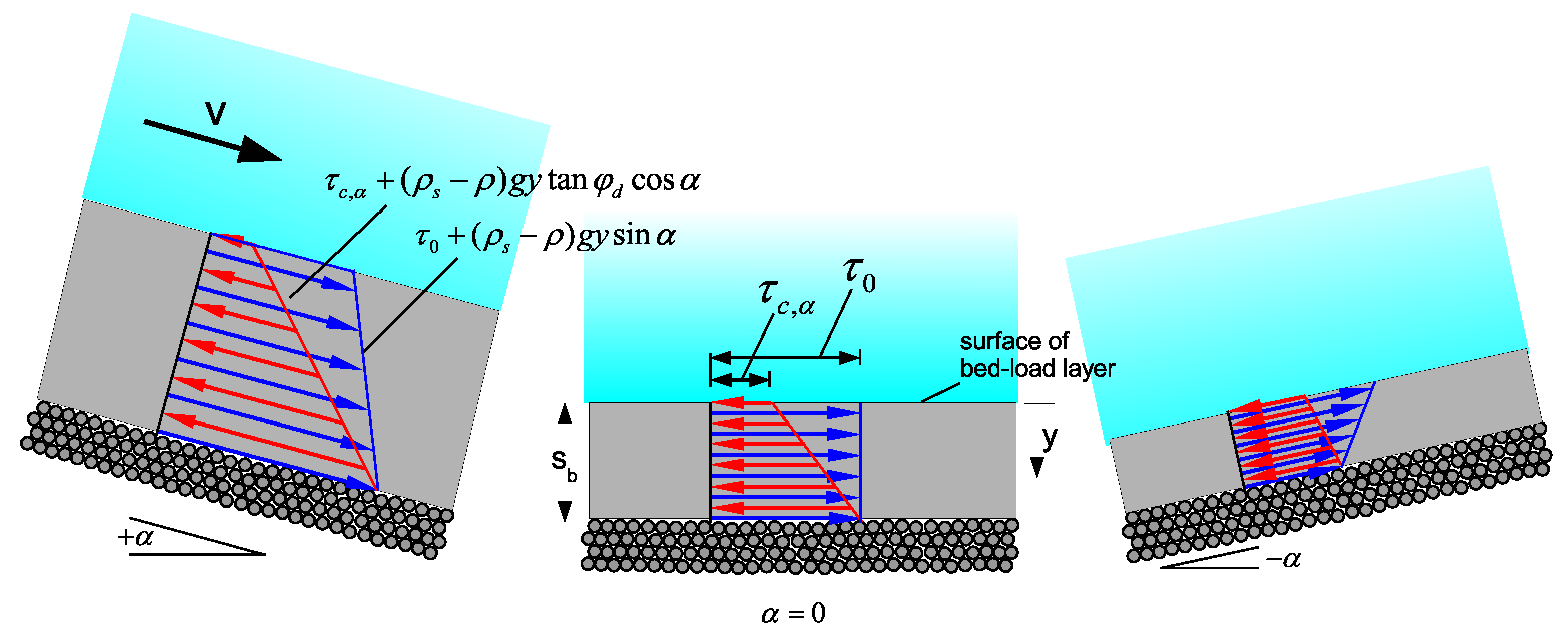
2.6. Zanke (1999, 2001, 2004) and the Inclusion of the Risk of Initial Movement
2.6.1. Consideration of the Probabilistic Character of Beginning of Sediment Motion
2.6.2. or ?
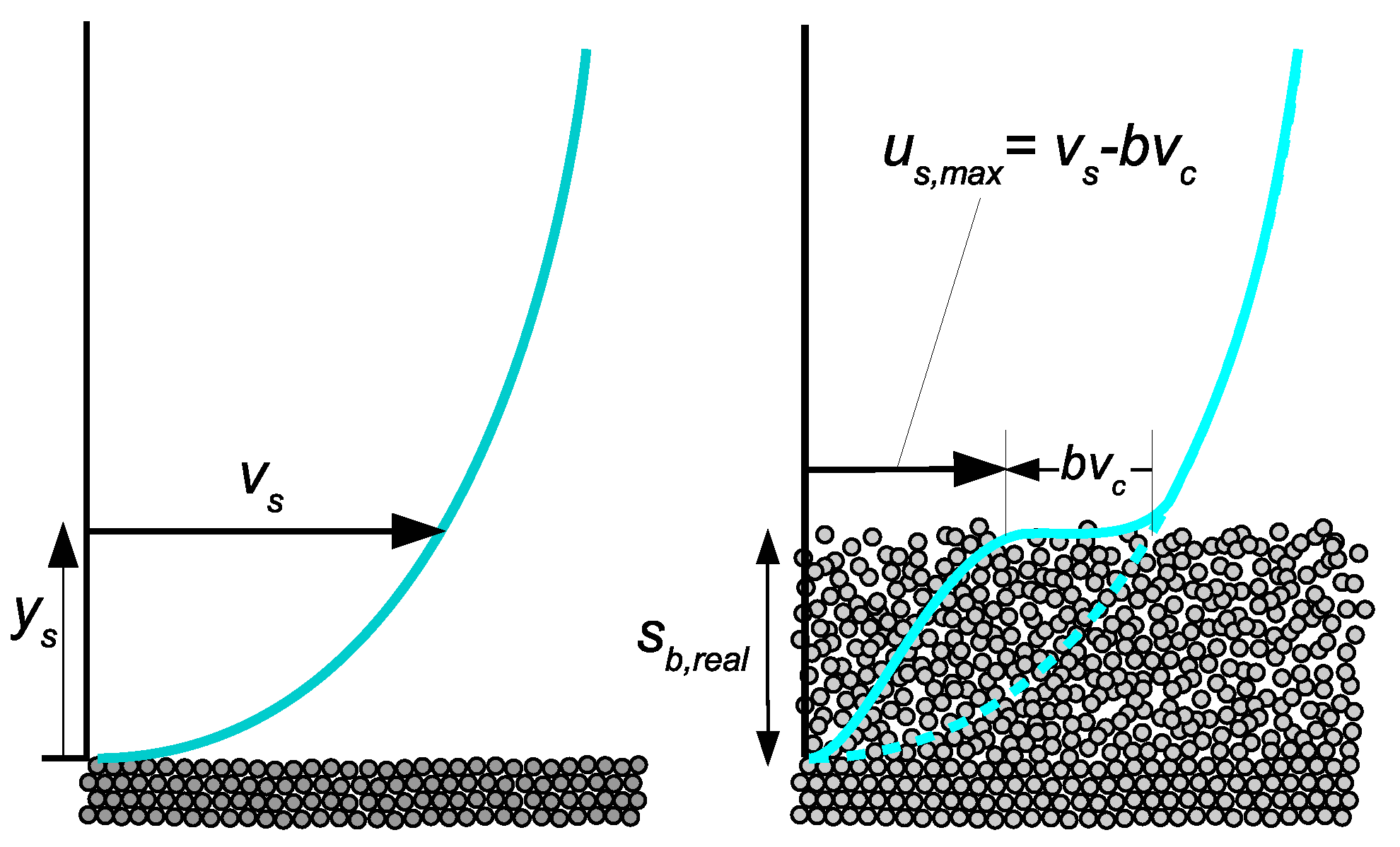
2.6.3. Average Transport Velocity of Bed-Load Layer,
2.6.4. Thickness of Bed-Load Layer
- Another confirmation of the linearity of Equation (16) can be drawn from numerical simulations by Duran et al., [25,26] and Pähtz/Duran [4], which indicate that the number of transported particles per area is a linear function of . Furthermore, Duran et al., 2012 stated that this linearity is true for both, bed-load and saltation and came to the conclusion ‘that dissipation due to collisions of the moving grains with the bed play the same role in both transport regimes’.
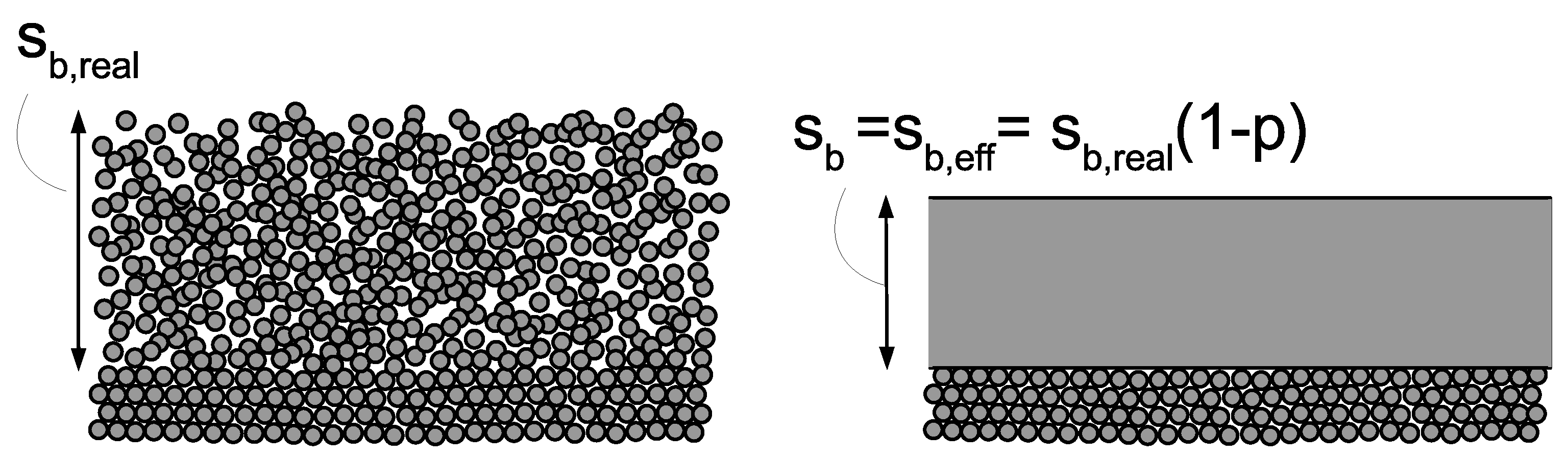
Porosity of Bed-Load Layer
2.6.5. Sediment Transport Rate
2.7. Lajeunesse, Malverti and Charru and Houssais and Lajeunesse
2.8. Duran, Andreotti and Claudin
3. A Standardized Structure of Bed-Load Transport Formulas
3.1. Formulas with (Widely) Identical Structures
3.2. Differences and Congruences of the Bed-Load Formulae
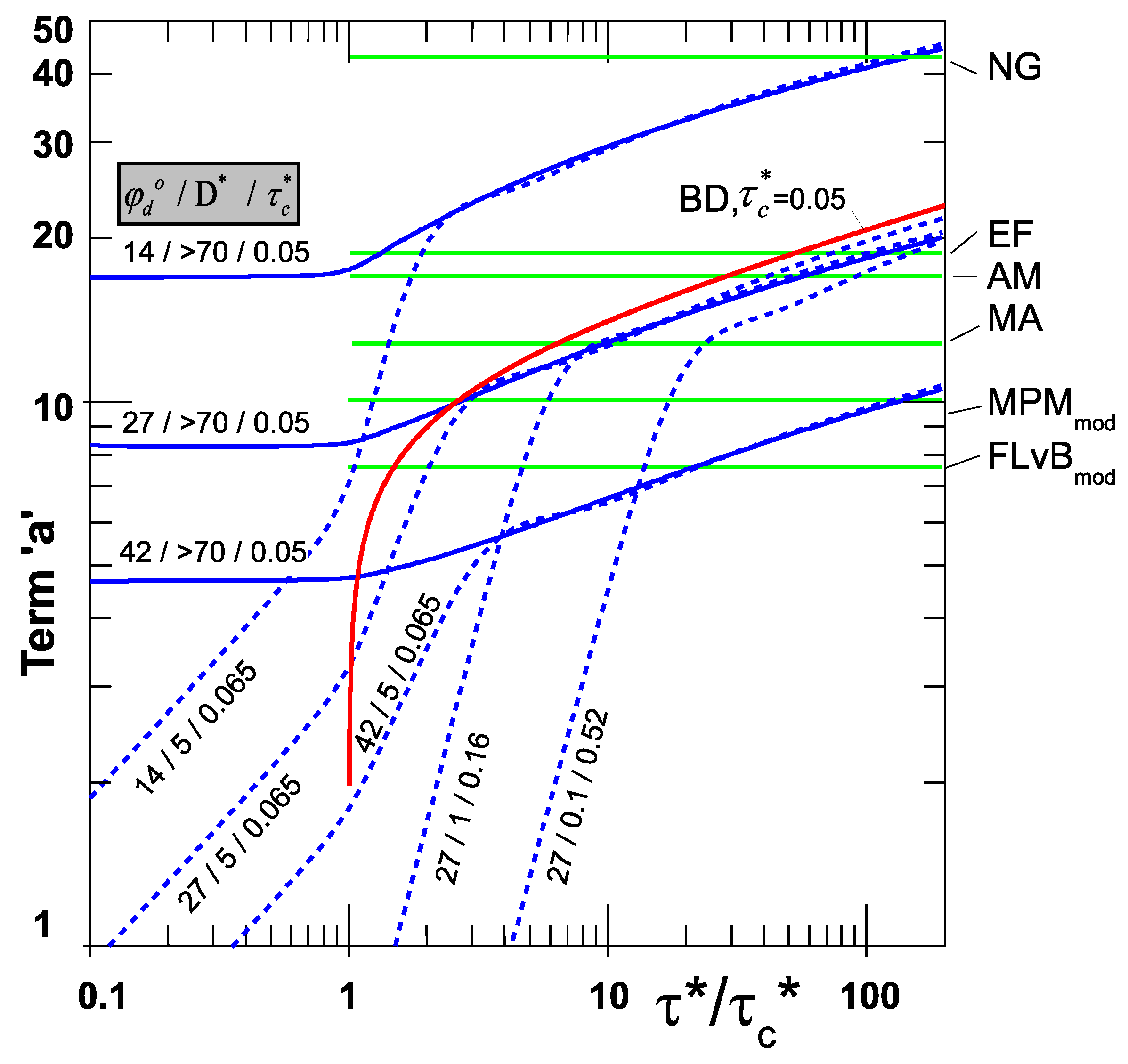
3.2.1. Differences with Respect to Term ‘a’ of the Equations of Table 1
3.2.2.
3.2.3. Differences with Respect to
3.2.4. Differences with Respect to
Shear Stress Affects
3.2.5. Differences with Respect to Slip Factor ‘b’ in Expressions for Bed-Load Velocity,
4. Bandwidth of Results of Bed-Load Formulas
5. Results and Conclusions
Author Contributions
Funding
Conflicts of Interest
Symbols
| a | factor, regarding effects on transport rate other than shear stress | - |
| B | integration constant of log. velocity profile | - |
| d | grain diameter | m |
| dimensionless grain diameter = | - | |
| g | acceleration of gravity | m/s2 |
| h | water depth | m |
| I | longitidinal bed slope | - |
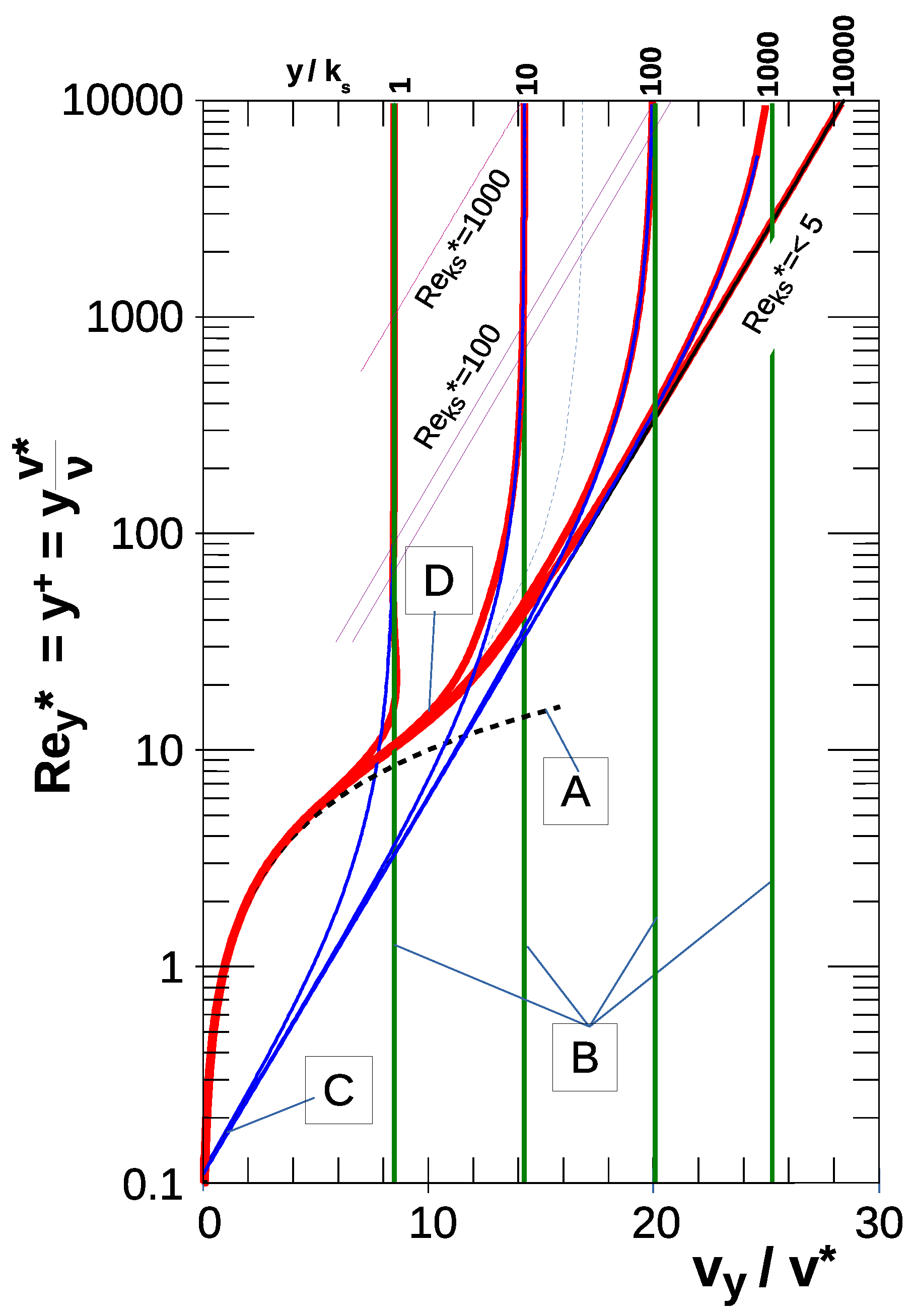
| equivalent sand roughness height (here is taken) | m | |
| = | - | |
| P | probability | - |
| probability of the flow to be turbulent at level y | - | |
| p | porosity, relation between pore volume and total volume of a sediment | |
| bulk, if unknown, estimate 30% … 36% | - | |
| bed-load transport rate (transported sediment volume without | m3/m/s | |
| pore volume), bulk transport rate = | ||
| = , dimensionless transport rate after EINSTEIN | - | |
| R | risk or probability of grain motion. when | - |
| = , particle Reynolds number | - | |
| = = effective (= net) thickness of moving bed-load layer, | m | |
| i.e., thickness without pores | ||
| = = real thickness of moving bed-load layer, | m | |
| i.e., thickness with pores | ||
| depth averaged sediment velocity of moving bed-load layer | m/s | |
| sediment velocity at top edge of moving bed-load layer | m/s | |
| depth averaged flow velocity | m/s | |
| depth averaged critical velocity for initiation of sediment motion | m/s | |
| flow velocity at level y | m/s | |
| flow velocity at a the upper edge of bed-load layer (Figure 1) | m/s | |
| critical velocity for initiation of sediment motion | m/s | |
| shear velocity = | m/s | |
| Shields critical shear velocity | m/s | |
| terminal settling velocity | m/s | |
| y | distance from the wall | m |
| distance from the wall where | m | |
| angle of longitudinal bed inclination (positive in direction of flow) | ||
| angle of repose = angle of internal friction of sediment | ||
| angle of repose at rest | ||
| angle of internal friction of sediment in motion | ||
| if unknown, estimate | ||
| factor regarding bed inclinations | - | |
| kinematic viscosity of fluid | m2/s | |
| density of fluid | kg/m3 | |
| density of sediment | kg/m3 | |
| , relative density | - | |
| , shear stress at the bed | N/m2 | |
| , dimensionless shear stress | - | |
| Shields dimensional critical shear stress at horizontal bed = | N/m2 | |
| dimensionless Shields critical shear stress at the bed | - | |
| Shields critical shear stress at a bed with inclination angle | - | |
References
- Einstein, H.A. The Bed-Load Function for Sediment Transportation in Open Channel Flows; U.S. Department of Agricultural Technology Bulletin: Washington, DC, USA, 1950; Volume 1950.
- Cheng, N.-S. Exponential Formula for Bed Load Transport. ASCE J. Hydraul. Eng. 2002, 128, 942–946. [Google Scholar] [CrossRef]
- Li, J.-D.; Sun, J.; Lin, B. Bed-Load transport rate based on the entrainment probabilities of sediment grains by rolling and lifting. Int. J. Sediment Res. 2018, 33, 126–136. [Google Scholar] [CrossRef]
- Pähtz, T.; Duran, O. Unification of Aeolian and Fluvial Sediment Transport Rate from Granular Physics. Phys. Rev. Lett. 2020, 124, 168001. [Google Scholar] [CrossRef]
- Fernandez-Luque, R. Erosion and Transport of Bed-Load Sediment; Dissertation: Delft, The Netherlands, 1974; Volume 14, pp. 127–144. [Google Scholar]
- Fernandez-Luque, R.; van Beek, R. Erosion and Transport of Bed-Load Sediment. J. Hydraul. Res. 1976, 14, 127–144. [Google Scholar] [CrossRef]
- Meyer-Peter, E.; Mueller, R. Formulas for Bed-Load Transport; IAHR: Stockholm, Sweden, 1948. [Google Scholar]
- Meyer-Peter, E.; Mueller, R. Eine Formel zur Berechnung des Geschiebetriebes; Schweizer Bauzeitung: Zürich, Switzerland, 1949; Volume 67. (In German) [Google Scholar]
- Hunziker, R. Fraktionsweiser Geschiebetransport. Ph.D. Thesis, Versuchsanst. f. Wasserbau der ETH Zuerich (VAW), Zuerich, Switzerland, 1995. (In German). [Google Scholar]
- Seminara, G.; Solari, L.; Parker, G. Bed load at low Shields stress on arbitralily sloping beds: Failure on the Bagnold hypothesis. Water Resour. Res. 2002, 38, 31-1–31-6. [Google Scholar] [CrossRef]
- Engelund, F.; Fredsoe, J. A sediment trensport model for straight alluvial channels. Hydrol. Res. 1976, 7, 294–298. [Google Scholar] [CrossRef]
- Zhang, X.; McConnachie, G.L. A reappraisal of the Engelund bed load equation. Hydrol. Sci. 1994, 39, 561–567. [Google Scholar] [CrossRef]
- Zanke, U. Zur Physik von Stromungsgetriebenem Sediment (On the Physics of Flow Driven Sediments); Mitt. des Instituts für Wasserbau und Wasserwirtschaft der TU Darmstadt, Heft 106; RWTH Publications: Aachen, Germany, 1999. [Google Scholar]
- Zanke, U. On the Physics of Flow Driven Sediments (Bed Load). Int. J. Sediment Res. 2001, 16, 1–18. [Google Scholar]
- Zanke, U. Vorlesungsskript ‘Sedimenttransport’ (Scriptum ‘Sediment Transport’); Institut fuer Wasserbau und Wasserwirtschaft der TU Darmstadt, RWTH Publications: Aachen, Germany, 2004; Unpublished work. [Google Scholar]
- Bridge, J.S.; Dominic, D.F. Bed Load Grain Velocities and Sediment Transport Rates. Water Resour. Res. 1984, 20, 476–490, Correction in 1985, 21, 775. [Google Scholar] [CrossRef]
- Zanke, U. Calculation of the Sink Rate of Sediments; Mitteilungen des Franzius-Instituts, Univ. Hannover, Heft 46; RWTH Publications: Aachen, Germany, 1977. (In German) [Google Scholar]
- Parker, G.; Seminara, G.; Solari, L. Bed load at low Shields stress on arbitralily sloping beds. Water Res. Res. 2003, 39, 1–11. [Google Scholar] [CrossRef]
- Zanke, U. On the Influence of Turbulence on the Initiation of Sediment Motion; Mitt. des Instituts für Wasserbau und Wasserwirtschaft der TU: Darmstadt, Germany, 2001. (In German) [Google Scholar]
- Zanke, U. The beginning of sediment movement as a probability problem. In Proceedings of the International Symposium on Sediment Transport Modeling, Sediment Commission, ASCE Hydraulics Division, New Orleans, LA, USA, 4–18 August 1989. [Google Scholar]
- Zanke, U. The Beginning of Sediment Motion as a Probability Problem; Wasser- und Boden, Mitteilungen des Franzius-Instituts, Univ. Hannover, Heft 1; RWTH Publications: Aachen, Germany, 1990. (In German) [Google Scholar]
- Luckner, T.; Zanke, U. An analytical solution for calculating the initiation of sediment motion. ASCE J. Hydraul. Eng. 2007, 22, 87–102. [Google Scholar]
- Grass, A.J. Initial instability of fine bed sand. J. Hydraul. Div. ASCE 1970, 96, 619–632. [Google Scholar]
- Shields, A. Application of Similarity Mechanics and Turbulence Research on Bed Load Movement; Mitt. der Preussischen Versuchsanstalt fuer Wasser-, Erd- und Schiffbau Heft, Volume 26; RWTH Publications: Aachen, Germany, 1936. (In German) [Google Scholar]
- Duran, A.; Andreotti, B.; Claudin, P. Numerical simulation of turbulent sediment transport, from bed load to saltation. Phys. Fluids 2012, 24, 103306. [Google Scholar] [CrossRef]
- Duran, A.; Andreotti, B.; Claudin, P. Numerical Simulations of Turbulent Sediment Transport; Marine and River Dune Dynamics-Marid IV: Bruges, Belgium, 2013. [Google Scholar]
- Hanes, D.M.; Inman, D.L. Experimental evaluation of a dynamic yield criterion for granular fluid flows. J. Geophys. Res. Solid Earth 1985, 90, 3670–3674. [Google Scholar] [CrossRef]
- Chiew, Y.-M.; Parker, G. Incipient sediment motion on non-horizontal slopes. IAHR J. Hydraul. Res. 1994, 32, 649–660. [Google Scholar] [CrossRef]
- Maurin, R.; Chauchat, J.; Frey, P. Revisiting Slope Influence in Turbulent Bedload Transport; Cambridge University Press: Cambridge, UK, 2018. [Google Scholar]
- Sumer, M.; Kozakievicz, A.; Fredsoe, J.; Deigaard, R. Velocity and concentration profiles in sheet flow layer of movable bed. J. Hydraul. Eng. 1996, 122, 549–558. [Google Scholar] [CrossRef]
- Wilson, K.C. Analysis of Bed-Load Motion at high Shear Stress Properties on Transport Dynamics, Dynamics of Gravel Bed Rivers. J. Hydraul. Eng. 1987, 113, 97–103. [Google Scholar] [CrossRef]
- Recking, A.; Liébault, F.; Peteuil, C.; Jolime, T. Testing bedload transport equations with consideration of time scales. Earth Surf. Process. Landf. 2012, 37, 774–789. [Google Scholar] [CrossRef]
- Lajeunesse, E.; Malverti, L.; Charru, F. Bed load transport in turbulent flow at the grain scale: Experiments and modeling. J. Geophys. Res. 2010, 115, 1–13. [Google Scholar] [CrossRef]
- Houssais, M.; Lajeunesse, E. Bed load transport of a bimodal sediment bed. J. Geophys. Res. 2012, 117. [Google Scholar] [CrossRef]
- Nino, Y.; Garcia, M. Using Lagrangian particle saltation observations for bedload sediment transport modelling. Hydrol. Process. 1998, 12, 1197–1218. [Google Scholar] [CrossRef]
- Simons, D.B. Theory and Design of Stable Channels; Colorado State University Dept. of Civil Engineering: Fort Collins, CO, USA, 1957. [Google Scholar]
- Madsen, O.S.; Grant, W.D. Mechanics of Cohesionless Sediment Transport in Coastal Waters; Coastal Sediments’91; ASCE: New York, NY, USA, 1991. [Google Scholar]
- Ashida, K.; Michiue, M. Study on Hydraulic Resistance and Bed Load Transport Rate in Alluvial Streams. Proc. Jpn. Soc. Civ. Eng. 1972, 1972, 206. (In Japanese) [Google Scholar] [CrossRef]
- Zanke, U. Zum Übergang Hydraulisch Glatt—Hydraulisch Rauh (On the Transition Hydraulically Smooth-Hydraulically Rough); Wasser und Boden, Heft 10; RWTH Publications: Aachen, Germany, 1996. (In German) [Google Scholar]
- Reichardt, H. Complete representation of the turbulent velocity distribution in smooth pipes. ZAMM 1951, 31, 208–219. (In German) [Google Scholar] [CrossRef]
- Bagnold, R.A. The nature of saltation and of bed load transport in water. Ser. A Proc. R. Soc. Lond. 1973, 332, 473–504. [Google Scholar]
- Bridge, J.S.; Bennet, S. A model for the entrainment and transport of sediment grains. Water Resour. Res. 1992, 28, 337–363. [Google Scholar] [CrossRef]
- van Rijn, L.C. Sediment Transport, Part I: Bed Load Transport. J. Hydraul. Eng. 1984, 110, 1431–1456. [Google Scholar] [CrossRef]
- Parker, G. Hydraulic geometry of active gravel rivers. ASCE J. Hydraul. Eng. 1979, 105, 1185–1201. [Google Scholar]
- Soulsby, R. Dynamics of Marine Sands; Thomas: Telford, UK, 1997. [Google Scholar]
- Wong, M. Does the bedload equation of Meyer-Peter & Müller fit its own data? In Proceedings of the 30th Congress IAHR, Thessaloniki, Greece, 24–29 August 2003; pp. 78–80. [Google Scholar]
- Cheng, N.-S.; Chen, X. Slope Correction for Calculation of Bedload Sediment Transport Rates in Steep Channels. ASCE J. Hydraul. Eng. 2014, 140, 04014018. [Google Scholar] [CrossRef]
- Sekine, M.; Kikkawa, H. Mechanics of saltating grains II. J. Hydraul. Eng. 1992, 118, 536–558. [Google Scholar] [CrossRef]


| Meyer-Peter and Müller 1948/1949 | |||
| MPM mod. | |||
| Ashida and Michiue 1972 | |||
| Fernandez-Luque and v. Beek mod. 1976 | |||
| Engelund and Fredsoe 1976 | |||
| Bridge/Dominic 1984, Bridge/Hanes 85 | |||
| Madsen 1991 | |||
| Fredsoe and Deigaard 1992 | |||
| Zhang and McConnachie 1994 | |||
| Nino and Garcia 1998 | |||
| Zanke 1999/2001 | |||
| Parker, Seminarai and Solari 2002 | |||
| Lajeunesse, Malverti and Charru 2010 | |||
| Duran, Andreotti and Claudin 2012 | |||
| Zanke 2020 |
| = 8 | and considered, or with and fully considered | |
| = 1.28 | ||
| = | recommended by the authors: | |
| = 7.59 | ||
| = | for sand, after EF is from which results | |
| = | approach BD: | |
| = | ≈ for sliding grains; 9.5 for saltating grains | |
| = 9.55 | ||
| = | ||
| = | recommended by NG: | |
| = | = bed inclination angle | |
| = | ||
| = 10.6 | (Houssais & Lajeunesse2012: 56.6 ) | |
| = | ||
| = | R = risk of initial sediment motion |
| Term ‘b’ | Author |
|---|---|
| 1 | MPM, AM, LMC |
| MPM, FLB, EF, MA, FD, NG, PSS, ZMC, ZA99 | |
| BDH | |
| DAC, ZA20 |
| Author(s) | Hydraulic Conditions | Sediment | Effective | ||
|---|---|---|---|---|---|
| Rough | Transition and Smooth | Grain Size | Shear Stress | ||
| Meyer-Peter and Mueller | MPM | + | − | > mm | |
| Ashida and Michiue | AM (*) | + | − | >1 … 2 mm | |
| Ashida and Michiue | AM (**) | + | + | any, cohesion free | |
| Fernandez-Luque and v.Beek | FLB | + | − | >1 … 2 mm | |
| Engelund and Fredsoe | EF | + | − | >1 … 2 mm | |
| Bridge and Dominic/Hanes | BD, BDH (*) | + | − | >1 … 2 mm | |
| Bridge and Dominic Hanes | BD, BDH (**) | + | + | any, cohesion free | |
| Madsen | MA | + | − | >1 … 2 mm | |
| Fredsoe and Deigaard | FD | + | − | >1 … 2 mm | |
| Zhang and McConnachie | ZMC | + | − | >1 … 2 mm | |
| Nino and Garcia | NG | + | − | >1 … 2 mm | |
| Zanke 1999 | ZA99 | + | + | any, cohesion free | |
| Parker, Seminara and Solari | PSS (*) | + | − | >1 … 2 mm | |
| Parker, Seminara and Solari | PSS (**) | + | + | any, cohesion free | |
| Lajeunesse, Malverti and Charru | LMC | + | − | >1 … 2 mm | |
| Duran, Andreotti and Claudin | DAC | + | + | any, cohesion free | |
| Zanke | ZA | + | + | any, cohesion free | no limitation |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zanke, U.; Roland, A. Sediment Bed-Load Transport: A Standardized Notation. Geosciences 2020, 10, 368. https://doi.org/10.3390/geosciences10090368
Zanke U, Roland A. Sediment Bed-Load Transport: A Standardized Notation. Geosciences. 2020; 10(9):368. https://doi.org/10.3390/geosciences10090368
Chicago/Turabian StyleZanke, Ulrich, and Aron Roland. 2020. "Sediment Bed-Load Transport: A Standardized Notation" Geosciences 10, no. 9: 368. https://doi.org/10.3390/geosciences10090368
APA StyleZanke, U., & Roland, A. (2020). Sediment Bed-Load Transport: A Standardized Notation. Geosciences, 10(9), 368. https://doi.org/10.3390/geosciences10090368





