Electromagnetic Emissions from Quartz Subjected to Shear Stress: Spectral Signatures and Geophysical Implications
Abstract
1. Introduction
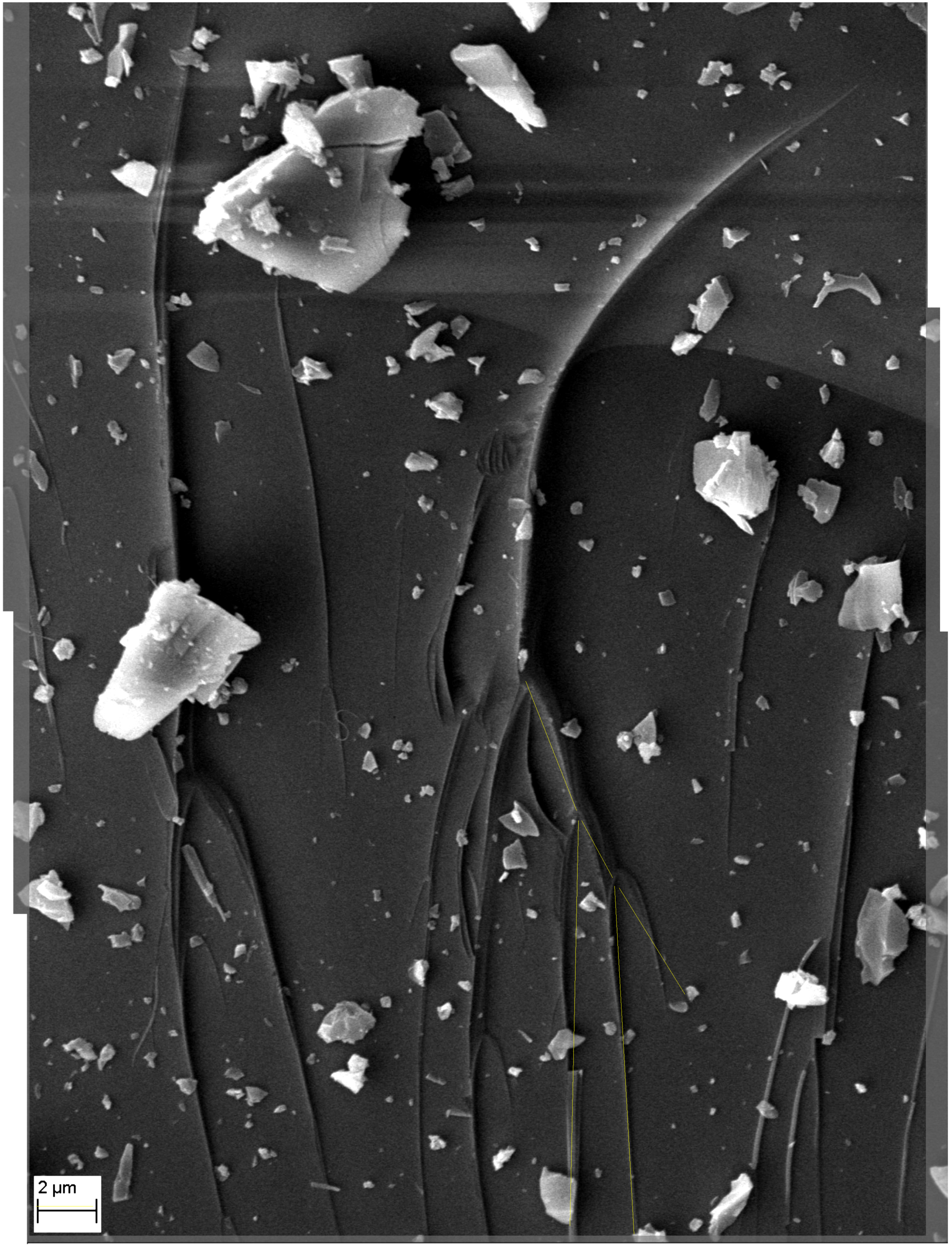
2. Experimental Study
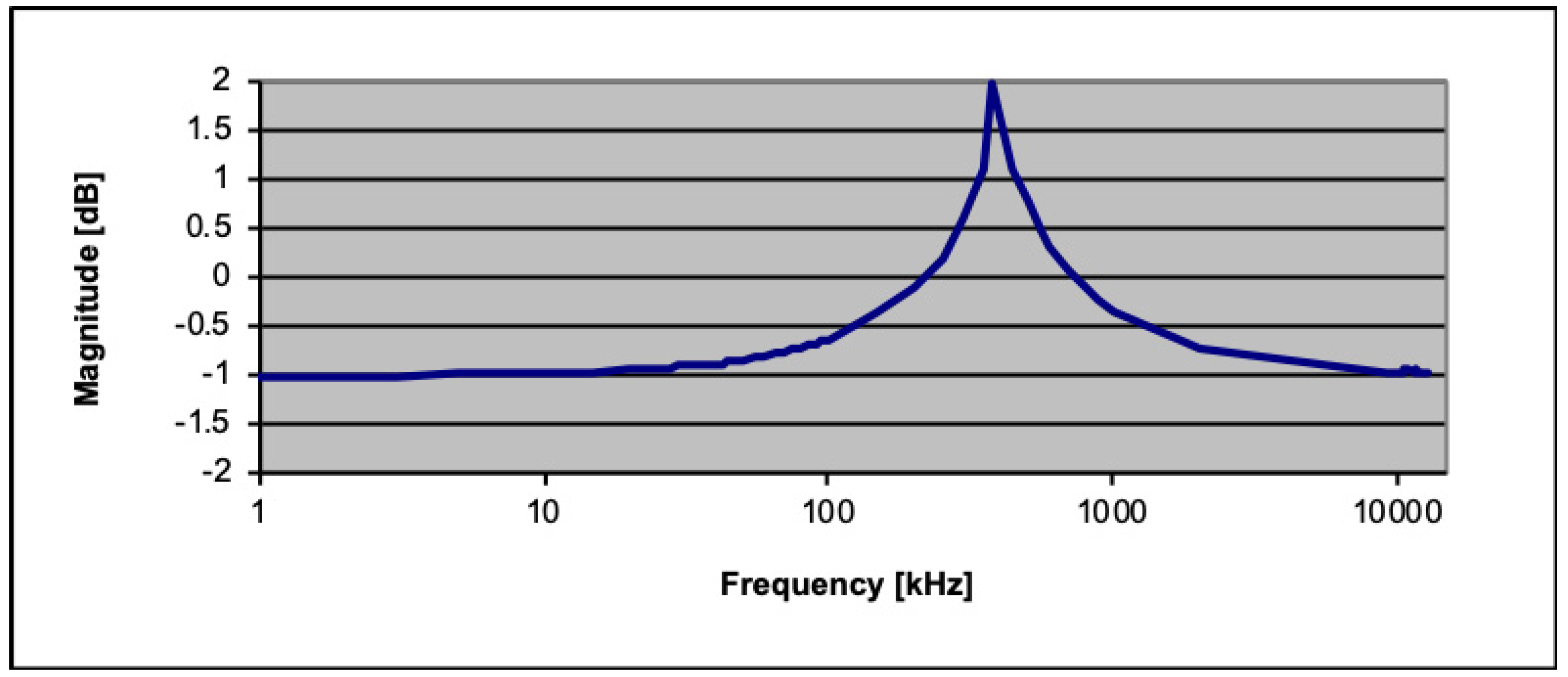
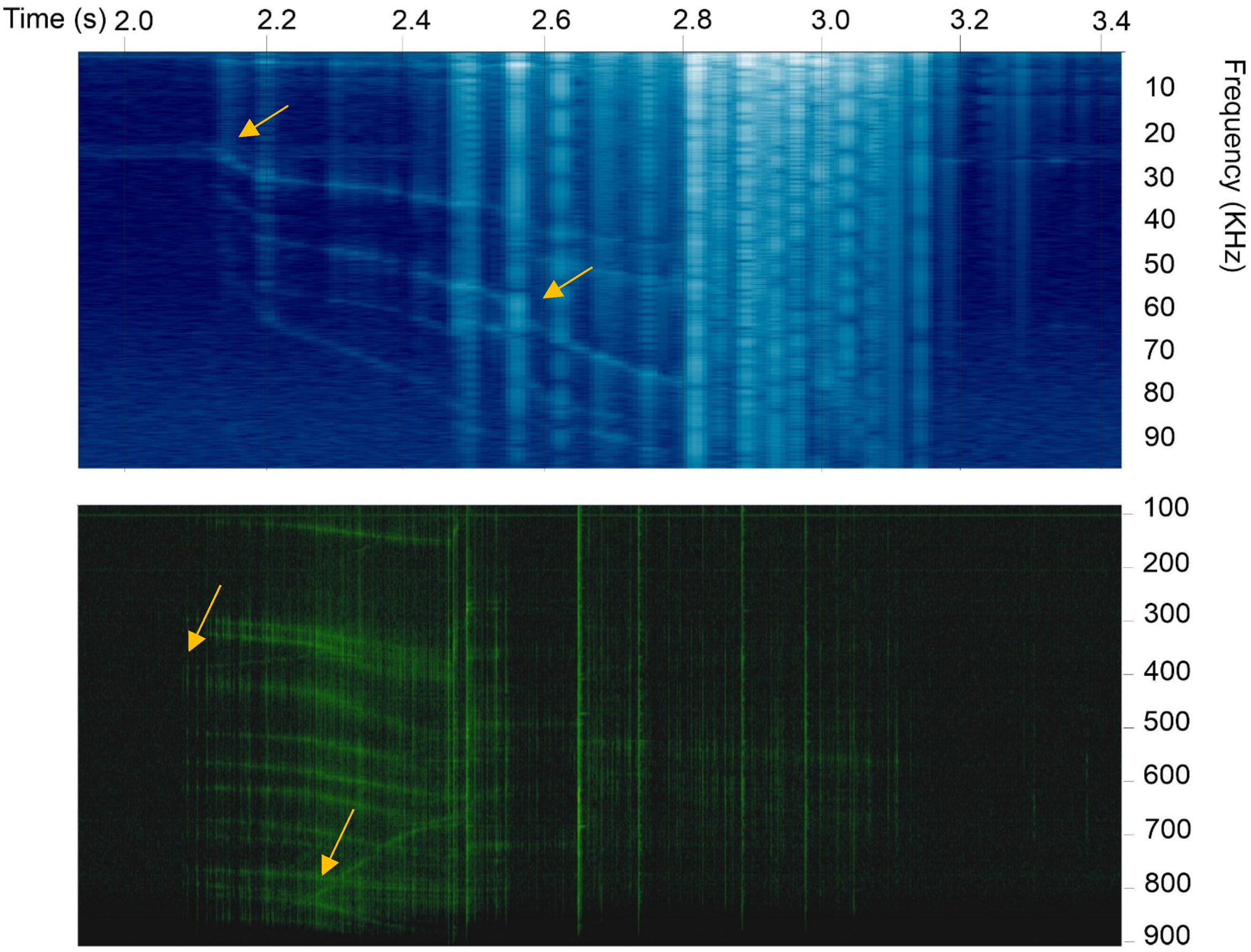
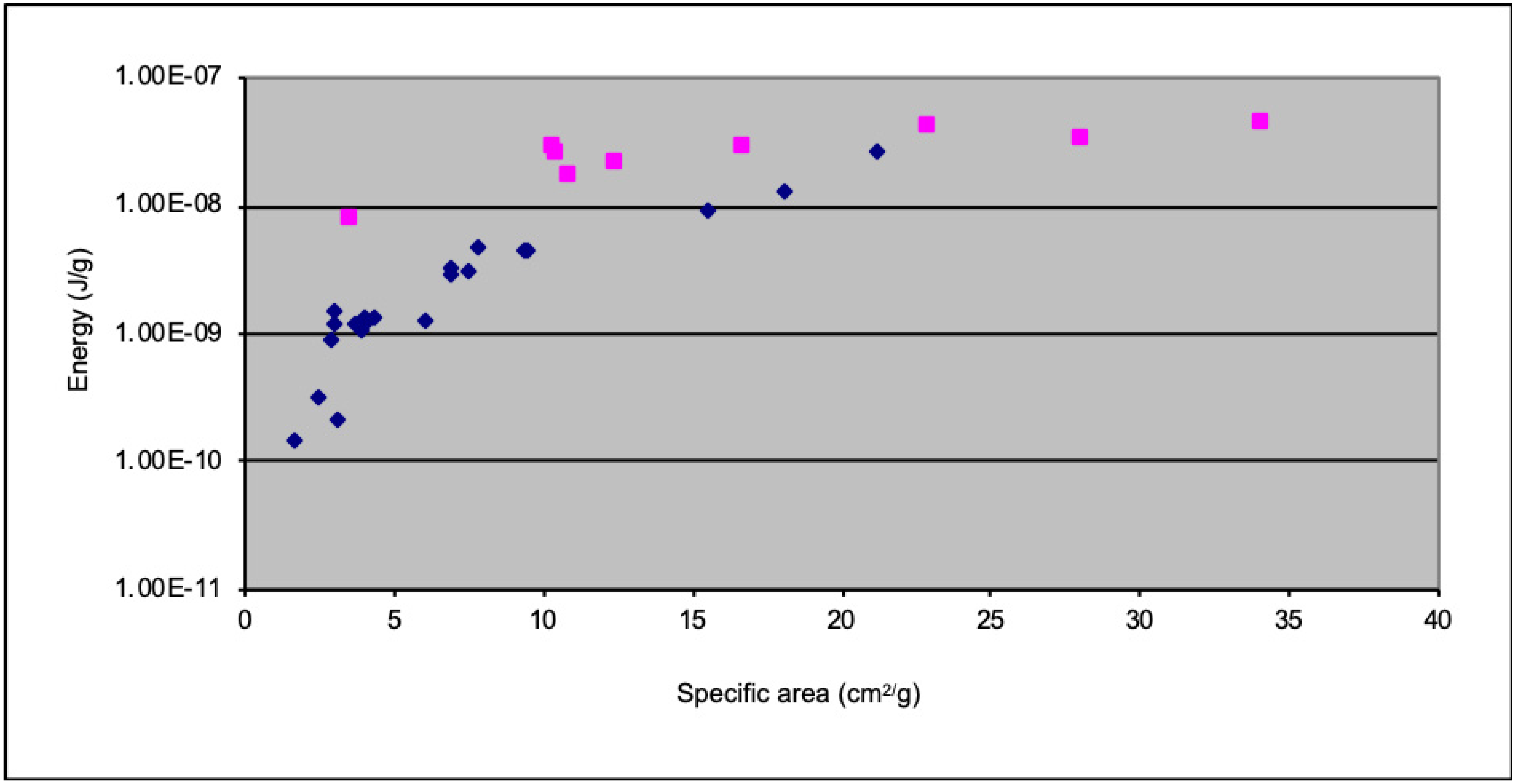
3. Results
4. Discussion of Data
5. Conclusions
Supplementary Materials
Author Contributions
Funding
Conflicts of Interest
Data Availability
References
- Uyeda, S.; Nagao, T.; Kamogawa, M. Short term earthquake prediction: Current status of seismo-electromagnetics. Tectonophysics 2009, 470, 205–213. [Google Scholar] [CrossRef]
- Carpinteri, A.; Borla, O. Fracto-Emissions as seismic precursors. Eng. Fract. Mech. 2017, 177, 239–250. [Google Scholar] [CrossRef]
- Hayakawa, M.; Asano, T.; Rozhnoi, A.; Solovieva, M. Very-Low to Low-Frequency Sounding of Ionospheric Perturbations and Possible Association with Earthquakes. In Pre-Earthquake Processes: A Multidisciplinary Approach to Earthquake Prediction Studies; Ouzounov, D., Pulinets, S., Hattori, K., Taylor, P., Eds.; Geophysical Monograph 234; Wiley-AGU: Hoboken, NJ, USA, 2018; pp. 273–304. [Google Scholar] [CrossRef]
- Potirakis, S.M.; Minadakis, G.; Eftakias, K. Analysis of electromagnetic pre-seismic emissions using Fisher information and Tsallis entropy. Phys. A Stat. Mech. Appl. 2012, 391, 300–306. [Google Scholar] [CrossRef]
- Vallianatos, F.; Triantis, D.; Tzanis, A.; Anastasiadis, C.; Stavrakas, I. Electric earthquake precursors: From laboratory results to field observations. Phys. Chem. Earth Parts A/B/C 2004, 29, 339–351. [Google Scholar] [CrossRef]
- Di Toro, G.; Hirose, T.; Nielsen, S.; Pennacchioni, G.; Shimamoto, T. Natural and Experimental Evidence of Melt Lubrication of Faults during Earthquakes. Science 2006, 311, 647–649. [Google Scholar] [CrossRef]
- Freund, F.T.; Takeuchi, A.; Lau, B.W.S. Elastic currents streaming out of stressed igneous rocks- A step towards understanding pre-earthquake low frequency EM emissions. Phys. Chem. Earth 2006, 31, 389–396. [Google Scholar] [CrossRef]
- Cress, G.O.; Brady, B.T.; Rowell, G.A. Sources of electromagnetic radiation from fracture of rock samples in the laboratory. Geophys. Res. Lett. 1987, 14, 331–334. [Google Scholar] [CrossRef]
- Enomoto, Y.; Hashimoto, H. Emission of charged particles from indentation fracture of rocks. Nature 1990, 346, 641–643. [Google Scholar] [CrossRef]
- O’Keefe, S.G.; Thiel, D.V. Mechanism for the production of electromagnetic radiation during fracture of brittle materials. Phys. Earth Planet. Inter. 1995, 89, 127–135. [Google Scholar] [CrossRef]
- Camara, C.G.; Escobar, J.V.; Hird, J.R.; Putterman, S.J. Correlation between nanosecond X-ray flashes and stick–slip friction in peeling tape. Nature 2008, 455, 1089–1092. [Google Scholar] [CrossRef]
- Rabinovitch, A.; Frid, V.; Bahat, D.; Goldbaum, J. Fracture area calculation from electromagnetic radiation and its use in chalk failure analysis. Int. J. Rock Mech. Min. Sci. 2000, 37, 1149–1154. [Google Scholar] [CrossRef]
- Goldbaum, J.; Frid, V.; Bahat, D.; Rabinovitch, A. An analysis of complex electromagnetic radiation signals induced by fracture. Meas. Sci. Technol. 2003, 14, 1839–1844. [Google Scholar] [CrossRef]
- Tromans, D.; Meech, J.A. Fracture toughness and surface energies of minerals: Theoretical estimates. Miner. Eng. 2002, 17, 1–15. [Google Scholar] [CrossRef]
- Tromans, D. Mineral comminution: Energy efficiency considerations. Miner. Eng. 2008, 21, 613–620. [Google Scholar] [CrossRef]
- Fuerstenau, D.W.; Abouzeid, A.Z.M. The energy efficiency of ball milling in comminution. Int. J. Min. Process. 2003, 67, 161–185. [Google Scholar] [CrossRef]
- Rumpf, H. Physics aspects of comminution and new formulation of a law of comminution. Powder Technol. 1973, 7, 21–26. [Google Scholar] [CrossRef]
- Tavares, L.M.; King, R.P. Single particle fracture under impact loading. Int. J. Miner. Process. 1998, 54, 1–28. [Google Scholar] [CrossRef]
- Tavares, L.M. Optimum routes for particles breakage by impact. Powder Technol. 2004, 142, 81–91. [Google Scholar] [CrossRef]
- Tugcam Tuzcu, E.; Rajamani, R.K. Modeling breakage rates in mills with impact energy spectra and ultra fast load cell data. Miner. Eng. 2011, 24, 252–260. [Google Scholar] [CrossRef]
- Plescia, P.; Tempesta, E. Analysis of Friction coefficient in a vibrating cup mill (ring mill) during grinding. Tribol. Int. 2017, 114, 458–468. [Google Scholar] [CrossRef]
- Aman, S.; Tomas, J.; Chaikina, M. Structure modification and Mechanoluminescence of Quartz. Chem. Sustain. Dev. 2005, 2, 125–130. [Google Scholar]
- Thériault, R.; St-Laurent, F.; Freund, F.T.; Derr, J.S. Prevalence of Earthquake Lights Associated with Rift Environments. Seismol. Res. Lett. 2014, 85, 159–178. [Google Scholar] [CrossRef]
- Bremmer, H. Terrestrial Radio Waves; Elsevier: Amsterdam, The Netherlands, 1949. [Google Scholar]
- Field, F.C.; Dore, M. Electromagnetic Communication in the Earth’s Crust, Pacific-Sierra Research Corp. Santa Monica, California. Available online: https://pdfs.semanticscholar.org/e89c/d0080beeb48d686bc66cece92372154e9fef.pdf (accessed on 11 April 2020).
- Hermance, J.F. Electrical Conductivity Models of the Crust and mantle. In Global Earth Physics A Handbook of Physical Constants; American Geophysical Union: Washington, DC, USA, 1995; Volume 1. [Google Scholar] [CrossRef]
- Wheeler, H. Radio-Wave propagation in the Earth’s Crust. J. Res. Natl. Bur. Stand. Sect. D Radio Propag. 1961, 65, 189. [Google Scholar] [CrossRef]
- Safari, A.; Akdoğan, K.A. Piezoelectric and Acoustic Materials for Transducer Applications; Springer: Boston, MA, USA, 2008. [Google Scholar]
- Brice, J.C. Crystals for quartz resonators. Rev. Mod. Phys. 1985, 57, 105–146. [Google Scholar] [CrossRef]
- Cambon, O.; Haines, J.; Keen, D.A.; Tucker, M.G.; Dove, M.T. Structural disorder and loss of piezoelectric properties in a-quartz at high temperature. Appl. Phys. Lett. 2002, 81, 2968–2970. [Google Scholar]
- Cambon, O.; Haines, J.; Fraysse, G.; Keen, D.A.; Tucker, M.G. Piezoelectric properties at high temperature in a-quartz materials. J. Phys. Colloq. 2005, 126, 27–30. [Google Scholar] [CrossRef]
- Weichert, R.; Schonert, K. Heat generation at the tip of a moving crack. J. Mech. Phys. Solids 1978, 26, 151–161. [Google Scholar] [CrossRef]
- Pandey, K.N.; Chand, S.N. Analysis of temperature distribution near the crack tip under constant amplitude loading. Fatigue Fract. Eng. Mater. Struct. 2008, 31, 316–326. [Google Scholar] [CrossRef]
| Test | Electrical Parameters | Frequency, kHz | |||
|---|---|---|---|---|---|
| 0.1 | 1 | 10 | 100 | ||
| Empty cell | L [μH] | 586 | 474 | 373 | 335 |
| C [pF] | 102 | 101 | 106 | 106 | |
| Cell filled with 1 g of quartz | L [μH] | 523 | 452 | 373 | 336 |
| C [pF] | 98 | 98 | 102 | 102 | |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Martinelli, G.; Plescia, P.; Tempesta, E. Electromagnetic Emissions from Quartz Subjected to Shear Stress: Spectral Signatures and Geophysical Implications. Geosciences 2020, 10, 140. https://doi.org/10.3390/geosciences10040140
Martinelli G, Plescia P, Tempesta E. Electromagnetic Emissions from Quartz Subjected to Shear Stress: Spectral Signatures and Geophysical Implications. Geosciences. 2020; 10(4):140. https://doi.org/10.3390/geosciences10040140
Chicago/Turabian StyleMartinelli, Giovanni, Paolo Plescia, and Emanuela Tempesta. 2020. "Electromagnetic Emissions from Quartz Subjected to Shear Stress: Spectral Signatures and Geophysical Implications" Geosciences 10, no. 4: 140. https://doi.org/10.3390/geosciences10040140
APA StyleMartinelli, G., Plescia, P., & Tempesta, E. (2020). Electromagnetic Emissions from Quartz Subjected to Shear Stress: Spectral Signatures and Geophysical Implications. Geosciences, 10(4), 140. https://doi.org/10.3390/geosciences10040140






