A Two-Segment Continuum Robot with Piecewise Stiffness for Tracheal Intubation and Active Decoupling

Abstract

This study presents a two-segment continuum robot with piecewise stiffness, designed to enhance the precision, adaptability, and safety of tracheal intubation procedures. The robot employs a continuum manipulator (CM) as its end-effector, featuring a proximal segment (PS) with an aluminum alloy interlocking joint, which provides high axial stiffness for stable insertion, and a distal segment (DS) with a micro-nano resin-based notched structure, offering increased flexibility and compliance to navigate complex anatomical structures such as the epiglottis and vocal cords, thereby reducing airway trauma. To describe the motion behavior of the robot, a piecewise variable curvature kinematic model is developed, capturing the deformation characteristics of each segment under actuation. Furthermore, a piecewise stiffness analysis is conducted to determine the axial and bending stiffness of each segment, ensuring an appropriate balance between stability and flexibility. To enhance control precision, an active tendon-driven decoupling control strategy is introduced, effectively minimizing the interaction forces between flexible segments and improving end-effector maneuverability. The results demonstrate that the proposed design significantly improves the adaptability of the tracheal intubation robot, ensuring controlled insertion while reducing the risk of excessive force on the airway walls. This study provides theoretical and technical insights into the mechanical design and control strategies of continuum robots, contributing to the safety and efficiency of tracheal intubation.

1. Introduction

Endotracheal intubation is one of the most effective methods for establishing an artificial airway. It involves the insertion of a specialized tracheal tube through the oral or nasal cavity, passing through the glottis into the trachea. This technique ensures optimal conditions for maintaining airway patency, ventilation, oxygenation, and airway suction, making it a critical measure for rescuing patients with respiratory dysfunction. It is widely used in emergency medicine, anesthesia, and critical care monitoring to maintain effective respiratory function in patients, prevent aspiration, and improve prognosis [1]. The current global annual surgical volume exceeds 313 million cases, with general anesthesia accounting for approximately 80%. Tracheal intubation is the primary airway establishment method used after general anesthesia [2,3]. Nasal intubation is a procedure in which the endotracheal tube is inserted into the trachea through the nasal cavity. It can be used in conscious and unsedated patients, and the tube fixation is simpler. However, it requires high-level technical skills. During the intubation process, it may cause injuries to the posterior pharyngeal wall and turbinate bones and increase the risk of sinusitis. Oral intubation is a procedure in which the endotracheal tube is placed into the trachea through the oral cavity. It is relatively easy to perform, has a fast intubation speed, and causes less pain to the patient. Nevertheless, the tube is prone to displacement or even accidental extubation. Long-term indwelling may affect the patient’s swallowing and chewing functions [4]. Consequently, orotracheal intubation is the more commonly employed technique in clinical practice.

TI techniques have evolved significantly alongside advances in clinical airway management. Initial approaches relied on blind insertion using rigid metal tubes or stylets, guided solely by tactile feedback and clinician experience. The introduction of direct laryngoscopes improved visual access to the glottis, enhancing procedural safety. More recently, video laryngoscopes and bronchoscopes have become mainstream for difficult airway management due to their superior visualization. Despite these advancements, current tools still face limitations in flexibility and control, particularly in complex or emergency cases. As such, bronchoscopes are now considered the preferred option for navigating challenging airways.

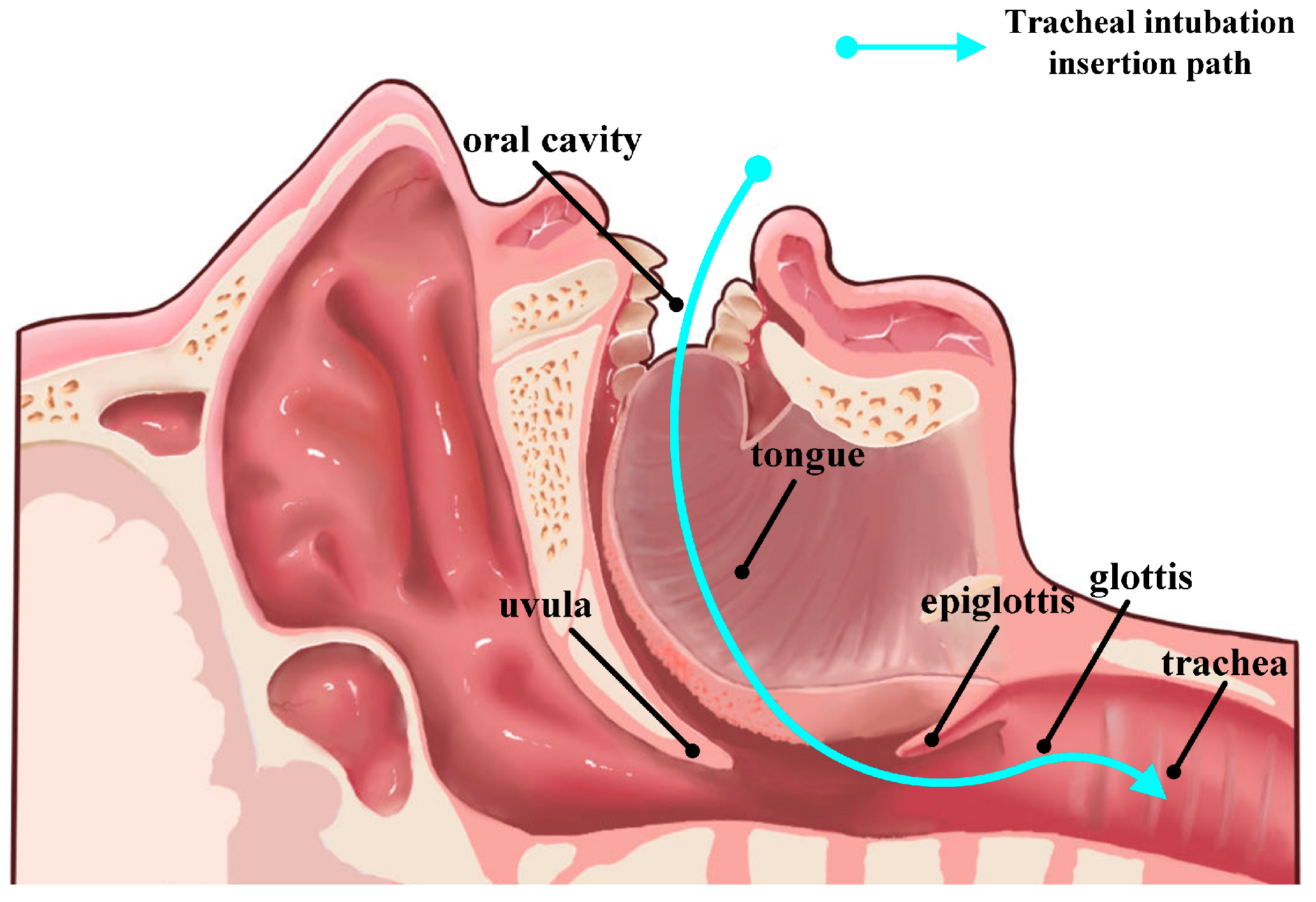
As illustrated in Figure 1, it demonstrates the anatomical structure and path of tracheal intubation using a bronchoscope. The insertion process involves passing through multiple anatomical landmarks in a sequential manner, including the uvula, epiglottis, vocal cords, and trachea. When the imaging system at the distal end of the bronchoscope provides a clear view of the carina, the endotracheal tube is advanced along the bronchoscope into the patient’s airway until an appropriate depth is reached (typically 22–24 cm in males and 20–22 cm in females). Subsequently, the bronchoscope is gently withdrawn, and the endotracheal tube is secured [5]. During this process, the trajectory of the bronchoscope typically follows a “hook-shaped” path. The key to successful intubation lies in the precise manipulation of the bronchoscope to navigate past the epiglottis and smoothly guide the endotracheal tube through the vocal cords into the trachea, while minimizing trauma to the airway structures.

With the continuous advancement of robotics technology, medical robots have demonstrated significant application potential in surgery, rehabilitation, and nursing care [6,7,8,9]. In recent years, robot-assisted TI has emerged as a prominent research focus, as it leverages the inherent dexterity, precision, and controllability of robotic systems to enable physicians to establish the airway more efficiently and accurately [10]. Such systems not only help alleviate the workload of medical personnel but also enhance the accuracy and success rate of intubation. Moreover, with the potential for remote and automated operation, they demonstrate significant application value in complex or high-risk airway scenarios. In early robot-assisted intubation systems, mechanical arms were used to support commercially available video laryngoscopes or bronchoscopes for direct insertion of the endotracheal tube into the patient’s airway through the oral cavity [11,12,13]. The primary advantage of such robotic systems lies in their simpler design, cleaner insertion process, and more convenient tool interchangeability and control [14]. However, due to the rigid structure of commercial video laryngoscopes, traditional support-based TIRs exhibit limited flexibility and adaptability in complex airway environments, restricting their motion capabilities and functional expansion. To address these limitations, researchers have conducted autonomous design studies on the end-effectors with increased degrees of freedom for TIRs. Wang et al. [15] proposed a portable teleoperated TIR equipped with a laryngoscope-like tongue depressor. It delivers the endotracheal tube via a pulley mechanism and adjusts the bending direction of the tube through the telescopic motion of rigid linkages. Boehler et al. [16] developed a two-degree-of-freedom variable-curvature TIR, whose end-effector is an interlocking articulated CM. By gradually reducing the gap between adjacent joints, the curvature of the joints is increased, enabling more agile rotation near the glottis. The interlocking design also enhances the structural stability of the manipulator. Additionally, Liu et al. [17] developed a pneumatically actuated TIR, where the CM comprises two different types of polymer materials fabricated using 3D-printed molds with an embedded lumen to facilitate airflow. To improve structural rigidity, reinforced fibers were embedded within the internal structure, providing enhanced elasticity and controllability. Furthermore, Liu et al. [18] introduced a NasoTIR, whose CM shares a similar internal structure to commercial bronchoscopes but features an 80 mm-long single-degree-of-freedom section with a 5 mm outer diameter, achieving ±110° bending through actuation via a 0.3 mm pneumatic channel. This design enables the seamless integration of the CM’s distal structure with detachable flexible guiding elements, facilitating a rapidly adaptable structural configuration. Lastly, Yang et al. [19] designed a three-degree-of-freedom continuum TIR, in which the CM consists of two flexible segments with different stiffness. The high-stiffness flexible segment adopts an articulated structure driven by elastic rods, with an internal rigid rod that enables variable bending length. The low-stiffness flexible segment utilizes a tendon-driven ball-and-socket structure. This piecewise stiffness design effectively reduces inter-segment coupling errors.

To date, continuum robots have been widely applied in various surgical scenarios, including laparoscopic surgery [20,21], pulmonary interventional surgery [22,23], and superior laryngeal nerve surgery [24]. The differences in target anatomical structures across different surgical environments have led to diverse design approaches for continuum robots’ CMs. For instance, Pan et al. [25] developed a notch-based CM for laparoscopic surgical robots, which offers several advantages, including ease of fabrication, enhanced flexibility, and improved compliance. Moreover, by adjusting the spacing between notch segments, the stiffness of flexible sections can be optimized to accommodate external loading requirements. Similarly, Hong et al. [26] designed a CM for endoscopic sinus surgery (ESS), which consists of a PS and a DS with distinct flexibility characteristics. The PS employs a single-degree-of-freedom articulated structure, characterized by greater length and higher rigidity, while the DS features a two-degree-of-freedom spherical structure, offering greater flexibility and maneuverability. These differential stiffness configurations and distinct bending unit structures significantly enhance adaptability in complex surgical environments. These CM design concepts provide valuable insights for the development of TIR end-effectors. To comprehensively and intuitively present the variations in the CM design parameters among contemporary medical robots, a comparative analysis has been conducted, as detailed in

Table 1. Comparison of the CM design parameters in current medical robots.

Source	Year	App	NoS	Materials	JF	D	L	DOF	DF
[16]	2020	TI	1	Metal	Interlocking articulated joint	5.5	/	2	tendon-driven
[17]	2024	TI	1	Silicone	Soft joint	11	40	2	hydraulic-driven
[18]	2023	TI	1	Metal	Articulated joint	5	80	2	tendon-driven
[19]	2024	TLS	2	Metal	Articulated joint + Spherical joint	6.5	104	3	tendon-driven
[25]	2024	LS	3	Metal	Notched joint	8	150	6	tendon-driven
[26]	2022	ESS	2	Metal	Spherical joint	4.5	100	5	tendon-driven
Ours	2025	TI	2	Metal + Resin	Semicircular joint + Notched joint	6.5	142	4	tendon-driven

App: Application; NoS: number of segments; JF: joint form; D: diameter (mm); L: length (mm); DOF: degree of freedom; DF: driving form.

.

In summary, [15] lacks a CM in its “track-based” guidance system, which directly inserts the endotracheal tube into the airway. This approach leads to significant human–machine interaction forces, increasing the risk of airway trauma for the patient. References [16,17,18] present a single-segment CM in TIR applications, where the number of motion degrees of freedom typically does not exceed two (either two bending degrees of freedom or one bending plus one rotational degree of freedom). Although these systems can achieve gradient bending by differentiated maximum bending angles across the CM units, enabling adaptation to the natural curvature of the airway, the limited length of a single-segment flexible structure relative to the overall insertion path necessitates the inclusion of a relatively long non-controllable flexible section, making it difficult to achieve precise distal control. Reference [19] employs a two-segment structure; however, the number of degrees of freedom of the flexible section is only one, which imposes strict constraints on the insertion trajectory, reducing feasibility in practical operations. Additionally, notch-based CMs exhibit high flexibility, are easy to manufacture, and feature small notch spacing, ensuring appropriate stiffness while providing excellent compliance and biocompatibility. These characteristics enable them to adapt to airway shape variations, thereby reducing the risk of patient injury. Beyond differences in the number of flexible segments, variations in the CM performance primarily stem from differences in design materials and joint structures. In TIR research, multi-segment CM designs based on differentiated material strategies remain relatively unexplored, as most studies employ a unified material approach. For instance, in the study of [18], all flexible segments are constructed using metallic materials, whereas, in the work of [17], the flexible segments are entirely composed of silicone-based materials. Furthermore, the design of flexible segment joints primarily serves to enable curved bending motion. Common joint structures include skeleton-based compliant mechanisms [27], spherical joints [28], interlocking joints [29], rigid ring-stacked joints [30], notched joints [25], and double-layer compliant joints [31].

In this paper, a novel two-segment, four-degree-of-freedom CM is proposed. The PS adopts an interlocking structure, consisting of aluminum alloy rigid ring units arranged in an orthogonal serial configuration. The DS is shorter than the PS and employs a notch-based design, fabricated using 3D-printed micro-nano resin material with an integrated molding process, where notch segments are arranged in an alternating configuration. Each flexible segment has two independently controlled bending degrees of freedom, enabling motion in both coronal and sagittal planes. The differences in materials and structures among the bending units of the flexible segment facilitate differentiated stiffness design, accommodating both large-angle bending and dexterous distal manipulation of the CM.

The remainder of this paper is organized as follows: Section 2 provides a detailed description of the TIR structural design, including the CM and actuation system. Section 3 presents kinematic modeling and workspace analysis of the continuum manipulator, incorporating analyses of tendon friction and tendon passage mechanics, segmental stiffness, and multi-segment coupling. Section 4 discusses experimental studies, evaluating the CM’s motion performance, including stiffness testing, coupling experiments, and in vitro tracheal simulation tests. 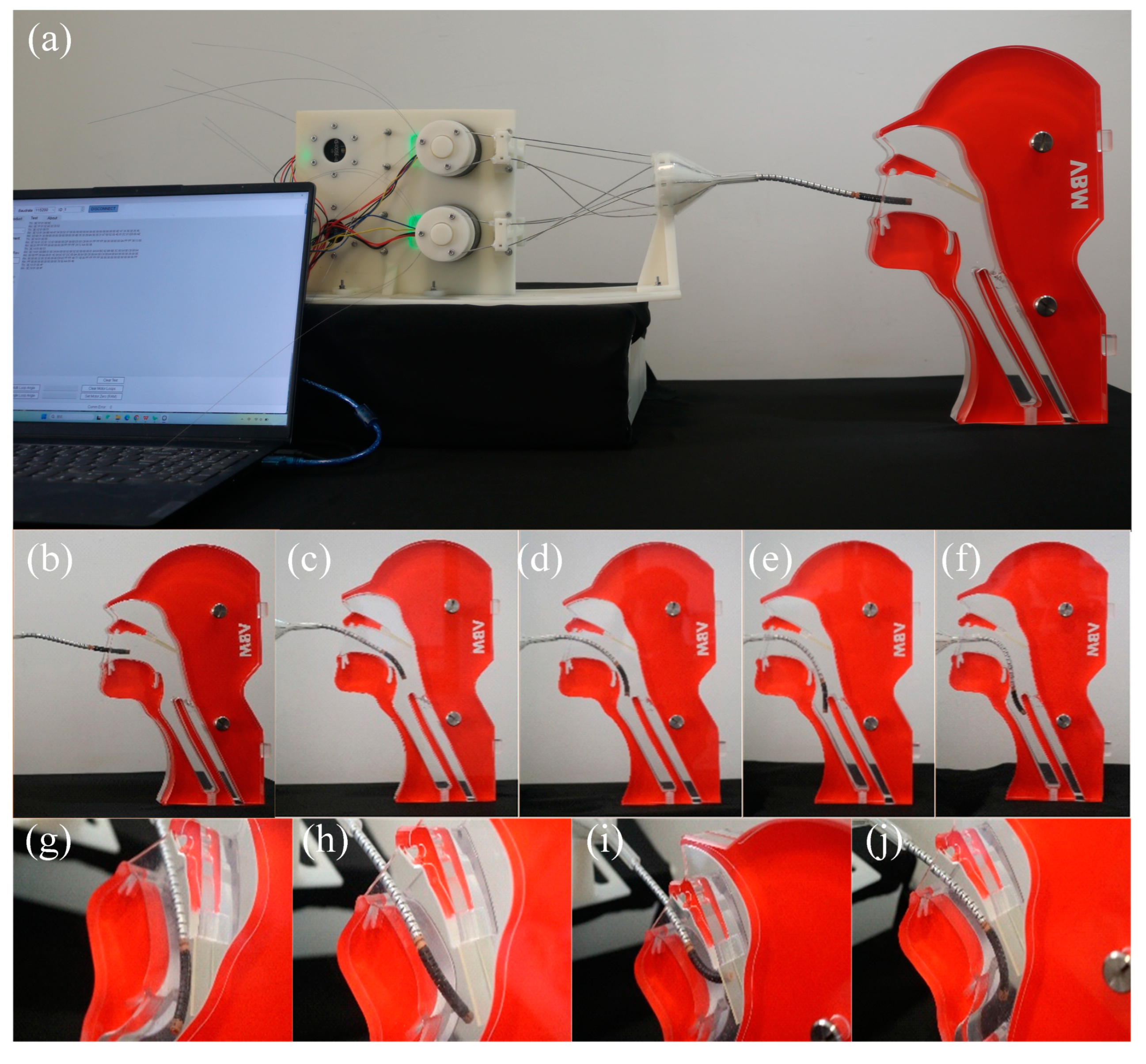Finally, Section 5 presents practical validation experiments using real human head models, demonstrating the bending capabilities, load-bearing capacity, and feasibility of TIR applications.

2. Design of the TIR

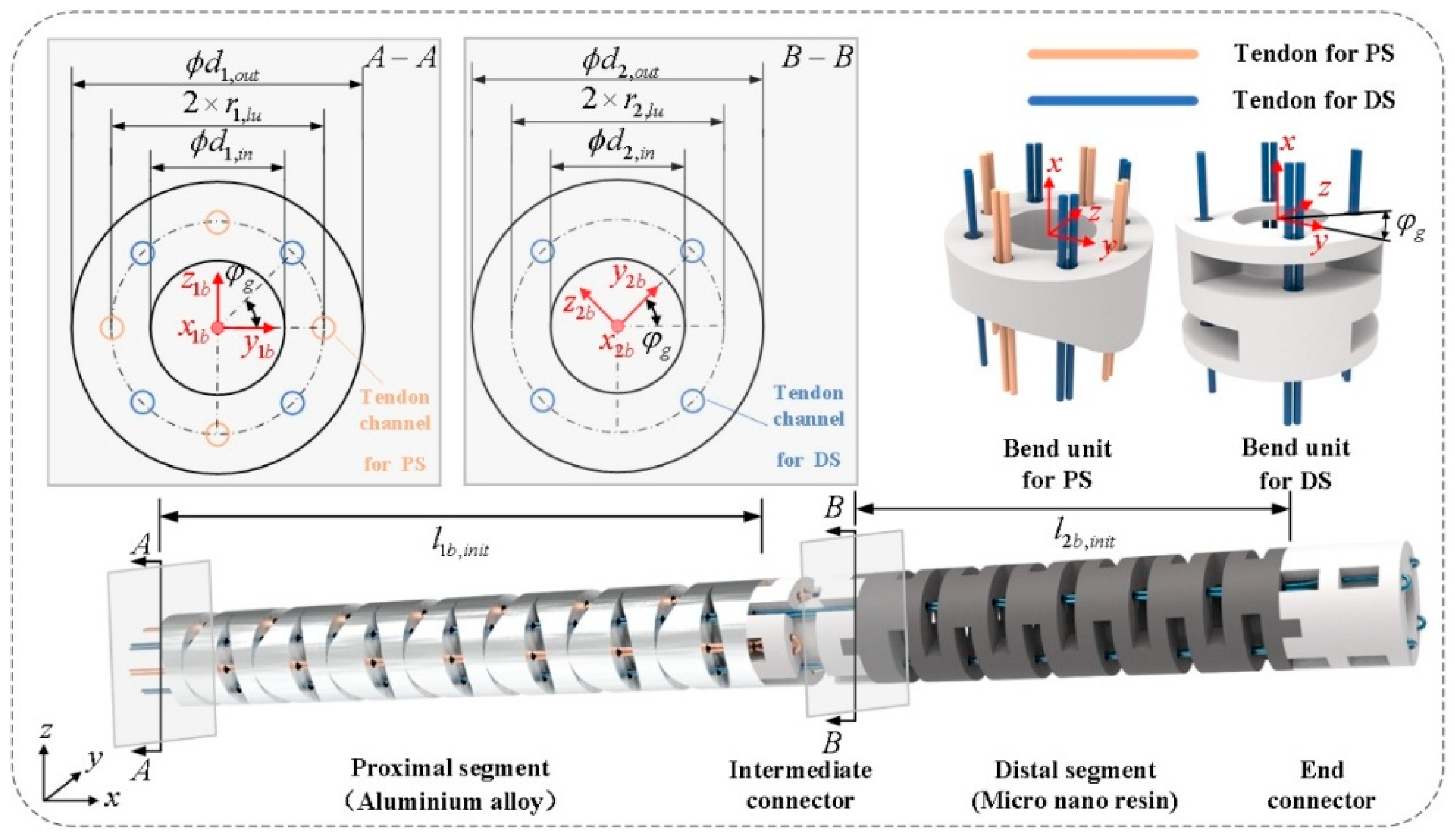
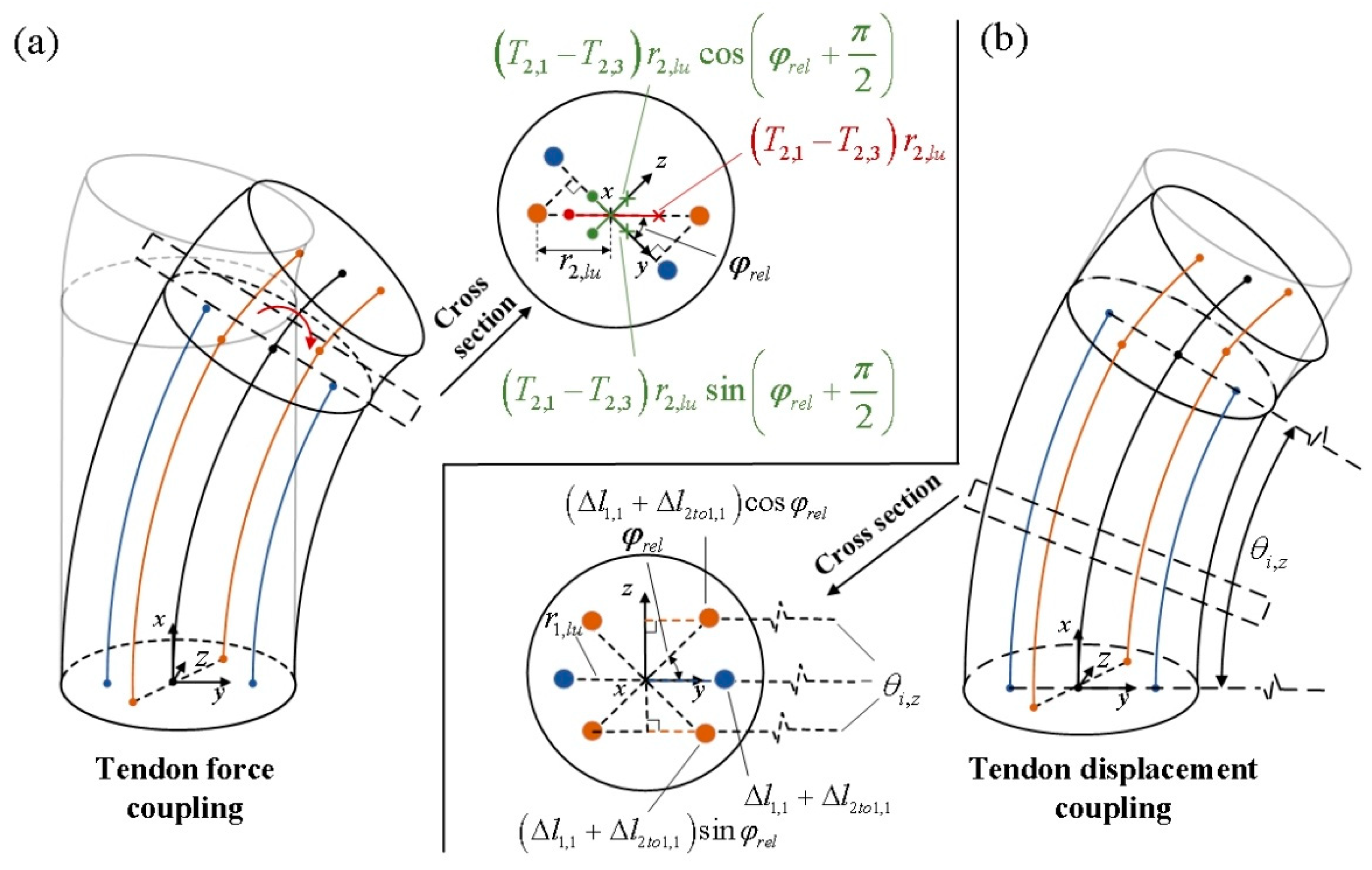
The proposed TIR consists of two main components: the CM and its actuation system. The CM is composed of the PS, the DS, the intermediate connector (IC), and the end connector (EC), forming a two-segment, four-degree-of-freedom CM. Both the PS and DS possess two independent degrees of freedom in angular deflection, as illustrated in Figure 2. The specific parameters and values of the CM are detailed in

Table 2. Design parameters of the CM.

Part	Parameters	Physical Meaning	Value	Unit
PS	$l_{1b,init}$	The original length of the segment body	90	mm
	$\varphi d_{1,in}$/$\varphi d_{1,out}$	Inside diameter and outside diameter	3/6.5	mm
	$r_{1,lu}$	Radial distance from the lumen to center line	2.375	mm
	$\varphi d_{1,lu}$/$\varphi d_{1,tdn}$	The diameter of lumens and tendons	0.6/0.25	mm
	$n_1$/$h_1$	The number and height of the bending units	30/3	1/mm
	$n_{1,tdn}$	The number of tendons	4	1
	$h_{1,b}$/$h_{1,c}$	The height of the semicircle and the height of the ring	1.5/1.5	mm
	${\phi }_1$	The bending plane angle of the segment	$\left[0,{360}^{\circ }\right]$	rad
	${\theta }_1$	The bending angle of the segment	$\left[0,{110}^{\circ }\right]$	rad
DS	$l_{2b,init}$	The original length of the segment body	40	mm
	$\varphi d_{2,in}$/$\varphi d_{2,out}$	Inside diameter and outside diameter	3/6.5	mm
	$r_{2,lu}$	Radial distance from the lumen to center line	2.375	mm
	$\varphi d_{2,lu}$/$\varphi d_{2,tdn}$	The diameter of lumens and tendons	0.6/0.25	mm
	$n_2$/$h_2$	The number and height of the bending units	20/2	1/mm
	$n_{2,tdn}$	The number of tendons	4	1
	$h_{2,b}$/$h_{2,c}$	The height of the semicircle and the height of the ring	1/1	mm
	${\phi }_2$	The bending plane angle of the segment	$\left[0,{360}^{\circ }\right]$	rad
	${\theta }_2$	The bending angle of the segment	$\left[0,{120}^{\circ }\right]$	rad
	${\phi }_{rel}$	The initial relative angle of the lumen of the DS relative to the lumen of the PS about the x-axis.	$\pi /4$	rad
IC	$h_{IC}$	The height of the connecting component	6	mm
EC	$h_{EC}$	The height of the end connecting component	6	mm

.

Considering that the two flexible segments need to perform different functions during the TI process, the PS is primarily responsible for providing structural support and stability, while addressing the issue of the DS being too short to reach the trachea. In contrast, the DS focuses on enhancing flexibility and ensuring smooth interaction with surrounding anatomical structures, particularly in navigating the vocal cords without excessive compression. To optimize the collaborative functionality of these two segments, the proposed CM employs distinct materials and joint configurations for the PS and DS. Specifically, the PS utilizes semi-circular stacked joints composed of aluminum alloy, where each unit maintains line contact between the lower convex surface of the trailing unit and the upper flat surface of the preceding unit, enabling single-directional bending. By arranging multiple joints in an orthogonally interleaved configuration, the PS achieves independent bending in two perpendicular directions. This design effectively reduces the cumulative impact of frictional forces from relative rotational motion, thereby minimizing the overall actuation force requirements while improving control precision. In addition, the DS adopts a flexible notch-based joint design fabricated from micro-nano resin material. Each bending unit consists of a symmetrical U-shaped notch pair, and multiple units are arranged in an orthogonally adjacent pattern. Compared to the original design with a single-direction notch, this configuration allows independent bending in two perpendicular directions, adding an extra degree of freedom for bending. As a result, the design significantly expands the workspace of the system, greatly enhancing its dexterity. Additionally, by leveraging the unique properties of micro-nano resin materials, the DS achieves a balance between flexibility and sufficient stiffness. This combination ensures excellent compliance, while maintaining the necessary rigidity, effectively reducing the risk of mechanical trauma to surrounding biological tissues and meeting the requirements for medical applications.

The three-dimensional structure of the bending units in the PS and DS is illustrated in Figure 2. Each bending unit of PS contains a total of nine lumens, consisting of one central lumen and eight peripheral lumens. The central lumen, located at the geometric center of the bending unit and coaxial with the outer cylindrical surface, is designed to potentially accommodate the distal micro-imaging sensor, LED data transmission cables, and the working channel, offering flexibility for future integration of such components. The eight peripheral lumens are evenly spaced at 45° intervals along the inner walls of the PS bending unit, with four serving as tendon actuation channels for PS and the remaining four dedicated to DS tendon actuation. In contrast, each bending unit of the DS is designed with only five lumens, including four peripheral lumens that serve as the DS tendon actuation channels. The uniform and symmetric lumen distribution not only enhances the structural stability of the CM but also ensures that the PS and DS maintain consistent mechanical properties in all directions, thereby meeting the functional requirements for isotropic performance during operation. Given that the CM actuation relies on frequent tendon displacement for real-time positional adjustments, NiTi alloy wires were ultimately selected due to their superelasticity and flexibility. The tendons of PS sequentially pass through its tendon actuation channels, looping around the pre-set pulley structure at the lower end of IC before folding back, forming a “single-path, double-tendon” configuration. This dual-tendon support structure effectively enhances the stiffness of PS. Since the tension in PS tendons is entirely applied to PS, its influence on the DS can be neglected. Similarly, the DS tendons follow the same routing pattern and terminate at EC, where their tension directly actuates the DS while also inducing secondary effects on PS. Regardless of whether the PS or DS is being actuated, all four tendons are arranged in opposing pairs, ensuring precise antagonistic force control to achieve stable and isotropic bending mechanics in both segments.

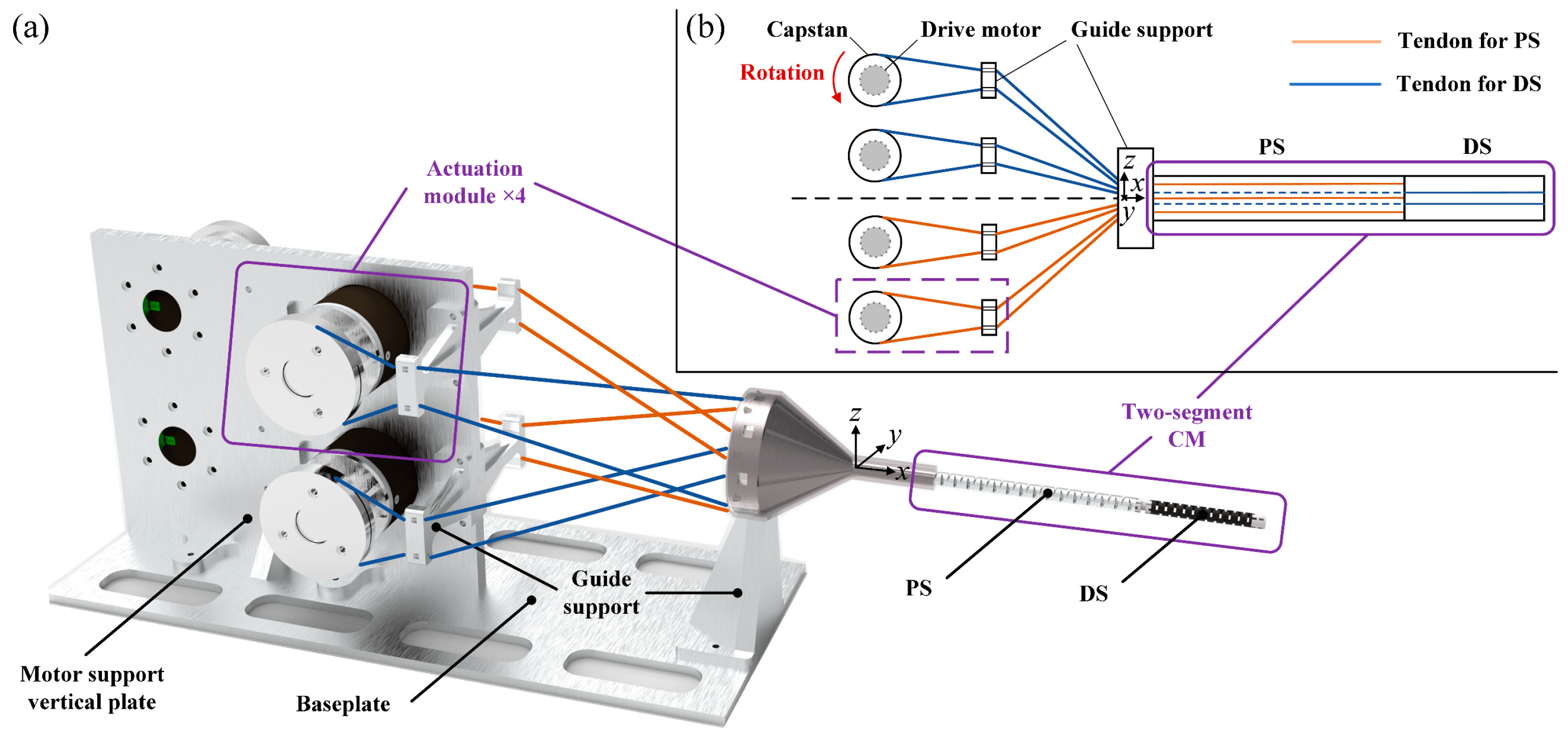
The actuation system of the CM is illustrated in Figure 3. The actuation system primarily consists of a base plate, motor support frame, guiding seat, and actuation module assembly. The actuation module comprises four independent actuation submodules, each consisting of a brushless servomotor and a spool. The proximal end of the CM is connected to the compliant beam at the distal end of the guiding seat, while the tendon origins pass through the guiding seat and are linked to their respective spools. Each spool has two opposing tendons attached at its ends. Under the actuation of brushless servomotors, the spools precisely regulate angular positioning, enabling accurate tendon displacement control. By independently driving the four spools, the system achieves precise and independent control of the CM’s four degrees of freedom.

3. Kinematics of the CM

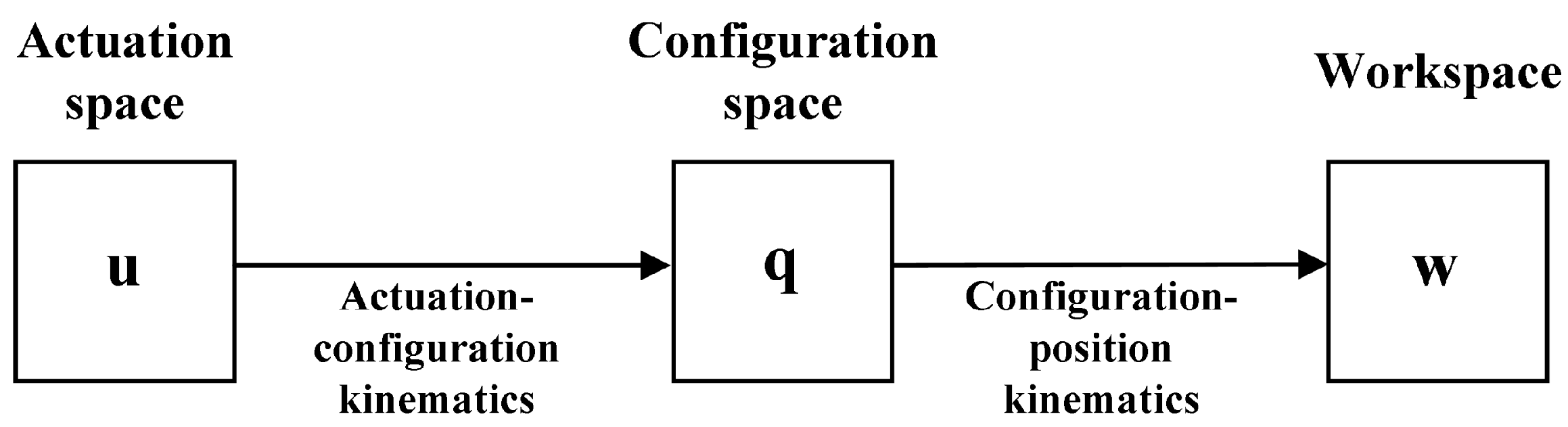
The kinematics of the CM can be divided into two main components: actuation-configuration kinematics and configuration-position kinematics, as illustrated in Figure 4. Actuation-configuration kinematics calculates the geometric configuration of the CM based on the actuation states and structural parameters, mapping the actuation space to the configuration space. configuration-position kinematics determines the final end-effector position based on the geometric configuration, mapping the configuration space to the workspace. The accuracy of these mappings among actuation space, configuration space, and workspace directly influences the precision of the CM.

The kinematic modeling methods of the CM are usually divided into constant curvature model and variable curvature model [20]. In this study, a piecewise variable curvature model is adopted for kinematic modeling under the following assumptions:

Assumption 1: 

The tendons controlling different flexible segments are independent. The tendons controlling different degrees of freedom within the same flexible segment are also independent. The interaction between different segments is neglected.

Assumption 2: 

Each flexible segment exhibits independent curvature variations.

Assumption 3: 

Each flexible segment is further subdivided into multiple bending units. Within a single flexible segment, all bending units controlled by the same tendon pair exhibit identical curvature. Bending units controlled by different tendon pairs may have different curvatures. The curvature within each bending unit remains constant, allowing it to be approximated as a circular arc.

Assumption 4: 

The tendons remain linear between two consecutive bending units. Intrinsic curvature effects of the tendons themselves are neglected.

Assumption 5: 

Gravity, inertial forces, and frictional effects are ignored in the model.

The workspace of the CM is represented as $w={\left[\begin{array}{ccc}x& y& z\end{array}\right]}^{\mathrm{T}}$, which defines the position coordinates of the centroid of the CM’s distal cross-section in the base coordinate system. The configuration space of the CM is denoted as $q={\left[\begin{array}{cc}{q}_1^{\mathrm{T}}& q_2^{\mathrm{T}}\end{array}\right]}^{\mathrm{T}}$, where $q_i={\left[\begin{array}{cc}{\phi }_i& {\theta }_i\end{array}\right]}^{\mathrm{T}}$ represents the configuration space of the flexible segment $i$, consisting of the bending plane angle ${\phi }_i$ and bending angle ${\theta }_i$. The actuation space of the CM is defined as $u={\left[\begin{array}{cc}{u}_1^{\mathrm{T}}& u_2^{\mathrm{T}}\end{array}\right]}^{\mathrm{T}}$, where $u_i$ represents the actuation space of the flexible segment $i$ and is given by

$$u_i={\left[\begin{array}{cccc}\Delta l_{i1,t}& \Delta l_{i2,t}& \Delta l_{i3,t}& \Delta l_{i4,t}\end{array}\right]}^{\mathrm{T}}$$

(1)

3.1. Configuration-Position Kinematics

3.1.1. A Single Flexible Segment

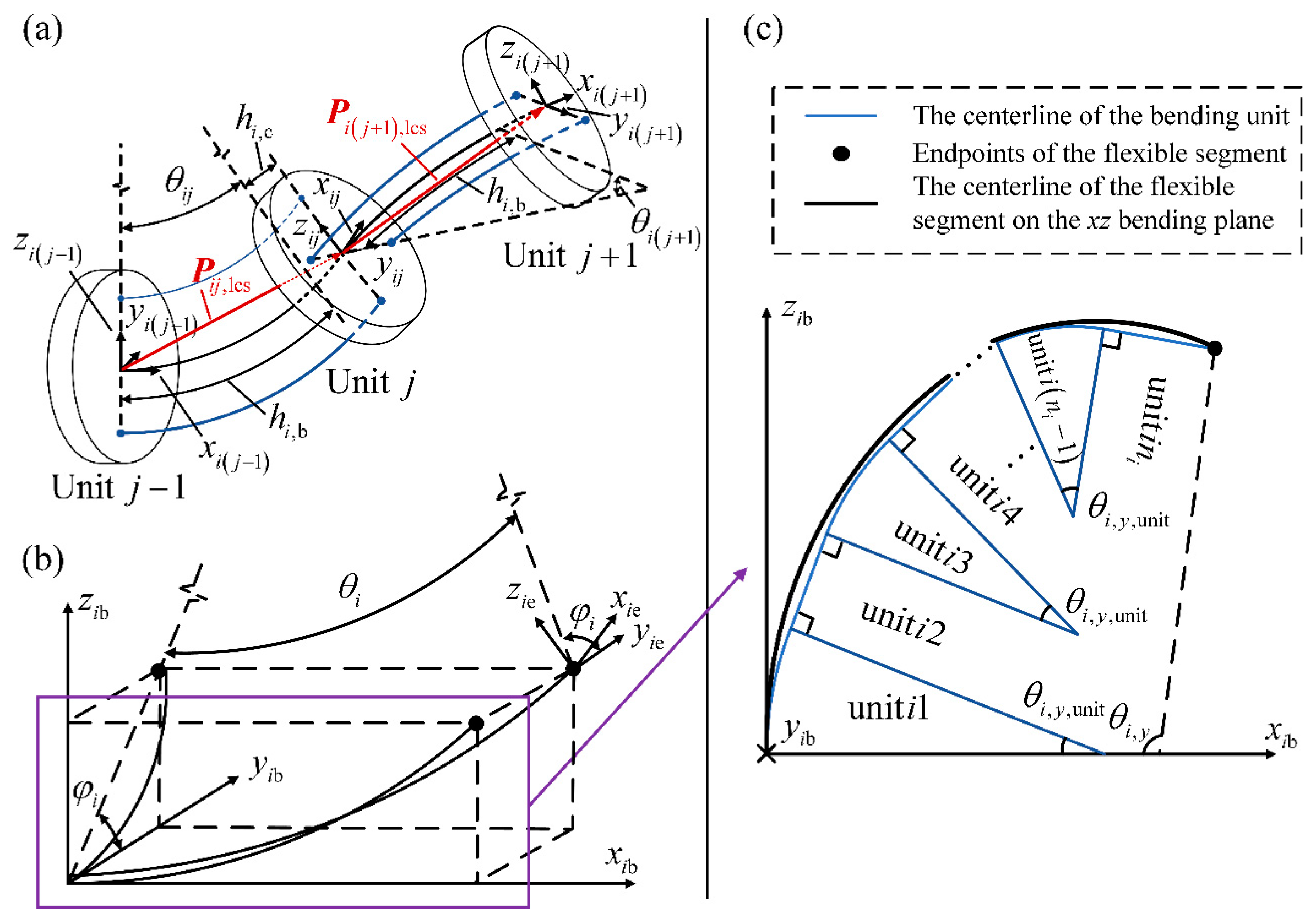
As shown in Figure 5a, the local position vector $P_{ij,lcs}$ of the centroid of the upper plane of bending unit $j$ relative to the local coordinate system $x_{ij-1}{y}_{ij-1}{z}_{ij-1}$ of bending unit $j-1$ is expressed as

$$P_{ij,lcs}={\left[\begin{array}{ccc}{x}_{ij,lcs}& y_{ij,lcs}& z_{ij,lcs}\end{array}\right]}^{\mathrm{T}}==\left\{\begin{array}{l}{\left[\begin{array}{ccc}{\displaystyle \frac{h_{i,b}\sin {\theta }_{i,y,unit}}{{\theta }_{i,y,unit}}}& 0& {\displaystyle \frac{h_{i,b}\left(1-\cos {\theta }_{i,y,unit}\right)}{{\theta }_{i,y,unit}}}\end{array}\right]}^{\mathrm{T}} j=j_{even}\\ {\left[\begin{array}{ccc}{\displaystyle \frac{h_{i,b}\sin {\theta }_{i,z,unit}}{{\theta }_{i,z,unit}}}& {\displaystyle \frac{h_{i,b}\left(1-\cos {\theta }_{i,z,unit}\right)}{{\theta }_{i,z,unit}}}& 0\end{array}\right]}^{\mathrm{T}} j=j_{odd}\end{array}\right.$$

(2)

Here, $j_{odd}=2n-1\left(n=1,2\cdots ,n_i/2\right)$ and $j_{even}=2n\left(n=1,2\cdots ,n_i/2\right)$ represent the indices of the $n\mathrm{-th}$ odd-numbered and even-numbered bending units, respectively. The first bending unit of each flexible segment is assumed to bend about the $z$ axis. The local rotation matrix $R_{ij,lcs}$ for bending unit $j$ is given by

$$R_{ij,lcs}=\left\{\begin{array}{l}{R}_{y_{j-1}}\left({\theta }_{i,y,unit}\right) j=j_{even}\\ R_{z_{j-1}}\left({\theta }_{i,z,unit}\right) j=j_{odd}\end{array}\right.$$

(3)

By applying recursive calculations, the global rotation matrix $R_{ij}$ and global position vector $P_{ij}$ of bending unit can be determined as

$$\left\{\begin{array}{l}{R}_{ij}=\left\{\begin{array}{l}{R}_{ij,lcs} j=1\\ R_{ij-1}{R}_{ij,lcs} j>1\end{array}\right.\\ P_{ij}={\left[\begin{array}{ccc}{x}_{ij}& y_{ij}& z_{ij}\end{array}\right]}^{\mathrm{T}}=\left\{\begin{array}{l}{P}_{ij,lcs} j=1\\ P_{ij-1}+R_{ij-1}{P}_{ij,lcs} j>1\end{array}\right.\end{array}\right.$$

(4)

Furthermore, the global rotation matrix $R_i$ and global position vector $P_i$ for the entire flexible segment can be derived from the above relationships:

$$\left\{\begin{array}{l}{R}_i={\displaystyle \prod _{j=1}^{n_i}{R}_{ij}}\\ P_i={\displaystyle \sum _{j=1}^{n_i}{P}_{ij}}\end{array}\right.$$

(5)

As shown in Figure 5c, since the bending units within the flexible segment adopt an orthogonal serial structure, each unit can only bend in a single direction. Consequently, within a specific bending direction, the bending directions of adjacent units are alternately arranged. To determine the global bending angles in two orthogonal directions for the flexible segment, the local bending angles of the bending units in each respective direction need to be accumulated. The global bending angles around the y-axis and z-axis are given by

$$\left\{\begin{array}{l}{\theta }_{i,y}={\displaystyle \sum _{j=j_{even}}^{n_i}{\theta }_{i,y,unit}}={\displaystyle \frac{n_i{\theta }_{i,y,unit}}{2}}\\ {\theta }_{i,z}={\displaystyle \sum _{j=j_{odd}}^{n_i}{\theta }_{i,z,unit}}={\displaystyle \frac{n_i{\theta }_{i,z,unit}}{2}}\end{array}\right.$$

(6)

Furthermore, As shown in Figure 5b, the global bending plane angle and the total global bending angle of the flexible segment can be obtained as

$$\left\{\begin{array}{l}{\phi }_i=\mathrm{atan}2\left({\displaystyle \frac{{\theta }_{i,y}}{{\theta }_{i,z}}}\right)=\mathrm{atan}2\left({\displaystyle \frac{{\theta }_{i,y,unit}}{{\theta }_{i,z,unit}}}\right)\\ {\theta }_i=\sqrt{{\theta }_{i,y}^2+{\theta }_{i,z}^2}=={\displaystyle \frac{n_i\sqrt{{\theta }_{i,y,unit}^2+{\theta }_{i,z,unit}^2}}{2}}\end{array}\right.$$

(7)

3.1.2. Multiple Flexible Segments

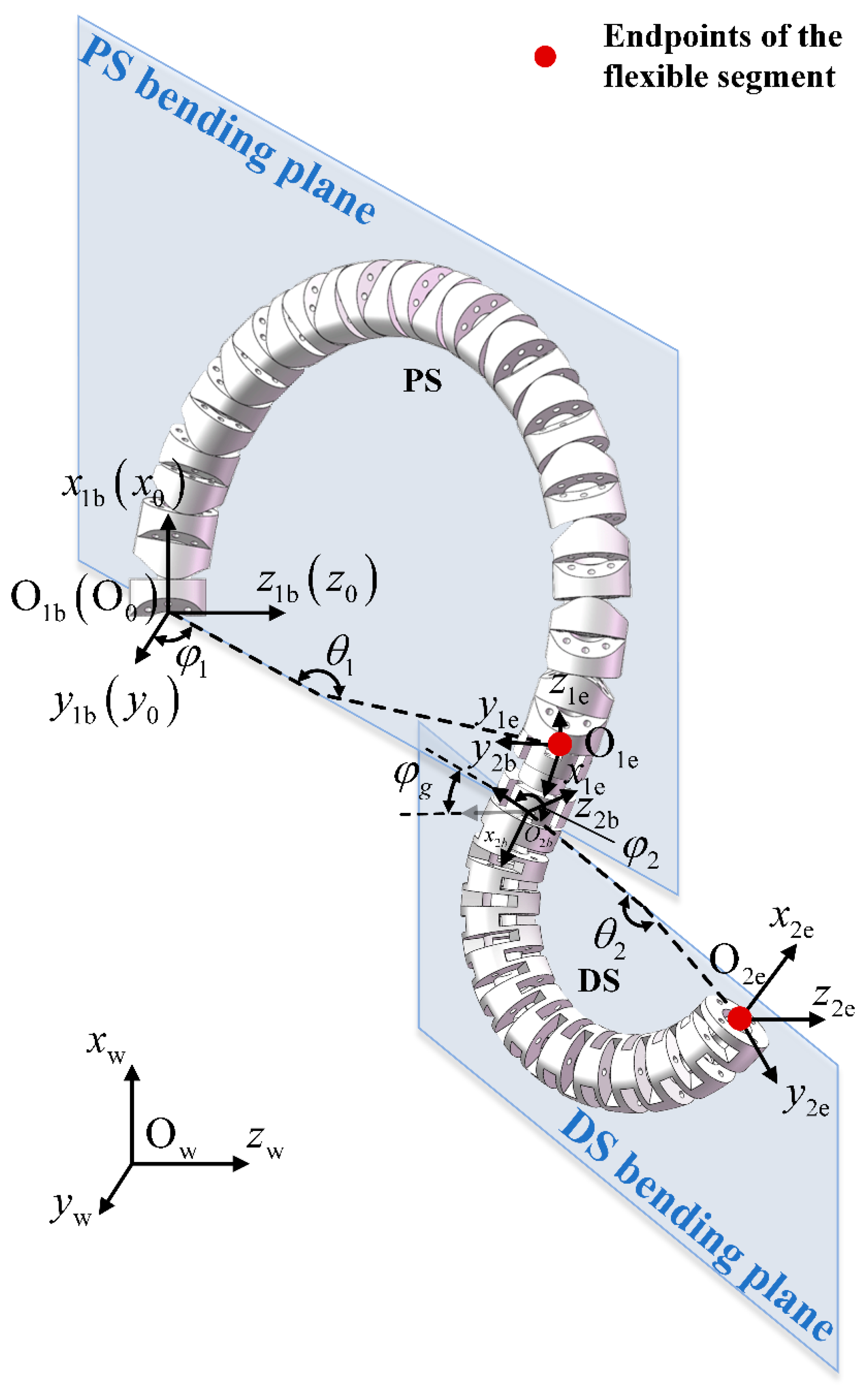
The CM coordinate system is established as shown in Figure 6. As seen in the diagram, the lower-left corner represents the world coordinate system $\left\{O_{\mathrm{w}}\right\}$. The base coordinate system $\left\{O_0\right\}$ has its origin $O_0$ fixed at the centroid of the initial cross-section of the PS. The $x_0$-axis is perpendicular to the cross-section and oriented inward towards the PS as the positive direction. From the perspective of the $x_0$-axis, the rightward horizontal direction is the positive $y_0$-axis. Initially, the PS beginning coordinate system $\left\{O_{1\mathrm{b}}\right\}$ coincides with the base coordinate system $\left\{O_0\right\}$. The PS end coordinate system $\left\{O_{1\mathrm{e}}\right\}$ has its origin $O_{1\mathrm{e}}$ fixed at the centroid of the final cross-section of the PS. The $x_{1e}$-axis is perpendicular to the cross-section and oriented outward as the positive direction, while the $y_{1e}$-axis is horizontally rightward as the positive direction. The DS beginning coordinate system $\left\{O_{2\mathrm{b}}\right\}$ has its origin $O_{2\mathrm{b}}$ fixed at the centroid of the initial cross-section of the DS. The $x_{2b}$-axis is perpendicular to the cross-section and oriented outward as the positive direction, while the $y_{2b}$-axis is defined as the direction obtained by rotating counterclockwise by ${\phi }_g$ from the rightward horizontal direction around the $x_{2b}$-axis. The DS end coordinate system $\left\{O_{2\mathrm{e}}\right\}$ has its origin $O_{2\mathrm{e}}$ fixed at the centroid of the initial cross-section of the DS. The $x_{2e}$-axis is perpendicular to the cross-section and oriented outward as the positive direction, while the $y_{2e}$-axis is defined as the direction obtained by rotating counterclockwise by ${\phi }_g$ from the rightward horizontal direction around the $x_{2e}$-axis. All $z$-axes are determined according to the right-hand rule. Once the coordinate system is established, the sequential transformation matrix from the world coordinate system to the end coordinate system of the DS can be expressed as

$${}_{2\mathrm{e}}{}^{\mathrm{w}}T={}_0{}^{\mathrm{w}}T{}_{1\mathrm{b}}{}^{0}T{}_{1\mathrm{e}}{}^{1\mathrm{b}}T{}_{2\mathrm{b}}{}^{1\mathrm{e}}T{}_{2\mathrm{e}}{}^{2\mathrm{b}}T$$

(8)

where ${}_0{}^{\mathrm{w}}T$ and ${}_{1\mathrm{b}}{}^{0}T$ represent the sequential transformation matrices from the world coordinate system to the base coordinate system and from the base coordinate system to the initial coordinate system of the PS, respectively. By combining these transformation matrices with the specific environment, a more detailed analysis can be derived, which is omitted here. The transformation matrix ${}_{1\mathrm{e}}{}^{1\mathrm{b}}T$ represents the sequential transformation from the initial coordinate system of the PS to its distal coordinate system. Similarly, ${}_{2\mathrm{b}}{}^{1\mathrm{e}}T$ denotes the transformation from the distal coordinate system of the PS to the initial coordinate system of the DS, and ${}_{2\mathrm{e}}{}^{2\mathrm{b}}T$ represents the transformation from the initial coordinate system of the DS to its distal coordinate system. Now, we analyze the transformation matrix from the initial coordinate system of the PS to the distal coordinate system of the DS.

$${}_{2\mathrm{e}}{}^{1\mathrm{b}}T={}_{1\mathrm{e}}{}^{1\mathrm{b}}T{}_{2\mathrm{b}}{}^{1\mathrm{e}}T{}_{2\mathrm{e}}{}^{2\mathrm{b}}T$$

(9)

Specifically, the transformation matrices ${}_{1\mathrm{e}}{}^{1\mathrm{b}}T$, ${}_{2\mathrm{b}}{}^{1\mathrm{e}}T$, and ${}_{2\mathrm{e}}{}^{2\mathrm{b}}T$ can be expressed as

$$\left\{\begin{array}{l}{}_{1\mathrm{e}}{}^{1\mathrm{b}}T=\left[\begin{array}{cc}{R}_1& P_1\\ 0& 1\end{array}\right]\\ {}_{2\mathrm{b}}{}^{1\mathrm{e}}T=\mathrm{Trans}\left(x_{1e},h_{IC}\right)\times \mathrm{Rot}\left(x_{1e},{\phi }_{rel}\right)\\ {}_{2\mathrm{e}}{}^{2\mathrm{b}}T=\left[\begin{array}{cc}{R}_2& P_2\\ 0& 1\end{array}\right]\end{array}\right.$$

(10)

Here, $R_i$ and $P_i$ represent the global rotation matrix and global position vector of flexible segment $i$ with respect to its own beginning coordinate system $\left\{O_{i\mathrm{b}}\right\}$, which can be obtained from the previous subsection.

3.2. Actuation-Configuration Kinematics

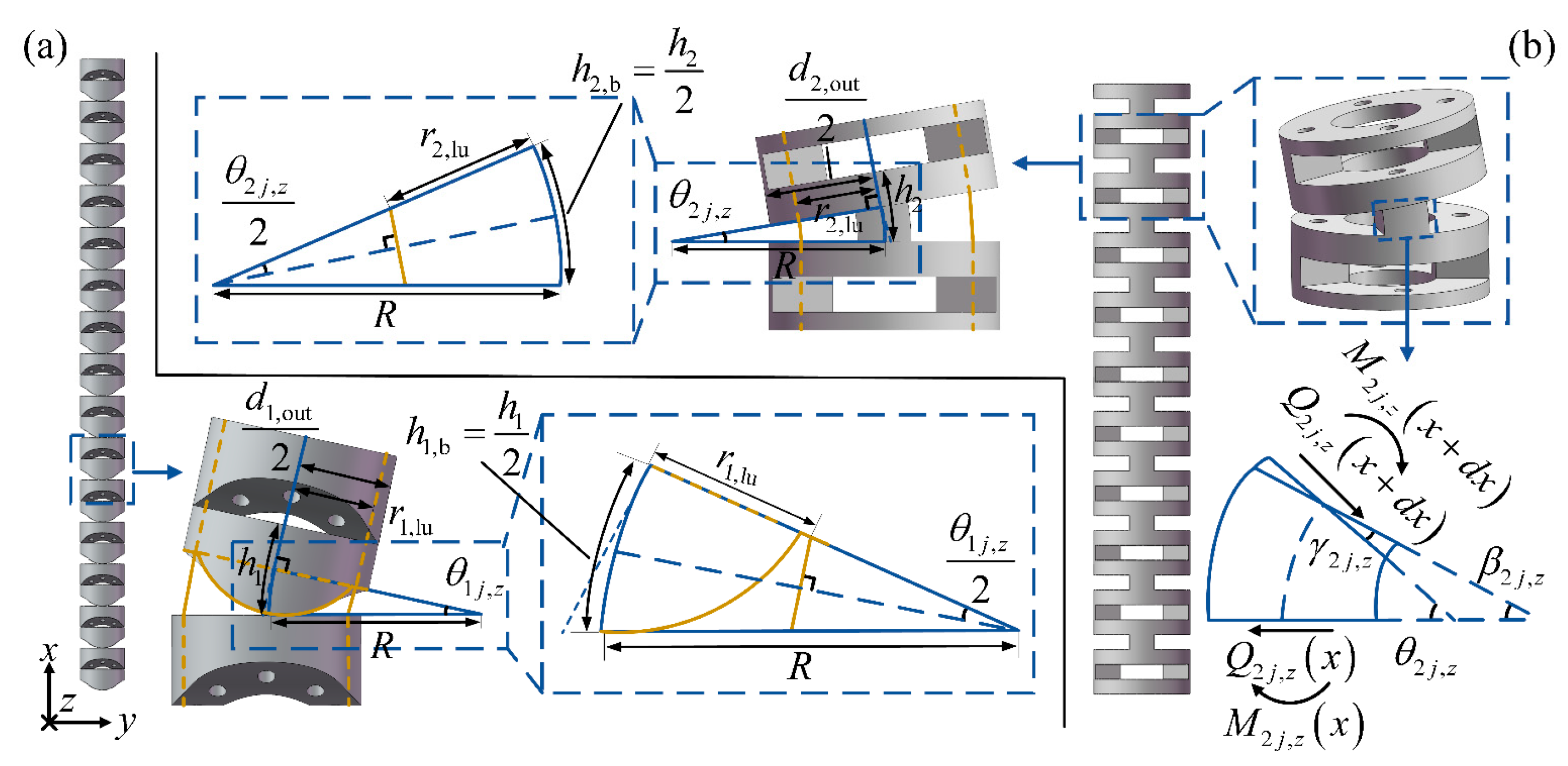
Next, the relationship between the local tendon displacement and the local bending angle of an arbitrary bending unit within the PS and DS is investigated. The PS achieves bending through smooth rolling contact of aluminum alloy semi-circular elements (while neglecting friction between units), whereas the DS achieves deformation-based bending via micro-scale polymer notched beam connections. Although the two flexible segments exhibit distinct bending mechanisms and unit geometric structures, the fundamental geometric relationships between tendons and bending units remain similar. Therefore, a unified analytical approach can be applied. Since each flexible segment inherently possesses two mutually orthogonal and equivalent bending degrees of freedom, it suffices to illustrate the bending process along a specific axis in a given plane. In this study, the bending process along the z-axis is analyzed and visualized in the plane perspective, as shown in Figure 7.

To simplify the analysis, the contact between adjacent bending units of the PS is idealized as a smooth hinge connection, and the chamfer of the contact contour edge is approximated as a straight line, as shown in Figure 7a. The structural characteristics of the PS bending unit determine that its primary bending region is limited to the lower semicircular arc region. Based on geometric relationships, the nonlinear relationship between the local bending angles ${\theta }_{1,z,unit}$ and ${\theta }_{1,y,unit}$ around the $z$-axis and $y$-axis of the PS bending unit and the local tendon displacement $\mathsf{\Delta }{l}_{1,k,unit}$ can be expressed as

$$\left\{\begin{array}{l}\mathsf{\Delta }{l}_{1,1,unit}=-\mathsf{\Delta }{l}_{1,3,unit}=h_{1,b}-2\sin \left({\displaystyle \frac{{\theta }_{1,z,unit}}{2}}\right)\left({\displaystyle \frac{h_{1,b}}{{\theta }_{1,z,unit}}}-r_{1,lu}\right)\\ \mathsf{\Delta }{l}_{1,4,unit}=-\mathsf{\Delta }{l}_{1,2,unit}=h_{1,b}-2\sin \left({\displaystyle \frac{{\theta }_{1,y,unit}}{2}}\right)\left({\displaystyle \frac{h_{1,b}}{{\theta }_{1,y,unit}}}-r_{1,lu}\right)\end{array}\right.$$

(11)

The subscripts in the local bending angles and local tendon displacements indicate their corresponding physical meanings. Specifically, subscript 1 represents the flexible segment to which the bending unit belongs, while $z$ and $y$ denote the bending directions around the $y$-axis and $z$-axis, respectively. $k$ represents the tendon number of the flexible segment, and unit signifies that the parameter is calculated at the scale of a single bending unit.

As shown in Figure 7b, since the DS bending unit has a relatively small span-to-depth ratio <5, the traditional Euler–Bernoulli beam theory (pure bending deformation) is insufficient to accurately describe its deformation behavior. Considering rotational inertia and shear force effects, the DS bending unit’s connecting beam is modeled as a Timoshenko beam, incorporating both shear and bending deformations. Based on this, the bending moment and shear force equations of the DS bending unit’s connecting beam can be derived as

$$\left\{\begin{array}{c}{M}_{2,y}=E_{MNR}{I}_{2,beam}{\kappa }_{2,y,unit}\\ M_{2,z}=E_{MNR}{I}_{2,beam}{\kappa }_{2,z,unit}\end{array}\right.,\left\{\begin{array}{c}{Q}_{2,y}=G_{MNR}{A}_2{k}_q{\gamma }_{2,y,unit}\\ Q_{2,z}=G_{MNR}{A}_2{k}_q{\gamma }_{2,z,unit}\end{array}\right.$$

(12)

$M_{2,y}=\left(T_{2,4}-T_{2,2}\right)r_{2,lu}\cos {\displaystyle \frac{{\theta }_{2,y,unit}}{2}}$ and $M_{2,z}=\left(T_{2,1}-T_{2,3}\right)r_{2,lu}\cos {\displaystyle \frac{{\theta }_{2,z,unit}}{2}}$ represent the bending moments of the DS bending unit’s connecting beam around the $y$-axis and $z$-axis, respectively. Meanwhile, $Q_{2,y}=\left(T_{2,4}-T_{2,2}\right)\sin {\displaystyle \frac{{\theta }_{2,y,unit}}{2}}$ and $Q_{2,z}=\left(T_{2,1}-T_{2,3}\right)\sin {\displaystyle \frac{{\theta }_{2,z,unit}}{2}}$ represent the corresponding shear forces. Here, $I_{2,beam}$ is the second moment of inertia of the cross-section (approximated as a rectangular cross-section), and $A_2$ is the cross-sectional area. $E_{MNR}$ and $G_{MNR}$ denote Young’s modulus and shear modulus of the micronano resin material, respectively. Additionally, ${\kappa }_{2,y,unit}$ and ${\kappa }_{2,z,unit}$ represent the local bending curvatures of the DS bending unit, while ${\gamma }_{2,y,unit}$ and ${\gamma }_{2,z,unit}$ denote the local shear bending angles under shear force. $k_q$ is the Timoshenko shear coefficient, which is typically taken as $k_q\approx 5/6$ for rectangular cross-sections. Considering both bending moment and shear force effects, the local bending angle of the DS bending unit can be expressed as

$$\left\{\begin{array}{l}{\theta }_{2,y,unit}={\beta }_{2,y,unit}+{\gamma }_{2,y,unit}\\ {\theta }_{2,z,unit}={\beta }_{2,z,unit}+{\gamma }_{2,z,unit}\end{array}\right.$$

(13)

${\beta }_{2,y,unit}=h_{2,b}{\kappa }_{2,y,unit}$ and ${\beta }_{2,z,unit}=h_{2,b}{\kappa }_{2,z,unit}$ represent the local bending angles of the DS bending unit due to bending moments. Similar to the PS case, based on geometric relationships, the local bending angles ${\theta }_{2,y,unit}$ and ${\theta }_{2,z,unit}$ of the DS bending unit, along with the local tendon displacement $\mathsf{\Delta }{l}_{2,k,unit}$, can be further expressed through a nonlinear relationship as

$$\left\{\begin{array}{l}\mathsf{\Delta }{l}_{2,1,unit}=-\mathsf{\Delta }{l}_{2,3,unit}=h_{2,b}-2\sin \left({\displaystyle \frac{{\theta }_{2,z,unit}}{2}}\right)\left({\displaystyle \frac{h_{2,b}}{{\theta }_{2,z,unit}}}-r_{2,lu}\right)\\ \mathsf{\Delta }{l}_{2,4,unit}=-\mathsf{\Delta }{l}_{2,2,unit}=h_{2,b}-2\sin \left({\displaystyle \frac{{\theta }_{2,y,unit}}{2}}\right)\left({\displaystyle \frac{h_{2,b}}{{\theta }_{2,y,unit}}}-r_{2,lu}\right)\end{array}\right.$$

(14)

3.3. Workspace

To determine the specific workspace of the CM, a Monte Carlo simulation was performed. The bending plane angle range for both the PS and DS was set to $\left[0,{360}^{\circ }\right]$, while the bending angle range was set to $\left[0,{120}^{\circ }\right]$ and $\left[0,{110}^{\circ }\right]$, respectively. The overall workspace of the CM is shown in Figure 8, which includes the independent workspaces of the PS and DS, as well as the combined workspace formed by their integration.

Figure 8a illustrates the workspace of the PS, which exhibits a hemispherical shell-like structure. The grid representation visually depicts the boundaries and distribution characteristics of the workspace. Figure 8b presents the workspace of the DS, generated using an envelope method, providing a clear depiction of the DS’s motion boundaries in three-dimensional space, revealing a hemispherical contour. Figure 8c demonstrates the overall workspace of the continuum manipulator, also generated based on the envelope method. The outer envelope represents the maximum workspace boundary of the entire continuum manipulator, while the internal grid structure corresponds to the independent workspace of the PS. Additionally, the three-dimensional grid structure illustrates the workspace limits of the combined PS and DS under different postures. The blue scatter points in the figure represent the distribution of critical flexible points.

4. Piecewise Stiffness and Active Decoupling of the CM

4.1. Piecewise Stiffness

Since the PS and DS have different geometric structures and bending unit configurations, their flexible segments exhibit different stiffness characteristics. Based on this, a piecewise stiffness analysis is conducted. The stiffness matrix $K_i$ of flexible segment $i$ can be expressed as

$$K_i=diag(\begin{array}{ccc}{K}_{i,x}& K_{i,y}& K_{i,z}\end{array})$$

(15)

where $K_{i,x}$, $K_{i,y}$, and $K_{i,z}$ represent the axial stiffness in the $x$-axis direction, the bending stiffness in the $y$-axis direction, and the bending stiffness in the $z$-axis direction, respectively.

Since the tendons cannot undergo elongation or compression, the axial stiffness of both the PS and DS is determined by the bending unit stiffness without considering the effect of tendon channels. First, the axial stiffness of individual bending units in the PS and DS is analyzed. As shown in Figure 9a, the cross-sectional areas of the semicircular bending units in the PS and the notched bending units in the DS vary along the local $x$-axis. For the former, the cross-sectional area $A_1\left(x\right)$ can be approximated in three segments: the first segment is a varying double-rectangular cross-section; the second segment is an inner circular and outer rectangular cross-section; the third segment is a constant circular cross-section. The detailed formulation is as follows:

$$A_1\left(x\right)=\left\{\begin{array}{l}2\sqrt{2rx-x^2}\left(d_{1,out}-d_{1,in}\right) x\in \left[0,r-\sqrt{r^2-d_{1,in}^2/4}\right)\\ 2\sqrt{2rx-x^2}\sqrt{d_{1,out}^2-4\left(2rx-x^2\right)}-\pi d_{1,in}^2/4 x\in \left[r-\sqrt{r^2-d_{1,in}^2/4},h_{1,b}\right)\\ \pi \left(d_{1,out}^2-d_{1,in}^2\right)/4 x\in \left[h_{1,b},h_1\right)\end{array}\right.$$

(16)

The parameter $r={\displaystyle \frac{d_{1,out}^2+h_1^2}{12}}$ represents the semicircular bending radius of the PS bending unit. For the latter, the cross-sectional area $A_2\left(x\right)$ can be divided into two segments: the first segment represents the notched double-rectangular cross-section of the connecting beam; the second segment corresponds to the non-notched constant circular cross-section. The detailed formulation is as follows:

$$A_2\left(x\right)=\left\{\begin{array}{l}2ab-2\pi {\left({\displaystyle \frac{d_{2,lu}}{2}}\right)}^2 x\in \left[0,h_{2,b}\right)\\ \pi \left(d_{2,out}^2-d_{2,in}^2\right)-4\pi {\left({\displaystyle \frac{d_{2,lu}}{2}}\right)}^2 x\in \left[h_{2,b},h_2\right)\end{array}\right.$$

(17)

By integrating, the axial stiffness of the PS and DS bending units can be obtained separately:

$$\left\{\begin{array}{l}{k}_{1,x}={\displaystyle \frac{E_{Al}}{h_1}}{\displaystyle {\int }_0^{h_1}{A}_1\left(x\right)dx}\\ k_{2,x}={\displaystyle \frac{E_{MNR}}{h_2}}{\displaystyle {\int }_0^{h_2}{A}_2\left(x\right)dx}\end{array}\right.$$

(18)

Among them, $k_{1,x}$ and $k_{2,x}$ represent the axial stiffness of the PS and DS bending units, respectively, while $E_{Al}$ and $E_{MNR}$ denote Young’s modulus of aluminum alloy and micro-nano resin materials, respectively. Therefore, based on the series stiffness formula, the axial stiffness of the PS and DS can be determined:

$$\left\{\begin{array}{l}{K}_{1,x}={\displaystyle \frac{k_{1,x}}{n_1}}\\ K_{2,x}={\displaystyle \frac{k_{2,x}}{n_2}}\end{array}\right.$$

(19)

As shown in Figure 9b, the PS joint is simplified as a smooth chain, thus neglecting joint friction. Consequently, the local bending stiffness of the PS bending unit is determined by the combined local bending stiffness of the tendons passing through both the PS and DS. In contrast, the DS primarily relies on the resistance to bending deformation provided by the interspace connecting beams and tendons. Therefore, the local bending stiffness of the DS bending unit is derived from the combined local bending stiffness of the connecting beams and the tendons in the DS. To begin with, the local bending stiffness of a single bending unit in the flexible segment is considered. Given the symmetric distribution of the bending unit’s cross-section and tendon arrangement, the bending stiffness in both the $y$-axis and $z$-axis directions is equivalent and can be expressed as

$$\left\{\begin{array}{l}{k}_{1,y}=k_{1,z}={\displaystyle \frac{E_{NiTi}{I}_{1,tdn}}{h_1}}\\ k_{2,y}=k_{2,z}={\displaystyle \frac{E_{NiTi}{I}_{2,tdn}+E_{MNR}{I}_{2,beam}}{h_2}}\end{array}\right.$$

(20)

In this, $E_{NiTi}$ is Young’s modulus of the aluminum alloy, $I_{1,tdn}$ and $I_{2,tdn}$ are the moments of inertia for the bending units of the PS and DS, respectively. $I_{2,beam}$ is the moment of inertia for the connection of the DS bending unit. Simplifying the DS’s cross-sectional shape into a rectangular shape, its length and width are denoted as $a$ and $b$, respectively. Based on the plane bending, the moment of inertia can be calculated.

$$\left\{\begin{array}{l}{I}_{1,tdn}=2{\displaystyle \sum _{k=1}^{n_{1,tdn}}\left(\frac{\pi d_{1,tdn}^4}{64}+A_{1,tdn}{\left(r_{1,lu}\sin \left(\frac{\pi }{2}k-\frac{\pi }{2}\right)\right)}^2\right)}\\ +2{\displaystyle \sum _{k=1}^{n_{2,tdn}}\left(\frac{\pi d_{2,tdn}^4}{64}+A_{2,tdn}{\left(r_{2,lu}\sin \left(\frac{\pi }{2}k-\frac{\pi }{4}\right)\right)}^2\right)}\\ I_{2,tdn}=2{\displaystyle \sum _{k=1}^{n_{2,tdn}}\left(\frac{\pi d_{2,tdn}^4}{64}+A_{2,tdn}{\left(r_{2,lu}\sin \left(\frac{\pi }{2}k-\frac{\pi }{2}\right)\right)}^2\right)}\\ I_{2,beam}=2\left({\displaystyle \frac{b{a}^3}{12}}-{\displaystyle \frac{\pi d_{1,tdn}^4}{64}}\right)\end{array}\right.$$

(21)

To account for the influence of the single-path dual-tendon configuration, a correction factor of 2 is introduced. The variable $k$ represents the tendon index of the PS and DS, while $k$ and $A_{2,tdn}=\pi d_{2,tdn}^2/4$ denote the cross-sectional areas of the tendons in the PS and DS, respectively.

Since both the PS and DS adopt the same orthogonal serially connected bending unit structure, an alternating bending phenomenon occurs during unidirectional bending. Specifically, one bending unit undergoes bending while the adjacent unit remains straight. Based on this observation, each pair of adjacent bending units within a flexible segment is considered as a single group, where only the bending unit contributes to the bending stiffness of the group, while the non-bending unit does not participate in stiffness transmission. Consequently, the bending stiffness of each bending unit group is equal to that of an individual bending unit. By applying the serially connected bending model, the overall bending stiffness of the flexible segment can be derived:

$$\left\{\begin{array}{l}{K}_{1,y}=K_{1,z}={\displaystyle \frac{2k_{1,y}}{n_1}}={\displaystyle \frac{2k_{1,z}}{n_1}}\\ K_{2,y}=K_{2,z}={\displaystyle \frac{2k_{2,y}}{n_2}}={\displaystyle \frac{2k_{2,z}}{n_2}}\end{array}\right.$$

(22)

4.2. Active Decoupling

The PS tendons are directly fixed to the IC, meaning their tendon force is confined to the PS and IC regions and has no direct effect on the DS. However, the DS tendons are fixed to the EC. When the DS tendons are actuated, they exert a certain tendon force on the PS, inevitably affecting its structural configuration and tendon displacement. Therefore, it is necessary to consider the tendon force coupling effect of the DS tendon force on the PS tendon displacement. Since the PS tendons do not pass through the DS lumens, the impact of the DS tendon displacement on the PS deformation is minimal and can be neglected, provided that manufacturing, installation, and dimensional errors are not considered. However, because the DS tendons extend through the PS lumens, the PS tendon displacement induces deformation in the DS, further influencing the internal DS tendon displacement, leading to passive deformation of the DS even in the absence of active actuation. Consequently, it is essential to consider the tendon displacement coupling effect of the PS tendon displacement on the DS tendon displacement coupling. To effectively mitigate this coupling effect, this section proposes an active decoupling strategy that utilizes tendon displacement compensation to attenuate the coupling between the PS and DS, thereby achieving independent and precise control of each flexible segment.

4.2.1. Tendon Force Coupling Effect

As shown in Figure 10a, by neglecting the IC and EC structures at the ends of the PS and DS, and simplifying the force coupling problem, we simplify the coupling effect of the DS on the PS as a resultant moment:

$$\left\{\begin{array}{l}{M}_{2to1}=M_{2to1,y}^2+M_{2to1,z}^2\\ M_{2to1,y}=\left(T_{2,4}-T_{2,2}\right)r_{2,lu}\cos {\phi }_{rel}+\left(T_{2,1}-T_{2,3}\right)r_{2,lu}\cos \left({\phi }_{rel}+{\displaystyle \frac{\pi }{2}}\right)\\ M_{2to1,z}=\left(T_{2,4}-T_{2,2}\right)r_{2,lu}\sin {\phi }_{rel}+\left(T_{2,1}-T_{2,3}\right)r_{2,lu}\sin \left({\phi }_{rel}+{\displaystyle \frac{\pi }{2}}\right)\end{array}\right.$$

(23)

$M_{2to1}$ is the resultant torque applied by the DS to the PS. $M_{2to1,y}$ and $M_{2to1,z}$ represent the components of this torque along the $y$-axis and $z$-axis, respectively. $T_{i,k}$ is the tension of tendon $k$ in flexible segment $i$, and the subscript $2\mathrm{to}1$ indicates that this torque is the resultant torque applied by the DS to PS. The resultant torque applied to the PS generates displacements in the direction of the combined axis and angular displacements in the bending axis, which are

$$\left\{\begin{array}{l}\Delta l_{2to1,1,x}=\Delta l_{2to1,2,x}=\Delta l_{2to1,3,x}=\Delta l_{2to1,4,x}=\left({\displaystyle \sum _{k=1}^{n_{2,tdn}}{T}_{2,k}}\right)/K_{1,x}\\ \Delta l_{2to1,4,y}=-\Delta l_{2to1,2,y}={\displaystyle \frac{M_{2to1,y}{r}_{1,lu}}{K_{1,y}}}\\ \Delta l_{2to1,1,z}=-\Delta l_{2to1,3,z}={\displaystyle \frac{M_{2to1,z}{r}_{1,lu}}{K_{1,z}}}\end{array}\right.$$

(24)

Thus, the total parasite displacement generated by the tendon force of the DS on the PS is

$$\left\{\begin{array}{c}\Delta l_{2to1,1}=\Delta l_{2to1,1,x}+\Delta l_{2to1,1,z}\\ \Delta l_{2to1,2}=\Delta l_{2to1,2,x}+\Delta l_{2to1,2,y}\\ \Delta l_{2to1,3}=\Delta l_{2to1,3,x}+\Delta l_{2to1,3,z}\\ \Delta l_{2to1,4}=\Delta l_{2to1,4,x}+\Delta l_{2to1,4,y}\end{array}\right.$$

(25)

4.2.2. Tendon Displacement Coupling Effect

As shown in Figure 10b, when the tendon displacement of the PS occurs, the structural state of the PS’s bending unit changes, resulting in coupling displacement of the DS tendon through the bending unit within the lumens of the PS. The tendon displacement of the PS consists of two parts: one part is the displacement caused by the active force, and the other part is the displacement caused by the force coupling of the DS to PS. Therefore, the tendon displacement of the PS is the coupling displacement produced by the DS’s tendon on the PS.

$$\left\{\begin{array}{l}\Delta l_{1to2,1}=\left(\Delta l_{1,1}+\Delta l_{2to1,1}\right)\cos {\phi }_{rel}+\left(\Delta l_{1,2}+\Delta l_{2to1,2}\right)\sin {\phi }_{rel}\\ \Delta l_{1to2,2}=\left(\Delta l_{1,2}+\Delta l_{2to1,2}\right)\cos {\phi }_{rel}+\left(\Delta l_{1,3}+\Delta l_{2to1,3}\right)\sin {\phi }_{rel}\\ \Delta l_{1to2,3}=\left(\Delta l_{1,3}+\Delta l_{2to1,3}\right)\cos {\phi }_{rel}+\left(\Delta l_{1,4}+\Delta l_{2to1,4}\right)\sin {\phi }_{rel}\\ \Delta l_{1to2,4}=\left(\Delta l_{1,4}+\Delta l_{2to1,4}\right)\cos {\phi }_{rel}+\left(\Delta l_{1,1}+\Delta l_{2to1,1}\right)\sin {\phi }_{rel}\end{array}\right.$$

(26)

In summary, the corrected tendon displacements of the PS and DS are obtained through compensation:

$$\left\{\begin{array}{l}\mathsf{\Delta }{}^{adj}l_{1,k}=\Delta l_{1,k}+\Delta l_{2to1,k}\\ \mathsf{\Delta }{}^{adj}l_{2,k}=\Delta l_{2,k}+\Delta l_{1to2,k}\end{array}\right.$$

(27)

5. Experimental Verification

5.1. Single-Segment Bending Test

The bending response characteristics of the PS and DS under a series of predefined input angles has been investigated; the experimental scenarios and results are presented in Figure 11. In Figure 11a, the PS was driven sequentially at target angles of 0°, 30°, 45°, 60°, 75°, 90°, and 120°, while the DS was driven at target angles of 0°, 30°, 45°, 60°, 75°, 90°, and 110°. The corresponding actual bending configurations and maximum bending angles are shown in the figure, demonstrating continuous and well-controlled bending behavior. Figure 11b presents the angle error between the expected and actual bending angles. The data show that the angle error for the PS ranges from 0.8° to 4.4°, and for the DS from 0.5° to 2.9°, with the maximum error for both flexible segments occurring at a target angle of 60°. These results indicate that both flexible segments achieve accurate angle tracking under independent actuation, exhibiting good bending consistency and control precision.

5.2. Stiffness Test

To evaluate the bending stiffness of each flexible segment of the CM, a series of static bending tests were conducted, analyzing the tip deflections of the segments under various external tip loads.

Figure 12a presents the actual bending configurations of the PS under different initial bending angles, including 0°, 30°, 60°, and 90°, as the applied load increases from 0 g to 80 g. It can be observed that the PS maintains good continuity and structural integrity under all tested conditions, with no significant undesired deformation. Figure 12b provides a quantitative analysis of the tip deflections in the horizontal direction (x-axis) and the vertical direction (z-axis) under the same conditions. In the bar chart, blue bars represent deflections in the x direction, while yellow bars represent those in the z direction. The results show that the x-direction deflections are generally small, mostly fluctuating within 2 to 3 mm and not exceeding 5 mm. The influence of load increase on x-direction deflection is minimal, with only a slight upward trend. In contrast, z-direction deflection increases more significantly with increasing load, whereas the deflection rises from nearly 0 mm at 0 g to approximately 6 to 7 mm at 80 g. When the initial bending angle reaches 90°, the overall z-direction deflection is relatively reduced, indicating that a proper pre-bent configuration helps enhance resistance to vertical deformation. Despite a certain degree of tip displacement under larger vertical loads, the tip deflections of the PS remain within an acceptable range across all tested conditions. The maximum z-direction deflection is approximately 6.9 mm, which is only about 7.7 percent of the total segment length of 90 mm. Considering that the upper airway pathway from the oral cavity to the tracheal inlet typically has a diameter ranging from 20 to 30 mm, this level of tip deflection remains within a safe margin and is unlikely to impede endotracheal tube advancement or path selection. Notably, for the PS under working configurations that closely approximate clinical application, such as when the initial bending angle is 90°, both x-direction and z-direction tip deflections are further reduced and stay within 5 mm, indicating superior stability and control precision. Therefore, the PS exhibits excellent structural stability and tip control performance under various initial postures and loading conditions, indicating strong potential to meet the spatial and positional requirements of clinical tracheal intubation.

To evaluate the bending stiffness of the DS, a series of static bending tests were conducted. Figure 13a presents the bending configurations of the DS under different initial bending angles (0°, 30°, 60°, and 90°) as the applied tip load increased from 0 g to 80 g. The DS maintained good continuity and structural consistency under all tested conditions, with no significant deformation or structural failure. Figure 13b quantitatively analyzes the tip deflections in both the x direction (horizontal) and z direction (vertical) under these conditions. In the bar chart, blue bars represent the x-direction deflections, while yellow bars represent the z-direction deflections. The x-direction deflections remained consistently low across all initial angles and loads, with a maximum value below 5 mm. In contrast, z-direction deflections increased significantly with higher loads, especially at lower initial angles. For example, under 0° initial bending, the z-direction deflection reached up to approximately 21 mm, whereas, under 90° initial bending, the maximum deflection was reduced to less than 13 mm. Notably, for loads below 40 g, all z-direction deflections were below 4 mm. In clinical tracheal intubation scenarios where only light contact typically occurs between the device and tissue, the DS tends to perform better, showing smaller positional deviation under lower loads. Furthermore, when the DS is in a highly pre-bent posture—more aligned with the anatomical curvature of the airway—it exhibits reduced deformation and better matches real clinical conditions.

5.3. Coupling Test

The coupling test has been investigated and is shown in Figure 14. The top row shows the angle behavior when the PS is actively bent to 30°, 60°, and 90°. The left three images represent the pre-decoupling state, where the passive DS exhibits coupling responses of 30.2°, 37.1°, and 49.9°, respectively. These results indicate a clear coupling effect from the PS to the DS, which intensifies with increasing input angle. The right three images show the decoupled state after the active decoupling is applied via tendon control, with the PS achieving actual bending angles of 33.6°, 56.2°, and 86.3°, resulting in angle errors of 3.6°, 3.8°, and 3.7°, respectively, demonstrating high bending accuracy. The bottom row shows the case when the DS is actively bent to 30°, 60°, and 90°. The left three images depict the pre-decoupling condition, where the passive PS exhibits coupling responses of 6.5°, 31.6°, and 38.7°, respectively. This confirms that DS activation also induces noticeable coupling effects on the PS. After active decoupling, the DS achieves bending angles of 33.1°, 56.7°, and 87.2°, with corresponding angle errors of 3.1°, 3.3°, and 2.8°, indicating excellent control precision. In summary, the tendon-driven active decoupling mechanism effectively mitigates inter-segment coupling, enabling both segments to achieve bending angles that closely match their expected values, and ensuring a stable and precise control performance.

The influence of different initial bending angles (30°, 60°, 90°) of the PS on the coupling behavior and post-decoupling control accuracy of the DS in the CM has been shown in Figure 15.

In Figure 15a, each major row represents one experiment with a specific PS initial upward bending angle (30°, 60°, or 90°). Each major row contains two sub-rows: the top showing the CM configuration before decoupling, and the bottom after decoupling. Within each configuration, the DS is actively controlled to bend 90° in one of four directions—upward, downward, leftward, and rightward—serving as the desired bending target for evaluating coupling and decoupling performance. Among them, the upward and downward directions lie in the same bending plane as the PS, while the leftward and rightward directions are in the orthogonal plane. Each image pair includes two views: a side view in the XZ plane and a top view in the XY plane, allowing spatial observation of the configuration of CM. In Figure 15b, the left bar chart quantifies the degree of DS coupling before decoupling, using a normalized deviation metric calculated as the difference between the expected and actual DS bending angles, divided by the expected target angle. In this experiment, the target angle is 90°, so the metric becomes (90°–actual DS bending angle)/90°. A larger value indicates greater deviation from the desired configuration, corresponding to a stronger coupling effect. In contrast to the left chart, which shows coupling deviation before decoupling, the right bar chart illustrates the post-decoupling control accuracy of the DS, quantified by the absolute difference between the DS’s actual bending angle and the expected target of 90°. Smaller values reflect better control accuracy and more effective decoupling.

The experimental results demonstrate that, as the PS initial bending angle increases from 30° to 90°, the DS coupling degree generally increases, indicating that stronger proximal pre-bending leads to greater mechanical interference and more pronounced coupling effects on the DS. Furthermore, the coupling in the upward and downward directions (same bending plane as the PS) is consistently greater than that in the left and right directions (orthogonal plane). The maximum coupling degree in the same plane reaches approximately 0.45, whereas that in the orthogonal plane remains below 0.25, suggesting that mechanical coupling is more readily transmitted within the same bending plane. In terms of post-decoupling control accuracy, the DS in the upward and downward directions exhibits a slight increase in angle error with increasing PS bending, but overall remains below 6.2°, with the upward (same-direction) error slightly higher than the downward (opposite-direction) case. In contrast, the DS in the leftward and rightward directions shows a decreasing error trend as PS bending increases, with all errors falling below 3° at a 90° initial PS bend. This suggests that the decoupling strategy maintains reliable performance even in directions with stronger coupling and achieves even better control accuracy in directions with weaker coupling. These findings confirm that the proposed decoupling approach effectively suppresses trajectory deviations and preserves distal posture control, supporting its applicability in complex airway scenarios requiring high-precision robotic intubation.

5.4. Phantom Model Test

To validate the effectiveness of the proposed CM in TI, we employed a life-sized medical airway model, which approximately simulates the upper respiratory tract anatomy of an adult male, as shown in Figure 16a. During the experiment, the CM was first positioned at the oral cavity inlet, and its bending shape was adjusted via process control to accommodate the complex airway pathway. As the CM navigated along the pathway, the PS advanced gradually along the airway, while the DS actively adjusted its posture to adapt to anatomical variations. With the progression of intubation, the distal end of the PS successfully reached the subglottic region, while the tip of the DS smoothly passed through the glottis and entered the trachea, as illustrated in Figure 16b–f. Subsequently, to evaluate the CM’s mobility in different spatial dimensions, we further measured its free-bending characteristics within the airway, as shown in Figure 16g–j. Experimental results indicate that the DS was able to perform precise lateral and vertical adjustments within the trachea, adapting to intubation requirements. The overall experiment validated the CM’s flexibility and adaptability, demonstrating that its distal end could be smoothly guided through the glottis into the trachea with minimal force, effectively reducing the risk of tissue damage during intubation while showcasing its high adaptability and controllability in TI tasks.

6. Conclusions and Discussion

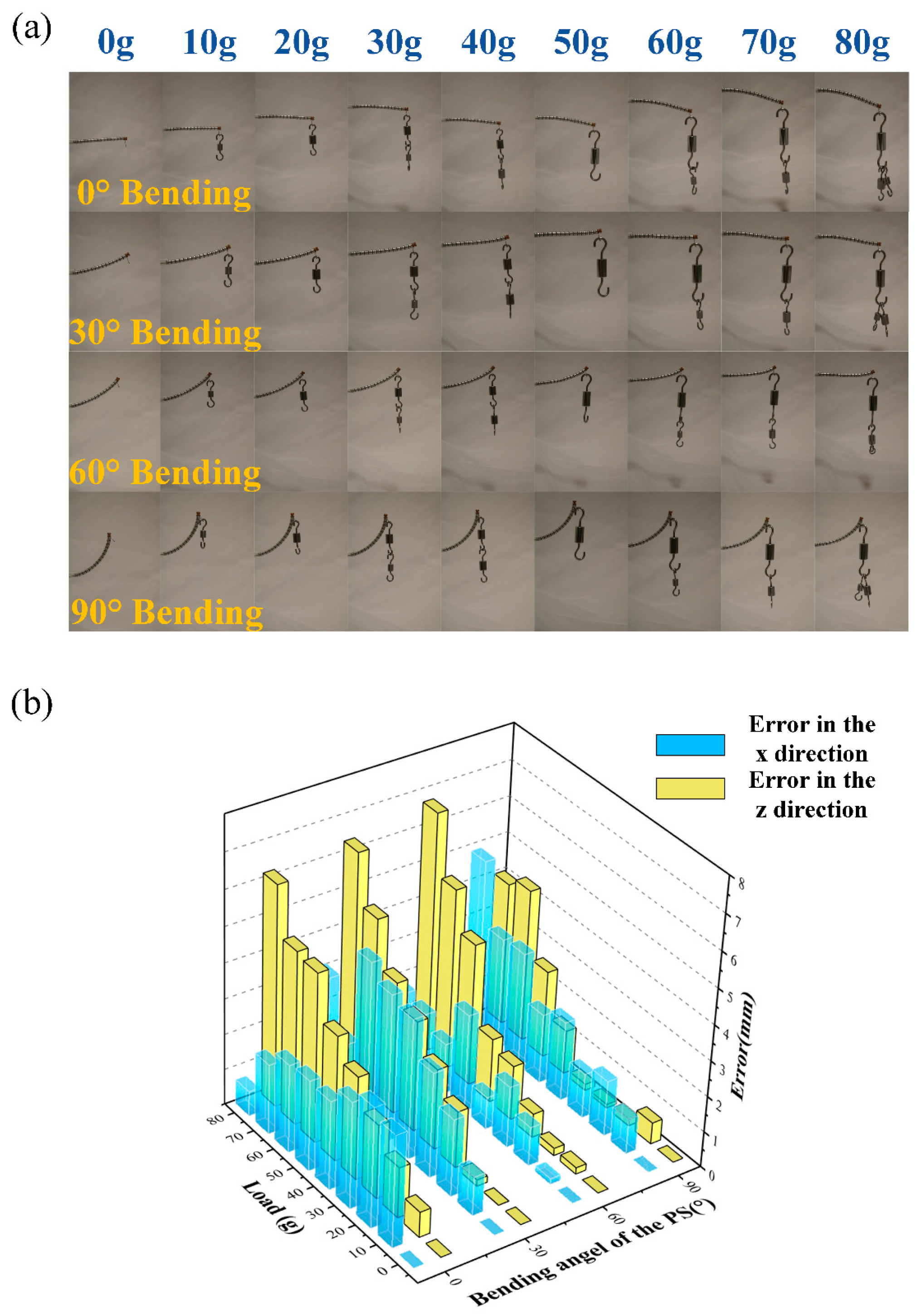
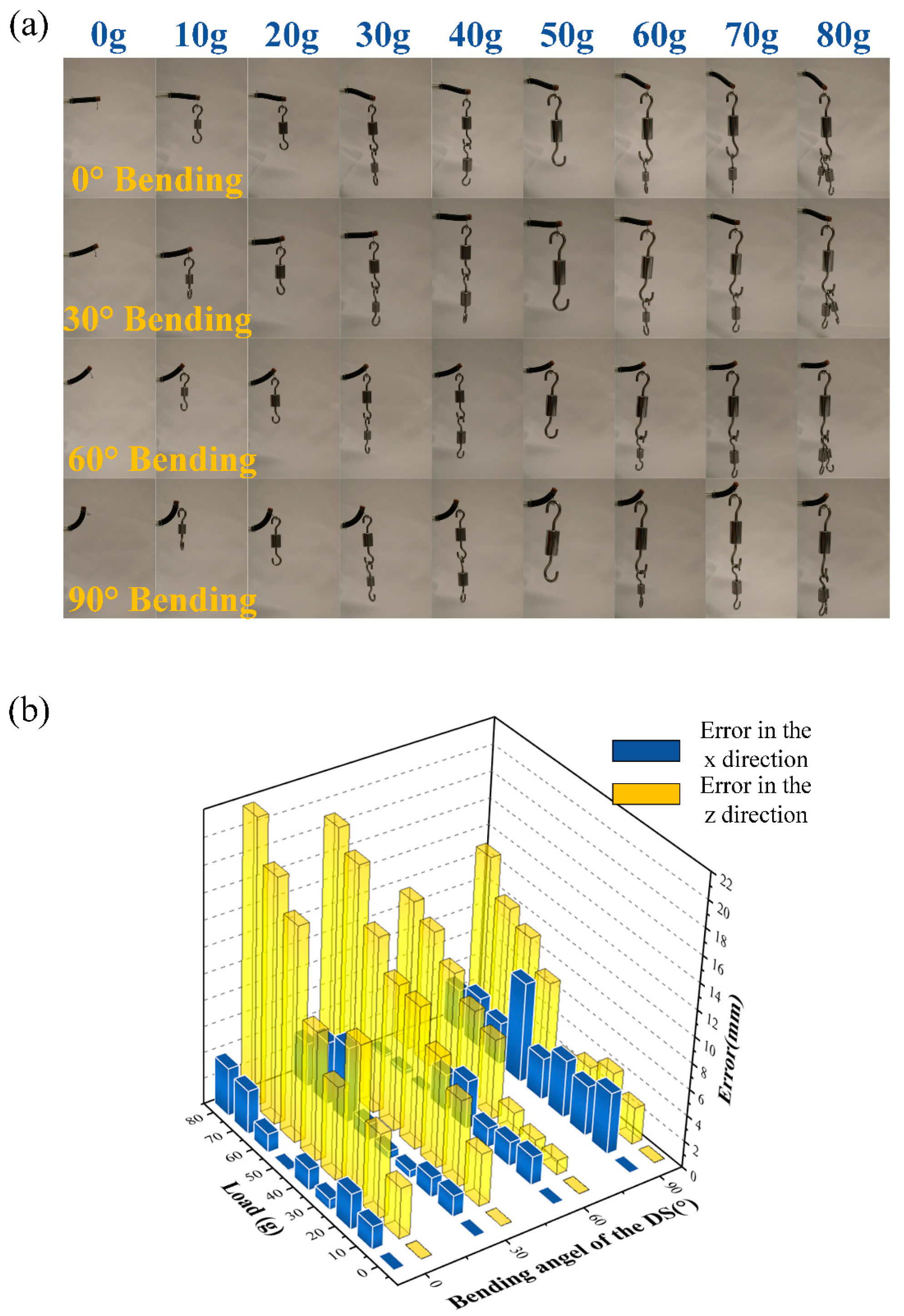
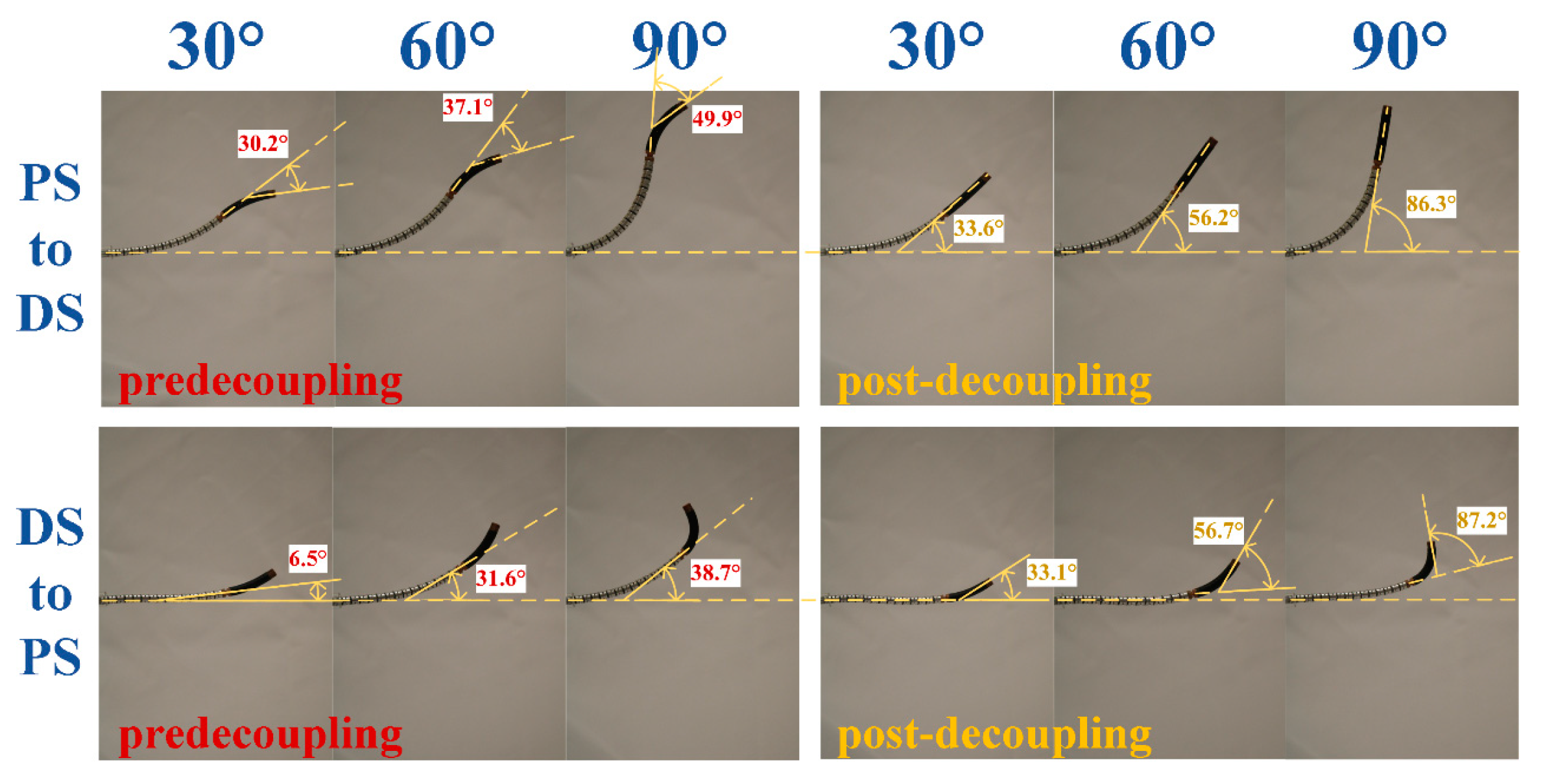
This study proposes a two-segment continuum robot with piecewise stiffness and its decoupling method, aiming to optimize the flexibility, operational precision, and patient safety of the end-effector during TTR procedures. Compared to traditional single-segment continuum structures, this study adopts a dual-segment flexible design, integrating different materials and joint structures to achieve stiffness segmentation and regulation. The experimental results demonstrate that this design effectively enhances stability and flexibility during intubation while reducing the risk of airway tissue damage. Furthermore, this paper further analyzes the coupling effects within the dual-segment continuum structure and proposes an active decoupling method based on independent tendon control, significantly mitigating motion interference between flexible segments and improving precise end-effector control capabilities.

Although the experimental results on intubation accuracy and flexible control are promising, further optimization is still necessary. First, while the current segmented stiffness strategy improves the adaptability of the flexible segments, further studies are needed on the influence of different material combinations and joint structures on stiffness distribution, in order to optimize the overall performance and apply more effective analytical methods [32]. Second, in the active decoupling approach, the nonlinear effects of tendon-driven mechanisms may affect control accuracy. Future work could explore advanced control strategies, such as vision-based or sensor-assisted control, to further enhance the stability of the intubation process [33]. In addition, embodied control and adaptive feedback mechanisms are considered to have potential for improving the autonomy and robustness of continuum robots, and may be integrated with existing control frameworks to support system-level intelligence [34].

Additionally, incorporating navigation planning algorithms may facilitate more intelligent intubation procedures, reducing the workload of medical practitioners and increasing intubation success rates [35]. In conclusion, the proposed dual-segment continuum robot and its decoupling method provide new insights for applications in minimally invasive surgery, airway procedures, and other complex medical scenarios. Future research will focus on further optimizing system performance. For example, the backbone of the CM should be fabricated from materials exhibiting high stiffness and superior elasticity to ensure enhanced postural stability and dynamic responsiveness. Localized encapsulation strategies employing UV-curable adhesives are recommended to achieve effective sealing performance and resistance to fluid ingress. Furthermore, critical components interfacing with biological tissues must utilize medical-grade silicone or equivalent biocompatible materials, ensuring compliance with medical regulatory standards and fulfilling clinical operational requirements.