A Robust Cooperative Control Protocol Based on Global Sliding Mode Manifold for Heterogeneous Nonlinear Multi-Agent Systems Under the Switching Topology
Abstract
1. Introduction
- (a).
- A GSMM for MASs is proposed, which remains unaffected by topology switching. The consensus performance under the sliding mode is determined by the design of the sliding mode manifold parameters. Moreover, order reduction is realized.
- (b).
- A completely distributed control protocol based on the GSMM is proposed. It features a sliding mode control format, a simple structure, and strong robustness. The control protocol and its parameters depend solely on information from neighboring agents.
- (c).
- The proposed cooperative control protocol is applied to WMRs.
2. Preliminary and Problem Formulation
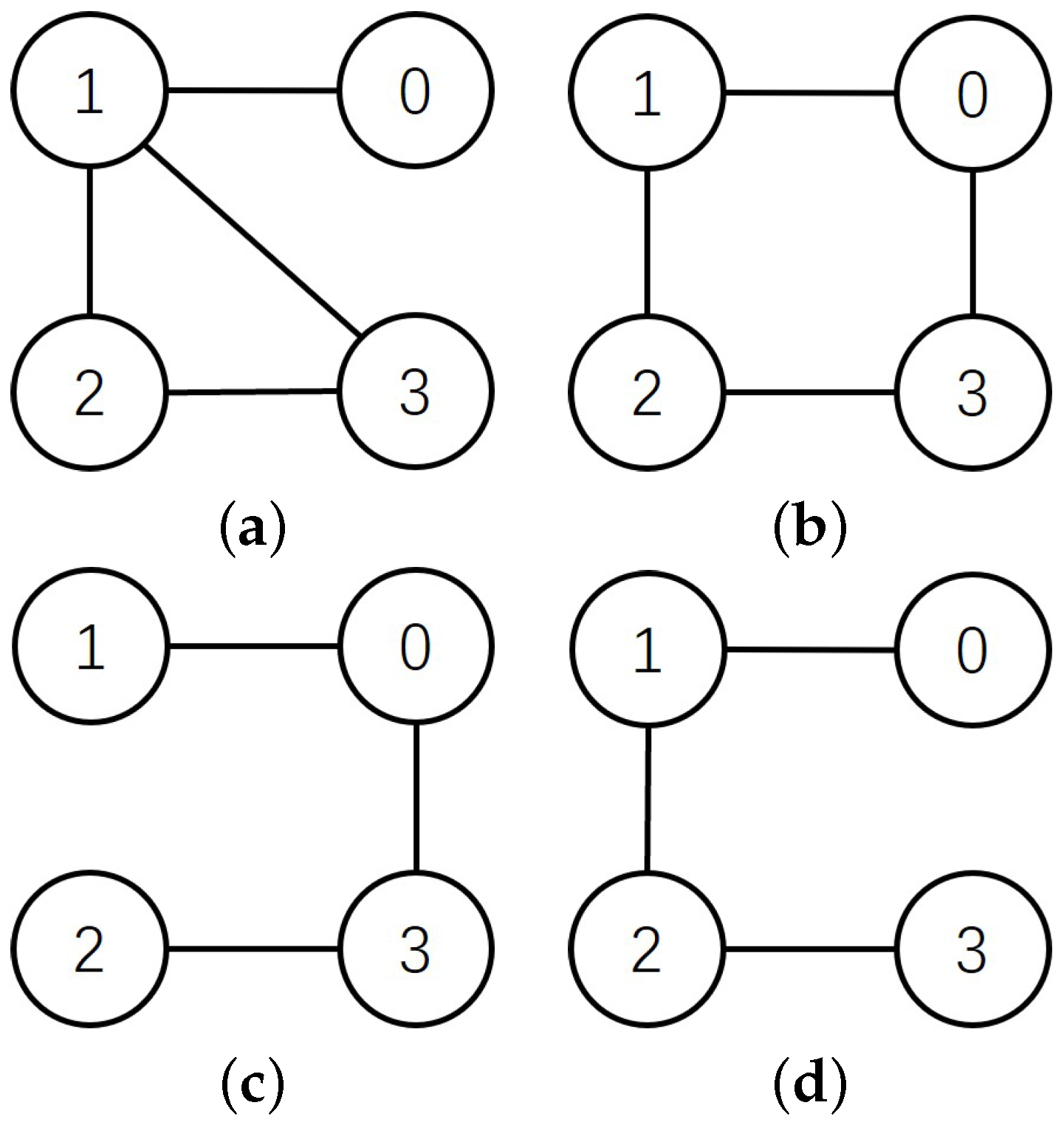
2.1. Graph Theory
2.2. The Consensus Control Problem
3. The Global Sliding Mode Surface Design for the Multi-Agent System
4. The Sliding Mode Control Protocol Design
4.1. The Control Protocol Design
4.2. Reachability of the Sliding Mode
5. Application to Multiple WMRs
5.1. Coordinate Transformation
5.2. The Control Protocol Design
5.3. The Stability of the Sliding Mode
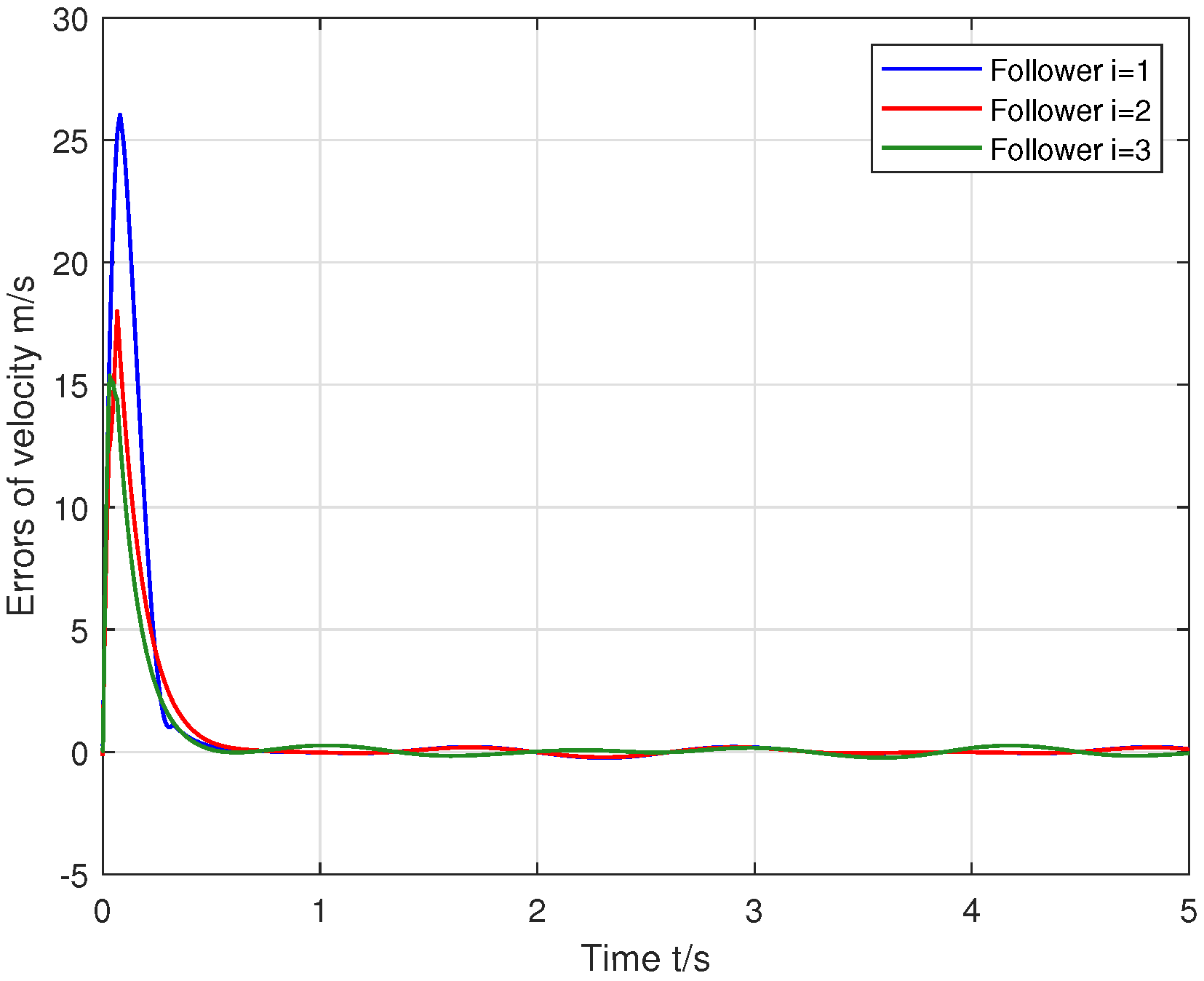
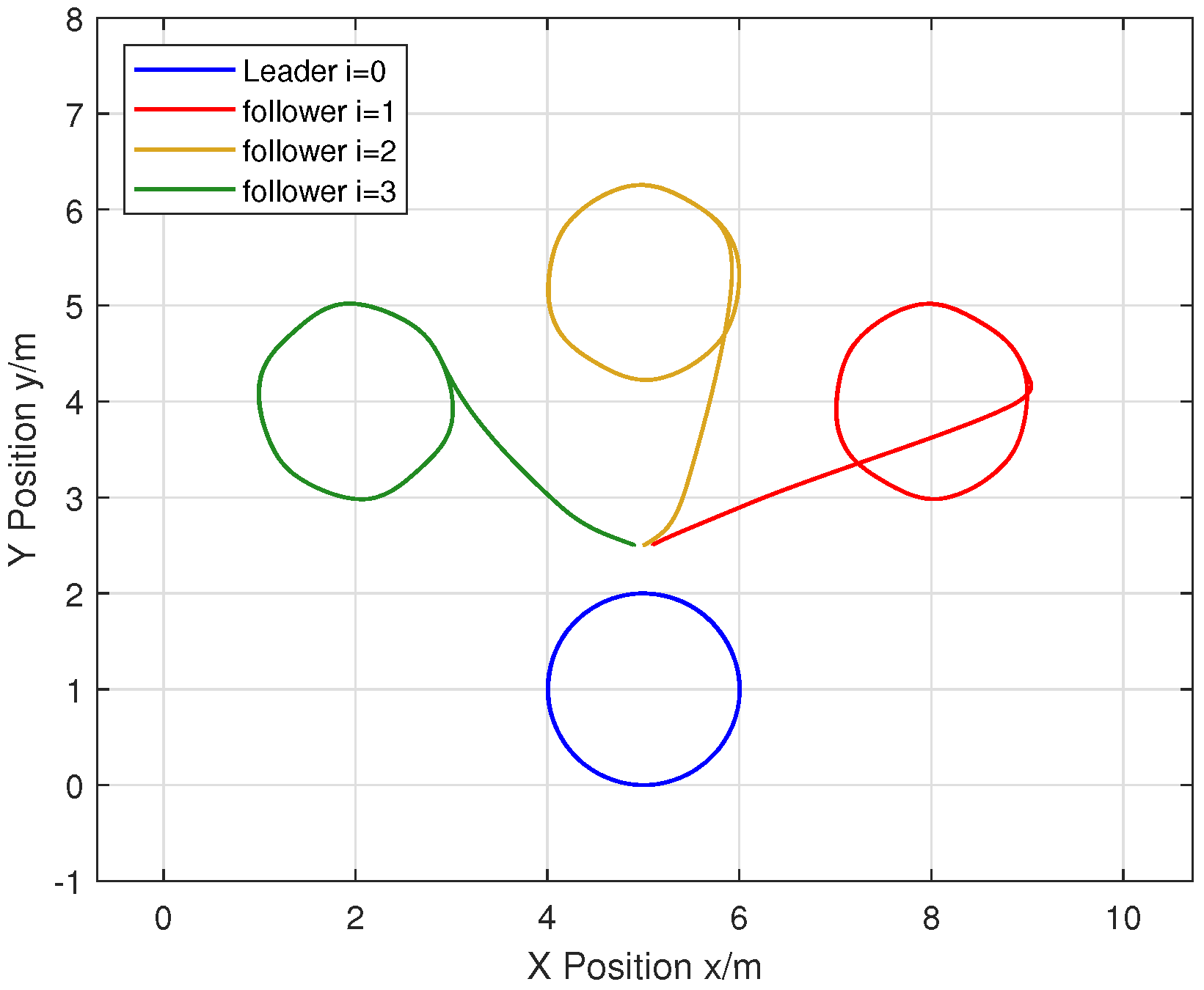
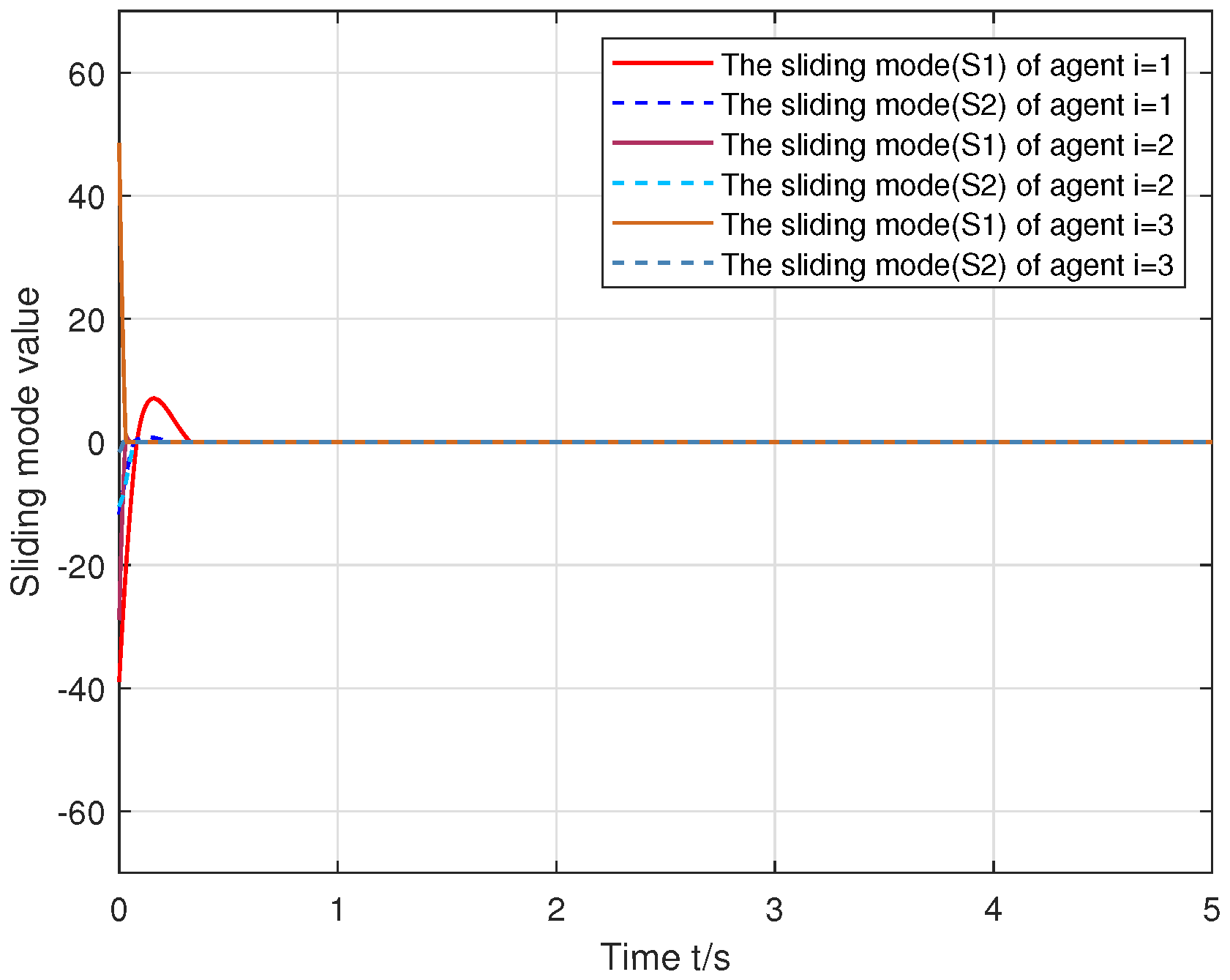
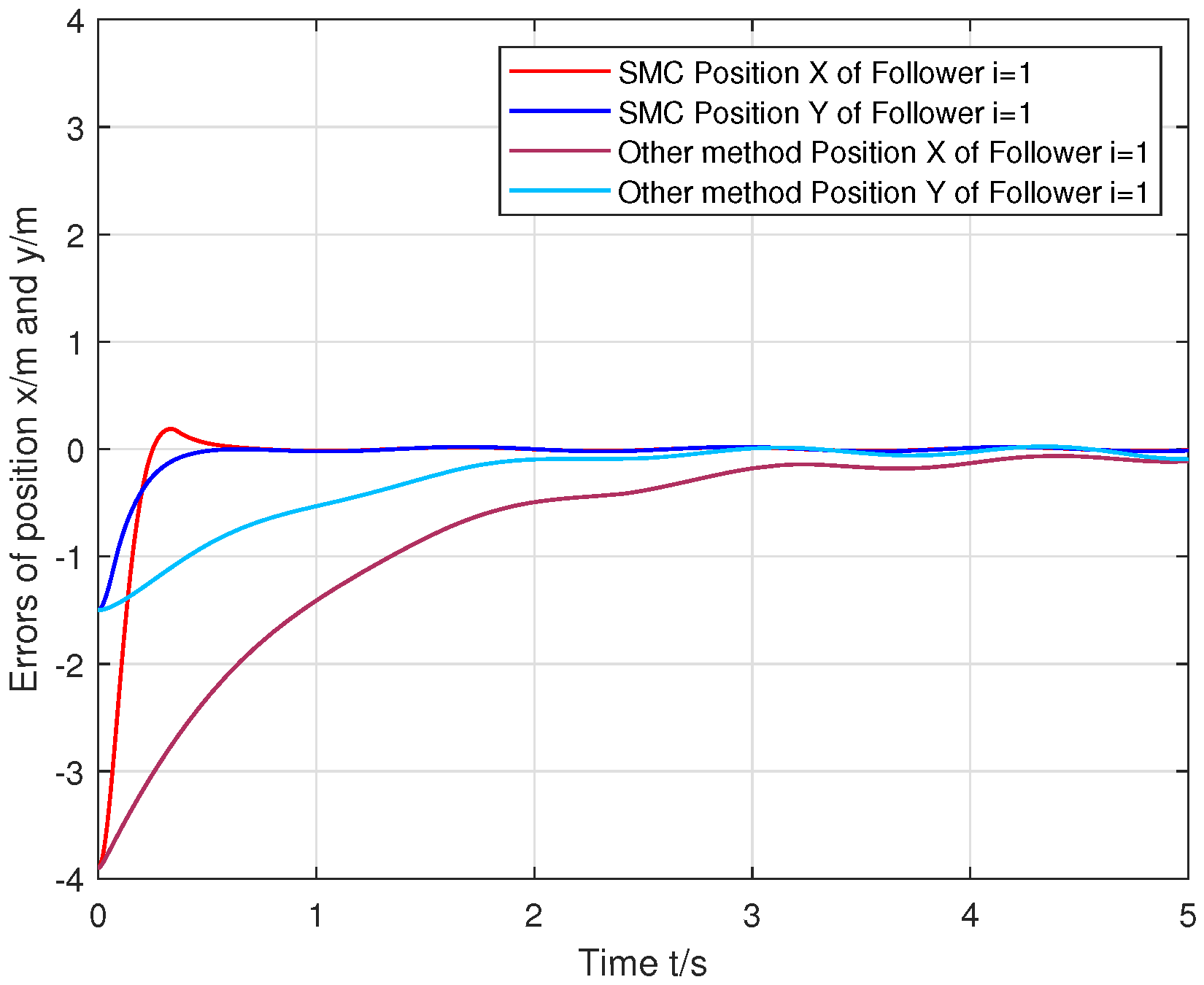
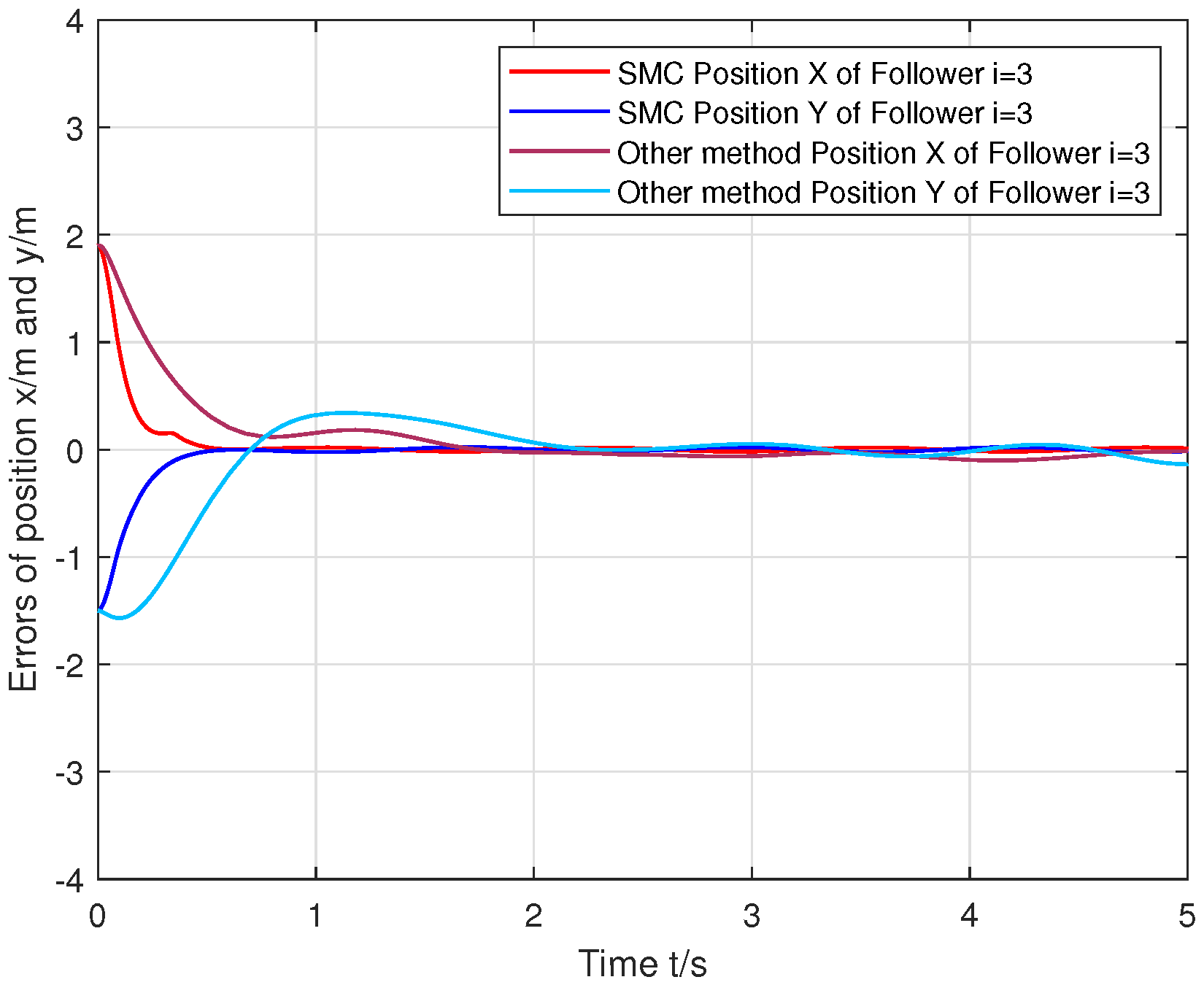
6. Simulation Tests
7. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Liu, Y.; Liu, J.; He, Z.; Li, Z.; Zhang, Q.; Ding, Z. A survey of multi-agent systems on distributed formation control. Unmanned Syst. 2024, 12, 913–926. [Google Scholar] [CrossRef]
- Shah, M.I.A.; Wahid, A.; Barrett, E.; Mason, K. Multi-agent systems in Peer-to-Peer energy trading: A comprehensive survey. Eng. Appl. Artif. Intell. 2024, 132, 107847. [Google Scholar] [CrossRef]
- Jiang, Y.; Liu, L.; Feng, G. Fully distributed adaptive control for output consensus of uncertain discrete-time linear multi-agent systems. Automatica 2024, 162, 111531. [Google Scholar] [CrossRef]
- Zhang, Y.; Wang, Q.; Shen, Y.; Dai, N.; He, B. Multi-AUV cooperative control and autonomous obstacle avoidance study. Ocean Eng. 2024, 304, 117634. [Google Scholar] [CrossRef]
- Nasir, M.; Maiti, A. Adaptive Sliding Mode Resilient Control of Multi-Robot Systems with a Leader–Follower Model under Byzantine Attacks in the Context of the Industrial Internet of Things. Machines 2024, 12, 205. [Google Scholar] [CrossRef]
- Zhao, H.; Liu, M.; Sun, Y.; Chen, Z.; Duan, G.; Cao, X. Automated Design of Fault Diagnosis CNN Network for Satellite Attitude Control Systems. IEEE Trans. Cybern. 2024, 54, 4028–4038. [Google Scholar] [CrossRef] [PubMed]
- Zhang, K.; Zhou, B.; Duan, G.R. Leader-following consensus of multi-agent systems with time delays by fully distributed protocols. Syst. Control Lett. 2023, 178, 105582. [Google Scholar] [CrossRef]
- Zhou, Y.; Wen, G.; Wan, Y.; Fu, J. Consensus tracking control for a class of general linear hybrid multi-agent systems: A model-free approach. Automatica 2023, 156, 111198. [Google Scholar] [CrossRef]
- Wang, J.; Deng, X.; Guo, J.; Zeng, Z. Resilient consensus control for multi-agent systems: A comparative survey. Sensors 2023, 23, 2904. [Google Scholar] [CrossRef] [PubMed]
- Zhou, D.; Chen, W.H.; Lu, X. Leader-Following Consensus of Linear Multiagent Systems with Aperiodically Sampled Outputs: A Distributed Impulsive-Observer-Based Approach. IEEE Trans. Cybern. 2024, 55, 161–171. [Google Scholar] [CrossRef] [PubMed]
- Long, J.; Wang, W.; Wen, C.; Huang, J.; Guo, Y. Output-Feedback-Based Adaptive Leaderless Consensus for Heterogenous Nonlinear Multiagent Systems With Switching Topologies. IEEE Trans. Cybern. 2024, 54, 7905–7918. [Google Scholar] [CrossRef]
- Liu, Y.; Xie, X.; Chadli, M.; Sun, J. Leaderless Consensus Control of Fractional-Order Nonlinear Multi-Agent Systems with Measurement Sensitivity and Actuator Attacks. IEEE Trans. Control Netw. Syst. 2024, 1–10. [Google Scholar] [CrossRef]
- Zhang, W.; Huang, Q.; Alhudhaif, A. Event-triggered fixed-time bipartite consensus for nonlinear disturbed multi-agent systems with leader-follower and leaderless controller. Inf. Sci. 2024, 662, 120243. [Google Scholar] [CrossRef]
- Rezaee, H.; Abdollahi, F. Average consensus over high-order multiagent systems. IEEE Trans. Autom. Control 2015, 60, 3047–3052. [Google Scholar] [CrossRef]
- Chen, T.; Wang, F.; Feng, M.; Xia, C.; Chen, Z. Fully distributed consensus of linear multi-agent systems via dynamic event-triggered control. Neurocomputing 2024, 569, 127129. [Google Scholar] [CrossRef]
- Liu, Y.J.; Shang, X.; Tang, L.; Zhang, S. Finite-Time Consensus Adaptive Neural Network Control for Nonlinear Multiagent Systems Under PDE Models. IEEE Trans. Neural Netw. Learn. Syst. 2024, 1–11. [Google Scholar] [CrossRef]
- Luo, Y.; Huang, W.; Cao, J.; Cao, Z. Finite-time consensus of second-order multi-agent connectivity preserving based on adaptive sliding mode control. Commun. Nonlinear Sci. Numer. Simul. 2024, 137, 108142. [Google Scholar] [CrossRef]
- Zhuang, J.; Peng, S.; Peng, H. Finite-time and fixed-time consensus of nonlinear multi-agent systems: A unified two-phase control. Int. J. Robust Nonlinear Control 2024, 34, 9438–9455. [Google Scholar] [CrossRef]
- Wang, C.; Zhan, H.; Guo, Q.; Li, T. Distributed Neural Fixed-Time Consensus Control of Uncertain Multiple Euler-Lagrange Systems with Event-Triggered Mechanism. IEEE/ASME Trans. Mechatron. 2024, 1–12. [Google Scholar] [CrossRef]
- Li, H.; Niu, G.; Chen, Y. Fixed-time consensus of leader-following multi-agent systems subject to failed follower: Reconstructed topology approach. Appl. Math. Comput. 2024, 482, 128955. [Google Scholar] [CrossRef]
- Wang, Q.; Wu, W. A distributed finite-time optimization algorithm for directed networks of continuous-time agents. Int. J. Robust Nonlinear Control 2024, 34, 4032–4050. [Google Scholar] [CrossRef]
- He, S.; Wang, H.; Yu, W. Distributed Fast Finite-Time Tracking Consensus of Multi-Agent Systems With a Dynamic Leader. IEEE Trans. Circuits Syst. II Express Briefs 2022, 69, 2176–2180. [Google Scholar] [CrossRef]
- Razmjooei, H.; Shafiei, M.H. Partial finite-time stabilization of perturbed nonlinear systems based on the novel concept of nonsingular terminal sliding mode method. J. Comput. Nonlinear Dyn. 2020, 15, 021005. [Google Scholar] [CrossRef]
- Chu, Y.; Fei, J.; Hou, S. Adaptive neural backstepping PID global sliding mode fuzzy control of MEMS gyroscope. IEEE Access 2019, 7, 37918–37926. [Google Scholar] [CrossRef]
- Cai, J.; Yi, C.; Wu, Y.; Liu, D.; Zhong, D. Leader-following consensus of nonlinear singular switched multi-agent systems via sliding mode control. Asian J. Control 2024, 26, 1997–2010. [Google Scholar] [CrossRef]
- Ma, Y.; Zhan, X.; Yang, Q.; Yan, H. Finite-time Consensus of Heterogeneous Multi-agent Systems by Integral Sliding Mode Control. Int. J. Control Autom. Syst. 2024, 22, 1819–1826. [Google Scholar] [CrossRef]
- Nie, R.; Du, W.; Li, Z.; He, S. Sliding mode-based finite-time consensus tracking control for multi-agent systems under actuator attacks. Inf. Sci. 2023, 640, 118971. [Google Scholar] [CrossRef]
- Jin, D.; Xiang, Z. Predefined-Time Consensus for Second-Order Nonlinear Multiagent Systems via Sliding Mode Technique. IEEE Trans. Fuzzy Syst. 2024, 32, 4534–4541. [Google Scholar] [CrossRef]
- Song, Y.; Li, Z.; Li, B.; Wen, G. Optimized leader-follower consensus control using combination of reinforcement learning and sliding mode mechanism for multiple robot manipulator system. Int. J. Robust Nonlinear Control 2024, 34, 5212–5228. [Google Scholar] [CrossRef]
- Li, X.; Zhu, Y.; Zhao, X.; Lu, J. Bearing-Based Prescribed Time Formation Tracking for Second-Order Multi-Agent Systems. IEEE Trans. Circuits Syst. II Express Briefs 2022, 69, 3259–3263. [Google Scholar] [CrossRef]




Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhang, X.; Li, Y.; Xiong, S.; Liu, X.; Guo, R. A Robust Cooperative Control Protocol Based on Global Sliding Mode Manifold for Heterogeneous Nonlinear Multi-Agent Systems Under the Switching Topology. Actuators 2025, 14, 57. https://doi.org/10.3390/act14020057
Zhang X, Li Y, Xiong S, Liu X, Guo R. A Robust Cooperative Control Protocol Based on Global Sliding Mode Manifold for Heterogeneous Nonlinear Multi-Agent Systems Under the Switching Topology. Actuators. 2025; 14(2):57. https://doi.org/10.3390/act14020057
Chicago/Turabian StyleZhang, Xiaoyu, Yining Li, Shuiping Xiong, Xiangbin Liu, and Rong Guo. 2025. "A Robust Cooperative Control Protocol Based on Global Sliding Mode Manifold for Heterogeneous Nonlinear Multi-Agent Systems Under the Switching Topology" Actuators 14, no. 2: 57. https://doi.org/10.3390/act14020057
APA StyleZhang, X., Li, Y., Xiong, S., Liu, X., & Guo, R. (2025). A Robust Cooperative Control Protocol Based on Global Sliding Mode Manifold for Heterogeneous Nonlinear Multi-Agent Systems Under the Switching Topology. Actuators, 14(2), 57. https://doi.org/10.3390/act14020057





