Comparative Study of Mechanical Scaling Effects of Origami-Inspired Motion Generation Mechanisms with Multi-Degree Vertices
Abstract
1. Introduction
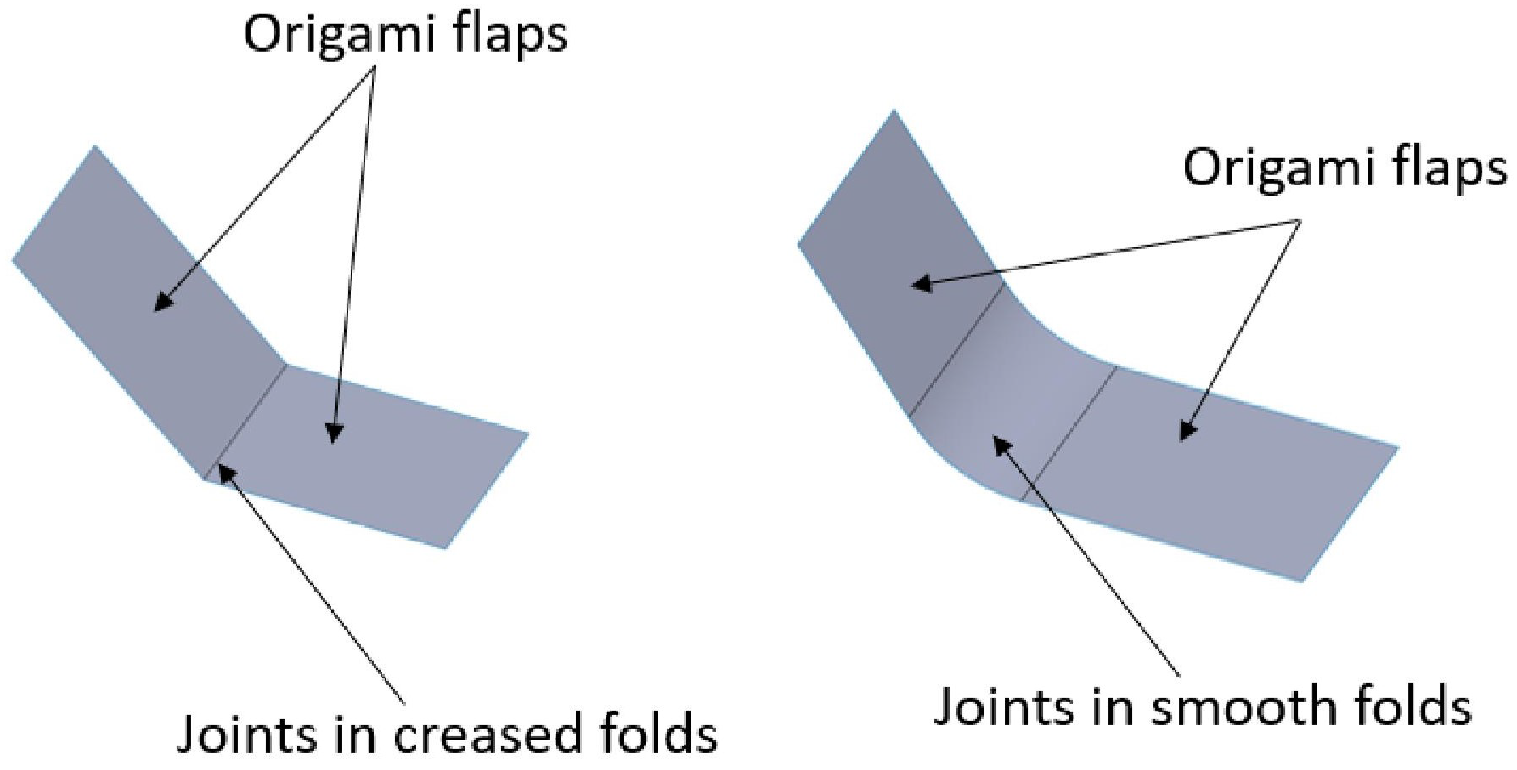
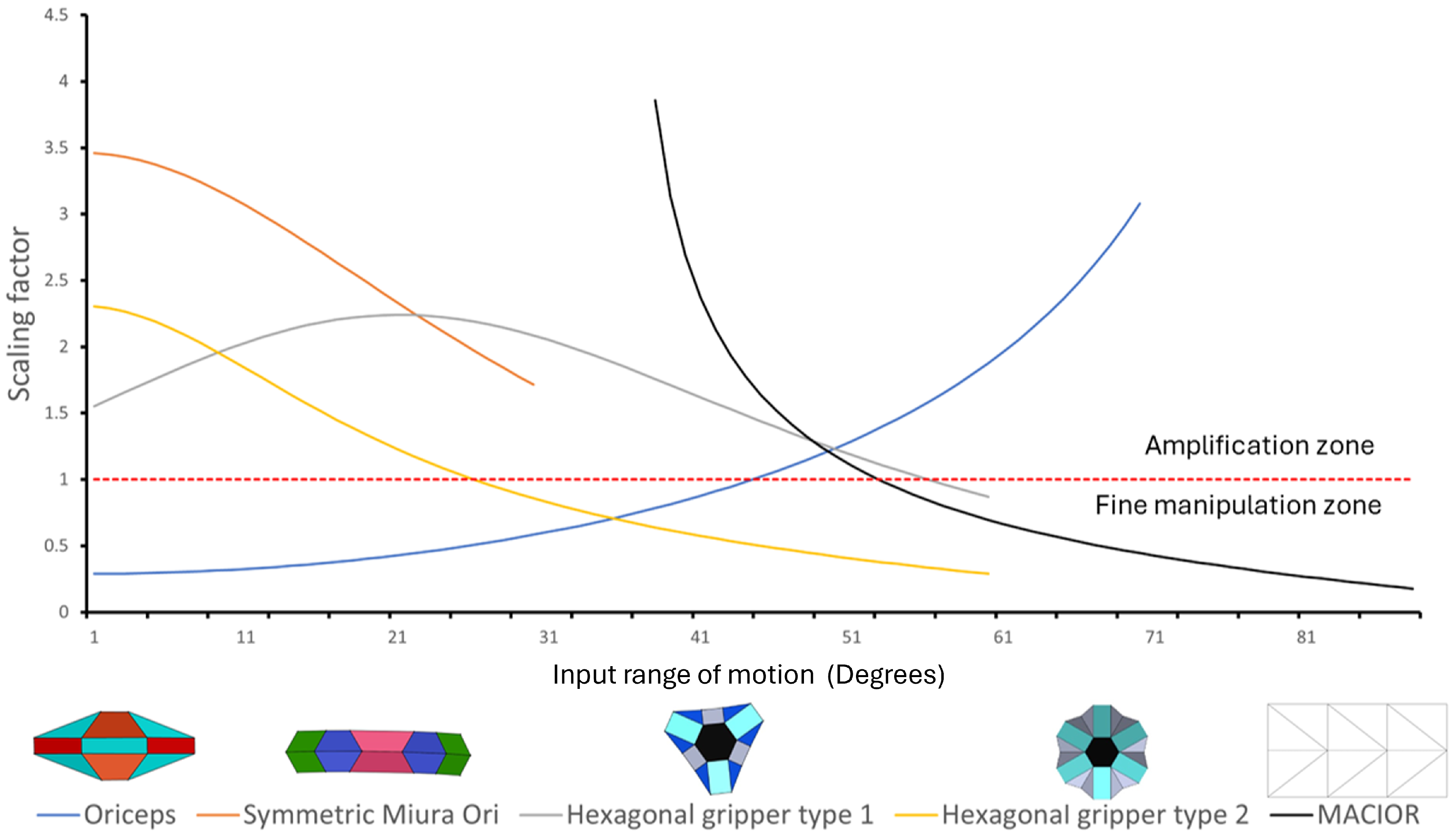
2. Scaling Factor of Origami Structures
3. Oriceps
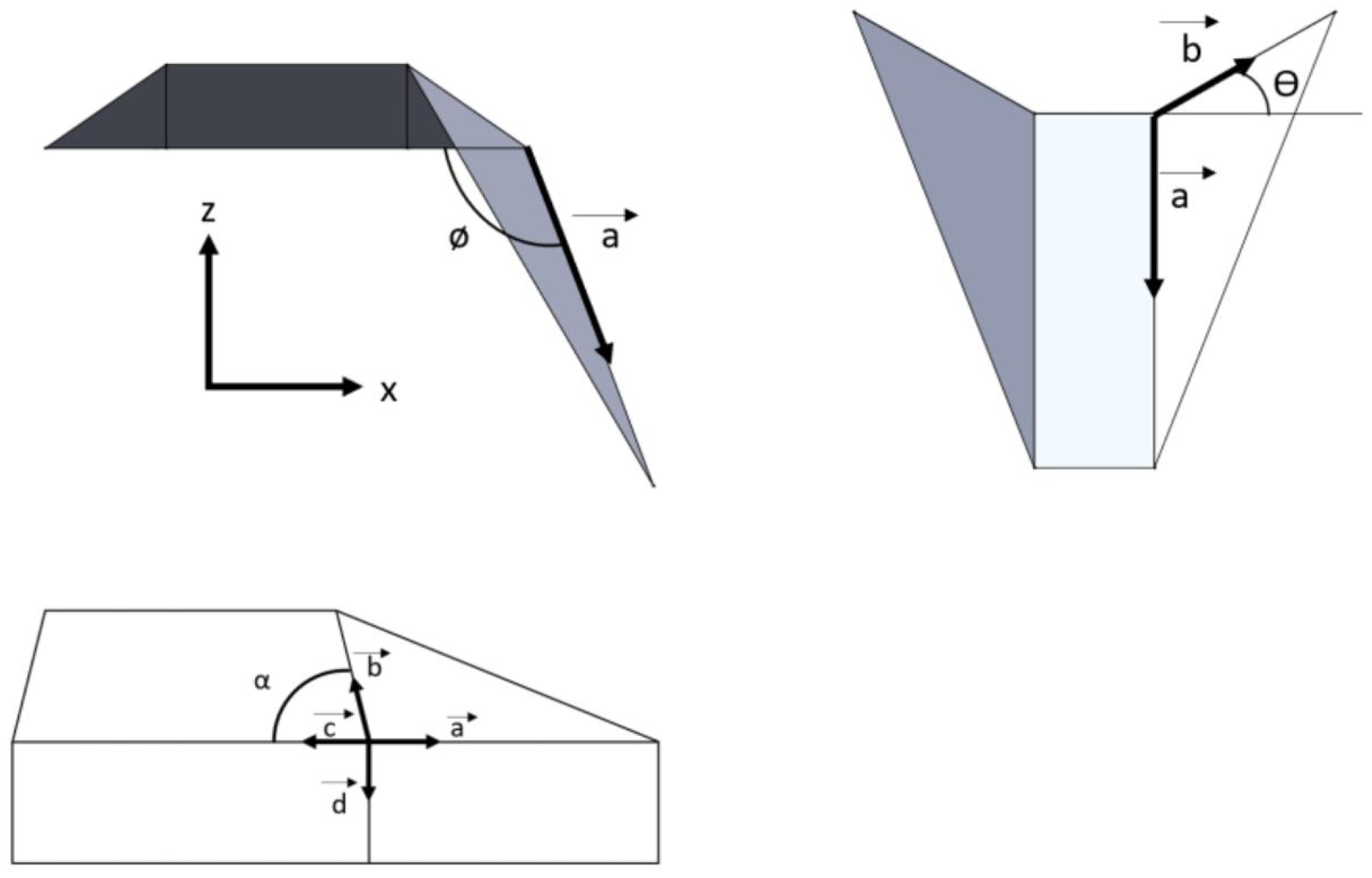
3.1. Kinematics
3.2. Scaling Factor
4. Miura Ori String
4.1. Kinematics
4.2. Scaling Factor
5. MACIOR Origami with a Six-Degree Vertex
5.1. Kinematics
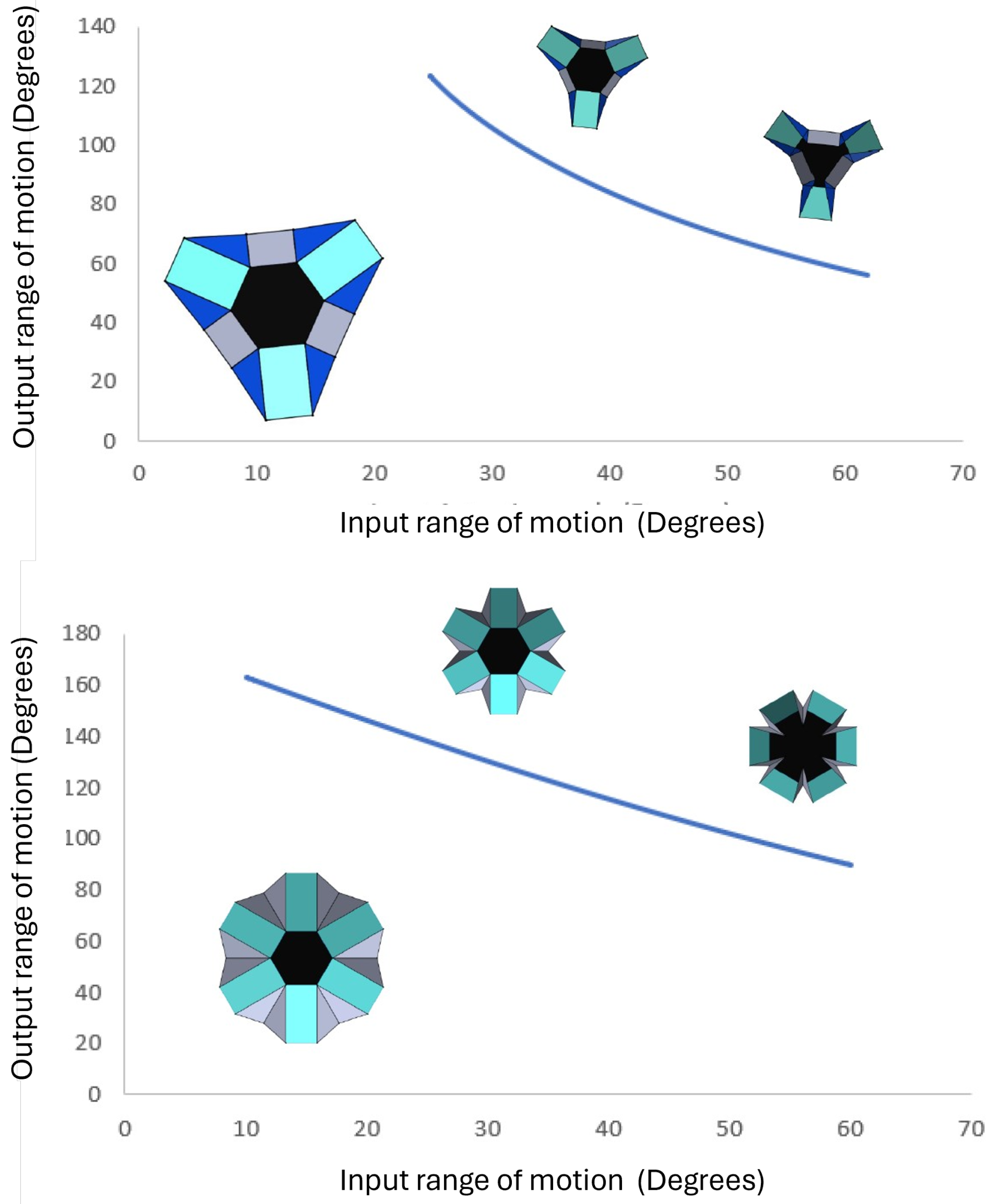
5.2. Scaling Factor
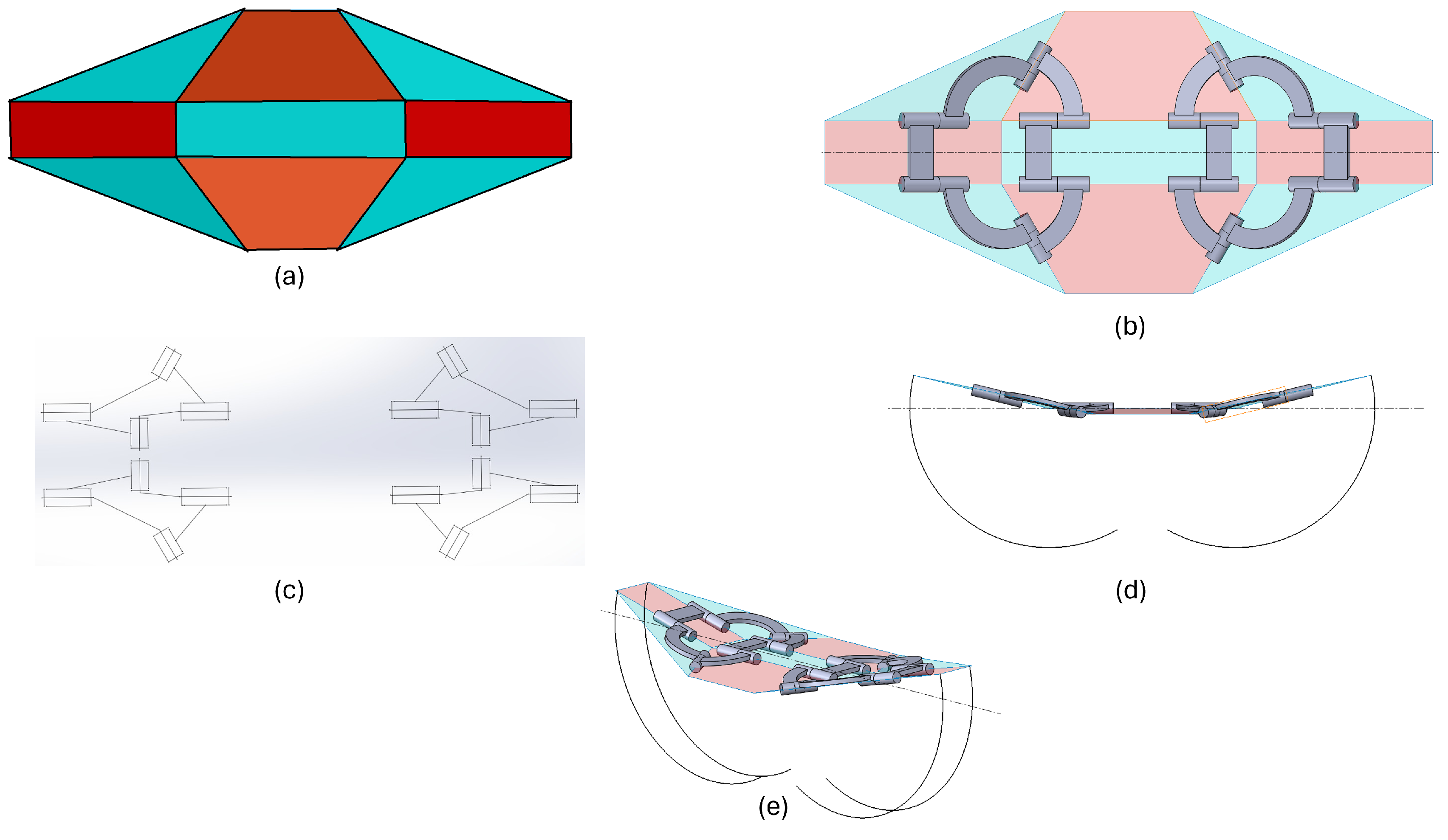
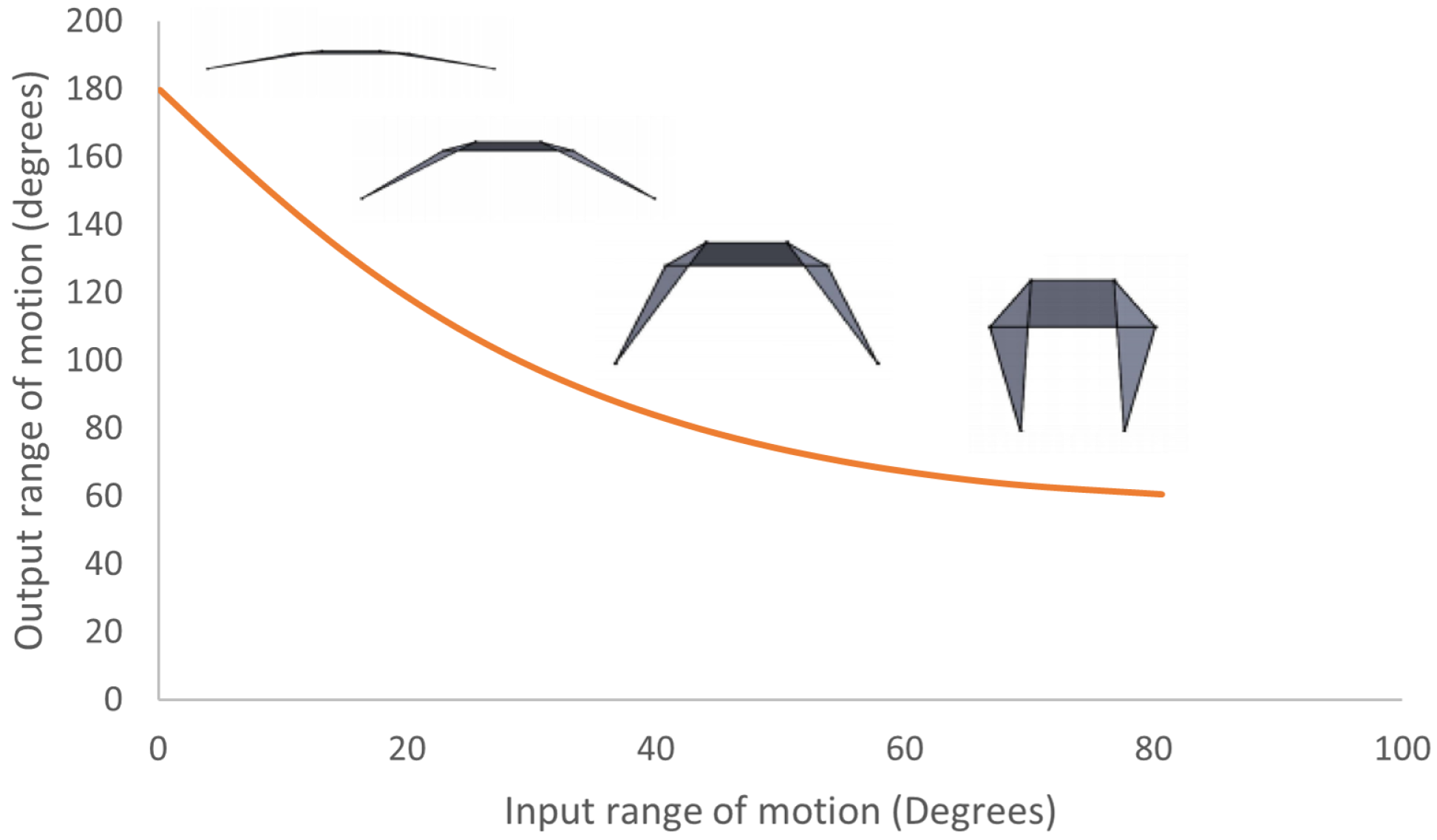
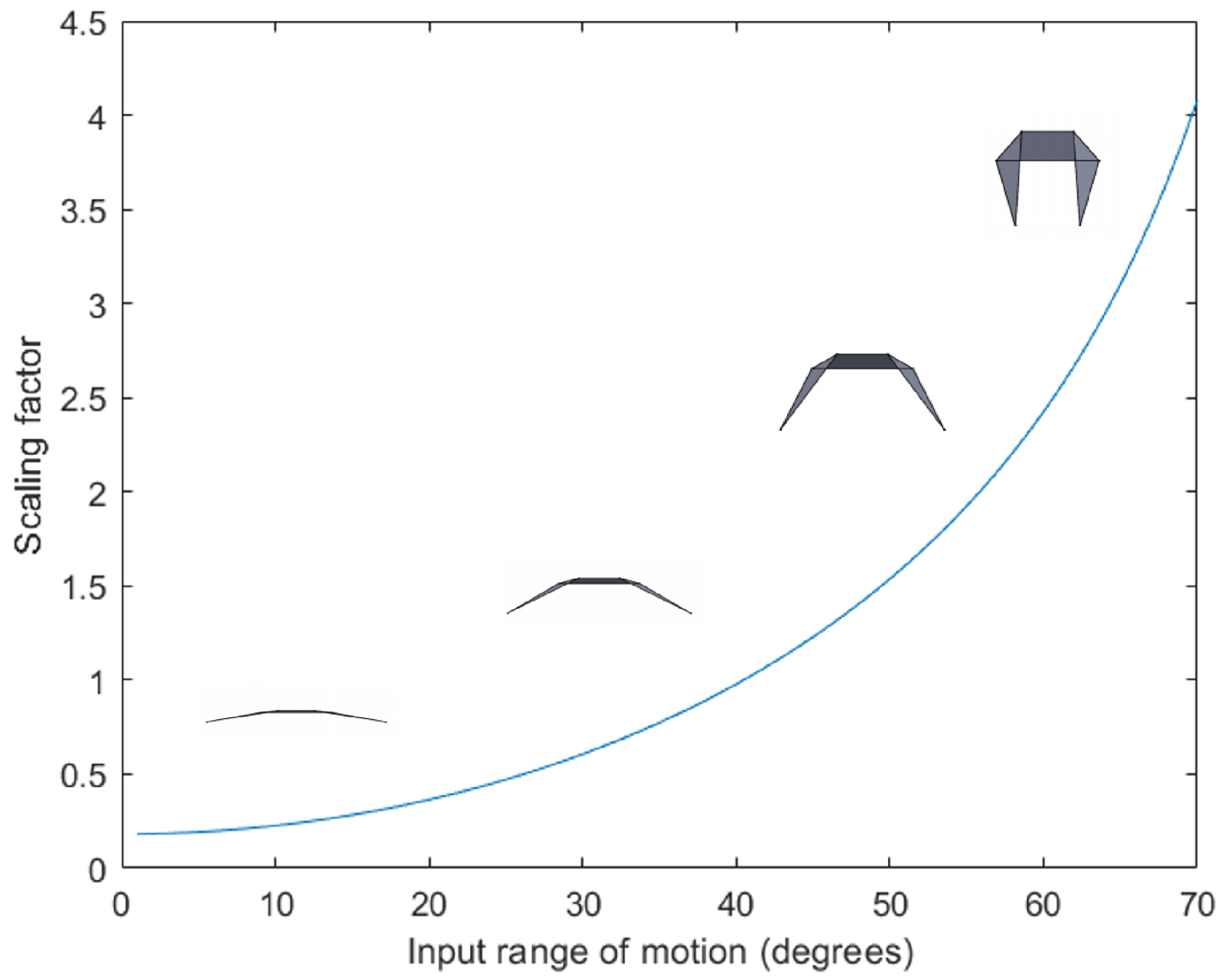
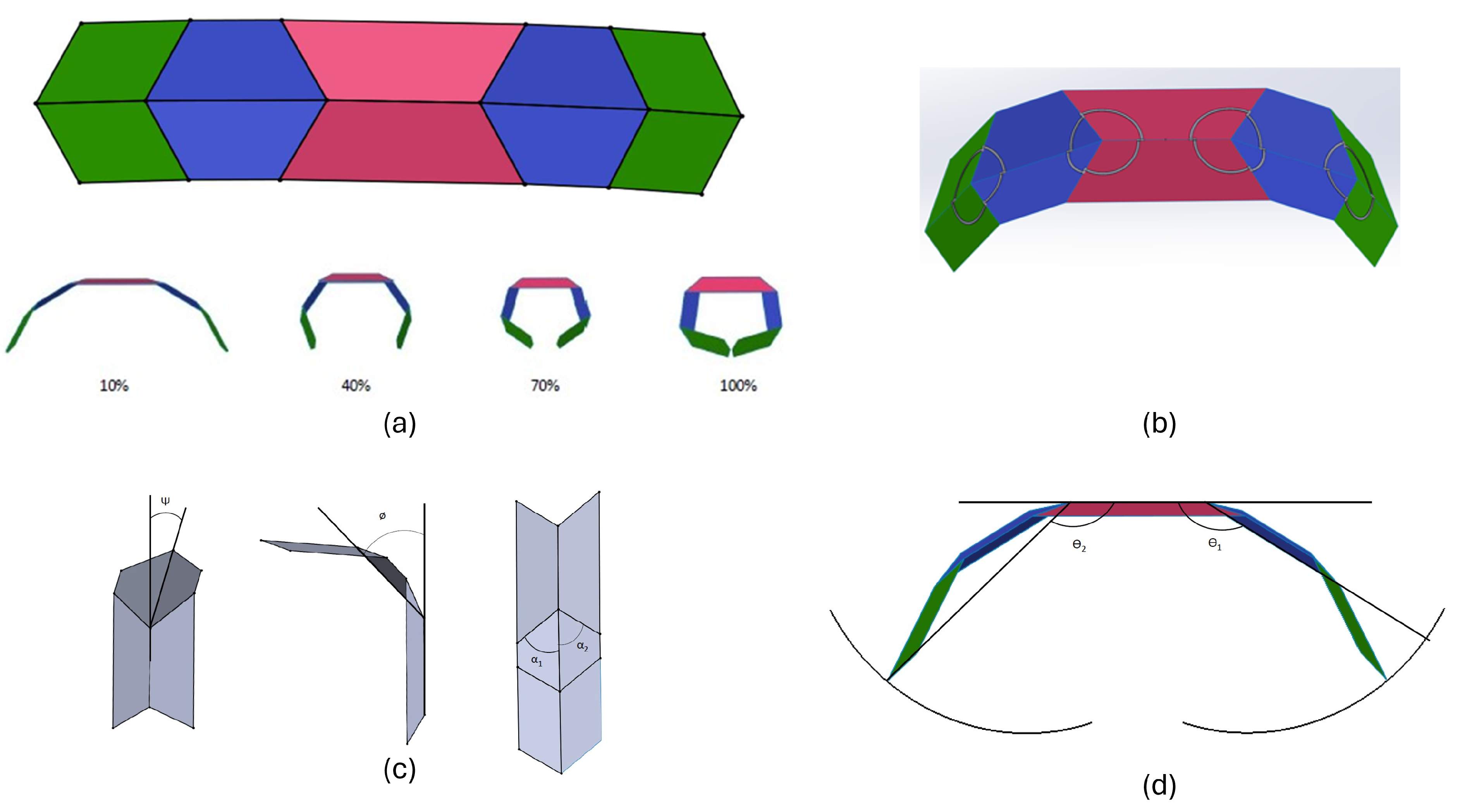
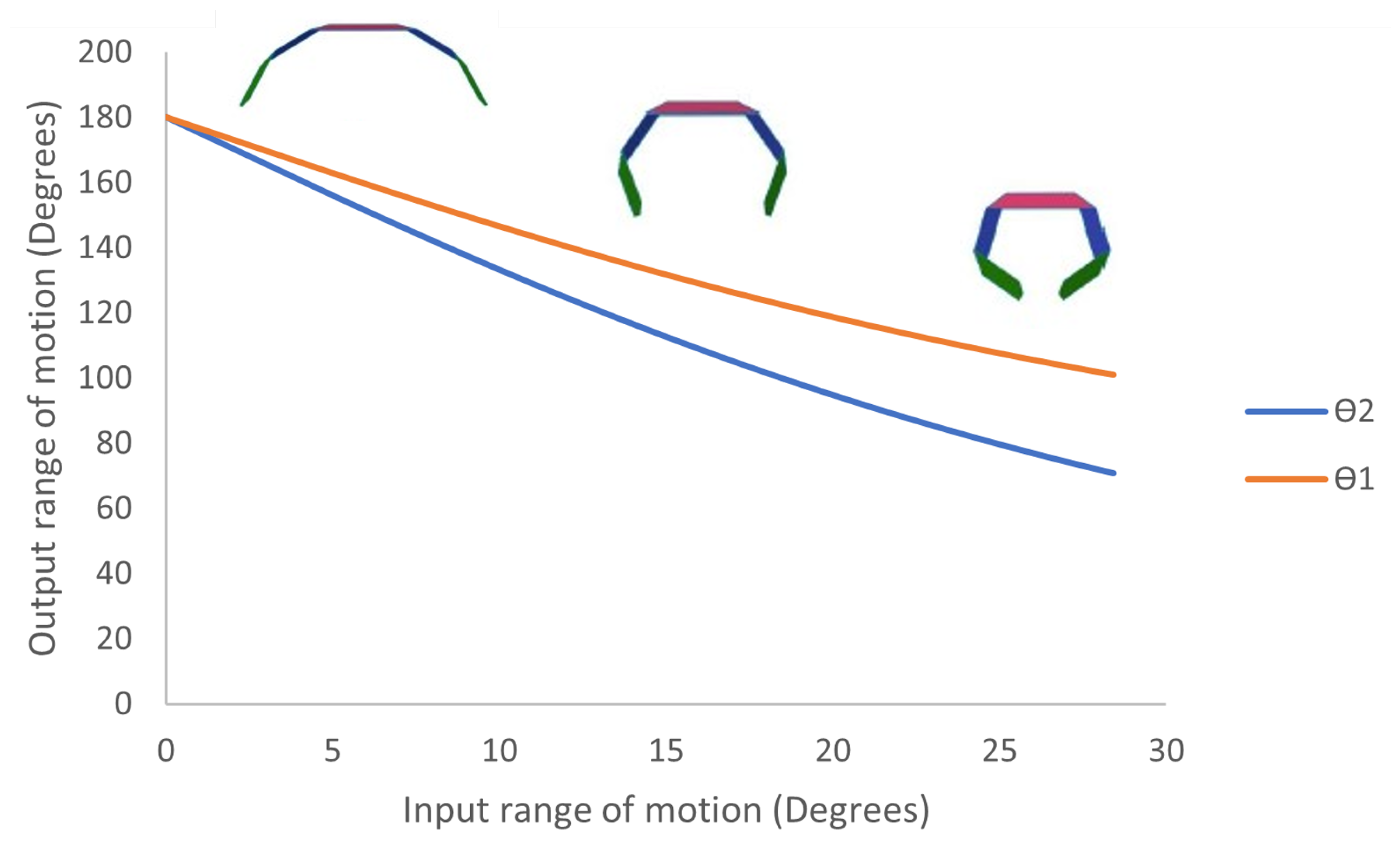
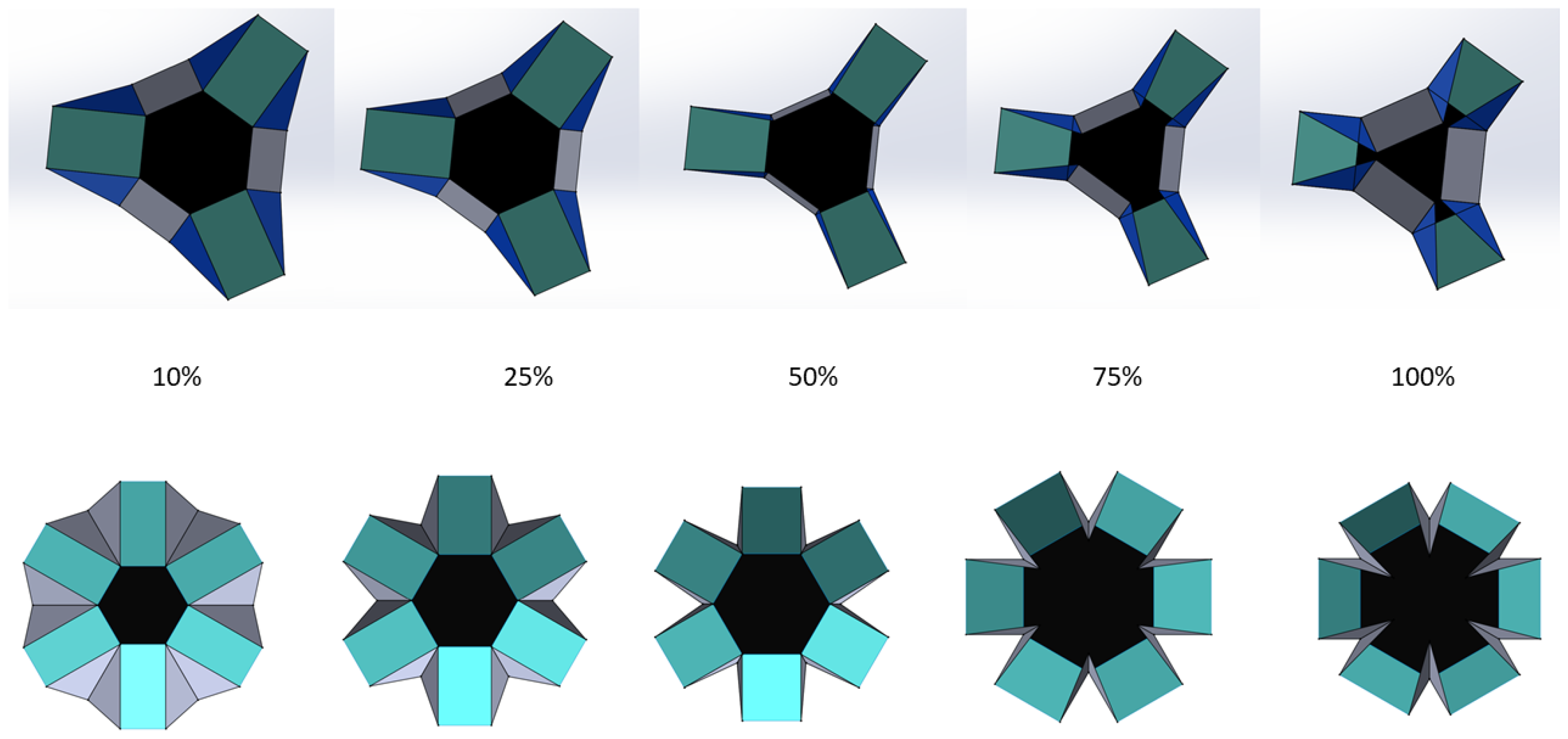
6. Hexagonal Structures
6.1. Kinematics
6.1.1. Type 1
6.1.2. Type 2
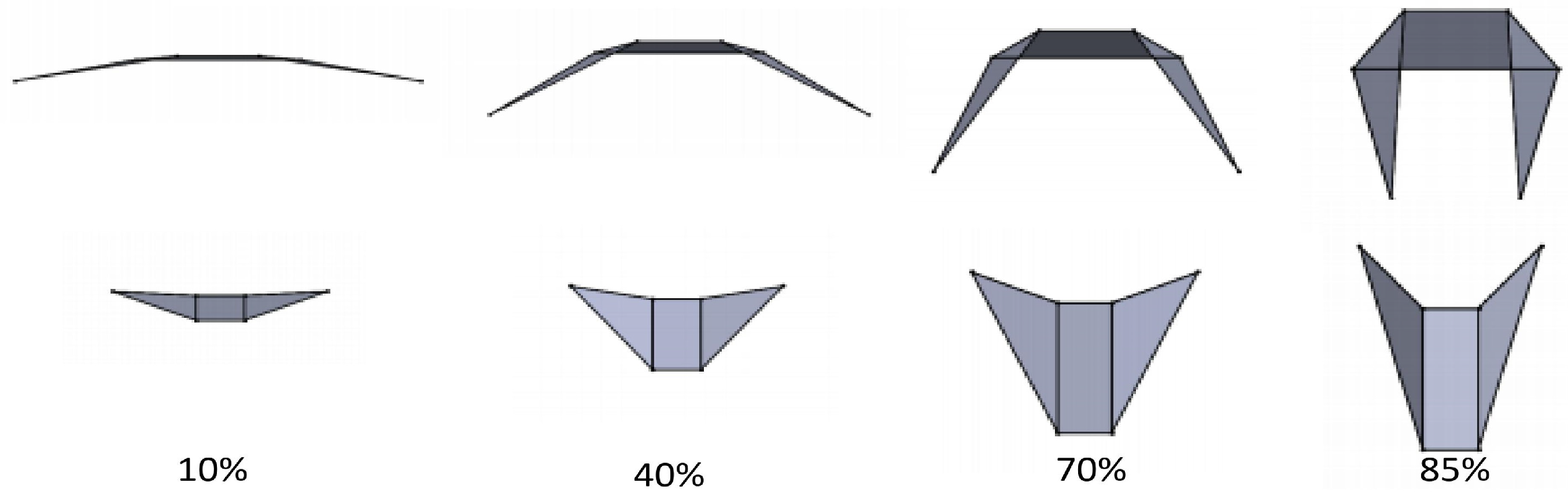
6.2. Scaling Factor
6.2.1. Type 1
6.2.2. Type 2
7. Comparison of Different Structures
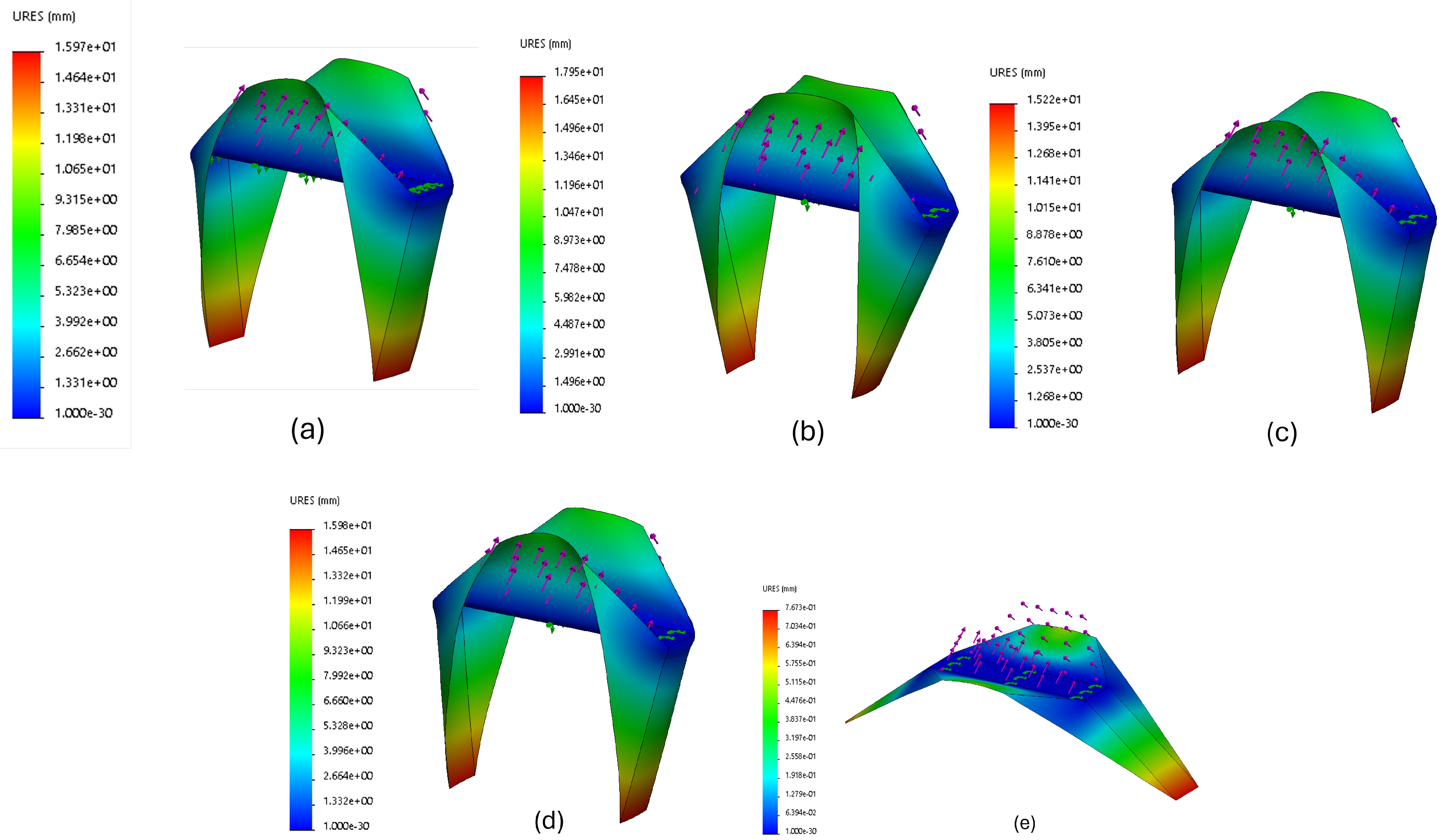
7.1. Simulation of Oriceps with Different Materials
7.2. Potential Design Concept and Usage in Four-Bar Mechanisms
7.3. Emerging Trends in Origami
8. Conclusions and Discussions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Turner, N.; Goodwine, B.; Sen, M. A review of origami applications in mechanical engineering. Proc. Inst. Mech. Eng. Part J. Mech. Eng. Sci. 2016, 230, 2345–2362. [Google Scholar] [CrossRef]
- Balkcom, D.; Mason, M. Robotic origami folding. Int. J. Robot. Res. 2008, 27, 613–627. [Google Scholar] [CrossRef]
- Sapienza, V.; Rodonò, G. Kinetic Architecture and Foldable Surface. Athens J. Archit. 2016, 2, 223–236. [Google Scholar] [CrossRef]
- Nelson, T.G.; Zimmerman, T.K.; Magleby, S.P.; Lang, R.J.; Howell, L.L. Developable mechanisms on developable surfaces. Sci. Robot. 2019, 4, eaau5171. [Google Scholar] [CrossRef] [PubMed]
- Yao, W.; Dai, J. Dexterous manipulation of origami cartons with robotic fingers based on the interactive configuration space. J. Mech. Des. 2008, 130, 022303. [Google Scholar] [CrossRef]
- Chen, Y.; Peng, R.; You, Z. Origami of thick panels. Science 2015, 349, 396–400. [Google Scholar] [CrossRef] [PubMed]
- Edmondson, B.J.; Bowen, L.A.; Grames, C.L.; Magleby, S.P.; Howell, L.L.; Bateman, T.C. Oriceps: Origami-Inspired Forceps. In Smart Materials, Adaptive Structures and Intelligent Systems; American Society of Mechanical Engineers: New York, NY, USA, 2013; Volume 1. [Google Scholar] [CrossRef]
- von der Woerd, J.D.; Chudoba, R.; Hegger, J. Single-curved shell structure made out of textile-reinforced concrete plate using a folding technique. Proc. Iass Annu. Symp. 2013, 2013, 1–6. [Google Scholar]
- Yang, H.; Yeow, B.; Chang, T.; Li, K.; Fu, F.; Chen, P. Graphene Oxide-Enabled Synthesis of Metal Oxide Origamis for Soft Robotics. ACS Nano 2019, 13, 5410–5420. [Google Scholar] [CrossRef]
- Lee, M.J.T.; Yeow, B.S.; Cai, C.J.; Tse, Z.T.H.; Ren, H. Prototyping soft origami quad-bellows robots from single-bellows characterization. In Flexible Robotics in Medicine: A Design Journey of Motion Generation Mechanisms and Biorobotic System Development; Academic Press: Cambridge, MA, USA, 2020; pp. 19–37. [Google Scholar]
- Thomson, A.; Hao, C.T.S.; Yanhui, L.O. Modular origami joint operator to create bendable motions with multiple radii. In Flexible Robotics in Medicine: A Design Journey of Motion Generation Mechanisms and Biorobotic System Development; Academic Press: Cambridge, MA, USA, 2020; pp. 101–148. [Google Scholar]
- Rus, D.; Tolley, M. Design, fabrication and control of origami robots. Nat. Rev. Mater. 2018, 3, 101–112. [Google Scholar] [CrossRef]
- Abdul-Sater, K.; Winkler, M.M.; Irlinger, F.; Lueth, T.C. Three-Position Synthesis of Origami-Evolved, Spherically Constrained Spatial Revolute–Revolute Chains. J. Mech. Robot. 2015, 8, 011012. [Google Scholar] [CrossRef]
- Banerjee, H.; Kakde, S.; Ren, H. OrumBot: Origami-Based Deformable Robot Inspired by an Umbrella Structure. In Proceedings of the 2018 IEEE International Conference on Robotics and Biomimetics (ROBIO), Kuala Lumpur, Malaysia, 12–15 December 2018; pp. 910–915. [Google Scholar] [CrossRef]
- Matsuo, H.; Asada, H.H.; Takeda, Y. Design of a Novel Mutliple-DOF Extendable Arm With Rigid Components Inspired by a Deployable Origami Structure. IEEE Robot. Autom. Lett. 2020, 5, 2730–2737. [Google Scholar] [CrossRef]
- Lang, R.J.; Magleby, S.; Howell, L. Single Degree-of-Freedom Rigidly Foldable Cut Origami Flashers. J. Mech. Robot. 2016, 8, 031005. [Google Scholar] [CrossRef]
- Zimmermann, L.; Stankovic, T. Rigid and Flat Foldability of a Degree-Four Vertex in Origami. J. Mech. Robot. 2020, 12, 011004. [Google Scholar] [CrossRef]
- Lee, K.; Wang, Y.; Zheng, C. TWISTER Hand: Underactuated Robotic Gripper Inspired by Origami Twisted Tower. IEEE Trans. Robot. 2020, 36, 488–500. [Google Scholar] [CrossRef]
- Su, Y.; Fang, Z.; Zhu, W.; Sun, X.; Zhu, Y.; Wang, H.; Tang, K.; Huang, H.; Liu, S.; Wang, Z. A High-Payload Proprioceptive Hybrid Robotic Gripper with Soft Origamic Actuators. IEEE Robot. Autom. Lett. 2020, 5, 3003–3010. [Google Scholar] [CrossRef]
- Deshpande, A.R.; Ho, Z.T.; Ren, H. Origami-inspired bi-directional soft pneumatic actuator with integrated variable stiffness mechanism. In Proceedings of the 2017 18th International Conference on Advanced Robotics (ICAR), Hong Kong, China, 10–12 July 2017; pp. 417–421. [Google Scholar] [CrossRef]
- Salerno, M.; Firouzeh, A.; Paik, J. A Low Profile Electromagnetic Actuator Design and Model for an Origami Parallel Platform. J. Mech. Robot. 2017, 9, 041005. [Google Scholar] [CrossRef]
- Tolley, M.T.; Shepherd, R.F.; Karpelson, M.; Bartlett, N.W.; Galloway, K.C.; Wehner, M.; Nunes, R.; Whitesides, G.M.; Wood, R.J. An untethered jumping soft robot. In Proceedings of the 2014 IEEE/RSJ International Conference on Intelligent Robots and Systems, Chicago, IL, USA, 14–18 September 2014; pp. 561–566. [Google Scholar] [CrossRef]
- Banerjee, H.; Pusalkar, N. Single-Motor Controlled Tendon Driven Peristaltic Soft Origami Robot. J. Mech. Robot. 2018, 10, 064501. [Google Scholar] [CrossRef]
- Banerjee, H.; Li, T.; Ponraj, G.; Kumar, K.S.; Lim, C. Origami-Layer-Jamming Deployable Retractor with Variable Stiffness and Tactile Sensing. J. Mech. Robot. 2019, 12, 031010. [Google Scholar] [CrossRef]
- Taylor, A.J.; Slutzky, T.; Feuerman, L.; Ren, H.; Tokuda, J.; Nilsson, K.; Tse, Z.T. MR-Conditional SMA-Based Origami Joint. IEEE/ASME Trans. Mechatron. 2019, 24, 883–888. [Google Scholar] [CrossRef] [PubMed]
- Salerno, M.; Zhang, K.; Menciassi, A.; Dai, J.S. A Novel 4-DOF Origami Grasper with an SMA-Actuation System for Minimally Invasive Surgery. IEEE Trans. Robot. 2016, 32, 484–498. [Google Scholar] [CrossRef]
- Cai, C.J.; Xiao, X.; Kalairaj, M.S.K.; Lee, I.J.; Mugilvannan, A.K.; Yeow, B.S.; Tan, J.H.; Huang, H.; Ren, H. Diversified and Untethered Motion Generation Via Crease Patterning from Magnetically Actuated Caterpillar-Inspired Origami Robot. IEEE/ASME Trans. Mechatron. 2021, 26, 1678–1688. [Google Scholar] [CrossRef]
- Yang, H.; Yeow, B.S.; Li, Z.; Li, K.; Chang, T.; Jing, L.; Li, Y.; Ho, J.S.; Ren, H.; Chen, P.Y. Multifunctional metallic backbones for origami robotics with strain sensing and wireless communication capabilities. Sci. Robot. 2019, 4, eaax7020. [Google Scholar] [CrossRef]
- Zhai, Z.; Wang, Y.; Lin, K.; Wu, L.; Jiang, H. In situ stiffness manipulation using elegant curved origami. Sci. Adv. 2020, 6, eabe2000. [Google Scholar] [CrossRef]
- Qiu, C.; Zhang, K.; Dai, J. Repelling-Screw Based Force Analysis of Origami Mechanisms. J. Mech. Robot. 2016, 8, 031001. [Google Scholar] [CrossRef]
- Salamon, B.A.; Midha, A. An Introduction to Mechanical Advantage in Compliant Mechanisms. J. Mech. Des. 1998, 120, 311–315. [Google Scholar] [CrossRef]
- Hernandez, E.P.; Hartl, D.; Lagoudas, D. Active Origami: Modeling, Design, and Applications; Springer: Berlin/Heidelberg, Germany, 2019. [Google Scholar] [CrossRef]
- Bai, S.; Li, X.; Angeles, J. A review of spherical motion generation using either spherical parallel manipulators or spherical motors. Mech. Mach. Theory 2019, 140, 377–388. [Google Scholar] [CrossRef]
- Yip, H.M.; Li, P.; Navarro-Alarcon, D.; Wang, Z.; Liu, Y. A new circular-guided remote center of motion mechanism for assistive surgical robots. In Proceedings of the 2014 IEEE International Conference on Robotics and Biomimetics (ROBIO 2014), Bali, Indonesia, 5–10 December 2014; pp. 217–222. [Google Scholar] [CrossRef]
- Bowen, L.A.; Grames, C.L.; Magleby, S.P.; Lang, R.J.; Howell, L.L. An Approach for Understanding Action Origami as Kinematic Mechanisms. In Proceedings of the Volume 6B: 37th Mechanisms and Robotics Conference, Portland, OR, USA, 4–7 August 2013. [Google Scholar] [CrossRef]
- Huffman. Curvature and Creases: A Primer on Paper. IEEE Trans. Comput. 1976, 100, 1010–1019. [Google Scholar] [CrossRef]
- Farhang, K.; Zargar, Y.S. Design of Spherical 4R Mechanisms: Function Generation for the Entire Motion Cycle. J. Mech. Des. 1999, 121, 521–528. [Google Scholar] [CrossRef]
- Hernández, S.; Bai, S.; Angeles, J. The Design of a Chain of Spherical Stephenson Mechanisms for a Gearless Robotic Pitch-Roll Wrist. J. Mech. Des. 2006, 128, 422–429. [Google Scholar] [CrossRef]
- Hull, T.C.; Tachi, T. Double-line rigid origami. arXiv 2017, arXiv:1709.03210. [Google Scholar]
- Feng, H.; Peng, R.; Ma, J.; Chen, Y. Rigid Foldability of Generalized Triangle Twist Origami Pattern and Its Derived 6R Linkages. J. Mech. Robot. 2018, 10, 051003. [Google Scholar] [CrossRef]
- Fang, H.; Li, S.; Wang, K. Self-locking degree-4 vertex origami structures. Proc. R. Soc. Math. Phys. Eng. Sci. 2016, 472, 20160682. [Google Scholar] [CrossRef]
- Edmondson, B.J. Development of a Thickness Accommodation Technique for Origami-Inspired Design; Brigham Young University: Provo, UT, USA, 2015. [Google Scholar]
- Kamrava, S.; Mousanezhad, D.; Felton, S.M.; Vaziri, A. Programmable Origami Strings. Adv. Mater. Technol. 2018, 3, 1700276. [Google Scholar] [CrossRef]
- Miura, K. The Science of Miura-Ori: A Review. Origami 2009, 4, 87. [Google Scholar]
- Butler, J.; Bowen, L.; Wilcox, E.; Shrager, A.; Frecker, M.I.; von Lockette, P.; Simpson, T.W.; Lang, R.J.; Howell, L.L.; Magleby, S.P. A Model for Multi-Input Mechanical Advantage in Compliant and Origami-Based Mechanisms. J. Mech. Robot. 2018, 10, 061007. [Google Scholar] [CrossRef]
- Wilcox, E.W.; Shrager, A.; Bowen, L.; Frecker, M.; Lockette, P.V.; Simpson, T.; Magleby, S.; Lang, R.J.; Howell, L.L. Considering mechanical advantage in the design and actuation of an origami-based mechanism. In Proceedings of the ASME Design Engineering Technical Conference, 39th Mechanisms and Robotics Conference, Boston, MA, USA, 2–5 August 2015; ASME: New York, NY, USA, 2016. [Google Scholar] [CrossRef]
- Orlofsky, A.; Liu, C.; Kamrava, S.; Vaziri, A.; Felton, S.M. Mechanically Programmed Miniature Origami Grippers. In Proceedings of the 2020 IEEE International Conference on Robotics and Automation (ICRA), Paris, France, 31 May–31 August 2020; pp. 2872–2878. [Google Scholar] [CrossRef]
- Kamrava, S.; Liu, C.; Orlofsky, A.; Vaziri, A.; Felton, S. A Closed-form Solution for the Kinematics of Asymmetric Miura Vertices. arXiv 2020, arXiv:2001.07657. [Google Scholar]


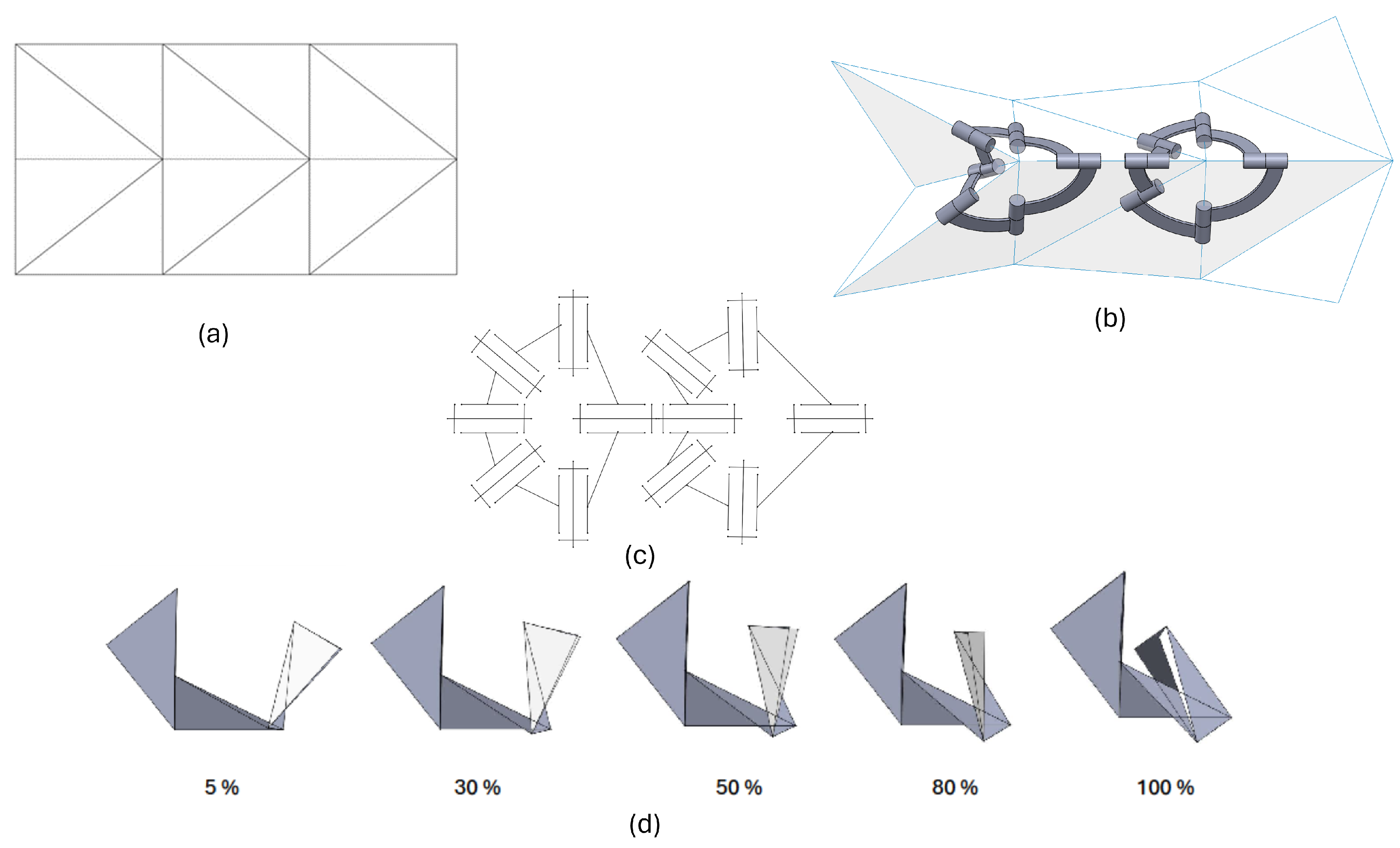
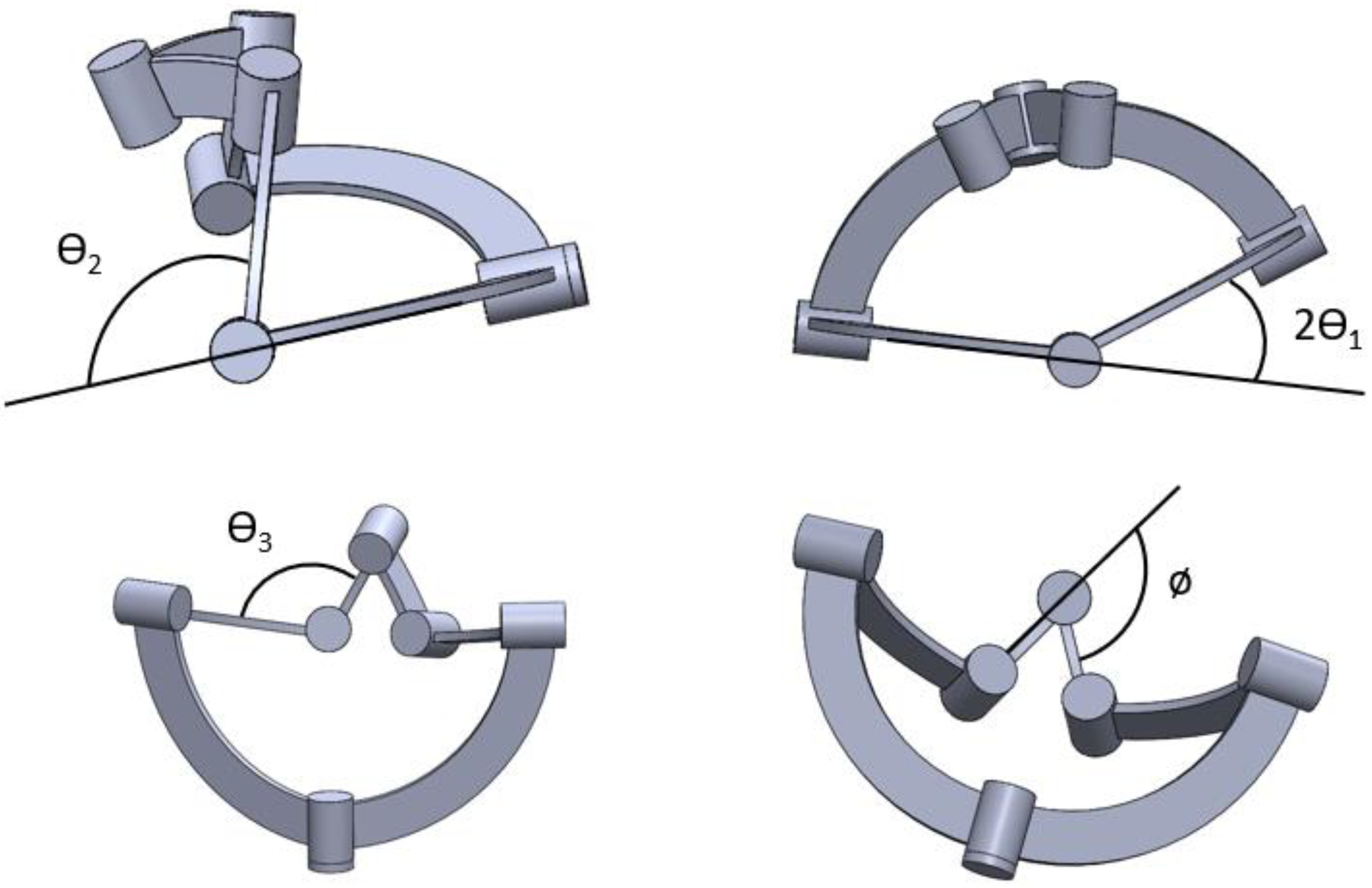

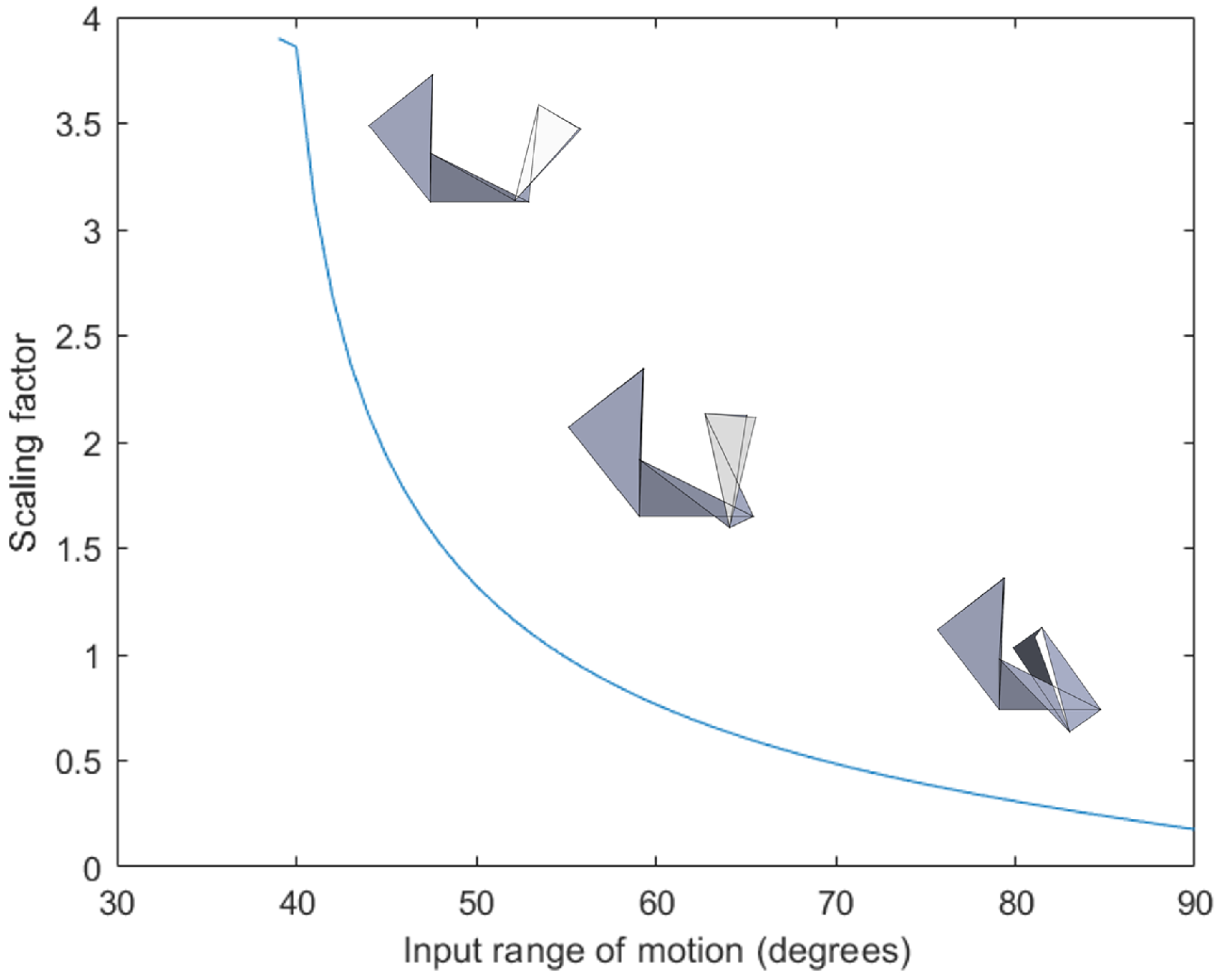

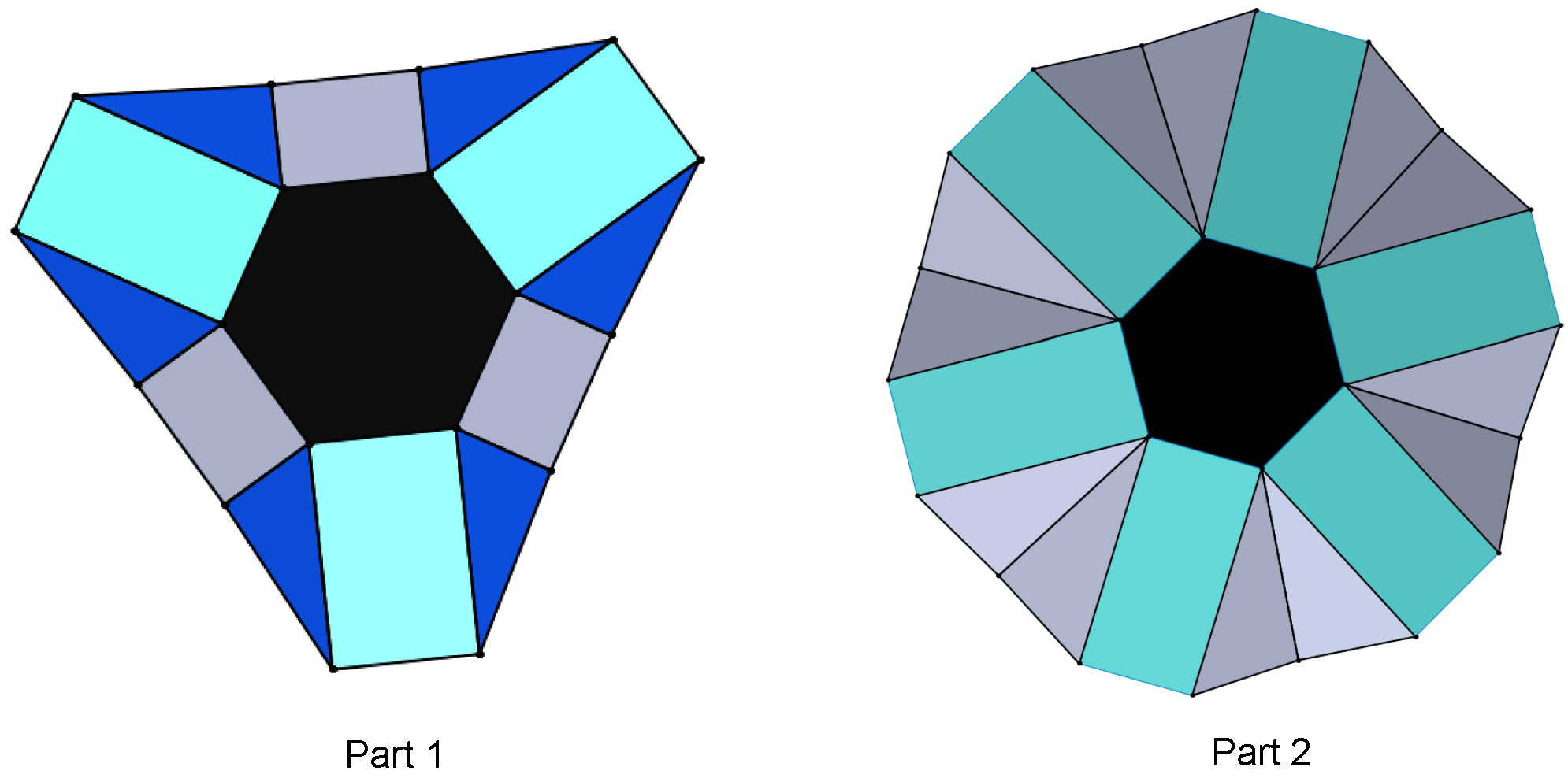


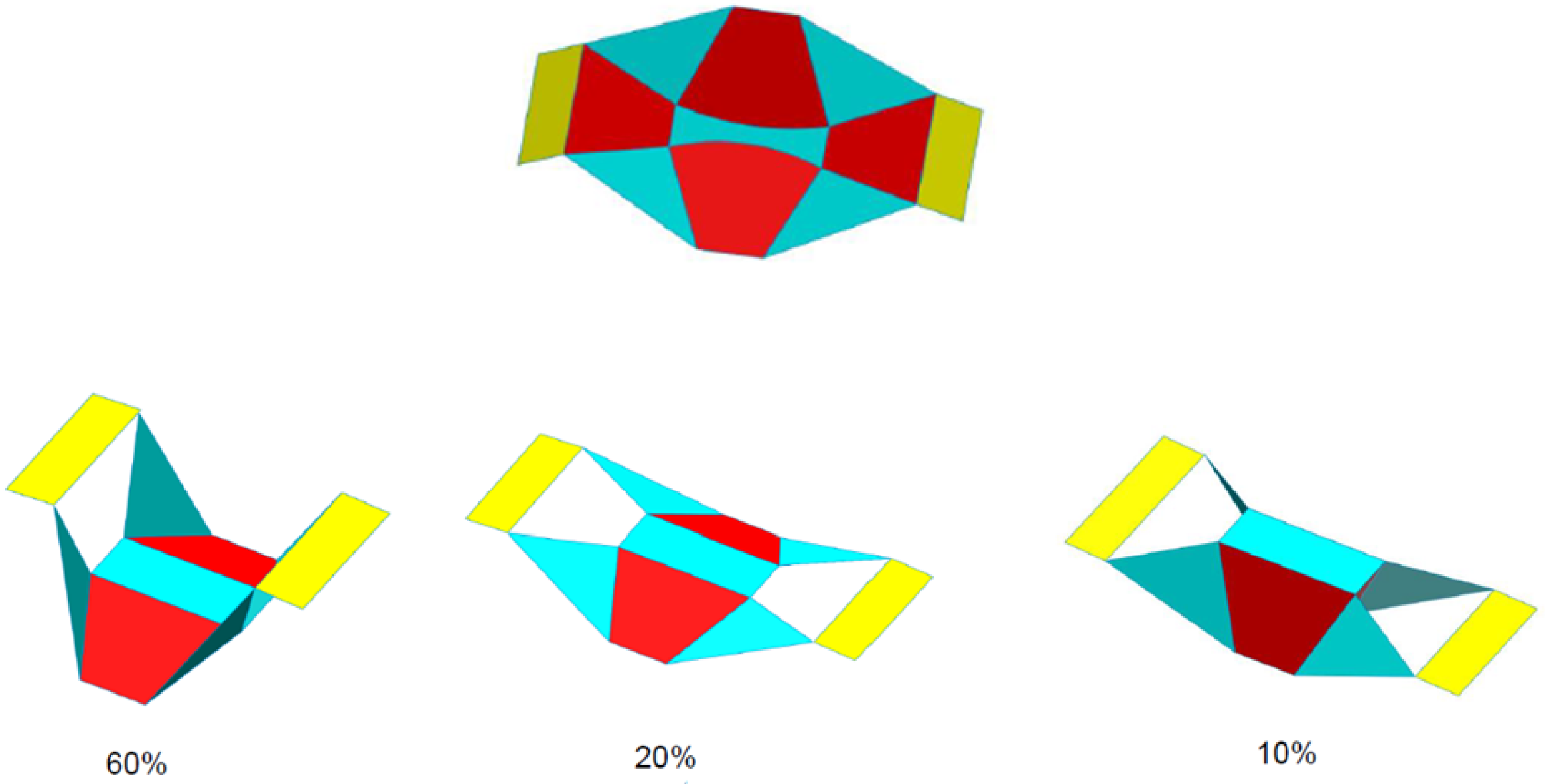
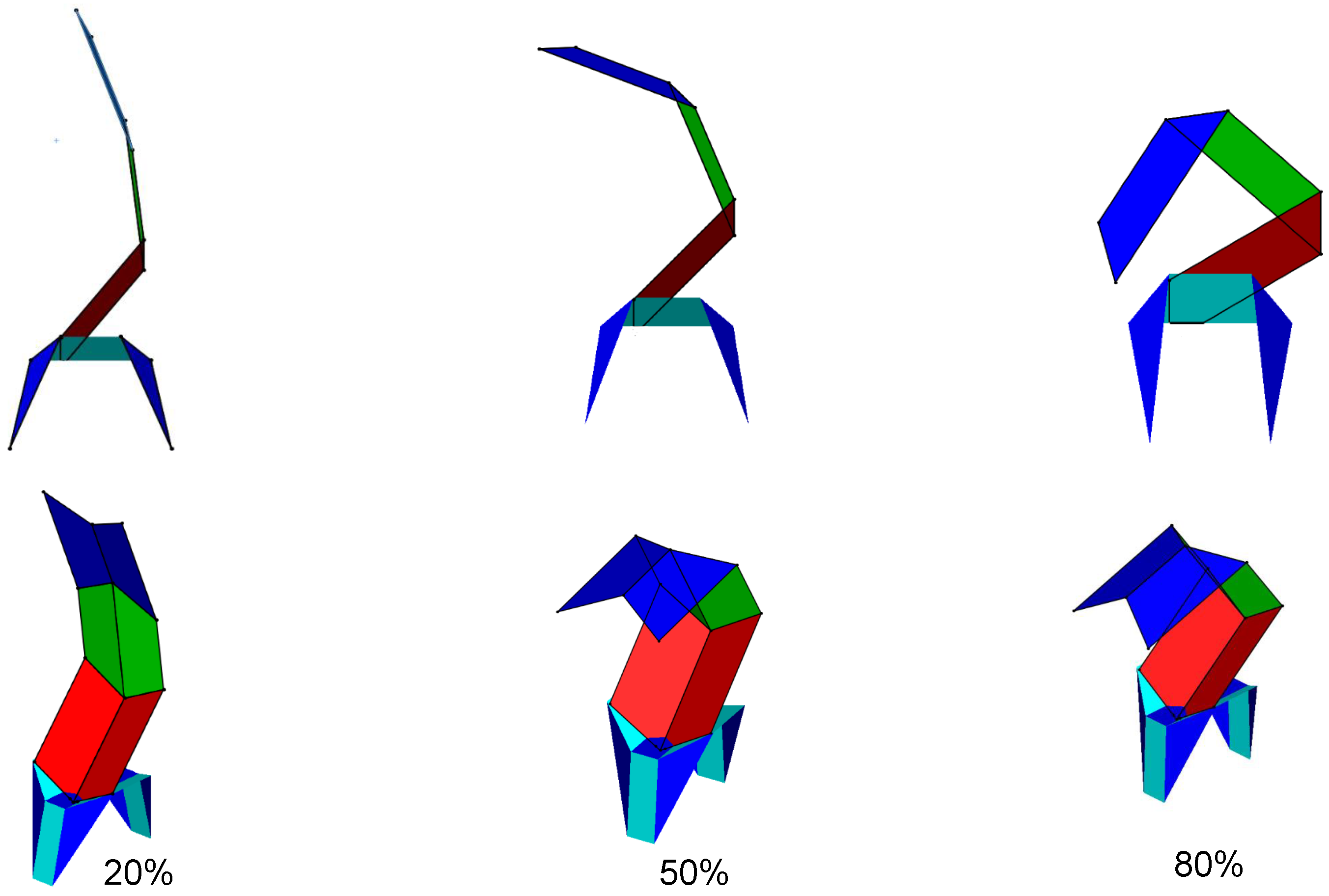
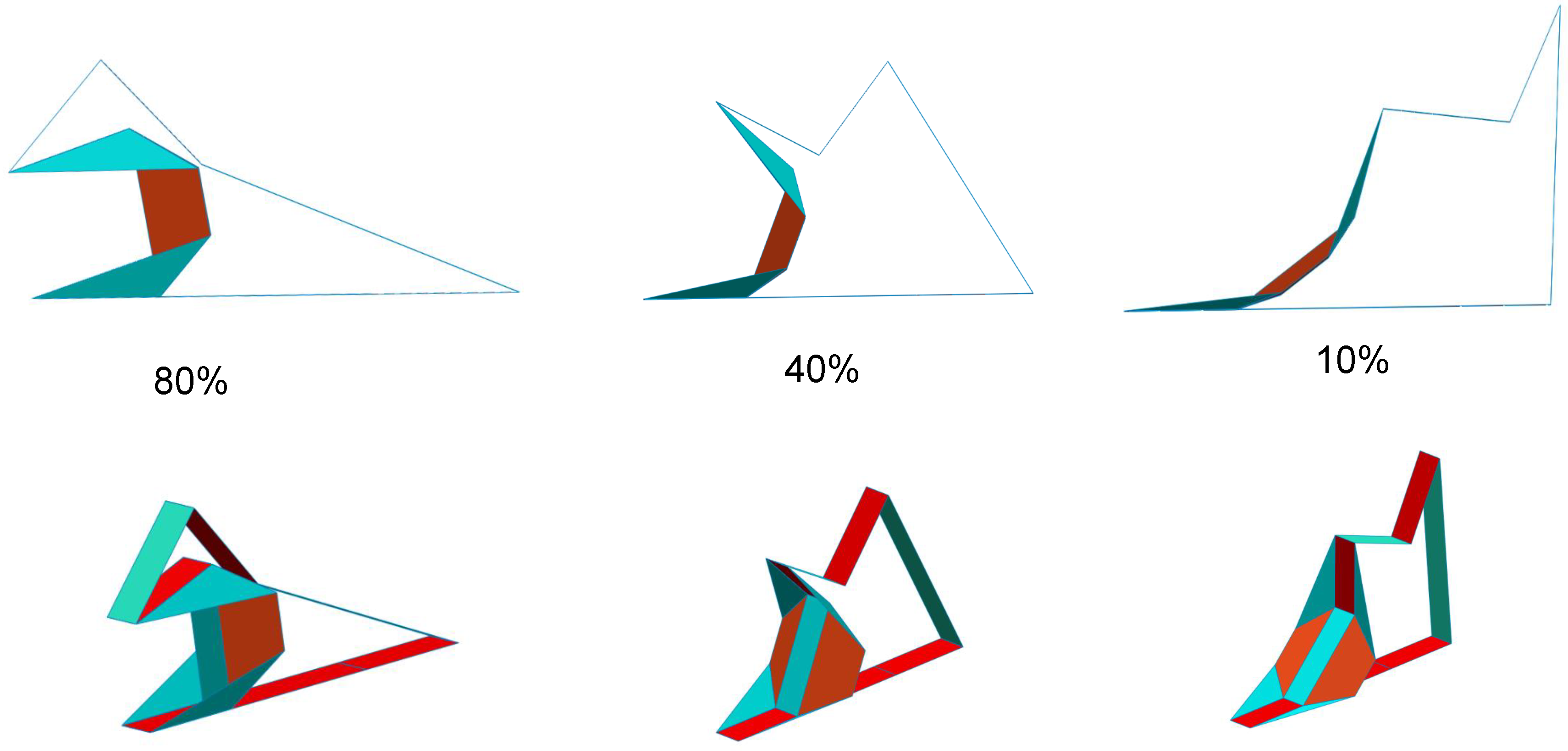
| Parameter | Oriceps | Miura Ori | MACIOR | Hexagon Type 1 | Hexagon Type 2 |
|---|---|---|---|---|---|
| Number of creases | 12 | 8 | 13 | 18 | 24 |
| Number of structures | 2 | 2 | 3 | 3 | 6 |
| Number of surfaces | 9 | 10 | 12 | 13 | 19 |
| Number of spherical mechanisms | 4 | 4 | 2 | 6 | 6 |
| Degree of a vertex | 4 | 4 | 6 | 4 | 5 |
| Parameter | Oriceps | Miura Ori | MACIOR | Hexagon Type 1 | Hexagon Type 2 |
|---|---|---|---|---|---|
| Arrangement of spherical mechanism | 4 corners of a rectangle | Linear | Linear | Hexagon vertices | Hexagon vertices |
| Classification [35] | Coupled | 4 long linear chain | 2 long linear chain | Single loop | Single loop |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Krishnapuram, S.; Xiao, X.; Ren, H. Comparative Study of Mechanical Scaling Effects of Origami-Inspired Motion Generation Mechanisms with Multi-Degree Vertices. Actuators 2024, 13, 266. https://doi.org/10.3390/act13070266
Krishnapuram S, Xiao X, Ren H. Comparative Study of Mechanical Scaling Effects of Origami-Inspired Motion Generation Mechanisms with Multi-Degree Vertices. Actuators. 2024; 13(7):266. https://doi.org/10.3390/act13070266
Chicago/Turabian StyleKrishnapuram, Seetharam, Xiao Xiao, and Hongliang Ren. 2024. "Comparative Study of Mechanical Scaling Effects of Origami-Inspired Motion Generation Mechanisms with Multi-Degree Vertices" Actuators 13, no. 7: 266. https://doi.org/10.3390/act13070266
APA StyleKrishnapuram, S., Xiao, X., & Ren, H. (2024). Comparative Study of Mechanical Scaling Effects of Origami-Inspired Motion Generation Mechanisms with Multi-Degree Vertices. Actuators, 13(7), 266. https://doi.org/10.3390/act13070266






