Study on Dynamics of Overrunning Spring Clutches and Suppression Methods for Their Abnormal Noise
Abstract
1. Introduction
2. Analysis of Abnormal Noise Causes
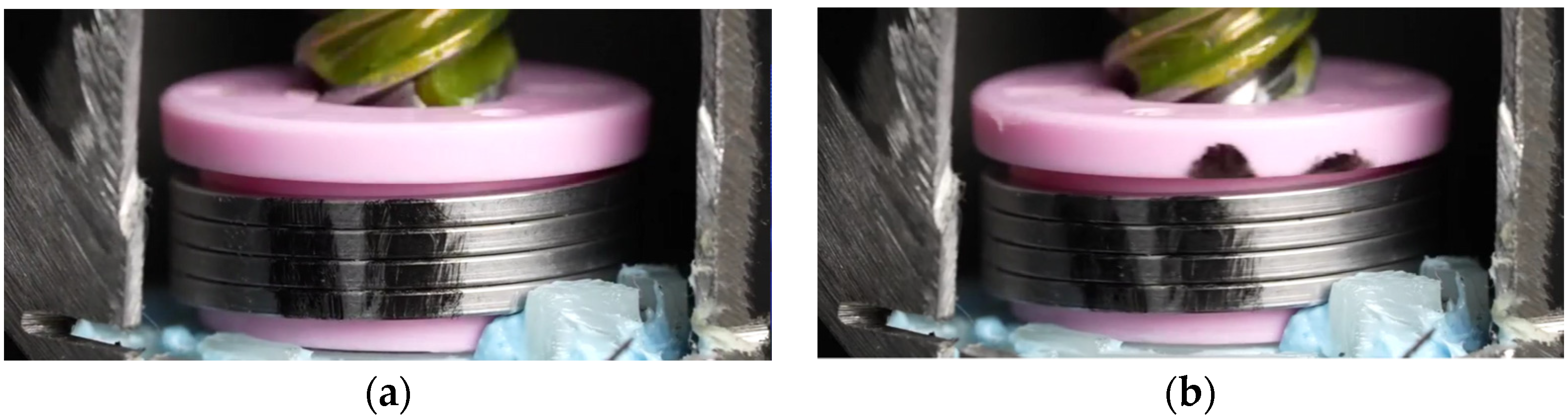
2.1. Phenomenon Analysis
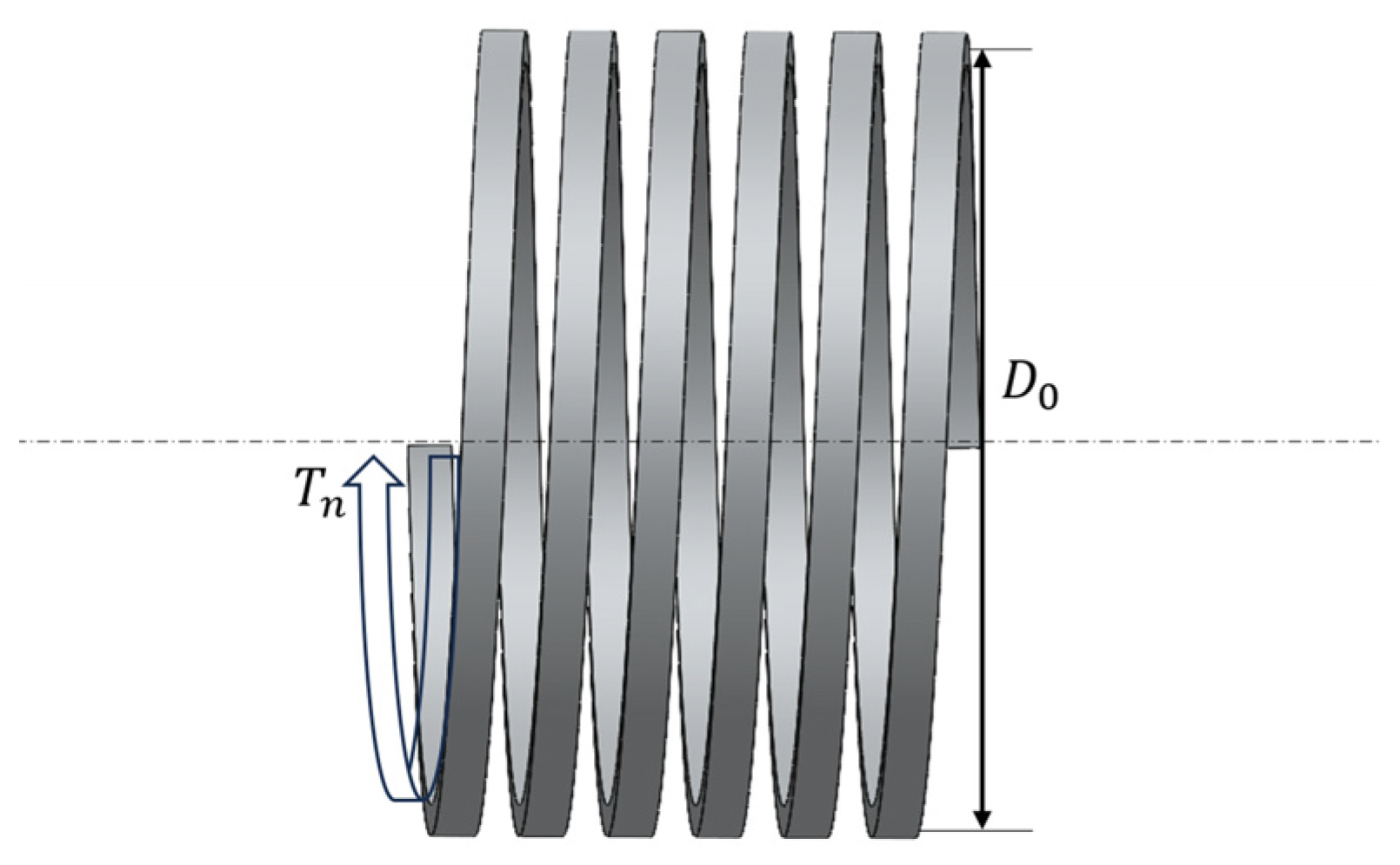
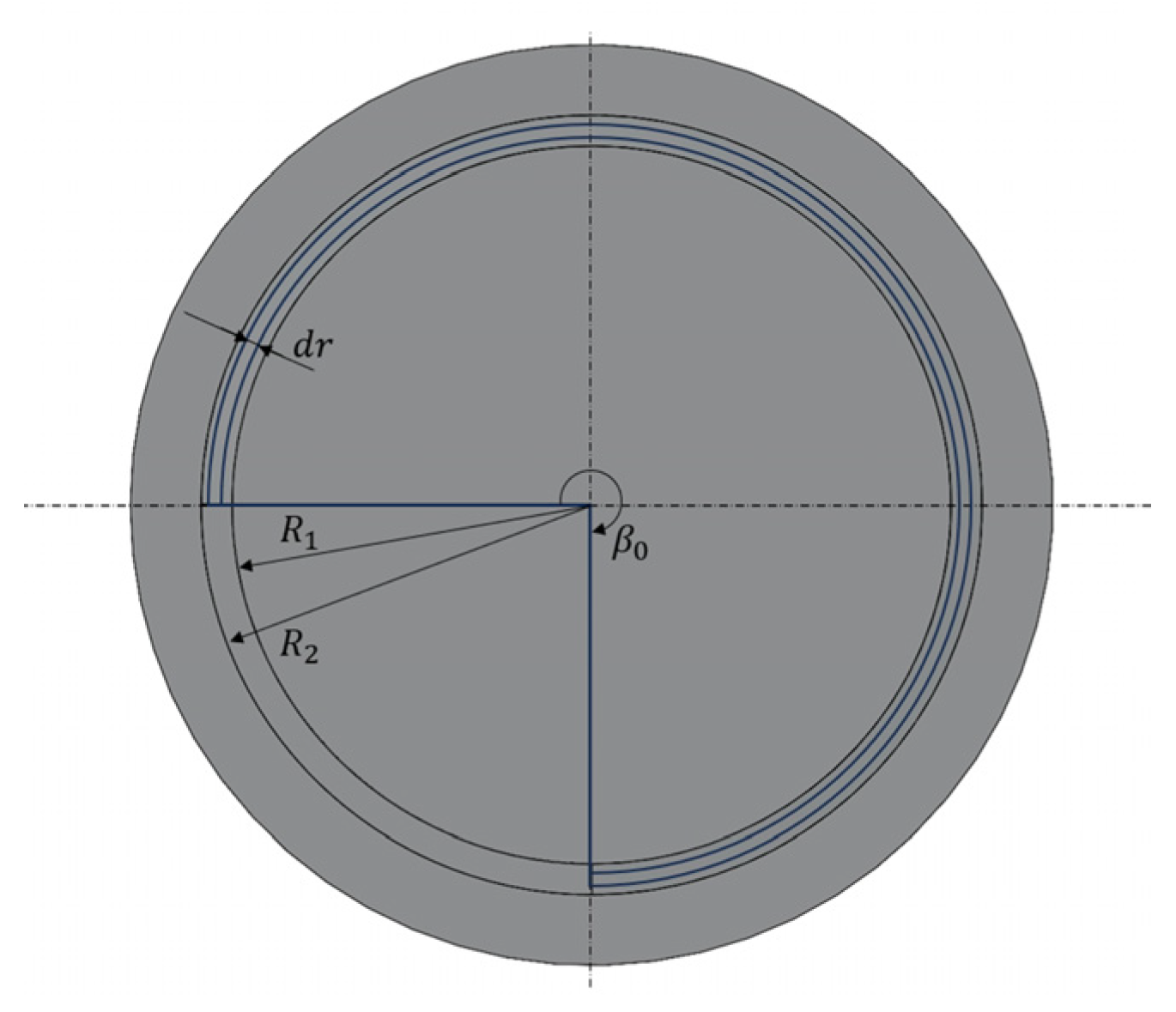
2.2. Mechanical Analysis
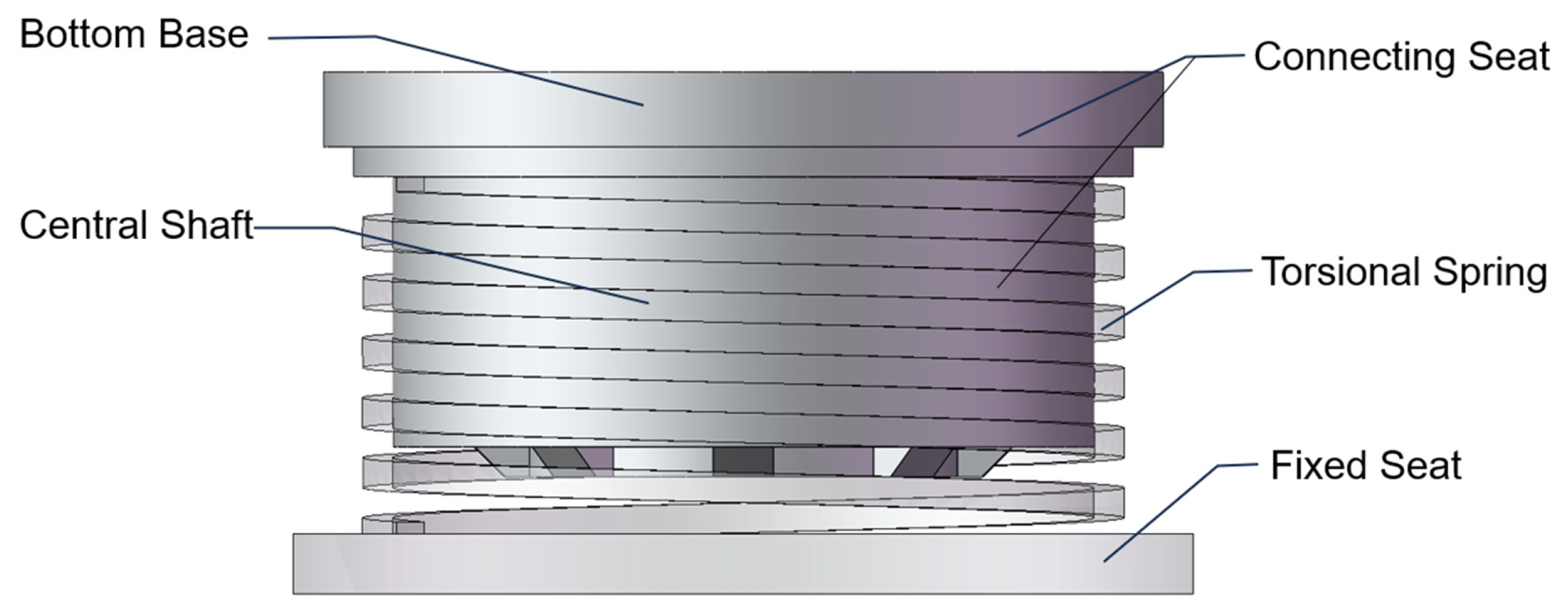
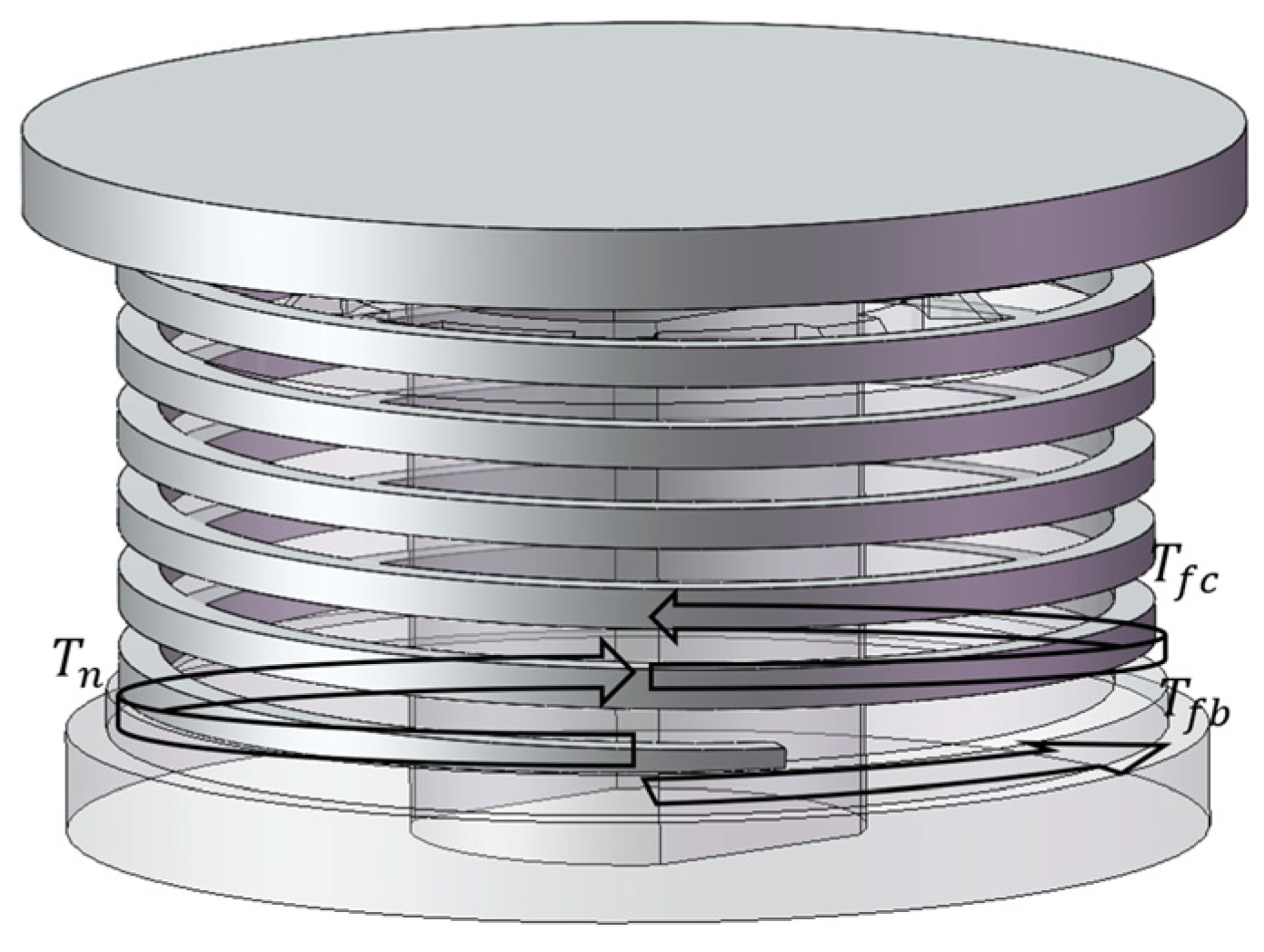
2.3. Dynamics Analysis
3. Structural Optimization Design
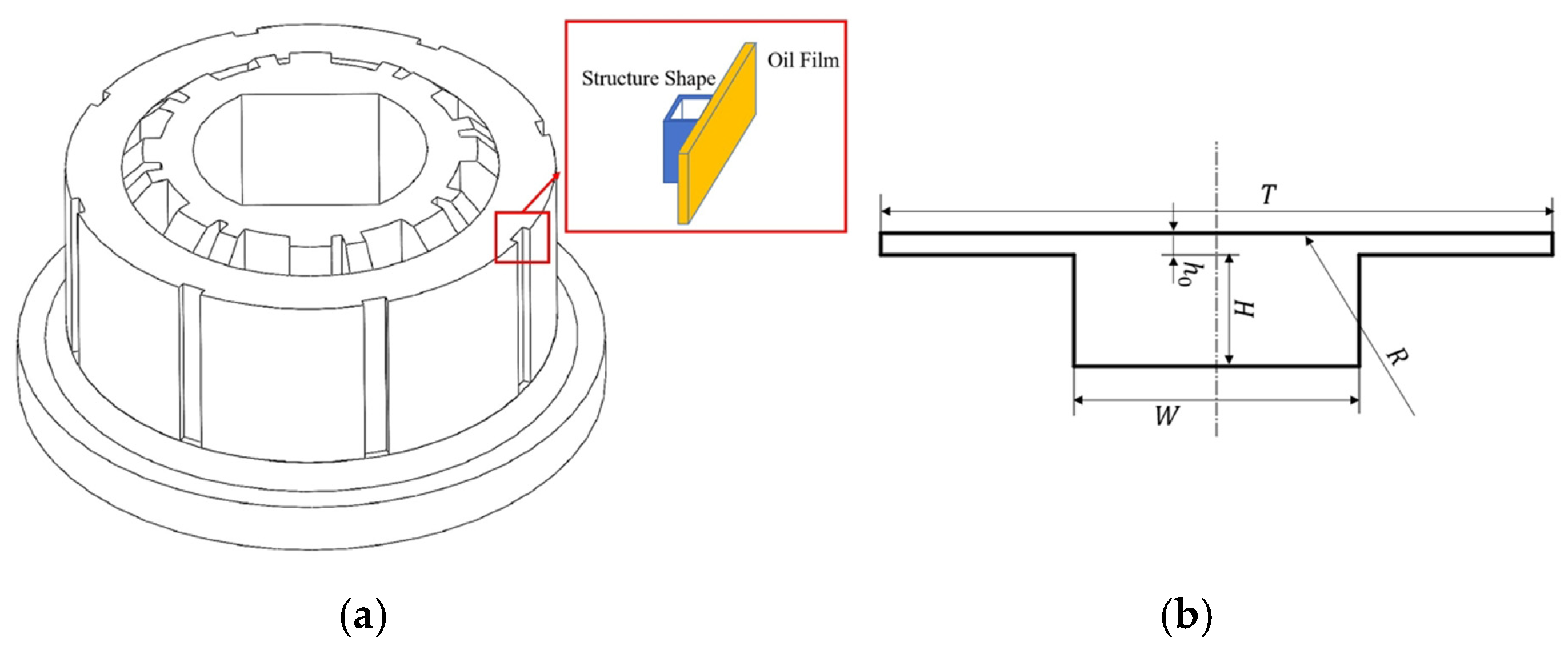
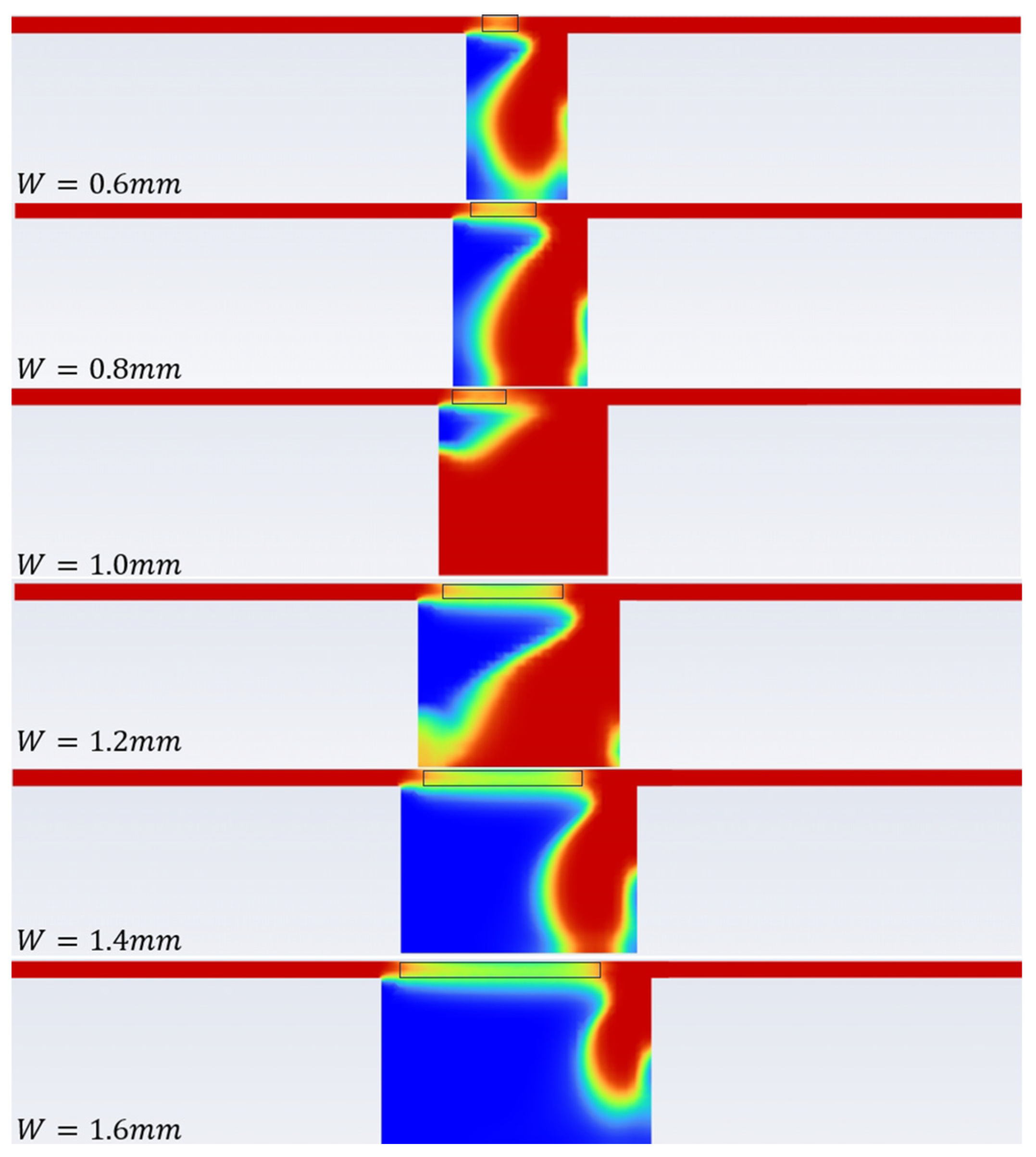
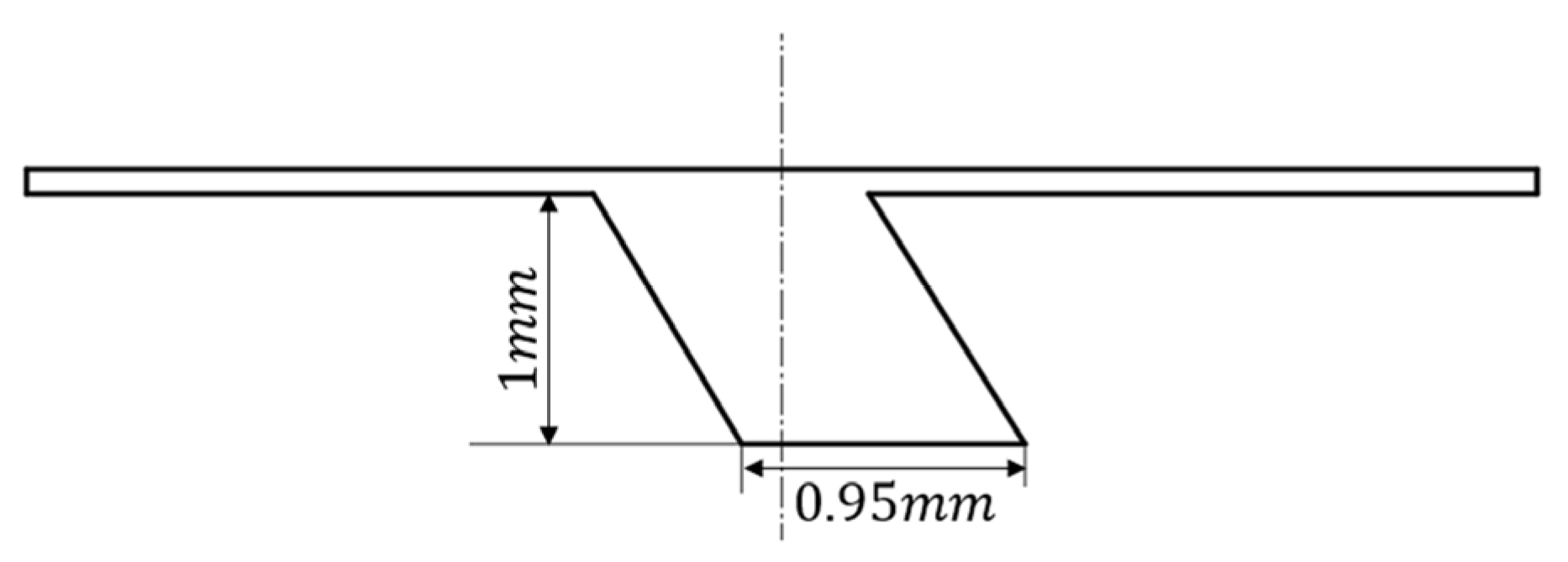
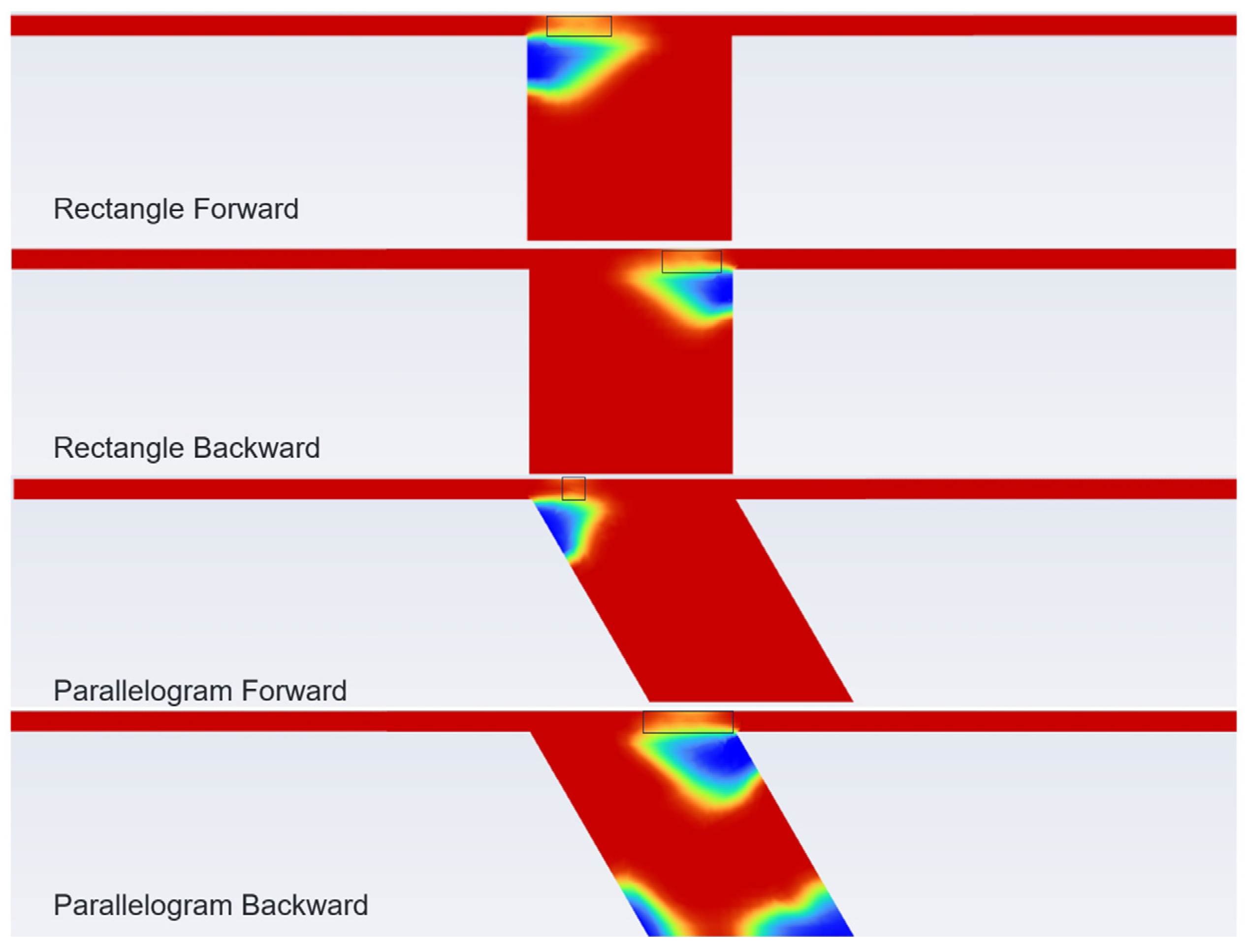
3.1. Structural Optimization Analysis
3.2. Structure CFD Simulation Analysis
4. Experimental Verification
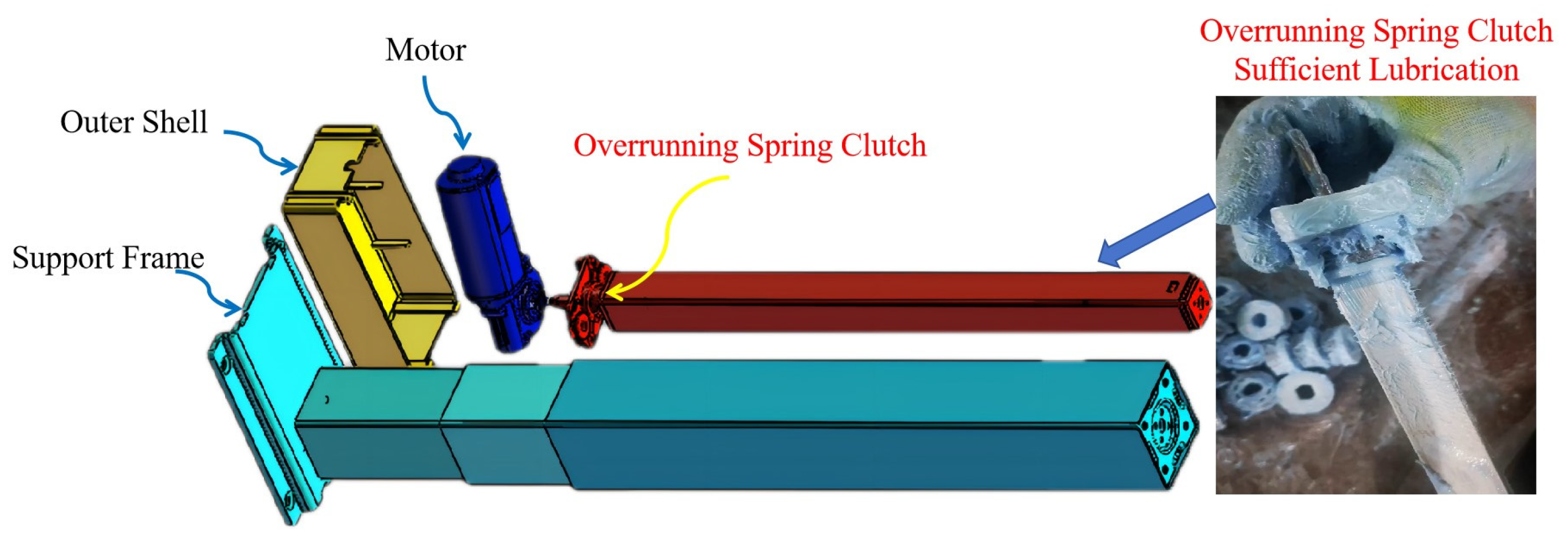
4.1. Experimental Apparatus Design
4.2. Experimental Analysis
5. Conclusions
- (1)
- The abnormal noise during the reverse overrunning process of overrunning spring clutches originates from the self-excited frictional vibration caused by the stick–slip phenomenon between the spring and the clutch seat.
- (2)
- Based on the system’s dynamic model of overrunning spring clutches, the generation of self-excited frictional vibration in the clutch system is related to the rotational speed. The critical speed is directly proportional to the system’s damping, and changing the system’s damping can alter the critical speed at which frictional self-excited vibration occurs.
- (3)
- Optimized surface texturing can increase system damping and effectively suppress abnormal noise while reducing its impact on clutch performance.
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Zhao, P.; Yan, H.; Xia, F.; Cao, Y.; Chen, X. Analysis of the Engagement Characteristic of Spring Clutch under Different Pre Expansion Quantity. J. Mech. Transm. 2015, 39, 138–143. [Google Scholar] [CrossRef]
- Pan, C.; Che, C.; Li, X.; Pan, Z. Principle and Calculation for Expanding Type of Self-energizing Overrunning Spring Clutch. J. Harbin Univ. Sci. Technol. 2000, 03, 55–58. [Google Scholar] [CrossRef]
- Chang, B.; Bao, H.; Zhu, R. Optimal Design of Spring for Spring Overrunning Clutch Based on Matlab. Mach. Build. Autom. 2012, 41, 4. [Google Scholar] [CrossRef]
- Yan, H.; Li, S.; Zhu, H.; Zhao, L.; Liu, Z.; Li, J. Analysis of the influence of key structural parameters on torsional performance of spring clutch. Mod. Manuf. Eng. 2020, 05, 33–38+132. [Google Scholar] [CrossRef]
- Yan, H.; Ye, H.; Chen, X.; Wang, W.; Zhao, P. Analyzing Friction and Wear Characteristics of Overrunning Spring Clutch. Mech. Sci. Technol. Aerosp. Eng. 2016, 35, 630–635. [Google Scholar] [CrossRef]
- Yan, H.; Long, S.; Tan, W.; Xia, F. Analysis of Combining Process Dynamics Character of Overrunning Variable Cross—Section Spring Clutch. J. Mech. Transm. 2013, 37, 82–86. [Google Scholar] [CrossRef]
- Cao, Y.; Yan, H.; Wang, Z.; Cai, M.; Wu, S. Modeling and analysis of engagement behavior of one-way clutch with a variable rectangular cross-section torsional spring. Adv. Mech. Eng. 2019, 11, 1687814019898361. [Google Scholar] [CrossRef]
- Cao, Y.; Yan, H.; Lin, M.; Wen, B. Calculation model of deformation and stress-resultants of cylindrical helical torsion spring with variable cross-section used in overrunning clutch. In Proceedings of the 2nd International Conference on Mechanical Engineering, Intelligent Manufacturing and Automation Technology (MEMAT 2022), Guilin, China, 7–9 January 2022; VDE: Frankfurt, Germany, 2022; pp. 1–9. [Google Scholar]
- Ali, S.; Kurniawan, R.; Chul, P.G.; Tae Jo, K.O. Tribological properties of hierarchical micro-dimples produced on a cylindrical surface by dual-frequency texturing. Friction 2023, 11, 246–258. [Google Scholar] [CrossRef]
- Ding, S.; Wei, H.; Yang, O.; Deng, L.; Mu, D. Tribological behaviors of laser textured surface under different lubrication conditions for rotary compressor. Sci. Rep. 2023, 13, 5378. [Google Scholar] [CrossRef] [PubMed]
- Song, J.; Huang, H.; Wang, X.; Shi, W. Status and prospects of surface texturing: Design, manufacturing and applications. Surf. Sci. Tech. 2023, 1, 21. [Google Scholar] [CrossRef]
- Liu, J.H.; Yu, P.J.; Zhou, Y.J.; Xu, Z.B.; Li, Y.J.; Li, P.; Zhao, Z.; He, C.G.; Shen, M.X. New insight on wheel flange/rail gauge lubrication: Effect of lasered microtexture shapes on the wear and fatigue behaviour of wheel/rail steels. Wear 2022, 498, 204310. [Google Scholar] [CrossRef]
- Seid Ahmed, Y.; DePaiva, J.M.; Amorim, F.L.; Torres, R.; Veldhuis, S. Laser surface texturing and characterization of austenitic stainless steel for the improvement of its surface properties. Int. J. Adv. Manuf. Technol. 2021, 115, 1795–1808. [Google Scholar] [CrossRef]
- Sudeep, U.; Tandon, N.; Pandey, R.K. Tribological studies of lubricated laser-textured point contacts in rolling/sliding reciprocating motion with investigations of wettability and nanohardness. Tribol. Trans. 2015, 58, 625–634. [Google Scholar] [CrossRef]
- Xu, J.; Sun, G.; Zhai, C.; Tian, J.; Nie, X.; Yu, H. Study on the Heat-Affected Zone, Microstructure, and Surface Quality of TB8 Titanium Alloy Treated by Laser-Assisted Micromachining. J. Mater. Eng. Perform. 2022, 31, 2978–2990. [Google Scholar] [CrossRef]
- Wang, Q.; Wang, H. Laser surface functionalization to achieve extreme surface wetting conditions and resultant surface functionalities. J. Cent. South Univ. 2022, 29, 3217–3247. [Google Scholar] [CrossRef]
- Rajurkar, A.; Chinchanikar, S. Investigation on the effect of laser parameters and hatch patterns on the dimensional accuracy of micro-dimple and micro-channel texture geometries. Int. J. Interact. Des. Manuf. 2023, 17, 1955–2505. [Google Scholar] [CrossRef]
- Gachot, C.; Rosenkranz, A.; Hsu, S.M.; Costa, H.L. A critical assessment of surface texturing for friction and wear improvement. Wear 2016, 372, 21–41. [Google Scholar] [CrossRef]
- Lu, S.; Wang, H.; He, J.; Shao, Z.; Yuan, J. Multi-dimensional Multi-resolution Fourier Analysis method in image processing. In Proceedings of the 2013 15th IEEE International Conference on Communication Technology, Guilin, China, 17–19 November 2013; pp. 75–79. [Google Scholar] [CrossRef]
- Norton, R.L. Machine Design; Prentice Hall: London, UK, 2010. [Google Scholar]
- Niknam, A.; Farhang, K. Friction-induced vibration in a two-mass damped system. J. Sound Vib. 2019, 456, 454–475. [Google Scholar] [CrossRef]
- Wang, H.; Wang, W. The Frictional Self-excited Vibration of Machine Tool. J. Yantai Univ. (Nat. Sci. Eng. Ed.) 1997, 1, 61–67. [Google Scholar] [CrossRef]
- Spelsberg-Korspeter, G. Structural optimization for the avoidance of self-excited vibrations based on analytical models. J. Sound Vib. 2010, 329, 4829–4840. [Google Scholar] [CrossRef]

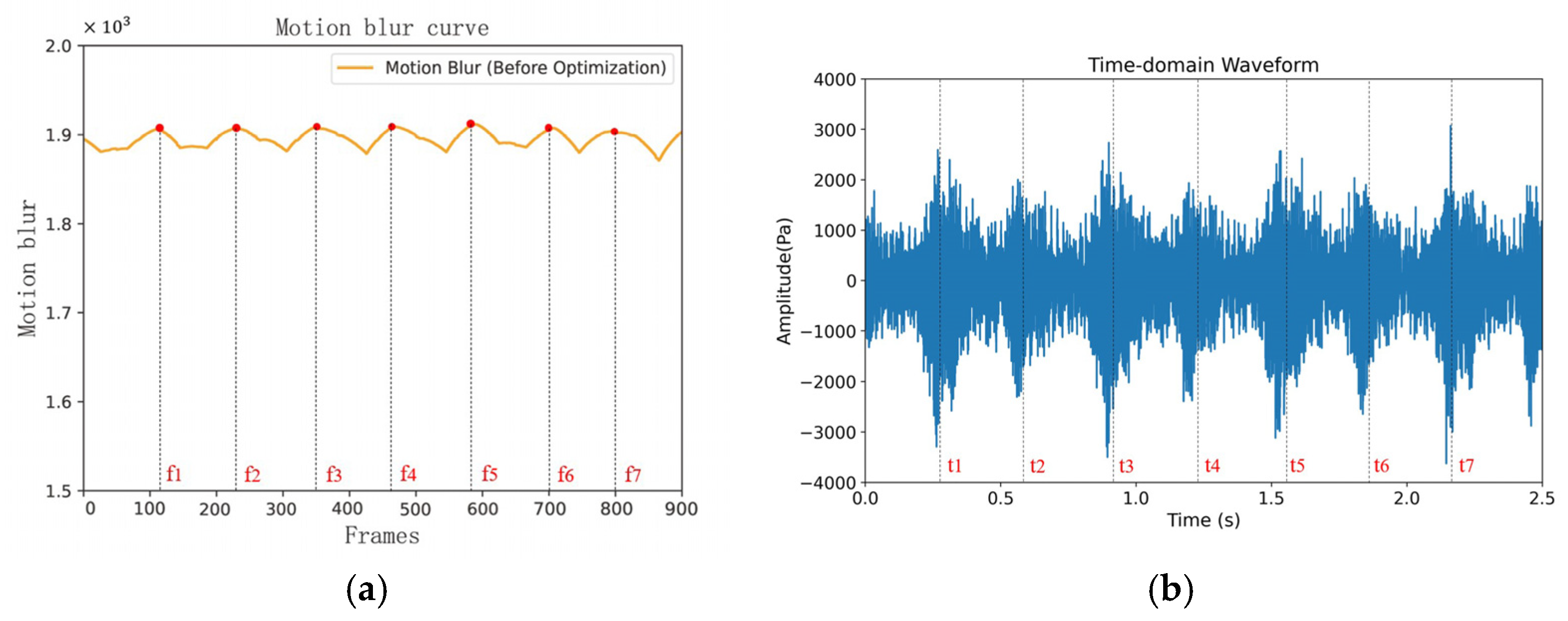




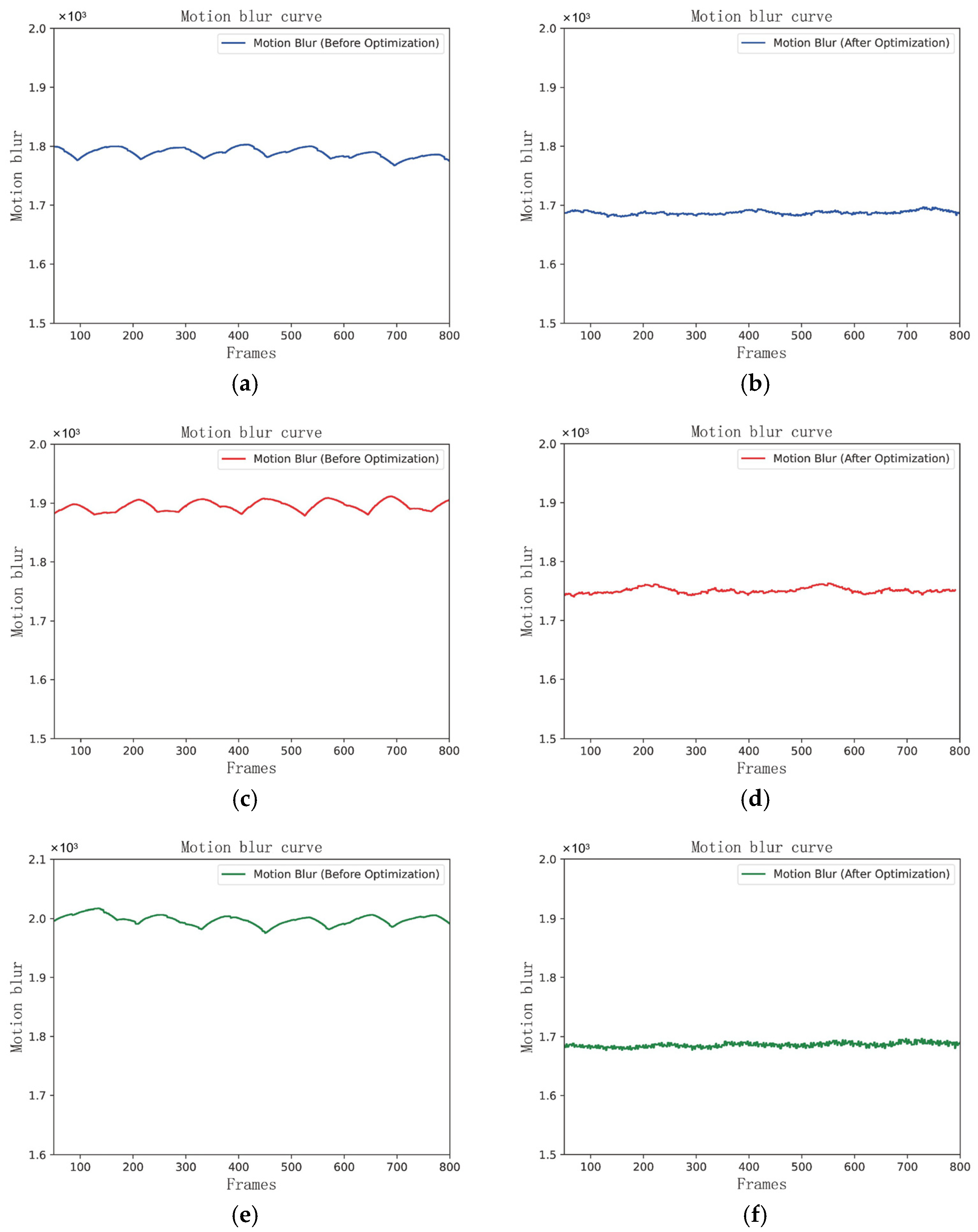

| Blur Curve | Time-Domain Waveform | |||
|---|---|---|---|---|
| Peak Identification | Peak Frame Number | Time (s) | Peak Identification | Time (s) |
| f1 | 108 | 0.30 | t1 | 0.29 |
| f2 | 221 | 0.61 | t2 | 0.58 |
| f3 | 348 | 0.97 | t3 | 0.94 |
| f4 | 465 | 1.29 | t4 | 1.24 |
| f5 | 585 | 1.63 | t5 | 1.56 |
| f6 | 700 | 1.94 | t6 | 1.87 |
| f7 | 799 | 2.22 | t7 | 2.21 |
| Group A | Group B | Group C | Abnormal Group Proportion | |
|---|---|---|---|---|
| Before lubricant optimization | Unusual noises | Unusual noises | Unusual noises | 3/3 |
| After lubricant optimization | Unusual noises | No noises | Unusual noises | 2/3 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhou, J.; Qiu, Z.; Zhang, H.; Zhan, J. Study on Dynamics of Overrunning Spring Clutches and Suppression Methods for Their Abnormal Noise. Actuators 2024, 13, 165. https://doi.org/10.3390/act13050165
Zhou J, Qiu Z, Zhang H, Zhan J. Study on Dynamics of Overrunning Spring Clutches and Suppression Methods for Their Abnormal Noise. Actuators. 2024; 13(5):165. https://doi.org/10.3390/act13050165
Chicago/Turabian StyleZhou, Jie, Zhehang Qiu, Huijuan Zhang, and Jianming Zhan. 2024. "Study on Dynamics of Overrunning Spring Clutches and Suppression Methods for Their Abnormal Noise" Actuators 13, no. 5: 165. https://doi.org/10.3390/act13050165
APA StyleZhou, J., Qiu, Z., Zhang, H., & Zhan, J. (2024). Study on Dynamics of Overrunning Spring Clutches and Suppression Methods for Their Abnormal Noise. Actuators, 13(5), 165. https://doi.org/10.3390/act13050165







