Negative-Stiffness Structure Vibration-Isolation Design and Impedance Control for a Lower Limb Exoskeleton Robot
Abstract
1. Introduction
2. Problem Formulation and Preliminaries
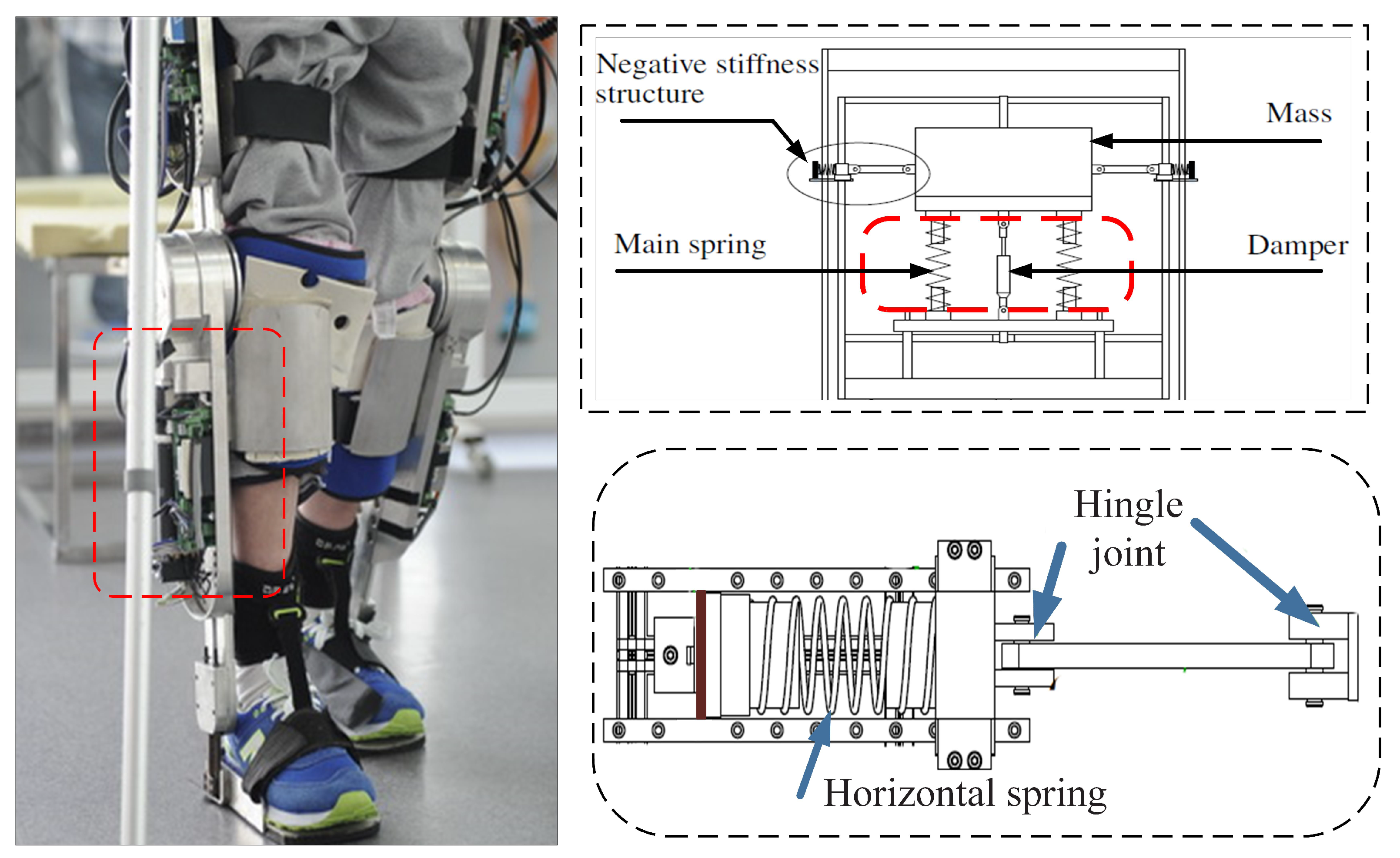
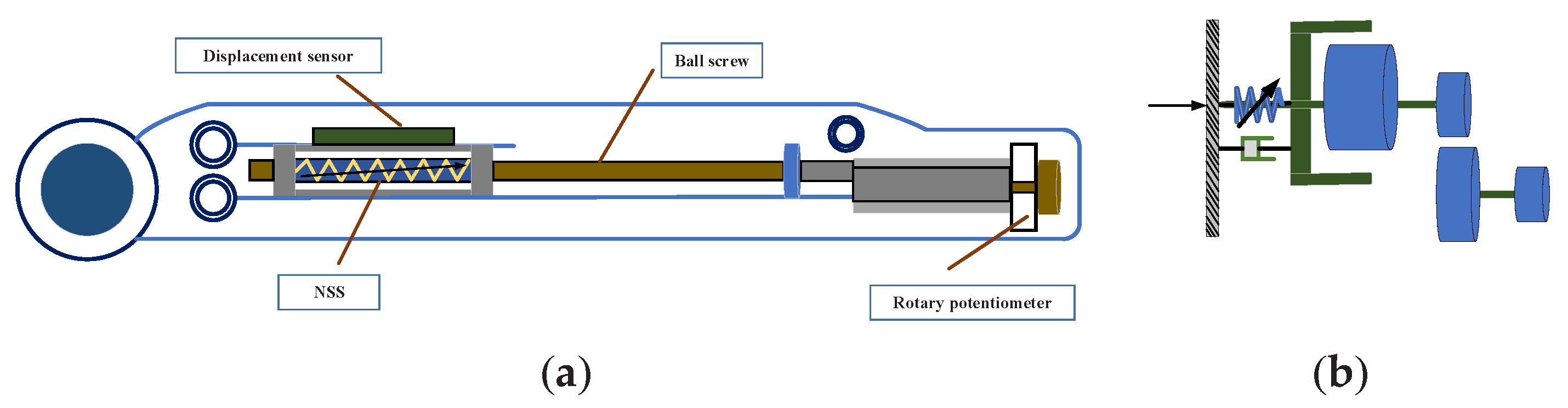
2.1. SEA Structure with NSS
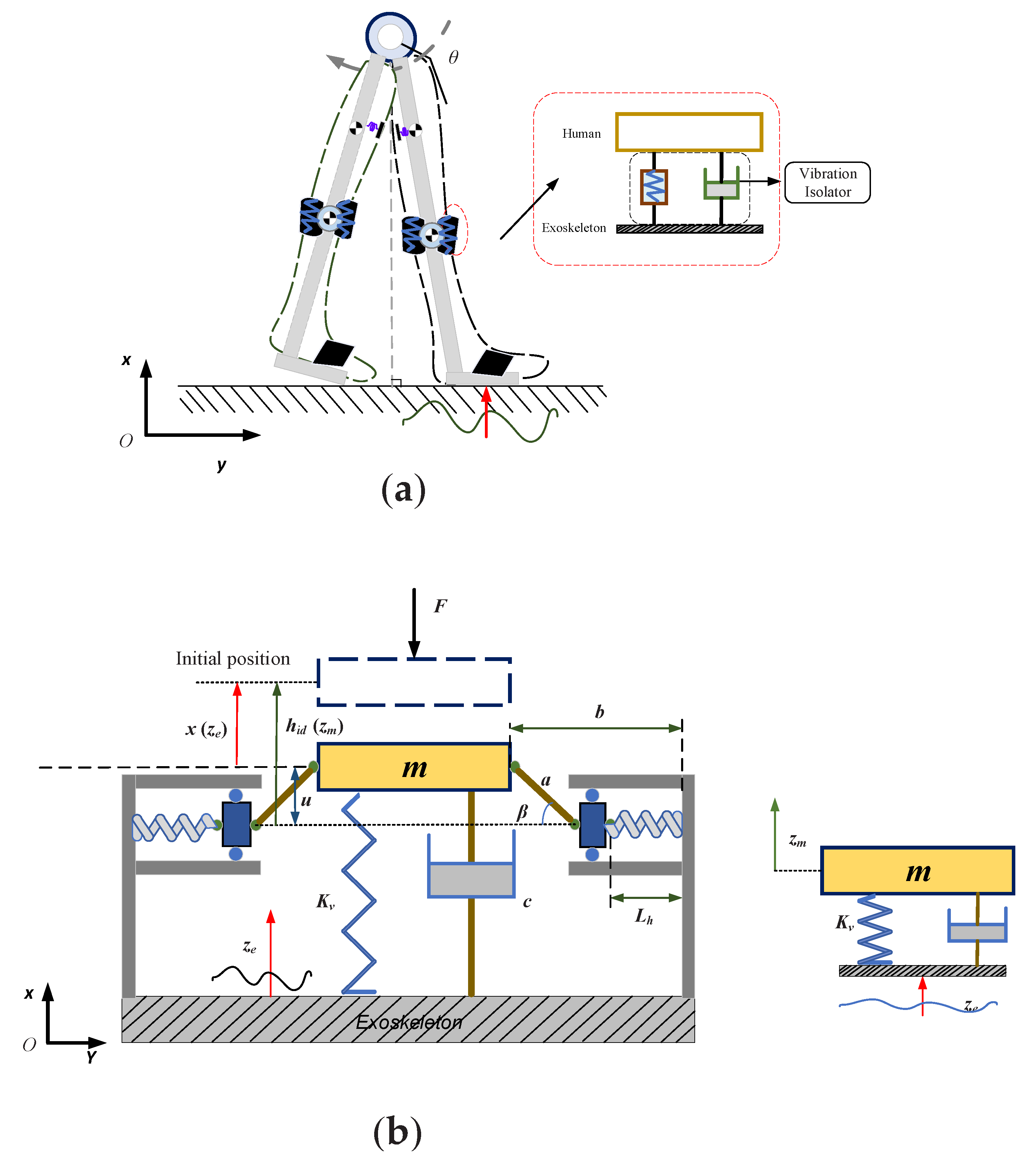
2.2. Design Procedure of the Vibration Isolation System
2.3. Human-Limb Model
2.4. Numerical Solution of Nonlinear Vibration Isolation System
2.5. Harmonic-Balance Solution for Nonlinear Vibration-Isolation Systems
3. Impedance Control of Integrated Robotic Systems
3.1. Dynamic Model of SEA-Driven Robot
3.2. Impedance Control
3.3. Lyapunov Stability Analysis
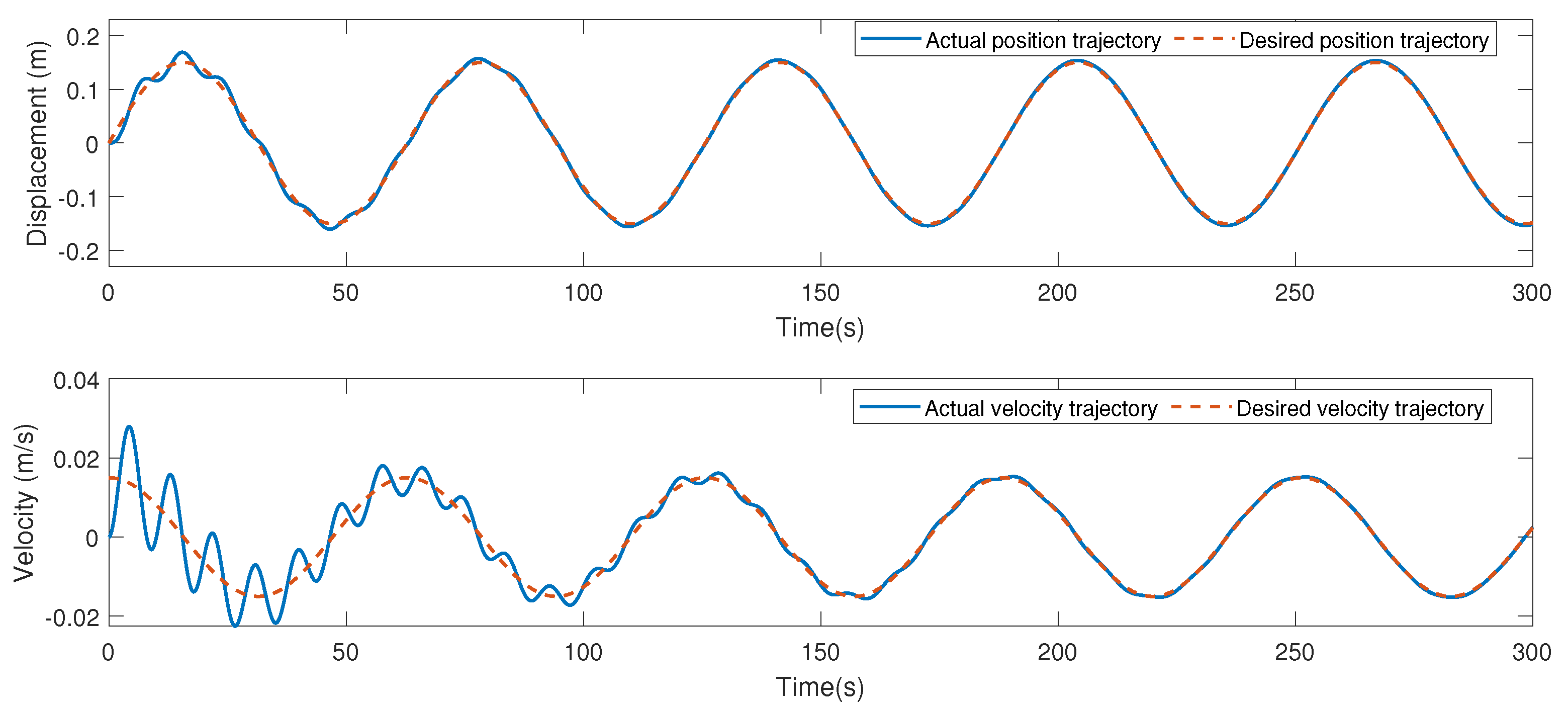
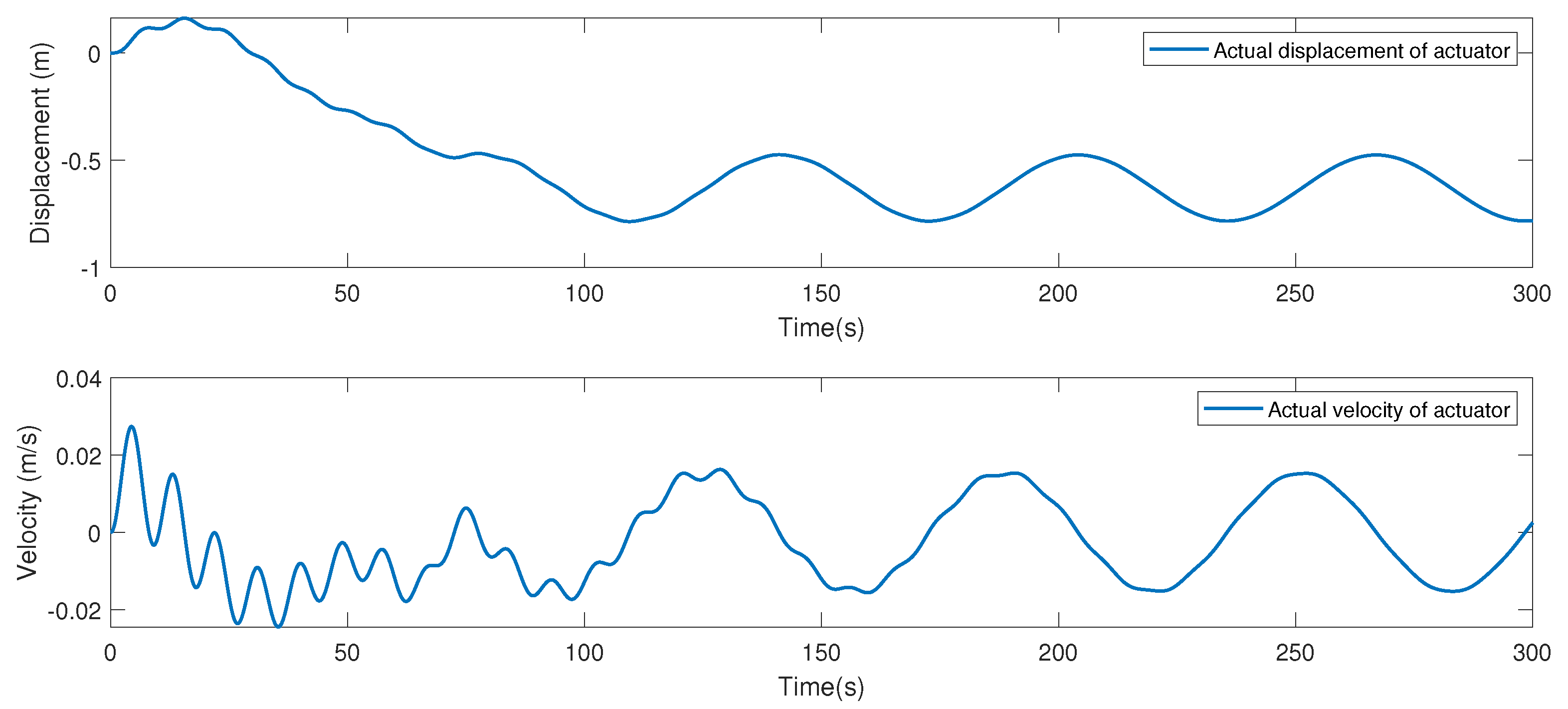
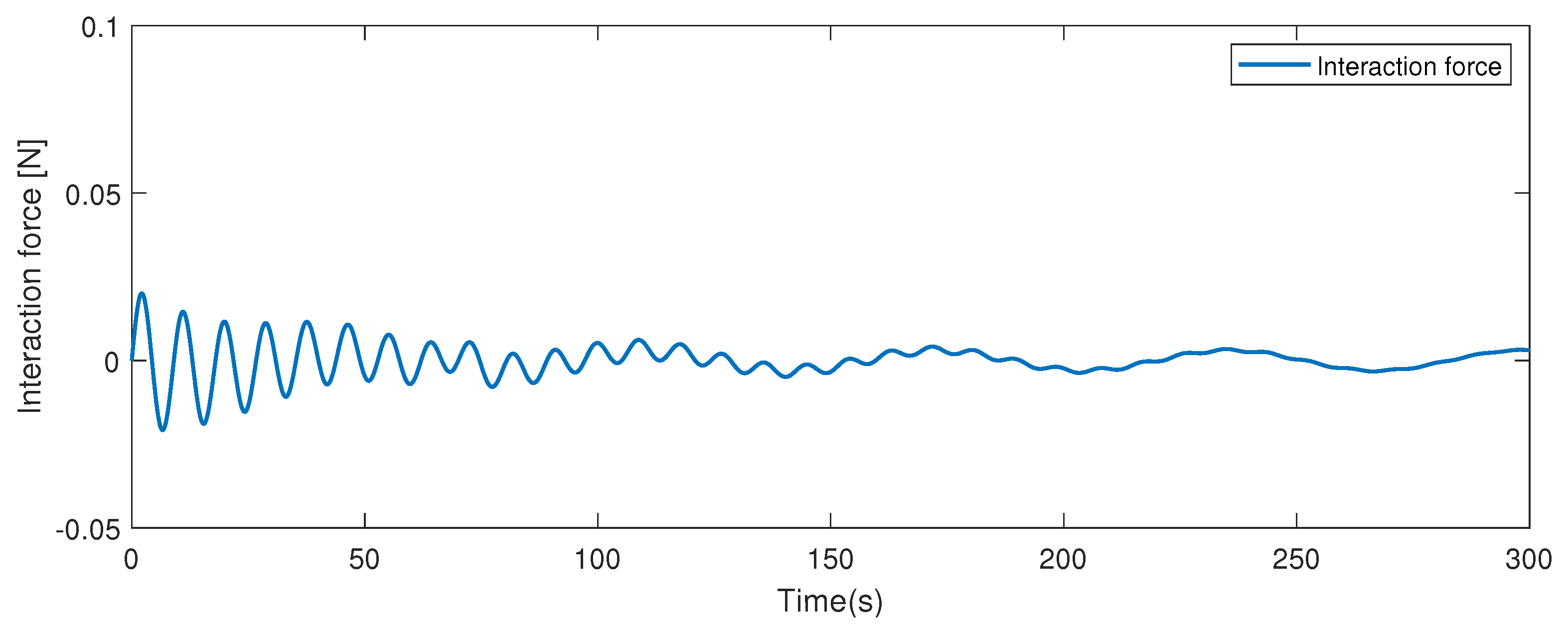
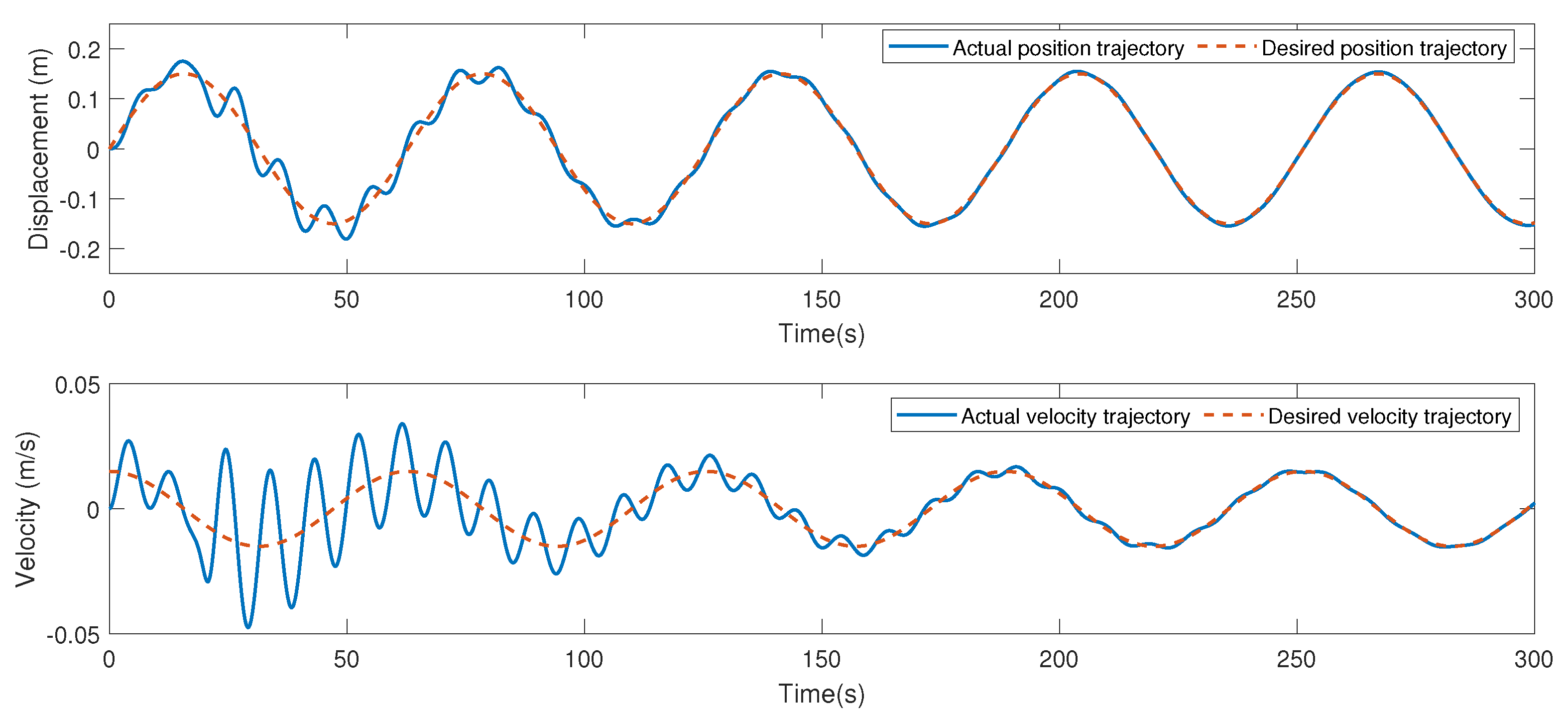
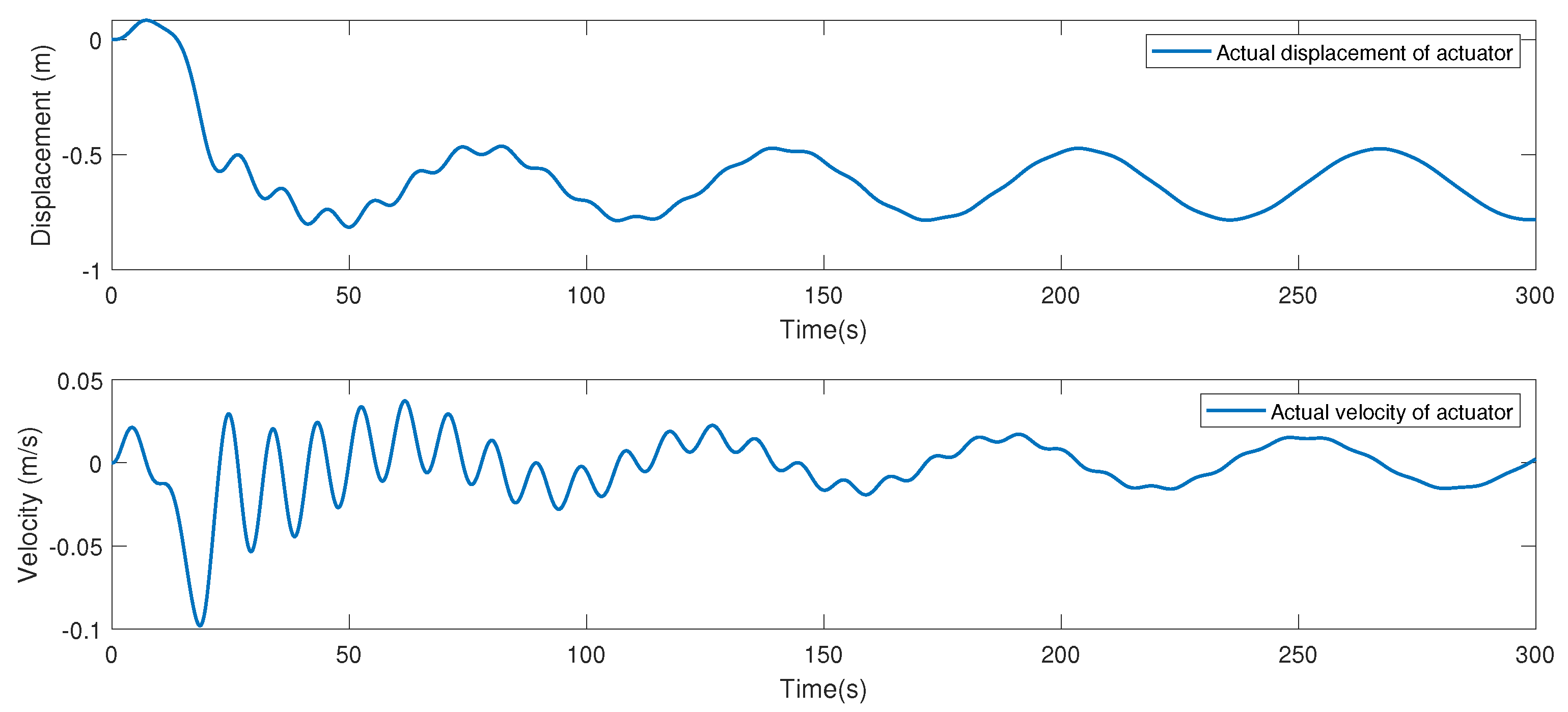
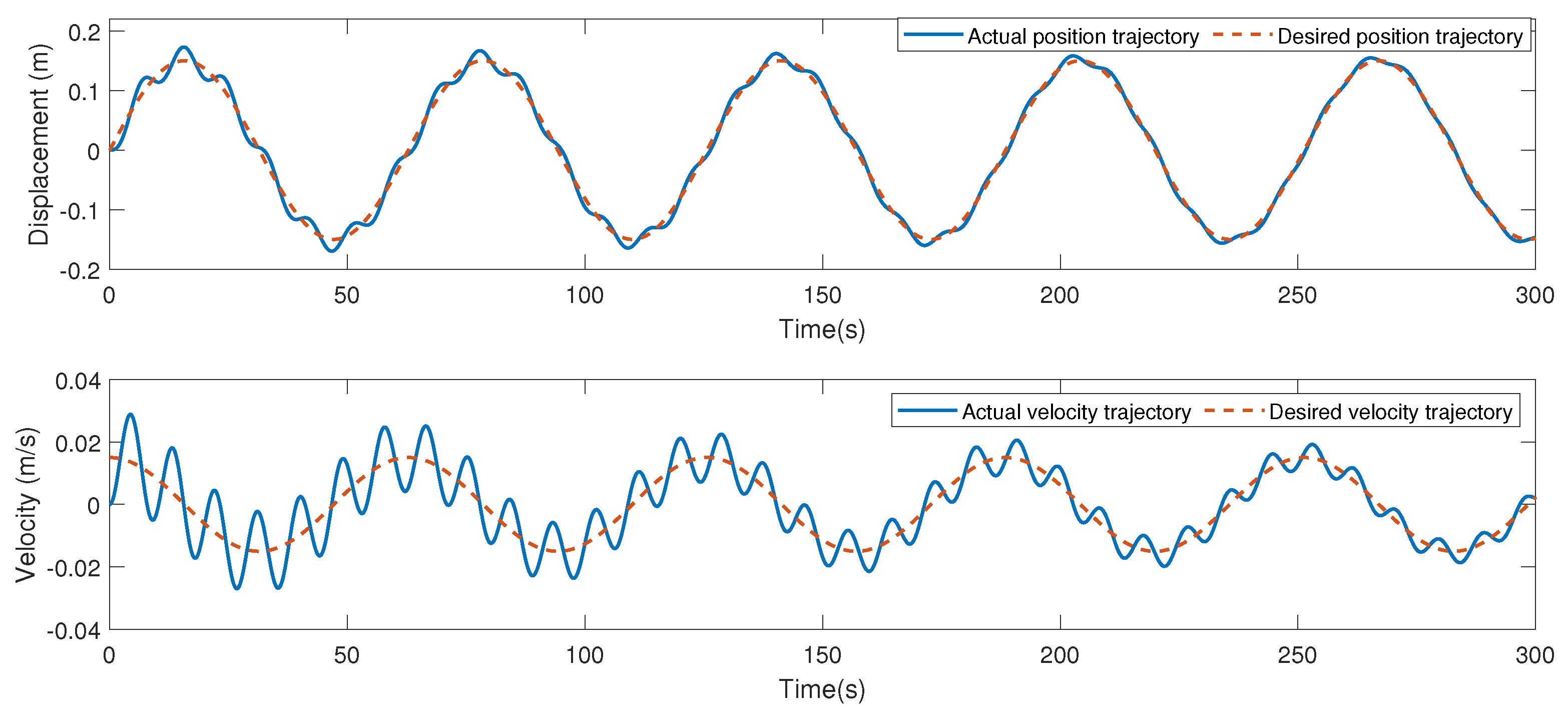
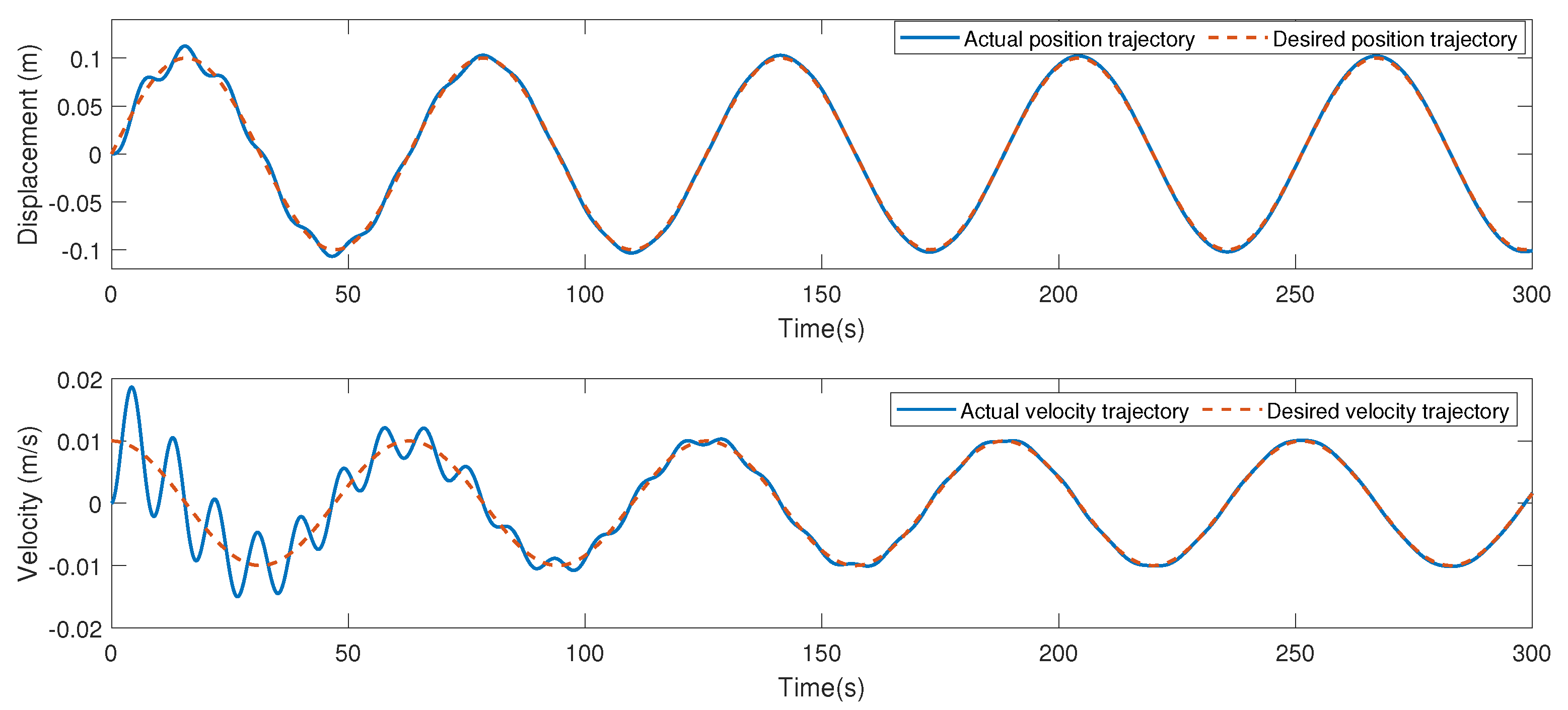
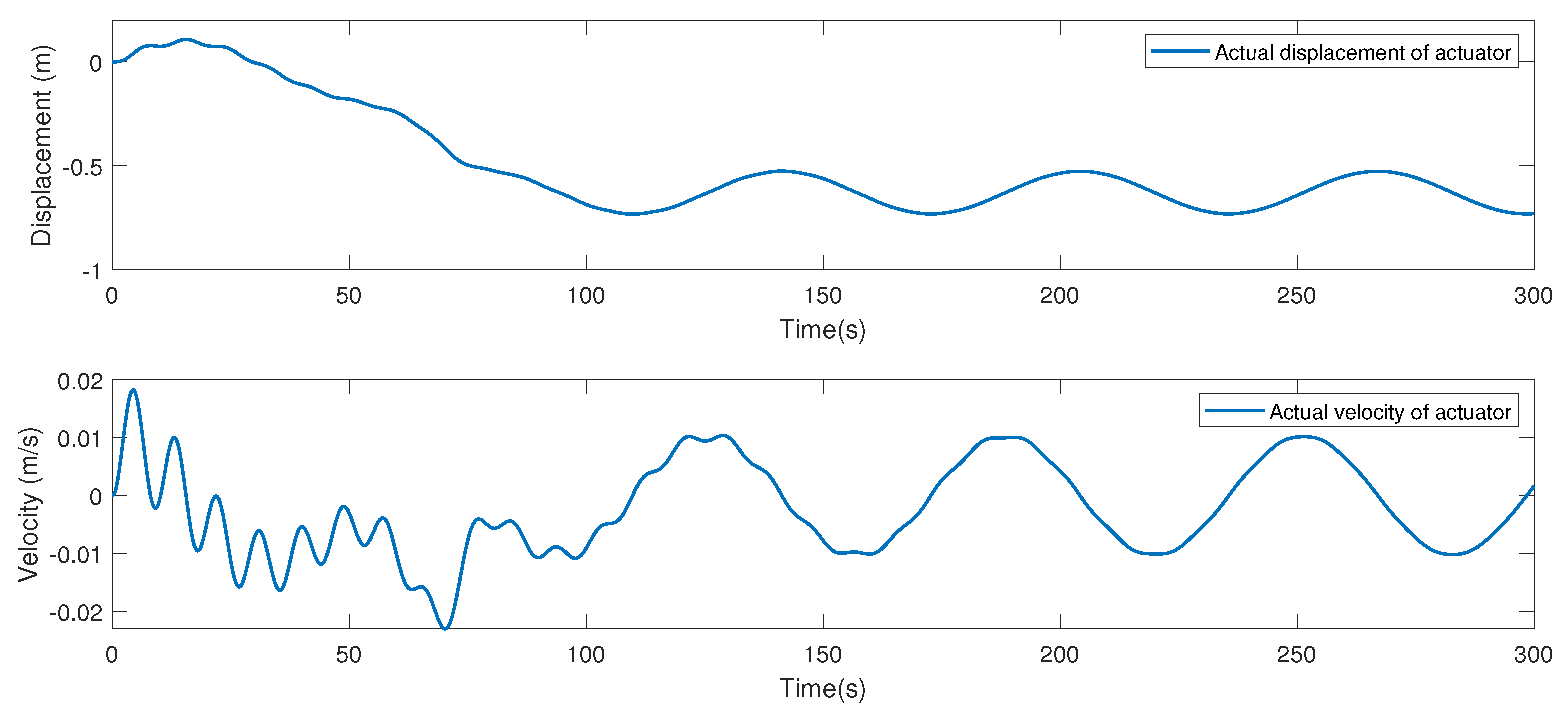
3.4. Simulation Results
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Qiao, H.; Wang, M.; Su, J.; Jia, S.; Li, R. The concept of “attractive region in environment” and its application in high-precision tasks with low-precision systems. IEEE/ASME Trans. Mechatron. 2015, 20, 2311–2327. [Google Scholar] [CrossRef]
- Guo, Q.; Yin, J.M.; Yu, T.; Jiang, D. Coupled-disturbance-observer-based position tracking control for a cascade electrohydraulic system. ISA Trans. 2017, 68, 367–380. [Google Scholar] [CrossRef]
- Chen, M.; Shao, S.-Y.; Jiang, B. Adaptive neural control of uncertain nonlinear systems using disturbance observer. IEEE Trans. Cybern. 2017, 47, 3110–3123. [Google Scholar] [CrossRef] [PubMed]
- Yu, X.; He, W.; Li, Y.; Xue, C.; Li, J.; Zou, J.; Yang, C. Bayesian estimation of human impedance and motion intention for human-robot collaboration. IEEE Trans. Cybern. 2021, 51, 1822–1834. [Google Scholar] [CrossRef] [PubMed]
- Maciejewski, I.; Meyer, L.; Krzyzynski, T. Modelling and multi-criteria optimisation of passive seat suspension vibro-isolating properties. J. Sound Vib. 2009, 324, 520–538. [Google Scholar] [CrossRef]
- Abbas, W.; Emam, A.; Badran, S.; Shebl, M.; Abouelatta, O. Optimal seat suspension design for a half-car with driver model using genetic algorithm. Intell. Control Autom. 2013, 4, 199–205. [Google Scholar] [CrossRef]
- Corbridge, C.; Griffin, M.J. Vibration and comfort: Vertical and lateral motion in the range 0.5 to 5.0 Hz. Ergonomics 1986, 29, 249–272. [Google Scholar] [CrossRef]
- Palomares, E.; Nieto, A.J.; Morales, A.L.; Chicharro, J.M.; Pintado, P. Dynamic behaviour of pneumatic linear actuators. Mechatronics 2017, 45, 37–48. [Google Scholar] [CrossRef]
- Zhang, J.; Liu, X.; Xia, Y.; Zuo, Z.; Wang, Y. Disturbance observer-based integral sliding-mode control for systems with mismatched disturbances. IEEE Trans. Ind. Electron. 2016, 63, 7040–7048. [Google Scholar] [CrossRef]
- Manevitch, L.I. The Description of Localized Normal Modes in a Chain of Nonlinear Coupled Oscillators Using Complex Variables. Nonlinear Dyn. 2001, 25, 95–109. [Google Scholar] [CrossRef]
- Fang, Z.W.; Zhang, Y.W.; Li, X.; Ding, H.; Chen, L.Q. Integration of a nonlinear energy sink and a giant magnetostrictive energy harvester. J. Sound Vib. 2017, 319, 35–49. [Google Scholar] [CrossRef]
- Fang, Z.W.; Zhang, Y.W.; Li, X.; Ding, H.; Chen, L.Q. Complexification-averaging analysis on a giant magnetostrictive harvester integrated with a nonlinear energy sink. J. Vib. Acoust. 2017, 140, 021009. [Google Scholar] [CrossRef]
- Lu, Z.Q.; Gu, D.H.; Ding, H.; Lacarbonara, W.; Chen, L.Q. A ring vibration isolator enhanced by shape memory pseudoelasticity. Appl. Math. Model. 2021, 100, 1–15. [Google Scholar] [CrossRef]
- Lu, Z.Q.; Brennan, M.J.; Yang, T.J.; Li, X.; Liu, Z.G. An investigation of a two stage nonlinear vibration isolation system. J. Sound Vib. 2013, 332, 1456–1464. [Google Scholar] [CrossRef]
- Lu, Z.Q.; Gu, D.H.; Ding, H.; Lacarbonara, W.; Chen, L.Q. Nonlinear vibration isolation via a circular ring. Mech. Syst. Signal. Process. 2020, 136, 106490. [Google Scholar] [CrossRef]
- Lu, Z.Q.; Brennan, M.J.; Ding, H.; Chen, L.Q. High-static-low-dynamic-stiffness vibration isolation enhanced by damping nonlinearity. Sci. China Tech. Sci. 2019, 62, 1103–1110. [Google Scholar] [CrossRef]
- Lu, Z.Q.; Yang, T.J.; Brennan, M.J.; Liu, Z.G.; Chen, L.Q. Experimental Investigation of a Two-stage Nonlinear Vibration Isolation System with High-static-Low-Dynamic Stiffness. ASME J. Appl. Mech. 2017, 84, 021001. [Google Scholar] [CrossRef]
- Matsumoto, Y.; Griffin, M.J. Dynamic response of the standing human body exposed to vertical vibration: Influence of posture and vibration magnitude. J. Sound Vib. 1998, 212, 81–94. [Google Scholar] [CrossRef]
- Lee, C.M.; Goverdovskiy, V.N.; Temnikov, A.I. Design of springs with “negative” stiffness to improve vehicle driver isolation. J. Sound Vib. 2007, 32, 865–874. [Google Scholar] [CrossRef]
- Lee, C.M.; Goverdovskiy, V.N. A multi-stage high-speed railroad vibration isolation system with “negative” stiffness. J. Sound Vib. 2012, 331, 914–921. [Google Scholar] [CrossRef]
- Le, T.D.; Ahn, K.K. Experimental investigation of a vibration isolation system using negative stiffness structure. Int. J. Mech. Sci. 2013, 70, 99–112. [Google Scholar] [CrossRef]
- Sun, Y.H.; Zhang, Y.W.; Ding, H.; Chen, L.Q. Nonlinear energy sink for a flywheel system vibration reduction. J. Sound Vib. 2018, 429, 305–324. [Google Scholar] [CrossRef]
- Oyelade, A.O. Vibration isolation using a bar and an Euler beam as negative stiffness for vehicle seat comfort. Adv. Mech. Eng. 2019, 11, 1–10. [Google Scholar] [CrossRef]
- Tu, L.; Ning, D.; Sun, S.; Li, W.; Huang, H.; Dong, M.; Du, H. A novel negative stiffness magnetic spring design for vehicle seat suspension system. Mechatronics 2020, 68, 102370. [Google Scholar] [CrossRef]
- Phu, D.X.; Choi, S.M.; Choi, S.B. A new adaptive hybrid controller for vibration control of a vehicle seat suspension featuring MR damper. J. Vib. Control. 2017, 23, 3392–3413. [Google Scholar] [CrossRef]
- Kopets, E.; Karimov, A.; Scalera, L.; Butusov, D. Estimating Natural Frequencies of Cartesian 3D Printer Based on Kinematic Scheme. Appl. Sci. 2022, 12, 4514. [Google Scholar] [CrossRef]
- Gholikord, M.; Etemadi, E.; Imani, M.; Hosseinabadi, M.; Hu, H. Design and analysis of novel negative stifness structures with significant energy absorption. Thin Wall Struct. 2022, 181, 110137. [Google Scholar] [CrossRef]
- Kong, K.; Bae, J.; Tomizuka, M. Control of rotary series elastic actuator for ideal force-mode actuation in human–robot interaction applications. IEEE/ASME Trans. Mechatron. 2009, 14, 105–118. [Google Scholar] [CrossRef]
- Wang, M.; Sun, L.; Yin, W.; Dong, S.; Liu, J.-T. Series elastic actuator torque control approach for interaction application. Acta Autom. Sin. 2017, 43, 1319–1328. [Google Scholar]
- Li, Y.; Tee, K.P.; Chan, W.L.; Yan, R.; Chua, Y.; Limbu, D.K. Continuous role adaptation for human-robot shared control. IEEE Trans. Robot. 2015, 31, 672–681. [Google Scholar] [CrossRef]
- Li, X.; Liu, Y.H.; Yu, H. Iterative learning impedance control for rehabilitation robots driven by series elastic actuators. Automatica 2018, 90, 1–7. [Google Scholar] [CrossRef]
- Hsieh, H.C.; Chen, D.F.; Chien, L.; Lan, C.C. Design of a parallel actuated exoskeleton for adaptive and safe robotic shoulder rehabilitation. IEEE/ASME Trans. Mechatron. 2017, 22, 2034–2045. [Google Scholar] [CrossRef]
- Ju, Z.; Ouyang, G.; Wilamowska-Korsak, M.; Liu, H. Surface EMG based hand manipulation identification via nonlinear feature extraction and classification. IEEE Sens. J. 2013, 13, 3302–3311. [Google Scholar] [CrossRef]
- Noohi, E.; Žefran, M.; Patton, J.L. A model for human–human collaborative object manipulation and its application to human–robot interaction. IEEE Trans. Robot. 2016, 32, 880–896. [Google Scholar] [CrossRef]
- Li, Y.; Tee, K.P.; Yan, R.; Chan, W.L.; Wu, Y. A framework of human–robot coordination based on game theory and policy iteration. IEEE Trans. Robot. 2016, 32, 1408–1418. [Google Scholar] [CrossRef]
- Li, Z.; Kang, Y.; Xiao, Z.; Song, W. Human-robot coordination control of robotic exoskeletons by skill transfers. IEEE Trans. Ind. Electron. 2017, 64, 5171–5181. [Google Scholar] [CrossRef]
- Li, Y.; Ge, S.S. Human–Robot collaboration based on motion intention estimation. IEEE/ASME Trans. Mechatron. 2014, 19, 1007–1014. [Google Scholar] [CrossRef]
- Le, T.D.; Ahn, K.K. Active pneumatic isolator system using negative stiffness structure for a vehicle seat. J. Sound Vib. 2014, 333, 1245–1268. [Google Scholar]
- Le, T.D.; Ahn, K.K. A vibration isolation system in low frequency excitation region using negative stiffness structure for vehicle seat. J. Sound Vib. 2011, 330, 6311–6335. [Google Scholar] [CrossRef]
- Huang, R.; Cheng, H.; Guo, H.; Lin, X.; Zhang, J. Hierarchical learning control with physical human-exoskeleton interaction. Inf. Sci. 2018, 432, 584–595. [Google Scholar] [CrossRef]
- Huang, R.; Cheng, H.; Qiu, J.; Zhang, J. Learning physical human-robot interaction with coupled cooperative primitives for a lower exoskeleton. IEEE Trans. Autom. Sci. Eng. 2019, 16, 1566–1574. [Google Scholar] [CrossRef]
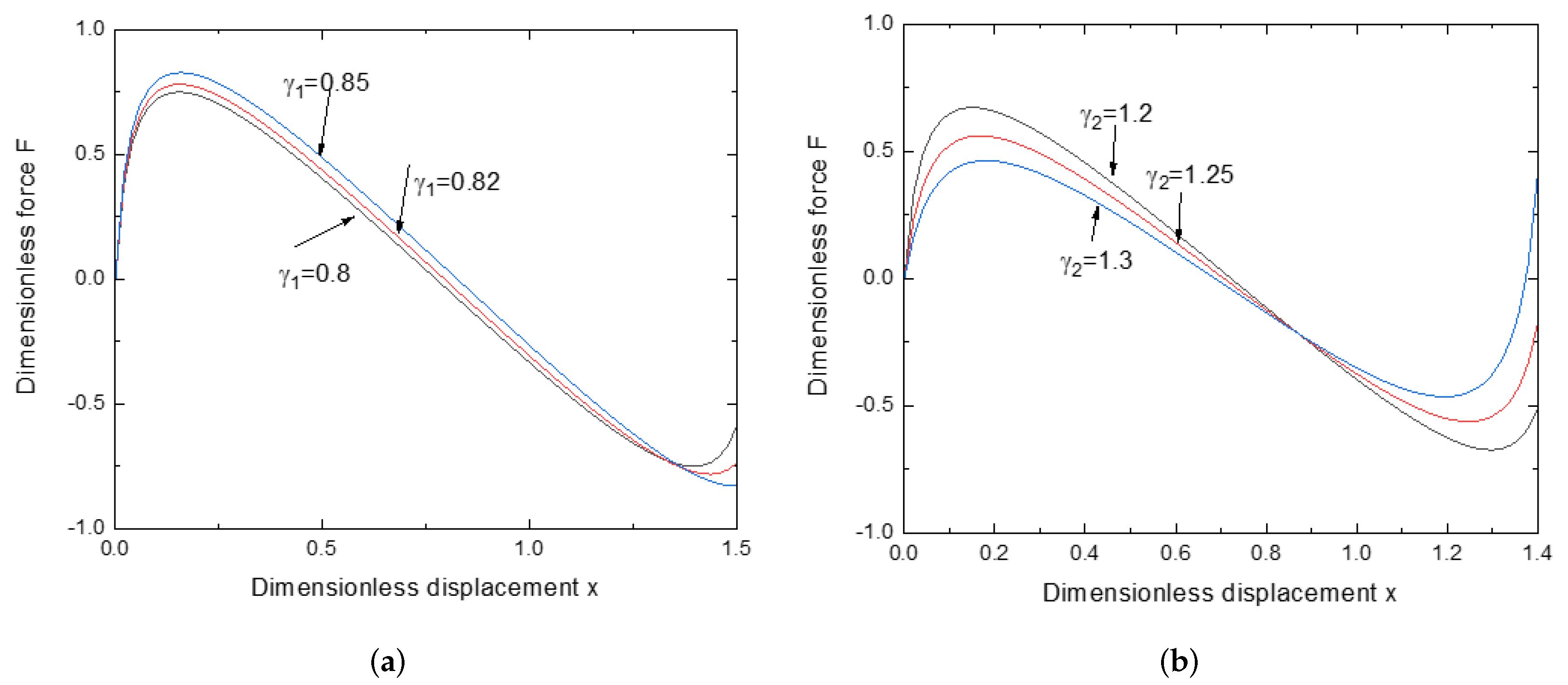
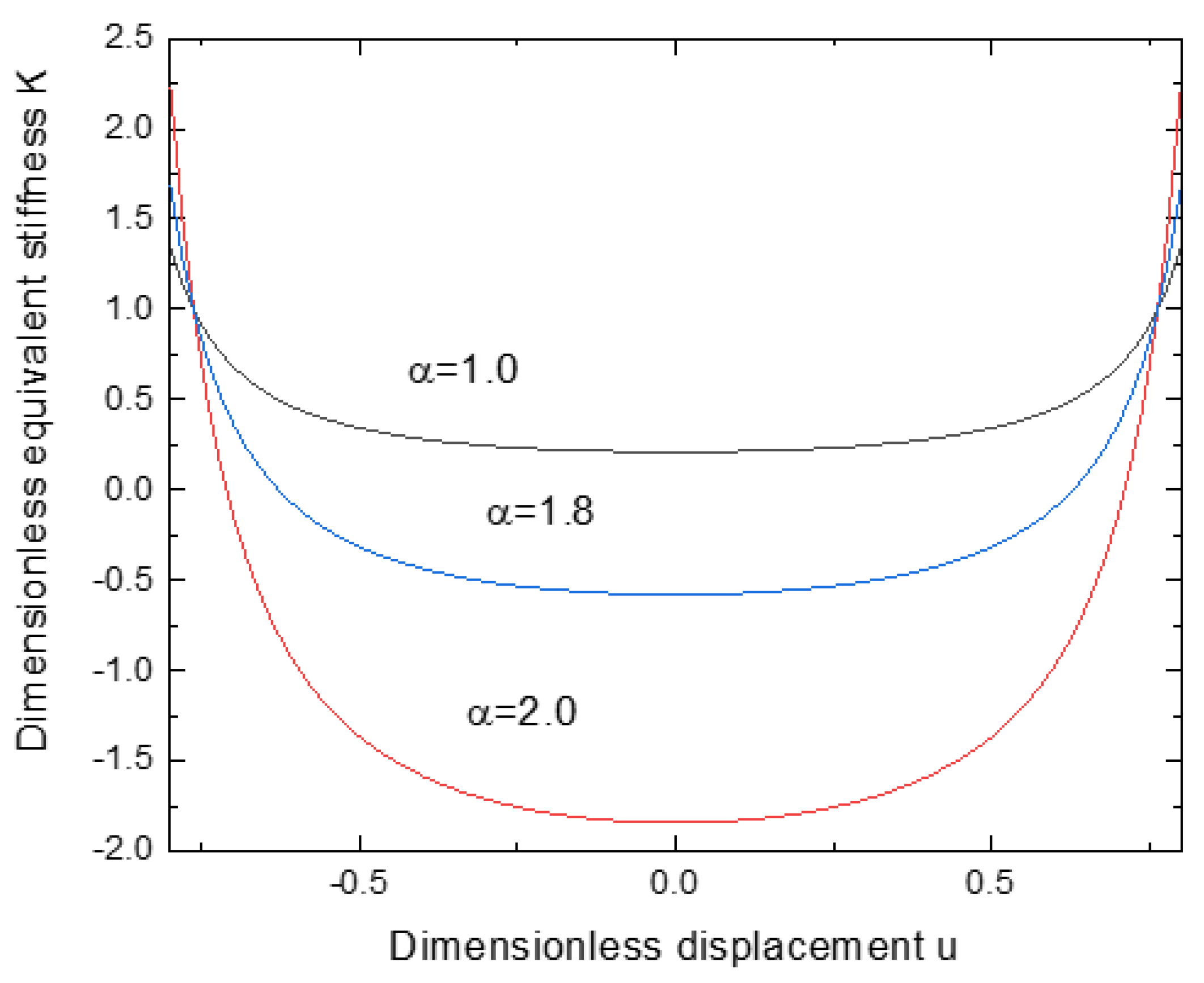
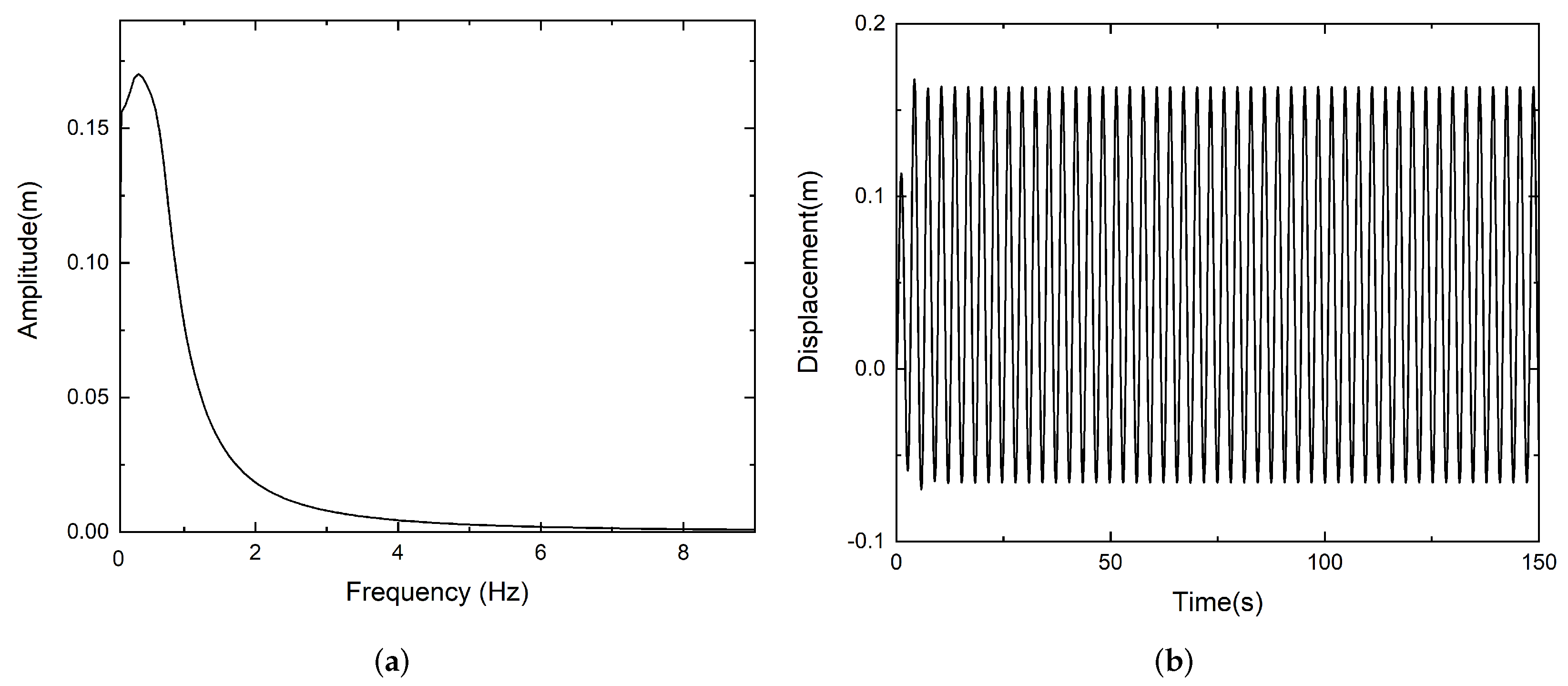

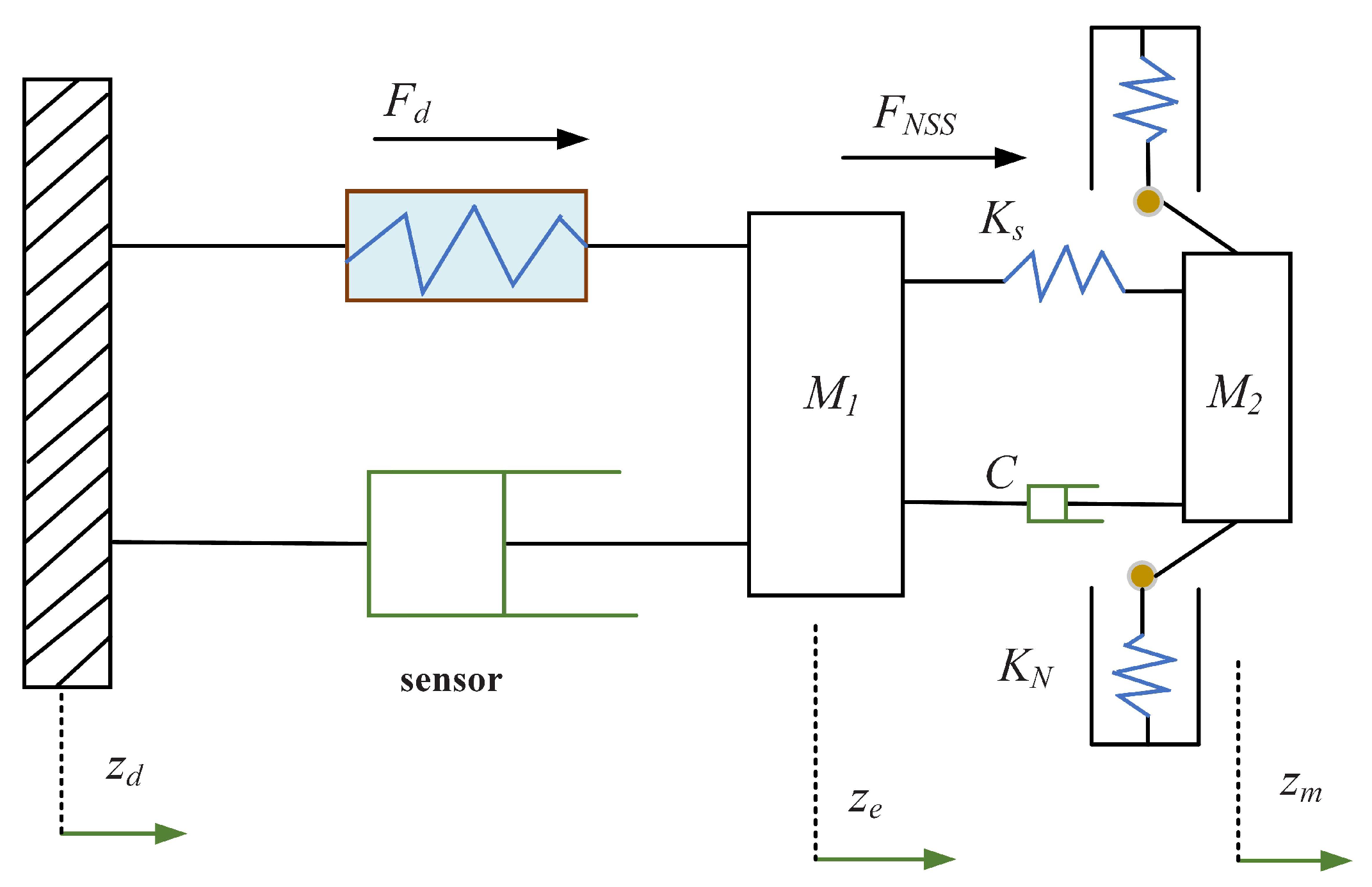
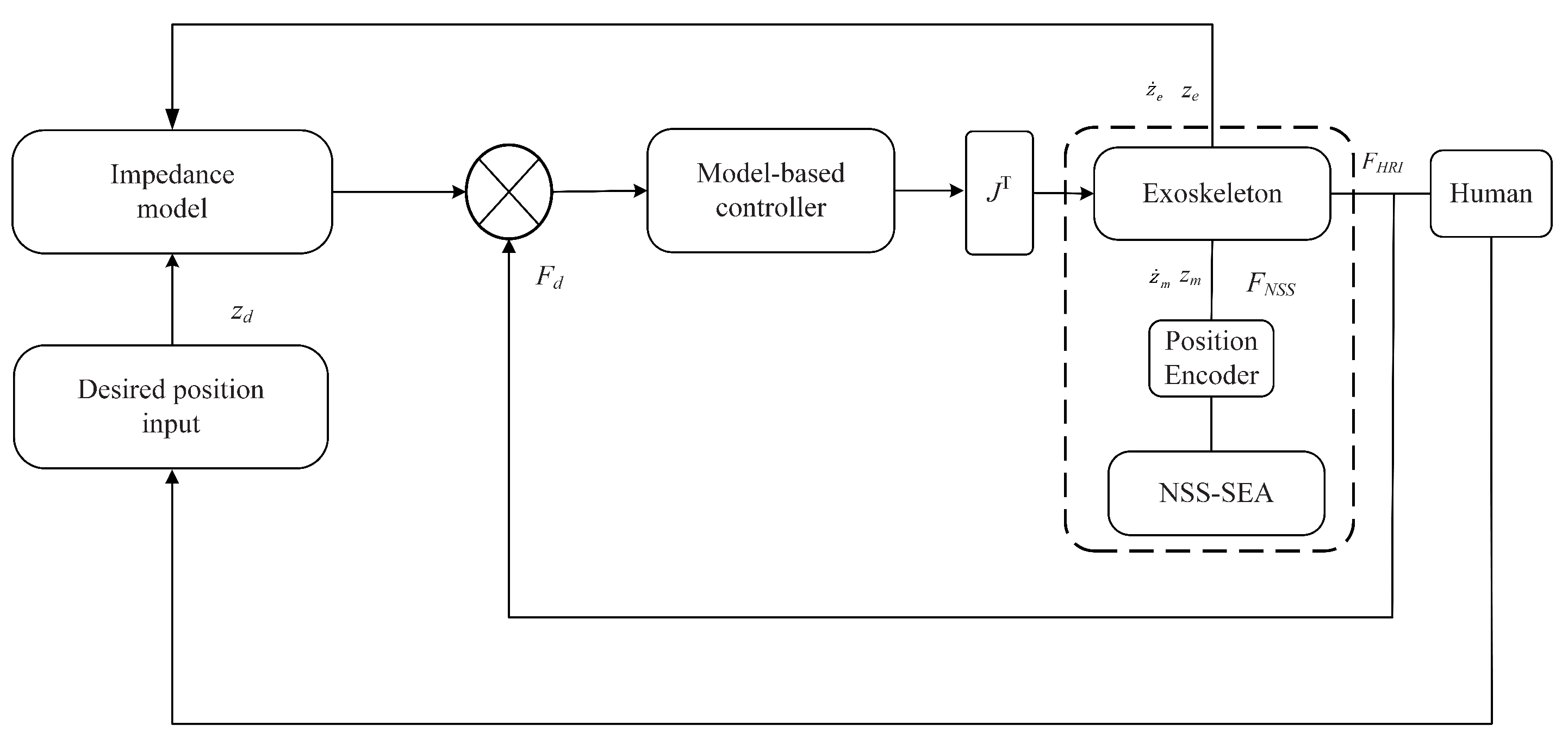

| Parameters | Change in the Value of | Change in the Value of |
|---|---|---|
| 0.8, 0.82 and 0.85 | 0.75 | |
| 1.2 | 1.2, 1.25 and 1.3 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Sun, Y.; Hu, J.; Huang, R. Negative-Stiffness Structure Vibration-Isolation Design and Impedance Control for a Lower Limb Exoskeleton Robot. Actuators 2023, 12, 147. https://doi.org/10.3390/act12040147
Sun Y, Hu J, Huang R. Negative-Stiffness Structure Vibration-Isolation Design and Impedance Control for a Lower Limb Exoskeleton Robot. Actuators. 2023; 12(4):147. https://doi.org/10.3390/act12040147
Chicago/Turabian StyleSun, Yaohui, Jiangping Hu, and Rui Huang. 2023. "Negative-Stiffness Structure Vibration-Isolation Design and Impedance Control for a Lower Limb Exoskeleton Robot" Actuators 12, no. 4: 147. https://doi.org/10.3390/act12040147
APA StyleSun, Y., Hu, J., & Huang, R. (2023). Negative-Stiffness Structure Vibration-Isolation Design and Impedance Control for a Lower Limb Exoskeleton Robot. Actuators, 12(4), 147. https://doi.org/10.3390/act12040147









