Reaction Force-Based Position Sensing for Magnetic Levitation Platform with Exceptionally Large Hovering Distance
Abstract
1. Introduction
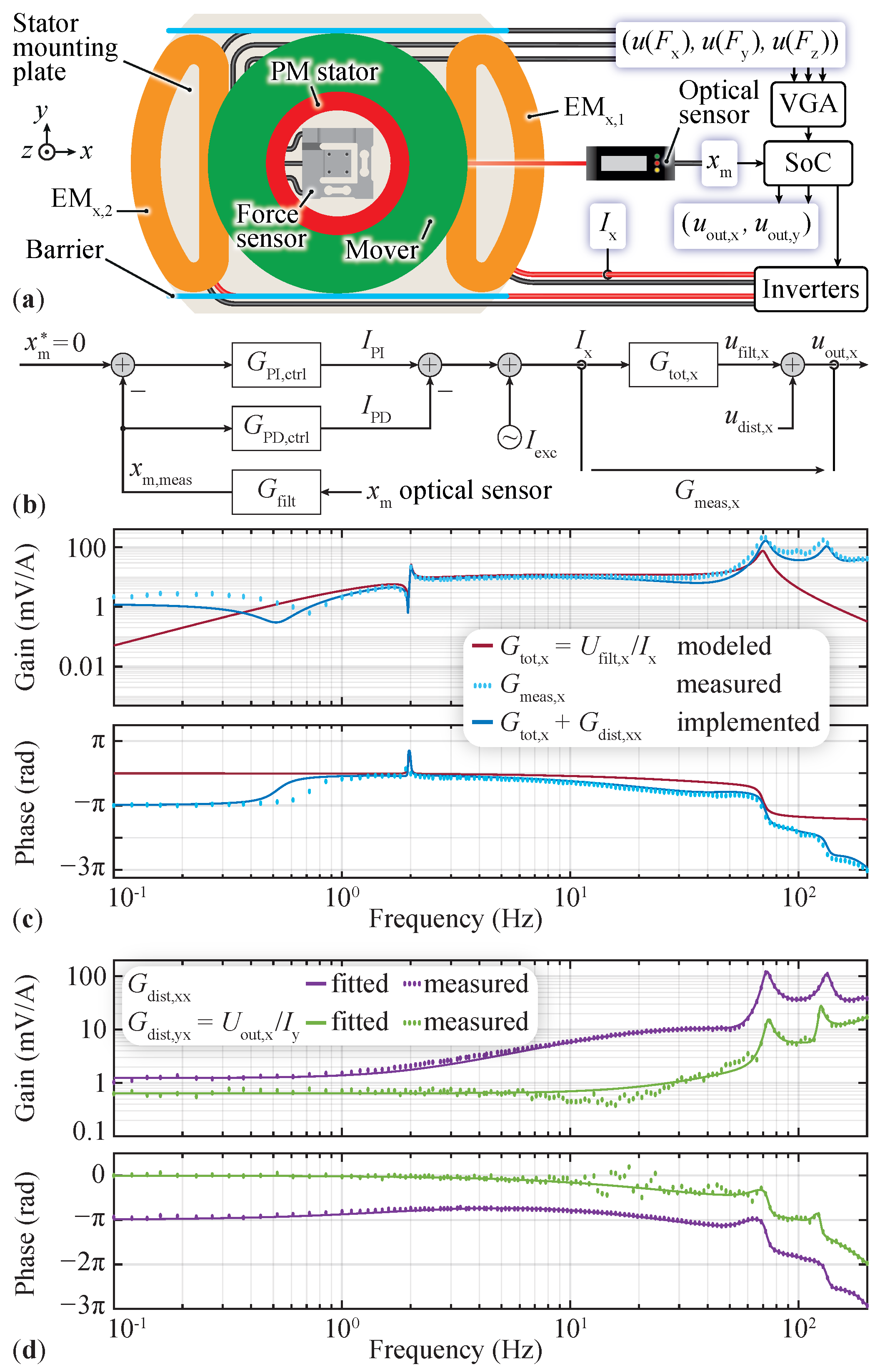
2. Magnetic Levitation Platform Overview
3. Dynamics Modeling
3.1. Radial Motion Dynamics
3.2. Rotational Dynamics
3.3. Force Sensor Mechanical Dynamics
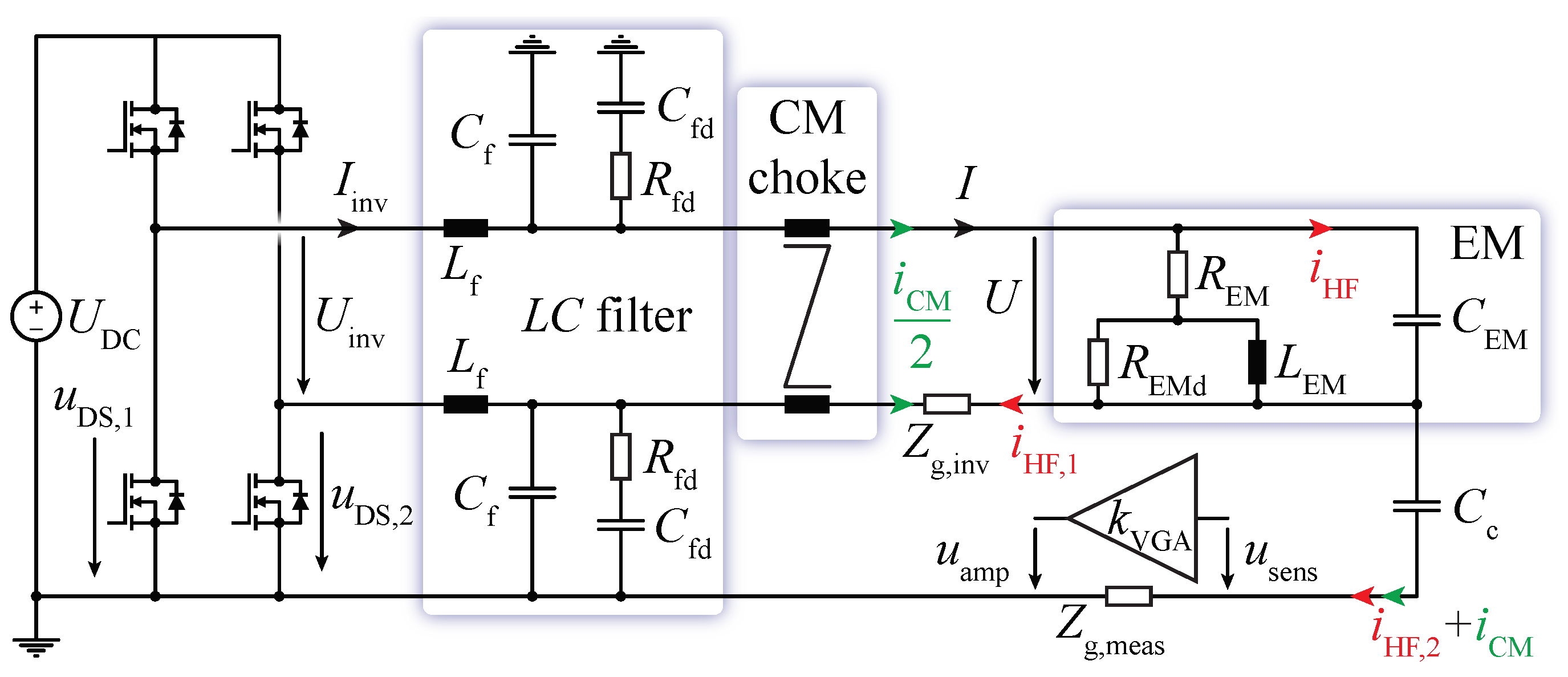
3.4. Force Sensor Electrical Dynamics
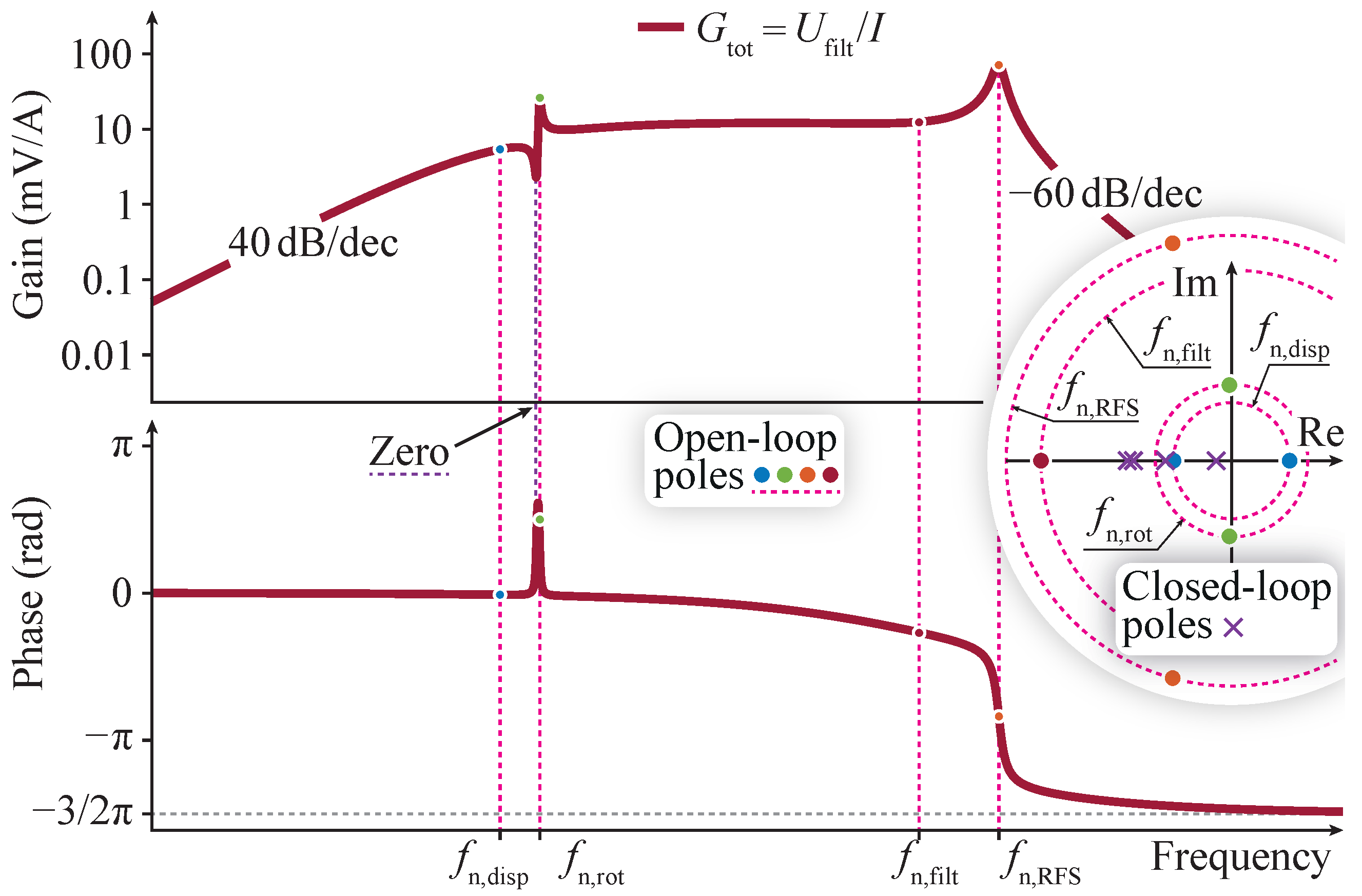
3.5. Summary of the Dynamics
4. Dynamic Model Verification and Tuning
4.1. Mover’s Position Controller
4.2. Dynamic Model Proof and Adaption
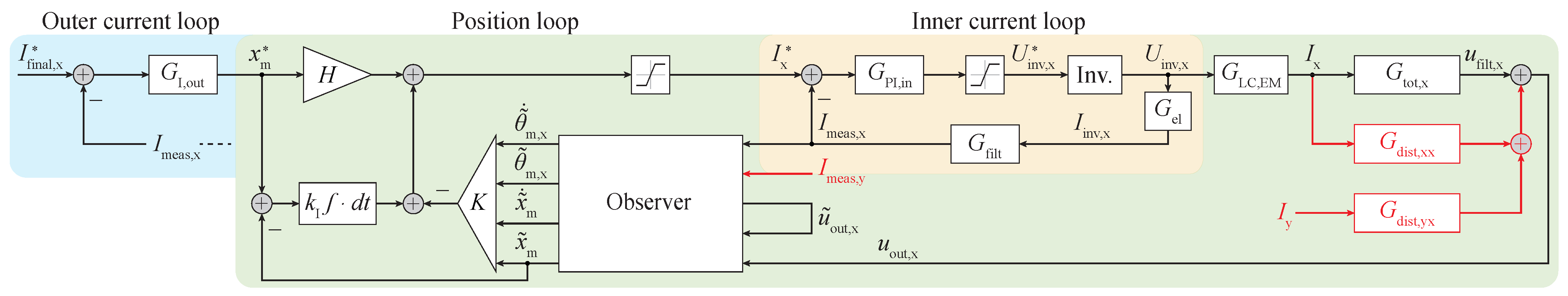
5. Observer and Controller Design
5.1. Observer
5.2. Mover’s Position Controller and Rotation Damping
5.3. Inverter Stage
5.4. Position Sensor Offset Compensation
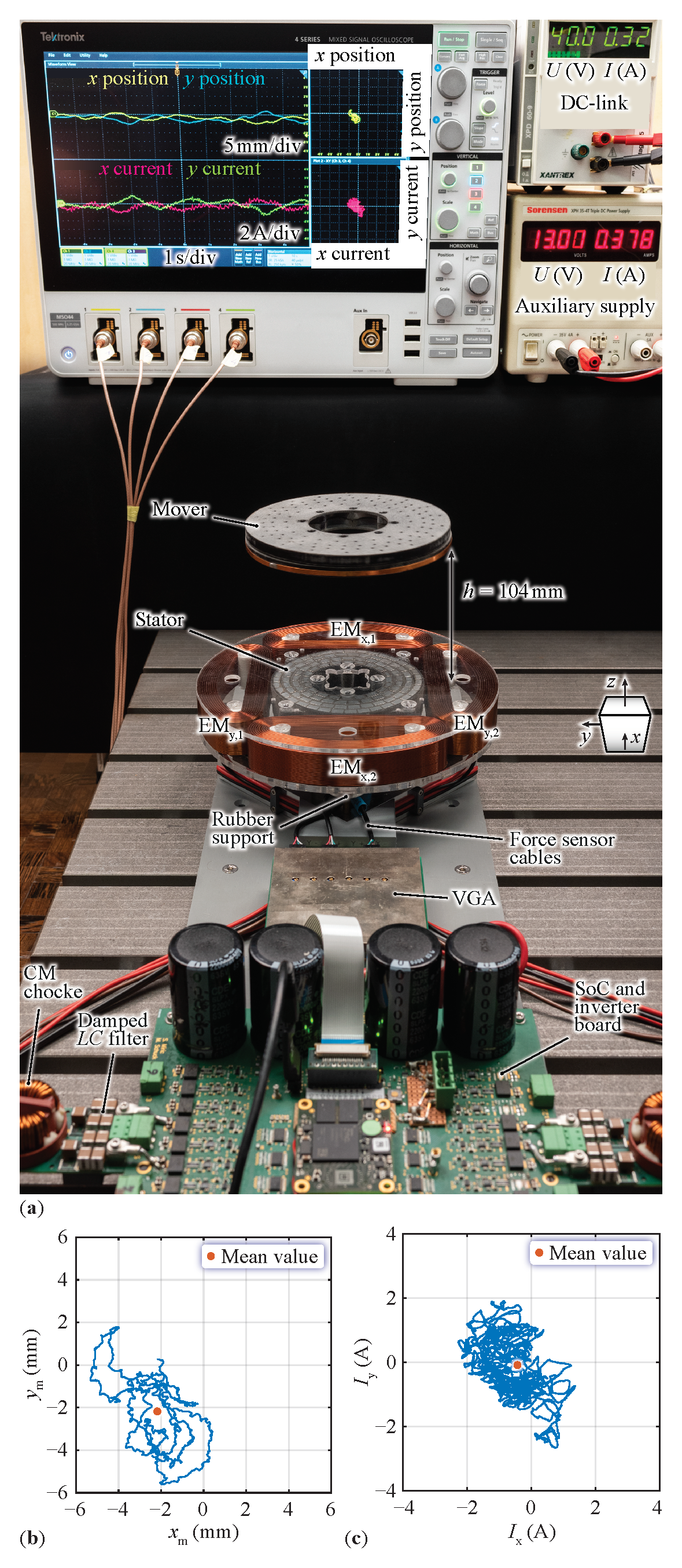
6. Hardware Demonstrator Realization
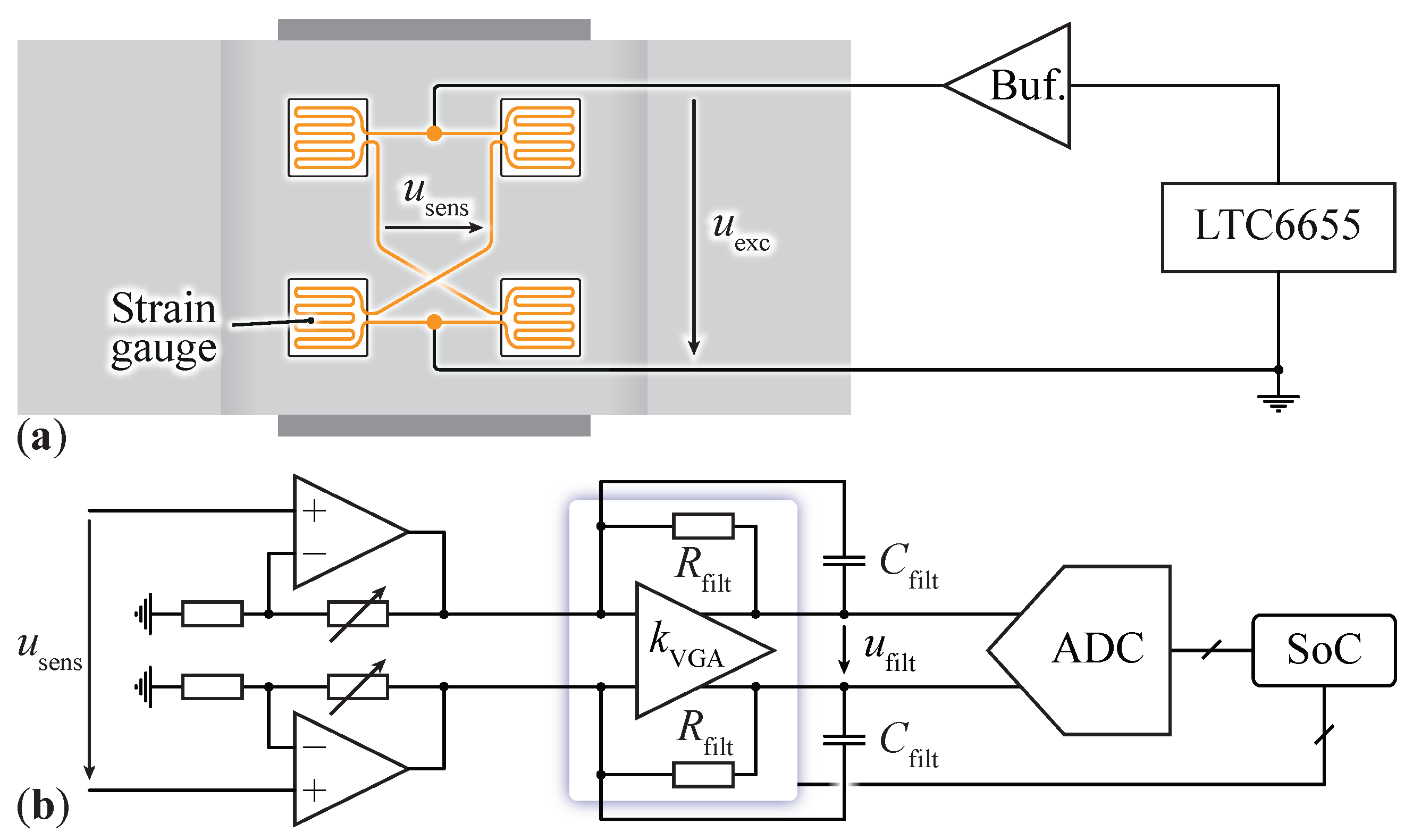
6.1. Force Sensor
6.2. Controller Tuning
6.3. Results
7. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
Appendix A. Design of the Electromagnets
| Design Parameters | |||
| 40 V | 6 A | ||
| 10 mm | mm | ||
| mm | 23 mm | ||
| mm | |||
| Calculated Characteristics | |||
| N | 550 | 20 | |
| 5.5 Ω | kg | ||
References
- Lai, Y.C.; Lee, Y.L.; Yen, J.Y. Design and Servo Control of a Single-Deck Planar MAGLEV Stage. IEEE Trans. Magn. 2007, 43, 2600–2602. [Google Scholar] [CrossRef]
- Zhu, H.; Teo, T.J.; Pang, C.K. Design and Modeling of a Six-Degree-of-Freedom Magnetically Levitated Positioner using Square Coils and 1-D Halbach Arrays. IEEE Trans. Ind. Electron. 2017, 64, 440–450. [Google Scholar] [CrossRef]
- Lahdo, M.; Ströhla, T.; Kovalev, S. Design and Implementation of an New 6-DOF Magnetic Levitation Positioning System. IEEE Trans. Magn. 2019, 55, 8107407. [Google Scholar] [CrossRef]
- Zhang, X.; Trakarnchaiyo, C.; Zhang, H.; Khamesee, M.B. MagTable: A Tabletop System for 6-DOF Large Range and Completely Contactless Operation using Magnetic Levitation. Mechatronics 2021, 77, 102600. [Google Scholar] [CrossRef]
- Trakarnchaiyo, C.; Wang, Y.; Khamesee, M.B. Design of a Compact Planar Magnetic Levitation System with Wrench-Current Decoupling Enhancement. Appl. Sci. 2023, 13, 2370. [Google Scholar] [CrossRef]
- Lomonova, E.A. Advanced Actuation Systems - State of the Art: Fundamental and Applied Research. In Proceedings of the IEEE International Conference on Electrical Machines and Systems, Incheon, Republic of Korea, 10–13 October 2010; pp. 13–24. [Google Scholar]
- Stadler, G.; Csencsics, E.; Ito, S.; Schitter, G. High Precision Hybrid Reluctance Actuator with Integrated Orientation Independent Zero Power Gravity Compensation. IEEE Trans. Ind. Electron. 2022, 69, 13296–13304. [Google Scholar] [CrossRef]
- Wertjanz, D.; Csencsics, E.; Schlarp, J.; Schitter, G. Design and Control of a MAGLEV Platform for Positioning in Arbitrary Orientations. In Proceedings of the IEEE/ASME International Conference on Advanced Intelligent Mechatronics (AIM), Boston, MA, USA, 6–9 July 2020; pp. 1935–1942. [Google Scholar]
- Chen, K.S.; Trumper, D.L.; Smith, S.T. Design and Control for an Electromagnetically Driven X-Y-θ Stage. Precis. Eng. 2002, 26, 355–369. [Google Scholar] [CrossRef]
- Kim, W.j.; Trumper, D.L. High-Precision Magnetic Levitation Stage for Photolithography. Precis. Eng. 1998, 22, 66–77. [Google Scholar] [CrossRef]
- Schaeffel, C.; Katzschmann, M.; Mohr, H.U.; Gloess, R.; Rudolf, C.; Mock, C.; Walenda, C. 6D Planar Magnetic Levitation System - PIMag 6D. Mech. Eng. J. 2016, 3, 15-00111. [Google Scholar] [CrossRef][Green Version]
- Berkelman, P.; Ohashi, N. Analysis and Testing of a Four Coil Magnetic Levitation Configuration. In Proceedings of the 48th Annual Conference of the IEEE Industrial Electronics Society (IECON), Brussels, Belgium, 17–20 October 2022; pp. 1–5. [Google Scholar]
- Song, H.; Lin, W.; Zhou, M.; Liu, G.; Pan, H.; Tong, M. Robust H∞ Control for Disturbance Rejection in a Magnetic Levitation Device. In Proceedings of the IEEE 28th International Symposium on Industrial Electronics (ISIE), Vancouver, BC, Canada, 12–14 June 2019; pp. 2170–2174. [Google Scholar]
- Britcher, C.P.; Ghofrani, M. A Magnetic Suspension System with a Large Angular Range. Rev. Sci. Instrum. 1993, 64, 1910–1917. [Google Scholar] [CrossRef]
- Chong, P.; Commandeur, C.; Davis, H.; Whitehead, L. Large-Gap Magnetic Positioning System having Advantageous Configuration. In Proceedings of the International Symposium on Magnetic Suspension Technology, Hampton, VA, USA, 19–23 August 1992; Part 2, pp. 941–953. [Google Scholar]
- Jansen, G.L.M. Magnetic Levitation Apparatus. WO2009038464A2, 26 March 2009. [Google Scholar]
- Bosley, R.W. Magnetic Levitation System. EP0193664A1, 10 September 1986. [Google Scholar]
- Davis, H.; Whitehead, L. Magnetic Levitation Apparatus. WO2004030198A2, 8 April 2004. [Google Scholar]
- Okuizumi, H.; Sawada, H.; Nagaike, H.; Konishi, Y.; Obayashi, S. Introduction of 1-m MSBS in Tohoku University, New Device for Aerodynamics Measurements of the Sports Equipment. In Proceedings of the 12th Conference of the International Sports Engineering Association, Brisbane, Australia, 26–29 March 2018; Volume 2, pp. 1–7. [Google Scholar]
- Inomata, C.; Kuwata, M.; Yokota, S.; Abe, Y.; Sawada, H.; Obayashi, S.; Asai, K.; Nonomura, T. Model Position Sensing Method for Low Fineness Ratio Models in a Magnetic Suspension and Balance System. Rev. Sci. Instrum. 2023, 94, 025102. [Google Scholar] [CrossRef] [PubMed]
- Pujol-Vázquez, G.; Vargas, A.N.; Mobayen, S.; Acho, L. Semi-Active Magnetic Levitation System for Education. Appl. Sci. 2021, 11, 5330. [Google Scholar] [CrossRef]
- van Lierop, C.M.M.; Jansen, J.W.; Damen, A.A.H.; Lomonova, E.A.; van den Bosch, P.P.J.; Vandenput, A.J.A. Model-Based Commutation of a Long-Stroke Magnetically Levitated Linear Actuator. IEEE Trans. Ind. Appl. 2009, 45, 1982–1990. [Google Scholar] [CrossRef]
- Berkelman, P.; Dzadovsky, M. Magnetic Levitation over Large Translation and Rotation Ranges in All Directions. IEEE/ASME Trans. Mechatron. 2013, 18, 44–52. [Google Scholar] [CrossRef]
- Kitagaki, K.; Ogasawara, T.; Suehiro, T. Methods to Detect Contact State by Force Sensing in an Edge Mating Task. In Proceedings of the IEEE International Conference on Robotics and Automation, Atlanta, GA, USA, 2–6 May 1993; Volume 2, pp. 701–706. [Google Scholar]
- Handa, H.; Nozaki, T.; Murakami, T. Identification of Contact Position and Force Estimation on Manipulator using Force Sensor Implemented on Base Frame. IEEJ J. Ind. Appl. 2021, 10, 324–330. [Google Scholar] [CrossRef]
- Komati, B.; Clévy, C.; Lutz, P. High Bandwidth Microgripper with Integrated Force Sensors and Position Estimation for the Grasp of Multistiffness Microcomponents. IEEE/ASME Trans. Mechatron. 2016, 21, 2039–2049. [Google Scholar] [CrossRef]
- Nam, K.; Oh, S.; Fujimoto, H.; Hori, Y. Estimation of Sideslip and Roll Angles of Electric Vehicles using Lateral Tire Force Sensors Through RLS and Kalman Filter Approaches. IEEE Trans. Ind. Electron. 2013, 60, 988–1000. [Google Scholar] [CrossRef]
- Bonetti, R.; Bortis, D.; Beglinger, L.; Kolar, J.W. Exploring the Physical Limits of Axial Magnetic Bearings Featuring Extremely Large Vertical Levitation Distances. IEEE Trans. Ind. Appl. 2021, 57, 6931–6943. [Google Scholar] [CrossRef]
- Kanj, A.; Thanalakshme, R.P.; Li, C.; Kulikowski, J.; Bahl, G.; Tawfick, S. Design, Dynamics, and Dissipation of a Torsional-Magnetic Spring Mechanism. Mech. Syst. Signal Process. 2022, 179, 109307. [Google Scholar] [CrossRef]
- Xiao, X. Dynamic Tensile Testing of Plastic Materials. Polym. Test. 2008, 27, 164–178. [Google Scholar] [CrossRef]
- Yang, X.; Hector, L.G.; Wang, J. A Combined Theoretical/Experimental Approach for Reducing Ringing Artifacts in Low Dynamic Testing with Servo-Hydraulic Load Frames. Exp. Mech. 2014, 54, 775–789. [Google Scholar] [CrossRef]
- Xia, Y.; Zhu, J.; Wang, K.; Zhou, Q. Design and Verification of a Strain Gauge Based Load Sensor for Medium-Speed Dynamic Tests with a Hydraulic Test Machine. Int. J. Impact Eng. 2016, 88, 139–152. [Google Scholar] [CrossRef]
- Allemang, R.; Avitabile, P. Handbook of Experimental Structural Dynamics; Springer Nature: Berlin/Heidelberg, Germany, 2022. [Google Scholar]
- Analog Devices. Low Power, 18 MHz Variable Gain Amplifier. AD8338 Datasheet. 2022. Available online: https://www.analog.com/en/products/ad8338.html (accessed on 1 January 2024).
- Baumer. Distance Sensors. OM70-P0140.HH0130.VI Datasheet. 2021. Available online: https://www.baumer.com/us/en/product-overview/distance-measurement/laser-distance-sensors/high-performance/high-measuring-accuracy/om70-p0140-hh0130-vi/p/36863 (accessed on 1 January 2024).
- Ewins, D.J. Mode of Vibration. In Encyclopedia of Vibration; Braun, S., Ed.; Elsevier: Oxford, UK, 2001; pp. 838–844. [Google Scholar]
- Chui, C.K.; Chen, G. Kalman Filtering, 5th ed.; Springer International Publishing: Cham, Switzerland, 2017. [Google Scholar]
- Anderson, B.D.O.; Moore, J.B. Optimal Control: Linear Quadratic Methods; Prentice-Hall Information and System Sciences Series; Prentice-Hall International: Englewood Cliffs, NJ, USA, 1989. [Google Scholar]
- Abut, T. Optimal LQR Controller Methods for Double Inverted Pendulum System on a Cart. Dicle Univ. J. Eng. 2023, 14, 247–255. [Google Scholar] [CrossRef]
- Abut, T.; Salkim, E. Control of Quarter-Car Active Suspension System Based on Optimized Fuzzy Linear Quadratic Regulator Control Method. Appl. Sci. 2023, 13, 8802. [Google Scholar] [CrossRef]
- Zhao, T.; Li, W. LQR-Based Attitude Controllers Design for a 3-DOF Helicopter System with Comparative Experimental Tests. Int. J. Dyn. Control. 2023, 13, 8802. [Google Scholar] [CrossRef]
- Franklin, G.F.; Powell, J.D.; Emami-Naeini, A. Feedback Control of Dynamic Systems, 8th ed.; Pearson Education: London, UK, 2019; Volume 33. [Google Scholar]
- Erickson, R.W.; Maksimovic, D. Fundamentals of Power Electronics, 3rd ed.; Springer Science & Business Media: Cham, Switzerland, 2007. [Google Scholar]
- Xing, L.; Feng, F.; Sun, J. Optimal Damping of EMI Filter Input Impedance. IEEE Trans. Ind. Appl. 2011, 47, 1432–1440. [Google Scholar] [CrossRef]
- Forsentek. 3 Axis Load Cell 0-100 N Multi-Axis Force Sensor. FNZ Datasheet. Available online: http://www.forsentek.com/prodetail_279.html (accessed on 1 January 2024).
- Analog Devices. 0.25 ppm Noise, Low Drift Precision References. LTC6655/LTC6655LN Datasheet. 2021. Available online: https://www.analog.com/en/products/ltc6655.html (accessed on 1 January 2024).
- Texas Instruments. ADSxxx3 Dual, 1-MSPS, 16-, 14-, and 12-Bit, 4×2 or 2×2 Channel, Simultaneous Sampling Analog-to-Digital Converter. ADS8363 Datasheet. 2017. Available online: https://www.ti.com/product/ADS8363 (accessed on 1 January 2024).
- Petruska, A.J.; Nelson, B.J. Minimum Bounds on the Number of Electromagnets Required for Remote Magnetic Manipulation. IEEE Trans. Robot. 2015, 31, 714–722. [Google Scholar] [CrossRef]
- Mirić, S.M.; Giuffrida, R.V.; Bortis, D.; Kolar, J.W. Enhanced Complex Space Vector Modeling and Control System Design of Multiphase Magnetically Levitated Rotary-Linear Machines. IEEE J. Emerg. Sel. Top. Power Electron. 2020, 8, 1833–1849. [Google Scholar] [CrossRef]
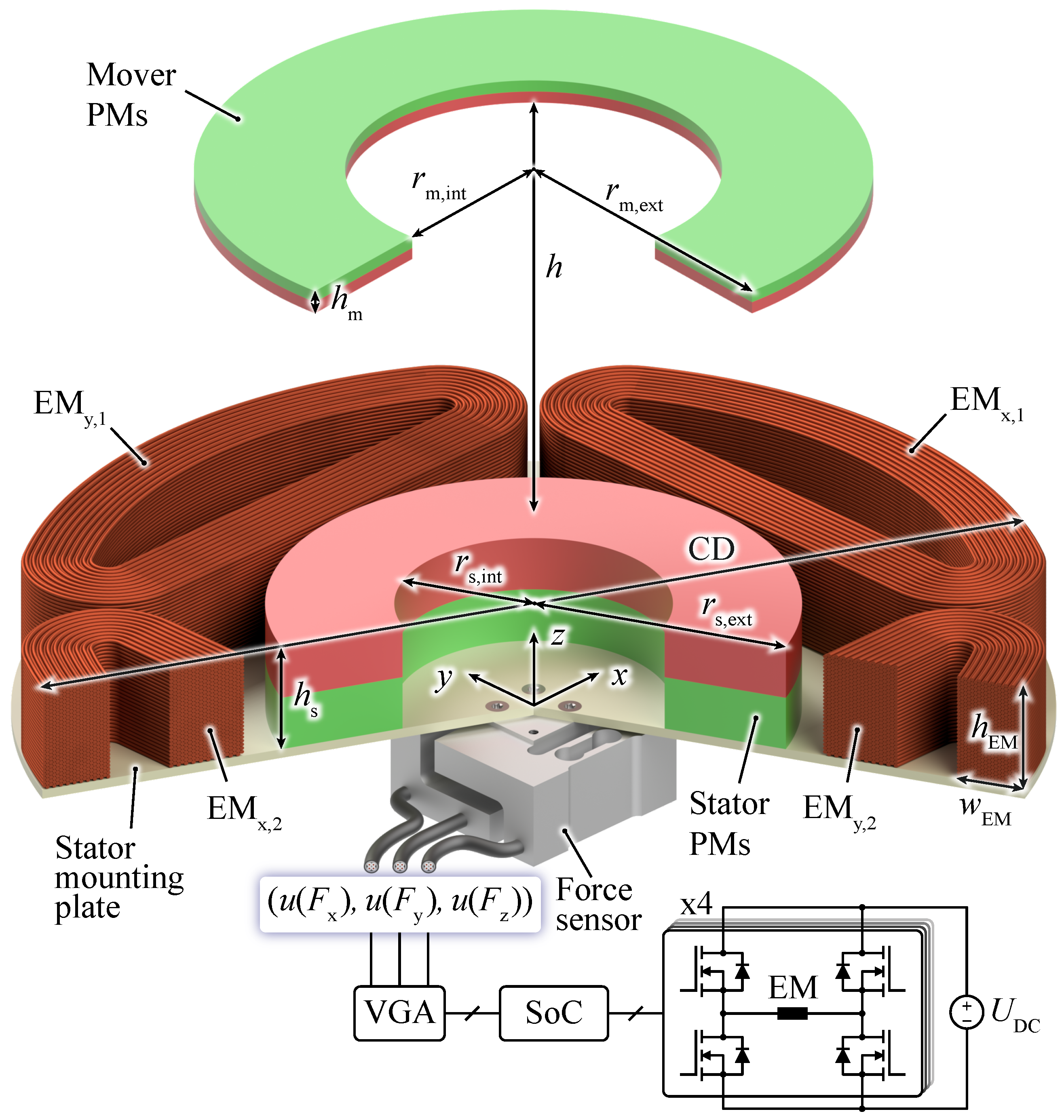
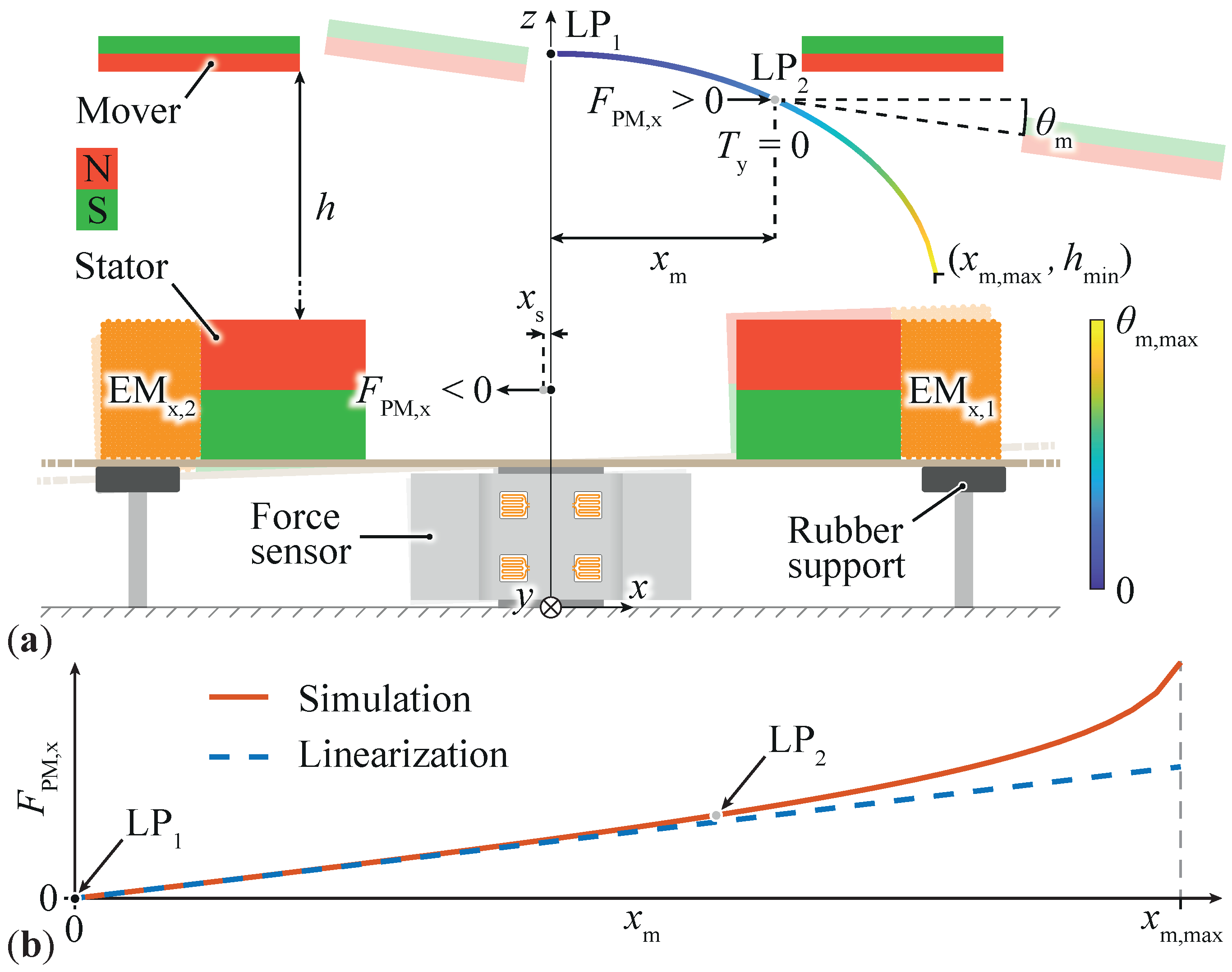
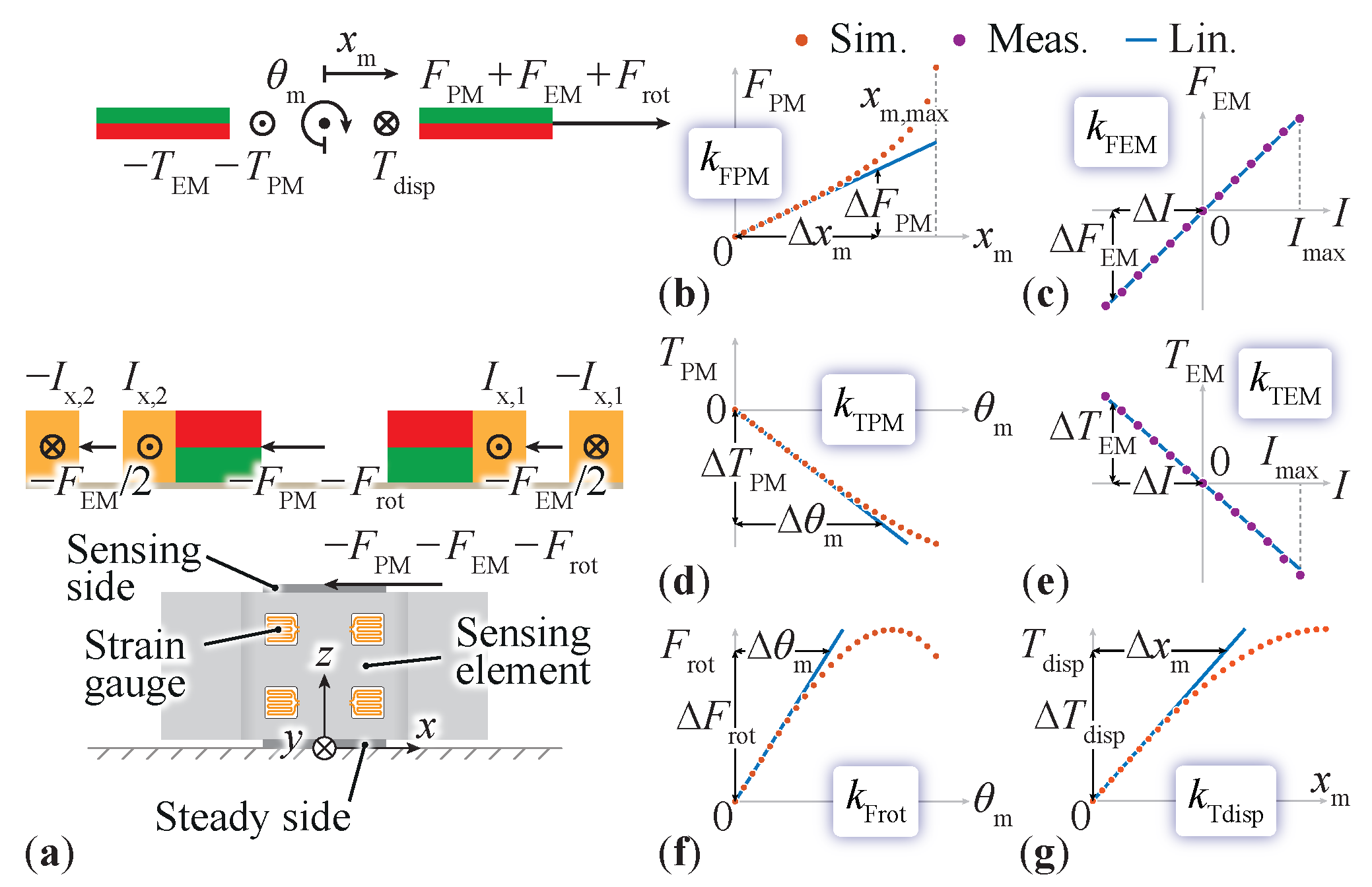
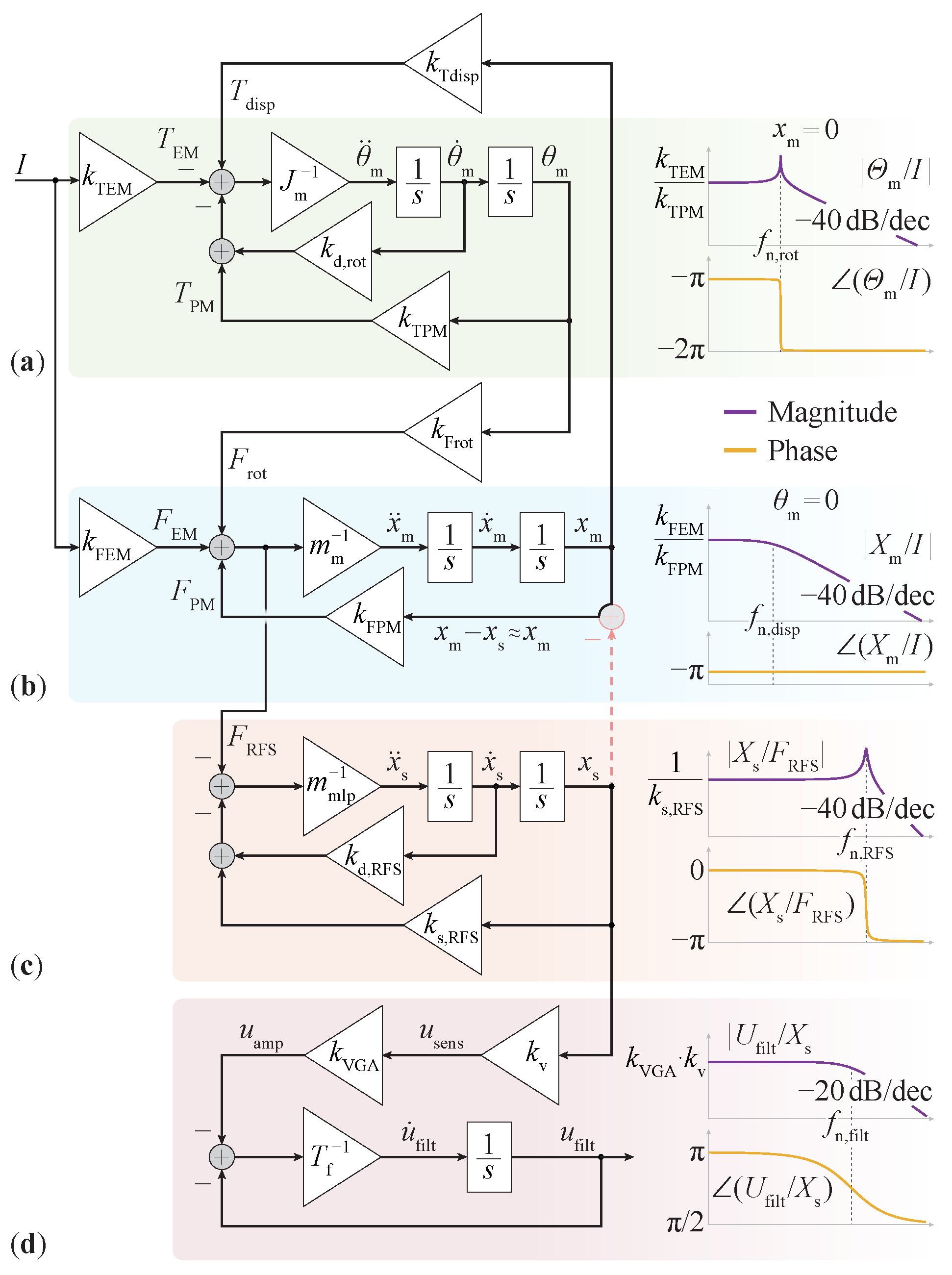

| Mover PM external radius | mm | |
| Mover PM internal radius | mm | |
| Mover height | 5 mm | |
| Stator PM external radius | 50 mm | |
| Stator PM internal radius | mm | |
| Stator height | 20 mm | |
| Mover weight | kg | |
| Stator weight | kg | |
| Mover moment of inertia | gm2 | |
| Total MLP weight | kg | |
| Levitation height | h | 104 mm |
| Characteristic dimension | CD | 207 mm |
| Radial stiffness | N/m | |
| Displacement torque const. | Nm/m | |
| Rotational stiffness | mNm/ | |
| Rotational damping | 2 Nms/ | |
| Rotational force const. | mN/ | |
| EMs force const. | 65 mN/A | |
| EMs torque const. | mNm/A | |
| Force sensor damping | Ns/m | |
| Force sensor stiffness | 694 kN/m | |
| Force sensor el. conv. const. | V/m | |
| Force sensor amplifier gain | 10 V/mV |
| Inverter | |||
| 40 | 100 | ||
| filter | |||
| 22 | 1 | ||
| 22 | 88 | ||
| n | 4 | ||
| Electromagnet | |||
| 87 | 85 | ||
| Resonant freq. | 125 | ||
| Outer Current Controller | |||
| Position controller (50) | |||
| As/ | A/ | ||
| As/m | kA/m | ||
| H | A/m | ||
| Inner current controller | |||
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Bonetti, R.; Beglinger, L.; Mirić, S.; Bortis, D.; Kolar, J.W. Reaction Force-Based Position Sensing for Magnetic Levitation Platform with Exceptionally Large Hovering Distance. Actuators 2024, 13, 114. https://doi.org/10.3390/act13030114
Bonetti R, Beglinger L, Mirić S, Bortis D, Kolar JW. Reaction Force-Based Position Sensing for Magnetic Levitation Platform with Exceptionally Large Hovering Distance. Actuators. 2024; 13(3):114. https://doi.org/10.3390/act13030114
Chicago/Turabian StyleBonetti, Reto, Lars Beglinger, Spasoje Mirić, Dominik Bortis, and Johann W. Kolar. 2024. "Reaction Force-Based Position Sensing for Magnetic Levitation Platform with Exceptionally Large Hovering Distance" Actuators 13, no. 3: 114. https://doi.org/10.3390/act13030114
APA StyleBonetti, R., Beglinger, L., Mirić, S., Bortis, D., & Kolar, J. W. (2024). Reaction Force-Based Position Sensing for Magnetic Levitation Platform with Exceptionally Large Hovering Distance. Actuators, 13(3), 114. https://doi.org/10.3390/act13030114







