Internal Model Principle-Based Extended State Observer for the Uncertain Systems with Nonconstant Disturbances
Abstract
1. Introduction
- Introduction of a design concept and methodology for an internal model principle-based ESO tailored to different types of disturbances, providing a novel approach to Active Disturbance Rejection Control (ADRC) that effectively suppresses non-constant disturbances.
- Further extension and improvement of the ESO by proposing a new disturbance-tracking paradigm.
- Theoretical analysis and simulation results indicate that the internal model principle-based extended state observer (IMP-ESO-ADRC) possesses several advantages, including high tracking accuracy, strong disturbance rejection capability, and good stability. This controller exhibits robustness in the face of disturbances and showcases a remarkable ability to mitigate their effects. The remaining sections of this paper are organized as follows:
2. Expansion State Observer
3. Controller Design and Stability Analysis
3.1. Controller Design
3.2. Stability Analysis
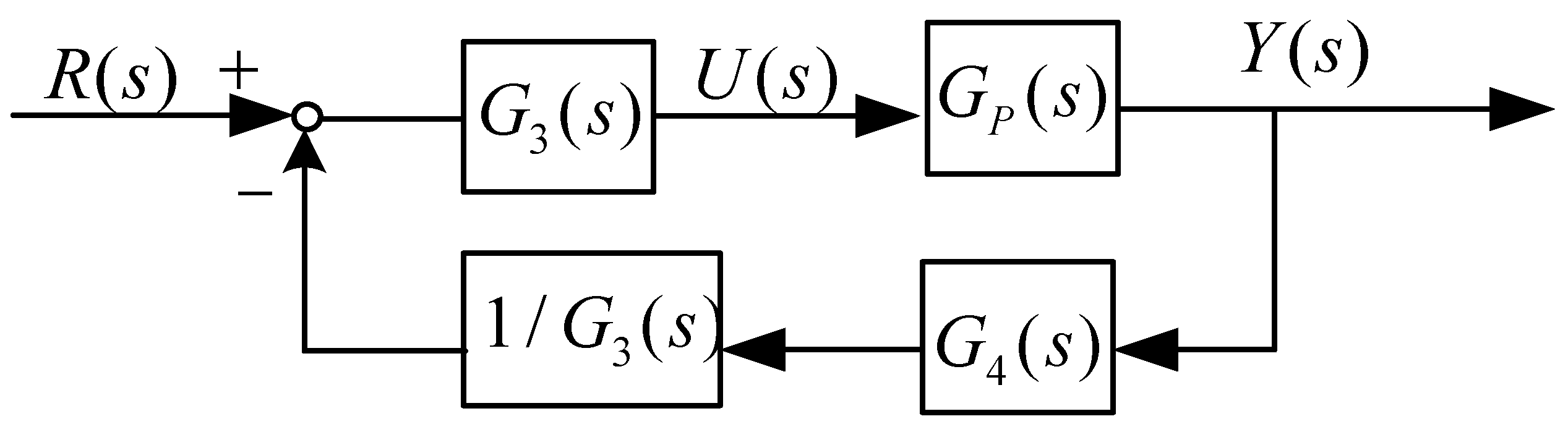
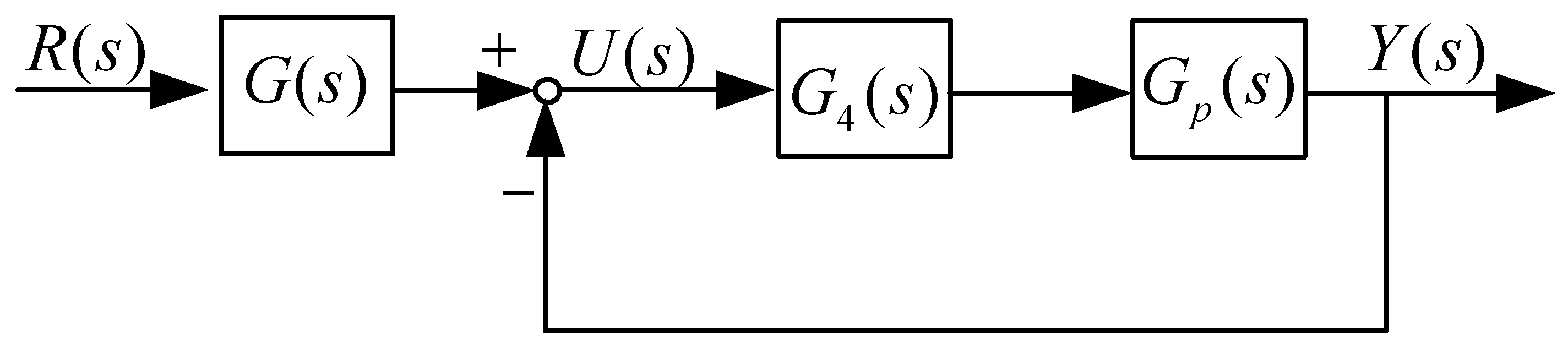
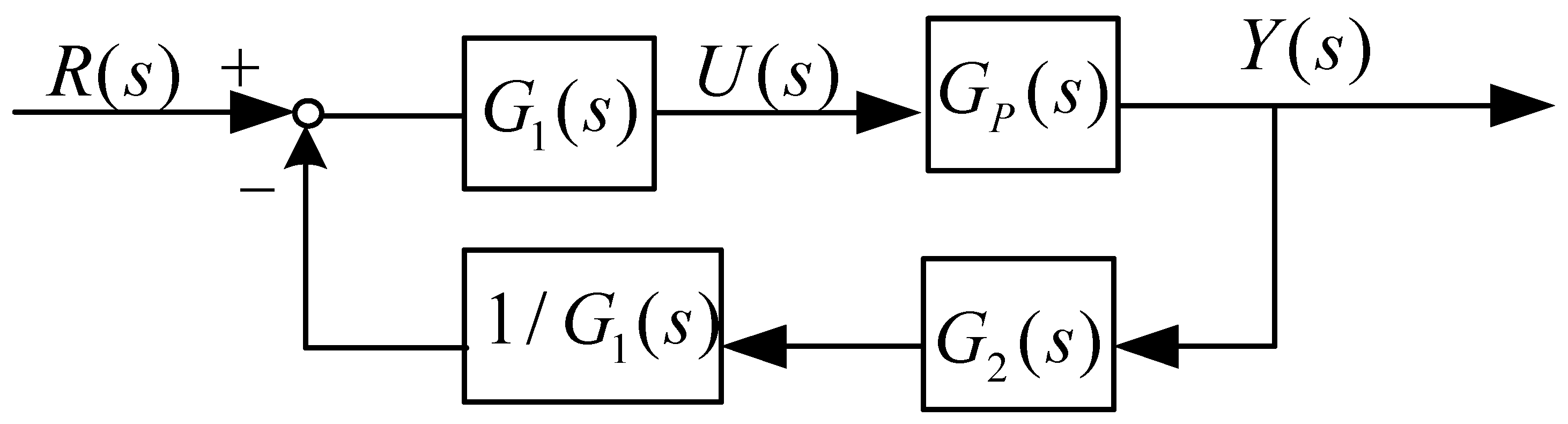
4. IMP-ESO Analysis in the Context of ADRC Closed-Loop Control System
4.1. IMP-ESO-ADRC Design
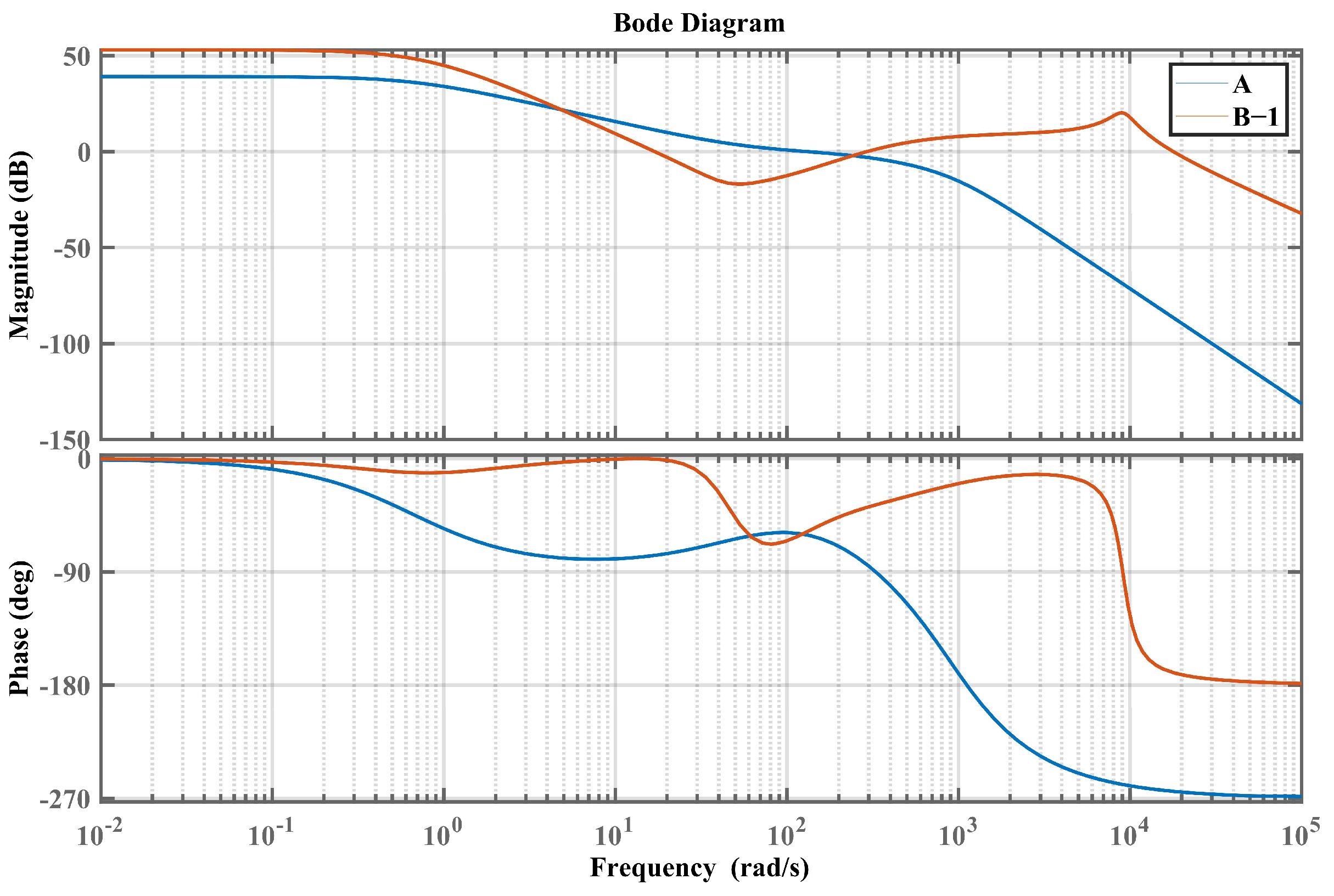
4.2. Frequency Domain Analysis
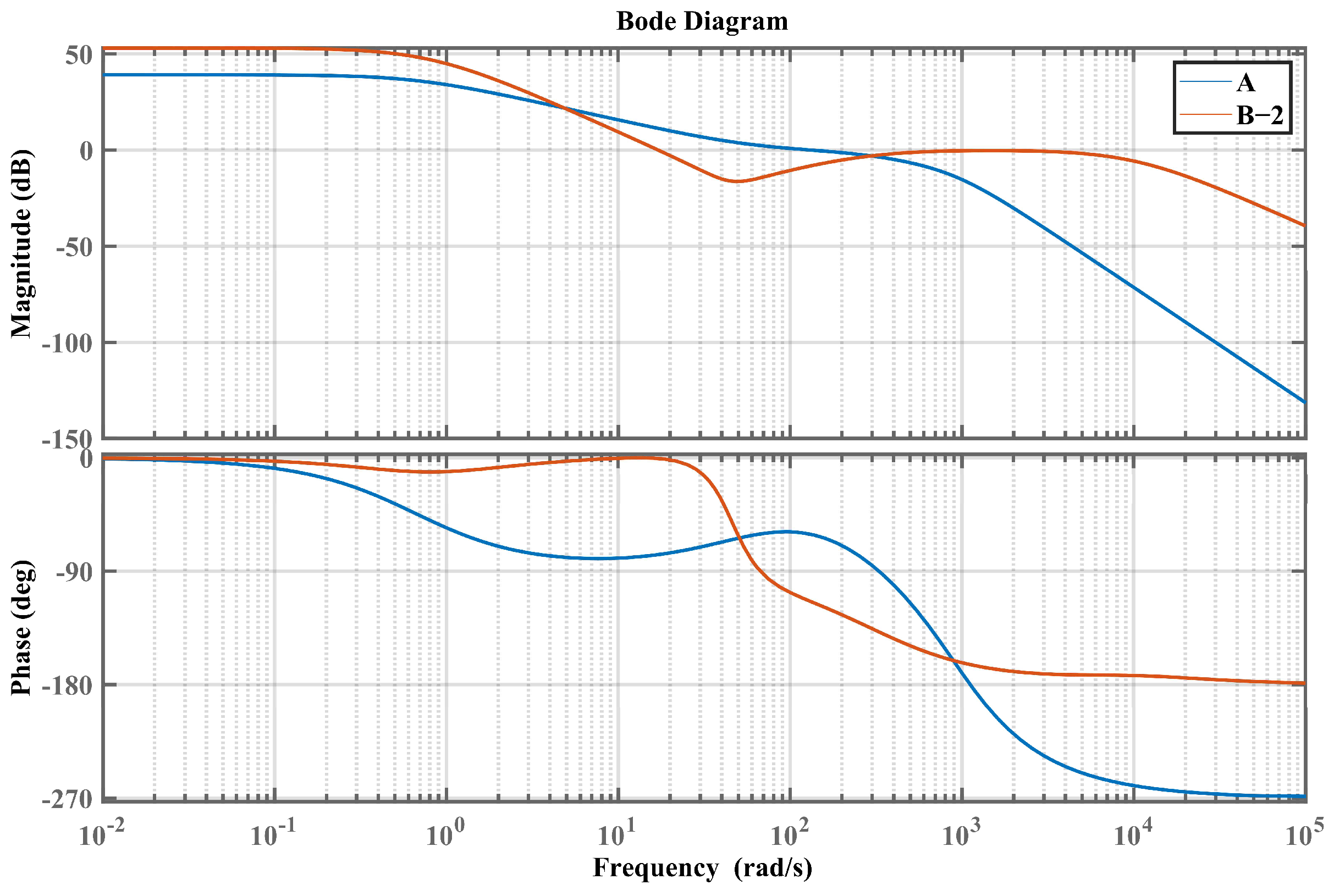
- Comparison between A and B-1;
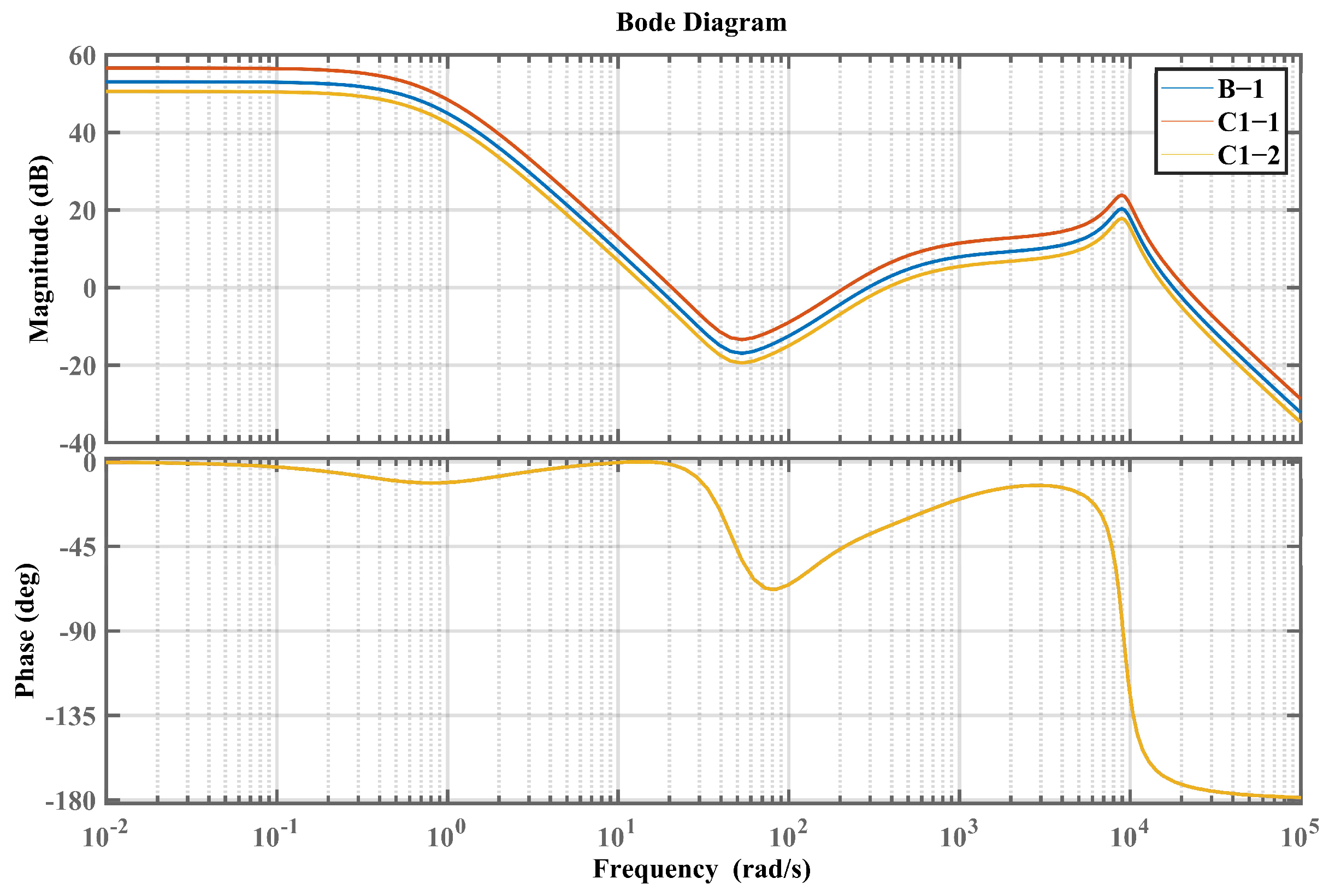
- Comparison between B-1 and C1-1, C1-2;
- Comparison between B-1 and C2-1, C2-2;
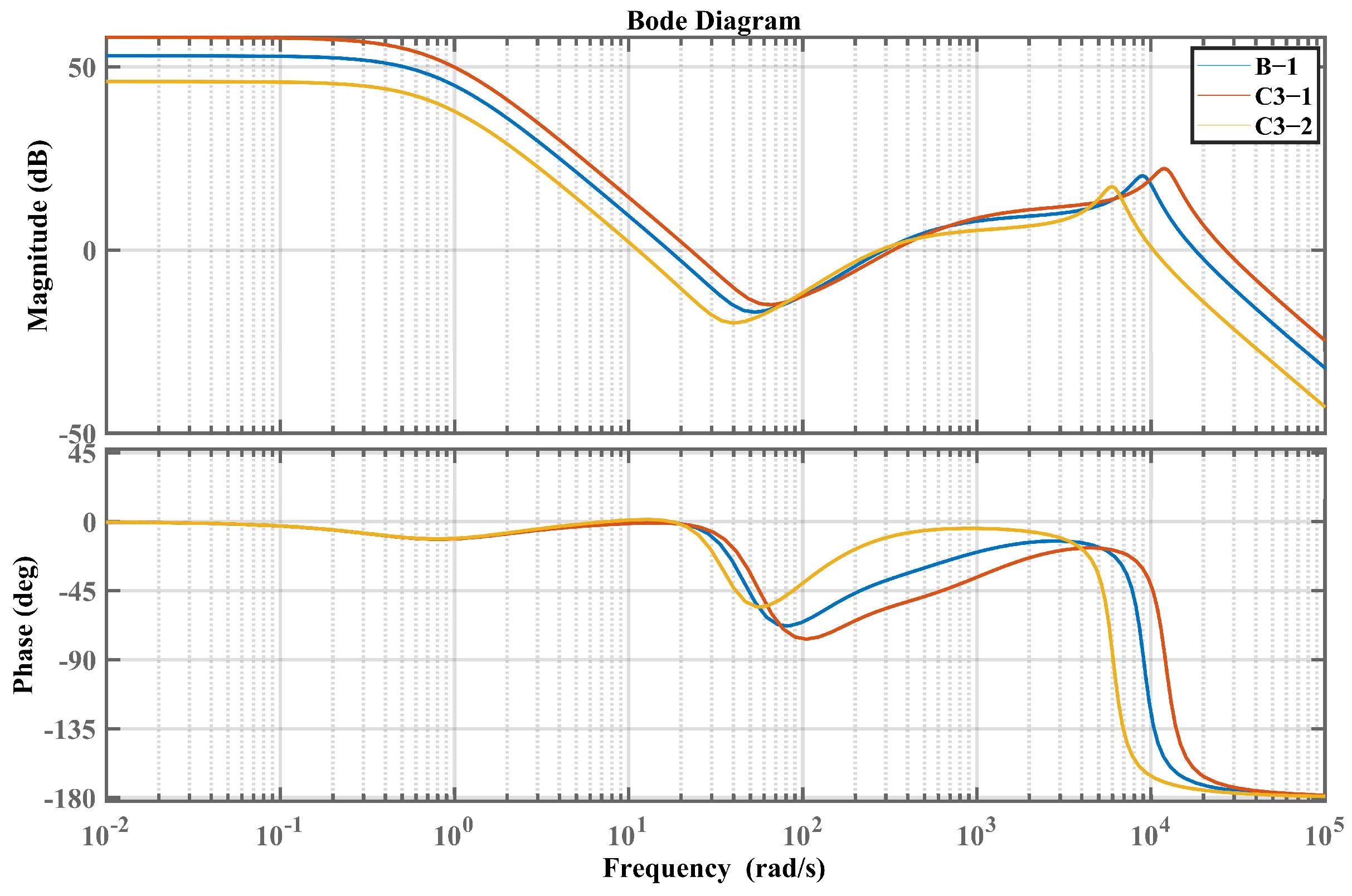
- Comparison between B-1 and C3-1, C3-2.
- Comparison between A and B-2;
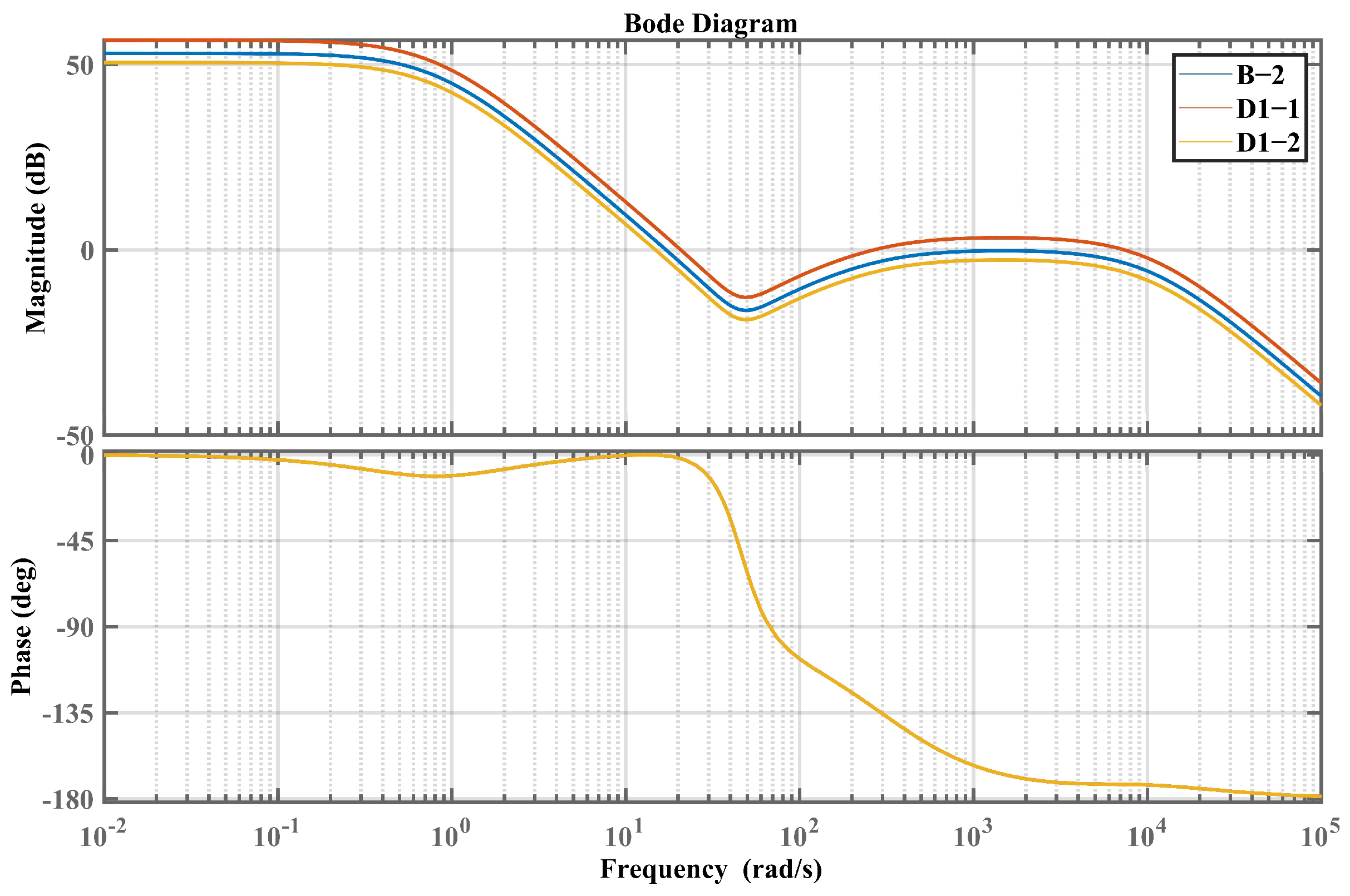
- Comparison between B-2 and D1-1, D1-2;
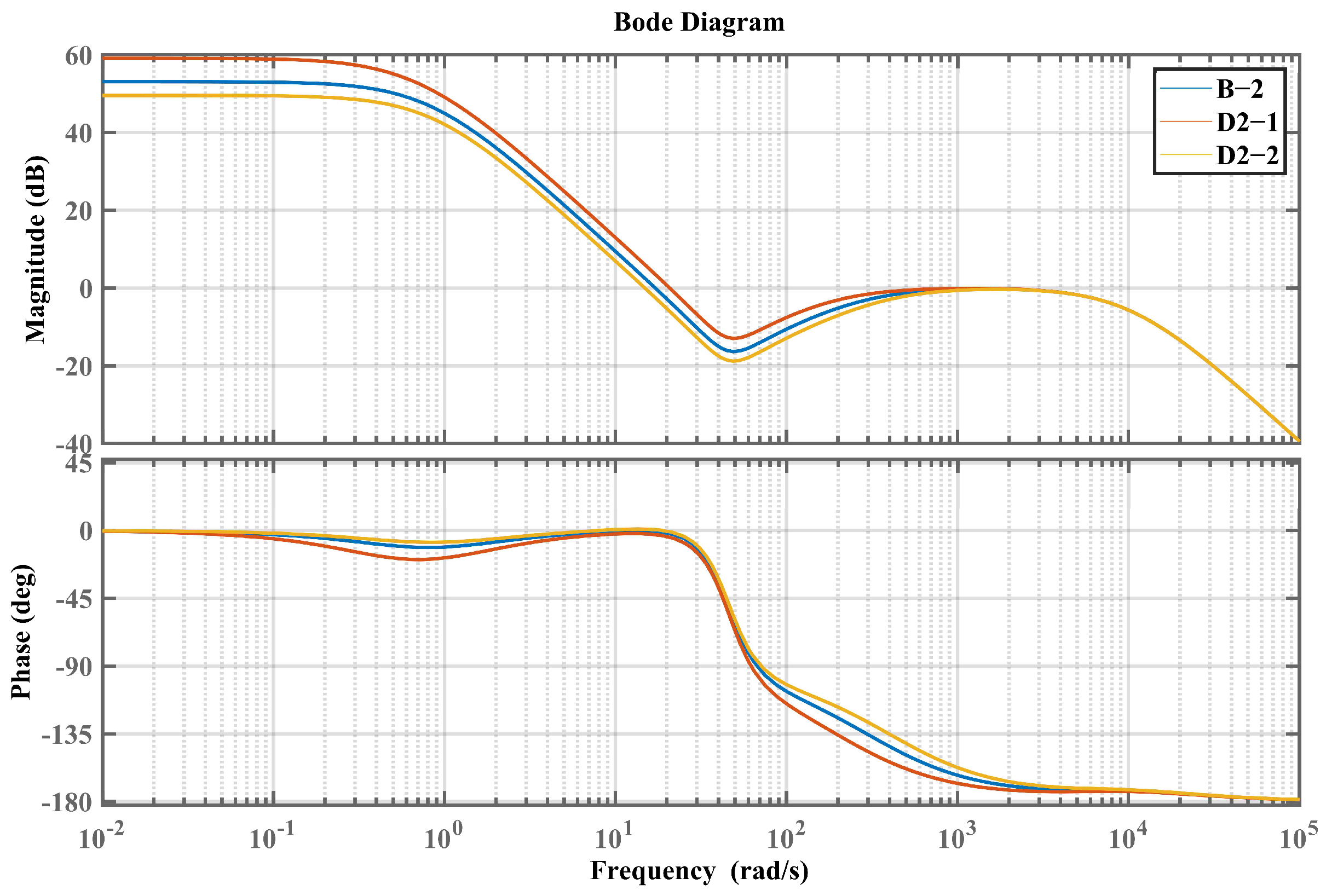
- Comparison between B-2 and D2-1, D2-2;
- Comparison between B-2 and D3-1, D3-2.
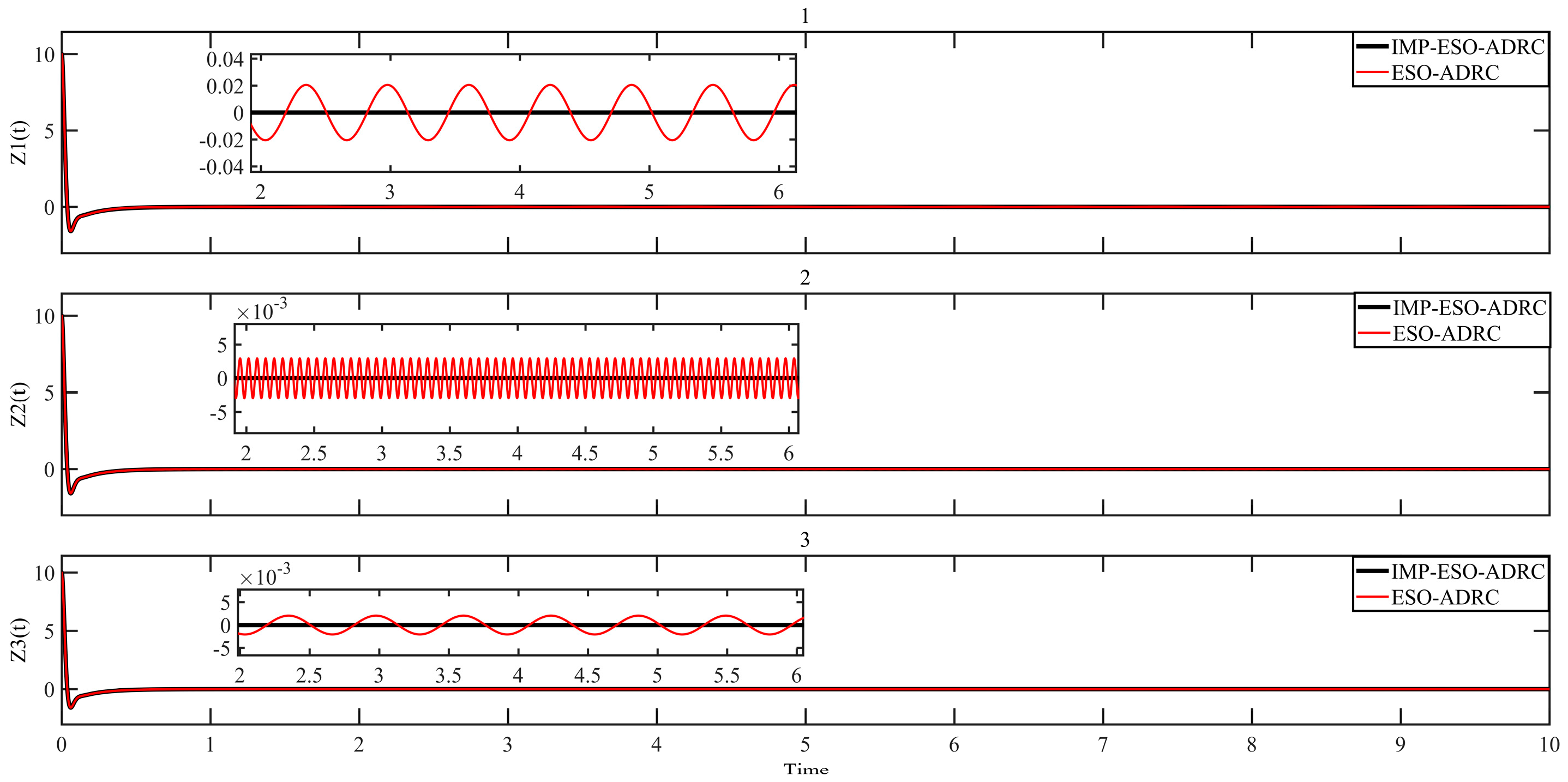
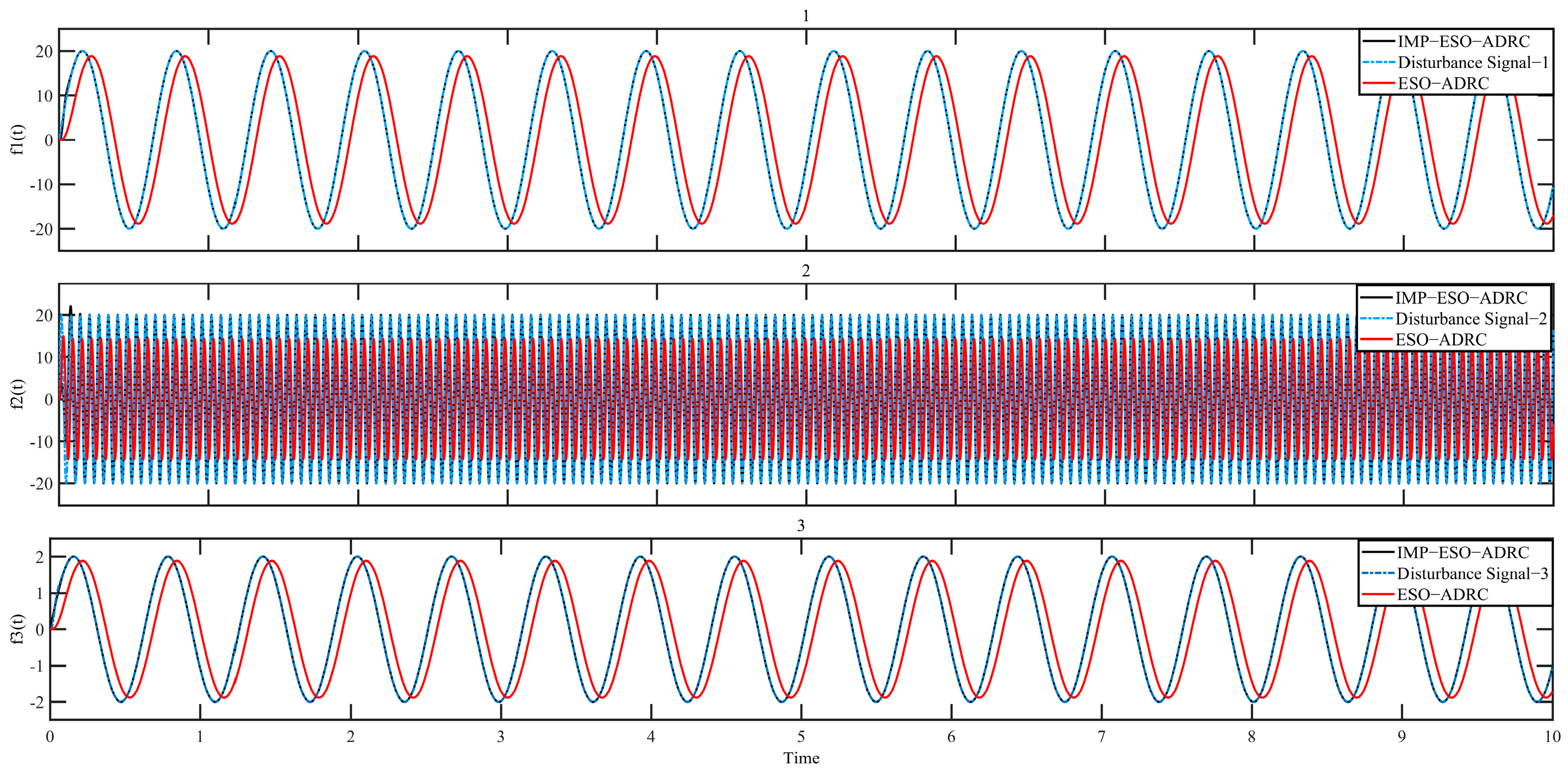
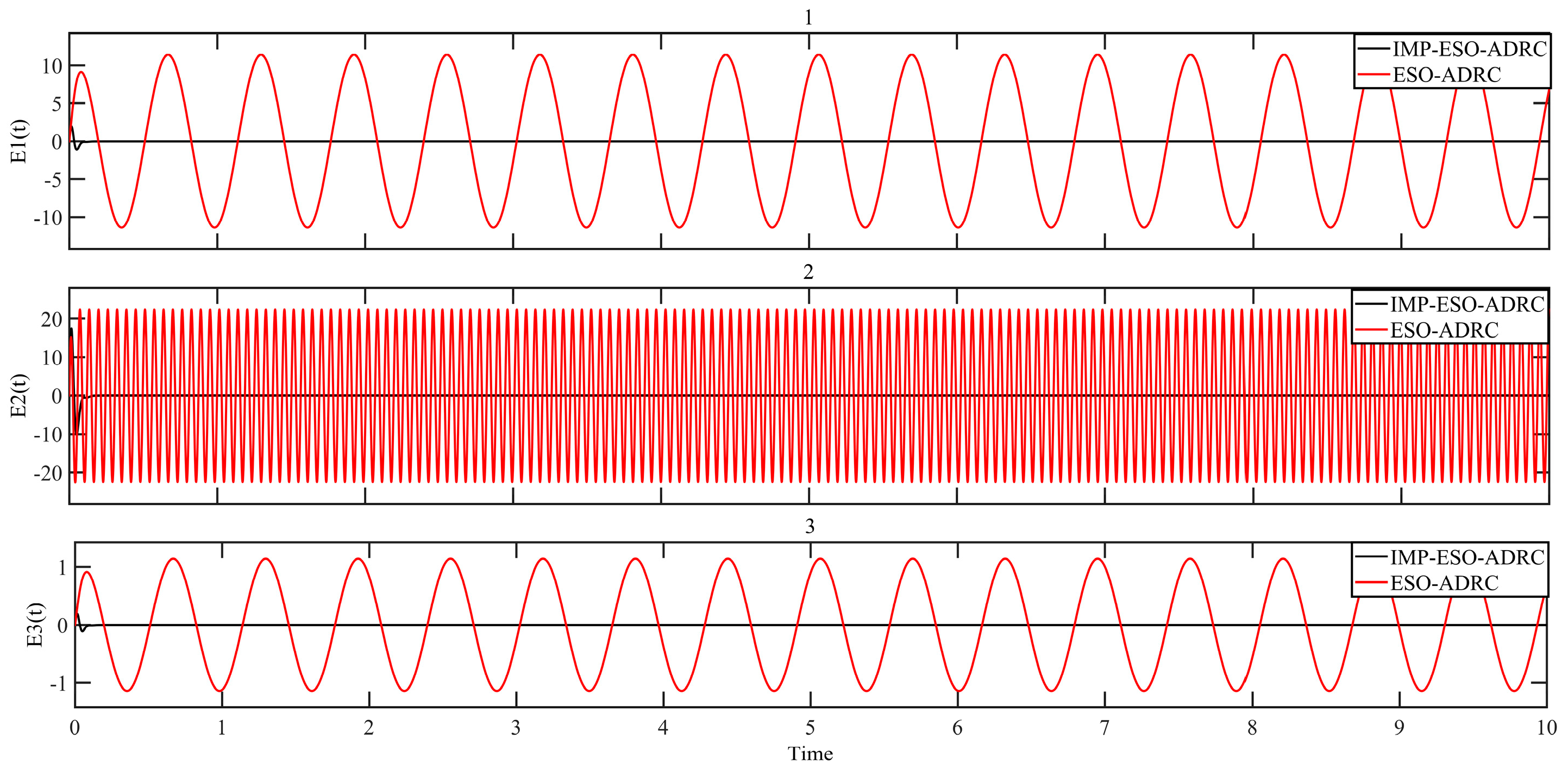
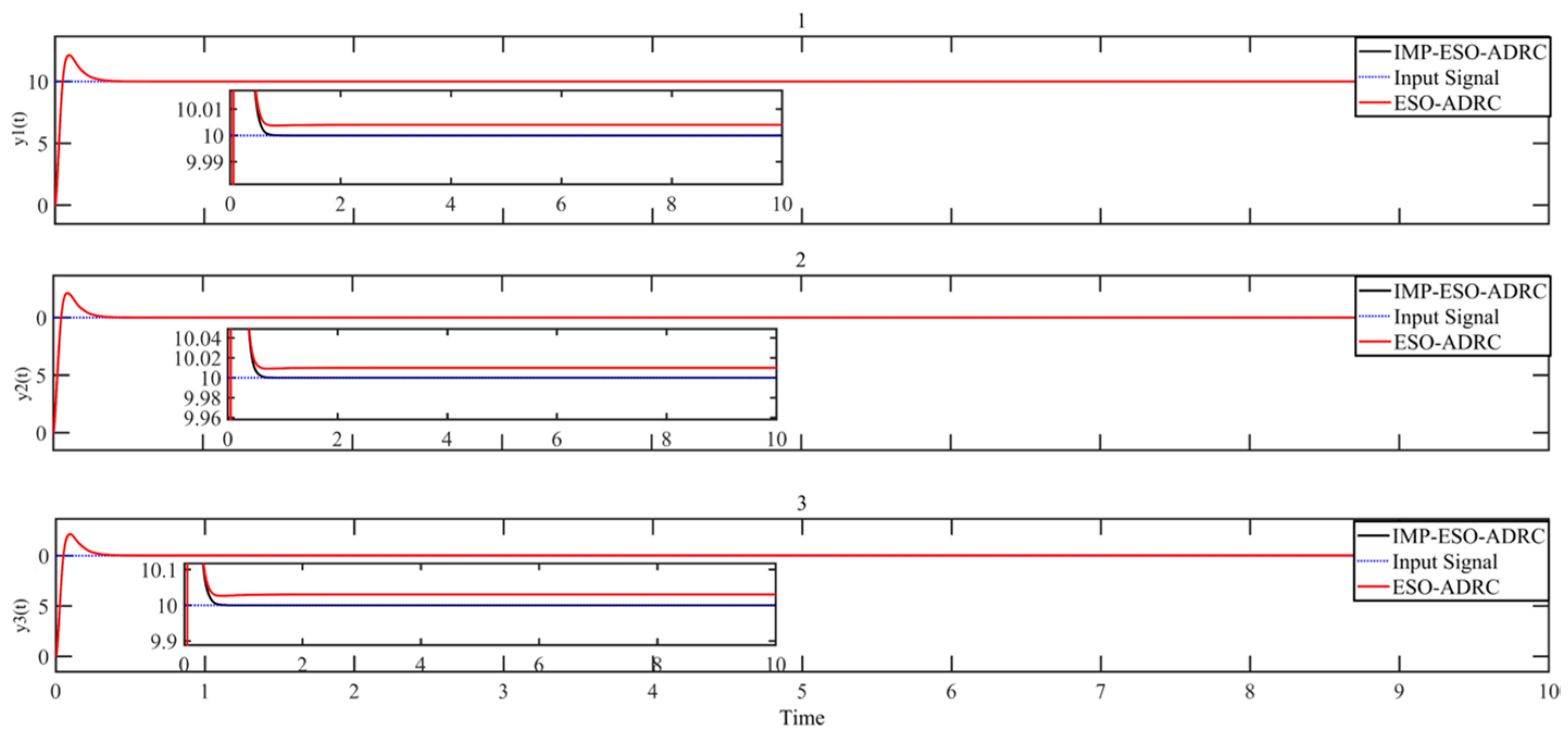
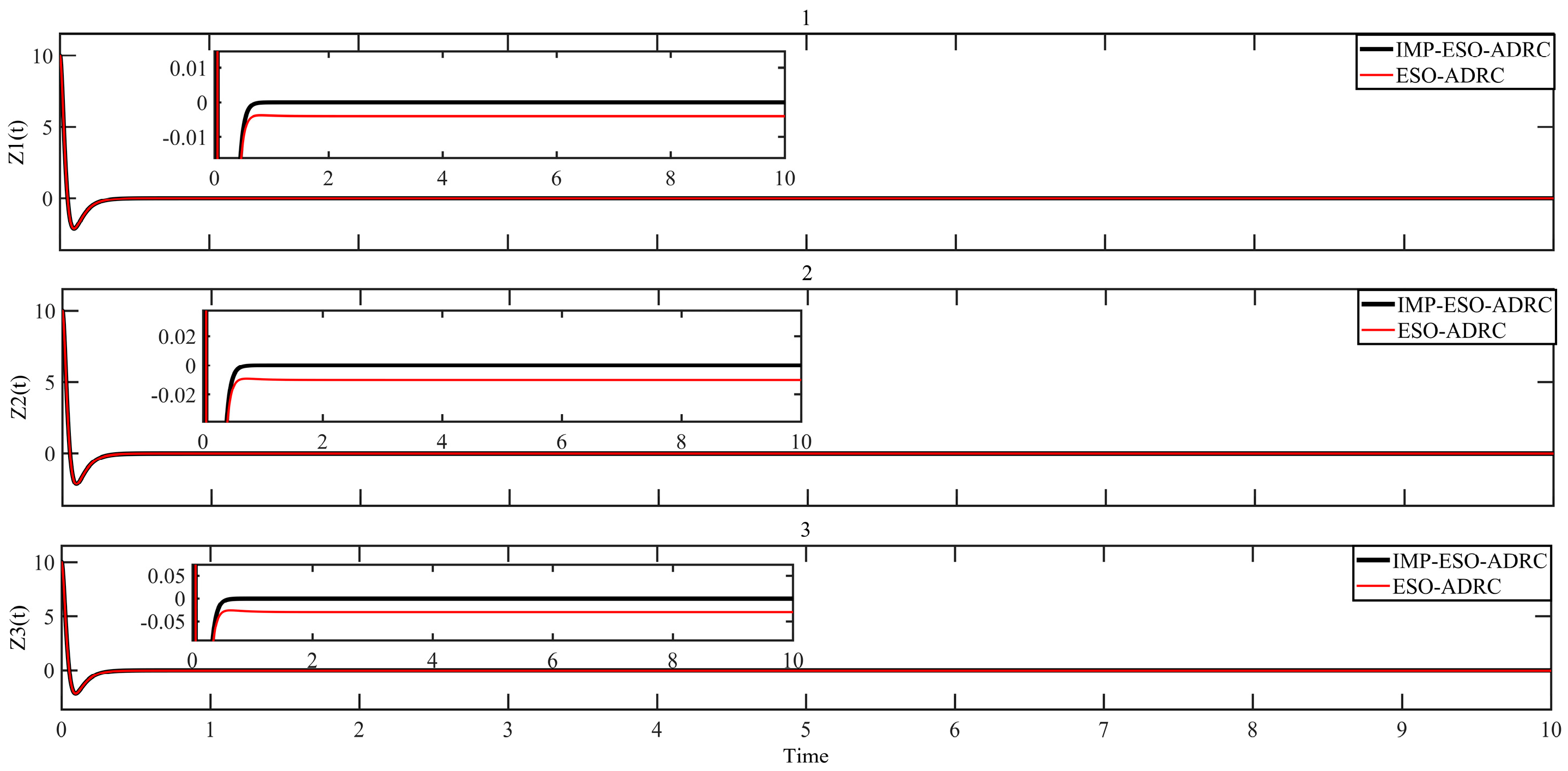
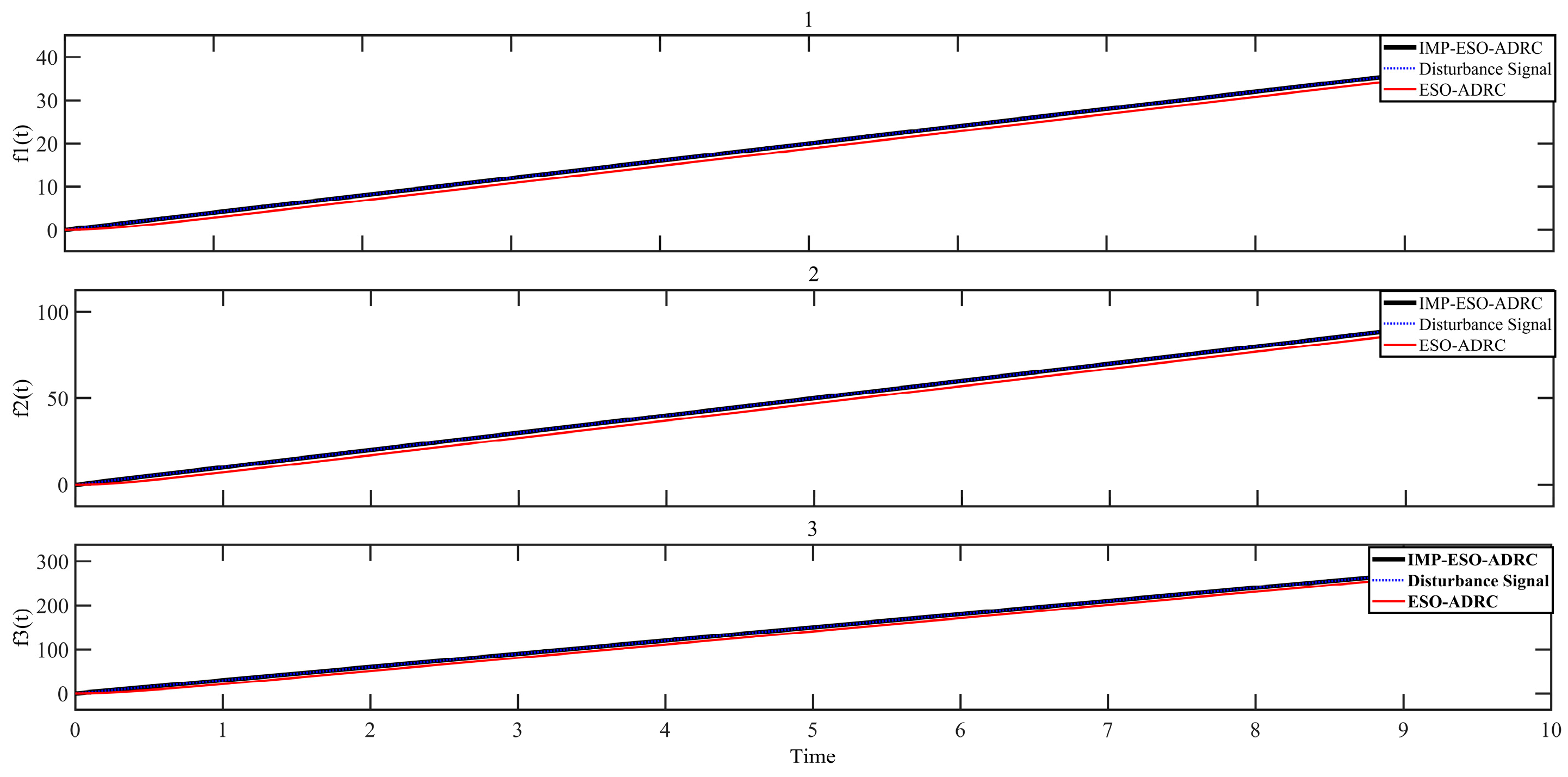
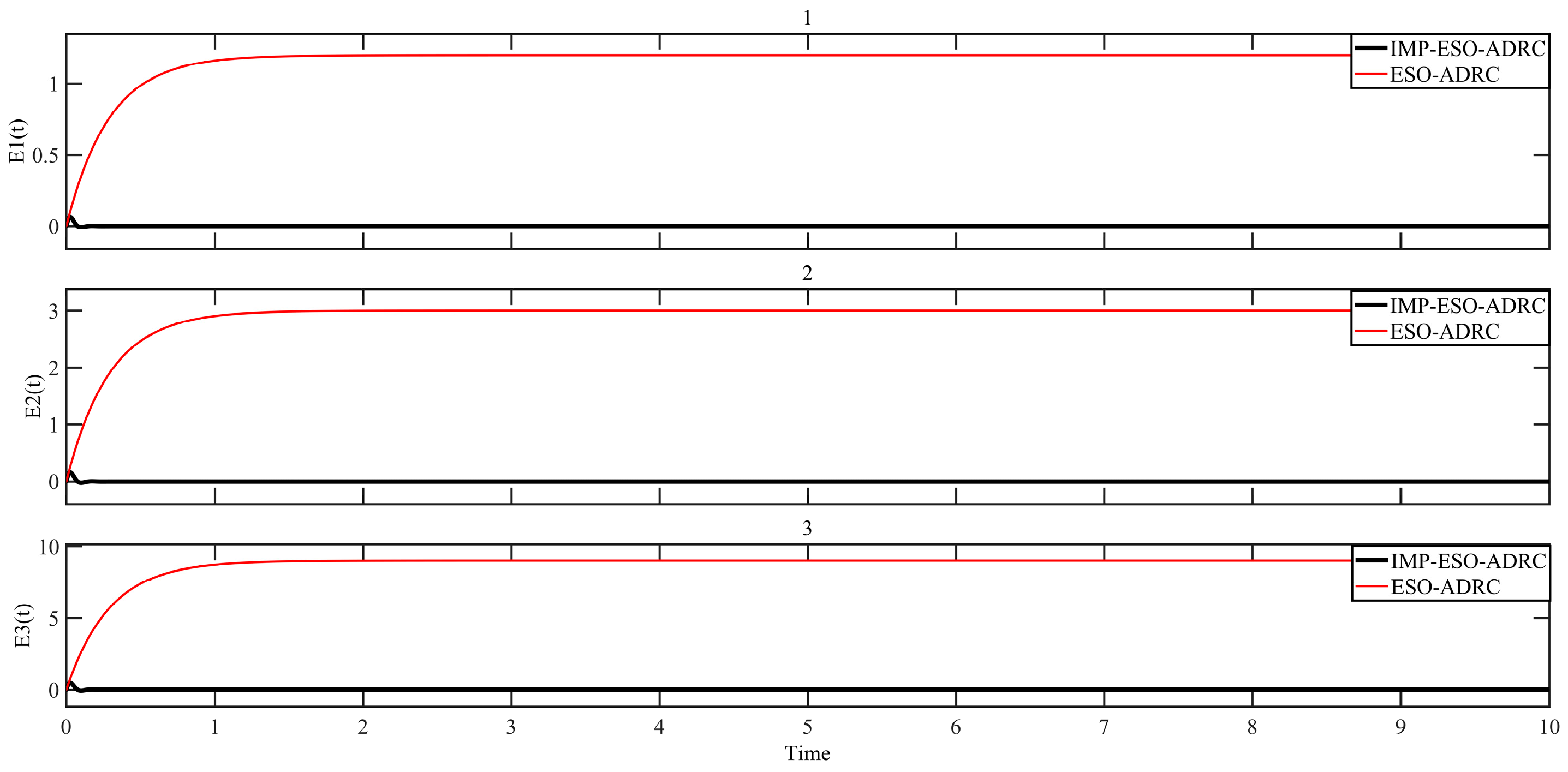
5. Simulation Test Results and Analysis
5.1. Simulation with Harmonic Disturbance
5.2. Simulation with Ramp Disturbance
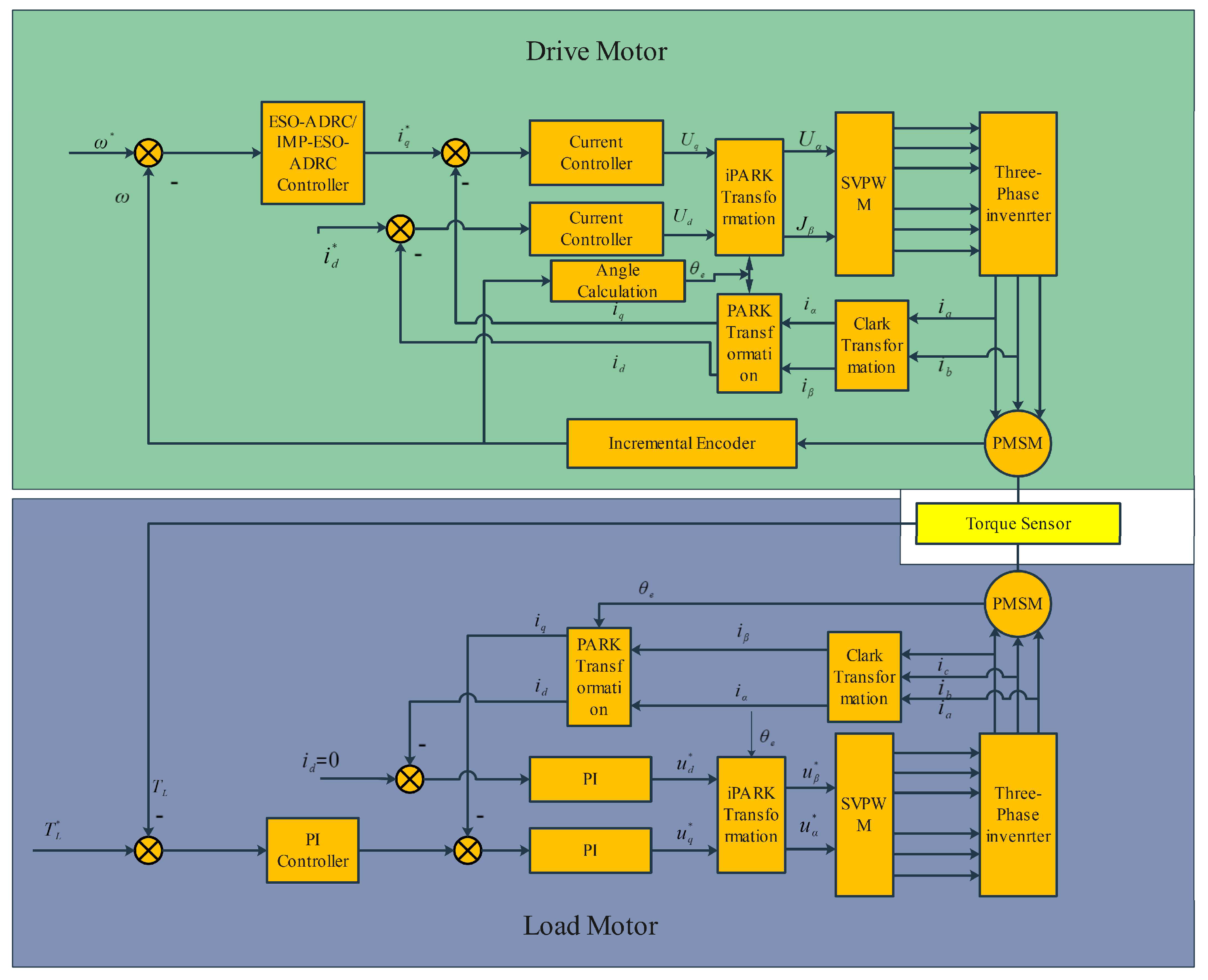
5.3. Experimental Results
6. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Han, J. From PID to active disturbance rejection control. IEEE Trans. Ind. Electron. 2009, 56, 900–906. [Google Scholar] [CrossRef]
- Zolotas, A. Disturbance Observer-Based Control: Methods and Applications [Bookshelf]. IEEE Control Syst. Mag. 2015, 35, 55–57. [Google Scholar] [CrossRef]
- Yin, X.; Shi, Y.; She, J.; Wang, H. Equivalent Input Disturbance-Based Control: Analysis, Development, and Applications. IEEE Trans. Cybern. 2023. [Google Scholar] [CrossRef] [PubMed]
- Hao, Z.; Tian, Y.; Yang, Y.; Gong, Y.; Hao, Z.; Zhang, J. Sensorless Control Based on the Uncertainty and Disturbance Estimator for IPMSMs with Periodic Loads. IEEE Trans. Ind. Electron. 2023, 70, 5085–5093. [Google Scholar] [CrossRef]
- Hezzi, A.; Elghali, S.B.; Bensalem, Y. ADRC Based Robust and Resilient Control of a 5 Phase PMSM Driven Electric Vehicle. Machines 2020, 8, 17. [Google Scholar] [CrossRef]
- Sira-Ramirez, H.; Linares-Flores, J.; Garcia-Rodriguez, C.; Contreras-Ordaz, M.A. On the Control of the Permanent Magnet Synchronous Motor: An Active Disturbance Rejection Control Approach. IEEE Trans. Control Syst. Technol. 2014, 22, 2056–2063. [Google Scholar] [CrossRef]
- Zurita-Bustamante, E.W.; Sira-Ramirez, H.; Linares-Flores, J. On the sensorless rotor position control of the permanent magnet synchronous motor: An active disturbance rejection approach. In Proceedings of the 13th International Conference on Power Electronics (CIEP), Guanajuato, Mexico, 20–23 June 2016; Volume 12, pp. 12–17. [Google Scholar]
- Du, B.; Wu, S.; Han, S.; Cui, S. Application of Linear Active Disturbance Rejection Controller for Sensorless Control of Internal Permanent-Magnet Synchronous Motor. IEEE Trans. Ind. Electron. 2016, 63, 3019–3027. [Google Scholar] [CrossRef]
- Zhiwang, C.; Zizhen, Z.; Jie, C.Y. Fal function improvement of ADRC and its application in quadrotor aircraft attitude control. Control Decis. 2018, 33, 1901–1907. [Google Scholar]
- Han, J. Active disturbance rejection controller and its applications. Control Decis. 1998, 13, 19–23. (In Chinese) [Google Scholar]
- Gao, Z. Scaling and bandwidth-parameterization based controller tuning. In Proceedings of the American Control Conference, Minneapolis, MN, USA, 14–16 June 2006; Institute of Electrical and Electronics Engineers (IEEE): New York, NY, USA, 2006; pp. 4989–4996. [Google Scholar]
- Guo, B.; Bacha, S.; Alamir, M.; Mohamed, A.; Boudinet, C. LADRC applied to variable speed micro-hydro plants: Experimental validation. Control Eng. Pract. 2019, 85, 290–298. [Google Scholar] [CrossRef]
- Guo, B.; Bacha, S.; Alamir, M. A review on ADRC based PMSM control designs. In Proceedings of the 43rd Annual Conference of the IEEE Industrial Electronics Society (IECON), Beijing, China, 29 October–1 November 2017; pp. 1747–1753. [Google Scholar]
- Guo, B.; Bacha, S.; Alamir, M.; Boudinet, C.; Mesnage, H. An enhanced phase-locked loop with extended state observer. In Proceedings of the 20th International Symposium on Power Electronics (Ee), Novi Sad, Serbia, 23–26 October 2019; pp. 1–6. [Google Scholar]
- Fareh, R.; Khadraoui, S.; Abdallah, M.Y.; Baziyad, M.; Bettayeb, M. Active Disturbance Rejection Controllers for robotic systems: A review. Mechatronics 2021, 80, 102671. [Google Scholar] [CrossRef]
- Li, B.; Zhu, L. An Improved Fractional-Order Active Disturbance Rejection Control: Performance Analysis and Experiment Verification. arXiv 2021, arXiv:2112.05553. [Google Scholar] [CrossRef]
- Mado’nski, R.; Herman, P. Survey on methods of increasing the efficiency of extended state disturbance observers. ISA Trans. 2015, 56, 18–27. [Google Scholar] [CrossRef]
- Yang, X.; Huang, Y. Capabilities of extended state observer for estimating uncertainties. In Proceedings of the 2009 American Control Conference, St. Louis, MO, USA, 10–12 June 2009; IEEE: Piscataway, NJ, USA, 2009. [Google Scholar] [CrossRef]
- Chen, W.-H.; Yang, J.; Guo, L.; Li, S. Disturbance Observer Based Control and Related Methods—An Overview. IEEE Trans. Ind. Electron. 2016, 63, 1083–1095. [Google Scholar] [CrossRef]
- Spurgeon, S.K. Sliding mode observers: A survey. Int. J. Syst. Sci. 2008, 39, 751–764. [Google Scholar] [CrossRef]
- Freidovich, L.B.; Khalil, H.K. Performance recovery of feedback-linearization-based designs. IEEE Trans. Autom. Control 2008, 53, 2324–2334. [Google Scholar] [CrossRef]
- Wu, Y.; Isidori, A.; Lu, R.; Khalil, H. Performance recovery of dynamic feedback–linearization methods for multivariable nonlinear systems. IEEE Trans. Autom. Control 2008, 65, 1365–1380. [Google Scholar] [CrossRef]
- Han, B. Optimization Strategies for Power and Load Control of Large Wind Turbines. Ph.D. Thesis, Hunan University, Changsha, China, 2019. [Google Scholar]
- Mei, Z.; Li, C.; Xu, S. Resonance Frequency Detection Method of Servo Drive System Based on SOGI-FLL. Electr. Mach. Control Appl. 2023, 50, 74–80. [Google Scholar]
- Huang, J. Nonlinear Output Regulation: Theory and Applications; SIAM: Philadelphia, PA, USA, 2004. [Google Scholar]
- Herbst, G. Transfer Function Analysis and Implementation of Active Disturbance Rejection Control. J. Control Theory Appl. 2021, 19, 19–34. [Google Scholar] [CrossRef]








| System | b | |||||
|---|---|---|---|---|---|---|
| A | 300 | 200 | 400 | 400 | 300 | 300 |
| B-1 | 300 | 200 | 400 | 400 | 300 | 300 |
| C1-1 | 300 | 200 | 600 | 400 | 300 | 300 |
| C1-2 | 300 | 200 | 300 | 400 | 300 | 300 |
| C2-1 | 200 | 100 | 400 | 400 | 300 | 300 |
| C2-2 | 400 | 300 | 400 | 400 | 300 | 300 |
| C3-1 | 300 | 200 | 400 | 400 | 400 | 400 |
| C3-2 | 300 | 200 | 400 | 400 | 200 | 200 |
| System | Cutoff Frequency (rad/s) | Crossover Frequency (rad/s) | Gain Margin (dB) | Phase Margin (Degrees) | Delay Margin (s) | Stability |
|---|---|---|---|---|---|---|
| A | 133 | 297 | 17.3 | 119 | 0.0156 | Stable |
| B-1 | 17 | 20.1 | - | 180 | 0.184 | Stable |
| C1-1 | 20.7 | 24.3 | - | 179 | 0.151 | Stable |
| C1-2 | 14.8 | 17.5 | - | 180 | 0.212 | Stable |
| C2-1 | 20.6 | 24.2 | - | 176 | 0.149 | Stable |
| C2-2 | 14.8 | 17.6 | - | 179 | 0.213 | Stable |
| C3-1 | 22.7 | 26.8 | - | 178 | 0.137 | Stable |
| C3-2 | 11.3 | 13.4 | - | 179 | 0.28 | Stable |
| System | b | |||||
|---|---|---|---|---|---|---|
| A | 300 | 200 | 400 | 400 | 300 | 300 |
| B-2 | 300 | 200 | 400 | 400 | 300 | 300 |
| D1-1 | 300 | 200 | 600 | 400 | 300 | 300 |
| D1-2 | 300 | 200 | 300 | 400 | 300 | 300 |
| D2-1 | 200 | 100 | 400 | 400 | 300 | 300 |
| D2-2 | 400 | 300 | 400 | 400 | 300 | 300 |
| D3-1 | 300 | 200 | 400 | 400 | 400 | 400 |
| D3-2 | 300 | 200 | 400 | 400 | 200 | 200 |
| System | Cutoff Frequency (rad/s) | Crossover Frequency (rad/s) | Gain Margin (dB) | Phase Margin (Degrees) | Delay Margin (s) | Stability |
|---|---|---|---|---|---|---|
| A | 133 | 296 | 17.3 | 119 | 0.0156 | Stable |
| B | 17.1 | 20 | - | 179 | 0.183 | Stable |
| D1-1 | 20.8 | 24.5 | - | 178 | 0.15 | Stable |
| D1-2 | 14.8 | 17.6 | - | 180 | 0.211 | Stable |
| D2-1 | 20.7 | 24.4 | - | 176 | 0.148 | Stable |
| D2-2 | 14.8 | 17.6 | - | 179 | 0.213 | Stable |
| D3-1 | 22.8 | 26.7 | - | 177 | 0.136 | Stable |
| D3-2 | 11.4 | 13.5 | - | 179 | 0.278 | Stable |
| Rated voltage | 24 V |
| Rated power | 200 W |
| Rated speed | 1500 RPM (Revolutions per minute) |
| Incremental encoder | 2500 PPR (Pulses per revolution) |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Liu, J.; Zeng, Z.; Shi, S.; Chen, P. Internal Model Principle-Based Extended State Observer for the Uncertain Systems with Nonconstant Disturbances. Actuators 2024, 13, 29. https://doi.org/10.3390/act13010029
Liu J, Zeng Z, Shi S, Chen P. Internal Model Principle-Based Extended State Observer for the Uncertain Systems with Nonconstant Disturbances. Actuators. 2024; 13(1):29. https://doi.org/10.3390/act13010029
Chicago/Turabian StyleLiu, Jiahao, Zhiqiang Zeng, Shangyao Shi, and Pengyun Chen. 2024. "Internal Model Principle-Based Extended State Observer for the Uncertain Systems with Nonconstant Disturbances" Actuators 13, no. 1: 29. https://doi.org/10.3390/act13010029
APA StyleLiu, J., Zeng, Z., Shi, S., & Chen, P. (2024). Internal Model Principle-Based Extended State Observer for the Uncertain Systems with Nonconstant Disturbances. Actuators, 13(1), 29. https://doi.org/10.3390/act13010029






