Performance Evaluation of a High-Torque Permanent Magnet Brake at Operating Temperature Based on Magneto-Thermal Coupling Method
Abstract
1. Introduction
2. Design for High Torque Permanent Magnet Brake
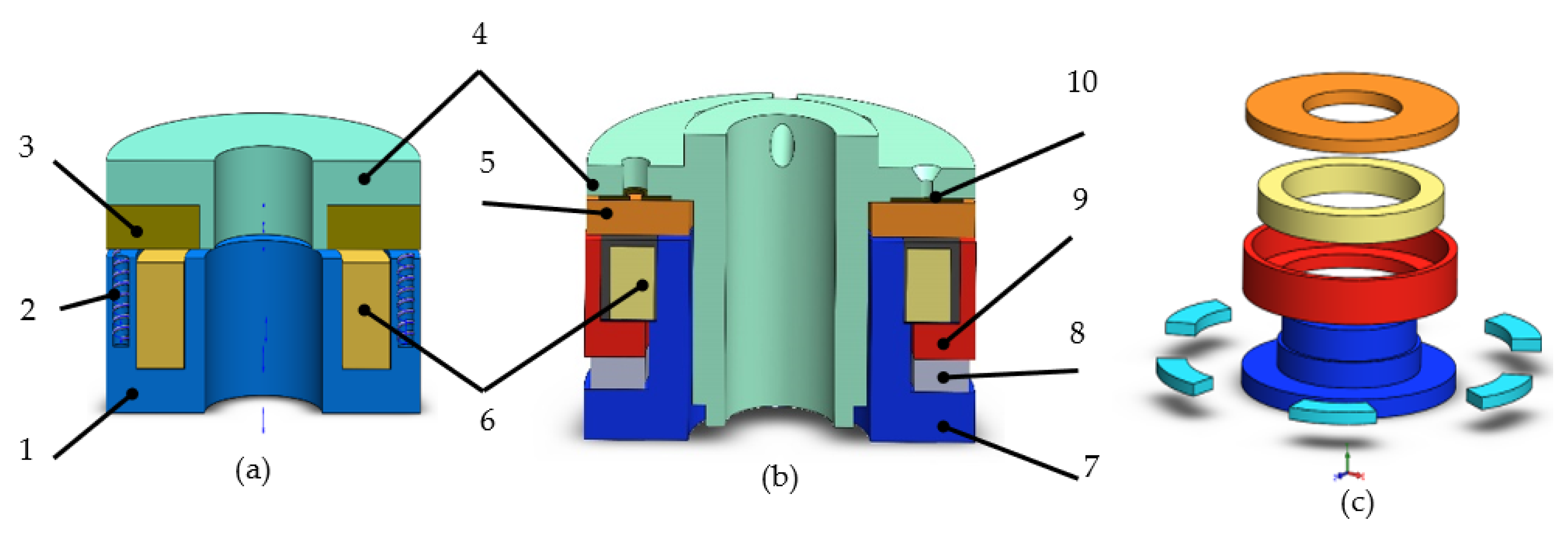
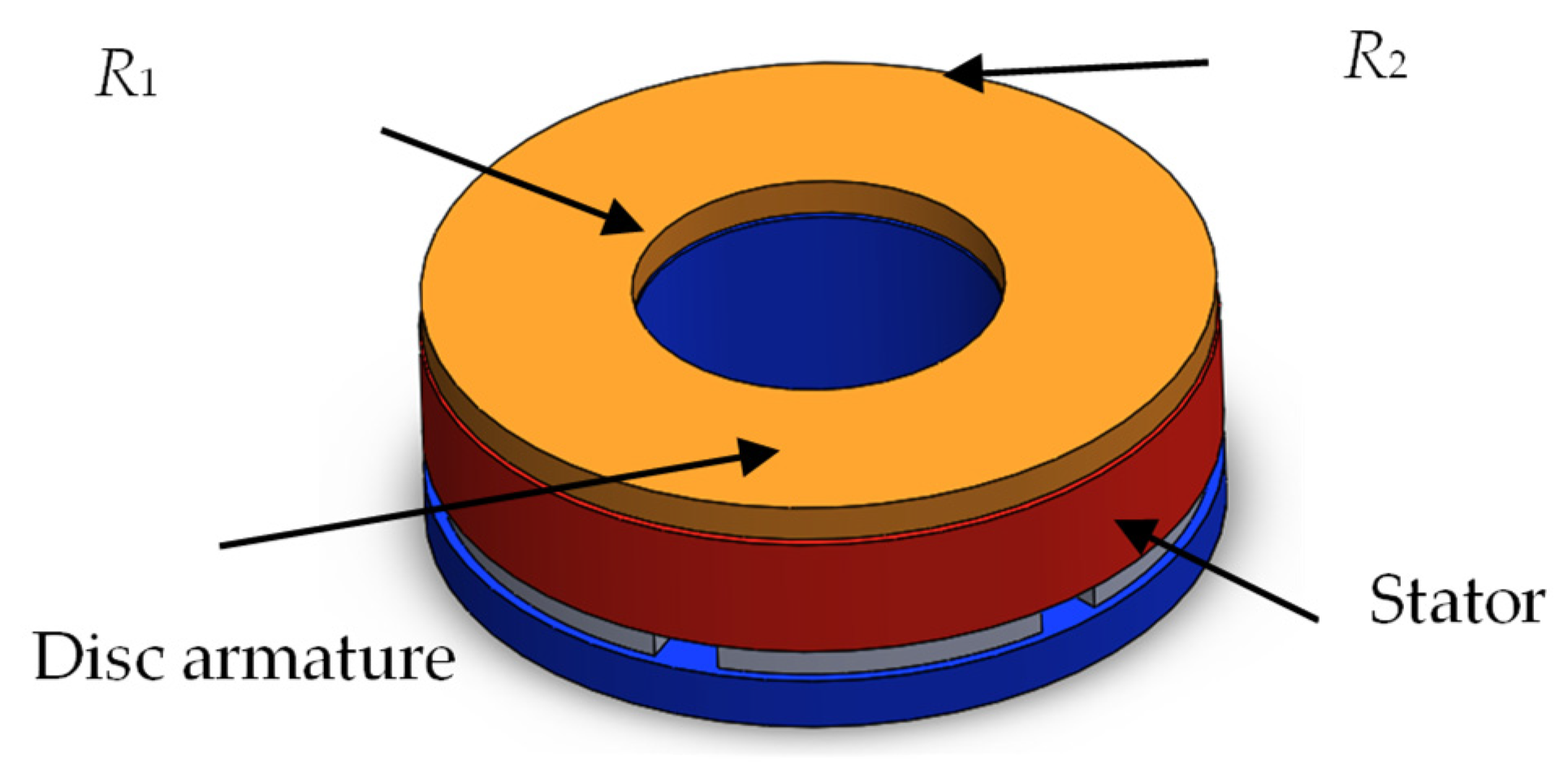
2.1. Structure Design
2.2. Coil Design
- (1)
- The coil number of turns
- (2)
- Coil resistance
3. Analysis Method
3.1. One-Way Method
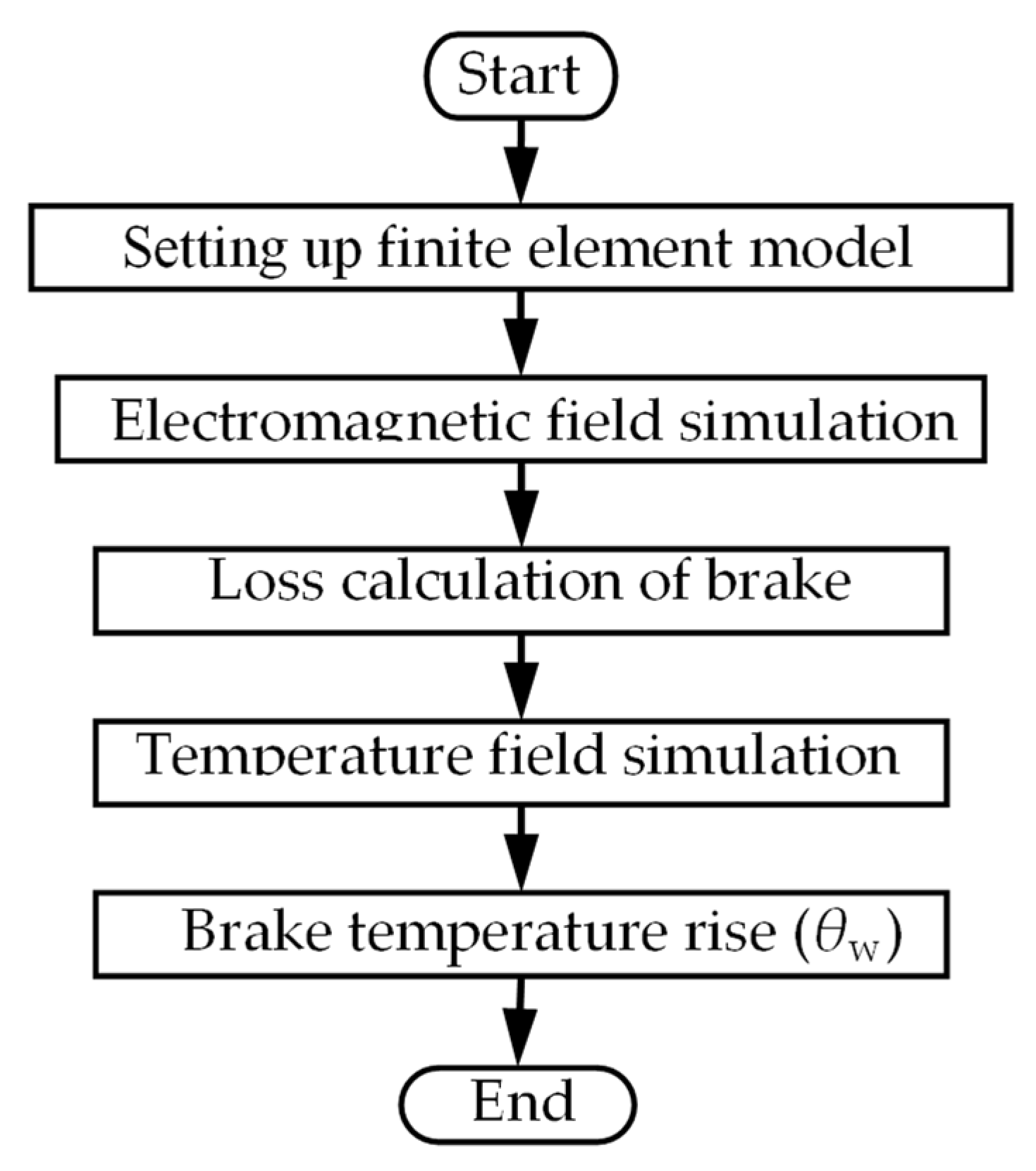
3.2. Magneto-Thermal Coupling Method
4. Magneto-Thermal Coupling Analysis
4.1. Finite Element Model
4.2. Temperature Rise Evaluation Based on Magneto-Thermal Coupling
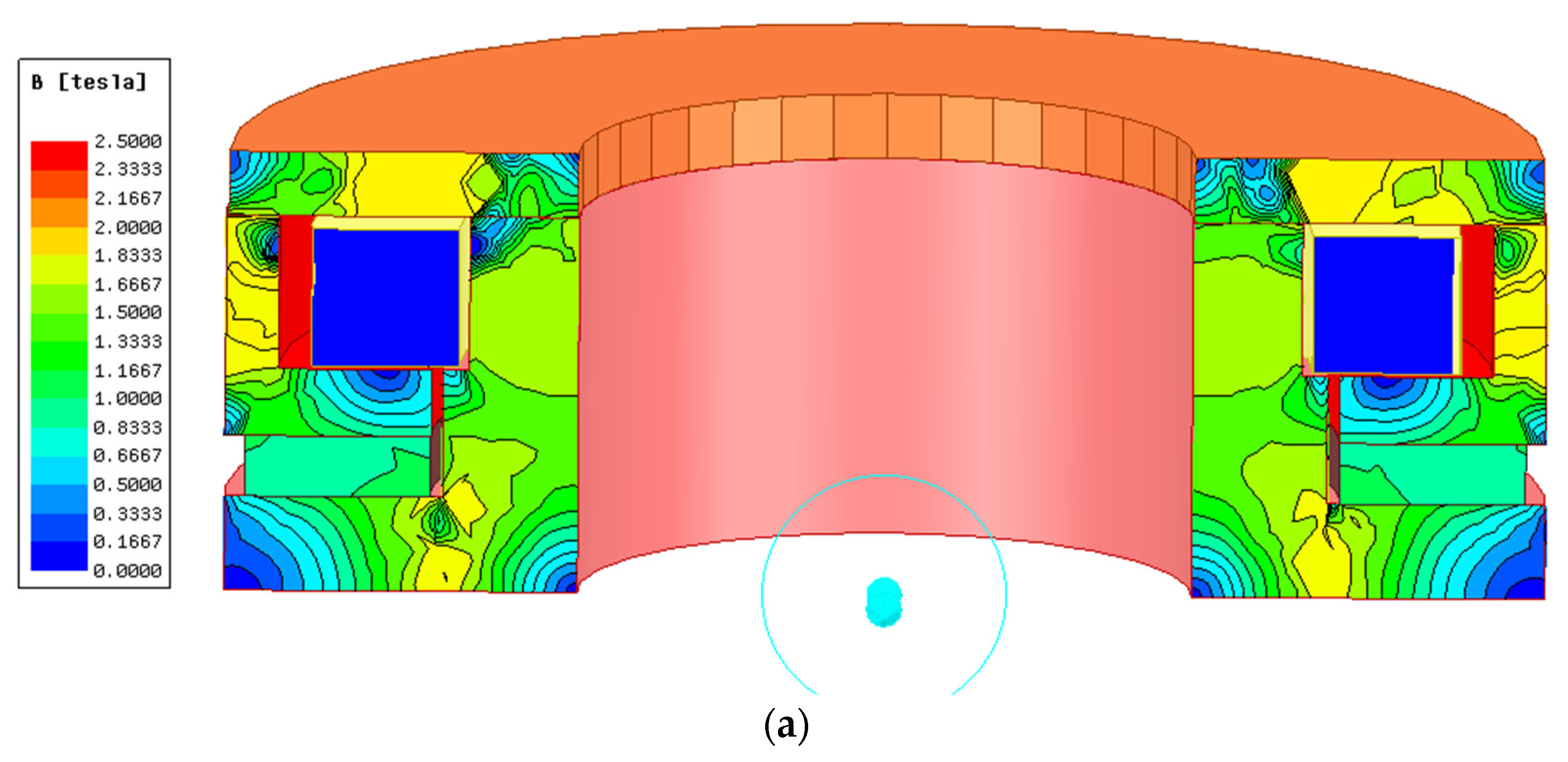
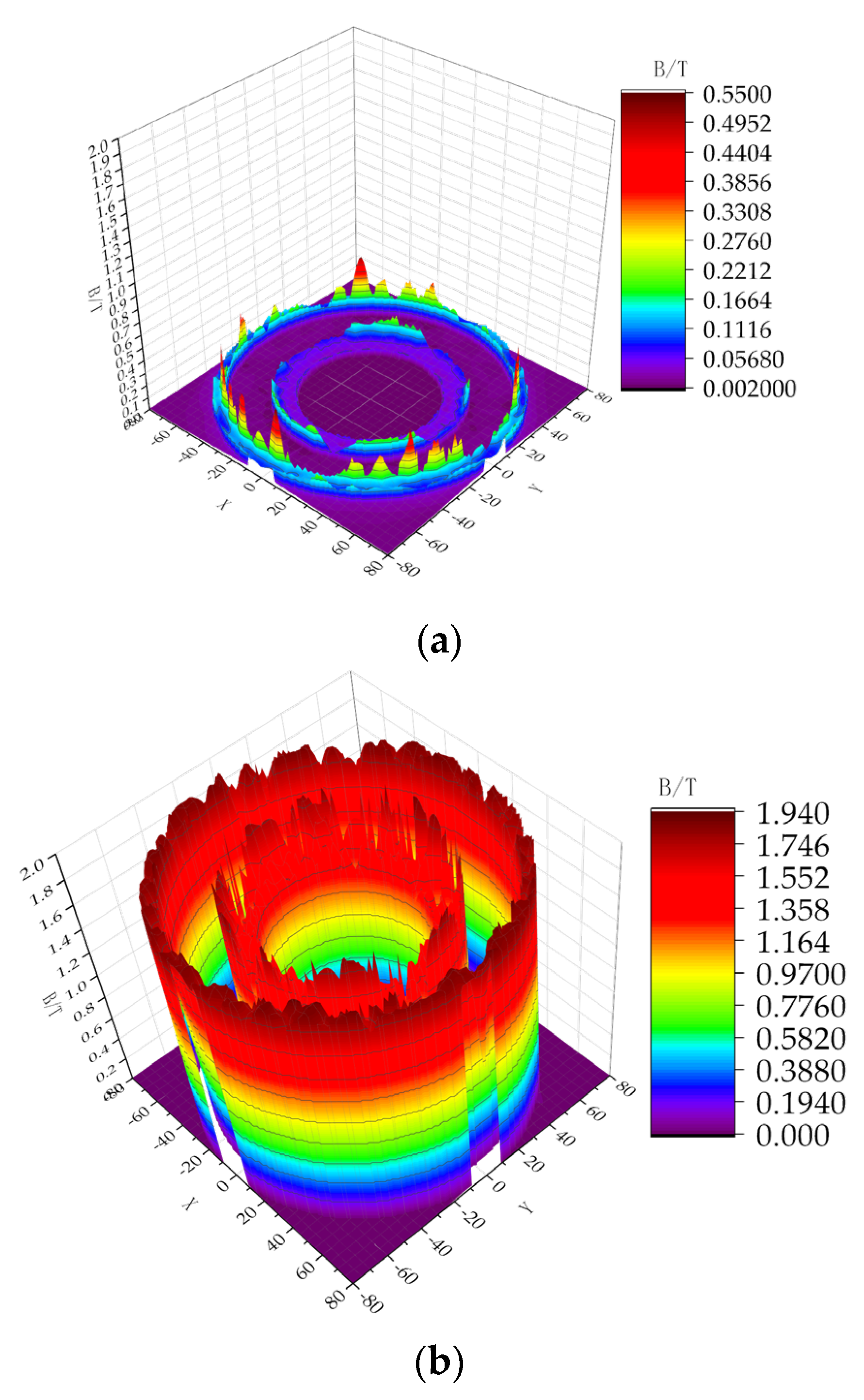
4.3. Performance Evaluation Based on Magneto-Thermal Coupling
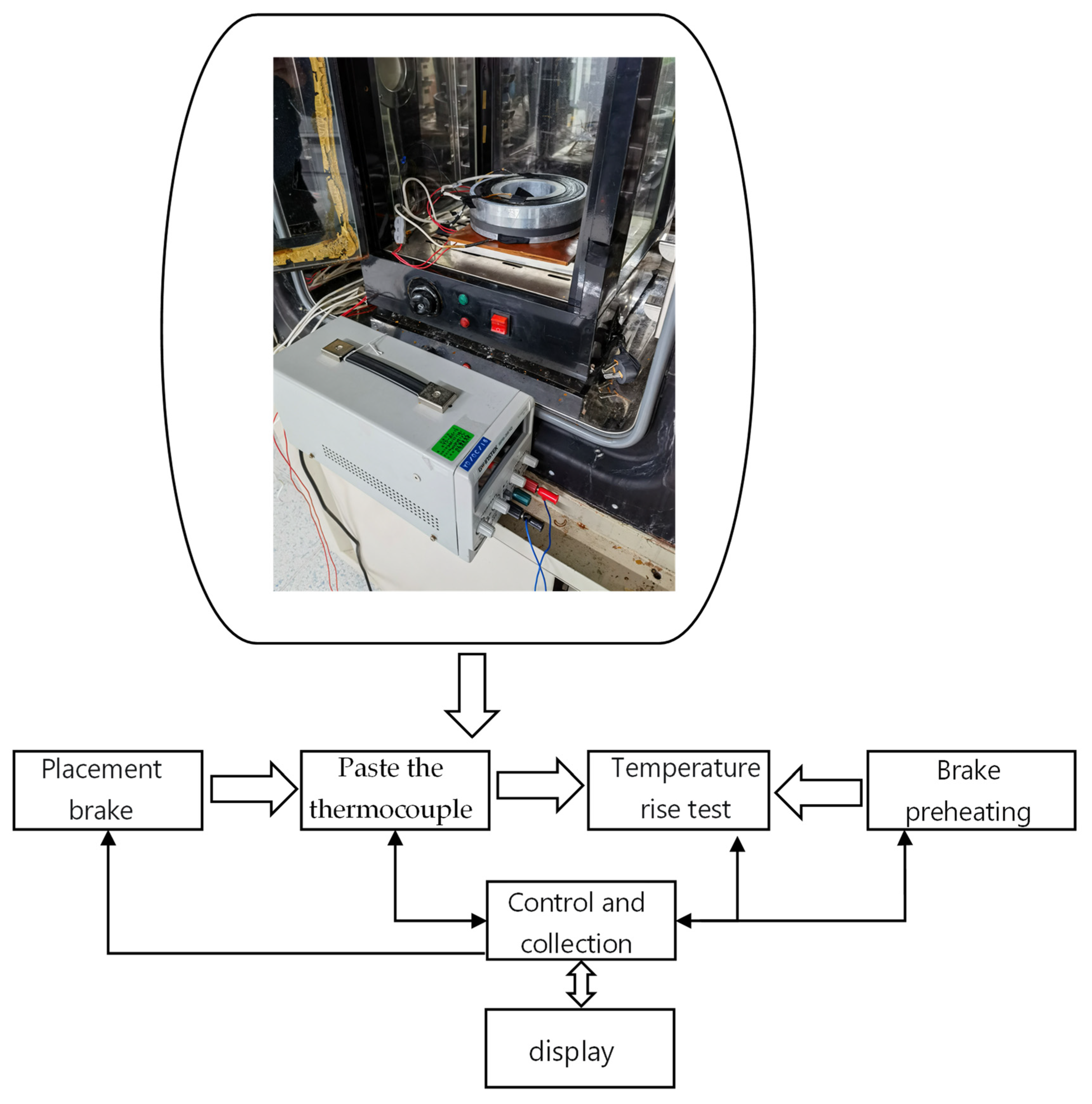
5. Experimental Validation
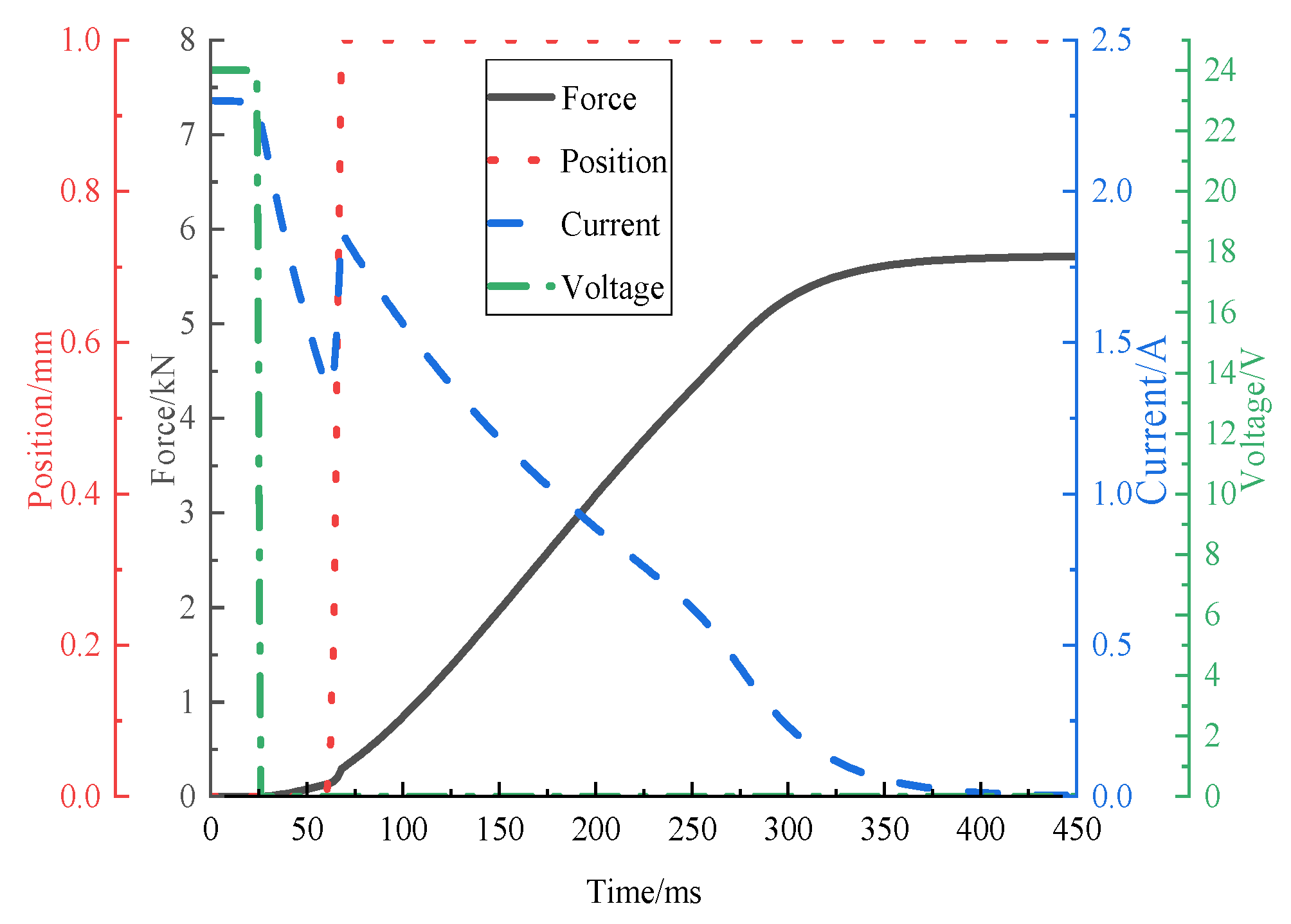
5.1. Temperature Rise Test and Model Validation
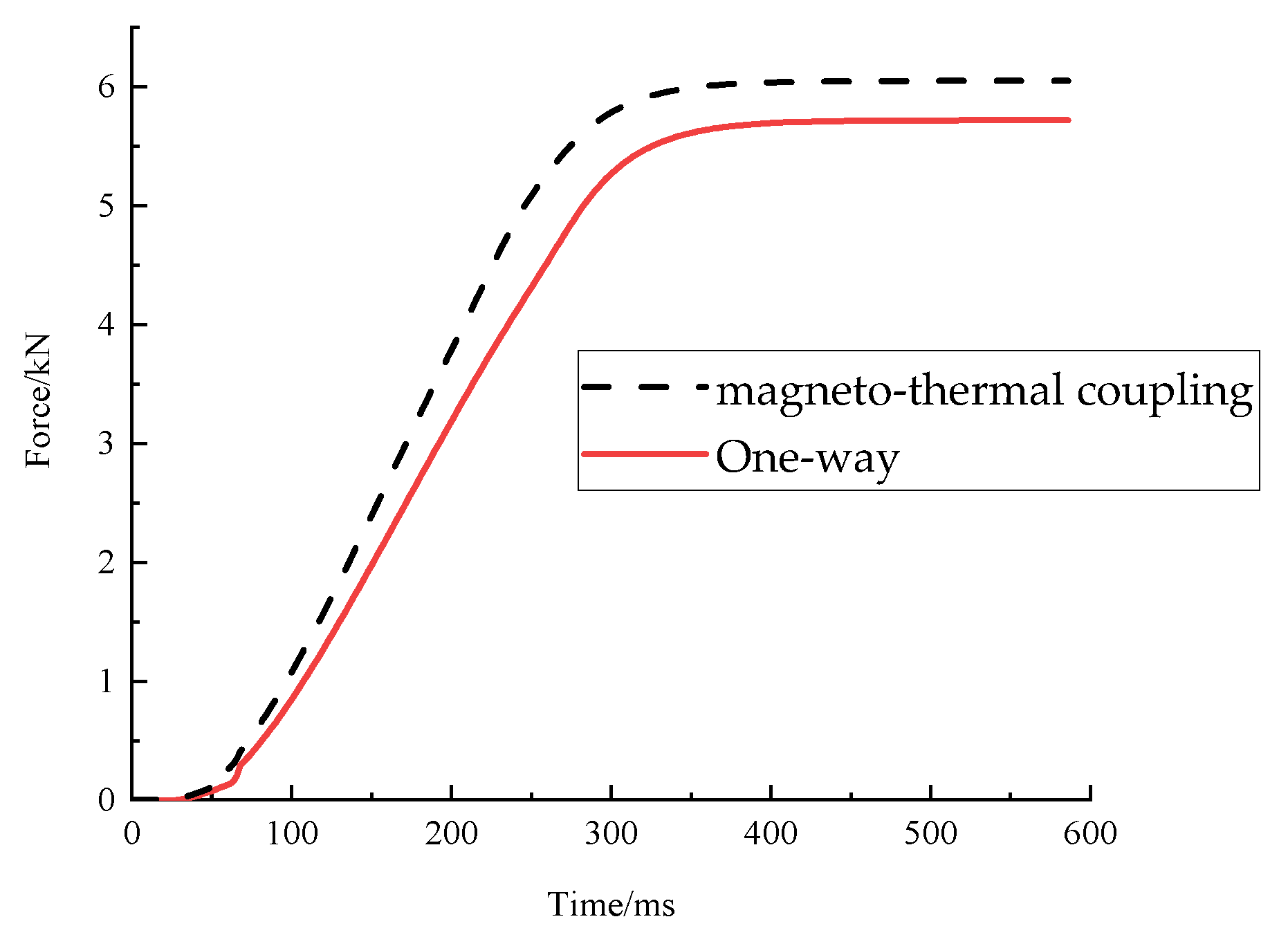
5.2. Torque Testing and Model Validation
6. Summary and Prospect
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Zhang, G.; Zhu, J.; Li, Y.; Yuan, Y.; Xiang, Y.; Lin, P.; Wang, L.; Liu, J.; Liang, L.; Deng, Z. Simulation of the Braking Effects of Permanent Magnet Eddy Current Brake and Its Effects on Levitation Characteristics of HTS Maglev Vehicles. Actuators 2022, 11, 295. [Google Scholar] [CrossRef]
- Wang, J.; Li, J.Q.; Gao, Z.W. A High Torque Magnetorheological Brake with Three Effective Areas. Appl. Mech. Mater. 2015, 742, 470–476. [Google Scholar] [CrossRef]
- Lv, M.P.; Gao, S.Y.; Wei, Y.J.; Zhang, D.; Qi, H.H. Model-Free Parallel Predictive Torque Control Based on Ultra-Local Model of Permanent Magnet Synchronous Machine. Actuators 2022, 11, 31. [Google Scholar] [CrossRef]
- Sinha, A.; Ischia, G.; Menapace, C.; Gialanella, S. Experimental Characterization Protocols for Wear Products from Disc Brake Materials. Atmosphere 2020, 11, 1102. [Google Scholar] [CrossRef]
- Ito, Y.; Oda, Y.; Narita, T.; Kato, H. Effect of Optimal Placement of Permanent Magnets on the Electromagnetic Force in the Horizontal Direction. Actuators 2018, 7, 54. [Google Scholar] [CrossRef]
- Mallik, S.; Mallik, K.; Barman, A.; Maiti, D.; Biswas, S.K.; Deb, N.K.; Basu, S. Efficiency and Cost Optimized Design of an Induction Motor Using Genetic Algorithm. IEEE Trans. Ind. Electron. 2017, 64, 9854–9863. [Google Scholar] [CrossRef]
- Li, Y.; Zhang, C.; Shen, Y.D. Study on electromagnetic separation mechanism of aerospace electrical Connector with large thrust range. Trans. China Electrotech. Soc. 2015, 30, 128–134. [Google Scholar]
- Li, S.W.; Qin, J.; Yu, L. Research on Temperature Rise of Electromagnet of Electromagnetic Clamp Disc Brake. Manuf. Upgrad. Today 2020, 10, 72–73. [Google Scholar]
- Dilshad, M.R.; Ashok, S.; Vijayan, V.; Pathiyil, P. An energy loss model based temperature estimation for Permanent Magnet Synchronous Motor (PMSM). In Proceedings of the 2016 2nd International Conference on Advances in Electrical, Electronics, Information, Communication and Bio-Informatics (AEEICB), Chennai, India, 27–28 February 2016; pp. 172–176. [Google Scholar]
- Tang, J.; Zuo, Y. The design and magnetic field analysis of a double rotor permanent magnet braking device. Processes 2022, 10, 346. [Google Scholar] [CrossRef]
- Li, L.; Yang, G.L.; Li, Z.X. Magneto-thermal coupling analysis of the permanent magnet eddy current brake under intensive impact load. In Proceedings of the 42nd International Conference on Vibroengineering, Shanghai, China, 19–21 October 2019; Volume 28, pp. 177–182. [Google Scholar]
- Fan, Y.M.; Yang, G.L. Design and analysis of magnetic circuit of permanent magnet eddy current brake. In Proceedings of the 42nd International Conference on Vibroengineering, Shanghai, China, 19–21 October 2019; Volume 28, pp. 111–117. [Google Scholar]
- Li, J.H.; Yang, G.L.; Sun, Q.Z. Characteristic and Thermal Analysis of Permanent Magnet Eddy Current Brake. Comput. Model. Eng. Sci. 2021, 126, 1011–1031. [Google Scholar] [CrossRef]
- Lee, C.-Y.; Lee, S.-J.; Tang, M.-S.; Chen, P.-C. In Situ Monitoring of Temperature inside Lithium-Ion Batteries by Flexible Micro Temperature Sensors. Sensors 2011, 11, 9942–9950. [Google Scholar] [CrossRef] [PubMed]
- Wang, K.Y.; He, R.; Tang, J.H.; Liu, R.C. Design and thermal analysis of a novel permanent magnet-friction integrated brake for vehicle. Therm. Sci. Belgrade 2020, 24, 1827–1834. [Google Scholar] [CrossRef]
- Wang, K.; Ju, H.; Yang, Y.; Guo, Z. An Optimized Permanent Magnet Brake Mechanism in Robot Joints. IEEE Access 2021, 9, 18278–18286. [Google Scholar] [CrossRef]
- Yasa, Y.; Sincar, E.; Ertugrul, B.T.; Mese, E. Design considerations of electromagnetic brakes for servo applications. In Proceedings of the 2014 IEEE 23rd International Symposium on Industrial Electronics (ISIE), Istanbul, Turkey, 1–4 June 2014; pp. 768–774. [Google Scholar]
- Woochu, K.; Eun, K.J.; Young, K.Y. Coil configuration design for the Lorentz force maximization by the topology optimization method: Applications to optical pickup coil design. Sens. Actuators A Phys. 2005, 121, 221–229. [Google Scholar]
- Jo, S.; Shin, H.; Chang, J. Dynamic Analysis of Surface-Mounted Permanent Magnet Type Coaxial Magnetic Gear With Damper Bar Considering Magnetic Field Modulation Effect. IEEE Access 2022, 10, 33616–33627. [Google Scholar] [CrossRef]
- Asif, A.; Mujeebu, M.A. Thermo-Mechanical and Structural Performances of Automobile Disc Brakes: A Review of Numerical and Experimental Studies. Arch. Comput. Methods Eng. 2018, 26, 1489–1513. [Google Scholar]
- Xu, Y.D.; Li, L.; Yuan, X. Magneto-thermal coupling simulation and experimental verification for a three-winding high-frequency transformer. Int. J. Appl. Electromagn. Mech. Amst. 2022, 68, 159–175. [Google Scholar] [CrossRef]
- Zhang, W.; Yu, Z.; Chen, X.; Huang, Q. The Magneto-Thermal Analysis of a High Torque Density Joint Motor for Humanoid Robots. In Proceedings of the 2018 IEEE-RAS 18th International Conference on Humanoid Robots (Humanoids), Beijing, China, 6–9 November 2018; pp. 112–117. [Google Scholar]
- Cisz, G.; Runcos, F.; Waite, S. Comparative analyses of standards temperature rise test methods for induction machines. In Proceedings of the 2009 Record of Conference Papers—Industry Applications Society 56th Annual Petroleum and Chemical Industry Conference, Anaheim, CA, USA, 14–16 September 2009; pp. 1–6. [Google Scholar]
- Huang, Z.Q.; Li, G.F.; Tong, Y.T.; Ouyang, W.P. Research on Dynamic Braking Torque Test Method of Brake Motor. Les Ulis EDP Sci. 2021, 257, 148–153. [Google Scholar] [CrossRef]








| Parameter | Value | Parameter | Value |
|---|---|---|---|
| Stator inner diameter/mm | 75 | Rotor inner diameter/mm | 148 |
| Stator outer diameter/mm | 160 | Rotor outer diameter/mm | 161 |
| Stator height/mm | 46.5 | Rotor height/mm | 27.3 |
| Permanent magnet Thickness/mm | 7.5 | Air gap/mm | 1 |
| Method | Braking Torque/N∙m |
|---|---|
| Magneto-thermal coupling | 162 |
| The one-way method | 153 |
| Parameter | Value |
|---|---|
| Maximum Torque | 145 N∙m |
| Rated torque | 50 N∙m |
| Rated Speed | 1500 r/min |
| Rated power | 7.9 kW |
| Rated Frequency | 100 Hz |
| Rated current | 15 A |
| Torque | 53 N∙m |
| Rotational Inertia | 56 kg∙cm2 |
| Parameter | Value | |
|---|---|---|
| Rated voltage | DC24 V | |
| Rated power | 55 W | |
| Response time | 20 ms | |
| Air clearance | 0.55 mm | |
| 25 °C | Rated current | 2.3 A |
| Coil resistance | 10.5 Ω | |
| Torque | 170 N∙m | |
| 120 °C | Rated current | 1.66 A |
| Coil resistance | 14.5 Ω | |
| Torque | 140 N∙m | |
| Time | A1 (N∙m) | A2 (N∙m) | A3 (N∙m) | A4 (N∙m) | A5 (N∙m) | Average (N∙m) |
|---|---|---|---|---|---|---|
| 0 | 220.8 | 216.9 | 226.8 | 216.5 | 218.5 | 219.903 |
| 1 | 185.9 | 174.4 | 188.2 | 187.9 | 162.6 | 179.784 |
| 2 | 175.2 | 172.1 | 162.8 | 180.6 | 170.9 | 172.315 |
| 3 | 196.3 | 164.6 | 161.6 | 168.9 | 162.9 | 170.864 |
| 4 | 181.6 | 161.0 | 164.6 | 173.0 | 166.6 | 169.369 |
| 5 | 169.6 | 167.8 | 175.8 | 173.1 | 164.4 | 170.14 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wu, Y.; Li, Y.; Wang, G.; Zhang, C.; Pang, J. Performance Evaluation of a High-Torque Permanent Magnet Brake at Operating Temperature Based on Magneto-Thermal Coupling Method. Actuators 2023, 12, 149. https://doi.org/10.3390/act12040149
Wu Y, Li Y, Wang G, Zhang C, Pang J. Performance Evaluation of a High-Torque Permanent Magnet Brake at Operating Temperature Based on Magneto-Thermal Coupling Method. Actuators. 2023; 12(4):149. https://doi.org/10.3390/act12040149
Chicago/Turabian StyleWu, Yichao, Yong Li, Guixian Wang, Chaohui Zhang, and Jihong Pang. 2023. "Performance Evaluation of a High-Torque Permanent Magnet Brake at Operating Temperature Based on Magneto-Thermal Coupling Method" Actuators 12, no. 4: 149. https://doi.org/10.3390/act12040149
APA StyleWu, Y., Li, Y., Wang, G., Zhang, C., & Pang, J. (2023). Performance Evaluation of a High-Torque Permanent Magnet Brake at Operating Temperature Based on Magneto-Thermal Coupling Method. Actuators, 12(4), 149. https://doi.org/10.3390/act12040149







