Trajectory Tracking and Adaptive Fuzzy Vibration Control of Multilink Space Manipulators with Experimental Validation
Abstract
1. Introduction
- A mathematical model of a multilink flexible space manipulator system with flexible joints and links was established.
- The dynamic responses and natural characteristics of the flexible manipulator under three different kinds of mode shapes are compared.
- Based on the underactuated characteristic of the system, the underactuated CTM is designed to achieve high-precision performance on flexible joint trajectory tracking, and the non-model adaptive fuzzy controller is adopted to suppress the elastic vibrations of the flexible links.
2. Dynamics of Flexible Space Manipulator System
2.1. Mathematical Model of Flexible Space Manipulator System
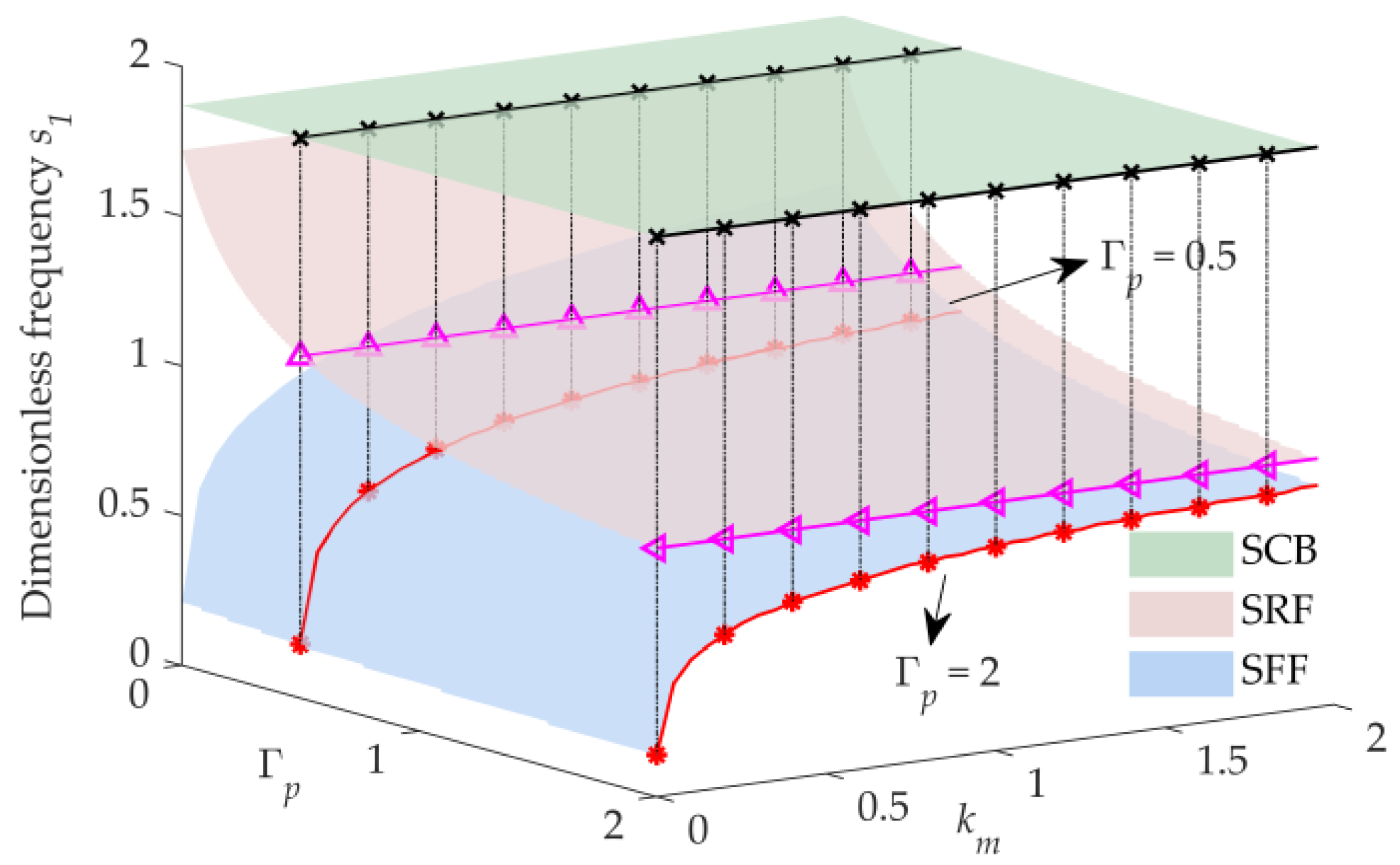
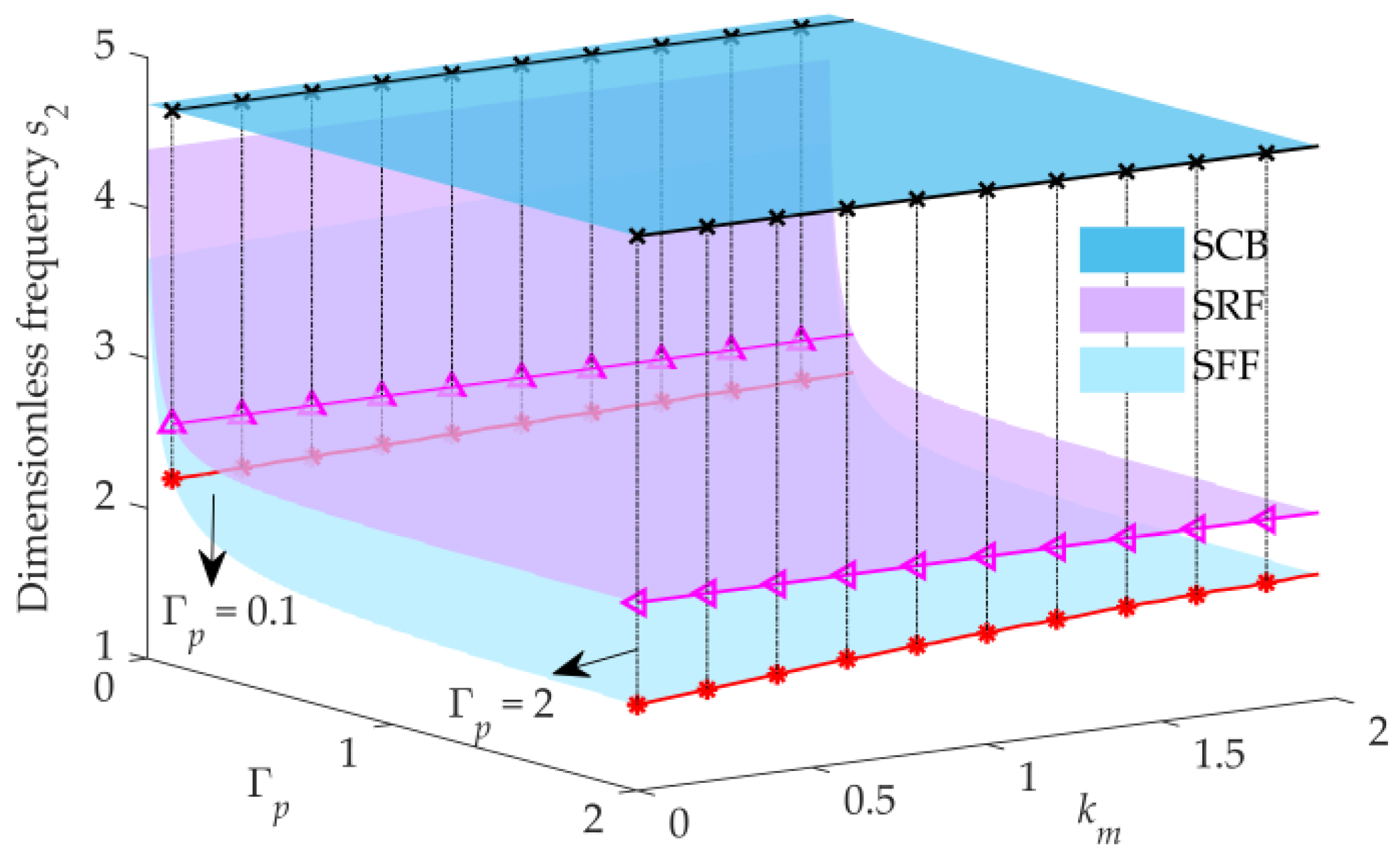
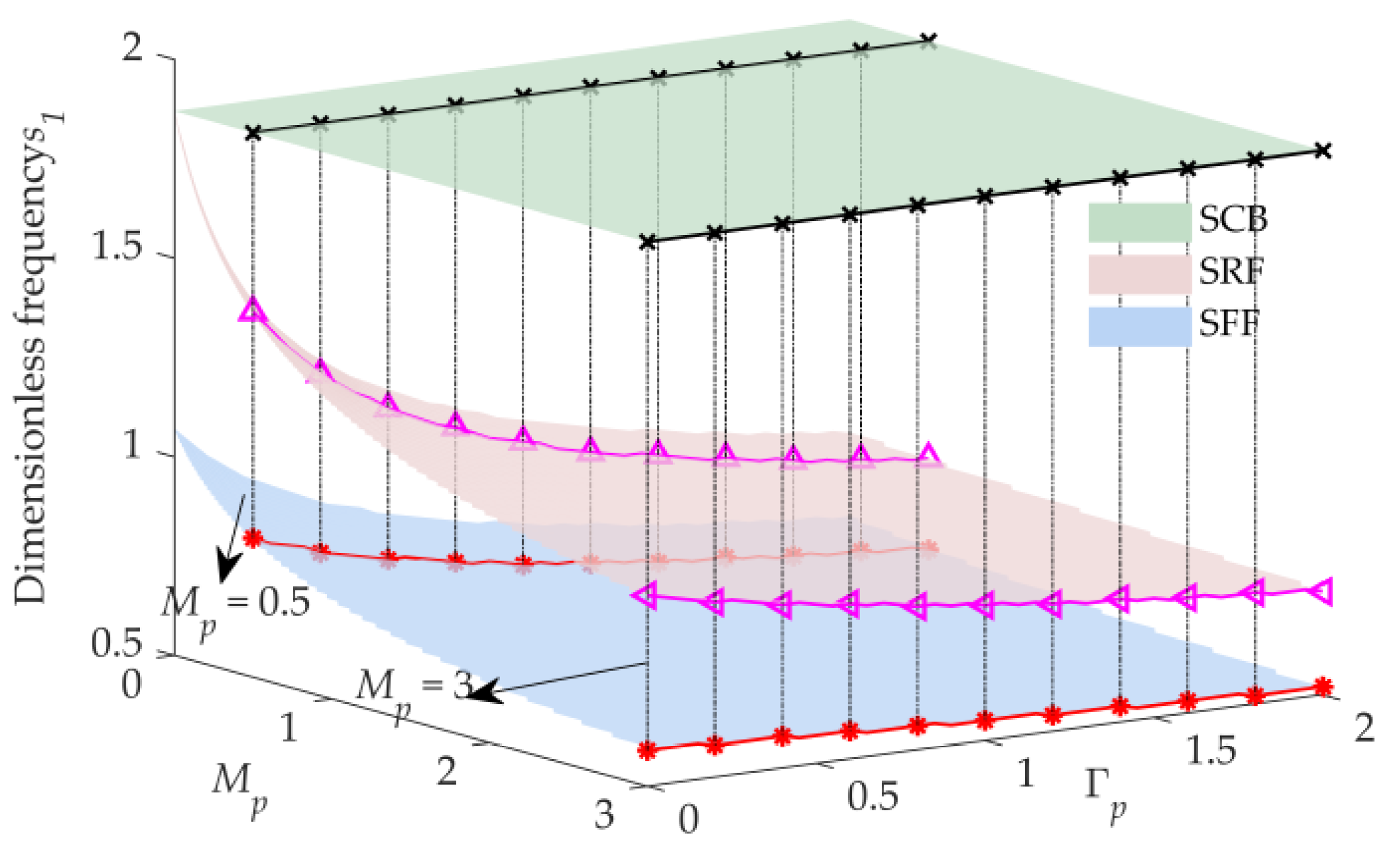
2.2. Natural Characteristics of the Flexible Links under Different Boundary Conditions
- Fixed-free boundary condition, which is a single cantilever beam (SCB) boundary condition.
- Fixed-inertial load boundary condition, which is a single rigid joint and flexible link manipulator (SRF) boundary condition.
- Elastic load-inertial load boundary condition, which is a single flexible joint and flexible link manipulator (SFF) boundary condition.
- SCB boundary condition:
- SRF boundary condition:
- SFF boundary condition:
- SCB boundary condition:
- SRF boundary condition:
- SFF boundary condition:
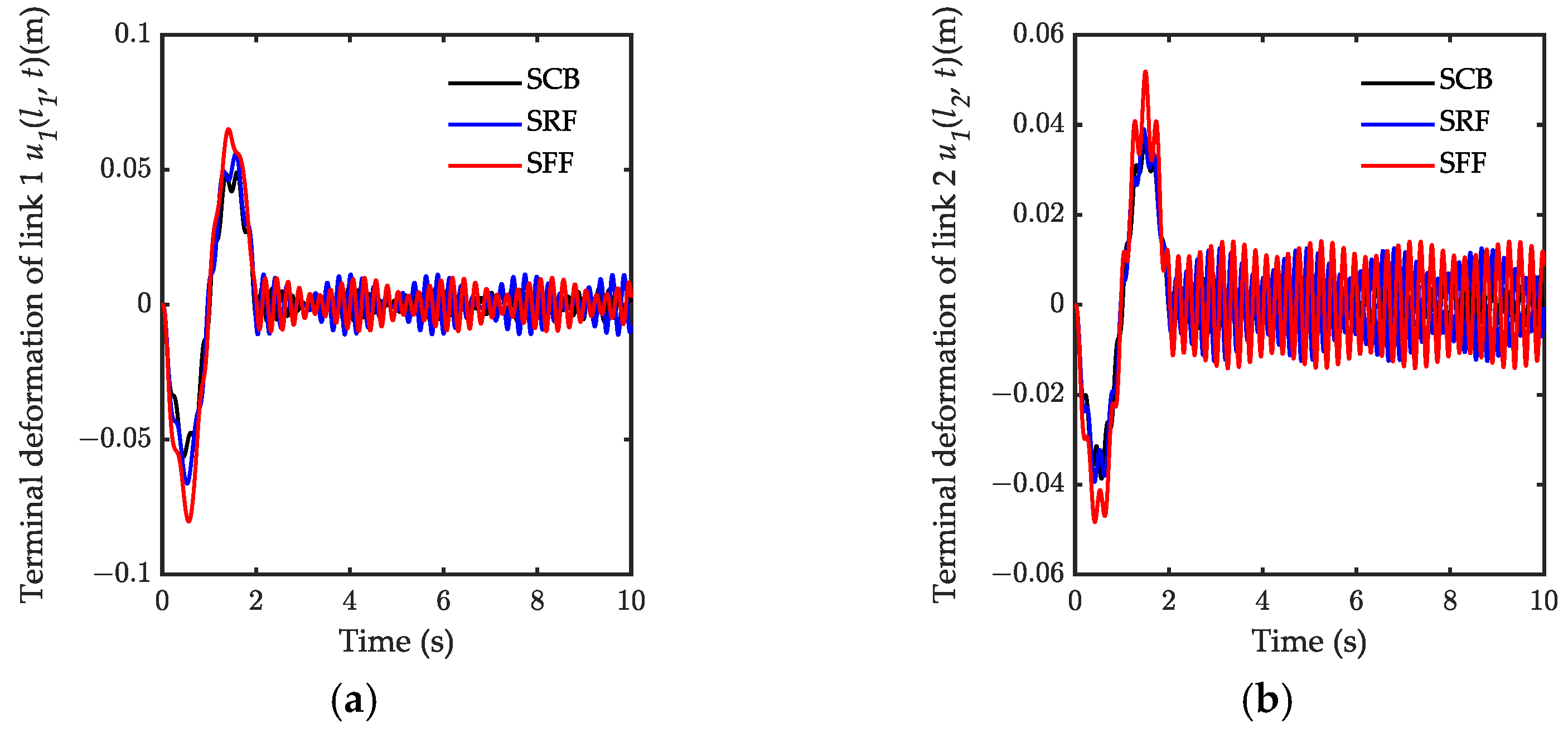
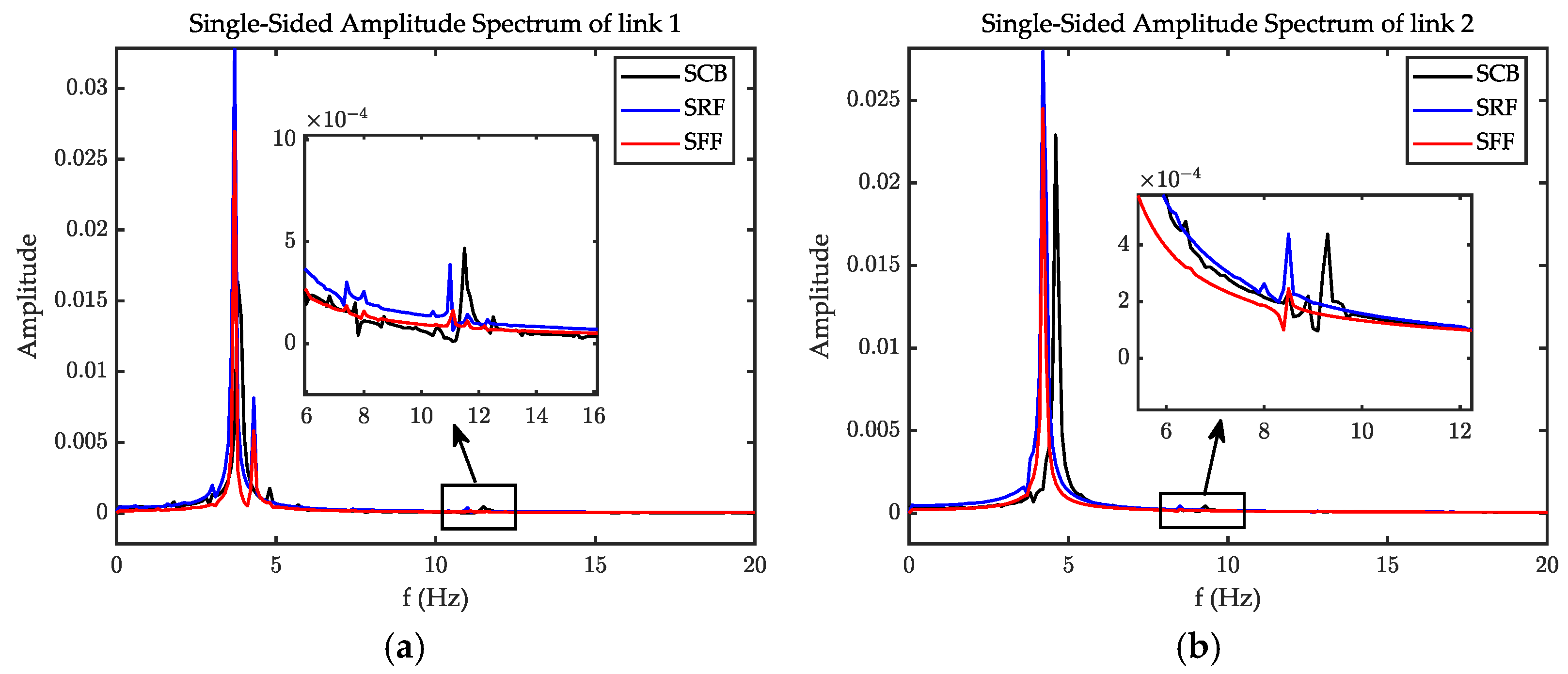
2.3. Dynamic Response of a Flexible Space Manipulator System
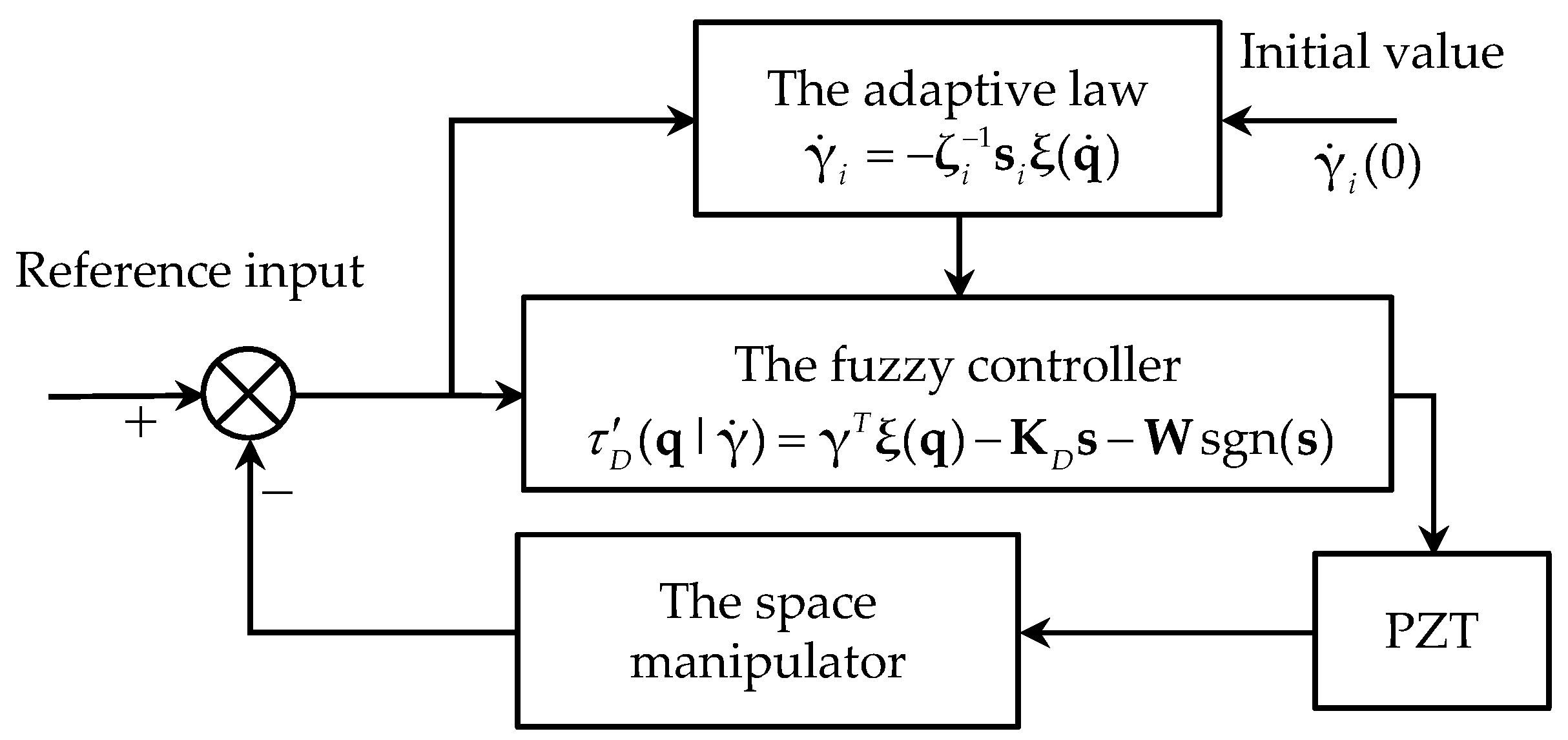
3. Controller Design
3.1. Singular Perturbation Model of Flexible Space Manipulator System
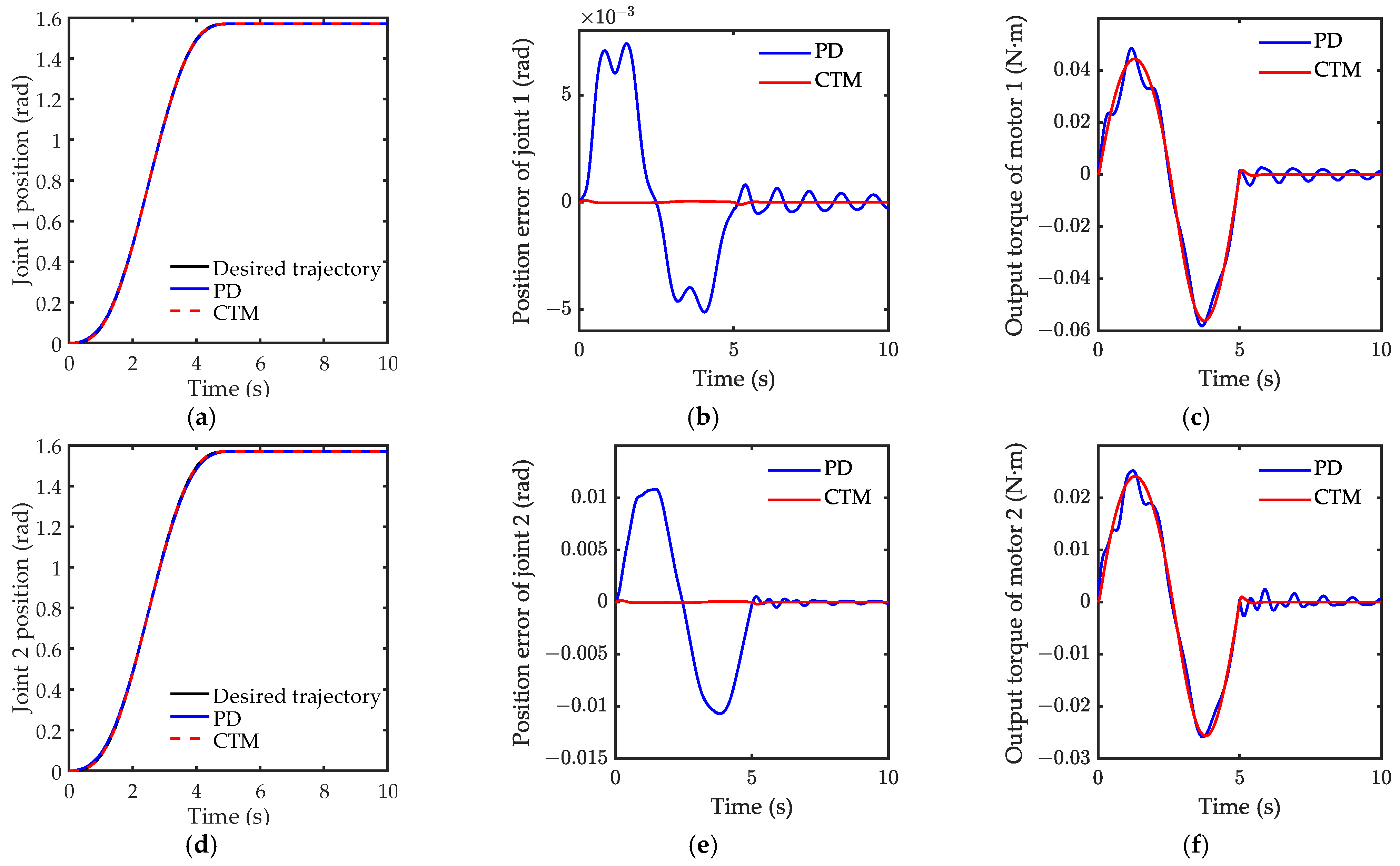
3.2. Computed Torque Controller Designed for Joints
3.3. Adaptive Fuzzy Controller Designed for Piezo Actuator
4. Numerical Simulation
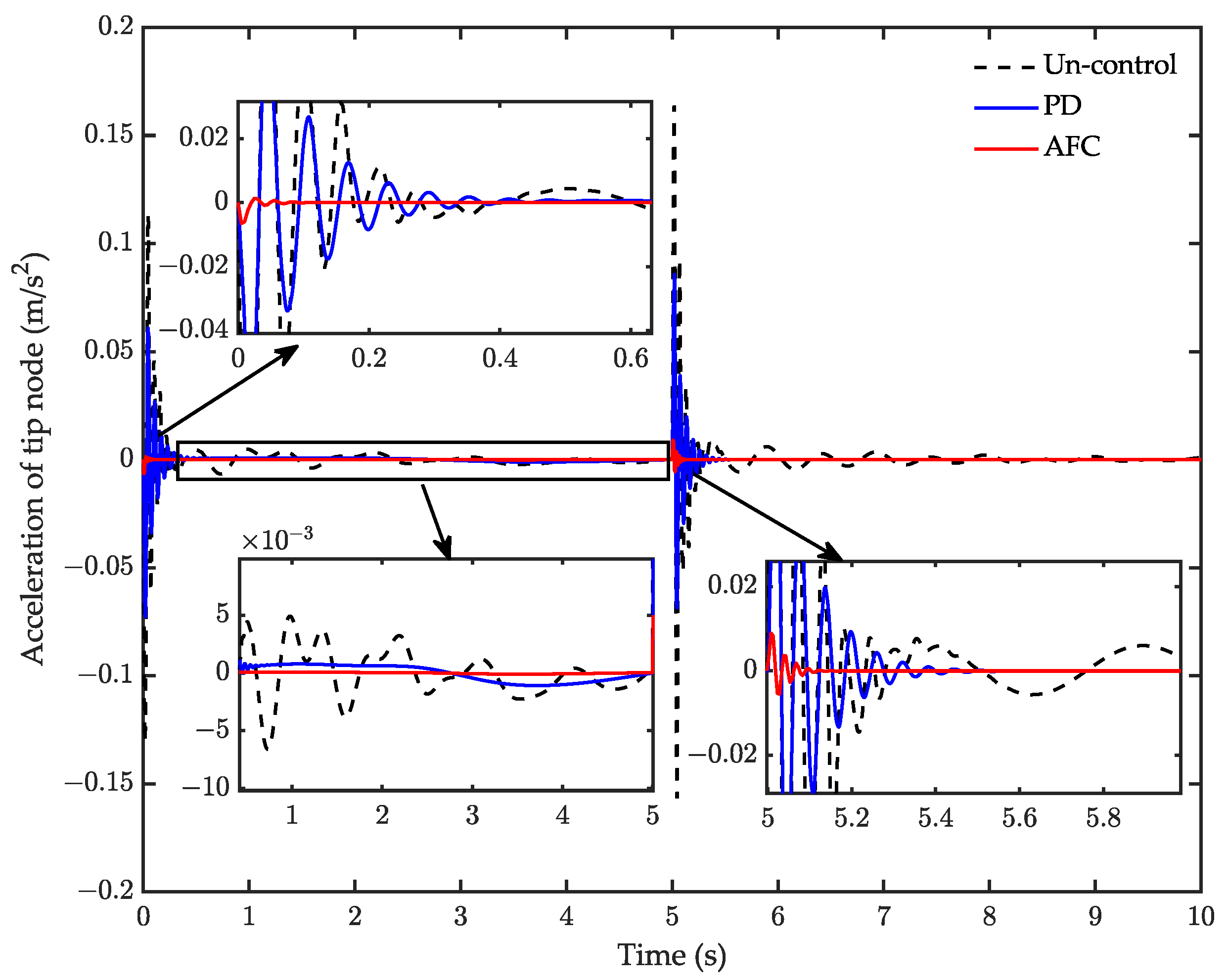
4.1. Case 1: Setpoint Motion Control
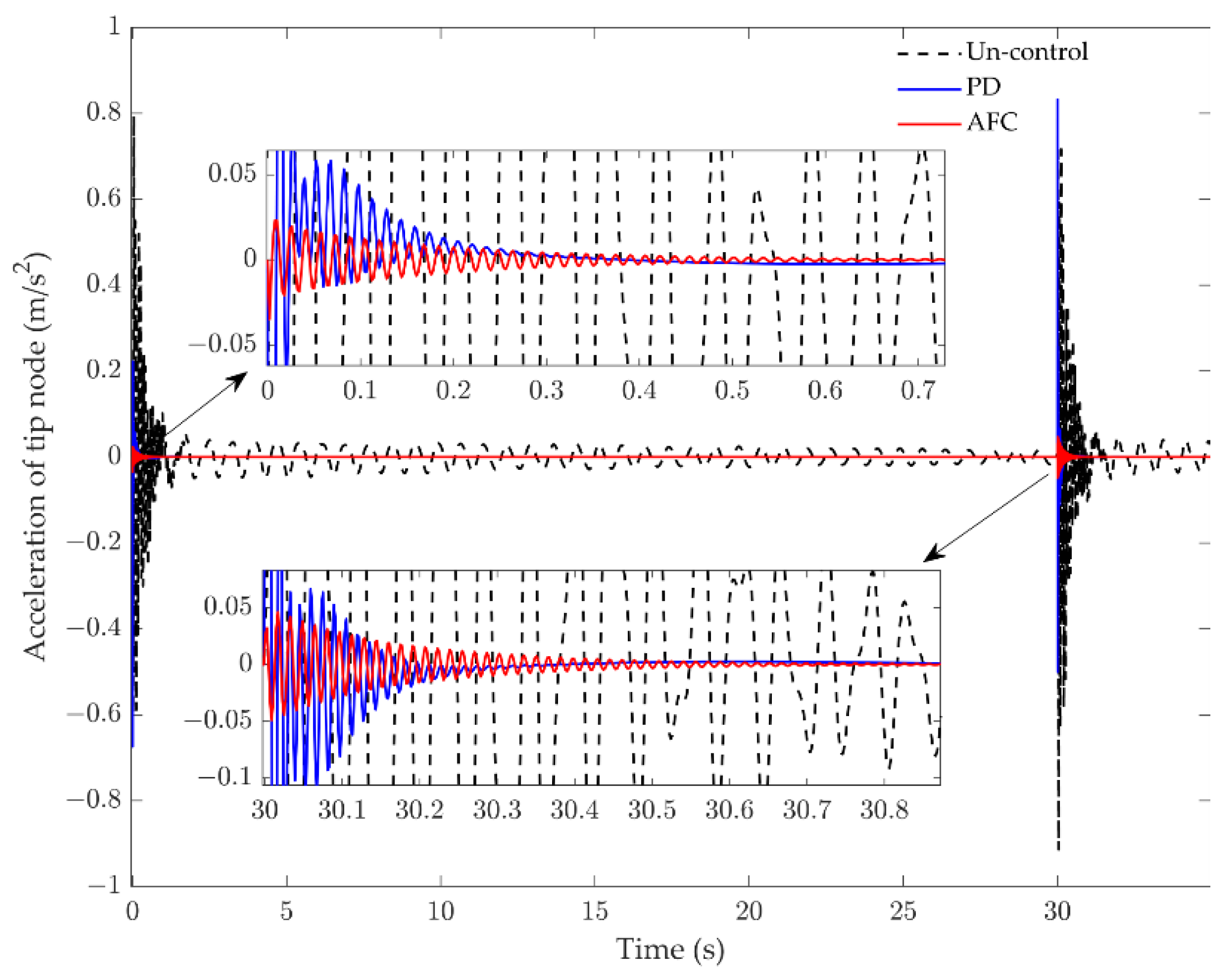
4.2. Case 2: Periodic Motion Control
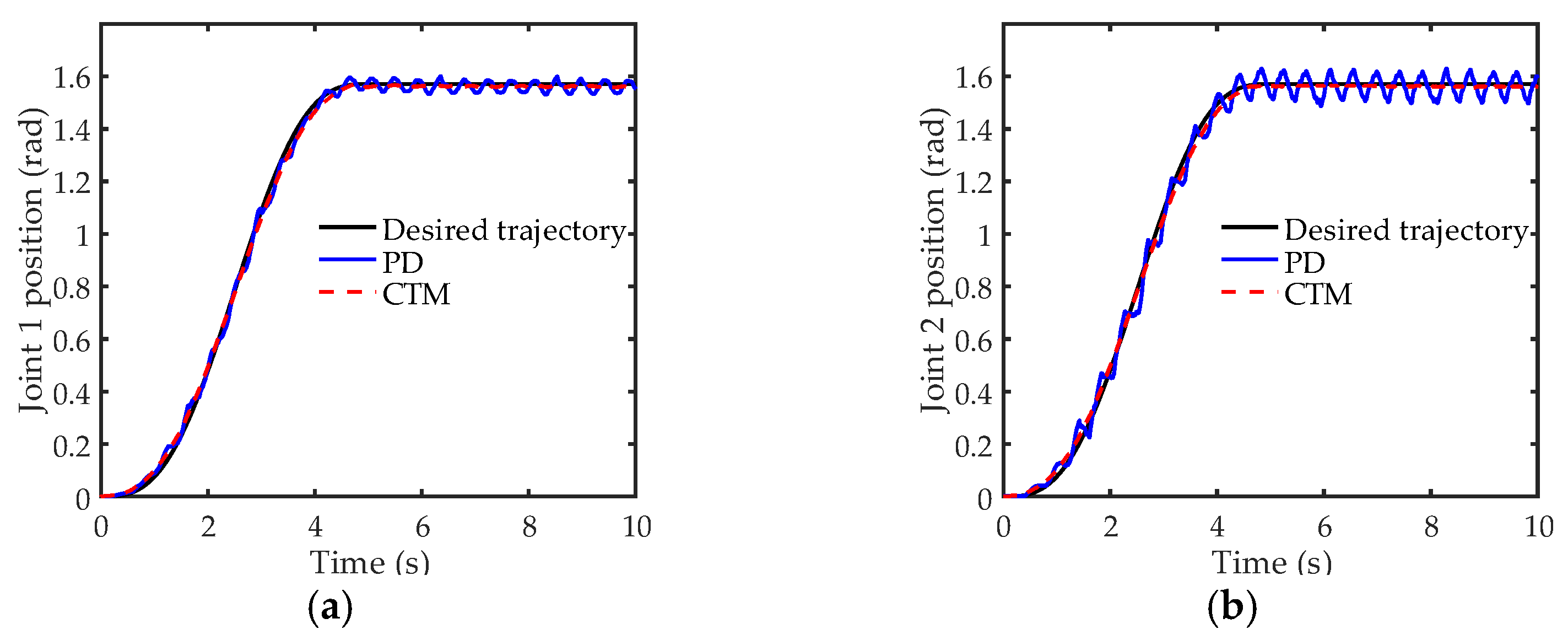
5. Experimental Results
6. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Sachdev, S.; Harvey, W.; Gibbs, G.; Marcotte, B.; Buckley, N.; Braithwaite, T.; Rey, D. Canada and the International Space Station Program: Overview and Status since IAC 2005. In Proceedings of the 57th International Astronautical Congress, Valencia, Spain, 2–6 October 2006. [Google Scholar]
- Friend, R.B. Orbital Express program summary and mission overview. In Proceedings of the SPIE Defense and Security Symposium, Orlando, FL, USA, 15 April 2008. [Google Scholar]
- Alandoli, E.A.; Lee, T.S. A Critical Review of Control Techniques for Flexible and Rigid Link Manipulators. Robotica 2020, 38, 2239–2265. [Google Scholar] [CrossRef]
- Subedi, D.; Tyapin, I.; Hovland, G. Review on Modeling and Control of Flexible Link Manipulators. Model. Identif. Control 2020, 41, 141–163. [Google Scholar] [CrossRef]
- Yu, X.; Chen, L. Dynamic modeling and control of a free-flying space robot with flexible-link and flexible-joints. In Proceedings of the IEEE International Conference on Robotics & Automation, Hong Kong, China, 31 May–7 June 2014. [Google Scholar]
- Dwivedy, S.K.; Eberhard, P. Dynamic analysis of flexible manipulators, a literature review. Mech. Mach. Theory 2006, 41, 749–777. [Google Scholar] [CrossRef]
- Wei, J.; Cao, D.; Wang, L.; Huang, H.; Huang, W. Dynamic modeling and simulation for flexible spacecraft with flexible jointed solar panels. Int. J. Mech. Sci. 2017, 130, 558–570. [Google Scholar] [CrossRef]
- Kiang, C.T.; Spowage, A.; Yoong, C.K. Review of control and sensor system of flexible manipulator. J. Intell. Robot. Syst. 2015, 77, 187–213. [Google Scholar] [CrossRef]
- He, F.; Huang, Q. Time-Optimal Trajectory Planning of 6-DOF Manipulator Based on Fuzzy Control. Actuators 2022, 11, 332. [Google Scholar] [CrossRef]
- Sabatini, M.; Gasbarri, P.; Monti, R.; Palmerini, G.B. Vibration control of a flexible space manipulator during on orbit operations. Acta Astronaut. 2012, 73, 109–121. [Google Scholar] [CrossRef]
- Zhang, Q.; Li, C.; Zhang, J.; Zhang, J. Smooth adaptive sliding mode vibration control of a flexible parallel manipulator with multiple smart linkages in modal space. J. Sound Vib. 2017, 411, 1–19. [Google Scholar] [CrossRef]
- Zhang, W. The impulse spectrum method for vibration suppression of a flexible multilink robot arm. J. Vib. Control. 2018, 24, 3865–3881. [Google Scholar] [CrossRef]
- Yang, G. Asymptotic tracking with novel integral robust schemes for mismatched uncertain nonlinear systems. Int. J. Robust Nonlinear Control. 2023, 33, 1988–2002. [Google Scholar] [CrossRef]
- Shawky, A.; Ordys, A.; Grimble, M.J. End-point control of a flexible-link manipulator using H∞ nonlinear control via a state-dependent Riccati equation. In Proceedings of the International Conference on Control Applications, Glasgow, UK, 18–20 September 2002. [Google Scholar]
- De Luca, A. Feedforward/Feedback Laws for the Control of Flexible Robots. In Proceedings of the 2000 ICRA, San Francisco, CA, USA, 24–28 April 2000. [Google Scholar]
- Qiu, Z.; Zhang, W. Trajectory planning and diagonal recurrent neural network vibration control of a flexible manipulator using structural light sensor. Mech. Syst. Signal Process. 2019, 132, 563–594. [Google Scholar] [CrossRef]
- Malzahn, J.; Phung, A.S.; Bertram, T. A Multi-Link-Flexible Robot Arm Catching Thrown Balls. In Proceedings of the 7th German Conference on Robotics, Munich, Germany, 21–22 May 2012. [Google Scholar]
- Qingsong, C.; Ailan, Y. Optimal actuator placement for vibration control of two-link piezoelectric flexible manipulator. In Proceedings of the International Conference on Mechanic Automation and Control Engineering, Wuhan, China, 3 August 2010. [Google Scholar]
- Wei, J.; Qiu, Z.; Han, J.; Wang, Y. Experimental Comparison Research on Active Vibration Control for Flexible Piezoelectric Manipulator Using Fuzzy Controller. J. Intell. Robot. Syst. 2010, 59, 31–56. [Google Scholar] [CrossRef]
- Qiu, Z.; Li, C.; Zhang, X. Experimental study on active vibration control for a kind of two-link flexible manipulator. Mech. Syst. Signal Process. 2019, 118, 623–644. [Google Scholar] [CrossRef]
- Zhang, J.; Dai, X.; Huang, Q.; Wu, Q. AILC for Rigid-Flexible Coupled Manipulator System in Three-Dimensional Space with Time-Varying Disturbances and Input Constraints. Actuators 2022, 11, 268. [Google Scholar] [CrossRef]
- Soltani, M.; Keshmiri, M.; Misra, A.K. Dynamic analysis and trajectory tracking of a tethered space robot. Acta Astronaut. 2016, 128, 335–342. [Google Scholar] [CrossRef]
- Shao, M.; Huang, Y.; Silberschmidt, V.V. Intelligent Manipulator with Flexible Link and Joint: Modeling and Vibration Control. Shock Vib. 2020, 2020, 4671358. [Google Scholar] [CrossRef]
- Spong, M.W. Review article: Modeling and control of elastic joint robots. Math. Comput. Model. 1989, 12, 912. [Google Scholar] [CrossRef]
- Gao, H.; He, W.; Zhou, C.; Sun, C. Neural Network Control of a Two-Link Flexible Robotic Manipulator Using Assumed Mode Method. IEEE Trans. Ind. Inform. 2019, 15, 755–765. [Google Scholar] [CrossRef]
- Li, Y.; Tang, B.; Shi, Z.; Lu, Y. Experimental study for trajectory tracking of a two-link flexible manipulator. Int. J. Syst. Sci. 2000, 31, 3–9. [Google Scholar] [CrossRef]
- Vakil, M.; Fotouhi, R.; Nikiforuk, P.N. On the zeros of the transfer function of a single flexible link manipulators and their non-minimum phase behaviour. Proc. Inst. Mech. Eng. Part C 2010, 224, 2083–2096. [Google Scholar] [CrossRef]
- Shao, Z.; Zhang, X. Intelligent control of flexible-joint manipulator based on singular perturbation. In Proceedings of the International Conference on Automation and Logistics, Hong Kong, China, 16–20 August 2010. [Google Scholar]
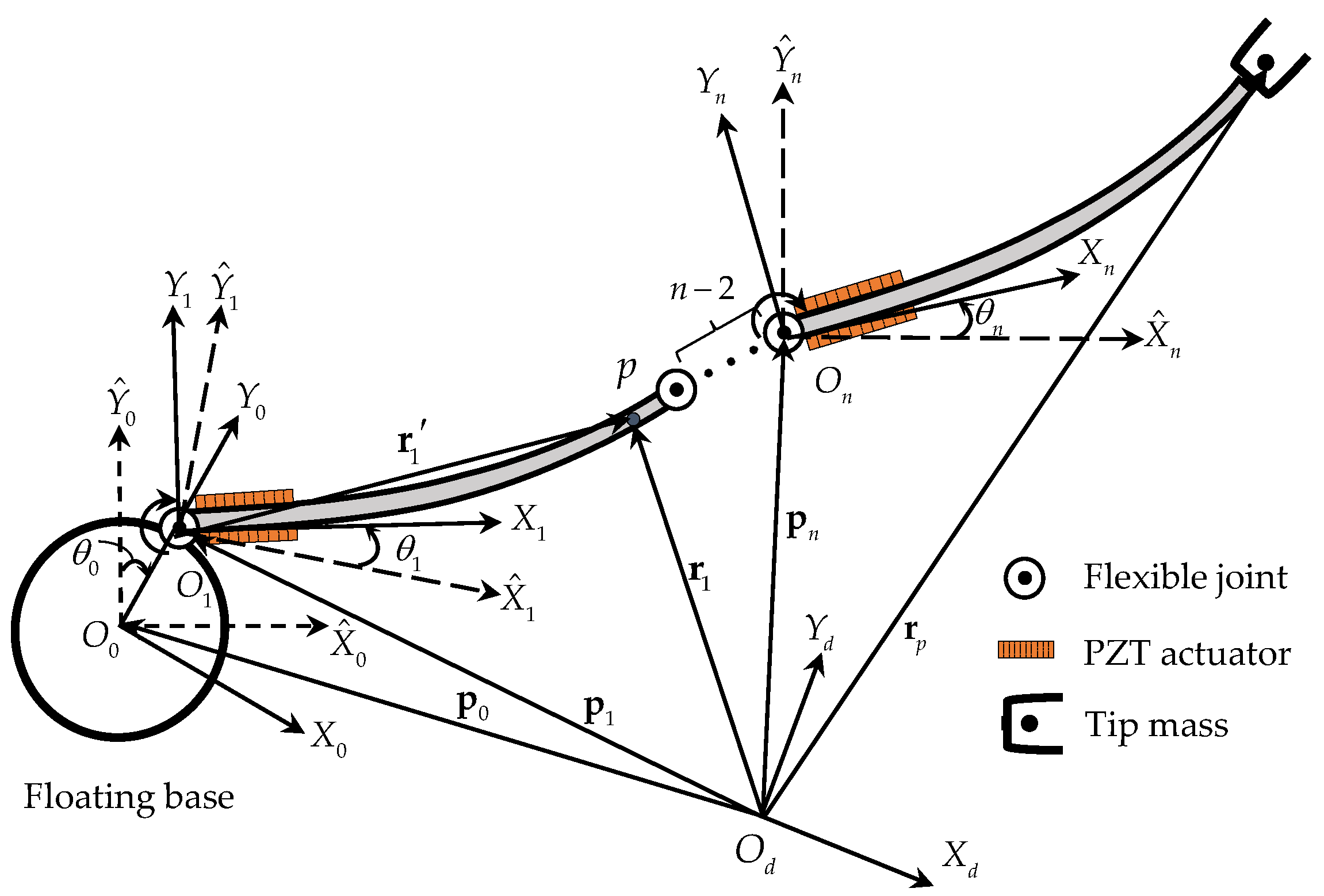

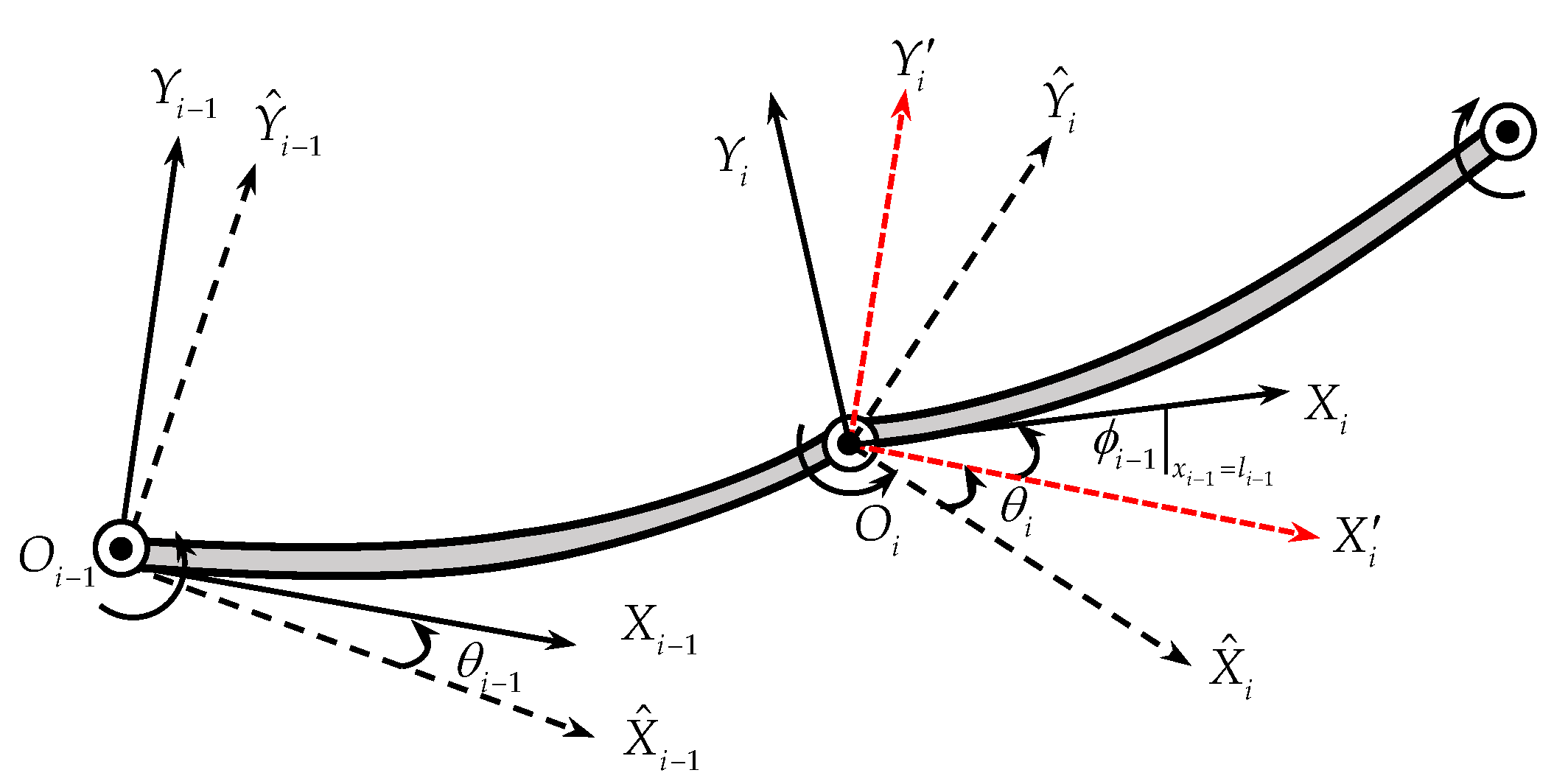









| Symbol | Representation |
|---|---|
| , | Moment of inertia of the base, the ith rotor |
| , | mass of the base, the ith rotor |
| , | length of the base, the ith link |
| The linear density of the ith link | |
| The moment of inertia of the ith link | |
| The elastic modulus of the ith link | |
| The mass of the tip payload | |
| Moment of inertia of tip payload | |
| The ith spring rate coefficient | |
| The theoretical rotation angle of the ith rotor | |
| The actual rotation angle of the ith joint | |
| The theoretical torque of the ith joint | |
| The elastic deformation of the ith joint | |
| , | Base, link frame system |
| Joint frame system | |
| Inertial frame system |
| Symbol | Value | Link1 | Link2 |
|---|---|---|---|
| 17.23 kg | \ | \ | |
| 2.0 kg | \ | \ | |
| 0.087 kg · m2 | \ | \ | |
| 0.005 kg · m2 | \ | \ | |
| , | \ | 72.0 GPa | 72.0 GPa |
| 0.12 m | \ | \ | |
| , | \ | 1.620 kg/m | 1.620 kg/m |
| , | \ | 0.005 kg · m2 | 0.005 kg · m2 |
| , | \ | 500 Nm/rad | 500 Nm/rad |
| , | \ | 4.50 × 10−8 m4 | 4.50 × 10−8 m4 |
| , | \ | 2.0 m | 2.0 m |
| Symbol | Value | Link1 | Link2 |
|---|---|---|---|
| 0.1 kg | \ | \ | |
| 1.25 × 10−6 kg · m2 | \ | \ | |
| , | \ | 200.0 GPa | 200.0 GPa |
| , | \ | 0.5688 kg/m | 0.3160 kg/m |
| , | \ | 0.001 kg · m2 | 0.001 kg · m2 |
| , | \ | 9.6 Nm/rad | 9.6 Nm/rad |
| , | \ | 2.40 × 10−11 m4 | 1.33 × 10−11 m4 |
| , | \ | 0.5 m | 0.25 m |
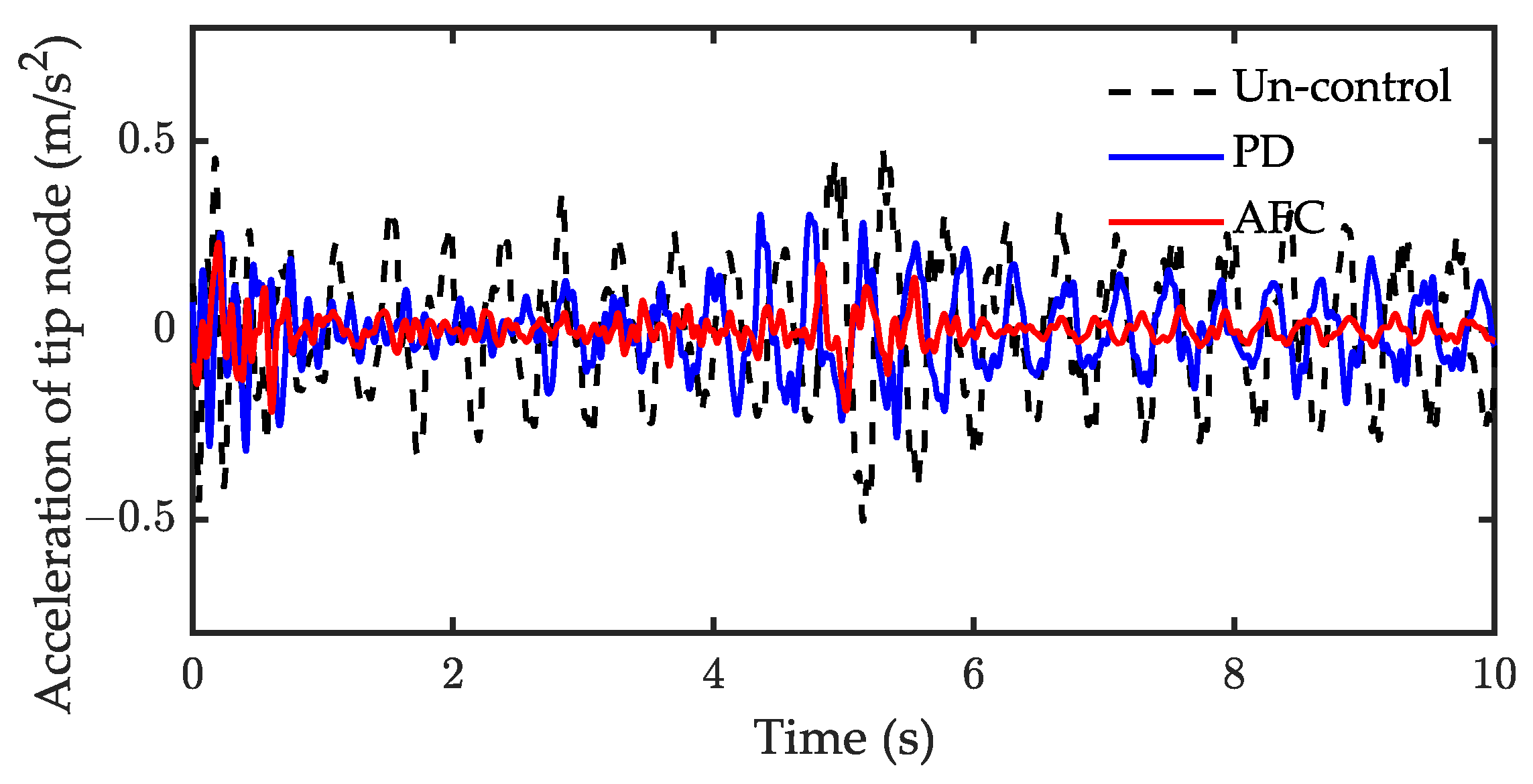
| Controller | Uncontrol | PD | AFC |
|---|---|---|---|
| RMS (m/s2) | 0.1847 | 0.1017 | 0.04 |
| Amplitude (m/s2) | 0.5048 | 0.3186 | 0.2309 |
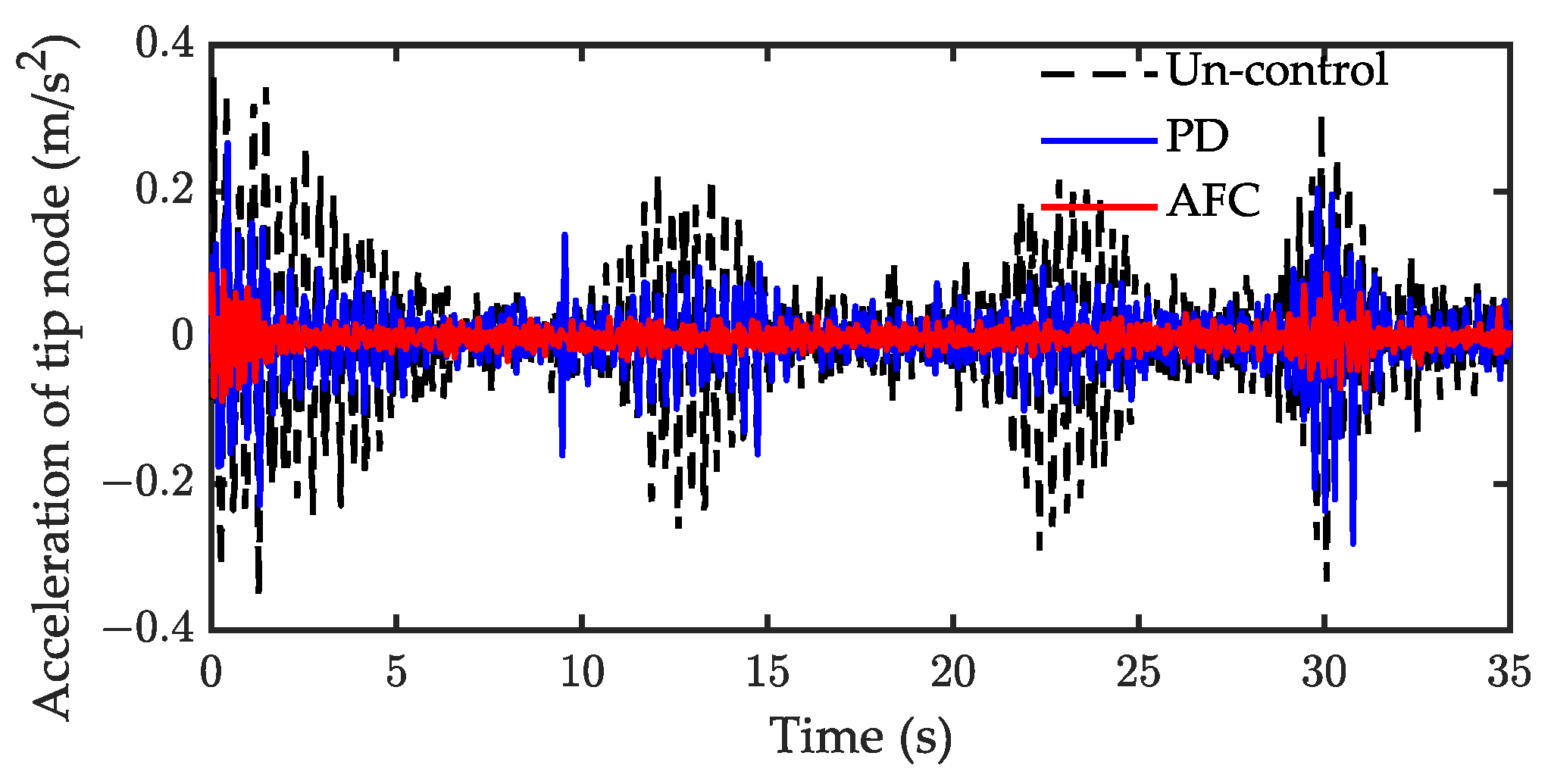
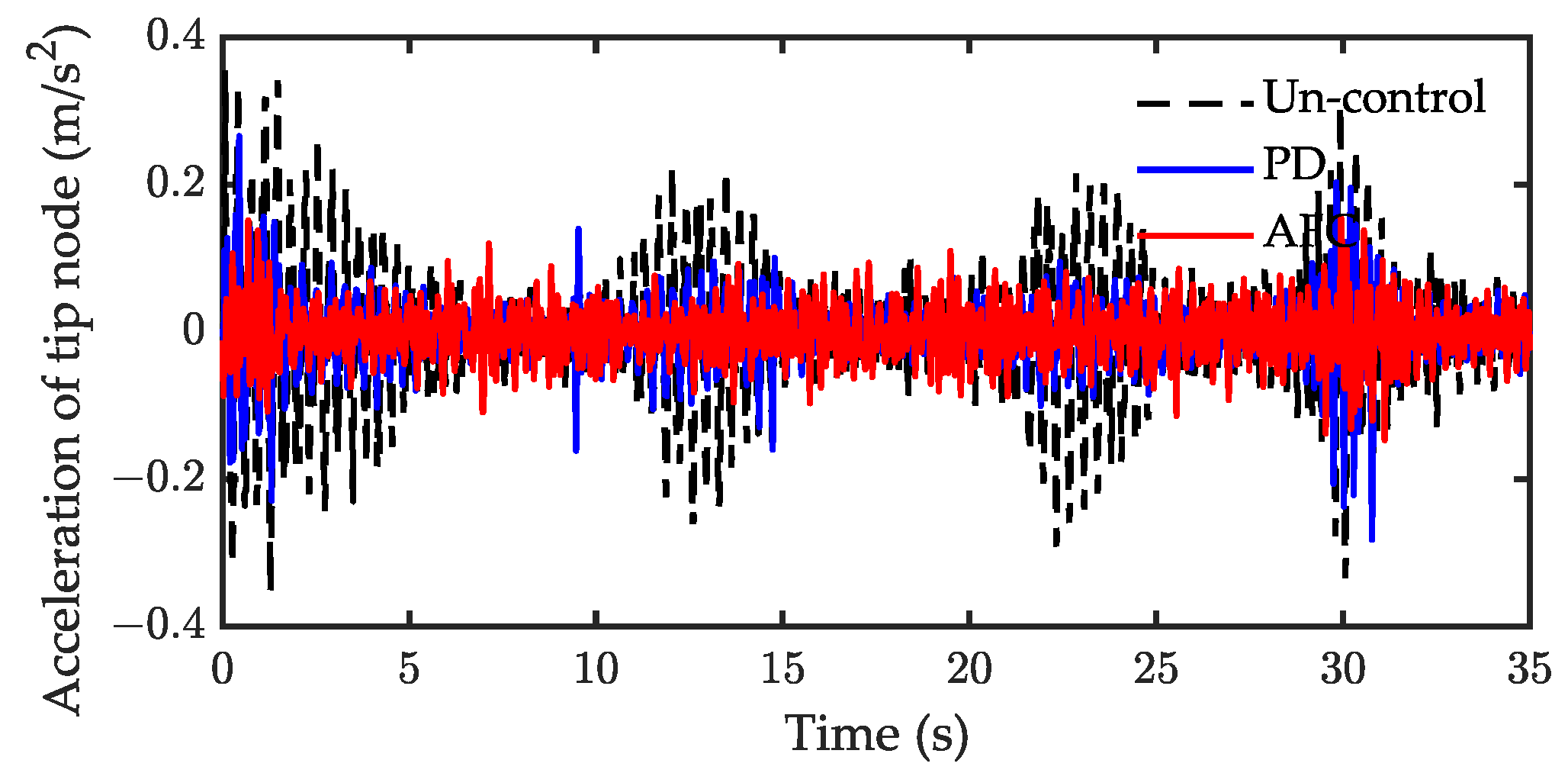
| Controller | Uncontrol | PD | AFC |
|---|---|---|---|
| RMS (m/s2) | 0.0808 | 0.0413 | 0.0303 |
| Amplitude (m/s2) | 0.3500 | 0.2819 | 0.2997 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Feng, C.; Chen, W.; Shao, M.; Ni, S. Trajectory Tracking and Adaptive Fuzzy Vibration Control of Multilink Space Manipulators with Experimental Validation. Actuators 2023, 12, 138. https://doi.org/10.3390/act12040138
Feng C, Chen W, Shao M, Ni S. Trajectory Tracking and Adaptive Fuzzy Vibration Control of Multilink Space Manipulators with Experimental Validation. Actuators. 2023; 12(4):138. https://doi.org/10.3390/act12040138
Chicago/Turabian StyleFeng, Chenlu, Weidong Chen, Minqiang Shao, and Shihao Ni. 2023. "Trajectory Tracking and Adaptive Fuzzy Vibration Control of Multilink Space Manipulators with Experimental Validation" Actuators 12, no. 4: 138. https://doi.org/10.3390/act12040138
APA StyleFeng, C., Chen, W., Shao, M., & Ni, S. (2023). Trajectory Tracking and Adaptive Fuzzy Vibration Control of Multilink Space Manipulators with Experimental Validation. Actuators, 12(4), 138. https://doi.org/10.3390/act12040138





