A Review of Cooperative Actuator and Sensor Systems Based on Dielectric Elastomer Transducers
Abstract
1. Introduction
2. Dielectric Elastomer Transducers
2.1. Dielectric Elastomer Material and Operating Principle
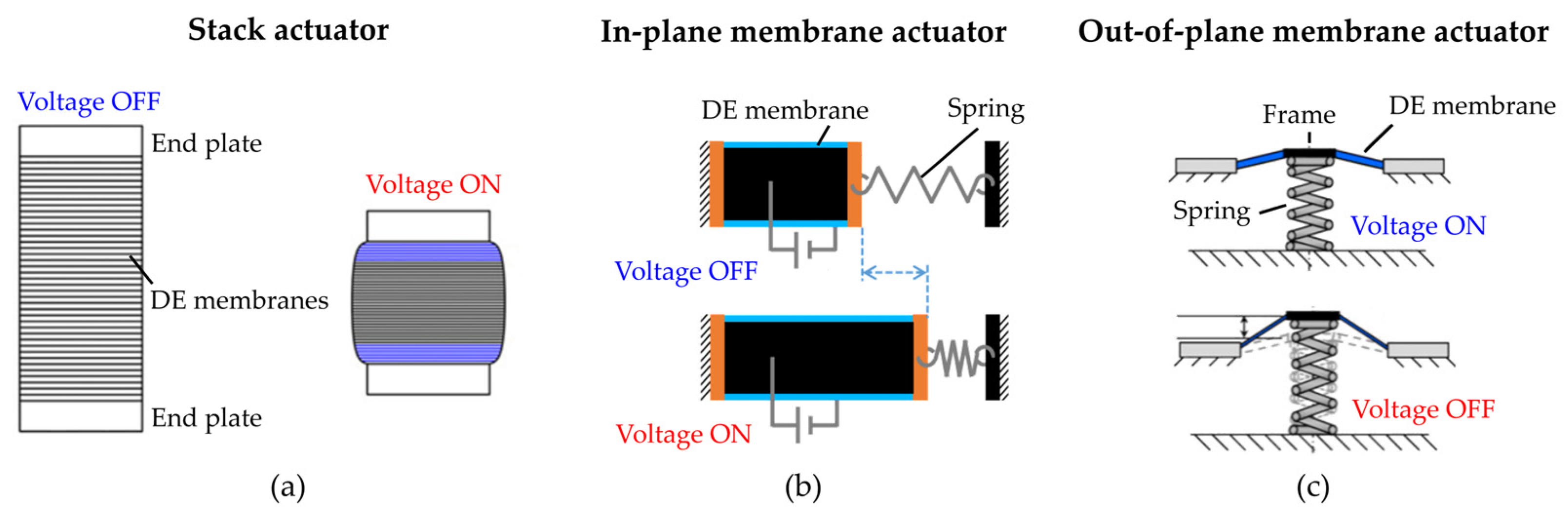
2.2. Dielectric Elastomer Actuators Configurations
3. Dielectric Elastomer Applications in Cooperative Actuator and Sensor Systems
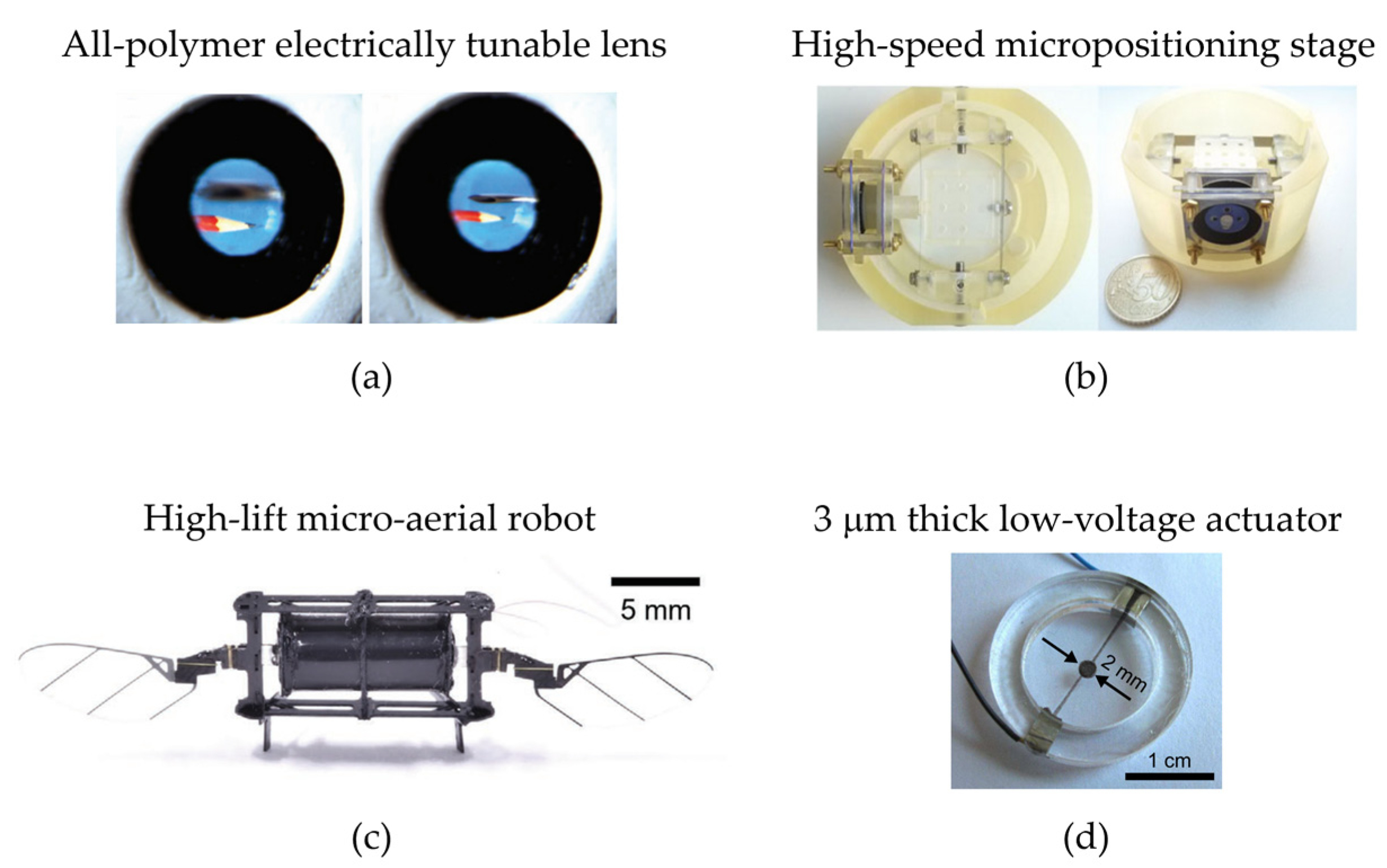
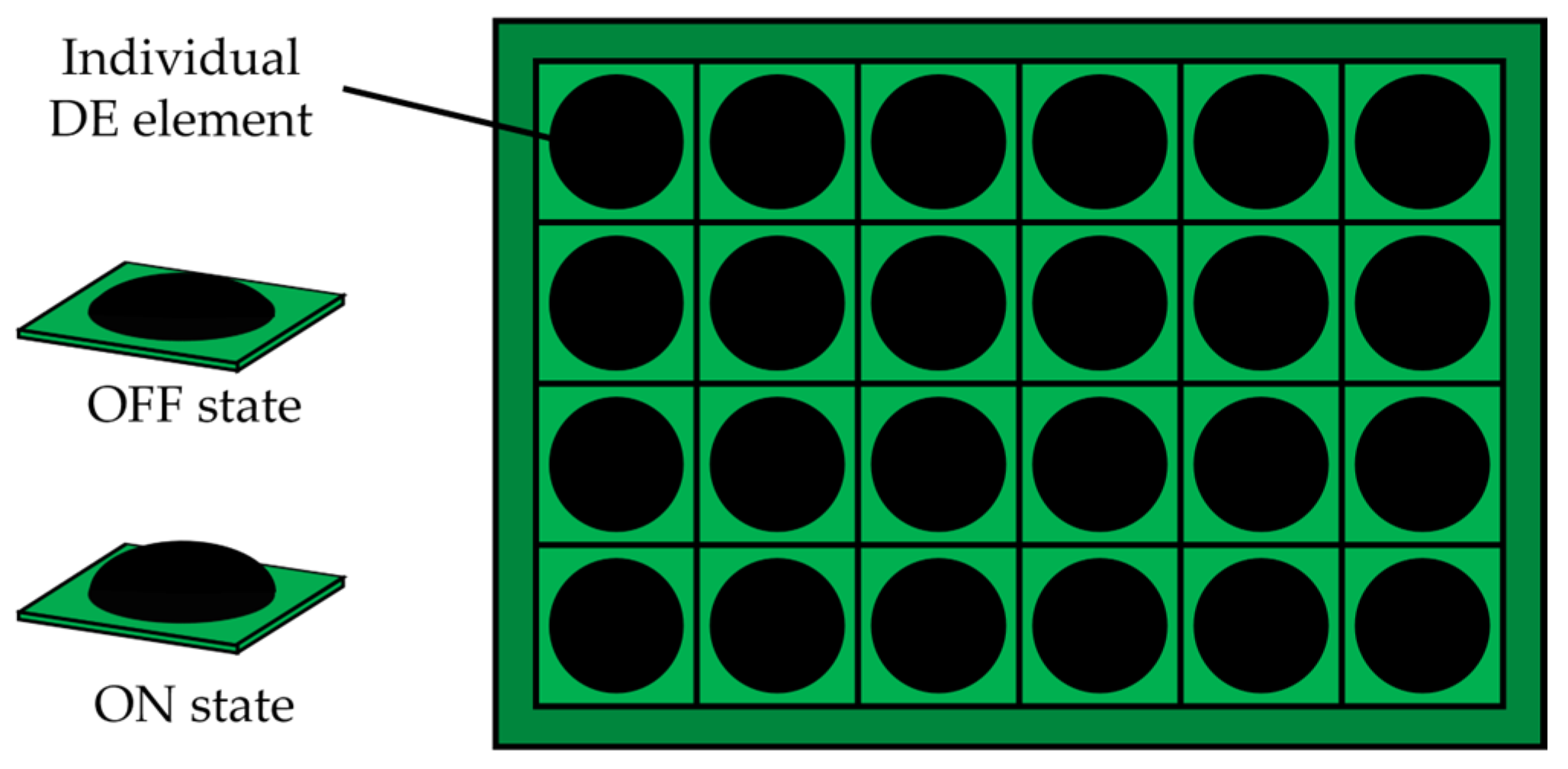
3.1. Towards Meso- and Micro-Scale Dielectric Elastomer Actuators
3.2. Cooperative Dielectric Elastomer Actuators
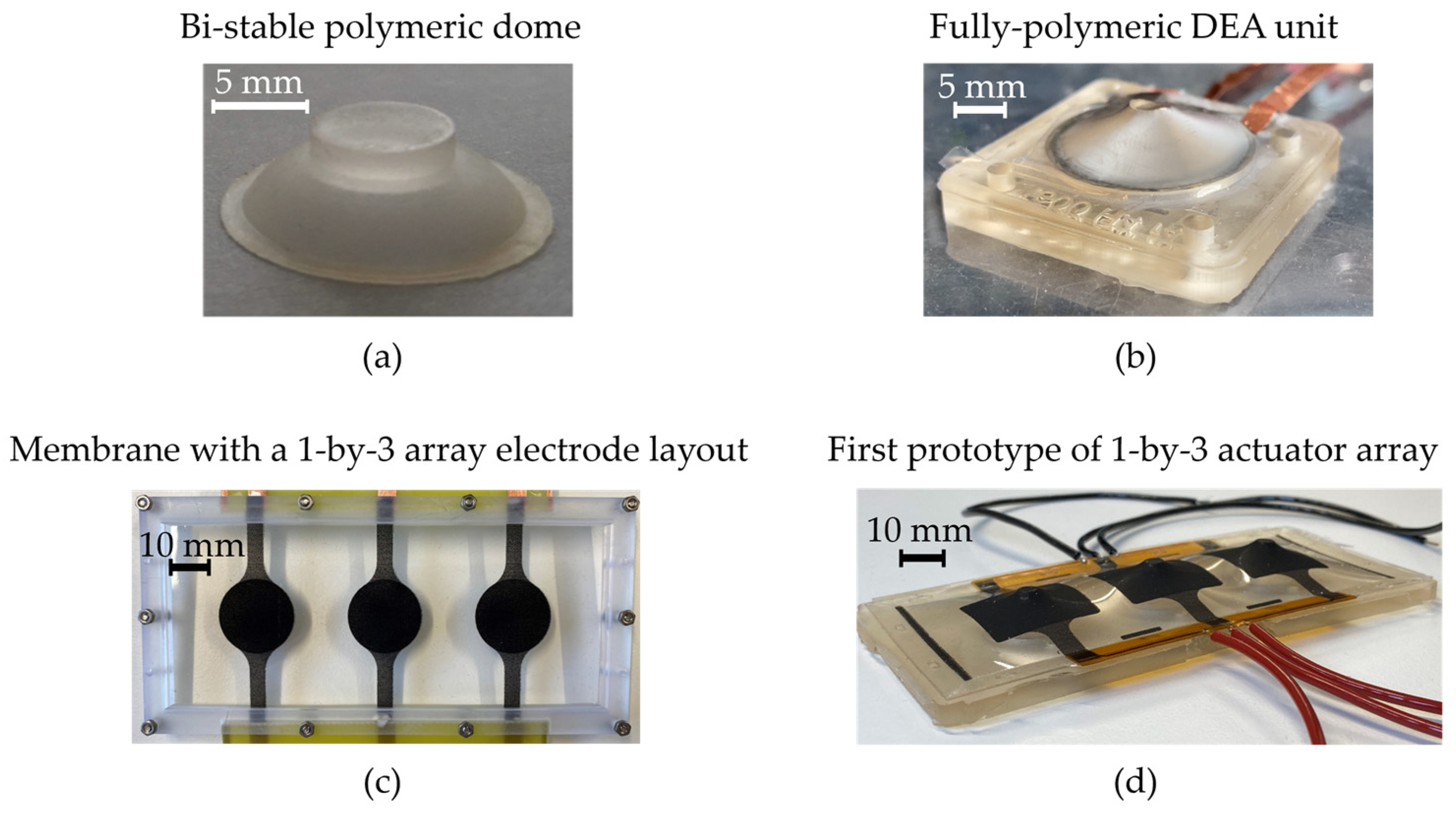
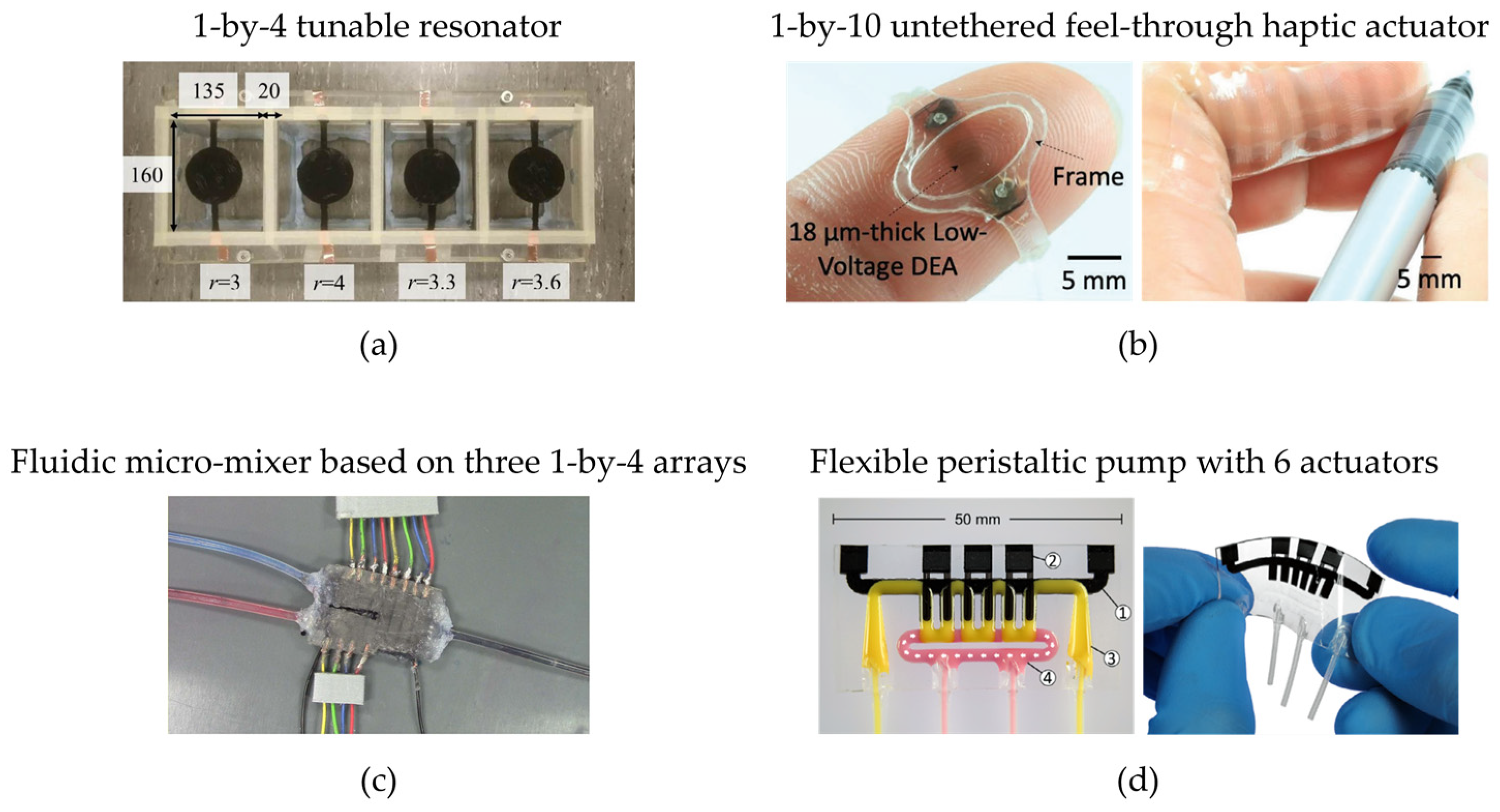
3.2.1. One-Dimensional Arrays
3.2.2. Cooperative Bio-Inspired Robots

3.2.3. Two-Dimensional Arrays for Out-of-Plane Actuation
3.2.4. Two-Dimensional Arrays for in-Plane Actuation
3.2.5. Two-Dimensional Arrays for Haptics and Wearables
3.2.6. Two-Dimensional Arrays for Refreshable Braille Displays
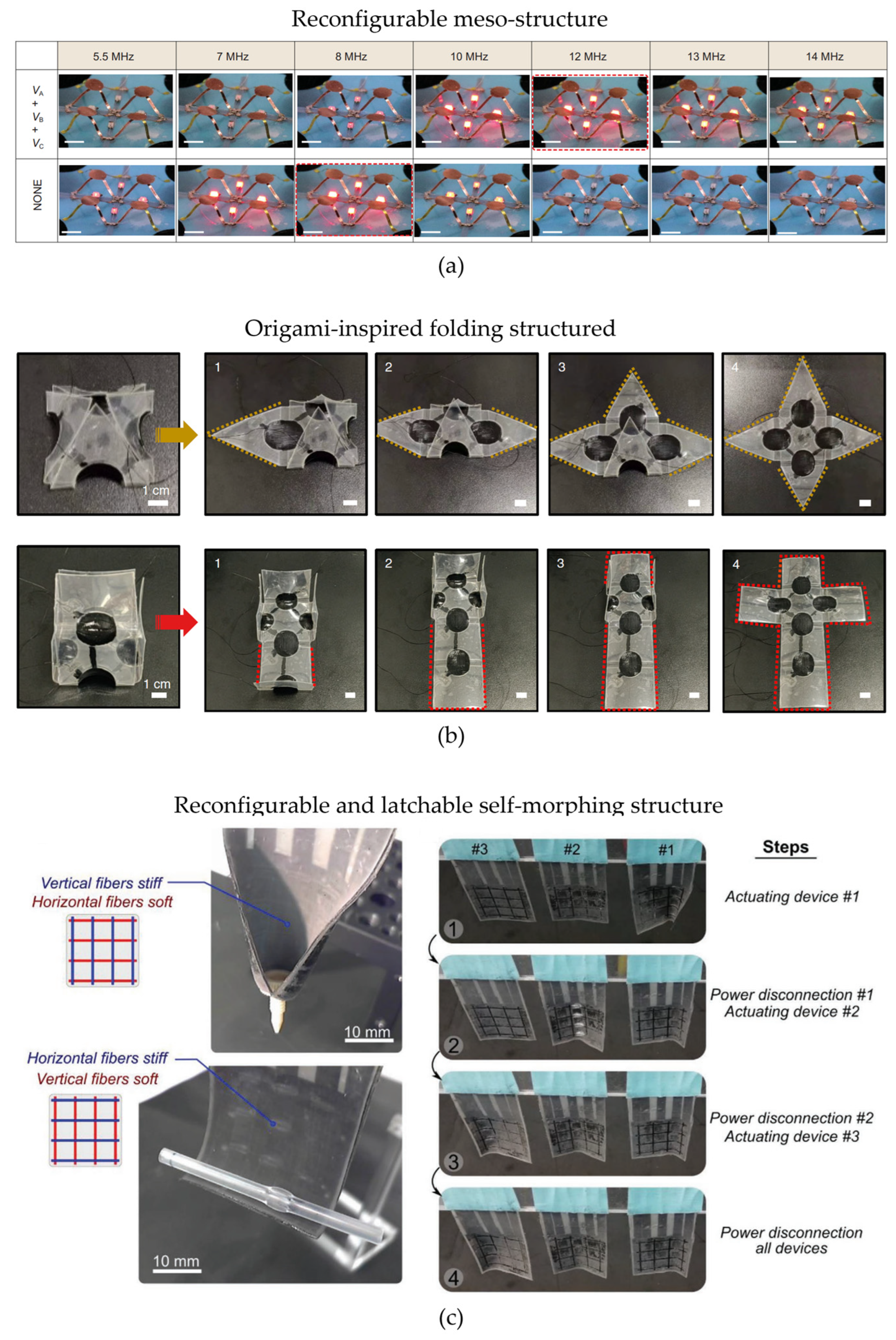
3.2.7. Three-Dimensional Reconfigurable Structures
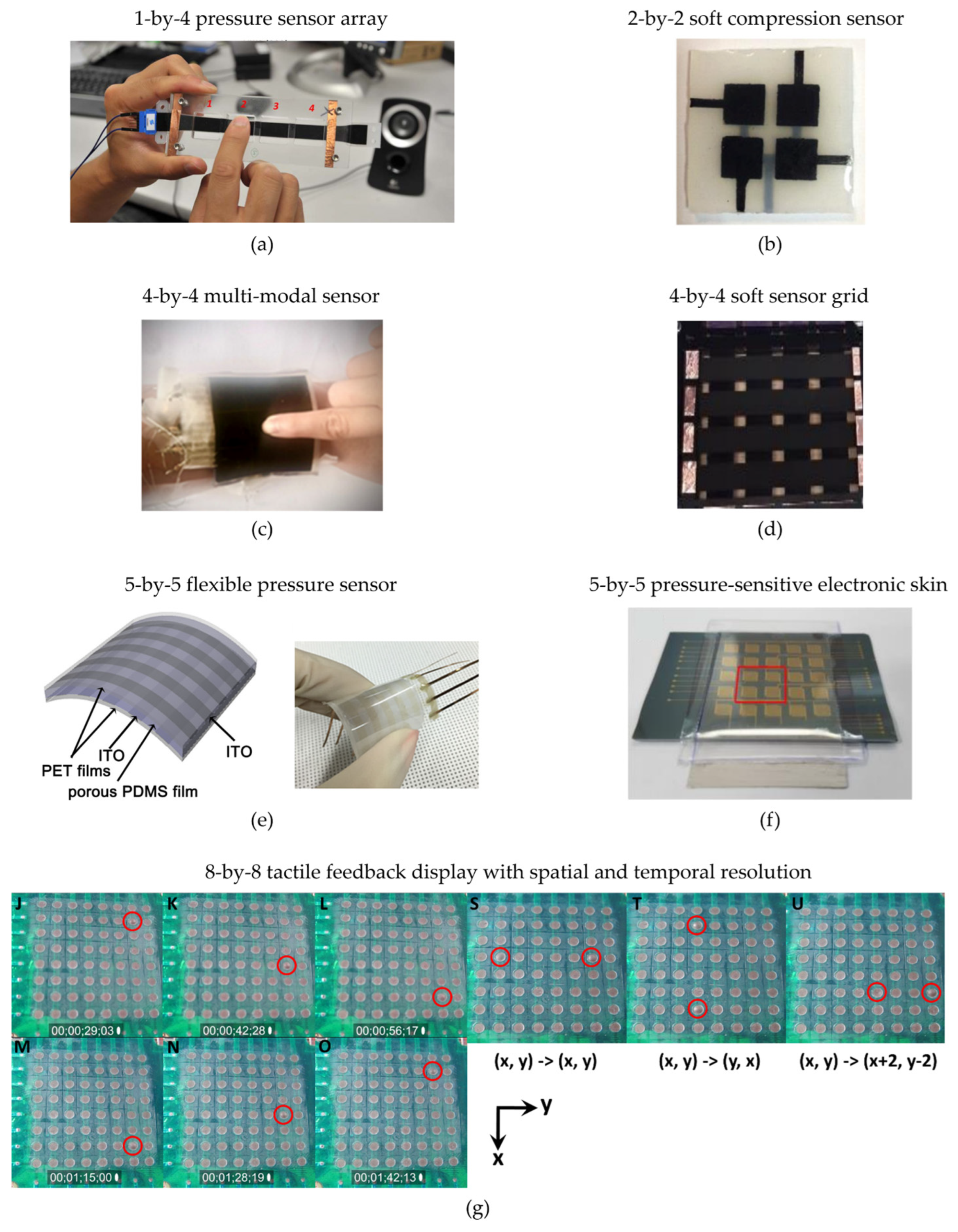
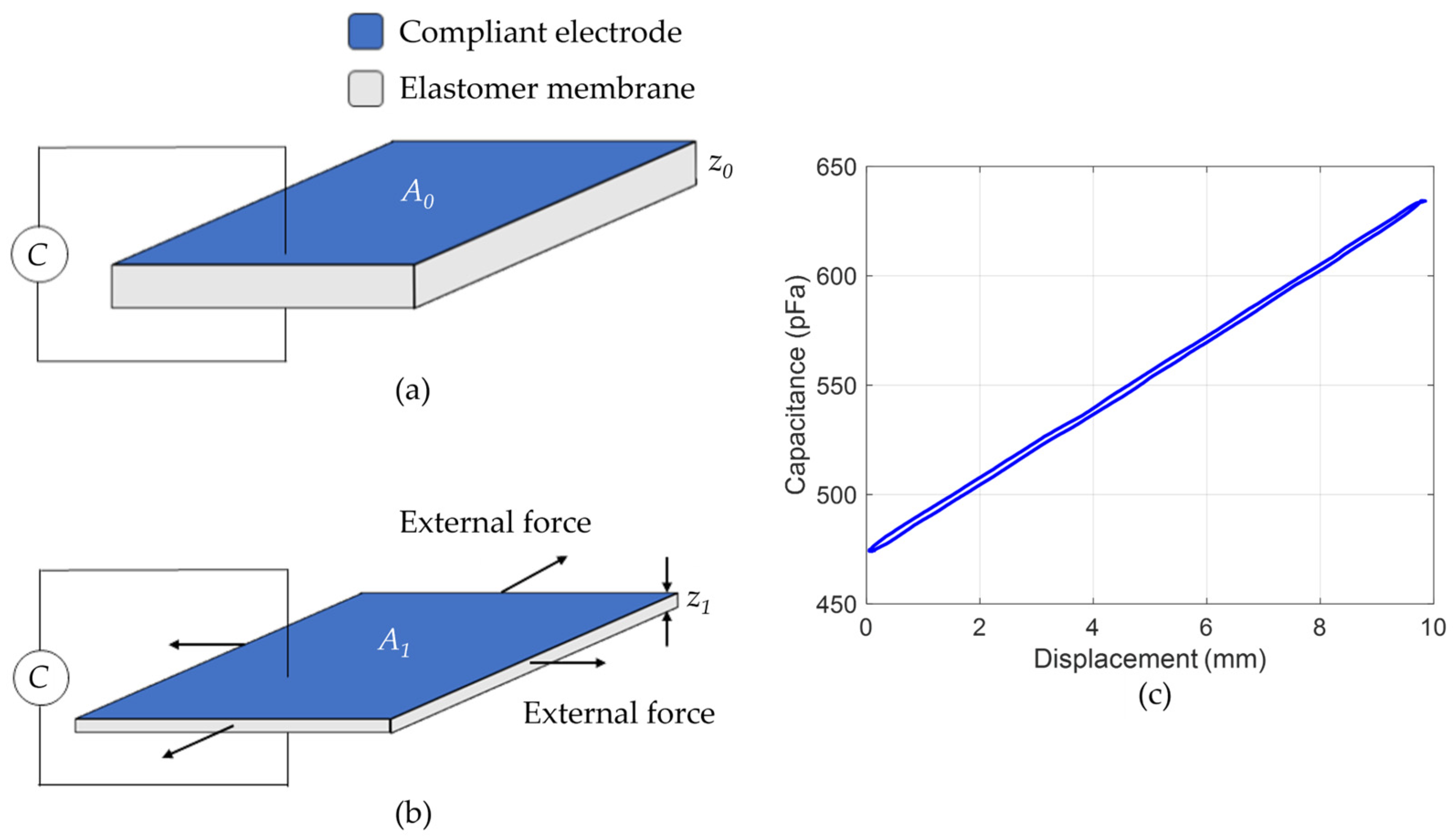
3.3. Cooperative Dielectric Elastomer Sensors
4. Conclusions
Funding
Data Availability Statement
Conflicts of Interest
References
- Butenko, S.; Murphey, R.; Pardalos, P.M. Cooperative Control: Models, Applications and Algorithms; Springer: Berlin/Heidelberg, Germany, 2003. [Google Scholar]
- Wilson, S.A.; Jourdain, R.P.J.; Zhang, Q.; Dorey, R.A.; Bowen, C.R.; Willander, M.; Wahab, Q.U.; Willander, M.; Al-hilli, S.M.; Nur, O.; et al. New materials for micro-scale sensors and actuators: An engineering review. Mater. Sci. Eng. R Rep. 2007, 56, 1–129. [Google Scholar] [CrossRef]
- Gao, J.; Xiao, Y.; Liu, J.; Liang, W.; Chen, C.L.P. A survey of communication/networking in Smart Grids. Futur. Gener. Comput. Syst. 2012, 28, 391–404. [Google Scholar] [CrossRef]
- Chen, F.; Ren, W. On the Control of Multi-Agent Systems: A Survey. Found. Trends® Syst. Control 2019, 6, 339–499. [Google Scholar] [CrossRef]
- Vaccaro, A.; Velotto, G.; Zobaa, A.F. A Decentralized and Cooperative Architecture for Optimal Voltage Regulation in Smart Grids. IEEE Trans. Ind. Electron. 2011, 58, 4593–4602. [Google Scholar] [CrossRef]
- Patwari, N.; Ash, J.N.; Kyperountas, S.; Hero, A.O.; Moses, R.L.; Correal, N.S. Locating the nodes: Cooperative localization in wireless sensor networks. IEEE Signal Process. Mag. 2005, 22, 54–69. [Google Scholar] [CrossRef]
- Lee, J.; Park, B. Development and Evaluation of a Cooperative Vehicle Intersection Control Algorithm Under the Connected Vehicles Environment. IEEE Trans. Intell. Transp. Syst. 2012, 13, 81–90. [Google Scholar] [CrossRef]
- Khamis, A.; Hussein, A.; Elmogy, A. Multi-Robot Task Allocation: A Review of the State-of-the-Art; Springer: Cham, Switzerland, 2015; pp. 31–51. [Google Scholar]
- Chandler, P.R.; Swaroop, D.; Howlett, J.K.; Pachter, M.; Fowler, J.M. Complexity in UAV Cooperative Control. In Proceedings of the 2002 American Control Conference, Anchorage, AK, USA, 8–10 May 2002; pp. 1831–1836. [Google Scholar]
- Laurent, G.J.; Delettre, A.; Zeggari, R.; Yahiaoui, R.; Manceau, J.-F.; Le Fort-Piat, N. Micropositioning and Fast Transport Using a Contactless Micro-Conveyor. Micromachines 2014, 5, 66–80. [Google Scholar] [CrossRef]
- Petit, L.; Hassine, A.; Terrien, J.; Lamarque, F.; Prelle, C. Development of a Control Module for a Digital Electromagnetic Actuators Array. IEEE Trans. Ind. Electron. 2014, 61, 4788–4796. [Google Scholar] [CrossRef]
- Ataka, M.; Legrand, B.; Buchaillot, L.; Collard, D.; Fujita, H. Design, Fabrication, and Operation of Two-Dimensional Conveyance System with Ciliary Actuator Arrays. IEEE/ASME Trans. Mechatron. 2009, 14, 119–125. [Google Scholar] [CrossRef]
- Konishi, S.; Fujita, H. A conveyance system using air flow based on the concept of distributed micro motion systems. J. Microelectromech. Syst. 1994, 3, 54–58. [Google Scholar] [CrossRef]
- Luntz, J.E.; Messner, W.; Choset, H. Distributed Manipulation Using Discrete Actuator Arrays. Int. J. Robot. Res. 2001, 20, 553–583. [Google Scholar] [CrossRef]
- Yahiaoui, R.; Zeggari, R.; Malapert, J.; Manceau, J.-F. A MEMS-based pneumatic micro-conveyor for planar micromanipulation. Mechatronics 2012, 22, 515–521. [Google Scholar] [CrossRef]
- Berlin, A.; Biegelsen, D.; Cheung, P.; Fromherz, M.; Goldberg, D.; Jackson, W.; Preas, B.; Reich, J.; Swartz, L.-E. Motion Control of Planar Objects Using Large-Area Arrays of Mems-Like Distributed Manipulators. Micromechatronics 2000, 1–5. [Google Scholar]
- Vandelli, N.; Wroblewski, D.; Velonis, M.; Bifano, T. Development of a MEMS Microvalve Array for Fluid Flow Control. J. Microelectromech. Syst. 1998, 7, 395–403. [Google Scholar] [CrossRef]
- Tellers, M.C.; Pulskamp, J.S.; Bedair, S.S.; Rudy, R.Q.; Kierzewski, I.M.; Polcawich, R.G.; Bergbreiter, S.E. Piezoelectric actuator array for motion-enabled reconfigurable RF circuits. In Proceedings of the 2015 Transducers-2015 18th International Conference on Solid-State Sensors, Actuators and Microsystems (Transducers), Anchorage, AK, USA, 21–25 June 2015; pp. 819–822. [Google Scholar] [CrossRef]
- El-Baz, D.; Piranda, B.; Bourgeois, J. A Distributed Algorithm for a Reconfigurable Modular Surface. In Proceedings of the 2014 IEEE International Parallel & Distributed Processing Symposium Workshops, Phoenix, AZ, USA, 19–23 May 2014; pp. 1591–1598. [Google Scholar] [CrossRef]
- Bourgeois, J.; Goldstein, S.C. Distributed Intelligent MEMS: Progresses and Perspectives. IEEE Syst. J. 2015, 9, 1057–1068. [Google Scholar] [CrossRef]
- Boutoustous, K.; Laurent, G.J.; Dedu, E.; Matignon, L.; Bourgeois, J.; Le Fort-Piat, N. Distributed Control Architecture for Smart Surfaces. In Proceedings of the 2010 IEEE/RSJ International Conference on Intelligent Robots and Systems, Taipei, Taiwan, 18–22 October 2010; pp. 2018–2024. [Google Scholar]
- Konishi, S.; Fujita, H. System design for cooperative control of a microactuator array. IEEE Trans. Ind. Electron. 1995, 42, 449–454. [Google Scholar] [CrossRef]
- Matignon, L.; Laurent, G.J.; Le Fort-Piat, N.; Chapuis, Y.-A. Designing Decentralized Controllers for Distributed-Air-Jet MEMS-Based Micromanipulators by Reinforcement Learning. J. Intell. Robot. Syst. 2010, 59, 145–166. [Google Scholar] [CrossRef]
- Fukuta, Y.; Chapuis, Y.-A.; Mita, Y.; Fujita, H. Design, Fabrication, and Control of MEMS-Based Actuator Arrays for Air-Flow Distributed Micromanipulation. J. Microelectromech. Syst. 2006, 15, 912–926. [Google Scholar] [CrossRef]
- Amato, M.; De Vittorio, M.; Petroni, S. Advanced MEMS Technologies for Tactile Sensing and Actuation. MEMS Fundam. Technol. Appl. 2013, 351–380. [Google Scholar]
- Chouvardas, V.G.; Miliou, A.N.; Hatalis, M.K. Tactile Display Applications: A State of the Art Survey. In Proceedings of the 2nd Balkan Conference in Informatics, Ohrid, Macedon, 26–28 September 2005; pp. 290–303. [Google Scholar]
- Velázquez, R. Wearable Assistive Devices for the Blind. In Wearable and Autonomous Biomedical Devices and Systems for Smart Environment; Springer: Berlin/Heidelberg, Germany, 2010; pp. 331–349. [Google Scholar]
- Wilhelm, E.; Schwarz, T.; Jaworek, G.; Voigt, A.; Rapp, B.E. Towards Displaying Graphics on a Cheap, Large-Scale Braille Display; Springer: Cham, Switzerland, 2014; pp. 662–669. [Google Scholar]
- Munasinghe, K.C.; Bowatta, B.G.C.T.; Abayarathne, H.Y.R.; Kumararathna, N.; Maduwantha, L.K.A.H.; Arachchige, N.M.P.; Amarasinghe, Y.W.R. New MEMS based micro gripper using SMA for micro level object manipulation and assembling. In Proceedings of the 2016 Moratuwa Engineering Research Conference (MERCon), Moratuwa, Sri Lanka, 5–6 April 2016; pp. 36–41. [Google Scholar]
- Kornbluh, R.D.; Pelrine, R.; Prahlad, H.; Heydt, R. Electroactive Polymers: An Emerging Technology for MEMS; Janson, S.W., Henning, A.K., Eds.; International Society for Optics and Photonics: Bellingham, WA, USA, 2004; Volume 5344, pp. 13–27. [Google Scholar]
- Liu, H.; Jui Tay, C.; Quan, C.; Kobayashi, T.; Lee, C. Piezoelectric MEMS Energy Harvester for Low-Frequency Vibrations With Wideband Operation Range and Steadily Increased Output Power. Artic. J. Microelectromech. Syst. 2011, 20, 1131–1142. [Google Scholar] [CrossRef]
- Dhanalakshmi, K. Demonstration of self-sensing in Shape Memory Alloy actuated gripper. In Proceedings of the IEEE International Symposium on Intelligent Control (ISIC), Hyderabad, India, 28–30 August 2013; pp. 218–222. [Google Scholar]
- Islam, M.N.; Seethaler, R.J. Sensorless Position Control for Piezoelectric Actuators Using A Hybrid Position Observer. IEEE/ASME Trans. Mechatron. 2014, 19, 667–675. [Google Scholar] [CrossRef]
- Rizzello, G.; Naso, D.; York, A.; Seelecke, S. A Self-Sensing Approach for Dielectric Elastomer Actuators Based on Online Estimation Algorithms. IEEE/ASME Trans. Mechatron. 2017, 22, 728–738. [Google Scholar] [CrossRef]
- Mineta, T.; Yanatori, H.; Hiyoshi, K.; Tsuji, K.; Ono, Y.; Abe, K. Tactile display MEMS device with SU8 micro-pin and spring on SMA film actuator array. In Proceedings of the 2017 19th International Conference on Solid-State Sensors, Actuators and Microsystems (TRANSDUCERS), Kaohsiung, Taiwan, 18–22 June 2017; pp. 2031–2034. [Google Scholar]
- Hishinuma, Y.; Yang, E.-H. Piezoelectric Unimorph Microactuator Arrays for Single-Crystal Silicon Continuous-Membrane Deformable Mirror. J. Microelectromech. Syst. 2006, 15, 370–379. [Google Scholar] [CrossRef]
- Besse, N.; Zarate, J.J.; Rosset, S.; Shea, H.R. Flexible haptic display with 768 independently controllable shape memory polymers taxels. In Proceedings of the 2017 19th International Conference on Solid-State Sensors, Actuators and Microsystems (TRANSDUCERS), Kaohsiung, Taiwan, 18–22 June 2017; pp. 323–326. [Google Scholar]
- Richter, A.; Paschew, G. Optoelectrothermic Control of Highly Integrated Polymer-Based MEMS Applied in an Artificial Skin. Adv. Mater. 2009, 21, 979–983. [Google Scholar] [CrossRef]
- Carpi, F.; De Rossi, D.; Kornbluh, R.; Pelrine, R.E.; Sommer-Larsen, P. Dielectric Elastomers as Electromechanical Transducers: Fundamentals, Materials, Devices, Models and Applications of an Emerging Electroactive Polymer Technology; Elsevier: Amsterdam, The Netherlands, 2011. [Google Scholar]
- Hill, M.; Rizzello, G.; Seelecke, S. Development and Experimental Characterization of a Pneumatic Valve Actuated by a Dielectric Elastomer Membrane. Smart Mater. Struct. 2017, 26, 085023. [Google Scholar] [CrossRef]
- Loverich, J.J.; Kanno, I.; Kotera, H. Concepts for a new class of all-polymer micropumps. Lab Chip 2006, 6, 1147–1154. [Google Scholar] [CrossRef]
- Gu, G.-Y.; Zhu, J.; Zhu, L.-M.; Zhu, X. A survey on dielectric elastomer actuators for soft robots. Bioinspir. Biomim. 2017, 12, 011003. [Google Scholar] [CrossRef] [PubMed]
- Guo, Y.; Liu, L.; Liu, Y.; Leng, J. Review of Dielectric Elastomer Actuators and their Applications in Soft Robots. Adv. Intell. Syst. 2021, 3, 2000282. [Google Scholar] [CrossRef]
- Huang, B.; Li, M.; Mei, T.; McCoul, D.; Qin, S.; Zhao, Z.; Zhao, J. Wearable stretch sensors for motion measurement of the wrist joint based on dielectric elastomers. Sensors 2017, 17, 2708. [Google Scholar] [CrossRef]
- Kelley, C.R.; Kauffman, J.L. Towards wearable tremor suppression using dielectric elastomer stack actuators. Smart Mater. Struct. 2020, 30, 025006. [Google Scholar] [CrossRef]
- Kovacs, G.; Lochmatter, P.; Wissler, M. An arm wrestling robot driven by dielectric elastomer actuators. Smart Mater. Struct. 2007, 16, S306–S317. [Google Scholar] [CrossRef]
- Pelrine, R.; Kornbluh, R.D.; Pei, Q.; Stanford, S.; Oh, S.; Eckerle, J.; Full, R.J.; Rosenthal, M.A.; Meijer, K. Smart Structures and Materials. In Proceedings of the SPIE’S 9th Annual International Symposium on Smart Structures and Materials, San Diego, CA, USA, 17–21 March 2002; Volume 4695, pp. 126–137. [Google Scholar]
- Wang, N.F.; Cui, C.Y.; Guo, H.; Chen, B.C.; Zhang, X.M. Advances in dielectric elastomer actuation technology. Sci. China Technol. Sci. 2018, 61, 1512–1527. [Google Scholar] [CrossRef]
- Balakrisnan, B.; Smela, E. Challenges in the Microfabrication of Dielectric Elastomer Actuators; Bar-Cohen, Y., Ed.; International Society for Optics and Photonics: Bellingham, WA, USA, 2010; Volume 7642, pp. 141–150. [Google Scholar]
- Patrick, L.; Gabor, K.; Silvain, M. Characterization of dielectric elastomer actuators based on a hyperelastic film model. Sens. Actuators A Phys. 2007, 135, 748–757. [Google Scholar] [CrossRef]
- York, A.; Dunn, J.; Seelecke, S. Experimental characterization of the hysteretic and rate-dependent electromechanical behavior of dielectric electro-active polymer actuators. Smart Mater. Struct. 2010, 19, 094014. [Google Scholar] [CrossRef]
- Kaltseis, R.; Keplinger, C.; Koh, S.J.A.; Baumgartner, R.; Goh, Y.F.; Ng, W.H.; Kogler, A.; Tröls, A.; Foo, C.C.; Suo, Z.; et al. Natural rubber for sustainable high-power electrical energy generation. RSC Adv. 2014, 4, 27905–27913. [Google Scholar] [CrossRef]
- Chen, Y.; Agostini, L.; Moretti, G.; Fontana, M.; Vertechy, R. Dielectric elastomer materials for large-strain actuation and energy harvesting: A comparison between styrenic rubber, natural rubber and acrylic elastomer. Smart Mater. Struct. 2019, 28, 114001. [Google Scholar] [CrossRef]
- Chen, T.; Qiu, J.; Zhu, K.; Li, J. Electro-mechanical performance of polyurethane dielectric elastomer flexible micro-actuator composite modified with titanium dioxide-graphene hybrid fillers. Mater. Des. 2016, 90, 1069–1076. [Google Scholar] [CrossRef]
- Fasolt, B.; Hodgins, M.; Rizzello, G.; Seelecke, S. Effect of screen printing parameters on sensor and actuator performance of dielectric elastomer (DE) membranes. Sens. Actuators A Phys. 2017, 265, 10–19. [Google Scholar] [CrossRef]
- Rosset, S.; Shea, H.R. Flexible and stretchable electrodes for dielectric elastomer actuators. Appl. Phys. A 2012, 110, 281–307. [Google Scholar] [CrossRef]
- Röntgen, W.C. Ueber die durch Electricität bewirkten Form- und Volumenänderungen von dielectrischen Körpern. Ann. Der Phys. Und Chem. 1880, 247, 771–786. [Google Scholar] [CrossRef]
- Pelrine, R.; Kornbluh, R.; Joseph, J. Electrostriction of polymer dielectrics with compliant electrodes as a means of actuation. Sens. Actuators A Phys. 1998, 64, 77–85. [Google Scholar] [CrossRef]
- Carpi, F.; Anderson, I.; Bauer, S.; Frediani, G.; Gallone, G.; Gei, M.; Graaf, C.; Jean-Mistral, C.; Kaal, W.; Kofod, G.; et al. Standards for dielectric elastomer transducers. Smart Mater. Struct. 2015, 24, 105025. [Google Scholar] [CrossRef]
- Rizzello, G.; Loew, P.; Agostini, L.; Fontana, M.; Seelecke, S. A lumped parameter model for strip-shaped dielectric elastomer membrane transducers with arbitrary aspect ratio. Smart Mater. Struct. 2020, 29, 115030. [Google Scholar] [CrossRef]
- Shian, S.; Huang, J.; Zhu, S.; Clarke, D.R. Optimizing the Electrical Energy Conversion Cycle of Dielectric Elastomer Generators. Adv. Mater. 2014, 26, 6617–6621. [Google Scholar] [CrossRef]
- Michel, S.; Zhang, X.Q.; Wissler, M.; Löwe, C.; Kovacs, G. A comparison between silicone and acrylic elastomers as dielectric materials in electroactive polymer actuators. Polym. Int. 2009, 59, 391–399. [Google Scholar] [CrossRef]
- Chen, Y.; Agostini, L.; Moretti, G.; Berselli, G.; Fontana, M.; Vertechy, R. Fatigue life performances of silicone elastomer membranes for dielectric elastomer transducers: Preliminary results. In Proceedings of the SPIE-The International Society for Optical Engineering, Denver, CO, USA, 3–7 March 2019; Volume 10966, pp. 158–167. [Google Scholar]
- Youn, J.-H.; Jeong, S.M.; Hwang, G.; Kim, H.; Hyeon, K.; Park, J.; Kyung, K.-U. Dielectric Elastomer Actuator for Soft Robotics Applications and Challenges. Appl. Sci. 2020, 10, 640. [Google Scholar] [CrossRef]
- Pelrine, R.; Kornbluh, R.; Joseph, J.; Heydt, R.; Pei, Q.; Chiba, S. High-field deformation of elastomeric dielectrics for actuators. Mater. Sci. Eng. C 2000, 11, 89–100. [Google Scholar] [CrossRef]
- Romasanta, L.J.; Lopez-Manchado, M.A.; Verdejo, R. Increasing the performance of dielectric elastomer actuators: A review from the materials perspective. Prog. Polym. Sci. 2015, 51, 188–211. [Google Scholar] [CrossRef]
- Hodgins, M.; Seelecke, S. Systematic experimental study of pure shear type dielectric elastomer membranes with different electrode and film thicknesses. Smart Mater. Struct. 2016, 25, 095001. [Google Scholar] [CrossRef]
- Schlatter, S.; Rosset, S.; Shea, H. Inkjet Printing of Carbon Black Electrodes for Dielectric Elastomer Actuators. In SPIE Electroactive Polymer Actuators and Devices; SPIE: Bellingham, WA, USA, 2017; Volume 10163, pp. 177–185. [Google Scholar]
- Klug, F.; Solano-Arana, S.; Hoffmann, N.J.; Schlaak, H.F. Multilayer dielectric elastomer tubular transducers for soft robotic applications. Smart Mater. Struct. 2019, 28, 104004. [Google Scholar] [CrossRef]
- Hubertus, J.; Neu, J.; Croce, S.; Rizzello, G.; Seelecke, S.; Schultes, G. Nanoscale Nickel-Based Thin Films as Highly Conductive Electrodes for Dielectric Elastomer Applications with Extremely High Stretchability up to 200%. ACS Appl. Mater. Interfaces 2021, 13, 39894–39904. [Google Scholar] [CrossRef] [PubMed]
- Hubertus, J.; Croce, S.; Neu, J.; Rizzello, G.; Seelecke, S.; Schultes, G. Electromechanical characterization and laser structuring of Ni-based sputtered metallic compliant electrodes for DE applications. In Proceedings of the International Conference and Exhibition on New Actuator Systems and Applications, online, 17–19 February 2021; pp. 321–324. [Google Scholar]
- Jung, K.; Kim, K.J.; Choi, H.R. A self-sensing dielectric elastomer actuator. Sens. Actuators A Phys. 2008, 143, 343–351. [Google Scholar] [CrossRef]
- Gisby, T.A.; O’Brien, B.M.; Anderson, I.A. Self sensing feedback for dielectric elastomer actuators. Appl. Phys. Lett. 2013, 102, 193703. [Google Scholar] [CrossRef]
- Hoffstadt, T.; Griese, M.; Maas, J. Online identification algorithms for integrated dielectric electroactive polymer sensors and self-sensing concepts. Smart Mater. Struct. 2014, 23, 104007. [Google Scholar] [CrossRef]
- Rizzello, G.; Fugaro, F.; Naso, D.; Seelecke, S. Simultaneous Self-Sensing of Displacement and Force for Soft Dielectric Elastomer Actuators. IEEE Robot. Autom. Lett. 2018, 3, 1230–1236. [Google Scholar] [CrossRef]
- Rizzello, G.; Naso, D.; York, A.; Seelecke, S. Closed loop control of dielectric elastomer actuators based on self-sensing displacement feedback. Smart Mater. Struct. 2016, 25, 035034. [Google Scholar] [CrossRef]
- Hoffstadt, T.; Maas, J. Sensorless force control for dielectric elastomer transducers. J. Intell. Mater. Syst. Struct. 2018, 30, 1419–1434. [Google Scholar] [CrossRef]
- Rizzello, G.; Serafino, P.; Naso, D.; Seelecke, S. Towards Sensorless Soft Robotics: Self-Sensing Stiffness Control of Dielectric Elastomer Actuators. IEEE Trans. Robot. 2020, 36, 174–188. [Google Scholar] [CrossRef]
- Gatti, D.; Haus, H.; Matysek, M.; Frohnapfel, B.; Tropea, C.; Schlaak, H.F. The dielectric breakdown limit of silicone dielectric elastomer actuators. Appl. Phys. Lett. 2014, 104, 052905. [Google Scholar] [CrossRef]
- Fasolt, B.; Welsch, F.; Jank, M.; Seelecke, S. Effect of actuation parameters and environment on the breakdown voltage of silicone dielectric elastomer films. Smart Mater. Struct. 2019, 28, 094002. [Google Scholar] [CrossRef]
- Suo, Z. Theory of dielectric elastomers. Acta Mech. Solida Sin. 2010, 23, 549–578. [Google Scholar] [CrossRef]
- Croce, S.; Neu, J.; Moretti, G.; Hubertus, J.; Schultes, G.; Rizzello, G. Finite element modeling and validation of a soft array of spatially coupled dielectric elastomer transducers. Smart Mater. Struct. 2022, 31, 084001. [Google Scholar] [CrossRef]
- Hajiesmaili, E.; Clarke, D.R. Dielectric elastomer actuators. J. Appl. Phys. 2021, 129, 151102. [Google Scholar] [CrossRef]
- Kovacs, G.; Düring, L.; Michel, S.; Terrasi, G. Stacked dielectric elastomer actuator for tensile force transmission. Sens. Actuators A Phys. 2009, 155, 299–307. [Google Scholar] [CrossRef]
- Maas, J.; Tepel, D.; Hoffstadt, T. Actuator design and automated manufacturing process for DEAP-based multilayer stack-actuators. Meccanica 2015, 50, 2839–2854. [Google Scholar] [CrossRef]
- Hau, S.; Bruch, D.; Rizzello, G.; Motzki, P.; Seelecke, S. Silicone based dielectric elastomer strip actuators coupled with nonlinear biasing elements for large actuation strains. Smart Mater. Struct. 2018, 27, 074003. [Google Scholar] [CrossRef]
- Kwak, J.W.; Chi, H.J.:; Jung, K.M.; Koo, J.C.; Jeon, J.W.; Lee, Y.; Nam, J.-D.; Ryew, Y.; Choi, H.-R. A Face Robot Actuated With Artificial Muscle Based on Dielectric Elastomer. J. Mech. Sci. Technol. 2005, 19, 578–588. [Google Scholar] [CrossRef]
- Kunze, J.; Prechtl, J.; Bruch, D.; Fasolt, B.; Nalbach, S.; Motzki, P.; Seelecke, S.; Rizzello, G. Design, Manufacturing, and Characterization of Thin, Core-Free, Rolled Dielectric Elastomer Actuators. Actuators 2021, 10, 69. [Google Scholar] [CrossRef]
- Rajamani, A.; Grissom, M.; Rahn, C.; Ma, Y.; Zhang, Q. Wound roll dielectric elastomer actuators: Fabrication, analysis and experiments. In Proceedings of the 2005 IEEE/RSJ International Conference on Intelligent Robots and Systems, IROS, Edmonton, AB, Canada, 2–6 August 2005; Volume 13, pp. 2587–2592. [Google Scholar]
- Moretti, G.; Sarina, L.; Agostini, L.; Vertechy, R.; Berselli, G.; Fontana, M. Styrenic-rubber dielectric elastomer actuator with inherent stiffness compensation. Actuators 2020, 9, 44. [Google Scholar] [CrossRef]
- Follador, M.; Cianchetti, M.; Mazzolai, B. Design of a compact bistable mechanism based on dielectric elastomer actuators. Meccanica 2015, 50, 2741–2749. [Google Scholar] [CrossRef]
- Berselli, G.; Vertechy, R.; Vassura, G.; Parenti-Castelli, V. Optimal Synthesis of Conically Shaped Dielectric Elastomer Linear Actuators: Design Methodology and Experimental Validation. IEEE/ASME Trans. Mechatron. 2011, 16, 67–79. [Google Scholar] [CrossRef]
- Rizzello, G.; Hodgins, M.; Naso, D.; York, A.; Seelecke, S. Modeling of the effects of the electrical dynamics on the electromechanical response of a DEAP circular actuator with a mass-spring load. Smart Mater. Struct. 2015, 24, 094003. [Google Scholar] [CrossRef]
- Nalbach, S.; Banda, R.M.; Croce, S.; Rizzello, G.; Naso, D.; Seelecke, S. Modeling and Design Optimization of a Rotational Soft Robotic System Driven by Double Cone Dielectric Elastomer Actuators. Front. Robot. AI 2020, 6, 150. [Google Scholar] [CrossRef] [PubMed]
- Cao, C.; Chen, L.; Li, B.; Chen, G.; Nie, Z.; Wang, L.; Gao, X. Toward broad optimal output bandwidth dielectric elastomer actuators. Sci. China Technol. Sci. 2022, 65, 1137–1148. [Google Scholar] [CrossRef]
- Kofod, G.; Wirges, W.; Paajanen, M.; Bauer, S. Energy minimization for self-organized structure formation and actuation. Appl. Phys. Lett. 2007, 90, 081916. [Google Scholar] [CrossRef]
- McGough, K.; Ahmed, S.; Frecker, M.; Ounaies, Z. Finite element analysis and validation of dielectric elastomer actuators used for active origami. Smart Mater. Struct. 2014, 23, 094002. [Google Scholar] [CrossRef]
- Hau, S.; Rizzello, G.; Seelecke, S. A novel dielectric elastomer membrane actuator concept for high-force applications. Extrem. Mech. Lett. 2018, 23, 24–28. [Google Scholar] [CrossRef]
- Hodgins, M.; York, A.; Seelecke, S. Experimental comparison of bias elements for out-of-plane DEAP actuator system. Smart Mater. Struct. 2013, 22, 094016. [Google Scholar] [CrossRef]
- Wang, Y.; Zhu, J. Artificial muscles for jaw movements. Extrem. Mech. Lett. 2016, 6, 88–95. [Google Scholar] [CrossRef]
- Zhang, R.; Lochmatter, P.; Kunz, A.; Kovacs, G. Spring roll dielectric elastomer actuators for a portable force feedback glove. In Proceedings of the Smart Structures and Materials 2006: Electroactive Polymer Actuators and Devices (EAPAD), San Diego, CA, USA, 26 February–2 March 2006; Volume 6168, pp. 505–516. [Google Scholar]
- He, T.; Cui, L.; Chen, C.; Suo, Z. Nonlinear deformation analysis of a dielectric elastomer membrane–spring system. Smart Mater. Struct. 2010, 19, 085017. [Google Scholar] [CrossRef]
- Keplinger, C.; Li, T.; Baumgartner, R.; Suo, Z.; Bauer, S. Harnessing snap-through instability in soft dielectrics to achieve giant voltage-triggered deformation. Soft Matter 2012, 8, 285. [Google Scholar] [CrossRef]
- Li, T.; Keplinger, C.; Baumgartner, R.; Bauer, S.; Yang, W.; Suo, Z. Giant voltage-induced deformation in dielectric elastomers near the verge of snap-through instability. J. Mech. Phys. Solids 2013, 61, 611–628. [Google Scholar] [CrossRef]
- Jordi, C.; Michel, S.; Kovacs, G.; Ermanni, P. Scaling of planar dielectric elastomer actuators in an agonist-antagonist configuration. Sens. Actuators A Phys. 2010, 161, 182–190. [Google Scholar] [CrossRef]
- Carpi, F.; Frediani, G.; De Rossi, D. Hydrostatically Coupled Dielectric Elastomer Actuators. IEEE/ASME Trans. Mechatron. 2010, 15, 308–315. [Google Scholar] [CrossRef]
- Loew, P.; Rizzello, G.; Seelecke, S. A novel biasing mechanism for circular out-of-plane dielectric actuators based on permanent magnets. Mechatronics 2018, 56, 48–57. [Google Scholar] [CrossRef]
- Cao, C.; Chen, L.; Hill, T.L.; Wang, L.; Gao, X. Exploiting Bistability for High-Performance Dielectric Elastomer Resonators. IEEE/ASME Trans. Mechatron. 2022, 27, 5994–6005. [Google Scholar] [CrossRef]
- Neu, J.; Hubertus, J.; Croce, S.; Schultes, G.; Seelecke, S.; Rizzello, G. Fully Polymeric Domes as High-Stroke Biasing System for Soft Dielectric Elastomer Actuators. Front. Robot. AI 2021, 8, 695918. [Google Scholar] [CrossRef]
- Dubois, P.; Rosset, S.; Koster, S.; Stauffer, J.; Mikhaïlovc, S.; Dadras, M.; de Rooij, N.-F.; Shea, H. Microactuators based on ion implanted dielectric electroactive polymer (EAP) membranes. Sens. Actuators A Phys. 2006, 131, 147–154. [Google Scholar] [CrossRef]
- Marette, A.; Poulin, A.; Besse, N.; Rosset, S.; Briand, D.; Shea, H. Thin Film Transistors: Flexible Zinc-Tin Oxide Thin Film Transistors Operating at 1 kV for Integrated Switching of Dielectric Elastomer Actuators Arrays. Adv. Mater. 2017, 29, 1700880. [Google Scholar] [CrossRef]
- Poulin, A.; Rosset, S.; Shea, H. Fully printed 3 microns thick dielectric elastomer actuator. In Proceedings of the SPIE Smart Structures and Materials + Nondestructive Evaluation and Health Monitoring, Las Vegas, NV, USA, 20–24 March 2016; Volume 9798, pp. 36–46. [Google Scholar]
- Lotz, P.; Matysek, M.; Schlaak, H.F. Fabrication and Application of Miniaturized Dielectric Elastomer Stack Actuators. IEEE/ASME Trans. Mechatron. 2011, 16, 58–66. [Google Scholar] [CrossRef]
- Wang, K.; Ouyang, G.; Chen, X.; Jakobsen, H. Engineering Electroactive Dielectric Elastomers for Miniature Electromechanical Transducers. Polym. Rev. 2017, 57, 369–396. [Google Scholar] [CrossRef]
- Carpi, F.; Frediani, G.; Turco, S.; De Rossi, D. Bioinspired Tunable Lens with Muscle-Like Electroactive Elastomers. Adv. Funct. Mater. 2011, 21, 4152–4158. [Google Scholar] [CrossRef]
- Carpi, F.; Frediani, G.; Tarantino, S.; De Rossi, D. Millimetre-scale bubble-like dielectric elastomer actuators. Polym. Int. 2009, 59, 407–414. [Google Scholar] [CrossRef]
- Kim, U.; Kang, J.; Lee, C.; Kwon, H.Y.; Hwang, S.; Moon, H.; Koo, J.C.; Nam, J.-D.; Hong, B.H.; Choi, J.-B.; et al. A transparent and stretchable graphene-based actuator for tactile display. Nanotechnology 2013, 24, 145501. [Google Scholar] [CrossRef]
- Hau, S.; Rizzello, G.; Hodgins, M.; York, A.; Seelecke, S. Design and control of a high-speed positioning system based on dielectric elastomer membrane actuators. IEEE/ASME Trans. Mechatron. 2017, 22, 1259–1267. [Google Scholar] [CrossRef]
- Soulimane, S.; Pinon, S.; Shih, W.P.; Camon, H. Dielectric Elastomer Micro Actuator Made In Micromachining Technology: Finite Element Modelling and Deformation Measurement. Procedia Eng. 2011, 25, 479–482. [Google Scholar] [CrossRef]
- Pimpin, A.; Suzuki, Y.; Kasagi, N. Microelectrostrictive actuator with large out-of-plane deformation for flow-control application. J. Microelectromech. Syst. 2007, 16, 753–764. [Google Scholar] [CrossRef]
- Murray, C.; McCoul, D.; Sollier, E.; Ruggiero, T.; Niu, X.; Pei, Q.; Di Carlo, D. Electro-adaptive microfluidics for active tuning of channel geometry using polymer actuators. Microfluid. Nanofluidics 2013, 14, 345–358. [Google Scholar] [CrossRef]
- Tanaka, Y.; Fujikawa, T.; Kazoe, Y.; Kitamori, T. An active valve incorporated into a microchip using a high strain electroactive polymer. Sens. Actuators B Chem. 2013, 184, 163–169. [Google Scholar] [CrossRef]
- Mohd Ghazali, F.A.; Mah, C.K.; AbuZaiter, A.; Chee, P.S.; Mohamed Ali, M.S. Soft dielectric elastomer actuator micropump. Sens. Actuators A Phys. 2017, 263, 276–284. [Google Scholar] [CrossRef]
- Ren, Z.; Kim, S.; Ji, X.; Zhu, W.; Niroui, F.; Kong, J.; Chen, Y. A High-Lift Micro-Aerial-Robot Powered by Low-Voltage and Long-Endurance Dielectric Elastomer Actuators. Adv. Mater. 2022, 34, 2106757. [Google Scholar] [CrossRef]
- Rosset, S.; Niklaus, M.; Dubois, P.; Shea, H.R. Mechanical characterization of a dielectric elastomer microactuator with ion-implanted electrodes. Sens. Actuators A Phys. 2008, 144, 185–193. [Google Scholar] [CrossRef]
- Poulin, A.; Rosset, S.; Shea, H.R. Printing low-voltage dielectric elastomer actuators. Appl. Phys. Lett. 2015, 107, 244104. [Google Scholar] [CrossRef]
- Neu, J.; Croce, S.; Willian, T.; Hubertus, J.; Schultes, G.; Seelecke, S.; Rizzello, G. Distributed Electro-Mechanical Coupling Effects in a Dielectric Elastomer Membrane Array. Exp. Mech. 2022, 63, 79–95. [Google Scholar] [CrossRef]
- Yu, X.; Lu, Z.; Cui, F.; Cheng, L.; Cui, Y. Tunable acoustic metamaterial with an array of resonators actuated by dielectric elastomer. Extrem. Mech. Lett. 2017, 12, 37–40. [Google Scholar] [CrossRef]
- Ji, X.; Liu, X.; Cacucciolo, V.; Civet, Y.; El Haitami, A.; Cantin, S.; Perriard, Y.; Shea, H. Untethered Feel-Through Haptics Using 18-µm Thick Dielectric Elastomer Actuators. Adv. Funct. Mater. 2021, 31, 2006639. [Google Scholar] [CrossRef]
- Solano-Arana, S.; Klug, F.; Mößinger, H.; Förster-Zügel, F.; Schlaak, H.F. A novel application of dielectric stack actuators: A pumping micromixer. Smart Mater. Struct. 2018, 27, 074008. [Google Scholar] [CrossRef]
- Schlatter, S.; Grasso, G.; Rosset, S.; Shea, H. Inkjet Printing of Complex Soft Machines with Densely Integrated Electrostatic Actuators. Adv. Intell. Syst. 2020, 2, 2000136. [Google Scholar] [CrossRef]
- O’Brien, B.; Gisby, T.; Calius, E.; Xie, S.; Anderson, I. FEA of Dielectric Elastomer Minimum Energy Structures as a Tool for Biomimetic Design. In SPIE Electroactive Polymer Actuators and Devices; SPIE: Bellingham, WA, USA, 2009; Volume 7287, pp. 61–71. [Google Scholar]
- Dai, B.; Li, S.; Xu, T.; Wang, Y.; Zhang, F.; Gu, Z.; Wang, S. Artificial Asymmetric Cilia Array of Dielectric Elastomer for Cargo Transportation. ACS Appl. Mater. Interfaces 2018, 10, 42979–42984. [Google Scholar] [CrossRef]
- Zhao, J.; Niu, J.; Liu, L.; Yu, J. A Soft Creeping Robot Actuated by Dielectric Elastomer. In SPIE Electroactive Polymer Actuators and Devices; SPIE: Bellingham, WA, USA, 2014; Volume 9056, pp. 40–45. [Google Scholar]
- Lu, X.J.; Wang, K.; Hu, T.T. Development of an annelid-like peristaltic crawling soft robot using dielectric elastomer actuators. Bioinspir. Biomim. 2020, 15, 046012. [Google Scholar] [CrossRef] [PubMed]
- Li, W.B.; Zhang, W.M.; Zou, H.X.; Peng, Z.K.; Meng, G. Multisegment annular dielectric elastomer actuators for soft robots. Smart Mater. Struct. 2018, 27, 115024. [Google Scholar] [CrossRef]
- Pfeil, S.; Henke, M.; Katzer, K.; Zimmermann, M.; Gerlach, G. A Worm-Like Biomimetic Crawling Robot Based on Cylindrical Dielectric Elastomer Actuators. Front. Robot. AI 2020, 7, 9. [Google Scholar] [CrossRef]
- Henke, E.-F.M.; Schlatter, S.; Anderson, I.A. Soft Dielectric Elastomer Oscillators Driving Bioinspired Robots. Soft Robot. 2017, 4, 353–366. [Google Scholar] [CrossRef] [PubMed]
- Nguyen, C.T.; Phung, H.; Nguyen, T.D.; Jung, H.; Choi, H.R. Multiple-degrees-of-freedom dielectric elastomer actuators for soft printable hexapod robot. Sens. Actuators A Phys. 2017, 267, 505–516. [Google Scholar] [CrossRef]
- Chen, F.; Cao, J.; Zhang, H.; Wang, M.Y.; Zhu, J.; Zhang, Y.F. Programmable Deformations of Networked Inflated Dielectric Elastomer Actuators. IEEE/ASME Trans. Mechatron. 2019, 24, 45–55. [Google Scholar] [CrossRef]
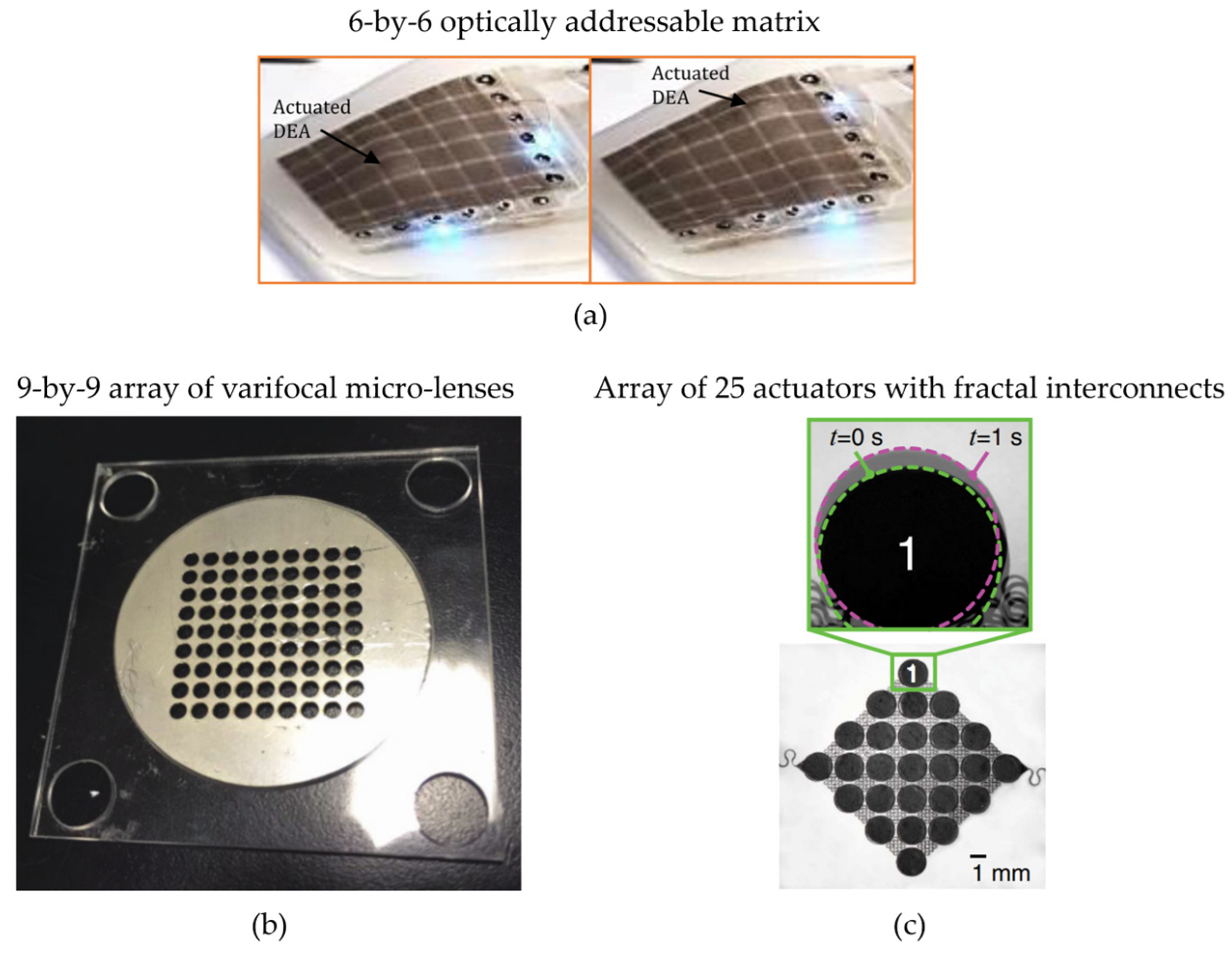
- Hajiesmaili, E.; Clarke, D.R. Optically addressable dielectric elastomer actuator arrays using embedded percolative networks of zinc oxide nanowires. Mater. Horiz. 2022, 9, 3110–3117. [Google Scholar] [CrossRef]
- Wang, L.; Hayakawa, T.; Ishikawa, M. Dielectric-elastomer-based fabrication method for varifocal microlens array. Opt. Express 2017, 25, 31708. [Google Scholar] [CrossRef]
- Burugupally, S.P.; Koppolu, B.; Danesh, N.; Lee, Y.; Indeewari, V.; Li, B. Enhancing the performance of dielectric elastomer actuators through the approach of distributed electrode array with fractal interconnects architecture. J. Micromech. Microeng. 2021, 31, 064002. [Google Scholar] [CrossRef]
- Yang, W.-P.; Chen, L.-W.; McGough, K.; Ahmed, S. The tunable acoustic band gaps of two-dimensional phononic crystals with a dielectricelastomer cylindrical actuator. Smart Mater. Struct. 2007, 17, 015011. [Google Scholar] [CrossRef]
- Wang, T.; Zhang, J.; Hong, J.; Wang, M.Y. Dielectric Elastomer Actuators for Soft Wave-Handling Systems. Soft Robot. 2017, 4, 61–69. [Google Scholar] [CrossRef]
- Akbari, S.; Shea, H.R. Microfabrication and characterization of an array of dielectric elastomer actuators generating uniaxial strain to stretch individual cells. J. Micromech. Microeng. 2012, 22, 045020. [Google Scholar] [CrossRef]
- Akbari, S.; Shea, H.R. An array of 100 m × 100 m dielectric elastomer actuators with 80% strain for tissue engineering applications. Sens. Actuators A 2012, 186, 236–241. [Google Scholar] [CrossRef]
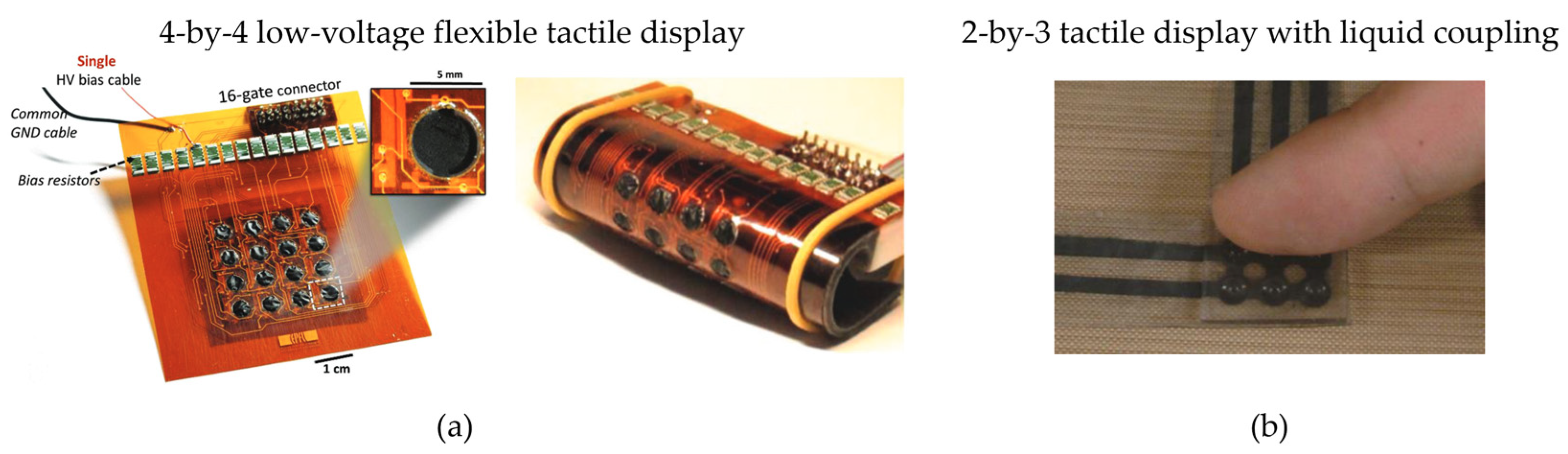
- Matysek, M.; Lotz, P.; Winterstein, T.; Schlaak, H.F. Dielectric elastomer actuators for tactile displays. In Proceedings of the World Haptics 2009-Third Joint EuroHaptics conference and Symposium on Haptic Interfaces for Virtual Environment and Teleoperator Systems, Online, 18–20 March 2009; pp. 290–295. [Google Scholar]
- Phung, H.; Hoang, P.T.; Jung, H.; Nguyen, T.D.; Nguyen, C.T.; Choi, H.R. Haptic Display Responsive to Touch Driven by Soft Actuator and Soft Sensor. IEEE/ASME Trans. Mechatron. 2021, 26, 2495–2505. [Google Scholar] [CrossRef]
- Lee, H.S.; Phung, H.; Lee, D.-H.; Kim, U.K.; Nguyen, C.T.; Moon, H.; Koo, J.C.; Choi, H.R. Design analysis and fabrication of arrayed tactile display based on dielectric elastomer actuator. Sens. Actuators A Phys. 2014, 205, 191–198. [Google Scholar] [CrossRef]
- Lee, D.-Y.; Jeong, S.H.; Cohen, A.J.; Vogt, D.M.; Kollosche, M.; Lansberry, G.; Mengüç, Y.; Israr, A.; Clarke, D.R.; Wood, R.J. A Wearable Textile-Embedded Dielectric Elastomer Actuator Haptic Display. Soft Robot. 2022, 9, 1186–1197. [Google Scholar] [CrossRef]
- Zhao, H.; Hussain, A.M.; Israr, A.; Vogt, D.M.; Duduta, M.; Clarke, D.R.; Wood, R.J. A Wearable Soft Haptic Communicator Based on Dielectric Elastomer Actuators. Soft Robot. 2020, 7, 451–461. [Google Scholar] [CrossRef]
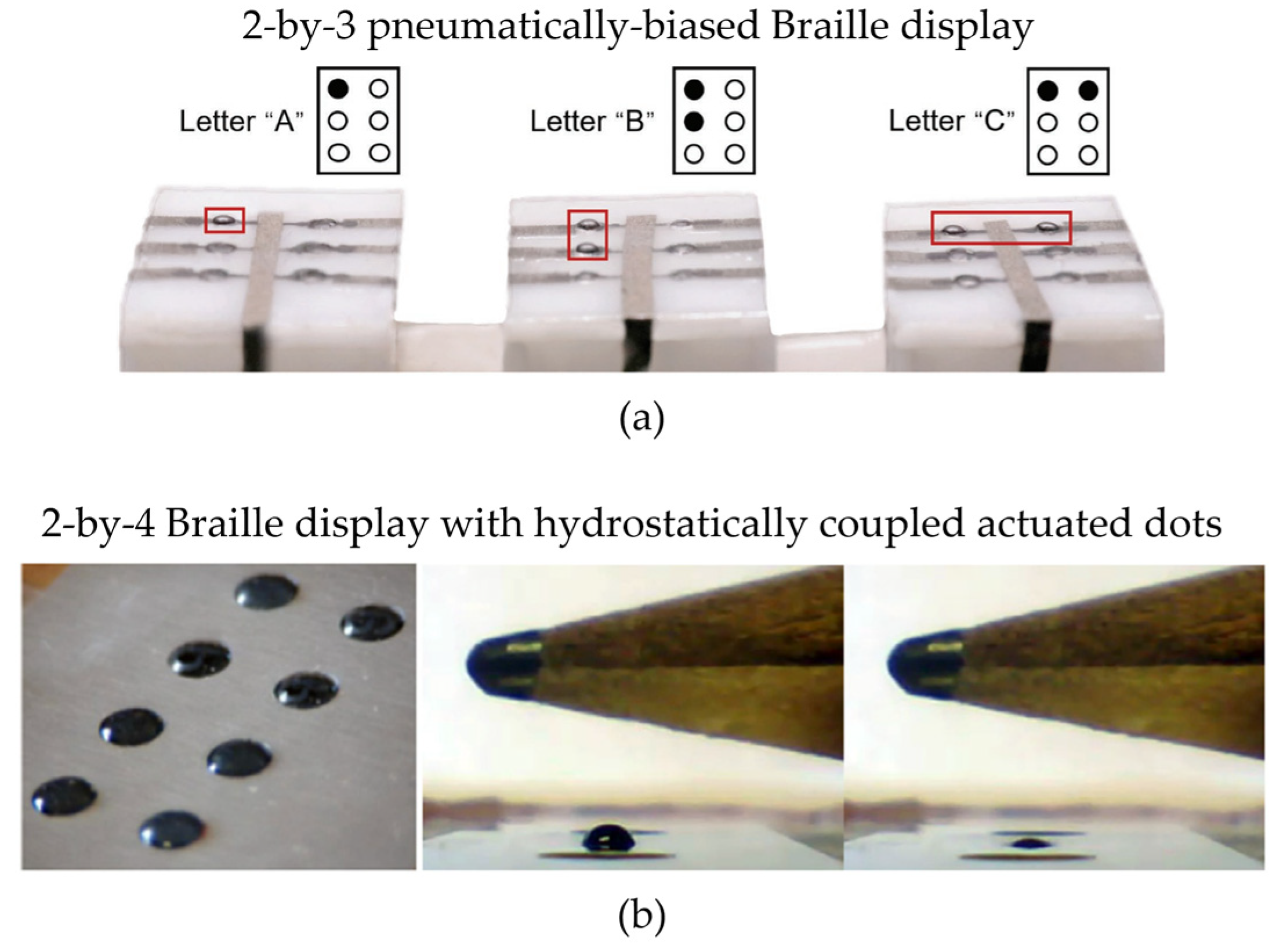
- Chakraborti, P.; Toprakci, H.A.K.; Yang, P.; Di Spigna, N.; Franzon, P.; Ghosh, T. A compact dielectric elastomer tubular actuator for refreshable Braille displays. Sens. Actuators A Phys. 2012, 179, 151–157. [Google Scholar] [CrossRef]
- Qu, X.; Ma, X.; Shi, B.; Li, H.; Zheng, L.; Wang, C.; Liu, Z.; Fan, Y.; Chen, X.; Li, Z.; et al. Refreshable Braille Display System Based on Triboelectric Nanogenerator and Dielectric Elastomer. Adv. Funct. Mater. 2020, 31, 2006612. [Google Scholar] [CrossRef]
- Frediani, G.; Busfield, J.; Carpi, F. Enabling portable multiple-line refreshable Braille displays with electroactive elastomers. Med. Eng. Phys. 2018, 60, 86–93. [Google Scholar] [CrossRef]
- Pang, W.; Cheng, X.; Zhao, H.; Guo, X.; Ji, Z.; Li, G.; Liang, Y.; Xue, Z.; Song, H.; Zhang, F.; et al. Electro-mechanically controlled assembly of reconfigurable 3D mesostructures and electronic devices based on dielectric elastomer platforms. Natl. Sci. Rev. 2020, 7, 342–354. [Google Scholar] [CrossRef]
- Sun, Y.; Li, D.; Wu, M.; Yang, Y.; Su, J.; Wong, T.; Xu, K.; Li, Y.; Li, L.; Yu, X.; et al. Origami-inspired folding assembly of dielectric elastomers for programmable soft robots. Microsyst. Nanoeng. 2022, 8, 1–11. [Google Scholar] [CrossRef] [PubMed]
- Aksoy, B.; Shea, H. Reconfigurable and Latchable Shape-Morphing Dielectric Elastomers Based on Local Stiffness Modulation. Adv. Funct. Mater. 2020, 30, 2001597. [Google Scholar] [CrossRef]
- Meng, J.; Qiu, Y.; Hou, C.; Zhang, Q.; Li, Y.; Wang, H. Bistable dielectric elastomer actuator with directional motion. Sens. Actuators A Phys. 2021, 330, 112889. [Google Scholar] [CrossRef]
- Xu, D.; Tairych, A.; Anderson, I.A. Where the rubber meets the hand: Unlocking the sensing potential of dielectric elastomers. J. Polym. Sci. Part B Polym. Phys. 2016, 54, 465–472. [Google Scholar] [CrossRef]
- Zhang, H.; Wang, M.Y.; Li, J.; Zhu, J. A soft compressive sensor using dielectric elastomers. Smart Mater. Struct. 2016, 25, 035045. [Google Scholar] [CrossRef]
- Ham, J.; Huh, T.M.; Kim, J.; Kim, J.-O.; Park, S.; Cutkosky, M.R.; Bao, Z. Porous Dielectric Elastomer Based Flexible Multiaxial Tactile Sensor for Dexterous Robotic or Prosthetic Hands. Adv. Mater. Technol. 2022, in press. [CrossRef]
- Kadooka, K.; Imamura, H.; Taya, M. Tactile Sensor Integrated Dielectric Elastomer Actuator for Simultaneous Actuation and Sensing. In Electroactive Polymer Actuators and Devices; SPIE: Bellingham, WA, USA, 2016; Volume 9798, pp. 489–498. [Google Scholar]
- Zhu, Y.; Giffney, T.; Aw, K. A Dielectric Elastomer-Based Multimodal Capacitive Sensor. Sensors 2022, 22, 622. [Google Scholar] [CrossRef] [PubMed]
- Meyer, A.; Lenz, S.; Gratz-Kelly, S.; Motzki, P.; Nalbach, S.; Seelecke, S.; Rizzello, G. Experimental Characterization of a Smart Dielectric Elastomer Multi-Sensor Grid. In SPIE Electroactive Polymer Actuators and Devices (EAPAD) XXII; SPIE: Bellingham, WA, USA, 2020; Volume 11375, pp. 262–268. [Google Scholar]
- Lee, B.Y.; Kim, J.; Kim, H.; Kim, C.; Lee, S.D. Low-cost flexible pressure sensor based on dielectric elastomer film with micro-pores. Sens. Actuators A Phys. 2016, 240, 103–109. [Google Scholar] [CrossRef]
- Kwon, D.; Lee, T.-I.; Shim, J.; Ryu, S.; Kim, M.S.; Kim, S.; Kim, T.-S.; Park, I. Highly Sensitive, Flexible, and Wearable Pressure Sensor Based on a Giant Piezocapacitive Effect of Three-Dimensional Microporous Elastomeric Dielectric Layer. ACS Appl. Mater. Interfaces 2016, 8, 16922–16931. [Google Scholar] [CrossRef]
- Kyaw, A.K.K.; Loh, H.H.C.; Yan, F.; Xu, J. A polymer transistor array with a pressure-sensitive elastomer for electronic skin. J. Mater. Chem. C 2017, 5, 12039–12043. [Google Scholar] [CrossRef]
- Larson, C.; Spjut, J.; Knepper, R.; Shepherd, R. A Deformable Interface for Human Touch Recognition Using Stretchable Carbon Nanotube Dielectric Elastomer Sensors and Deep Neural Networks. Soft Robot. 2019, 6, 611–620. [Google Scholar] [CrossRef] [PubMed]
- Vishniakou, S.; Lewis, B.W.; Niu, X.; Kargar, A.; Sun, K.; Kalajian, M.; Park, N.; Yang, M.; Jing, Y.; Brochu, P.; et al. Tactile Feedback Display with Spatial and Temporal Resolutions. Sci. Rep. 2013, 3, 2521. [Google Scholar] [CrossRef] [PubMed]



Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Rizzello, G. A Review of Cooperative Actuator and Sensor Systems Based on Dielectric Elastomer Transducers. Actuators 2023, 12, 46. https://doi.org/10.3390/act12020046
Rizzello G. A Review of Cooperative Actuator and Sensor Systems Based on Dielectric Elastomer Transducers. Actuators. 2023; 12(2):46. https://doi.org/10.3390/act12020046
Chicago/Turabian StyleRizzello, Gianluca. 2023. "A Review of Cooperative Actuator and Sensor Systems Based on Dielectric Elastomer Transducers" Actuators 12, no. 2: 46. https://doi.org/10.3390/act12020046
APA StyleRizzello, G. (2023). A Review of Cooperative Actuator and Sensor Systems Based on Dielectric Elastomer Transducers. Actuators, 12(2), 46. https://doi.org/10.3390/act12020046