Reduction in Airfoil Trailing-Edge Noise Using a Pulsed Laser as an Actuator
Abstract
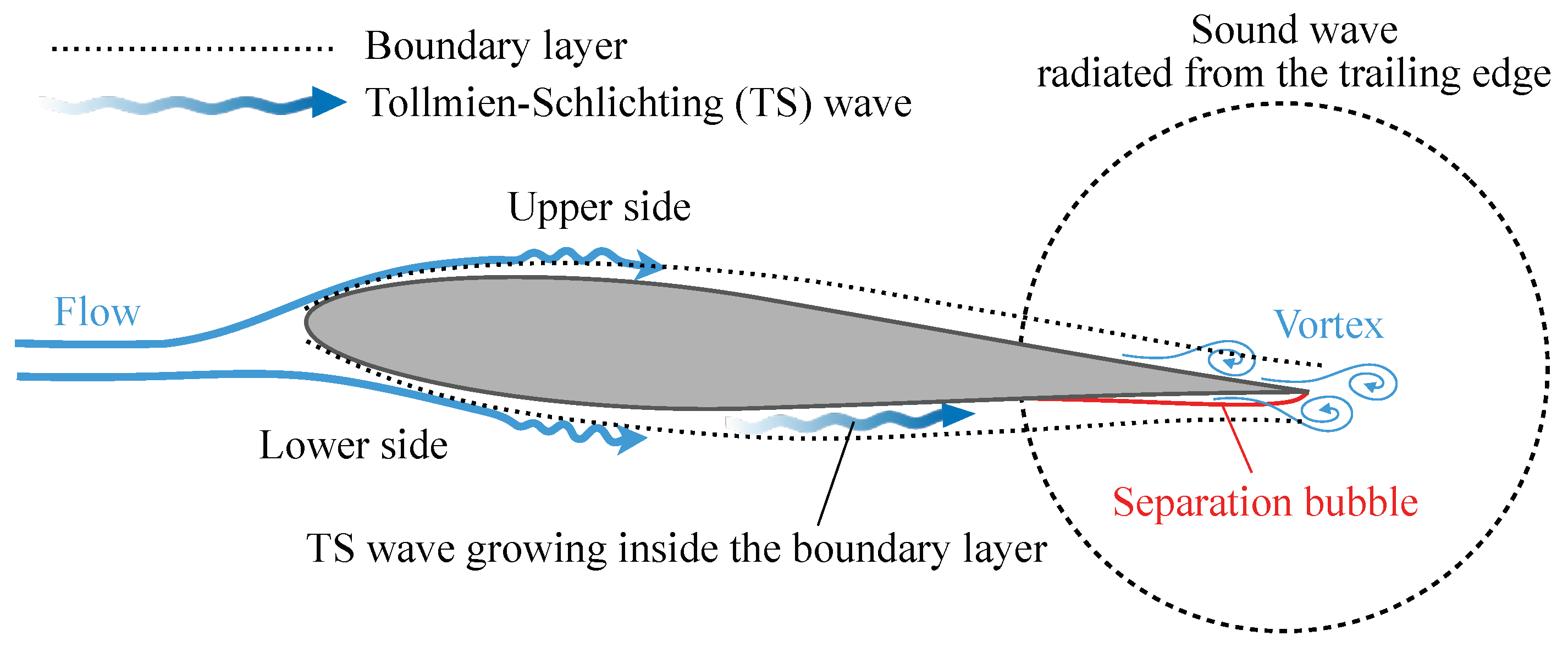
1. Introduction
2. Wind Tunnel Test
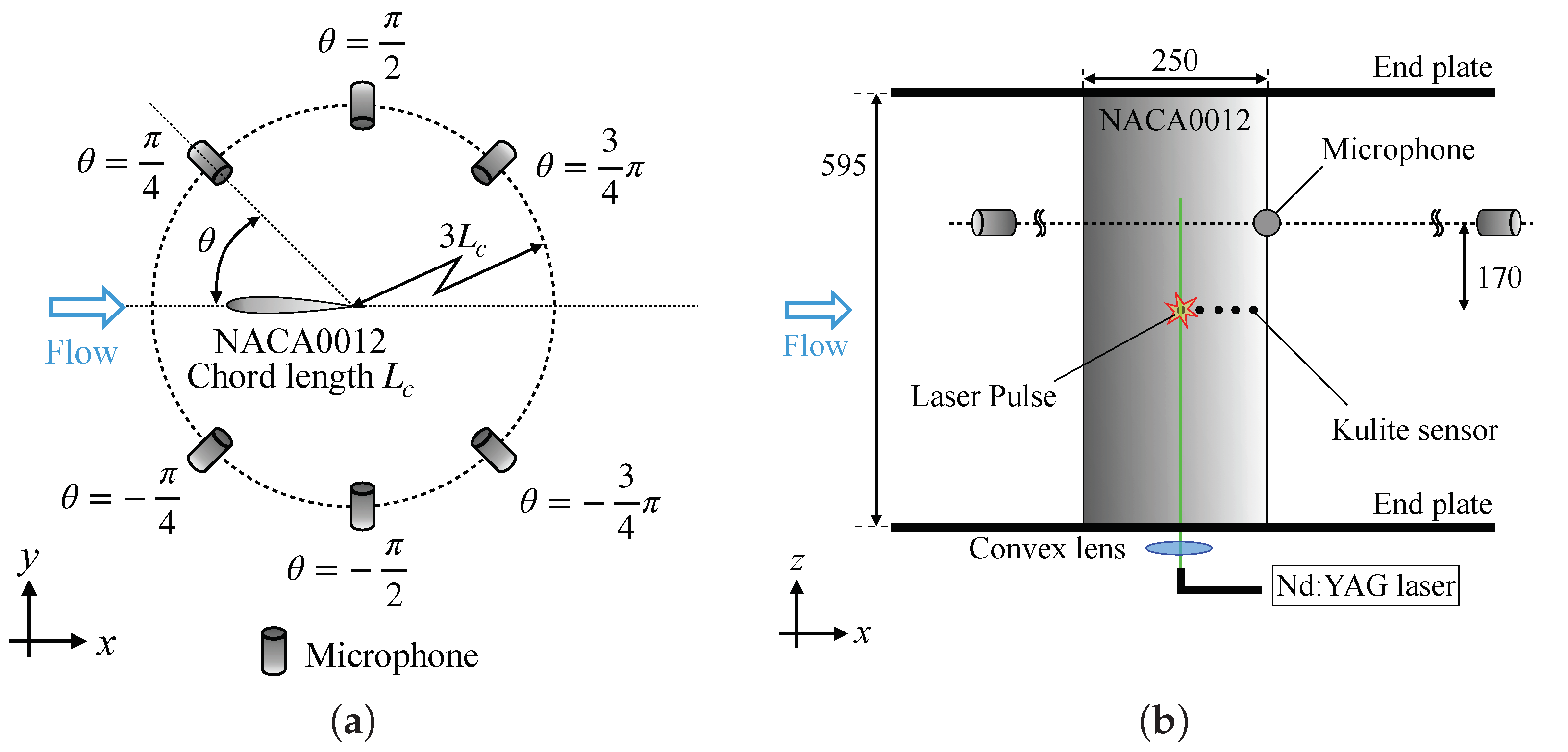
2.1. Experimental Setup
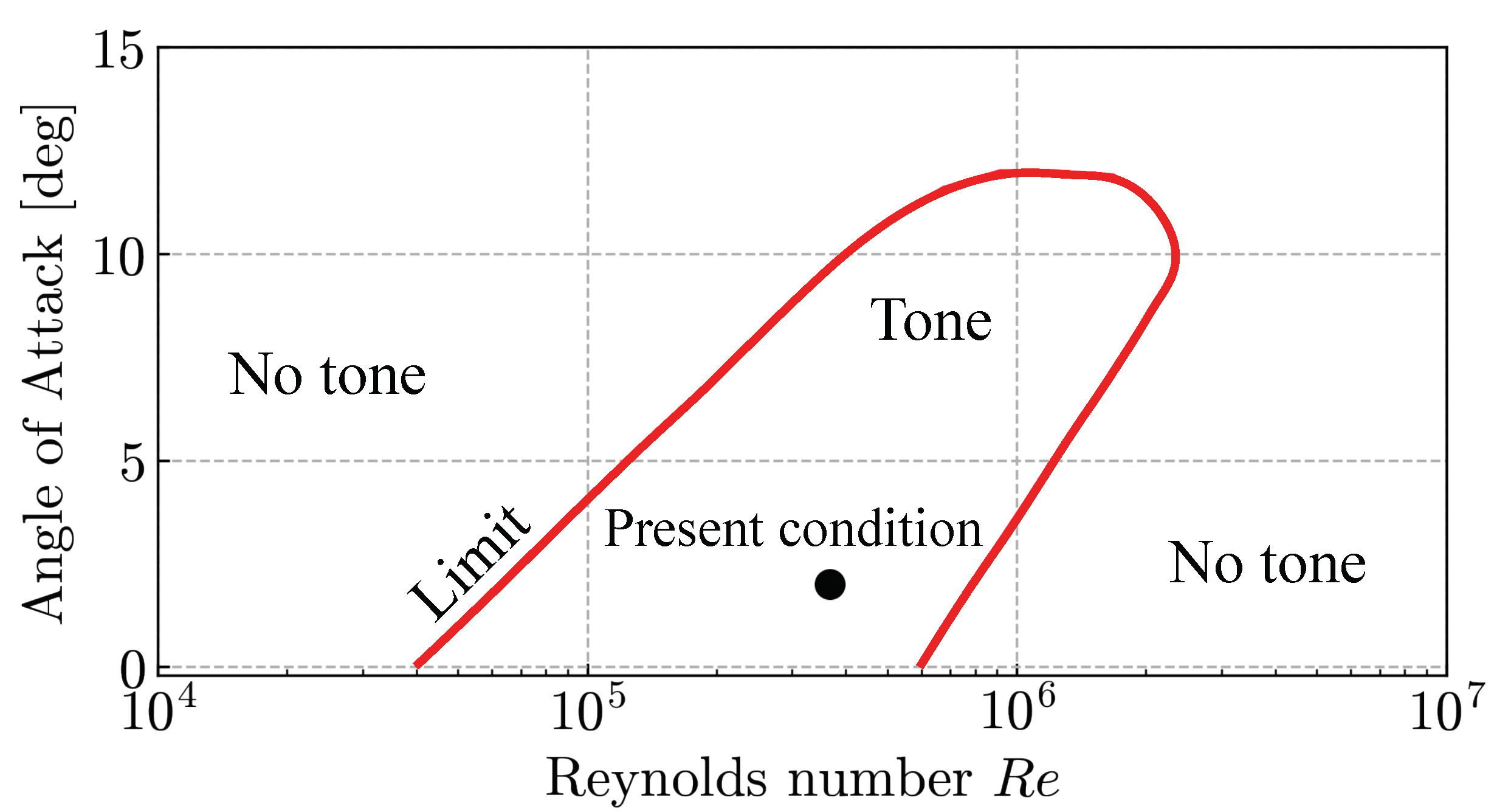
2.2. Experimental Conditions
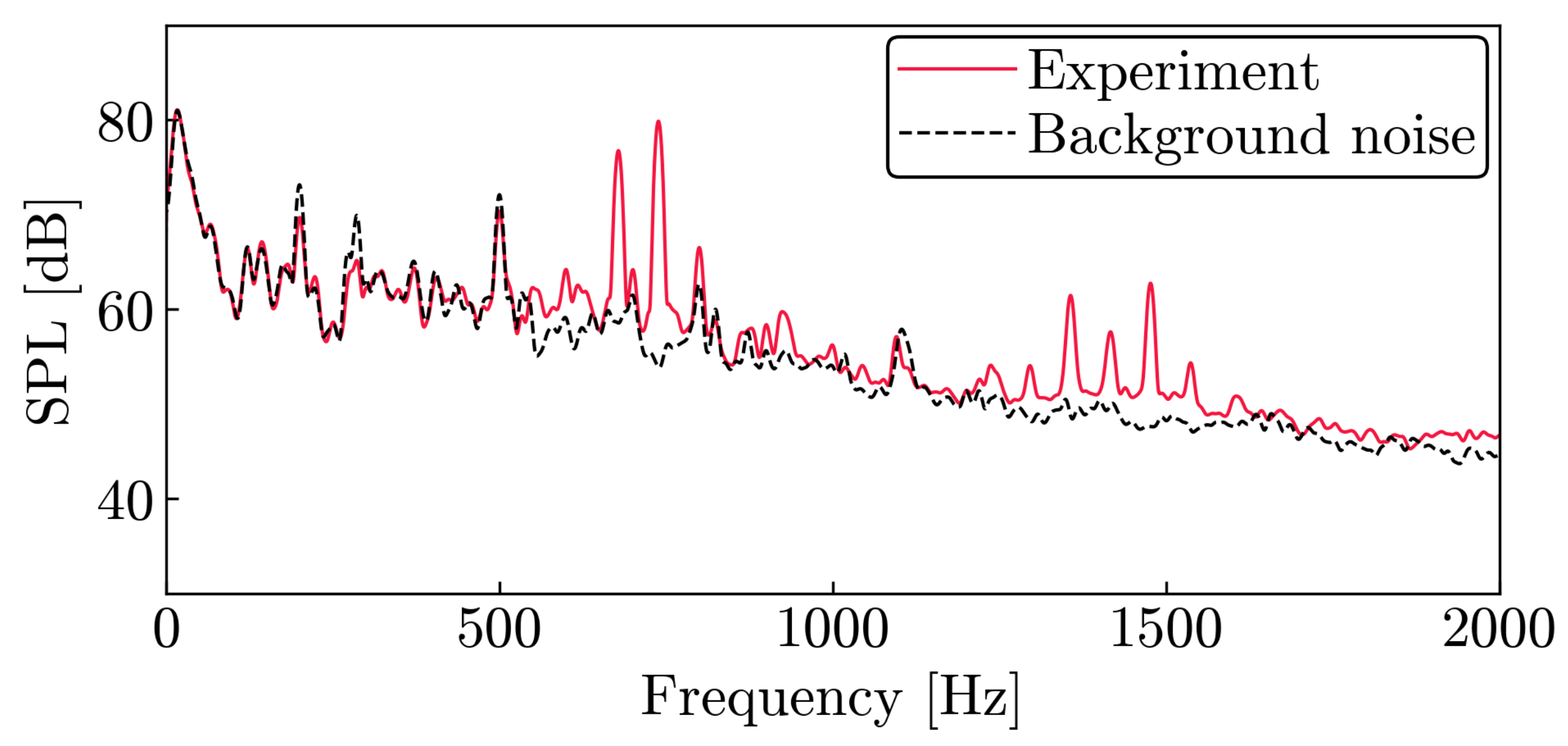
2.3. Experimental Results
3. Numerical Setup for the Simulation
3.1. Governing Equations
3.2. Modeling of Energy Deposition
3.3. Numerical Method and Conditions
4. Numerical Results and Discussion
4.1. Validation of the Present Computation
4.2. Energy Deposition near the Boundary Layer
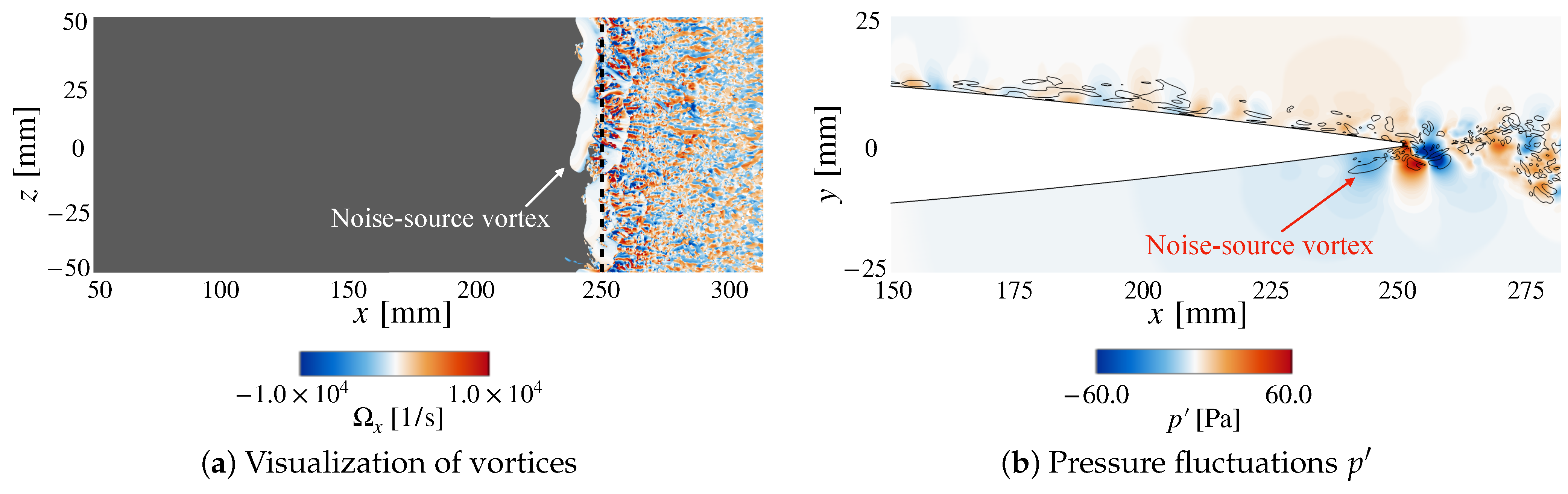
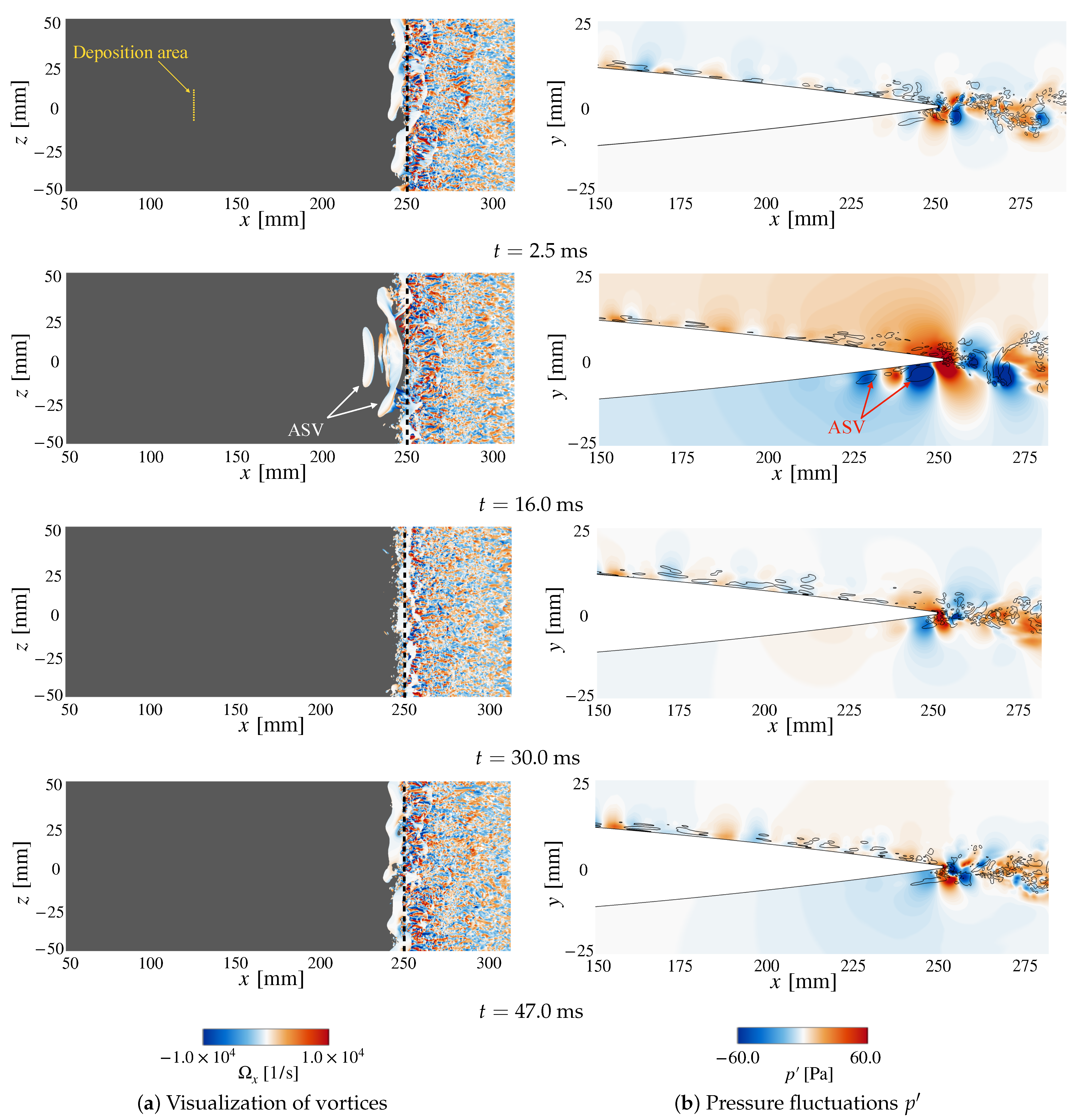
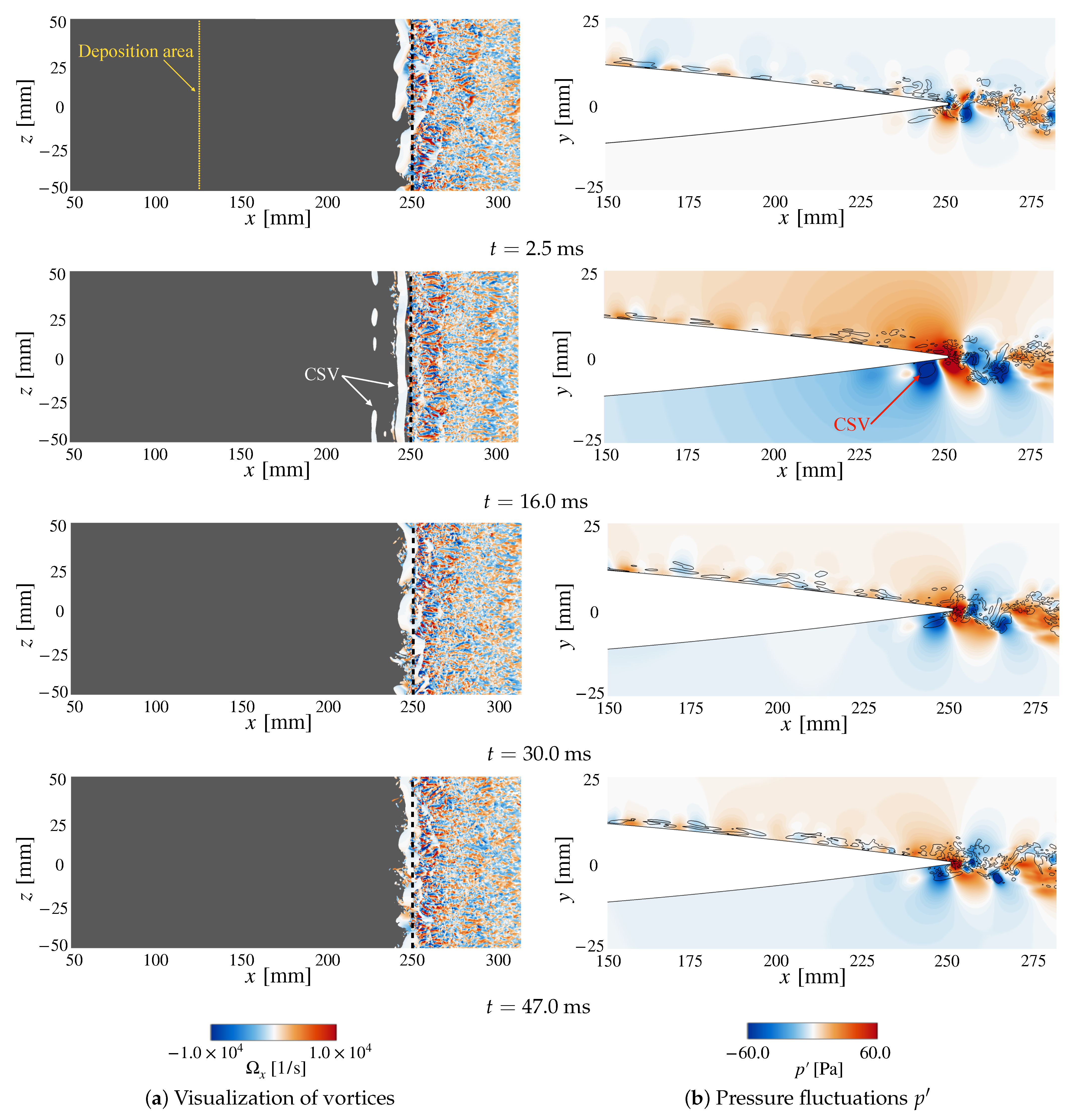
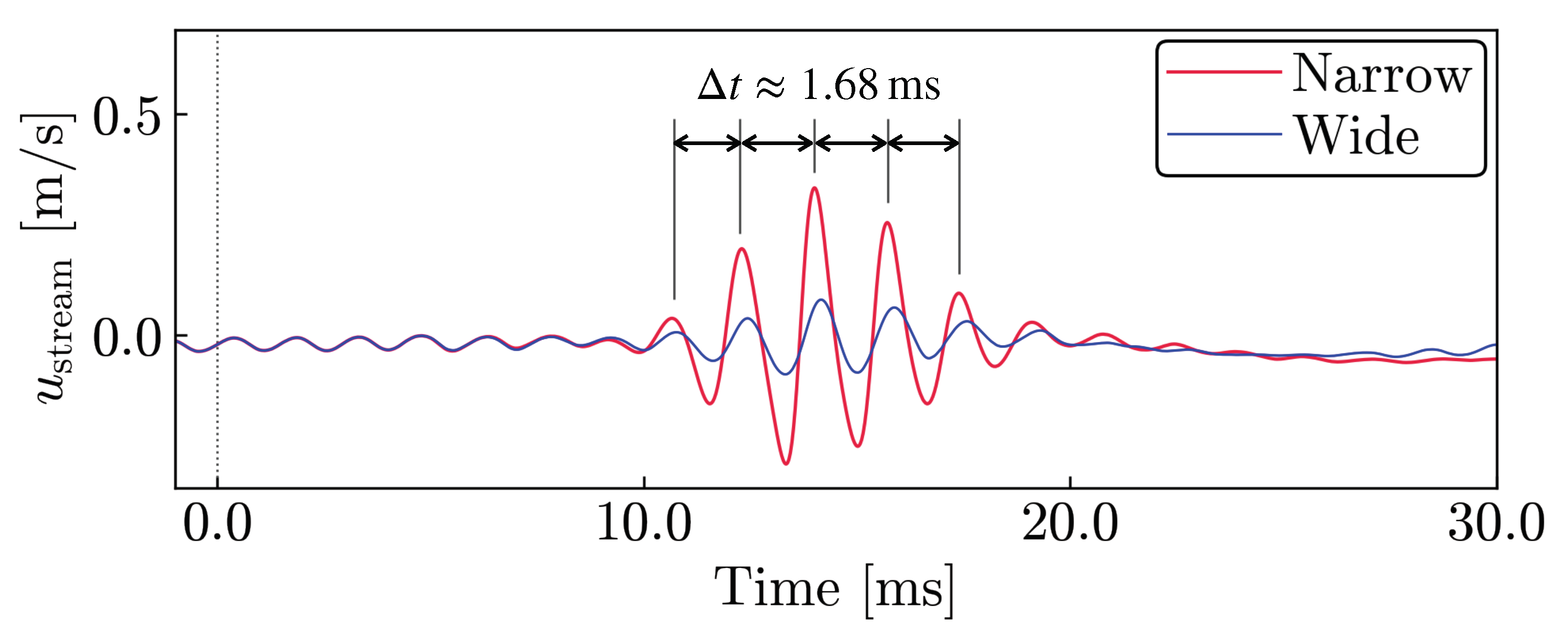
4.3. Dynamics of Vortices near the Trailing Edge
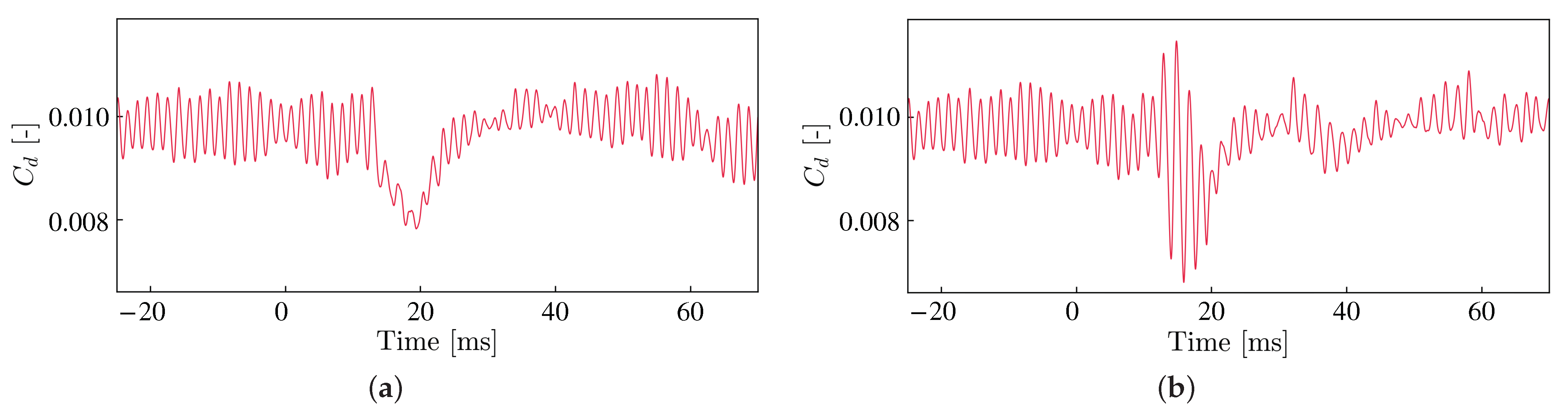
4.4. Aerodynamic Characteristics
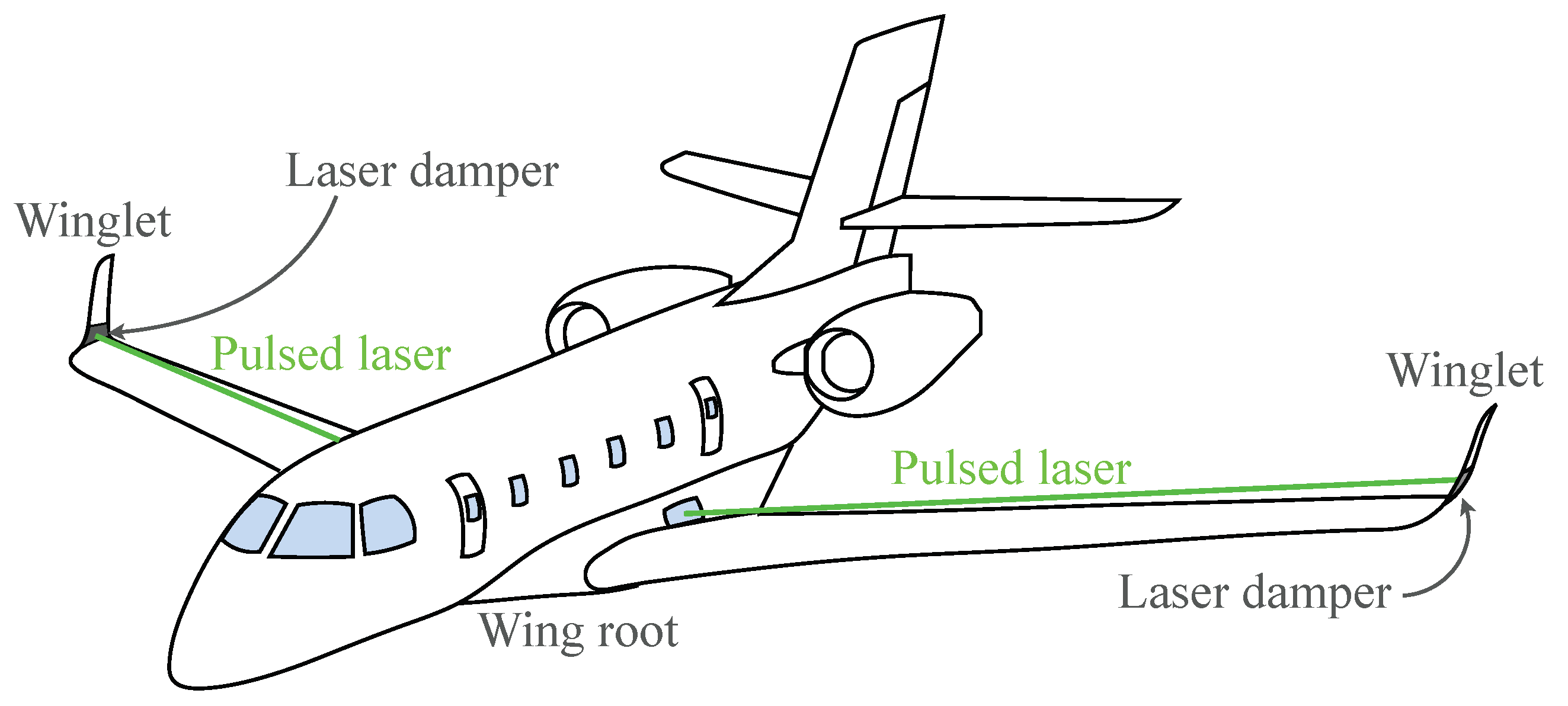
4.5. Implementation of the Laser Actuator for Actual Aircraft
5. Conclusions
- The experimental result indicates that a laser irradiation can reduce the intensity of the surface pressure fluctuations that are the source of the TE noise.
- The numerical investigations suggest that a laser irradiation induces a characteristic vortex depending on the energy deposition area shapes. In the Narrow case, the energy deposition to the narrow domain introduces vortices with a curved shape named an accurate spread vortex (ASV). In contrast, the relatively spanwise uniform vortex called a cylindrical spread vortex (CSV) is observed in the Wide case. The ASV swept out the source vortices of the TE noise and broke the acoustic feedback mechanism that sustains the TE noise. The CSV disturbs the spanwise uniformity of the noise-source vortices, resulting in a spanwise-incoherent noise radiation, which might cancel out each other.
- The Wide case is more effective in reducing the source vortex intensity if the amount of energy decompositions is the same as the Narrow case.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Abbreviations
| ILES | Implicit large-eddy simulation |
| SPL | Sound pressure level |
| PSD | Power spectral density |
| ASV | Arcuate spread vortex |
| CSV | Cylindrical spread vortex |
Appendix A. Detail of Governing Equations
Appendix B. Details of Numerical Method

Appendix C. Nomenclature
| Symbol | Unit | Parameter Description |
|---|---|---|
| Coordinate values | ||
| x, y, z wise velocity | ||
| Chord length | ||
| Angle of attack | ||
| - | Mach number | |
| - | Chord length-based Reynolds number | |
| Density | ||
| e | Total energy per unit mass | |
| p | Pa | Pressure |
| T | K | Temperature |
| Dynamics viscosity | ||
| - | Specific heat ratio | |
| V | Volume | |
| - | Inviscid and viscous flux of the Navier–Stokes equation | |
| - | Source term of the energy application by pulsed laser | |
| Input energy per unit mass and time | ||
| Peak intensity of Gaussian beam per unit mass and time | ||
| Deposited energy per unit mass and time | ||
| s | Pulse duration time | |
| s | Pulse interval time | |
| Pressure impulse | ||
| r | m | Distance from the laser’ center |
| m | Laser’s radius | |
| m | Pitch of the focal spots | |
| m | Length of the plasma beads | |
| t | s | Time |
| f | Hz | Frequency |
| Vorticity | ||
| Q | Q-criterion, a second invariant of the strain rate tensor | |
| ∞ | - | Uniform flow value |
| - | Time-averaged value | |
| + | - | Wall unit value |
References
- Yamamoto, K.; Hayama, K.; Kumada, T.; Hayashi, K. A flight demonstration for airframe noise reduction technology. CEAS Aeronaut. J. 2019, 10, 77–92. [Google Scholar] [CrossRef]
- Paterson, R.W.; Vogt, P.G.; Fink, M.R.; Munch, C.L. Vortex Noise of Isolated Airfoils. J. Aircr. 1973, 10, 296–302. [Google Scholar] [CrossRef]
- Desquesnes, G.; Terracol, M.; Sagaut, P. Numerical investigation of the tone noise mechanism over laminar airfoils. J. Fluid Mech. 2007, 591, 155–182. [Google Scholar] [CrossRef]
- Lowson, M.; Fiddes, S.; Nash, E. Laminar boundary layer aero-acoustic instabilities. In Proceedings of the 32nd Aerospace Sciences Meeting and Exhibit, Reno, NV, USA, 10–13 January 1994. [Google Scholar] [CrossRef]
- Brooks, T.F.; Pope, D.S.; Marcolini, M.A. Airfoil Self-noise and Prediction. Technical Report. 1989. Available online: https://ntrs.nasa.gov/citations/19890016302 (accessed on 28 December 2022).
- Arbey, H.; Bataille, J. Noise generated by airfoil profiles placed in a uniform laminar flow. J. Fluid Mech. 1983, 134, 33–47. [Google Scholar] [CrossRef]
- Nash, E.C.; Lowson, M.V.; McALPINE, A. Boundary-layer instability noise on aerofoils. J. Fluid Mech. 1999, 382, 27–61. [Google Scholar] [CrossRef]
- Pröbsting, S.; Yarusevych, S. Laminar separation bubble development on an airfoil emitting tonal noise. J. Fluid Mech. 2015, 780, 167–191. [Google Scholar] [CrossRef]
- Nakano, T.; Fujisawa, N.; Lee, S. Measurement of tonal-noise characteristics and periodic flow structure around NACA0018 airfoil. Exp. Fluids 2006, 40, 482–490. [Google Scholar] [CrossRef]
- Noda, T.; Nakakita, K.; Wakahara, M.; Kameda, M. Detection of small-amplitude periodic surface pressure fluctuation by pressure-sensitive paint measurements using frequency-domain methods. Exp. Fluids 2018, 59, 94. [Google Scholar] [CrossRef]
- Taira, K.; Colonius, T. Three-dimensional flows around low-aspect-ratio flat-plate wings at low Reynolds numbers. J. Fluid Mech. 2009, 623, 187–207. [Google Scholar] [CrossRef]
- Fosas de Pando, M.; Schmid, P.J.; Sipp, D. A global analysis of tonal noise in flows around aerofoils. J. Fluid Mech. 2014, 754, 5–38. [Google Scholar] [CrossRef]
- Fosas de Pando, M.; Schmid, P.J.; Sipp, D. On the receptivity of aerofoil tonal noise: An adjoint analysis. J. Fluid Mech. 2017, 812, 771–791. [Google Scholar] [CrossRef]
- Ricciardi, T.R.; Wolf, W.R.; Taira, K. Transition, intermittency and phase interference effects in airfoil secondary tones and acoustic feedback loop. J. Fluid Mech. 2022, 937, A23. [Google Scholar] [CrossRef]
- Goldin, N.; King, R.; Pätzold, A.; Nitsche, W.; Haller, D.; Woias, P. Laminar flow control with distributed surface actuation: Damping Tollmien-Schlichting waves with active surface displacement. Exp. Fluids 2013, 54, 1478. [Google Scholar] [CrossRef]
- Wylie, J.D.B.; Mishra, S.; Amitay, M. Tollmien–Schlichting Wave Control on an Airfoil Using Dynamic Surface Modification. AIAA J. 2021, 59, 2890–2900. [Google Scholar] [CrossRef]
- Inasawa, A.; Ninomiya, C.; Asai, M. Suppression of Tonal Trailing-Edge Noise From an Airfoil Using a Plasma Actuator. AIAA J. 2013, 51, 1695–1702. [Google Scholar] [CrossRef]
- Simon, B.; Markus, D.; Tropea, C.; Grundmann, S. Cancellation of Tollmien-Schlichting Waves in Direct Vicinity of a Plasma Actuator. AIAA J. 2018, 56, 1760–1769. [Google Scholar] [CrossRef]
- Simon, B.; Fabbiane, N.; Nemitz, T.; Bagheri, S.; Henningson, D.S.; Grundmann, S. In-flight active wave cancelation with delayed-x-LMS control algorithm in a laminar boundary layer. Exp. Fluids 2016, 57, 160. [Google Scholar] [CrossRef]
- Déda, T.C.; Wolf, W.R. Extremum Seeking Control Applied to Airfoil Trailing-Edge Noise Suppression. AIAA J. 2022, 60, 823–843. [Google Scholar] [CrossRef]
- Tang, H.; Lei, Y.; Fu, Y. Noise Reduction Mechanisms of an Airfoil with Trailing Edge Serrations at Low Mach Number. Appl. Sci. 2019, 9, 3784. [Google Scholar] [CrossRef]
- Kremeyer, K.P. Energy Deposition II: Physical Mechanisms Underlying Techniques to Achieve High-Speed Flow Control. In Proceedings of the International Space Planes and Hypersonic Systems and Technologies Conferences, Glasgow, UK, 6–9 July 2015. [Google Scholar] [CrossRef]
- Kim, J.H.; Matsuda, A.; Sakai, T.; Sasoh, A. Wave Drag Reduction with Acting Spike Induced by Laser-Pulse Energy Depositions. AIAA J. 2011, 49, 2076–2078. [Google Scholar] [CrossRef]
- Bright, A.; Tichenor, N.; Kremeyer, K.; Wlezien, R. Boundary-Layer Separation Control Using Laser-Induced Air Breakdown. AIAA J. 2018, 56, 1472–1482. [Google Scholar] [CrossRef]
- Welch, P. The use of fast Fourier transform for the estimation of power spectra: A method based on time averaging over short, modified periodograms. IEEE Trans. Audio Electroacoust. 1967, 15, 70–73. [Google Scholar] [CrossRef]
- Tagawa, Y.; Yamamoto, S.; Hayasaka, K.; Kameda, M. On pressure impulse of a laser-induced underwater shock wave. J. Fluid Mech. 2016, 808, 5–18. [Google Scholar] [CrossRef]
- Hashimoto, A.; Murakami, K.; Aoyama, T.; Ishiko, K.; Hishida, M.; Sakashita, M.; Lahur, P. Toward the Fastest Unstructured CFD Code ‘FaSTAR’. In Proceedings of the 50th AIAA Aerospace Sciences Meetings, Nashville, TN, USA, 9–12 January 2012. [Google Scholar] [CrossRef]
- Ricciardi, T.R.; Wolf, W.; Taira, K. Compressibility Effects in Airfoil Secondary Tones. In Proceedings of the AIAA Scitech 2021 Forum, Virtual Event, 11–15, 19–21 January 2021. [Google Scholar] [CrossRef]
- Hunt, J.C.R. Eddies, Streams and Convergence Zones in Turbulent Flows. In Proceeding of the Summer Program in Center for Turbulence Research, NASA Ames, Stanford, CA, USA, 27 June–22 July 1988; pp. 193–207. [Google Scholar]
- Jameson, A. The Construction of Discretely Conservative Finite Volume Schemes that Also Globally Conserve Energy or Entropy. J. Sci. Comput. 2008, 34, 152–187. [Google Scholar] [CrossRef]
- Jameson, A. Formulation of Kinetic Energy Preserving Conservative Schemes for Gas Dynamics and Direct Numerical Simulation of One-Dimensional Viscous Compressible Flow in a Shock Tube Using Entropy and Kinetic Energy Preserving Schemes. J. Sci. Comput. 2008, 34, 188–208. [Google Scholar] [CrossRef]
- Kojima, Y.; Hashimoto, A. Embedded Large Eddy Simulation of Transonic Flow over an OAT15A Airfoil. In Proceedings of the AIAA SciTech Forum 2022, San Diego, CA, USA, 3–7 January 2022. [Google Scholar] [CrossRef]
- Shima, E.; Kitamura, K.; Haga, T. Green–Gauss/Weighted-Least-Squares Hybrid Gradient Reconstruction for Arbitrary Polyhedra Unstructured Grids. AIAA J. 2013, 51, 2740–2747. [Google Scholar] [CrossRef]
- Yoon, S.; Jameson, A. Lower-upper Symmetric-Gauss-Seidel method for the Euler and Navier–Stokes equations. AIAA J. 1988, 26, 1025–1026. [Google Scholar] [CrossRef]

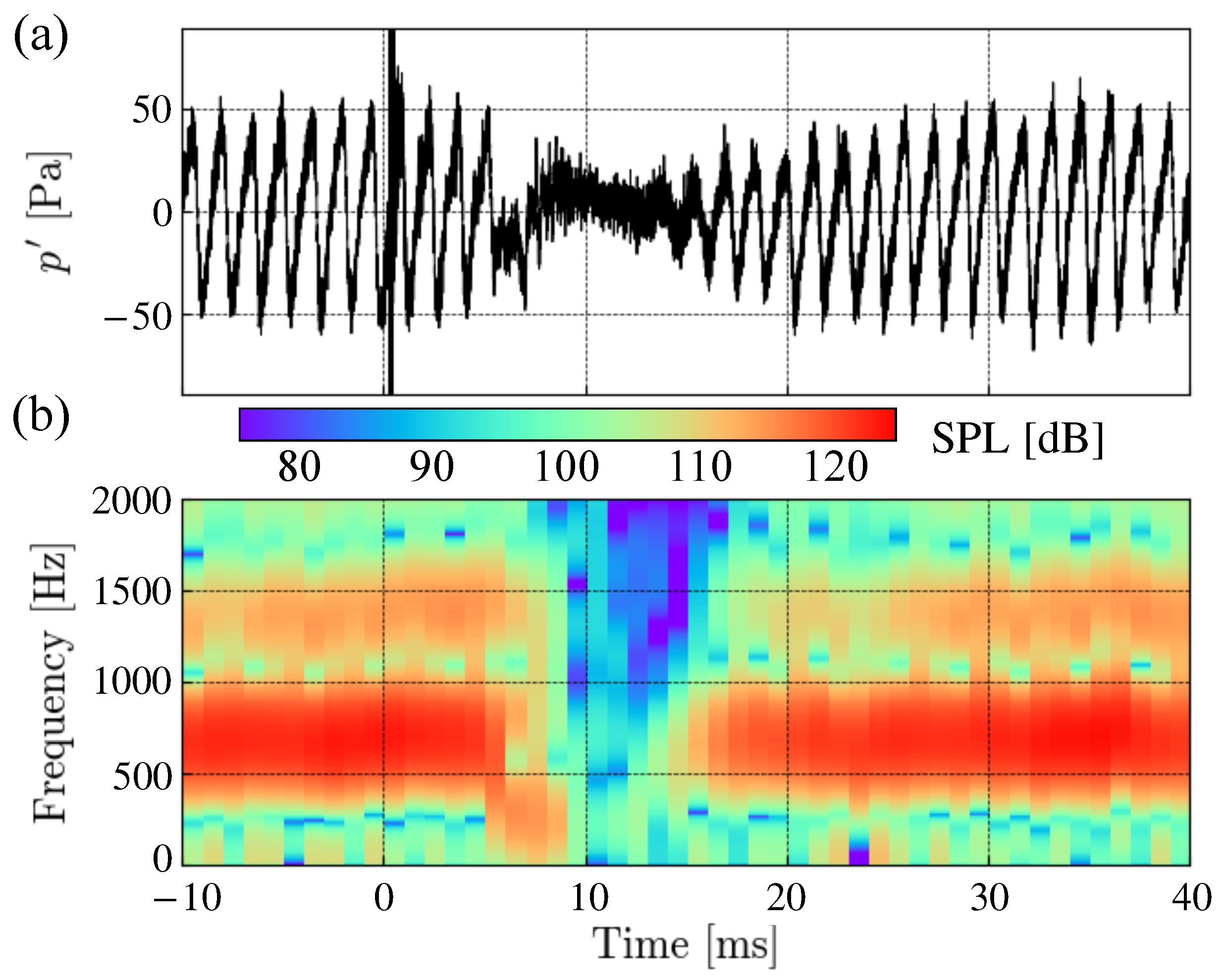

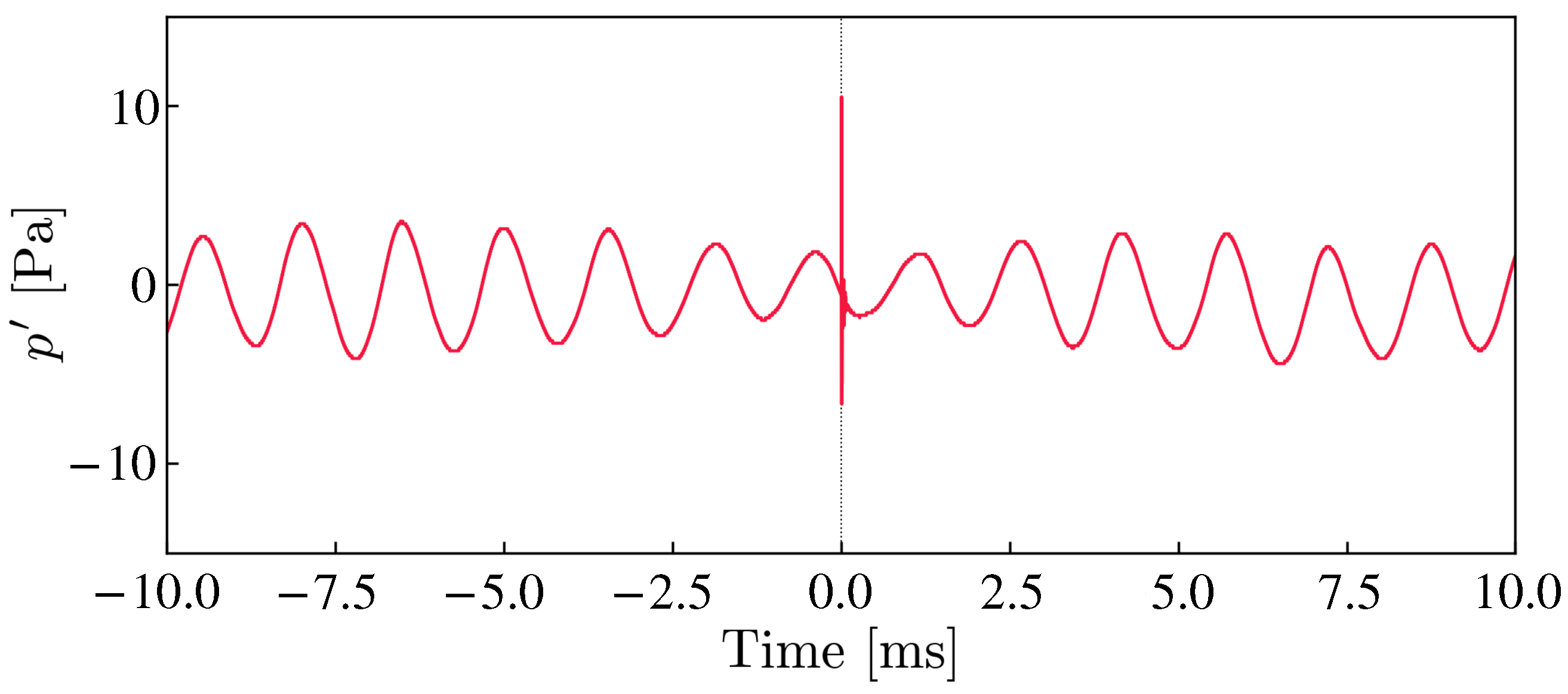


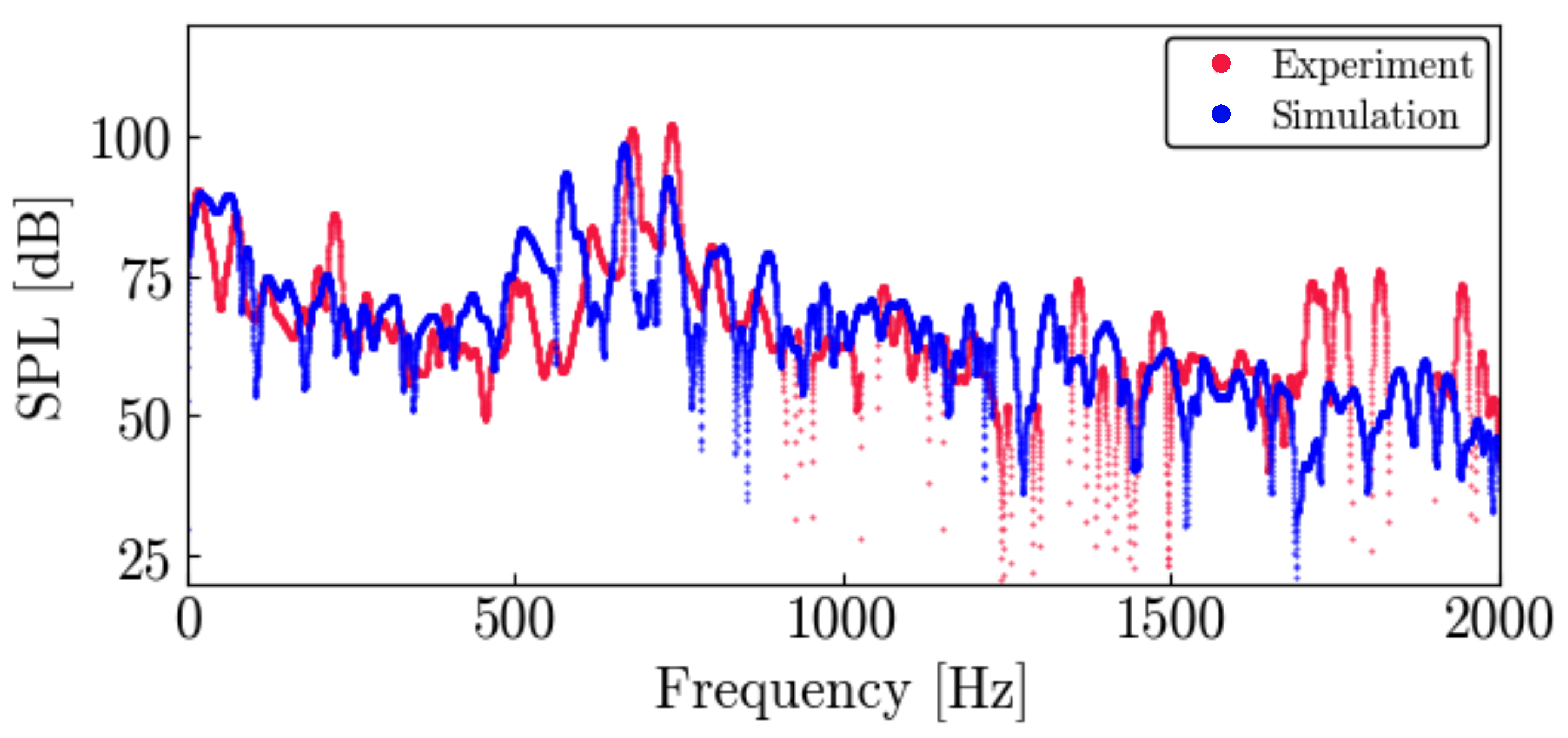

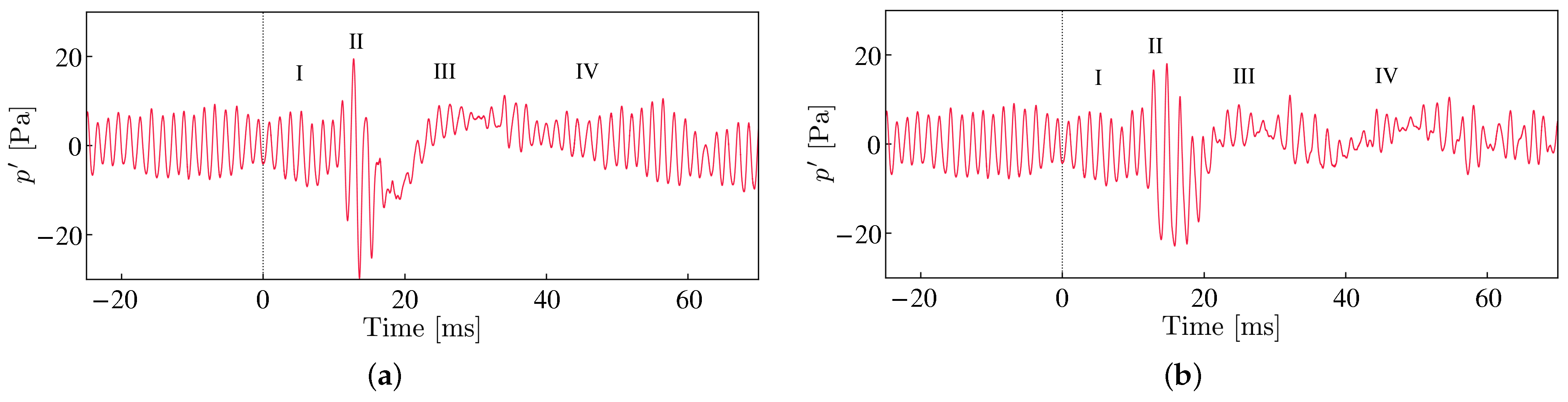

| Parameters | Unit | Narrow | Wide |
|---|---|---|---|
| mm | 0.5 | ||
| mm | 1.5 | ||
| mm | 20 | 100 | |
| Number of Pulse | – | 13 | 67 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ogura, K.; Kojima, Y.; Imai, M.; Konishi, K.; Nakakita, K.; Kameda, M. Reduction in Airfoil Trailing-Edge Noise Using a Pulsed Laser as an Actuator. Actuators 2023, 12, 45. https://doi.org/10.3390/act12010045
Ogura K, Kojima Y, Imai M, Konishi K, Nakakita K, Kameda M. Reduction in Airfoil Trailing-Edge Noise Using a Pulsed Laser as an Actuator. Actuators. 2023; 12(1):45. https://doi.org/10.3390/act12010045
Chicago/Turabian StyleOgura, Keita, Yoimi Kojima, Masato Imai, Kohei Konishi, Kazuyuki Nakakita, and Masaharu Kameda. 2023. "Reduction in Airfoil Trailing-Edge Noise Using a Pulsed Laser as an Actuator" Actuators 12, no. 1: 45. https://doi.org/10.3390/act12010045
APA StyleOgura, K., Kojima, Y., Imai, M., Konishi, K., Nakakita, K., & Kameda, M. (2023). Reduction in Airfoil Trailing-Edge Noise Using a Pulsed Laser as an Actuator. Actuators, 12(1), 45. https://doi.org/10.3390/act12010045





