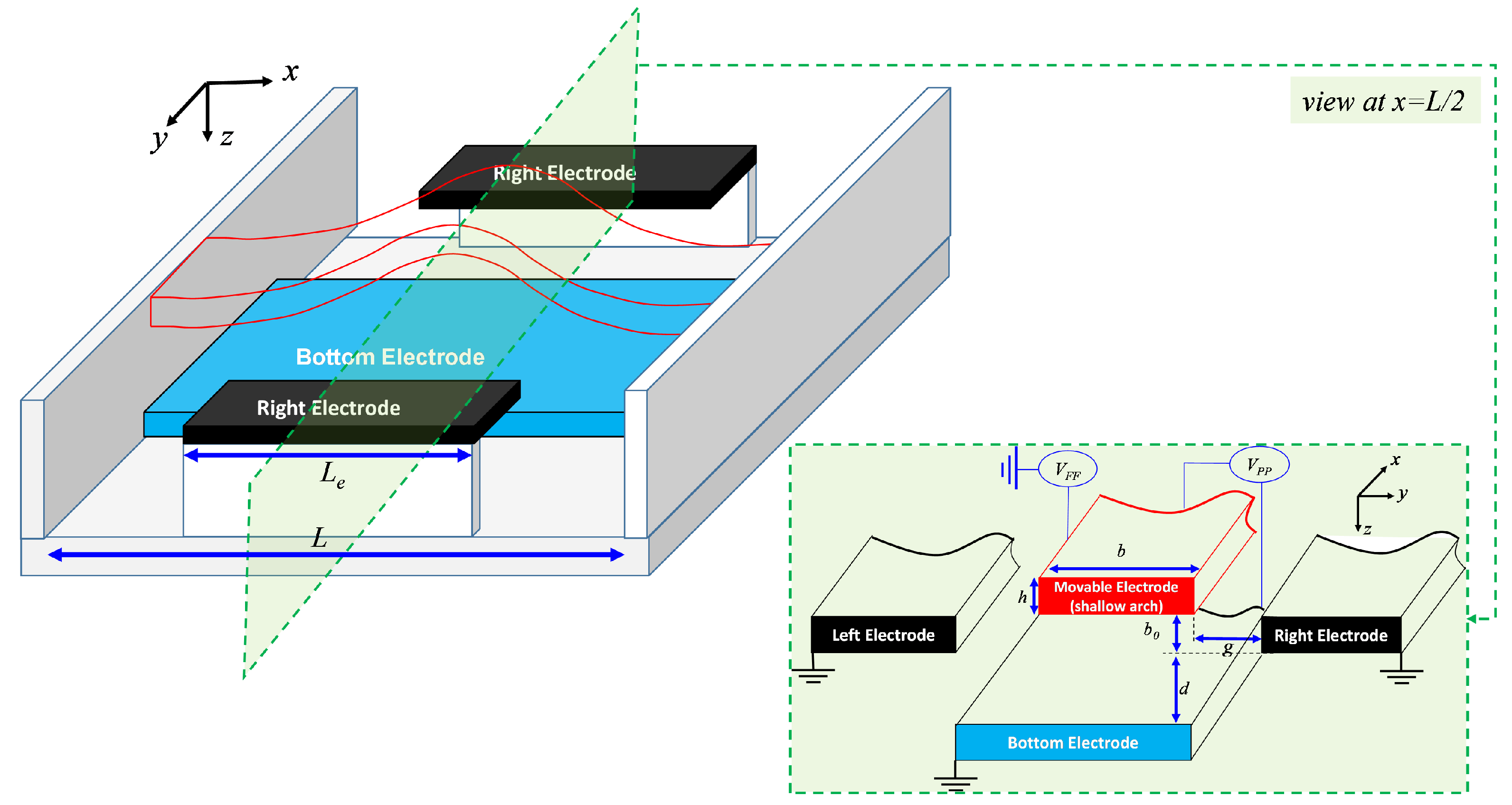
As a case study, we consider a shallow arch to be made of polycrystalline silicon with length
m, width
m, thickness
m, and two horizontal offsets of
m and
m. First, in one of our previous papers [
26], we have compared the simulated static deflection of the out-of-plane fringing-fields-based actuator arrangement with some experimental data that showed an acceptable level of agreement. Furthermore, in a separate publication [
28], we have conducted a comparative analysis between the numerical simulations and data presented by another research group [
17], resulting in a satisfactory level of agreement.
4.1. In-Plane Parallel Plates Actuation
Next, we consider investigating, as a first study, the variation of the maximum static deflection and the first three natural frequencies of the actuator under only parallel-plate DC electrostatic force.
Figure 2 clearly demonstrates that the shallow arch would not experience a “bi-stability”-like behavior for all values of the actuator’s initial curvature, when the in-plane and out-of-plane voltages
and
are varied. As can be seen in
Figure 2, for the case of
m and lower, only one stable equilibrium position at one DC voltage parameter load is registered. For this particular case, the beam’s mid-point deflection starts to increase gradually, with a softening-like behavior, as revealed by a decrease in the first three natural frequencies, mainly governed by the quadratic nonlinearity which is dominated by the initial curvature,
Figure 3a. Formerly, when reaching the flat arrangement, the arch displacement upsurge was swiftly accompanied by an increase in all natural frequencies,
Figure 3a, indicating a strong cubic stiffening nonlinearity governed by the mid-plane stretching effect. Finally, the microbeam’s maximum deflection curve shows a slope going to infinity which indicates that pull-in instability has been reached (when the arch reaches the lower stationary electrode) and this is complemented by a drop to zero for the fundamental first frequency,
Figure 3a.
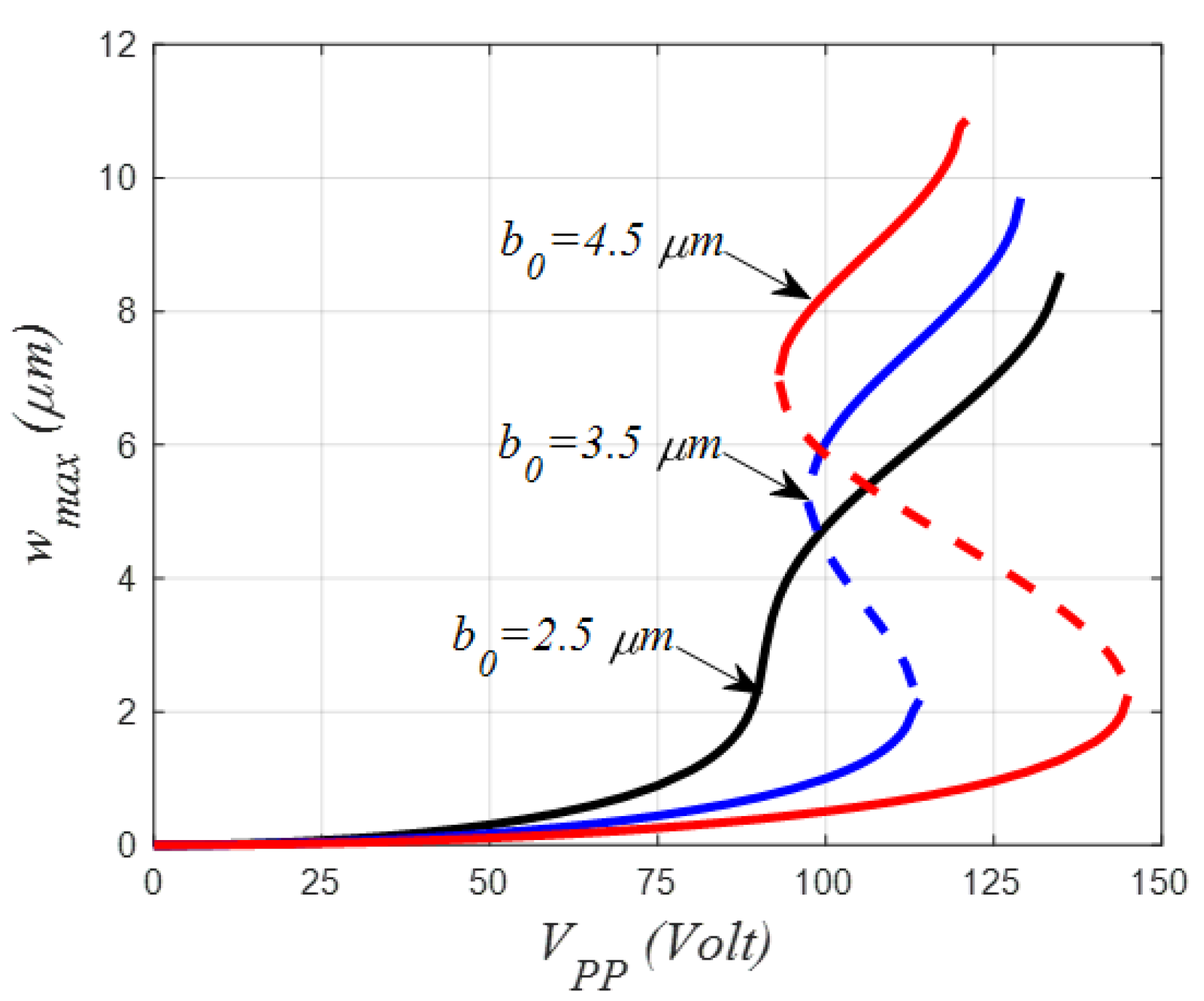
For the cases of
m and
m, respectively, the shallow arch static curves show a bi-stable-like behavior with a possibility of coexistence between two stable equilibrium positions: one when the beam is curved up away from the lower stationary electrode (initial curvature) and a second one when the beam is concave down close to the lower electrode (counter curvature),
Figure 2. In both cases, the static curves depicted in this figure are showing two slopes going to infinity illustrating both snap-through (when the beam travels from initial to counter curvature solutions or vice versa) and pull-in instabilities, respectively.
Nevertheless, only the case of
m is showing that the arch can undergo the snap-through instability before the pull-in with the increase in the DC voltage because the registered snap-through voltage, in this particular case, is found to be lower than the pull-in voltage. Contrarily, for the case of
m, the arch is forced to pull-in without snap-through, having recorded a snap-through voltage greater than the pull-in one. Regarding the variation of the natural frequencies, both cases show a monotonic decrease in all three first natural frequencies when the beam is in the first equilibrium state, concluded by a drop to zero for the fundamental frequency, indicating that instability corresponding to the snap-through voltage has been reached. Afterward, the shallow arch regains stability, accompanied by an increase in the three lowest natural frequencies when the arch is deflected in the neighborhood of the second stable state. This increase is then completed by a drop to zero for the fundamental frequency, corresponding to the pull-in instability. It is worth noting that all the frequency variation curves portrayed in
Figure 3 are not displaying possible mode interactions, neither mode-veering nor mode-crossing.
4.2. Out-of-Plane Parallel-Plates Actuation
Next, the variation of the shallow arch maximum static mid-point deflection (
Figure 4 and
Figure 5) and their three lowest natural frequencies (
Figure 6,
Figure 7 and
Figure 8) are examined for various out-of-plane DC fringing fields loads, while considering two lateral gap sizes of
m and
m.
As can be seen from
Figure 4 and
Figure 5, all the static curves show a slow increase (bending regime) followed by a rapid one (catenary regime) for small DC loads [
26]. Also, all displacement curves are concluded by a slow variation trend until the shallow arch static deflection converges to its initial rise-assumed value
(elastic regime). In both
Figure 4 and
Figure 5, we have also investigated the effect of assuming quarter and half-sized side stationary electrodes, both considered symmetrically from the midspan of the flexible electrode. It is worth noticing that only a single stable equilibrium position was registered for each case, with a faster increase in the static deflection, with a higher DC load, for the case of the half-size electrode as compared to the quarter one. Nevertheless, when considering
m, quarter-sized electrode, and higher initial rise value, two stable equilibria were possible at certain DC loads, illustrating a symmetry-breaking of the shallow arch under out-of-plane DC load actuation. Therefore, this is a good indication that such out-of-plane fringing field types of actuation are very interesting when considered from two different perspectives: increasing the electrostatic load through decreasing the lateral gap size and/or increasing the side electrode sizes, both resulting in faster stroke regarding the applied DC voltage. On the contrary, when considering the opposite case (higher lateral gap size and lower side electrode size), one can get a possible bi-stable stroke-like behavior.
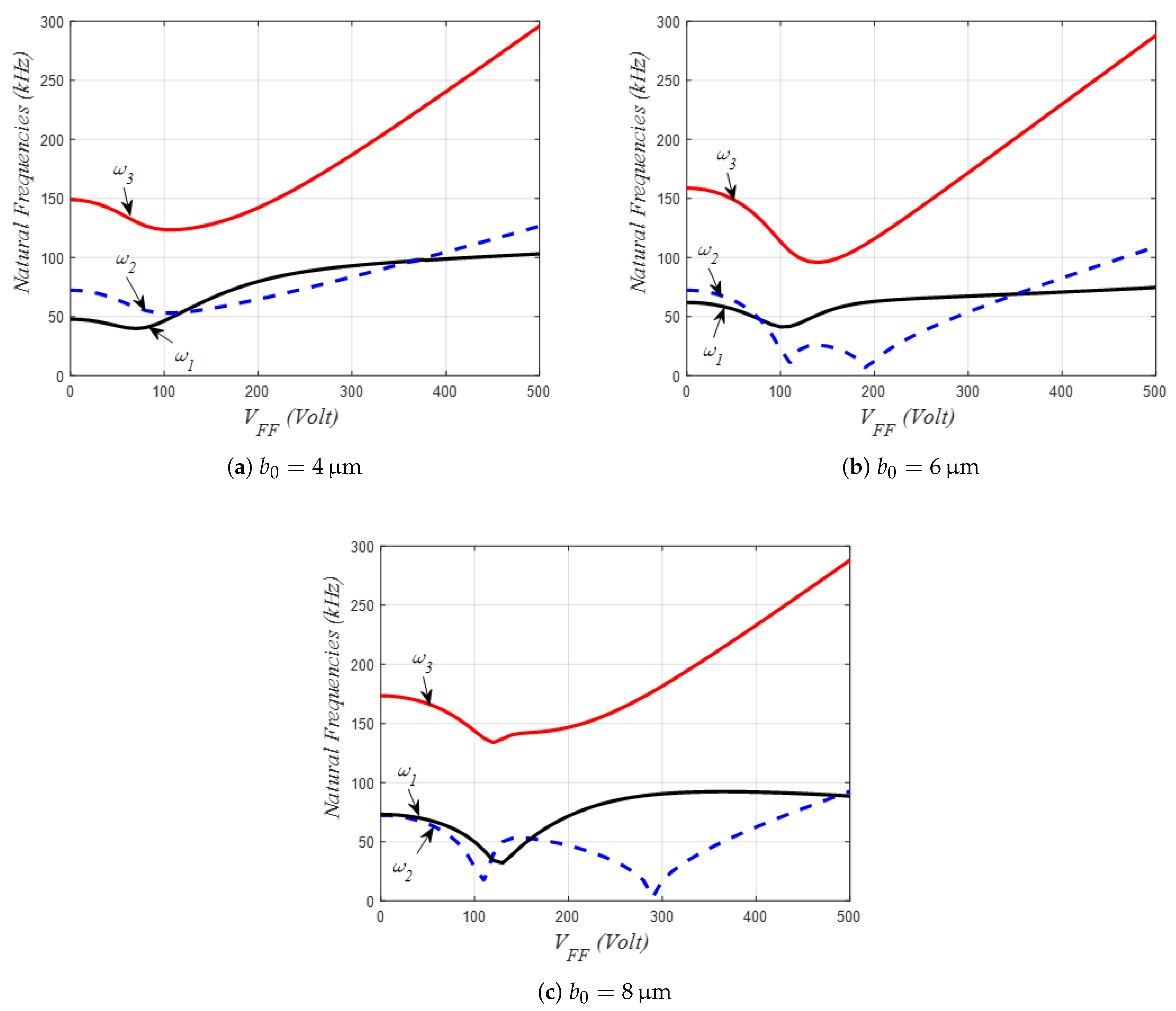
In the following,
Figure 6,
Figure 7 and
Figure 8 portray the effects of the shallow arch initial rise
, the lateral gap size, and side electrodes size on the lowest three natural frequency variations.
Figure 6 examines the case of
m with quarter-sized side electrodes. As can be seen, for a lower value of initial rise,
m, all three lowest frequencies are formerly decreasing with the DC load, certainly because of the dominance of the initial curvature effect,
Figure 6a. Then, increasing further the DC load, the shallow arch reaches the near-flat shape, and consequently the mid-plane stretching effect induces an increase in the fundamental frequency until crossing the second natural frequency before departing away from each other. This is well known as the crossover phenomena, where both modes are considered to be linearly decoupled. Next, additional DC amplitude brings the second mode frequency to higher values until again crossing the first frequency in a second crossover occurrence. For the case of higher values of
,
Figure 6b,c, the same crossover occurrences were recorded, moreover the second mode frequency is showing two drops to lower values indicating the occurrence of the symmetry-breaking singularity: the arch static shape switching from a symmetric pattern to an antisymmetric one. It is worth noting here that for all above cases, the first and third frequencies did not show any clear indication of mode interaction among their variation with the DC load. On the contrary, when considering the case of the half-sized side electrode,
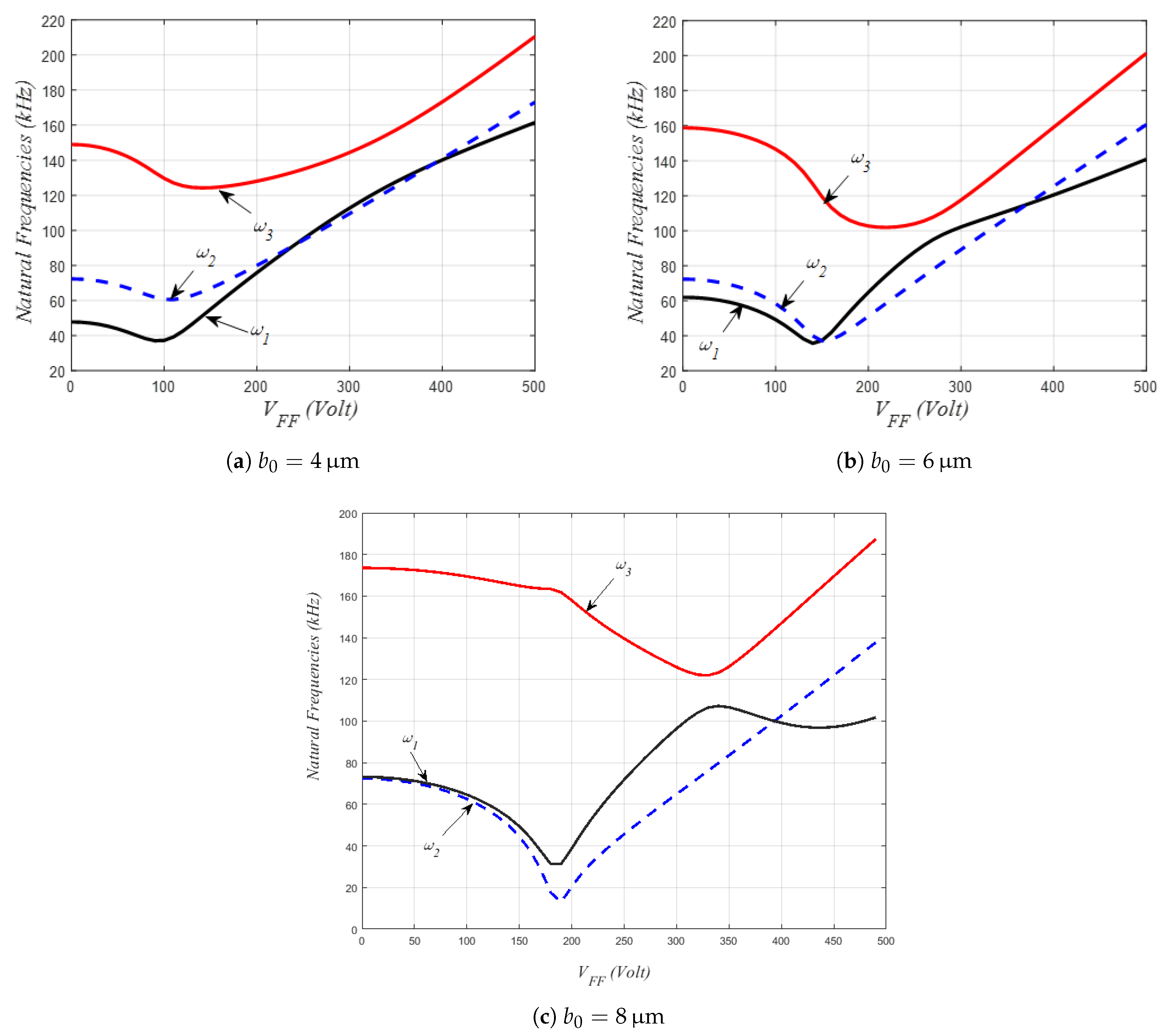
Figure 7, and for higher values of
and
m, the first and third frequencies showed affirmative signs of modal interaction as their variation clearly exhibited an attraction-like behavior where both frequencies are coming close to each other with the increase in the DC load. The frequencies deviate away from each other in a manner of mode-veering as the DC voltage is further increased,
Figure 7b,c. One concludes that such frequency veering occurs when both first and third frequencies are very close to each other. This fact could represent a positive mark of a strong mode localization, which could in turn be very practical for many MEMS applications ranging from energy harvesting to mass sensing.
The same process is also obtained when considering the case of
m with half-sized side electrodes, as the first–second mode-crossings and the first–third mode-veering are arising at higher values of DC voltage, as compared to the case of
m,
Figure 8.
4.3. Combined Parallel-Plate and Fringing Field Actuations
It would be of great interest to many MEMS designers to understand the difference between the behavior of the shallow-arch actuator once actuated with a combined in-plane and out-of-plane electrostatic arrangement. Therefore, the case to be examined subsequently is the superposition of both parallel plates (PP) and fringing fields (FF) DC actuation arrangements,
Figure 9 and
Figure 10, for the case of
m and half-sized side electrodes.
Figure 9 shows the mid-point static deflection of the shallow arch considering three different values of
, two fixed values of the PP DC load and when varying the out-of-plane FF DC voltage. Recall, from
Figure 2, that for lower values of
, such as
m, the shallow arch possesses only one stable equilibrium under PP DC load. Therefore, for this particular case, and when assuming lower (50 V) or higher (100 V) PP DC voltages (
Figure 9a and
Figure 9b, respectively), the shallow arch mid-point static displacement showed only one stable solution in spite of having two opposite stroke directions: downward toward the lower stationary electrode for the case of 50 V and upward away from the same electrode for the case of 100 V, both when increasing the FF DC load.
However, when increasing the initial rise to higher values, such as
m and
m. the shallow arch static deflection may exhibit two distinct but coexisting stable positions: one for lower values of PP DC load (i.e.,
V) and one for higher values of PP DC load (i.e.,
V). For the case of
V PP DC load and increasing the FF DC load,
Figure 11a, the shallow-arch static deflection is showing a mono-stable-like behavior with greater stroke when considering the higher initial rise values. Controversially, when increasing the PP DC load to
V, the shallow arch static profile displayed a bi-stable-like hysteresis behavior: two distinct and coexisting stable strokes: one downward increasing motion toward the lower stationary when the arch is initially in its first stable upper position and one upward, turning away from the same electrode when the arch is initially in its second lower stable position, both when increasing the FF DC load. When the FF DC load reaches a threshold value, the registered bi-stable behavior switches to a mono-stable one, attracting the shallow arch to the straight-like outline under the effect of the FF DC load.
Figure 10 shows the mid-point static deflection of the shallow arch for the case of
m, four fixed values of the FF DC load (
m and half sized side electrodes), and when varying the in-plane PP DC amplitude. Recall, from
Figure 2, that for such an initial rise case, the shallow arch possesses a hysteresis bi-stable-like static behavior under PP DC load. Then, for this particular case, and when we gradually increased the FF DC amplitude (
Figure 10), the shallow-arch mid-point static displacement bi-stable band is reduced until showing a mono-stable-like response with limited stroke. The existence of an invariant displacement for different
voltages, corresponding to the point where the microbeam is almost straight, can also be noticed (
Figure 10), with a maximum displacement equal to the initial rise
. At this point, the out-of-plane fringing fields have no effect on the microbeam because the electrostatic forces are balanced from the upper and lower side of the microbeam.
The combined effect of the microbeam static deflection under in-plane parallel-plate and out-of-plane fringing fields DC amplitudes, is shown in
Figure 11. One can remark that with a highly applied
voltage (more than 100 V), the microbeam is strongly bound to a straight beam-like configuration where the maximum displacement is equal to the initial rise
. This behavior is verified for the three different tested initial rises
m,
m, and
m shown in
Figure 11a–c, respectively. For lower
voltages, the behavior is dominated by
with a classical response of a parallel plate actuator. Nevertheless, the introduction of relatively low values of
can mitigate the effect of
, leading to an attenuation of the amplitudes which reduces nonlinear responses such as snap-through and pull-in. In
Figure 11b,c, the introduction of
can even switch the response from mono- to bi-stable potential well solutions. Since the transition between these regimes requires relatively low applied
voltages, this behavior could be further investigated with a dynamic actuation to develop new detection mechanisms, such as mass-sensing applications.
Figure 12 shows the variation of the lowest three natural frequencies of the shallow arch when considering a combined effect of PP and FF actuations. The effect of varying the FF and PP voltages, and for several values of the initial rise, is portrayed. As seen, the first/second frequencies’ crossover and first/third frequencies’ veering are further noticeable at higher values of the arch’s initial rise and PP DC load, respectively, when varying the FF load. On the contrary, when allowing the PP DC load to vary for discrete values of FF amplitude lower than
V, all of the lowest three frequencies are monotonically varying without a single sign of mode-veering nor mode-crossing. For
around
V,
V and for relatively large initial rises (
m and
m in
Figure 12b,c, the existence of a bi-stable solution in the static domain generates new natural frequencies corresponding to each of the static solutions. On the other hand, the responses shown in
Figure 12 indicate that, for such a combined actuation design, it would be more practical to vary the FF DC load rather than the PP load if one needs to design a localized mode-based sensor with better stroke and improved tunability.
To detect possible veering and crossover between the different modes of the shallow arch microbeam calculated and displayed by
Figure 12, density plots are generated for three possible configurations: (i) crossing between the first and second natural frequencies shown in
Figure 13a–c, (ii) veering between first and third natural frequencies shown in
Figure 13d–f, and (iii) crossing between two times the first natural frequency and the third one shown in
Figure 13g–i. For the first and third cases, the idea is to show possible one-to-one and three-to-one internal resonances that could arise in the microbeam dynamic response, such as those reported in the literature [
29]. The second case can be used to detect possible localization phenomena between the first and third modes [
30].
Figure 13a,b shows that crossing can be obtained between the first and second natural frequencies for any applied
voltage until
V. For the applied voltage
, the crossing occurs around
V for the case where
m. However, for the other initial rises (
m and
m), the crossing occurs for two different values of
:
V and
V for
m and
V and
V for
m.
Crossing between the third and double of the first natural frequencies, corresponding to the second case, is found to be specific to nearly a single
voltage and almost independent of the
voltage, as shown in
Figure 13g–i. It is located around
V for all value of
, with a very small decrease of
as
is increased, and also a very small increase of
as
is increased.
For the second case, where the difference between first and third natural frequencies is shown in
Figure 13d–f, three different behaviors are observed here for the plot region where the minimum frequency value of the difference is calculated. For
m, in
Figure 13d, the applied
voltage is around
V and
can vary from 0 to
V, with a minimum frequency difference of
kHz. While for
m, in
Figure 13e, we detected that
can vary from 0 to
V and
should be around
V. The minimum frequency difference is around
kHz. For
m, in
Figure 13f, the minimum frequency difference is about
kHz, and it is obtained for the applied voltages
V and
V.