Enhancing Dynamic Bandwidth of Amplified Piezoelectric Actuators by a Hybrid Lever and Bridge-Type Compliant Mechanism
Abstract
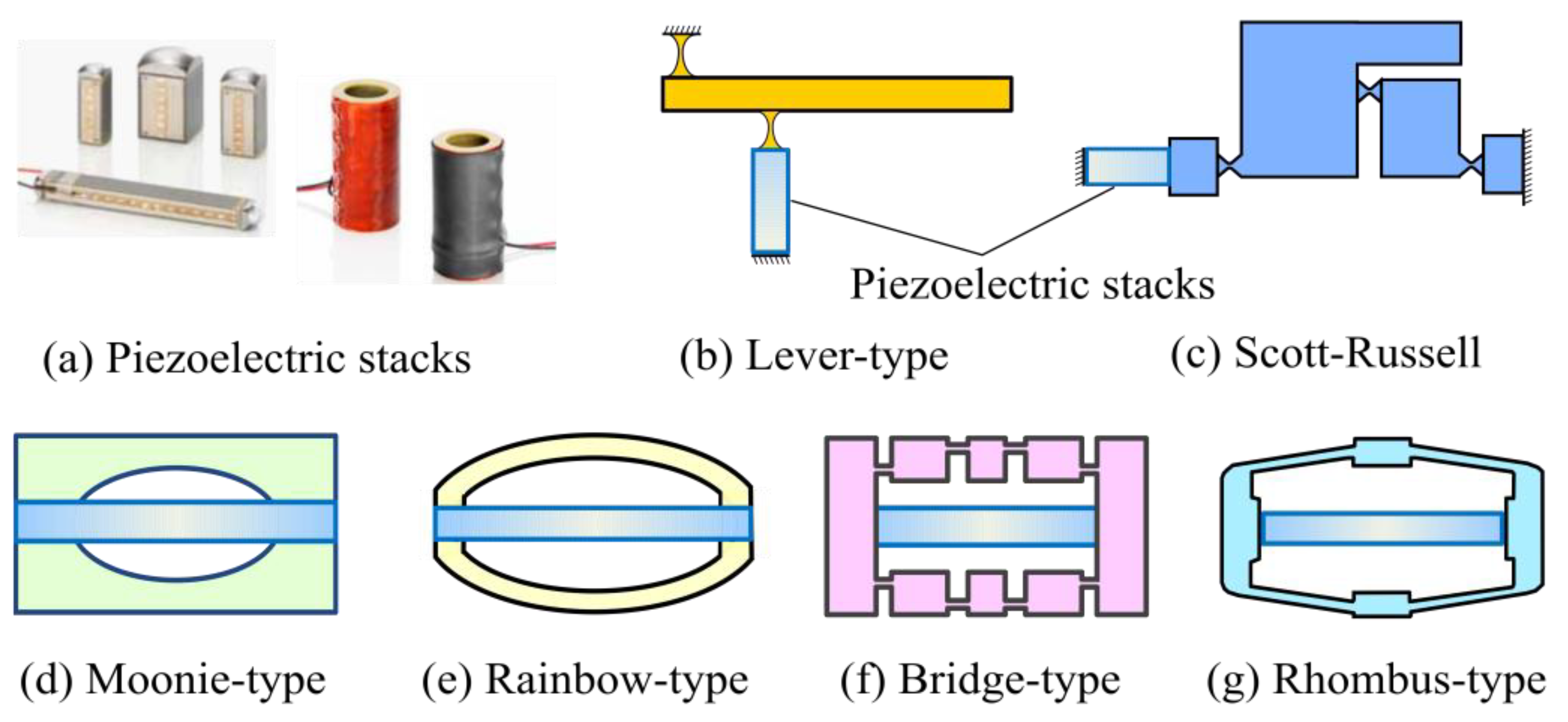
:1. Introduction
2. Operational Principle and Configuration
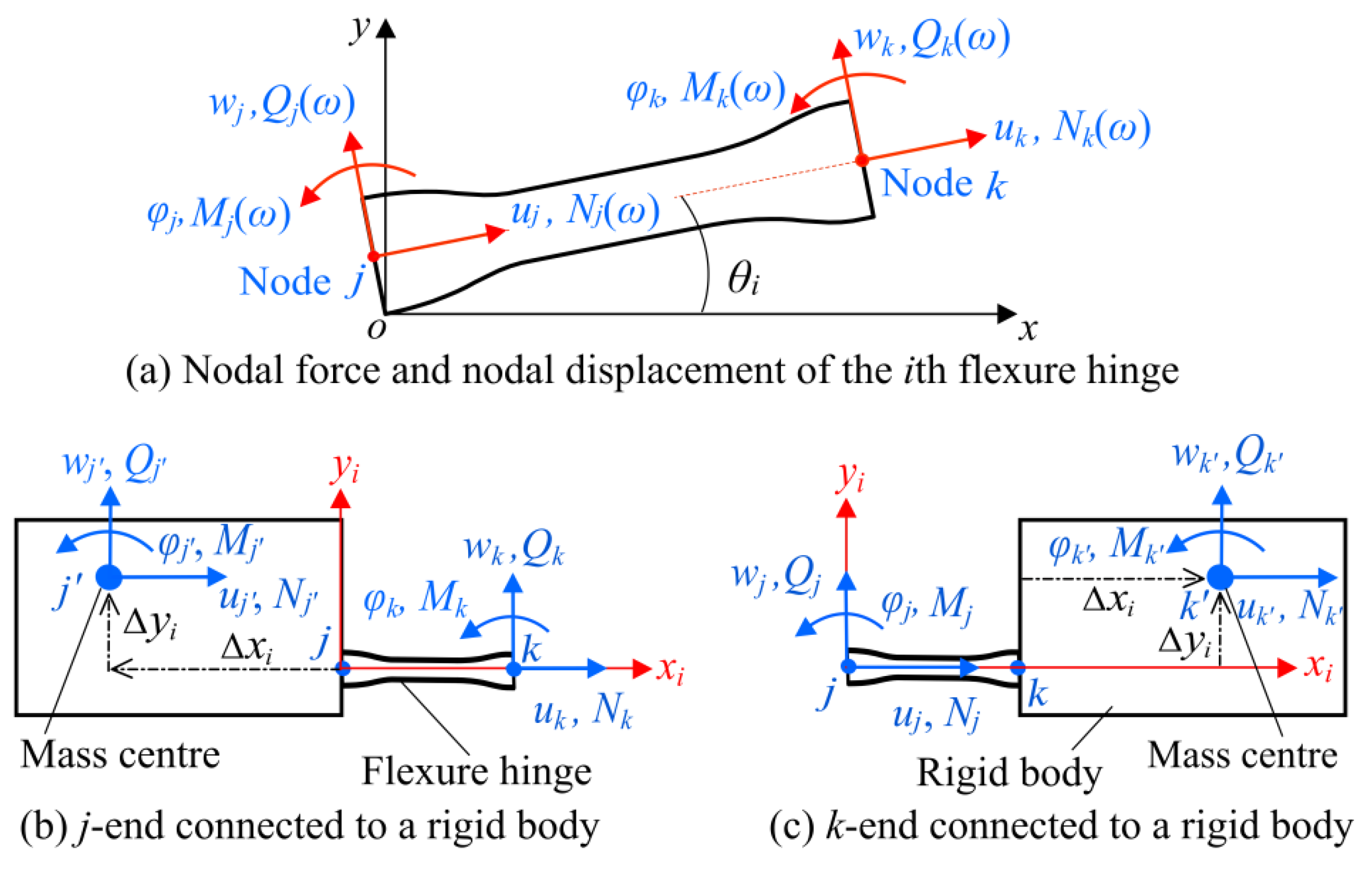
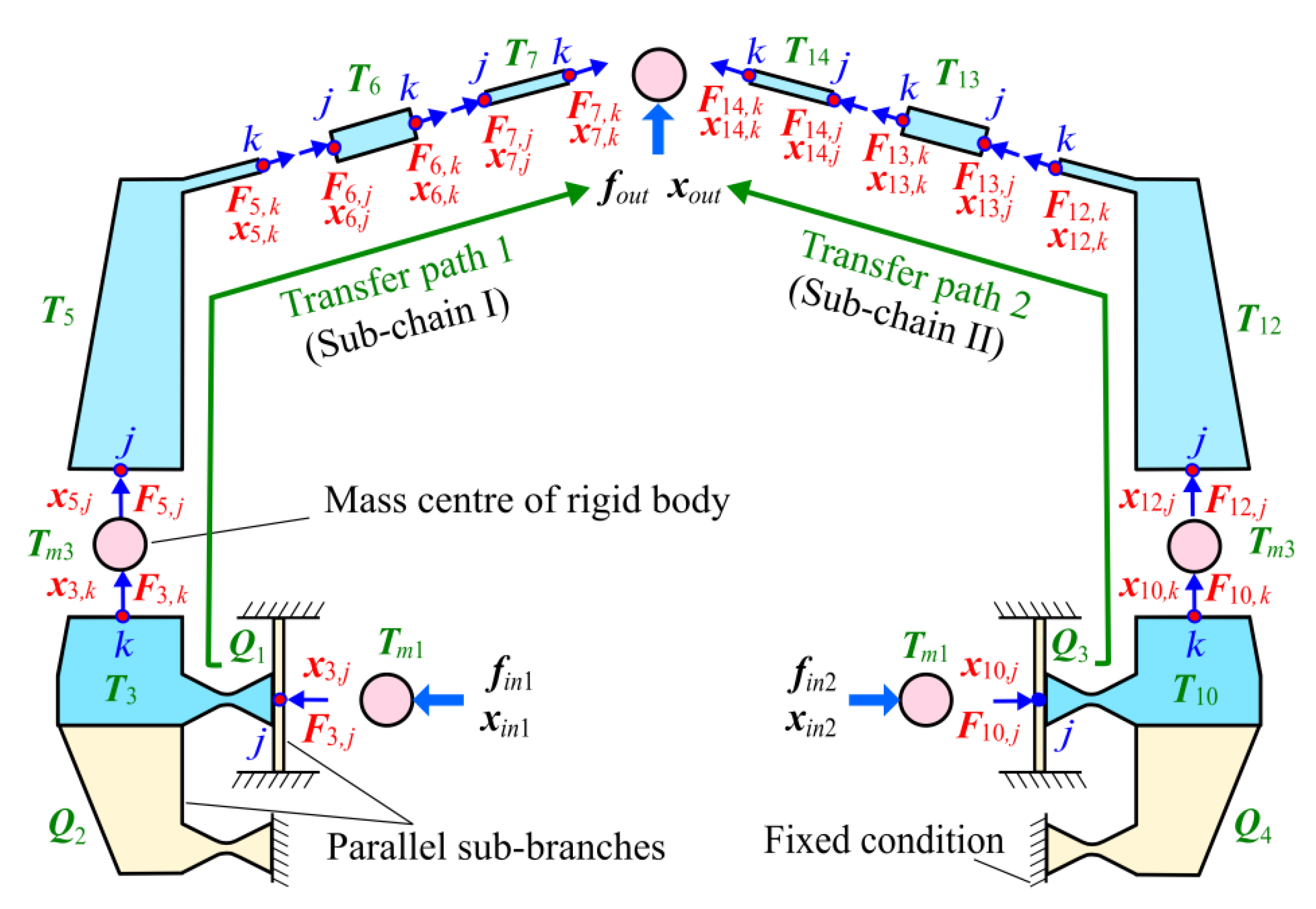
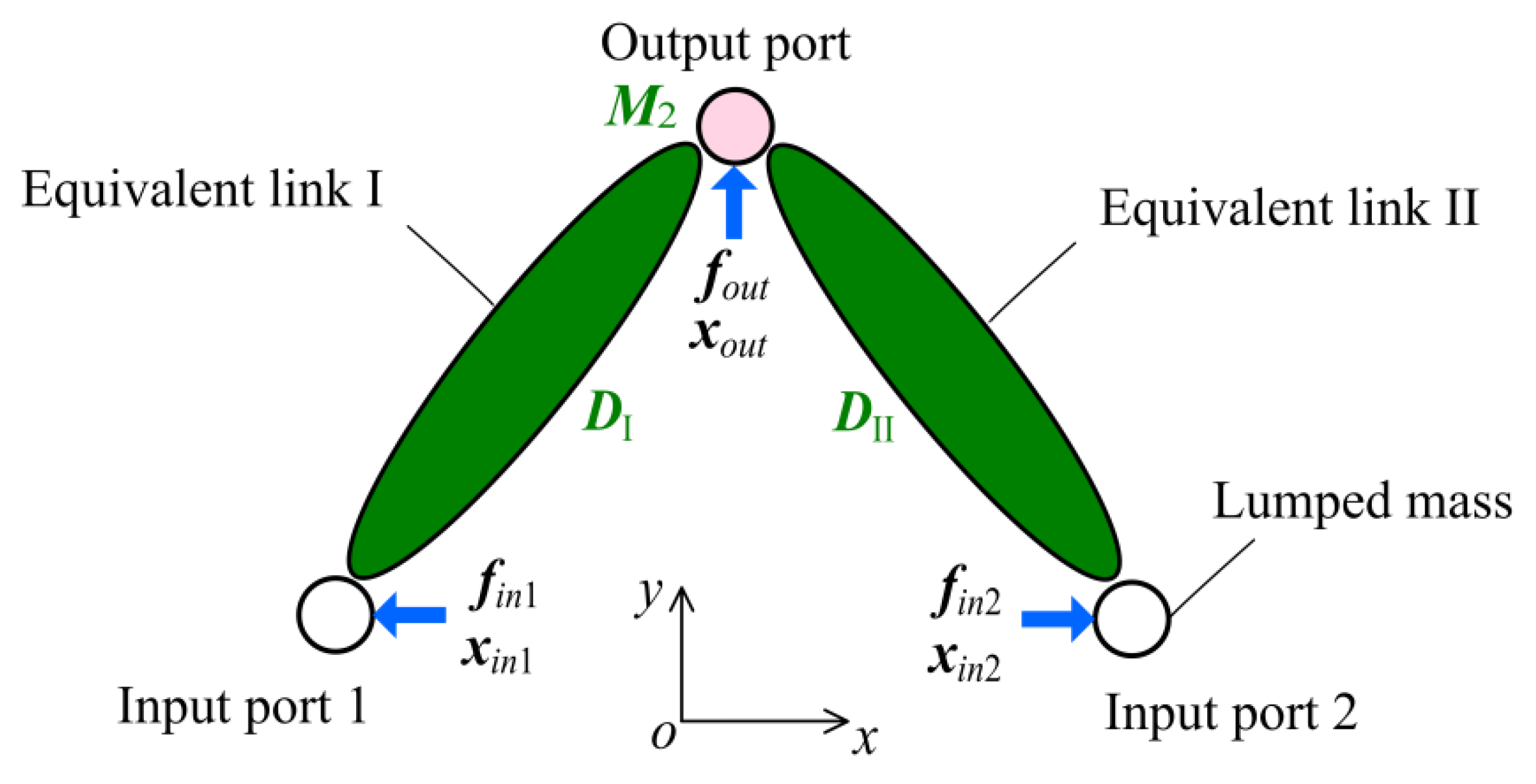
3. Parametric Formulation
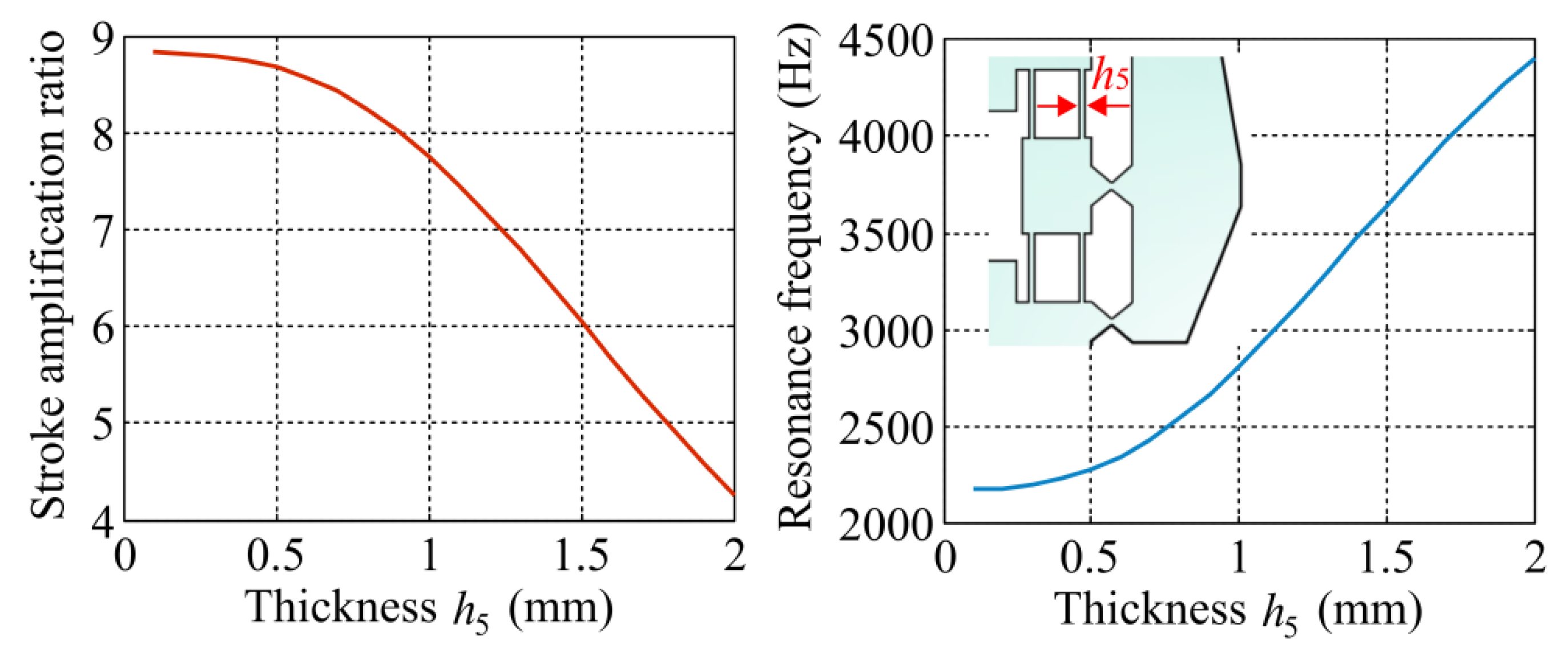
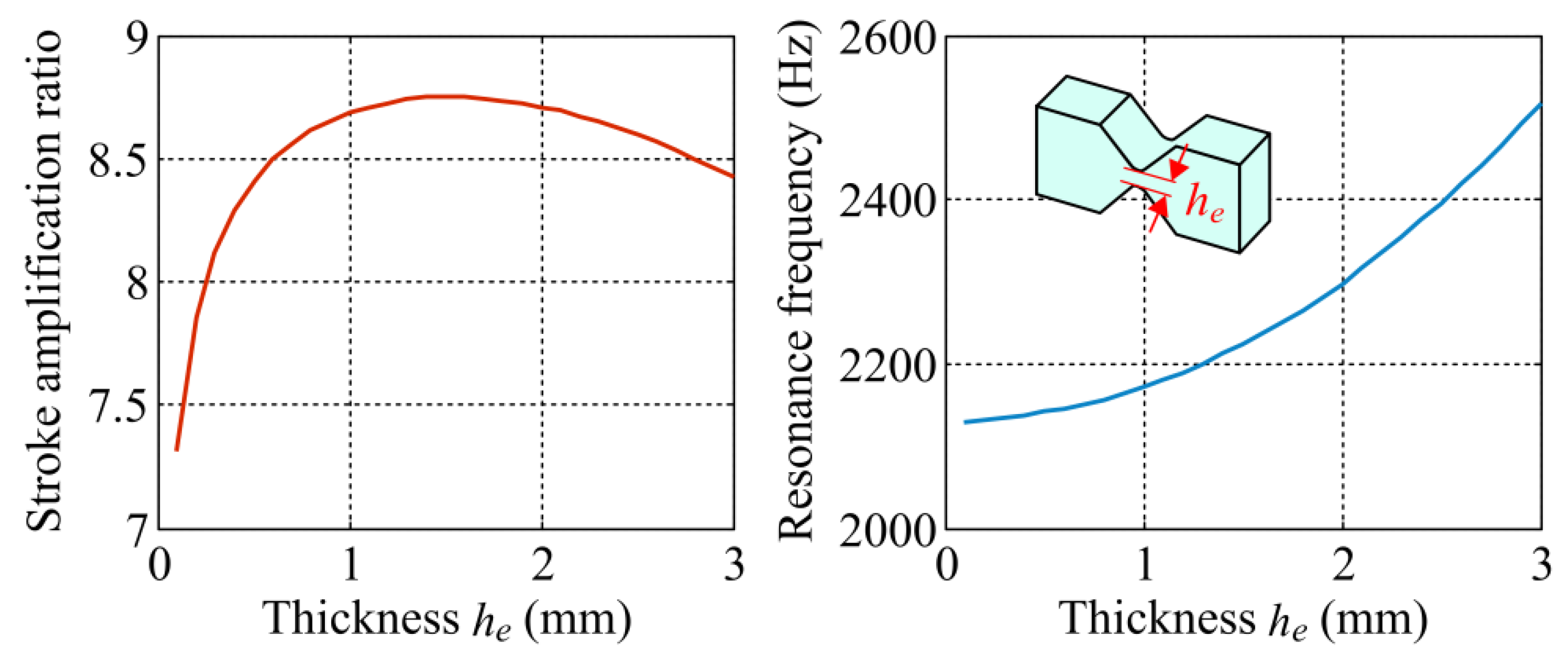
4. Parameter Influence Analysis
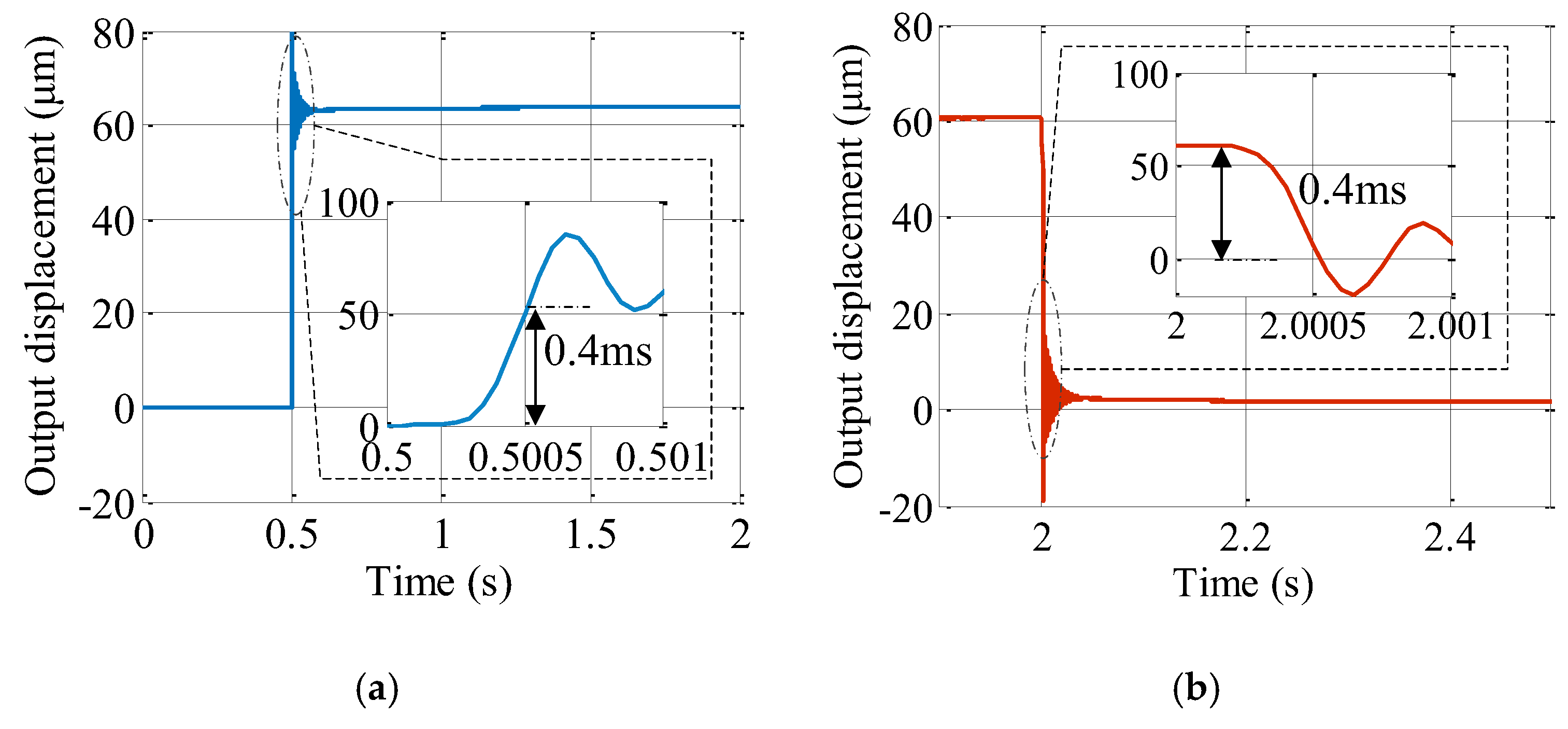
5. Prototype and Experimental Testing
6. Conclusions
- (1)
- Combining lever-type and semi bridge-type complaint amplifying mechanisms, we proposed a new amplified piezoelectric actuator. Although these two types of compliant mechanisms might not be perfect from a practical point of view, our combination enables an improved performance. We showcase by comparing with previous designs such a hybrid displacement amplification mechanism can reach a resonance frequency of 2.1 kHz and the displacement amplification ratio of 6 within a compact size of 50 mm × 44 mm × 7 mm.
- (2)
- The benefits of using a comprehensive two-port dynamic stiffness model for the dimension synthesis have been validated by extensive studies of the parameter influence analysis. The traditional transfer matrix method, which is rarely used in the presence of serial-parallel compliant mechanisms including rigid bodies and complex branches, now is able to successfully utilize for such a complicated application scenario in a way of conciseness.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Feng, Z.; Ming, M.; Ling, J.; Xiao, X.; Yang, Z.X.; Wan, F. Fractional delay filter based repetitive control for precision tracking: Design and application to a piezoelectric nanopositioning stage. Mech. Syst. Signal. Process. 2022, 164, 108249. [Google Scholar] [CrossRef]
- Zhang, L.; Liu, P.; Yan, P. A novel compact tilt stage with additive manufacturable spatial flexure mechanism driven by asymmetric stiffness. Mech. Machine. Theory 2021, 166, 104443. [Google Scholar] [CrossRef]
- Navale, R.; Patil, A.K.; Minase, J.; Pandhare, A. Development of a novel three degree of freedom XYθZ micro-motion stage. Indian J. Sci. Technol. 2021, 14, 1758–1774. [Google Scholar] [CrossRef]
- Das, T.K.; Shirinzadeh, B.; Al-Jodah, A.; Ghafarian, M.; Pinskier, J. A novel compliant piezoelectric actuated symmetric microgripper for the parasitic motion compensation. Mech. Mach. Theory 2021, 155, 104069. [Google Scholar]
- Nguyen, D.N.; Ho, N.L.; Dao, T.P.; Le Chau, N. Multi-objective optimization design for a sand crab-inspired compliant microgripper. Microsyst. Technol. 2019, 25, 3991–4009. [Google Scholar] [CrossRef]
- Lyu, Z.K.; Xu, Q.S. Design and testing of a new piezoelectric-actuated symmetric compliant microgripper. Actuators 2022, 11, 77. [Google Scholar] [CrossRef]
- Yang, X.; Zhu, L.; Li, S.; Zhu, W.; Ji, C. Development of a novel pile-up structure based nanopositioning mechanism driven by piezoelectric actuator. IEEE/ASME Trans. Mechatron. 2020, 25, 502–512. [Google Scholar] [CrossRef]
- Zhao, D.; Zhu, Z.; Huang, P.; Guo, P.; Zhu, L.; Zhu, Z. Development of a piezoelectrically actuated dual-stage fast tool servo. Mech. Syst. Signal. Process. 2020, 144, 106873. [Google Scholar] [CrossRef]
- Xu, C.; Damle, T.; Bosworth, M.; Soto, D.; Agarwal, R.; Steurer, M.; Graber, L. Piezoelectrically actuated fast mechanical switch for MVDC protection. IEEE Trans. Power Deliv. 2020, 36, 2955–2964. [Google Scholar] [CrossRef]
- Ren, J.; Cao, Q. Dynamic modeling and frequency characteristic analysis of a novel 3-PSS flexible parallel micro-manipulator. Micromachines 2021, 12, 678. [Google Scholar] [CrossRef]
- Gao, Q.; Li, Y.; Lu, X.; Zhang, C.; Zhang, X.; Cheng, T. A piezoelectric stick-slip linear actuator with a rhombus-type flexure hinge mechanism by means of parasitic motion. Rev. Sci. Instrum. 2019, 90, 096102. [Google Scholar] [CrossRef]
- Wu, H.; Lai, L.; Zhu, L. Analytical model and experimental verification of an elliptical bridge-type compliant displacement amplification mechanism. Rev. Sci. Instrum. 2021, 92, 055109. [Google Scholar] [CrossRef]
- Zhang, Q.; Zhao, J.; Peng, Y.; Pu, H.; Yang, Y. A novel amplification ratio model of a decoupled XY precision positioning stage combined with elastic beam theory and Castigliano’s second theorem considering the exact loading force. Mech. Syst. Signal. Process. 2020, 136, 106473. [Google Scholar] [CrossRef]
- Claeyssen, F.; Letty, R.L.; Barillot, F.; Sosnicki, O. Amplified piezoelectric actuators, Static & dynamic applications. Ferroelectrics 2007, 351, 3–14. [Google Scholar]
- Deng, G.; Wang, N.; Zhou, C.; Li, J. A simplified analysis method for the piezo jet dispenser with a diamond amplifier. Sensors 2018, 18, 2115. [Google Scholar] [CrossRef] [Green Version]
- Xu, Z.; Yang, Z.; Wang, K.; Li, X.; Liu, Y.; Dong, J.; Huang, H. A bionic inertial piezoelectric actuator with improved frequency bandwidth. Mech. Syst. Signal. Process. 2021, 156, 107620. [Google Scholar] [CrossRef]
- Koyuncu, A.; Şahin, M.; Özgüven, H.N. Dynamic behavior of a compliant mechanism driven by stacked piezoelectric actuators. In Structural Health Monitoring, Photogrammetry & DIC; Springer: Cham, Switzerland, 2019; Volume 6, pp. 77–79. [Google Scholar]
- Ming, M.; Feng, Z.; Ling, J.; Xiao, X.H. Hysteresis modelling and feedforward compensation of piezoelectric nanopositioning stage with a modified Bouc-Wen model. Micro Nano Lett. 2018, 13, 1170–1174. [Google Scholar] [CrossRef]
- Rusu, C.; Besoiu, S.; Tatar, M.O. Design and closed-loop control of a piezoelectric actuator [C]. IOP Conference Series, Materials Science and Engineering. IOP Publ. 2021, 1018, 012002. [Google Scholar]
- Chen, F.; Zhang, Q.; Gao, Y.; Dong, W. A review on the flexure-based displacement amplification mechanisms. IEEE Access 2020, 8, 205919–205937. [Google Scholar] [CrossRef]
- Pan, B.; Zhao, H.; Zhao, C.; Zhang, P.; Hu, H. Nonlinear characteristics of compliant bridge-type displacement amplification mechanisms. Precis. Eng. 2019, 60, 246–256. [Google Scholar] [CrossRef]
- Xiao, R.; Shao, S.; Xu, M.; Jing, Z. Design and analysis of a novel piezo-actuated XYθz micropositioning mechanism with large travel and kinematic decoupling. Adv. Mater. Sci. Eng. 2019, 2019, 5461725. [Google Scholar] [CrossRef] [Green Version]
- Schmitt, P.; Hoffmann, M. Engineering a compliant mechanical amplifier for MEMS sensor applications. J. Microelectromech. Syst. 2020, 29, 214–227. [Google Scholar] [CrossRef]
- Yang, Y.; Wu, G.; Wei, Y. Design, modeling, and control of a monolithic compliant XYθ microstage using a double-rocker mechanism. Precis. Eng. 2021, 71, 209–231. [Google Scholar] [CrossRef]
- Li, H.; Guo, F.; Wang, Y.; Wang, Z.; Li, C.; Ling, M.; Hao, G. Design and modeling of a compact compliant stroke amplification mechanism with completely distributed compliance for ground-mounted actuators. Mech. Mach. Theory 2022, 167, 104566. [Google Scholar] [CrossRef]
- Chen, X.; Li, Y.; Xie, Y.; Wang, R. Design and analysis of new ultra-compact decoupled XYZθ stage to achieve large-scale high precision motion. Mech. Mach. Theory 2022, 167, 104527. [Google Scholar] [CrossRef]
- Duan, X.; Cao, D.; Li, X.; Shen, Y. Design and dynamic analysis of integrated architecture for vibration energy harvesting including piezoelectric frame and mechanical amplifier. Appl. Math. Mech. 2021, 42, 755–770. [Google Scholar] [CrossRef]
- Chen, W.; Qu, J.; Chen, W.; Zhang, J. A compliant dual-axis gripper with integrated position and force sensing. Mechatronics 2017, 47, 105–115. [Google Scholar] [CrossRef]
- Liang, C.; Wang, F.; Shi, B.; Huo, Z.; Zhou, K.; Tian, Y.; Zhang, D. Design and control of a novel asymmetrical piezoelectric actuated microgripper for micromanipulation. Sens. Actuators A Phys. 2018, 269, 227–237. [Google Scholar] [CrossRef]
- Tran, N.T.; Le Chau, N.; Dao, T.P. A hybrid computational method of desirability, fuzzy logic, ANFIS, and LAPO algorithm for multiobjective optimization design of scott russell compliant mechanism. Math. Probl. Eng. 2020, 2020, 3418904. [Google Scholar] [CrossRef]
- Ding, Y.; Lai, L.J. Design and analysis of a displacement amplifier with high load capacity by combining bridge-type and Scott-Russell mechanisms. Rev. Sci. Instrum. 2019, 90, 065102. [Google Scholar] [CrossRef]
- Liu, Y.; Xu, Q. Mechanical design, analysis and testing of a large-range compliant microgripper. Mech. Sci. 2016, 7, 119–126. [Google Scholar] [CrossRef] [Green Version]
- Tian, Y.; Lu, K.; Wang, F.; Zhou, C.; Ma, Y.; Jing, X.; Yang, C.; Zhang, D. A spatial deployable three-dof compliant nano-positioner with a three-stage motion amplification mechanism. IEEE/ASME Trans. Mechatron. 2020, 25, 1322–1334. [Google Scholar] [CrossRef]
- Liu, G.; He, Z.; Bai, G.; Zheng, J.; Zhou, J.; Chang, M. Modeling and experimental study of double-row bow-type micro-displacement amplifier for direct-drive servo valves. Micromachines 2020, 11, 312. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Kim, J.H.; Kim, S.H.; Kwak, Y.K. Development of a piezoelectric actuator using a three-dimensional bridge-type hinge mechanism. Rev. Sci. Instrum. 2003, 74, 2918–2924. [Google Scholar] [CrossRef]
- Choi, S.B.; Han, S.S.; Han, Y.M.; Thompson, B.S. A magnification device for precision mechanisms featuring piezoactuators and flexure hinges, Design and experimental validation. Mech. Mach. Theory 2007, 42, 1184–1198. [Google Scholar] [CrossRef]
- Ling, M.; Cao, J.; Jiang, Z.; Lin, J. Theoretical modeling of attenuated displacement amplification for multistage compliant mechanism and its application. Sens. Actuators A Phys. 2016, 249, 15–22. [Google Scholar] [CrossRef]
- Ling, M.; Cao, J.; Pehrson, N. Kinetostatic and dynamic analyses of planar compliant mechanisms via a two-port dynamic stiffness model. Precis. Eng. 2019, 57, 149–161. [Google Scholar] [CrossRef]
- Ling, M.; Zhang, X.; Cao, J. Extended dynamic stiffness model for analyzing flexure-hinge mechanisms with lumped compliance. J. Mech. Des. 2022, 144, 013304. [Google Scholar] [CrossRef]
- Zhu, X.; Xu, X.; Wen, Z.; Ren, J.; Liu, P. A novel flexure-based vertical nanopositioning stage with large travel range. Rev. Sci. Instrum. 2015, 86, 105112. [Google Scholar] [CrossRef]
- Ouyang, P.R.; Zhang, W.J.; Gupta, M.M. A new compliant mechanical amplifier based on a symmetric five-bar topology. J. Mech. Des. 2008, 130, 104501. [Google Scholar] [CrossRef]







| Variables | Values | Variables | Values | Variables | Values |
|---|---|---|---|---|---|
| θ1 | −90 | θ6 | θr | θ11 | 0 |
| θ2 | 90 | θ7 | θr | θ12 | 180 − θr |
| θ3 | 180 | θ8 | −90 | θ13 | 180 − θr |
| θ4 | 180 | θ9 | 90 | θ14 | 180 − θr |
| θ5 | θr | θ10 | 0 |
| Variables | Values | Variables | Values |
|---|---|---|---|
| (Δx3, Δy3) | (Δ1, − Δ2) | (Δx11, Δy11) | (Δ1, Δ2 + l3) |
| (Δx4, Δy4) | (Δ1, −Δ2 − l3) | (Δx5, Δy5) | (−Δ3sinθh − Δ1cosθh, Δ1sinθh − Δ3cosθh) |
| (Δx10, Δy10) | (Δ1, Δ2) | (Δx12, Δy12) | (−Δ3sinθh − Δ1cosθh, Δ3cosθh − Δ1sinθh) |
| Parameters | Values | Parameters | Values | Parameters | Values |
|---|---|---|---|---|---|
| l1 | 5.0 mm | h1 | 0.6 mm | d | 7.0 mm |
| l2 | 5.0 mm | h2 | 1.5 mm | r | 0.4 mm |
| l3 | 10.0 mm | h3 | 4.0 mm | L | 15.0 mm |
| l4 | 24.0 mm | h4 | 4.0 mm | m1 | ρ × d × 5 mm × 7 mm |
| l5 | 5.0 mm | h5 | 0.4 mm | m2 | ρ × d × 2 mm × 4 mm |
| le | 3.0 mm | he | 1.5 mm | m3 | 4.2 g |
| θr | 8.0 deg | θe | 40.0 deg | J3 | 0.4 kg·mm2 |
| Δ 1 | 3.6 mm | Δ 2 | 6.5 mm | Δ 3 | 17 mm |
| Angle | Methods | Static Performances | The First Two-Order Natural Frequencies | |||
|---|---|---|---|---|---|---|
| R | Kin (N/μm) | Kout (N/μm) | fn1 (Hz) | fn2 (Hz) | ||
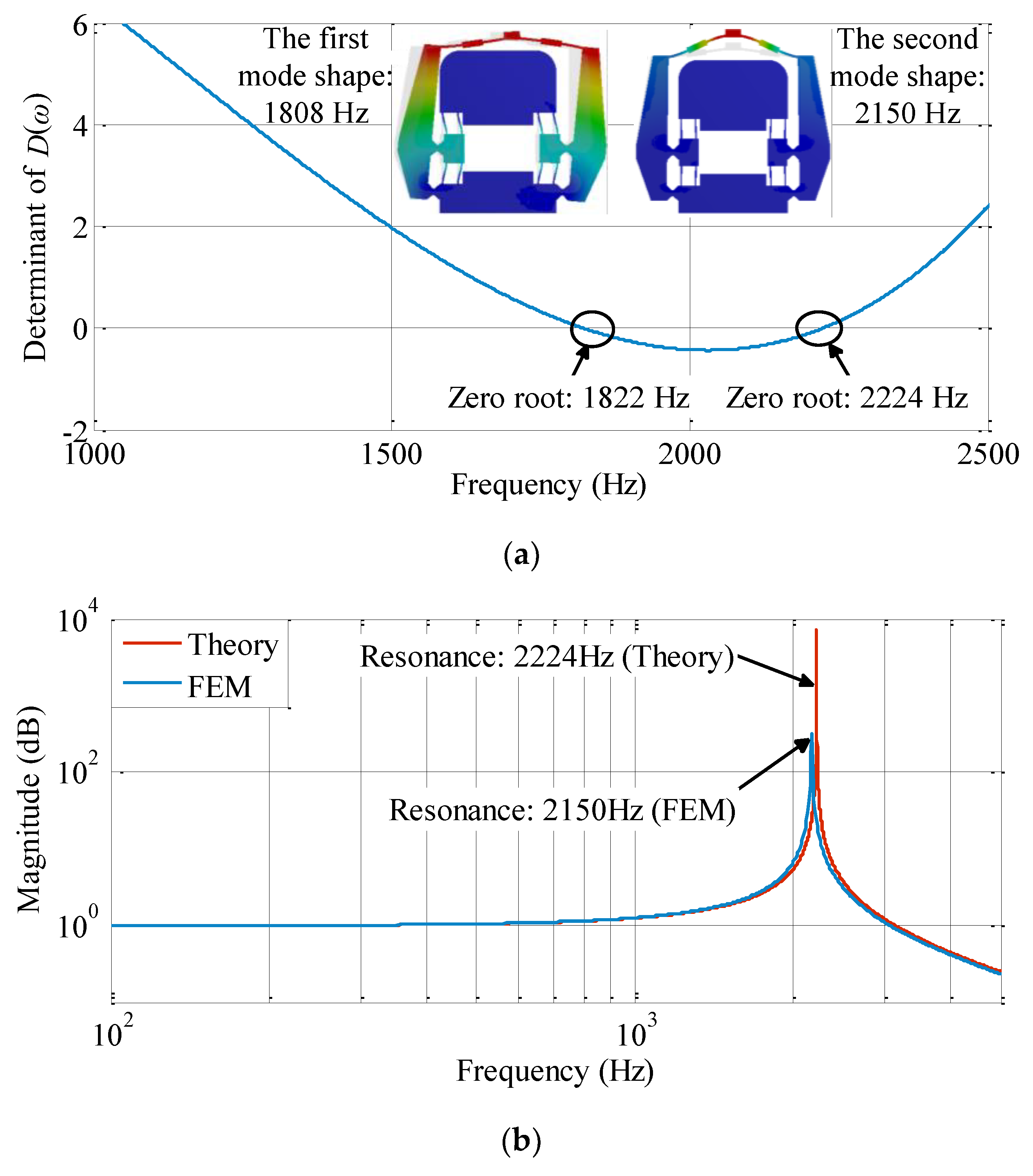
| θr = 8 deg | Theory1 | 10.54 | 20.27 | 0.078 | 1822 | 2224 |
| Theory2 | 5.39 | 11.23 | 0.077 | 1790 | 2223 | |
| FEM | 5.84 | 10.45 | 0.072 | 1808 | 2150 | |
| Error1 | 80.48% | 93.97% | 8.33% | 0.77% | 3.44% | |
| Error2 | 7.71% | 7.46% | 6.94% | 1.00% | 3.40% | |
| θr = 20 deg | Theory1 | 4.62 | 5.92 | 0.132 | 1803 | 2089 |
| Theory2 | 3.88 | 5.28 | 0.126 | 1777 | 2094 | |
| FEM | 3.91 | 4.82 | 0.123 | 1794 | 2073 | |
| Error1 | 18.20% | 22.82% | 7.31% | 0.50% | 0.77% | |
| Error2 | 0.77% | 9.54% | 2.43% | 0.95% | 1.01% | |
| Amplifiers | Size: Length × Width × Height | Output Stroke (Displacement Amplifying Ratio) | Resonance Frequency |
|---|---|---|---|
| Ref. [7] | 120 mm × 80 mm × 25 mm (Approximate value) | 30 μm (R = 10.4) | 1152 Hz |
| Ref. [35] | 30 mm × 30 mm × 15 mm | 80 μm (R = 10) | 190 Hz |
| Ref. [31] | 98 mm × 52 mm × 20 mm | 69 μm (R = 3.51) | 457 Hz |
| Ref. [31] (Traditional bridge-type) | 98 mm × 52 mm × 20 mm | 74 μm (R = 3.70) | 355 Hz |
| Ref. [36] | 134 mm × 50 mm × 20 mm (Approximate value) | 200 μm (R = 20) | 189 Hz |
| Ref. [40] | 92 mm × 50 mm × 18 mm | 214 μm (R = 12.1) | 205 Hz |
| Ref. [41] | 65 mm × 22 mm × 10 mm | 200 μm (R = 16.2) | 628 Hz |
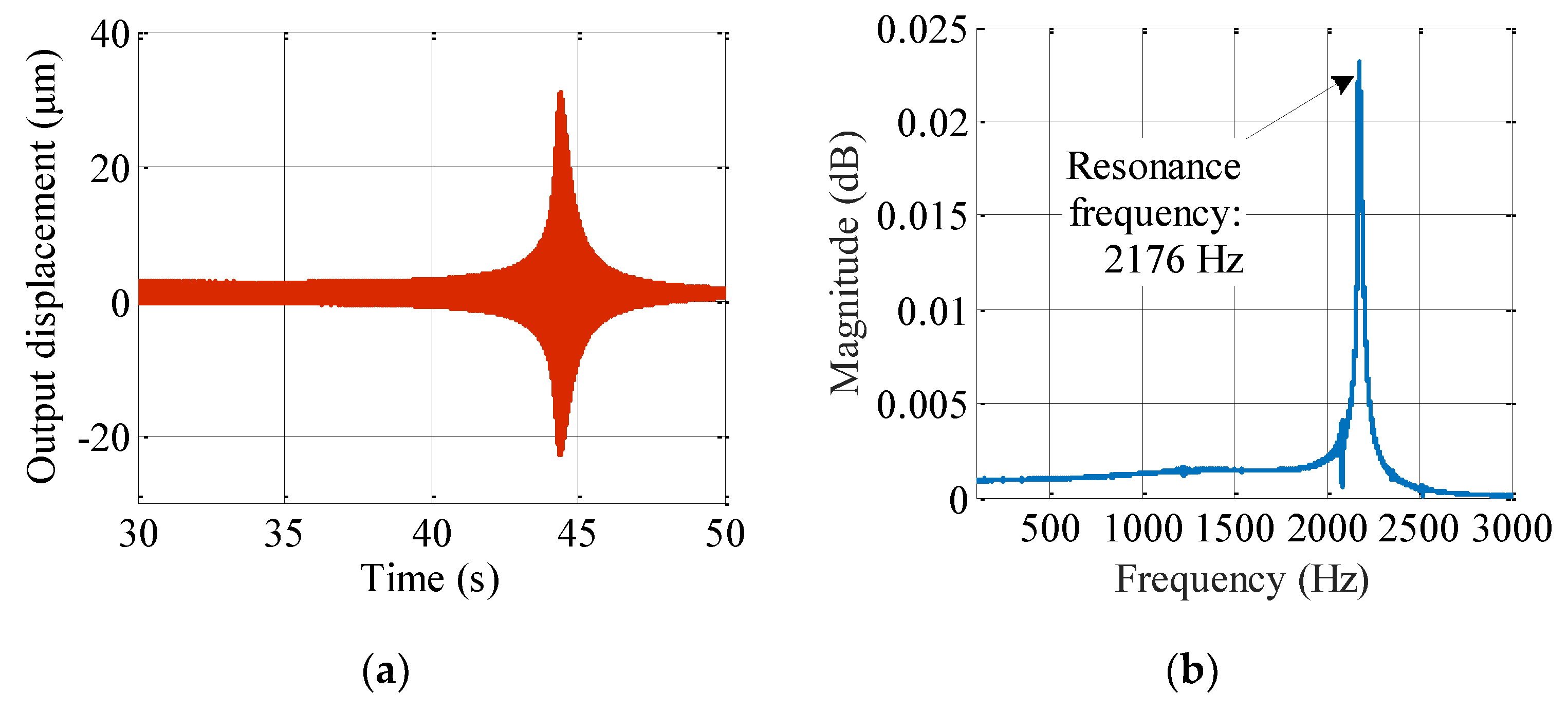
| The presented | 50 mm × 44 mm × 7 mm | 61 μm (R = 6) | 2176 Hz |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ling, M.; Yuan, L.; Luo, Z.; Huang, T.; Zhang, X. Enhancing Dynamic Bandwidth of Amplified Piezoelectric Actuators by a Hybrid Lever and Bridge-Type Compliant Mechanism. Actuators 2022, 11, 134. https://doi.org/10.3390/act11050134
Ling M, Yuan L, Luo Z, Huang T, Zhang X. Enhancing Dynamic Bandwidth of Amplified Piezoelectric Actuators by a Hybrid Lever and Bridge-Type Compliant Mechanism. Actuators. 2022; 11(5):134. https://doi.org/10.3390/act11050134
Chicago/Turabian StyleLing, Mingxiang, Lei Yuan, Zhihong Luo, Tao Huang, and Xianmin Zhang. 2022. "Enhancing Dynamic Bandwidth of Amplified Piezoelectric Actuators by a Hybrid Lever and Bridge-Type Compliant Mechanism" Actuators 11, no. 5: 134. https://doi.org/10.3390/act11050134
APA StyleLing, M., Yuan, L., Luo, Z., Huang, T., & Zhang, X. (2022). Enhancing Dynamic Bandwidth of Amplified Piezoelectric Actuators by a Hybrid Lever and Bridge-Type Compliant Mechanism. Actuators, 11(5), 134. https://doi.org/10.3390/act11050134








