A Review on the Development of Pneumatic Artificial Muscle Actuators: Force Model and Application
Abstract
1. Introduction
- PAMs are lightweight and produce a strong force, which provides high power-to-weight ratio for the applications.
- PAMs provide flexibility and compliance for the applications in a pressurized or unpressurized condition.
- PAMs deliver a safe and compatible interaction with the human environment only by controlling the volume flow rate of operating air into the muscle.
- Simple PAMs can be fabricated from inexpensive materials, which reduce the overall manufacturing cost.
- PAMs provide the linear contractile motion with a monotonically decreasing load-contraction relation like the skeletal muscle, which can control joint compliance.
- PAMs exhibit the nature of essential compliance for installation for providing required power, and the values of speed along with force are in the range of what is needed.
- Because of the direct connection, replacement of a defective PAM with a new one can be done easily and swiftly by uncoupling the muscle along with pneumatic tubing.
- Due to the intrinsic and adjustable compliance, PAMs can be made to have a soft touch and, consequently, are well-suited for safe human–robot interaction.
2. Concept and Operation
3. Classification of Pneumatic Artificial Muscle
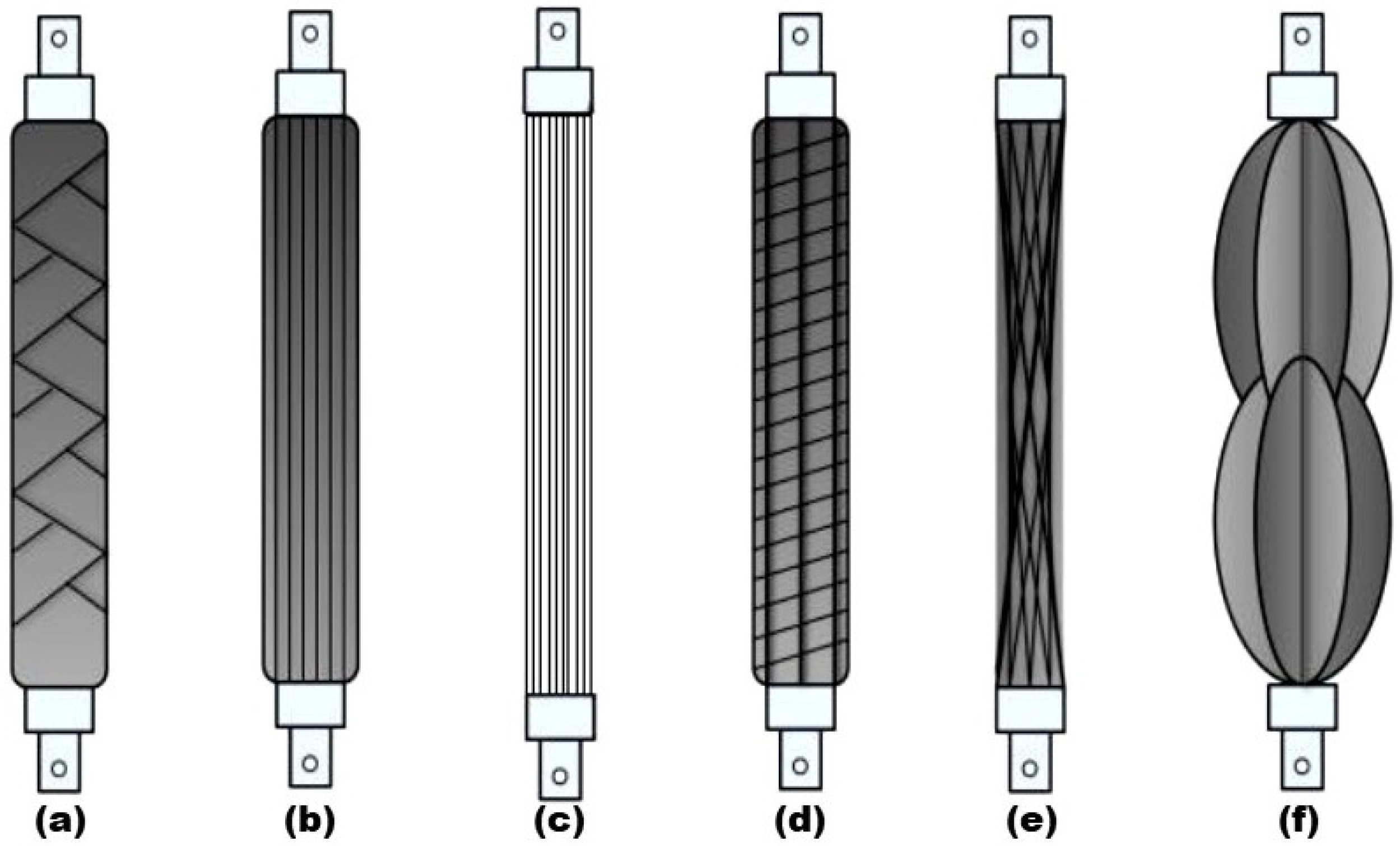
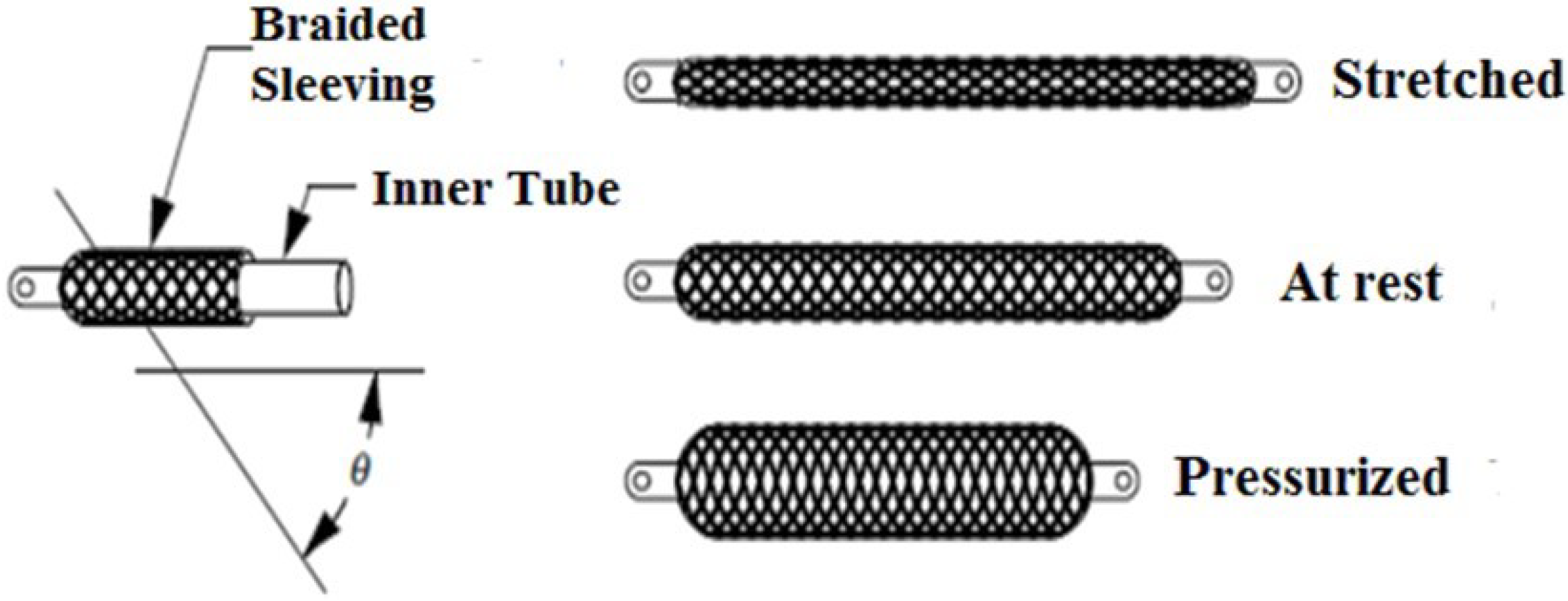
3.1. Braided Muscle
3.2. Pleated Muscle
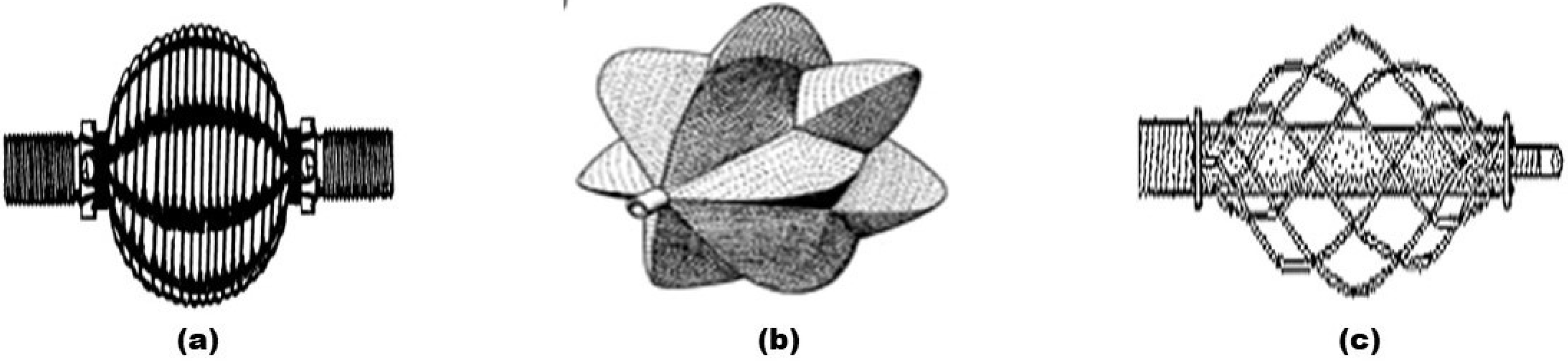
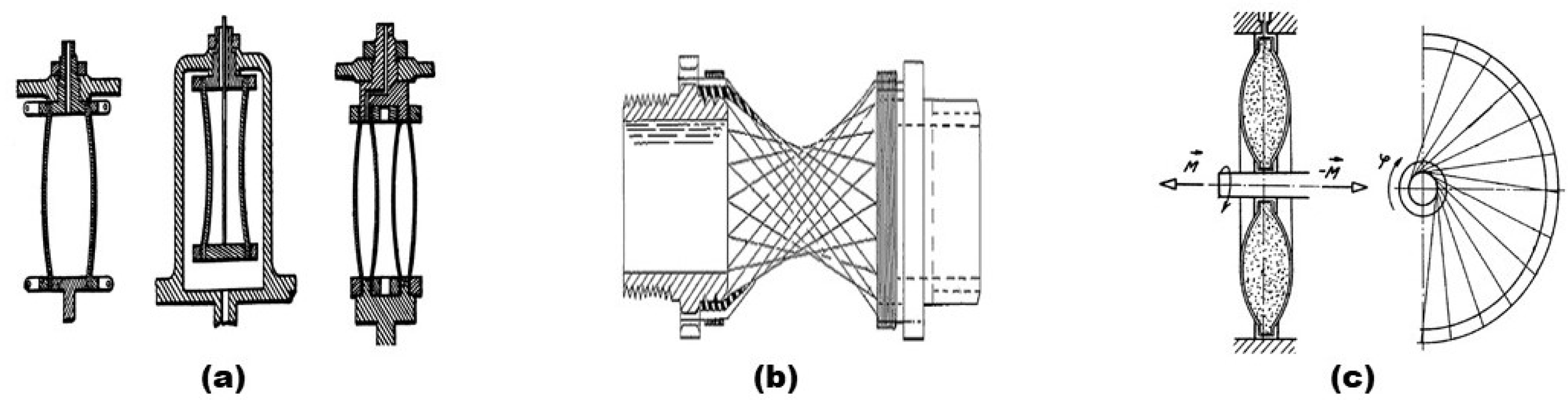
3.3. Netted Muscle
3.4. Embedded Muscle
4. Force Model of Pneumatic Artificial Muscle
4.1. Geometrical Model
4.2. Biomimetic/Biomechanical Model
4.3. Empirical Model
4.4. Nonlinear Model
5. Applications of Pneumatic Artificial Muscle
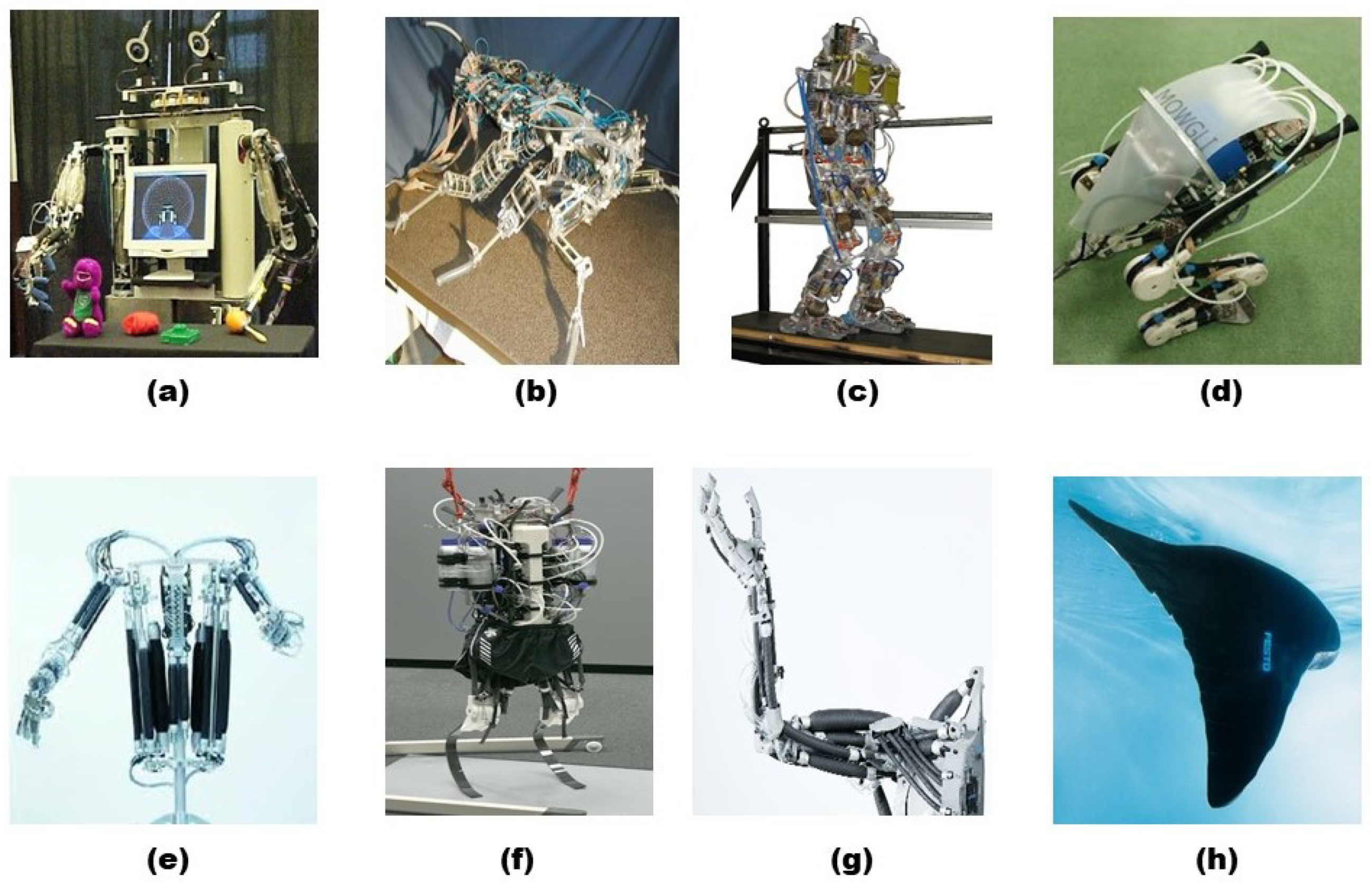
5.1. Biorobotic Applications
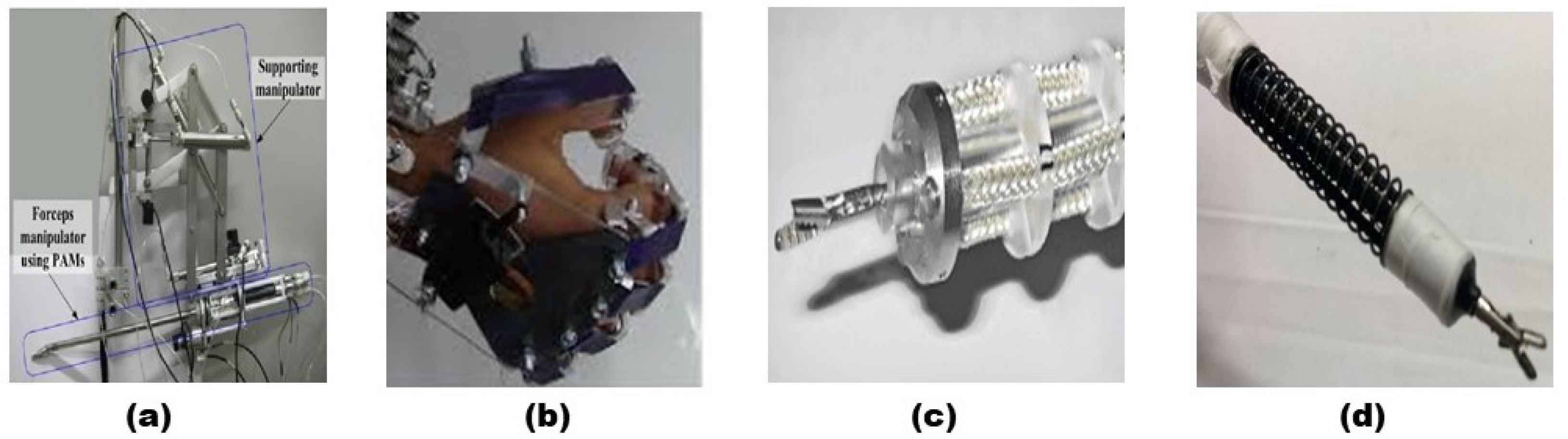
5.2. Medical Applications
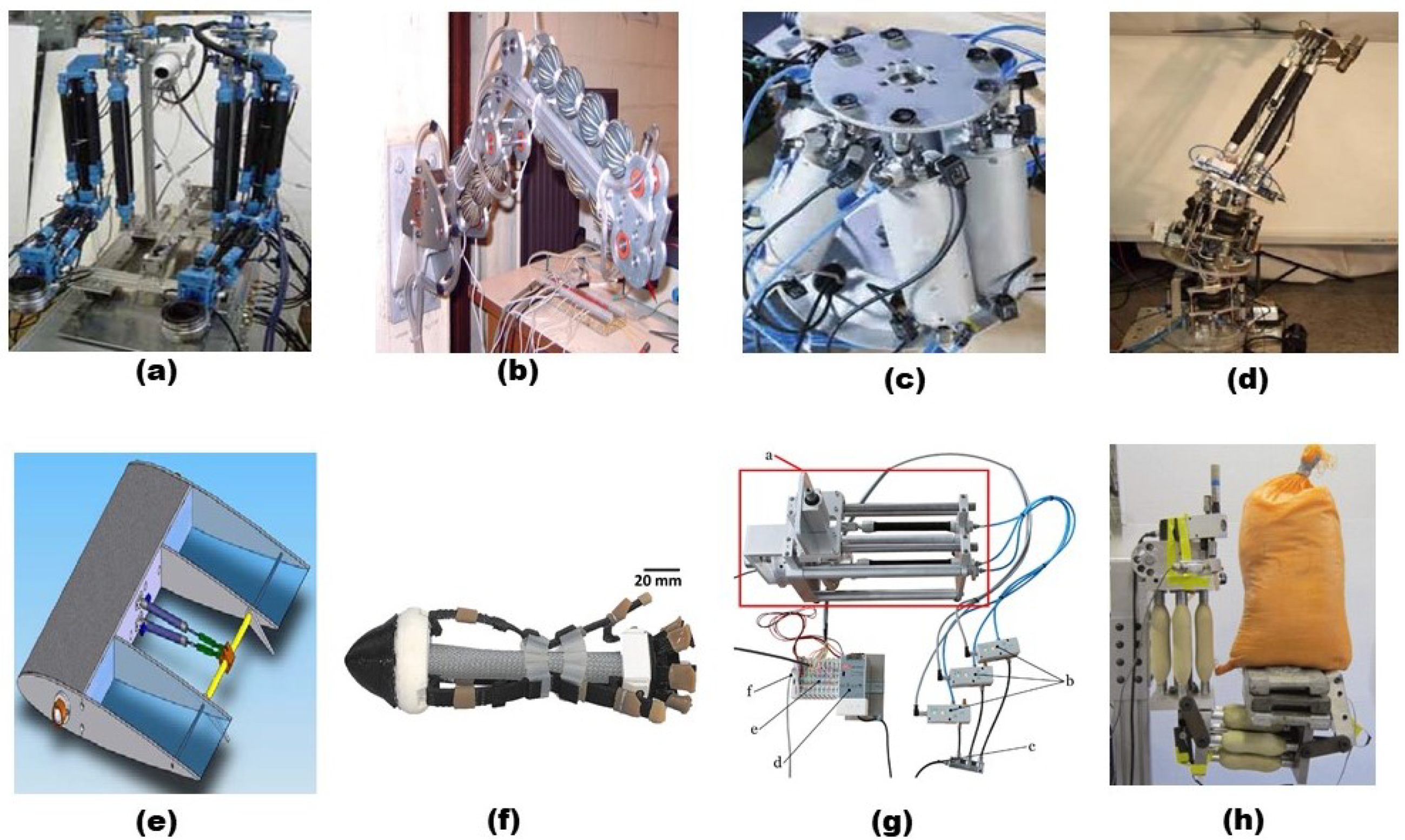
5.3. Industrial Applications
6. Discussion and Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Mirvakili, S.M.; Hunter, I.W. Artificial muscles: Mechanisms, applications, and challenges. Adv. Mater. 2018, 30, 1704407. [Google Scholar] [CrossRef]
- Chou, C.P.; Hannaford, B. Measurement and modeling of McKibben pneumatic artificial muscles. IEEE Trans. Robot. Autom. 1996, 12, 90–102. [Google Scholar] [CrossRef]
- Tondu, B.; Lopez, P. Modeling and control of McKibben artificial muscle robot actuators. IEEE Control Syst. Mag. 2000, 20, 15–38. [Google Scholar]
- Daerden, F.; Lefeber, D. Pneumatic artificial muscles: Actuators for robotics and automation. Eur. J. Mech. Environ. Eng. 2002, 47, 11–21. [Google Scholar]
- Ashwin, K.; Ghosal, A. A survey on static modeling of miniaturized pneumatic artificial muscles with new model and experimental results. Appl. Mech. Rev. 2018, 70, 040802. [Google Scholar]
- Andrikopoulos, G.; Nikolakopoulos, G.; Manesis, S. A survey on applications of pneumatic artificial muscles. In Proceedings of the 2011 19th Mediterranean Conference on Control & Automation (MED), Corfu, Greece, 20–23 June 2011; IEEE: Piscataway, NJ, USA, 2011; pp. 1439–1446. [Google Scholar]
- Kalita, B. Nonlinear Dynamic Analysis of Pneumatic Artificial Muscle Acutator Under Forced and Parametric Excitations. Ph.D. Thesis, Indian Institute of Technology Guwahati, Guwahati, India, 2020. [Google Scholar]
- Isermann, R.; Raab, U. Intelligent actuators—Ways to autonomous actuating systems. Automatica 1993, 29, 1315–1331. [Google Scholar] [CrossRef]
- Raab, U.; Isermann, R. Actuator principles with low power. vdi/vde Tag. Actuator 1990, 90. [Google Scholar]
- Hunter, I.W.; Hollerbach, J.M.; Ballantyne, J. A comparative analysis of actuator technologies for robotics. Robot. Rev. 1991, 2, 299–342. [Google Scholar]
- Caldwell, D.; Medrano-Cerda, G.; Goodwin, M. Braided pneumatic actuator control of a multi-jointed manipulator. In Proceedings of the IEEE Systems Man and Cybernetics Conference—SMC, Le Touquet, France, 17–20 October 1993; IEEE: Piscataway, NJ, USA, 1993; Volume 1, pp. 423–428. [Google Scholar]
- Caldwell, D.G. Natural and artificial muscle elements as robot actuators. Mechatronics 1993, 3, 269–283. [Google Scholar] [CrossRef]
- Woods, B.K.S. Pneumatic Artificial Muscle Driven Trailing Edge Flaps for Active Rotors; University of Maryland: College Park, MD, USA, 2012. [Google Scholar]
- Kalita, B.; Narayan, J.; Dwivedy, S.K. Development of active lower limb robotic-based orthosis and exoskeleton devices: A systematic review. Int. J. Soc. Robot. 2021, 13, 775–793. [Google Scholar] [CrossRef]
- Narayan, J.; Kalita, B.; Dwivedy, S.K. Development of robot-based upper limb devices for rehabilitation purposes: A systematic review. Augment. Hum. Res. 2021, 6, 4. [Google Scholar] [CrossRef]
- Woods, B.K.; Gentry, M.F.; Kothera, C.S.; Wereley, N.M. Fatigue life testing of swaged pneumatic artificial muscles as actuators for aerospace applications. J. Intell. Mater. Syst. Struct. 2012, 23, 327–343. [Google Scholar] [CrossRef]
- Kalita, B.; Borgohain, A.; Dwivedy, S.K. Antagonistic Actuation of Pneumatic Artificial Muscle (PAM) with Chain-Sprocket Mechanism. In Machines, Mechanism and Robotics; Springer: Singapore, 2022; pp. 1659–1668. [Google Scholar]
- Yariott, J.M. Fluid Actuator. U.S. Patent 3,645,173, 29 February 1972. [Google Scholar]
- Paynter, H.M. Hyperboloid of Revolution Fluid-Driven Tension Actuators and Method of Making. U.S. Patent 4,721,030, 26 January 1988. [Google Scholar]
- Immega, G. Bellows Actuator. U.S. Patent 5,181,452, 26 January 1993. [Google Scholar]
- Kukolj, M. Axially Contractile Actuator. U.S. Patent 4,733,603, 27 April 1988. [Google Scholar]
- Immega, G. Tension Actuator Load Suspension System. U.S. Patent 4,826,206, 17 August 1988. [Google Scholar]
- Paynter, H.M. High Pressure Fluid-Driven Tension Actuators and Method for Constructing Them. U.S. Patent 4,751,869, 21 June 1988. [Google Scholar]
- Marcincin, J.; Palko, A. Negative pressure artificial muscle-An unconventional drive of robotic and handling systems. In Transactions of the University of Kosice; Riecansky Science Publishing Co: Bratislava, Slovkia, 1993; pp. 350–354. [Google Scholar]
- Morin, A.H. Elastic Diaphragm. U.S. Patent 2,642,091, 16 June 1953. [Google Scholar]
- Caldwell, D.G.; Razak, A.; Goodwin, M. Braided pneumatic muscle actuators. IFAC Proc. Vol. 1993, 26, 522–527. [Google Scholar] [CrossRef]
- Hannaford, B.; Winters, J. Actuator properties and movement control: Biological and technological models. In Multiple Muscle Systems; Springer: New York, NY, USA, 1990; pp. 101–120. [Google Scholar]
- Beullens, T. Hydraulic or Pneumatic Drive Device. U.S. Patent 4,841,845, 27 June 1989. [Google Scholar]
- Baldwin, H.A. Realizable models of muscle function. In Biomechanics; Springer: New York, NY, USA, 1969; pp. 139–147. [Google Scholar]
- Schulte, H. The characteristics of the McKibben artificial muscle. In The Application of External Power in Prosthetics and Orthotics; National Academy of Sciences-National Research Council: Washington, DC, USA, 1961; pp. 94–115. [Google Scholar]
- Gavrilović, M.; Marić, M. Positional servo-mechanism activated by artificial muscles. Med. Biol. Eng. 1969, 7, 77–82. [Google Scholar] [CrossRef] [PubMed]
- Daerden, F. Conception and Realization of Pleated Pneumatic Artificial Muscles and Their Use as Compliant Actuation Elements. Ph.D. Thesis, Vrije Universiteit Brussel, Brussels, Belgium, 1999. [Google Scholar]
- Daerden, F.; Lefeber, D. The concept and design of pleated pneumatic artificial muscles. Int. J. Fluid Power 2001, 2, 41–50. [Google Scholar] [CrossRef]
- Terryn, S.; Brancart, J.; Lefeber, D.; Van Assche, G.; Vanderborght, B. A pneumatic artificial muscle manufactured out of self-healing polymers that can repair macroscopic damages. IEEE Robot. Autom. Lett. 2017, 3, 16–21. [Google Scholar] [CrossRef]
- Immega, G.B. ROMAC actuators for micro robots. In Proceedings of the IEEE Micro Robotics and Teleoperators Workshop, Hyannis, MA, USA, 9–11 November 1987. [Google Scholar]
- Kleinwachter, H.; Geerk, J. Device with a Pressurizable Variable Capacity Chamber for Transforming a Fluid Pressure into a Motion. U.S. Patent 3,638,536, 1 February 1972. [Google Scholar]
- Han, K.; Kim, N.H.; Shin, D. A novel soft pneumatic artificial muscle with high-contraction ratio. Soft Robot. 2018, 5, 554–566. [Google Scholar] [CrossRef]
- Nakamura, T.; Shinohara, H. Position and force control based on mathematical models of pneumatic artificial muscles reinforced by straight glass fibers. In Proceedings of the 2007 IEEE International Conference on Robotics and Automation, Rome, Italy, 10–14 April 2007; IEEE: Piscataway, NJ, USA, 2007; pp. 4361–4366. [Google Scholar]
- Kalita, B.; Dwivedy, S. Dynamic analysis of a parametrically excited golden Muga silk embedded pneumatic artificial muscle. In Proceedings of the MATEC Web of Conferences, Lisbon, Portugal, 10–13 September 2018; EDP Sciences: Lez Ili, France, 2018; Volume 211, p. 02008. [Google Scholar]
- Kalita, B.; Dwivedy, S. Forced Vibration Analysis of a Silk Fibre Embedded Pneumatic Artificial Muscle. In RITA 2018; Springer: Singapore, 2020; pp. 281–301. [Google Scholar]
- Veale, A.J.; Xie, S.Q.; Anderson, I.A. Modeling the Peano fluidic muscle and the effects of its material properties on its static and dynamic behavior. Smart Mater. Struct. 2016, 25, 065014. [Google Scholar] [CrossRef]
- Oguntosin, V.; Akindele, A. Design and characterization of artificial muscles from wedge-like pneumatic soft modules. Sensors Actuators A Phys. 2019, 297, 111523. [Google Scholar] [CrossRef]
- Wirekoh, J.; Park, Y.L. Design of flat pneumatic artificial muscles. Smart Mater. Struct. 2017, 26, 035009. [Google Scholar] [CrossRef]
- Wirekoh, J.; Valle, L.; Pol, N.; Park, Y.L. Sensorized, flat, pneumatic artificial muscle embedded with biomimetic microfluidic sensors for proprioceptive feedback. Soft Robot. 2019, 6, 768–777. [Google Scholar] [CrossRef] [PubMed]
- Greer, J.D.; Morimoto, T.K.; Okamura, A.M.; Hawkes, E.W. Series pneumatic artificial muscles (sPAMs) and application to a soft continuum robot. In Proceedings of the 2017 IEEE International Conference on Robotics and Automation (ICRA), Singapore, 29 May–3 June 2017; IEEE: Piscataway, NJ, USA, 2017; pp. 5503–5510. [Google Scholar]
- Skorina, E.H.; Luo, M.; Oo, W.Y.; Tao, W.; Chen, F.; Youssefian, S.; Rahbar, N.; Onal, C.D. Reverse pneumatic artificial muscles (rPAMs): Modeling, integration, and control. PLoS ONE 2018, 13, e0204637. [Google Scholar] [CrossRef] [PubMed]
- Yang, H.D.; Greczek, B.T.; Asbeck, A.T. Modeling and analysis of a high-displacement pneumatic artificial muscle with integrated sensing. Front. Robot. AI 2019, 5, 136. [Google Scholar] [CrossRef] [PubMed]
- Caldwell, D.G.; Medrano-Cerda, G.A.; Goodwin, M. Control of pneumatic muscle actuators. IEEE Control Syst. Mag. 1995, 15, 40–48. [Google Scholar]
- Kelasidi, E.; Andrikopoulos, G.; Nikolakopoulos, G.; Manesis, S. A survey on pneumatic muscle actuators modeling. In Proceedings of the 2011 IEEE International Symposium on Industrial Electronics, Gdansk, Poland, 27–30 June 2011; IEEE: Piscataway, NJ, USA, 2011; pp. 1263–1269. [Google Scholar]
- Daerden, F.; Verrelst, B.; Lefeber, D.; Kool, P. Controlling motion and compliance with folded pneumatic artificial muscles. In Proceedings of the CLAWAR’99: International Workshop and Conference, Portsmouth, UK, 14–15 September 1999; pp. 667–677. [Google Scholar]
- Pujana-Arrese, A.; Mendizabal, A.; Arenas, J.; Prestamero, R.; Landaluze, J. Modelling in Modelica and position control of a 1-DoF set-up powered by pneumatic muscles. Mechatronics 2010, 20, 535–552. [Google Scholar] [CrossRef]
- Ganguly, S.; Garg, A.; Pasricha, A.; Dwivedy, S. Control of pneumatic artificial muscle system through experimental modelling. Mechatronics 2012, 22, 1135–1147. [Google Scholar] [CrossRef]
- Zhang, J.F.; Yang, C.J.; Chen, Y.; Zhang, Y.; Dong, Y.M. Modeling and control of a curved pneumatic muscle actuator for wearable elbow exoskeleton. Mechatronics 2008, 18, 448–457. [Google Scholar] [CrossRef]
- Serres, J.; Reynolds, D.; Phillips, C.; Rogers, D.; Repperger, D. Characterisation of a pneumatic muscle test station with two dynamic plants in cascade. Comput. Methods Biomech. Biomed. Eng. 2010, 13, 11–18. [Google Scholar] [CrossRef]
- Serres, J.; Reynolds, D.; Phillips, C.; Gerschutz, M.; Repperger, D. Characterisation of a phenomenological model for commercial pneumatic muscle actuators. Comput. Methods Biomech. Biomed. Eng. 2009, 12, 423–430. [Google Scholar] [CrossRef]
- Wickramatunge, K.; Leephakpreeda, T. Empirical modeling of pneumatic artificial muscle. In Proceedings of the International MultiConference of Engineers and Computer Scientists, Hong Kong, China, 18–20 March 2009; Citeseer: Princeton, NJ, USA, 2009; Volume 2. [Google Scholar]
- Wickramatunge, K.C.; Leephakpreeda, T. Study on mechanical behaviors of pneumatic artificial muscle. Int. J. Eng. Sci. 2010, 48, 188–198. [Google Scholar] [CrossRef]
- Wickramatunge, K.C.; Leephakpreeda, T. Empirical modeling of dynamic behaviors of pneumatic artificial muscle actuators. ISA Trans. 2013, 52, 825–834. [Google Scholar] [CrossRef] [PubMed]
- Li, H.; Kawashima, K.; Tadano, K.; Ganguly, S.; Nakano, S. Achieving haptic perception in forceps’ manipulator using pneumatic artificial muscle. IEEE/ASME Trans. Mechatronics 2011, 18, 74–85. [Google Scholar] [CrossRef]
- Kalita, B.; Dwivedy, S.K. Instability regions of pneumatic artificial muscle actuator subject to direct and parametric excitations. Arch. Appl. Mech. 2022, 92, 2019–2039. [Google Scholar] [CrossRef]
- Sárosi, J.; Biro, I.; Nemeth, J.; Cveticanin, L. Dynamic modeling of a pneumatic muscle actuator with two-direction motion. Mech. Mach. Theory 2015, 85, 25–34. [Google Scholar] [CrossRef]
- Palomares, E.; Nieto, A.; Morales, A.; Chicharro, J.; Pintado, P. Dynamic behaviour of pneumatic linear actuators. Mechatronics 2017, 45, 37–48. [Google Scholar] [CrossRef]
- Cullinan, M.F.; Bourke, E.; Kelly, K.; McGinn, C. A McKibben type sleeve pneumatic muscle and integrated mechanism for improved stroke length. J. Mech. Robot. 2017, 9, 011013. [Google Scholar] [CrossRef]
- Cveticanin, L.; Zukovic, M.; Biro, I.; Sarosi, J. Mathematical investigation of the stability condition and steady state position of a pneumatic artificial muscle–mass system. Mech. Mach. Theory 2018, 125, 196–206. [Google Scholar] [CrossRef]
- Colbrunn, R.W.; Nelson, G.M.; Quinn, R.D. Modeling of braided pneumatic actuators for robotic control. In Proceedings of the 2001 IEEE/RSJ International Conference on Intelligent Robots and Systems. Expanding the Societal Role of Robotics in the the Next Millennium (Cat. No. 01CH37180), Maui, HI, USA, 29 October–3 November 2001; IEEE: Piscataway, NJ, USA, 2001; Volume 4, pp. 1964–1970. [Google Scholar]
- Tóthová, M.; Hošovskỳ, A. Dynamic simulation model of pneumatic actuator with artificial muscle. In Proceedings of the 2013 IEEE 11th International Symposium on Applied Machine Intelligence and Informatics (SAMI), Herl’any, Slovakia, 31 January–2 February 2013; IEEE: Piscataway, NJ, USA, 2013; pp. 47–51. [Google Scholar]
- Hildebrandt, A.; Sawodny, O.; Neumann, R.; Hartmann, A. A cascaded tracking control concept for pneumatic muscle actuators. In Proceedings of the 2003 European Control Conference (ECC), Cambridge, UK, 1–4 September 2003; IEEE: Piscataway, NJ, USA, 2003; pp. 2517–2522. [Google Scholar]
- Carneiro, J.F.; de Almeida, F.G. Using two servovalves to improve pneumatic force control in industrial cylinders. Int. J. Adv. Manuf. Technol. 2013, 66, 283–301. [Google Scholar] [CrossRef]
- Tóthová, M.; Pitel’, J.; Hošovskỳ, A.; Sárosi, J. Numerical approximation of static characteristics of McKibben pneumatic artificial muscle. Int. J. Math. Comput. Simul. 2015, 9, 228–233. [Google Scholar]
- Rimár, M.; Šmeringai, P.; Fedak, M.; Hatala, M.; Kulikov, A. Analysis of step responses in nonlinear dynamic systems consisting of antagonistic involvement of pneumatic artificial muscles. Adv. Mater. Sci. Eng.g 2017, 2017, 7168462. [Google Scholar] [CrossRef]
- Kang, B.S.; Kothera, C.S.; Woods, B.K.; Wereley, N.M. Dynamic modeling of Mckibben pneumatic artificial muscles for antagonistic actuation. In Proceedings of the 2009 IEEE International Conference on Robotics and Automation, Kobe, Japan, 12–17 May 2009; IEEE: Piscataway, NJ, USA, 2009; pp. 182–187. [Google Scholar]
- Vocke III, R.D.; Kothera, C.S.; Wereley, N.M. Mechanism and bias considerations for design of a bi-directional pneumatic artificial muscle actuator. Smart Mater. Struct. 2014, 23, 125039. [Google Scholar] [CrossRef]
- Šitum, Ž.; Trslić, P. Ball and beam balancing mechanism actuated with pneumatic artificial muscles. J. Mech. Robot. 2018, 10, 055001. [Google Scholar] [CrossRef]
- Liu, Y.; Zang, X.; Lin, Z.; Liu, X.; Zhao, J. Modelling length/pressure hysteresis of a pneumatic artificial muscle using a modified Prandtl-Ishlinskii model. Stroj. Vestn. J. Mech. Eng. 2017, 63, 56–64. [Google Scholar] [CrossRef]
- Xie, S.; Mei, J.; Liu, H.; Wang, Y. Hysteresis modeling and trajectory tracking control of the pneumatic muscle actuator using modified Prandtl–Ishlinskii model. Mech. Mach. Theory 2018, 120, 213–224. [Google Scholar] [CrossRef]
- Jouppila, V.; Gadsden, S.; Ellman, A. Modeling and identification of a pneumatic muscle actuator system controlled by an on/off solenoid valve. In Proceedings of the 7th International Fluid Power Conference, Aachen, Germany, 22–24 March 2010; pp. 1–11. [Google Scholar]
- Hošovskỳ, A.; Havran, M. Dynamic modelling of one degree of freedom pneumatic muscle-based actuator for industrial applications. Teh. Vjesn. 2012, 19, 673–681. [Google Scholar]
- Veale, A.J.; Xie, S.Q.; Anderson, I.A. Characterizing the Peano fluidic muscle and the effects of its geometry properties on its behavior. Smart Mater. Struct. 2016, 25, 065013. [Google Scholar] [CrossRef]
- Ferraresi, C.; Franco, W.; Manuello Bertetto, A. Flexible Pneumatic Actuators: A comparison between the McKibben and the straight fibres muscles. J. Robot. Mechatron. 2001, 13, 56–63. [Google Scholar] [CrossRef]
- Liu, W.; Rahn, C. Fiber-reinforced membrane models of McKibben actuators. J. Appl. Mech. 2003, 70, 853–859. [Google Scholar] [CrossRef]
- Delson, N.; Hanak, T.; Loewke, K.; Miller, D.N. Modeling and implementation of McKibben actuators for a hopping robot. In Proceedings of the ICAR’05. Proceedings, 12th International Conference on Advanced Robotics, Seattle, WA, USA, 18–20 July 2005; IEEE: Piscataway, NJ, USA, 2005; pp. 833–840. [Google Scholar]
- Davis, S.; Caldwell, D.G. Braid effects on contractile range and friction modeling in pneumatic muscle actuators. Int. J. Robot. Res. 2006, 25, 359–369. [Google Scholar] [CrossRef]
- Trivedi, D.; Lotfi, A.; Rahn, C.D. Geometrically exact models for soft robotic manipulators. IEEE Trans. Robot. 2008, 24, 773–780. [Google Scholar] [CrossRef]
- Doumit, M.D. Characterization, Modeling and Design of the Braided Pneumatic Muscle. Ph.D. Thesis, University of Ottawa, Ottawa, ON, Canada, 2009. [Google Scholar]
- De Volder, M.; Moers, A.; Reynaerts, D. Fabrication and control of miniature McKibben actuators. Sens. Actuators A Phys. 2011, 166, 111–116. [Google Scholar] [CrossRef]
- Chen, D.; Ushijima, K. Prediction of the mechanical performance of McKibben artificial muscle actuator. Int. J. Mech. Sci. 2014, 78, 183–192. [Google Scholar] [CrossRef]
- Wang, G.; Wereley, N.M.; Pillsbury, T. Non-linear quasi-static model of pneumatic artificial muscle actuators. J. Intell. Mater. Syst. Struct. 2015, 26, 541–553. [Google Scholar] [CrossRef]
- Hocking, E.G.; Wereley, N.M. Analysis of nonlinear elastic behavior in miniature pneumatic artificial muscles. Smart Mater. Struct. 2012, 22, 014016. [Google Scholar] [CrossRef]
- Sangian, D.; Naficy, S.; Spinks, G.M.; Tondu, B. The effect of geometry and material properties on the performance of a small hydraulic McKibben muscle system. Sens. Actuators A Phys. 2015, 234, 150–157. [Google Scholar] [CrossRef]
- Andrikopoulos, G.; Nikolakopoulos, G.; Manesis, S. Novel considerations on static force modeling of pneumatic muscle actuators. IEEE/ASME Trans. Mechatron. 2016, 21, 2647–2659. [Google Scholar] [CrossRef]
- Ball, E.; Garcia, E. Effects of bladder geometry in pneumatic artificial muscles. J. Med. Devices 2016, 10, 041001. [Google Scholar] [CrossRef]
- Klute, G.K.; Hannaford, B. Accounting for elastic energy storage in McKibben artificial muscle actuators. J. Dyn. Sys. Meas. Control 2000, 122, 386–388. [Google Scholar] [CrossRef]
- Kothera, C.S.; Jangid, M.; Sirohi, J.; Wereley, N.M. Experimental characterization and static modeling of McKibben actuators. J. Mech. Des. 2009, 131, 091010. [Google Scholar] [CrossRef]
- Chou, C.P.; Hannaford, B. Static and dynamic characteristics of McKibben pneumatic artificial muscles. In Proceedings of the 1994 IEEE International Conference on Robotics and Automation, San Diego, CA, USA, 8–13 May 1994; IEEE: Piscataway, NJ, USA, 1994; pp. 281–286. [Google Scholar]
- Kalita, B.; Dwivedy, S. Nonlinear dynamics of a parametrically excited pneumatic artificial muscle (PAM) actuator with simultaneous resonance condition. Mech. Mach. Theory 2019, 135, 281–297. [Google Scholar]
- Kalita, B.; Dwivedy, S. Dynamic analysis of pneumatic artificial muscle (PAM) actuator for rehabilitation with principal parametric resonance condition. Nonlinear Dyn. 2019, 97, 2271–2289. [Google Scholar] [CrossRef]
- Kalita, B.; Dwivedy, S.K. Nonlinear dynamic response of pneumatic artificial muscle: A theoretical and experimental study. Int. J. Non-Linear Mech. 2020, 125, 103544. [Google Scholar] [CrossRef]
- Kalita, B.; Dwivedy, S.K. Numerical investigation of nonlinear dynamics of a pneumatic artificial muscle with hard excitation. J. Comput. Nonlinear Dyn. 2020, 15, 041003. [Google Scholar] [CrossRef]
- Mooney, M. A theory of large elastic deformation. J. Appl. Phys. 1940, 11, 582–592. [Google Scholar] [CrossRef]
- Rivlin, R.S. Large elastic deformations of isotropic materials IV. Further developments of the general theory. Philos. Trans. R. Soc. Lond. Ser. A Math. Phys. Sci. 1948, 241, 379–397. [Google Scholar]
- Woods, B.K.; Kothera, C.S.; Wereley, N.M. Wind tunnel testing of a helicopter rotor trailing edge flap actuated via pneumatic artificial muscles. J. Intell. Mater. Syst. Struct. 2011, 22, 1513–1528. [Google Scholar] [CrossRef]
- Kim, B.; Lee, S.B.; Lee, J.; Cho, S.; Park, H.; Yeom, S.; Park, S.H. A comparison among Neo-Hookean model, Mooney-Rivlin model, and Ogden model for chloroprene rubber. Int. J. Precis. Eng. Manuf. 2012, 13, 759–764. [Google Scholar] [CrossRef]
- Inoue, K. Rubbertuators and applications for robots. In Proceedings of the 4th International Symposium on Robotics Research, Santa Cruz, CA, USA, 9–14 August 1987; pp. 57–63. [Google Scholar]
- Shadow Robot. Available online: https://www.shadowrobot.com/ (accessed on 1 September 2022).
- Fluidic Muscle. Available online: https://www.festo.com/group/en/cms/10248.htm (accessed on 1 September 2022).
- Deaconescu, A.; Deaconescu, T. Contributions to the behavioural study of pneumatically actuated artificial muscles. In Proceedings of the 6th International DAAAM Baltic Conference Industrial Engineering, Tallinn, Estonia, 24–26 April 2008. [Google Scholar]
- Waycaster, G.; Wu, S.K.; Shen, X. Design and control of a pneumatic artificial muscle actuated above-knee prosthesis. J. Med. Devices 2011, 5, 031003. [Google Scholar] [CrossRef]
- Wu, S.K.; Driver, T.; Shen, X. Design and control of a pneumatically actuated lower-extremity orthosis. J. Med. Devices 2012, 6, 041004. [Google Scholar] [CrossRef]
- Fantoni, G.; Santochi, M.; Dini, G.; Tracht, K.; Scholz-Reiter, B.; Fleischer, J.; Lien, T.K.; Seliger, G.; Reinhart, G.; Franke, J.; et al. Grasping devices and methods in automated production processes. CIRP Ann. 2014, 63, 679–701. [Google Scholar] [CrossRef]
- Giannaccini, M.E.; Georgilas, I.; Horsfield, I.; Peiris, B.; Lenz, A.; Pipe, A.G.; Dogramadzi, S. A variable compliance, soft gripper. Auton. Robot. 2014, 36, 93–107. [Google Scholar] [CrossRef]
- Deimel, R.; Brock, O. A compliant hand based on a novel pneumatic actuator. In Proceedings of the 2013 IEEE International Conference on Robotics and Automation, Karlsruhe, Germany, 6–10 May 2013; IEEE: Piscataway, NJ, USA, 2013; pp. 2047–2053. [Google Scholar]
- Ilievski, F.; Mazzeo, A.D.; Shepherd, R.F.; Chen, X.; Whitesides, G.M. Soft robotics for chemists. Angew. Chem. 2011, 123, 1930–1935. [Google Scholar] [CrossRef]
- Suzumori, K.; Endo, S.; Kanda, T.; Kato, N.; Suzuki, H. A bending pneumatic rubber actuator realizing soft-bodied manta swimming robot. In Proceedings of the 2007 IEEE International Conference on Robotics and Automation, Rome, Italy, 10–14 April 2007; IEEE: Piscataway, NJ, USA, 2007; pp. 4975–4980. [Google Scholar]
- Durfee, W.K.; Rivard, A. Preliminary design and simulation of a pneumatic, stored-energy, hybrid orthosis for gait restoration. In Proceedings of the ASME International Mechanical Engineering Congress and Exposition, Anaheim, CA, USA, 13–19 November 2004; Volume 47101, pp. 235–241. [Google Scholar]
- Ashwin, K.; Ghosal, A. A soft-robotic end-effector for independently actuating endoscopic catheters. J. Mech. Robot. 2019, 11, 061004. [Google Scholar] [CrossRef]
- Ashwin, K.; Ghosal, A. Static modeling of miniaturized pneumatic artificial muscles, kinematic analysis, and experiments on an endoscopic end-effector. IEEE/ASME Trans. Mechatron. 2019, 24, 1429–1439. [Google Scholar] [CrossRef]
- Verrelst, B.; Van Ham, R.; Vanderborght, B.; Lefeber, D.; Daerden, F.; Van Damme, M. Second generation pleated pneumatic artificial muscle and its robotic applications. Adv. Robot. 2006, 20, 783–805. [Google Scholar] [CrossRef]
- Noritsugu, T.; Tanaka, T. Application of rubber artificial muscle manipulator as a rehabilitation robot. IEEE/ASME Trans. Mechatron. 1997, 2, 259–267. [Google Scholar] [CrossRef]
- Martens, M.; Seel, T.; Zawatzki, J.; Boblan, I. A novel framework for a systematic integration of pneumatic-muscle-actuator-driven joints into robotic systems via a torque control interface. Actuators 2018, 7, 82. [Google Scholar] [CrossRef]
- Winters, J.M. Braided artificial muscles: Mechanical properties and future uses in prosthetics/orthotics. In Proceedings of the RESNA 13th Annual Conference, Washington, DC, USA, 15–20 June 1990; ERIC: Washington, DC, USA, 1990; pp. 173–174. [Google Scholar]
- Caldwell, D.G.; Tsagarakis, N.; Badihi, D.; Medrano-Cerda, G.A. Pneumatic muscle actuator technology: A light weight power system for a humanoid robot. In Proceedings of the 1998 IEEE International Conference on Robotics and Automation (Cat. No. 98CH36146), Leuven, Belgium, 20–20 May 1998; IEEE: Piscataway, NJ, USA, 1998; Volume 4, pp. 3053–3058. [Google Scholar]
- Hannaford, B.; Winters, J.M.; Chou, C.P.; Marbot, P.H. The anthroform biorobotic arm: A system for the study of spinal circuits. Ann. Biomed. Eng. 1995, 23, 399–408. [Google Scholar] [CrossRef]
- Grodski, J.; Immega, G. Myoelectric control of compliance on a ROMAC protoarm. In Proceedings of the International Symposium on Teleoperation and Control, Bristol, UK, 12–15 July 1988; pp. 297–308. [Google Scholar]
- Kawamura, K.; Peters, R.A.; Wilkes, D.M.; Alford, W.A.; Rogers, T.E. ISAC: Foundations in human-humanoid interaction. IEEE Intell. Syst. Their Appl. 2000, 15, 38–45. [Google Scholar] [CrossRef]
- Berns, K. Airbug insect-like machine actuated by fluidic muscle. In Proceedings of the 4th International Conference on Climbing and Walking Robots, from Biology to Industrial Application, Karlsruhe, Germany, 24–26 September 2001. [Google Scholar]
- Haghshenas-Jaryani, M. Dynamics and Computed-Muscle-Force Control of a Planar Muscle-Driven Snake Robot. Actuators 2022, 11, 94. [Google Scholar] [CrossRef]
- Daerden, F.; Lefeber, D.; Verrelst, B.; Van Ham, R. Pleated pneumatic artificial muscles: Actuators for automation and robotics. In Proceedings of the 2001 IEEE/ASME International Conference on Advanced Intelligent Mechatronics, Proceedings (Cat. No. 01TH8556), Como, Italy, 8–12 July 2001; IEEE: Piscataway, NJ, USA, 2001; Volume 2, pp. 738–743. [Google Scholar]
- Witte, H.; Hackert, R.; Lilje, K.E.; Schilling, N.; Voges, D.; Klauer, G.; Ilg, W.; Albiez, J.; Seyfarth, A.; Germann, D.; et al. Transfer of biological principles into the construction of quadruped walking machines. In Proceedings of the Second International Workshop on Robot Motion and Control, RoMoCo’01 (IEEE Cat. No. 01EX535), Bukowy Dworek, Poland, 20 October 2001; IEEE: Piscataway, NJ, USA, 2001; pp. 245–249. [Google Scholar]
- Kingsley, D.A. A Cockroach Inspired Robot with Artificial Muscles; Case Western Reserve University: Cleveland, OH, USA, 2005. [Google Scholar]
- Verrelst, B.; Ham, R.V.; Vanderborght, B.; Daerden, F.; Lefeber, D.; Vermeulen, J. The pneumatic biped “Lucy” actuated with pleated pneumatic artificial muscles. Auton. Robot. 2005, 18, 201–213. [Google Scholar] [CrossRef]
- Tsujita, K.; Inoura, T.; Masuda, T. Oscillator-controlled bipedal walker with pneumatic actuators. In Proceedings of the SICE Annual Conference 2007, Takamatsu, Japan, 17–20 September 2007; IEEE: Piscataway, NJ, USA, 2007; pp. 2165–2169. [Google Scholar]
- Niiyama, R.; Nagakubo, A.; Kuniyoshi, Y. Mowgli: A bipedal jumping and landing robot with an artificial musculoskeletal system. In Proceedings of the 2007 IEEE International Conference on Robotics and Automation, Rome, Italy, 10–14 April 2007; IEEE: Piscataway, NJ, USA, 2007; pp. 2546–2551. [Google Scholar]
- Niiyama, R.; Kuniyoshi, Y. A pneumatic biped with an artificial musculoskeletal system. In Proceedings of the 4th International Symposium on Adaptive Motion of Animals and Machines, Cleveland, Ohio, USA, 1–6 June 2008; pp. 80–81. [Google Scholar]
- Tsujita, K.; Kobayashi, T.; Inoura, T.; Masuda, T. Gait transition by tuning muscle tones using pneumatic actuators in quadruped locomotion. In Proceedings of the 2008 IEEE/RSJ International Conference on Intelligent Robots and Systems, Nice, France, 22–26 September 2008; IEEE: Piscataway, NJ, USA, 2008; pp. 2453–2458. [Google Scholar]
- Boblan, I.; Schulz, A. A humanoid muscle robot torso with biologically inspired construction. In Proceedings of the ISR 2010 (41st International Symposium on Robotics) and ROBOTIK 2010 (6th German Conference on Robotics), VDE, Munich, Germany, 7–9 June 2010; pp. 1–6. [Google Scholar]
- Niiyama, R.; Nishikawa, S.; Kuniyoshi, Y. Athlete robot with applied human muscle activation patterns for bipedal running. In Proceedings of the 2010 10th IEEE-RAS International Conference on Humanoid Robots, Nashville, TN, USA, 6–8 December 2010; IEEE: Piscataway, NJ, USA, 2010; pp. 498–503. [Google Scholar]
- Andrikopoulos, G.; Manesis, S. A study of the behavior of penumatic artificial msucles in industrial applications controlled by programmable logic controller. In Proceedings of the International Congress on Computer Applications and Computational Science, Fukuoka, Japan, 23–26 March 2010. [Google Scholar]
- Fukuoka, Y.; Komatsu, R.; Machii, K.; Yokota, M.; Tobe, M.; Ibrahim, A.N.; Fukui, T.; Habu, Y. Pace Running of a Quadruped Robot Driven by Pneumatic Muscle Actuators: An Experimental Study. Appl. Sci. 2022, 12, 4146. [Google Scholar] [CrossRef]
- Prior, S.D.; White, A.S. Measurements and simulation of a pneumatic muscle actuator for a rehabilitation robot. Simul. Pract. Theory 1995, 3, 81–117. [Google Scholar] [CrossRef]
- Misuraca, J.J.; Mavroidis, C. Lower limb human muscle enhancer. In Proceedings of the ASME International Mechanical Engineering Congress and Exposition, New York, NY, USA, 11–16 November 2001; American Society of Mechanical Engineers: New York, NY, USA, 2001; Volume 35609, pp. 963–969. [Google Scholar]
- Caldwell, D.; Tsagarakis, N. Biomimetic actuators in prosthetic and rehabilitation applications. Technol. Health Care 2002, 10, 107–120. [Google Scholar] [CrossRef] [PubMed]
- Wongsiri, S.; Laksanacharoen, S. Design and construction of an artificial limb driven by artificial muscles for amputees. In Proceedings of the International Conference on Energy and the Environment, Songkla, Thailand, 11–12 December 2003; pp. 11–12. [Google Scholar]
- Laksanacharoen, S. Artificial muscle construction using natural rubber latex in Thailand. In Proceedings of the 3rd Thailand and Material Science and Technology Conference, Bangkok, Thailand, 10–11 August 2004; pp. 10–11. [Google Scholar]
- Kobayashi, H.; Hiramatsu, K. Development of muscle suit for upper limb. In Proceedings of the IEEE International Conference on Robotics and Automation, Proceedings, ICRA’04. New Orleans, LA, USA, 26 April–1 May 2004; IEEE: Piscataway, NJ, USA, 2004; Volume 3, pp. 2480–2485. [Google Scholar]
- Herr, H.M.; Kornbluh, R.D. New horizons for orthotic and prosthetic technology: Artificial muscle for ambulation. In Proceedings of the Smart Structures and Materials 2004: Electroactive Polymer Actuators and Devices (EAPAD), San Diego, CA, USA, 14–18 March 2004; SPIE: Bellingham, WA, USA, 2004; Volume 5385, pp. 1–9. [Google Scholar]
- He, J.; Koeneman, E.J.; Schultz, R.S.; Huang, H.; Wanberg, J.; Herring, D.; Sugar, T.; Herman, R.; Koeneman, J. Design of a robotic upper extremity repetitive therapy device. In Proceedings of the 9th International Conference on Rehabilitation Robotics, ICORR, Chicago, IL, USA, 28 June–1 July 2005; IEEE: Piscataway, NJ, USA, 2005; pp. 95–98. [Google Scholar]
- Balasubramanian, S.; Wei, R.; Perez, M.; Shepard, B.; Koeneman, E.; Koeneman, J.; He, J. RUPERT: An exoskeleton robot for assisting rehabilitation of arm functions. In Proceedings of the 2008 Virtual Rehabilitation, Vancouver, BC, Canada, 25–27 August 2008; IEEE: Piscataway, NJ, USA, 2008; pp. 163–167. [Google Scholar]
- Vimieiro, C.; do Nascimento, B.G.; Nagem, D.A.P.; Pinotti, M. Development of a hip orthosis using pneumatic artificial muscles. In Proceedings of the TMSi, São Paulo, Spain, 18–19 July 2005; pp. 18–19. [Google Scholar]
- Ferris, D.P.; Czerniecki, J.M.; Hannaford, B. An ankle-foot orthosis powered by artificial pneumatic muscles. J. Appl. Biomech. 2005, 21, 189–197. [Google Scholar] [CrossRef]
- Ferris, D.P.; Gordon, K.E.; Sawicki, G.S.; Peethambaran, A. An improved powered ankle–foot orthosis using proportional myoelectric control. Gait Posture 2006, 23, 425–428. [Google Scholar] [CrossRef]
- Gordon, K.E.; Sawicki, G.S.; Ferris, D.P. Mechanical performance of artificial pneumatic muscles to power an ankle–foot orthosis. J. Biomech. 2006, 39, 1832–1841. [Google Scholar] [CrossRef]
- Gupta, A.; O’Malley, M.K. Design of a haptic arm exoskeleton for training and rehabilitation. IEEE/ASME Trans. Mechatron. 2006, 11, 280–289. [Google Scholar] [CrossRef]
- Knestel, M.; Hofer, E.; Barillas, S.K.; Rupp, R. The artificial muscle as an innovative actuator in rehabilitation robotics. IFAC Proc. Vol. 2008, 41, 773–778. [Google Scholar] [CrossRef]
- Deaconescu, T.; Deaconescu, A. Pneumatic muscle actuated isokinetic equipment for the rehabilitation of patients with disabilities of the bearing joints. In Proceedings of the International MultiConference of Engineers and Computer Scientists, Hong Kong, China, 18–20 March 2009; Citeseer: Princeton, NJ, USA, 2009; Volume 2, pp. 1823–1827. [Google Scholar]
- Deaconescu, A.; Deaconescu, T. Pneumatic muscle actuated robotized arm for rehabilitation systems. Environment 2009, 1, 3. [Google Scholar]
- Do Nascimento, B.G.; Vimieiro, C.B.S.; Nagem, D.A.P.; Pinotti, M. Hip orthosis powered by pneumatic artificial muscle: Voluntary activation in absence of myoelectrical signal. Artif. Organs 2008, 32, 317–322. [Google Scholar] [CrossRef]
- Kao, P.C.; Lewis, C.L.; Ferris, D.P. Invariant ankle moment patterns when walking with and without a robotic ankle exoskeleton. J. Biomech. 2010, 43, 203–209. [Google Scholar] [CrossRef] [PubMed]
- Kao, P.C.; Lewis, C.L.; Ferris, D.P. Joint kinetic response during unexpectedly reduced plantar flexor torque provided by a robotic ankle exoskeleton during walking. J. Biomech. 2010, 43, 1401–1407. [Google Scholar] [CrossRef][Green Version]
- Norris, J.A.; Granata, K.P.; Mitros, M.R.; Byrne, E.M.; Marsh, A.P. Effect of augmented plantarflexion power on preferred walking speed and economy in young and older adults. Gait Posture 2007, 25, 620–627. [Google Scholar] [CrossRef]
- Lewis, C.L.; Ferris, D.P. Invariant hip moment pattern while walking with a robotic hip exoskeleton. J. Biomech. 2011, 44, 789–793. [Google Scholar] [CrossRef]
- Kim, K.; Yu, C.H.; Jeong, G.Y.; Heo, M.; Kwon, T.K. Analysis of the assistance characteristics for the knee extension motion of knee orthosis using muscular stiffness force feedback. J. Mech. Sci. Technol. 2013, 27, 3161–3169. [Google Scholar] [CrossRef]
- Leclair, J.; Pardoel, S.; Helal, A.; Doumit, M. Development of an unpowered ankle exoskeleton for walking assist. Disabil. Rehabil. Assist. Technol. 2018, 15, 1–13. [Google Scholar] [CrossRef] [PubMed]
- Kim, K.; Kim, J.J.; Kang, S.R.; Jeong, G.Y.; Kwon, T.K. Analysis of the assistance characteristics for the plantarflexion torque in elderly adults wearing the powered ankle exoskeleton. In Proceedings of the ICCAS 2010, Gyeonggi-do, Korea, 27–30 October 2010; IEEE: Piscataway, NJ, USA, 2010; pp. 576–579. [Google Scholar]
- Malcolm, P.; Derave, W.; Galle, S.; De Clercq, D. A simple exoskeleton that assists plantarflexion can reduce the metabolic cost of human walking. PLoS ONE 2013, 8, e56137. [Google Scholar] [CrossRef]
- Malcolm, P.; Fiers, P.; Segers, V.; Van Caekenberghe, I.; Lenoir, M.; De Clercq, D. Experimental study on the role of the ankle push off in the walk-to-run transition by means of a powered ankle-foot-exoskeleton. Gait Posture 2009, 30, 322–327. [Google Scholar] [CrossRef]
- Dragone, D.; Randazzini, L.; Capace, A.; Nesci, F.; Cosentino, C.; Amato, F.; De Momi, E.; Colao, R.; Masia, L.; Merola, A. Design, Computational Modelling and Experimental Characterization of Bistable Hybrid Soft Actuators for a Controllable-Compliance Joint of an Exoskeleton Rehabilitation Robot. Actuators 2022, 11, 32. [Google Scholar] [CrossRef]
- Chi, H.; Su, H.; Liang, W.; Ren, Q. Control of a rehabilitation robotic device driven by antagonistic soft actuators. Actuators 2021, 10, 123. [Google Scholar] [CrossRef]
- Nguyen, H.T.; Trinh, V.C.; Le, T.D. An adaptive fast terminal sliding mode controller of exercise-assisted robotic arm for elbow joint rehabilitation featuring pneumatic artificial muscle actuator. Actuators 2020, 9, 118. [Google Scholar] [CrossRef]
- Dao, Q.T.; Yamamoto, S.i. Assist-as-needed control of a robotic orthosis actuated by pneumatic artificial muscle for gait rehabilitation. Appl. Sci. 2018, 8, 499. [Google Scholar] [CrossRef]
- Tsai, T.C.; Chiang, M.H. Design and control of a 1-DOF robotic lower-limb system driven by novel single pneumatic artificial muscle. Appl. Sci. 2019, 10, 43. [Google Scholar] [CrossRef]
- Tjahyono, A.P.; Aw, K.C.; Devaraj, H.; Surendra, W.; Haemmerle, E.; Travas-Sejdic, J. A five-fingered hand exoskeleton driven by pneumatic artificial muscles with novel polypyrrole sensors. Ind. Robot. Int. J. 2013, 40, 251–260. [Google Scholar] [CrossRef]
- Obiajulu, S.C.; Roche, E.T.; Pigula, F.A.; Walsh, C.J. Soft pneumatic artificial muscles with low threshold pressures for a cardiac compression device. In Proceedings of the International Design Engineering Technical Conferences and Computers and Information in Engineering Conference, Portland, OR, USA, 4–7 August 2013; American Society of Mechanical Engineers: New York, NY, USA, 2013; Volume 55935, p. V06AT07A009. [Google Scholar]
- Chakravarthy, S.; Aditya, K.; Ghosal, A. Experimental characterization and control of miniaturized pneumatic artificial muscle. J. Med. Devices 2014, 8, 041011. [Google Scholar] [CrossRef]
- Pomiers, P. Modular robot arm based on pneumatic artificial rubber muscles (PARM). Proc. CLAWAR Catania 2003, 2003, 879–886. [Google Scholar]
- Kawashima, K. Development of robot using pneumatic artificial rubber muscles to operate construction machinery. J. Robot. Mechatron. 2004, 16, 8–16. [Google Scholar] [CrossRef]
- Damme, M.V.; Ham, R.V.; Vanderborght, B.; Daerden, F.; Lefeber, D. Design of a “soft” 2-DOF planar pneumatic manipulator. In Climbing and Walking Robots; Springer: Berlin/Heidelberg, Germany, 2006; pp. 559–566. [Google Scholar]
- Van Damme, M.; Daerden, F.; Lefeber, D. A pneumatic manipulator used in direct contact with an operator. In Proceedings of the 2005 IEEE International Conference on Robotics and Automation, Barcelona, Spain, 18–22 April 2005; IEEE: Piscataway, NJ, USA, 2005; pp. 4494–4499. [Google Scholar]
- Caldwell, D.G.; Tsagarakis, N.; Medrano-Cerda, G.A.; Schofield, J.; Brown, S. Development of a pneumatic muscle actuator driven manipulator rig for nuclear waste retrieval operations. In Proceedings of the 1999 IEEE International Conference on Robotics and Automation (Cat. No. 99CH36288C), Detroit, MI, USA, 10–15 May 1999; IEEE: Piscataway, NJ, USA, 1999; Volume 1, pp. 525–530. [Google Scholar]
- Deckers, K.; Guillaume, P.; Lefeber, D.; De Roeck, G.; Reynders, E. Modal testing of bridges using low-weight pneumatic artificial muscle actuators. In Proceedings of the IMAC 26, the International Modal Analysis Conference, Orlando, FL, USA, 4–7 February 2008. [Google Scholar]
- Wedler, A.; Friederichs, J.; Hackelöer, F.; Denkena, B. New compliant mc-kibben actuator driven by pneumatic actuators as a hexapod platform in robotic applications. In Proceedings of the 10th Workshop on Advanced Space Technologies for Robotics and Automation, ASTRA, Noordwijk, The Netherlands, 11–14 November 2008; Citeseer: Princeton, NJ, USA, 2008. [Google Scholar]
- Radojicic, J.; Surdilovic, D.; Schreck, G. Modular hybrid robots for safe human-robot interaction. Int. J. World Acad. Sci. Eng. Technol. (WASET) 2009, 60, 832–838. [Google Scholar]
- Yerkes, N.; Wereley, N. Pneumatic artificial muscle activation for trailing edge flaps. In Proceedings of the 46th AIAA aerospace sciences meeting and exhibit, Reno, NV, USA, 7–10 January 2008; p. 1418. [Google Scholar]
- Wereley, N.; Kothera, C.; Bubert, E.; Woods, B.; Gentry, M.; Vocke, R. Pneumatic artificial muscles for aerospace applications. In Proceedings of the 50th AIAA/ASME/ASCE/AHS/ASC Structures, Structural Dynamics, and Materials Conference, Palm Springs, CA, USA, 4–7 May 2009; p. 2140. [Google Scholar]
- Lin, Y.; Xu, Y.X.; Juang, J.Y. Single-Actuator Soft Robot for In-Pipe Crawling. Soft Robot. 2022. [Google Scholar] [CrossRef]
- Stoll, J.T.; Schanz, K.; Pott, A. Mechatronic control system for a compliant and precise pneumatic rotary drive unit. Actuators 2019, 9, 1. [Google Scholar] [CrossRef]
- Robinson, R.M.; Kothera, C.S.; Wereley, N.M. Control of a heavy-lift robotic manipulator with pneumatic artificial muscles. Actuators 2014, 3, 41–65. [Google Scholar] [CrossRef]
- Brown, G.; Haggard, R.; Almassy, R.; Benney, R.; Dellicker, S. The affordable guided airdrop system (AGAS). In Proceedings of the 15th Aerodynamic Decelerator Systems Technology Conference, Toulouse, France, 8–11 June 1999; p. 1742. [Google Scholar]
- Pohl, M. A motion seat using pneumatic membran actuators in a hexapod system structure. In Proceedings of the 6th International Workshop on Research and Education in Mechatronics REM, Annecy, France, 30 June–1 July 2005; Citeseer: Princeton, NJ, USA; pp. 183–188. [Google Scholar]
- Engineered Arts. Available online: https://www.engineeredarts.co.uk/robothespian/ (accessed on 2 September 2022).
- Designboom. Available online: https://www.designboom.com/technology/standing-motorcycle-concept-by-jake-loniak/ (accessed on 2 September 2022).

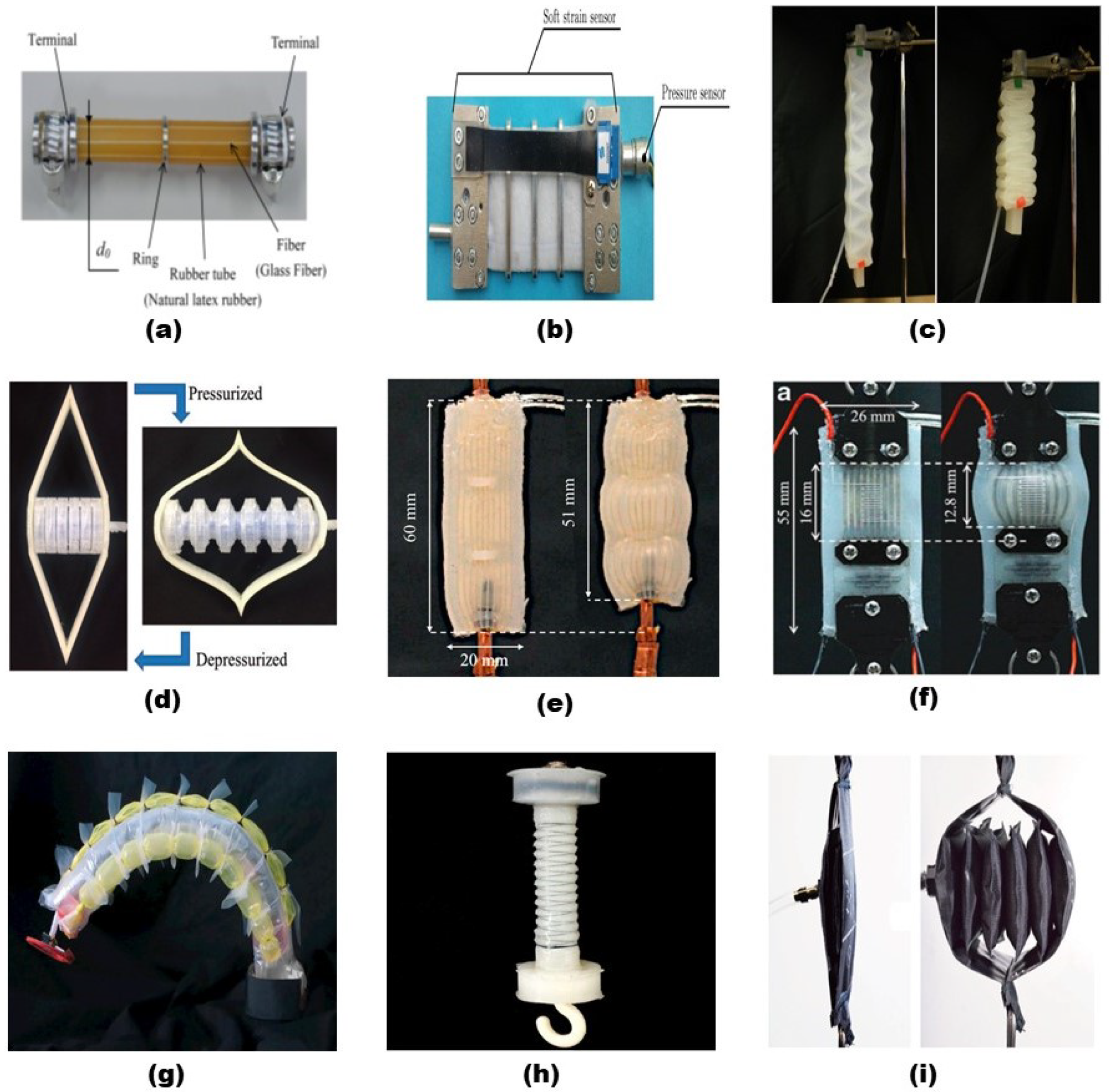
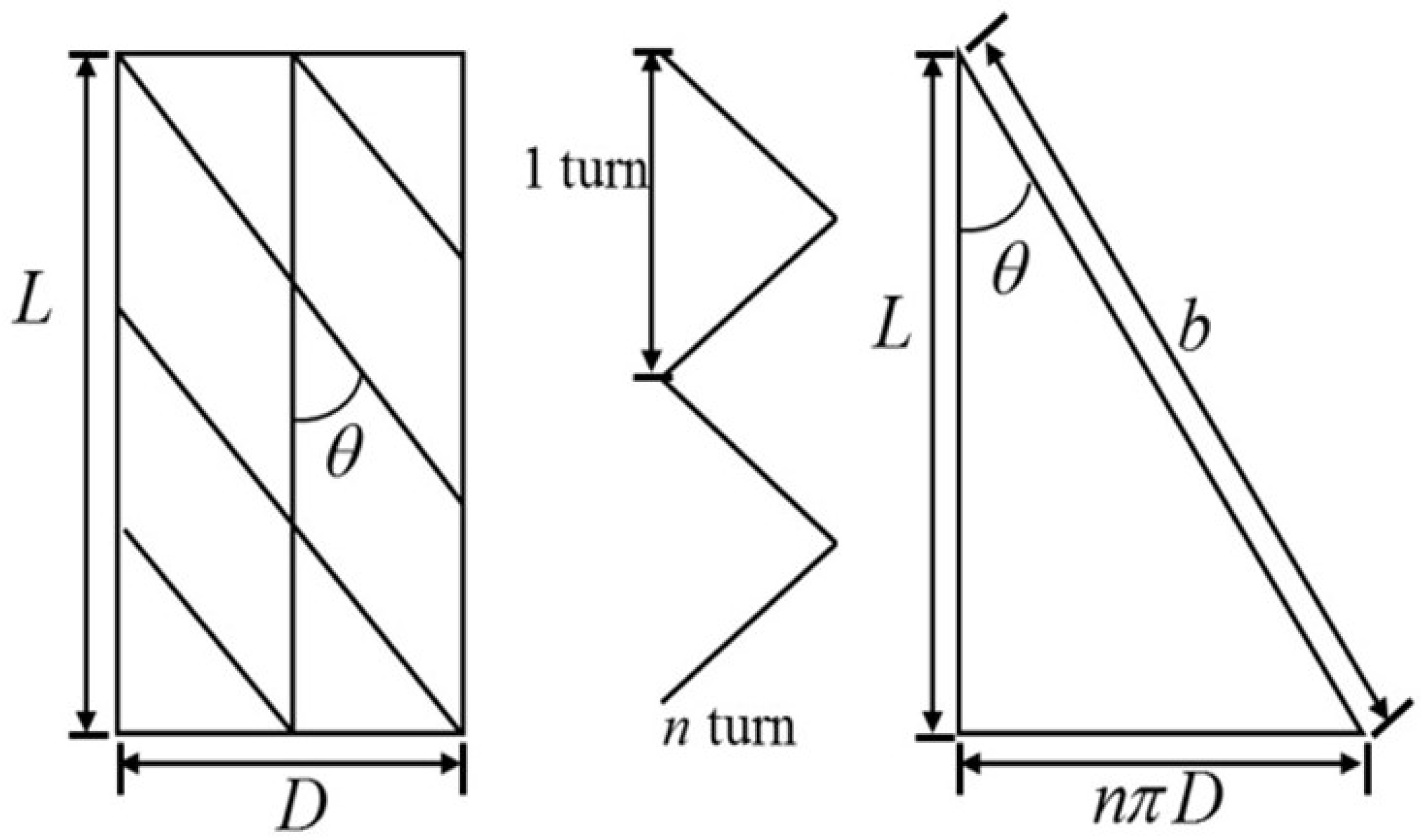
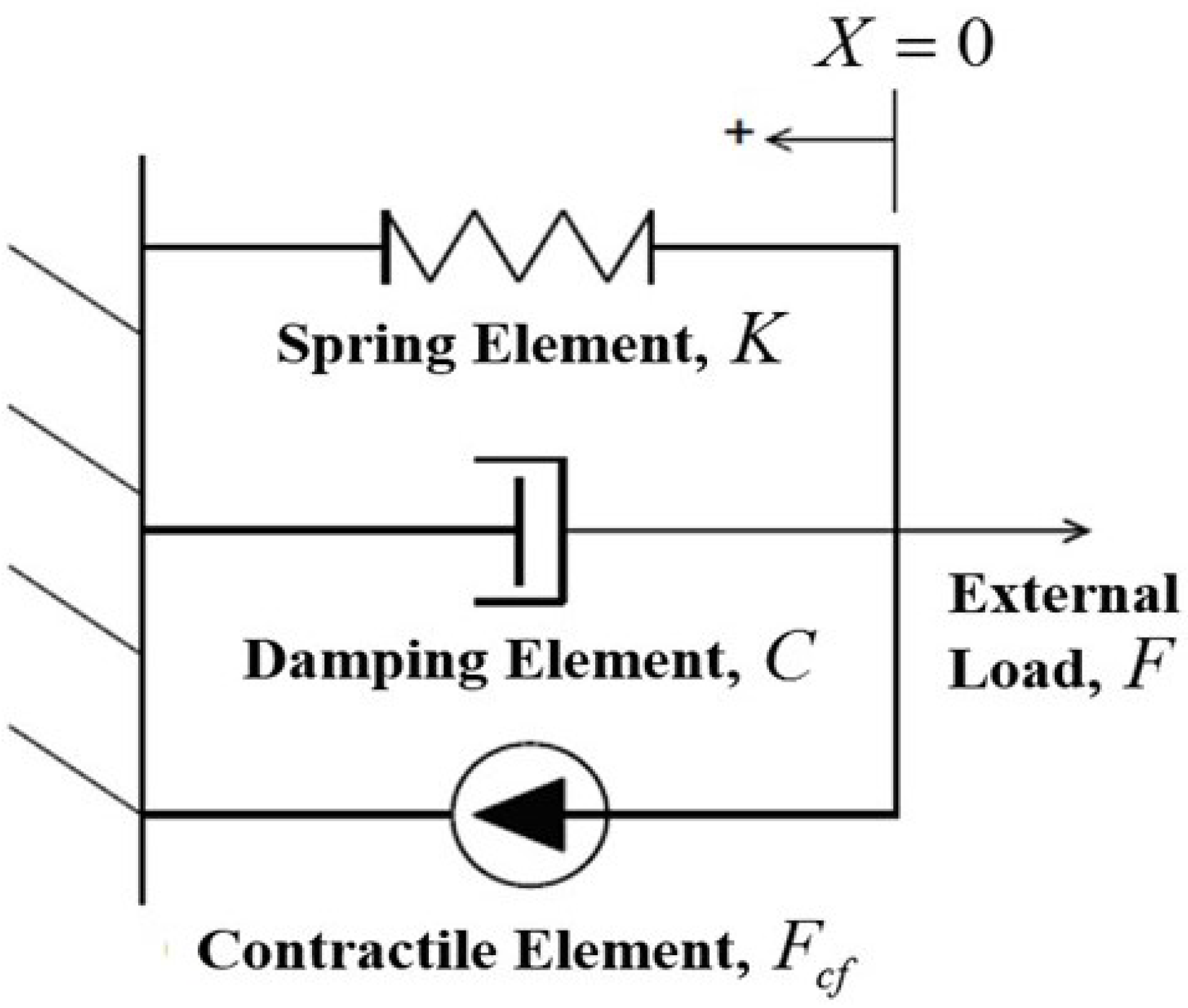
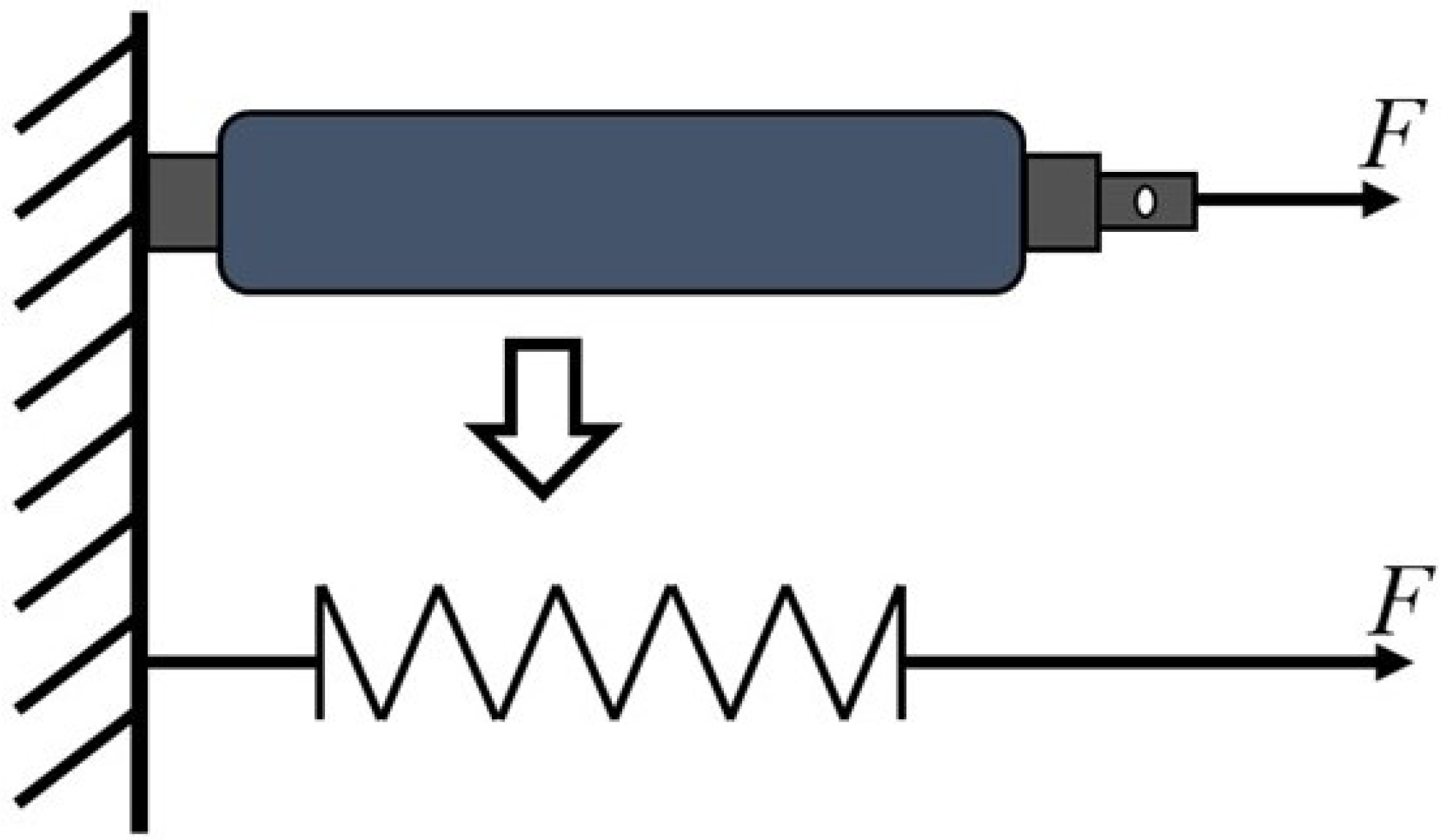

| Actuation Technology | Maximum Strain | Actuation Stress (MPa) | Specific Work (J/kg) |
|---|---|---|---|
| Hydraulic | 1 | 70 | 35,000 |
| Electromechanical | 0.5 | 1 | 300 |
| Solenoid | 0.4 | 0.1 | 5 |
| Piezoelectric | 0.002 | 9 | 1 |
| Magnetostrictive | 0.002 | 200 | 20 |
| SMA | 0.07 | 700 | 4500 |
| Pneumatic Cylinder | 1 | 0.9 | 1200 |
| PAM | 0.4 | 16 | 4400 |
| Author | Muscle Force Equation |
|---|---|
| Schulte [30] | |
| Carlo Ferraresi et al. [79] | |
| Liu and Rahn [80] | |
| Delson et al. [81] | |
| Davis and Caldwell [82] | |
| Trivedi et al. [83] | |
| Doumit [84] | |
| de Volder et al. [85] | |
| Chen and Ushijima [86] | |
| Wang et al. [87] | |
| Hocking and Wereley [88] | |
| Sangian et al. [89] | |
| Andrikopoulos et al. [90] |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Kalita, B.; Leonessa, A.; Dwivedy, S.K. A Review on the Development of Pneumatic Artificial Muscle Actuators: Force Model and Application. Actuators 2022, 11, 288. https://doi.org/10.3390/act11100288
Kalita B, Leonessa A, Dwivedy SK. A Review on the Development of Pneumatic Artificial Muscle Actuators: Force Model and Application. Actuators. 2022; 11(10):288. https://doi.org/10.3390/act11100288
Chicago/Turabian StyleKalita, Bhaben, Alexander Leonessa, and Santosha K. Dwivedy. 2022. "A Review on the Development of Pneumatic Artificial Muscle Actuators: Force Model and Application" Actuators 11, no. 10: 288. https://doi.org/10.3390/act11100288
APA StyleKalita, B., Leonessa, A., & Dwivedy, S. K. (2022). A Review on the Development of Pneumatic Artificial Muscle Actuators: Force Model and Application. Actuators, 11(10), 288. https://doi.org/10.3390/act11100288