Design and Experimental Analysis of Charge Recovery for Piezoelectric Fan
Abstract
1. Introduction
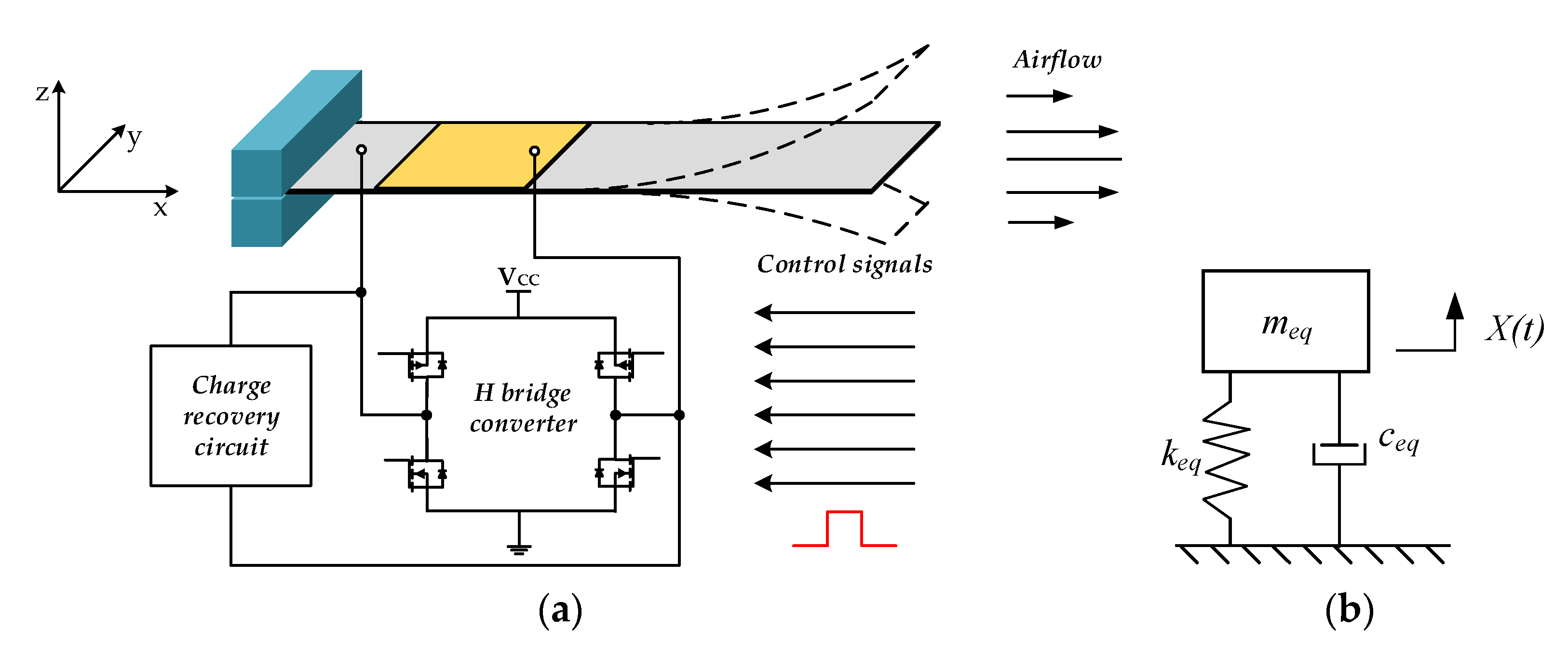
2. Structure and Modelling
3. Driving Circuit and Power Consumption Analysis
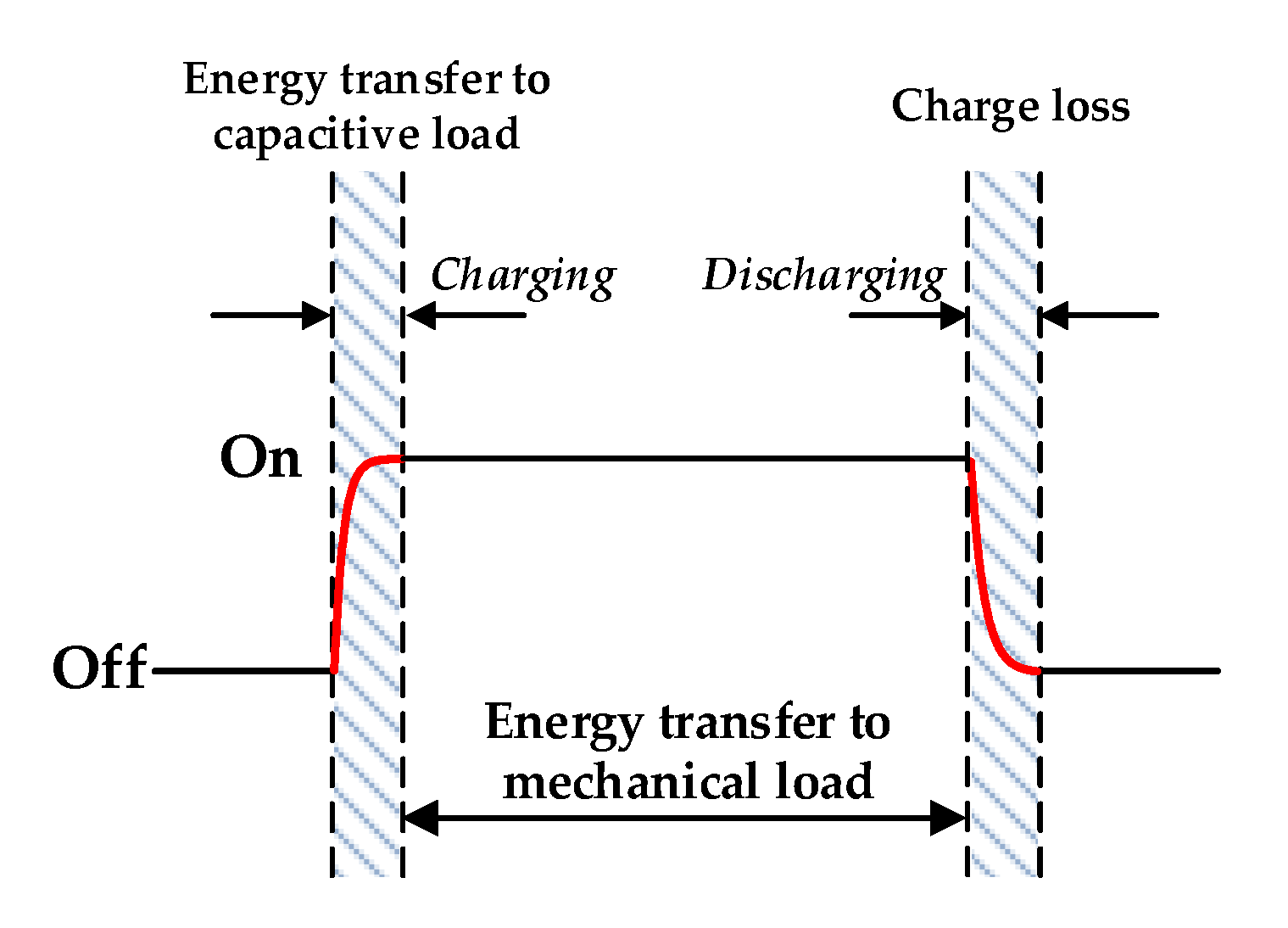
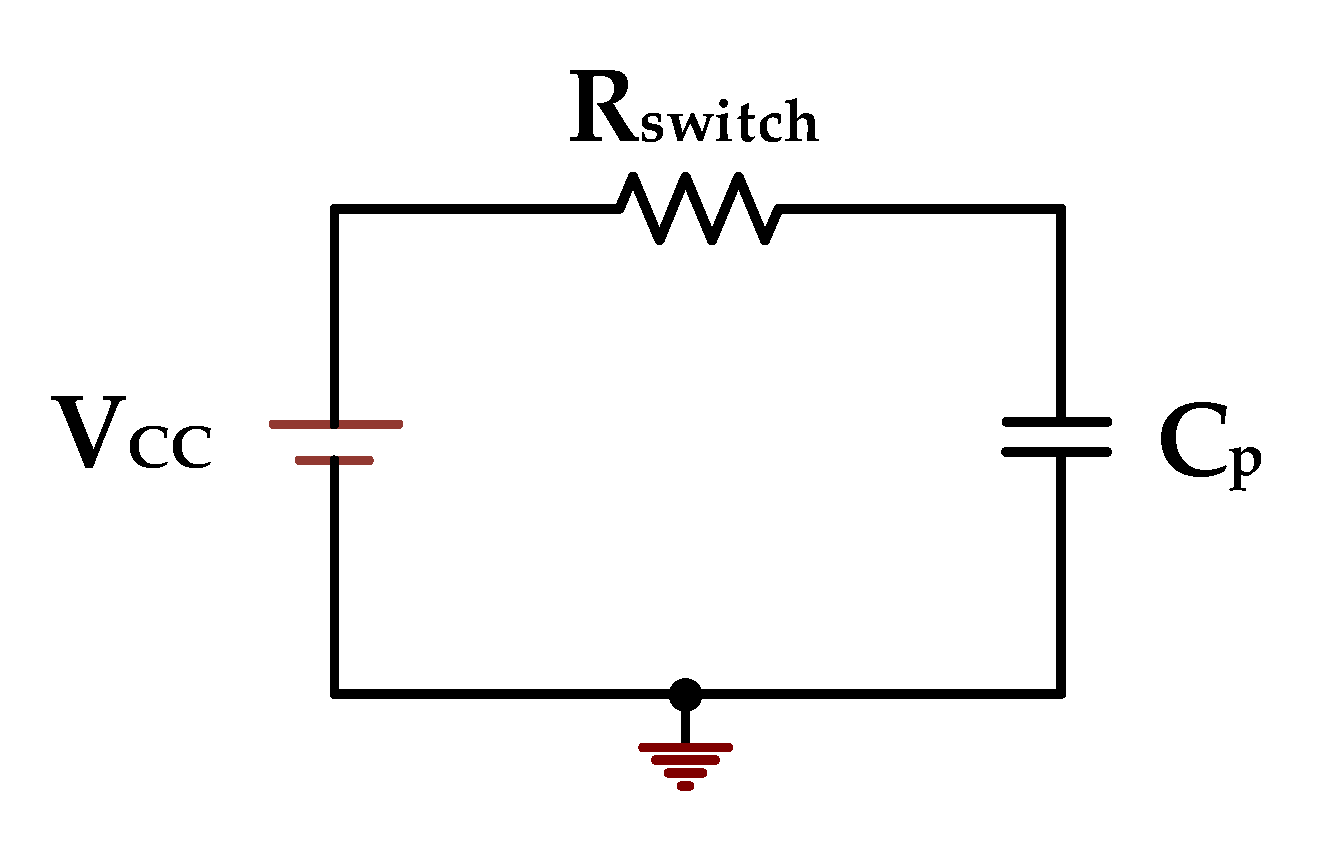
3.1. Conventional Driving Circuit Power Consumption
3.2. Inductor-Based Charge Recovery
4. Proposed Driving Circuit with Charge Recovery for the PE Fan
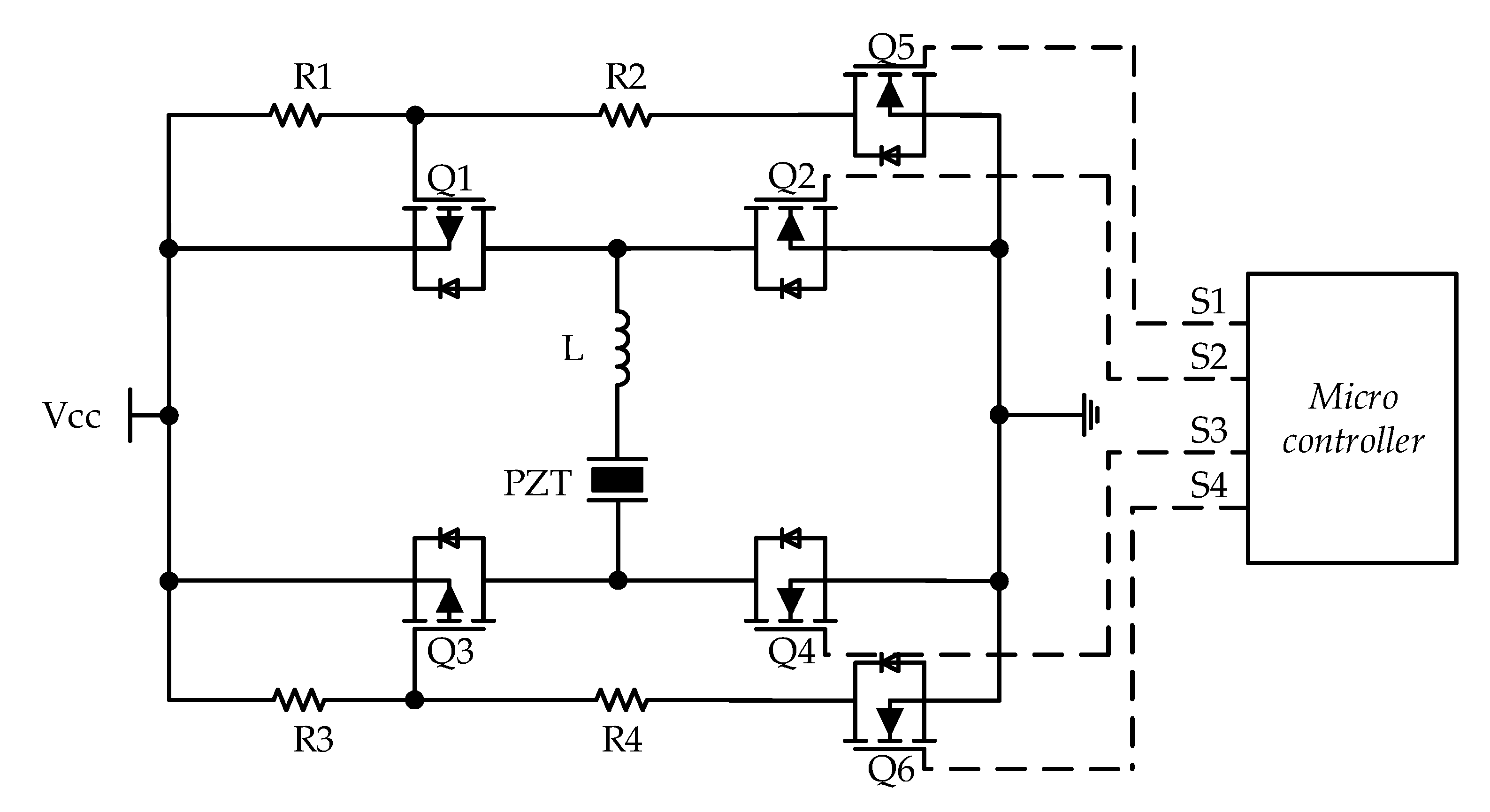
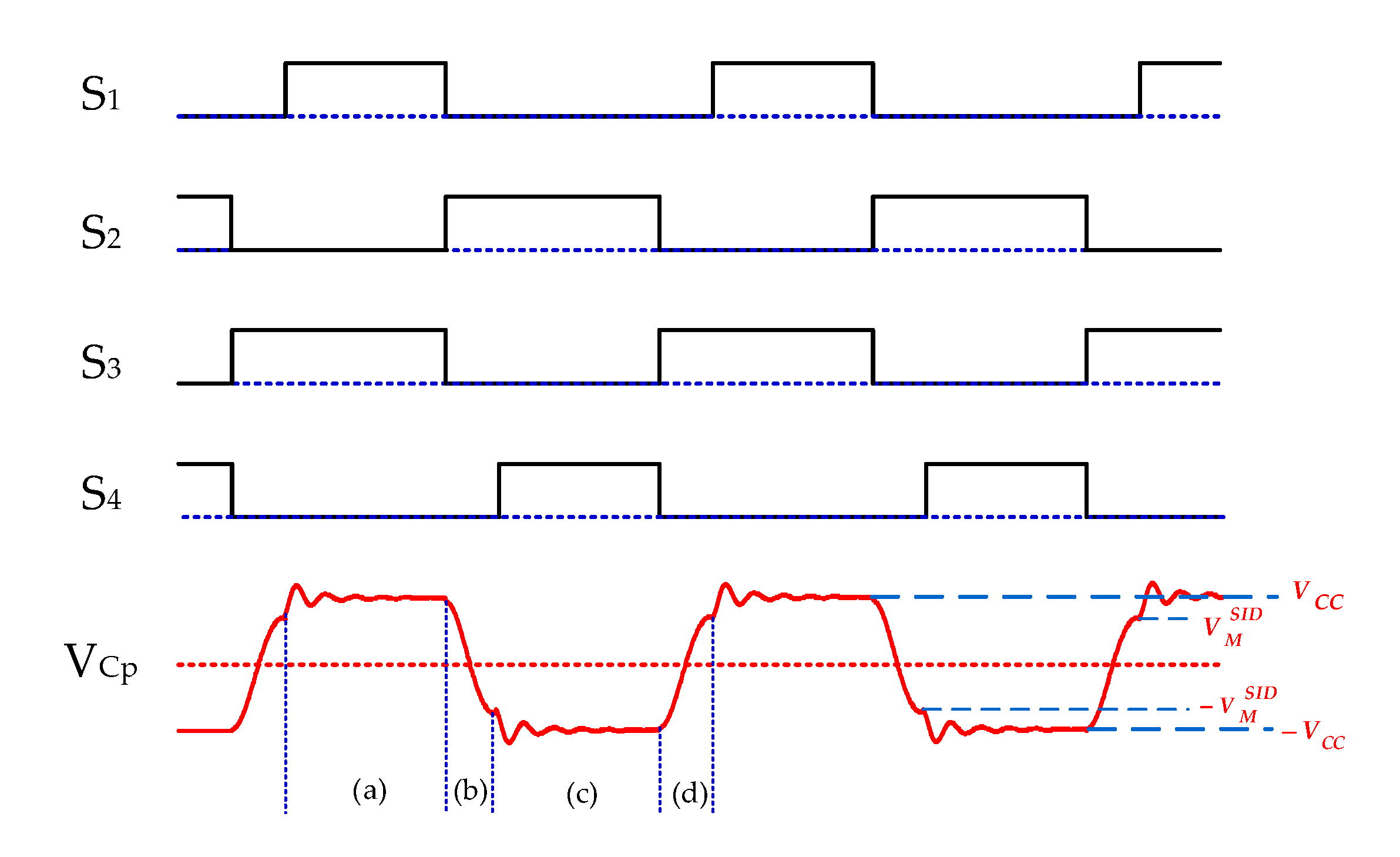
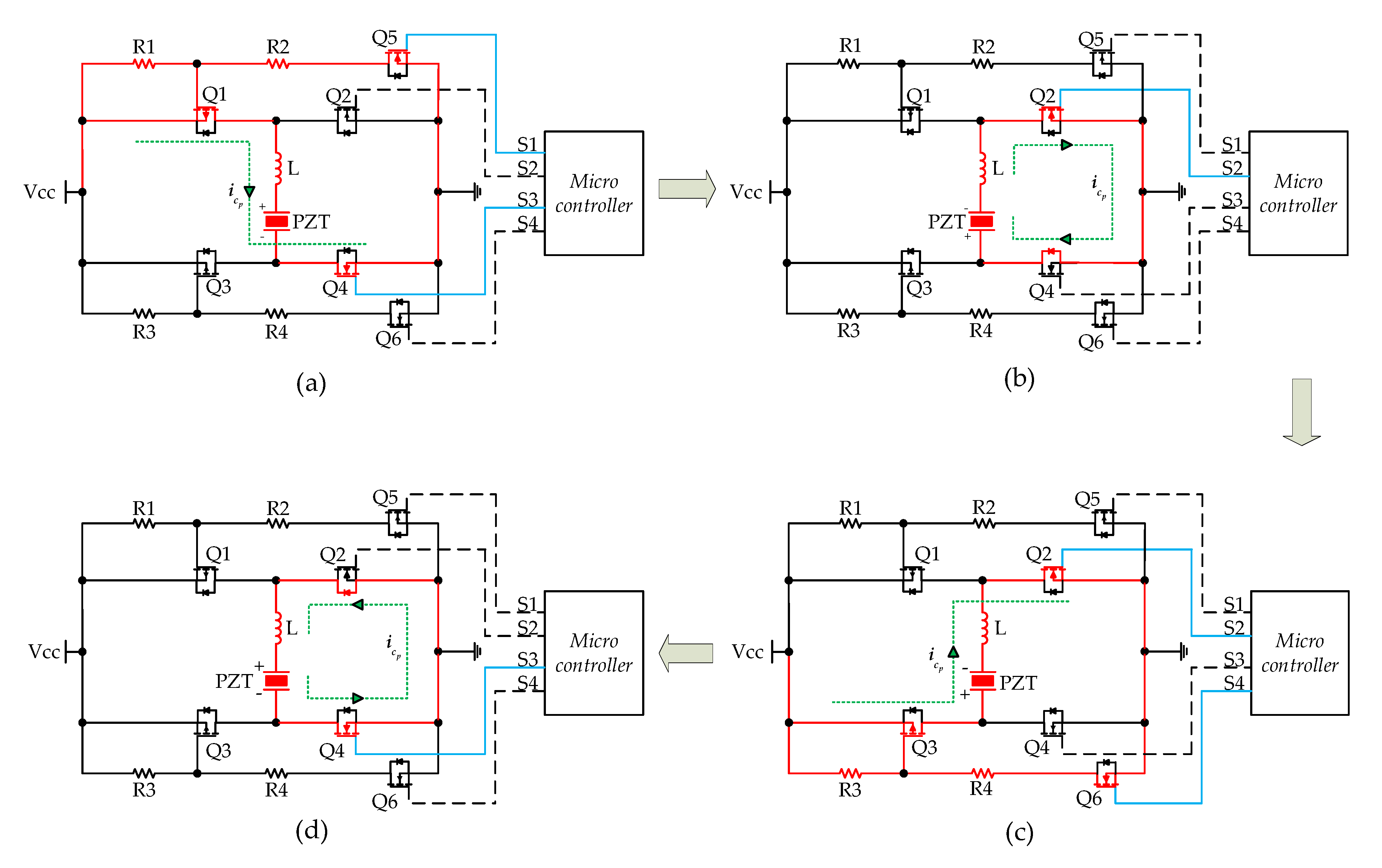
4.1. Single Inductor Based Driving (SID) Circuit
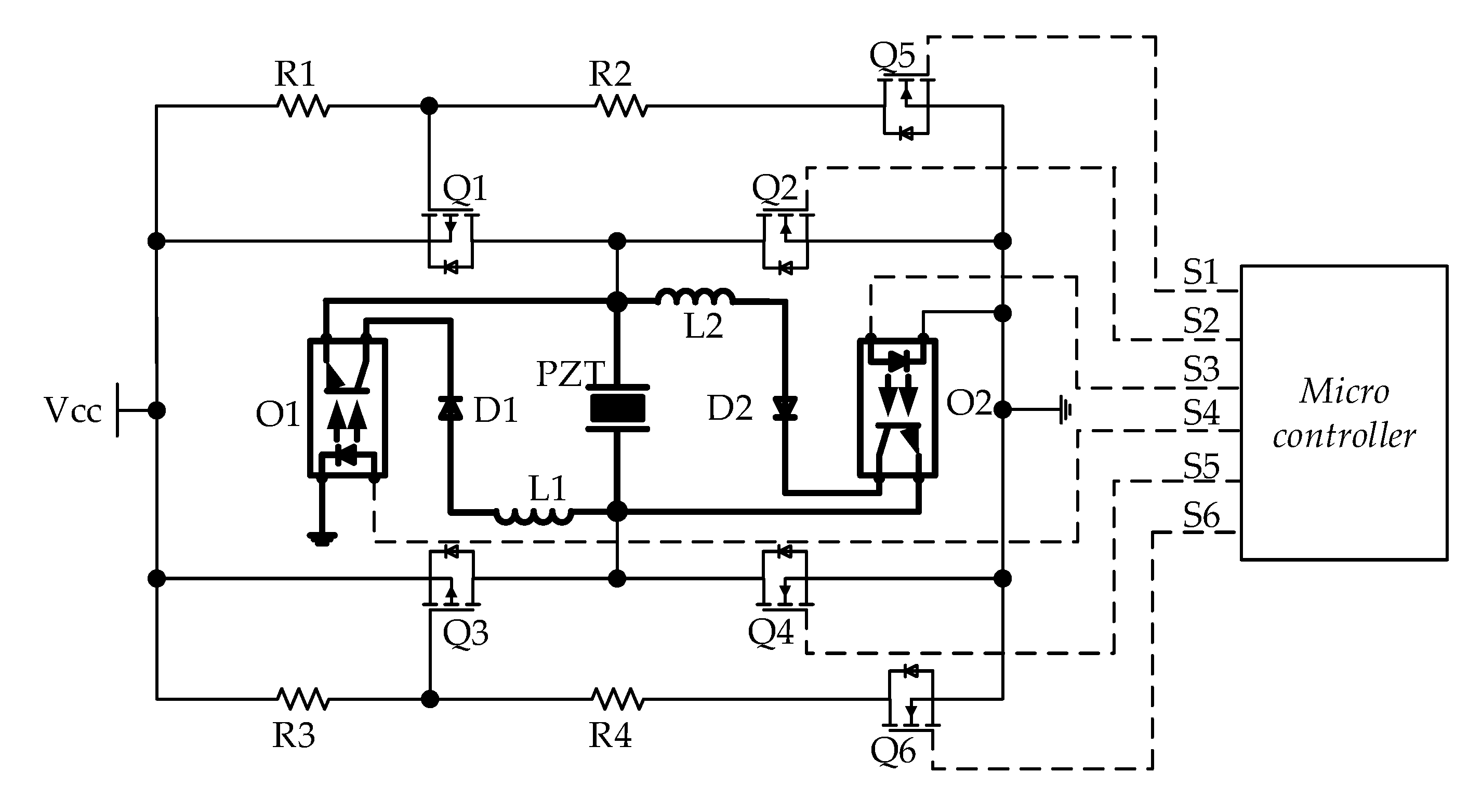
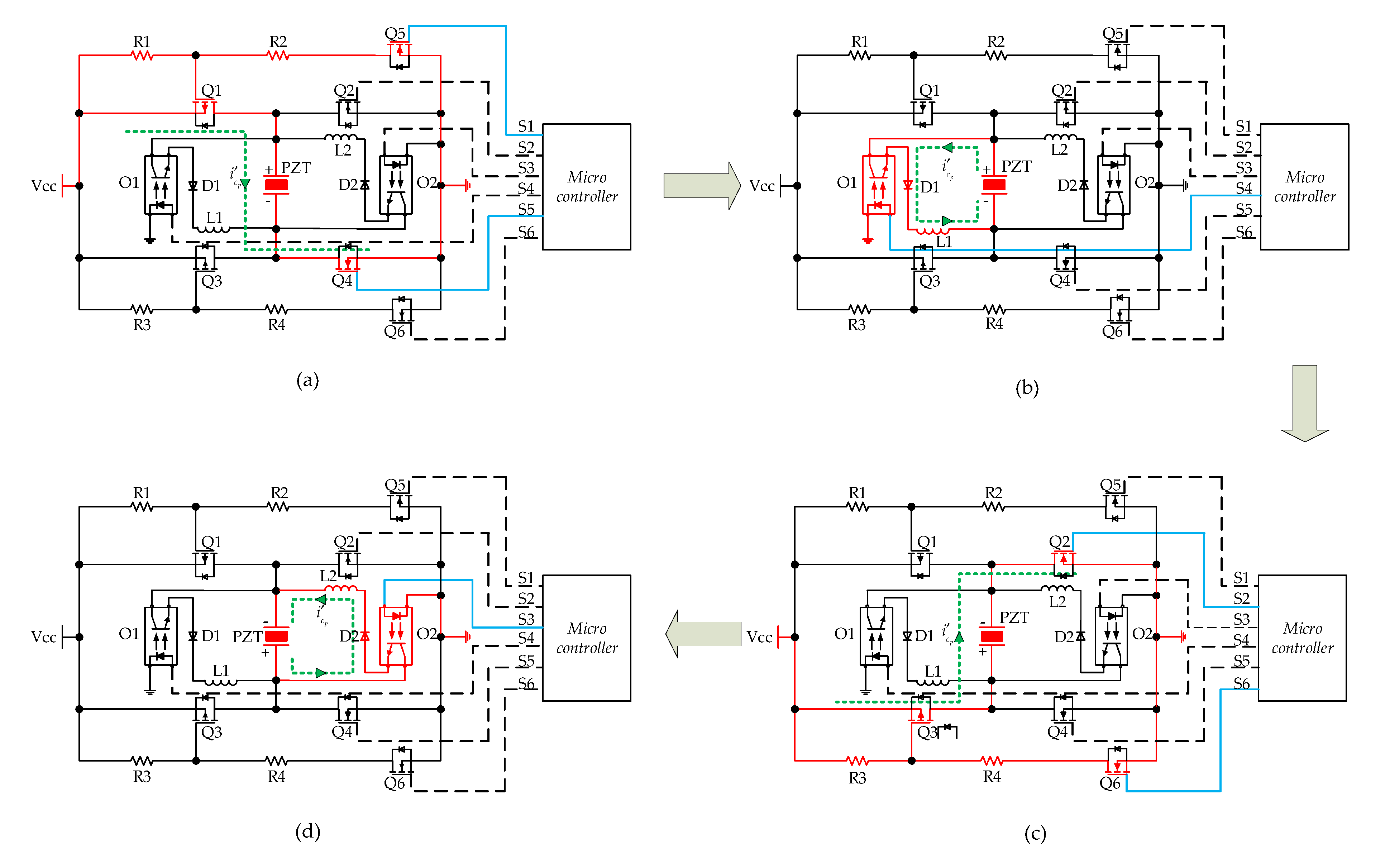
4.2. Double Inductor-Based Driving (DID) Circuit
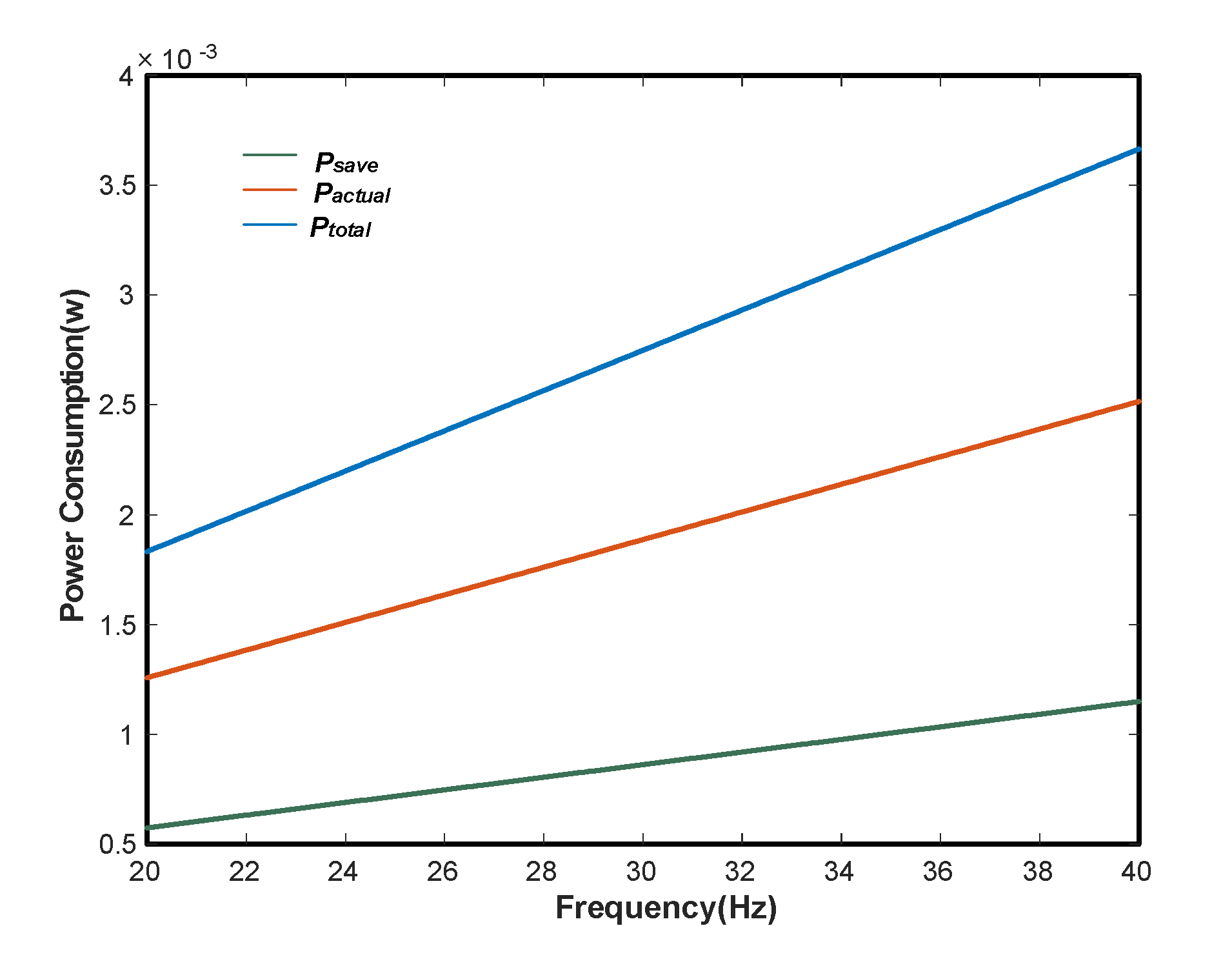
4.3. Energy Efficiency Analysis
5. Experimental Results and Discussion
5.1. Experimental Setup
5.2. Experimental Results
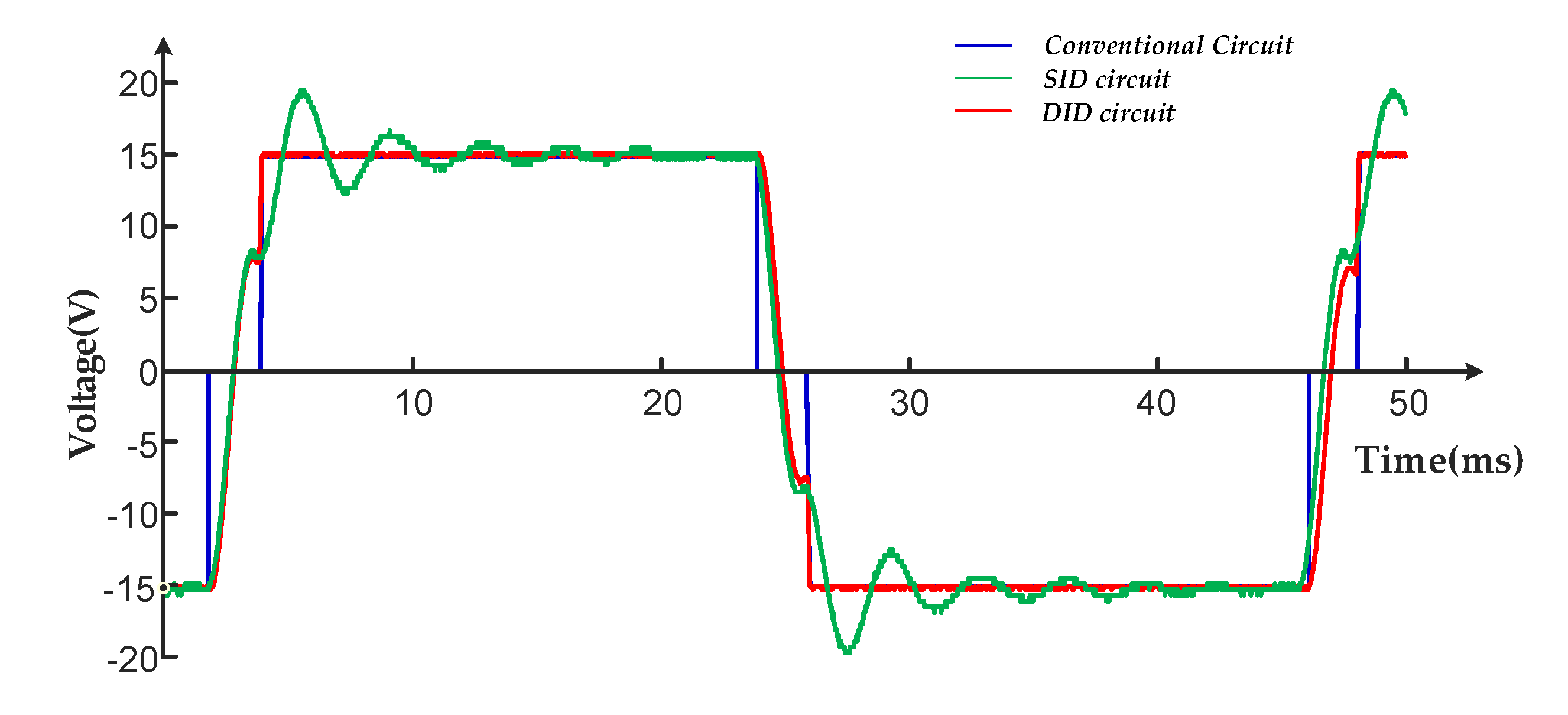
5.2.1. Validity of the Proposed Quasi-Square Wave Inductor-Based Driving Circuit
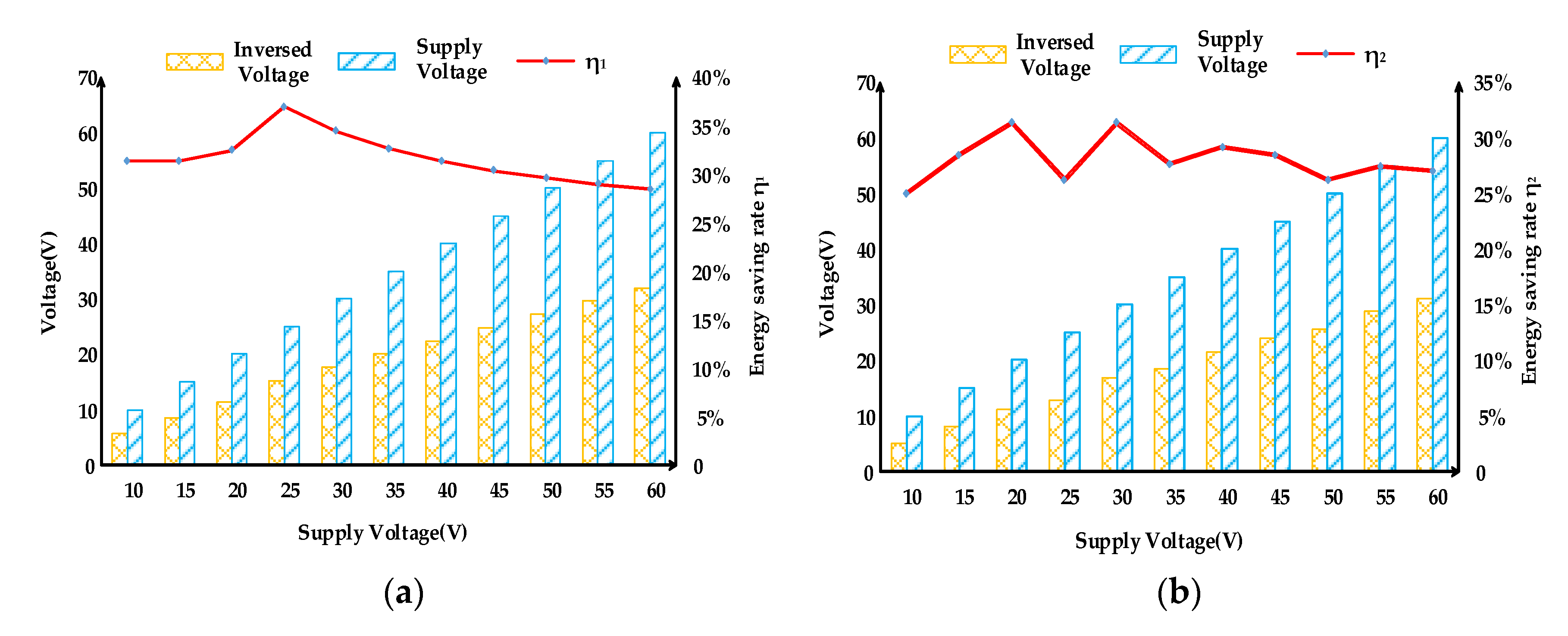
5.2.2. Energy Efficiency Calculation
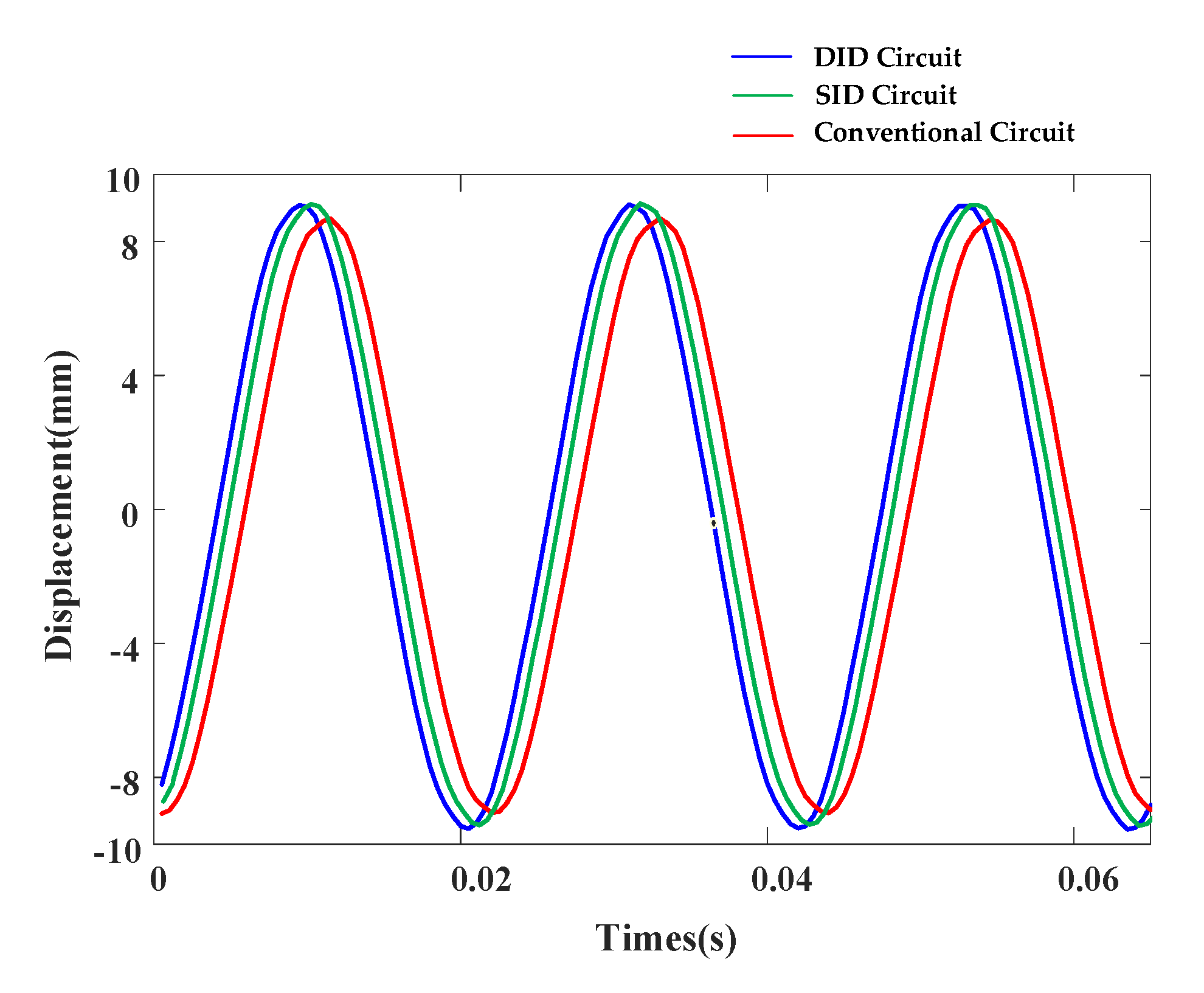
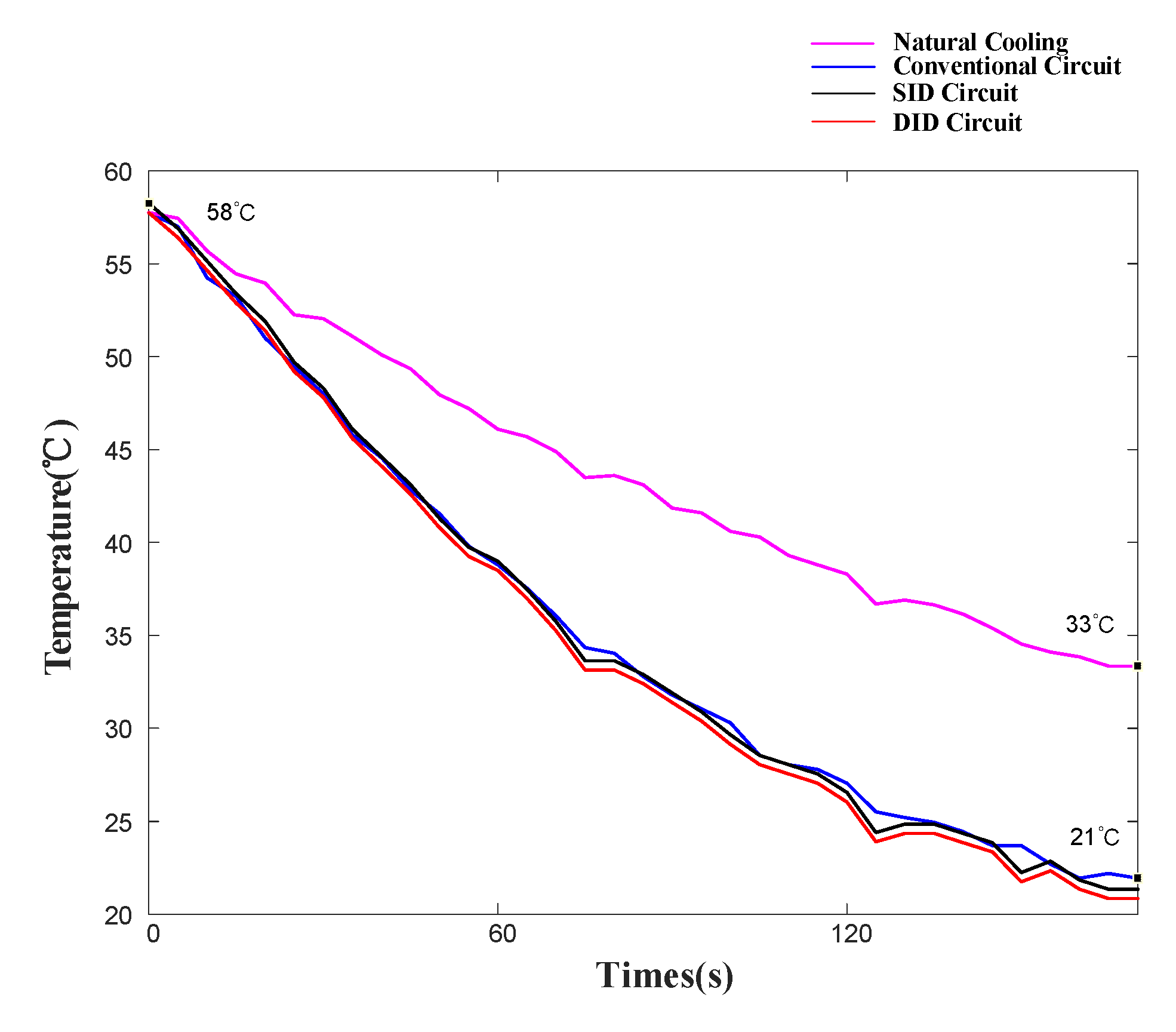
5.2.3. Vibration Displacement and Cooling Effect of the PE Fan
6. Conclusions
- (1)
- The proposed quasi-square wave inductor-based driving circuits reuse the energy stored in the piezoelectric patches without using the driving chip of the conventional driving circuit, which improves the efficiency and reduces the cost.
- (2)
- The SID circuit has the advantages of simple structure, fewer electrical components, and higher energy-saving rate, but accompanied by oscillation during the driving process, while the DID circuit has a more complicated structure and slightly lower energy-saving rate, but is stable without oscillation.
- (3)
- The energy-saving rate of both circuits fluctuates little with the supply voltage. The energy-saving rate of the SID circuit is around 30%, and that of DID circuit is around 28%.
- (4)
- When the piezoelectric fan is driven by the conventional driving circuit, SID circuit, and DID circuit respectively, the vibration displacement is the same, and there is no difference in cooling performance under the same conditions.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Conflicts of Interest
References
- Xie, H.; Zamkotsian, F. Editorial for the Special Issue on Optical MEMS. Micromachines 2019, 10, 458. [Google Scholar] [CrossRef] [PubMed]
- Crespí-Llorens, D.; Vicente, P.; Viedma, A. Flow Pattern of Non-Newtonian Fluids in Reciprocating Scraped Surface Heat Exchangers. Exp. Therm. Fluid Sci. 2016, 76, 306–323. [Google Scholar] [CrossRef]
- Quan, X.; Gao, M.; Cheng, P.; Li, J. An Experimental Investigation of Pool Boiling Heat Transfer on Smooth/Rib Surfaces under an Electric Field. Int. J. Heat Mass Transf. 2015, 85, 595–608. [Google Scholar] [CrossRef]
- Legay, M.; Gondrexon, N.; Le Person, S.; Boldo, P.; Bontemps, A. Enhancement of Heat Transfer by Ultrasound: Review and Recent Advances. Int. J. Chem. Eng. 2011, 2011, e670108. [Google Scholar] [CrossRef]
- Gugulothu, S.K.; Chamkha, A.J. Thermal Intensification of Heat Transfer Characteristics on the Plate-Fin Heat Sink with Piezoelectric Fan. Heat Transfer—Asian Res. 2019, 48, 2629–2638. [Google Scholar] [CrossRef]
- Krishan, G.; Aw, K.C.; Sharma, R.N. Synthetic Jet Impingement Heat Transfer Enhancement—A Review. Appl. Therm. Eng. 2019, 149, 1305–1323. [Google Scholar] [CrossRef]
- Sufian, S.F.; Abdullah, M.Z. Heat Transfer Enhancement of LEDs with a Combination of Piezoelectric Fans and a Heat Sink. Microelectron. Reliab. 2017, 68, 39–50. [Google Scholar] [CrossRef]
- Jeng, T.-M.; Tzeng, S.-C.; Wang, C.-X.; Peng, H.-Y. Flow Visualization and Thermal Resistance Measurement of the LED Heat Sinks with a Built-in Piezoelectric Fan and Various Flow-Runner Opens. Smart Sci. 2016, 4, 62–70. [Google Scholar] [CrossRef]
- Hales, A.; Jiang, X. A Review of Piezoelectric Fans for Low Energy Cooling of Power Electronics. Appl. Energy 2018, 215, 321–337. [Google Scholar] [CrossRef]
- Abdullah, M.K.; Ismail, N.C.; Abdullah, M.Z.; Mujeebu, M.A.; Ahmad, K.A.; Ripin, Z.M. Effects of Tip Gap and Amplitude of Piezoelectric Fans on the Performance of Heat Sinks in Microelectronic Cooling. Heat Mass Transf. 2012, 48, 893–901. [Google Scholar] [CrossRef]
- Sufian, S.F.; Abdullah, M.Z.; Abdullah, M.K.; Mohamed, J.J. Effect of Side and Tip Gaps of a Piezoelectric Fan on Microelectronic Cooling. IEEE Trans. Compon. Packag. Manuf. Technol. 2013, 3, 1545–1553. [Google Scholar] [CrossRef]
- Wait, S.M.; Basak, S.; Garimella, S.V.; Raman, A. Piezoelectric Fans Using Higher Flexural Modes for Electronics Cooling Applications. IEEE Trans. Compon. Packag. Technol. 2007, 30, 119–128. [Google Scholar] [CrossRef]
- Fairuz, Z.M.; Sufian, S.F.; Abdullah, M.Z.; Zubair, M.; Abdul Aziz, M.S. Effect of Piezoelectric Fan Mode Shape on the Heat Transfer Characteristics. Int. Commun. Heat Mass Transf. 2014, 52, 140–151. [Google Scholar] [CrossRef]
- Li, X.; Chen, W.; Lu, S. Characterization of the Thermal Performance of Multi Piezoelectric Fans for Cooling a Semi-Cylindrical Concave Surface. Int. J. Mech. Sci. 2021, 208, 106672. [Google Scholar] [CrossRef]
- Conway, C.; Jeffers, N.; Agarwal, A.; Punch, J. Influence of Thickness on the Flow Field Generated by an Oscillating Cantilever Beam. Exp. Fluids 2020, 61, 167. [Google Scholar] [CrossRef]
- Huang, C.-H.; Fan, G.-Y. Determination of Relative Positions and Phase Angle of Dual Piezoelectric Fans for Maximum Heat Dissipation of Fin Surface. Int. J. Heat Mass Transf. 2016, 92, 523–538. [Google Scholar] [CrossRef]
- Kim, Y.-H.; Wereley, S.T.; Chun, C.-H. Phase-Resolved Flow Field Produced by a Vibrating Cantilever Plate between Two Endplates. Phys. Fluids 2004, 16, 145–162. [Google Scholar] [CrossRef]
- Shyu, J.-C.; Syu, J.-Z. Plate-Fin Array Cooling Using a Finger-like Piezoelectric Fan. Appl. Therm. Eng. 2014, 62, 573–580. [Google Scholar] [CrossRef]
- Kimber, M.; Lonergan, R.; Garimella, S.V. Experimental Study of Aerodynamic Damping in Arrays of Vibrating Cantilevers. J. Fluids Struct. 2009, 25, 1334–1347. [Google Scholar] [CrossRef][Green Version]
- Liu, S.-F.; Huang, R.-T.; Sheu, W.-J.; Wang, C.-C. Heat Transfer by a Piezoelectric Fan on a Flat Surface Subject to the Influence of Horizontal/Vertical Arrangement. Int. J. Heat Mass Transf. 2009, 52, 2565–2570. [Google Scholar] [CrossRef]
- Abdullah, M.K.; Ismail, N.C.; Abdul Mujeebu, M.; Abdullah, M.Z.; Ahmad, K.A.; Husaini, M.; Hamid, M.N.A. Optimum Tip Gap and Orientation of Multi-Piezofan for Heat Transfer Enhancement of Finned Heat Sink in Microelectronic Cooling. Int. J. Heat Mass Transf. 2012, 55, 5514–5525. [Google Scholar] [CrossRef]
- Jeng, T.-M.; Tzeng, S.-C.; Huang, Y.-C. The Orientation Effect of Driven Air Flow on Cooling Performance of the Cylindrical Heat Sink with a Built-in Horizontally-Placed Fan. Int. Commun. Heat Mass Transf. 2017, 81, 190–200. [Google Scholar] [CrossRef]
- Açıkalın, T.; Garimella, S.V.; Raman, A.; Petroski, J. Characterization and Optimization of the Thermal Performance of Miniature Piezoelectric Fans. Int. J. Heat Fluid Flow 2007, 28, 806–820. [Google Scholar] [CrossRef]
- Chen, Y.; Peng, D.; Liu, Y. Heat Transfer Enhancement of Turbulent Channel Flow Using a Piezoelectric Fan. Int. J. Heat Mass Transf. 2020, 147, 118964. [Google Scholar] [CrossRef]
- Tiwari, J.; Yeom, T. Enhancement of Channel-Flow Convection Heat Transfer Using Piezoelectric Fans. Appl. Therm. Eng. 2021, 191, 116917. [Google Scholar] [CrossRef]
- Dehdari Ebrahimi, N.; Eldredge, J.D.; Ju, Y.S. Three-Dimensional Characteristics of the Jet Flows Induced by a Pitching Plate in a Quiescent Fluid. J. Fluid Mech. 2020, 887, A25. [Google Scholar] [CrossRef]
- Liang, Z.; Xu, C.; Ren, B.; Di, W.; Li, L.; Luo, H.; Chen, Z.; Su, J. Optimization of Cantilevered Piezoelectric Energy Harvester with a Fixed Resonance Frequency. Sci. China Technol. Sci. 2014, 57, 1093–1100. [Google Scholar] [CrossRef]
- Maaspuro, M. Piezoelectric Oscillating Cantilever Fan for Thermal Management of Electronics and LEDs—A Review. Microelectron. Reliab. 2016, 63, 342–353. [Google Scholar] [CrossRef]
- Yong, Y.K.; Fleming, A.J. Piezoelectric Actuators With Integrated High-Voltage Power Electronics. IEEEASME Trans. Mechatron. 2015, 20, 611–617. [Google Scholar] [CrossRef]
- Chao, C.-S.; Huang, P.-C.; Chen, M.-K.; Jang, L.-S. Design and Analysis of Charge-Recovery Driving Circuits for Portable Peristaltic Micropumps with Piezoelectric Actuators. Sens. Actuators Phys. 2011, 168, 313–319. [Google Scholar] [CrossRef]
- Campolo, D.; Sitti, M.; Fearing, R.S. Efficient Charge Recovery Method for Driving Piezoelectric Actuators with Quasi-Square Waves. IEEE Trans. Ultrason. Ferroelectr. Freq. Control 2003, 50, 237–244. [Google Scholar] [CrossRef]
- Biancuzzi, G.; Lemke, T.; Woias, P.; Ruthmann, O.; Schrag, H.J.; Vodermayer, B.; Schmid, T.; Goldschmidtboeing, F. Performance of Piezoelectric Micropumps Actuated by Charge Recovery. Procedia Chem. 2009, 1, 698–701. [Google Scholar] [CrossRef][Green Version]
- Biancuzzi, G.; Lemke, T.; Woias, P.; Ruthmann, O.; Schrag, H.J.; Vodermayer, B.; Schmid, T.; Goldschmidtboeing, F. Design and Simulation of Advanced Charge Recovery Piezoactuator Drivers. J. Micromech. Microeng. 2010, 20, 105022. [Google Scholar] [CrossRef]
- Xu, Z.; Wang, Y.; Chen, C. Micro Converter with a High Step-up Ratio to Drive a Piezoelectric Bimorph Actuator Applied in Mobile Robots. Int. J. Adv. Robot. Syst. 2018, 15, 172988141876345. [Google Scholar] [CrossRef]
- Ren, Y.; Tang, W.; Wang, Y.; Wang, L.; Cui, M.; Li, Z. Switch Controlled Charge Recovery Technique for Piezoelectric Fan. In Proceedings of the 2019 31st Chinese Control and Decision Conference (CCDC 2019), Nanchang, China, 3–5 June 2019; IEEE: New York, NY, USA, 2019; pp. 2259–2264. [Google Scholar]
- Tang, W.; Wang, L.; Ren, Y.; Bao, B.; Cao, J. Design and Experimental Analysis of Self-Sensing SSDNC Technique for Semi-Active Vibration Control. Smart Mater. Struct. 2018, 27, 085028. [Google Scholar] [CrossRef]
- Lei, T.; Jing-zhou, Z.; Xiao-ming, T. Numerical Investigation of Convective Heat Transfer on a Vertical Surface Due to Resonating Cantilever Beam. Int. J. Therm. Sci. 2014, 80, 93–107. [Google Scholar] [CrossRef]




| Parameter | Symbol | Value |
|---|---|---|
| Resistor | R1,R3 | 500 KΩ |
| Resistor | R2,R4 | 2 MΩ |
| PMOS | Q1,Q3 | IRF4905 |
| NMOS | Q2,Q4,Q5,Q6 | IRF740 |
| Inductance | L,L1,L2 | 3H |
| Optocoupler | O1,O2 | TLP521 |
| Diode | D1,D2 | IN4148 |
| Parameter | Symbol | Value |
|---|---|---|
| Young’s modulus of the beam | ES | 70 GPa |
| Length of the beam | LS | 150 mm |
| Width of the beam | wS | 26 mm |
| Thickness of the beam | hS | 0.6 mm |
| Density of the beam | 2700 kg/m3 | |
| 1st bending mode | 24.2 Hz | |
| Young’s modulus of the PZT | EP | 67 GPa |
| Length of the PZT | lP | 50.8 mm |
| Width of the PZT | wP | 25.4 mm |
| Thickness of the PZT | hP | 0.3 mm |
| Density of the PZT | 7800 kg/m3 | |
| Capacitance of the PZT | Cp | 101.8 nF |
| Internal Resistance of the PZT | Rp | 4 MΩ |
| Piezoelectric constant | d31 | −190 pm/V |
| Constant strain permittivity | 15.9 nF/m |
| Supply Voltage (V) | Inversed Voltage (V) | Energy-Saving Rate of SID η1 | |
|---|---|---|---|
| 10 | 5.6 | 0.077 | 31.36% |
| 15 | 8.4 | 0.174 | 31.36% |
| 20 | 11.4 | 0.320 | 32.49% |
| 25 | 15.2 | 0.569 | 36.97% |
| 30 | 17.6 | 0.763 | 34.42% |
| 35 | 20 | 0.985 | 32.65% |
| 40 | 22.4 | 1.236 | 31.36% |
| 45 | 24.8 | 1.515 | 30.37% |
| 50 | 27.2 | 1.823 | 29.59% |
| 55 | 29.6 | 2.158 | 28.96% |
| 60 | 32 | 2.523 | 28.44% |
| Supply Voltage (V) | Inversed Voltage (V) | Energy-Saving Rate of DID η2 | ||
|---|---|---|---|---|
| 10 | 5 | 0.062 | 25.00% | |
| 15 | 8 | 0.158 | 28.44% | |
| 20 | 11.2 | 0.309 | 31.36% | |
| 25 | 12.8 | 0.404 | 26.21% | |
| 30 | 16.8 | 0.695 | 31.36% | |
| 35 | 18.4 | 0.834 | 27.64% | |
| 40 | 21.6 | 1.149 | 29.16% | |
| 45 | 24 | 1.419 | 28.44% | |
| 50 | 25.6 | 1.615 | 26.21% | |
| 55 | 28.8 | 2.043 | 27.42% | |
| 60 | 31.2 | 2.398 | 27.04% |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Chen, Z.; Tang, W.; Li, Z.; Lan, J. Design and Experimental Analysis of Charge Recovery for Piezoelectric Fan. Actuators 2022, 11, 20. https://doi.org/10.3390/act11010020
Chen Z, Tang W, Li Z, Lan J. Design and Experimental Analysis of Charge Recovery for Piezoelectric Fan. Actuators. 2022; 11(1):20. https://doi.org/10.3390/act11010020
Chicago/Turabian StyleChen, Zhenwei, Wei Tang, Ze Li, and Jiaqi Lan. 2022. "Design and Experimental Analysis of Charge Recovery for Piezoelectric Fan" Actuators 11, no. 1: 20. https://doi.org/10.3390/act11010020
APA StyleChen, Z., Tang, W., Li, Z., & Lan, J. (2022). Design and Experimental Analysis of Charge Recovery for Piezoelectric Fan. Actuators, 11(1), 20. https://doi.org/10.3390/act11010020






