A Review of Piezoelectric Material-Based Structural Control and Health Monitoring Techniques for Engineering Structures: Challenges and Opportunities
Abstract
1. Introduction
2. Piezoelectric Materials
2.1. Piezoelectric–Mechanical Constitutive Equations
2.2. Reduced-Order Modal Equations
2.3. Piezoelectric Material Type-Based Investigation and Issues
- In comparison to the host structure, the PZT transducer should be non-reactive and have marginal stiffness and strength. It should also be protected from environmental factors such as humidity, precipitation, and temperature.
- The frequency spectrum of excitation determines the sensing region of the PZT transducer. A wider sensing domain is covered by frequencies below 30 kHz. High-frequency EMI models are only applicable to a small region.
- An arrangement has parts that are weak or essential that need more effort than others. High-stress fields, corrosive environment areas, and so on, must all be monitored closely. The length, distance, and thickness of the PZT actuation are all three directions. As a result, a detailed estimate of the PZT-sensing region must be determined using these three types of actuations, based on the geometry and material properties of the host structure.
- In the absence of damages, PZT transducers are effective at measuring the loading on a structure, or vice versa. The study on PZT-based EMI for load applications is largely limited to 1D structures, but [31] shows some work on 2D, 3D, and complex structures.
- To track any structure using EMI-based SHM, the current usual practice is to first acquire a baseline signature. This is then compared to later levels of the structure’s EM admittance signatures to see if the structure has any flaws. It is very difficult to achieve the no-damage baseline signature for older current systems, making comparisons with later stage signatures almost impossible. As a result, any signature obtained from any structure at any point in time should provide overt or implied knowledge about the structure.
- As the embedded or surface bonded PZT transducer is excited, the ‘structural responses’ are extracted and expressed as conductance and susceptibility signatures. The structural reaction varies with the frequency of excitation. The effectiveness of any non-parametric index is determined by its ability to detect harm using all modified peaks.
- Ultrasonic technology, acoustic absorption, magnetic field analysis, global structural reaction analysis, and visual inspection techniques have also been proven to be useful at identifying damage early on. Regardless of their usefulness, both of these approaches should be used in conjunction with the EMI technique.
3. Structural Control Using Piezoelectric Material
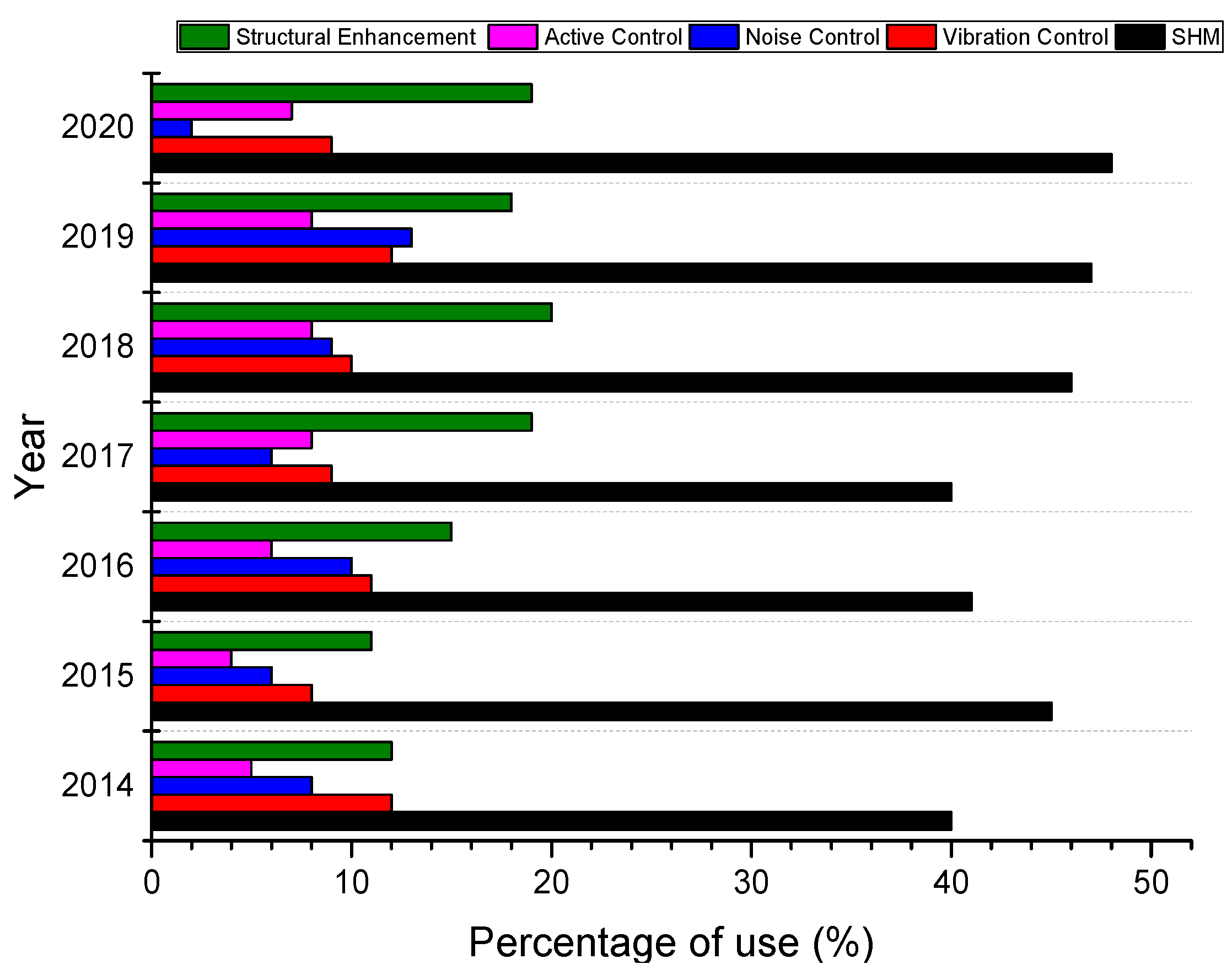
3.1. Vibration Control
3.2. Noise Control
3.3. Active Control
4. Structural Health Monitoring
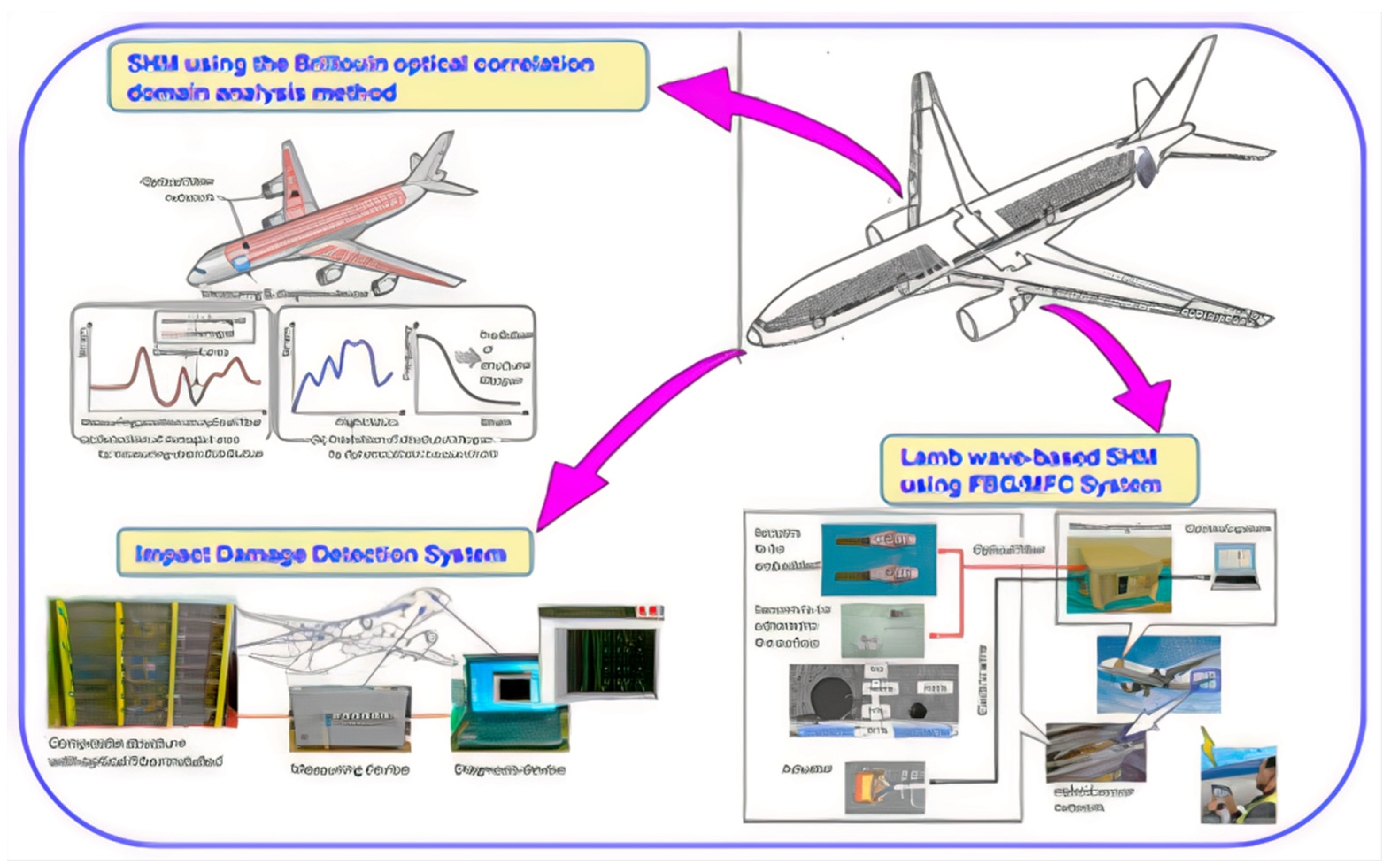
4.1. Aerospace Structures
4.2. Concrete Structures
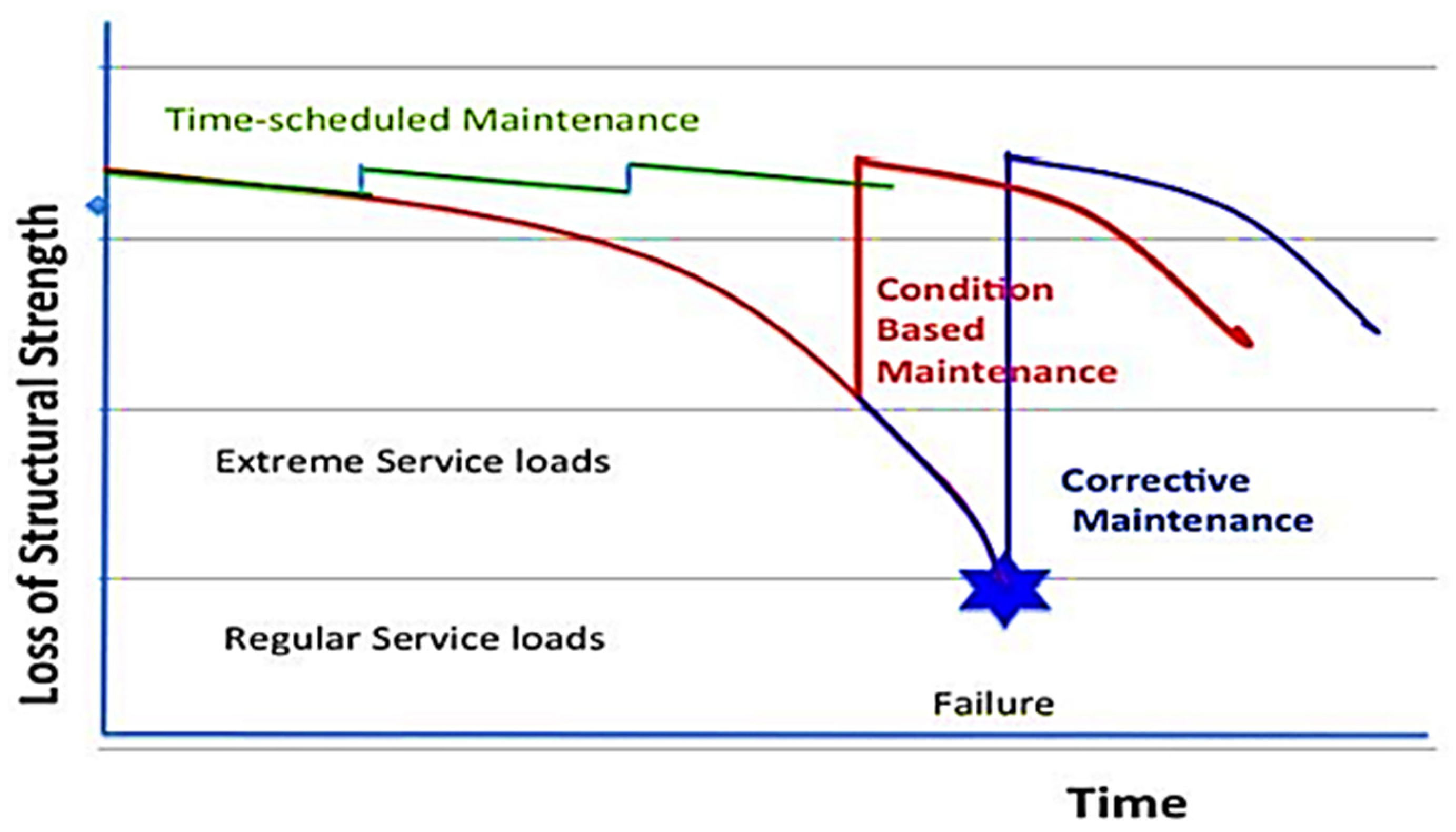
4.3. Practical Issues
5. Challenges and Opportunities
- It is difficult to install the piezoelectric to the host structures and generate a high-frequency range; therefore, the piezoelectric is packaged in a way that makes installation easier [148].
- Modern civil infrastructure projects were designed to provide specialized functionality for multi-purpose applications in extreme weather situations, including earthquakes, hurricanes, and typhoons. These dynamic structural structures raise significant questions about their stability. Integrating a network of smart and embeddable sensors into civil infrastructure systems with local artificial intelligence (AI)/machine learning (ML) data-processing platforms is a promising solution to this problem, allowing next-generation smart, civil infrastructure systems to be installed on the skeleton of conventional systems [149].
- The lack of a computational platform on which to develop new techniques for realizing massively distributed smart sensors is one of the crucial issues listed in [150] and the possible development of the next generation of SHM systems.
- Although they have been the focus of intense study for many decades, computational approximation and simulation of fluid–structure interactions remain an undeniably difficult topic with many unsolved problems and concerns, and the “arbitrary Lagrangian-Eulerian” method is a standard framework for solving fluid–structure interaction problems, as we emphasize [112].
- SHM can be used to measure civil industries that are live loads on bridge systems as well as environmental loads and to spot any significant differences from the values assumed in bridge-building codes. Given raising questions about climate change and its possible effect on the stability and serviceability of concrete bridge systems due to increased wind loads, floods, thermal gradients, freeze–thaw cycles, deicing salt use, and other factors, this is becoming an important topic [151].
6. Conclusions
- The procedure of control and repair used to preserve the structural integrity of damaged components is distinctive. It is established on the converse piezoelectric effect, in which the local moment and force induced in the piezoelectric materials by an applied electric field would make it easier for the structure to prevent the development of high stress and strain levels because of external load and thus lessens the criticality of the damage.
- Structural health monitoring is also proving highly significant in avoiding the premature collapse of structures based on aerospace and civil industries such as offshore platforms, houses, bridges, and underground structures.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- PI Piezo Technology: “DuraAct Piezoelectric Transducers”: PI Piezo Technology. 2017. Available online: https://www.piceramic.com/en/products/piezoceramic-actuators/patch-transducers/ (accessed on 31 March 2017).
- Saraiva, F. Development of Press Forming Techniques for Thermoplastic Composites Investigation of a Multiple Step Forming Approach. Master’s Thesis, TU Delft, Delft, The Netherlands, 2017. [Google Scholar]
- Sun, D.; Wang, D. Distributed Piezoelectric Element Method for Vibration Control of Smart Plates. AIAA J. 1999, 37, 1459–1463. [Google Scholar] [CrossRef]
- Wang, Q.; Quek, S.T. Repair of delaminated beams via piezoelectric patches. Smart Mater. Struct. 2004, 13, 1222–1229. [Google Scholar] [CrossRef]
- Wang, Q.; Duan, W.H.; Quek, S.T. Repair of notched beam under dynamic load using piezoelectric patch. Int. J. Mech. Sci. 2004, 46, 1517–1533. [Google Scholar] [CrossRef]
- Alaimo, A.; Milazzo, A.; Orlando, C. Boundary elements analysis of adhesively bonded piezoelectric active repair. Eng. Fract. Mech. 2009, 76, 500–511. [Google Scholar] [CrossRef]
- Alaimo, A.; Milazzo, A.; Orlando, C. Numerical analysis of a piezoelectric structural health monitoring system for composite flange-skin delamination detection. Compos. Struct. 2013, 100, 343–355. [Google Scholar] [CrossRef]
- Kapuria, S.; Yasin, M.Y.; Hagedorn, P. Active Vibration Control of Piezolaminated Composite Plates Considering Strong Electric Field Nonlinearity. AIAA J. 2015, 53, 603–616. [Google Scholar] [CrossRef]
- Zhang, C.; Nanthakumar, S.S.; Lahmer, T.; Rabczuk, T. Multiple cracks identification for piezoelectric structures. Int. J. Fract. 2017, 206, 151–169. [Google Scholar] [CrossRef]
- Krishna, P.; Mallik, S.; Rao, D.S. Vibration Control on Composite Beams With Multiple Piezoelectric Patches Using Finite Element Analysis. Int. Res. J. Eng. Technol. 2017, 4, 906–911. [Google Scholar]
- Dineva, P.; Gross, D.; Müller, R.; Rangelov, T. Dynamic Fracture of Piezoelectric Materials; Springer International Publishing: Cham, Switzerland, 2014; Volume 212. [Google Scholar]
- Holterman, J.; Groen, P. An Introduction to Piezoelectric Materials and Applications; Stichting Applied Piezo: Apeldoorn, The Netherlands, 2013; ISBN 978-9081936118. [Google Scholar]
- Curie, J.; Curie, P. Développement, par pression, de l’électricité polaire dans les cristaux hémièdres à faces inclinées. Comptes Rendus de l’Académie des Sciences 1880, 91, 294–295. [Google Scholar]
- Chee, C.Y.K.; Tong, L.; Steven, G.P. A review on the modelling of piezoelectric sensors and actuators incorporated in intelligent structures. J. Intell. Mater. Syst. Struct. 1998, 9, 3–19. [Google Scholar] [CrossRef]
- De Jong, M.; Chen, W.; Geerlings, H.; Asta, M.; Persson, K.A. A database to enable discovery and design of piezoelectric materials. Sci. Data 2015, 2, 1–13. [Google Scholar]
- Qin, Q.H. Advanced Mechanics of Piezoelectricity; Springer-Verlag: Berlin/Heidelberg, Germany, 2013; Volume 9783642297, ISBN 9783642297670. [Google Scholar]
- Dahiya, A.; Thakur, O.P.; Juneja, J.K. Sensing and actuating applications of potassium sodium niobate: Use of potassium sodium niobate in sensor and actuator. Proc. Int. Conf. Sens. Technol. ICST 2013, 383–386. [Google Scholar] [CrossRef]
- Samal, M.K.; Seshu, P.; Dutta, B.K. Modeling and application of piezoelectric materials in smart structures. Int. J. COMADEM 2007, 10, 30. [Google Scholar]
- Benjeddou, A. Shear-mode piezoceramic advanced materials and structures: A state of the art. Mech. Adv. Mater. Struct. 2007, 14, 263–275. [Google Scholar] [CrossRef]
- Lang, S.B.; Muensit, S. Review of some lesser-known applications of piezoelectric and pyroelectric polymers. Appl. Phys. A Mater. Sci. Process. 2006, 85, 125–134. [Google Scholar] [CrossRef]
- Hall, D.A. Nonlinearity in piezoelectric ceramics. J. Mater. Sci. 2001, 36, 4575–4601. [Google Scholar] [CrossRef]
- Ramegowda, P.C.; Ishihara, D.; Takata, R.; Niho, T.; Horie, T. Hierarchically decomposed finite element method for a triply coupled piezoelectric, structure, and fluid fields of a thin piezoelectric bimorph in fluid. Comput. Methods Appl. Mech. Eng. 2020, 365, 113006. [Google Scholar] [CrossRef]
- Lee, T.; Lakes, R. Damping properties of lead metaniobate. IEEE Trans. Ultrason. Ferroelectr. Freq. Control 2001, 48, 48–52. [Google Scholar]
- Nogas-Ćwikiel, E. Fabrication of Mn Doped PZT for Ceramic-Polymer Composites. Arch. Metall. Mater. 2011, 56, 2–6. [Google Scholar] [CrossRef]
- Arnau, A.; Soares, D. Fundamentals of piezoelectricity. In Piezoelectric Transducers and Applications; Springer-Verlag: Berlin/Heidelberg, Germany, 2008; pp. 1–38. ISBN 9783540775072. [Google Scholar]
- Bafandeh, M.R.; Gharahkhani, R.; Lee, J.S. Dielectric and piezoelectric properties of sodium potassium niobate-based ceramics sintered in microwave furnace. Mater. Chem. Phys. 2015, 156, 254–260. [Google Scholar] [CrossRef]
- Arian Nijmeijer, H.K. Synthesis and Properties of Lead Magnesium Niobate Zirconate. J. Am. Ceram. Soc. 1997, 21, 2717–2721. [Google Scholar]
- Pmn, W. Lead Magnesium Niobate. 1958. Available online: http://research.physics.illinois.edu/Publications/theses/copies/fanning/4plmn.pdf (accessed on 5 April 2021).
- Pasquali, M.; Gaudenzi, P. A nonlinear formulation of piezoelectric plates. J. Intell. Mater. Syst. Struct. 2012, 23, 1713–1723. [Google Scholar] [CrossRef]
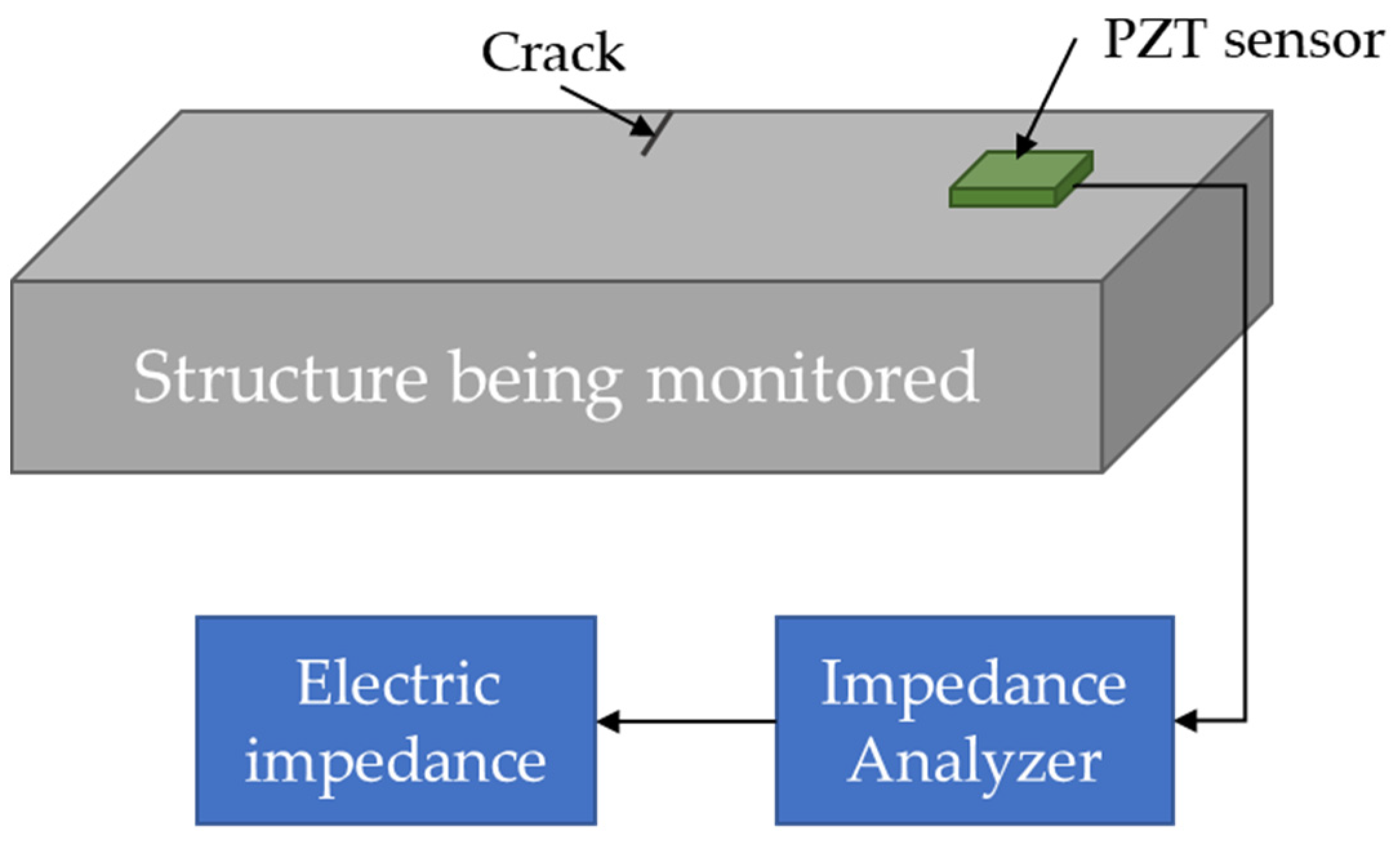
- Annamdas, V.G.M.; Soh, C.K. Application of electromechanical impedance technique for engineering structures: Review and future issues. J. Intell. Mater. Syst. Struct. 2010, 21, 41–59. [Google Scholar] [CrossRef]
- Giurgiutiu, V. Structural Health Monitoring: With Piezoelectric Wafer Active Sensors; Elsevier: Amsterdam, The Netherlands, 2007. [Google Scholar]
- Swigert, C.J.; Forward, R.L. Electronic damping of orthogonal bending modes in a cylindrical mast-theory. J. Spacecr. Rockets 1981, 18, 5–10. [Google Scholar] [CrossRef]
- Iyengar, N.G.R.; Kamle, S. Development and application of flexible piezo patches for vibration control. In Society of Expt. Mechanical Proceedin; 2003; pp. 48–49. [Google Scholar]
- Sharma, A.; Kumar, R.; Vaish, R.; Chauhan, V.S. Experimental and numerical investigation of active vibration control over wide range of operating temperature. J. Intell. Mater. Syst. Struct. 2016, 27, 1846–1860. [Google Scholar] [CrossRef]
- Bao, B.; Guyomar, D.; Lallart, M. Vibration reduction for smart periodic structures via periodic piezoelectric arrays with nonlinear interleaved-switched electronic networks. Mech. Syst. Signal Process. 2016, 1–29. [Google Scholar] [CrossRef]
- Casagrande, D.; Gardonio, P.; Zilletti, M. Smart panel with time-varying shunted piezoelectric patch absorbers for broadband vibration control. J. Sound Vib. 2017, 400, 288–304. [Google Scholar] [CrossRef]
- Bailey, T.; Hubbard, J.E. Distributed Piezoelectric-Polymer Active Vibration Control of a Cantilever Beam. J. Guid. Control Dyn. 1985, 8, 605–611. [Google Scholar] [CrossRef]
- Yue, H.; Lu, Y.; Deng, Z.; Tzou, H. Experiments on vibration control of a piezoelectric laminated paraboloidal shell. Mech. Syst. Signal Process. 2016, 1–17. [Google Scholar] [CrossRef]
- Hagood, N.W.; Flotow, A. Von Damping of Structural Vibrations with Piezoelectric Materials and Passive Electrical Networks. J. Sound Vib. 1991, 146, 243–268. [Google Scholar] [CrossRef]
- Clark, W.W. Vibration Control with State-Switched Piezoelectric Materials. J. Intell. Mater. Syst. Struct. 2000, 11, 263–271. [Google Scholar] [CrossRef]
- Tsushima, N.; Su, W.; Introduction, I.; Member, S.; Member, S. Highly Flexible Piezoelectric Multifunctional Wings for. In Proceedings of the 58th AIAA/ASCE/AHS/ASC Structures, Structural Dynamics, and Materials Conference AIAA 2017-0624 Downloaded, Grapevine, TX, USA, 9–13 January 2017; pp. 1–15. [Google Scholar]
- Na, W.S.; Baek, J. Impedance-Based Non-Destructive Testing Method Combined with Unmanned Aerial Vehicle for Structural Health Monitoring of Civil Infrastructures. Appl. Sci. 2017, 7, 15. [Google Scholar] [CrossRef]
- Liu, T.; Li, C.; Wang, C.; Lai, J.W.; Cheong, K.H. A simple-fsdt-based isogeometric method for piezoelectric functionally graded plates. Mathematics 2020, 8, 1–24. [Google Scholar] [CrossRef]
- Song, X.; Tan, S.; Wang, E.; Wu, S.; Wu, Z. Active shape control of an antenna reflector using piezoelectric actuators. J. Intell. Mater. Syst. Struct. 2019, 30, 2733–2747. [Google Scholar] [CrossRef]
- Song, G.; Sethi, V.; Li, H.-N. Vibration control of civil structures using piezoceramic smart materials: A review. Eng. Struct. 2006, 28. [Google Scholar] [CrossRef]
- Preumont, A.; Voltan, M.; Sangiovanni, A.; Bastaits, R.; Mokrani, B.; Alaluf, D. An investigation of the active damping of suspension bridges. Adv. Inf. Knowl. Process. 2015, 3, 1–36. [Google Scholar] [CrossRef]
- Sethi, V.; Song, G. Optimal vibration control of a model frame structure using piezoceramic sensors and actuators. JVC/Journal Vib. Control 2005, 11. [Google Scholar] [CrossRef]
- Sethi, V.; Song, G. Multimode vibration control of a smart model frame structure. Smart Mater. Struct. 2006, 15, 473–479. [Google Scholar] [CrossRef]
- Sethi, V.; Song, G.; Franchek, M.A. Loop shaping control of a model-story building using smart materials. J. Intell. Mater. Syst. Struct. 2008, 19, 765–777. [Google Scholar] [CrossRef]
- Aridogan, U.; Basdogan, I. A review of active vibration and noise suppression of plate-like structures with piezoelectric transducers. J. Intell. Mater. Syst. Struct. 2015, 26, 1455–1476. [Google Scholar] [CrossRef]
- Search, H.; Journals, C.; Contact, A.; Iopscience, M.; Address, I.P.; Yang, A.M. Review on the use of piezoelectric materials for active vibration, noise, and flow control. Smart Mater. Struct. 2020, 29, 053001. [Google Scholar]
- Gripp, J.A.B.; Rade, D.A. Vibration and noise control using shunted piezoelectric transducers: A review. Mech. Syst. Signal Process. 2018, 112, 359–383. [Google Scholar] [CrossRef]
- Casadei, F.; Dozio, L.; Ruzzene, M.; Cunefare, K.A. Periodic shunted arrays for the control of noise radiation in an enclosure. J. Sound Vib. 2010, 329, 3632–3646. [Google Scholar] [CrossRef]
- Ang, L.Y.L.; Koh, Y.K.; Lee, H.P. Acoustic metamaterials: A potential for cabin noise control in automobiles and armored vehicles. Int. J. Appl. Mech. 2016, 8, 1–35. [Google Scholar] [CrossRef]
- Lai, S.C.; Mirshekarloo, M.S.; Yao, K. Effects of equivalent series resistance on the noise mitigation performance of piezoelectric shunt damping. Smart Mater. Struct. 2017, 26. [Google Scholar] [CrossRef]
- Salvador, C.S.; Abas, M.C.A.; Teresa, J.A.; Castillo, M.; Dimaano, K.; Velasco, C.L.; Sangalang, J. Development of a traffic noise energy harvesting standalone system using piezoelectric transducers and super-capacitor. In Proceedings of the 2017 25th International Conference on Systems Engineering (ICSEng), Las Vegas, NV, USA, 22–24 August 2017; pp. 370–376. [Google Scholar]
- Araújo, A.L.; Madeira, J.F.A. Multiobjective optimization solutions for noise reduction in composite sandwich panels using active control. Compos. Struct. 2020, 247, 112440. [Google Scholar] [CrossRef]
- Al-Furjan, M.S.H.; Habibi, M.; Safarpour, H. Vibration Control of a Smart Shell Reinforced by Graphene Nanoplatelets. Int. J. Appl. Mech. 2020, 12. [Google Scholar] [CrossRef]
- Li, S.; Liu, S.; Yang, L. Active Control of Vibration and Noise of Energy Equipment. IOP Conf. Ser. Earth Environ. Sci. 2020, 446. [Google Scholar] [CrossRef]
- Kumamoto, M.; Kida, M.; Hirayama, R.; Kajikawa, Y.; Tani, T.; Kurumi, Y. Active noise control system for reducing MR noise. IEICE Trans. Fundam. Electron. Commun. Comput. Sci. 2011, E94, 1479–1486. [Google Scholar] [CrossRef]
- Timothy Zahl, “Car Body Cracked”. 2015. Available online: https://www.carid.com/articles/what-type-of-body-kit-material-should-i-choose.html (accessed on 5 April 2021).
- Fesharaki, J.J.; Madani, S.G.; Golabi, S. Effect of stiffness and thickness ratio of host plate and piezoelectric patches on reduction of the stress concentration factor. Int. J. Adv. Struct. Eng. 2016, 8, 229–242. [Google Scholar] [CrossRef]
- Ihn, J.; Chang, F. Pitch-catch Active Sensing Methods in Structural Health Monitoring for Aircraft Structures. Struct. Heal. Monit. 2008, 7, 5–15. [Google Scholar] [CrossRef]
- Rogers, C.A. Intelligent Material Systems—The Dawn of a New Materials Age. J. Intell. Mater. Syst. Struct. 1993, 4, 4–12. [Google Scholar] [CrossRef]
- Kessler, S.S.; Johnson, C.E.; Dunn, C.T. Experimental Application of Optimized Lamb Wave Actuating/Sensing Patches for Health Monitoring of Composite Structures. In Proceedings of the 4th International Workshop, Stanford University, Stanford, CA, USA, 31 August–2 September 2003. [Google Scholar]
- Kessler, S.S.; Spearing, S.M.; Soutis, C. Damage Detection in Composite Materials Using Lamb Wave Methods. Smart Mater. {…}. 2002. Available online: http://iopscience.iop.org/0964-1726/11/2/310 (accessed on 5 April 2021).
- Bös, J.; Mayer, D. Comparison of various active vibration and noise reduction approaches applied to a planar test structure. In Proceedings of the 13th International Congress on Sound and Vibration, Wien, Österreich, 2–6 July 2006. [Google Scholar]
- Vergé, M.; Mechbal, N.; Coffignal, G. Active control of structures applied to an adaptable structure|Contrôle actif des structures appliqué à une structure souple. J. Eur. Syst. Autom. 2003, 37. [Google Scholar] [CrossRef]
- Song, G.; Qiao, P.Z.; Binienda, W.K.; Zou, G.P. Active vibration damping of composite beam using smart sensors and actuators. J. Aerosp. Eng. 2002, 15, 97–103. [Google Scholar] [CrossRef]
- Wu, N. Structural Repair using Smart Materials. J. Aeronaut. Aerosp. Eng. 2012, 1, 1–2. [Google Scholar] [CrossRef]
- Rao, U.K.; Bangaru Babu, P.; Nagaraju, C. Active Repair of Engineering Structures Using Piezoelectric Patches. In Proceedings of the 17th ISME Conference ISME17, IIT Delhi, New Delhi, India, 3–4 October 2015; pp. 1–5. [Google Scholar]
- Cheng, J.; Taheri, F. A novel smart adhesively bonded joint system. Smart Mater. Struct. 2005, 14, 971–981. [Google Scholar] [CrossRef]
- Shaik Dawood, M.S.I.; Iannucci, L.; Greenhalgh, E.; Ariffin, A.K. Low Velocity Impact Induced Delamination Control Using MFC Actuator. Appl. Mech. Mater. 2012, 165, 346–351. [Google Scholar] [CrossRef]
- Sohn, H.; Park, G.; Wait, J.R.; Limback, N.P.; Farrar, C.R. Wavelet-based active sensing for delamination detection in composite structures. Smart Mater. Struct. 2003, 13, 153–160. [Google Scholar] [CrossRef]
- Liu, T.J.C. Fracture mechanics and crack contact analyses of the active repair of multi-layered piezoelectric patches bonded on cracked structures. Theor. Appl. Fract. Mech. 2007, 47, 120–132. [Google Scholar] [CrossRef]
- Duan, W.H.; Quek, S.T.; Wang, Q. Finite element analysis of the piezoelectric-based repair of a delaminated beam. Smart Mater. Struct. 2008, 17, 015017. [Google Scholar] [CrossRef]
- Alaimo, A.; Milazzo, A.; Orlando, C. Piezoelectric Patches for the Active Repair of Delaminated Structures. J. Aerosp. Sci. Technol. Syst. 2011, 22, 2137–2146. [Google Scholar]
- Alaimo, A.; Milazzo, A.; Orlando, C.; Messineo, A. Numerical analysis of piezoelectric active repair in the presence of frictional contact conditions. Sensors 2013, 13, 4390–4403. [Google Scholar] [CrossRef]
- Wu, N.; Wang, Q. Repair of a delaminated plate under static loading with piezoelectric patches. Smart Mater. Struct. 2010, 19, 105025. [Google Scholar] [CrossRef]
- Muthu, N.; Maiti, S.K.; Falzon, B.G.; Yan, W. Crack propagation in non-homogenous materials: Evaluation of mixed-mode SIFs, T-stress and kinking angle using a variant of EFG Method. Eng. Anal. Bound. Elem. 2016, 72, 11–26. [Google Scholar] [CrossRef]
- Barsoum, R.S. Triangular Quarter Point Elements as Elastic and Perfectly Plastic Crack Tip Elements. Int. J. Numer. Meth. Engng. 1977, 11, 85. [Google Scholar] [CrossRef]
- Dally, J.W.; Sanford, R.J. Strain-gage methods for measuring the opening-mode stress-intensity factor, KI. Exp. Mech. 1987, 27, 381–388. [Google Scholar] [CrossRef]
- Crawley, E.F.; De Luis, J. Use of piezoelectric actuators as elements of intelligent structures. AIAA J. 1987, 25, 1373–1385. [Google Scholar] [CrossRef]
- Isaksson, P.; Hägglund, R. Crack-tip fields in gradient enhanced elasticity. Eng. Fract. Mech. 2013, 97, 186–192. [Google Scholar] [CrossRef]
- Wang, Q.S. Active buckling control of beams using piezoelectric actuators and strain gauge sensors. Smart Mater. Struct. 2010, 19, 065022. [Google Scholar] [CrossRef]
- Abuzaid, A.; Hrairi, M.; Dawood, M.S.I. Survey of Active Structural Control and Repair Using Piezoelectric Patches. Actuators 2015, 4, 77–98. [Google Scholar] [CrossRef]
- Abuzaid, A.; Hrairi, M.; Dawood, M.S. Mode I Stress Intensity Factor for a Cracked Plate with an Integrated Piezoelectric Actuator. Adv. Mater. Res. 2015, 1115, 517–522. [Google Scholar] [CrossRef]
- Abuzaid, A.; Hrairi, M.; Dawood, M. Evaluating the Reduction of Stress Intensity Factor in Center-Cracked Plates Using Piezoelectric Actuators. Actuators 2018, 7, 25. [Google Scholar] [CrossRef]
- Aabid, A.; Hrairi, M.; Ali, J.S.M.; Abuzaid, A. Stress Concentration Analysis of a Composite Patch on a Hole in an Isotropic Plate. Int. J. Mech. Prod. Eng. Res. Dev. 2018, 6, 249–255. [Google Scholar]
- Abuzaid, A.; Shaik Dawood, M.S.I.; Hrairi, M. The effect of piezoelectric actuation on stress distribution in aluminum plate with circular hole. ARPN J. Eng. Appl. Sci. 2015, 10, 9723–9729. [Google Scholar]
- Abuzaid, A.; Hrairi, M.; Dawood, M.S. Modeling approach to evaluating reduction in stress intensity factor in center-cracked plate with piezoelectric actuator patches. J. Intell. Mater. Syst. Struct. 2017, 28, 1334–1345. [Google Scholar] [CrossRef]
- Abuzaid, A.; Hrairi, M. Experimental and numerical analysis of piezoelectric active repair of edge-cracked plate. J. Intell. Mater. Syst. Struct. 2018, 29, 3656–3666. [Google Scholar] [CrossRef]
- Abuzaid, A.; Dawood, M.S.; Hrairi, M. Effects of Adhesive Bond on Active Repair of Aluminium Plate Using Piezoelectric Patch. Appl. Mech. Mater. 2015, 799–800, 788–793. [Google Scholar] [CrossRef]
- Aabid, A.; Hrairi, M.; Abuzaid, A.; Mohamed Ali, J.S. Estimation of stress intensity factor reduction for a center-cracked plate integrated with piezoelectric actuator and composite patch. Thin-Walled Struct. 2021, 158. [Google Scholar] [CrossRef]
- Aabid, A.; Hrairi, M.; Dawood, M.S.I.S. Modeling Different Repair Configurations of an Aluminum Plate with a Hole. Int. J. Recent Technol. Eng. 2019, 7, 235–240. [Google Scholar]
- Kang, K.; Chun, H.; Lee, J.A.; Byun, J.; Um, M.; Lee, S. Damage Detection of Composite Plates Using Finite Element Analysis Based on Structural Health Monitoring. J. Mater. Sci. Eng. 2011, 1, 14–21. [Google Scholar]
- Martinez-Luengo, M.; Kolios, A.; Wang, L. Structural health monitoring of offshore wind turbines: A review through the Statistical Pattern Recognition Paradigm. Renew. Sustain. Energy Rev. 2016, 64, 91–105. [Google Scholar] [CrossRef]
- Güemes, A.; Fernandez-Lopez, A.; Pozo, A.R.; Sierra-Pérez, J. Structural health monitoring for advanced composite structures: A review. J. Compos. Sci. 2020, 4, 15. [Google Scholar] [CrossRef]
- Miorelli, R.; Kulakovskyi, A.; Chapuis, B.; D’Almeida, O.; Mesnil, O. Supervised learning strategy for classification and regression tasks applied to aeronautical structural health monitoring problems. Ultrasonics 2021, 113, 106372. [Google Scholar] [CrossRef] [PubMed]
- Memmolo, V.; Ricci, F.; Boffa, N.D.; Maio, L.; Monaco, E. Structural Health Monitoring in Composites Based on Probabilistic Reconstruction Techniques. Procedia Eng. 2016, 167, 48–55. [Google Scholar] [CrossRef]
- Anjum, A.; Syed, J.; Ali, M.; Zayan, J.M.; Aabid, A. Statistical Analysis of Adhesive Bond Parameters in a Single Lap Joint System. J. Mod. Mech. Eng. Technol. 2020, 7, 53–58. [Google Scholar]
- Maio, L.; Memmolo, V.; Boccardi, S.; Meola, C.; Ricci, F.; Boffa, N.D.; Monaco, E. Ultrasonic and IR Thermographic Detection of a Defect in a Multilayered Composite Plate. Procedia Eng. 2016, 167, 71–79. [Google Scholar] [CrossRef]
- Cot, L.D.; Wang, Y.; Bès, C.; Gogu, C. Scheduled and SHM structural airframe maintenance applications using a new probabilistic model. In Proceedings of the 17th European Workshop on Structural Health Monitoring (EWSHM 2014), Nantes, France, 8–11 July 2014; pp. 2306–2313. [Google Scholar]
- Su, Z.; Ye, L.; Lu, Y. Guided Lamb waves for identification of damage in composite structures: A review. J. Sound Vib. 2006, 295, 753–780. [Google Scholar] [CrossRef]
- Mitra, M.; Gopalakrishnan, S. Guided wave based structural health monitoring: A review. Smart Mater. Struct. 2016, 25. [Google Scholar] [CrossRef]
- Memmolo, V.; Pasquino, N.; Ricci, F. Experimental characterization of a damage detection and localization system for composite structures. Meas. J. Int. Meas. Confed. 2018, 129, 381–388. [Google Scholar] [CrossRef]
- Cawley, P. Structural health monitoring: Closing the gap between research and industrial deployment. Struct. Heal. Monit. 2018, 17, 1225–1244. [Google Scholar] [CrossRef]
- Memmolo, V.; Monaco, E.; Boffa, N.D.; Maio, L.; Ricci, F. Guided wave propagation and scattering for structural health monitoring of stiffened composites. Compos. Struct. 2018, 184, 568–580. [Google Scholar] [CrossRef]
- Salmanpour, M.S.; Sharif Khodaei, Z.; Aliabadi, M.H. Guided wave temperature correction methods in structural health monitoring. J. Intell. Mater. Syst. Struct. 2017, 28, 604–618. [Google Scholar] [CrossRef]
- Miniaci, M.; Mazzotti, M.; Radzieński, M.; Kudela, P.; Kherraz, N.; Bosia, F.; Pugno, N.M.; Ostachowicz, W. Application of a laser-based time reversal algorithm for impact localization in a stiffened aluminum plate. Front. Mater. 2019, 6, 1–12. [Google Scholar] [CrossRef]
- Cai, J.; Qiu, L.; Yuan, S.; Shi, S.; Liu, P.; Liang, D. Structural health monitoring for composite materials. In Composites and Their Applications; Hu, N., Ed.; IntechOpen: London, UK, 2012; pp. 37–58. ISBN 978-953-51-0706-4. [Google Scholar]
- Hai, B.S.M.E.; Bause, M.; Kuberry, P. Finite element approximation of the extended fluid-structure interaction (eXFSI) problem. Am. Soc. Mech. Eng. Fluids Eng. Div. FEDSM 2016, 1A-2016. [Google Scholar] [CrossRef]
- Kessler, S.; Mark, S.; Seth, S. Structural Health Monitoring in Composite Materials Using Lamb Wave Methods Structural Health Monitoring in Composite Materials Using Lamb Wave Methods. ASC 2001, 43, 1–4. [Google Scholar]
- Duan, W.H.; Wang, Q.; Quek, S.T. Applications of piezoelectric materials in structural health monitoring and repair: Selected research examples. Materials 2010, 3, 5169–5194. [Google Scholar] [CrossRef] [PubMed]
- Zhou, B.; Ma, X.; Wang, S.; Xue, S. Least-squares method for laminated beams with distributed braided piezoelectric composite actuators. J. Intell. Mater. Syst. Struct. 2020, 31, 2165–2176. [Google Scholar] [CrossRef]
- Porchez, T.; Bencheikh, N.; Claeyssen, F. Piezo-Composite Patches Applied to the Detection of Defects Using Lamb Wave Focusing; Cedrat Technologies: Grenoble, France, 2013. [Google Scholar]
- Shanker, R. An Integrated Approach for Structural Health Monitoring Rama Shanker. Ph.D. Thesis, Indian Institute of Technology Delhi, New Delhi, India, 2009. [Google Scholar]
- Chen, C.-D.; Chiu, Y.-C.; Huang, Y.-H.; Wang, P.-H.; Chien, R.-D. Assessments of Structural Health Monitoring for Fatigue Cracks in Metallic Structures by Using Lamb Waves Driven by Piezoelectric Transducers. J. Aerosp. Eng. 2021, 34, 04020091. [Google Scholar] [CrossRef]
- Venu, A.; Madhav, G.; John, P.; Lye, H.; Youxiang, C.; Jen, H.O.H.H.; Kun, Z.; Bin, S. Fatigue Monitoring of double surface defects using PZT based Electromechanical Impedance and Digital image correlation methods. Adv. Mater. Res. Vols. 2014, 892, 551–556. [Google Scholar]
- Qing, X.; Li, W.; Wang, Y.; Sun, H. Piezoelectric transducer-based structural health monitoring for aircraft applications. Sensors 2019, 19, 1–27. [Google Scholar] [CrossRef] [PubMed]
- Mewer, R.C. Analysis and Structural Health Monitoring of Composite Plates with Piezoelectric Sensors and Actuators. Master’s Thesis, The University of Maine, Orono, ME, USA, 2003. [Google Scholar]
- Bergmayr, T.; Kralovec, C.; Schagerl, M. Vibration-based thermal health monitoring for face layer debonding detection in aerospace sandwich structures. Appl. Sci. 2021, 11, 1–15. [Google Scholar]
- Wang, Y.; Qiu, L.; Luo, Y.; Ding, R.; Jiang, F. A piezoelectric sensor network with shared signal transmission wires for structural health monitoring of aircraft smart skin. Mech. Syst. Signal Process. 2020, 141, 106730. [Google Scholar] [CrossRef]
- Rocha, H.; Semprimoschnig, C.; Nunes, J.P. Sensors for process and structural health monitoring of aerospace composites: A review. Eng. Struct. 2021, 237, 112231. [Google Scholar] [CrossRef]
- Ferri Aliabadi, M.H.; Khodaei, Z.S. Structural health monitoring for advanced composite structures. Struct. Heal. Monit. Adv. Compos. Struct. 2017, 1–274. [Google Scholar] [CrossRef]
- Giurgiutiu, V.; Zagrai, A.; Jing, B.J. Piezoelectric Wafer Embedded Active Sensors for Aging Aircraft Structural Health Monitoring. Struct. Heal. Monit. 2002, 1, 41–61. [Google Scholar] [CrossRef]
- Aeronautics, S.M. Piezoelectric-Based In-Situ Damage Detection of Composite Materials for Structural Health Monitoring Systems. Ph.D Thesis, Massachusetts Institute of Technology, Cambridge, MA, USA, 2002. [Google Scholar]
- Boukabache, H.; Escriba, C.; Fourniols, J.Y. Toward smart aerospace structures: Design of a piezoelectric sensor and its analog interface for flaw detection. Sensors 2014, 14, 20543–20561. [Google Scholar] [CrossRef]
- Xu, Y.G.; Liu, G.R. A modified electro-mechanical impedance model of piezoelectric actuator-sensors for debonding detection of composite patches. J. Intell. Mater. Syst. Struct. 2002, 13, 389–396. [Google Scholar] [CrossRef]
- Yang, Y.; Annamdas, V.G.M.; Wang, C.; Zhou, Y. Application of multiplexed FBG and PZT impedance sensors for health monitoring of rocks. Sensors 2008, 8, 271–289. [Google Scholar] [CrossRef]
- Annamdas, V.G.M.; Yang, Y.; Soh, C.K. Influence of loading on the electromechanical admittance of piezoceramic transducers. Smart Mater. Struct. 2007, 16, 1888–1897. [Google Scholar] [CrossRef]
- Yang, Y.; Lim, Y.Y.; Soh, C.K. Practical issues related to the application of the electromechanical impedance technique in the structural health monitoring of civil structures: I. Experiment. Smart Mater. Struct. 2008, 17. [Google Scholar] [CrossRef]
- Yang, Y.; Hu, Y.; Lu, Y. Sensitivity of PZT impedance sensors for damage detection of concrete structures. Sensors 2008, 8, 327–346. [Google Scholar] [CrossRef]
- Xu, B.; Jiang, F. Concrete-steel composite girder bolt loosening monitoring using electromechanical impedance measurements. In Earth and Space 2012: Engineering, Science, Construction, and Operations in Challenging Environments; American Society of Civil Engineers: Reston, VA, USA, 2012; pp. 629–634. [Google Scholar]
- Hu, X.; Zhu, H.; Wang, D. A study of concrete slab damage detection based on the electromechanical impedance method. Sensors 2014, 14, 19897–19909. [Google Scholar] [CrossRef]
- Gu, H.; Song, G.; Dhonde, H.; Mo, Y.L.; Yan, S. Concrete early-age strength monitoring using embedded piezoelectric transducers. Smart Mater. Struct. 2006, 15, 1837–1845. [Google Scholar] [CrossRef]
- Ai, D.; Luo, H.; Wang, C.; Zhu, H. Monitoring of the load-induced RC beam structural tension / compression stress and damage using piezoelectric transducers. Eng. Struct. 2018, 154, 38–51. [Google Scholar] [CrossRef]
- Song, G.; Gu, H.; Mo, Y.L.; Hsu, T.T.C.; Dhonde, H. Concrete structural health monitoring using embedded piezoceramic transducers. Smart Mater. Struct. 2015, 16, 959–968. [Google Scholar] [CrossRef]
- Talakokula, V.; Bhalla, S.; Gupta, A. Corrosion assessment of reinforced concrete structures based on equivalent structural parameters using electro-mechanical impedance technique. Intell. Mater. Syst. Struct. 2014, 25, 484–500. [Google Scholar] [CrossRef]
- Na, W.S.; Baek, J. A Review of the Piezoelectric Electromechanical Impedance Based Structural Health Monitoring Technique for Engineering Structures. Sensors 2018, 18, 18. [Google Scholar] [CrossRef]
- Kim, H.; Liu, X.; Ahn, E.; Shin, M.; Shin, S.W.; Sim, S.H. Performance assessment method for crack repair in concrete using PZT-based electromechanical impedance technique. NDT E Int. 2019, 104, 90–97. [Google Scholar] [CrossRef]
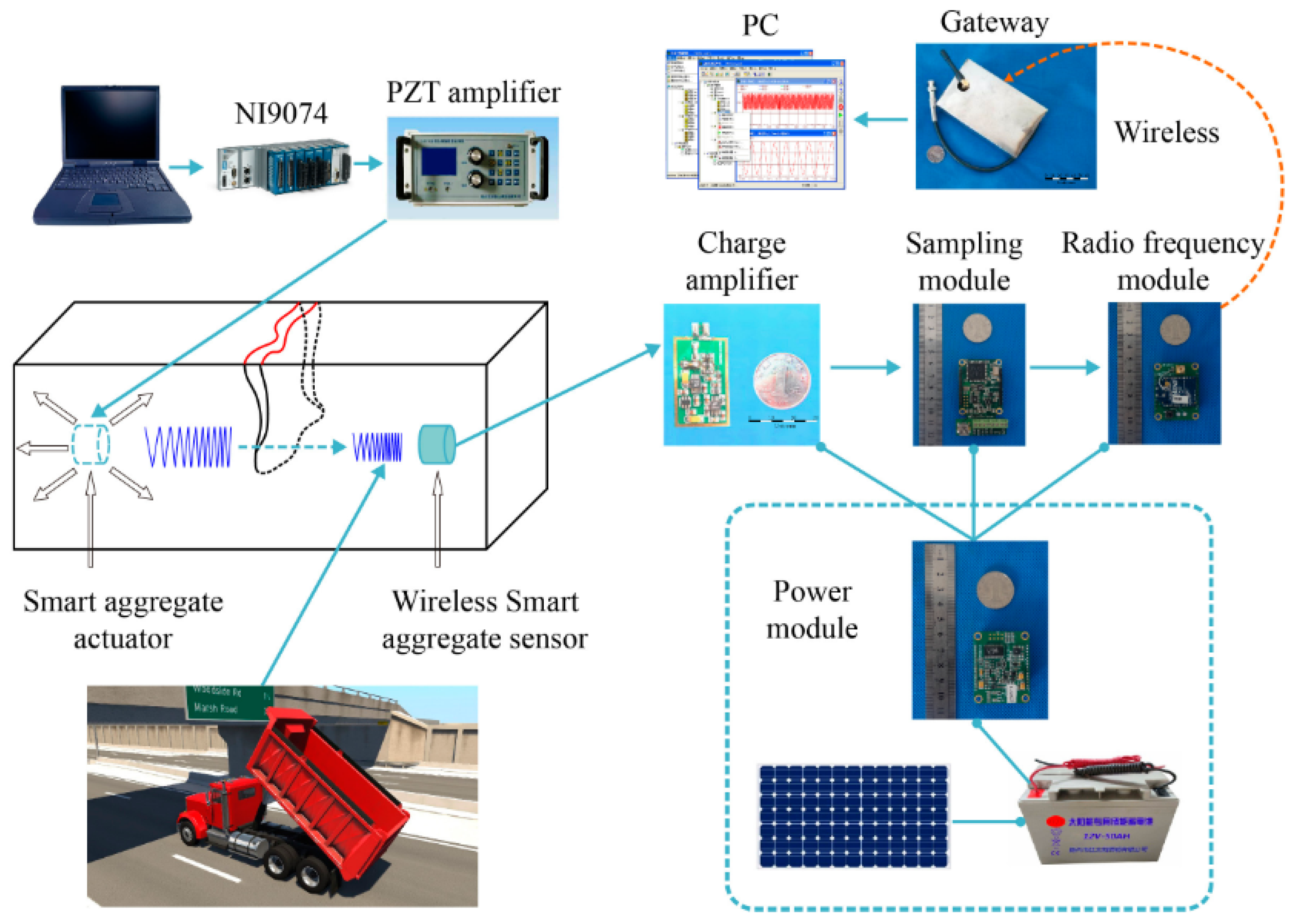
- Yan, S.; Ma, H.; Li, P.; Song, G.; Wu, J. Development and application of a structural health monitoring system based on wireless smart aggregates. Sensors 2017, 17, 1641. [Google Scholar] [CrossRef]
- Sun, F.P.; Chaudhry, Z.; Liang, C.; Rogers, C.A. Truss Structure Integrity Identification Using PZT Sensor-Actuator. J. Intell. Mater. Syst. Struct. 1995, 6, 134–139. [Google Scholar] [CrossRef]
- Park, G.; Kabeya, K.; Cudney, H.H.; Inman, D.J. Impednce-Based Structural Health Monitoring for Temperature Varying Applications. Chem. Pharm. Bull. 1992, 40, 1569–1572. [Google Scholar]
- Grisso, B.L.; Inman, D.J. Temperature corrected sensor diagnostics for impedance-based SHM. J. Sound Vib. 2010, 329, 2323–2336. [Google Scholar] [CrossRef]
- Wandowski, T.; Malinowski, P.H.; Ostachowicz, W.M. Temperature and damage influence on electromechanical impedance method used for carbon fibre-reinforced polymer panels. J. Intell. Mater. Syst. Struct. 2017, 28, 782–798. [Google Scholar] [CrossRef]
- Na, W.S.; Lee, H. Experimental investigation for an isolation technique on conducting the electromechanical impedance method in high-temperature pipeline facilities. J. Sound Vib. 2016, 383, 210–220. [Google Scholar] [CrossRef]
- Park, G.; Inman, D.J. Structural health monitoring using piezoelectric impedance measurements. Philos. Trans. R. Soc. A Math. Phys. Eng. Sci. 2007, 365, 373–392. [Google Scholar] [CrossRef]
- Jiao, P.; Egbe, K.J.I.; Xie, Y.; Nazar, A.M.; Alavi, A.H. Piezoelectric sensing techniques in structural health monitoring: A state-of-the-art review. Sensors 2020, 20, 1–21. [Google Scholar] [CrossRef] [PubMed]
- Spencer, B.F.; Ruiz-Sandoval, M.E.; Kurata, N. Smart sensing technology: Opportunities and challenges. Struct. Control Heal. Monit. 2004, 11, 349–368. [Google Scholar] [CrossRef]
- Cusson, D.; Daigle, L. Continuous Condition Assessment of Highway Bridges Using Field Monitoring NRCC-50863; INFRA: Quebec City, QC, Canada, 2008; p. 14. [Google Scholar]
- Albedah, A.; Khan, S.M.A.; Bouiadjra, B.B. Fatigue crack propagation in aluminum plates with composite patch including plasticity effect. Proc. Inst. Mech. Eng. Part G 2018, 232, 2122–2131. [Google Scholar] [CrossRef]
- Oudad, W.; Belhadri, D.E.; Fekirini, H.; Khodja, M. Analysis of the Plastic Zone under Mixed Mode Fracture in Bonded Composite Repair of Aircraft Structures. Aerosp. Sci. Technol. 2017. [Google Scholar] [CrossRef]
- Hu, D.; Yang, Q.; Liu, H.; Mao, J.; Meng, F. Crack closure effect and crack growth behavior in GH2036 superalloy plates under combined high and low cycle fatigue. Int. J. Fatigue 2016. [Google Scholar] [CrossRef]
- Daryabor, P.; Safizadeh, M.S. Investigation of defect characteristics and heat transfer in step heating thermography of metal plates repaired with composite patches. Infrared Phys. Technol. 2016, 76, 608–620. [Google Scholar] [CrossRef]



| Type of Structure | Technique Adopted | Number of Piezoelectric | Focused Parameters | Remark | Reference |
|---|---|---|---|---|---|
| Simply supported and cantilever beam | Multi-domain boundary integral formulation and spring model | 2 (single and multi-layered) | Displacements, electric potential, and friction coefficient | Friction contact does not affect the repairing mechanism | [78] |
| Graphite/epoxy plate | wavelet-based signal processing | 16 | Piezoelectric patches and delamination size | Simple and needs minimum interaction | [74] |
| Cantilever and simply supported beam | Euler–Bernoulli beam theory and numerical simulation | 2 | Delamination locations, shear stress, and voltage | Piezoelectric materials are capable of repair of delaminated beam | [4] |
| Cantilever model | FE analysis using ANSYS | 2 (single and multi-layered) | Crack location and length, repair voltage, patch thickness, and length | FEM analysis allows a detailed understanding of active repairs as well | [75] |
| Beam | FEM analysis using ABAQUS | 2 (top and bottom) | Repair voltage, repair index, | The repair index depends upon the delamination location | [76] |
| Cantilever beam | Multi-domain boundary integral formulation and spring model | 2 (single and multi-layered) | Shear stress, peal stress, crack displacement | The piezoelectric patch actuation capacity decreases due to shear stress transfer at the interface of structure and crack | [6] |
| Composite (drop ply) and cantilever beam | Springle model and multi-domain boundary integral formulation | 2 (single and multi-layered) | Total ERR distribution, normal and tangential crack surface displacements | The optimal position for the patch is on the top of the skin, which eliminates crack opening. | [77] |
| Rectangular plate | Stiffness ratio and induce strain | 2 (top/bottom)2 (left/right) around a circular hole | Reduction of stress concentration factor | SIF | [62] |
| Aluminum alloy 2024-T3 and 7075-T6 plate | J-integral using the FE method | 1 composite patch (carbon/epoxy) | Crack propagation | Parametric study and SIF with experimentation give more ideas | [152] |
| Aluminum 2024-T3 plate | Von-mises stress, J-integral using FE analysis | 1 compsite patch (boron/epoxy) | Fatigue life | SIF | [153] |
| GH2036 superalloy (novel model) | low and high cycle fatigue loading | No patch | crack closure and behavior of growth | Reproduce with bonded composite patches and piezoelectric actuators | [154] |
| Aluminum plate | Step heating thermography with FE modeling | 1 (Composite patch) | delamination and disbond) with thermal heat transfer | SIF | [155] |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Aabid, A.; Parveez, B.; Raheman, M.A.; Ibrahim, Y.E.; Anjum, A.; Hrairi, M.; Parveen, N.; Mohammed Zayan, J. A Review of Piezoelectric Material-Based Structural Control and Health Monitoring Techniques for Engineering Structures: Challenges and Opportunities. Actuators 2021, 10, 101. https://doi.org/10.3390/act10050101
Aabid A, Parveez B, Raheman MA, Ibrahim YE, Anjum A, Hrairi M, Parveen N, Mohammed Zayan J. A Review of Piezoelectric Material-Based Structural Control and Health Monitoring Techniques for Engineering Structures: Challenges and Opportunities. Actuators. 2021; 10(5):101. https://doi.org/10.3390/act10050101
Chicago/Turabian StyleAabid, Abdul, Bisma Parveez, Md Abdul Raheman, Yasser E. Ibrahim, Asraar Anjum, Meftah Hrairi, Nagma Parveen, and Jalal Mohammed Zayan. 2021. "A Review of Piezoelectric Material-Based Structural Control and Health Monitoring Techniques for Engineering Structures: Challenges and Opportunities" Actuators 10, no. 5: 101. https://doi.org/10.3390/act10050101
APA StyleAabid, A., Parveez, B., Raheman, M. A., Ibrahim, Y. E., Anjum, A., Hrairi, M., Parveen, N., & Mohammed Zayan, J. (2021). A Review of Piezoelectric Material-Based Structural Control and Health Monitoring Techniques for Engineering Structures: Challenges and Opportunities. Actuators, 10(5), 101. https://doi.org/10.3390/act10050101









