Back Electro Motive Force Estimation Method for Cascade Proportional Integral Control in Permanent Magnet Synchronous Motors
Abstract
:1. Introduction
2. Mathematical Modeling and Controller Design
2.1. PMSM Modeling
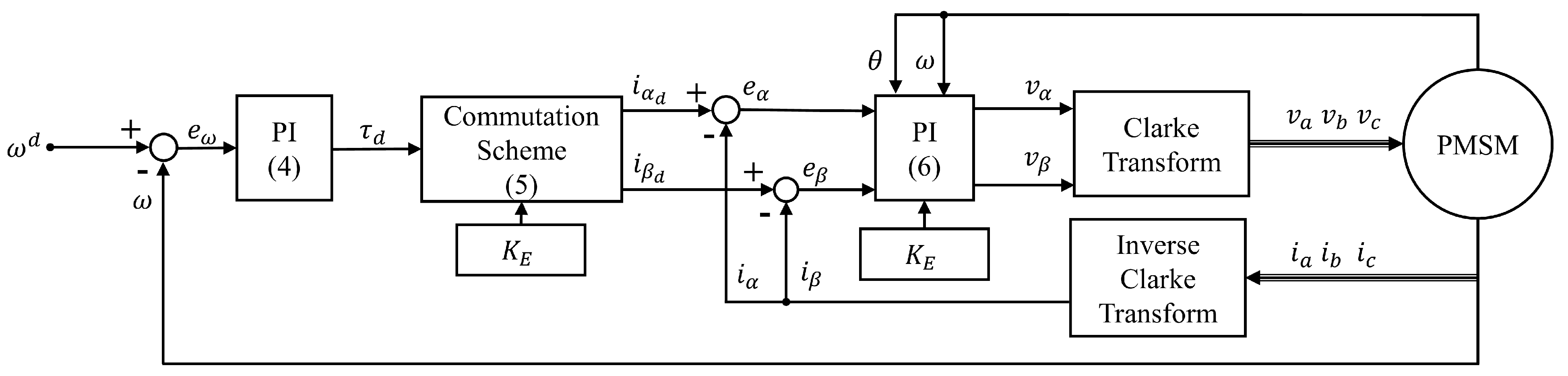
2.2. PI Controller with Back-EMF Compensation
3. Design and Analysis of Back-EMF Constant Estimator
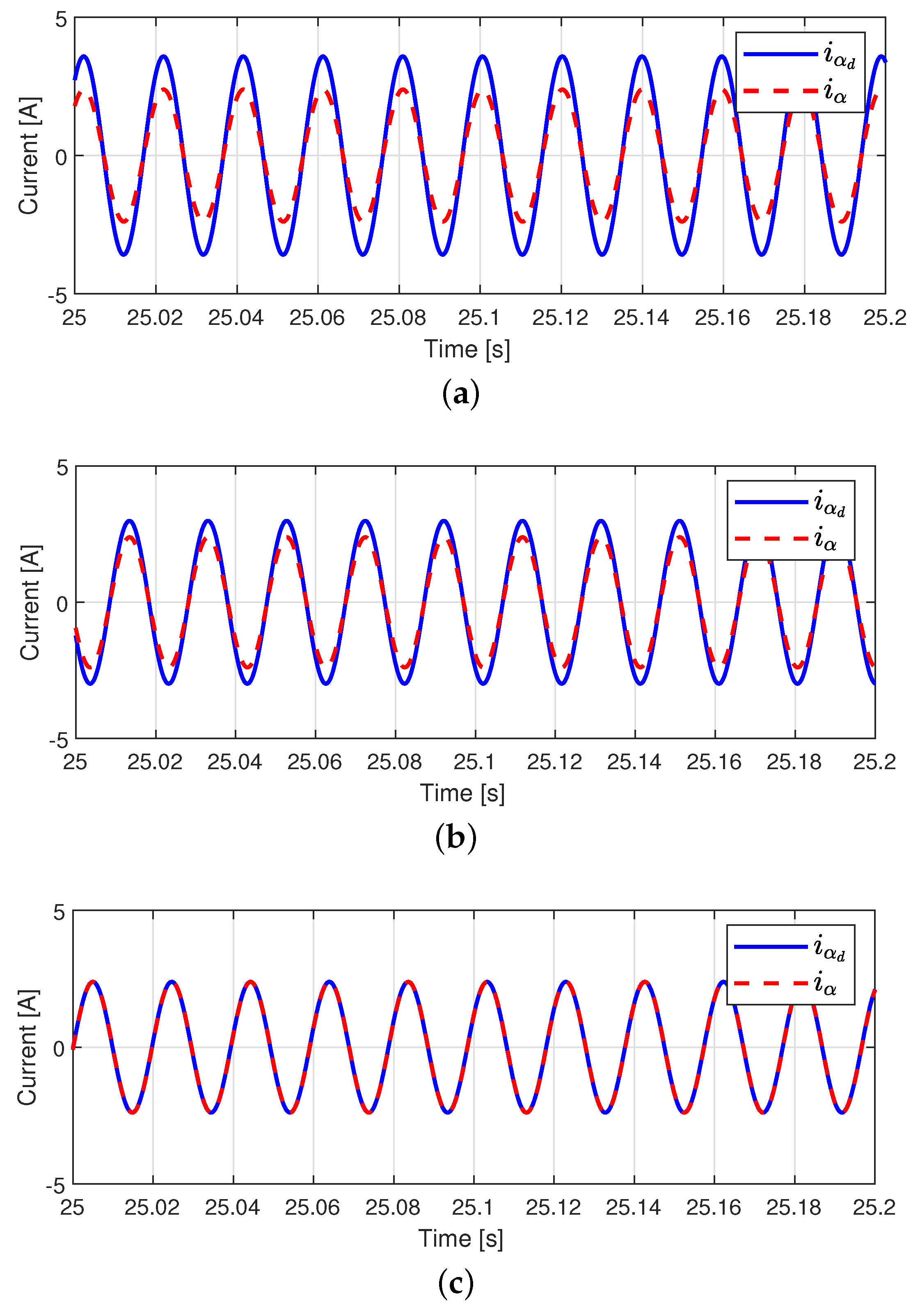
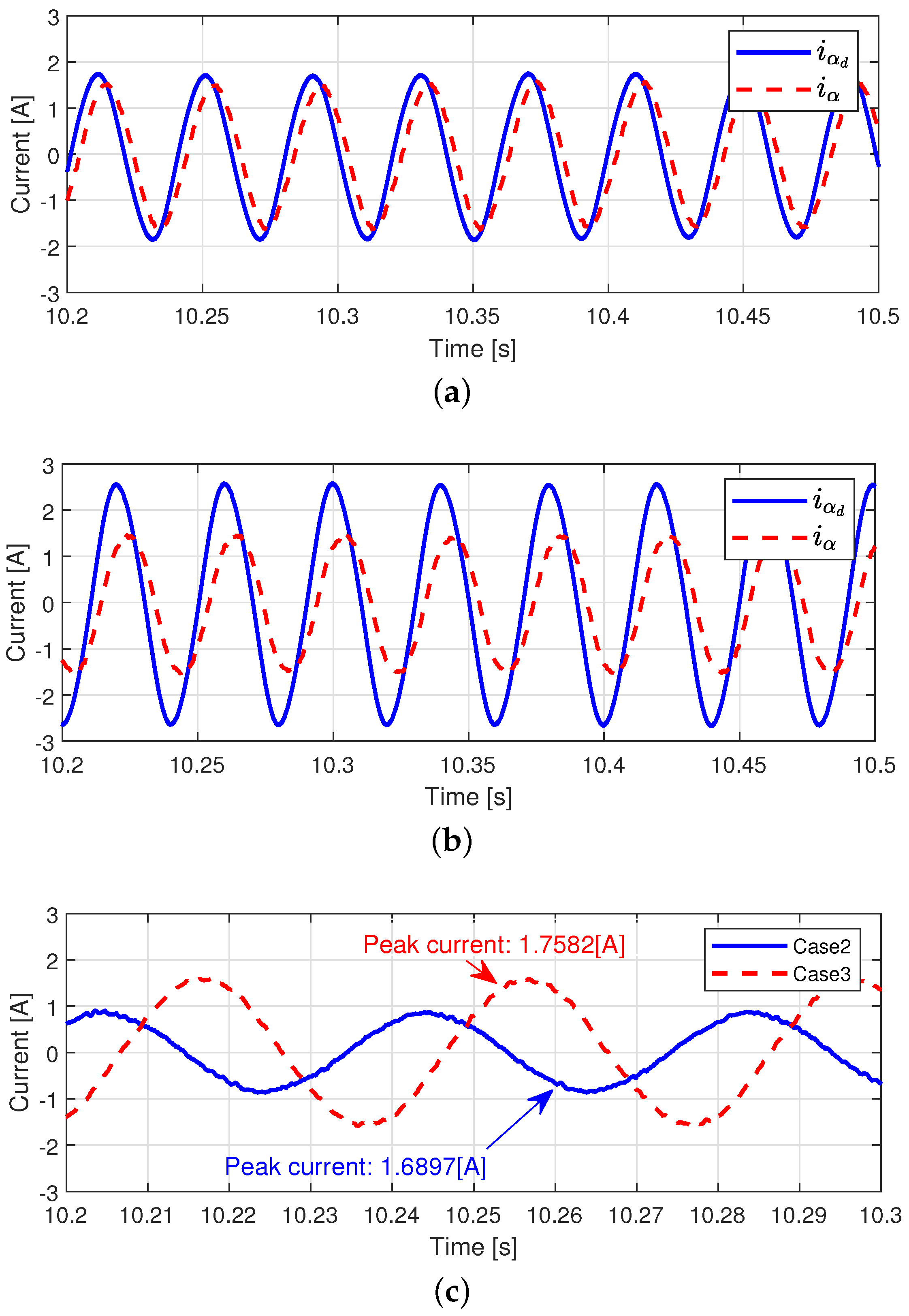
3.1. Performance Analysis of Back-EMF Compensation
- Case S.1: Cascade PI control without back-EMF compensation
- Case S.2: Cascade PI control with back-EMF compensation by 50% of the back-EMFswhere
- Case S.3: Cascade PI control with back-EMF compensation
3.2. Back-EMF Constant Estimator Design
3.3. Tuning Guideline
- Step 1: μ selectionSince operating frequency and electrical parameters are invariable in constant velocity range, estimation performance will be decided by choosing and . By (12), homogenous solution of differential equation can be represented aswhere t goes to infinity. is initial value of . .If magnitude of is relatively large, then is also large. So the convergence rate of is more faster. And if magnitude of is relatively small, then is also small. So the convergence rate of is more slower. Therefore, should be determined by the magnitude of the phase currents because there is a tradeoff relationship between and the phase currents.
- Step 2: selectionIn many industrial applications, it is common for measurement noise to be present in the signal measured from the sensor. Suppose that the measured angular velocity can be rewritten aswhere and are fundamental frequency and noise frequency obtained from the sensor, respectively. By this definition, the convergence rate, is redefined asIt means that high results in amplification of the measurement noise. Therefore, should be determined within a range that does not amplify the frequency of the measurement noise.
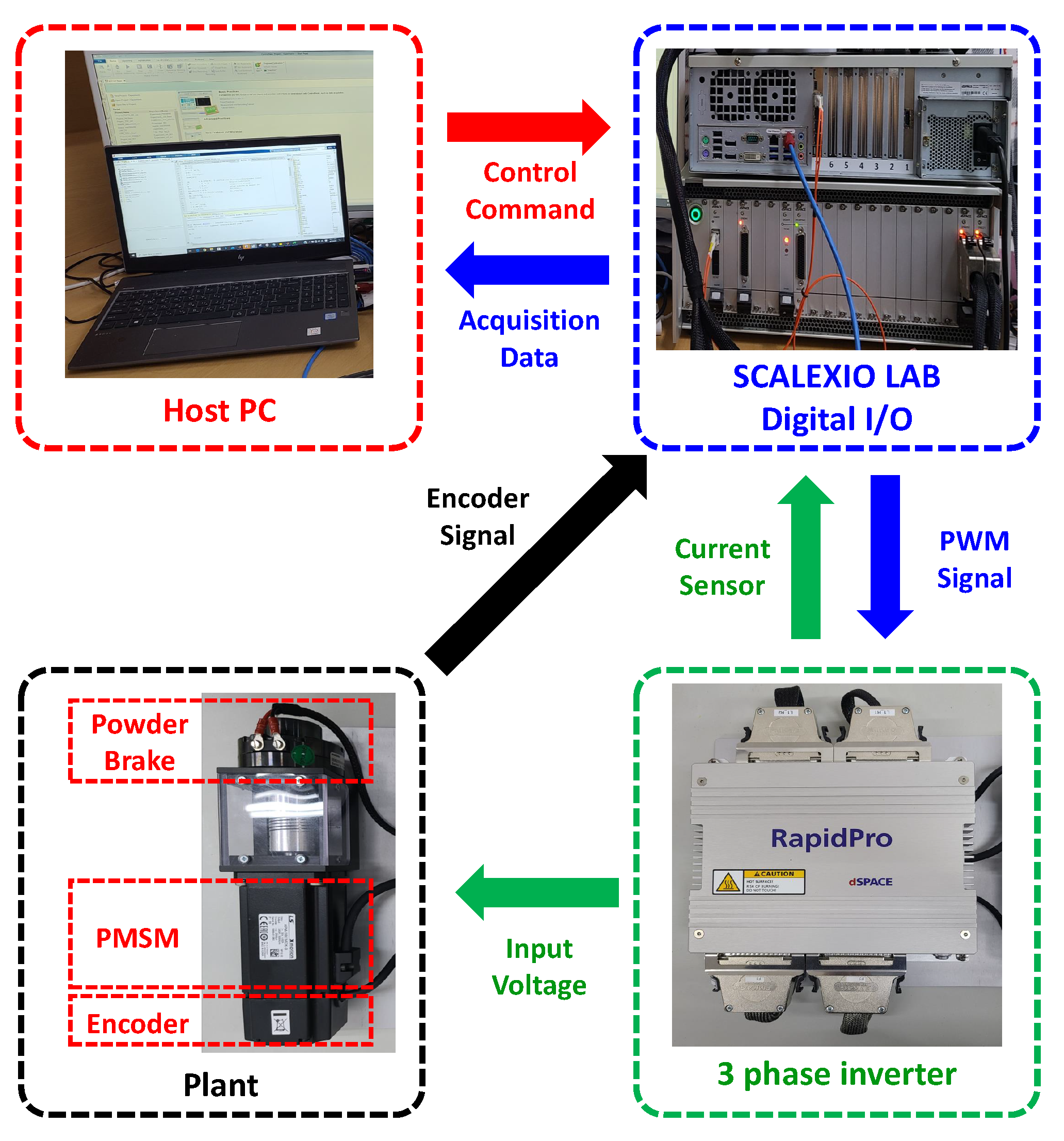
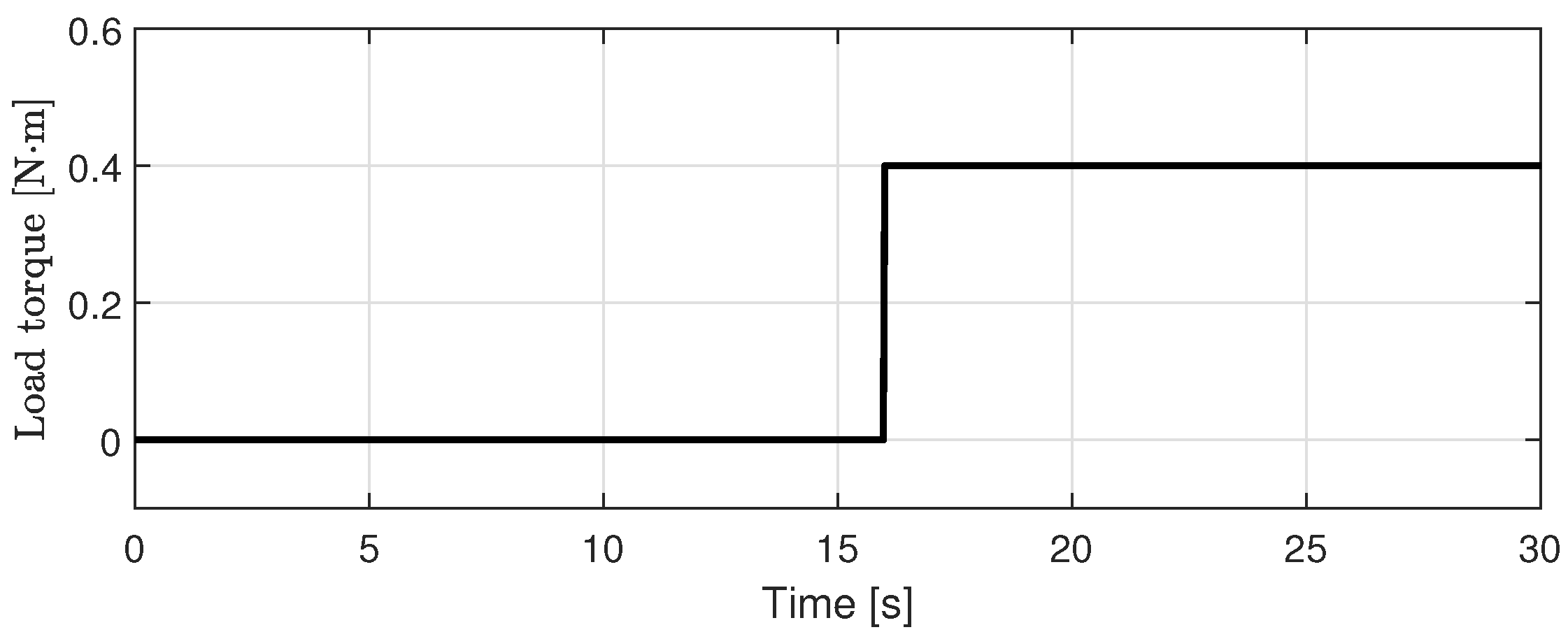
4. Experimental Results
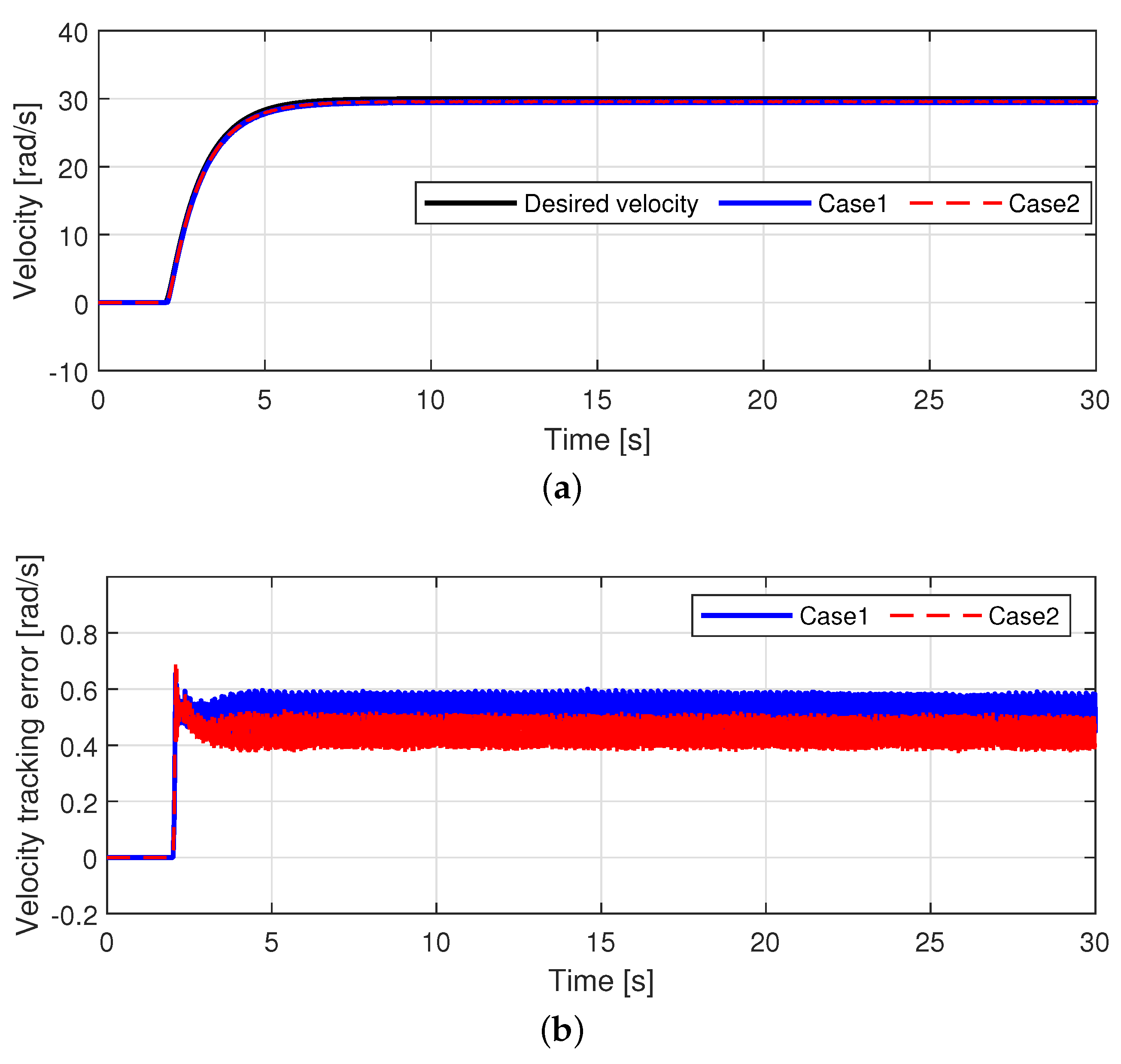
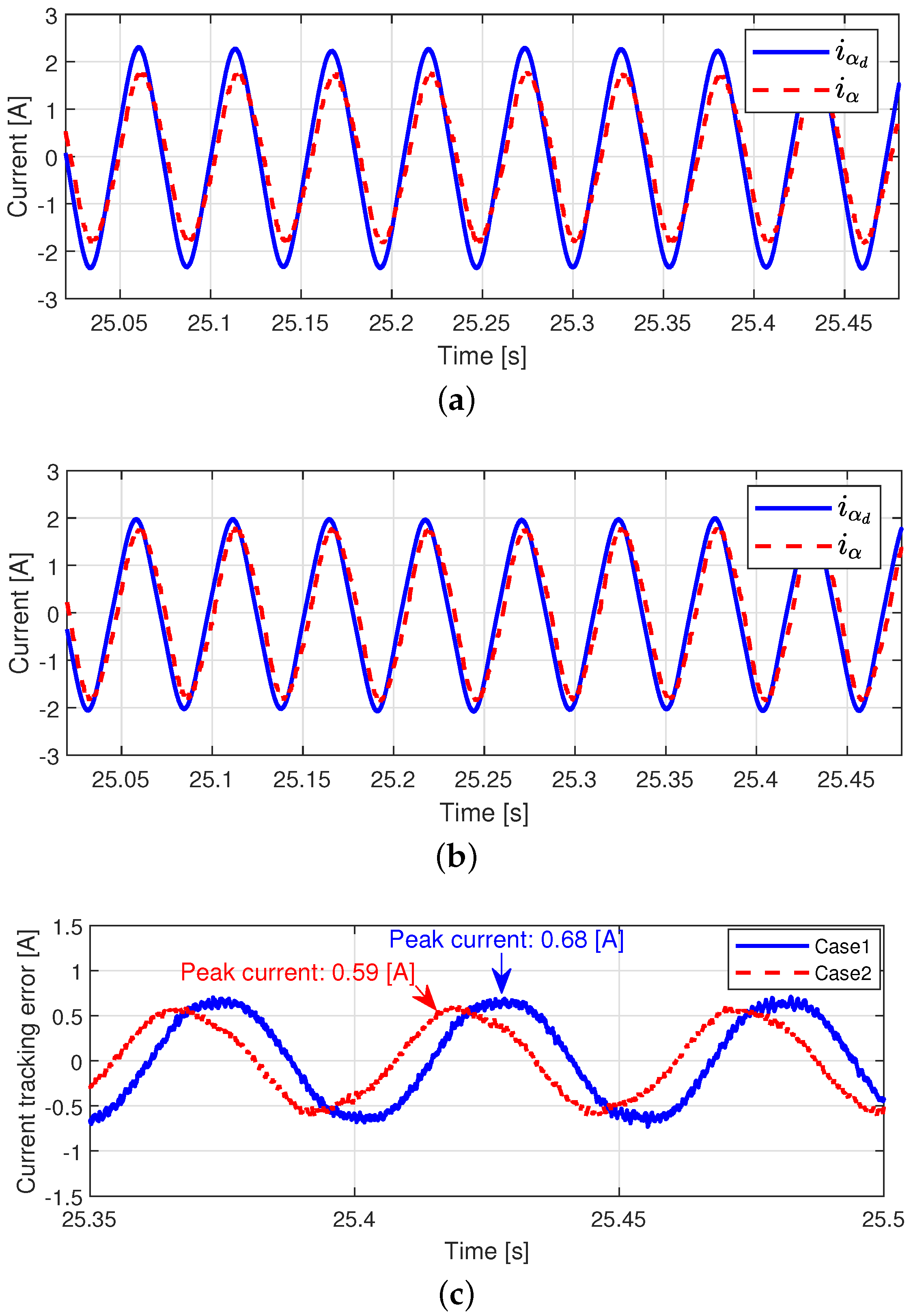
- Case 1: PI controller with = 0.105 (70% of nominal back-emf constant),
- Case 2: PI controller with (proposed back-EMF estimator).
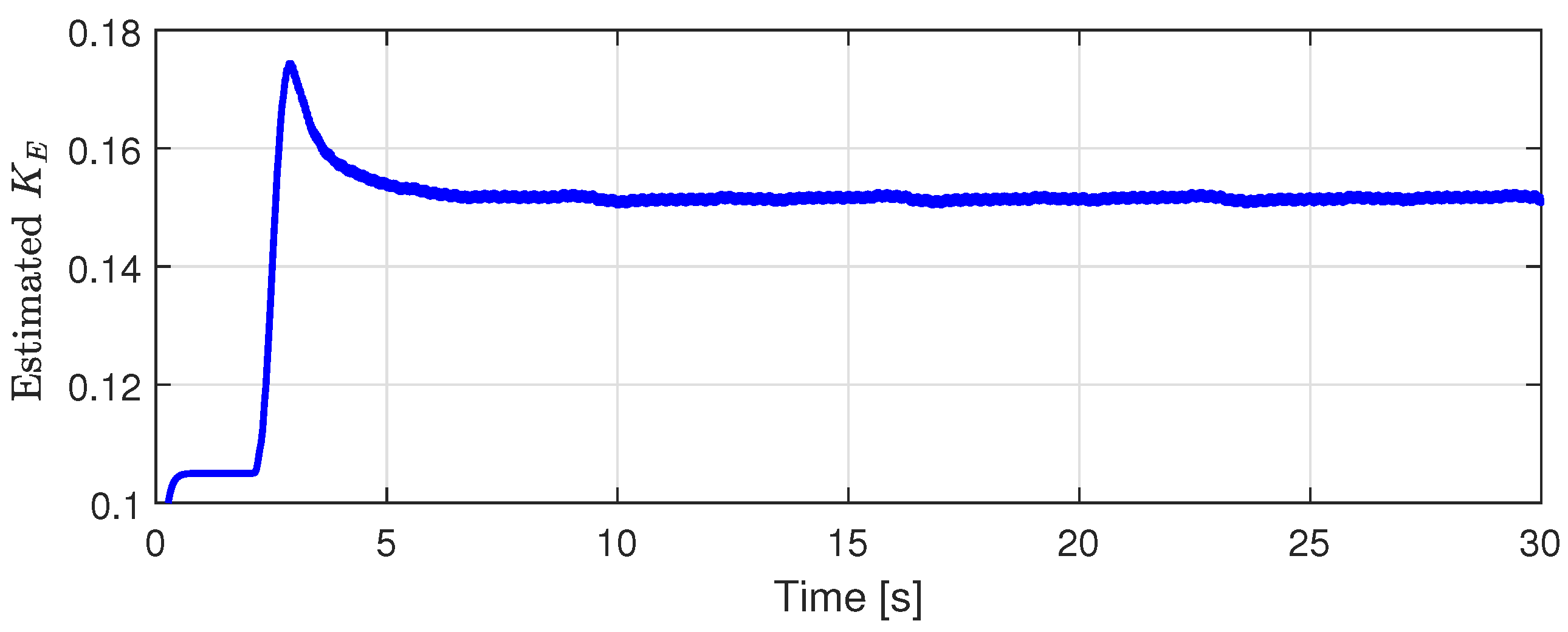
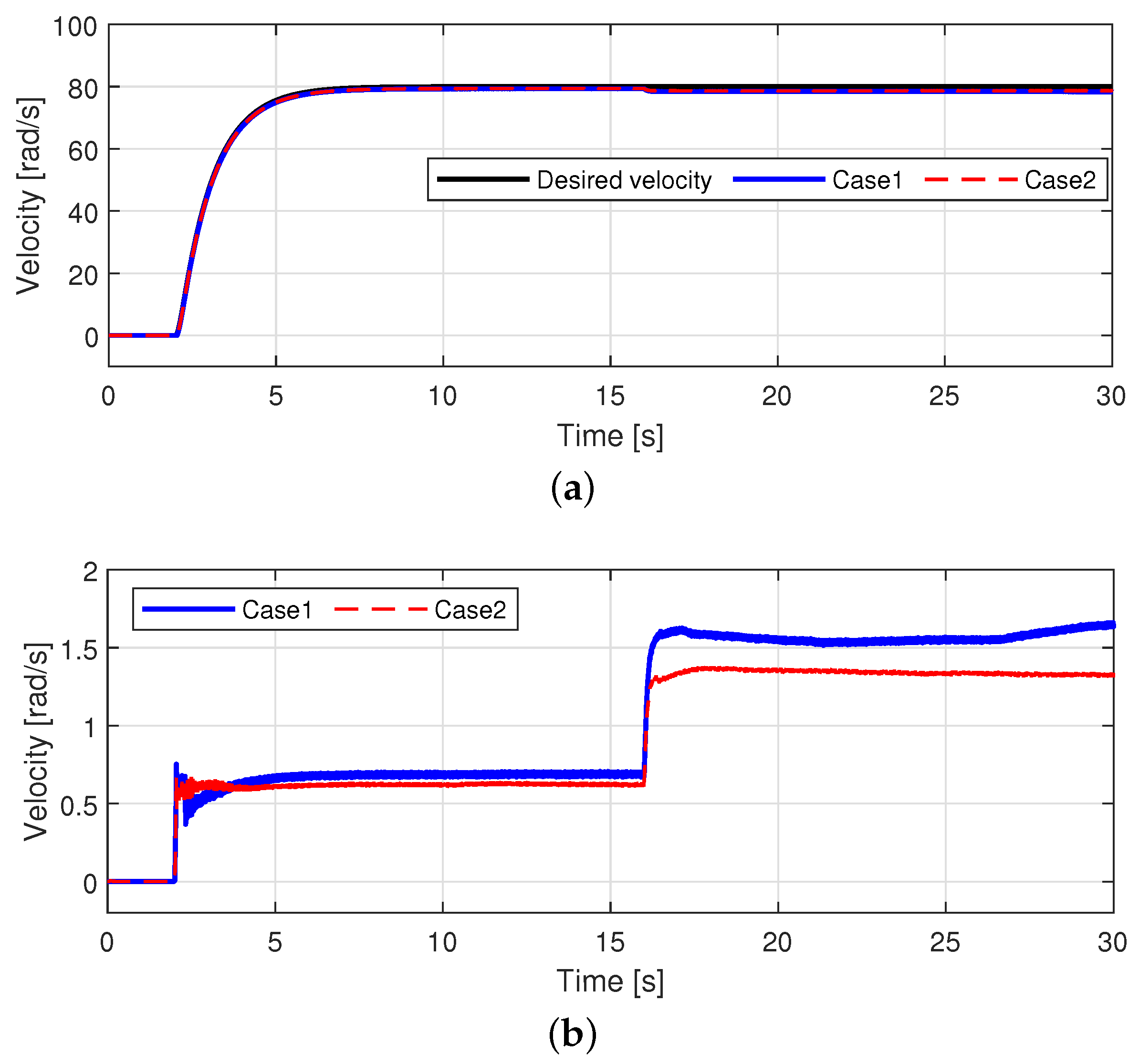
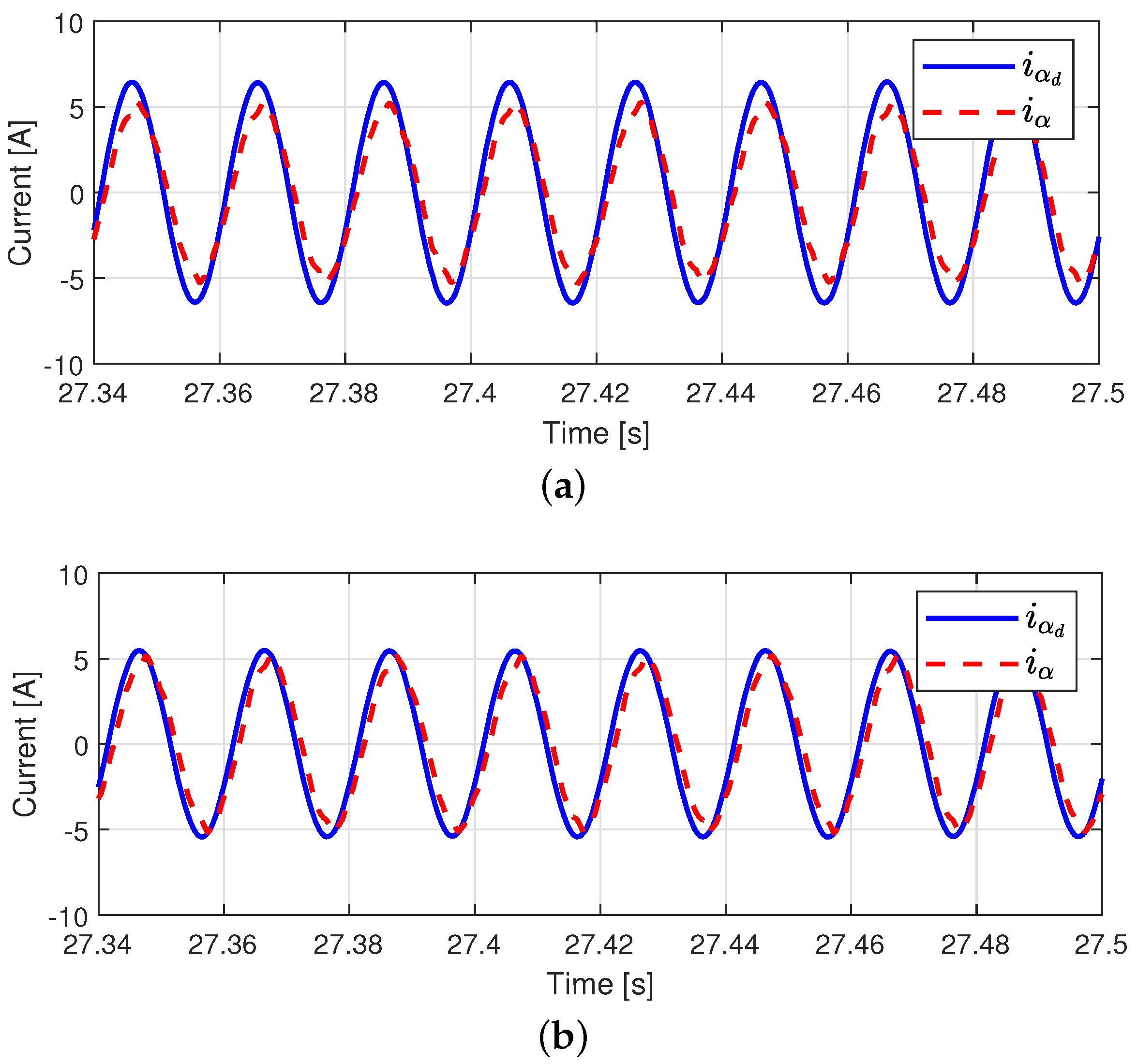
4.1. 30 rad/s Operation
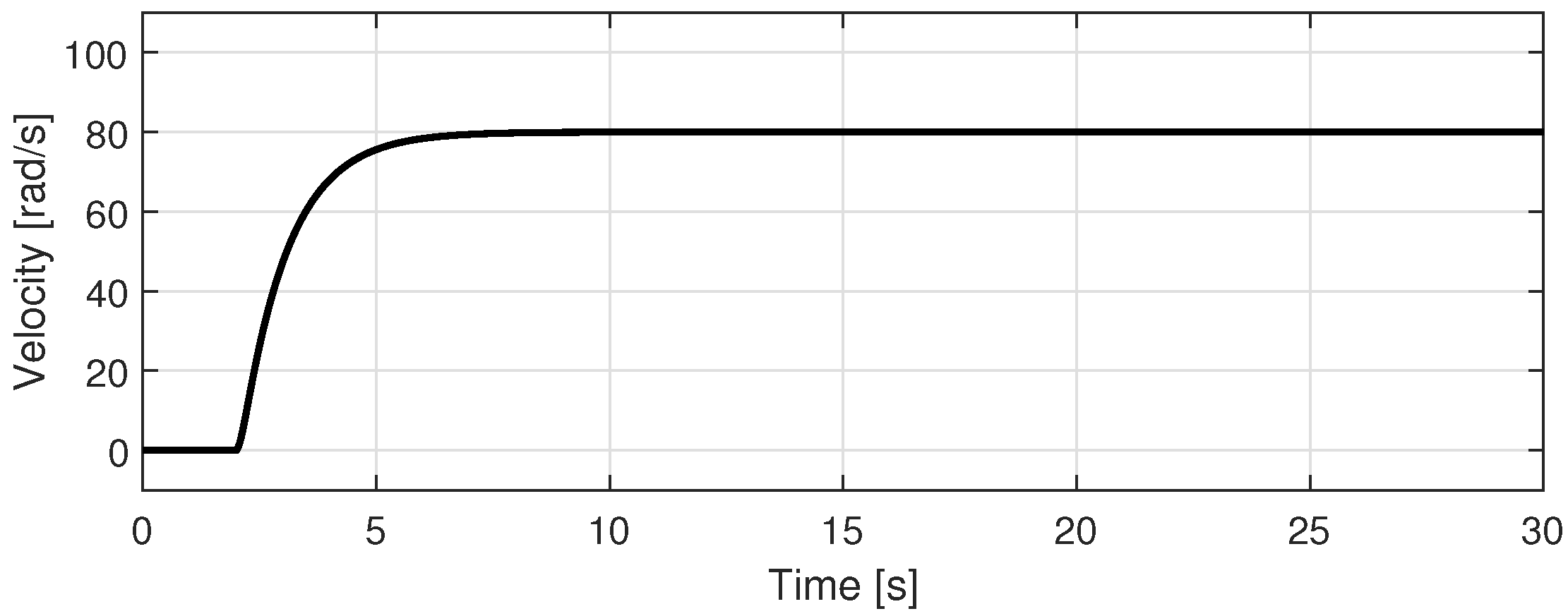
4.2. 80 rad/s Operation
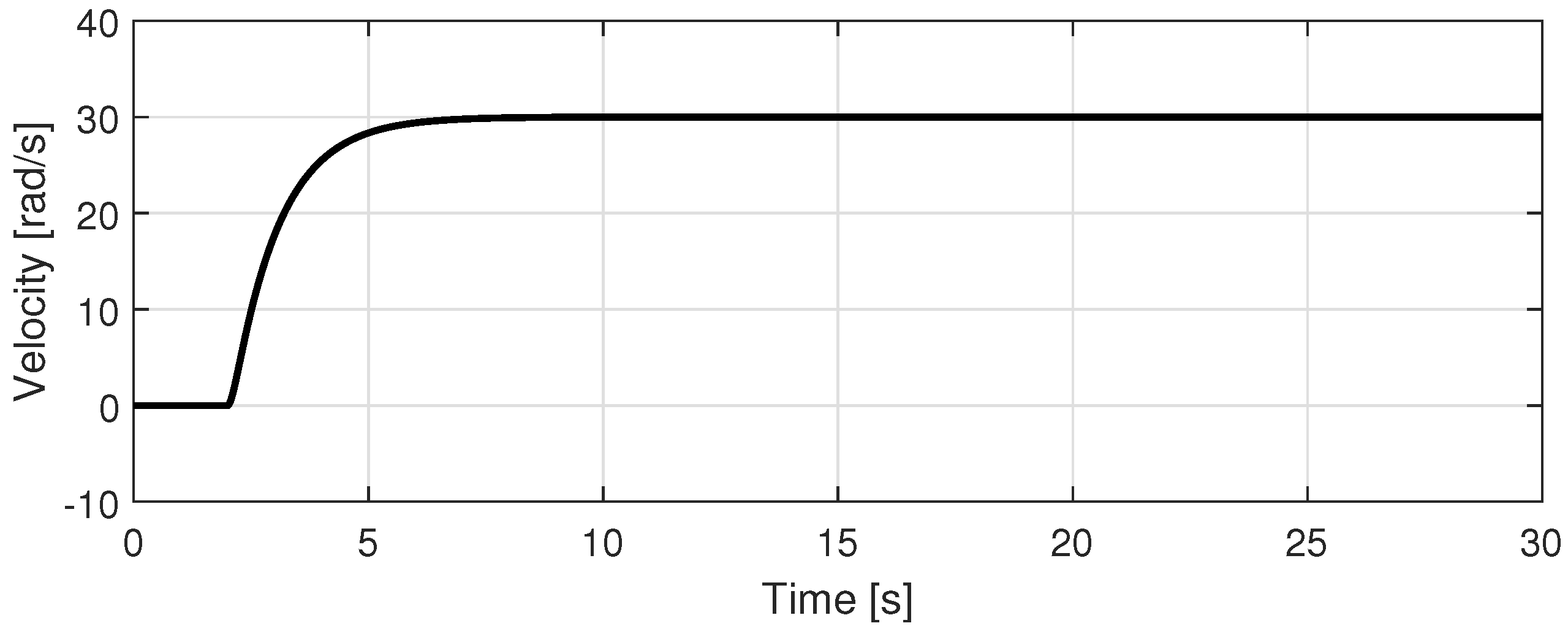
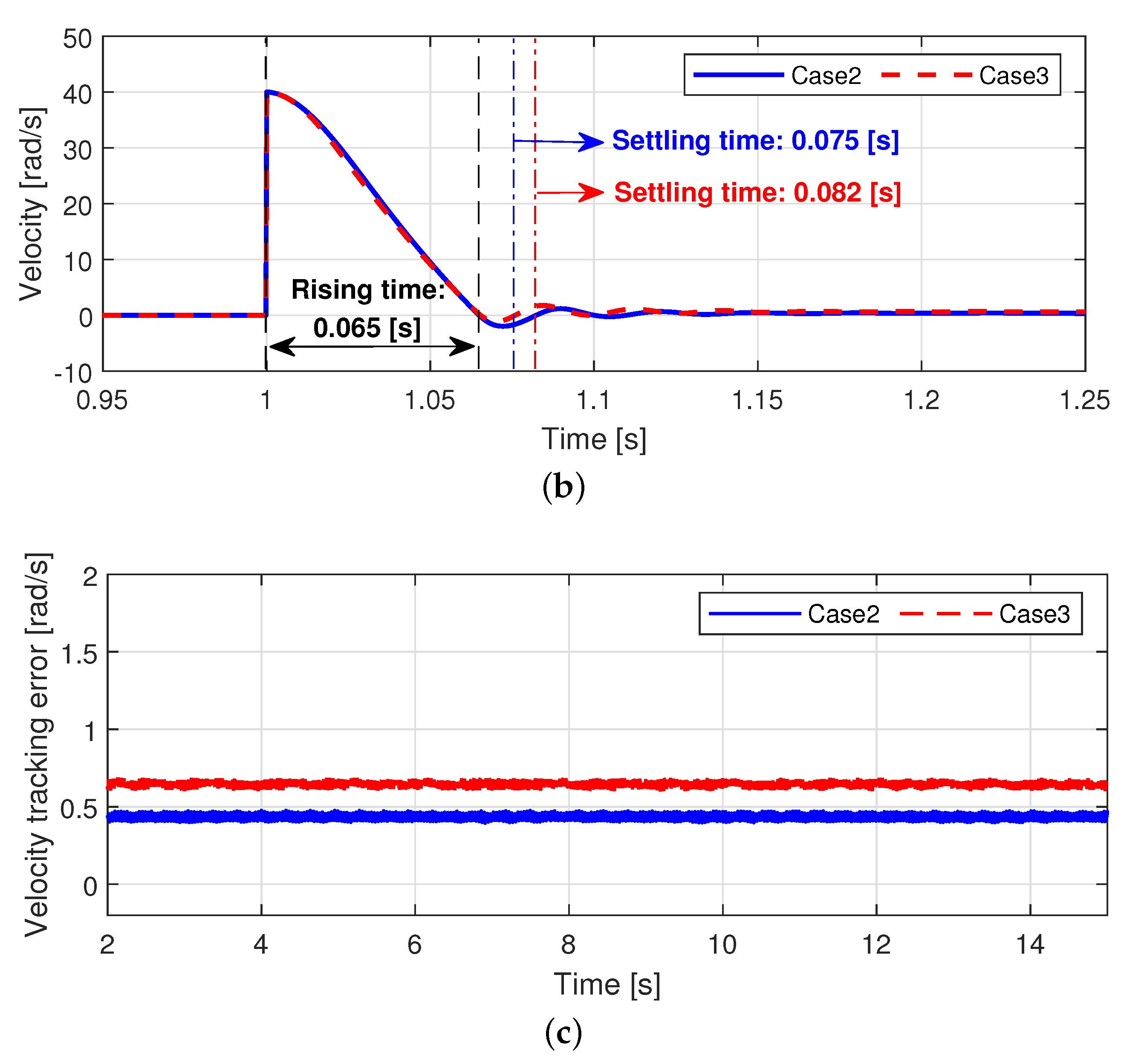
4.3. Step Response
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
Abbreviations
| PI | Proportional integral |
| PID | Proportional integral differential |
| EMF | Electro motive force |
| PMSMs | Permanent magnet synchronous motors |
| LPV | Linear parameter varying |
| Nomenclature | |
| Rotor angular position [rad] | |
| Rotor angular velocity [rad/s] | |
| Mechanical torque [N· m] | |
| Current of phase [A] | |
| Current of phase [A] | |
| Voltage of phase [V] | |
| Voltage of phase [V] | |
| Desired rotor angular velocity [rad/s] | |
| Desired current of phase [A] | |
| Desired current of phase [A] | |
| Desired mechanical torque [N· m] | |
| Back-EMF constant [V·s/rad] | |
| P | Pole pair |
| Flux linkage [Wb] | |
| B | viscous friction of the motor [N·m·s/rad] |
| J | Inertia of the motor [kg·m2/rad] |
| R | Resistance of the phase winding [] |
| L | Inductance of the phase winding [H] |
| Estimation error of back-EMF constant [V·s/rad] | |
| Estimation state of back-EMF constant [V·s/rad] | |
| Proportional gain of velocity control loop | |
| Integral gain of velocity control loop | |
| Proportional gain of current control loop | |
| Integral gain of current control loop | |
| Estimator gains |
References
- Li, X.; Kennel, R. General formulation of Kalman-filter-based online parameter identification methods for VSI-fed PMSM. IEEE Trans. Ind. Electron. 2021, 58, 2856–2864. [Google Scholar] [CrossRef]
- Lee, Y.; Lee, S.-H.; Chung, C.C. LPV H∞ control with disturbance estimation for permanent magnet synchronous motors. IEEE Trans. Ind. Electron. 2018, 65, 488–497. [Google Scholar] [CrossRef]
- Lee, Y.; Gil, J.; Kim, W. Velocity control for sideband harmonics compensation in permanent magnet synchronous motors with low switching frequency inverter. IEEE Trans. Ind. Electron. 2021, 68, 3434–3444. [Google Scholar] [CrossRef]
- Liu, M.; Chan, K.W.; Hu, J.; Xu, W.; Rodriguez, J. Model predictive direct speed control with torque oscillation reduction for PMSM drives. IEEE Trans. Ind. Inform. 2019, 15, 4944–4956. [Google Scholar] [CrossRef]
- Liang, D.; Li, J.; Qu, R.; Kong, W. Adaptive second-order sliding-mode observer for PMSM sensorless control considering VSI nonlinearity. IEEE Trans. Power Electron. 2018, 33, 8994–9004. [Google Scholar] [CrossRef]
- Yan, Y.; Yang, J.; Sun, Z.; Zhang, C.; Li, S.; Yu, H. Robust Speed Regulation for PMSM Servo System with multiple sources of disturbances via an augmented disturbance observer. IEEE/ASME Trans. Mechatron. 2018, 23, 769–780. [Google Scholar] [CrossRef]
- Choi, J.-W.; Sul, S.-K. Generalized solution of minimum time current control in three-phase balanced systems. IEEE Trans. Ind. Electron. 1998, 45, 738–744. [Google Scholar] [CrossRef]
- Kong, W.Y.; Holmes, D.G.; McGrath, B.P. Enhanced three phase ac stationary frame PI current regulators. In Proceedings of the 2009 IEEE Energy Conversion Congress and Exposition, San Jose, CA, USA, 20–24 September 2009; pp. 91–98. [Google Scholar]
- Xu, W.; Jiang, Y.; Mu, C.; Blaabjerg, F. Improved nonlinear flux observer-based second-order SOIFO for PMSM sensorless control. IEEE Trans. Power Electron. 2019, 34, 565–579. [Google Scholar] [CrossRef] [Green Version]
- Hanselman, D. Brushless Permanent Magnet Motor Design; McGraw-Hill: New York, NY, USA, 1994. [Google Scholar]
- Liu, K.; Zhu, Z.Q.; Stone, D.A. Parameter estimation for condition monitoring of PMSM stator winding and rotor permanent magnets. IEEE Trans. Ind. Electron. 2013, 60, 5902–5913. [Google Scholar] [CrossRef] [Green Version]
- Milanfar, P.; Lang, J.H. Monitoring the thermal condition of permanent-magnet synchronous motors. IEEE Trans. Aerosp. Electron. Syst. 1996, 32, 1421–1429. [Google Scholar] [CrossRef]
- Fernandez, D.; Reigosa, D.; Tanimoto, T.; Kato, T.; Briz, F. Wireless permanent magnet temperature & field distribution measurement system for IPMSMs. In Proceedings of the 2015 IEEE Energy Conversion Congress and Exposition (ECCE), Montreal, QC, Canada, 20–24 September 2015; pp. 3996–4003. [Google Scholar]
- Pulvirenti, M.; Scarcella, G.; Scelba, G.; Testa, A.; Harbaugh, M.M. On-line stator resistance and permanent magnet flux linkage identification on open-end winding PMSM drives. IEEE Trans. Ind. Appl. 2019, 55, 504–515. [Google Scholar] [CrossRef]
- Gai, H.; Li, X.; Jiao, F.; Cheng, X.; Yang, X.; Zheng, G. Application of a New Model Reference Adaptive Control Based on PID Control in CNC Machine Tools. Machines 2021, 9, 274. [Google Scholar] [CrossRef]
- Xiao, R.; Wang, Z.; Zhang, H.; Shen, J.; Chen, Z. A Novel Adaptive Control of PMSM for Electric Vehicle. In Proceedings of the 2017 IEEE Vehicle Power and Propulsion Conference (VPPC), Belfort, France, 11–14 December 2017; pp. 1–8. [Google Scholar]
- Piippo, A.; Hinkkanen, M.; Luomi, J. Adaptation of motor parameters in sensorless PMSM drives. IEEE Trans. Ind. Appl. 2009, 45, 203–212. [Google Scholar] [CrossRef] [Green Version]
- Cao, P.; Zhang, X.; Yang, S. A unified-model-based analysis of MRAS for online rotor time constant estimation in an induction motor drive. IEEE Trans. Ind. Electron. 2017, 64, 4361–4371. [Google Scholar] [CrossRef]
- Shi, Y.; Sun, K.; Huang, L.; Li, Y. Online identification of permanent magnet flux based on extended Kalman filter for IPMSM drive with position sensorless control. IEEE Trans. Ind. Electron. 2012, 59, 4169–4178. [Google Scholar] [CrossRef]
- Wang, Q.; Wang, G.; Zhao, N.; Zhang, G.; Cui, Q.; Xu, D. An impedance model-based multiparameter identification method of PMSM for both offline and online conditions. IEEE Trans. Power Electron. 2021, 36, 727–738. [Google Scholar] [CrossRef]
- Jang, M.; Akatsu, K. Magnet operating point estimation using flux linkage observer and magnetic and thermal equivalent circuit in PMSM. IEEJ J. Ind. Appl. 2021, 21006173. [Google Scholar] [CrossRef]
- Zhu, X.; Hua, W.; Wang, W.; Huang, W. Analysis of back-EMF in flux-reversal permanent magnet machines by air gap field modulation theory. IEEE Trans. Ind. Electron. 2019, 66, 3344–3355. [Google Scholar] [CrossRef]
- Park, J.S.; Lee, S.H.; Moon, C.; Kwon, Y.A. State observer with stator resistance and back-EMF constant estimation for sensorless PMSM. In Proceedings of the TENCON 2010—2010 IEEE Region 10 Conference, Fukuoka, Japan, 21–24 November 2010; pp. 31–36. [Google Scholar]






| PMSM Parameters, Control Gains, and Estimator Gains | ||
|---|---|---|
| PMSM parameters | [N·m·s/rad] | |
| J | [kg·m2/rad] | |
| R | [] | |
| L | [H] | |
| [V/rad/s] | ||
| Controller gains | 2000 | |
| 40 | ||
| 5 | ||
| 10 | ||
| Estimator gains | 1 | |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Gil, J.; You, S.; Lee, Y.; Kim, W. Back Electro Motive Force Estimation Method for Cascade Proportional Integral Control in Permanent Magnet Synchronous Motors. Actuators 2021, 10, 319. https://doi.org/10.3390/act10120319
Gil J, You S, Lee Y, Kim W. Back Electro Motive Force Estimation Method for Cascade Proportional Integral Control in Permanent Magnet Synchronous Motors. Actuators. 2021; 10(12):319. https://doi.org/10.3390/act10120319
Chicago/Turabian StyleGil, Jeonghwan, Sesun You, Youngwoo Lee, and Wonhee Kim. 2021. "Back Electro Motive Force Estimation Method for Cascade Proportional Integral Control in Permanent Magnet Synchronous Motors" Actuators 10, no. 12: 319. https://doi.org/10.3390/act10120319
APA StyleGil, J., You, S., Lee, Y., & Kim, W. (2021). Back Electro Motive Force Estimation Method for Cascade Proportional Integral Control in Permanent Magnet Synchronous Motors. Actuators, 10(12), 319. https://doi.org/10.3390/act10120319







