Numerical Modeling of Masonry Infilled Reinforced Concrete Building during Construction Stages Using ABAQUS Software
Abstract
:1. Introduction
- Provide information about the dynamic characteristics of the Turkish RC masonry buildings at different construction stages.
- Determine the modeling errors through comparison study results found in the laboratory.
- Understand the effect of the structural component and material such as wall and plaster work on dynamic characteristics of the completed building.
- Compare the ultimate displacements and principal stresses for each stage.
Novelty of This Study Paper
2. Description of the Structure
2.1. Geometry of the Prototype
2.2. Material Properties
2.3. Finite Element Modeling
3. Results and Discussion
3.1. Modal Analyses
3.1.1. The Natural Frequencies
3.1.2. Mode Shapes
3.2. Static Analysis
3.2.1. Ultimate Displacements
3.2.2. Maximum Principal Stresses
4. Conclusions
- For the first three modes, the relative errors between the frequencies obtained numerically and experimentally were around 1% to 5%. It indicates that natural frequencies obtained from ABAQUS agree well with the experimental results. However, at the fourth and fifth mode, the difference was between 12% to 16%. This could be ascribed to different reasons; for example, plastering defects or else uncertainties in mechanical properties may be due to the incorrect positioning of samples during laboratory tests.
- As a result of this study, it is clearly shown that the presence of infill walls that have plaster work significantly increases the natural frequencies by about 70%, which affects the dynamic behavior of the building, and they must be taken into account in structural analysis.
- By comparing the displacements for each stage of construction analysis, it can be concluded that the magnitudes of the displacements depend not only on the storey’s level and masonry walls, but also on materials such as plaster coating in infield RC buildings. Moreover, it is clearly seen that the maximum principal stress shows no correlation with the masonry unit type. However, the application of a plastering mortar layer seems to increase to some extent the maximum stress.
Author Contributions
Funding
Conflicts of Interest
References
- Kechidi, S.; Bourahla, N. Effective use of ambient vibration measurement for modal updating. In Proceedings of the 1st National Meeting of Civil Engineering, Bejaia, Algeria, 22–23 October 2014. [Google Scholar]
- Diaferio, M.; Foti, D.; Mongelli, M.; Giannoccaro, I.N.; Andersen, P. Operational Modal Analysis of a Historical Tower in Bari, in Civil Engineering Topics. In Proceedings of the Society for Experimental Mechanics Series, “IMAC XXIX”, Jacksonville, FL, USA, 31 January–3 February 2011. [Google Scholar]
- Oyarzo-Vera, C.; Jason, I.; Nawawi, C. Vibration-Based Damage Identification of an Unreinforced Masonry House Model. In Proceedings of the 9th International Conference on Structural Dynamics, Porto, Portugal, 30 June–2 July 2014; pp. 2477–2484. [Google Scholar]
- Günaydin, M.; Adanur, S.; Altunişik, A.C.; Sevim, B.; Bayraktar, A. Finite Modeling Updating Effects on the Dynamic Response of Building Models. J. Test. Eval. 2017, 45, 1630–1649. [Google Scholar] [CrossRef]
- Vlad, I.; Vlad, M. Ambient vibration instrumental investigations on full scale structures. In Proceedings of the 8th International Conference on Structural Dynamics, Leuven, Belgium, 4–6 July 2011; pp. 2424–2431, ISBN 978-9-07-601931-4. [Google Scholar]
- De Sortis, A.; Antonacci, E.; Vestroni, F. Dynamic identification of a masonry building using forced vibration tests. Eng. Struct. 2005, 27, 155–165. [Google Scholar] [CrossRef]
- Rombach, G.A. Finite-Element Design of Concrete Structures Practical Problems and Their Solutions; ICE Publishing: London, UK, 2011. [Google Scholar]
- Arslan, M.E.; Durmuş, A. Modal parameter identification of in-filled RC frames with low strength concrete using ambient vibration. Struct. Eng. Mech. 2014, 50, 137–149. [Google Scholar] [CrossRef]
- Kaplan, O.; Guney, Y.; Doğangün, A.; Livaoğlu, R. Effect of Infill Walls and Plaster for a Four Storey R/C Building. In Proceedings of the 6th the International Operational Modal Analysis Conference (IOMAC), Gijón, Spain, 12–14 May 2015. [Google Scholar]
- Bayraktar, A.; Turker, T.; Altunısık, A.C.; Sevim, B.; Sahin, A.; Ozcan, D.M. Determination of dynamic parameters of buildings by operational modal analysis method. Tek. Dergi 2010, 21, 5185–5205. [Google Scholar]
- Al-Nimry, H.; Resheidat, M.; Al-Jamal, M. Ambient vibration testing of low and medium rise in-filled RC frame buildings in Jordan Soil. Soil Dyn. Earthq. Eng. 2014, 59, 21–29. [Google Scholar] [CrossRef]
- Bikçe, M.; Geneş, M.C.; Kaçın, S.; Zubaroglu, A. A concrete-walled structure without walls and dynamic testing of these cases. In Proceedings of the 1st Turkey Earthquake Engineering and Seismology Conference, Ankara, Turkey, 11–14 October 2011. [Google Scholar]
- Amanat, K.M.; Hoque, E. A rationale for determining the natural period of RC building frames having infill. Eng. Struct. 2006, 28, 495–502. [Google Scholar] [CrossRef]
- Hao, H.; Brownjohn, J.M.W.; Xia, P.Q.; Xia, Y. Civil Structure Condition Assessment by FE Model Updating: Methodology and Case Studies. Finite Elem. Anal. Des. 2001, 37, 761–775. [Google Scholar]
- Timurağaoğlu, M.Ö.; Doğangün, A.; Livaoğlu, R. Finite element analysis of ınfilled RC frames under lateral loads. In Proceedings of the 2nd International Conference on Engineering and Natural Sciences (ICENS 2016), Sarajevo, Bosnia and Herzegovina, 24–28 May 2016. [Google Scholar]
- Kubalski, T.; Marinković, M.; Butenweg, C. Numerical investigation of masonry infilled R.C. frames. In Proceedings of the 16th International Brick and Block Masonry Conference, Padova, Italy, 26–30 June 2016; pp. 1219–1229, ISBN 978-1-38-02999-6. [Google Scholar]
- Khatiwada, E.; Jiang, H. Numerical Modeling of Masonry Infilled Reinforced Concrete Moment Resisting Frame. In Proceedings of the Thirtieth KKHTCNN Symposium on Civil Engineering, Taipei, Taiwan, 2–4 November 2017. [Google Scholar]
- Furtado, A.; Vila-Pouca, N.; Varum, H.; Arêde, A. Study of the Seismic Response on the Infill Masonry Walls of a 15-Storey Reinforced Concrete Structure in Nepal. Buildings 2019, 9, 39. [Google Scholar] [CrossRef]
- SAP2000. Integrated Finite Element Analysis and Design of Structures Basic Analysis Reference Manual; Computer and Structures, Inc.: Berkeley, CA, USA, 2009. [Google Scholar]
- Ghosh, A.K.; Amde, A.M. Finite element analysis of infilled frames. J. Struct. Eng. 2001, 128, 881–889. [Google Scholar] [CrossRef]
- Güler, K.; Yuksel, E.; Kocak, A. Estimation of the fundamental vibration period of existing RC buildings in Turkey utilizing ambient vibration records. J. Earthq. Eng. 2008, 12, 140–150. [Google Scholar] [CrossRef]
- Hallebrand, E.; Jacobsson, W. Structural Design of High-Rise Buildings. Master’s Dissertation, Faculty of Engineering LTH, Lund University, Lund, Sweden, 2016. [Google Scholar]
- Türker, T.; Bayraktar, A. Vibration based modal testing of a scaled reinforced concrete building for construction stages. Bull. Earthq. Eng. 2016, 5, 1–18. [Google Scholar] [CrossRef]
- Turkish Standard Institution (TS-705). Solid Brick and Vertically Perforated Bricks (the Classification, Properties, Sampling, Testing and Marking of Solid Bricks and Vertically Perforated Bricks); Turkish Standard Institution: Ankara, Turkey, 1985. [Google Scholar]
- Dilrukshi, K.G.S.; Dias, W.P.S. Field survey and numerical modelling of cracking in masonry walls due to thermal movements of an overlying slab. J. Natl. Sci. Found. Sri Lanka 2008, 36, 205–213. [Google Scholar] [CrossRef]
- Ministry of Public Works and Settlement Government of Republic of Turkey. Specification for Structures to be Built in Disaster Areas; Ministry of Public Works and Settlement Government of Republic of Turkey: Ankara, Turkey, 2007.
- ABAQUS. Version 6.14 User’s Manual; Dassault Systèmes Simulia Corp.: Providence, RI, USA, 2014. [Google Scholar]
- Laurenco, P.; Rots, J.G.; Blaauwendraad, J. Two approaches for the analysis of masonry structures: Micro and macro-modeling. Heron 1995, 40, 313–340. [Google Scholar]
- Lourenço, P.B. Structural masonry analysis: Recent developments and prospects. In Proceedings of the 14th International Brick and Block Masonry Conference, Callaghan, Australia, 17–20 February 2008; Masia, M., Bokan-Bosiljkov, V., Bosiljkov, V., Eds.; University of Newcastle: Newcastle, UK, 2008. [Google Scholar]
- Penna, A.; Lagomarsino, S.; Galasco, A. A nonlinear macro element model for the seismic analysis of masonry buildings. Earthq. Eng. Struct. Dyn. 2014, 43, 159–179. [Google Scholar] [CrossRef]
- Raj, D.; Bharathi, M. Effects of Soil-Structure Interaction on Regular and Braced RC Building. In Proceedings of the Indian Geotechnical Conference, Roorkee, India, 22–24 December 2013. [Google Scholar]
- Ministry of Public Works and Settlement Government of Republic of Turkey. Specification for Structures to be Built in Disaster Areas; Ministry of Public Works and Settlement Government of Republic of Turkey: Ankara, Turkey, 1997.
- Pan, T.C.; You, X.; Brownjohn, M.A.W. Effects of infill walls and floor diaphragms on the dynamic characteristics of a narrow-rectangle building. Earthq. Eng. Struct. Dyn. 2006, 35, 637–651. [Google Scholar] [CrossRef]
- TS 498. Design Loads for Buildings; Turkish Standard Institute: Ankara, Turkey, 1997. (In Turkish) [Google Scholar]
- Ural, A.; Dogangün, A. Practices of Brick Masonry Construction in Turkey and their Seismic Behaviors during Earthquakes. In Proceedings of the 1st International Conference on Restoration of Heritage Masonry Structures, Cairo, Egypt, 18 April 2015. [Google Scholar]


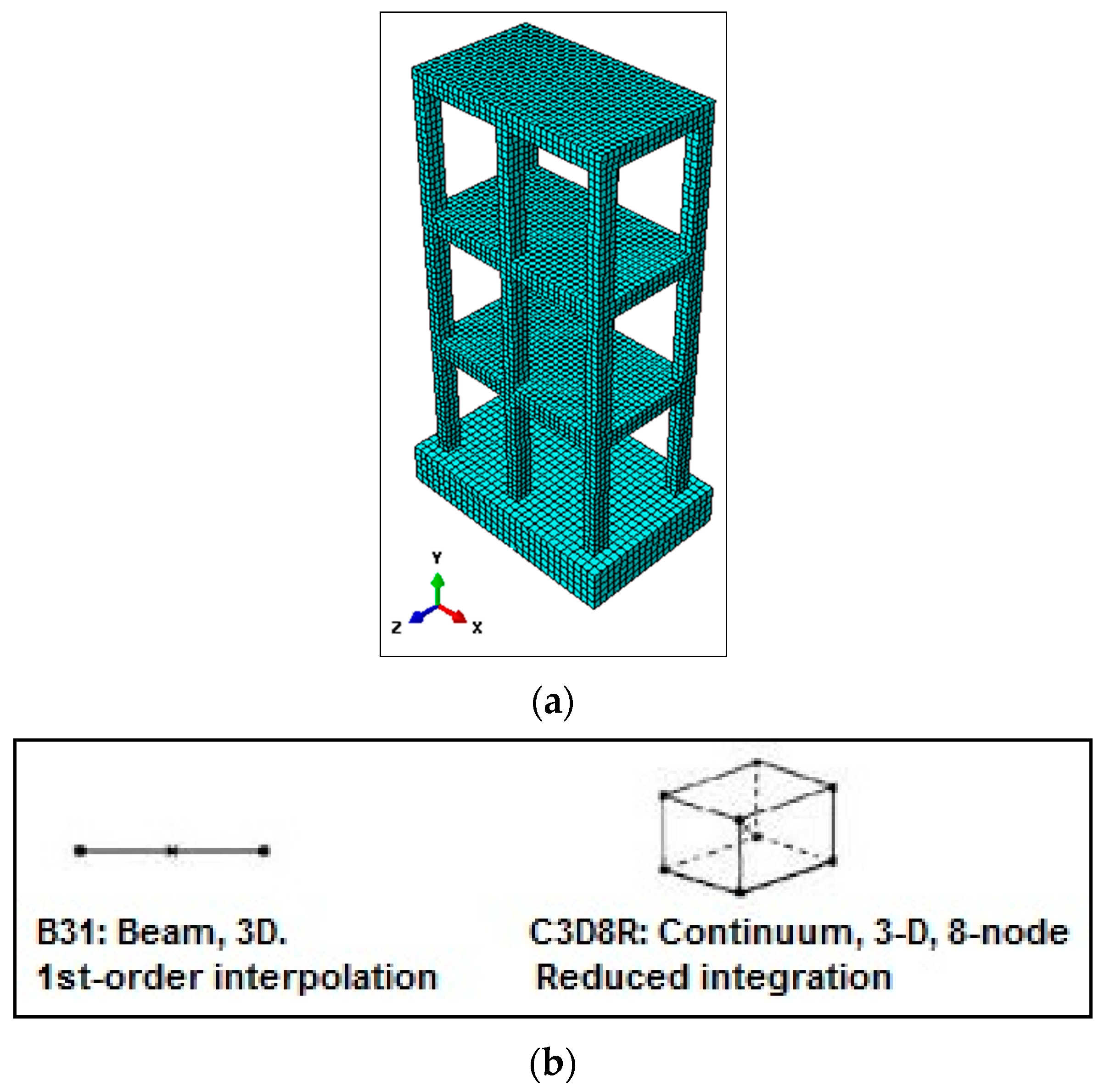


| Column and Beam (mm) | Foundation (mm) | Slabs (mm) | |
|---|---|---|---|
| Reinforcements | Dimensions | Thickness | |
| 4 Φ 10 | 150 × 200 | 500 | 75 |
| Elements | Modulus of Elasticity (MPa) | Poisson’s Ratio (-) | Density (kg/m3) | Compressive Strength (MPa) |
|---|---|---|---|---|
| Concrete | 22,000 | 0.20 | 2350 | 20 |
| Steel | 210,000 | 0.30 | 7850 | 420 |
| Brick | 1000 | 0.20 | 2000 | 05 |
| Cement plaster | 10,000 | 0.20 | 1950 | 7.50 |
| Construction Stage Cases | Vibration Modes | Frequency (Hz) | Relative of Errors (%) | |||
|---|---|---|---|---|---|---|
| Experimental | Analytical | |||||
| One-storey case | 1 | 18.91 |  | 18.83 |  | 0.42 |
| 2 | 23.40 | 22.63 | 3.40 | |||
| 3 | 28.72 | 28.37 | 1.23 | |||
| Two-storey case | 1 | 9.69 |  | 10.18 |  | 4.81 |
| 2 | 11.36 | 11.54 | 1.56 | |||
| 3 | 14.08 | 14.79 | 4.80 | |||
| 4 | 27.33 | 28.76 | 4.95 | |||
| 5 | 34.51 | 36.35 | 5.06 | |||
| Three-storey Case | 1 | 6.43 |  | 6.98 |  | 3.58 |
| 2 | 7.32 | 7.47 | 2.00 | |||
| 3 | 9.33 | 9.84 | 5.18 | |||
| 4 | 19.70 | 20.48 | 3.81 | |||
| 5 | 23.49 | 23.09 | 1.73 | |||
| Brick-walled case | 1 | 11.18 |  | 11.96 | 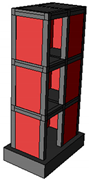 | 0.58 |
| 2 | 13.17 | 12.97 | 1.54 | |||
| 3 | 20.67 | 20.05 | 3.09 | |||
| 4 | 37.76 | 36.47 | 3.53 | |||
| 5 | 44.35 | 42.90 | 5.80 | |||
| Coated-wall Case | 1 | 13.89 |  | 14.54 |  | 4.47 |
| 2 | 16.79 | 17.25 | 2.67 | |||
| 3 | 31.23 | 29.68 | 5.22 | |||
| 4 | 52.01 | 44.62 | 16.56 | |||
| 5 | 56.80 | 50.67 | 12.10 | |||
| Cases | Measurement Model | Analytical Model |
|---|---|---|
| One-storey |  |  |
| Two-storey |  | 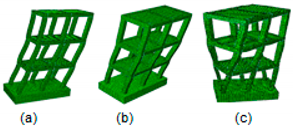 |
| Three-storey |  | 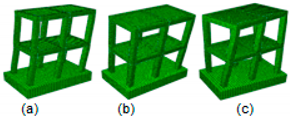 |
| Cases | Measurement Model | Analytical Model |
|---|---|---|
| Brick walled |  |  |
| Coated Brick |  | 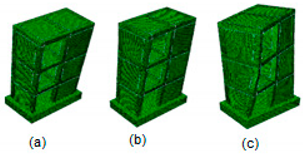 |
| Results | Frame Bare Cases | Brick-Wall Case | Coated-Wall Case | ||
|---|---|---|---|---|---|
| One-Storey | Two-Storey | Three-Storey | |||
| Ultimate displacement (mm) | 1.04 | 1.11 | 1.17 | 1.36 | 1.25 |
| Maximum principal stress (MPa) | 3.61 | 2.40 | 1.24 | 3.36 | 3.41 |
© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Roudane, B.; Adanur, S.; Altunışık, A.C. Numerical Modeling of Masonry Infilled Reinforced Concrete Building during Construction Stages Using ABAQUS Software. Buildings 2019, 9, 181. https://doi.org/10.3390/buildings9080181
Roudane B, Adanur S, Altunışık AC. Numerical Modeling of Masonry Infilled Reinforced Concrete Building during Construction Stages Using ABAQUS Software. Buildings. 2019; 9(8):181. https://doi.org/10.3390/buildings9080181
Chicago/Turabian StyleRoudane, Boudjamaa, Süleyman Adanur, and Ahmet Can Altunışık. 2019. "Numerical Modeling of Masonry Infilled Reinforced Concrete Building during Construction Stages Using ABAQUS Software" Buildings 9, no. 8: 181. https://doi.org/10.3390/buildings9080181
APA StyleRoudane, B., Adanur, S., & Altunışık, A. C. (2019). Numerical Modeling of Masonry Infilled Reinforced Concrete Building during Construction Stages Using ABAQUS Software. Buildings, 9(8), 181. https://doi.org/10.3390/buildings9080181





