2. Critical Analysis
The use of well-known conventional design methods [
6,
7,
8,
9,
10,
21], known from the traditional courses of theory of structures, in the shaping of shell roof forms is ineffective because it usually results in high values of normal and shear stresses, local buckling and distortion of thin-walled flanges and webs of transformed shell folds. The assembly of the designed shell sheeting into skewed roof directrices is often impossible because of the plasticity of the fold’s edges between flanges and webs. Reichhart developed a specific method for calculating the arrangement and the length of the supporting lines of all folds in transformed corrugated shell sheeting [
11], but it is effective only for the cases where the fold’s longitudinal axes are perpendicular to roof directrices or very close to those [
13]. The author significantly improved the Reichhart concept and has proposed an innovative method [
13,
18], so that the transformation would cause the smallest possible initial stresses on the shell folds resulting from this transformation.
For effective fold transformations, interdependence between the geometrical supporting conditions and the obtained shell forms of a transformed fold in a shell can be used [
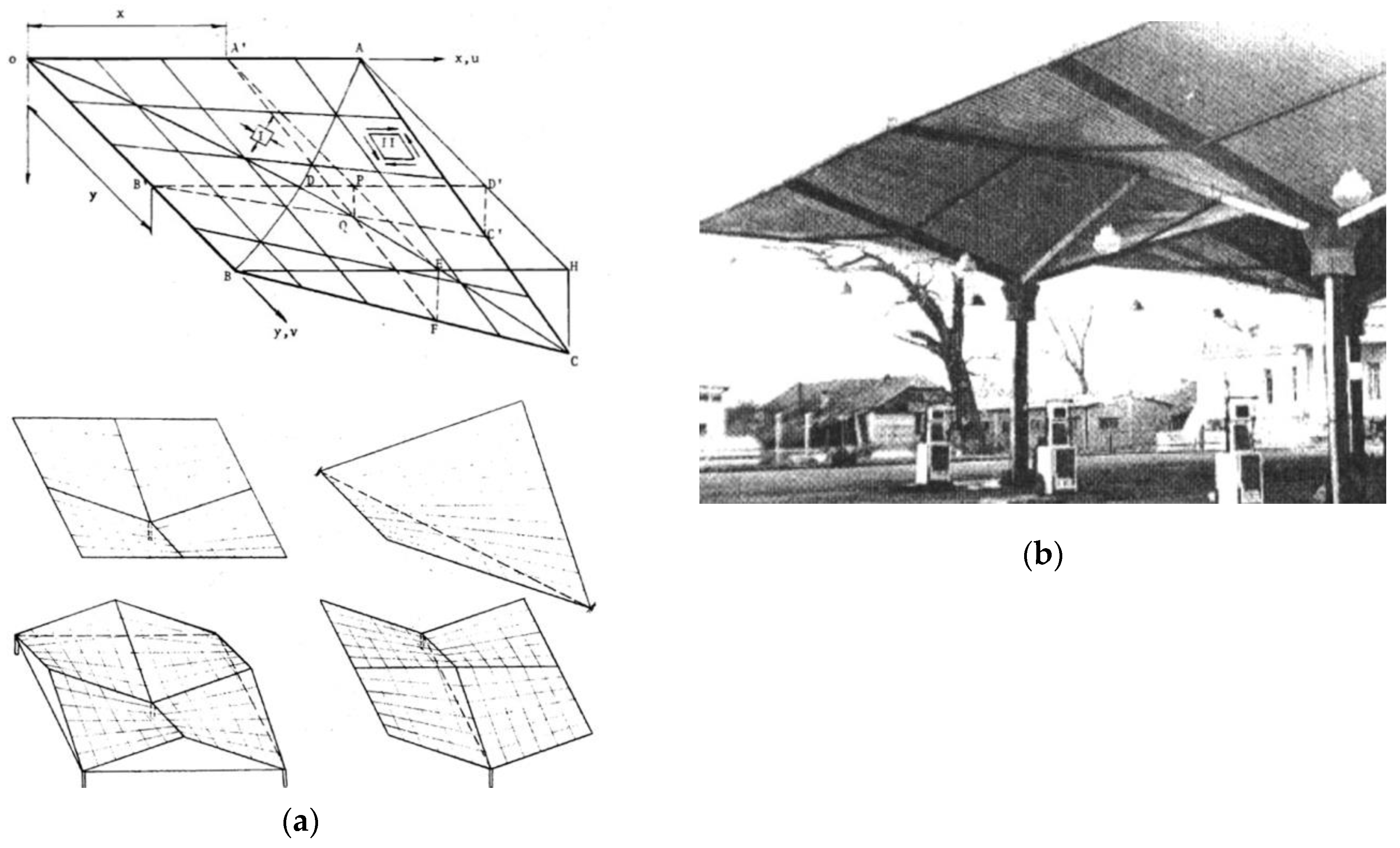
11]. In these cases, the freedom of the transverse width and height increments of each shell fold forming the transformed sheeting is ensured, and various attractive and innovative shapes of shell roofs and contraction curves of relatively big curvatures on these roofs can be achieved (
Figure 3) [
13]. If the fold does not have the freedom of transverse width increments due to strong stiffening of its longitudinal edges shared with its adjacent folds, the aforementioned interdependence cannot be used. Neither can it be used if the assembly technique causes additional forces varying the effective widths of the fold ends and their supporting lines.
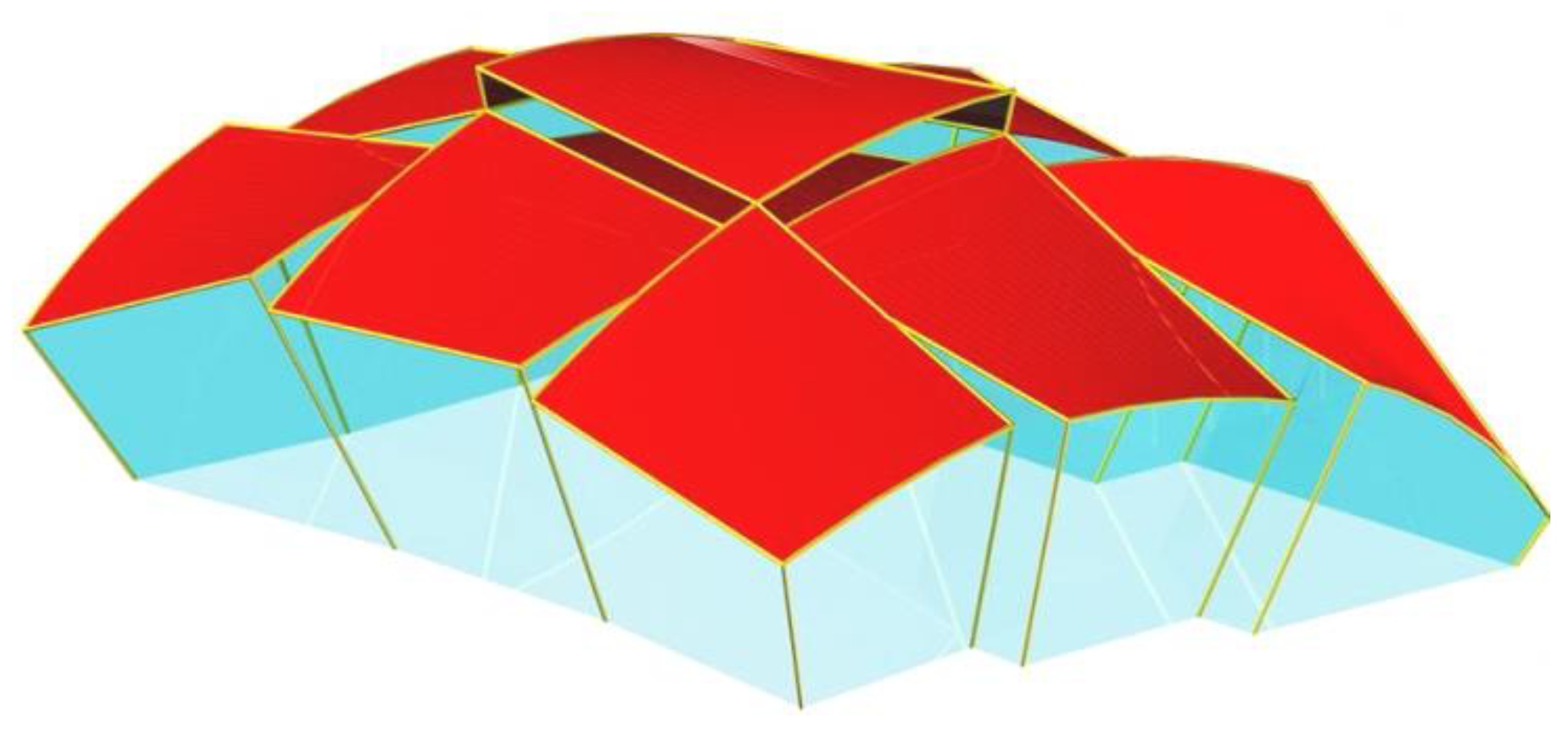
In the 1970s, Gergely, Banavalkar and Parker [
24] accomplished shape transformations of folded sheets to create shallow right hyperbolic paraboloid roofs and their structures, named “hypars”. The very limited shapes of shell roof structures using various configurations of hypars units are also discussed by Bryan and Davies [
25]. Right hyperbolic paraboloids are a specific kind of hyperbolic paraboloids whose two rulings belonging to various families of rulings are perpendicular to each other. These two rulings are various lines of contraction of each right hyperbolic paraboloid [
13]. Quarters and halves of these central sections are also used and joined together to obtain various shell structures, including hypars [
24,
25,
26] (
Figure 4).
The methods proposed by these authors drastically limit the variety of the designed transformed folded shell forms to central sectors of right hyperbolic paraboloids [
26] and their one-fourths [
24,
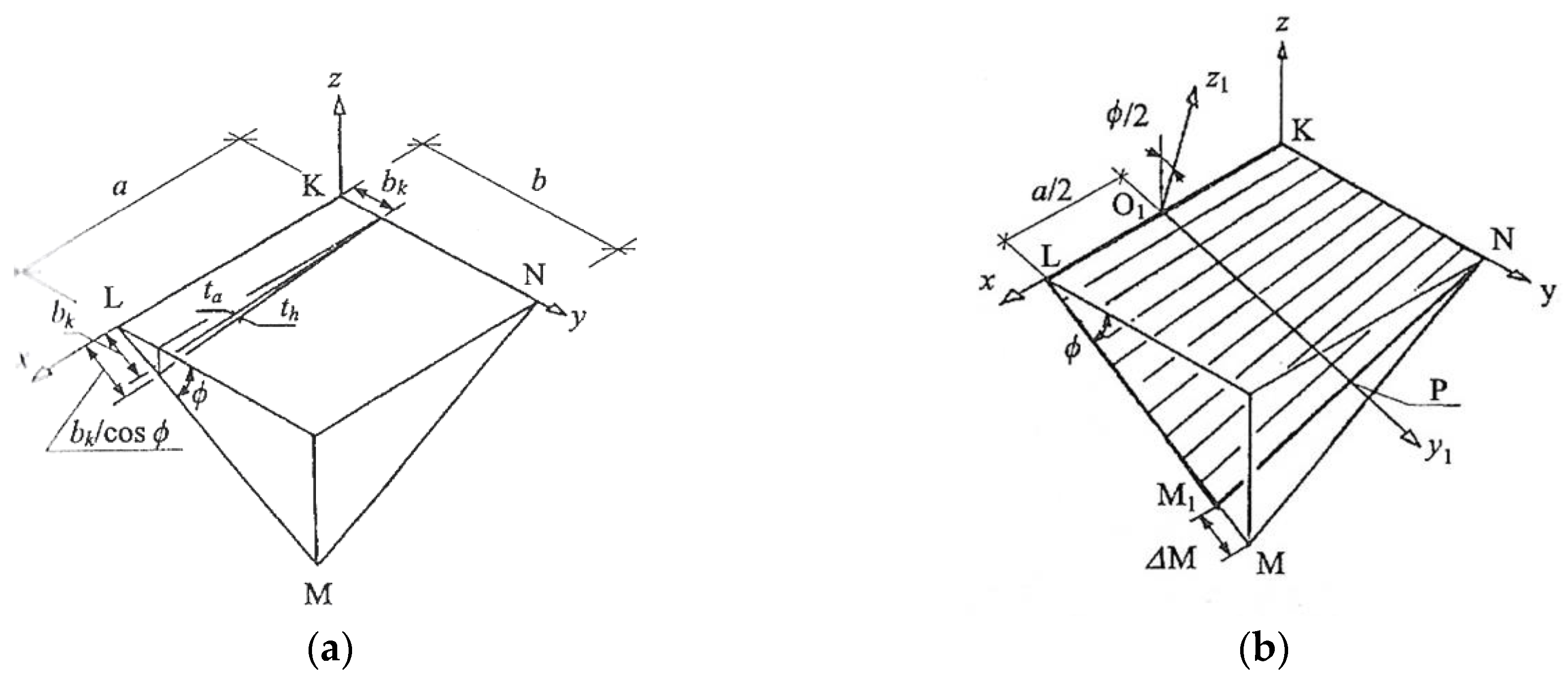
25]. Moreover, the models obtained by means of these methods enforce unjustified additional stresses of the folds resulting from the need to adjust the longitudinal axes of the shell folds to the positions of selected rulings of the hyperbolic paraboloid used. The above adjustment of the longitudinal shell fold’s axes to these rulings imposes a significant change in the width Δ
M (
Figure 5) [
11] of the transverse fold’s ends passing along shell directrix
LM. These additional forces cause a significant increase in initial stresses and limitation of the searched surfaces to shallow hypars.
Simple shell structures composed of a few corrugated shells have been used in different architectural configurations, most often as shells supported by stiff constructions based on very few columns [
27,
28]. Shell structures are used for achieving: (a) large spans; (b) greater architectural attractiveness; and (c) skylights letting sunlight into the building interior [
29,
30].
There are very few methods for the geometrical shaping of folded steel roofs transformed into shell forms. Among them, only the Reichhart method allows complete shells different from the central sectors of right hyperbolic paraboloids to be obtained [
11]. In the 1990s, Reichhart started to shape corrugated steel sheeting for shell roofing, where all folds underwent big transformations into shell shapes. An additional advantage of the Reichhart method is that the initial stresses induced by the shape transformations are the smallest possible. Reichhart called such transformations free deformations, because they assure freedom of the transversal width and height increments of all folds in the transformed shell. In this way, the initial fold’s effort is reduced to a possibly low level.
Reichhart arranged the complete corrugated shells on horizontal or oblique planes [
11] as continuous ribbed structures (
Figure 2). He developed a simple method for geometrical and strength shaping of the transformed shell roofs. He designed corrugated shell sheeting supported by very stiff frameworks or planar girders with additional intermediate members and roof bracings. [
20].
The transverse ends of transformed folds cannot be extended to the positions predicted by conventional methods, because this action causes a radical increase in stresses of the deformed thin-walled profiles [
11,
13,
14]. The fold’s fixing points along all roof directrices must be precisely calculated either by the Reichhart method [
11], if the longitudinal fold’s axes are close to perpendicular to the directrices, or by the author’s method [
13,
18]. For these calculations based on precisely calculated supporting conditions, diversified for the subsequent folds in a roof shell, and the stiffness of these folds resulting from their geometrical and mechanical properties can be applied.
Unfortunately, the Reichhart method is correct only when the longitudinal axes of the transformed shell folds are perpendicular to the roof directrices, or very close to those, and the algebraic equations of these directrices are of the second order at most. In other cases, the method leads to serious errors, as demonstrated by the author [
13]. These errors result from the lack of conditions providing similar values of stress at both transverse ends of the same fold. The visible result of different stress values at both transverse ends of the same shell fold is that the transverse contraction of the fold does not pass halfway along its length, on the contrary, it is shifted closer to one of these ends. The condition defined by the author is employed in his innovative method of shaping individual roof shells (monograph) [
13] and implemented in the application he developed [
18] in the Rhino/Grasshopper program used for parametric modeling of engineering objects.
The author started with experimental tests [
13] and computer analyses [
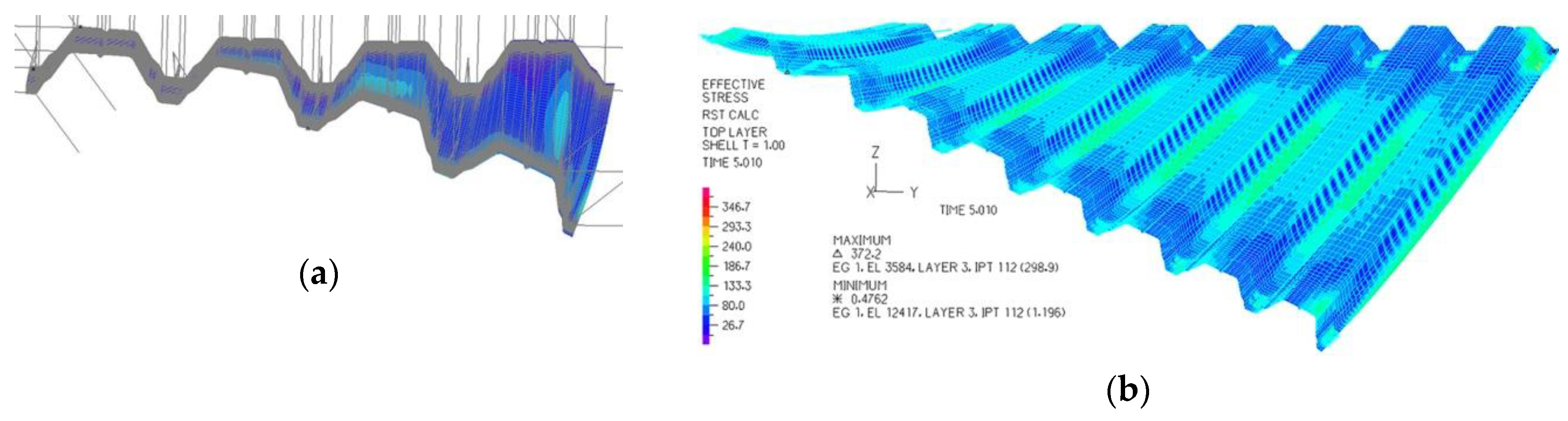
14] on static and strength work of folded sheets transformed into various shell forms and structural systems dedicated to supporting the transformed complete roof shells and their complex structures. This issue goes beyond the scope of the article. As Reichhart incorrectly accepted each shell fold as prismatic beams not cooperating with each other and having linear geometrical and mechanical characteristics, the author began his work with a preliminary understanding of the geometrical and static-strength characteristics of thin-walled folded shells. He analyzed the possibilities of modeling the transformed steel sheeting with accurate, thin-walled, folded computer models created in the ADINA program used for advanced dynamic incremental nonlinear analyses [
15] (
Figure 1). In order to accurately configure his computational models, he intends to perform experimental tests in the near future on the innovative experimental stand of his design at a laboratory hall (
Figure 3).
The present article concerns geometrical shaping the building’s free forms roofed with folded steel sheeting transformed into various shell forms [
31]. Therefore, the possibilities of shaping complex free forms, that is free form structures composed of several single free forms roofed with separate individual transformed shells, are analyzed. The justification for creating roof structures composed of several shell segments results from the geometrical and mechanical properties of the transformed folded sheets employed. The shell folds are twisted around their longitudinal axes or twisted and bent transversely to these axes. That is the reason why the transverse ends of these folds expand and their middles contract as the degree of the transformation increases [
13].
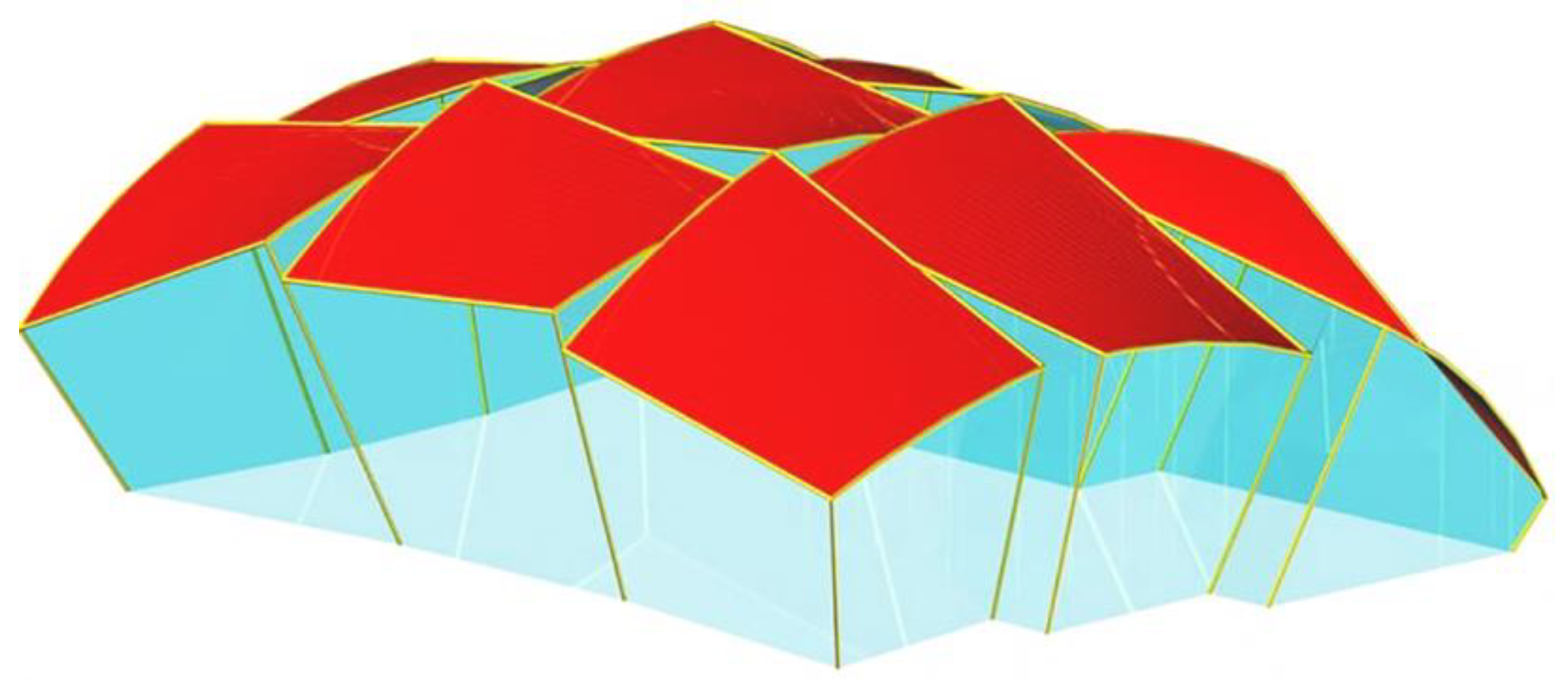
Therefore, it is not possible to combine two transformed corrugated shells along their transverse edges into one smooth shell (
Figure 2 and
Figure 4) [
32]. It is possible, however, to join both shells with transverse edges, so that there is an edge between them. The edge disturbs the smoothness of the resultant shell that becomes a structure of two shells. Most often, roof directrices separate the adjacent shell segments in the roof structure, or the adjacent shell segments are separated by additional roof or wall areas that let sunlight into the building’s interior.
The main goals of combining complete transformed shells in the roof structure include: increasing the span of the roof and entire building, integrating the roof and façade forms, increasing the visual attractiveness of the entire building free form and making it sensitive to the natural or built environments. The concept most commonly used in the shaping of transformed folded shell structures is the combination of central sections of right hyperbolic paraboloids, their halves or quadrants in various configurations along their common edges (
Figure 2a) [
25,
26].
The variety of shell structures constructed in this way is minimal. Reichhart’s actions are also limited to structures composed of several identical central segments of right hyperbolic paraboloids additionally arranged on the same plane (
Figure 2) [
9].
The author presented wide possibilities of shaping free form structures composed of many individual free forms [
13,
17]. He developed the concept and coherent rules for creating such complex structures covered with plane-walled folded elevations and multi-segment transformed shell roof structures [
18,
19]. The developed algorithms allowed a radical increase in the variety of shapes of these forms [
19,
22,
32,
33]. Based on these algorithms, the author developed three methods presented in this work. These methods differ in quality and serve to obtain mutually different and specific goals. Each of these methods is aimed at creating building structures of very specific forms, in a convenient and relatively simple way. Therefore, in the author’s opinion, only a qualitative comparison of these methods is justified.
The methods and examples presented in the sections that follow describe step by step and define objects, actions and algorithms used to solve increasingly complex issues of shaping internally consistent forms of building structures sensitive to the natural or built environments. The structure of the present article has been adopted so as to discuss step by step the specifics of the search for more and more sophisticated forms of building free forms roofed with transformed corrugated steel shell structures.
The designer may have to face, and cope with, some problems that arise from using unconventional methods for shaping general architectural forms of buildings roofed with transformed folded steel sheets and striving for relatively simple implementation of the designed innovative forms. The main task is to achieve geometrical, architectural and structural cohesion of all elements of each free form building, its shell roof in particular [
15,
32]. This aim can be accomplished by creating a parametric description of such building free forms and their specific structural systems based on the geometrical and mechanical characteristics of the transformed sheeting [
14]. The proposed methods contain geometrical descriptions and algorithms that can be employed in the creating of parametric description of the free form structures covered with plane-walled folded elevations and complex transformed steel roof shell structures and writing parametric computer applications assisting the designer in the engineering developments.
Prokopska and the author continue the problems initiated by Reichhart. They propose a method of geometrical integration of each shell roof form with plane and oblique walls to obtain innovative, attractive and multi-variant architectural forms considered as morphological systems of buildings [
18]. Some main principles of shaping complete and compound innovative free forms are the result of the cooperation between Prokopska and the author [
32].
On the basis of these principles the author invented two methods for parametric shaping of the complete architectural free forms and their complex structures covered with transformed folded shell steel sheeting [
18,
22,
31]. He assumed that the great freedom in shaping diversified transformed shell forms for roofing, resulting from great freedom in adopting shapes and mutual positions of roof directrices, can be used to integrate the entire building free form and make the form very sensitive to the natural or built environments [
32]. Consequently, to achieve more consistent and sensitive architectural free forms, he decided to fold and incline elevation walls to the vertical depending on the shape of the shell roof and entire building. He noticed that the interdependence between the efficiency of the roof sheeting transformation and the location of its contraction along the length of each shell roof fold greatly enhances the attractiveness of the entire form and the integrity of the shapes of the roof and elevation [
17].
Prokopska conducted multivariate interdisciplinary analyses of some consistent morphological systems that can be designed in harmony with the natural or man-made environments. Her research involves many interdisciplinary topics needed to develop experience in shaping various attractive architectural free forms [
34,
35]. Some of the proposed structural systems [
36,
37] can be modified and employed in the discussed building free forms [
17].
4. The Concept and the Range of the Article
The article proposes three methods for shaping complex free form buildings roofed with structures of corrugated shells made up of nominally flat folded sheets connected to each other by longitudinal edges, and transformed into spatial forms. The presentation of the methods on specific examples began with the simplest formulation based on a few very simple rules. The next two methods are increasingly complex and serve to achieve different sophisticated goals. Therefore, only a qualitative comparison of these three methods can be made. Comparison of the quantitative results achieved with the methods and included those in the specific examples presented is not justified. The quantitative comparison of these methods with the methods of other authors mentioned in the previous sections also seems unjustified.
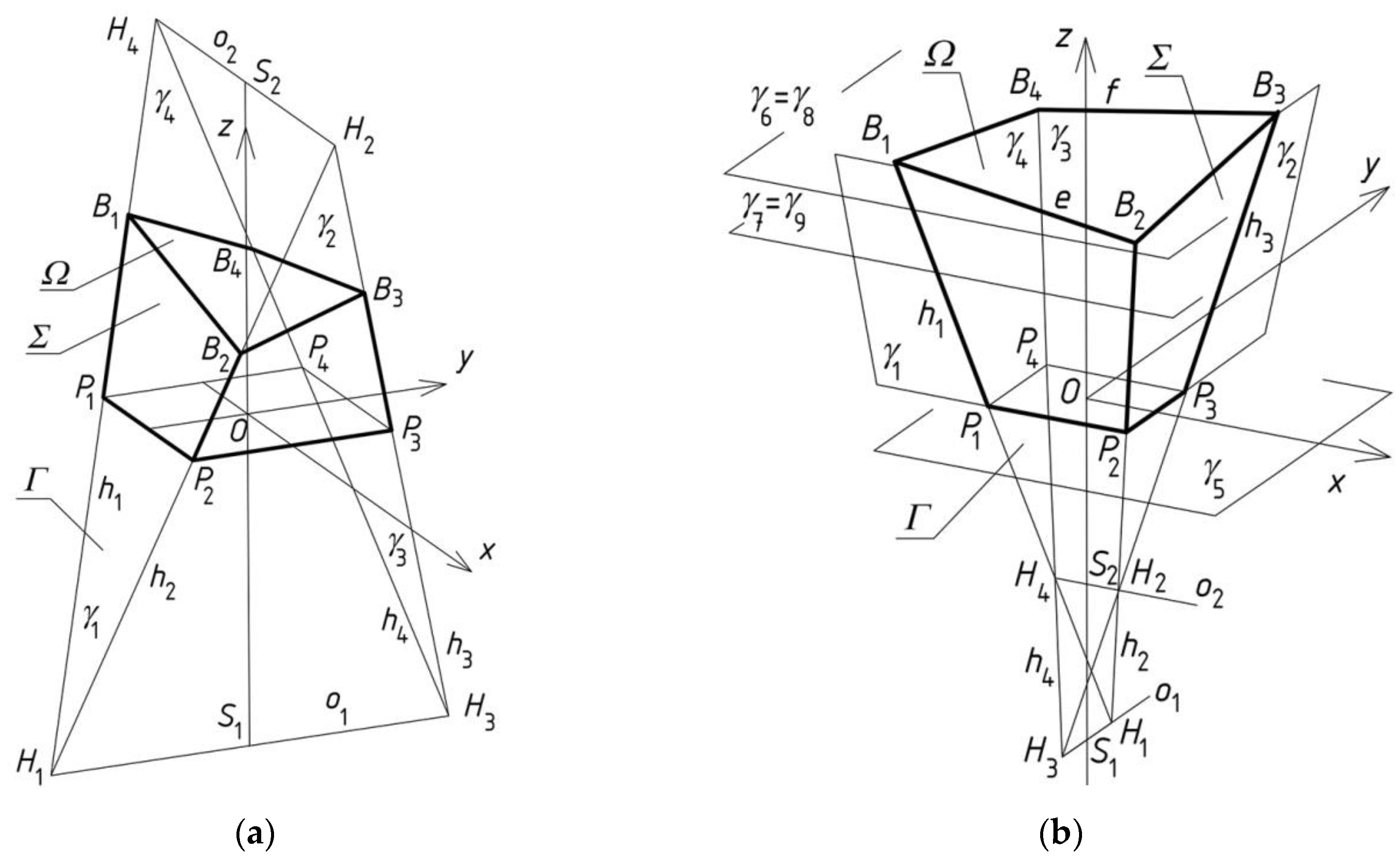
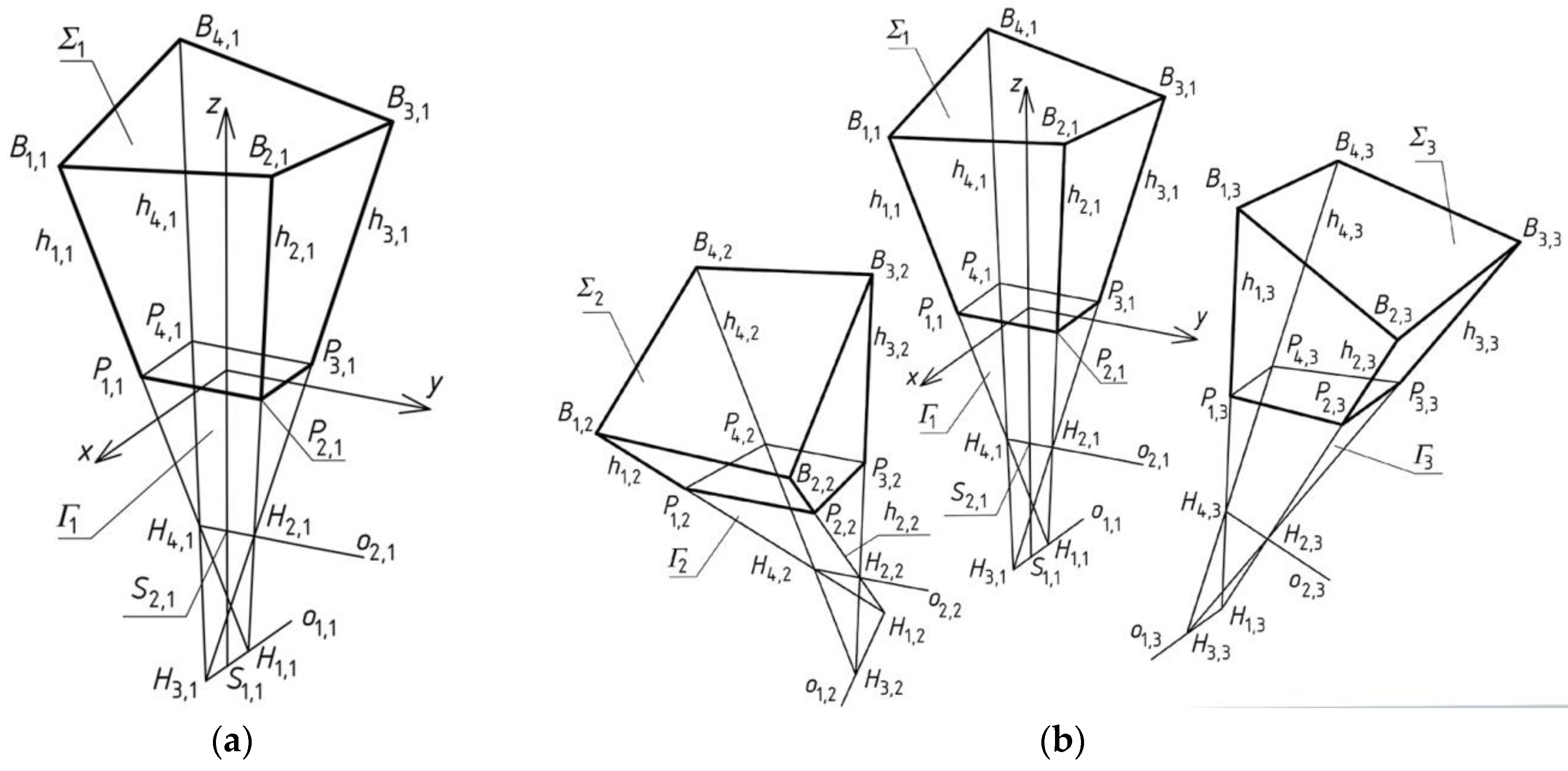
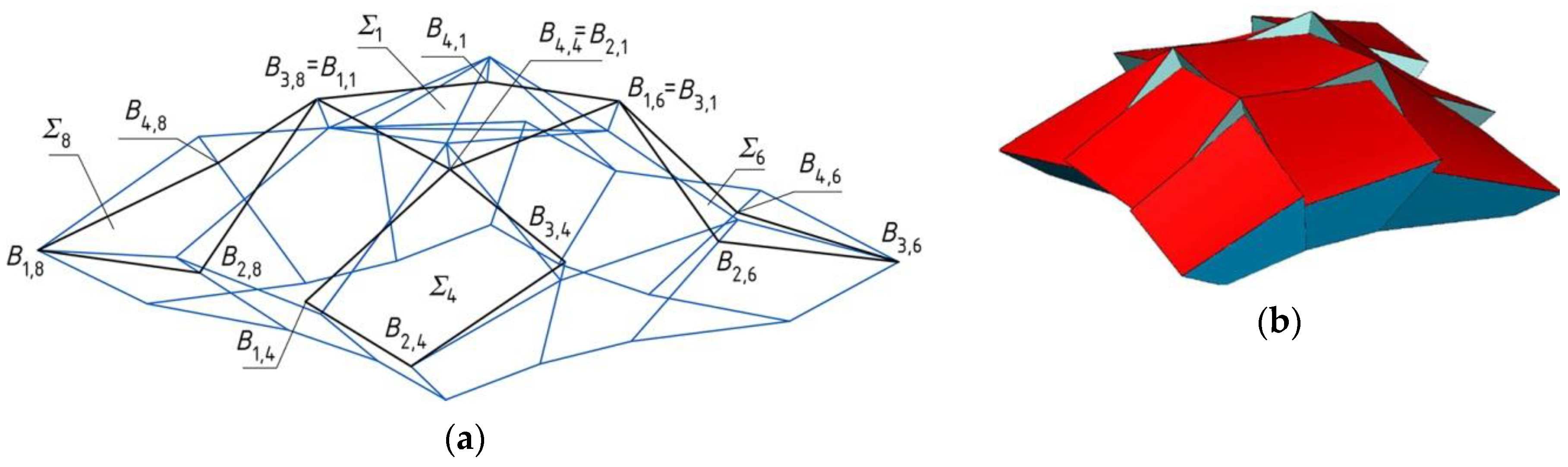
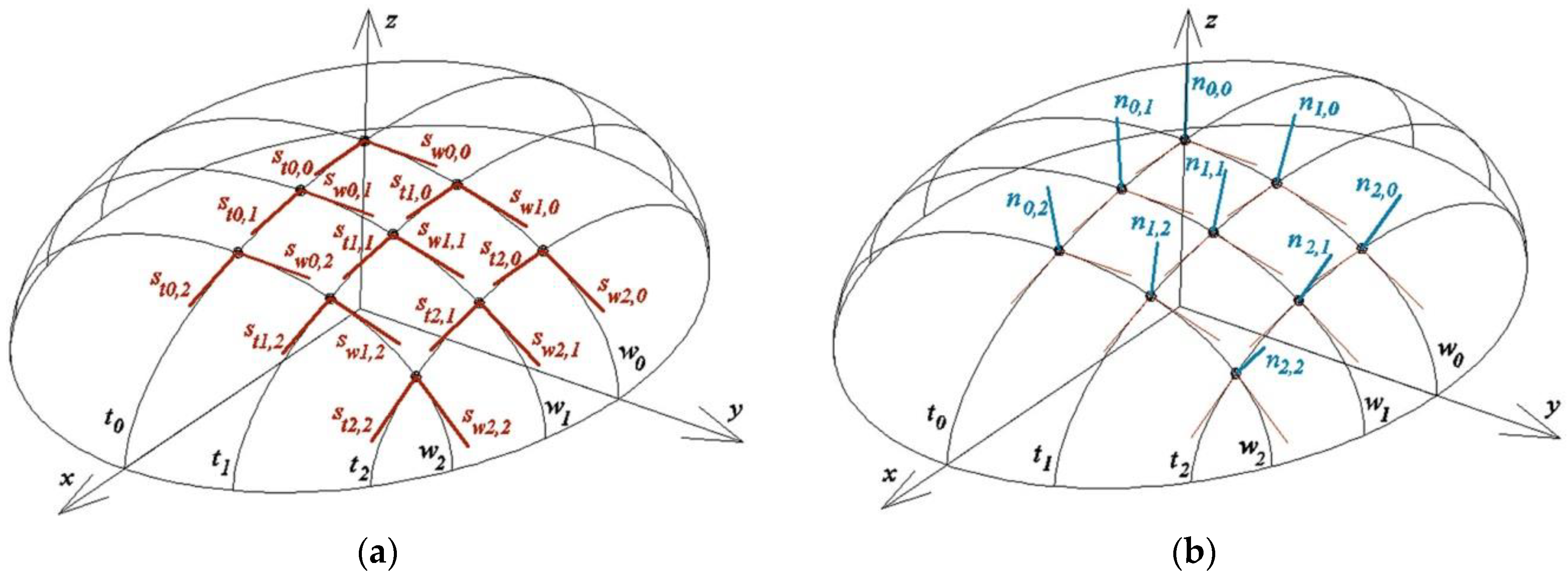
The first method formulates the basic conditions that must be met by single free forms
Σ covered with single transformed shells
Ω (
Figure 6), so that they can be combined into a structure in a simple way. The combined structure has a free form, and is roofed with a structure composed of several shell segments. The purpose of creating a roof structure is to increase the span of a complex building form in relation to the span of a single form.
The basic action of the first method is to create a model of roof eaves of each complete free form. The model is a closed spatial quadrangle Bev, whose geometrical properties depend on the form of rectangular, nominally flat sheets folded in one direction and transformed into a shell form. Points Bi are four vertices of Bev.
Since the folded sheets are rectangular, the angles between two adjacent sides having shared ends at corners
Bj (
j = 1–4) of the spatial quadrangle
Bev are very close to right angles, and the lengths of each pair of the opposite sides of the quadrangle are equal to each other or differ very little. Two opposite sides of the aforementioned quadrangle, corresponding to the transverse edges of a transformed shell are almost equal to each other. Furthermore, if the transverse edges of the shell are not obliquely cut [
13], the lengths of all folds of the shell are identical, so the second pair of the opposite sides of this quadrangle is formed from two skew straight sections of equal length. The transverse fold ends are often cut obliquely to adjust the transverse edges of the shell to the direction of the roof directrices. However, the cuts are only minor and cause little variation in the fold’s length, followed by a slight difference in the lengths of the opposite sides and the measures of the corner angles of the aforementioned spatial quadrangle.
As all folds of each transformed roof shell are almost always twisted along the longitudinal axes, expanded at the transverse ends and contracted at half-length, each quadrangle cannot be flat; on the contrary, it must be spatial. Each pair of its two opposite sides is created as two skewed lines. The lengths of these sides must be precisely calculated based on the border conditions adopted for the roof’s corrugated shell. The conditions are determined mainly by the shape and mutual position of the roof directrices [
14,
18].
For the above models and in the initial example presented in
Section 5, the directrices are adopted as two straight segments
e =
B1B2 and
f =
B3B4 in order to obtain a relatively simple description of the first method. The
Bev spatial quadrilateral is made up of two pairs of skew straight sections. One pair of these sections is formed of the
e and
f directrices corresponding to the shell’s edges running transversely to the shell fold directions. The second pair of the opposite straight sections corresponds to the longitudinal edges of the shell belonging to the extreme folds.
Since the directrices are skewed straight lines, the calculated supporting conditions of the subsequent folds in a shell, mainly affecting the fold’s twist, are varied. As a result, the subsequent folds have different lengths of their supporting lines, twists and lengths. The author attempts to use quadrangles Bev whose shapes are symmetrical towards an axis skew with respect to each side of quadrangle Bev in order to obtain pairs of its opposite edges of equal length and congruent apex angles. Such an operation leads to a symmetrical complete roof shell, identical inclination of each shell fold to both directrices and identical supporting conditions of the fold at both ends.
In order to build a very simple free form whose roof eaves are spatial quadrangle
Bev characterized by the aforementioned properties, the definition of four planes
γi modeling four façade walls of this form is most convenient (
Figure 6). In these planes, four straight sections of
Bev with common vertices are defined. On the basis of these elements, the author defined a simplified model of a single free form, and called it the reference tetrahedron
Γ [
13]. He distinguished three basic types of the reference tetrahedrons. Two of them are presented in
Figure 6.
He also drew attention to the following basic geometrical properties of each reference tetrahedron
Γi of the free form structure
Γ, where
i indicates the number of all reference tetrahedrons used for creating free form structure
Σ. For each reference tetrahedron, two of the six edges formed as a result of the intersection of the above four planes are called axes
o1 and
o2 (
Figure 6), and the other are side edges
hj (
j = 1–4). The axes are the intersecting lines of two opposite planes of the tetrahedron. The side edges are the intersection of the adjacent planes of tetrahedron
Γi. The axes intersect with the side edges at points
Hj called the vertices of
Γi. Vertices
Bj of each quadrangle
Bevi are defined at side edges
hj at adequate distances from the respective vertices
Hj. Four sides of
Bev are created on the basis of four vertices
Bj.
In order to obtain spatial network
Γ composed of many complete reference tetrahedrons
Γi, the reference tetrahedrons are arranged so that one wall of each two neighboring tetrahedrons is contained in a common plane. A detailed description of the relations between the axes, edges and vertices of neighboring reference tetrahedrons
Γi in
Γ is presented in
Section 5 concerning the first method proposed by the author.
The method enables the full recognition of the possibilities of combining the reference tetrahedrons into one spatial reference network Γ whose unconventional and innovative form is determined by the specific properties of these tetrahedrons Γi. The method leaves the designer complete freedom to give the shape of the spatial reference network Γ and the roof shell structure Σ. As a result, the network may be regular or not. The arrangement of the shell segments in the three-dimensional space may be regular or not. The forms of the individual shells may be regular and similar to each other or not, depending on the geometrical properties of the subsequently adopted complete free forms.
As reference network Γ is used to create regular free form building structures roofed with regular shell roof structures, it is necessary to define additional rules supporting the designer’s spatial reasoning and intuition. Therefore, the author developed the second method of shaping the aforementioned structures roofed with transformed curved shells. In order to impose the regularity of a complex free form and its roof shell structure, a so-called reference surface is introduced into the method. According to the method, the shapes and arrangement of the reference tetrahedrons in the three-dimensional space should be determined on the basis of the reference surface.
Using this method, it is possible to exploit specific geometrical properties of the reference surface, such as its planes of symmetry, so that the symmetry axes of the largest possible number of reference tetrahedrons Γi are contained in these planes. Moreover, relatively simple operations are possible to obtain the symmetry of quadrangles Bevi contained in Γi. In this method, however, reference tetrahedrons Γi are arranged on the basis of the reference surface in an intuitive manner to a large extent, whereby their construction is related to the curvatures of the reference surface only to a small extent. This may lead to unjustified differences in the shapes and irregular distribution of the reference tetrahedrons in relation to the reference surface, so certain additional rules are needed. Therefore, the author decided to employ some planes normal to the reference surface for selected planes of the reference tetrahedrons.
In order to prevent inefficient and irregular forms of the reference network and shell roof structure, the author created the third method and introduced another condition allowing him to optimize the regular shapes and arrangement of the reference tetrahedrons in relation to the arbitrary reference surface. He imposed a constraint that the directions of all side edges of the reference network should be close to the directions of the adopted normals of the reference surface at the points of the intersection of the above side edges and the reference surface within the adopted optimization accuracy. Such operations, undertaken by the author, result in the fact that the projections of the single roof shell segments on a reference surface, in the directions compatible with the directions of selected straight lines normal to this surface, do not overlap each other. Instead, they form a continuous two-dimensional surface. In other words, the projections are not disjoint and do not create discontinuous areas.
The third method allows an optimization of the positions and directions of the side edges hi,j of reference tetrahedrons Γi,j relative to the arbitrary reference surface. The optimization is carried out using the aforementioned straight lines ni,j normal to the reference surface. The optimizing condition is the allowable size of the deviation of all edge sides from the corresponding normals to the reference surface.
The basic difficulty in maintaining the above optimizing condition is the fact that two subsequent straight lines ni,j normal to the reference surface are skew lines, in contrast to each pair of neighboring edges hi,j of each reference tetrahedron Γi,j, which intersect each other at the appropriate vertex Hi,j of Γi,j. Therefore the perfect approximation or replacement of ni,j with hi,j is impossible, and the optimization of the side edges hi,j in relation to the reference surface is needed. In the third method, the author therefore included an innovative way of looking for a regular spatial network composed of regular reference tetrahedrons whose side edges hi,j, intersecting each other at vertices Hi,j of reference tetrahedrons Γi,j, are optimized and defined on the basis of not crossing each other’s normals with regard to the reference surface.
5. Structures as Compositions of Many Regular Free Forms
The following steps, activities and objects have been identified in the algorithm of the first developed method for shaping complex free form structures. In the first step, some actions are undertaken to build the general form of a single free form with the help of reference tetrahedron Γ1, whose four walls model four façade walls of the designed free form.
In the second step, the roof of a single free form Σ1 is modeled as a sector of a smooth regular warped surface. The roof is made of nominally flat corrugated sheets transformed effectively into a shell shape; that is, the shell shape resulting from this transformation should contract at half-length of each fold, transversally to the fold directions, which positively affects the static-strength work of the folds in the shell.
In the third step of the algorithm, a method for determining the positions of several individual forms and combining these forms into a structure sensitive to the predicted natural or man-made environments is carried out. The complex building free form is, therefore, the sum of several individual free forms appropriately set together with the common façade walls. The roof of the building structure created this way is a shell roof structure composed of several smooth shell segments.
In the last step of the algorithm, modification of the forms of the roof and façade of the previously achieved structure is possible. This modification is based on displacements of selected roof edges or façades, in the planes of the reference tetrahedrons employed. The purpose of this modification is to make the building structure more sensitive to the built or natural environments. Complete reference tetrahedron
Γ1 (
Figure 7a) is formed by means of four vertices:
H1,1,
H2,1,
H3,1,
H4,1. To determine the positions of the above four vertices, two skewed straight lines
o1,1 and
o2,1 located in distance
dn1 and perpendicular to each other are assumed. Middle point
S2,1 of
H2,1H4,1, is lain in the distance
dp1 from the origin
O of the orthogonal coordinate system [
x,y,z]. A straight line
z perpendicular to
o1,1 and
o2,1 intersects these lines at points
S1,1, and
S2,1. Finally, the vertices
H3,1,
H1,1 are measured along
ot1,1 in the distances
d3,1 and
d1,1 from
S1,1, and the vertices
H4,1,
H2,1 are measured along
o2,1 in the distances
d4,1 and
d2,1 from
S2,1.
Lines
o1,1, and
o2,1 are called the axes of
Γ1. However, straight lines:
h1,1(
H1,1,
H4,1),
h2,1(
H1,1,
H2,1),
h3,1(
H2,1,
H3,1),
h4,1(
H3,1,
H4,1) are said to be the side edges of
Γ1. In order to obtain the vertices:
P1,1,
P2,1,
P3,1,
P4,1 of a planar base of
Γ1,1, plane (
x, y) ⊥
z is passed through point
O located in the distance
dp1 from
S2,1, (
Figure 7a). Vertices
B1,1,
B2,1,
B3,1,
B4,1 of the free form
Σ1 are constructed on
h1,1,
h2,1,
h3,1,
h4,1 in the distances
dh1,1,
dh2,1,
dh3,1,
dh4,1 from the above points
Pi,1 (
i = 1–4). Values of all above input data are presented in
Table 1. The above four values are adopted so that the eaves of the resultant
Σ1 are characterized by two pairs of opposite segments of equal length to obtain a central sector of an oblique hyperbolic paraboloid [
19].
To determine the subsequent reference tetrahedrons of reference tetrahedral structure
Γ being sought, one should create nine complete free forms
Γj (
j = 1–9) of
Γ. In order to create reference tetrahedron
Γ2, four vertices
Hi,2 (
i = 1–4) (
Figure 7b) should be adopted as previously the vertices of
Γ2. In order to create free form
Σ2, vertices
Bi,1 and
Pi,1 (
i = 1–4) should be adopted on the basis of the appropriate parameters as previously.
The same action must be performed to obtain reference tetrahedron
Γ3 and free form
Σ3 (
Figure 7b). However, for the designed reference polyhedral structure
Γ, other activities are undertaken to simplify the assembly of reference tetrahedrons
Γj (
j = 1–9) into one structure
Γ and complete free forms
Σj (
j = 1–9) into one structure
Σ.
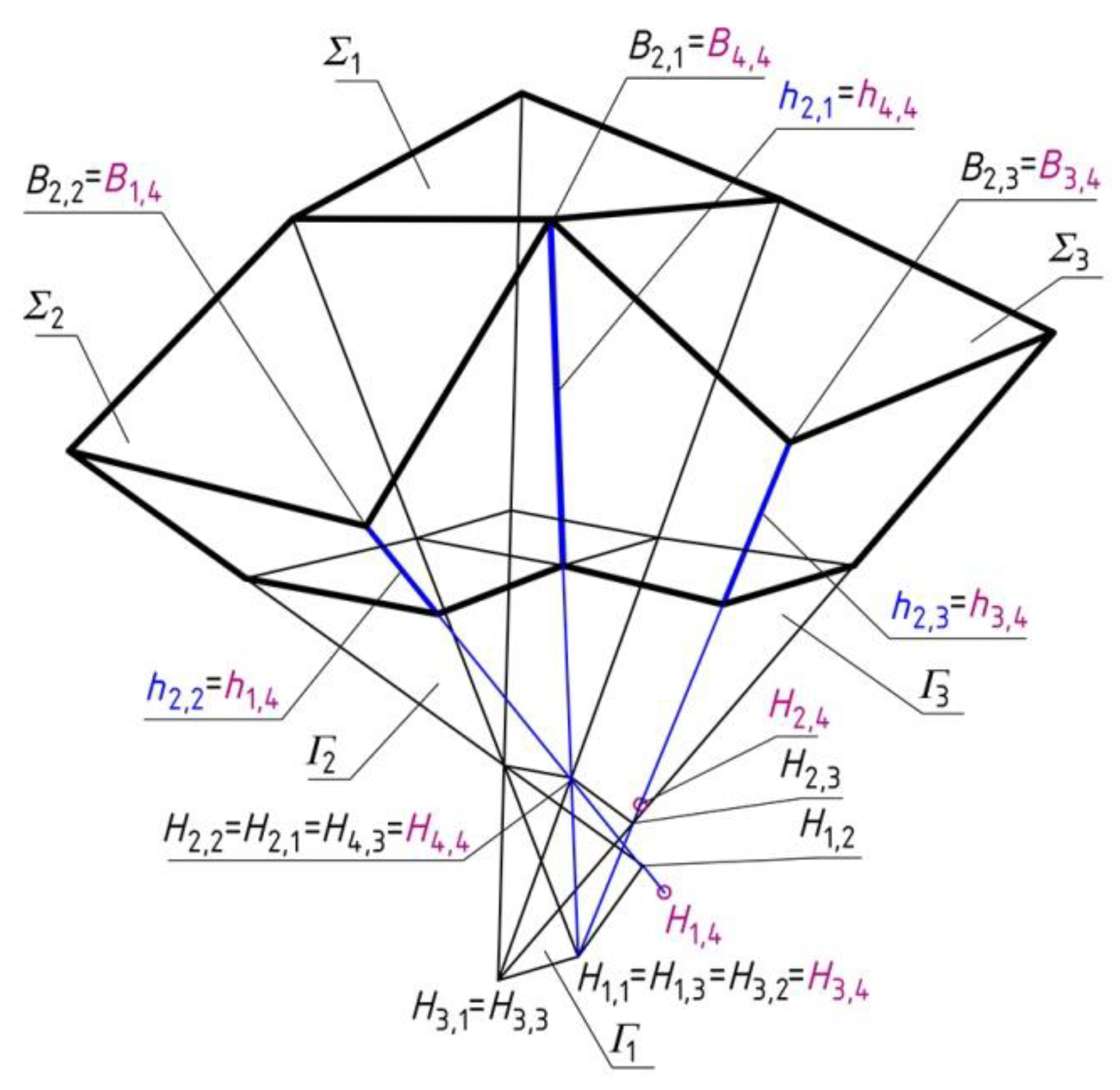
According to the aforementioned concept, it is taken that
H3,2 =
H1,1,
H2,2 ∈
h1,2,
H4,2 ∈
h1,1 (
Figure 8). It is assumed that
H2,2 =
H2,1,
H4,2 =
H4,1. To obtain
Γ2 symmetrical to
Γ1 towards plane
ξ2 (
H3,2,
H2,2,
H4,2), vertex
H1,2 being sought has to be symmetrical to
H3,1 towards
ξ2.
The transformation related to plane ξ2 of symmetry is denoted as L2, so H1,2 = L2(H3,1), B1,2 = L2(B4,1), B2,2 = L2(B3,1), Γ2 = L2(Γ1). In addition, B3,2 = B2,1, B4,2 = B1,1, P3,2 = P2,1, P4,2 = P1,1 and P1,2 = (H1,2, H4,2) ∩ (x, y) and P2,2 = (H1,2, H2,2) ∩ (x, y).
Reference tetrahedron Γ3 is created in the same way as Γ2 so that plane ξ3 (H4,3 = H2,1, H3,3 = H3,1, H1,3 = H1,1) is used for transformation L3. Thus, B3,3 = L3(B4,1), B2,3 = L3(B1,1), Γ3 = L3(Γ1).
Γ2 and
Γ3 are located orthogonally in
Γ. The reference tetrahedron
Γ4 being sought is located diagonally in
Γ. The way of creating
Γ4 is different from
Γ2 and
Γ3 because the locations of two its vertices are known:
H4,4 =
H2,1,
H3,4 =
H1,1 (
Figure 9). The searched vertices
H1,4 and
H2,4 have to belong to
h1,4 =
h2,2 and
h3,4 =
h2,3, respectively.
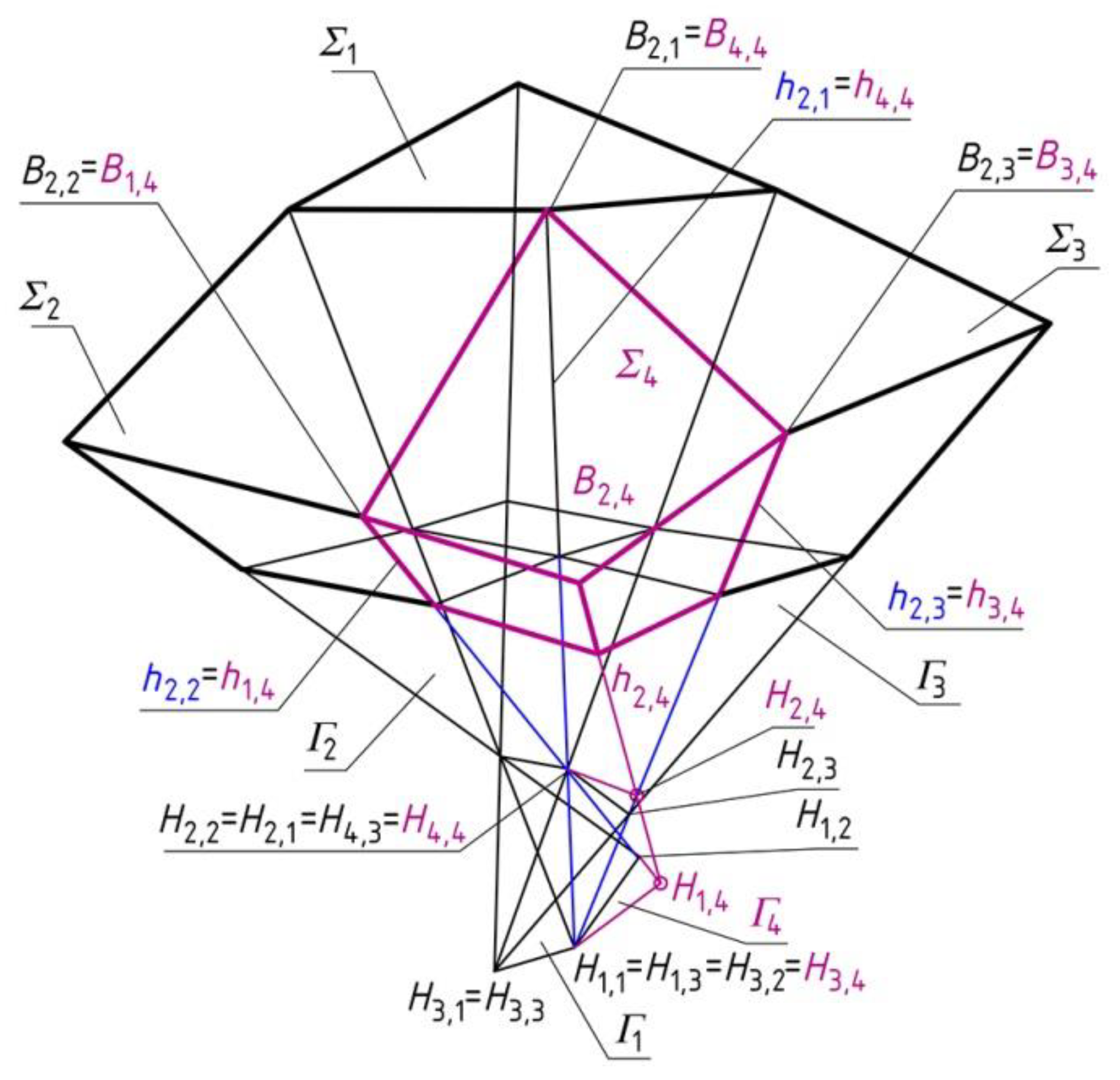
To obtain
Σ4, vertices
H1,4 and
H2,4 are accepted at side edges
h1,4 and
h3,4 (
Figure 10). It is assumed that
B4,4 =
B2,1,
B3,4 =
B2,3,
B1,4 =
B2,2. The position of
B2,4 is determined at
h2,4(
H1,4,
H2,4). Point
P2,4 is the intersection of
h2,4 and the base plane (
x,y). The data used in the present example are given in
Table 2 and
Table 3.
In addition, the following dependences should be adopted:
B1,3 =
B2,1,
B4,3 =
B3,1,
P1,3 =
P2,1,
P4,3 =
P3,1,
B1,4 =
B2,2,
B3,4 =
B2,3,
B4,4 =
B2,1,
P1,4 =
P2,2,
P3,4 =
P2,3,
P4,4 =
P2,1,
H1,3 =
H1,1,
H3,3 =
H3,1,
H4,3 =
H2,1,
H3,4 =
H3,2,
H4,4 =
H2,1. Other vertices are symmetrical towards plane (
x,z) or (
y,z). Thus, in order to obtain the entire reference structure
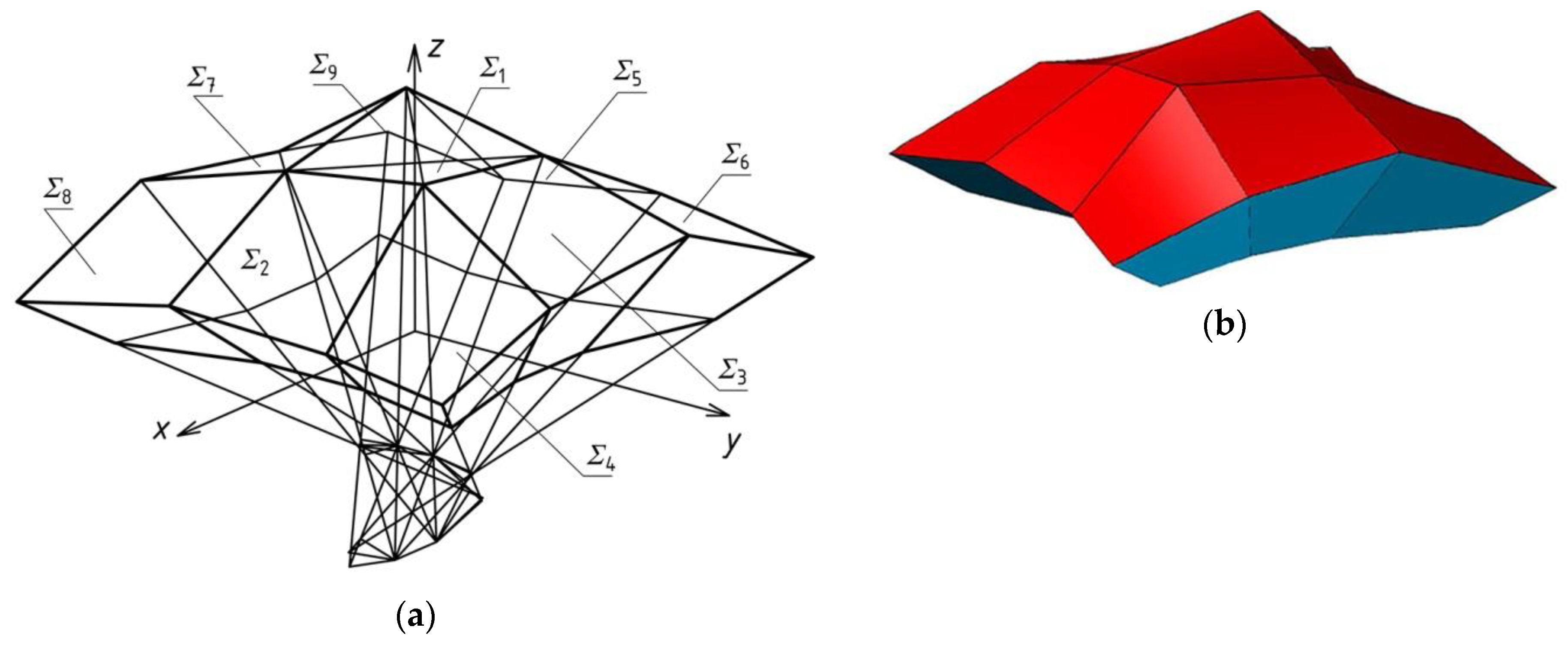
Γ and free form structure
Σ, four reference polyhedrons
Γi (
i = 1–4) and four free forms
Σi have to be transformed towards these planes of symmetry (
x,z) and (
y,z) into new positions of
Γr and
Σr (
r = 5–9). The final reference structure
Γ and free form structure
Σ, are shown in
Figure 11.
It is worth stressing that the reference tetrahedrons located orthogonally or diagonally in Γ do not have to be congruent to each other, so the form of the final Γ and Σ can take unsymmetrical shapes. Thus, the possible shapes of Σ may be really free, diversified and sensitive to the built and natural environments.
The free form presented previously is the basis of creating some derivative forms. The derivative free forms are shaped as a result of displacing or rotating some selected side edges of the basic free form in selected planes of its reference structure. The basic form is covered with the continuous shell structure (
Figure 11b), whose individual shells are divided by shared edges locally disturbing the smoothness of the structure and forming a regular pattern on the roof. In addition, the elevations have relatively simple shapes.
The first derivative form is constructed as a result of displacing the selected vertices belonging to five roof border quadrangles located diagonally in
Γ and distinguished by means of a black thick line in
Figure 12. Their selected vertices
Bi,j are displaced along the relevant side edges
hi,j in the distances equal to
dh = 3000.0 mm. The values of the coordinates of the points used are presented in
Table 4.
For the aforementioned free form, the parametric shape characteristics can be improved and extended by new shape parameters, for example describing the proportion of the roof discontinuity areas intended for windows to the area of the entire shell roof. Propositions of such additional shape parameters go beyond the scope of the paper.
The second derivative form (
Figure 13) is created as a result of: (a) the translations of all vertices of the eaves of
Σ1 discussed earlier, along the relevant side edges of
Γ1 in equal distances
dh; and (b) the translations of the selected elevation side edges along the selected axes of the reference structure in the distances
do1,2 =
H3,2H1,1 = 4534.1 mm and
do2,1 =
H2,3H4,3 = 3126.7 mm, so that these side edges will be contained in the planes of control compositions
Γ2 and
Γ3. The values of the parameters used are as follows:
B3,1B4,4 =
B3,1 B2,1 =
dh1,1 = 3000.0 mm. The values of the coordinates of the transformed vertices of the eaves are included in
Table 5.
7. Optimized Structures Based on Regular Reference Surface
A non-rotational ellipsoid is used as a reference surface in the example presented below. The equation of this ellipsoid
σ is the same as previously (1), but
a = 24,000 mm,
b = 18,000 mm,
c = 11,000 mm. The gable wall of the designed structure can be located in one plane (
Figure 20a) or divided into two planar pieces symmetrical towards the plane (
x,
z) (
Figure 20b). The both forms are presented in the first part of the section. The shell roofs corresponding to these forms take very simple shapes. The roofs are created as the sums of a few shell strips whose directrices became the ellipses of the intersection of the gable wall plane or other almost vertical planes with the aforementioned reference ellipsoid
σ.
The discussed method proposes to create more extended forms based on the reference surface
σ, covered with compound shell structures supported by walls formed from many planar or shell segments, as shown in
Figure 21. As a result, innovative, attractive and integrated building forms can be provided.
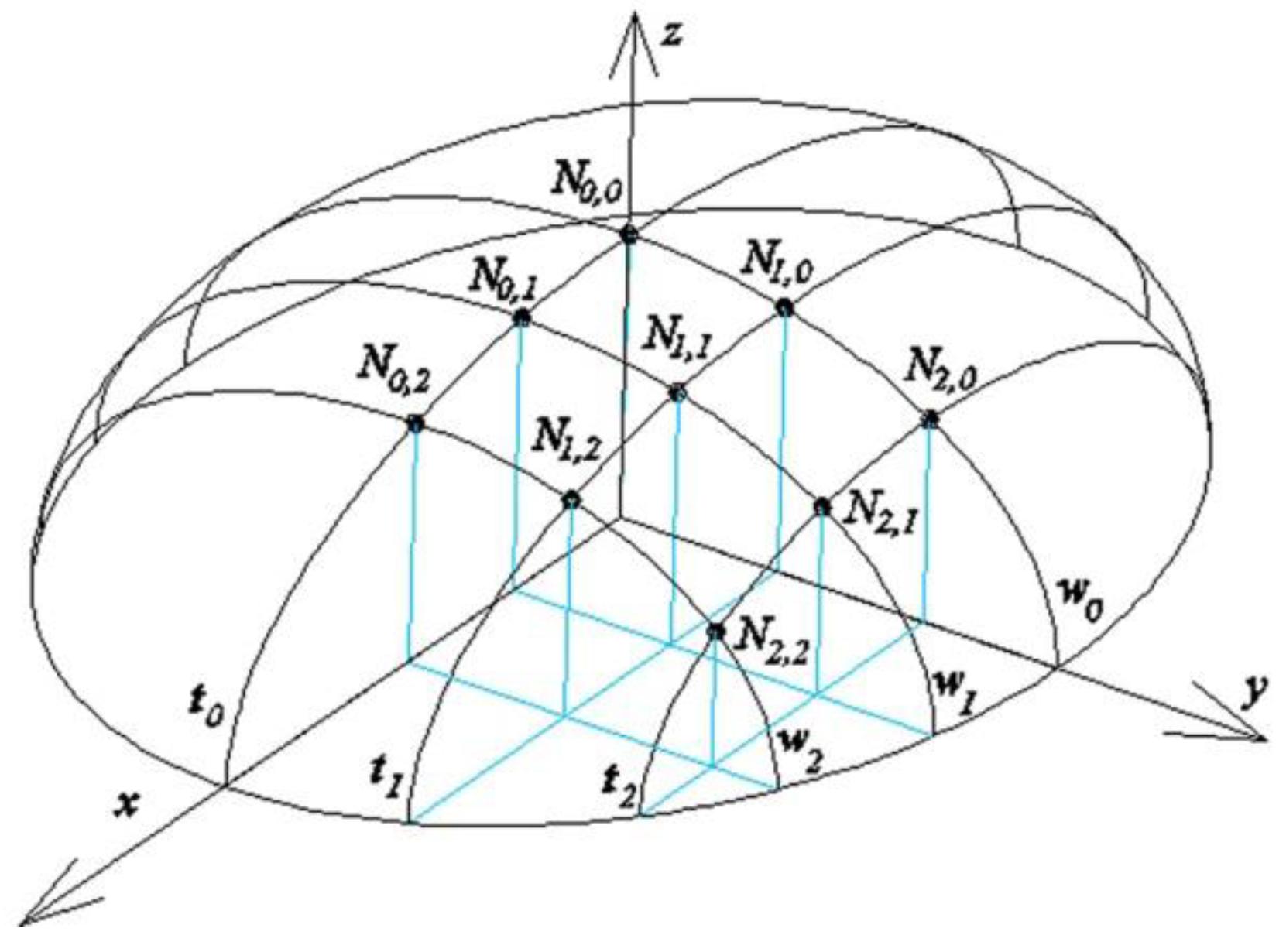
If the number of the planar pieces of the gable wall is increased, then the integration of this wall with the entire building may be improved. In the next example, a regular reference structure is created. For that purpose, a finite number of points
Ni,j is defined on the reference ellipsoid with the help of ellipses
wi and
ti (
i = 0, 1, 2) contained in vertical planes (
Figure 22). The coordinates of the considered points
Ni,j (
i = 0, 1, 2,
j = 0, 1, 2) selected on the reference surface
σ are included in
Table 6.
The parametric equations of the considered ellipses
ti of reference ellipsoid
σ are given by:
where
at =
a,
ct =
c,
τi—the interdependent variable,
yNi,0—
y-coordinate of point
Ni,0 (
i = 0, 1, 2). The parametric equation of the ellipses
wj of
σ are given by:
where
bw =
b,
cw =
c,
ωj - the interdependent variable,
xNi,0 –
x-coordinate of point
N0,j (
j = 0, 1, 2).
One plane of the reference network can be created for each pair of two subsequent lines {
ni,j,
ni+1,j} normal to
σ (
Figure 23) to increase the integration degree of the entire structure with reference surface
σ. On the basis of the above equations, straight lines
stNi,j and
swNi,j tangent to ellipses
ti and
wj of
σ at
Ni,j are determined, (
Figure 23a). Based on these tangents, the directional vectors of straight lines
ni,j normal to this ellipsoid at
Ni,j were calculated. The values of the components [
lni,j, mni,j, mni,j] of these directional vectors are given in
Table 7.
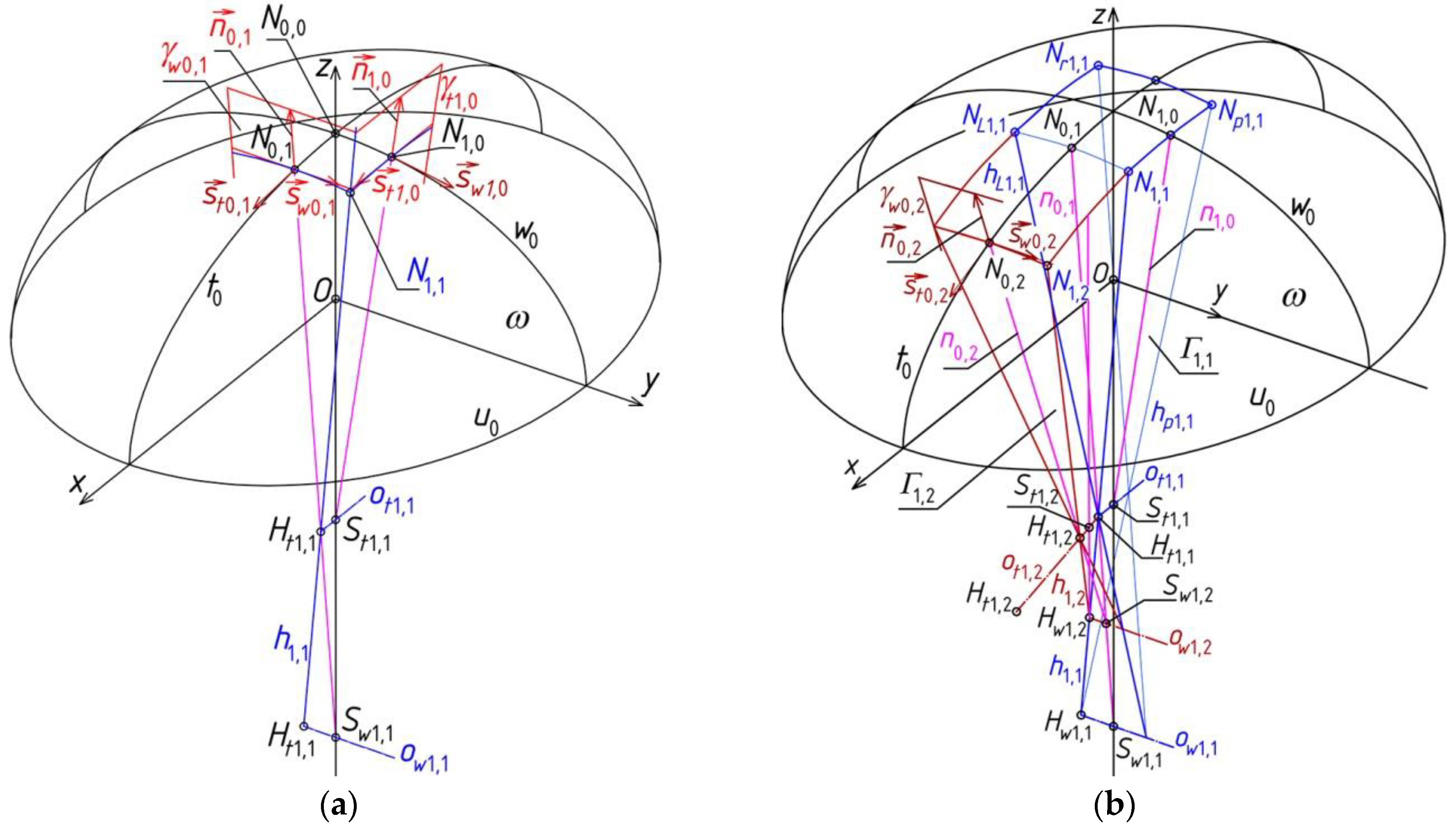
In order to construct the first tetrahedron
Γ1,1 of reference network
Γ, whose side edges
Hi_1,1 (
i = 1–4) pass through points
N0,0,
N1,0,
N0,1,
N1,1,, four pairs of straight lines {
n0,0,
n0,1}, {
n0,1,
n1,1}, {
n1,0,
n1,1} and {
n0,0,
n1,0} normal to
σ at these points must be considered. Next, for each pair of these normals, the intersection of both lines with a straight line perpendicular to them should be constructed. For the pair {
n0,1,
n1,1}, there is a straight line
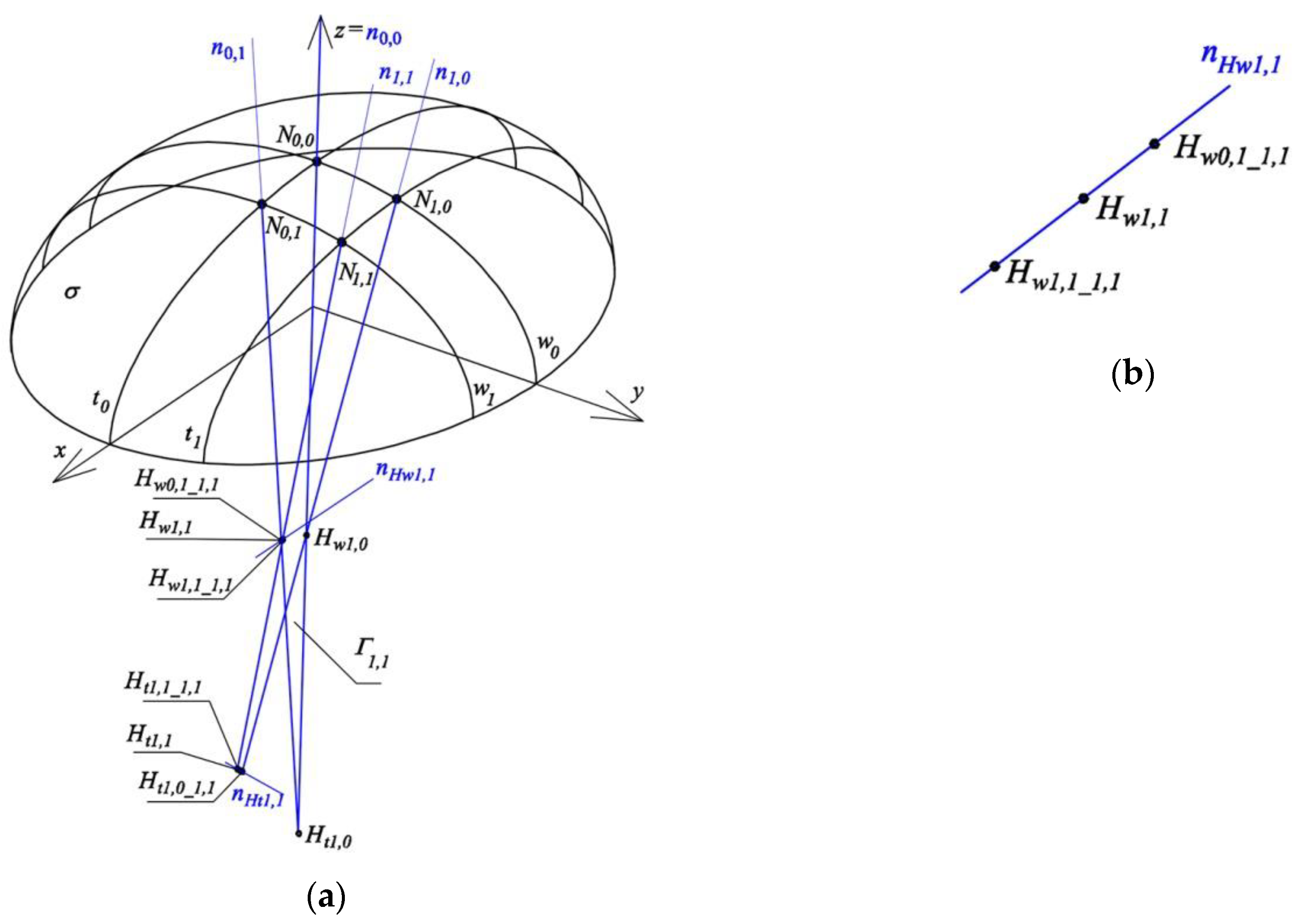
nHw1,1 and points
Hw0,1_1,1 ∈
n0,1 and
Hw,11_1,1 ∈
n1,1. For the pair {
n1,0,
n1,1} there is the straight line
nHt1,1 and points
Ht1,0_1,1 ∈
n1,0 and
Ht1,1_1,1 ∈
n1,1 (
Figure 24).
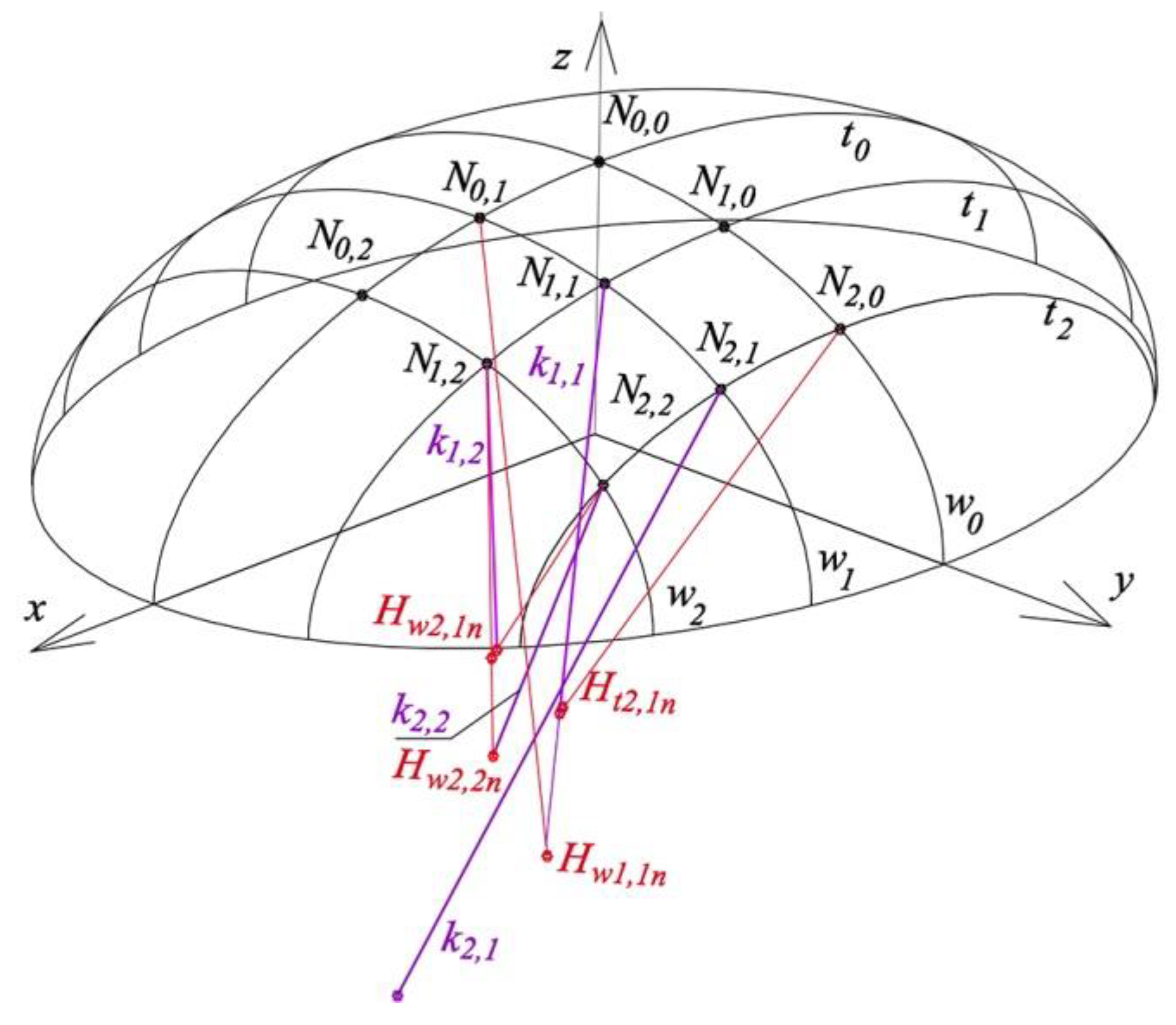
For the other two cases: {n0,0, n0,1}, {n0,0, n1,0}, both straight lines of each of these pairs are coplanar, and therefore these lines cross each other at vertices Hw0,0 and Ht0,0 of the reference tetrahedron Γ1,1, and it is not necessary to carry out relevant constructions. The notation of point Hw0,1_1,1 should be interpreted as follows: (a) symbol w indicates that this element is related to curve wj; (b) subscript 0,1 indicates that this point is referred to N0,1; and (c) subscript 1,1 means that the considered point is used for determining Γ1,1.
A tetrad of planes ζtN0,1, ζwN1,0, ζtN1,1, ζwN1,1 of tetrahedron Γ1,1 is formed from two planes (x, z), (y, z) of the orthogonal coordinate system [x,y,z] and two planes defined by the following triads of points: (N1,0, N1,1, Ht1,1), (N0,1, N1,1, Hw1,1), where Ht1,1 is the middle point of section Ht1,0_1,1Ht1,1_1,1, however, Hw1,1 is the middle point of section Hw0,1_1,1Hw1,1_1,1. Ultimately, edges h0,0_1,1 = ζtN0,1 ∩ ζwN1,0, h0,1_1,1 = ζtN0,1 ∩ ζwN1,1, h1,0_1,1 = ζtN1,1 ∩ ζwN1,0, h1,1_1,1 = ζtN1,1 ∩ ζwN1,1 are constructed. They are preliminary approximations of the side edges of the polyhedral reference structure Γ, and take positions close to the positions of straight lines ni,j normal to the arbitrary reference ellipsoid σ at points Ni,j (i = 0,1, j = 0,1).
Each two subsequent straight lines from {h0,0_1,1, h0,1_1,1, h1,0_1,1, h1,1_1,1} passing through adjacent points Νi,j of ellipsoid σ intersect at four corresponding vertices {Hw1,0, Ht1,0, Hw1,1, Ht1,1} of the tetrahedron Γ1,1. Points Ni,j (i = 0,1, j = 0,1) together with vertices Ni,j define the four planes ζtN0,1, ζwN1,0, ζwN1,1 and ζtN1,1 of Γ1,1.
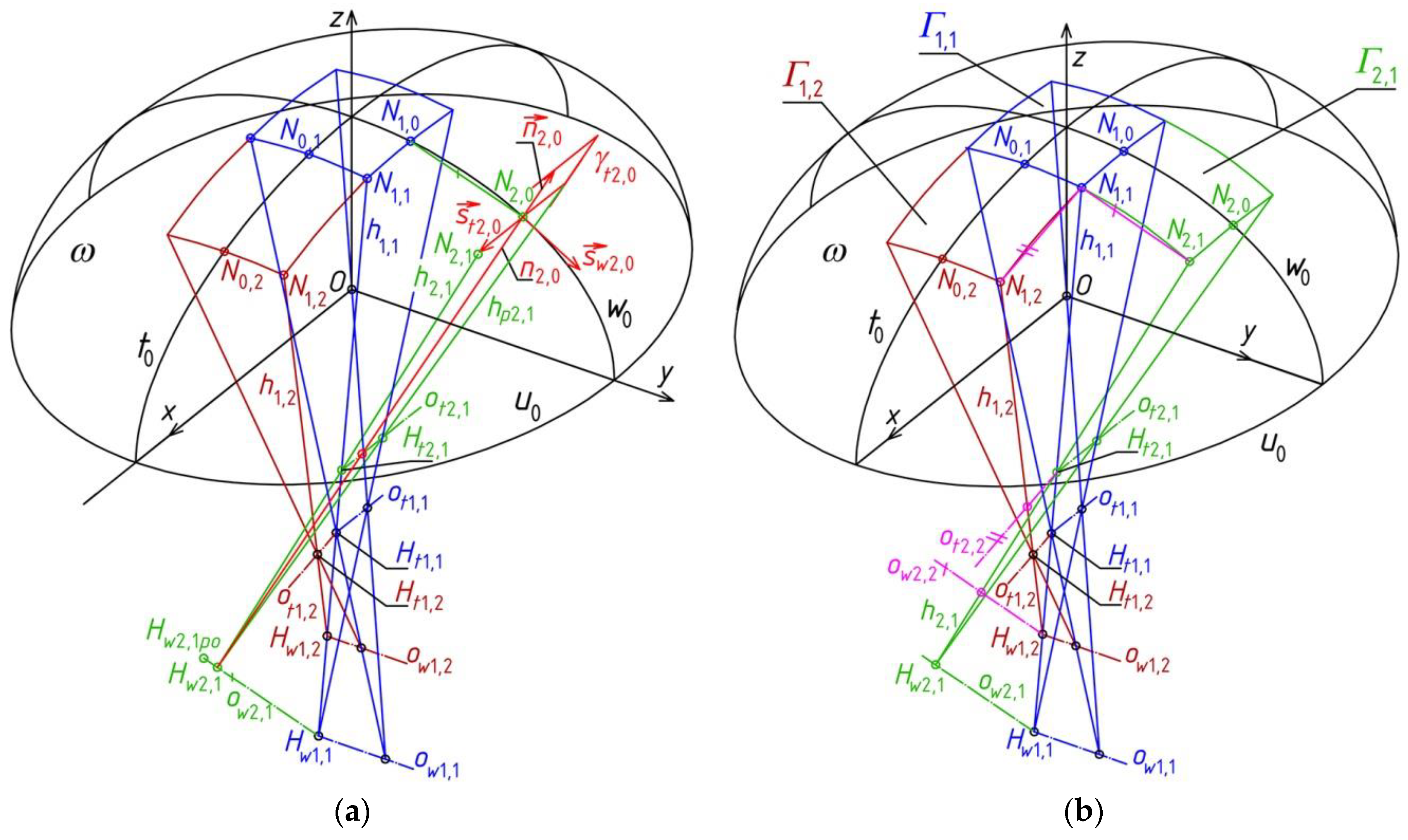
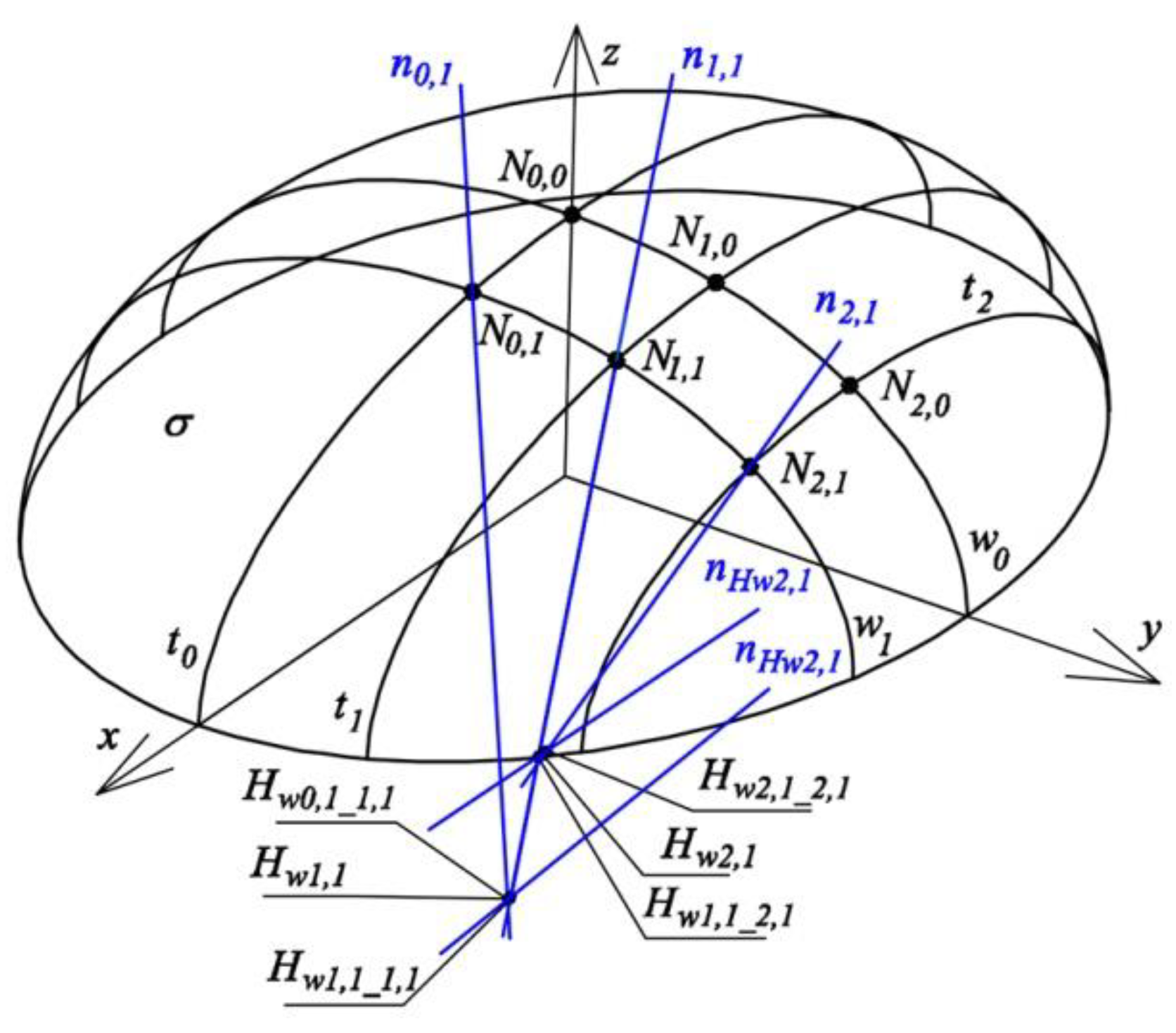
In order to construct the second tetrahedron
Γ2,1, whose side edges pass through points
N1,0,
N1,1, N2,1,
N2,0 (
Figure 25), it is necessary to take into account the previously considered pair {
n1,0,
n1,1} and next three pairs of straight lines {
n2,0,
n2,1}, {
n1,1,
n2,1} and {
n1,0,
n2,0}.
Next, for each of these pairs, the points of both straight lines intersecting with a straight line perpendicular to them should be constructed. In the case of pair {
n1,1,
n2,1}, these are points
Hw1,1_2,1 ∈
n1,1 and
Hw,21_2,1 ∈
n2,1 and straight line
nHw2,1 (
Figure 25). In the case of pair {
n2,0,
n2,1}, these are points
Ht2,0_2,1 ∈
n2,0 and
Ht2,1_2,1 ∈
n2,1. Straight lines {
n1,0,
n2,0} are coplanar and intersect at vertex
Hw2,0 of tetrahedron
Γ2,1. The side facets of tetrahedron
Γ2,1 are:
ζtN1,1,
ζwN2,0,
ζtN2,1,
ζwN2,1, where
ζwN2,0 is the plane (
y, z) of the coordinate system [
x, y, z],
ζtN1,1 was described earlier.
In turn, two planes:
ζtN2,1,
ζwN2,1 are defined respectively by the following triads of points: (
N2,0,
N2,1,
Ht2,1), (
N1,1,
N2,1,
Hw2,1), where
Ht2,1 is the middle of section
Ht2,0_2,1Ht2,1_1,1, and
Hw2,1 is the middle of segment
Hw1,1_2,1Hw2,1_2,1. Straight lines
h1,0_2,1 =
ζtN1,1 ∩
ζwN2,0,
h1,1_2,1 =
ζtN1,1 ∩
ζwN2,1,
h2,0_2,1 =
ζtN2,1 ∩
ζwN2,0,
h2,1_1,1 =
ζtN2,1 ∩
ζwN2,1 are initial approximations of straight lines
nNi,j normal to the reference surface σ at points
Ni,j (
i = 1, 2,
j = 0, 1) and are taken as preliminary approximations of the four side edges of tetrahedron
Γ2,1. Each two subsequent straight lines from the four following lines {
h1,0_2,1,
h1,1_2,1,
h2,0_2,1,
h2,1_1,1} pass through adjacent points
Νi,j of ellipsoid
σ and intersect in the appropriate four vertices {
Hw2,0,
Ht2,0,
Hw2,1,
Ht2,1} of tetrahedron
Γ2,1 (
Figure 26).
The sum of constructed tetrahedrons
Γ1,1 and
Γ2,1 does not create reference structure
Γ, because, for example, straight lines
h1,1_1,1, ⊂
Γ1,1, i
h1,1_2,1 ⊂
Γ2,1 are not identical (
Figure 26), so the above tetrahedrons do not have a common side edge passing through point
N1,1. In this case, the set of three adjacent planes
ζtN1,1 ∘
ζwN1,1 ∘
ζwN2,1 does not have a common edge, so the above tetrahedrons do not share any common wall.
Therefore, some operation is necessary to replace the two above various straight lines with one straight line in an effective way, so that the above system was replaced by a new system of three planes
ζtN1,1n ∘
ζwN1,1n ∘
ζwN2,1n having one common edge
k1,1. However, the position of this edge of the new system should be the closest possible to the position of normal
n1,1 of ellipsoid
σ at point
N1,1. Therefore, an optimization process is necessary, so that sum
SMin of square of angles
φi,j between each plane
ζtNi,jn or
ζwi,jn of the new system and the corresponding plane
ζtNi,jn or
ζwi,jn of the old system was the smallest possible. The optimization condition reads:
where:
r =
w or
t, so it is obtained
i,jn for points
Hwi,jn and
ζwi,jn, and
i,jn for points
Hti,jn and
ζtNi,jn.
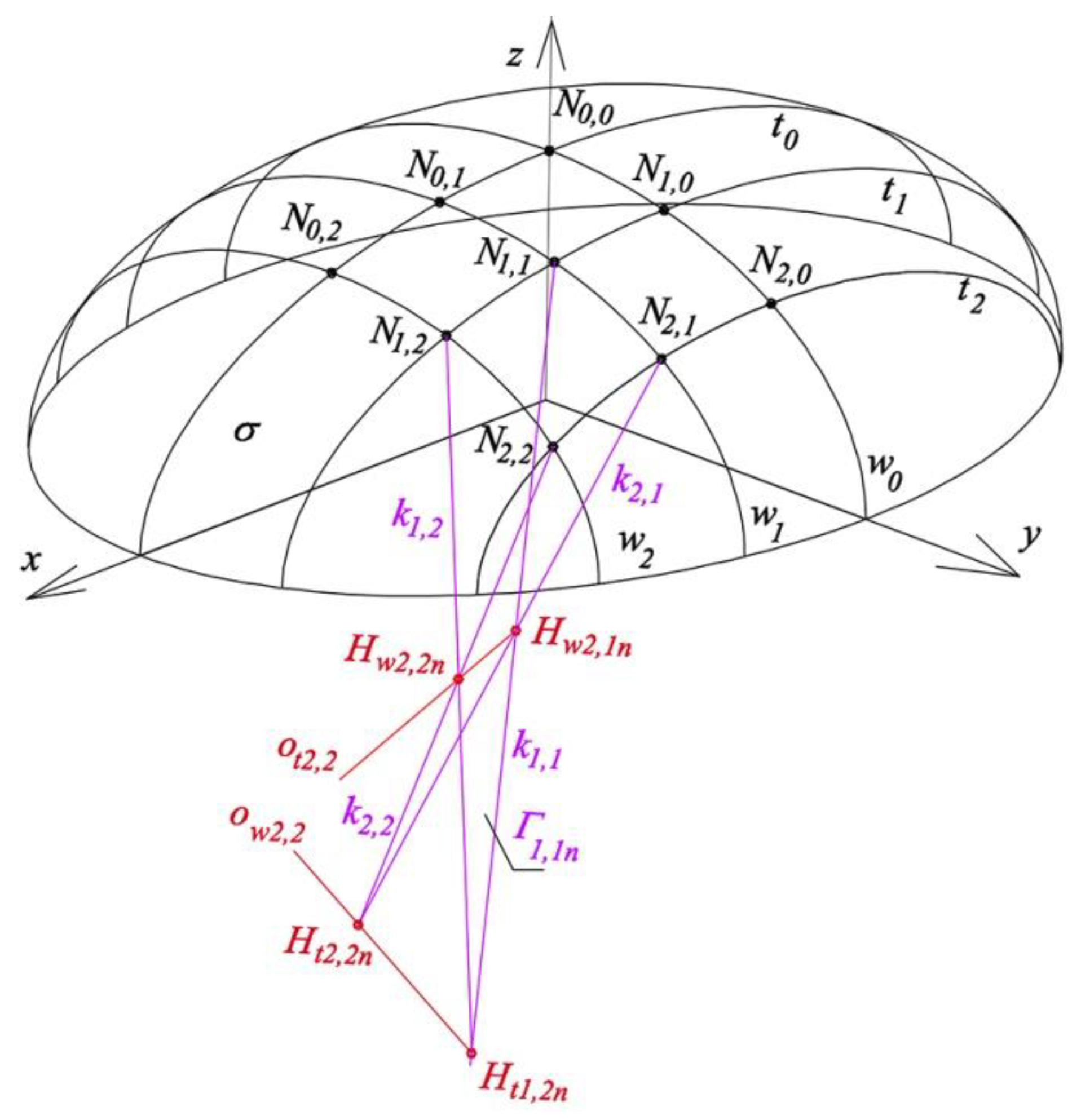
It is then assumed that edge k1,1 of the three new planes will be the closest possible to the line n1,1 normal to ellipsoid σ within acceptable modeling accuracy. The description of the way of determining straight line k1,1 will be presented after considering two next reference tetrahedrons Γ1,2, Γ2,2, because this straight line will replace four corresponding side edges of tetrahedrons Γ1,1, Γ2,1, Γ1,2, Γ2,2 with no common side edge passing through point N1,1. The results of the optimization process performed for the reference structure Γn composed of four reference tetrahedrons Γi,jn replacing Γi,j (i = 1.2, j = 1.2) are also presented at the end of this section.
The last two considered tetrahedrons
Γ2,1,
Γ2,2 are defined in a manner analogous to the one presented earlier for
Γ1,1. Therefore, the following pairs of straight lines are investigated: {
n0,2,
n1,2}, {
n1,1,
n1,2}, {
n1,2,
n2,2}, {
n2,1,
n2,2} and their intersecting points with a corresponding straight line perpendicular to each pair are sought. In the case of pair {
n0,2,
n1,2}, these are points
Hw0,2_1,2 ∈
n0,2 and
Hw1,2_1,2 ∈
n1,2. In the case of pair {
n1,1,
n1,2}, these are points
Ht1,1_1,2 ∈
n1,1 i
Ht1,2_1,2 ∈
n1,2 (
Figure 27a). In the case of pair {
n1,2,
n2,2}, these are points
Hw1,2_2,2 ∈
n1,2 i
Hw2,2_1,2 ∈
n2,2. For pair {
n2,1,
n2,2}, these are points
Ht2,1_2,2 ∈
n2,1 i
Ht2,2_2,2 ∈
n2,2.
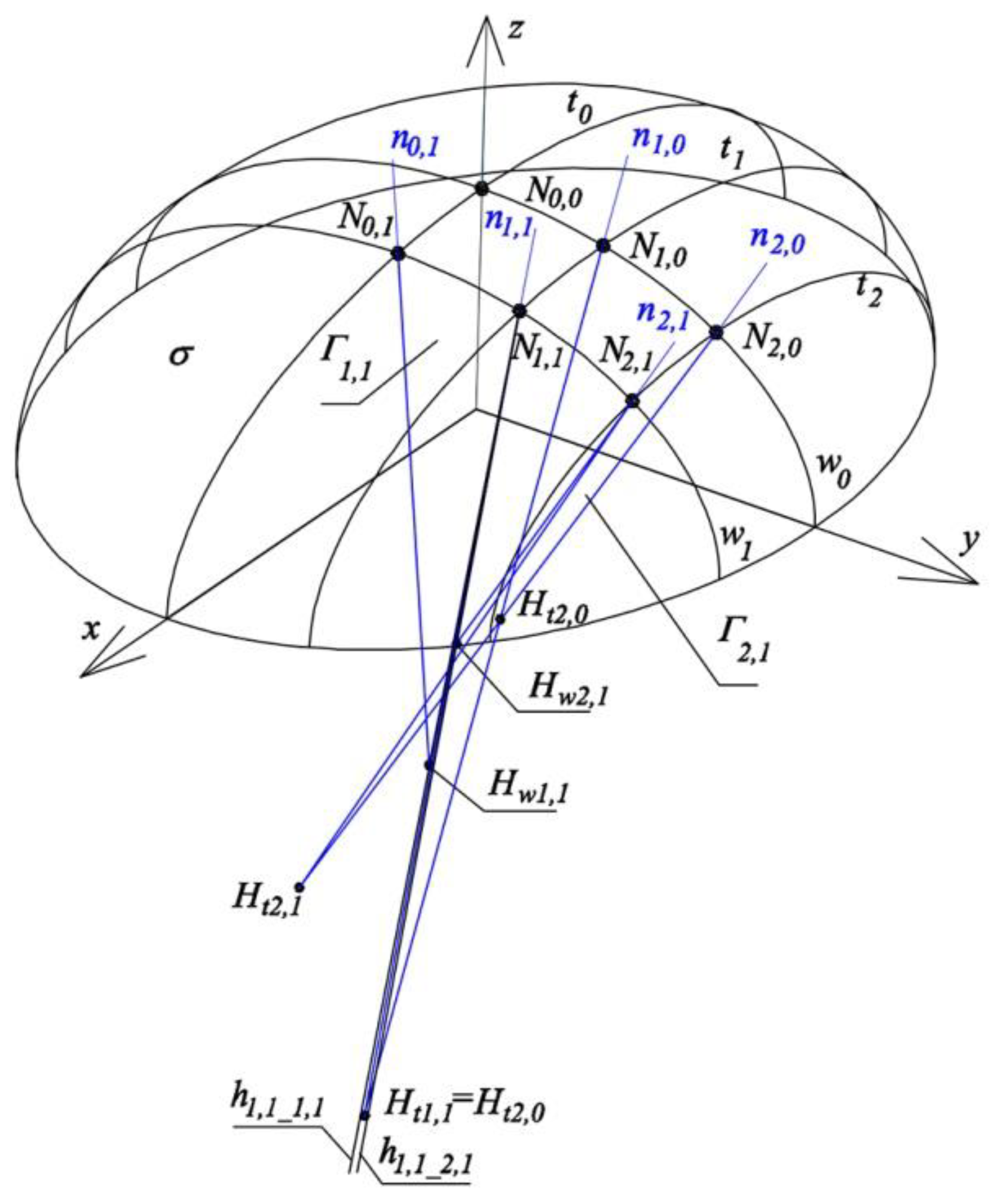
Tetrahedron Γ1,2 is created by means of planes ζtN0,2, ζtN1,2, ζwN1,2, ζwN1,1, where ζtN0,2 is the plane (x, z) of the coordinate system, ζwN1,1 was defined before, while two planes ζ tN1,2, ζwN1,2 are defined by the following triads of points: (N1,1, N1,2, Ht1,2), (N0,2, N1,2, Hw1,2), where Ht1,2 is the middle of Ht1,1_1,2Ht1,2_1,2 section, Hw1,2 is the middle of Hw0,2-_1,2Hw1,2-_1,2. Straight lines h0,1_1,2 = ζtN0,2 ∩ ζwN1,1, h0,2_1,2 = ζtN0,2 ∩ ζwN1,2, h1,1_1,2 = ζtN1,2 ∩ ζwN1,1, h1,2_1,2 = ζtN1,2 ∩ ζwN1,2 are adopted as the edges of tetrahedron Γ1,2. These edges take positions close to the positions of straight lines ni,j normal to reference surface σ at points Ni,j (for i = 0,1, j = 1,2). However, they are not side edges of the searched polyhedral reference structure because Γ1,2 has no common edge with Γ1,1 or Γ2,1.
Tetrahedron Γ2,2 is defined by means of planes ζtN1,2, ζtN2,2, ζwN2,1, ζwN2,2, where ζtN1,2 and ζwN2,1 were defined before. The next two planes ζtN2,2, ζwN2,2 should be determined using the following triplets of points: (N2,1, N2,2, Ht2,2), (N1,2, N2,2, Hw2,2), where Ht2,2 is the middle of segment Ht2,1_2,2Ht2,2_2,2, while Hw2, is the middle of segment Hw1,2-_2,2Hw2,2-_2,2. The following straight lines h1,1_2,2 = ζtN1,2 ∩ ζwN2,1, h1,2_2,2 = ζtN1,2 ∩ ζwN2,2, h2,1_2,2 = ζtN2,2 ∩ ζwN2,1, h2,2_2,2 = ζtN2,2 ∩ ζwN2,2 are adopted as the edges of reference of tetrahedron Γ2,2 and are the initial approximation of the edges of the polyhedral reference structure Γ.
The structure created as a result of adding the reference tetrahedrons
Γ1,1,
Γ2,1,
Γ1,2,
Γ2,2 is not reference structure
Γ because, similarly as for the case of the sum of tetrahedrons
Γ1,1,
Γ2,1 considered earlier, two triads of planes:
ζtN1,2 ∘
ζwN1,2 ∘
ζwN2,2,
ζtN2,1 ∘
ζwN2,1 ∘
ζtN2,2 and four planes
ζtN1,1 ∘
ζwN1,1 ∘
ζwN2,1 ∘
ζtN1,2 do not have common side edges. Thus, tetrahedrons
Γ1,2,
Γ2,2 and tetrahedrons
Γ2,1,
Γ2,2 as well as
Γ1,1,
Γ2,1,
Γ1,2,
Γ2,2 do not have appropriate common side edges passing through point
N2,1 or
N1,2 or
N1,1 (
Figure 27b). Therefore, it is necessary to replace the above two triads and one tetrad with two new triads
ζtN1,2n ∘
ζwN1,2n ∘
ζwN2,2n,
ζtN2,1n ∘
ζwN2,1n ∘
ζtN2,2n and one new tetrad
ζtN1,1n ∘
ζwN1,1n ∘
ζwN2,1n ∘
ζtN1,2n in such a way that the position of the new planes and their edges is close to the positions of the respective straight lines
ni,j normal to reference surface σ with the most possible precision.
Therefore, a second step of the initiated process of replacing straight lines hr,s_i,j (i, j, r, t = 1, 2) of planes ζtNi,j or ζwNi,j belonging to the created tetrahedrons Γi,j not producing the polyhedral reference structure Γ, with side edges ki,j of planes ζtNi,jn and ζwNi,jn of the searched polyhedral reference structure Γn of several Γi,jn, is necessary. Since the above straight lines and planes should be as close as possible to straight lines ni,j normal to reference surface σ, it was assumed that sum SMin (4) of squares of angles between the planes of the achieved new systems of planes ζtN1,2n ∘ ζwN1,2n ∘ ζwN2,2n, ζtN2,1n ∘ ζwN2,1n ∘ ζtN2,2n, ζtN1,1n ∘ ζwN1,1n ∘ ζwN2,1n ∘ ζtN1,2n and the corresponding planes of the previously created old systems of planes ζtN1,2 ∘ ζwN1,2 ∘ ζwN2,2, ζtN2,1 ∘ ζwN2,1 ∘ ζtN2,2, ζtN1,1 ∘ ζwN1,1 ∘ ζwN2,1 ∘ ζtN1,2 should be the smallest possible.
To calculate the angles between the
ζtNi,j,
ζwNi,j planes determined in the first step for the tetrahedrons
Γi,j and
ζtNi,jn,
ζwNi,jn for the
Γi,jn reference tetrahedrons forming the meshes of the searched reference network
Γn, and estimated in the second step of the algorithm of the presented method the following formula was used
where
nki,j is the unit vector normal to plane
ζtNi,j or
ζwNi,j,
nki,jn is the unit directional vector of
ζtNi,jn or
ζwNi,jn, Π = 3.14159.
As a result of the optimization process, the following objects were obtained (
Figure 28): (a) edge
k1,2 of three new planes
ζtN1,2n ∘
ζwN1,2n ∘
ζwN2,2n, which replaces two straight lines
h1,2_1,2 ⊂
Γ1,2,
h1,2_2,2 ⊂
Γ1,2 as accurately as possible; (b) edge
k2,1 of three new planes
ζtN2,1n ∘
ζwN2,1n ∘
ζtN2,2n, which replaces two straight lines
h2,1_2,1 ⊂
Γ2,1,
h2,1_2,2 ⊂
Γ2,2 as precisely as possible; and (c) edge
k1,1 of four new planes
ζtN1,1n ∘
ζwN1,1n ∘
ζwN2,1n ∘
ζtN1,2n, which substitutes four straight lines
h1,1_1,1 ⊂
Γ1,1,
h1,1_1,2 ⊂
Γ1,2,
h1,1_2,1 ⊂
Γ2,1,
h1,1_2,2 ⊂
Γ2,2 as exactly as possible. On the basis of the above planes, it is possible to determine edge
k2,2 of
Γn as the straight line being the intersection of planes
ζwN2,2n, ∩
ζtN2,2n, where
ζtN2,2n is defined by edge
k2,1 and point
N2,2, and
ζwN2,2n is defined by edge
k1,2 and point
N2,2. As a result, the straight lines
k1,1,
k1,2,
k2,1,
k2,2, whose positions are very close to the positions of
n1,1,
n1,2,
n2,1,
n2,2 normal to ellipsoid
σ, were obtained.
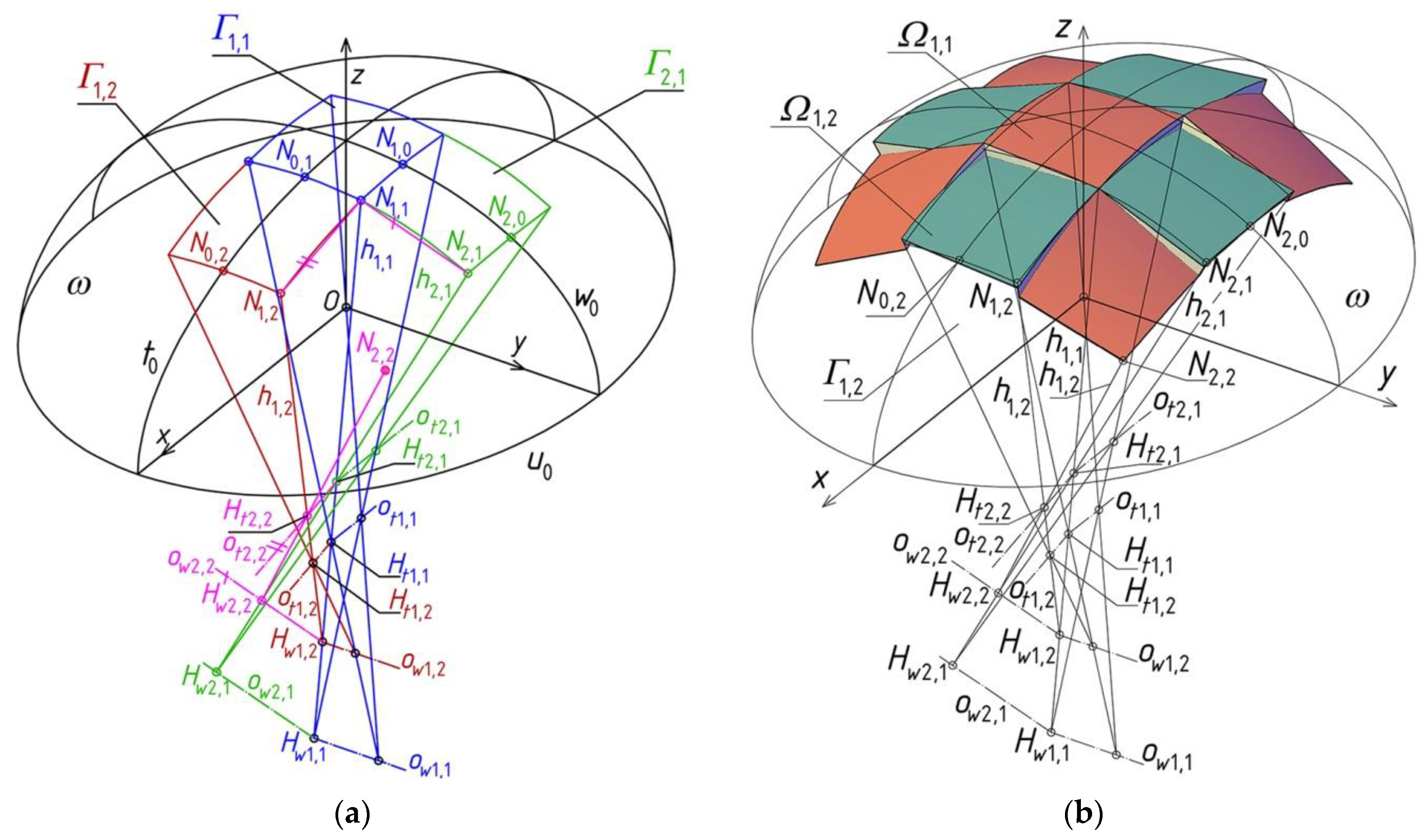
During the process of optimizing the positions of the facets and edges of reference tetrahedrons Γi,jn to the positions of straight lines ni,j normal to the reference surface σ, the positions of the following points were changed: Hw1,1, Hw2,1, Hw2,2, Ht2,1 respectively on lines (Hw0,1_1,1, Hw1,1_1,1), (Hw1,1_2,1, Hw2,1_2,1), (Hw1,2-_2,2, Hw2,2_2,2), (Ht2,0_2,1, Ht2,1_2,1). As a result, their new positions Hw1,1n, Hw2,1n, Hw2,2n, Ht2,1n made it possible to build triples of points (N0,1, N1,1, Hw1,1n), (N1,1, N2,1, Hw2,1n), (N1,2, N2,2, Hw2,2n), (N2,0, N2,1, Ht2,1n) determining the planes ζwN1,1n, ζwN2,1n, ζwN2,2n, ζtN2,1n sought. The location of points Hw1,1, Hw2,1, Hw2,2, Ht2,1 was controlled by parameters wHw1,1n, wHw2,1n, wHw2,2n, wHt2,1n, that is the division coefficients of sections Hw0,1_1,1Hw1,1_1,1, Hw1,1_2,1Hw2,1_2,1, Hw1,2-_2,2Hw2,2_2,2 i Ht2,0_2,1Ht2,1_2,1, in contrast to the vertices Hw1,1, Hw2,1, Hw2,2, Ht2,1 adopted in the middles of the respective sections considered in the previous step. For other points Hwi,jn, Hti,jn, the values of the division coefficients are the result of the optimization process and depend on the aforementioned four coefficients.
The algorithm of defining the optimal reference structure
Γn based on the obtained points
Hw1,1,
Hw2,1,
Hw2,2,
Ht2,1 is presented below (
Figure 28). Structure
Γn is the sum of reference tetrahedrons
Γi,jn, whose edges
ki,j and planes
ζtNi,jn can be achieved on the basis of the above four optimized division coefficients in the following way.
Points N1,1 and Hw1,1n define edge k1,1. Straight line k1,1 and point N0,1 determine plane ζwN1,1n of the reference structure Γn. Analogously, the following planes: ζwN2,1n, ζtN1,1n, ζtN1,2n of structure Γn pass through edge k1,1 and points N1,0, N1,2, N2,1, respectively. Similarly, plane ζwN2,2n passing through points N1,2, N2,2, Hw2,2n is the plane of structure Γn. Edge k1,2 of structure Γn is the intersection of planes ζtN1,2n, ζwN2,2n. Edge k1,2 and point N0,2 define plane ζwN1,2n. Planes ζtN2,1n and ζwN2,1n intersect each other in edge k2,1. Plane ζtN2,2n is determined by points N2,0, N2,1 and Ht2,1n. Plane ζwN2,2n is determined by points N1,2, N2,2, Hw2,2n. Edge k2,2 is the intersection of planes ζtN2,2n and ζwN2,2n.
Edges
k0,1 and
k0,2 of structure
Γn are the straight lines of the intersection of planes
ζwN1,1n,
ζwN1,2n with plane (
x, z). Edges
k1,0 and
k2,0 of
Γn are the straight lines of the intersection of planes
ζtN1,1n,
ζtN2,1n with plane (
y, z). Axis
z is adopted as edge
k0,0 of
Γn. The reference tetrahedron
Γ2,2 (
Figure 29) is created as the last part of the one-fourth of
Γn.
The reference structure
Γn is the sum of all reference tetrahedrons
Γi,jn whose walls, contained in the aforementioned planes
ζwNi,jn,
ζtNi,jn (
i = 0, 1, 2 and
j = 0, 1, 2), are common to each pair of the adjacent reference tetrahedrons, and side edges
ki,j are the shared corners of pairs, triples or tetrads of the neighboring reference tetrahedrons. The results of the optimization process performed for one of the four quarters of the considered reference structure
Γn symmetrical towards two planes of coordinate system [
x, y, z] are presented in
Figure 30.
The values of selected coefficients
wHwi,jn or
wHti,jn evaluated in the presented iterative optimization process are included in
Table 8. The obtained values of some angles between planes
ζtNi,j,
ζwNi,j of old tetrahedrons
Γi,j and planes
ζtNi,jn, ζwNi,j of new reference tetrahedrons
Γi,jn are included in
Table 9. The investigated one-fourth of the structure
Γn is composed of four reference tetrahedrons
Γi,jn (
i = 1, 2 and
j = 1, 2). The values of components
lki,j, mki,j, mki,j of the directional vectors of side edges
ki,j of structure
Γn, passing through points
Ni,j are given in
Table 10.
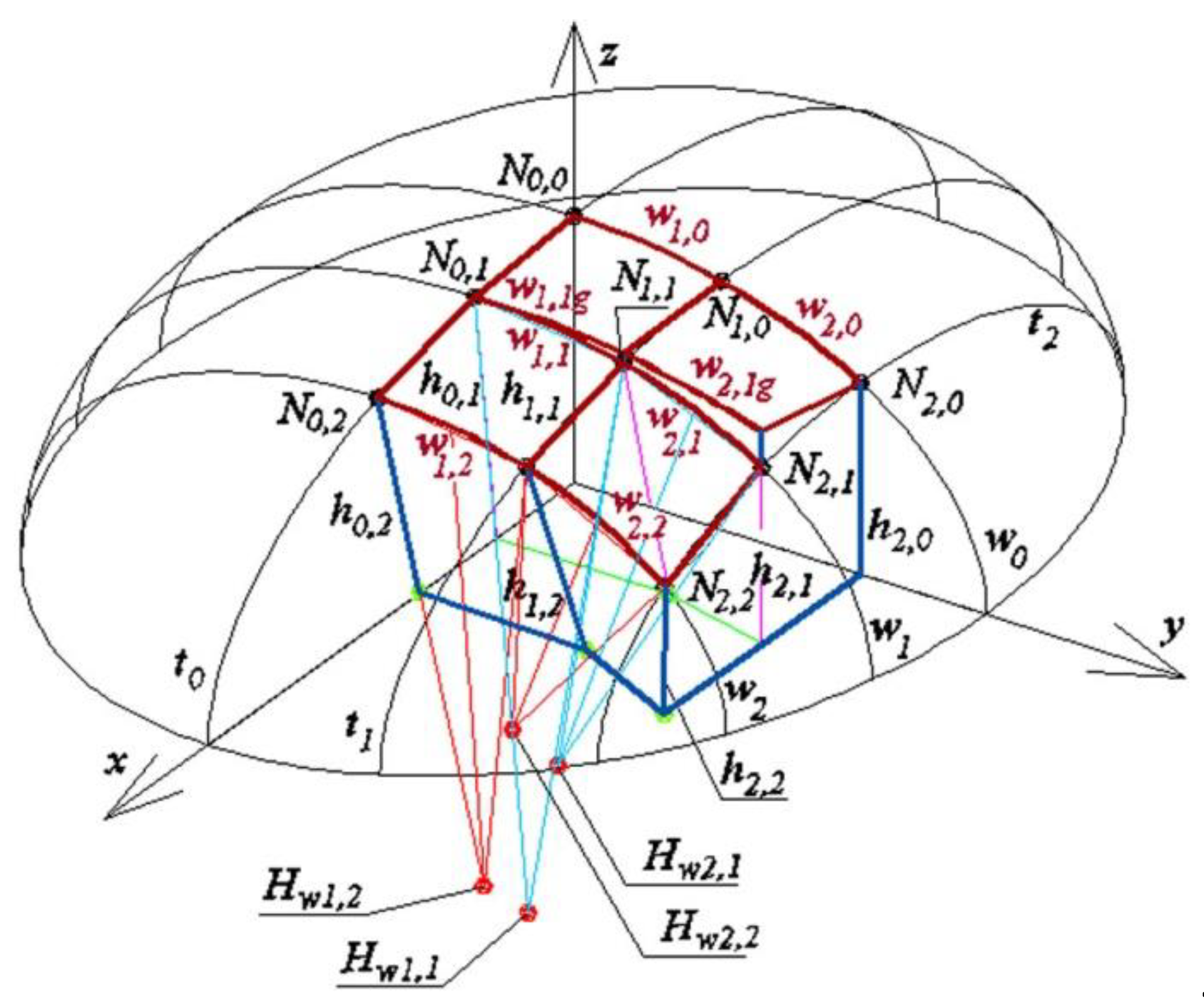
The directrices of each shell segment of the searched roof structure based on ellipsoid
σ are sums of sections
wi,j of arbitrary ellipses, for instance one directrix corresponding to
w2 is the sum of
w1,2 =
ζwN1,2n ∩
σ and
w2,2 =
ζwN2,2n ∩
σ (
Figure 31). In addition,
w1,0 w2,0 w0,
w1,1 ≠
w1,
w2,1 ≠
w1, {
w1,1g,
w1,1}
ζwN1,1n, {
w2,1g,
w2,1}
ζwN2,1n,
w1,2 ≠
w2,2 ≠
w2. The lines
w1,1 and
w1,1g as well as
w2,1 and
w2,1g are coplanar sections of the directrices. The index
g denotes that the proper curve e.g.
w2,1g is located outside of the reference ellipsoid. It is possible to obtain such a structure that the conditions
w1,1 =
w1,1g =
ζwN1,1n ∩
σ and
w2,1 =
w2,1g =
ζw2,1 ∩
σ are met. The visualization of the resultant architectural form of the discussed structure is presented in
Figure 32.