Multi-Hazard Vibration Control of Transmission Infrastructure: A Pounding Tuned Mass Damper Approach with Lifelong Reliability Analysis

Abstract

Power transmission tower-line systems are exposed to various dynamic hazards, including wind and earthquakes, among others. Despite the multitude of dampers proposed to mitigate vibrations, the dual control effect on both seismic and wind-induced vibrations has rarely been addressed. This paper introduces a comprehensive methodology for evaluating the reliability of power transmission towers under a range of dynamic disasters, encompassing both earthquakes and wind loads. Subsequently, a lifelong reliability approach was employed to assess the efficacy of a pounding tuned mass damper (PTMD). The proposed algorithm leverages the incremental dynamic analysis (IDA) method to compute structural fragility with regard to each type of disaster and integrates these findings with hazard functions to determine the probability of overall failure. The results conclusively demonstrate that the PTMD substantially diminished the towers’ dynamic response to both earthquakes and wind loads, thereby enhancing their overall reliability. Specifically, the PTMD reduced the vibration reduction ratio by 10% to 30% under wind loads and by 20% to 80% under seismic actions, with more pronounced effects at higher wind speeds and peak ground accelerations (PGAs). Furthermore, the reliability index (β) of the transmission tower increased from 2.1849 to 2.4295 when the PTMD was implemented, highlighting its effectiveness in dual-hazard scenarios. This study underscores the potential for reliability to be considered as a key metric for optimizing damping devices in power transmission structures, particularly in the context of multi-hazard scenarios.

1. Introduction

As the foundational support structures of electrical power systems, transmission towers are indispensable in guaranteeing stable power transmission, enhancing system safety and reliability, and integrating renewable energy sources. They not only facilitate the spanning of power lines across extensive distances but also constitute crucial infrastructure for the efficient distribution and utilization of energy.

Due to their inherent flexibility, transmission towers are prone to damage from a variety of dynamic hazards [1,2], which can result in significant consequences. Over the past few decades, numerous reports have chronicled instances ranging from minor damage to catastrophic failures. Two important types of hazard are as follows:

• Wind-induced hazards: Wind loads represent one of the most prevalent types of disaster leading to the collapse of power transmission towers [3,4]. When exposed to extreme wind loads, such as those from hurricanes, typhoons [5], galloping [6,7] or downbursts, the primary structural members of the transmission tower experience a substantial surge in stress levels, which may result in yielding, buckling, and ultimately, complete structural collapse. Furthermore, wind-driven particles, such as wind-blown raindrops [8] or sand [9], can exacerbate the dynamic responses of power transmission towers, and prolonged exposure to wind-induced excitations can induce fatigue damage in operational transmission towers, progressively diminishing the structural capacity of their components. In severe cases, this cumulative damage can escalate to collapse and result in catastrophic failure;
• Earthquake activity: As potent natural disasters, earthquakes generate intense ground motions that can cause immediate physical damage, such as the collapse of transmission towers and the fracture of conductors [10,11]. Additionally, these seismic events have the potential to trigger secondary geological hazards, such as landslides or structural failures, which can further compound the damage to transmission lines. Moreover, the electrical equipment situated along these lines can be adversely affected by earthquakes, thereby jeopardizing the stability of the entire power system. The ensuing disruptions not only lead to widespread power outages that inflict substantial economic losses on society but also have the potential to initiate a chain of consequences that disrupts the normal functioning of other vital infrastructure.

In order to reduce the losses caused by these dynamic hazards, a wide array of dampers have been developed and tested both in laboratory settings and in real-world applications.

Wind load is typically considered the dominant lateral load in the design of transmission towers, leading to the proposal of various dampers to mitigate wind-induced vibration and reduce structural response under such conditions. In 2021, Roy and Kundu [12] summarized state-of-the-art vibration control techniques with regard to the response of transmission towers under wind load. In early efforts, tuned mass dampers (TMDs) were initially utilized because of their simplicity and effectiveness. Stockbridge dampers, which employ a damping mechanism similar to that of a TMD, are widely used to suppress the vibration of long-span conductors [13]. Recently, in order to enhance damping performance or minimize space requirements, numerous supplementary devices have been integrated into the traditional TMD. Upgraded TMD-type dampers include tuned mass damper inerters (TMDIs) [14], shape memory alloy-spring pendulums (SMA-SPs) [15], etc. In addition, Song et al. [16] explored the use of electromagnetic inertial mass dampers (EIMDs) for control of vibration in wind-disturbed transmission towers. They presented an analytical model, control approach, and parametric studies that demonstrated the effectiveness and robustness of EIMDs in reducing structural dynamic responses under varying intensities of wind load. Lei et al. [17] developed a new device named ECD-TMD, incorporating eddy current damping (ECD) and characterized by its non-contact ECD and structural simplicity. Field tests verified the significant vibration suppression achieved with this damper. Lou et al. [18] conducted a wind-tunnel test to examine the damping effect of an omnidirectional cantilever-type eddy current tuned mass damper (ECTMD). Furthermore, Grotto et al. [19] proposed a methodology to optimize the stiffness and damping coefficients of connection dampers in lattice steel towers subjected to wind action, aiming to reduce the dynamic response and horizontal displacements of the structure. Numerical results demonstrated that through incorporating semi-rigid connections with optimized connection dampers, a significant reduction in horizontal displacements can be achieved without significantly altering the fundamental frequency of the vibration, thereby enhancing the structural performance against wind-induced vibrations.

Regarding mitigation of earthquake-induced vibration, while wind load is commonly considered the primary lateral load in the design of transmission towers, numerous tall transmission towers in regions with high seismic intensity are susceptible to damage or even collapse. To mitigate earthquake-induced vibration, Wu et al. [20] utilized the energy dissipation property of SMA wires, due to their superelasticity, and developed a tube-type SMA damper. Zhang et al. [21,22] introduced impact into the traditional TMD and proposed a pounding tuned mass damper (PTMD). Lin and Liu [23] conducted an exhaustive parametric study of a TMD to meticulously examine its damping effect against near-fault earthquakes. Their paper further introduced an innovative multi-objective optimization framework, leveraging the NSGA-III algorithm to concurrently optimize the vibration reduction ratios and the cost of the TMD. Additionally, an artificial neural network (ANN)-based predictive framework was established to efficiently forecast the intricate relationship between TMD parameters and vibration reduction ratios. Miguel et al. [24] introduced a method for optimizing the placement and friction forces of dampers in structures under seismic loading, employing a recently developed optimization algorithm. Their findings revealed substantial decreases in structural response, showcasing the potential of the proposed methodology as an efficient tool for the design of friction dampers.

Despite the considerable advancements in research on vibration control for power transmission towers, a significant limitation is evident. The majority of existing dampers have been tailored to address only a single type of hazard, such as earthquakes or wind load, but not both. This narrow focus stems from the absence of evaluation criteria that specifically address multi-hazard scenarios. As a result, the application of these dampers in mitigating the combined effects of multiple dynamic hazards remains limited. To bridge this gap, this paper introduces a comprehensive methodology for assessing the reliability of power transmission towers under a wide range of dynamic disasters (shown in Figure 1), including both earthquakes and wind load. We employ a lifelong reliability approach to rigorously evaluate the effectiveness of a PTMD. Our proposed algorithm utilizes incremental dynamic analysis (IDA) to calculate structural fragility with regard to each type of disaster. These fragility estimates are then integrated with hazard functions to determine the overall failure probabilities of the towers.

The results of this study demonstrate that the PTMD can significantly reduce the dynamic response of towers to both earthquakes and wind loads, thereby enhancing the towers’ overall reliability. This research highlights the potential of reliability as a crucial metric for optimizing damping devices in power transmission structures, particularly in the context of multi-hazard scenarios. By providing a comprehensive framework for evaluating and optimizing damping solutions, this study contributes to the advancement of vibration control technologies for power transmission towers.

The remainder of this paper is structured as follows: Section 2, Section 3, Section 4, Section 5 and Section 6 elucidate the detailed procedure of the proposed methodology. Specifically, Section 2 provides an explanation of the simplified model of the power transmission tower-line system. Section 3 subsequently introduces the damping mechanisms facilitated by the PTMD and derives the corresponding motion equation for the tower–PTMD system. In Section 4, we address considerations of structural uncertainties and uncertainties that may affect the PTMD. Section 5 details the method for generating seismic and wind excitations, and Section 6 outlines the calculation of the reliability of the tower–PTMD system through the integration of fragility and hazard functions. This is followed by Section 7, which presents numerical results and discussions. Finally, Section 8 concludes this paper with remarks on the current findings and their implications.

2. Numerical Model of Power Transmission Tower

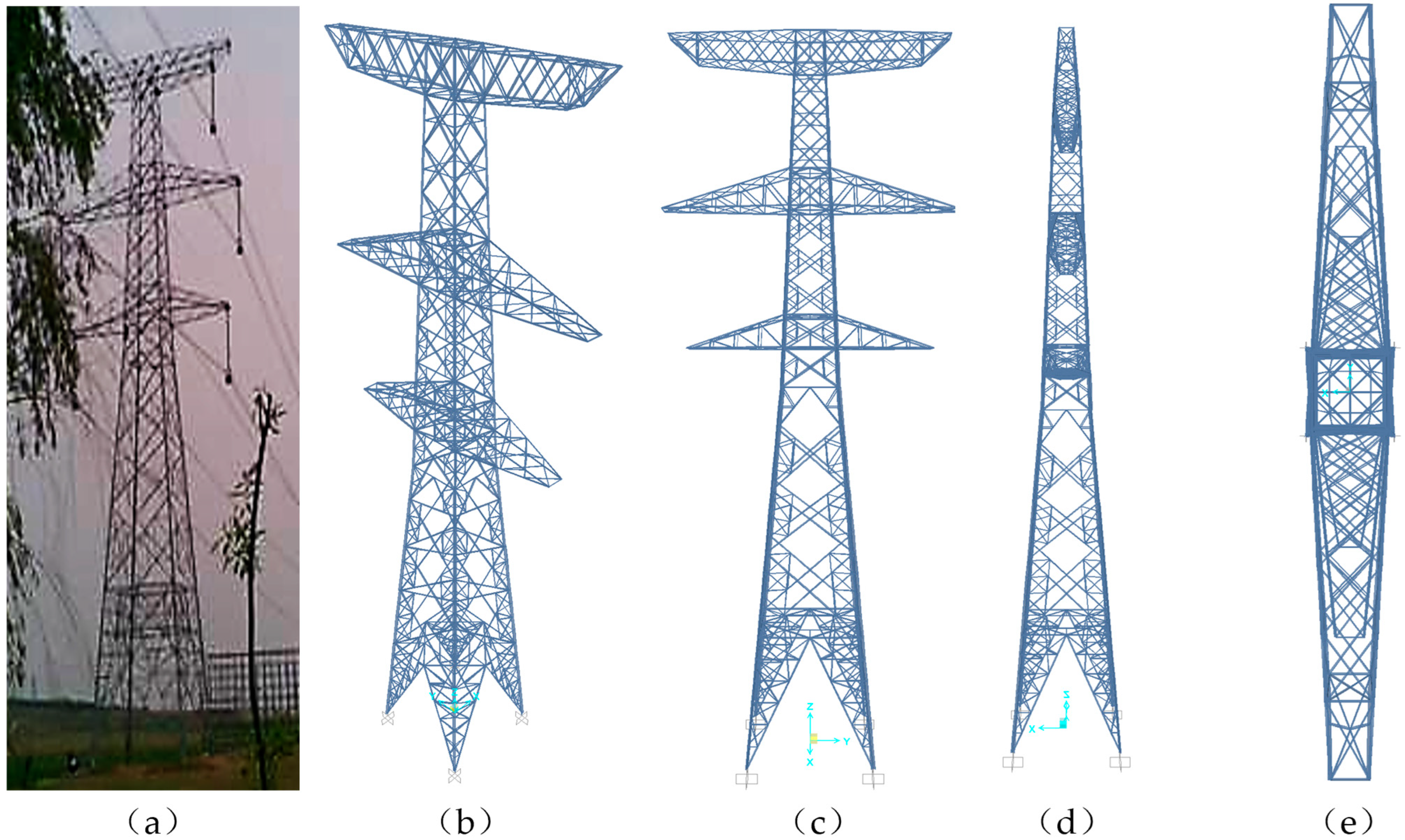
2.1. FE Model

This study simulated a 35.71 km long transmission line as the engineering background. The voltage level of this project was 500 kV. The transmission line utilized a double-circuit, four-bundle conductor configuration, with a horizontal span of 400 m. The conductors were composed of 4 × LGJ-400/35 aluminum conductor steel reinforced (ACSR) cables, while the ground wires were LGJ-95/55 ACSR.

The selected power transmission tower was a tangent-type transmission tower standing at a total height of 53.9 m, with a nominal (structural) height of 30 m. It featured a base width of 8.36 m and a top width of 2.0 m, incorporating three strategically positioned crossarms at the top, middle, and bottom. These crossarms extended outwards by 8.7 m, 10.45 m, and 10 m, respectively. The primary structural components, including the main members, diagonal members, and auxiliary materials, were constructed of Q345 and Q235 angle steel. For visual reference, a photograph of this type of tower is provided in Figure 2a. Figure 2b–e illustrates the finite element (FE) model of the transmission tower from different angles. The whole FE model consisted of 618 nodes and 1588 elements.

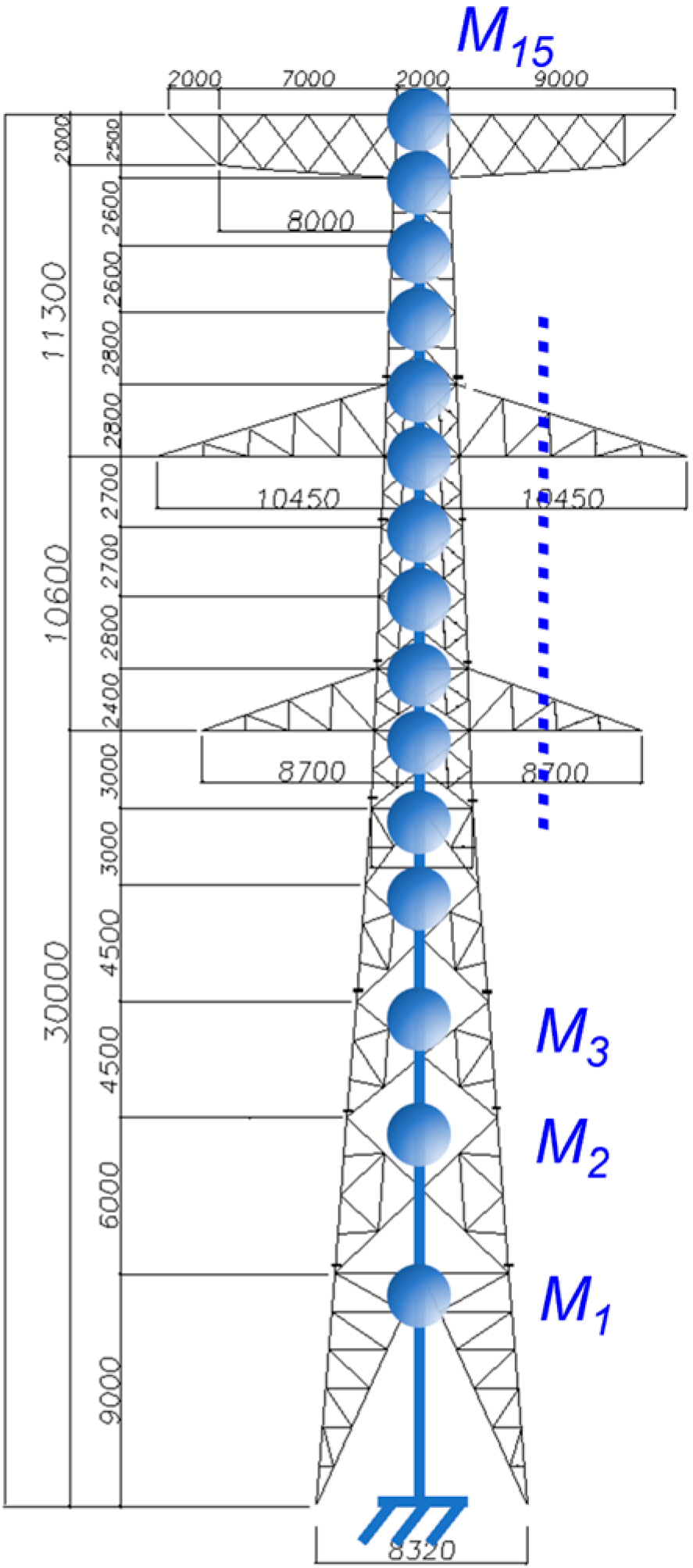
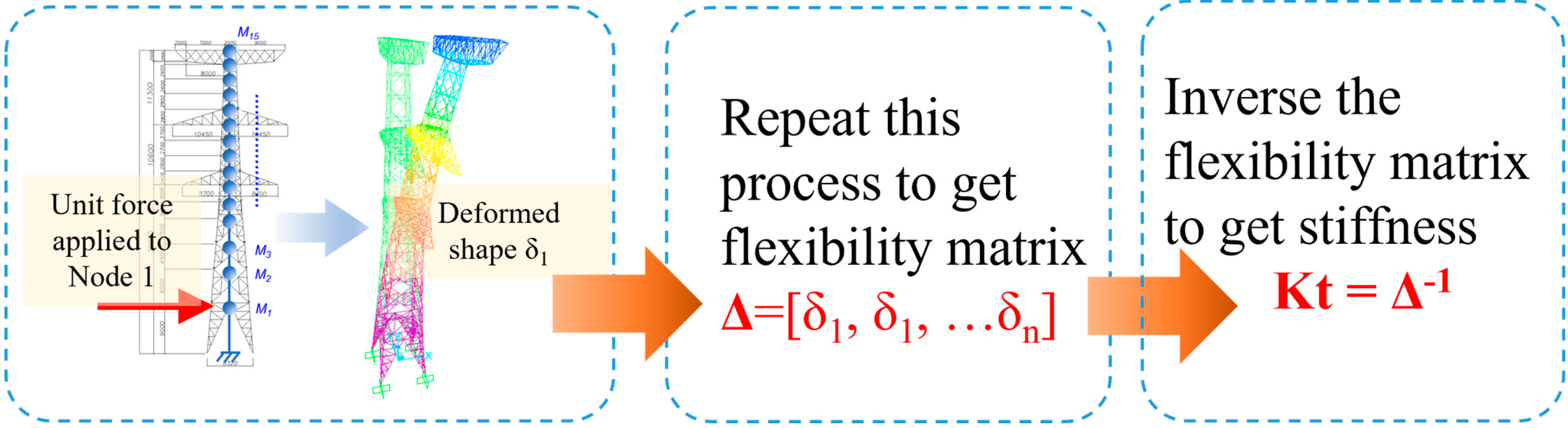
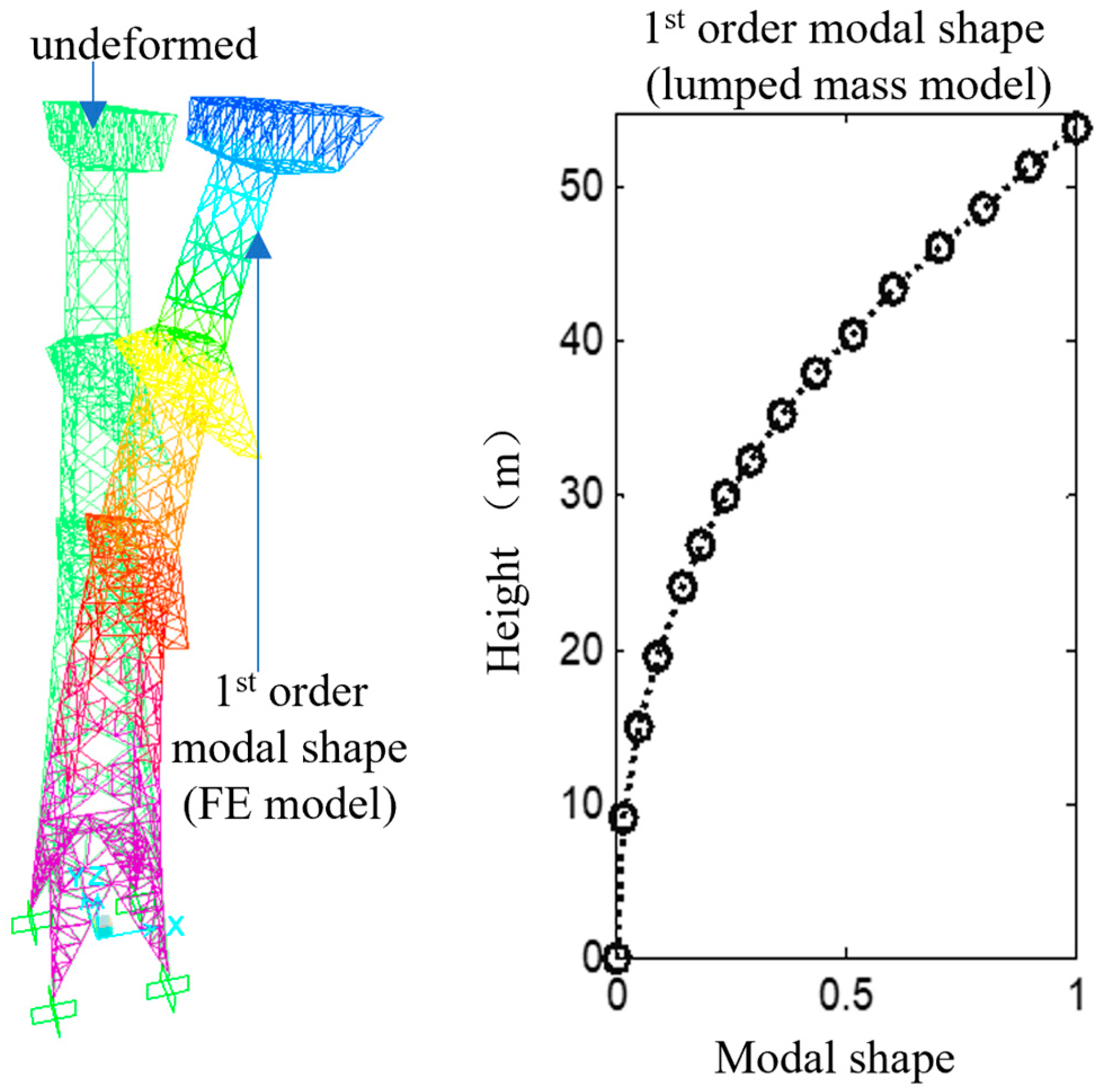
2.2. Lumped Mass Model

Although a finite element (FE) model can facilitate time-domain analysis of a power transmission tower-line system, considering the complexity of multi-hazards, a simplified numerical model remains necessary for the following reasons. The proposed PTMD is intricate and challenging to simulate using FE software. Additionally, conducting time-domain analysis with an FE model is computationally intensive and time-consuming. Therefore, this study established a lumped mass model to simulate the behavior of the transmission tower under wind load and seismic action.

The lumped mass model represents a simplification of the detailed FE model. It conceptualizes the power transmission tower as a series of concentrated masses interconnected by rods. Figure 3 provides a visualization of the lumped mass model applied to the tangent tower. To obtain the mass, stiffness, and damping matrices for this model, the following steps were followed:

Step 1

The power transmission tower was divided into $n$ nodes based on its structural characteristics. The positions of the crossarms and crossbraces were considered as a single node layer. In Figure 3, the power transmission tower considered in this study is represented by a lumped mass model of 15 mass components.

Step 2

The mass of the steel members between two adjacent nodes $n_i$ and $n_{i+1}$ was calculated. Here, $n_i$ represents the ith node, and $n_{i+1}$ represents the i + 1th node. Then, half of the calculated mass was assigned to node $n_i$ and the other half to node $n_{i+1}$. Thus, the mass matrix ${\mathit{M}}_{\mathrm{t}}$ was obtained.

Step 3

A unit force was aaplied at the jth node. The deformed shape under this load case was considered the jth flexibility vector, ${\mathit{\delta }}_j$. The total flexibility matrix $\mathsf{\Delta }$ was then obtained as followed:

$$\mathsf{\Delta }=\left[{\mathit{\delta }}_1, {\mathit{\delta }}_2, \dots ,{\mathit{\delta }}_n\right]$$

(1)

Then, the stiffness matrix ${\mathit{K}}_{\mathbf{t}}$ was calculated as the inverse of the flexibility matrix (as shown in Figure 4):

$${\mathit{K}}_{\mathbf{t}}={\mathsf{\Delta }}^{-1}$$

(2)

Step 4

Assuming Rayleigh damping, the damping matrix was then calculated as a superposition of the mass matrix and stiffness matrix, as follows:

$${\mathit{C}}_{\mathbf{t}}=a_1+{\mathit{K}}_{\mathbf{t}}$$

(3)

where

$$\begin{array}{c}{a}_1={\displaystyle \frac{2\left({\xi }_j{\omega }_i-{\xi }_i{\omega }_j\right)}{{{\omega }_i}^2-{{\omega }_j}^2}}{\omega }_i{\omega }_j\\ a_2={\displaystyle \frac{2\left({\xi }_i{\omega }_i-{\xi }_j{\omega }_j\right)}{{{\omega }_i}^2-{{\omega }_j}^2}}\end{array}$$

(4)

where ${\xi }_i$ is the damping ratio of the ith order, and ${\omega }_i$ is the circular frequency of the i-th mode of vibration.

As shown in Figure 3, the FE model was simplified to a lumped mass model of 15 degrees of freedom (d.o.f.). The lumped mass and the height of each node are presented in

Table 1. Mass and height of each node of the simplified model.

Node No.	Height (m)	Mass (kg)
1	9.00	3163.85
2	15.0	1033.85
3	19.5	1708.71
4	24.0	1123.44
5	26.8	767.98
6	30.0	1393.82
7	32.4	1413.27
8	35.2	478.67
9	37.9	448.92
10	40.6	1441.11
11	43.4	1202.66
12	46.2	289.97
13	48.8	203.59
14	51.4	1506.49
15	53.9	891.70

.

In order to validate the effectiveness of the lumped mass model in predicting the dynamic response of the power transmission tower, the first-order modal shapes computed using the finite element (FE) model and the lumped mass model were compared, as shown in Figure 5. The results revealed a striking similarity between the two modal shapes. Furthermore, the first-order frequencies obtained from the FE model and the lumped mass model were 1.877 Hz and 1.872 Hz, respectively, with a minimal relative error of only 0.26%, demonstrating the suitability of the lumped mass model for numerical analysis purposes.

To further validate the accuracy of the lumped mass model for predicting the dynamic response of the transmission tower, the time-history responses of the tower under dynamic loading were computed and the results obtained from the lumped mass model and the finite element model were compared. As shown in Figure 6, the displacement responses from the lumped mass model closely matched those from the finite element model, with minimal deviations observed. The maximum displacement calculated with the lumped mass model and the FE model was 0.1622 m and 0.1595 m, respectively, with a relative difference of only 1.66%. This demonstrates that the lumped mass model provided a high level of computational accuracy, making it a reliable and efficient alternative for dynamic analysis of transmission towers, especially when computational efficiency is a priority.

2.3. Lumped Mass Model Considering Tower-Line Coupling Effect

In actual high-voltage power transmission projects, multi-split conductors are often used for transmission lines, with the mass per unit length reaching several kilograms. Considering that the span of the conductors often extends to hundreds of meters, the total mass of the conductors borne by the transmission tower is substantial, sometimes exceeding half of the tower’s own mass. Therefore, the dynamic coupling effect of the conductors on the transmission tower’s structure cannot be ignored.

This study employed the classical coupling model, as delineated in the literature [25], to compute the dynamic response of the power transmission tower-line system. A schematic of this model is presented in Figure 7. Within this framework, the conductor is approximated as a series of lumped masses interconnected with rigid bars. Previous experimental studies have validated both the accuracy and efficiency of this model.

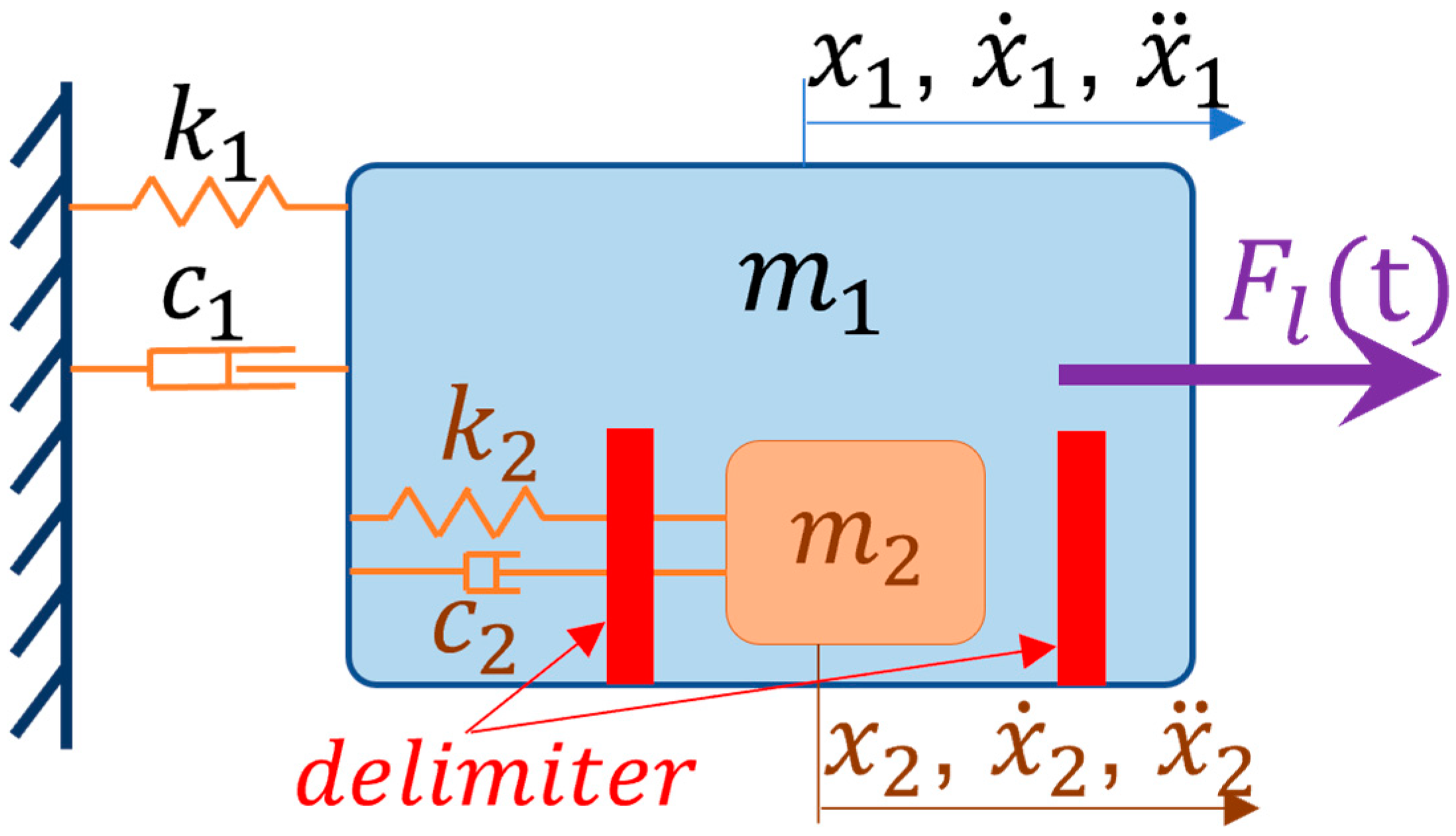
3. Power Transmission Tower Controlled with Pounding Tuned Mass Damper

3.1. Dampin Mechanism of Pounding Tuned Mass Damper

The pounding tuned mass damper (PTMD) is rooted in the principle of pounding damping. Extensive research has shown that PTMDs surpass traditional tuned mass dampers (TMDs) in terms of efficient vibration reduction, providing a wider damping bandwidth and exceptionally resilient performance. Furthermore, the model is distinguished by its straightforward design and minimal maintenance needs.

Figure 8 illustrates a schematic of the PTMD. Its damping mechanism operates in two distinct phases. In instances where the structural amplitude is minor, the amplitude of $m_2$ remains similarly low, causing the PTMD to function like a conventional TMD, mitigating vibration by absorbing energy. Under these conditions, $m_2$ generates an inertial force that aligns with the primary structure $m_1$, consistently directed towards the equilibrium position and thereby effectively diminishing its dynamic responses, including displacement and acceleration. Conversely, when the primary structure experiences substantial vibrations, $m_2$ within the PTMD collides with the delimiter on either side, further dissipating the seismic kinetic energy through impact. In contrast to a traditional TMD, the innovative PTMD offers multiple avenues for energy dissipation, leading to superior damping capabilities.

3.2. Motion Equation of Structure–PTMD System

The motion equations of the structure–PTMD damping system illustrated in Figure 8 are as follows:

$$\left\{\begin{array}{c}{m}_1{\ddot{x}}_1\left(t\right)+c_1{\dot{x}}_1\left(t\right)+k_1{x}_1\left(t\right)=F_{l1}+k_2\left(x_2-x_1\right)+c_2\left({\dot{x}}_2-{\dot{x}}_1\right)+F_{impact}\\ m_2{\ddot{x}}_2\left(t\right)+c_2{\dot{x}}_2\left(t\right)+k_2{x}_2\left(t\right)=F_{l2}-k_2\left(x_2-x_1\right)-c_2\left({\dot{x}}_2-{\dot{x}}_1\right)-F_{impact}\end{array}\right.$$

(5)

where $m_1,c_1$, and $k_1$ are the mass, damping, and stiffness of the primary structure, respectively. ${\ddot{x}}_1\left(t\right)$, ${\dot{x}}_1\left(t\right)$, and $x_1\left(t\right)$ are its acceleration, velocity, and displacement response. Similarly, $m_2,c_2$, and $k_2$ denote the mass, damping, and stiffness of the PTMD, and ${\ddot{x}}_2\left(t\right)$, ${\dot{x}}_2\left(t\right)$, and $x_2\left(t\right)$ are the corresponding acceleration, velocity, and displacement. $F_{impact}$ denotes the nonlinear impact force when auxiliary mass $m_2$ impacts the delimiter. $F_{l1}$ and $F_{l2}$ are the loading forces added onto $m_1$ and $m_2$.

Suppose the distance between $m_2$ and the delimiter is $d_{gap}$. Then, the impact deformation can be calculated as follows:

$$\delta =x_2-x_1-d_{gap}$$

(6)

Additionally, the velocity of the impact deformation can be calculated as follows:

$$\dot{\delta }={\dot{x}}_2-{\dot{x}}_1$$

(7)

Then, the impact force $F_{impact}$ can be calculated as follows:

$$\left\{\begin{array}{c}{F}_{impact}={\beta }_{impact}\delta +c_{impact}\dot{\delta } \left(\mathrm{P}\mathrm{h}\mathrm{a}\mathrm{s}\mathrm{e} \mathrm{I}\right)\\ F_{impact}={\beta }_{impact}\delta \left(\mathrm{P}\mathrm{h}\mathrm{a}\mathrm{s}\mathrm{e} \mathrm{I}\mathrm{I}\right)\end{array}\right.$$

(8)

where ${\beta }_{impact}$ and $c_{impact}$ denote the impact stiffness and impact damping. Their values can be obtained from experimental tests. As shown in Equation (8), the impact force is calculated in two phases. Phase I refers to the colliding phase where $m_2$ penetrates into the delimiter, whereas Phase II is the restitution phase during which $m_2$ leaves the delimiter.

4. Consideration of Uncertainties

This study considers two types of uncertainties, namely, uncertain structural parameters and uncertain damper parameters. Since the tower was made mainly of Q235 and Q345 angle steel, structural uncertainties were considered regarding the material properties and geometry of the members, including the elastic modulus, Poisson’s ratio, mass density, yield strength, web thickness, and the width of the angle steel.

Table 2. Probability distribution of random variables.

Random Variable	Average Value	Standard Deviation	Distribution Type
Elastic modulus	206 GPa	6.18 GPa	Lognormal
Poisson’s ratio	0.3	0.009	Lognormal
Mass density	7800 kg/mm3		Deterministic
Yield strength for Q235	263.7 MPa	18.46 MPa	Lognormal
Yield strength for Q345	387.1 MPa	27.10 MPa	Lognormal
Web thickness	0.985	0.031	Normal
Width	1.001	0.008	Normal

lists the stochastic parameters of these factors.

The parameters of the PTMD constituted another source of uncertainty. Specifically, impact stiffness, denoted as ${\beta }_{impact}$, fluctuates with changes in environmental temperature. Additionally, repeated impacts also modify this value. Nevertheless, this parameter was excluded from the present study because prior parametric analysis indicated that vibration control performance is relatively insensitive to ${\beta }_{impact}$. Conversely, the gap between the auxiliary mass $m_2$ and the delimiter significantly impacts vibration control performance. As such, it was recognized as the key uncertain parameter in this study. However, due to the limited application of PTMDs in practical engineering, stochastic data on this parameter ($d_{gap}$) are currently unavailable. Essentially, $d_{gap}$ is a geometric parameter; thus, this paper assumes that it follows the same probability distribution and coefficient as the web thickness.

5. Simulation of Dynamic Hazards

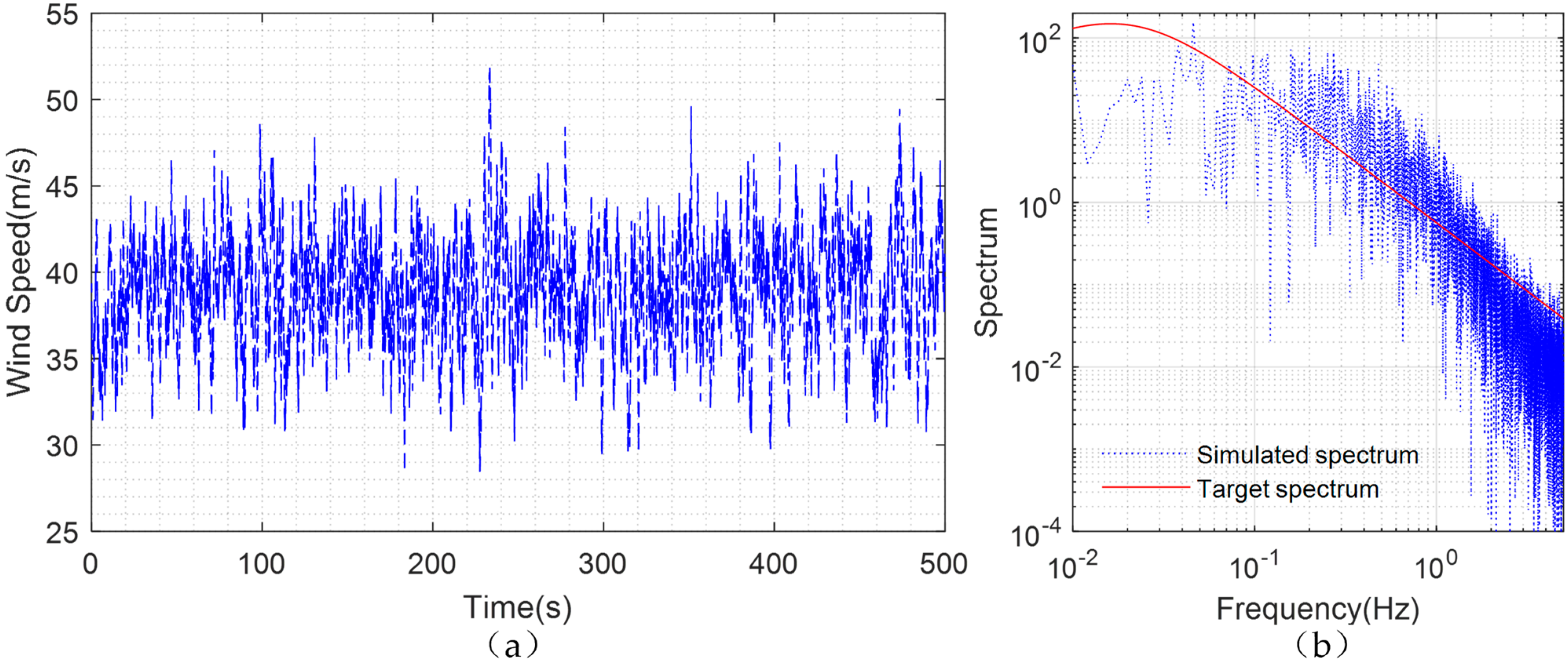
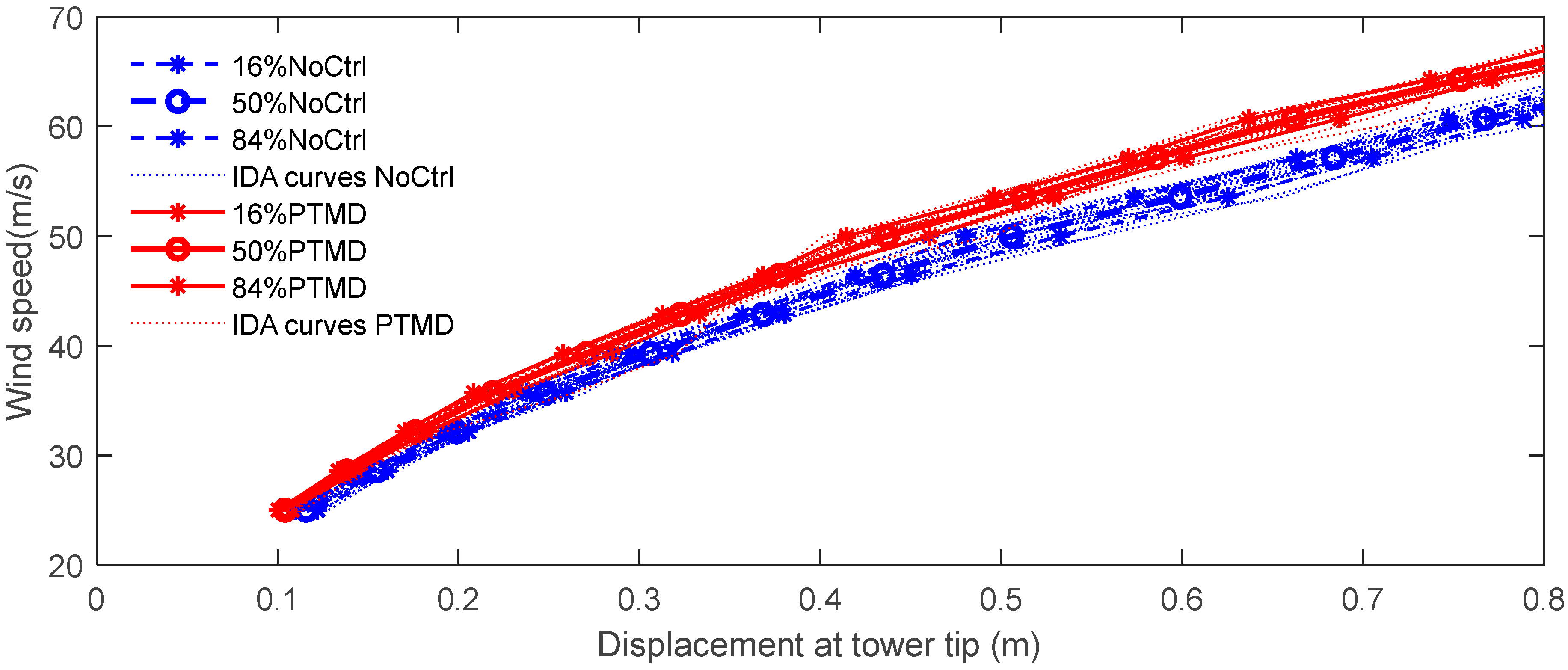
5.1. Simulation of Wind Loads

The time-varying wind speed is composed of a mean speed and a fluctuating speed, expressed as follows:

$$v\left(z,t\right)=\overline{v}\left(z\right)+v_f\left(z,t\right)$$

(9)

where $\overline{v}\left(z\right)$ is the mean wind speed, $v_f\left(z,t\right)$ is the fluctuating speed, and $z$ represents the height. The mean wind speed is considered a function of height. It can be calculated with the following formula:

$$\overline{v}\left(z\right)=v_{10}{\left({\displaystyle \frac{z}{10}}\right)}^{\alpha }$$

(10)

where $v_{10}$ is the mean wind speed at 10 m above the ground, and $\alpha$ is the power-law exponent, which is normally set to 0.15.

The fluctuating speed $v_f\left(z,t\right)$ can be simulated using the Davenport spectrum and the method proposed by Shinozuka et al. [26]. More details of this method can be found in [27]. Figure 9 shows the simulated wind speed at the top of the tower, when the basic wind speed $v_{10}=25 \mathrm{m}/\mathrm{s}$. Comparison of the simulated spectrum and the target spectrum validated the accuracy of this method.

Given the total wind speed at a simulation point of height $z$, the wind load at this point can be calculated using the following equation:

$$F_w={\displaystyle \frac{{\mu }_{s}A{v\left(z,t\right)}^2}{1.6}}$$

(11)

where $F_w$ represents the time-varying wind load, ${\mu }_s$ is the shape coefficient, which is taken to be 1.3, and $A$ is the projected area in the windward direction.

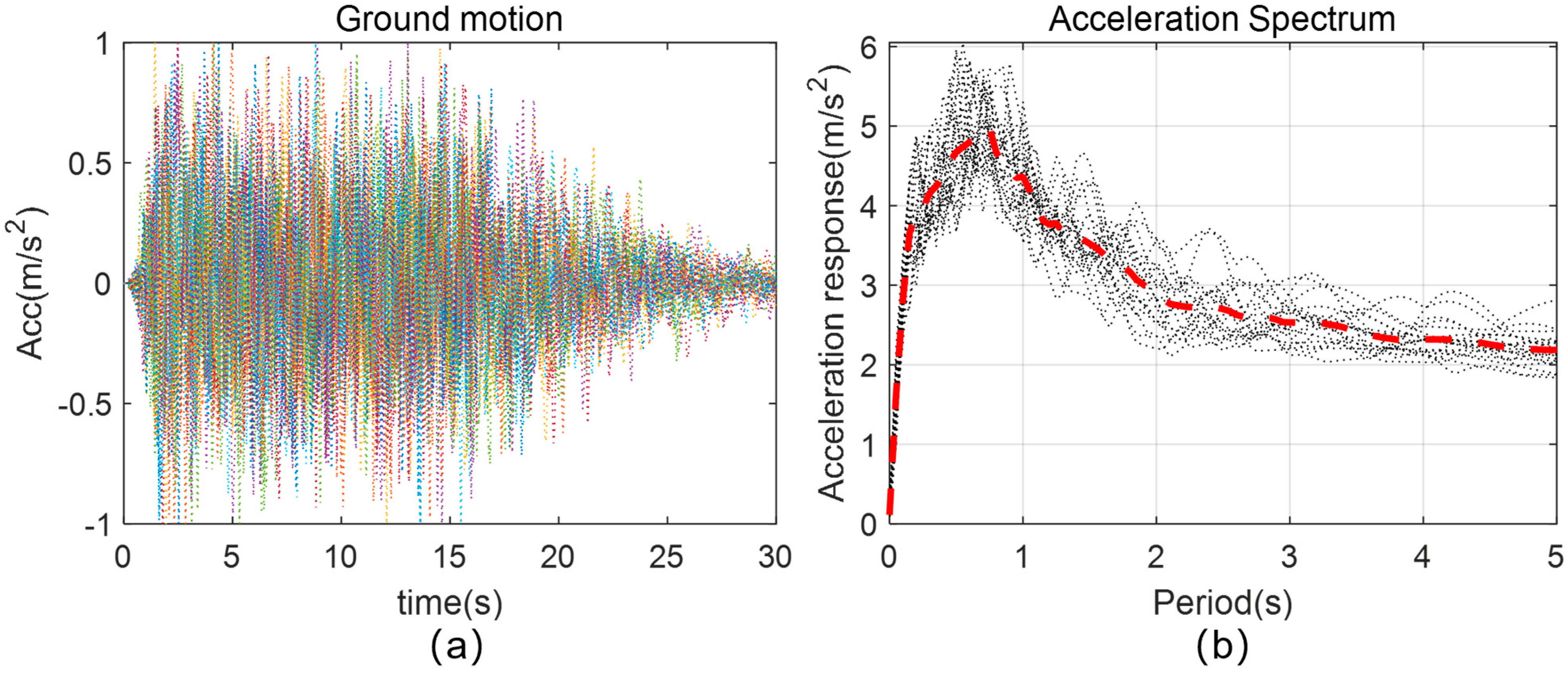
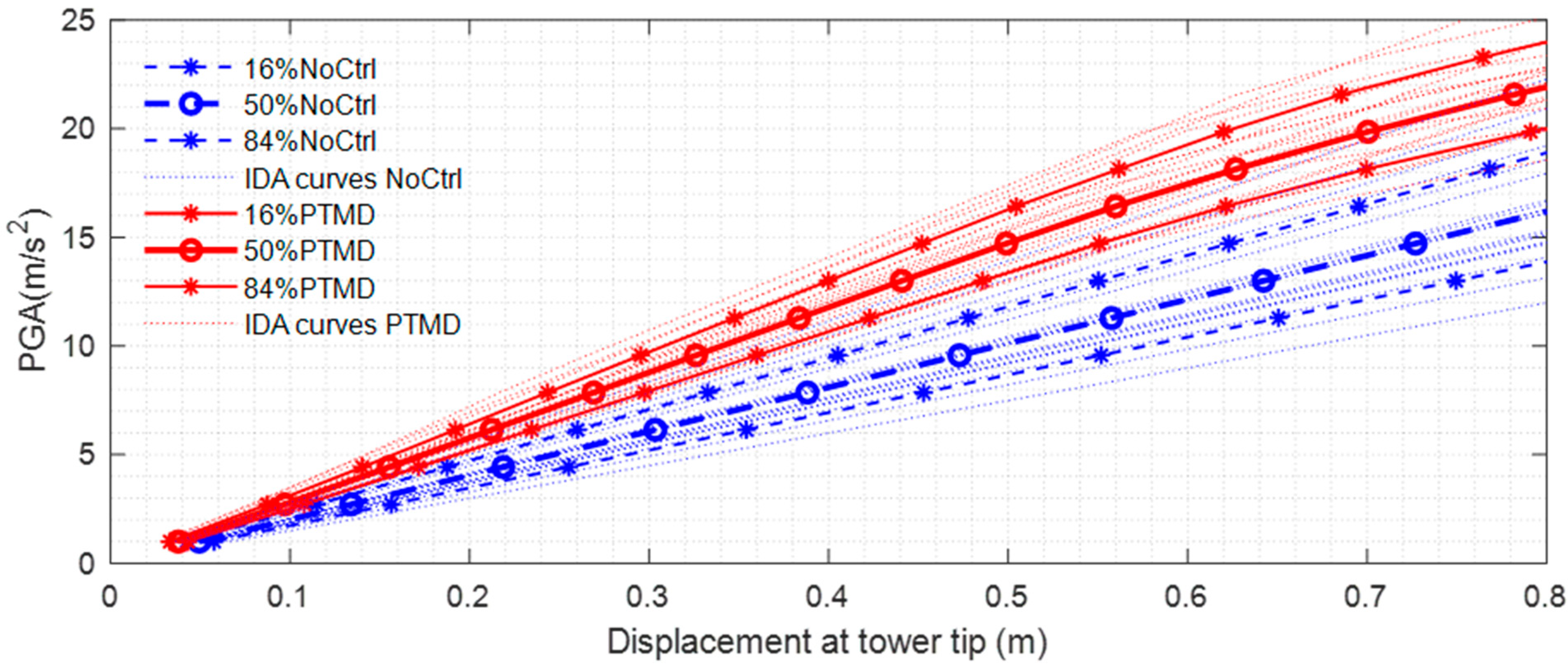
5.2. Simulation of Earthquake Actions

Earthquakes are highly random processes. To consider uncertainty in seismic load, this study simulated 20 ground motions using the following parameters: (1) characteristic period of the site = 0.4 s; (2) structural damping ratio = 5%; (3) duration of the earthquake = 30 s. Figure 10 shows the simulated ground motion record and the response spectrum.

6. Reliability Analysis

In this study, the probability of failure of a power transmission structure incorporating a PTMD was assessed by integrating its fragility curve with the corresponding seismic and wind hazard functions, thus enabling evaluation of its structural reliability. The fragility analysis was conducted using the incremental dynamic analysis (IDA) method. The hazard function comprehensively considered both seismic activity and wind load. The detailed procedure is outlined below.

6.1. Fragility Analysis

The fragility of the power transmission tower with and without a PTMD was calculated using incremental dynamic analysis (IDA) [28]. The core principle of IDA involves modulating the amplitude of excitations, such as earthquakes or wind load, and subjecting the structure to nonlinear time-history analysis. By progressively increasing the amplitude, this method allows comprehensive observation of the structure’s response, ranging from elastic deformation to potential collapse. The culmination of the IDA process is the generation of IDA curves, wherein each data point represents the outcome of an individual nonlinear time-history analysis. A solitary IDA curve indicates correlation between the excitation intensity measure (IM) and the structural damage measure (DM). Conversely, a collection of multiple IDA curves serves to illustrate variability in structural responses under diverse earthquake excitation or wind load.

The IDA method assumes that the engineering demand parameter (EDP) and the IM of the excitation can be defined by the following a log-linear relationship:

$$\ln \left( EDP\right)=\ln \left( b\right)+a\ln \left( IM\right)$$

(12)

where $a$ and $b$ are the regression coefficients obtained from linear regression. For a power transmission tower structure, the EDP normally refers to the maximum displacement at the top of the tower. The conditional probability for a transmission tower reaching a specific damage state is as follows:

$$P\left[EDP\ge LS|IM\right]=\mathsf{\Phi }\left({\displaystyle \frac{\ln \left( EDP\right)-\ln \left( LS\right)}{{\beta }_{\left(EDP|IM\right)}}}\right)$$

(13)

$${\beta }_{EDP|IM}=\sqrt{{\displaystyle \frac{\sum {\left[\mathit{ln}ED{P}_i-\left(a\right(\mathit{ln}(I{M}_i){)}^2+b\mathit{ln}(I{M}_i)\right]}^2}{n-2}}}$$

(14)

where LS represents engineering demand in a given damage state. For slight damage, it is set to 50% of the buckling displacement; for moderate damage, it is set to 75% of the buckling point; and collapse occurs at 100% of the buckling point. The determination of the buckling point involves conducting static nonlinear pushover analysis on the tower model. During the pushover analysis, the displacement at the top of the tower with the increasing load is recorded. By examining the pushover curve, a distinct buckling point can be identified, marking the transition from elastic deformation to plastic buckling of the tower structure (Figure 11).

6.2. Consideration of Hazards

Quantification of the hazard function of earthquakes and wind load is necessary to enable the reliability analysis of the power transmission tower-PTMD system.

Based on [29], the hazard function for seismic action can be expressed as follows:

$$H_q\left(IM\right)=k_0{IM}^{-k}$$

(15)

where IM denotes the intensity of the ground motion, and $k_0$ and $k$ are shape factors that can be obtained using the following equations:

$$k={\displaystyle \frac{\ln \left(p_1/p_2\right)}{\ln \left({IM}_2/{IM}_1\right)}}$$

(16)

$$\ln \left(k_0\right) ={\displaystyle \frac{\ln \left({IM}_1\right)\cdot \ln \left(p_2\right)-\ln \left({IM}_2\right)\cdot \ln \left(p_1\right)}{\ln \left({IM}_1/{IM}_2\right)}}$$

(17)

In Equation (15), the annual mean occurrence probabilities of ground motion under ${IM}_1$ and ${IM}_2$ are denoted as $p_1$ and $p_2$, respectively. In this study, $p_1$ and $p_2$ were determined in accordance with the current seismic design code for steel structures.

The hazard function of the wind load can also be determined following a similar procedure. According to the current design code, the basic wind speed is defined as the wind speed determined using probability statistics based on the average wind speed observed at a height of 10 m on open and flat ground for 10 min (i.e., $v_{10}$), with a maximum value determined by a 50-year return period. $v_{10}$ follows a Gumbel distribution, and its cumulative probability distribution function is expressed as follows:

$$H_w\left(v_{10}\right)=\exp \left\{\exp \left[-\alpha \left(x-u\right)\right]\right\}$$

(18)

$$\alpha ={\displaystyle \frac{1.28255}{\sigma }}$$

(19)

$$u=\mu -{\displaystyle \frac{0.57722}{\alpha }}$$

(20)

where $u$ represents the location parameter of the distribution, which is the mode of the distribution, $\alpha$ is the scale parameter of the distribution, and $\mu$ and $\sigma$ are the mean value and standard deviation of the sample, respectively.

The probability density function in relation to Equation (18) is as follows:

$$d\left[H_w\left(v_{10}\right)\right]=\left(1-\alpha \right)·\exp \left[-{\displaystyle \frac{x-u}{\alpha }}-\exp \left(-{\displaystyle \frac{x-u}{\alpha }}\right)\right]$$

(21)

where have the same meanings as those employed in Equation (18).

6.3. Reliability Analysis Based on Fragility and Harzard Analysis

The probability of a structure’s failure can be calculated by integrating the hazard function and cumulative density function of the fragility curve. Specifically, for seismic induced failure, the following applies:

$$P_{f,q}=\int F_{q}d\left[H_q\left(x\right)\right]$$

(22)

and for wind induced failure:

$$P_{f,w}=\int F_{w}d\left[H_w\left(x\right)\right]$$

(23)

where $P_{f,q}$ and $P_{f,w}$ denote the failure probability regarding earthquake activity and wind load, respectively. $F_q$ and $F_w$ are the fragility functions, determined using Equation (13), and $H_q\left(x\right)$ and $H_w\left(x\right)$ are the hazard functions of earthquake and wind load.

Based on Equations (22) and (23), the overall failure probability of the power transmission tower during its whole service life can be determined as follows:

$$P_f=1-\left[\left(1-P_{f,q}\right)·\left(P_{f,w}\right)\right]$$

(24)

where $P_f$ denotes the probability of failure, which can be further applied to express reliability as follows:

$$\beta =1-{\mathsf{\Phi }}^{-1}\left[1-P_f\right]$$

(25)

7. Results and Discussion

The primary findings of this study indicate that installation of PTMDs can significantly reduce the dynamic response of transmission towers subjected to both seismic and wind load. This reduction in dynamic response is reflected in the numerical results presented in this paper, demonstrating a substantial decrease in the displacement and acceleration of the tower under various loading conditions.

The significance of these findings lies in their implications for the design and maintenance of transmission towers. By effectively mitigating vibrations induced by earthquakes and wind, PTMDs have the potential to enhance the structural safety and durability of transmission towers, thereby reducing the risk of damage and failure. This is particularly important given the critical role transmission towers play in ensuring the reliable delivery of electrical power.

In comparison to existing research, the present study introduces a novel approach for evaluating the effectiveness of PTMDs in multi-hazard contexts. By considering both seismic activity and wind load, this study provides a more comprehensive assessment of PTMD performance than previous studies, which have typically focused on a single type of hazard.

It should be noted that the proposed reliability-driven design framework currently relies on numerical simulations without systematic experimental validation. This limitation stems primarily from the challenges in replicating the strongly nonlinear structural behaviors under extreme loads (e.g., seismic and wind-induced dynamic effects) and the stochastic nature of real-world environmental conditions in controlled laboratory settings. Future efforts will prioritize three experimental validation pathways: (1) collaboration with power grid operators to conduct long-term monitoring of in-service towers to capture real-world responses under extreme loads; (2) destructive testing of critical components (e.g., buckling-prone members, high-stress connections) at laboratory scale; and (3) probabilistic modeling of manufacturing tolerances and material variability to enhance the comparability of numerical and experimental data. This phased hybrid validation strategy aims to systematically improve the method’s engineering applicability while maintaining cost-effectiveness.

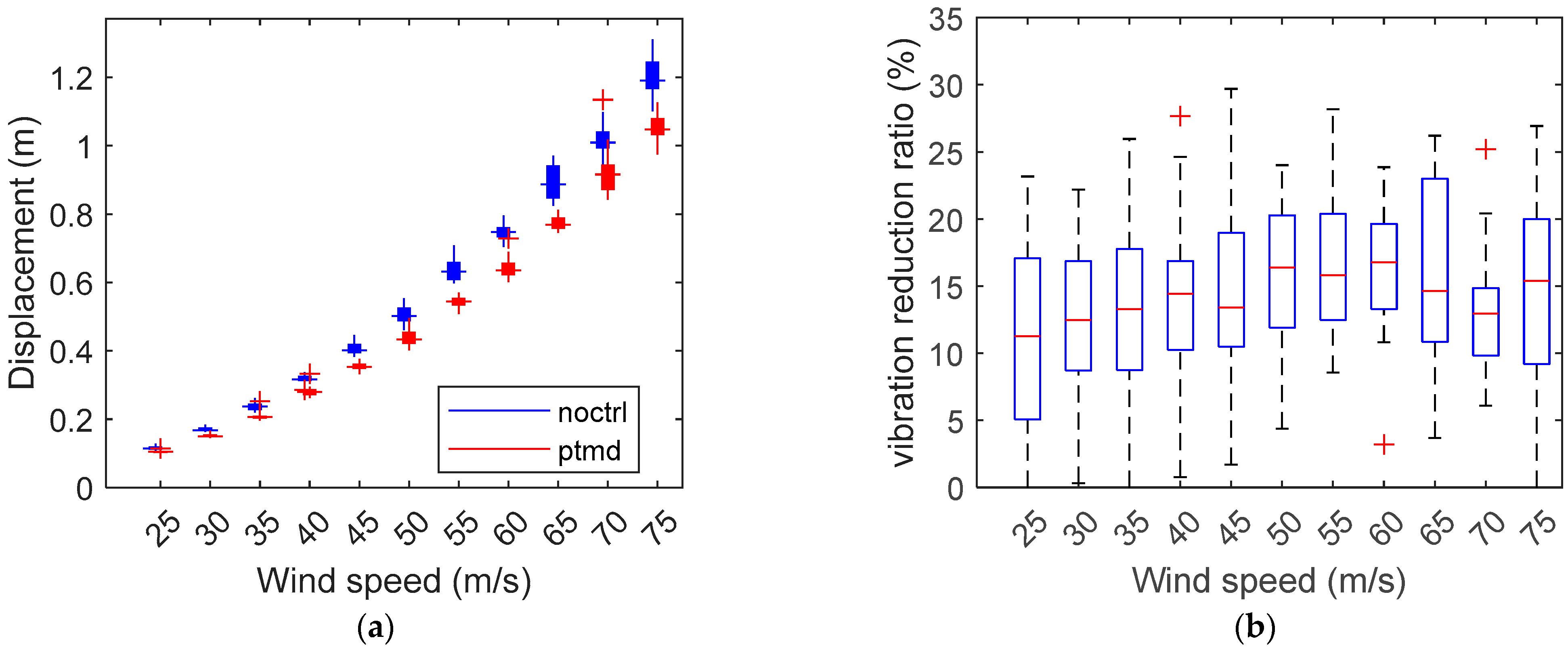
7.1. Performace Evaluation Based on Time-Domain Response

The displacement at the apex of the power transmission tower is frequently chosen as the key parameter for assessing structural safety. Although acceleration is occasionally taken into account to evaluate occupants’ comfort during moderate earthquakes or wind events, this was not a consideration in the current study because power transmission towers are not designed for human habitation.

Figure 12 presents an overview of the displacement responses of all structure samples subjected to varying levels of wind. In Figure 12a, a box plot compares the displacements with and without control, showing that the maximum displacement increases as the basic wind speed $v_{10}$ rises from 25 m/s to 75 m/s. Across all windspeed conditions, the responses observed with the implementation of a PTMD were consistently smaller than those in the non-control case. Figure 12b describes the vibration reduction ratio. The data reveal that the PTMD effectively reduced wind-induced vibrations. Specifically, the reduction in vibration fluctuated between 10% and 30%, with a more pronounced reduction effect at higher wind speeds. This further underscores the significant efficacy of PTMDs in controlling structural vibrations, particularly under high-windspeed conditions.

Similarly, Figure 13 showcases the results pertaining to seismic-induced vibrations. Panel (a) features a box plot that compares the displacement of structures with and without control measures. As the peak ground acceleration (PGA) increases from 0.1 m/s² to 25 m/s², the maximum displacement also escalates. However, across all PGA conditions, the responses observed with the implementation of the PTMD were consistently lower than those without control. Panel (b) of Figure 13 presents the vibration reduction ratio, demonstrating that the PTMD effectively dampened earthquake-induced vibrations. The reduction in vibration ranged from 20% to 80%, with a more pronounced reduction effect at higher PGAs. Comparing Figure 12b and Figure 13b, it is evident that the reduction ratio relating to seismic activity is greater than for wind load, suggesting that the PTMD is more effective in mitigating the effects of earthquakes.

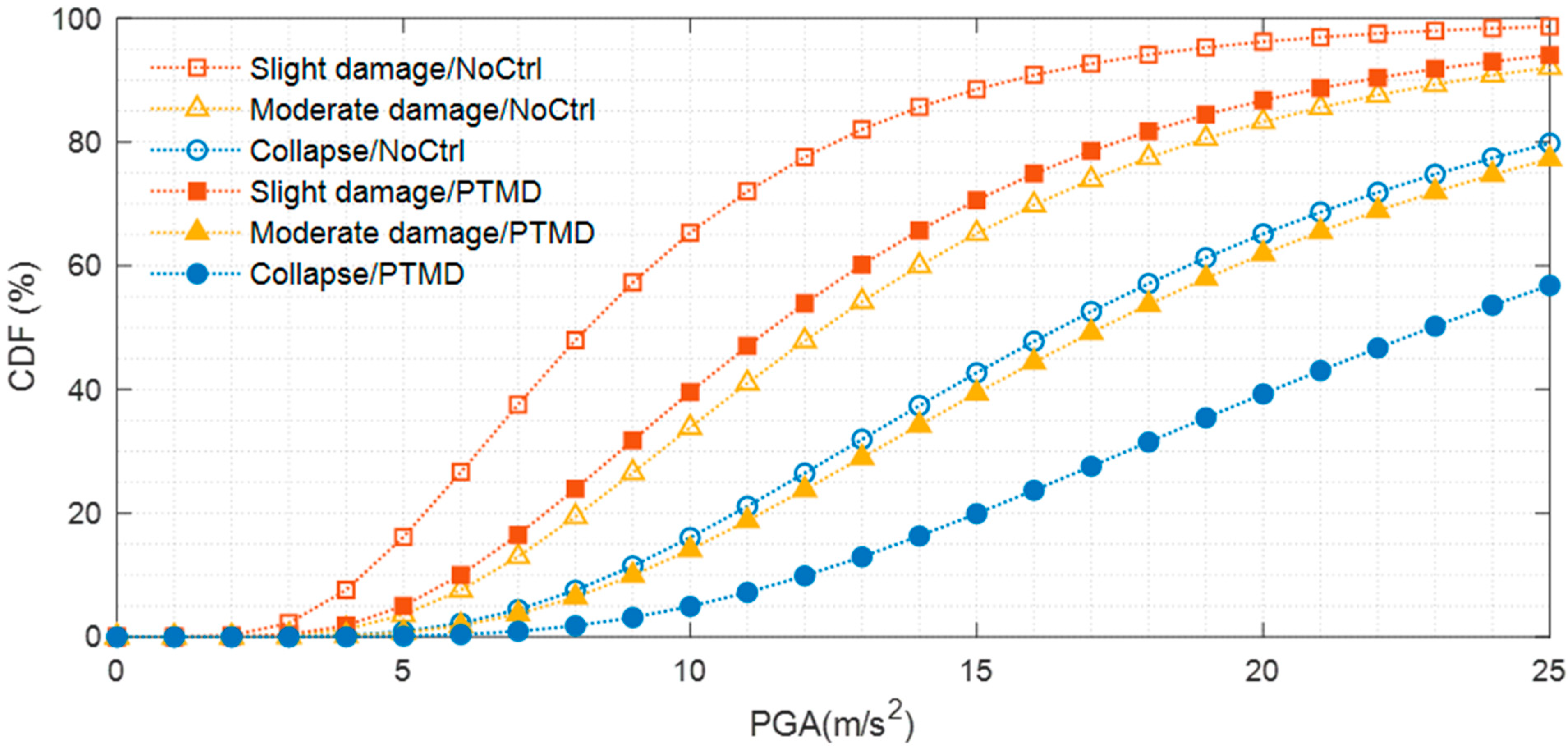
7.2. Fragility Curves with and Without Control

Figure 14 shows the IDA curves for the power transmission tower with and without the PTMD, under wind load. The blue symbols and lines denote the no-control conditions, revealing a positive correlation between increasing wind speed and structural displacement. In contrast, the introduction of PTMD control, represented by red symbols and lines, significantly mitigated this displacement. Figure 15 presents the fragility curves computed using Equation (11), quantifying the probability of different damage states as a function of wind speed. Both figures collectively demonstrate the effectiveness of the PTMD in mitigating wind-induced failure. For instance, at a wind speed of 75 m/s, the power transmission tower without the PTMD had a high 75.0% probability of experiencing collapse (Collapse/NoCtrl curve). However, when the PTMD was installed, the failure probability dropped significantly to 70.1% (Collapse/PTMD curve), indicating a notable reduction in the likelihood of structural failure.

Similar results are evident in both Figure 16 and Figure 17, which present the calculated outcomes of the earthquake-induced vibrations. The dashed red lines represent the IDA curves for the tower with the PTMD, all of which are elevated compared with the blue dashed lines, thereby highlighting the damping capability of the PTMD during seismic events. Compared with Figure 14 (depicting the wind load scenario), the IDA curves under seismic activity exhibit greater dispersion, suggesting that the uncertainty associated with earthquake activity significantly influences the seismic response. Therefore, the selection of earthquake records should be given careful consideration in structural design.

Figure 17 presents the fragility curves of the power transmission tower subjected to earthquakes, both with and without the incorporation of the PTMD; these results imply that a PTMD can effectively reduce the likelihood of failure during seismic events. For instance, when the tower is subjected to a ground motion of 25 m/s², the probability of failure soars to 79.7%; however, with the addition of a PTMD, this probability drops to just 56.8%.

7.3. Structural Reliability with and Without Control

This study considers a power transmission tower-line system with the following wind load and seismic activity parameters: 50-year recurrence interval; basic wind speed $v_{10}=25.3 \mathrm{m}/\mathrm{s}$; mean value $\mu =14.591 \mathrm{m}/\mathrm{s}$; standard deviation $\sigma =4.115 \mathrm{m}/\mathrm{s}$; scale parameter $\alpha =3.2085 \mathrm{m}/\mathrm{s}$; location parameter $u=12.739 \mathrm{m}/\mathrm{s}$; and shape factors for seismic hazard k = 2.3219 and $k_0=4.7651\times {10}^{-5}$.

Table 3. Reliablility of the power transmission tower with and without PTMD, considering both wind load and seismic activity.

Service Life (Year)	Wind		Seismic		Wind + Seismic
	No Ctrl	PTMD	No Ctrl	PTMD	No Ctrl	PTMD
1	3.4400	3.6124	5.1700	5.3467	3.4399	3.6123
30	2.3786	2.6089	4.4930	4.6934	2.3784	2.6088
50	2.1851	2.4296	4.3830	4.5879	2.1849	2.4295
100	1.9006	2.1686	4.2296	4.4410	1.9005	2.1685

presents a summary of the reliability indicator β for the power transmission tower, both with and without a PTMD. The following conclusions can be drawn from the table:

• As the service life extends from 30 to 100 years, a decrease in reliability is observed. Specifically, in the absence of control measures, $\beta$ is 3.4399 for a 1-year service life. However, when the tower is expected to operate for 100 years, $\beta$ drops significantly to 1.9005;
• Comparing wind load to earthquakes, wind load emerged as the dominant factor. For instance, with a 50-year service life, the reliability $\beta$ is 2.1851 when only wind load is considered. Conversely, the tower’s reliability under earthquake conditions reaches 4.3830, indicating that the tower is more susceptible to damage from wind load than from earthquakes. Considering both wind and earthquake load, the reliability factor $\beta$ is slightly lower at 2.1849 compared with the wind-only case ($\beta$= 2.1851);
• In all scenarios, the PTMD exhibited a satisfactory damping effect. For example, it enhanced the reliability from 2.1849 to 2.4295 under combined wind and earthquake load, demonstrating its effectiveness in providing dual control against vibrations and seismic activity.

8. Conclusions

Power transmission tower-line systems face significant challenges from various dynamic hazards, notably, wind and earthquakes. Despite the proposal of numerous damping solutions, effective dual control systems for both seismic and wind-induced vibrations have been largely unexplored. This study introduces a comprehensive framework for assessing the reliability of power transmission towers under a spectrum of dynamic disasters, including earthquakes and wind load. Through employing a lifelong reliability approach, the efficacy of a PTMD was rigorously evaluated. The following conclusions can be drawn from the results of this study:

(1)

While deterministic structural analysis demonstrated the damping ability of the PTMD using the vibration reduction ratio, this study further verified the effectiveness of the PTMD when considering structural uncertainties and excitation uncertainties;

(2)

This paper proposes utilizing reliability as a metric to assess the effectiveness of dampers in enhancing structural safety. With the implementation of the PTMD, the lifelong reliability of the power transmission tower increased from 2.1849 to 2.4295;

(3)

The proposed method takes into account the influence of multiple dynamic disasters. The results indicate that the PTMD is effective for dual control of seismic and wind-induced vibrations;

(4)

The PTMD utilized in this study was not optimized. However, reliability can serve as a valuable target for optimizing PTMDs and other damping devices, with the potential to further improve performance in dual seismic and vibration control.

The present study focused on wind- and earthquake-induced vibrations. Meanwhile, the proposed methodology shows potential for assessing hazards from other excitation sources such as traffic, machinery, or human activities. Additionally, the reliability-based optimization framework is not restricted to the investigated transmission tower configuration; it can be extended to other tower types and structural systems by adjusting the design parameters and performance criteria.

The current PTMD design parameters represent an initial stage, requiring further optimization through advanced simulations and experiments to maximize the dual-control system’s effectiveness. Future work will refine these parameters to enhance resilience against earthquakes and wind-induced vibrations, with practical implementations. Subsequent studies will integrate real-time structural health monitoring (SHM) capabilities into PTMD control frameworks, enabling adaptive damping strategies under operational uncertainties. This integration will embed sensor-driven feedback into adaptive control algorithms, advancing responsive vibration regulation in dynamic environments.