Study on Flexural Capacity of UHPC-NC Composite Slab with Reinforced Truss in the Normal Section
Abstract
1. Introduction
2. Numerical Model of the Cross-Shaped-Steel-Reinforced RPC Column
2.1. Constitutive Relationship of Materials
2.2. Establishment of the Numerical Model
3. Verification of the FEM
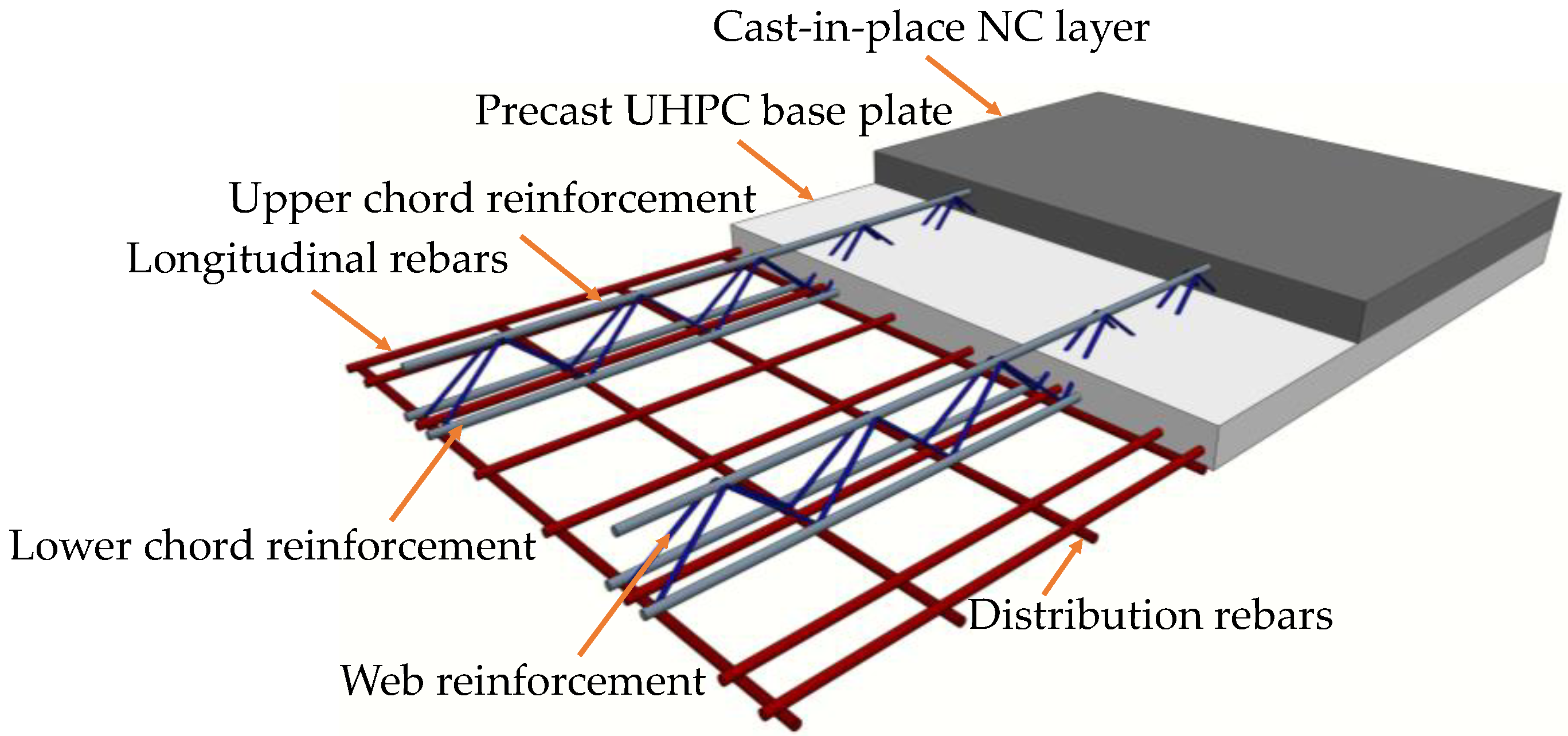
3.1. Reinforced Truss NC Composite Slab
3.2. Reinforced Truss UHPC Composite Slab
4. FE Analysis of Reinforced Truss UHPC-NC Composite Slab
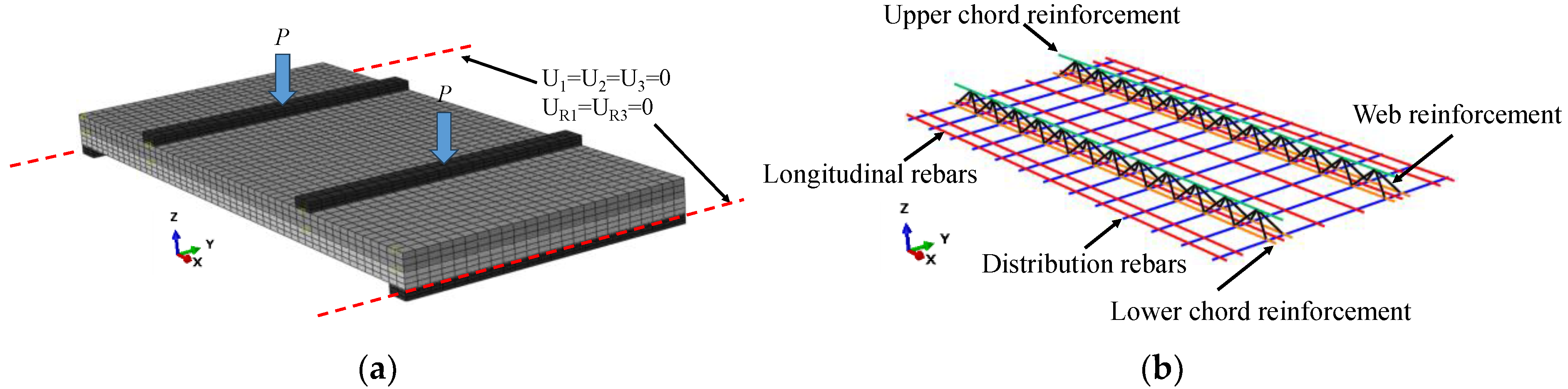
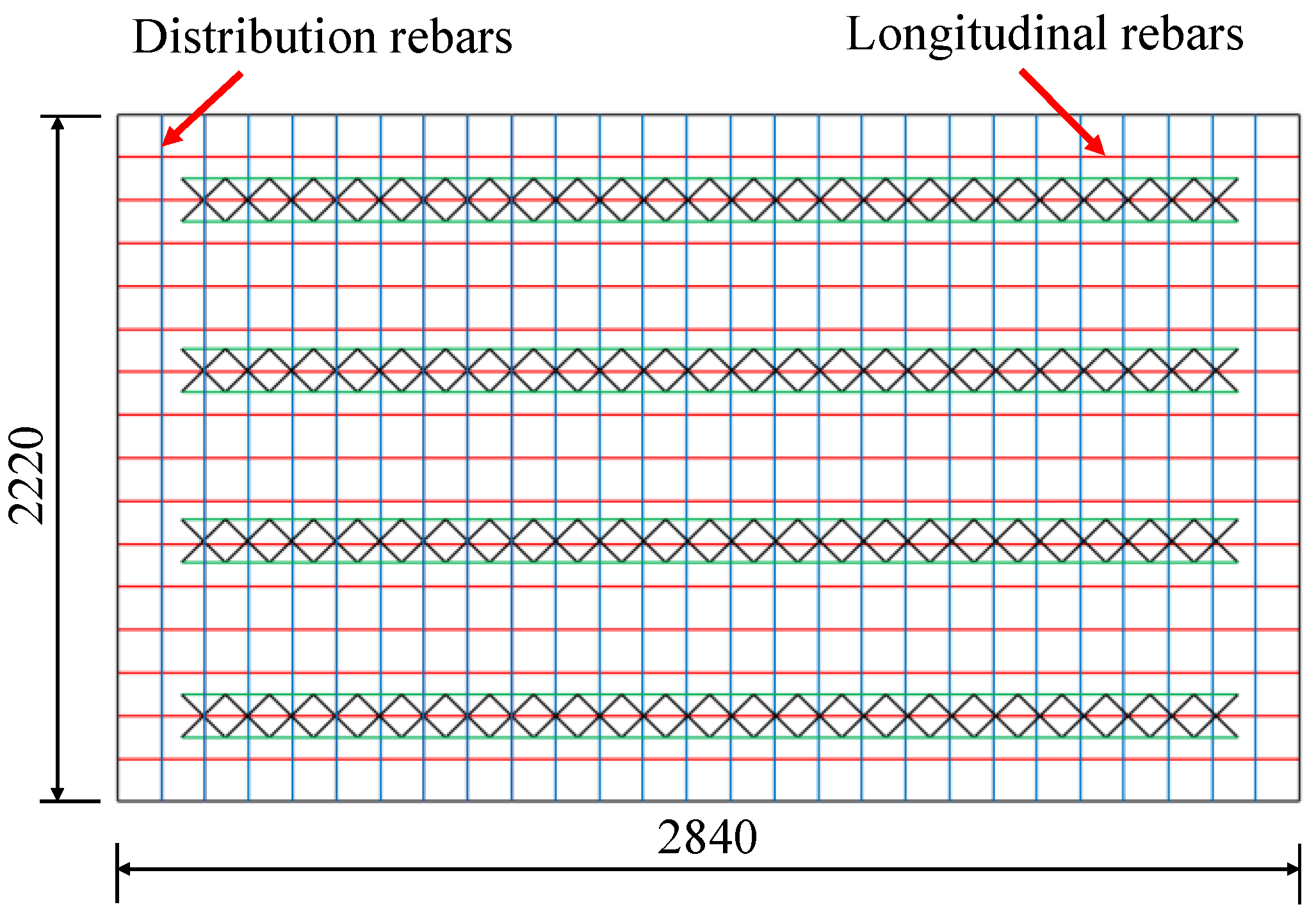
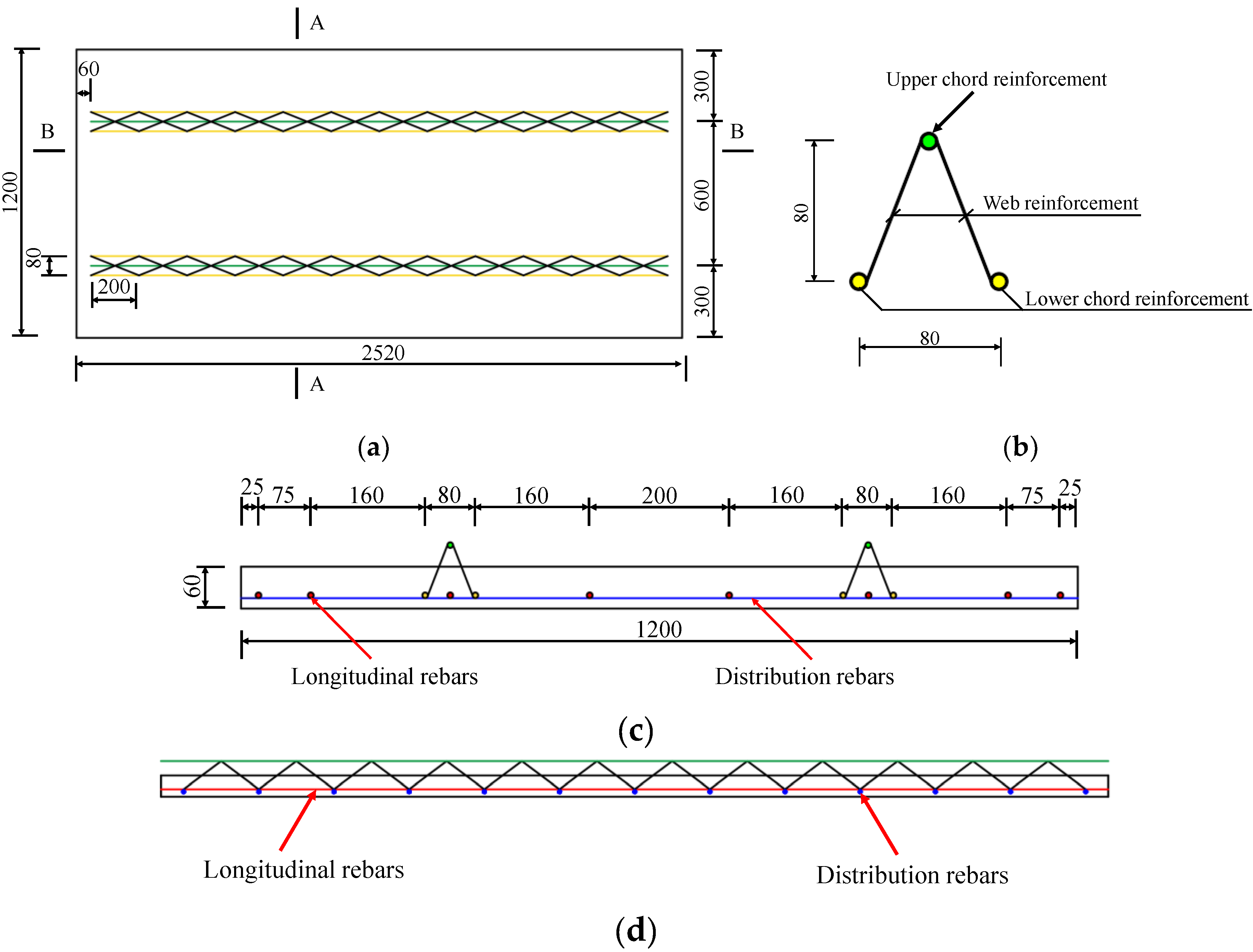
4.1. Scheme of the FEM
 represents the reinforcement strength grade of HRB400.
represents the reinforcement strength grade of HRB400.4.2. Mid-Span Deflection–Load Curve
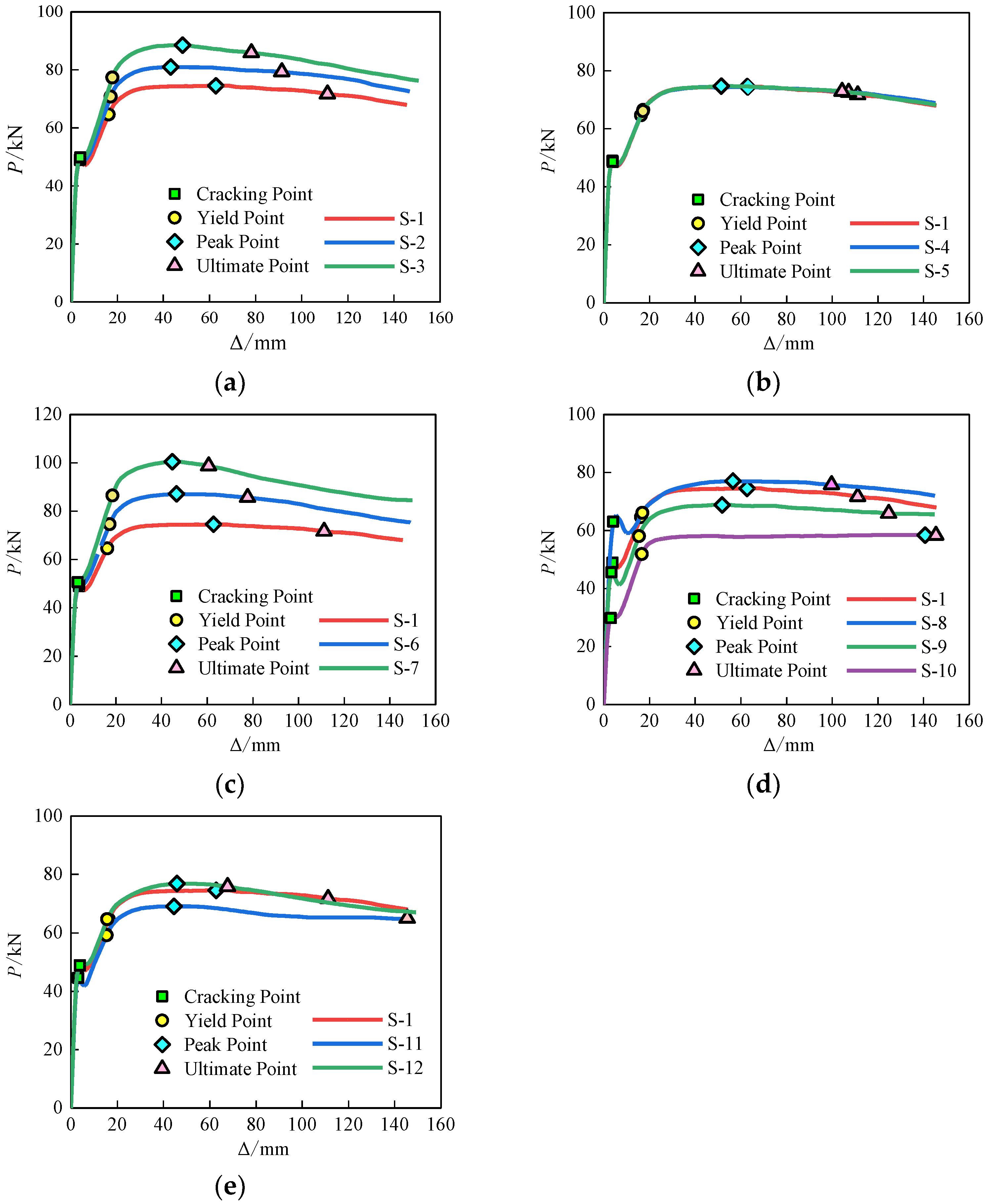
4.3. Analysis of Different Parameters
4.3.1. Cross-Sectional Area of the Lower Chord Reinforcement
4.3.2. Cross-Sectional Area of the Upper Chord Reinforcement
4.3.3. Reinforcement Ratio of Longitudinal Reinforcement in the Precast Base Plate
4.3.4. Concrete Strength Grade of the Precast Base Plate
4.3.5. Thickness of the Precast UHPC Base Plate
4.4. Full Process Analysis of Load-Bearing Behavior
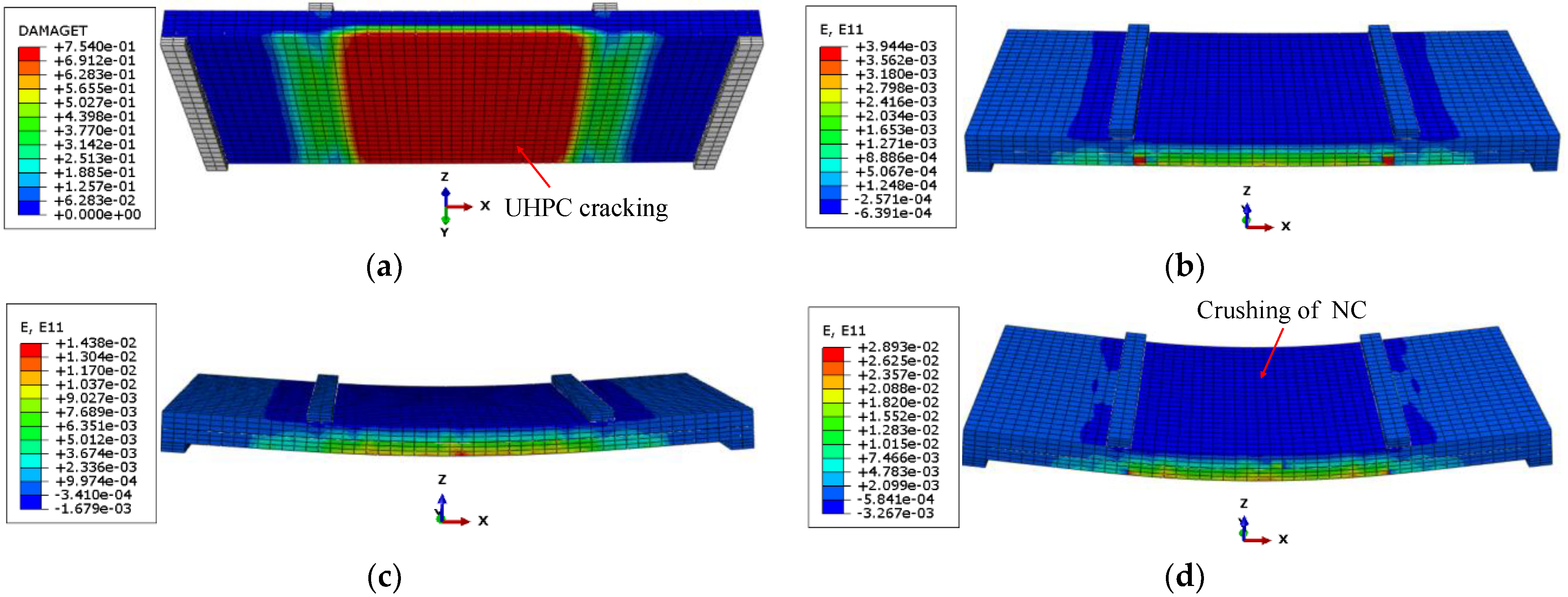
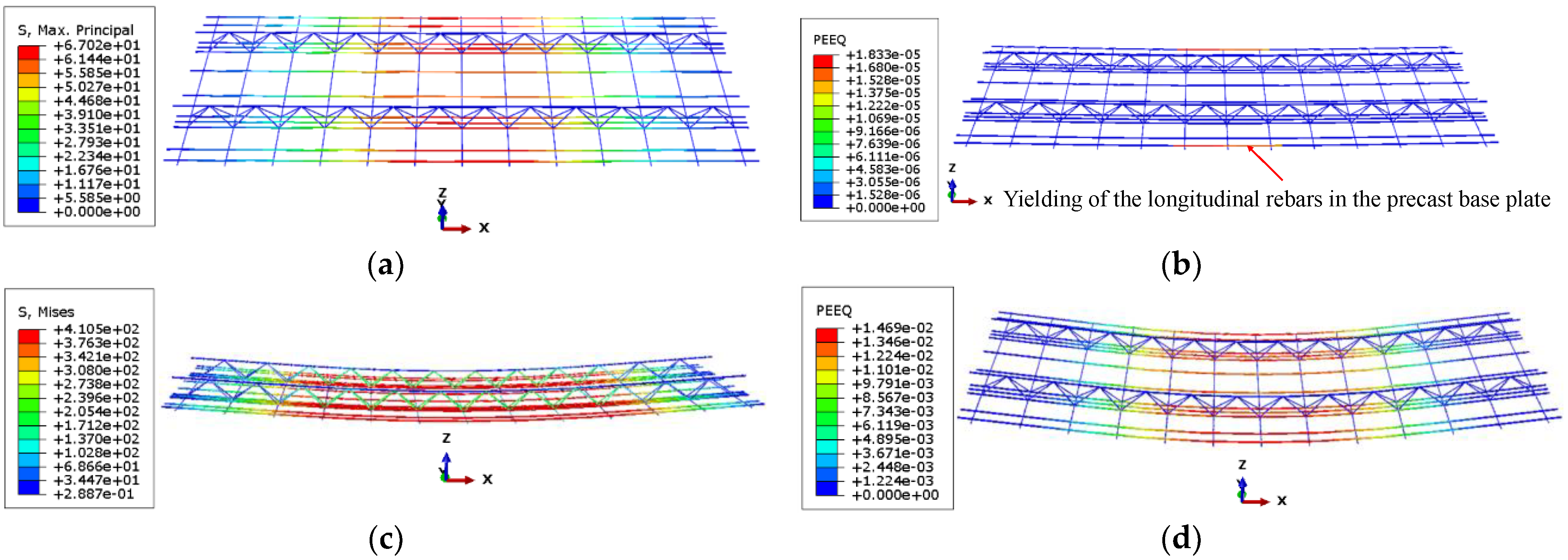
4.4.1. Cracking of UHPC
4.4.2. Yielding of Longitudinal Reinforcement
4.4.3. Peak Load
4.4.4. Ultimate Load
5. Calculation of Flexural Capacity of the Normal Section
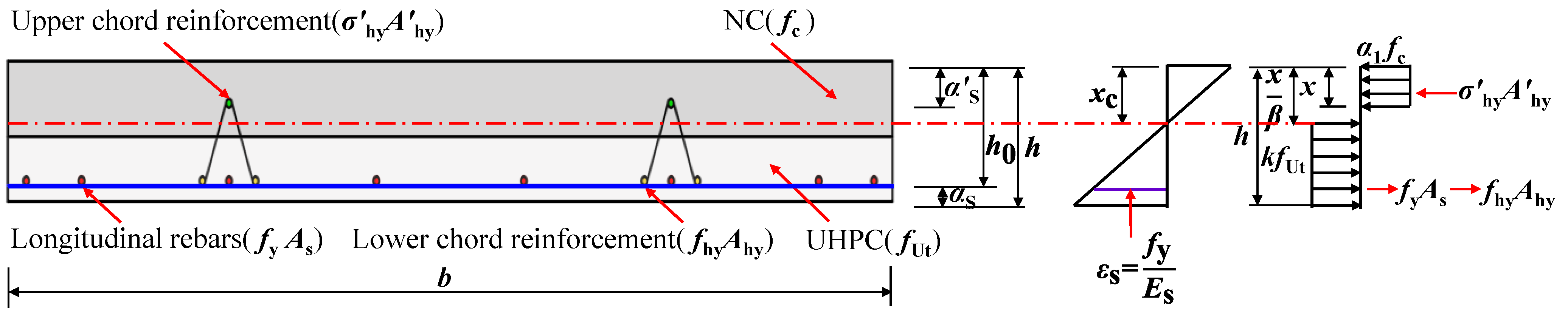
5.1. Basic Assumptions
5.2. Calculation Formula for Flexural Capacity of the Normal Section
5.3. Comparison of Results
6. Discussion
7. Conclusions
- 1.
- FE analyses were conducted to validate the flexural performance of UHPC-NC composite slabs with reinforced trusses. The results showed strong agreement between simulated and experimental values, confirming the reliability of the model.
- 2.
- Increasing the area of lower chord reinforcement and the longitudinal reinforcement ratio significantly enhanced both yield and ultimate loads. Specifically, increasing the lower chord reinforcement diameter from 8 mm to 10 mm and 12 mm raised the yield load by 9.61% and 19.64%, and the ultimate load by 10.72% and 19.93%. Similarly, increasing the longitudinal reinforcement ratio in the precast bottom slab from 0.32% to 0.50% and 0.73% improved the yield load by 15.49% and 33.80%, as well as the ultimate load by 19.48% and 37.70%. In contrast, the effect of upper chord reinforcement area was limited.
- 3.
- As the concrete strength grade of the precast base plate increased, the cracking, yield, and ultimate loads of the composite slab were significantly enhanced. Specifically, when the concrete strength grade increased from C30 to C100, C120, and C140, the cracking load increased by 53.17%, 64.36%, and 73.97%, respectively. The yield load increased by 11.79%, 24.51%, and 27.38%, while the ultimate load increased by 12.83%, 22.77%, and 29.87%.
- 4.
- When the thickness of the UHPC base plate increased from 40 mm to 60 mm and 80 mm, the cracking load increased by 9.59% and 9.41%, respectively; the yield load increased by 9.14% and 9.09%, respectively; and the ultimate load increased by 10.43% and 16.78%, respectively.
- 5.
- A calculation formula for the flexural capacity of UHPC-NC composite slabs was proposed. The ratio of calculated to simulated values averaged 1.005, with a mean square deviation of 0.0521 and a coefficient of variation of 0.0518, indicating good reliability. This formula provides a theoretical basis for the design of such slabs.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Al-Mukhtar, A. The Effected Parameters for Designing The Single Layer Composite Materials. Int. J. Mech. Eng. Rob. Res. 2012, 1, 359–370. [Google Scholar]
- Li, S.; Li, C.Z.; Xiong, M.; Zhen, Y.; Guo, Z. Performance of Prefabricated Construction: A Critical Review From 2010 to 2020. In International Symposium on Advancement of Construction Management and Real Estate, Beijing, China, 20–22 November 2021; Springer: Singapore, 2022; pp. 1013–1027. [Google Scholar]
- Jiang, T.; Yang, Z.; Wang, J.; Zhao, Y. Behavior of fiber-reinforced ultra-high-performance concrete beams subjected to bending. J. Build. Eng. 2022, 49, 104080. [Google Scholar]
- Cao, X.; Xie, X.; Zhang, T.; Du, G. Bond-slip behavior between high-strength steel tube and Ultra-high Performance Concrete. In Structures; Elsevier: Amsterdam, The Netherlands, 2023; Volume 47, pp. 1498–1510. [Google Scholar]
- Zheng, Y.; Lv, X.; Hu, S.; Zhuo, J.; Wan, C.; Liu, J. Mechanical properties and durability of steel fiber reinforced concrete: A review. J. Build. Eng. 2023, 82, 108025. [Google Scholar] [CrossRef]
- Meng, W.; Khayat, K.; Bao, Y. Flexural Behaviors of Fiber-Reinforced Polymer Fabric Reinforced Ultra-High-Performance Concrete Panels. Cem. Concr. Compos. 2018, 93, 43–53. [Google Scholar] [CrossRef]
- Zeng, J.J.; Zeng, W.B.; Ye, Y.Y.; Liao, J.; Zhuge, Y.; Fan, T.H. Flexural Behavior of FRP Grid Reinforced Ultra-High-Performance Concrete Composite Plates with Different Types of Fibers. Eng. Struct. 2022, 272, 115020. [Google Scholar] [CrossRef]
- Wu, C.; Wang, X.; Zhang, Z.; Zou, Y.; Yang, J.; Jin, M.; Lun, X. Flexural Behavior of Embedded GFRP Grid Framework-UHPC Composite Plate without Steel Rebar. Case Stud. Constr. Mater. 2024, 20, e02900. [Google Scholar] [CrossRef]
- Dogu, M.; Menkulasi, F. Punching Shear Strength of Post-Tensioned Ultra-High-Performance Concrete Plates. ACI Struct. J. 2022, 119, 165–178. [Google Scholar] [CrossRef]
- Mészöly, T.; Ofner, S.; Randl, N. Effect of Combining Fiber and Textile Reinforcement on the Flexural Behavior of UHPC Plates. Adv. Mater. Sci. Eng. 2020, 2020, 9891619. [Google Scholar] [CrossRef]
- Wang, Y.; Shao, X.; Cao, J.; Zhao, X.; Qiu, M. Static and Fatigue Flexural Performance of Ultra-High Performance Fiber Reinforced Concrete Slabs. Eng. Struct. 2021, 231, 111728. [Google Scholar] [CrossRef]
- Sun, Z.; Li, X.; Liu, C. Study on the Flexural Performance of Ultrahigh-Performance Concrete–Normal Concrete Composite Slabs. Materials 2024, 17, 4675. [Google Scholar] [CrossRef] [PubMed]
- Yin, H.; Teo, W.; Shirai, K. Experimental Investigation on the Behaviour of Reinforced Concrete Slabs Strengthened with Ultra-High Performance Concrete. Constr. Build. Mater. 2017, 155, 463–474. [Google Scholar] [CrossRef]
- Yan, B.; Qiu, M.; Zeng, T.; Shao, X.; Zhu, Y.; Li, M. Full-Scale Experimental Verification of UHPC-RC Composite Slab Culvert with a Clear Span of 8 m. J. Bridge Eng. 2020, 25, 05020010. [Google Scholar] [CrossRef]
- Zhu, Y.; Zhang, Y.; Hussein, H.H.; Chen, G. Numerical Modeling for Damaged Reinforced Concrete Slab Strengthened by Ultra-High Performance Concrete (UHPC) Layer. Eng. Struct. 2020, 209, 110031. [Google Scholar] [CrossRef]
- Hoang, V.H.; Do, T.A.; Tran, A.T.; Nguyen, X.H. Flexural Capacity of Reinforced Concrete Slabs Retrofitted with Ultra-High-Performance Concrete and Fiber-Reinforced Polymer. Innov. Infrastruct. Solut. 2024, 9, 113. [Google Scholar] [CrossRef]
- Zhang, J.; Li, Q.; Wu, Z.; Ma, Z. Numerical investigation on the flexural behavior of ultra-high performance fiber reinforced concrete (UHPFRC) beams. Eng. Struct. 2018, 156, 271–283. [Google Scholar]
- Zheng, W.; Li, L.; Lu, S. Investigation on Flexural Capacity of Reinforced Ultra-High Performance Concrete Beams. Eng. Mech. 2011, 32, 125–134. (In Chinese) [Google Scholar]
- Guo, Z. Experimental Foundations and Constitutive Relationships of Concrete Strength and Deformation; Tsinghua University Press: Beijing, China, 1997. (In Chinese) [Google Scholar]
- Li, Z. Experimental Study and Industrialized Construction Technology of Truss Reinforced Concrete Composite Slab. Master’s Thesis, Xi’an University of Architecture and Technology, Xi′an, China, 2020. (In Chinese). [Google Scholar]
- Lv, X.; Wang, Y.; Fu, C.; Zheng, W. Basic mechanical property indexes of reactive powder concrete. J. Harbin Inst. Technol. 2014, 46, 1–9. [Google Scholar]
- Prem, P.; Murthy, A. Acoustic emission and flexural behaviour of RC beams strengthened with UHPC overlay. Constr. Build. Mater. 2016, 123, 481–492. [Google Scholar] [CrossRef]
- Safdar, M.; Matsumoto, T.; Kakuma, K. Flexural behavior of reinforced concrete beams repaired with ultra-high performance fiber reinforced concrete (UHPFRC). Compos. Struct. 2016, 157, 448–460. [Google Scholar] [CrossRef]
- Wahalathantri, B.; Thambiratnam, D.; Chan, T.; Fawzia, S. A material model for flexural crack simulation in reinforced concrete elements using ABAQUS. In Proceedings of the First International Conference on Engineering, Designing and Developing the Built Environment for Sustainable Wellbeing, Brisbane, Australia, 27–29 April 2011; Queensland University of Technology: Brisbane, Australia, 2011; pp. 260–264. [Google Scholar]
- GB 50010-2010; Code for Design of Concrete Structures. China Building Industry Press: Beijing, China, 2010.
- Wang, K.; Zhu, Z.; Omar, A.A.; Yang, Y.; Zhang, L. Experimental study on the flexural performance of concrete-encased square steel tube truss beams. J. Build. Eng. 2022, 49, 104053. [Google Scholar] [CrossRef]
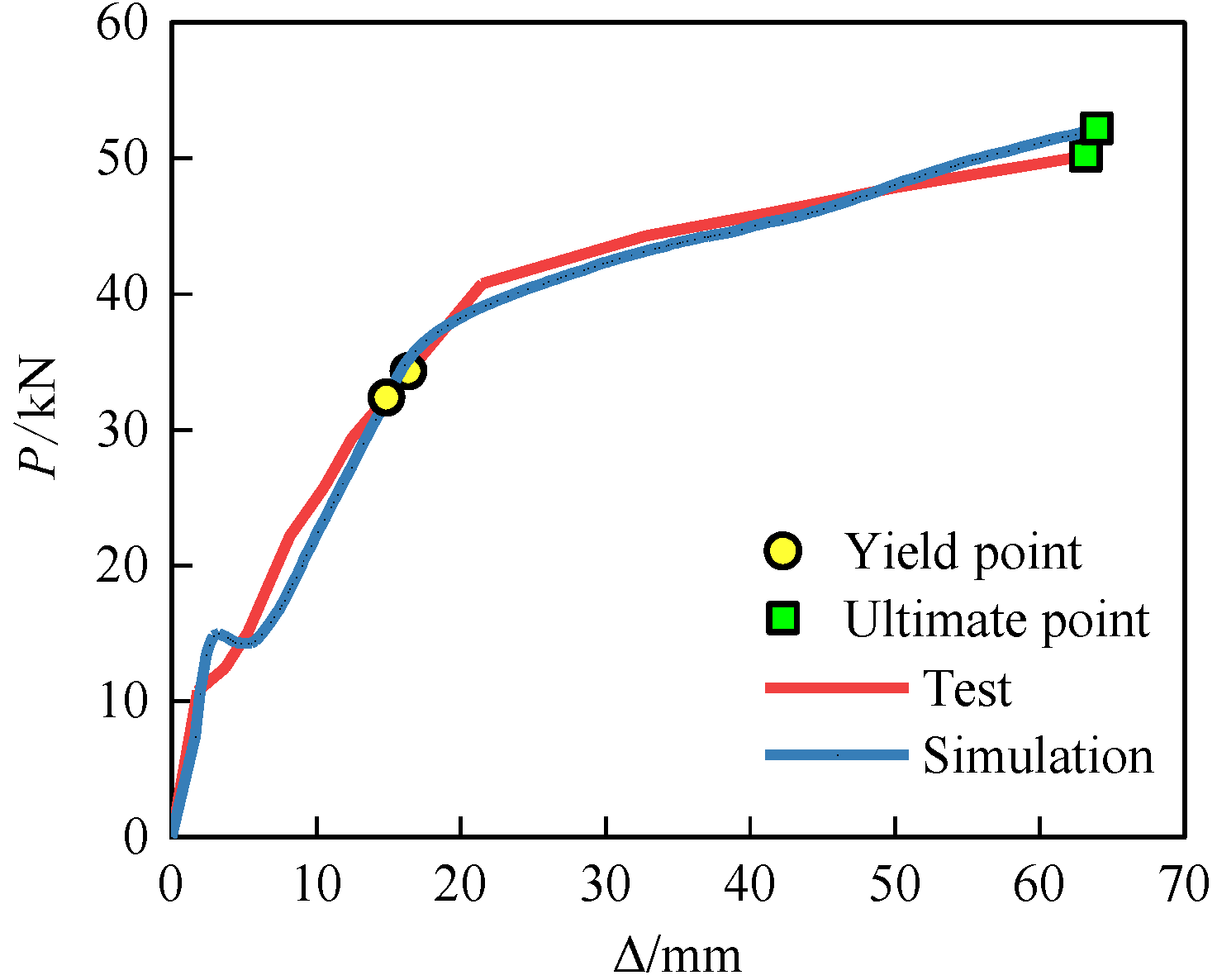

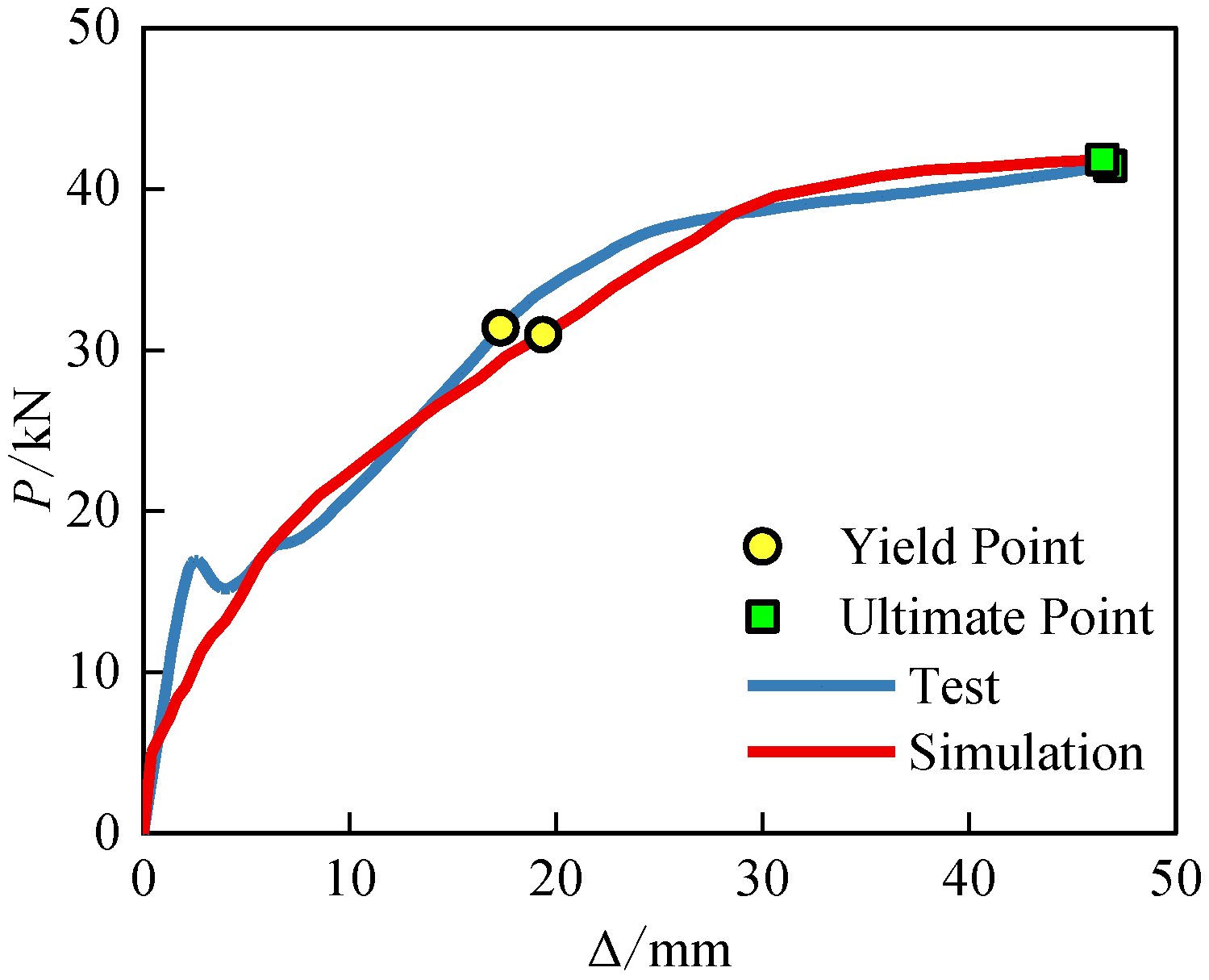

| No. | Pyt/kN | PyFE/kN | Pyt/PyFE | Pyt/kN | PuFE/kN | Put/PuFE |
|---|---|---|---|---|---|---|
| LS-1 | 34.28 | 37.42 | 0.92 | 50.16 | 52.17 | 0.96 |
| DB-2 | 30.95 | 31.36 | 0.99 | 41.84 | 41.44 | 1.01 |
| No. | Lower Chord of the Reinforced Truss | Upper Chord of the Reinforced Truss | Longitudinal Reinforcement in the Precast Base Plate | The Strength Grade of UHPC Base Plate/Mpa | The Thickness of the UHPC Base Plate/mm |
|---|---|---|---|---|---|
| S-1 |  8 8 |  8 8 | 8 8 8 | 120 | 60 |
| S-2 |  10 10 |  8 8 | 8 8 8 | 120 | 60 |
| S-3 |  12 12 |  8 8 | 8 8 8 | 120 | 60 |
| S-4 |  8 8 |  10 10 | 8 8 8 | 120 | 60 |
| S-5 |  8 8 |  12 12 | 8 8 8 | 120 | 60 |
| S-6 |  8 8 |  8 8 | 8 10 10 | 120 | 60 |
| S-7 |  8 8 |  8 8 | 8 12 12 | 120 | 60 |
| S-8 |  8 8 |  8 8 | 8 8 8 | 140 | 60 |
| S-9 |  8 8 |  8 8 | 8 8 8 | 100 | 60 |
| S-10 |  8 8 |  8 8 | 8 8 8 | 30 | 60 |
| S-11 |  8 8 |  8 8 | 8 8 8 | 120 | 40 |
| S-12 |  8 8 |  8 8 | 8 8 8 | 120 | 80 |
| Type | Cube Compressive Strength fcu (N/mm2) | Axial Compressive Strength fc (N/mm2) | Axial Tensile Strength ft (N/mm2) | Modulus of Elasticity Ec (×104 N/mm2) |
|---|---|---|---|---|
| NC | 30.0 | 14.3 | 1.4 | 3.0 |
| UHPC | 100.0 | 88.1 | 7.3 | 3.8 |
| 120 | 105.7 | 9.2 | 4.1 | |
| 140 | 123.3 | 11.0 | 4.3 |
| No. | Cracking Load/kN | Cracking Displacement/mm | Yield Load/kN | Yield Displacement/mm | Peak Load/kN | Peak Displacement/mm | Ultimate Load/kN | Ultimate Displacement/mm |
|---|---|---|---|---|---|---|---|---|
| S-1 | 48.93 | 3.89 | 64.62 | 16.25 | 74.54 | 62.76 | 71.65 | 111.21 |
| S-2 | 49.14 | 6.43 | 70.83 | 17.14 | 81.01 | 43.17 | 79.33 | 91.42 |
| S-3 | 49.82 | 3.94 | 77.31 | 17.95 | 88.53 | 48.32 | 85.93 | 78.19 |
| S-4 | 48.58 | 3.93 | 66.44 | 17.32 | 74.42 | 63.11 | 72.59 | 107.22 |
| S-5 | 48.62 | 3.94 | 66.07 | 17.13 | 74.75 | 51.42 | 72.89 | 104.37 |
| S-6 | 49.75 | 3.93 | 74.63 | 17.34 | 87.17 | 46.55 | 85.61 | 77.70 |
| S-7 | 50.53 | 3.37 | 86.46 | 18.51 | 100.50 | 44.71 | 98.66 | 60.69 |
| S-8 | 65.25 | 5.39 | 66.11 | 16.88 | 77.03 | 56.55 | 75.79 | 99.75 |
| S-9 | 45.60 | 3.41 | 58.02 | 15.39 | 68.87 | 51.83 | 65.85 | 124.75 |
| S-10 | 29.77 | 3.12 | 51.90 | 16.63 | 58.46 | 140.71 | 58.36 | 145.38 |
| S-11 | 44.65 | 3.10 | 59.21 | 15.42 | 69.06 | 46.74 | 64.88 | 145.47 |
| S-12 | 48.85 | 3.88 | 64.59 | 15.74 | 76.91 | 45.81 | 75.77 | 67.71 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wang, X.; Wang, R.; Zhu, Z.; Wang, K. Study on Flexural Capacity of UHPC-NC Composite Slab with Reinforced Truss in the Normal Section. Buildings 2024, 14, 3732. https://doi.org/10.3390/buildings14123732
Wang X, Wang R, Zhu Z, Wang K. Study on Flexural Capacity of UHPC-NC Composite Slab with Reinforced Truss in the Normal Section. Buildings. 2024; 14(12):3732. https://doi.org/10.3390/buildings14123732
Chicago/Turabian StyleWang, Xin, Ruochen Wang, Zhiyu Zhu, and Kun Wang. 2024. "Study on Flexural Capacity of UHPC-NC Composite Slab with Reinforced Truss in the Normal Section" Buildings 14, no. 12: 3732. https://doi.org/10.3390/buildings14123732
APA StyleWang, X., Wang, R., Zhu, Z., & Wang, K. (2024). Study on Flexural Capacity of UHPC-NC Composite Slab with Reinforced Truss in the Normal Section. Buildings, 14(12), 3732. https://doi.org/10.3390/buildings14123732






