Dynamics of Structures, Frames, and Plates with Viscoelastic Dampers or Layers: A Literature Review
Abstract
1. Introduction
2. General Characteristics of VE Materials and New VE Materials Used in Dampers and Layers
3. Models of VE Materials and Identification of Theirs Parameters
4. Influence of Temperature on VE Parameters and Behavior of VE Systems
5. Formulation of Equations of Motion and Their Solution for Beams and Frames with VE Elements
5.1. Linear Vibrations
5.2. Geometrically Non-Linear Vibrations
5.3. Structural Elements on Viscoelastic Foundations
6. Determination of Dynamic Characteristics of Systems with VE Elements
7. Methods of Numerical Integration of Motion Equations of Systems with VE Elements
8. Methods of Sensitivity and Uncertainty Analysis of Structures with Viscoelastic Elements
9. Optimization of Structures with VE Elements
10. Concluding Remarks
- (i)
- Significant progress has been gained in the field of designing of rubber-like materials with high damping properties; some new materials have the maximum damping ratio of up to 30% (see [36]);
- (ii)
- Advanced rheological models (both classical and fractional) are now adopted to characterize dynamic properties of VE elements and sophisticated identification procedures are developed to determine the parameters of those models;
- (iii)
- The identification procedures are quite complicated and need further improvements in the future; a nonlinear model of dampers which takes into account the influence of vibration amplitude in dampers is very much desired;
- (iv)
- Currently, the influence of ambient temperature on parameters of VE elements and overall dynamic behavior of systems with these elements is taken into account only for the case of rheologically simple materials, usually adopting the frequency–temperature superposition principle and the shifting techniques; studies concerning the influence of ambient temperature on parameters of rheologically complex materials are required;
- (v)
- Non-linear dynamic analysis of beams and plates with VE layers became a highly popular research subject due to its practical importance; in particular, very recently, the refined zig-zag theory was successfully applied to the analysis of free and steady-state vibrations; see also the very recent papers [392,393];
- (vi)
- Solution of an eigenvalue problem, in many cases a non-linear one, is required to determine dynamic characteristics of systems with VE elements; there are several methods available to solve this problem efficiently;
- (vii)
- Numerical integration methods for solving the equation of motion of systems with VE elements must take into account a complete history of the considered dynamic process, which significantly complicates the numerical procedure and for which further improvements aiming at the reduction in the total time of integration of equations of motion are very much desired;
- (viii)
- Recent achievements in sensitivity analysis and uncertainty quantification of design parameters involve the refinement of advanced methods to assess the influence of the parameter variability on the dynamic response of systems with VE elements; in the case of uncertain parameters, it is crucial to develop efficient methods because the recently proposed methods are computationally complex and involve multiple analyses to obtain reliable results;
- (ix)
- Heuristic methods are often used in optimization problems because of their reasonable physical relevance and simplicity, as dampers are added one by one with an even distribution or according to a certain pattern; however, their effectiveness is lower for asymmetrical structures or those featuring varying stiffness.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Spencer, B.F.; Nagarajaiah, S. State of the art of structural control. J. Struct. Eng. 2003, 129, 845–856. [Google Scholar] [CrossRef]
- Saaed, T.E.; Nikolakopoulos, G.; Jonasson, J.E.; Hedlund, H. A state-of-the-art review of structural control systems. J. Vib. Control 2015, 21, 919–937. [Google Scholar] [CrossRef]
- Housner, G.W.; Bergmann, L.A.; Caughey, T.K.; Chassiakos, A.G.; Claus, R.O.; Masri, S.F.; Skelton, R.E.; Soong, T.T.; Spencer, B.F.; Yao, J.T.P. Structural control: Past, present, and future. J. Eng. Mech. 1997, 123, 897–971. [Google Scholar] [CrossRef]
- Symans, M.D.; Charney, F.A.; Whittaker, A.S.; Constantinou, M.C. Energy dissipation systems for seismic applications: Current practice and recent developments. J. Struct. Eng. 2008, 134, 3–21. [Google Scholar] [CrossRef]
- Li, H.; Huo, L. Advances in structural control in civil engineering in China. Math. Probl. Eng. 2010, 2010, 936081. [Google Scholar] [CrossRef]
- El-Khoury, O.; Adeli, H. Recent advances on vibration control of structures under dynamic loading. Arch. Comput. Methods Eng. 2013, 20, 353–360. [Google Scholar] [CrossRef]
- Korkmaz, S. A review of active structural control: Challenges for engineering informatics. Comput. Struct. 2011, 89, 2113–2132. [Google Scholar] [CrossRef]
- Javanmardi, A.; Ibrahim, Z.; Ghaedi, K.; Ghadim, H.B.; Hanif, M.U. State-of-the-Art Review of Metallic Dampers: Testing, Development and Implementation. Arch. Comp. Meth. Eng. 2020, 27, 455–478. [Google Scholar] [CrossRef]
- Soong, T.T.; Spencer, B.F. Supplemental energy dissipation: State-of-the-art and state-of-the-practice. Eng. Struct. 2002, 24, 243–259. [Google Scholar] [CrossRef]
- Alhasan, A.A.; Vafaei, M.; Alih, S.C. Viscoelastic dampers for protection of structures against seismic actions. Innov. Infrastruct. Solut. 2022, 7, 309. [Google Scholar] [CrossRef]
- Jaisee, S.; Yue, F.; Ooi, Y.H. A state-of-the-art review on passive friction dampers and their applications. Eng. Struct. 2021, 235, 112022. [Google Scholar] [CrossRef]
- Ma, R.; Bi, K.; Hao, H. Inerter-based structural vibration control: A state-of-the-art review. Eng. Struct. 2021, 243, 112655. [Google Scholar] [CrossRef]
- Wagg, D.J. A review of the mechanical inerter: Historical context, physical realisations and nonlinear applications. Nonlinear Dyn. 2021, 104, 13–34. [Google Scholar] [CrossRef]
- Christopoulos, C.; Filiatrault, A. Principles of Passive Supplemental Damping and Seismic Isolation; IUSS Press: Pavia, Italy, 2006. [Google Scholar]
- Soong, T.T.; Dargush, G.F. Passive Energy Dissipation Systems in Structural Engineering; John Wiley: Chichester, UK; New York, NY, USA, 1997. [Google Scholar]
- De Domenico, D.; Ricciardi, G.; Takewaki, I. Design strategies of viscous dampers for seismic protection of building structures: A review. Soil Dyn. Earthq. Eng. 2019, 118, 144–165. [Google Scholar] [CrossRef]
- Xu, Z.D.; Wang, D.X.; Shi, C.F. Model, tests and application design for viscoelastic dampers. J. Vib. Control 2010, 17, 1359–1370. [Google Scholar] [CrossRef]
- Choo, J.F.; Koh, H.M.; Kang, S.C.; Kim, B.S. Vibration control of long-span high-speed railway bridges under periodic moving loading using viscoelastic dampers. In Structures for High-Speed Railway Transportation; International Association for Bridge and Structural Engineering: Antwerp, Belgium, 2003. [Google Scholar]
- Moliner, E.; Museros, P.; Martinez-Rodrigo, M.D. Retrofit of existing railway bridges of short to medium spans for high-speed traffic using viscoelastic dampers. Eng. Struct. 2012, 40, 519–528. [Google Scholar] [CrossRef]
- Cortés, F.; Martinez, M.; Elejabarrieta, M.J. Viscoelastic Surface Treatments for Passive Control of Structural Vibration; Nova Publishers: New York, NY, USA, 2012. [Google Scholar]
- Sandeep, S.H.; Srinivasa, C.V. Hybrid sandwich panels: A review. Int. J. Appl. Mech. Eng. 2020, 25, 64–85. [Google Scholar] [CrossRef]
- Lewandowski, R.; Słowik, M.; Przychodzki, M. Parameters identification of fractional models of viscoelastic dampers and fluids. Struc. Eng. Mech. 2017, 63, 181–193. [Google Scholar] [CrossRef]
- Eshaghi, M.; Sedaghati, R.; Rakheja, S. Dynamic characteristics and control of magnetorheological/electrorheological sandwich structures: A state-of-the-art review. J. Intell. Mater. Syst. Struct. 2016, 27, 2003–2037. [Google Scholar] [CrossRef]
- Osial, M.; Pregowska, A.; Warczak, M.; Giersig, M. Magnetorheological fluids: A concise review of composition, physicochemical properties, and models. J. Intell. Mater. Syst. Struct. 2023, 34, 1045389X231157357. [Google Scholar] [CrossRef]
- Jones, D.I.G. Handbook of Viscoelastic Vibration Damping; Wiley: New York, NY, USA, 2001. [Google Scholar]
- Shu, Z.; You, R.; Zhou, Y. Viscoelastic materials for structural dampers: A review. Constr. Build. Mater. 2022, 342, 127955. [Google Scholar] [CrossRef]
- Shu, Z.; Ning, B.; Li, S.; Li, Z.; Gan, Z.; Xie, Y. Experimental and numerical investigations of replaceable moment-resisting viscoelastic damper for steel frames. J. Constr. Steel Res. 2020, 170, 106100. [Google Scholar] [CrossRef]
- De Cazenove, J.; Rade, D.A.; de Lima, A.M.G.; Araujo, C.A. A numerical and experimental investigation on self-heating effects in viscoelastic dampers. Mech. Sys. Sig. Proc. 2012, 27, 433–445. [Google Scholar] [CrossRef]
- Chakraborty, B.C.; Ratna, D. Polymers for Vibration Damping Applications; Elsevier: Cambridge, MA, USA, 2020. [Google Scholar]
- Shedbale, N.; Muley, P.V. Review on Viscoelastic Materials used in Viscoelastic Dampers. Int. Res. J. Eng. Technol. 2017, 4, 7. [Google Scholar]
- Chen, Y.; Chen, C.; Ma, Q.; Jang, H.; Wan, Z. Study on mechanical properties of high damping viscoelastic dampers. Adv. Struct. Eng. 2019, 22, 2925–2936. [Google Scholar] [CrossRef]
- Wu, C.; Otani, Y.; Namiki, N.; Emi, H.; Nitta, K. Phase modification of acrylate rubber/chlorinated polypropylene blends by a hindered phenol compound. J. Polym. 2001, 33, 322–329. [Google Scholar] [CrossRef]
- Xu, Z.D.; Liao, Y.X.; Ge, T.; Xu, C. Experimental and theoretical study on viscoelastic dampers with different matrix rubbers. J. Eng. Mech. 2016, 142, 04016051. [Google Scholar] [CrossRef]
- Xu, Z.D.; Ge, T.; Liu, J. Experimental and theoretical study of high-energy dissipation-viscoelastic dampers based on acrylate-rubber matrix. J. Eng. Mech. 2020, 146, 04020057. [Google Scholar] [CrossRef]
- Xu, Z.D.; Yang, Y.; Zhu, Y.N.; Ge, T. Experimental study and mathematical modeling of viscoelastic dampers with wider temperature range based on blended rubber matrix. J. Build. Eng. 2023, 70, 106414. [Google Scholar] [CrossRef]
- Ge, T.; Xu, Z.D.; Yuan, F.G. Development of viscoelastic damper based on NBR and organic small-molecule composites. J. Mater. Civ. Eng. 2022, 34, 04022192. [Google Scholar] [CrossRef]
- Ge, T.; Xu, Z.D.; Yuan, F.G. Predictive model of dynamic mechanical properties of VE damper based on acrylic rubber–graphene oxide composites considering aging damage. J. Aero. Eng. 2022, 35, 04021132. [Google Scholar] [CrossRef]
- Xu, Y.S.; Xu, Z.D.; Guo, Y.Q.; Ge, T.; Xu, C.; Huang, X. Theoretical and experimental study of viscoelastic damper based on fractional derivative approach and micromolecular structures. J. Vib. Acoust. 2019, 141, 031010. [Google Scholar] [CrossRef]
- Teh, T.W.; Tan, C.G.; Jumaat, M.Z. Feasibility evaluation of novel high-damping rubbers as energy-dissipation material under axial dynamic load for damper devices. Buildings 2022, 12, 1917. [Google Scholar] [CrossRef]
- He, Z.; Shi, F.; Lin, Z.; Zhang, C.; Zhou, Y.; Zhao, F. Experimental characterization on cyclic stability behavior of a high-damping viscoelastic damper. Constr. Build. Mater. 2023, 371, 130749. [Google Scholar] [CrossRef]
- Nakamura, Y.; Hanzawa, T.; Nomura, T.; Takada, T. Performance-based placement of manufactured viscoelastic dampers for design response spectrum. Front. Built Environ. 2016, 2, 10. [Google Scholar] [CrossRef]
- Shitikova, M.V.; Krusser, A.I. Models of viscoelastic materials: A review on historical development and formulation. In Theoretical Analyses, Computations, and Experiments of Multiscale Materials; Giorgio, I., Placidi, L., Barchiesi, E., Abali, B.E., Altenbach, H., Eds.; Springer: Cham, Switzerland, 2022; Volume 175. [Google Scholar] [CrossRef]
- Genovese, A.; Carputo, F.; Maiorano, A.; Timpone, F.; Farroni, F.; Sakhnevych, A. Study on the generalized formulations with the aim to reproduce the viscoelastic dynamic behavior of polymers. Appl. Sci. 2020, 10, 2321. [Google Scholar] [CrossRef]
- Vasques, C.M.A.; Moreira, R.A.S.; Rodrigues, J.D. Viscoelastic damping Technologies—Part I: Modeling and finite element implementation. J. Adv. Res. Mech. Eng. 2010, 1, 76–95. [Google Scholar]
- Bland, D.R. The Theory of Linear Viscoelasticity; Pergamon Press: Oxford, UK, 1960. [Google Scholar]
- Tschoegl, N.W. Representation of Linear Viscoelastic Behavior by Series-Parallel Models. In The Phenomenological Theory of Linear Viscoelastic Behavior; Springer: Berlin/Heidelberg, Germany, 1989. [Google Scholar] [CrossRef]
- Steinmann, P.; Runesson, K. The Catalogue of Computational Material Models: Basic Geometrically Linear Models in 1D; Springer: Cham, Switzerland, 2021. [Google Scholar] [CrossRef]
- Lin, C.-Y. Rethinking and researching the physical meaning of the standard linear solid model in viscoelasticity. Mech. Adv. Mater. Struct. 2023, 1–16, in print. [Google Scholar] [CrossRef]
- Park, S.W. Analytical modeling of viscoelastic dampers for structural and vibration control. Int. J. Solids Struct. 2001, 38, 8065–8092. [Google Scholar] [CrossRef]
- Burgers, J.M. First and Second Report on Viscosity and Plasticity; Academy of Sciences at Amsterdam: Amsterdam, NY, USA, 1935. [Google Scholar]
- Zener, C. Elasticity and Anelasticity of Metals; University of Chicago Press: Chicago, IL, USA, 1948. [Google Scholar]
- Huang, C.; Gao, D.; Meng, T.; Yang, C. Investigation into Viscoelastic Properties of Fiber-Reinforced Asphalt Composite Concrete Based on the Burgers Model. Buildings 2023, 13, 449. [Google Scholar] [CrossRef]
- Renaud, F.; Dion, J.L.; Chevallier, G.; Tawfiq, I.; Lemaire, R. A new identification method of viscoelastic behavior: Application to the generalized Maxwell model. Mech. Syst. Signal Process. 2011, 25, 991–1010. [Google Scholar] [CrossRef]
- Lewandowski, R.; Bartkowiak, A.; Maciejewski, H. Dynamic analysis of frames with viscoelastic dampers: A comparison of damper models. Struct. Eng. Mech. 2012, 41, 113–137. [Google Scholar] [CrossRef]
- Ottosen, N.; Ristinmaa, M. The Mechanics of Constitutive Modeling; Elsevier: Amsterdam, NY, USA, 2005. [Google Scholar] [CrossRef]
- Bagley, R.L.; Torvik, P.J. Fractional calculus—A different approach to the analysis of viscoelastically damped materials. AIAA J. 1983, 21, 741–748. [Google Scholar] [CrossRef]
- Rossikhin, Y.A.; Shitikova, M.V. A new method for solving dynamic problems of fractional derivative viscoelasticity. Int. J. Eng. Sci. 2001, 39, 149–176. [Google Scholar] [CrossRef]
- Pritz, T. Five-parameter fractional derivative model for polymeric damping materials. J. Sound Vib. 2003, 265, 935–952. [Google Scholar] [CrossRef]
- Lewandowski, R.; Chorążyczewski, B. Identification of the parameters of the Kelvin–Voigt and the Maxwell fractional models, used to modeling of viscoelastic dampers. Comp. Struct. 2010, 88, 1–17. [Google Scholar] [CrossRef]
- Caputo, M. Linear models of dissipation whose is almost frequency independent-II. Geophys. J. R. Astron. Soc. 1967, 13, 529–539. [Google Scholar] [CrossRef]
- Lewandowski, R.; Przychodzki, M. Approximate method for temperature-dependent characteristics of structures with viscoelastic dampers. Arch. Appl. Mech. 2018, 88, 1695–1711. [Google Scholar] [CrossRef]
- Xu, Y.; He, Q.; Guo, Y.-Q.; Huang, X.-H.; Dong, Y.-R.; Hu, Z.-W.; Kim, J. Experimental and theoretical investigation of viscoelastic damper by applying fractional derivative method and internal variable theory. Buildings 2023, 13, 239. [Google Scholar] [CrossRef]
- Tang, S.; Greene, M.S.; Liu, W.K. Two-scale mechanism-based theory of nonlinear viscoelasticity. J. Mech. Phys. Solids 2012, 60, 199–226. [Google Scholar] [CrossRef]
- Vasile, O.; Bugaru, M. A New Modeling Approach for Viscous Dampers Using an Extended Kelvin–Voigt Rheological Model Based on the Identification of the Constitutive Law’s Parameters. Computation 2023, 11, 3. [Google Scholar] [CrossRef]
- Ghobadi, E.; Shutov, A.; Steeb, H. Parameter Identification and Validation of Shape-Memory Polymers within the Framework of Finite Strain Viscoelasticity. Materials 2021, 14, 2049. [Google Scholar] [CrossRef]
- Zhang, L.H.; Yao, X.H.; Zang, S.G.; Han, Q. Temperature and strain rate dependent tensile behavior of a transparent polyurethane interlayer. Mater. Des. 2015, 65, 1181–1188. [Google Scholar] [CrossRef]
- Martinez-Agirre, M.; Illescas, S.; Elejabarrieta, M.J. Characterisation and modelling of prestrained viscoelastic films. Int. J. Adhes. Adhes. 2014, 50, 183–190. [Google Scholar] [CrossRef]
- Chen, T. Determining a Prony Series for a Viscoelastic Material from Time Varying Strain Data; Technical Report; NASA: Washington, DC, USA, 2000. [Google Scholar]
- Rothermel, R.; Panfilenko, W.; Sharma, P.; Wald, A.; Schuster, T.; Jung, A.; Diebels, S. A method for determining the parameters in a rheological model for viscoelastic materials by minimizing Tikhonov functionals. Appl. Math. Sci. Eng. 2022, 30, 141–165. [Google Scholar] [CrossRef]
- Najib, M.F.; Nobari, A.S.; Nikbin, K. Modification and evaluation of a FRF-based model updating method for identification of viscoelastic constitutive models for a nonlinear polyurethane adhesive in a bonded joint. Int. J. Adhes. Adhes. 2017, 74, 181–193. [Google Scholar] [CrossRef]
- Pawlak, Z.M.; Denisiewicz, A. Identification of the fractional Zener model parameters for a viscoelastic material over a wide range of frequencies and temperatures. Materials 2021, 14, 7024. [Google Scholar] [CrossRef]
- Vasques, C.M.A.; Moreira, R.A.S.; Rodrigues, J. Viscoelastic damping technologies—Part II: Experimental identification procedure and validation. J. Adv. Res. Mech. Eng. 2010, 1, 96–110. [Google Scholar]
- Gerlach, S.; Matzenmiller, A. Comparison of numerical methods for identification of viscoelastic line spectra from static test data. Int. J. Numer. Meth. Eng. 2005, 63, 428–454. [Google Scholar] [CrossRef]
- Marino, E.; Flaschel, M.; Kumar, S.; de Lorenzis, L. Automated identification of linear viscoelastic constitutive laws with EUCLID. arXiv 2022, arXiv:2212.10969. [Google Scholar] [CrossRef]
- Najib, M.F.; Nobari, A.S. Nonlinear viscoelastic constitutive model identification for a polyurethane adhesive in a bonded joint using structural dynamic model updating. Mech. Mater. 2016, 100, 72–85. [Google Scholar] [CrossRef]
- Kim, S.-Y.; Lee, D. Identification of fractional-derivative-model parameters of viscoelastic materials from measured FRFs. J. Sound Vib. 2009, 324, 570–586. [Google Scholar] [CrossRef]
- Naraghi, T.; Nobari, A.S. Identification of the dynamic characteristics of a viscoelastic, nonlinear adhesive joint. J. Sound Vib. 2015, 352, 92–102. [Google Scholar] [CrossRef]
- Sun, W.; Wang, Z.; Liu, R.; Yan, X. Inverse Identification of the frequency-dependent mechanical parameters of a viscoelastic core layer based on the vibration response. Appl. Sci. 2017, 7, 455. [Google Scholar] [CrossRef]
- Sun, W.; Wang, Z.; Yan, X.; Zhu, M. Inverse identification of the frequency-dependent mechanical parameters of viscoelastic materials based on the measured FRFs. Mech. Syst. Signal Process. 2018, 98, 816–833. [Google Scholar] [CrossRef]
- Mehrez, L.; Kassem, E.; Masad, E.; Little, D. Stochastic Identification of Linear-Viscoelastic Models of Aged and Unaged Asphalt Mixtures. J. Mater. Civ. Eng. 2015, 27, 04014149. [Google Scholar] [CrossRef]
- Xiao, Z.; Haitian, Y.; Yiqian, H. Identification of constitutive parameters for fractional viscoelasticity. Commun. Nonlinear Sci. Numer. Simul. 2014, 19, 311–322. [Google Scholar] [CrossRef]
- Deng, R.; Davies, P.; Bajaj, A.K. Flexible polyurethane foam modelling and identification of viscoelastic parameters for automotive seating applications. J. Vib. Acoust. 2003, 262, 391–417. [Google Scholar] [CrossRef]
- Sessa, S.; Vaiana, N.; Paradiso, M.; Rosati, L. An inverse identification strategy for the mechanical parameters of a phenomenological hysteretic constitutive model. Mech. Syst. Signal Process. 2020, 139, 106622. [Google Scholar] [CrossRef]
- Grosso, P.; de Felice, A.; Sorrentino, S. A method for the experimental identification of equivalent viscoelastic models from vibration of thin plates. Mech. Syst. Signal Process. 2021, 153, 107527. [Google Scholar] [CrossRef]
- Lewandowski, R.; Chorążyczewski, B.; Wasilewicz, P. Evaluation of parameters of viscous fluid and viscoelastic dampers. Vib. Phys. Syst. 2006, 22, 223–228. [Google Scholar]
- Vaiana, N.; Sessa, S.; Marmo, F.; Rosati, L. An accurate and computationally efficient uniaxial phenomenological model for steel and fiber reinforced elastomeric bearings. Compos. Struct. 2019, 211, 196–212. [Google Scholar] [CrossRef]
- Galadima, Y.K.; Oterkus, S.; Oterkus, E.; Amin, I.; El-Aassar, A.-H.; Shawky, H. A nonlocal method to compute effective properties of viscoelastic composite materials based on peridynamic computational homogenization theory. Compos. Struct. 2023, 319, 117147. [Google Scholar] [CrossRef]
- Makris, N.; Roussos, Y.; Whittaker, A.S.; Kelly, J.M. Viscous heating of fluid dampers, II: Large-amplitude motions. J. Eng. Mech. 1998, 124, 1217–1223. [Google Scholar] [CrossRef]
- Kasai, K.; Sato, D. A constitutive rule for viscoelastic material considering heat conduction and heat transfer. In Proceedings of the Second International Conference on Urban Earthquake Engineering, Tokyo, Japan, 7–8 March 2005; pp. 459–466. [Google Scholar]
- Guo, J.W.; Daniel, Y.; Montgomery, M.; Christopoulos, C. Thermal-mechanical model for predicting the wind and seismic response of viscoelastic dampers. J. Eng. Mech. 2016, 142, 04016067. [Google Scholar] [CrossRef]
- Black, C.J.; Makris, N. Viscous heating of fluid dampers under small and large amplitude motions: Experimental studies and parametric modeling. J. Eng. Mech. 2007, 133, 566–577. [Google Scholar] [CrossRef]
- De Lima, A.M.G.; Rade, D.A.; Bouhaddi, N. Stochastic modeling of surface viscoelastic treatments combined with model condensation procedures. Shock Vib. 2010, 17, 429–444. [Google Scholar] [CrossRef]
- De Lima, A.M.G.; Rade, D.A.; Lepore-Neto, F.P. An efficient modeling methodology of structural systems containing viscoelastic dampers based on frequency response function substructuring. Mech. Syst. Sig. Process. 2009, 23, 1272–1281. [Google Scholar] [CrossRef]
- Brinson, H.F.; Brinson, L.C. Polymer Engineering Science and Viscoelasticity: An Introduction; Springer: New York, NY, USA, 2008. [Google Scholar]
- Shaw, M.T. Introduction to Polymer Rheology; Wiley: Hoboken, NJ, USA, 2012. [Google Scholar]
- De Lima, A.M.G.; Stoppa, M.H.; Rade, D.A.; Steffen, V., Jr. Sensitivity analysis of viscoelastic structures. Shock Vib. 2006, 13, 545–558. [Google Scholar] [CrossRef]
- Moreira, R.A.S.; Corte-Real, J.D.; Dias Rodrigues, J. A generalized frequency-temperature viscoelastic Model. Shock Vib. 2010, 17, 407–418. [Google Scholar] [CrossRef]
- Cardone, D.; Gesualdi, G.; Nigro, D. Effects of air temperature on the cyclic behavior of elastomeric seismic isolators. Bull. Earthq. Eng. 2011, 9, 1227–1255. [Google Scholar] [CrossRef]
- Park, H.-I.; Min, C.-H. Temperature effect on vibration characteristics of viscoelastic laminated beam. Int. J. Offshore Polar Eng. 2010, 20, 118–124. [Google Scholar]
- Tsai, C.S. Temperature effect of viscoelastic dampers during earthquakes. J. Struct. Eng. 1994, 120, 394–409. [Google Scholar] [CrossRef]
- Chang, K.C.; Soong, T.T.; Oh, S.-T.; Lai, M.L. Seismic behavior of steel frame with added viscoelastic dampers. J. Struct. Eng. 1995, 121, 1418–1426. [Google Scholar] [CrossRef]
- De Lima, A.M.G.; Rade, D.A.; Lacerda, H.B.; Araújo, C.A. An investigation of the self-heating phenomenon in viscoelastic materials subjected to cyclic loadings accounting for prestress. Mech. Syst. Sig. Process 2015, 58–59, 115–127. [Google Scholar] [CrossRef]
- Cunha-Filho, A.G.; de Lima, A.M.G.; Donadon, M.V.; Leao, L.S. Flutter suppression of plates using passive constrained viscoelastic layers. Mech. Syst. Sig. Process. 2016, 79, 99–111. [Google Scholar] [CrossRef]
- Diani, J.; Gilormini, P.; Frédy, C.; Rousseau, I. Predicting thermal shape memory of crosslinked polymer networks from linear viscoelasticity. Int. J. Solids Struct. 2012, 49, 793–799. [Google Scholar] [CrossRef]
- Arikoglu, A. A new fractional derivative model for linearly viscoelastic materials and parameter identification via genetic algorithms. Rheol. Acta 2014, 53, 219–233. [Google Scholar] [CrossRef]
- Xu, Z.-D.; Xu, C.; Hu, J. Equivalent fractional Kelvin model and experimental study on viscoelastic damper. J. Vib. Control 2015, 21, 2536–2552. [Google Scholar] [CrossRef]
- Xu, Y.; Xu, Z.-D.; Guo, Y.-Q.; Huang, X.; Dong, Y.; Li, Q. Dynamic properties and energy dissipation study of sandwich viscoelastic damper considering temperature influence. Buildings 2021, 11, 470. [Google Scholar] [CrossRef]
- Lakes, R.S. Viscoelastic Materials; Cambridge University Press: London, UK, 2009. [Google Scholar]
- Xu, Y.; Xu, Z.-D.; Guo, Y.-Q.; Huang, X.; Zhang, Z.; Sun, B.; Kim, J. Thermodynamic behaviors of a viscoelastic plate for vibration control with nonlocal effect and temperature-dependent properties when subjected to a moving heat source. J. Eng. Mech. 2022, 148, 04022022. [Google Scholar] [CrossRef]
- Xu, Y.; Xu, Z.-D.; Guo, Y.-Q.; Sarwar, W.; She, W.; Geng, Z.-F. Study on viscoelastic materials at micro scale pondering supramolecular interaction impacts with DMA tests and fractional derivative modelling. J. Appl. Polym. Sci. 2023, 140, e53660. [Google Scholar] [CrossRef]
- Drake, M.L.; Soovere, J. A Design Guide for Damping of Aerospace Structures. In Proceedings of the Vibration Damping Workshop, Long Beach, CA, USA, 27–29 February 1984; Air Force Wright Aeronautical Laboratories, Wright-Patterson Air Force Base: Greene County, OH, USA, 1984. AFWAL-TR-84-3064. pp. VV-1–VV-10. [Google Scholar]
- Sheoran, S.S.; Kalkal, K.K.; Deswal, S. Fractional order thermo-viscoelastic problem with temperature dependent modulus of elasticity. Mech. Adv. Mater. Struct. 2016, 23, 407–414. [Google Scholar] [CrossRef]
- Wei, K.; Yang, Q.; Dou, Y.; Wang, F.; Wang, P. Experimental investigation into temperature- and frequency-dependent dynamic properties of high-speed rail pads. Constr. Build. Mater. 2017, 151, 848–858. [Google Scholar] [CrossRef]
- Pettermann, H.E.; de Simone, A. An anisotropic linear thermo-viscoelastic constitutive law. Elastic relaxation and thermal expansion creep in the time domain. Mech. Time-Depend. Mater. 2018, 22, 421–433. [Google Scholar] [CrossRef]
- Ghaemmaghami, A.R.; Kwon, O.-S. Nonlinear modeling of MDOF structures equipped with viscoelastic dampers with strain, temperature and frequency-dependent properties. Eng. Struct. 2018, 168, 903–914. [Google Scholar] [CrossRef]
- Nakamura, N. Improved methods to transfer frequency-dependent complex stiffness to time domain. Earthq. Eng. Struct. Dyn. 2006, 35, 1037–1050. [Google Scholar] [CrossRef]
- Li, Z.L.; Qin, Y.; Sun, B.; Jia, C.L.; Zhang, W.J.; Yan, B.J.; Shi, Q.L. A fractional approach to the time-temperature dependence of dynamic viscoelastic behaviour. J. Mech. Sci. Technol. 2019, 33, 139–147. [Google Scholar] [CrossRef]
- Litewka, P.; Lewandowski, R. Dynamic characteristics of viscoelastic Mindlin plates with influence of temperature. Comput. Struct. 2020, 229, 106181. [Google Scholar] [CrossRef]
- Litewka, P.; Lewandowski, R. Temperature influence on non-linear harmonic vibrations of plates made of viscoelastic materials. Eng. Trans. 2020, 68, 159–176. [Google Scholar]
- Dai, J.; Xu, Z.-D.; Gai, P.-P.; Yan, X. Seismic performance of viscoelastically damped structures at different ambient temperatures. J. Vib. Control 2021, 27, 2819–2834. [Google Scholar] [CrossRef]
- Dai, J.; Gai, P.-P.; Xu, Z.-D.; Chen, Z.Q. A reduced-order improved rational polynomial method for viscoelastically damped structures considering ambient temperature effect. Soil Dyn. Earthq. Eng. 2022, 159, 107315. [Google Scholar] [CrossRef]
- Lewandowski, R. Influence of temperature on the dynamic characteristics of structures with viscoelastic dampers. J. Struct. Eng. 2019, 145, 04018245. [Google Scholar] [CrossRef]
- Zhang, H.; Li, S.; Zhang, Z.; Luo, H.; Wang, Y. A five-parameter fractional derivative temperature spectrum model for polymeric damping materials. Polym. Test. 2020, 89, 106654. [Google Scholar] [CrossRef]
- Cupiał, P.; Nizioł, J. Vibration and damping analysis of three-layered composite plate with a viscoelastic mid-layer. J. Sound Vib. 1995, 183, 99–114. [Google Scholar] [CrossRef]
- Sainsbury, M.G.; Zhang, Q.J. The Galerkin element method, applied to the vibration of damped sandwich beams. Comput. Struct. 1999, 71, 239–256. [Google Scholar] [CrossRef]
- Duigou, L.; Daya, E.M.; Potier-Ferry, M. Iterative algorithms for non-linear eigenvalue problems. Application to vibrations of viscoelastic shells. Comput. Methods Appl. Mech. Eng. 2003, 192, 1323–1335. [Google Scholar] [CrossRef]
- Moreira, R.A.S.; Rodrigues, J.D. A layerwise model for thin soft core sandwich plates. Comput. Struct. 2006, 84, 1256–1263. [Google Scholar] [CrossRef]
- Galucio, A.C.; Deü, J.-F.; Ohayon, R. Finite element formulation of viscoelastic sandwich beams using fractional derivative operators. Comput. Mech. 2004, 33, 282–291. [Google Scholar] [CrossRef]
- Banerjee, J.R.; Sobey, A.J. Dynamic stiffness formulation and free vibration analysis of a three-layered sandwich beam. Int. J. Solids Struct. 2005, 42, 2181–2197. [Google Scholar] [CrossRef]
- Cortés, F.; Elejabarrieta, M.J. Homogenised finite element for transient dynamic analysis of unconstrained layer damping beams involving fractional derivative models. Comput. Mech. 2007, 40, 313–324. [Google Scholar] [CrossRef]
- Backstrom, D.; Nilsson, A.C. Modeling the vibration of sandwich beams using frequency-dependent parameters. J. Sound Vib. 2007, 300, 589–611. [Google Scholar] [CrossRef]
- Barbosa, F.S.; Farage, M.C.R. A finite element model for sandwich viscoelastic beams: Experimental and numerical assessment. J. Sound Vib. 2008, 317, 91–111. [Google Scholar] [CrossRef]
- Hu, H.; Belouettar, S.; Potier-Ferry, M.; Daya, E.M. Review and assessment of various theories for modeling sandwich composites. Compos. Struct. 2008, 84, 282–292. [Google Scholar] [CrossRef]
- Carrera, E. Historical review of Zig-Zag theories for multilayered plates and shells. Appl. Mech. Rev. ASME 2003, 56, 287–308. [Google Scholar] [CrossRef]
- Kiasat, S.; Filippi, M.; Nobari, A.S.; Carrera, E. Layer-wise dynamic analysis of a beam with global and local viscoelastic contributions using an FE/Laplace transform approach. Acta Mech. 2022, 233, 4747–4761. [Google Scholar] [CrossRef]
- D’Ottavio, M.; Krasnobrizha, A.; Valot, E.; Polit, O.; Vescovini, R.; Dozio, L. Dynamic response of viscoelastic multiple-core sandwich structures. J. Sound Vib. 2021, 491, 115753. [Google Scholar] [CrossRef]
- Plagianakos, T.S.; Saravanos, D.A. High-order layerwise finite element for the damped free-vibration response of thick composite and sandwich composite plates. Int. J. Numer. Methods Eng. 2009, 77, 1593–1626. [Google Scholar] [CrossRef]
- Alvelid, M. Sixth order differential equation for sandwich beam deflection including transverse shear. Compos. Struct. 2013, 102, 29–37. [Google Scholar] [CrossRef]
- Won, S.G.; Bae, S.H.; Cho, J.R.; Bae, S.R.; Jeong, W.B. Three-layered damped element for forced vibration analysis of symmetric sandwich structures with a viscoelastic core. Finite Elem. Anal. Des. 2013, 68, 39–51. [Google Scholar] [CrossRef]
- Wang, Y.; Inman, D.J. Finite element analysis and experimental study on dynamic properties of a composite beam with viscoelastic damping. J. Sound Vib. 2013, 332, 6177–6191. [Google Scholar] [CrossRef]
- Akoussan, K.; Boudaoud, H.; Daya, E.M.; Carrera, E. Vibration Modeling of Multilayer Composite Structures with Viscoelastic Layers. Mech. Adv. Mater. Struct. 2015, 22, 136–149. [Google Scholar] [CrossRef]
- Zamani, H.A.; Aghdam, M.M.; Sadighi, M. Free vibration analysis of thick viscoelastic composite plates on visco-Pasternak foundation using higher-order theory. Compos. Struct. 2017, 182, 25–35. [Google Scholar] [CrossRef]
- Naji, J.; Zabihollah, A.; Behzad, M. Vibration characteristics of laminated composite beams with magnetorheological layer using layerwise theory. Mech. Adv. Mater. Struct. 2018, 25, 202–211. [Google Scholar] [CrossRef]
- Zarraga, O.; Sarría, I.; García-Barruetabeña, J.; Cortés, F. Dynamic analysis of plates with thick unconstrained layer damping. Eng. Struct. 2019, 201, 109809. [Google Scholar] [CrossRef]
- Zarraga, O.; Sarría, I.; García-Barruetabeña, J.; Elejabarrieta, M.J.; Cortés, F. General homogenised formulation for thick viscoelastic layered structures for finite element applications. Mathematics 2020, 8, 714. [Google Scholar] [CrossRef]
- Alavi, S.H.; Eipakchi, H. An analytical approach for free vibrations analysis of viscoelastic circular and annular plates using FSDT. Mech. Adv. Mater. Struct. 2020, 27, 250–264. [Google Scholar] [CrossRef]
- Huang, Z.; Wang, X.; Wu, N.; Chu, F.; Luo, D. The finite element modeling and experimental study of sandwich plates with frequency-dependent viscoelastic material model. Materials 2020, 13, 2296. [Google Scholar] [CrossRef]
- Lewandowski, R.; Litewka, P.; Wielentejczyk, P. Free vibrations of laminate plates with viscoelastic layers using the refined zig-zag theory. Part 1 Theoretical background. Compos. Struct. 2021, 278, 114547. [Google Scholar] [CrossRef]
- Lewandowski, R.; Litewka, P.; Wielentejczyk, P. Dynamic characteristics of multi-layered viscoelastic beams using the refined zig-zag theory. Composite Struct. 2021, 259, 113212. [Google Scholar] [CrossRef]
- Tessler, A.; di Sciuva, M.; Gherlone, M. A refined zigzag beam theory for composite and sandwich beams. J. Compos. Mater. 2009, 43, 1051–1081. [Google Scholar] [CrossRef]
- Tessler, A.; di Sciuva, M.; Gherlone, M. A consistent refinement of first-order shear-deformation theory for laminated composite and sandwich plates using improved zigzag kinematics. J. Mech. Mater. Struct. 2010, 5, 341–367. [Google Scholar] [CrossRef]
- Ren, S.; Zhao, G.; Zhang, S. A layerwise finite element formulation for vibration and damping analysis of sandwich plate with moderately thick viscoelastic core. Mech. Adv. Mater. Struct. 2020, 27, 1201–1212. [Google Scholar] [CrossRef]
- Rouzegar, J.; Vazirzadeh, M.; Heydari, M.H. A fractional viscoelastic model for vibrational analysis of thin plate excited by supports movement. Mech. Res. Commun. 2020, 110, 103618. [Google Scholar] [CrossRef]
- Dang, R.; Cui, Y.; Qu, J.; Yang, A.; Chen, Y. Variable fractional modeling and vibration analysis of variable-thickness viscoelastic circular plate. Appl. Math. Modell. 2022, 110, 767–778. [Google Scholar] [CrossRef]
- Zenkour, A.M.; El-Shahrany, H.D. Vibration of viscoelastic magnetostrictive plates embedded in viscoelastic foundations in hygrothermal environments. Acta Mech. Sin. 2023, 39, 522305. [Google Scholar] [CrossRef]
- Huang, W.; Ren, J.; Forooghi, A. Vibrational frequencies of FG-GPLRC viscoelastic rectangular plate subjected to different temperature loadings based on higher-order shear deformation theory and utilizing GDQ procedure. Mech. Based Des. Struct. Mach. 2023, 51, 1775–1800. [Google Scholar] [CrossRef]
- Xia, Z.Q.; Lukasiewicz, S. Nonlinear damped vibrations of simply-supported rectangular sandwich plates. Nonlinear Dyn. 1995, 8, 417–433. [Google Scholar] [CrossRef]
- Kim, T.-W.; Kim, J.-H. Nonlinear vibration of viscoelastic laminated composite plates. Int. J. Solids Struct. 2002, 39, 2857–2870. [Google Scholar] [CrossRef]
- Daya, E.M.; Azrar, L.; Potier-Ferry, M. An amplitude equation for the non-linear vibration of viscoelastically damped sandwich beams. J. Sound Vib. 2004, 271, 789–813. [Google Scholar] [CrossRef]
- Mahmoodi, S.N.; Khadem, S.E.; Kokabi, M. Non-linear free vibrations of Kelvin-Voigt visco-elastic beams. Int. J. Mech. Sci. 2007, 49, 722–732. [Google Scholar] [CrossRef]
- Jacques, N.; Daya, E.M.; Potier-Ferry, M. Nonlinear vibration of viscoelastic sandwich beams by the harmonic balance and finite element methods. J. Sound Vib. 2010, 329, 4251–4265. [Google Scholar] [CrossRef]
- Bilasse, M.; Daya, E.M.; Azrar, L. Linear and nonlinear vibrations analysis of viscoelastic sandwich beams. J. Sound Vib. 2010, 329, 4950–4969. [Google Scholar] [CrossRef]
- Bilasse, M.; Azrar, L.; Daya, E.M. Complex modes based numerical analysis of viscoelastic sandwich plates vibrations. Comput. Struct. 2011, 89, 539–555. [Google Scholar] [CrossRef]
- Li, J.-J.; Cheng, C.-J. Differential quadrature method for analyzing nonlinear dynamic characteristics of viscoelastic plates with shear effects. Nonlinear Dyn. 2010, 61, 57–70. [Google Scholar] [CrossRef]
- Ghayesh, M.H. Nonlinear forced dynamics of an axially moving viscoelastic beam with an internal resonance. Int. J. Mech. Sci. 2011, 53, 1022–1037. [Google Scholar] [CrossRef]
- Ghayesh, M.H. Nonlinear dynamic response of a simply-supported Kelvin-Voigt viscoelastic beam, additionally supported by a nonlinear spring. Nonlinear Anal. Real World Appl. 2012, 13, 1319–1333. [Google Scholar] [CrossRef]
- Sarangi, S.K.; Ray, M.C. Active damping of geometrically nonlinear vibrations of laminated composite plates using vertically reinforced 1–3 piezoelectric composites. Acta Mech. 2011, 222, 363–380. [Google Scholar] [CrossRef]
- Kumar, R.S.; Ray, M.C. Active constrained layer damping of geometrically nonlinear vibrations of smart laminated composite sandwich plates using 1–3 piezoelectric composites. Int. J. Mech. Mater. Des. 2012, 8, 359–380. [Google Scholar] [CrossRef]
- Datta, P.; Ray, M.C. Three-dimensional fractional derivative model of smart constrained layer damping treatment for composite plates. Compos. Struct. 2016, 156, 291–306. [Google Scholar] [CrossRef]
- Kanasogi, R.M.; Ray, M.C. Control of geometrically nonlinear vibrations of skew laminated composite plates using skew or rectangular 1–3 piezoelectric patches. Int. J. Mech. Mater. Des. 2013, 9, 325–354. [Google Scholar] [CrossRef]
- Shivakumar, J.; Ashok, M.H.; Ray, M.C. Active control of geometrically nonlinear transient vibrations of laminated composite cylindrical panels using piezoelectric fiber reinforced composite. Acta Mech. 2013, 224, 1–15. [Google Scholar] [CrossRef]
- Kumar, A.M.S.; Panda, S.; Chakraborty, D. Piezo-viscoelastically damped nonlinear frequency response of functionally graded plates with a heated plate-surface. J. Vib. Control 2016, 22, 320–343. [Google Scholar]
- Kumar, R.S.; Ray, M.C. Smart damping of geometrically nonlinear vibrations of functionally graded sandwich plates using 1–3 piezoelectric composites. Mech. Adv. Mater. Struct. 2016, 23, 652–669. [Google Scholar] [CrossRef]
- Youzera, H.; Meftah, S.A.; Challamel, N.; Tounsi, A. Nonlinear damping and forced vibration analysis of laminated composite beams. Compos. Part B 2012, 43, 1147–1154. [Google Scholar] [CrossRef]
- Leung, A.Y.T.; Yang, H.X.; Zhu, P.; Guo, Z.J. Steady state response of fractionally damped nonlinear viscoelastic arches by residue harmonic homotopy. Comput. Struct. 2013, 121, 10–21. [Google Scholar] [CrossRef]
- Mahmoudkhani, S.; Haddadpour, H.; Navazi, H.M. The effects of nonlinearities on the vibration of viscoelastic sandwich plates. Int. J. Non-Linear Mech. 2014, 62, 41–57. [Google Scholar] [CrossRef]
- Lewandowski, R.; Baum, M. Dynamic characteristics of multilayered beams with viscoelastic layers described by the fractional Zener model. Arch. Appl. Mech. 2015, 85, 1793–1814. [Google Scholar] [CrossRef]
- Amabili, M. Nonlinear vibrations of viscoelastic rectangular plates. J. Sound Vib. 2016, 362, 142–156. [Google Scholar] [CrossRef]
- Litewka, P.; Lewandowski, R. Nonlinear harmonically excited vibrations of plates with Zener material. Nonlinear Dyn. 2017, 89, 691–712. [Google Scholar] [CrossRef]
- Litewka, P.; Lewandowski, R. Steady-state non-linear vibrations of plates using Zener material with fractional derivative. Comput. Mech. 2017, 60, 333–354. [Google Scholar] [CrossRef]
- Mokhtari, M.; Permoon, M.R.; Haddadpour, H. Aeroelastic analysis of sandwich cylinder with fractional viscoelastic core described by Zener model. J. Fluids Struct. 2019, 85, 1–16. [Google Scholar] [CrossRef]
- Shafei, E.; Faroughi, S.; Rabczuk, T. Nonlinear transient vibration of viscoelastic plates: A NURBS-based isogeometric HSDT approach. Comput. Math. Appl. 2021, 84, 1–15. [Google Scholar] [CrossRef]
- Younesian, D.; Hosseinkhani, A.; Askari, H.; ·Esmailzadeh, E. Elastic and viscoelastic foundations: A review on linear and nonlinear vibration modeling and applications. Nonlinear Dyn. 2019, 97, 853–895. [Google Scholar] [CrossRef]
- Sarparast, H.; Ebrahimi-Mamaghani, A.; Safarpour, M.; Ouakad, H.M.; Dimitri, R.; Tornabene, F. Nonlocal study of the vibration and stability response of small-scale axially moving supported beams on viscoelastic-Pasternak foundation in a hygro-thermal environment. Math. Meth. Appl. Sci. 2020, 1–12, early view. [Google Scholar] [CrossRef]
- Amir, S.; Arshid, E.; Maraghi, Z.K. Free vibration analysis of magneto-rheological smart annular three-layered plates subjected to magnetic field in viscoelastic medium. Smart Struct. Syst. 2020, 25, 581–592. [Google Scholar] [CrossRef]
- Keshtegar, B.; Motezaker, M.; Kolahchi, R.; Trung, N.-T. Wave propagation and vibration responses in porous smart nanocomposite sandwich beam resting on Kerr foundation considering structural damping. Thin Wall. Struct. 2020, 154, 106820. [Google Scholar] [CrossRef]
- Patil, M.A.; Kadoli, R. Influence of Winkler and viscoelastic foundation on free vibration of functionally graded beam integrated with Terfenol-D layer. J. Braz. Soc. Mech. Sci. 2020, 42, 591. [Google Scholar] [CrossRef]
- Ghabussi, A.; Ashrafi, N.; Shavalipour, A.; Hosseinpour, A.; Habibi, M.; Moayedi, H.; Babaei, B.; Safarpour, H. Free vibration analysis of an electro-elastic GPLRC cylindrical shell surrounded by viscoelastic foundation using modified length-couple stress parameter. Mech. Based Des. Struct. Mach. 2021, 49, 738–762. [Google Scholar] [CrossRef]
- Zhang, D.; Liu, M.; Wang, Z.; Lei, Y. Thermo-electro-mechanical vibration of piezoelectric nanobeams resting on a viscoelastic foundation. J. Phys. Conf. Ser. 2021, 1759, 012029. [Google Scholar] [CrossRef]
- Kargar, J.; Arani, A.G.; Arshid, E.; Rahaghi, M.I. Vibration analysis of spherical sandwich panels with MR fluids core and magneto-electro-elastic face sheets resting on orthotropic viscoelastic foundation. Struct. Eng. Mech. 2021, 78, 557–572. [Google Scholar] [CrossRef]
- Li, H.; Gao, Z.; Zhao, J.; Ma, H.; Han, Q.; Liu, J. Vibration suppression effect of porous graphene platelet coating on fiber reinforced polymer composite plate with viscoelastic damping boundary conditions resting on viscoelastic foundation. Eng. Struct. 2021, 237, 112167. [Google Scholar] [CrossRef]
- Souad, H.; Ismail, M.; Hichem, A.; Noureddine, E. Vibration analysis of viscoelastic FGM nanoscale plate resting on viscoelastic medium using higher-order theory. Period. Polytech. Civ. Eng. 2021, 65, 255–275. [Google Scholar] [CrossRef]
- Praharaj, R.K.; Datta, N. Dynamic response of plates resting on a fractional viscoelastic foundation and subjected to a moving load. Mech. Based Des. Struct. Mach. 2022, 50, 2317–2332. [Google Scholar] [CrossRef]
- Bensaid, I.; Saimi, A. Dynamic investigation of functionally graded porous beams resting on viscoelastic foundation using generalised differential quadrature method. Aust. J. Mech. Eng. 2023, 21, 1440–1459. [Google Scholar] [CrossRef]
- Li, M.-L.; Wei, P.-J.; Zhou, X.-L. Wave propagation and free vibration of a Timoshenko beam mounted on the viscoelastic Pasternak foundation modelled by fraction-order derivatives. Mech. Time-Depend. Mat. 2022, 1–15. [Google Scholar] [CrossRef]
- Ghali, D.; Ismail, M.; Hichem, A.; Noureddine, E.; Belaid, M. Investigating the free vibration of viscoelastic FGM Timoshenko nanobeams resting on viscoelastic foundations with the shear correction factor using finite element method. Mech. Based Des. Struct. Mach. 2022, 1–26, in print. [Google Scholar]
- Van Tuyen, B. Free Vibration Behaviors of Nanoplates Resting on Viscoelastic Medium. Arab. J. Sci. Eng. 2023, 48, 11511–11524. [Google Scholar] [CrossRef]
- Al Mukahal, F.H.H.; Sobhy, M. Wave propagation and free vibration of FG graphene platelets sandwich curved beam with auxetic core resting on viscoelastic foundation via DQM. Arch. Civ. Mech. Eng. 2022, 22, 12. [Google Scholar] [CrossRef]
- Mei, M.; Alamri, S.; Turki Jalil, A.; Hadrawi, S.K.; Khan, I.; Baghaei, S. Wave propagation and vibration analysis of sandwich structure with a bio-based flexible core and composite face sheets subjected to visco-Pasternak foundation and magnetic field. Compos. Struct. 2022, 300, 116132. [Google Scholar] [CrossRef]
- Foroutan, K.; Carrera, E.; Ahmadi, H. Nonlinear hygrothermal vibration and buckling analysis of imperfect FG-CNTRC cylindrical panels embedded in viscoelastic foundations. Eur. J. Mech. A-Solid 2021, 85, 104107. [Google Scholar] [CrossRef]
- Zhang, P.; Schiavone, P.; Qing, H. Unified two-phase nonlocal formulation for vibration of functionally graded beams resting on nonlocal viscoelastic Winkler-Pasternak foundation. Appl. Math. Mech. 2023, 44, 89–108. [Google Scholar] [CrossRef]
- Song, P.; Wei, P.; Zhou, X. Vibration of rectangular plate on fractional order viscoelastic foundation subjected to standing and moving loads. Mech. Time-Depend. Mat. 2023, 1–21, in print. [Google Scholar] [CrossRef]
- Song, P.; Wei, P.; Zhou, X.; Bi, X. Free vibration of fully clamped rectangular plate on fractional viscoelastic Pasternak foundations. Chin. Q. Mech. 2022, 43, 681–690. [Google Scholar] [CrossRef]
- Zamani, H.A. Frequency analysis of FG-CNT–reinforced composite doubly curved panels on visco-Pasternak medium. Adv. Compos. Hybrid Mater. 2021, 4, 830–844. [Google Scholar] [CrossRef]
- Zamani, H.A.; Salehi, M. Free vibration of foam plates on viscoelastic foundations considering thickness stretching. Mech. Time-Depend. Mat. 2023, 1–18, in print. [Google Scholar] [CrossRef]
- Yu, Y.; Fu, Y.; Zhang, W. Analysis of transverse natural frequency of two-span continuous Timoshenko beam on viscoelastic Pasternak foundation. J. Vib. Shock 2023, 42, 1–10. [Google Scholar] [CrossRef]
- Wu, D.; Lei, Y.; Wang, Z.; Yu, B.; Zhang, D. Free vibration analysis of carbon-nanotube reinforced beams resting on a viscoelastic Pasternak foundation by the nonlocal Eshelby–Mori–Tanaka method. Mech. Compos. Mater. 2023, 59, 479–494. [Google Scholar] [CrossRef]
- Pham, Q.H.; Tran, V.K.; Tran, T.T.; Nguyen, V.C.; Zenkour, A.M. Nonlocal higher-order finite element modeling for vibration analysis of viscoelastic orthotropic nanoplates resting on variable viscoelastic foundation. Compos. Struct. 2023, 318, 117067. [Google Scholar] [CrossRef]
- Ghandourah, E.E.; Daikh, A.A.; Khatir, S.; Alhawsawi, A.M.; Banoqitah, E.M.; Eltaher, M.A. A dynamic analysis of porous coated functionally graded nanoshells rested on viscoelastic medium. Mathematics 2023, 11, 2407. [Google Scholar] [CrossRef]
- Alazwari, M.A.; Zenkour, A.M. A quasi-3d refined theory for the vibration of functionally graded plates resting on visco-Winkler-Pasternak foundations. Mathematics 2022, 10, 716. [Google Scholar] [CrossRef]
- Zenkour, A.M.; El-Shahrany, H.D. Hygrothermal vibration control of cross-ply magneto-viscoelastic beams resting on Kerr’s foundations. J. Eng. Math. 2023, 140, 2. [Google Scholar] [CrossRef]
- Zaitoun, M.W.; Chikh, A.; Tounsi, A.; Sharif, S.; Al-Osta, M.A.; Al-Dulaijan, S.U.; Al-Zahrani, M.M. An efficient computational model for vibration behaviour of a functionally graded sandwich plate in a hygrothermal environment with viscoelastic foundation effects. Eng. Comput. 2023, 39, 1127–1141. [Google Scholar] [CrossRef]
- Alimoradzadeh, M.; Tornabene, F.; Esfarjani, S.M.; Dimitri, R. Finite strain-based theory for the superharmonic and subharmonic resonance of beams resting on a nonlinear viscoelastic foundation in thermal conditions, and subjected to a moving mass loading. Int. J. Non-Linear Mech. 2023, 148, 104271. [Google Scholar] [CrossRef]
- Praharaj, R.K.; Datta, N. On the transient response of plates on fractionally damped viscoelastic foundation. Comput. Appl. Math. 2020, 39, 256. [Google Scholar] [CrossRef]
- Kiarasi, F.; Asadi, A.; Babaei, M.; Asemi, K.; Hosseini, M. Dynamic analysis of functionally graded carbon nanotube (FGCNT) reinforced composite beam resting on viscoelastic foundation subjected to impulsive loading. J. Comput. Appl. Mech. 2022, 53, 1–22. [Google Scholar] [CrossRef]
- Xiao, C.; Zhang, G.; Hu, P.; Yu, Y.; Mo, Y.; Mohammadi, R. Vibration suppression of the viscoelastic sandwich doubly-curved shells using magnetostrictive layers subjected to Kerr’s foundation. Int. J. Struct. Stab. Dyn. 2022, 22, 2250058. [Google Scholar] [CrossRef]
- Ebrahimi, F.; Hosseini, S.H.S. Investigation of flexoelectric effect on nonlinear forced vibration of piezoelectric/functionally graded porous nanocomposite resting on viscoelastic foundation. J. Strain Anal. 2020, 55, 53–68. [Google Scholar] [CrossRef]
- Salawu, S.A.; Sobamowo, G.M.; Sadiq, O.M. Forced vibration analysis of isotropic thin circular plate resting on nonlinear viscoelastic foundation. Iran. J. Sci. Technol. Trans. Civ. Eng. 2020, 44 (Suppl. S1), 277–288. [Google Scholar] [CrossRef]
- Aris, H.; Ahmadi, H. Combination resonance analysis of imperfect functionally graded conical shell resting on nonlinear viscoelastic foundation in thermal environment under multi-excitation. J. Vib. Control 2022, 28, 2121–2144. [Google Scholar] [CrossRef]
- Moayeri, M.; Darabi, B.; Sianaki, A.H.; Adamian, A. Third order nonlinear vibration of viscoelastic circular microplate based on softening and hardening nonlinear viscoelastic foundation under thermal loading. Eur. J. Mech. A-Solid 2022, 95, 104644. [Google Scholar] [CrossRef]
- Jahangiri, R.; Rezaee, M.; Manafi, H. Nonlinear and chaotic vibrations of FG double curved sandwich shallow shells resting on visco-elastic nonlinear Hetenyi foundation under combined resonances. Compos. Struct. 2022, 295, 115721. [Google Scholar] [CrossRef]
- Foroutan, K.; Dai, L. Nonlinear dynamic responses of porous FG sandwich cylindrical shells with a viscoelastic core resting on a nonlinear viscoelastic foundation. Mech. Adv. Mater. Struct. 2023, 30, 3184–3203. [Google Scholar] [CrossRef]
- Foroutan, K.; Ahmadi, H. Nonlinear vibration of SSMFG cylindrical shells with internal resonances resting on the nonlinear viscoelastic foundation. Struct. Eng. Mech. 2022, 84, 767–782. [Google Scholar] [CrossRef]
- Alimoradzadeh, M.; Akbas, S.D. Nonlinear vibration analysis of carbon nanotube-reinforced composite beams resting on nonlinear viscoelastic foundation. Geomech. Eng. 2023, 32, 125–135. [Google Scholar] [CrossRef]
- Alimoradzadeh, M.; Akbas, S.D. Nonlinear thermal vibration of FGM beams resting on nonlinear viscoelastic foundation. Steel Compos. Struct. 2022, 44, 557–567. [Google Scholar] [CrossRef]
- Khani Arani, H.; Shariyat, M. Nonlinear 2D-DQ volume-preservative global–local dynamic analysis of composite sandwich plates with soft hyperelastic cores and viscoelastic Winkler-Pasternak foundations. Structures 2023, 55, 727–746. [Google Scholar] [CrossRef]
- Shitikova, M.V.; Kandu, V.V.; Krusser, A.I. On nonlinear vibrations of an elastic plate on a fractional viscoelastic foundation in a viscoelastic medium in the presence of the one-to-one internal resonance. J. Sound Vib. 2023, 549, 117564. [Google Scholar] [CrossRef]
- Javidi, R.; Rezaei, B.; Zand, M.M. Nonlinear dynamics of a beam subjected to a moving mass and resting on a viscoelastic foundation using optimal homotopy analysis method. Int. J. Struct. Stab. 2023, 23, 2350084. [Google Scholar] [CrossRef]
- Zhao, Y.; Hou, X.; Zhang, S.; Sun, T.; Du, L.; Deng, Z. Nonlinear forced vibration of thermo-electro-elastic piezoelectric graphene composite nanoplate based on viscoelastic foundation. Acta Mech. Sin. 2023, 39, 522228. [Google Scholar] [CrossRef]
- Liu, G.; Wu, S.; Shahsavari, D.; Karami, B.; Tounsi, A. Dynamics of imperfect inhomogeneous nanoplate with exponentially-varying properties resting on viscoelastic foundation. Eur. J. Mech. A-Solid 2022, 95, 104649. [Google Scholar] [CrossRef]
- Hai, T.; Al-Masoudy, M.M.; Alsulamy, S.; Hechmi El Ouni, M.; Ayvazyan, A.; Kumar, A. Moving load analysis on cross/angle-ply laminated composite nanoplates resting on viscoelastic foundation. Compos. Struct. 2023, 305, 116540. [Google Scholar] [CrossRef]
- Bi, X.; Wei, P.; Zhou, X.; Song, P. Dynamic response of Timoshenko beams fixed at both ends on a fractional-order viscoelastic Pasternak foundation. Chin. Q. Mech. 2023, 44, 122–132. [Google Scholar] [CrossRef]
- Adhikari, S.; Pascual, B. Eigenvalues of linear viscoelastic systems. J. Sound Vib. 2009, 325, 1000–1011. [Google Scholar] [CrossRef]
- Chang, T.; Singh, M.P. Seismic analysis of structures with a fractional derivative model of viscoelastic dampers. Earthq. Eng. Eng. Vib. 2002, 1, 251–260. [Google Scholar] [CrossRef]
- Ruhe, A. Algorithms for the nonlinear eigenvalue problem. SIAM J. Numer. Anal. 1973, 10, 674–689. [Google Scholar] [CrossRef]
- Voss, H. Nonlinear eigenvalue problems. In Handbook of Linear Algebra 164; Chapman and Hall/CRC: Boca Raton, FL, USA, 2003. [Google Scholar]
- Daya, E.M.; Potier-Ferry, M. A numerical method for nonlinear eigenvalue problems application to vibrations of viscoelastic structures. Comput. Struct. 2001, 79, 533–541. [Google Scholar] [CrossRef]
- Muravyov, A.; Hutton, S.G. Closed-form solutions and the eigenvalue problem for vibration of discrete viscoelastic systems. J. Appl. Mech. 1997, 64, 684–691. [Google Scholar] [CrossRef]
- Menon, S.; Tang, J. A state-space approach for the dynamic analysis of viscoelastic systems. Comput. Struct. 2004, 82, 1123–1130. [Google Scholar] [CrossRef]
- Hryniewicz, Z. Dynamic analysis of systems with deterministic and stochastic viscoelastic dampers. J. Sound Vib. 2004, 278, 1013–1023. [Google Scholar] [CrossRef]
- Gürgöze, M.; Doğruoğlu, A.N.; Zeren, S. On the eigencharacteristics of a cantilevered visco-elastic beam carrying a tip mass and its representation by a spring-damper-mass system. J. Sound Vib. 2007, 301, 420–426. [Google Scholar] [CrossRef]
- Wang, P.; Wang, Q.; Xu, X.; Chen, N. Fractional critical damping theory and its application in active suspension control. Shock Vib. 2017, 2017, 2738976. [Google Scholar] [CrossRef]
- Chen, Y.; Xu, J.; Tai, Y.; Xu, X.; Chen, N. Critical damping design method of vibration isolation system with both fractional-order inerter and damper. Mech. Adv. Mater. Struct. 2022, 29, 1348–1359. [Google Scholar] [CrossRef]
- Adhikari, S.; Pascual, B. Iterative methods for eigenvalues of viscoelastic systems. J. Vib. Acoust. 2011, 133, 021002-1–021002-7. [Google Scholar] [CrossRef]
- Lázaro, M.; Pérez-Aparicio, J.L.; Epstein, M. Computation of eigenvalues in proportionally damped viscoelastic structures based on the fixed-point iteration. Appl. Math. Comput. 2012, 219, 3511–3529. [Google Scholar] [CrossRef][Green Version]
- Lázaro, M. Eigensolutions of nonviscously damped systems based on the fixed-point iteration. J. Sound Vib. 2018, 418, 100–121. [Google Scholar] [CrossRef]
- Cortés, F.; Elajabarrieta, M.J. An approximate numerical method for the complex eigenproblem in systems characterized by a structural damping matrix. J. Sound Vib. 2006, 296, 166–182. [Google Scholar] [CrossRef]
- Cortés, F.; Elejabarrieta, M.J. Computational methods for complex eigenproblems in finite element analysis of structural systems with viscoelastic damping treatments. Comput. Methods Appl. Mech. Eng. 2006, 195, 6448–6462. [Google Scholar] [CrossRef]
- Singh, K.V. Eigenvalue and eigenvector computation for discrete and continuous structures composed of viscoelastic materials. Int. J. Mech. Sci. 2016, 110, 127–137. [Google Scholar] [CrossRef]
- Lin, R.M.; Ng, T.Y. An iterative method for exact eigenvalues and eigenvectors of general nonviscously damped structural systems. Eng. Struct. 2019, 180, 630–641. [Google Scholar] [CrossRef]
- Lázaro, M. Closed-form eigensolutions of nonviscously, nonproportionally damped systems based on continuous damping sensitivity. J. Sound Vib. 2018, 413, 368–382. [Google Scholar] [CrossRef]
- Bailey, P.B.; Chen, P.J. Natural modes of vibration of linear viscoelastic circular plates with free edges. Int. J. Solids Struct. 1987, 23, 785–795. [Google Scholar] [CrossRef]
- Segalman, D.J. Calculation of damping matrices for linearly viscoelastic structures. J. Appl. Mech. 1987, 54, 585–588. [Google Scholar] [CrossRef]
- Lewandowski, R.; Wielentejczyk, P. Free vibration of frame structures made of Zener type viscoelastic material. MATEC WEB of Conferences. In Proceedings of the 16th Symposium of Structural Dynamics (DYNKON 2019), Kombornia, Poland, 22–24 May 2019; Volume 285, p. 00009. [Google Scholar] [CrossRef]
- Lewandowski, R.; Wielentejczyk, P. Analysis of dynamic characteristics of viscoelastic frame structures. Arch. Appl. Mech. 2020, 90, 147–171. [Google Scholar] [CrossRef]
- Rossikhin, Y.A.; Shitikova, M.V. Analysis of free non-linear vibrations of a viscoelastic plate under the conditions of different internal resonances. In. J. Non-Linear Mech. 2006, 41, 313–325. [Google Scholar] [CrossRef]
- Tubaldi, E. Dynamic behavior of adjacent buildings connected by linear viscous/viscoelastic dampers. Struct. Control Health Monit. 2015, 22, 1086–1102. [Google Scholar] [CrossRef]
- Lewandowski, R. Approximate method for determination of dynamic characteristics of structures with viscoelastic dampers. Vib. Phys. Syst. 2016, 27, 219–226. [Google Scholar]
- Pawlak, Z.; Lewandowski, R. The continuation method for the eigenvalue problem of structures with viscoelastic dampers. Comput. Struct. 2013, 125, 53–61. [Google Scholar] [CrossRef]
- Litewka, P.; Lewandowski, R.; Wielentejczyk, P. Free vibrations of laminate plates with viscoelastic layers using the refined zig-zag theory—Part 2. Numerical analysis. Compos. Struct. 2021, 278, 114550. [Google Scholar] [CrossRef]
- Lewandowski, R.; Kawa, O.; Studziński, R. Dynamic characteristics of reticulated domes built of composite elastic/viscoelastic rods modeled by the fractional Zener model. Buildings 2022, 12, 1999. [Google Scholar] [CrossRef]
- Fischer, P. Eigensolution of nonclassically damped structures by complex subspace iteration. Comput. Methods Appl. Mech. Eng. 2000, 189, 149–166. [Google Scholar] [CrossRef]
- Łasecka-Plura, M.; Lewandowski, R. The subspace iteration method for nonlinear eigenvalue problems occurring in the dynamics of structures with viscoelastic elements. Comput. Struct. 2021, 254, 106571. [Google Scholar] [CrossRef]
- Chen, X.; Chen, H.L.; Hu, X.L. Damping predication of sandwich structures by order-reduction-iteration approach. J. Sound Vib. 1999, 222, 803–812. [Google Scholar] [CrossRef]
- Mokhtari, M.; Permoon, M.R.; Haddadpour, H. Dynamic analysis of isotropic sandwich cylindrical shell with fractional viscoelastic core using Rayleigh-Ritz method. Compos. Struct. 2018, 186, 165–174. [Google Scholar] [CrossRef]
- Lázaro, M.; Pérez-Aparicio, J.L. Dynamic analysis of frame structures with free viscoelastic layers: New closed-form solutions of eigenvalues and a viscous approach. Eng. Struct. 2013, 54, 69–81. [Google Scholar] [CrossRef]
- Akoussan, K.; Boudaoud, H.; Daya, E.M.; Koutsawa, Y.; Carrera, E. Numerical method for nonlinear complex eigenvalues problems depending on two parameters: Application to three-layered viscoelastic composite structures. Mech. Adv. Mater. Struct. 2018, 25, 1361–1373. [Google Scholar] [CrossRef]
- Friswell, M.I.; Adhikari, S.; Lei, Y. Non-local finite element analysis of damped beams. Int. J. Solids Struct. 2007, 44, 7564–7576. [Google Scholar] [CrossRef]
- Friswell, M.I.; Adhikari, S.; Lei, Y. Vibration analysis of beams with non-local foundations using the finite element method. Int. J. Numer. Methods Eng. 2007, 71, 1365–1386. [Google Scholar] [CrossRef]
- Lei, Y.; Murmu, T.; Adhikari, S.; Friswell, M.I. Dynamic characteristics of damped viscoelastic nonlocal Euler-Bernoulli beams. Eur. J. Mech. A Solids 2013, 42, 125–136. [Google Scholar] [CrossRef]
- Ansari, R.; Oskouie, M.F.; Sadeghi, F.; Bazdid-Vahdati, M. Free vibration of fractional viscoelastic Timoshenko nanobeams using the nonlocal elasticity theory. Phys. E Low-Dimens. Syst. Nanostructures 2015, 74, 318–327. [Google Scholar] [CrossRef]
- Elyasi, P.; Neya, B.N.; Firoozjaee, A.R. Free vibration of viscoelastic nonlocally damped tapered axially functionally graded beams using the state-space formulation. Eng. Struct. 2023, 288, 116183. [Google Scholar] [CrossRef]
- Naghinejad, M.; Ovesy, H.R. Viscoelastic free vibration behavior of nano-scaled beams via finite element nonlocal integral elasticity approach. J. Vib. Control 2019, 25, 445–459. [Google Scholar] [CrossRef]
- Cajić, M.; Karličiś, D.; Lazarević, M. Nonlocal vibration of a fractional order viscoelastic nanobeam with attached nanoparticle. Theor. Appl. Mech. 2015, 42, 167–190. [Google Scholar] [CrossRef]
- Martin, O. Nonlocal effects on the dynamic analysis of a viscoelastic nanobeam using a fractional Zener model. Appl. Math. Model. 2019, 73, 637–650. [Google Scholar] [CrossRef]
- Rajabi, K.; Hosseini-Hashemi, S. Application of the generalized Hooke’s law for viscoelastic materials (GHVMs) in nonlocal free damped vibration analysis of viscoelastic orthotropic nanoplates. Int. J. Mech. Sci. 2017, 124–125, 158–165. [Google Scholar] [CrossRef]
- Rajabi, K.; Hosseini-Hashemi, S. Application of the generalized Hooke’s law for viscoelastic materials (GHVMs) in nanoscale mass sensing applications of viscoelastic nanoplates: A theoretical study. Eur. J. Mech. A Solids. 2018, 67, 71–83. [Google Scholar] [CrossRef]
- Johnson, C.D.; Kienholz, D.A. Finite element prediction of damping in structures with constrained viscoelastic layers. AIAA J. 1982, 20, 1284–1290. [Google Scholar] [CrossRef]
- Shen, K.L.; Soong, T.T. Seismic behaviour of reinforced concrete frame with added viscoelastic dampers. Eng. Struct. 1995, 17, 372–380. [Google Scholar] [CrossRef]
- Tsai, M.H.; Chang, K.C. A study of the modal strain energy method for viscoelastically damped structures. J. Chin. Inst. Eng. 2001, 24, 311–320. [Google Scholar] [CrossRef]
- Lee, D.G.; Hong, S.; Kim, J. Efficient seismic analysis of building structures with added viscoelastic dampers. Eng. Struct. 2002, 24, 1217–1227. [Google Scholar] [CrossRef]
- Fan, Z. Transient vibration and sound radiation of a rectangular plate with viscoelastic boundary supports. Int. J. Numer. Methods Eng. 2001, 51, 619–630. [Google Scholar] [CrossRef]
- Rao, M.D.; Echempati, R.; Nadella, S. Dynamic analysis and damping of composite structures embedded with viscoelastic layers. Compos. B 1997, 28, 547–554. [Google Scholar] [CrossRef]
- Saravanan, C.; Ganesan, N.; Ramamurti, V. Study on energy dissipation pattern in vibrating fluid filled cylindrical shells with a constrained viscoelastic layer. Comput. Struct. 2000, 75, 575–591. [Google Scholar] [CrossRef]
- Saravanan, C.; Ganesan, N.; Ramamurti, V. Vibration and damping analysis of multilayered fluid filled cylindrical shells with constrained viscoelastic damping using modal strain energy method. Comput. Struct. 2000, 75, 395–417. [Google Scholar] [CrossRef]
- Sun, B.; Wang, J.; Li, Z.I.; Qin, Y.; Liu, S.Z.; Tomker, D. Modified modal strain energy method for analyzing the dynamic damping behavior of constrained viscoelastic structures. J. Eng. Sci. Technol. 2017, 10, 174–180. [Google Scholar] [CrossRef]
- Aprile, A.; Benedetti, A. Implicit dynamic analysis of VE-damped structures using Maxwell parallel systems. Eng. Comp. 1999, 16, 374–396. [Google Scholar] [CrossRef]
- Cortés, F.; Mateos, M.; Elejabarrieta, M.J. A direct integration formulation for exponentially damped structural systems. Comp. Struct. 2009, 87, 391–394. [Google Scholar] [CrossRef]
- Puthanpurayil, A.M.; Carr, A.J.; Dhakal, R.P. A generic time domain implementation scheme for non-classical convolution damping models. Eng. Struct. 2014, 71, 88–98. [Google Scholar] [CrossRef]
- Adhikari, S.; Wagner, N. Direct time-domain integration method for exponentially damped linear systems. Comp. Struct. 2004, 82, 2453–2461. [Google Scholar] [CrossRef]
- Palmeri, A.; Muscolino, G.A. numerical method for the time-domain dynamic analysis of buildings equipped with viscoelastic dampers. Struct. Control Health Monit. 2011, 18, 519–539. [Google Scholar] [CrossRef]
- Palmeri, A.; Ricciardelli, F.; de Luca, A.; Muscolino, G. State space formulation for linear viscoelastic dynamic systems with memory. J. Eng. Mech. 2003, 129, 715–724. [Google Scholar] [CrossRef]
- Liu, Q. Computational method of the dynamic response for nonviscously damped structure systems. J. Eng. Mech. 2014, 140, 04014085. [Google Scholar] [CrossRef]
- Sorvari, J.; Hämäläinen, J. Time integration in linear viscoelasticity—A comparative study. Mech. Time-Depend Mater. 2010, 14, 307–328. [Google Scholar] [CrossRef]
- Liu, Q. Explicit computational method of dynamic response for non-viscously damped structure systems. Mech. Res. Commun. 2016, 71, 48–55. [Google Scholar] [CrossRef]
- Patlashenko, I.; Givoli, D.; Barbone, P. Time-stepping schemes for systems of Volterra integro-differential equations. Comp. Meth. Appl. Mech. Eng. 2001, 190, 5691–5718. [Google Scholar] [CrossRef]
- Ding, Z.; Li, L.; Kong, J.; Qin, L. A modal projection-based reduction method for transient dynamic responses of viscoelastic systems with multiple damping models. Comp. Struct. 2018, 194, 60–73. [Google Scholar] [CrossRef]
- Ding, Z.; Li, L.; Hu, Y. A modified precise integration method for transient dynamic analysis in structural systems with multiple damping models. Mech. Syst. Signal Process. 2018, 98, 613–633. [Google Scholar] [CrossRef]
- Zhang, J.; Zheng, G.T. The Biot Model and its application in viscoelastic Composite structures. J. Vib. Acoust. 2007, 129, 533–540. [Google Scholar] [CrossRef]
- Padovan, J. Computational algorithms fir FE formulations involving fractional operators. Comp. Mech. 1987, 2, 271–287. [Google Scholar] [CrossRef]
- Enelund, M.; Olsson, P. Damping described by fading memory—Analysis and application to fractional derivative models. Int. J. Solids Struct. 1999, 36, 939–970. [Google Scholar] [CrossRef]
- Enelund, M.; Lesieutre, G.A. Time domain modeling of damping using anelastic displacement fields and fractional calculus. Int. J. Solids Struct. 1999, 36, 4447–4472. [Google Scholar] [CrossRef]
- Schmidt, A.; Gaul, L. Finite element formulation of viscoelastic constitutive equations using fractional time derivatives. Nonlinear Dyn. 2002, 29, 37–55. [Google Scholar] [CrossRef]
- Schmidt, A.; Lotsch, M.; Gaul, L. Implementation of a new method for the computation of fractionally damped structures into the finite element method. Shock Vib. 2010, 17, 419–428. [Google Scholar] [CrossRef][Green Version]
- Cortés, F.; Elejabarrieta, M.J. Finite element formulations for transient dynamic analysis in structural systems with viscoelastic treatments containing fractional derivative models. Int. J. Numer. Meth. Eng. 2007, 69, 2173–2195. [Google Scholar] [CrossRef]
- Datta, P.; Ray, M.C. Fractional order derivative model of viscoelastic layer for active damping of geometrically nonlinear vibrations of smart composite plates. Comp. Mater. Cont. 2015, 49–50, 47–80. [Google Scholar] [CrossRef]
- Bucher, C.; Pirrotta, A. Dynamic finite element analysis of fractionally damped structural systems in the time domain. Acta Mech. 2015, 226, 3977–3990. [Google Scholar] [CrossRef]
- Singh, M.P.; Chang, T.S.; Nandan, H. Algorithms for seismic analysis of MDOF systems with fractional derivatives. Eng. Struct. 2011, 33, 2371–2381. [Google Scholar] [CrossRef]
- Cortés, F.; Brun, M.; Elejabarrieta, M.J. A finite element formulation for the transient response of free layer damping plates including fractional derivatives. Comp. Struct. 2023, 282, 107039. [Google Scholar] [CrossRef]
- Schmidt, A.; Gaul, L. On the numerical evaluation of fractional derivatives in multi-degree-of-freedom systems. Signal Proc. 2006, 86, 2592–2601. [Google Scholar] [CrossRef]
- Adolfsson, K.; Enelund, M.; Larsson, S. Adaptive discretization of fractional order viscoelasticity using sparse time history. Comp. Meth. Appl. Mech. Eng. 2004, 193, 4567–4590. [Google Scholar] [CrossRef]
- Liu, T.; Wen, W.; Wang, P.; Feng, F. A time-marching procedure based on a sub-step explicit time integration scheme for non-viscous damping systems. Eng. Comp. 2023, 1–21, in print. [Google Scholar] [CrossRef]
- Shen, R.; Qian, X.; Zhou, J. Direct integration for non-viscous structural systems and its simplification. Mech. Res. Comm. 2019, 95, 8–15. [Google Scholar] [CrossRef]
- Xu, J.; Li, J. Stochastic dynamic response and reliability assessment of controlled structures with fractional derivative model of viscoelastic dampers. Mech. Syst. Signal Process. 2016, 72–73, 865–896. [Google Scholar] [CrossRef]
- Shen, R.; Qian, X.; Zhou, J.; Lee, C. A time integration method based on the weak form Galerkin method for non-viscous damping systems. Mech. Syst. Signal Process. 2021, 151, 107361. [Google Scholar] [CrossRef]
- Adhikari, S. Derivative of eigensolutions of nonviscously damped linear systems. AIAA J. 2002, 40, 2061–2069. [Google Scholar] [CrossRef]
- Adhikari, S.; Friswell, M.I. Calculation of eigensolution derivatives for nonviscously damped systems using Nelson’s method. AIAA J. 2006, 44, 1799–1806. [Google Scholar] [CrossRef]
- Li, L.; Hu, Y.J.; Wang, X.L.; Ling, L. Computation of eigensolution derivatives for nonviscously damped systems using the algebraic method. AIAA J. 2012, 50, 2282–2284. [Google Scholar] [CrossRef]
- Li, L.; Hu, Y.; Wang, X.; Ling, L. Eigensensitivity analysis for asymmetric nonviscous systems. AIAA J. 2013, 51, 738–741. [Google Scholar] [CrossRef]
- Li, L.; Hu, Y.; Wang, X. Design sensitivity and Hessian matrix of generalized eigenproblems. Mech. Syst. Signal Process. 2014, 43, 272–294. [Google Scholar] [CrossRef]
- Wang, P.; Dai, H. Eigensensitivity analysis for symmetric nonviscously damped systems with repeated eigenvalues. J. Vibroengineering 2014, 16, 4065–4076. [Google Scholar]
- Li, L.; Hu, Y.; Wang, X. A study on design sensitivity analysis for general nonlinear eigenproblems. Mech. Syst. Signal Process. 2013, 34, 88–105. [Google Scholar] [CrossRef]
- Kobelev, V. Sensitivity analysis of the linear nonconservative systems with fractional damping. Struct. Multidiscipl. Optim. 2007, 33, 179–188. [Google Scholar] [CrossRef]
- Lewandowski, R.; Łasecka-Plura, M. Design sensitivity analysis of structures with viscoelastic dampers. Comput. Struct. 2016, 164, 95–107. [Google Scholar] [CrossRef]
- Łasecka-Plura, M.; Lewandowski, R. Sensitivity analysis of dynamic characteristics of composite beams with viscoelastic layers. Procedia Eng. 2017, 199, 366–371. [Google Scholar] [CrossRef]
- Akoussan, K.; Boudaoud, H.; Daya, E.M.; Koutsawa, Y.; Carrera, E. Sensitivity analysis of the damping properties of viscoelastic composite structures according to the layers thicknesses. Compos. Struct. 2016, 149, 11–25. [Google Scholar] [CrossRef]
- Lampoh, K.; Charpentier, I.; Mostafa, D.E. Eigenmode sensitivity of damped sandwich structures. Comptes Rendus Mécanique 2014, 342, 700–705. [Google Scholar] [CrossRef]
- Cha, P.D.; Carbon, K.C.; Hsieh, R. Eigenvalues and eigenvalue sensitivities of a beam supported by viscoelastic solids. J. Vib. Acoust. 2014, 136, 021017. [Google Scholar] [CrossRef]
- Łasecka-Plura, M. A comparative study of the sensitivity analysis for systems with viscoelastic elements. Arch. Mech. Eng. 2023, 70, 5–25. [Google Scholar] [CrossRef]
- Yun, K.S.; Youn, S.K. Design sensitivity analysis for transient response of non-viscously damped dynamic systems. Struct. Multidiscipl. Optim. 2017, 55, 2197–2210. [Google Scholar] [CrossRef]
- Ding, Z.; Li, L.; Li, X.; Kong, J. A comparative study of design sensitivity analysis based on adjoint variable method for transient response of non-viscously damped systems. Mech. Syst. Signal Process. 2018, 110, 390–411. [Google Scholar] [CrossRef]
- Ding, Z.; Zhang, L.; Gao, Q.; Lia, W.H. State-space based discretize-then-differentiate adjoint sensitivity method for transient responses of non-viscously damped systems. Comput. Struct. 2021, 250, 106540. [Google Scholar] [CrossRef]
- Ding, Z.; Li, L.; Zou, G.; Kong, J. Design sensitivity analysis for transient response of non-viscously damped systems based on direct differentiate method. Mech. Syst. Signal Process. 2019, 121, 322–342. [Google Scholar] [CrossRef]
- Ding, Z.; Shi, J.; Gao, Q.; Huang, Q.; Liao, W.H. Design sensitivity analysis for transient responses of viscoelastically damped systems using model order reduction techniques. Struct. Multidiscipl. Optim. 2020, 64, 1501–1526. [Google Scholar] [CrossRef]
- Li, L.; Hu, Y.; Wang, X. Design sensitivity analysis of dynamic response of nonviscously damped systems. Mech. Syst. Signal Process. 2013, 41, 613–638. [Google Scholar] [CrossRef]
- Łasecka-Plura, M.; Lewandowski, R. Design sensitivity analysis of frequency response functions and steady-state response for structures with viscoelastic dampers. Vib. Phys. Syst. 2014, 26, 129–136. [Google Scholar]
- De Lima, A.M.G.; Faria, A.W.; Rade, D.A. Sensitivity analysis of frequency response functions of composite sandwich plates containing viscoelastic layers. Compos. Struct. 2010, 92, 364–376. [Google Scholar] [CrossRef]
- Martinez-Agirre, M.; Elejabarrieta, M.J. Higher order eigensensitivities-based numerical method for the harmonic analysis of viscoelastically damped structures. Int. J. Numer. Methods Eng. 2011, 88, 1280–1296. [Google Scholar] [CrossRef]
- Huang, X.; Zhou, S.; Sun, G.; Li, G.; Xie, Y.M. Topology optimization for microstructures of viscoelastic composite materials. Comput. Methods Appl. Mech. Eng. 2015, 283, 503–516. [Google Scholar] [CrossRef]
- Yun, K.S.; Youn, S.K. Topology optimization of viscoelastic damping layers for attenuating transient response of shell structures. Finite Elem. Anal. Des. 2018, 141, 154–165. [Google Scholar] [CrossRef]
- James, K.A.; Waisman, H. Topology optimization of viscoelastic structures using a time-dependent adjoint method. Comput. Methods Appl. Mech. Eng. 2015, 285, 166–187. [Google Scholar] [CrossRef]
- Pingaro, M.; Venini, P. A fast approach to analysis and optimization of viscoelastic beams. Comput. Struct. 2016, 168, 46–55. [Google Scholar] [CrossRef]
- Jamshidi, B.; Hematiyan, M.R.; Mahzoon, M.; Shiah, Y.C. Load identification for a viscoelastic solid by an accurate meshfree sensitivity analysis. Eng. Struct. 2020, 203, 109895. [Google Scholar] [CrossRef]
- Chongshuai, W.; Yiqian, H.; Haitian, Y. A SBFEM and sensitivity analysis based algorithm for solving inverse viscoelastic problems. Eng. Anal. Bound. Elem. 2019, 106, 588–598. [Google Scholar] [CrossRef]
- Elishakoff, I. Essay on uncertainties in elastic and viscoelastic structures: From A. M. Freudenthal’s criticism to modern convex modeling. Comput. Struct. 1995, 56, 871–895. [Google Scholar] [CrossRef]
- Sepahvand, K.; Marbur, S. On uncertainty quantification in sandwich structures with spatial random damping behavior. In Proceedings of the 9th International Conference on Structural Dynamics, EURODYN 2014, Porto, Portugal, 30 June–2 July 2014. [Google Scholar]
- Hernández, W.P.; Castello, D.A.; Ritto, T.G. Uncertainty propagation analysis in laminated structures with viscoelastic core. Comput. Struct. 2016, 164, 23–37. [Google Scholar] [CrossRef]
- Hernández, W.P.; Castello, D.A.; Ritto, T.G. Stochastic fields to analyze uncertainties in laminated structures with viscoelastic core. J. Comput. Interdiscip. Sci. 2018, 9, 195–204. [Google Scholar] [CrossRef]
- Silva, V.A.C.; de Lima, A.M.G.; Bouhaddi, N.; Lacerda, H.B. Uncertainty propagation and experimental verification of nonlinear viscoelastic sandwich beams. Mech. Syst. Signal Process. 2019, 132, 654–669. [Google Scholar] [CrossRef]
- Wang, T.; Xu, C.; Hamdaoui, M.; Guo, N.; Gu, L. Uncertainty propagation in modal analysis of viscoelastic sandwich structures using a stochastic collocation method. J. Sandw. Struct. 2021, 23, 1141–1165. [Google Scholar] [CrossRef]
- Adhikari, S. On the quantification of damping model uncertainty. J. Sound Vib. 2007, 306, 153–171. [Google Scholar] [CrossRef]
- Wang, T.; Xu, C.; Guo, N. A novel frequency-labeled adaptive sparse grid collocation method for uncertainty quantification of the frequency response of general viscoelastic damping structures. Int. J. Mech. Sci. 2021, 193, 106168. [Google Scholar] [CrossRef]
- Wang, T.; Xu, C.; Guo, N.; Hamdaoui, M.; Daya, E.I. Uncertainty propagation of frequency response of viscoelastic damping structures using a modified high-dimensional adaptive sparse grid collocation method. Mech. Adv. Mater. Struct. 2022, 29, 506–524. [Google Scholar] [CrossRef]
- Soize, C.; Poloskov, I.E. Time-domain formulation in computational dynamics for linear viscoelastic media with model uncertainties and stochastic excitation. Comput. Math. With Appl. 2012, 64, 3594–3612. [Google Scholar] [CrossRef]
- Capillon, R.; Desceliers, C.; Soize, C. Uncertainty quantification for viscoelastic composite structures in computational linear structural dynamics. In Proceedings of the ISMA2016-USD2016, Leuven, Belgium, 19–24 September 2016. [Google Scholar]
- Capillon, R.; Desceliers, C.; Soize, C. Uncertainty quantification in computational linear structural dynamics for viscoelastic composite structures. Comput. Methods Appl. Mech. Eng. 2016, 305, 154–172. [Google Scholar] [CrossRef]
- Capillon, R.; Desceliers, C.; Soize, C. Model uncertainties in computational viscoelastic linear structural dynamics. Procedia Eng. 2017, 199, 1210–1215. [Google Scholar] [CrossRef]
- Guerdi, M.; Lima, A.M.G.; Bouhaddi, N.; Rade, D.A. Robust design of viscoelastic structures based on stochastic finite element models. Mech. Syst. Signal Process. 2010, 24, 59–77. [Google Scholar] [CrossRef]
- Guo, A.X.; Xu, Y.L.; Wu, B. Seismic reliability analysis of hysteretic structure with viscoelastic dampers. Eng. Struct. 2002, 24, 373–383. [Google Scholar] [CrossRef]
- Gonçalves, L.K.S.; Rosa, U.L.; de Lima, A.M.G. Fatigue damage investigation and optimization of a viscoelastically damped system with uncertainties. J. Brazilian Soc. Mech. 2019, 41, 382. [Google Scholar] [CrossRef]
- Nasab, M.S.E.; Kim, J. Fuzzy analysis of a viscoelastic damper in seismic retrofit of structures. Eng. Struct. 2022, 250, 113473. [Google Scholar] [CrossRef]
- Nasab, M.S.E.; Guo, Y.Q.; Kim, J. Seismic retrofit of a soft first-story building using viscoelastic dampers considering inherent uncertainties. J. Build. Eng. 2022, 47, 103866. [Google Scholar] [CrossRef]
- Elishakoff, I.; Elisseeff, P.; Glegg, S.A.L. Nonprobabilistic, Convex-Theoretic modeling of scatter in material properties. AIAA J. 1994, 32, 843–849. [Google Scholar] [CrossRef]
- Fujita, K.; Takewaki, I. An efficient methodology for robustness evaluation by advanced interval analysis using updated second-order Taylor series expansion. Eng. Struct. 2011, 33, 3299–3310. [Google Scholar] [CrossRef]
- Łasecka-Plura, M.; Lewandowski, R. Dynamic characteristics and frequency response function for frame with dampers with uncertain design parameters. Mech. Based Des. Struct. Mach. 2017, 45, 296–312. [Google Scholar] [CrossRef]
- Łasecka-Plura, M. Dynamic characteristics of a composite beam with viscoelastic layers under uncertain-but-bounded design parameters. Appl. Sci. 2023, 13, 6473. [Google Scholar] [CrossRef]
- Sofi, A.; Muscolino, G.; di Paola, M. Reliability analysis of structures controlled by external fractional viscoelastic dampers with interval parameters. Acta Mech. Sin. 2023, 39, 722486. [Google Scholar] [CrossRef]
- Peng, R.; He, Y.; Yang, H. An efficient numerical method to solve inverse fuzzy-uncertain viscoelastic problems of identification. Inverse Probl. Sci. Eng. 2021, 29, 920–943. [Google Scholar] [CrossRef]
- Kookalani, S.; Shen, D.; Zhu, L.L.; Lindsey, M. An Overview of Optimal Damper Placement Methods in Structures. Iran. J. Sci. Technol. Trans. Civ. Eng. 2022, 46, 1785–1804. [Google Scholar] [CrossRef]
- Takewaki, I. Building Control with Passive Dampers: Optimal Performance-Based Design for Earthquakes; John Wiley & Sons (Asia) Pte. Ltd.: Singapore, 2009. [Google Scholar] [CrossRef]
- Takewaki, I. Optimal damper placement for planar building frames using transfer functions. Struct. Multidiscip. Optim. 2000, 20, 280–287. [Google Scholar] [CrossRef]
- Sánchez, W.E.D.; Avila, S.M.; de Brito, J.L.V. Optimal placement of damping devices in buildings. J. Braz. Soc. Mech. Sci. Eng. 2018, 40, 337. [Google Scholar] [CrossRef]
- Takewaki, I. Optimal damper placement for minimum transfer functions. Earthq. Eng. Struct Dyn. 1997, 26, 1113–1124. [Google Scholar] [CrossRef]
- Cimellaro, G.P. Simultaneous stiffness-damping optimization of structures with respect to acceleration, displacement and base shear. Eng. Struct. 2007, 29, 2853–2870. [Google Scholar] [CrossRef]
- Sonmez, M.; Aydin, E.; Karabork, T. Using an artificial bee colony algorithm for the optimal placement of viscous dampers in planar building frames. Struct. Multidiscip. Optim. 2013, 48, 395–409. [Google Scholar] [CrossRef]
- Lavan, O.; Levy, R. Optimal design of supplemental viscous dampers for irregular shear-frames in the presence of yielding. Earthq. Eng. Struct. Dyn. 2005, 34, 889–907. [Google Scholar] [CrossRef]
- Aydin, E. Optimal damper placement based on base moment in steel building frames. J. Constr. Steel Res. 2012, 79, 216–225. [Google Scholar] [CrossRef]
- Lavan, O.; Dargush, G.F. Multi-objective evolutionary seismic design with passive energy dissipation systems. J. Earthq. Eng. 2009, 13, 758–790. [Google Scholar] [CrossRef]
- Xie, Z.; Shepard, W.; Woodbury, K. Design Optimization for Vibration Reduction of Viscoelastic Damped Structures Using Genetic Algorithms. Shock Vib. 2009, 16, 455–466. [Google Scholar] [CrossRef]
- Pricopie, A.; Costache, A. Viscous Damper Distribution Using Genetic Algorithms and Pattern Search Optimization. In The 1940 Vrancea Earthquake. Issues, Insights and Lessons Learnt; Vacareanu, R., Ionescu, C., Eds.; Springer: Cham, Switzerland, 2016. [Google Scholar] [CrossRef]
- Lenartowicz, A.; Przychodzki, M.; Guminiak, M.; Garbowski, T. Optimal placement of viscoelastic vibration dampers for Kirchhoff plates based on PSO method. Materials 2021, 14, 6616. [Google Scholar] [CrossRef] [PubMed]
- Fujita, K.; Moustafa, A.; Takewaki, I. Optimal placement of visco-elastic dampers and supporting members under variable critical excitations. Earthq. Struct. 2010, 1, 43–67. [Google Scholar] [CrossRef]
- Akehashi, H.; Takewaki, I. Comparative investigation on optimal viscous damper placement for elastic-plastic MDOF structures: Transfer function amplitude or double impulse. Soil Dynam. Earthq. Eng. 2020, 130, 105987. [Google Scholar] [CrossRef]
- Lavan, O. Optimal Design of Viscous Dampers and Their Supporting Members for the Seismic Retrofitting of 3D Irregular Frame Structures. J. Struct. Eng. 2015, 141, 04015026. [Google Scholar] [CrossRef]
- Lopez-Garcia, D.; Soong, T.T. Efficiency of a simple approach to damper allocation in MDOF structures. J. Struct. Control 2002, 9, 19–30. [Google Scholar] [CrossRef]
- Hussien, O.S.; Elamy, M.I. Optimal placement of dampers on multistorey frames using dynamic analysis. Strength Mater. 2020, 52, 470–479. [Google Scholar] [CrossRef]
- Hwang, J.S.; Lin, W.C.; Wu, N.J. Comparison of distribution methods for viscous damping coefficients to buildings. Struct. Infrastruct. Eng. 2013, 9, 28–41. [Google Scholar] [CrossRef]
- Singh, M.P.; Moreschi, L.M. Optimal seismic response control with dampers. Earthq. Eng. Struct. Dyn. 2001, 30, 553–572. [Google Scholar] [CrossRef]
- Rayegani, A.; Nouri, G. Seismic collapse probability and life cycle cost assessment of isolated structures subjected to pounding with smart hybrid isolation system using a modified fuzzy based controller. Structures 2022, 44, 30–41. [Google Scholar] [CrossRef]
- Gong, W.; Xiong, S. Probabilistic seismic risk assessment of modified pseudonegative stiffness control of a base-isolated building. Struct. Infrastruct. Eng. 2016, 12, 1295–1309. [Google Scholar] [CrossRef]
- Rayegani, A.; Nouri, G. Application of Smart Dampers for Prevention of Seismic Pounding in Isolated Structures Subjected to Near-fault Earthquakes. J. Earthq. Eng. 2022, 26, 4069–4084. [Google Scholar] [CrossRef]
- Lewandowski, R.; Litewka, P. Nonlinear harmonic vibrations of laminate plates with viscoelastic layers using refined zig-zag theory. Part 1—Theoretical background. Compos. Struct. 2023, 320, 117200. [Google Scholar] [CrossRef]
- Lewandowski, R.; Litewka, P. Nonlinear harmonic vibrations of laminate plates with VE layers using refined zig-zag theory. Part 2—Numerical solution. Compos. Struct. 2023, 319, 117062. [Google Scholar] [CrossRef]

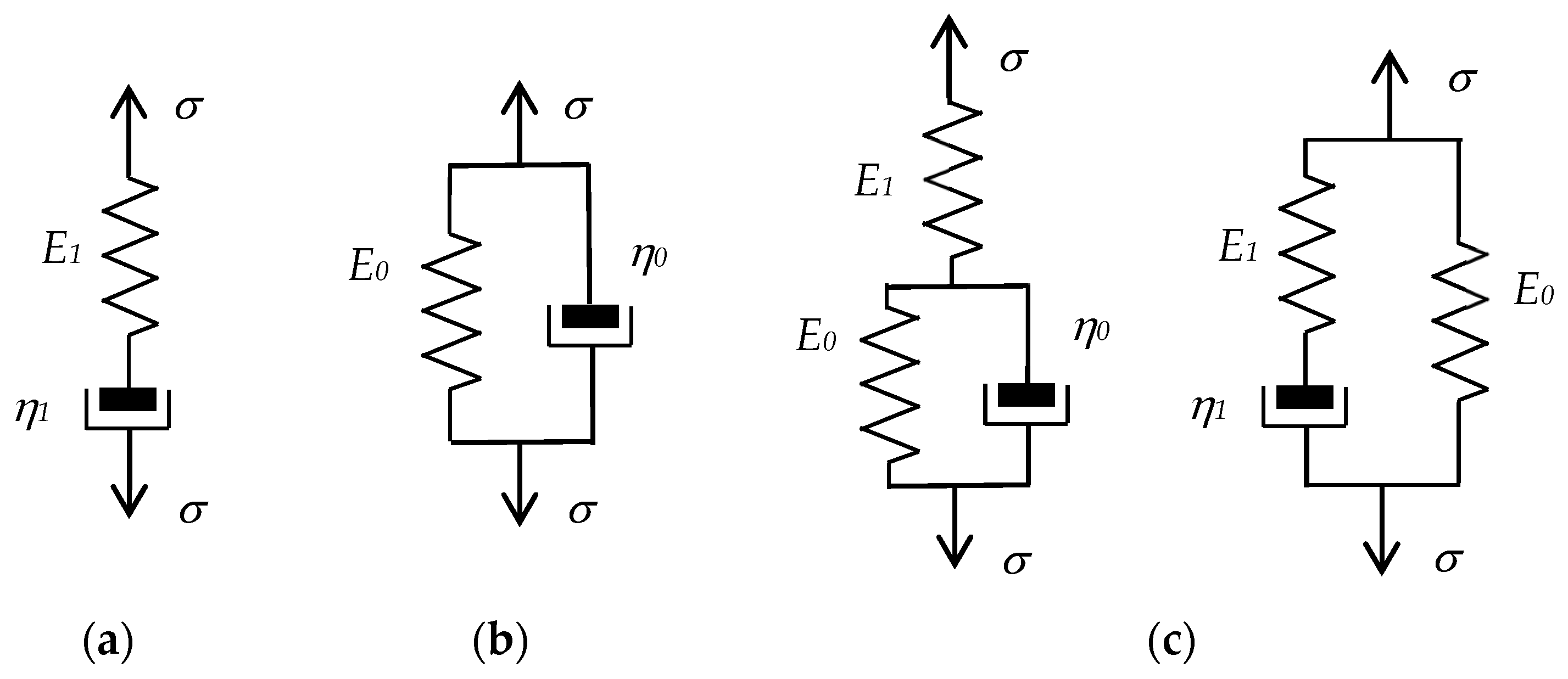
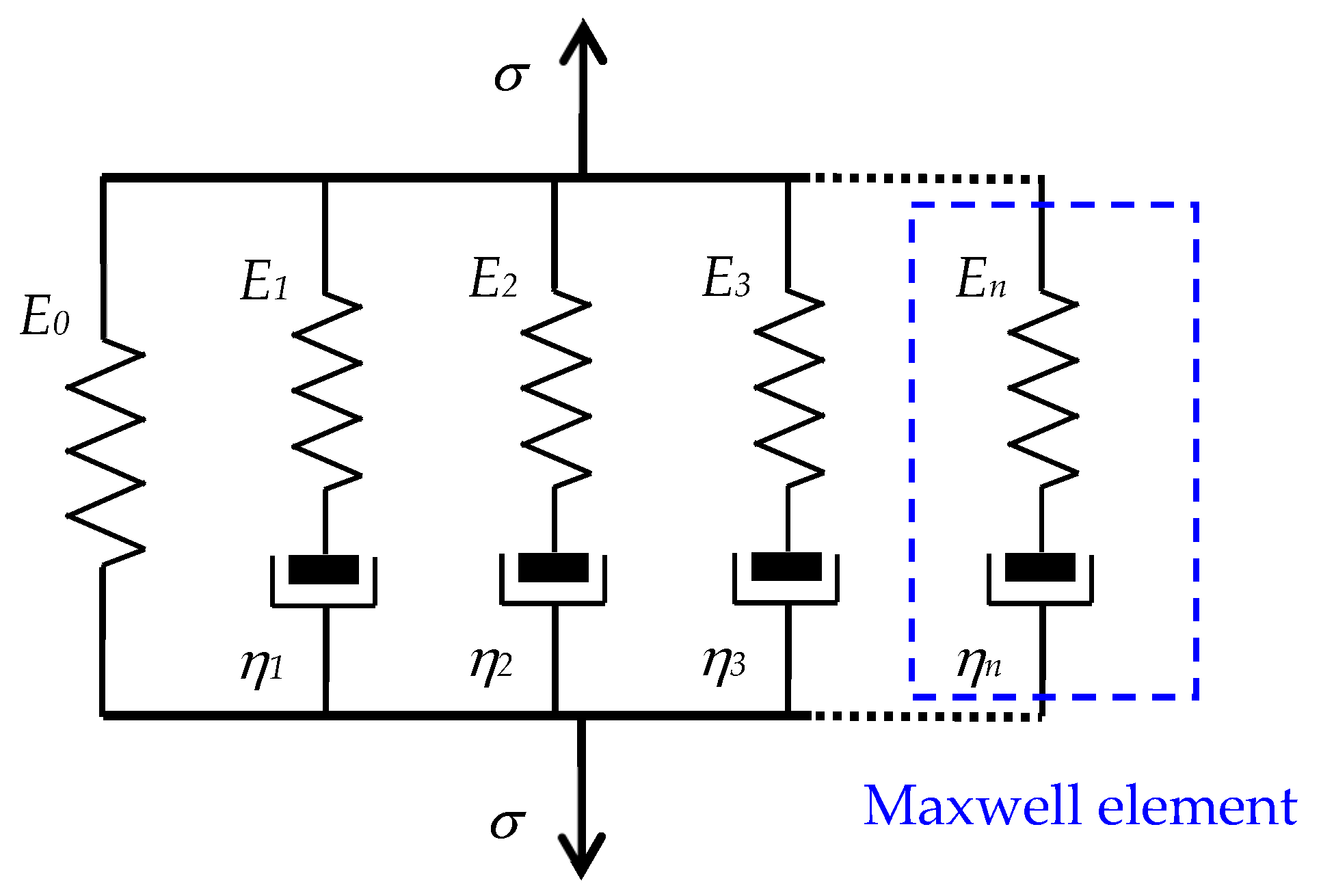
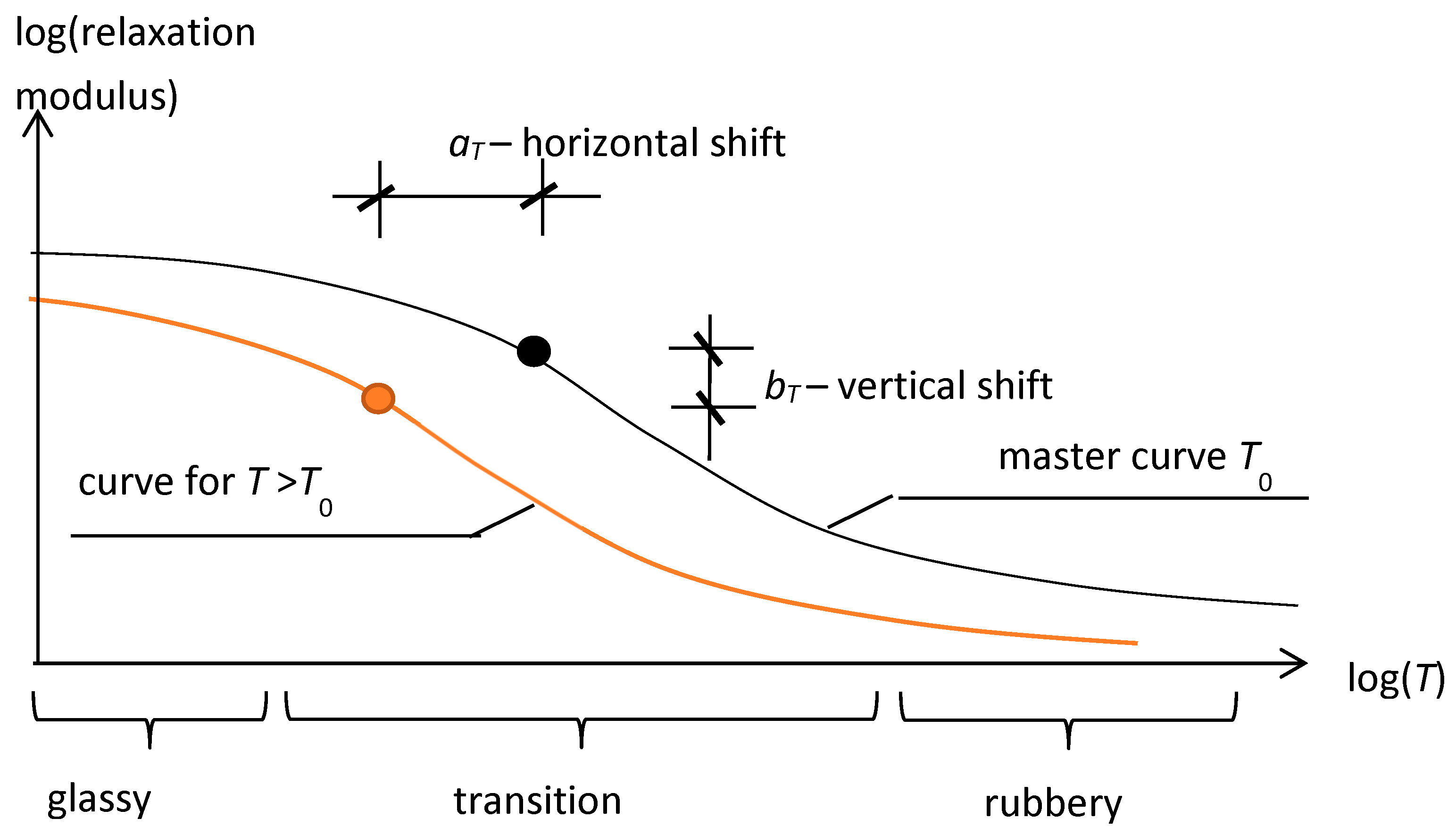



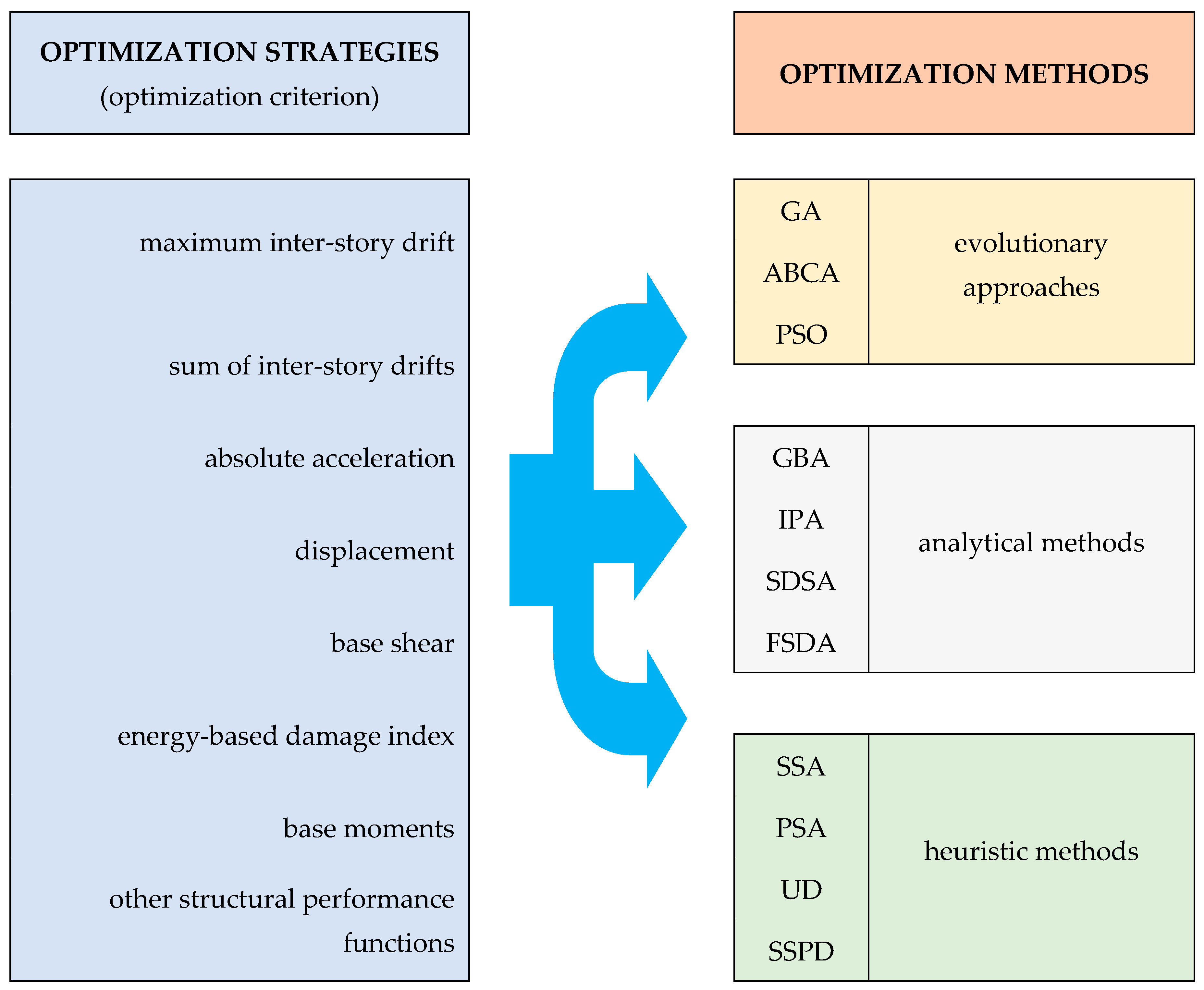
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Lewandowski, R.; Litewka, P.; Łasecka-Plura, M.; Pawlak, Z.M. Dynamics of Structures, Frames, and Plates with Viscoelastic Dampers or Layers: A Literature Review. Buildings 2023, 13, 2223. https://doi.org/10.3390/buildings13092223
Lewandowski R, Litewka P, Łasecka-Plura M, Pawlak ZM. Dynamics of Structures, Frames, and Plates with Viscoelastic Dampers or Layers: A Literature Review. Buildings. 2023; 13(9):2223. https://doi.org/10.3390/buildings13092223
Chicago/Turabian StyleLewandowski, Roman, Przemysław Litewka, Magdalena Łasecka-Plura, and Zdzisław M. Pawlak. 2023. "Dynamics of Structures, Frames, and Plates with Viscoelastic Dampers or Layers: A Literature Review" Buildings 13, no. 9: 2223. https://doi.org/10.3390/buildings13092223
APA StyleLewandowski, R., Litewka, P., Łasecka-Plura, M., & Pawlak, Z. M. (2023). Dynamics of Structures, Frames, and Plates with Viscoelastic Dampers or Layers: A Literature Review. Buildings, 13(9), 2223. https://doi.org/10.3390/buildings13092223







