The Quality Assessment of Timber Structural Joints Using the Coaxial Correlation Method
Abstract
1. Introduction
2. Materials and Methods
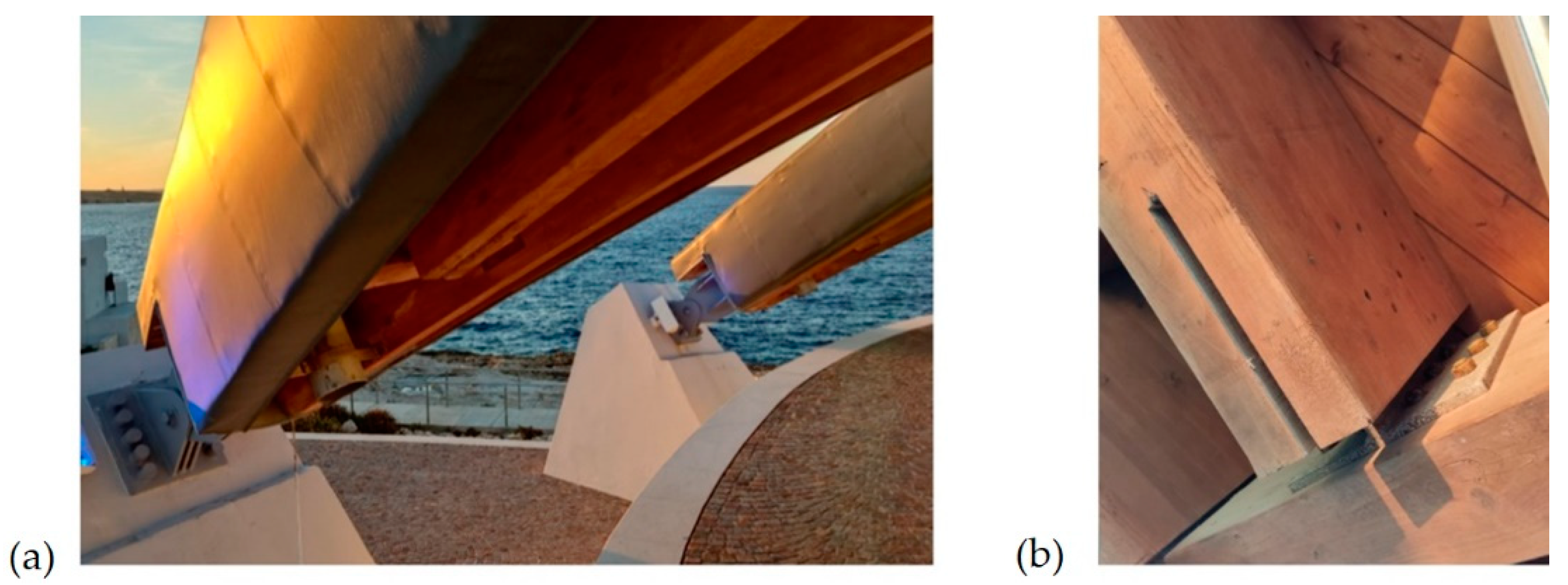
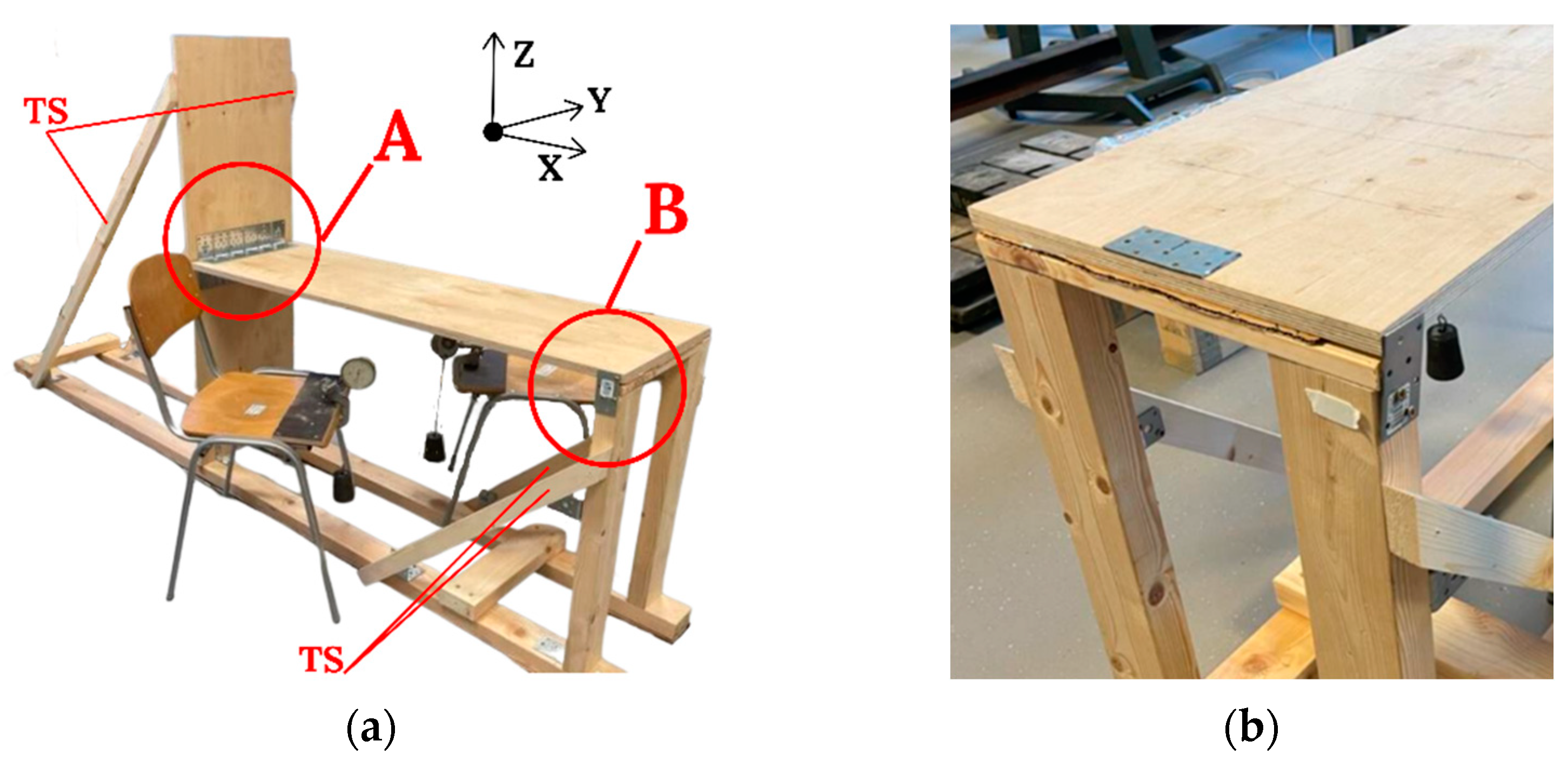
2.1. Choice of the Object of Investigation
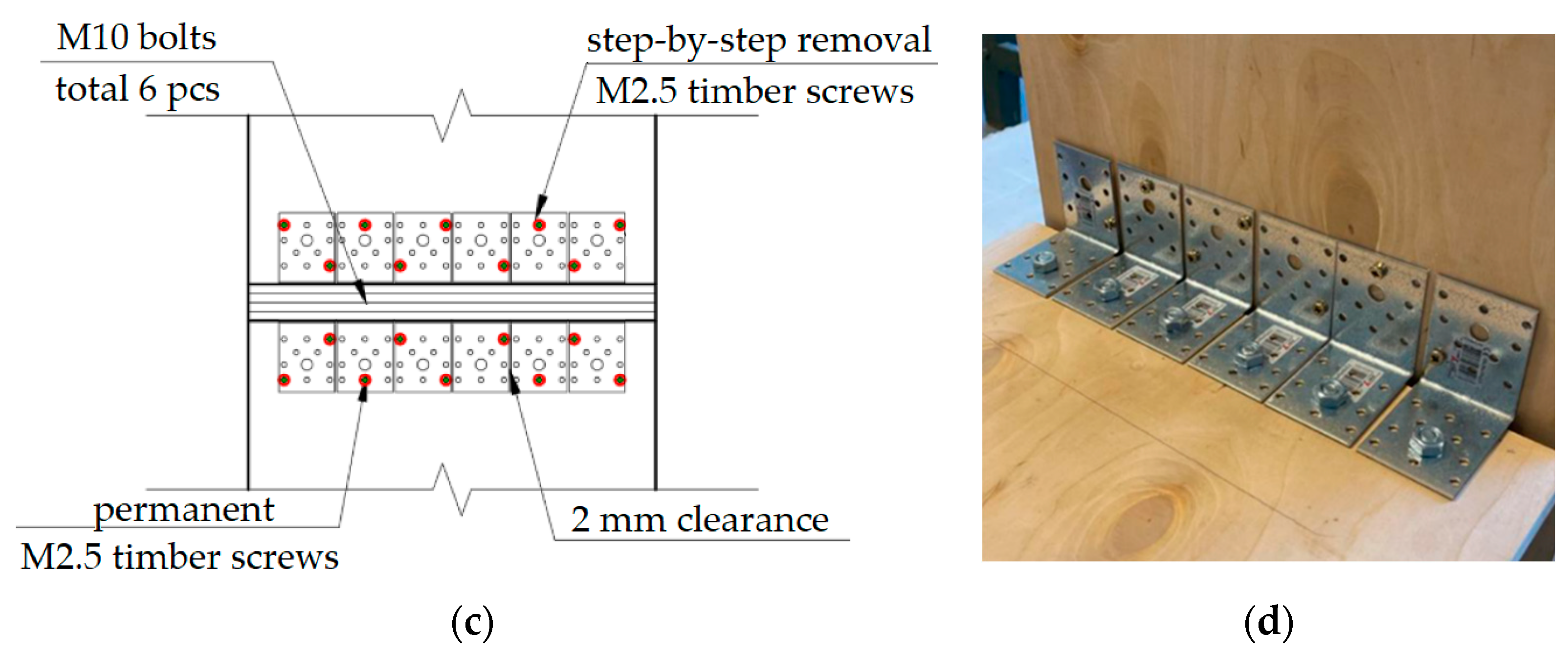
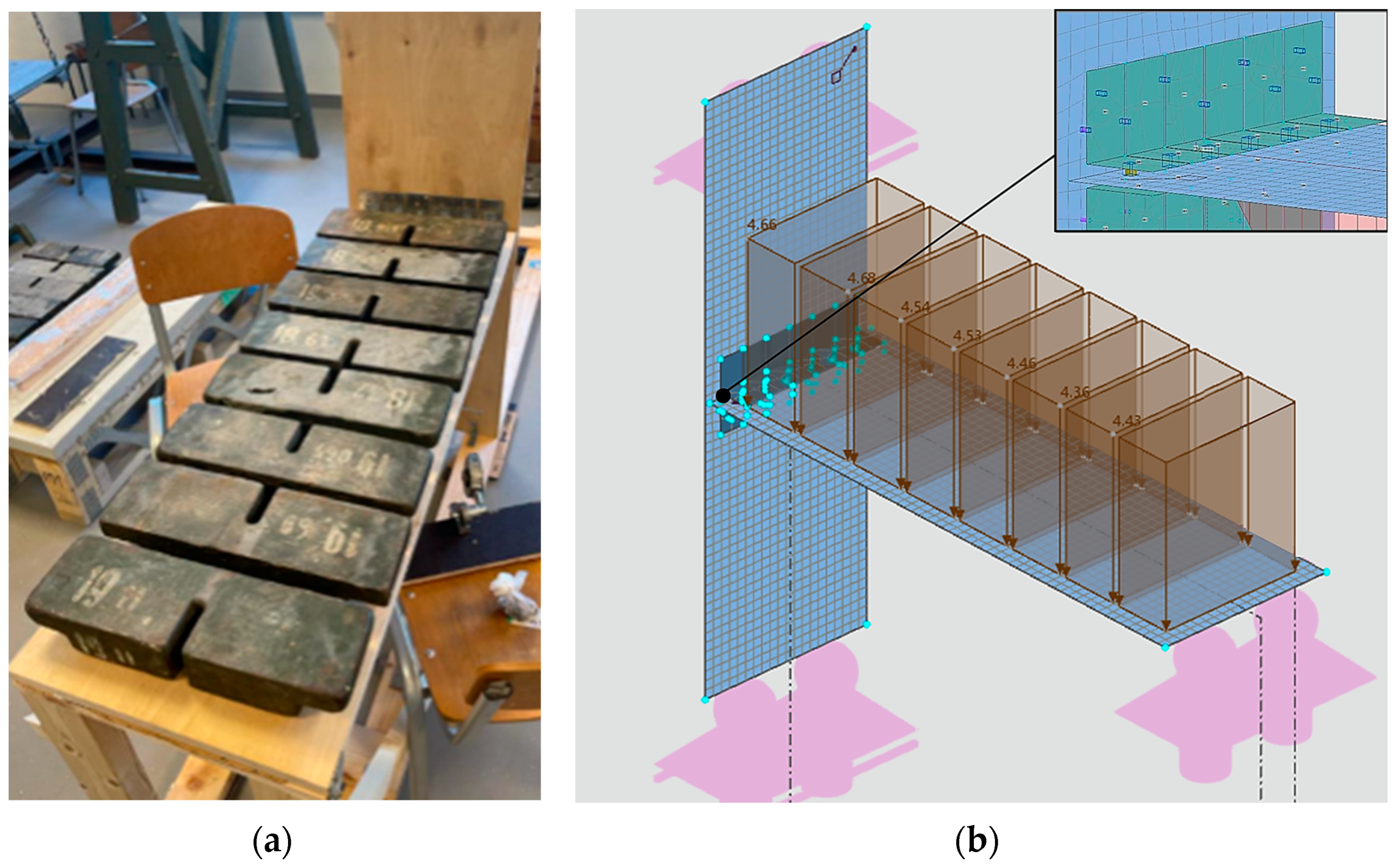
2.2. Evaluation of Stiffness of Investigated Joint Using Static Loading
2.3. Coaxial Correlation Method for Structural Joint Quality Evaluation
3. Results and Discussion
4. Conclusions
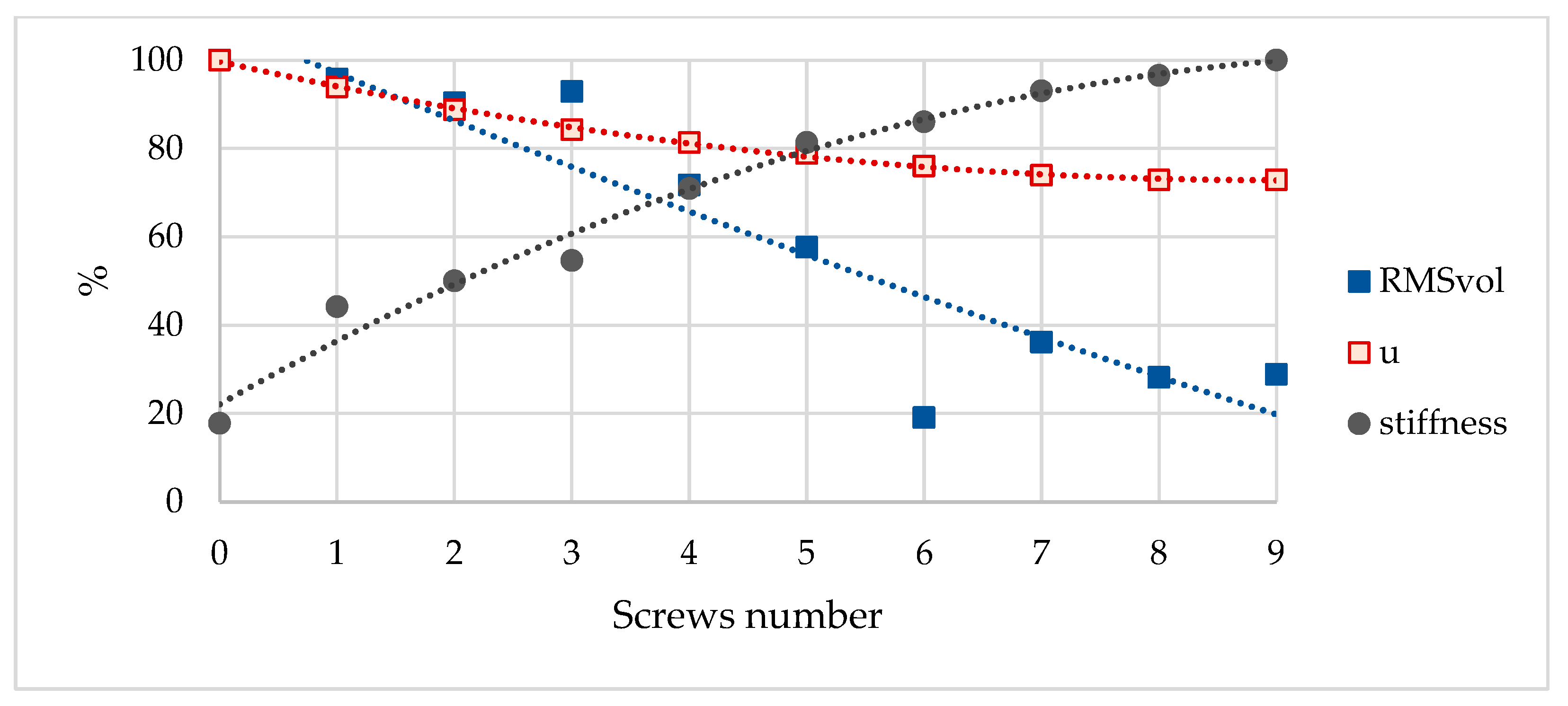
- There is a clear relationship between joint stiffness, vertical displacements, and the RMSvol parameter, as well as the RMSvol parameter more sensitively reflecting changes in the joint’s condition compared to maximal vertical displacements.
- In the case of the input signal as a sweep signal in the frequency range of 10 Hz to 2000 Hz and the placement of the accelerometer pair symmetrically on either side of the joint, aligned with its axis, an inverse correlation between the calculated RMSvol values of convolution signals and the panel-to-panel moment joint rotational stiffness is obtained.
- The reason for the opposite direction of the relation mentioned above can be signal scattering.
- The changes in the RMSvol parameter can be used for monitoring the system’s condition during operation as a reference point using determined parameters at the initial stage, with an approved design stiffness condition. But, it is recommended that, at the stage of the reference measurement, it should be determined whether the input signal is well transferred through the joint by comparing the power of the response signals from both sides of the joint of interest to save a more user-friendly interpretation of the results.
Author Contributions
Funding

Data Availability Statement
Conflicts of Interest
References
- Basterra, L.-A.; Baño, V.; López, G.; Cabrera, G.; Vallelado-Cordobés, P. Identification and Trend Analysis of Multistorey Timber Buildings in the SUDOE Region. Buildings 2023, 13, 1501. [Google Scholar] [CrossRef]
- Le, S.T.; Nguyen, T.N.; Bui, D.-K.; Ha, Q.P.; Ngo, T.D. Modelling and Multi-Objective Optimisation of Finger Joints: Improving Flexural Performance and Minimising Wood Waste. Buildings 2023, 13, 1186. [Google Scholar] [CrossRef]
- Dobeš, P.; Lokaj, A.; Vavrušová, K. Stiffness and Deformation Analysis of Cross-Laminated Timber (CLT) Panels Made of Nordic Spruce Based on Experimental Testing, Analytical Calculation and Numerical Modeling. Buildings 2023, 13, 200. [Google Scholar] [CrossRef]
- Kiviste, M.; Musakka, S.; Ruus, A.; Vinha, J. A Review of Non-Residential Building Renovation and Improvement of Energy Efficiency: Office Buildings in Finland, Sweden, Norway, Denmark, and Germany. Energies 2023, 16, 4220. [Google Scholar] [CrossRef]
- Tupenaite, L.; Kanapeckiene, L.; Naimaviciene, J.; Kaklauskas, A.; Gecys, T. Timber Construction as a Solution to Climate Change: A Systematic Literature Review. Buildings 2023, 13, 976. [Google Scholar] [CrossRef]
- Brics, A.; Serdjuks, D.; Gravit, M.; Buka-Vaivade, K.; Goremikins, V.; Vatin, N.I.; Podkoritovs, A. The Behaviour of Load-Carrying Members from Cordwood. Buildings 2022, 12, 1702. [Google Scholar] [CrossRef]
- Savolainen, J.M.; Ilgın, H.E.; Oinas, E.; Karjalainen, M. Finnish Multi-Story Timber-Framed Apartment Buildings: Tampere Residents’ Perspectives. Buildings 2022, 12, 1998. [Google Scholar] [CrossRef]
- Li, Z.; Tsavdaridis, K.D. Design for Seismic Resilient Cross Laminated Timber (CLT) Structures: A Review of Research, Novel Connections, Challenges and Opportunities. Buildings 2023, 13, 505. [Google Scholar] [CrossRef]
- Kysela, P.; Ponechal, R.; Michálková, D. Airtightness of a Critical Joint in a Timber-Based Building Affected by the Seasonal Climate Change. Buildings 2023, 13, 698. [Google Scholar] [CrossRef]
- Loebjinski, M.; Rug, W.; Pasternak, H. The Influence of Improved Strength Grading In Situ on Modelling Timber Strength Properties. Buildings 2020, 10, 30. [Google Scholar] [CrossRef]
- Yeh, Y.-H.; Yeh, Y.-C.; Wang, T.-C.; Hsu, Y.-C. Comparison of Self-Tapping Screws and Bamboo Nails in Reinforcing the Zuo-Dou Connections in Traditional Oriental Timber Frames of Asia. Buildings 2023, 13, 709. [Google Scholar] [CrossRef]
- Korolkov, D.; Gravit, M.; Aleksandrovskiy, M. Estimation of the Residual Resource of Wooden Structures by Changing Geometric Parameters of the Cross-Section. E3S Web Conf. 2021, 244, 04010. [Google Scholar] [CrossRef]
- Buka-Vaivade, K.; Gaile, L.; Serdjuks, D.; Tatarinovs, A.; Pakrastins, L. Non-Destructive Quality Control of the Adhesive Rigid Timber-to-Concrete Connection in TCC Structures. Buildings 2022, 12, 2151. [Google Scholar] [CrossRef]
- Deng, Z.; Huang, M.; Wan, N.; Zhang, J. The Current Development of Structural Health Monitoring for Bridges: A Review. Buildings 2023, 13, 1360. [Google Scholar] [CrossRef]
- Korolkov, D.I.; Chernykh, A.G.; Kazakevich, T.N.; Mamedov, S.M.; Gravit, M.V. Use of Two-Parameter Distributions for a Joint Assessment of the Residual Resource of Building Structures and Engineering Systems of Buildings and Structures. IOP Conf. Ser. Mater. Sci. Eng. 2020, 775, 012142. [Google Scholar] [CrossRef]
- Josifovski, A.; Todorović, N.; Milošević, J.; Veizović, M.; Pantelić, F.; Aškrabić, M.; Vasov, M.; Rajčić, A. An Approach to In Situ Evaluation of Timber Structures Based on Equalization of Non-Destructive and Mechanical Test Parameters. Buildings 2023, 13, 1405. [Google Scholar] [CrossRef]
- Sola-Caraballo, J.; Rincón-Calderón, J.M.; Rivera-Gómez, C.; López-Martínez, J.A.; Galán-Marín, C. On-Site Risk Assessment Methodology of Historic Timber Structures: The Case Study of Santa Cruz Church. Buildings 2022, 12, 935. [Google Scholar] [CrossRef]
- Jaskowska-Lemańska, J.; Przesmycka, E. Semi-Destructive and Non-Destructive Tests of Timber Structure of Various Moisture Contents. Materials 2021, 14, 96. [Google Scholar] [CrossRef]
- Perković, N.; Stepinac, M.; Rajčić, V.; Barbalić, J. Assessment of Timber Roof Structures before and after Earthquakes. Buildings 2021, 11, 528. [Google Scholar] [CrossRef]
- Han, F.; Zhang, Q.; Wang, C.; Lu, G.; Jiang, J. Structural Health Monitoring of Timber Using Electromechanical Impedance (EMI) Technique. Adv. Civ. Eng. 2020, 2020, e1906289. [Google Scholar] [CrossRef]
- Bakkar, A.R.; Elyamani, A.; El-Attar, A.G.; Bompa, D.V.; Elghazouli, A.Y.; Mourad, S.A. Dynamic Characterisation of a Heritage Structure with Limited Accessibility Using Ambient Vibrations. Buildings 2023, 13, 192. [Google Scholar] [CrossRef]
- Saidin, S.S.; Jamadin, A.; Abdul Kudus, S.; Mohd Amin, N.; Anuar, M.A. An Overview: The Application of Vibration-Based Techniques in Bridge Structural Health Monitoring. Int. J. Concr. Struct. Mater. 2022, 16, 69. [Google Scholar] [CrossRef]
- Morgantini, M.; Betti, R. The Inner Product Vector as an Output-Only Cross-Correlation-Based Feature to Structural Damage Assessment. J. Vibroeng. 2020, 22, 1373–1398. [Google Scholar] [CrossRef]
- Zielińska, M.; Rucka, M. Non-Destructive Testing of Wooden Elements. IOP Conf. Ser. Mater. Sci. Eng. 2021, 1203, 032058. [Google Scholar] [CrossRef]
- Baas, E.J.; Riggio, M.; Barbosa, A.R. A Methodological Approach for Structural Health Monitoring of Mass-Timber Buildings under Construction. Constr. Build. Mater. 2021, 268, 121153. [Google Scholar] [CrossRef]
- Petrović, M.; Pavićević, D.; Ilić, I.; Terzović, J.; Šekularac, N. Elements of a Timber Lamella Structure: Analysis and Systematization of Joints. Buildings 2023, 13, 885. [Google Scholar] [CrossRef]
- Han, F.; Jiang, J.; Xu, K.; Wang, N. Damage Detection of Common Timber Connections Using Piezoceramic Transducers and Active Sensing. Sensors 2019, 19, 2486. [Google Scholar] [CrossRef]
- Dong, L.; Yang, Z.; Wang, Z.; Ding, Y.; Weiqiang, Q. Study on Internal Force of Tunnel Segment by Considering the Influence of Joints. Adv. Mater. Sci. Eng. 2020, 2020, 1020732. [Google Scholar] [CrossRef]
- Hou, Y.; Hu, W.; Wang, X.; Hou, T.; Sun, C. Damage Identification of Ancient Timber Structure Based on Autocorrelation Function. Adv. Civ. Eng. 2021, 2021, e6683666. [Google Scholar] [CrossRef]
- Kurajevs, A. Quality Assessment of Structural Joints of Timber Structures Using Forced Vibrations; Riga Technical University: Riga, Latvia, 2023. [Google Scholar]
- Serdjuks, D.; Kurtenoks, V.; Tatarinovs, A.; Mironovs, V.; Lapkovskis, V.; Buka-Vaivade, K.; Macevics, A.; Topcijs, K.; Vilnitis, M. Method of Coaxial Accelerations Correlation for Quality Assessment of Structural Joints. Procedia Struct. Integr. 2022, 37, 547–554. [Google Scholar] [CrossRef]
- Serdjuks, D.; Kurtenoks, V.; Tatarinovs, A.; Buka-Vaivade, K.; Lapkovskis, V.; Mironovs, V.; Podkoritovs, A.; Topcijs, K. Non-Model Vibration Analysis Method for Health Monitoring of Structural Joints. Procedia Struct. Integr. 2022, 37, 555–562. [Google Scholar] [CrossRef]
- Buka-Vaivade, K.; Kurtenoks, V.; Serdjuks, D. Non-Destructive Damage Detection of Structural Joint by Coaxial Correlation Method in 6D Space. Buildings 2023, 13, 1151. [Google Scholar] [CrossRef]
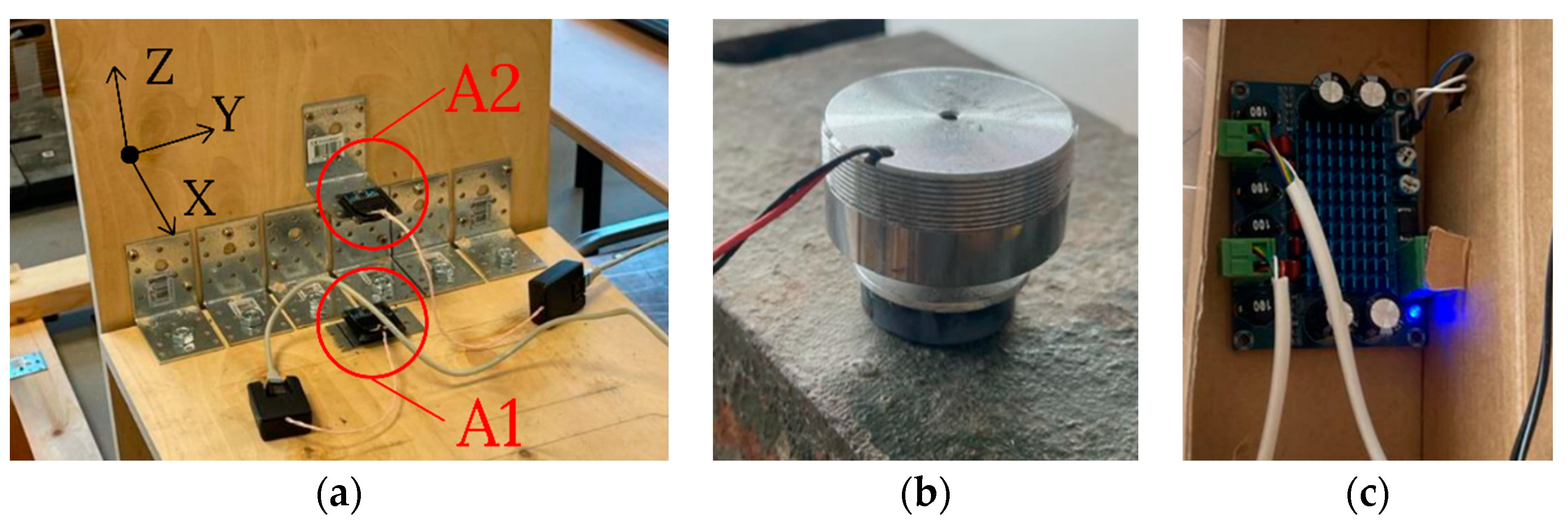
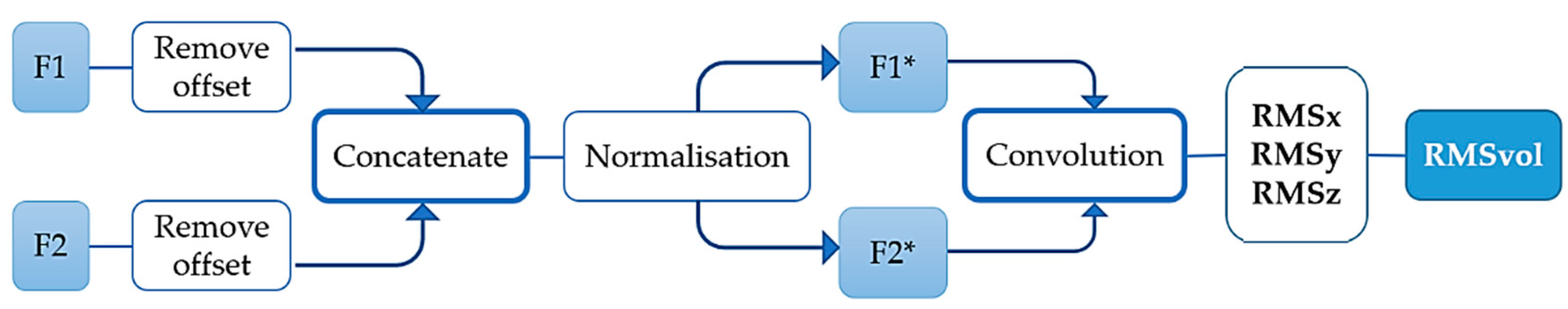

| Nr. | Screws Number | Displacement, mm | Difference, % | Rotational Stiffness of the Investigated Joint Obtained by the 2nd Numerical Model, [kNm × rad−1 × m−1] | |
|---|---|---|---|---|---|
| uexp | uFEM,1 | ||||
| 1 | 9 | 17.85 | 18.3 | 2.46 | 8.60 |
| 2 | 8 | 17.83 | 18.5 | 3.65 | 8.30 |
| 3 | 7 | 18.10 | 18.6 | 2.69 | 8.00 |
| 4 | 6 | 18.61 | 18.9 | 1.56 | 7.40 |
| 5 | 5 | 19.30 | 19.1 | 1.05 | 7.00 |
| 6 | 4 | 19.90 | 19.7 | 1.02 | 6.10 |
| 7 | 3 | 20.63 | 20.6 | 0.15 | 4.70 |
| 8 | 2 | 21.73 | 20.9 | 3.95 | 4.30 |
| 9 | 1 | 22.99 | 21.4 | 7.43 | 3.80 |
| 10 | 0 | 24.48 | 23.9 | 2.43 | 1.53 |
| Nr. | Screws Number | RMSx | RMSy | RMSz | RMSvol |
|---|---|---|---|---|---|
| 1 | 9 | 1.5739 | 4.2289 | 6.0235 | 7.5262 |
| 2 | 8 | 2.5188 | 2.7883 | 6.3096 | 7.3437 |
| 3 | 7 | 3.7834 | 2.2017 | 8.3543 | 9.4316 |
| 4 | 6 | 1.0254 | 3.8577 | 2.9503 | 4.9636 |
| 5 | 5 | 0.9018 | 5.4463 | 13.9686 | 15.0199 |
| 6 | 4 | 2.6823 | 5.8421 | 17.5589 | 18.6986 |
| 7 | 3 | 4.7522 | 4.2519 | 23.3603 | 24.2150 |
| 8 | 2 | 0.9565 | 4.3896 | 23.1195 | 23.5519 |
| 9 | 1 | 2.2475 | 4.2156 | 24.4985 | 24.9599 |
| 10 | 0 | 7.4473 | 4.4542 | 24.5854 | 26.0720 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Kurtenoks, V.; Kurajevs, A.; Buka-Vaivade, K.; Serdjuks, D.; Lapkovskis, V.; Mironovs, V.; Podkoritovs, A.; Vilnitis, M. The Quality Assessment of Timber Structural Joints Using the Coaxial Correlation Method. Buildings 2023, 13, 1929. https://doi.org/10.3390/buildings13081929
Kurtenoks V, Kurajevs A, Buka-Vaivade K, Serdjuks D, Lapkovskis V, Mironovs V, Podkoritovs A, Vilnitis M. The Quality Assessment of Timber Structural Joints Using the Coaxial Correlation Method. Buildings. 2023; 13(8):1929. https://doi.org/10.3390/buildings13081929
Chicago/Turabian StyleKurtenoks, Viktors, Aleksis Kurajevs, Karina Buka-Vaivade, Dmitrijs Serdjuks, Vjaceslavs Lapkovskis, Viktors Mironovs, Andrejs Podkoritovs, and Martins Vilnitis. 2023. "The Quality Assessment of Timber Structural Joints Using the Coaxial Correlation Method" Buildings 13, no. 8: 1929. https://doi.org/10.3390/buildings13081929
APA StyleKurtenoks, V., Kurajevs, A., Buka-Vaivade, K., Serdjuks, D., Lapkovskis, V., Mironovs, V., Podkoritovs, A., & Vilnitis, M. (2023). The Quality Assessment of Timber Structural Joints Using the Coaxial Correlation Method. Buildings, 13(8), 1929. https://doi.org/10.3390/buildings13081929








