Rocking Analysis of Towers Subjected to Horizontal Forces
Abstract
1. Introduction
2. Materials and Methods
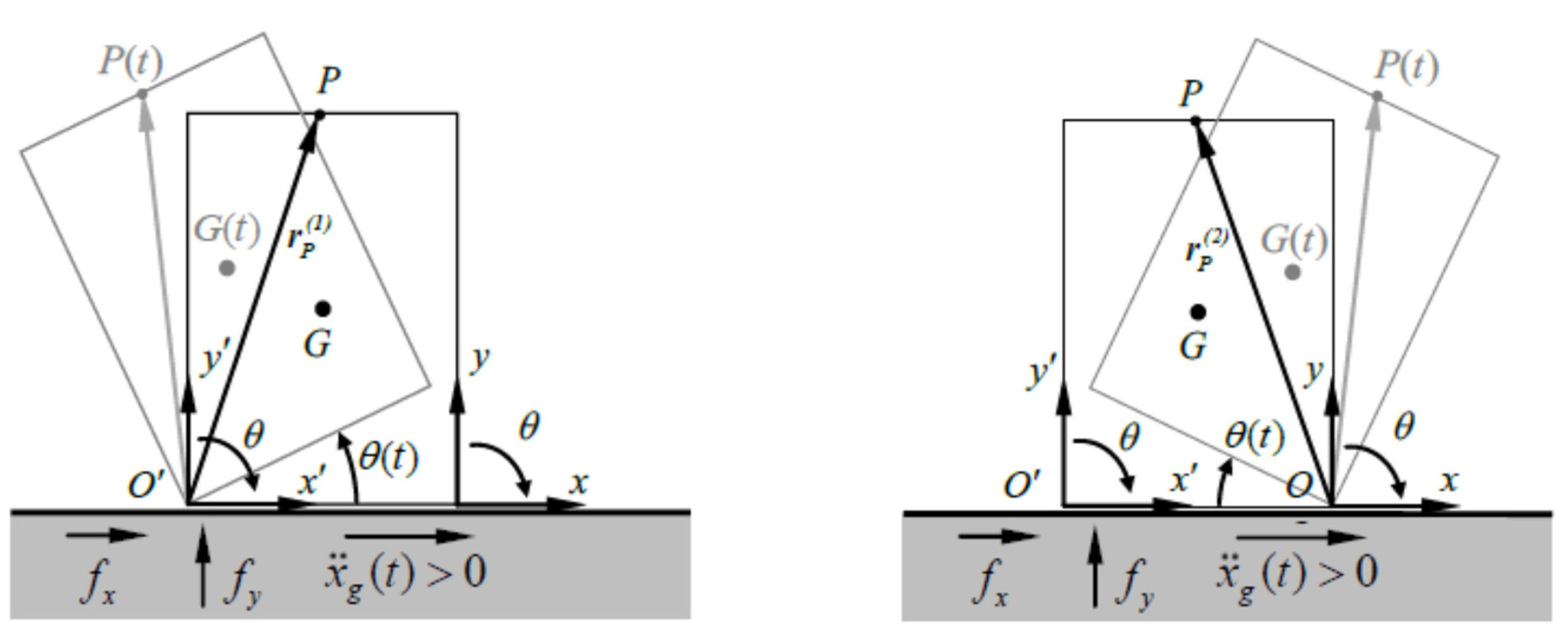
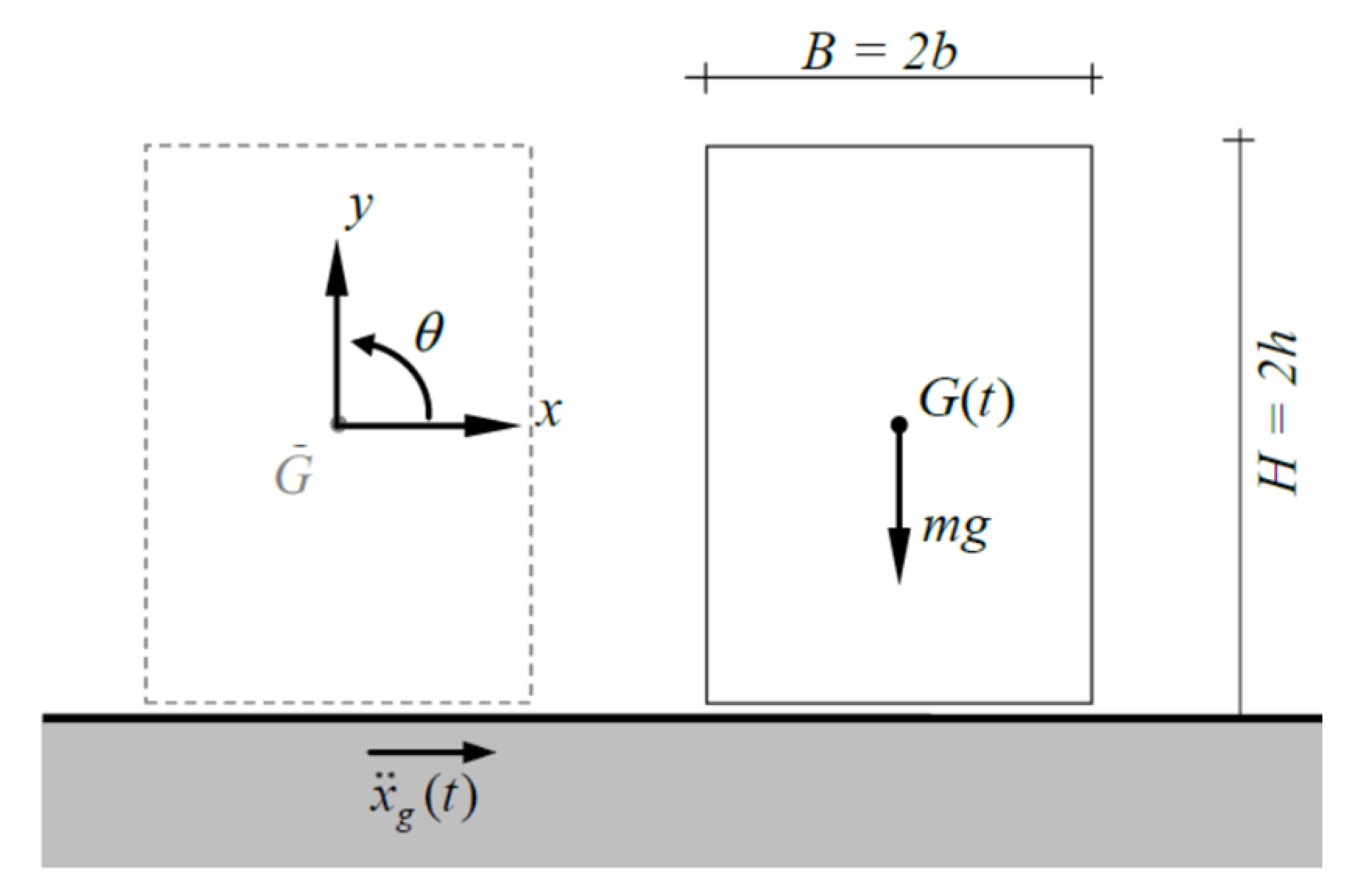
2.1. Rocking Motion of the Bell Tower
- -
- I0 is the polar inertia moment relative to O;
- -
- m is the mass of the block;
- -
- R is the distance of the centre of gravity from the rotation edge;
- -
- α = arctan (b/h) is the angle that the radius R forms with the vertical direction, depending on the block’s slenderness.
- -
- is the horizontal base acceleration;
- -
- are the polar inertia moments, with respect to the two points O and O’.
2.2. Sliding Motion of the Base Building
2.3. Combined Rocking and Sliding Motions
- -
- m1 is the mass of the bell tower
- -
- m2 is the mass of the monumental complex
- -
- M is the total mass of the complex (sum of m1 and m2)
- -
- is the signum function as defined above.
3. Application of the Proposed Method: S. Anna in Cervino Complex
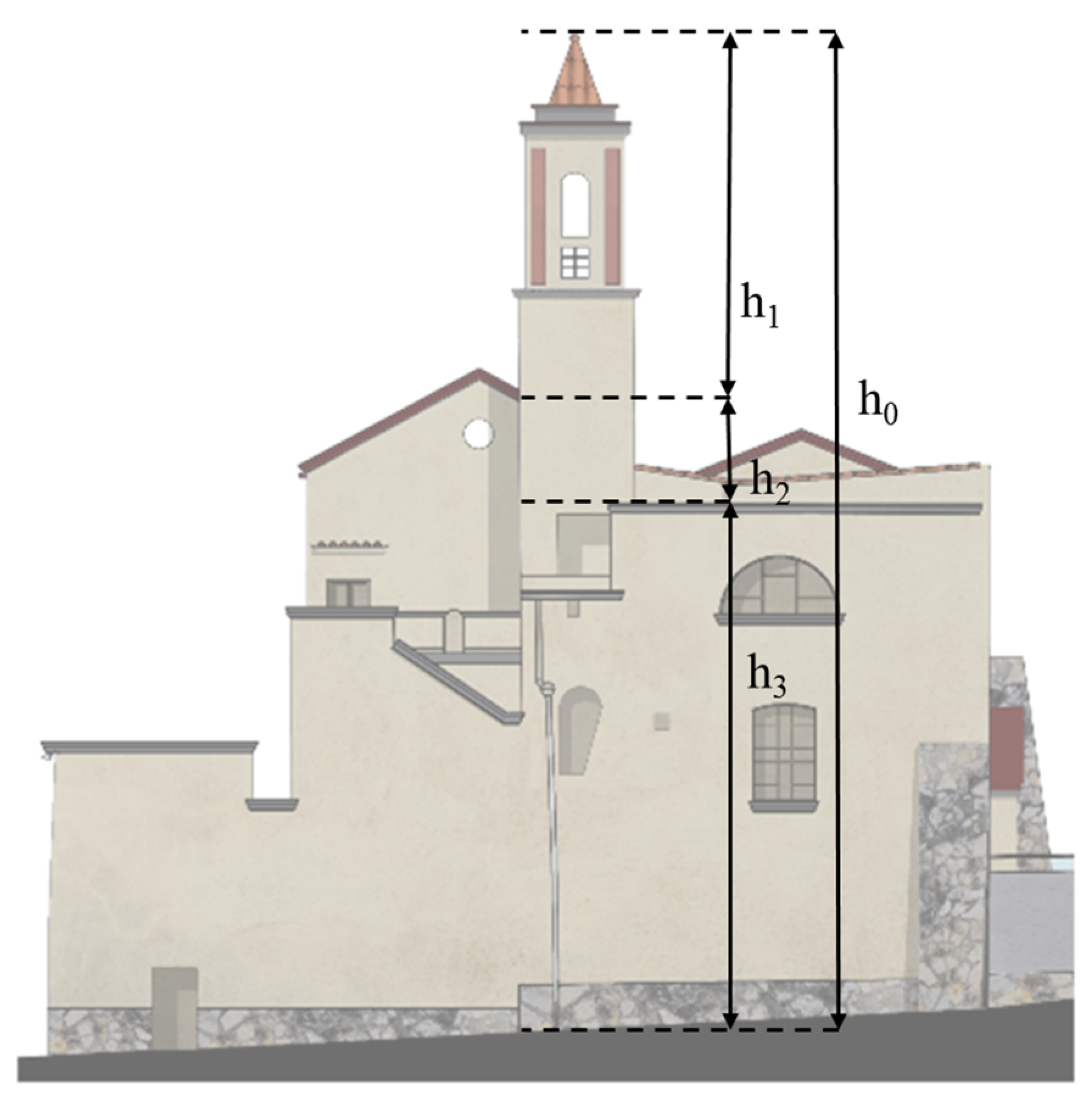
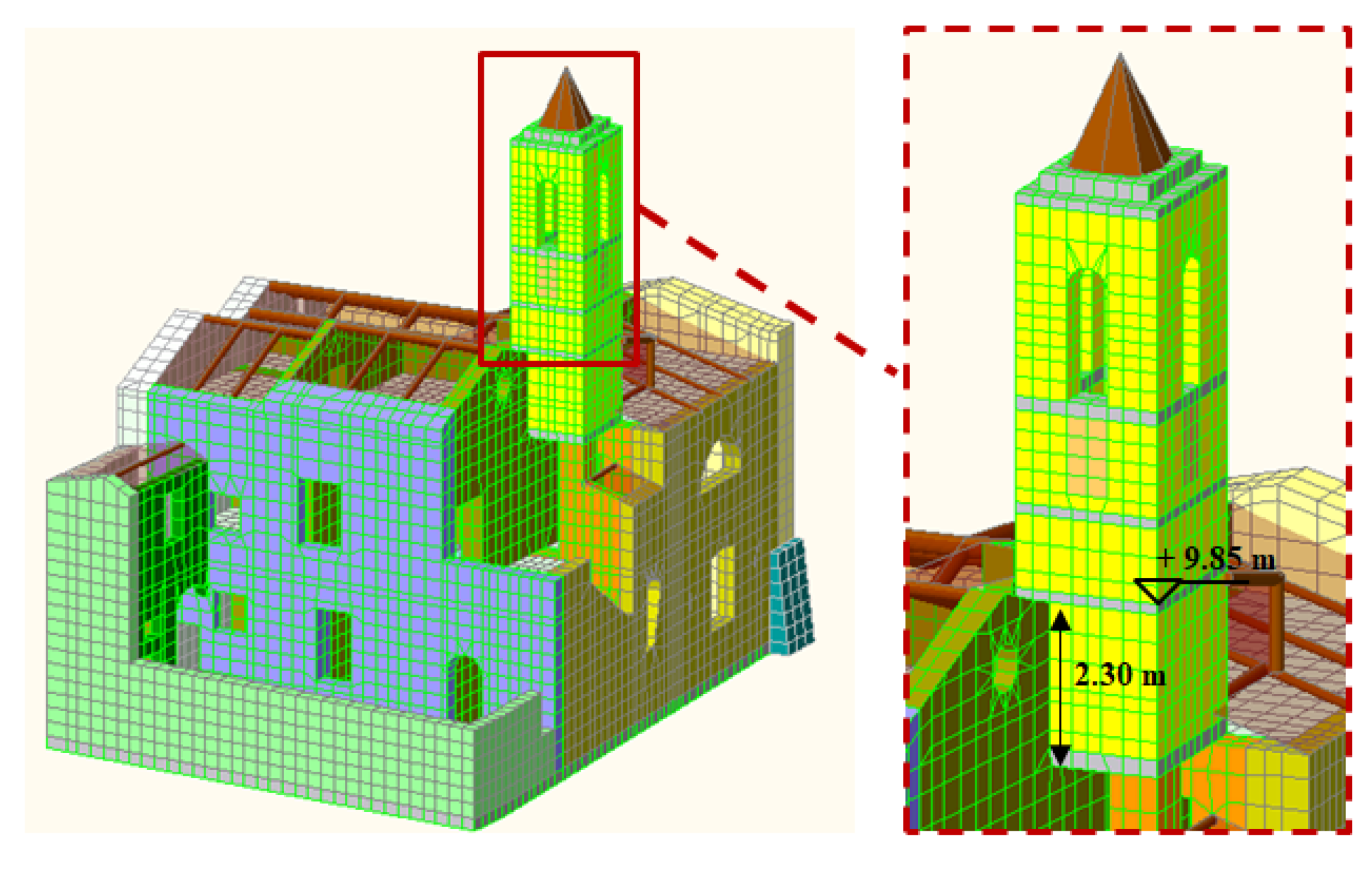
3.1. Case Study Presentation
3.2. Seismic Safety Assessment of the Bell Tower
- Type 1: oscillation of the bell tower with respect to the lower building perfectly fully supported on the moving foundation;
- Type 2: sliding of the entire masonry complex, including the bell tower rigidly connected to the lower building;
- Type 3: combined motion: the bell tower oscillates with respect to the building that undergoes relative displacements with respect to the foundation plane.
4. Results
- -
- A is the oscillation amplitude;
- -
- ω is the pulse, , with f [Hz] frequency of the excitation;
- -
- m1 = 96,840.67 kN
- -
- m2 = 339 kN
- -
- 2 h = 5.80 m
- -
- 2b = 2.06 m
- -
- r = 0.9
5. Discussion and Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Gesualdo, A.; Monaco, M. Seismic Vulnerability Reduction of Existing Masonry Buildings. Modelling of Retrofitting Techniques. In Proceedings of the COST ACTION C26: Urban Habitat Constructions under Catastrophic Events—Proceedings of the Final Conference; CRC Press: Taylor & Francis Group: London, UK, 2010. [Google Scholar]
- Gesualdo, A.; Brandonisio, G.; De-Luca, A.; Iannuzzo, A.; Montanino, A.; Olivieri, C. Limit Analysis of Cloister Vaults: The Case Study of Palazzo Caracciolo Di Avellino. J. Mech. Mater. Struct. 2019, 14, 739–750. [Google Scholar] [CrossRef]
- Iannuzzo, A.; Olivieri, C.; Fortunato, A. Displacement Capacity of Masonry Structures under Horizontal Actions via Prd Method. J. Mech. Mater. Struct. 2019, 14, 703–718. [Google Scholar] [CrossRef]
- Gesualdo, A.; Calderoni, B.; Sandoli, A.; Monaco, M. Minimum Energy Approach for the In-Plane Shear Resistance of Masonry Panels. Ing. Sismica 2019, 36, 42–53. [Google Scholar]
- Mascolo, I.; Fortunato, A.; Olivieri, C.; Gesualdo, A. Seismic Retrofitting Techniques for Existing Masonry Buildings. In Proceedings of the COMPDYN Proceedings, Athens, Greece, 28–30 June 2021; Volume 2. [Google Scholar]
- Frunzio, G.; Di Gennaro, L.; Guadagnuolo, M. Palazzo Ducale in Parete: Remarks on Code Provisions. Int. J. Mason. Res. Innov. 2019, 4, 159. [Google Scholar] [CrossRef]
- Preciado, A.; Peña, F.; Colmenero Fonseca, F.; Silva, C. Damage Description and Schematic Crack Propagation in Colonial Churches and Old Masonry Buildings by the 2017 Puebla-Morelos Earthquakes (Mw= 8.2 and 7.1). Eng. Fail. Anal. 2022, 141, 106706. [Google Scholar] [CrossRef]
- Valente, M. Seismic Vulnerability Assessment and Earthquake Response of Slender Historical Masonry Bell Towers in South-East Lombardia. Eng. Fail. Anal. 2021, 129, 105656. [Google Scholar] [CrossRef]
- Mascolo, I.; Gesualdo, A.; Olivieri, C.; Fortunato, A. On Blocks Detection in Unilateral Masonry-like Structures: A Rigid-Elastic Displacement Approach. Int. J. Mason. Res. Innov. 2022, 7, 395–405. [Google Scholar] [CrossRef]
- Minutolo, V.; Cerri, E.; Coscetta, A.; Damiano, E.; De Cristofaro, M.; Di Gennaro, L.; Esposito, L.; Ferla, P.; Mirabile, M.; Olivares, L.; et al. NSHT: New Smart Hybrid Transducer for Structural and Geotechnical Applications. Appl. Sci. 2020, 10, 4498. [Google Scholar] [CrossRef]
- Di Gennaro, L.; Damiano, E.; De Cristofaro, M.; Netti, N.; Olivares, L.; Zona, R.; Iavazzo, L.; Coscetta, A.; Mirabile, M.; Giarrusso, G.A.; et al. An Innovative Geotechnical and Structural Monitoring System Based on the Use of NSHT. Smart Mater. Struct. 2022, 31, 065022. [Google Scholar] [CrossRef]
- Montabert, A.; Mercerat, E.D.; Clément, J.; Langlaude, P.; Lyon-Caen, H.; Lancieri, M. High Resolution Operational Modal Analysis of Sant’Agata Del Mugello in Light of Its Building History. Eng. Struct. 2022, 254, 113767. [Google Scholar] [CrossRef]
- Torres, W.; Almazán, J.L.; Sandoval, C.; Boroschek, R. Operational Modal Analysis and FE Model Updating of the Metropolitan Cathedral of Santiago, Chile. Eng. Struct. 2017, 143, 169–188. [Google Scholar] [CrossRef]
- Angjeliu, G.; Coronelli, D.; Cardani, G. Development of the Simulation Model for Digital Twin Applications in Historical Masonry Buildings: The Integration between Numerical and Experimental Reality. Comput. Struct. 2020, 238, 106282. [Google Scholar] [CrossRef]
- Monaco, M.; Bergamasco, I.; Betti, M. A No-Tension Analysis for a Brick Masonry Vault with Lunette. J. Mech. Mater. Struct. 2018, 13, 703–714. [Google Scholar] [CrossRef]
- Olivieri, C.; Fortunato, A.; DeJong, M. A New Membrane Equilibrium Solution for Masonry Railway Bridges: The Case Study of Marsh Lane Bridge. Int. J. Mason. Res. Innov. 2021, 6, 446. [Google Scholar] [CrossRef]
- Montanino, A.; Olivieri, C.; Zuccaro, G.; Angelillo, M. From Stress to Shape: Equilibrium of Cloister and Cross Vaults. Appl. Sci. 2021, 11, 3846. [Google Scholar] [CrossRef]
- Angelillo, M.; Olivieri, C.; DeJong, M.J. A New Equilibrium Solut.ion for Masonry Spiral Stairs. Eng. Struct. 2021, 238, 112176. [Google Scholar] [CrossRef]
- Zizi, M.; Cacace, D.; Rouhi, J.; Lourenço, P.B.; De Matteis, G. Automatic Procedures for the Safety Assessment of Stand-Alone Masonry Arches. Int. J. Archit. Herit. 2022, 16, 1306–1324. [Google Scholar] [CrossRef]
- Zona, R.; Ferla, P.; Minutolo, V. Limit Analysis of Conical and Parabolic Domes Based on Semi-Analytical Solution. J. Build. Eng. 2021, 44, 103271. [Google Scholar] [CrossRef]
- Monaco, M.; Guadagnuolo, M. Out of Plane Behaviour of Unreinforced Masonry Walls. In Prohitech—Protection of Historical Buildings—First International Conference; CRC Press: Taylor & Francis Group: London, UK, 2009; pp. 1177–1180. ISBN 9780415558051. [Google Scholar]
- Frunzio, G.; Di Gennaro, L. The out of Plane Behaviour of Masonry Infilled Frames. J. Phys. Conf. Ser. 2021, 2090, 012148. [Google Scholar] [CrossRef]
- Gesualdo, A.; Calderoni, B.; Iannuzzo, A.; Fortunato, A.; Monaco, M. Minimum Energy Strategies for the In-Plane Behaviour of Masonry. Frat. Integrita Strutt. 2020, 14, 376–385. [Google Scholar] [CrossRef]
- Fortunato, A.; Gesualdo, A.; Mascolo, I.; Monaco, M. P-Bézier Energy Optimisation for Elastic Solutions of Masonry-like Panels. Int. J. Mason. Res. Innov. 2022, 7, 113–125. [Google Scholar] [CrossRef]
- Monaco, M.; Calderoni, B.; Iannuzzo, A.; Gesualdo, A. Behaviour of In-Plane Loaded Masonry Panels. Procedia Struct. Integr. 2018, 11, 388–393. [Google Scholar] [CrossRef]
- Zizi, M.; Chisari, C.; Rouhi, J.; De Matteis, G. Comparative Analysis on Macroscale Material Models for the Prediction of Masonry In-Plane Behavior. Bull. Earthq. Eng. 2022, 20, 963–996. [Google Scholar] [CrossRef]
- Chierchiello, G.; Gesualdo, A.; Iannuzzo, A.; Monaco, M.; Savino, M.T. Structural Modeling and Conservation of Single Columns in Archaeological Areas. In Proceedings XIV International Forum “Le vie dei Mercanti”; La Scuola di Pitagora Editrice: Napoli, Italy, 2015; pp. 2012–2020. ISBN 978-88-6542-416-2. [Google Scholar]
- Chisari, C.; Cacace, D.; De Matteis, G. A Mechanics-Based Model for Simplified Seismic Vulnerability Assessment of Masonry Bell Towers. Eng. Struct. 2022, 270, 114876. [Google Scholar] [CrossRef]
- Ceroni, F.; Casapulla, C.; Cescatti, E.; Follador, V.; Prota, A.; da Porto, F. Damage Assessment in Single-Nave Churches and Analysis of the Most Recurring Mechanisms after the 2016–2017 Central Italy Earthquakes. Bull. Earthq. Eng. 2022, 20, 8031–8059. [Google Scholar] [CrossRef]
- Ministry of Infrastructure and Transport. NTC 2018–Update of the «Technical Standards for Construction»; 2018. Available online: https://www.gazzettaufficiale.it/eli/gu/2018/02/20/42/so/8/sg/pdf (accessed on 17 January 2023).
- Guadagnuolo, M.; Nuzzo, M.; Faella, G. The Corpus Domini Bell Tower: Conservation and Safety. Procedia Struct. Integr. 2018, 11, 444–451. [Google Scholar] [CrossRef]
- Nuzzo, M.; Faella, G. The Carmine Maggiore Bell Tower: An Inclusive and Sustainable Restoration Experience. Sustainability 2021, 13, 1445. [Google Scholar] [CrossRef]
- Housner, G.W. The Behavior of Inverted Pendulum Structures during Earthquakes. Bull. Seismol. Soc. Am. 1963, 53, 403–417. [Google Scholar] [CrossRef]
- Aydin, K. The Rocking Response of an Unanchored Body Subjected to Simulated Excitation. Ph.D. Thesis, North Carolina State University, Raleigh, North Carolina, 2000. [Google Scholar]
- Gesualdo, A.; Iannuzzo, A.; Monaco, M.; Savino, M.T. Dynamic Analysis of Freestanding Rigid Blocks. Civ.-Comp Proc. 2014, 106, 1–17. [Google Scholar]
- Gesualdo, A.; Iannuzzo, A.; Monaco, M.; Penta, F. Rocking of a Rigid Block Freestanding on a Flat Pedestal. J. Zhejiang Univ. Sci. A 2018, 19, 331–345. [Google Scholar] [CrossRef]
- D’Angela, D.; Magliulo, G.; Cosenza, E. Incremental Dynamic Analysis of Rigid Blocks Subjected to Ground and Floor Motions and Shake Table Protocol Inputs. Bull. N. Z. Soc. Earthq. Eng. 2022, 55, 64–79. [Google Scholar] [CrossRef]
- D’Angela, D.; Magliulo, G.; Cosenza, E. Seismic Damage Assessment of Unanchored Nonstructural Components Taking into Account the Building Response. Struct. Saf. 2021, 93, 102126. [Google Scholar] [CrossRef]
- McCombie, P.F. Rocking of a Bell Tower—Investigation by Non-Contact Video Measurement. Eng. Struct. 2019, 193, 271–280. [Google Scholar] [CrossRef]
- Bru, D.; Ivorra, S.; Betti, M.; Adam, J.M.; Bartoli, G. Parametric Dynamic Interaction Assessment between Bells and Supporting Slender Masonry Tower. Mech. Syst. Signal Process. 2019, 129, 235–249. [Google Scholar] [CrossRef]
- Yim, C.-S.; Chopra, A.K.; Penzien, J. Rocking Response of Rigid Blocks to Earthquakes. Earthq. Eng. Struct. Dyn. 1980, 8, 565–587. [Google Scholar] [CrossRef]
- Bachmann, J.A.; Strand, M.; Vassiliou, M.F.; Broccardo, M.; Stojadinović, B. Is Rocking Motion Predictable? Earthq. Eng. Struct. Dyn. 2018, 47, 535–552. [Google Scholar] [CrossRef]
- Vassiliou, M.F.; Broccardo, M.; Cengiz, C.; Dietz, M.; Dihoru, L.; Gunay, S.; Mosalam, K.M.; Mylonakis, G.; Sextos, A.; Stojadinovic, B. Shake Table Testing of a Rocking Podium: Results of a Blind Prediction Contest. Earthq. Eng. Struct. Dyn. 2021, 50, 1043–1062. [Google Scholar] [CrossRef]
- Monaco, M.; Aurilio, M.; Tafuro, A.; Guadagnuolo, M. Sustainable Mortars for Application in the Cultural Heritage Field. Materials 2021, 14, 598. [Google Scholar] [CrossRef] [PubMed]
- Sandoli, A.; Pacella, G.; Cordasco, E.A.; Calderoni, B. PROS and CONS of Linear and Non-linear Seismic Analyses for Existing URM Structures: Application to a Historical Building. Structures 2021, 32, 532–547. [Google Scholar] [CrossRef]
- De Angelis, A.; Lourenço, P.B.; Sica, S.; Pecce, M.R. Influence of the Ground on the Structural Identification of a Bell-Tower by Ambient Vibration Testing. Soil Dyn. Earthq. Eng. 2022, 155, 107102. [Google Scholar] [CrossRef]
- De Angelis, A.; Santamato, F.; Pecce, M. Assessment of an Historical Masonry Bell Tower by Modal Testing. In Proceedings of the International Workshop on Civil Structural Health Monitoring Workshop (CSHM-8); Springer: Cham, Switzerland, 2021; pp. 219–231. [Google Scholar]
- Sandoli, A.; Lignola, G.P.; Calderoni, B.; Prota, A. Fragility Curves for Italian URM Buildings Based on a Hybrid Method. Bull. Earthq. Eng. 2021, 19, 4979–5013. [Google Scholar] [CrossRef]
- Tafuro, A. La Valutazione della Sicurezza Sismica di Complessi EcclesiasticI Il Complesso Di Sant’Anna in Cervino (CE). Ph.D. Dissertation, Università degli Studi della Campania Luigi Vanvitelli, Napoli, Italy, 2020. [Google Scholar]
- Midas Fea. Advanced Non-linear and Detail Program. Analysis and Algorithm. 1989. Available online: https://www.midasstructure.com/en/product/overview/gen (accessed on 17 January 2023).
- Ministry of Cultural Heritage and Activities. Guidelines for the Assessment and Reduction of Seismic Risk of Cultural Heritage—Alignment to New Technical Standards for Construction. Available online: https://www.soprintendenzapdve.beniculturali.it/la-soprintendenza-informa/atti-di-Address/linee-guida-per-la-valutazione-e-riduzione-del-rischio-sismico-del-patrimonio-culturale/ (accessed on 17 January 2023).
- Preciado, A.; Santos, J.C.; Silva, C.; Ramírez-Gaytán, A.; Falcon, J.M. Seismic Damage and Retrofitting Identification in Unreinforced Masonry Churches and Bell Towers by the September 19, 2017 (Mw = 7.1) Puebla-Morelos Earthquake. Eng. Fail. Anal. 2020, 118, 104924. [Google Scholar] [CrossRef]
- Bartoli, G.; Betti, M.; Monchetti, S. Seismic Risk Assessment of Historic Masonry Towers: Comparison of Four Case Studies. J. Perform. Constr. Facil. 2017, 31. [Google Scholar] [CrossRef]
- Peña, F.; Lourenço, P.B.; Mendes, N.; Oliveira, D.V. Numerical Models for the Seismic Assessment of an Old Masonry Tower. Eng. Struct. 2010, 32, 1466–1478. [Google Scholar] [CrossRef]
- Ferraioli, M.; Lavino, A.; Abruzzese, D.; Avossa, A.M. Seismic Assessment, Repair and Strengthening of a Medieval Masonry Tower in Southern Italy. Int. J. Civ. Eng. 2020, 18, 967–994. [Google Scholar] [CrossRef]
- Guadagnuolo, M.; Aurilio, M.; Tafuro, A.; Faella, G. Analysis of Local Mechanisms through Floor Spectra for the Preservation of Historical Masonries. A Case Study. In Proceedings of the COMPDYN Proceedings, Crete, Greece, 24–26 June 2019; Volume 1, pp. 1501–1513. [Google Scholar]
- Guadagnuolo, M.; Aurilio, M.; Nuzzo, M.; Faella, G. Historic Chimney Stacks: Seismic Assessment and Kinematic Analysis. J. Archit. Eng. 2023, 29, 04022037. [Google Scholar] [CrossRef]


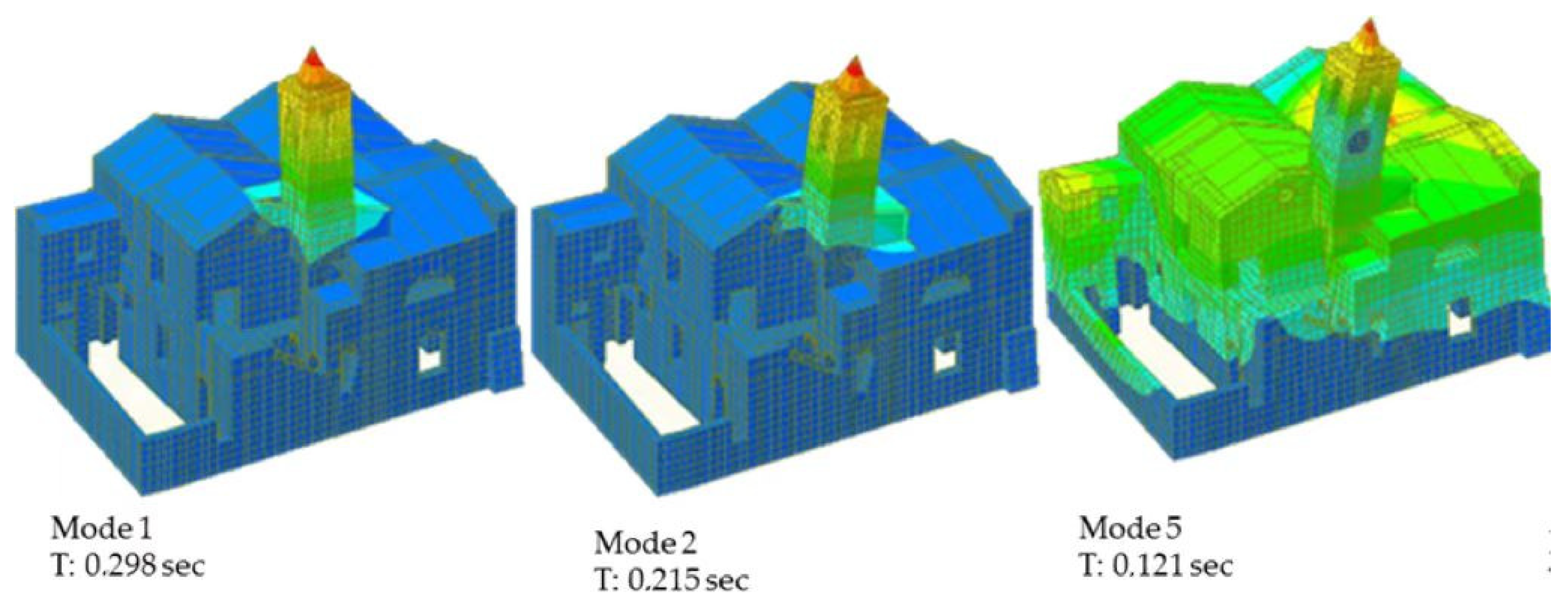

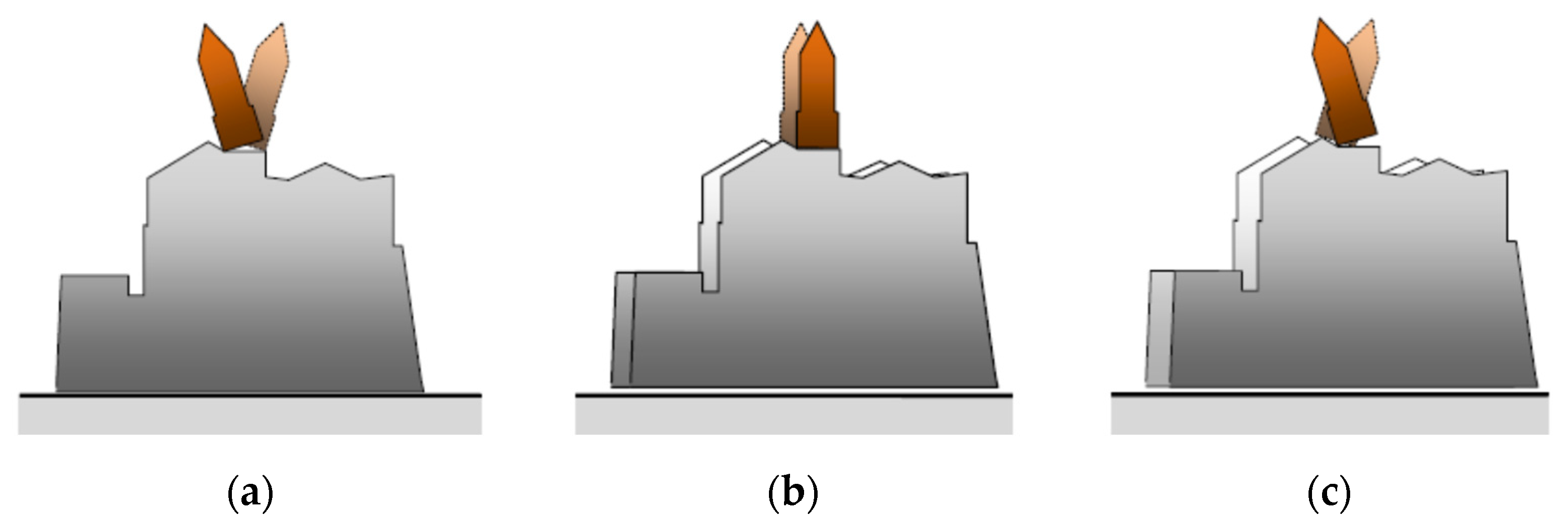
| Type of Model | Description | Parameters |
|---|---|---|
| Complex | FEM-Global |
|
| Complex | FEM-Bell Tower |
|
| Simplified | Single-Block Model |
|
| Simplified | Double-Block Model (proposed method) |
|
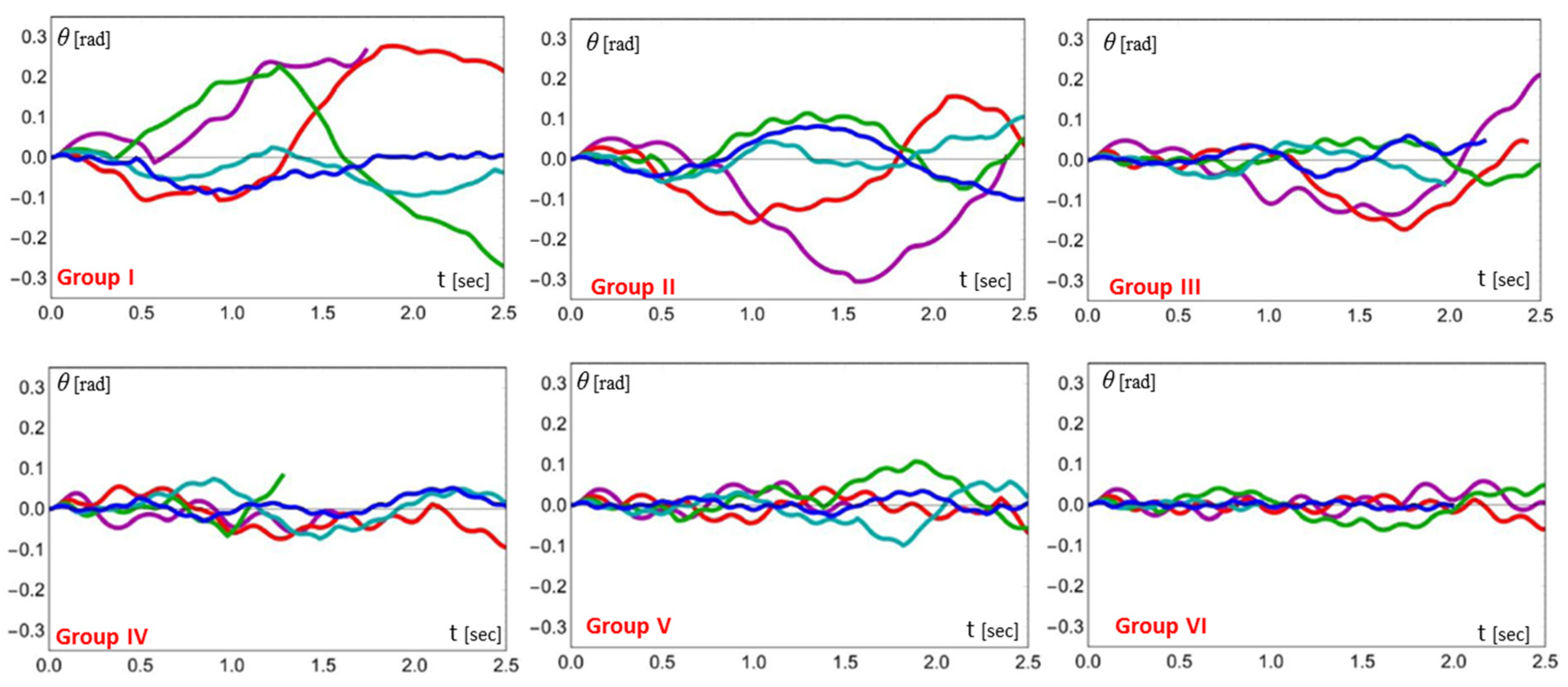
| Group | Combination | μs | μk | f [Hz] | Color |
|---|---|---|---|---|---|
| I | 1 2 3 4 5 | 1 | 0.7 | 3 4 5 6 7 | Purple Red Green Light blue Blue |
| II | 6 7 8 9 10 | 1.25 | 1 | 3 4 5 6 7 | Purple Red Green Light blue Blue |
| III | 11 12 13 14 15 | 2 | 1.5 | 3 4 5 6 7 | Purple Red Green Light blue Blue |
| IV | 16 17 18 19 20 | 2.8 | 2 | 3 4 5 6 7 | Purple Red Green Light blue Blue |
| V | 21 22 23 24 25 | 3.2 | 2 | 3 4 5 6 7 | Purple Red Green Light blue Blue |
| VI | 26 27 28 29 30 | 3.6 | 2.2 | 3 4 5 6 7 | Purple Red Green Light blue Blue |
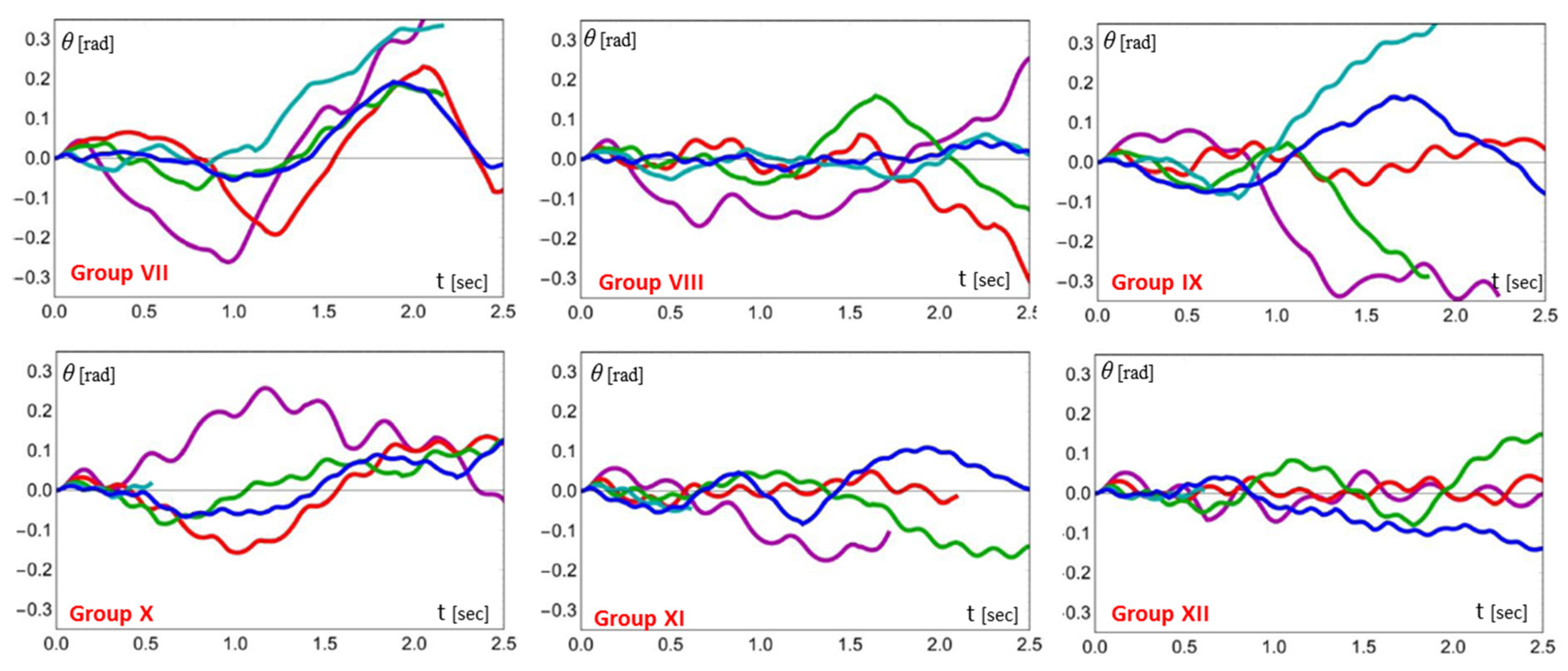
| Group | Combination | μs | μk | f [Hz] | Color |
|---|---|---|---|---|---|
| VII | 31 32 33 34 35 | 1 | 0.7 | 3 4 5 6 7 | Purple Red Green Light blue Blue |
| VIII | 36 37 38 39 40 | 1.25 | 1 | 3 4 5 6 7 | Purple Red Green Light blue Blue |
| IX | 41 42 43 44 45 | 2 | 1.5 | 3 4 5 6 7 | Purple Red Green Light blue Blue |
| X | 46 47 48 49 50 | 2.8 | 2 | 3 4 5 6 7 | Purple Red Green Light blue Blue |
| XI | 51 52 53 54 55 | 3.2 | 2 | 3 4 5 6 7 | Purple Red Green Light blue Blue |
| XII | 56 57 58 59 60 | 3.6 | 2.2 | 3 4 5 6 7 | Purple Red Green Light blue Blue |
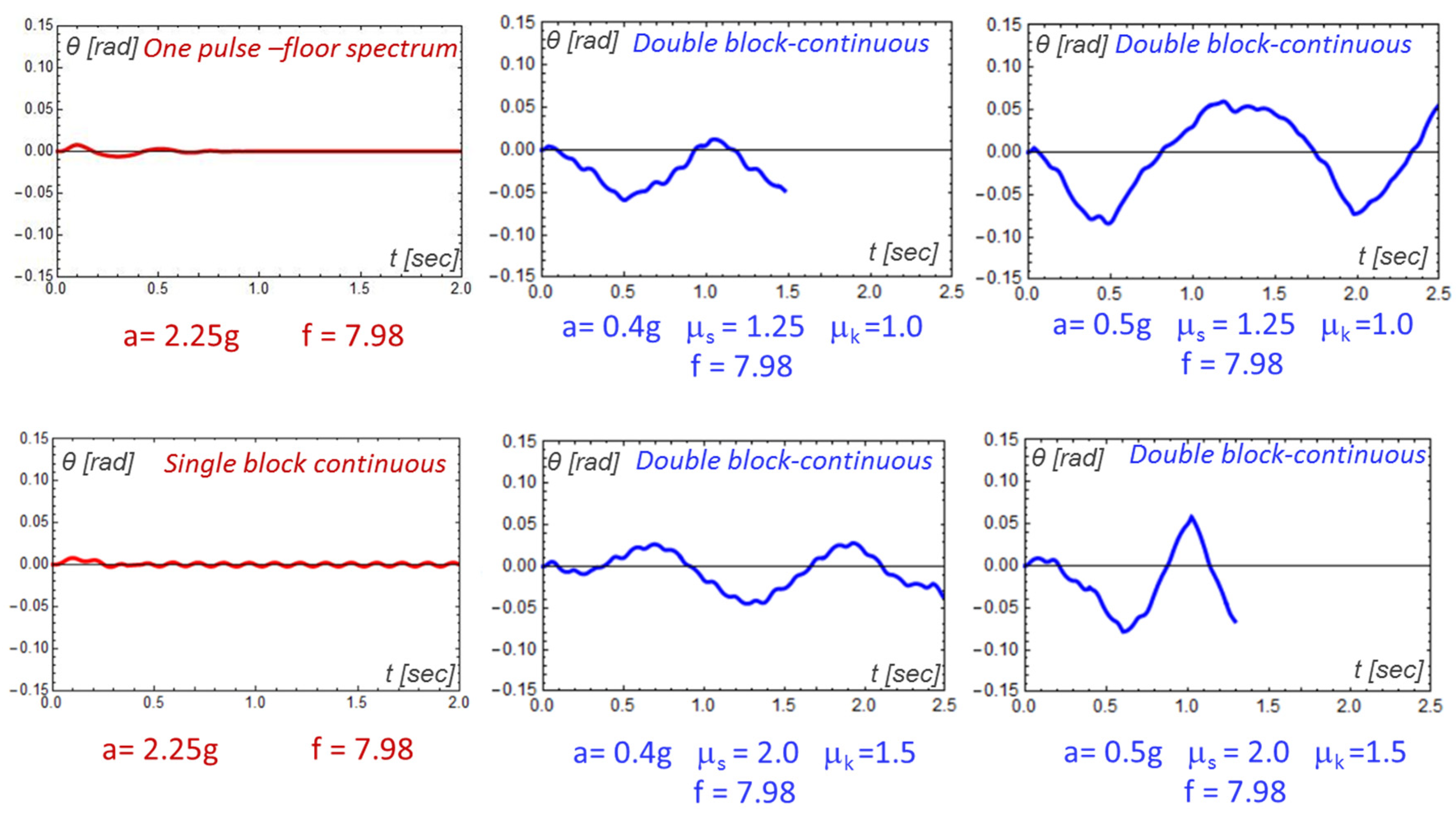
| Mechanical Model | Type of Motion | Amplitude | Frequency | µs | µk |
|---|---|---|---|---|---|
| Single block | One sine | 2.25 g | 7.98 Hz | - | - |
| Double block | Continuous sine | 0.4 g | 7.98 Hz | 1.25 | 1.0 |
| Continuous sine | 0.4 g | 7.98 Hz | 2.0 | 1.5 | |
| Continuous sine | 0.5 g | 7.98 Hz | 1.25 | 1.0 | |
| Continuous sine | 0.5 g | 7.98 Hz | 2.0 | 1.5 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Di Gennaro, L.; Guadagnuolo, M.; Monaco, M. Rocking Analysis of Towers Subjected to Horizontal Forces. Buildings 2023, 13, 762. https://doi.org/10.3390/buildings13030762
Di Gennaro L, Guadagnuolo M, Monaco M. Rocking Analysis of Towers Subjected to Horizontal Forces. Buildings. 2023; 13(3):762. https://doi.org/10.3390/buildings13030762
Chicago/Turabian StyleDi Gennaro, Luciana, Mariateresa Guadagnuolo, and Michela Monaco. 2023. "Rocking Analysis of Towers Subjected to Horizontal Forces" Buildings 13, no. 3: 762. https://doi.org/10.3390/buildings13030762
APA StyleDi Gennaro, L., Guadagnuolo, M., & Monaco, M. (2023). Rocking Analysis of Towers Subjected to Horizontal Forces. Buildings, 13(3), 762. https://doi.org/10.3390/buildings13030762








