Innovative Seismic Strengthening Techniques to Be Used in RC Beams’ Critical Zones
Abstract
1. Introduction
2. Significant Research Studies
3. Experimental Program
3.1. Specimens’ Description
3.2. Strengthened Specimens’ Description
3.3. Test Setup
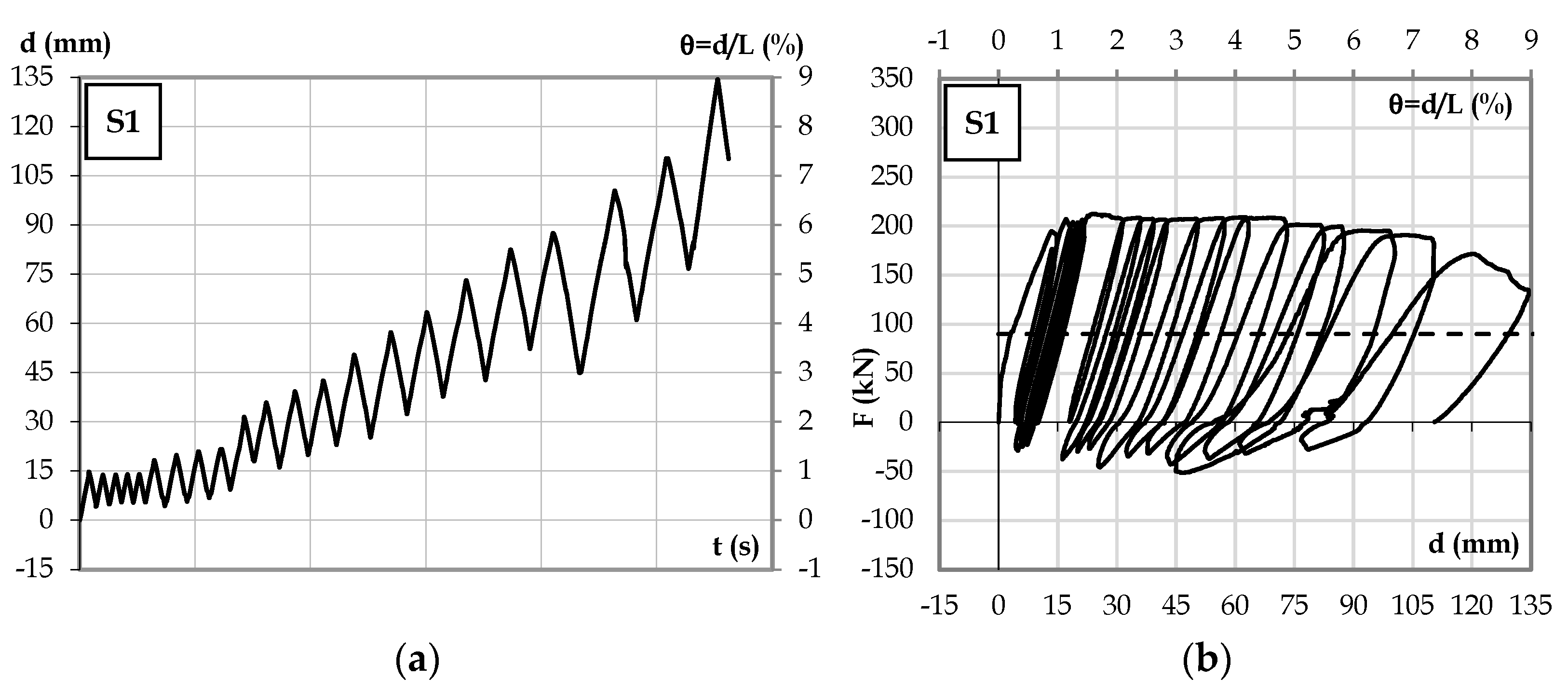
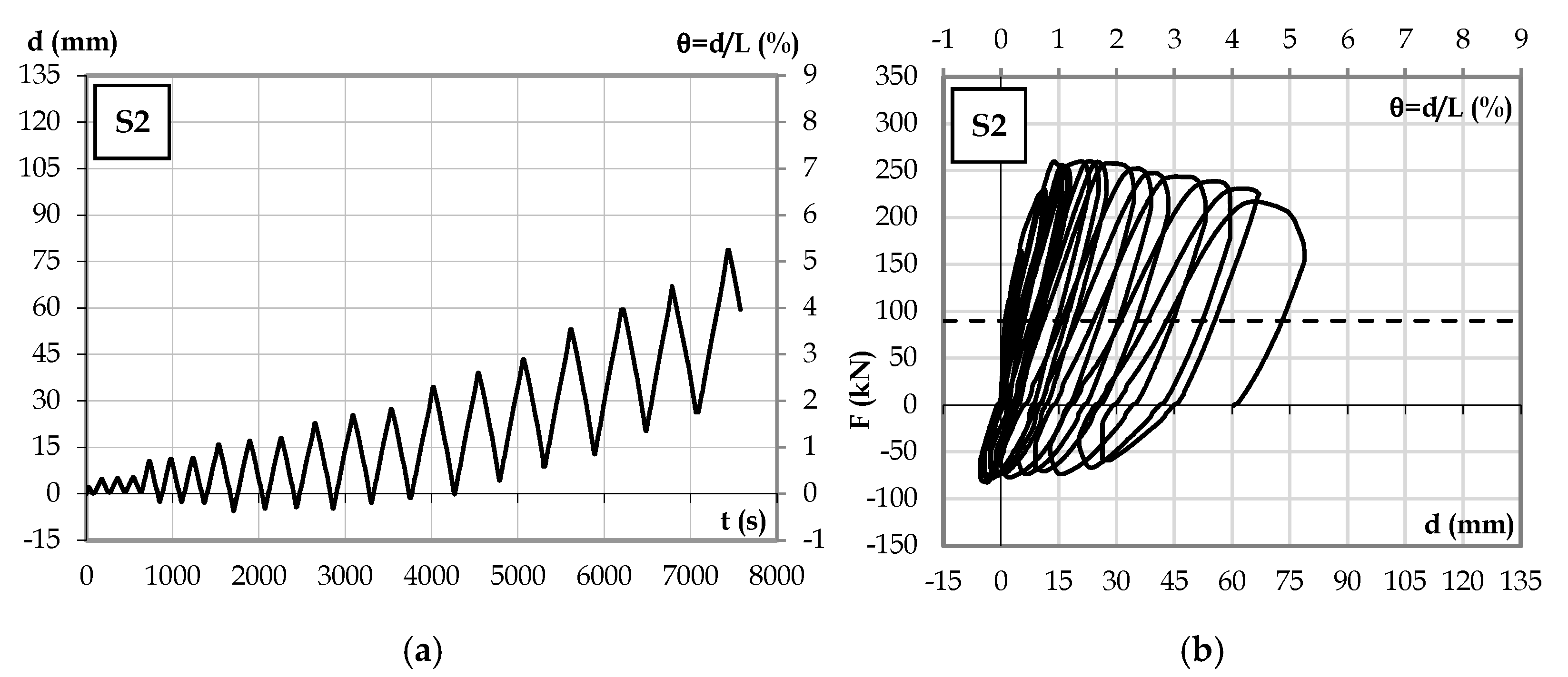
3.4. Displacement History
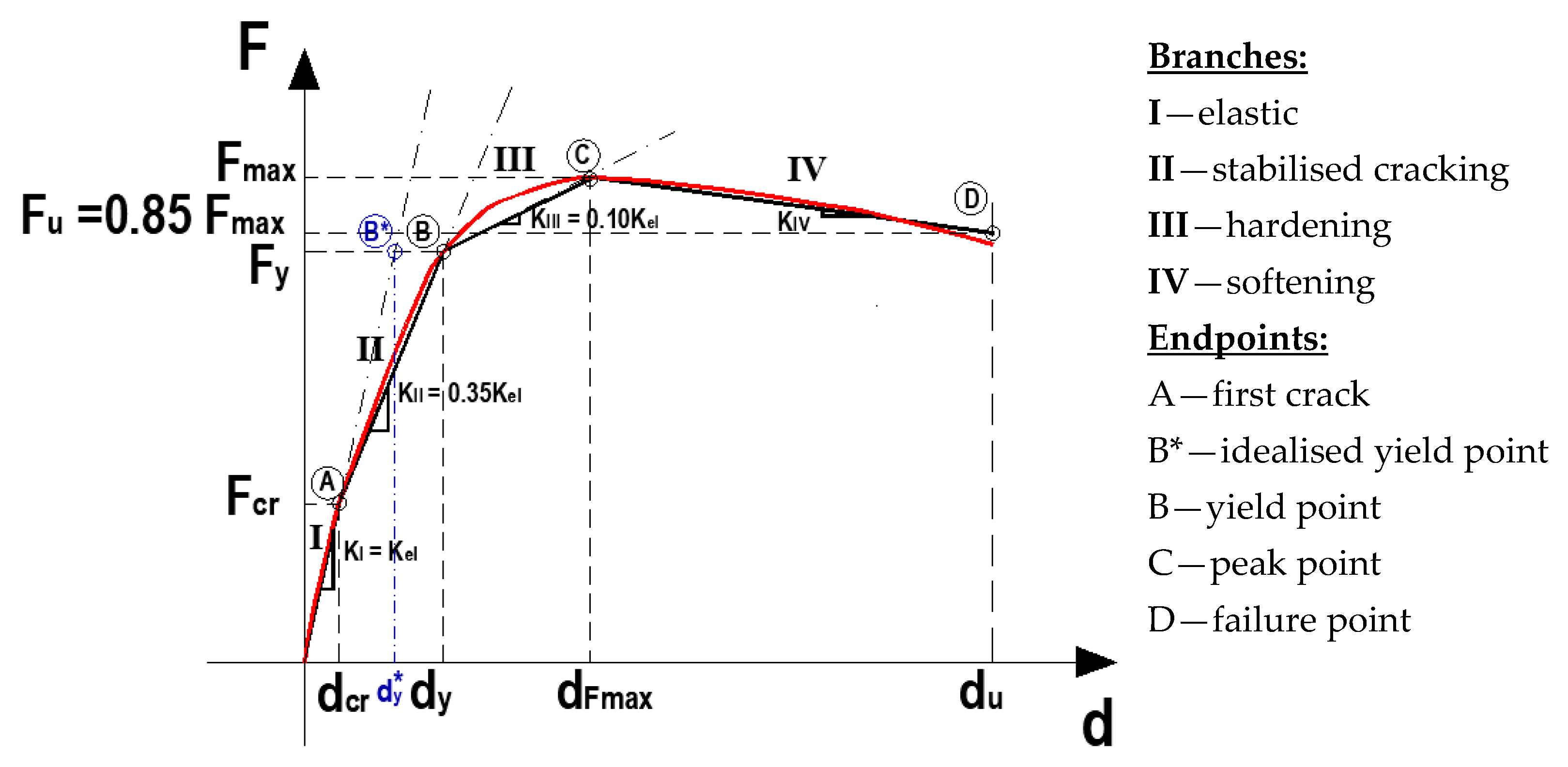
4. Test Results
4.1. Failure Mechanisms
4.2. Experimental Results
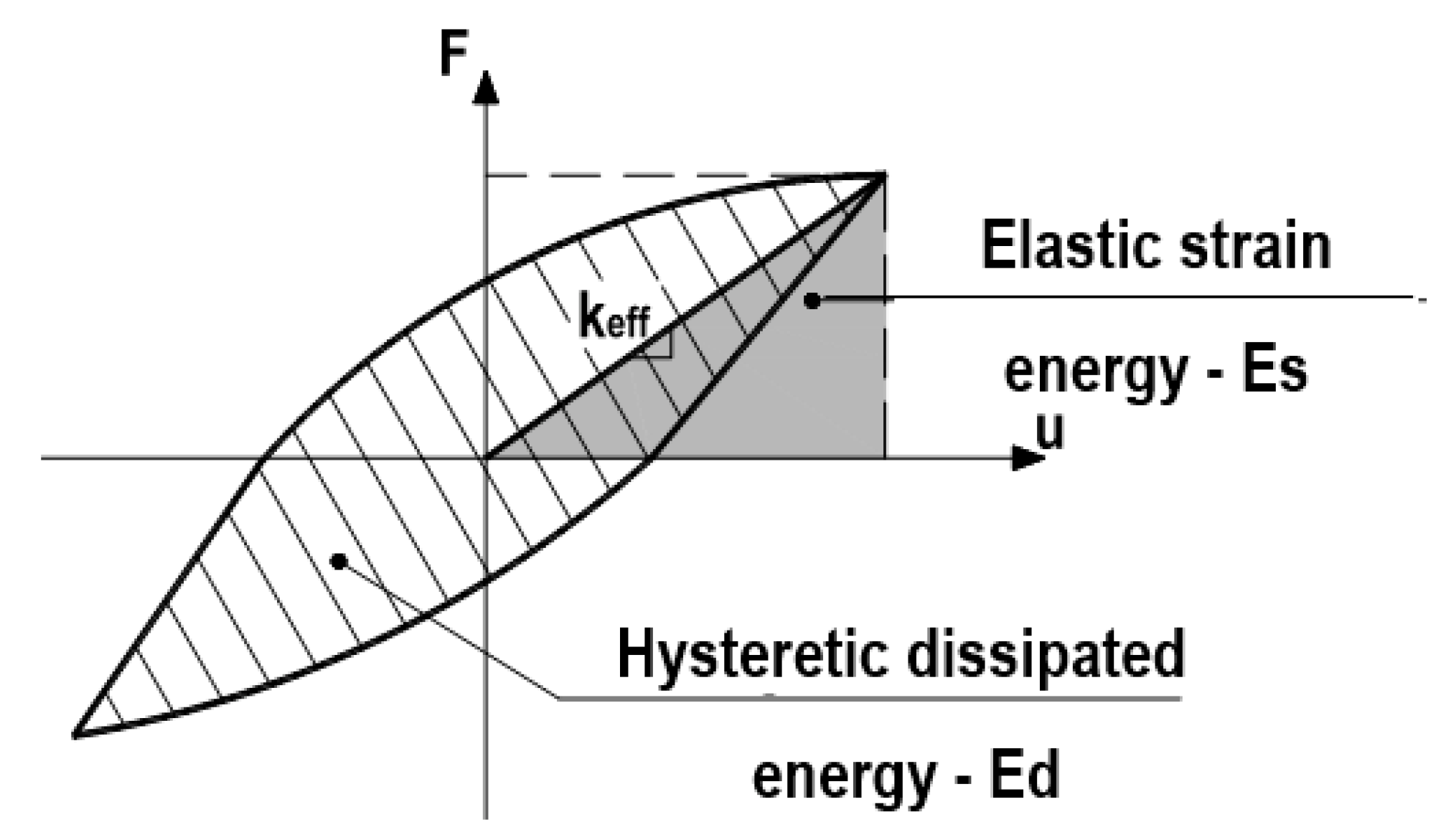
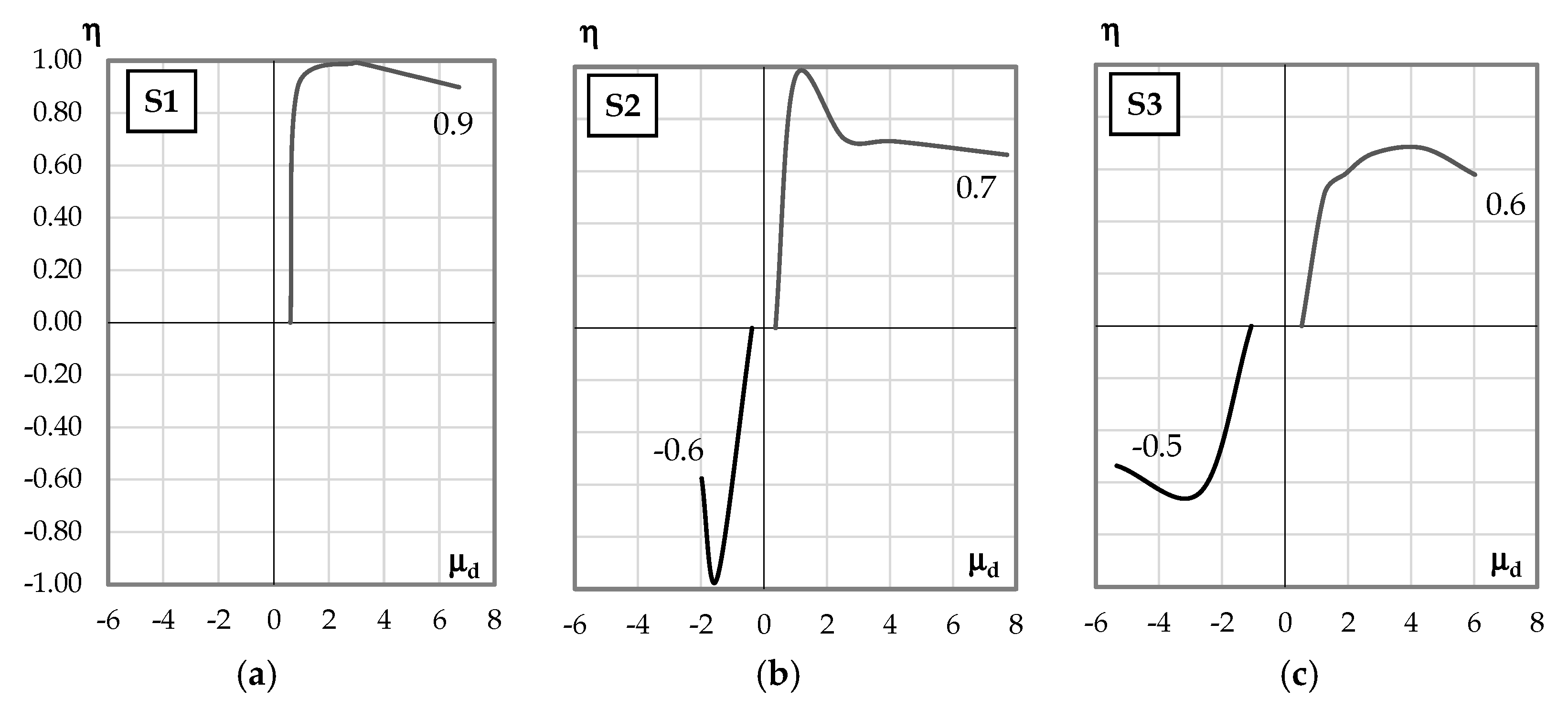
4.3. Performance Evaluation

5. Theoretical Prediction
5.1. Determination of the Yield Bending Capacity
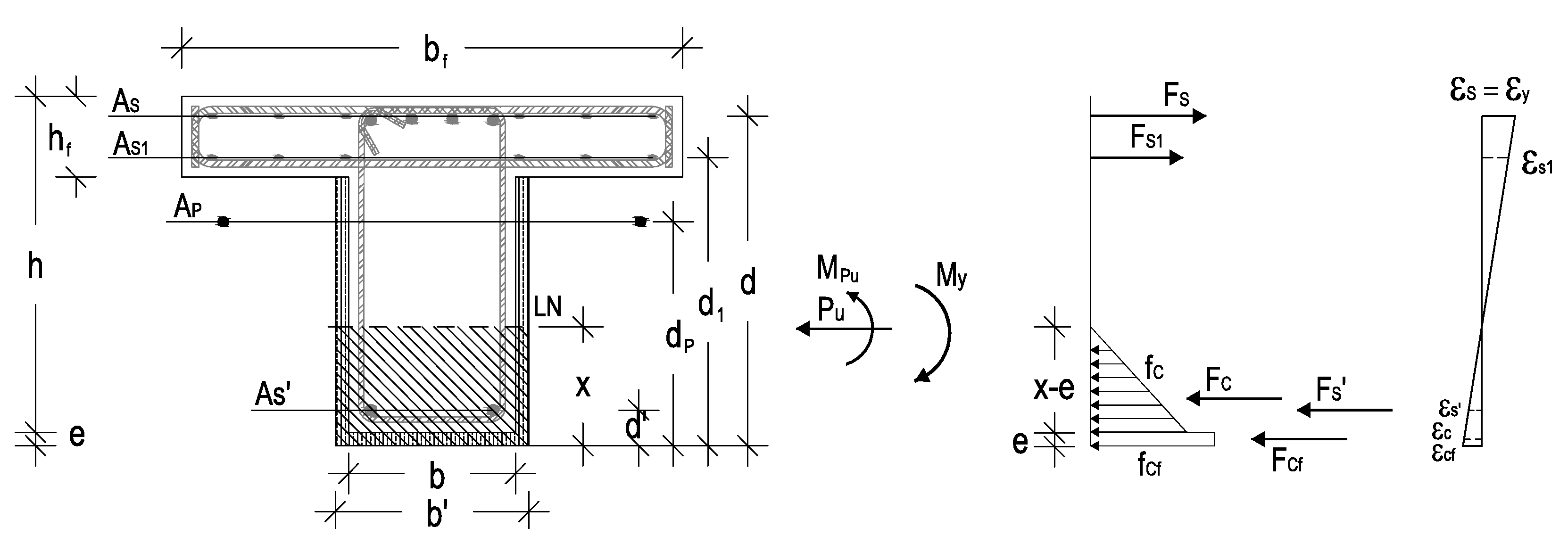
5.2. Determination of the Peak Bending Moment Capacity
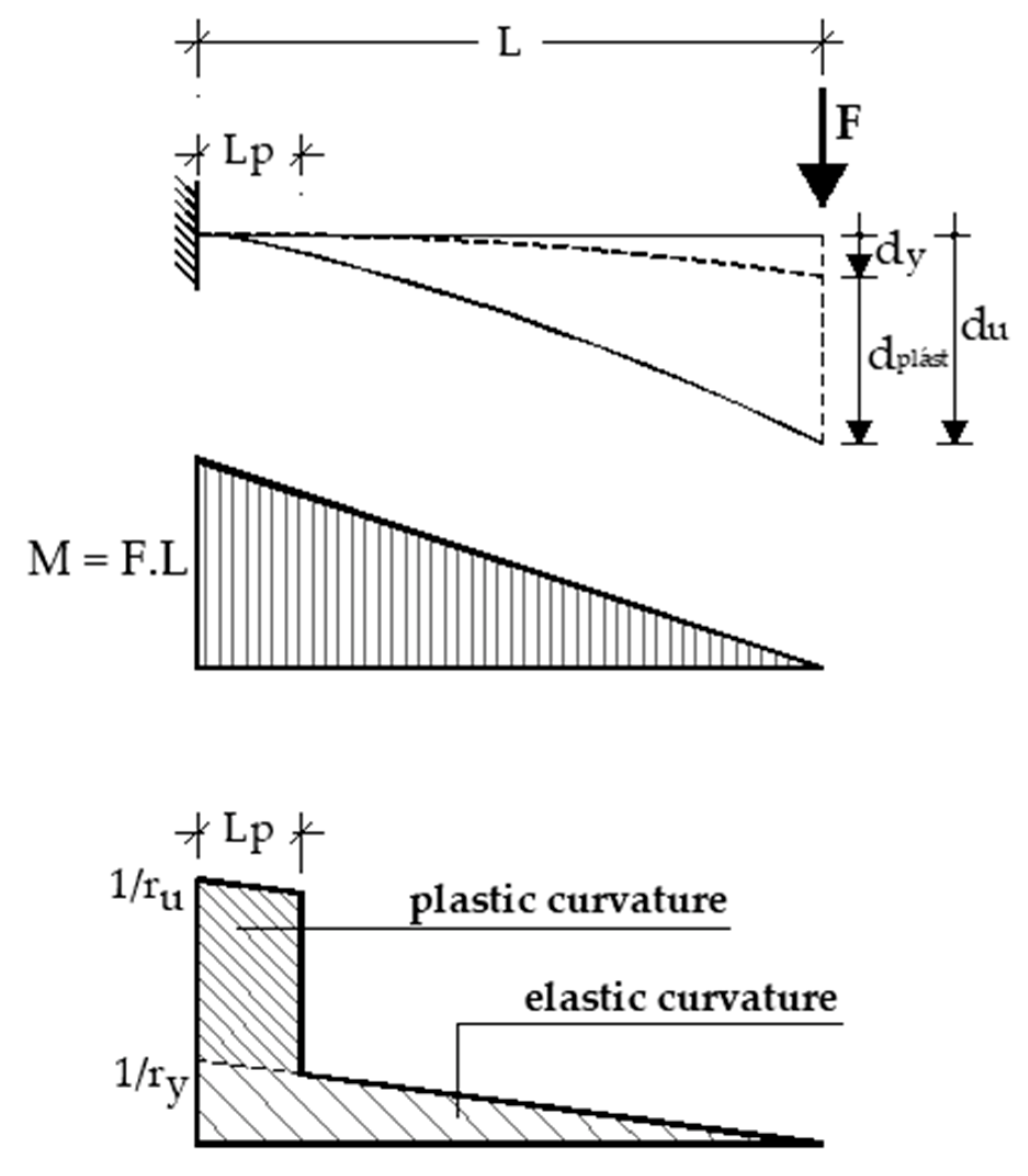
5.3. Determination of the Deformation Capacity
5.4. Proposed Multilinear Model
6. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Comité Européen du Béton-Fédération Internationale du Béton (CEB-FIB). Seismic Assessment and Retrofit of Reinforced Concrete Buildings, CEB-FIB Bulletin No. 24; State-of-art Report, Task Group 7.1: 2003; International Federation for Structural Concrete (fib): Lausanne, Switzerland, 2003. [Google Scholar]
- CEN EN 1998-3; Eurocode 8: Design of Structures for Earthquake Resistance—Part 3: Assessment and Retrofitting of Buildings. CEN: Brussels, Belgium, 2005.
- CEB Bulletin D’Information Nº. 220. Behaviour and Analysis of Reinforced Concrete Structures under Alternate Actions Inducing Inelastic Response; Volume 2: Frame Members; Comité Euro-Internacional du Béton (CEB): Lausanne, Switzerland, 1994.
- CEN EN 1998-1; Design of Structures for Earthquake Resistance—Part 1: General Rules, Seismic Actions and Rules for Buildings. CEN: Brussels, Belgium, 2004.
- Bracci, J.M.; Reinhorn, A.M.; Mander, J.B. Seismic Resistance of Reinforced Concrete Frame Structures Designed for Gravity Loads: Performance of Structural System. ACI Struct. J. 1995, 92, 597–609. [Google Scholar]
- El-Attar, A.G.; White, R.N.; Gergely, P. Behavior of Gravity Load Designed Reinforced Concrete Buildings Subjected to Earthquakes. ACI Struct. J. 1997, 94, 133–145. [Google Scholar]
- Calvi, G.M.; Magenes, G.; Pampanin, S. Experimental test on a three storey RC frame designed for gravity only. In Proceedings of the 12th European Conference of Earthquake Engineering, London, UK, 9–13 September 2002; p. 727. [Google Scholar]
- ECCS. Recommended Testing Procedure for Assessing the Behaviour of Structural Steel Elements under Cyclic Loads. Technical Working Group 1.3—Seismic Design, Nº 45; European Convention for Constructional Steelwork (ECCS): Brussels, Belgium, 1985. [Google Scholar]
- ACI T1.1-01; Acceptance Criteria for Moment Frames Based on Structural Testing. American Concrete Institute (ACI): Farmington Hills, MI, USA, 2001.
- Applied Technology Council. Guidelines for Seismic Testing of Components of Steel Structures; ATC Report Nº 24; Applied Technology Council: Redwood City, CA, USA, 1992. [Google Scholar]
- Gião, A.R.; Lúcio, V.; Chastre, C. Assessing the Behaviour of RC Beams subject to significant gravity loads under cyclic loads. Eng. Struct. 2014, 59, 512–521. [Google Scholar] [CrossRef]
- Priestley, M.J.N. Overview of the PRESSS Research Programme. PCI J. 1991, 36, 50–57. [Google Scholar] [CrossRef]
- Priestley, M.J.N. The PRESSS Program—Current Status and Proposed Plans for Phase III. PCI J. 1996, 41, 22–40. [Google Scholar] [CrossRef]
- Priestley, M.N.; Sritharan, S.; Conley, J.R.; Pampanin, S. Preliminary Results and Conclusions from the PRESSS Five Story Precast Concrete Test Building. PCI J. 1999, 44, 42–67. [Google Scholar] [CrossRef]
- Conley, J.; Sritharan, S.; Priestley, M.J.N. Precast seismic structural systems PRESSS-3: The Five-Story Precast Test Building. Vol. 3–5: Wall Direction Response. Final Report Submitted to the Precast/Prestressed Concrete Institute Department of Structural Engineering University of California, San Diego, La Jolla, California. 92093-0085. 2002. [Google Scholar]
- Nakaki, S.D.; Englekirk, R.E. PRESSS Industry Seismic Workshops: Concept Development. PCI J. 2014, 36, 54–61. [Google Scholar] [CrossRef]
- Pinho, R.; Elnashai, A.S. Repair and retrofitting of RC walls using selective techniques. J. Earthq. Eng. 1998, 2, 525–568. [Google Scholar]
- Pampanin, S. Controversial Aspects in Seismic Assessment and Retrofit of Structures in Modern Times: Understanding and Implementing Lessons from Ancient Heritage. Bull. New Zealand Soc. Earthq. Eng. 2006, 39, 120–133. [Google Scholar] [CrossRef][Green Version]
- Ireland, M.G.; Pampanin, S.; Bull, D.K. Concept and Implementation of a Selective Weakening Approach for the Seismic Retrofit of R.C. Buildings; University of Canterbury, Civil Engineering: Christchurch, New Zealand, 2006; Volume 1, pp. 1–9. [Google Scholar]
- Pampanin, S. Emerging Solutions for High Seismic Performance of Precast Prestressed Concrete Buildings. J. Adv. Concr. 2005, 3, 207–223. [Google Scholar] [CrossRef]
- Kam, W.Y.; Pampanin, S.; Bull, D. Selective weakening retrofit for existing R.C. structures—Concept, validation and design example. In Proceedings of the 9th US National and 10th Canadian Conference on Earthquake Engineering: Reaching Beyond Borders, Toronto, ON, Canada, 25–29 July 2010; pp. 25–29. [Google Scholar]
- Kam, W.Y.; Pampanin, S. The seismic performance of RC buildings in the 22 February 2011 Christchurch earthquake. Struct. Concr. 2011, 12, 223–233. [Google Scholar] [CrossRef]
- Kam, W.Y.; Pampanin, S.; Elwood, K. Seismic performance of reinforced concrete buildings in the 22 February Christchurch (Lyttelton) earthquake. Bull. New Zealand Soc. Earthq. Eng. 2011, 44, 239–278. [Google Scholar] [CrossRef]
- Leon, R.T.; Kam, W.Y.; Pampanin, S. Performance of beam-column joints in 314 the 2010–2012 Christchurch earthquakes, Special Publication. Am. Concr. Inst. 2012, 296, 47–66. [Google Scholar]
- Dogan, E.; Krstulovic-Opara, N. Seismic retrofit with continuous slurry-infiltrated mat concrete jackets. ACI Struct. J. 2003, 100, 713–722. [Google Scholar]
- Fischer, G.; Li, V.C. Intrinsic Response Control of Moment-Resisting Frames Utilizing Advanced Composite Materials and Structural Elements. ACI Struct. J. 2003, 100, 166–176. [Google Scholar]
- Parra-Montesinos, G. High Performance Fibre Reinforced Cement Composites: An Alternative for Seismic Design of Structures. ACI Struct. J. 2005, 102, 668–675. [Google Scholar]
- Shannag, M.J.; Alhassan, M.A. Seismic Upgrade of Interior Beam-Column Subassemblages with High-Performance Fibre-Reinforced Concrete Jackets. ACI Struct. J. 2005, 102, 130–138. [Google Scholar]
- Shang, X.-Y.; Yu, J.-T.; Li, L.-Z.; Lu, Z.-D. Strengthening of RC Structures by Using Engineered Cementitious Composites: A Review. Sustainability 2019, 11, 3384. [Google Scholar] [CrossRef]
- Konstantinos, K.; Manos, G.; Papakonstantinou, C. Seismic Retrofit of R/C T-Beams with Steel Fiber Polymers under Cyclic Loading Conditions. Buildings 2019, 9, 101. [Google Scholar] [CrossRef]
- Chalioris, C.E.; Kosmidou, P.-M.K.; Karayannis, C.G. Cyclic Response of Steel Fiber Reinforced Concrete Slender Beams: An Experimental Study. Materials 2019, 12, 1398. [Google Scholar] [CrossRef]
- Chalioris, C.E.; Zapris, A.G.; Karayannis, C.G. U-Jacketing Applications of Fiber-Reinforced Polymers in Reinforced Concrete T-Beams against Shear—Tests and Design. Fibers 2020, 8, 13. [Google Scholar] [CrossRef]
- Kalogeropoulos, G.; Tsonos, A.-D. Seismic Performance Enhancement of RC Columns Using Thin High-Strength RC Jackets and CFRP Jackets. Fibers 2021, 9, 29. [Google Scholar] [CrossRef]
- Bencardino, F.; Nisticò, M. Evaluation of the Maximum Strain for Different Steel-FRCM Systems in RC Beams Strengthened in Flexure. Fibers 2022, 10, 67. [Google Scholar] [CrossRef]
- Fares, S.; Fugger, R.; De Santis, S.; de Felice, G. Strength, bond and durability of stainless-steel reinforced grout. Constr. Build. Mater. 2022, 322, 126465. [Google Scholar] [CrossRef]
- Muhaj, H. Seismic Strengthening of Reinforced Concrete Beams by Post-Tensioning with Anchorages by Bonding. Ph.D Thesis, Universidade Nova de Lisboa, Lisbon, Portugal, 2020. [Google Scholar]
- Gião, R.; Lúcio, V.; Chastre, C.; Brás, A. UFRG—Unidirectional Fibre Reinforced Grout as strengthening material for reinforced concrete structures. In Proceedings of the 8th RILEM International Symposium on Fibre Reinforced Concrete: Challenges and Opportunities-BEFIB, Guimarães, Portugal, 19–21 September 2012. [Google Scholar]
- Gião, A.R.; Lúcio, V.; Chastre, C. Characterisation of unidirectional fibre reinforced grout as a strengthening material for RC structures. Constr. Build. Mater. 2017, 137, 272–287. [Google Scholar] [CrossRef]
- Park, Y.J.; Ang, A.H. Mechanistic seismic damage model for reinforced concrete. J. Struct. Eng. ASCE 1985, 111, 722–739. [Google Scholar] [CrossRef]
- Cosenza, E.; Manfredi, G.; Ramasco, R. The Use of Damage Functionals in Earthquake-Resistant Design: A Comparison Among Different Procedures. Earthq. Eng. Struct. Dyn. 1993, 22, 855–868. [Google Scholar] [CrossRef]
- Park, R.; Paulay, T. Reinforced Concrete Structures; John Wiley & Sons: Hoboken, NJ, USA, 1975. [Google Scholar]
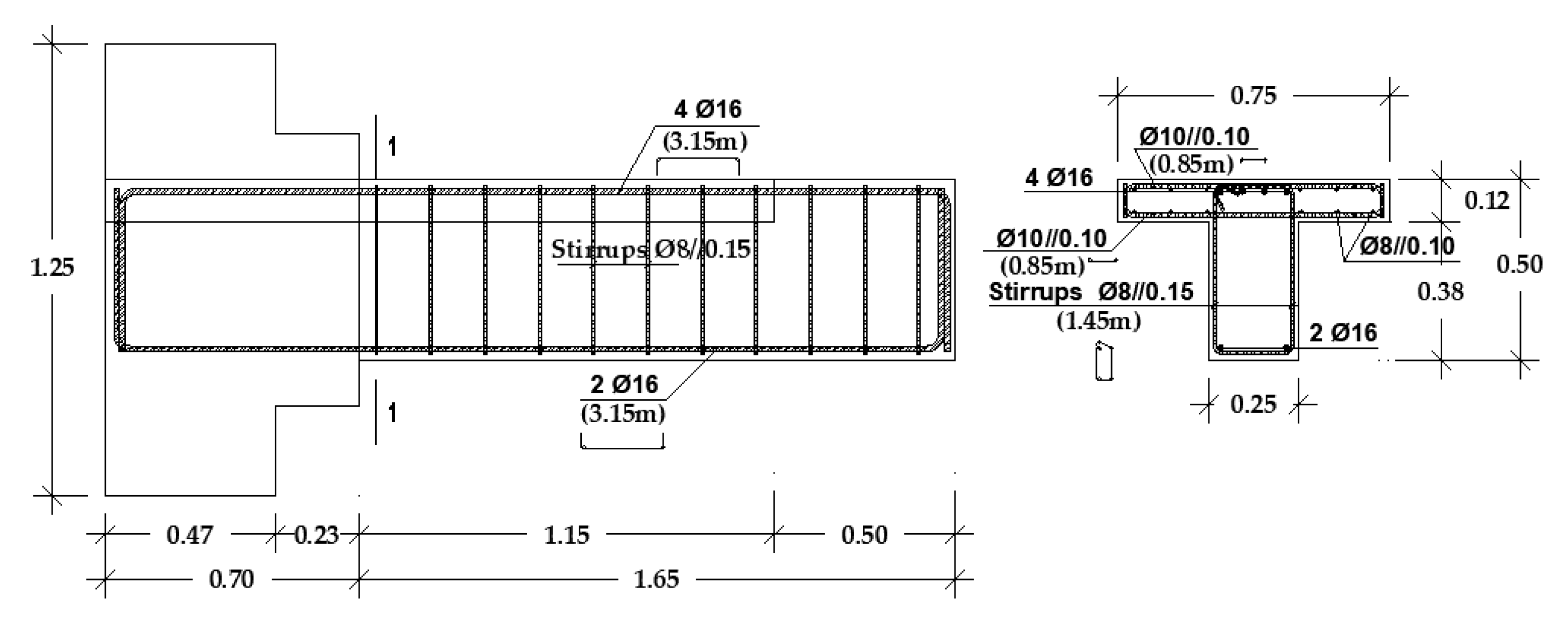
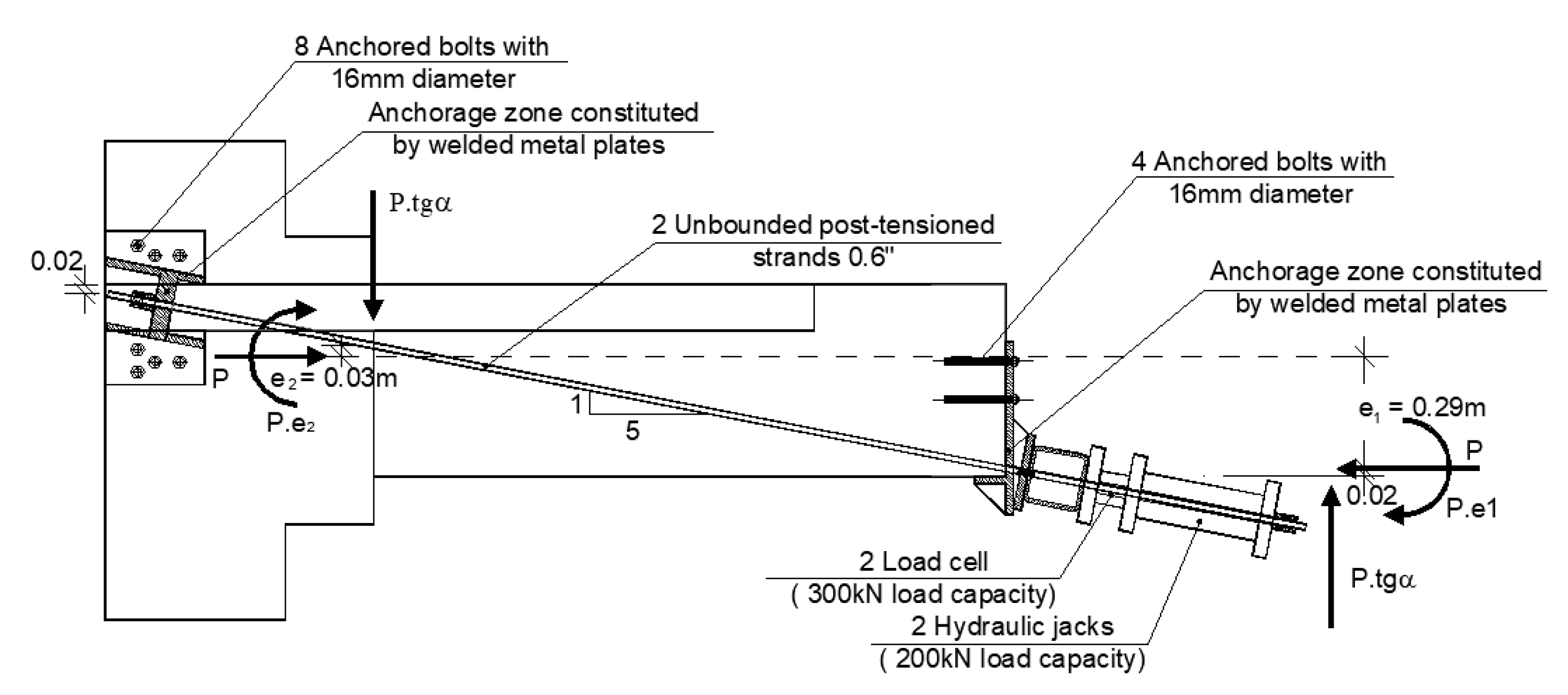
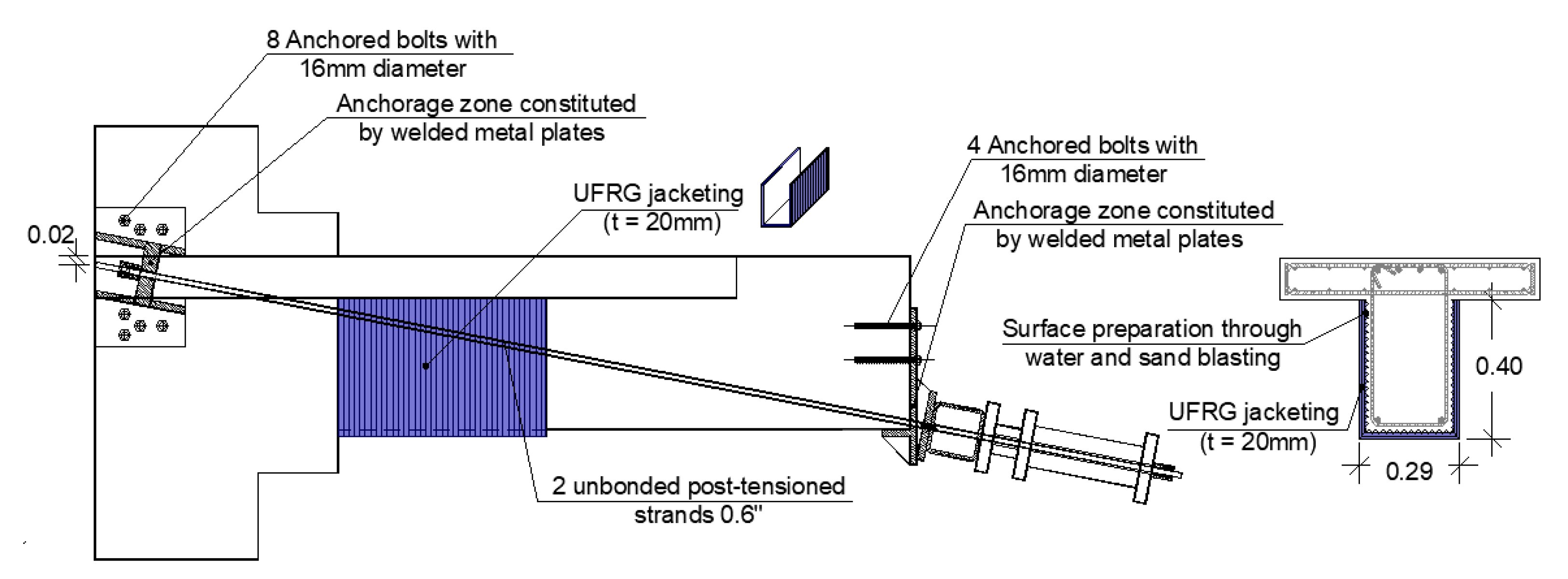
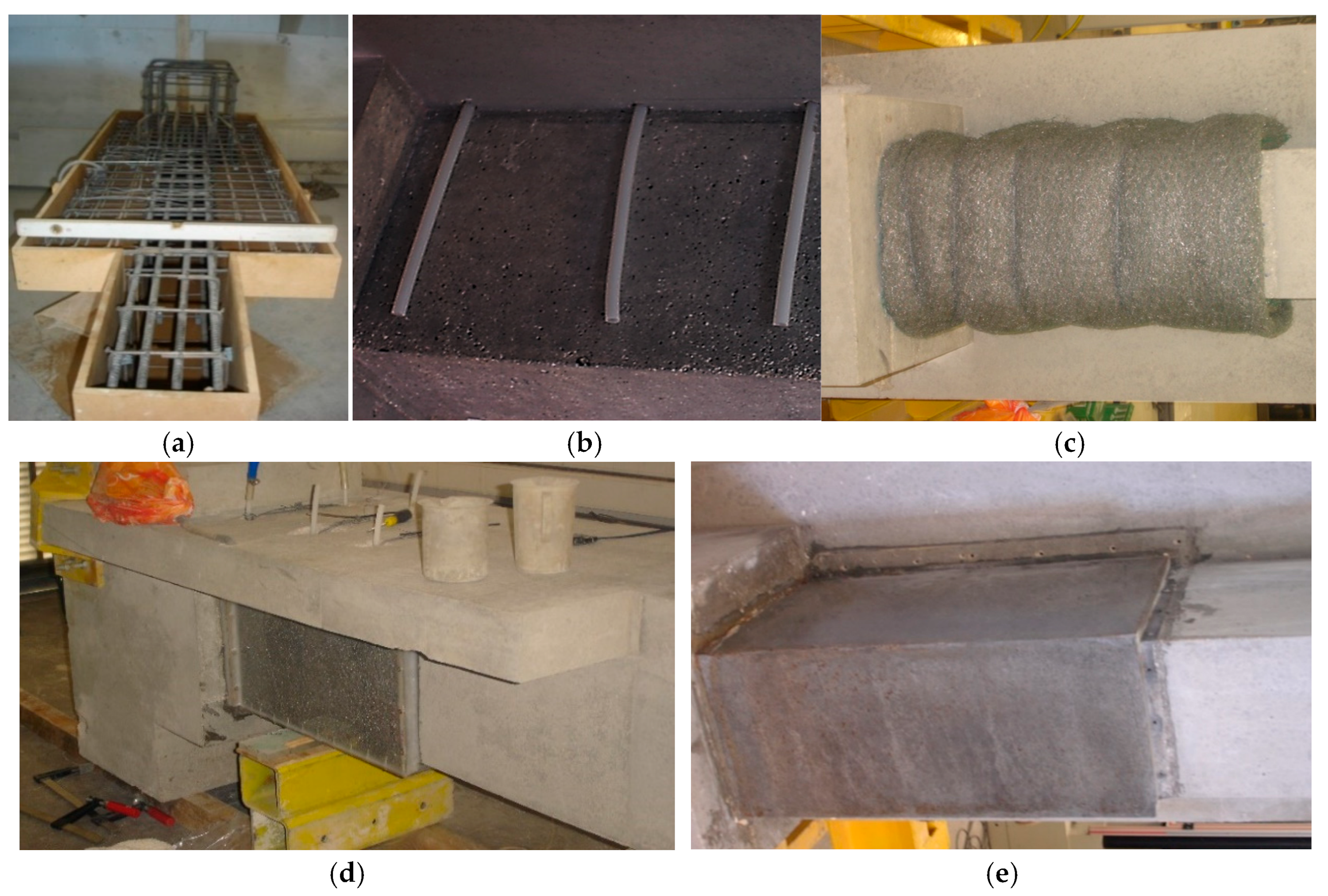

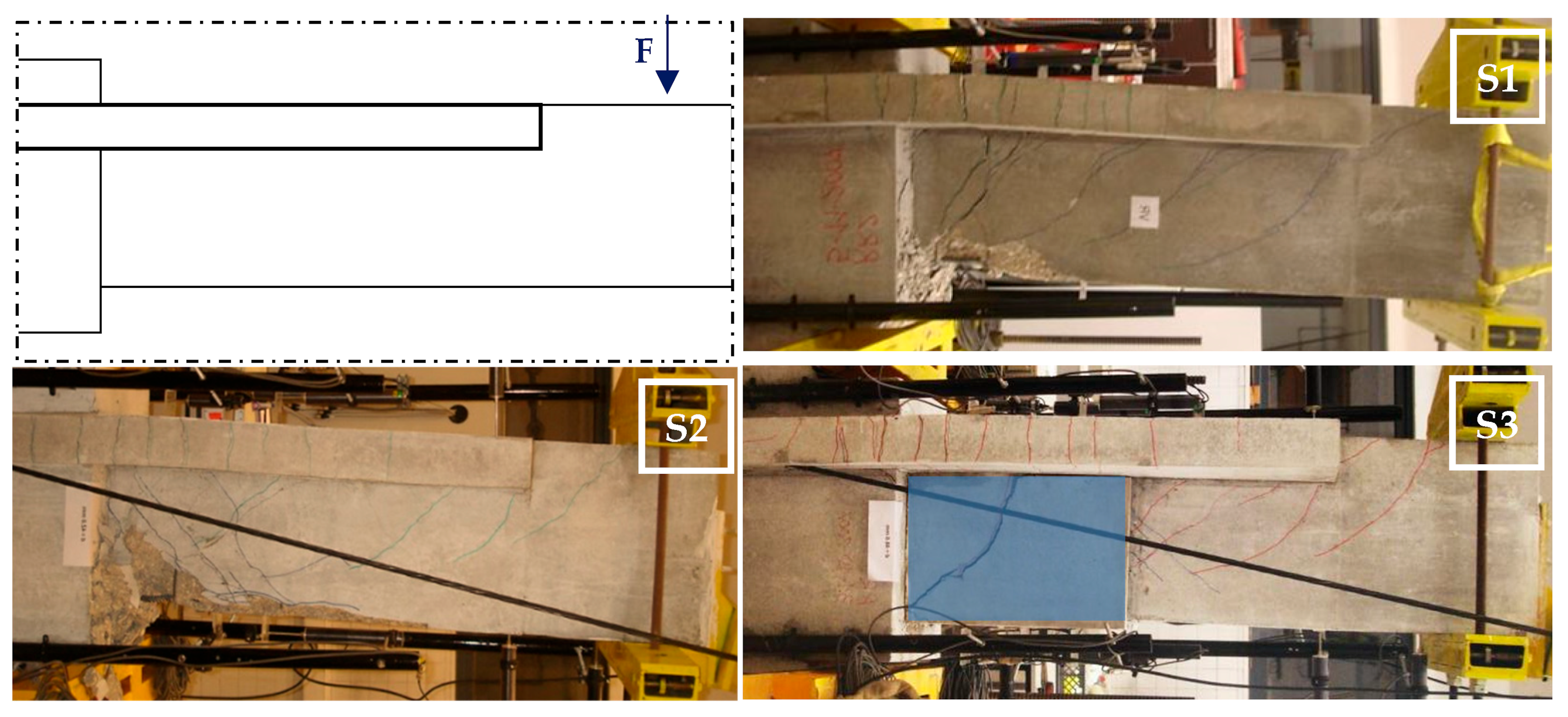






| Specimen | Description | Initial Post-Tensioning Force (kN) | UFRG Jacketing |
|---|---|---|---|
| S1 | Reference | - | - |
| S2 | Strengthened with external PT | 300 | - |
| S3 | Strengthened with UFRG jacketing + external PT | 300 | 20 mm thickness |
| Description | Fmax (kN) | Fmax/ FS1 | Displacement Ductility | Residual Deformation | Energy Dissipation | ||||
|---|---|---|---|---|---|---|---|---|---|
| µ− (*) | µ+ (*) | dr (mm) | dr/drS1 | W (kNm) | W/WS1 | ||||
| S1 | Reference | 212.50 | - | 10.40 | - | 126.20 | - | 28.60 | - |
| S2 | PT | 260.10 | 1.22 | 7.70 | 2.40 | 71.20 | 0.56 | 43.80 | 1.53 |
| S3 | PT + UFRG | 293.00 | 1.38 | 6.40 | 6.10 | 58.70 | 0.47 | 57.20 | 2.00 |
| x (m) | εc (‰) | My (kNm) | My, exp (kNm) | D (%) | |
|---|---|---|---|---|---|
| S1 | 0.15 | 1.10 | 277.80 | 303.30 | 8 |
| S2 | 0.18 | 1.50 | 353.70 | 375.30 | 6 |
| S3 | 0.17 | 1.10 | 384.60 | 413.60 | 7 |
| x (m) | εS (%) | Mmax (kNm) | Mmax,exp (kNm) | D (%) | |
|---|---|---|---|---|---|
| S1 | 0.07 | 1.90 | 311.70 | 318.80 | 2 |
| S2 | 0.10 | 1.20 | 392.30 | 390.20 | −1 |
| S3 | 0.08 | 2.40 | 425.00 | 439.50 | 3 |
| 1/ry (m−1) | 1/ru (m−1) | dy* (m) | dplast (m) | du (m) | θ = d/L (%) | θexp (%) | |
|---|---|---|---|---|---|---|---|
| S1 | 0.0075 | 0.155 | 0.005 | 0.060 | 0.065 | 4.4 | 8.0 (*) |
| S2 | 0.0084 | 0.171 | 0.006 | 0.066 | 0.072 | 4.8 | 5.3 |
| S3 | 0.0076 | 0.148 | 0.006 | 0.057 | 0.063 | 4.2 | 5.0 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Gião, R.; Lúcio, V.; Chastre, C. Innovative Seismic Strengthening Techniques to Be Used in RC Beams’ Critical Zones. Buildings 2023, 13, 95. https://doi.org/10.3390/buildings13010095
Gião R, Lúcio V, Chastre C. Innovative Seismic Strengthening Techniques to Be Used in RC Beams’ Critical Zones. Buildings. 2023; 13(1):95. https://doi.org/10.3390/buildings13010095
Chicago/Turabian StyleGião, Rita, Válter Lúcio, and Carlos Chastre. 2023. "Innovative Seismic Strengthening Techniques to Be Used in RC Beams’ Critical Zones" Buildings 13, no. 1: 95. https://doi.org/10.3390/buildings13010095
APA StyleGião, R., Lúcio, V., & Chastre, C. (2023). Innovative Seismic Strengthening Techniques to Be Used in RC Beams’ Critical Zones. Buildings, 13(1), 95. https://doi.org/10.3390/buildings13010095







