Bending Strength of Connection Joints of Prestressed Reinforced Concrete Pipe Piles
Abstract
1. Introduction
2. Specimens and Materials
2.1. Specimen Design
2.2. Material Properties
3. Methods
3.1. Loading Scheme of Tests
3.2. Numerical Simulation
3.2.1. Finite Element Model
3.2.2. Constitutive Models and Parameters
3.2.3. Contact Properties and Boundary Conditions
4. Results and Discussion
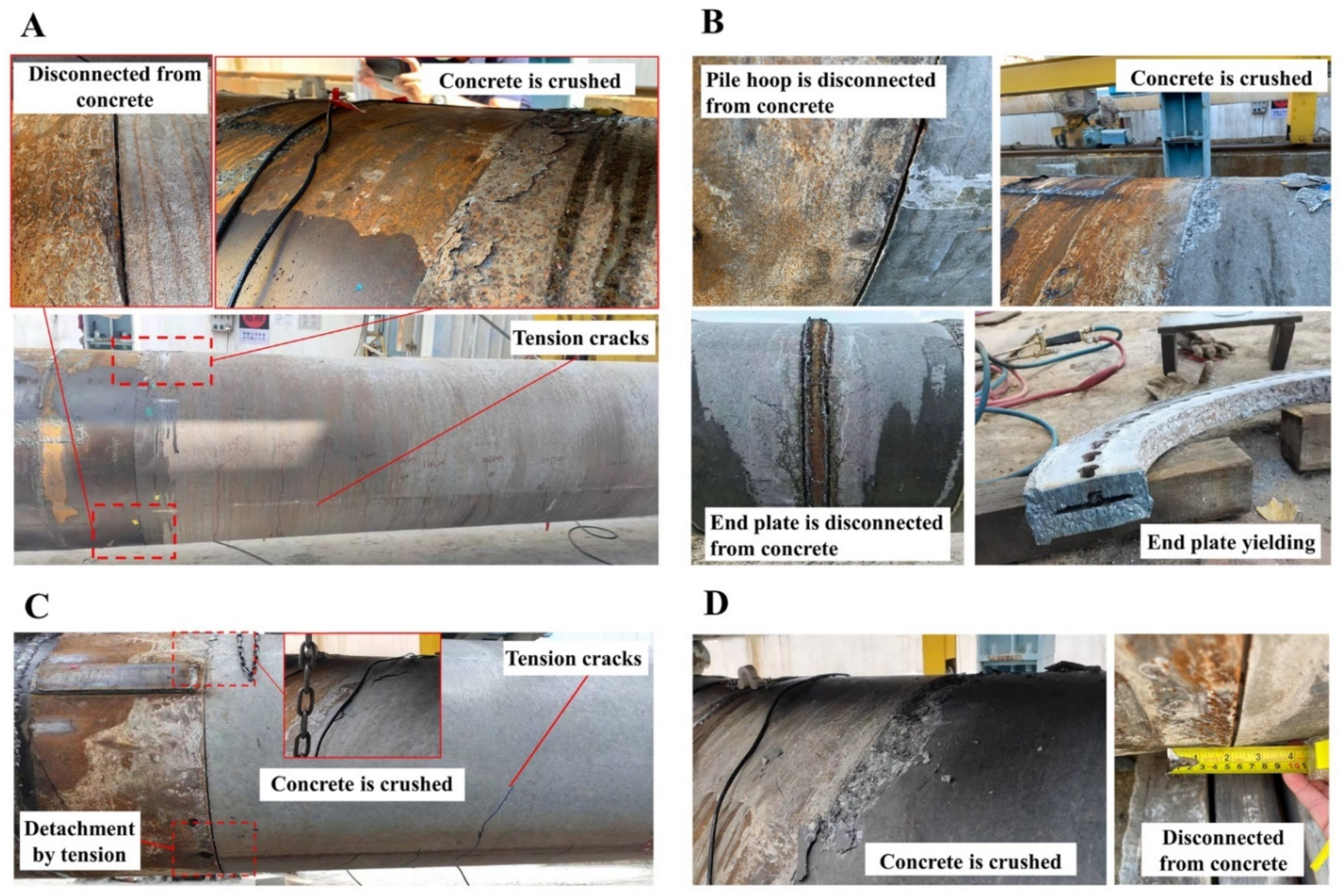
4.1. Failure Characteristics of Test Specimens
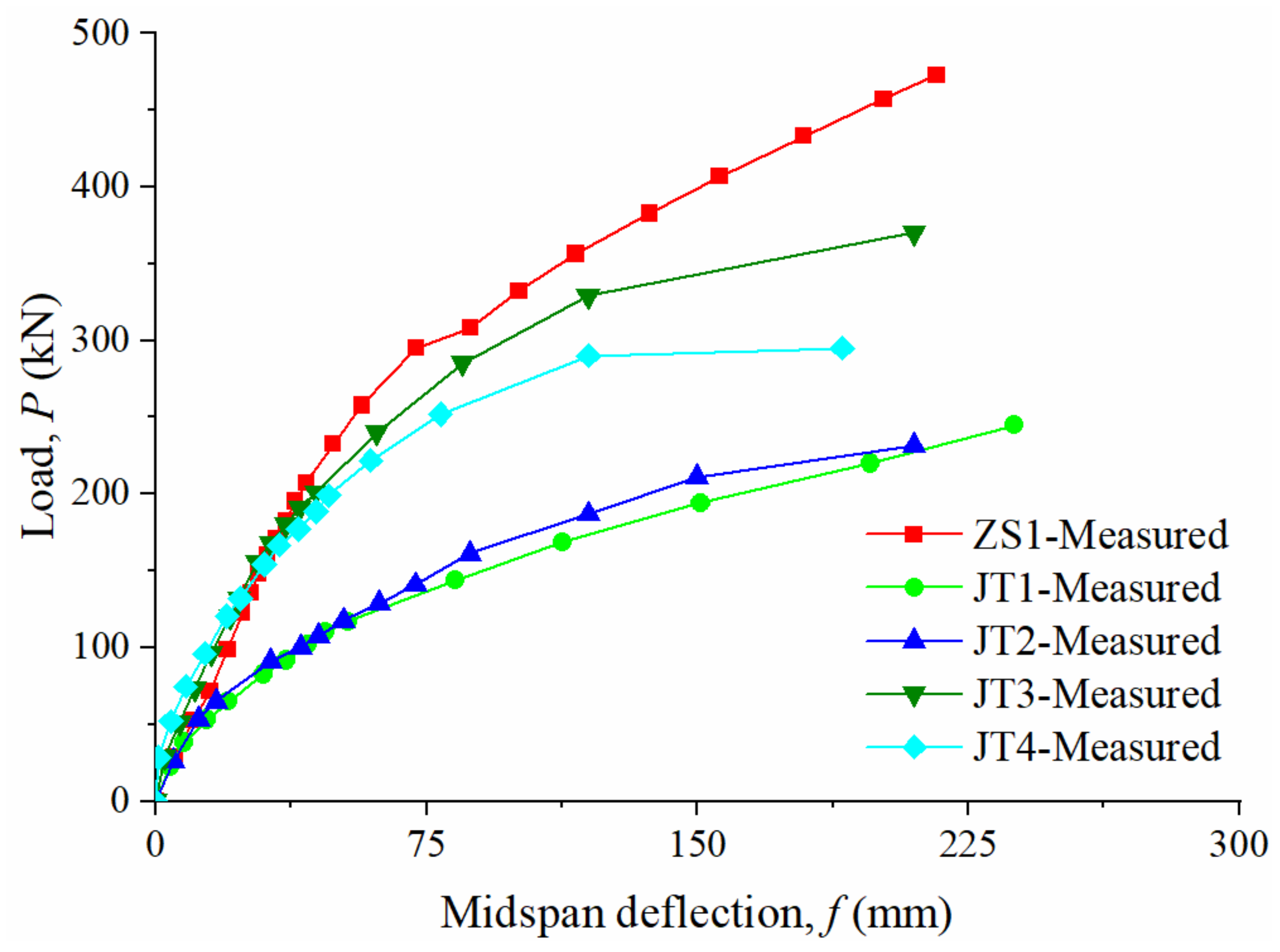
4.2. Bending Bearing Capacity
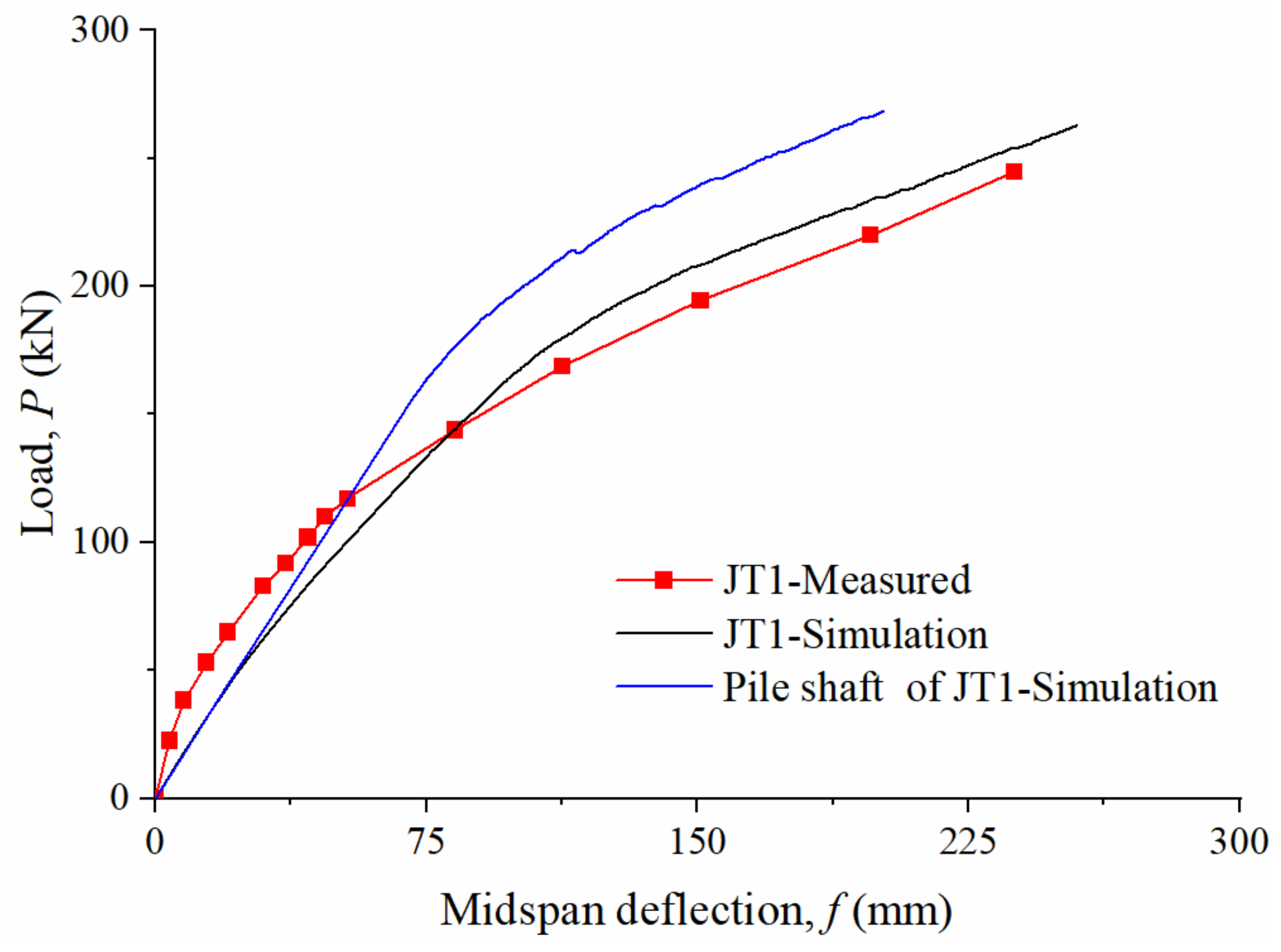
4.3. Numerical Model Validation
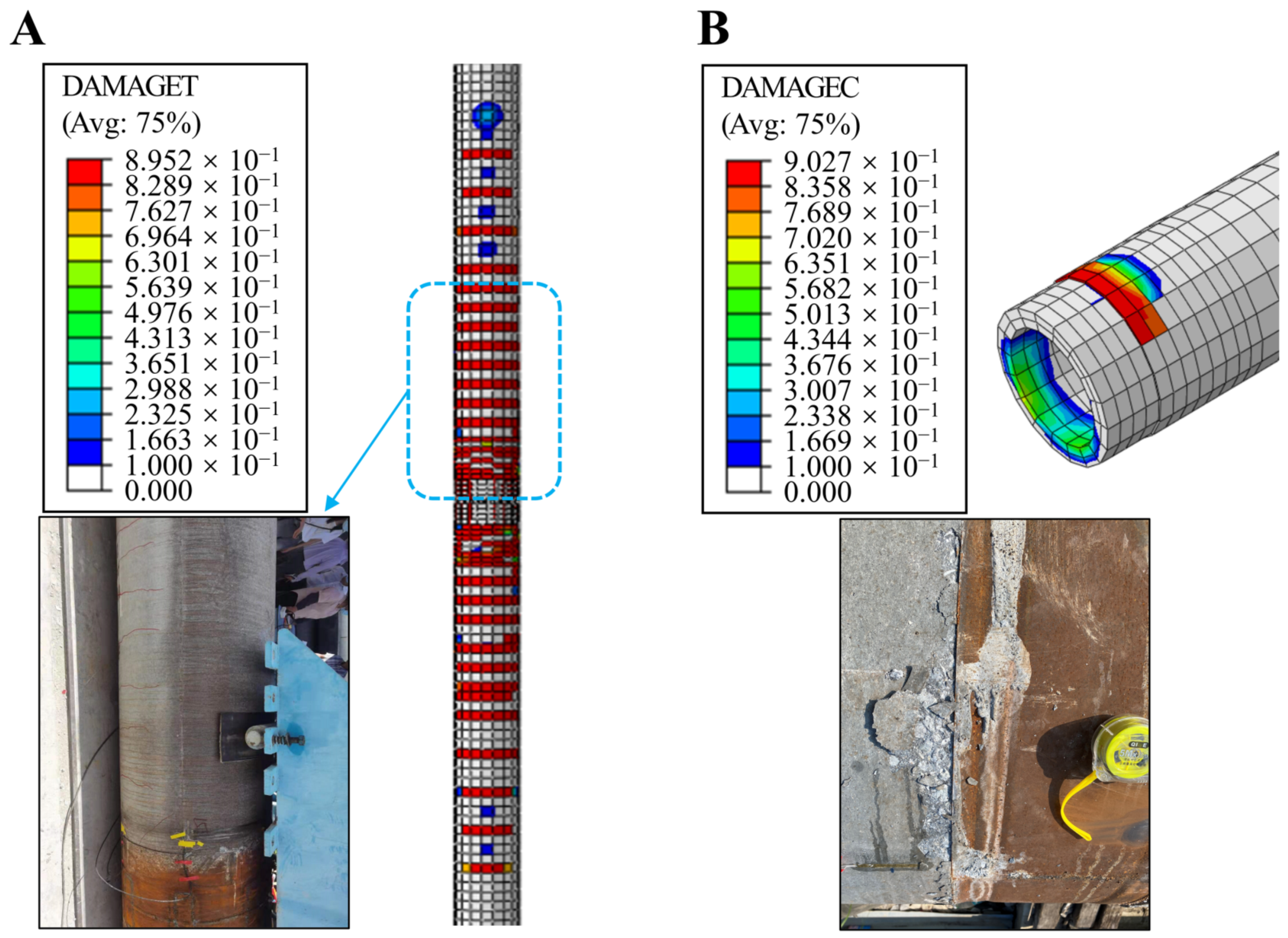
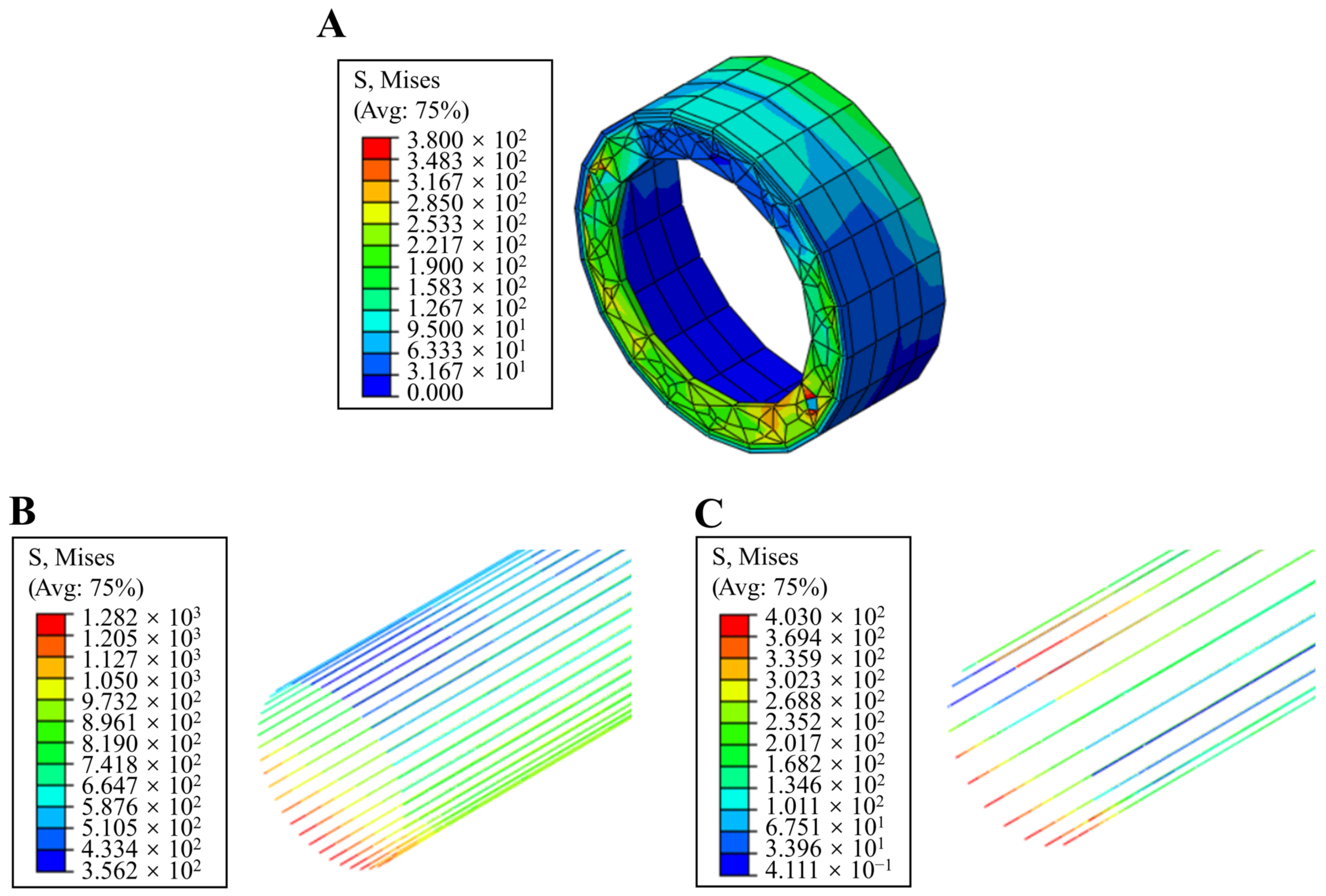
4.4. Mechanisms of Stress and Deformation of Connection Joints
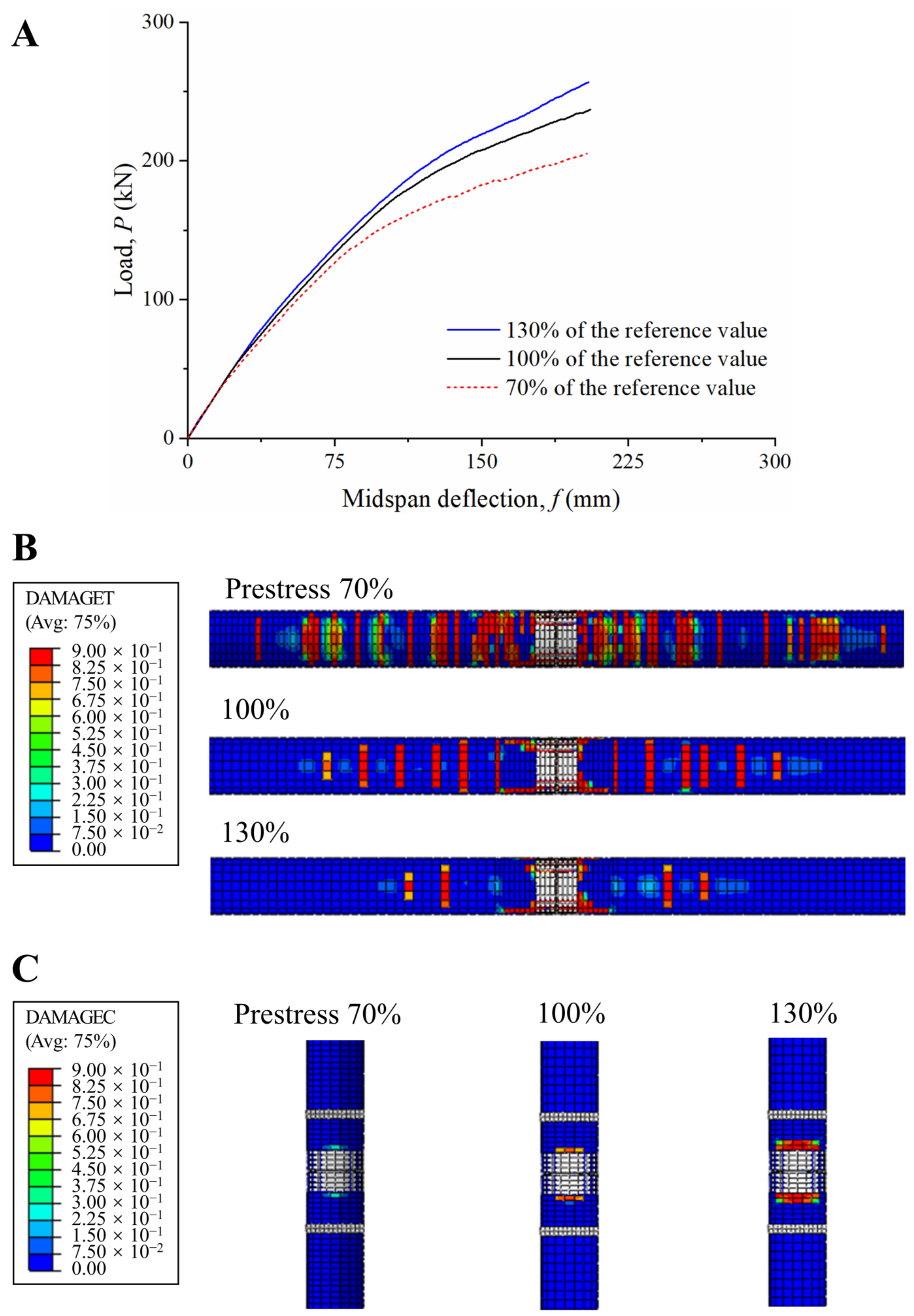
4.5. Influencing Factors on Bending Strength of Joints
4.5.1. Concrete Strength
4.5.2. Effective Precompression Stress of Concrete
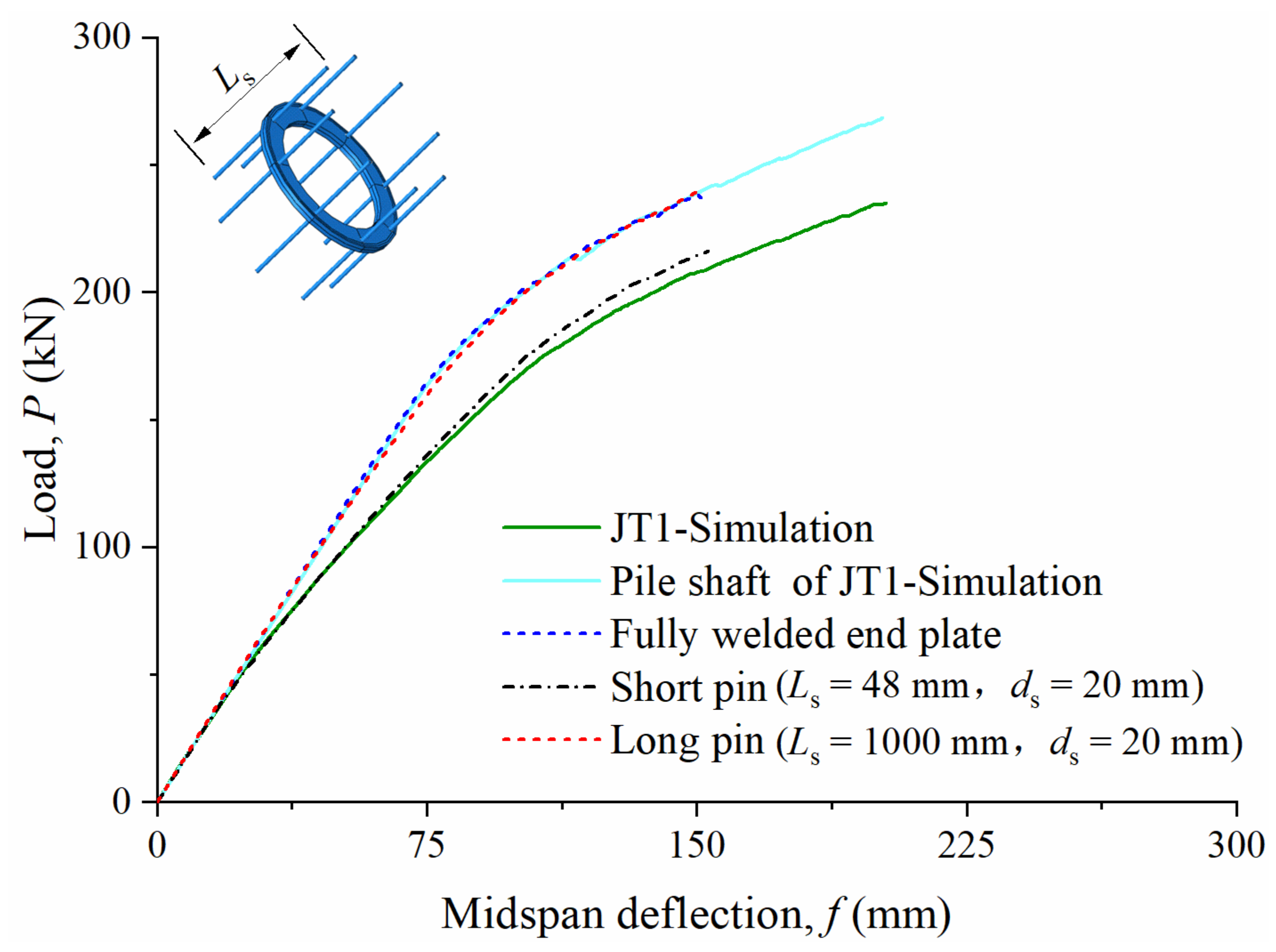
4.5.3. Joint Connection Mode
5. Conclusions
- (1)
- The crack resistance of PRC pipe pile welded joints was comparable to that of the pipe pile shaft, and it is safe and reasonable to adopt the crack moment of the pile without considering the effect of non-prestressed reinforcement as the design value of the joint bending moment of the pipe pile.
- (2)
- The bending strength of welded joint of PRC pipe pile was lower than that of the pile shaft. The main failure mode was that the tensile yield of the end plate was in the shape of a drum, the pile hoop and end plate were obviously separated from the pipe pile, and the concrete on the upper edge of the pile hoop was crushed.
- (3)
- The bending strength of the joint with fully welded end plates and long pin connection was close to that of the pile shaft. Rachel reinforcement connection implanted into concrete and filled with high-strength structural bar glue between end plates can be used to maintain the continuity of the joint section and force and improve the bending strength of the joint.
- (4)
- The bending capacity of the joint can be improved by increasing the strength grade and reinforcement ratio of concrete at the same time, or by strengthening the precompression stress of concrete. However, the comprehensive reinforcement ratio of PRC pipe pile with a pile diameter of 1000 mm and a wall thickness of 130 mm should not be more than 3.4%, otherwise brittle failure will occur easily.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Wu, Z.P.; Yang, X.D.; Bao, L. Application research of prestressed high strength concrete pipe pile in construction. Appl. Mech. Mater. 2012, 193–194, 1393–1396. [Google Scholar] [CrossRef]
- Zhao, S.; Huang, G.; Ma, S.; Zhou, W.; Zhang, X. Application of precast concrete pipe piles in a deep excavation project. Chin. J. Geotech. Eng. 2014, 36 (Suppl. S1), 91–96. [Google Scholar] [CrossRef]
- Gao, W.S.; Liu, J.L.; Zhao, X.G.; Qiu, M.B. Some understanding of prestressed concrete pipe pile in engineering application. Rock Soil Mech. 2015, 36 (Suppl. S2), 610–616. [Google Scholar] [CrossRef]
- Du, X.X.; Hu, R.; Yuan, H.X.; Cheng, X.Y.; Lin, C. Study on flexural behavior of prestressed concrete pipe pile with hybrid reinforcement. China Civ. Eng. J. 2019, 52, 44–52. [Google Scholar] [CrossRef]
- Ren, J.W.; Xu, Q.B.; Chen, G.; Yu, X.D.; Gong, S.F.; Lu, Y. Full-scale experimental study of the seismic performance of pretensioned spun high-strength concrete piles. Soil Dyn. Earthq. Eng. 2022, 162, 107467. [Google Scholar] [CrossRef]
- CABR (China Academy of Building Research). JGJ/T 406-2017 Technical Standard for Prestressed Concrete Pipe Pile; China Architecture & Building Press: Beijing, China, 2017. [Google Scholar]
- Liu, F.R.; Jia, L.; Li, C. The test study on welding joint flexural bearing capacity of prestressed concrete hollow square pile. J. Wuhan Univ. Technol. 2008, 30, 105–108. [Google Scholar] [CrossRef]
- Huang, G.L.; Yan, R.H.; Zhou, W.Y.; Chen, Q. Experimental study on joint flexural bearing capacity of prestressed concrete pipe piles. J. Jiangsu Univ. Technol. 2011, 25, 209–212. [Google Scholar]
- Li, Z.Y.; Ke, Z.B.; Liu, D.J.; Lu, Z.T.; Guo, Y. Tests on PHC pipe pile joint quality by low-strain reflected wave method. Chin. J. Geotech. Eng. 2011, 33 (Suppl. S2), 209–212. [Google Scholar]
- Chen, C.; Yang, L.D. Experimental research on concrete permeability of underground structure. Rock Soil Mech. 2011, 32, 2379–2385. [Google Scholar] [CrossRef]
- Huang, F.M.; Cao, Z.S.; Jiang, S.H.; Zou, G.B.; Guo, Z. Landslide susceptibility prediction based on a semi-supervised multiple-layer perceptron model. Landslides 2020, 17, 2919–2930. [Google Scholar] [CrossRef]
- Huang, F.M.; Cao, Z.S.; Guo, J.F.; Jiang, S.H.; Guo, Z. Comparisons of heuristic, general statistical and machine learning models for landslide susceptibility prediction and mapping. CATENA 2020, 191, 104580. [Google Scholar] [CrossRef]
- Chang, Z.; Du, Z.; Zhang, F.; Huang, F.; Chen, J.; Li, W.; Guo, Z. Landslide susceptibility prediction based on remote sensing images and GIS: Comparisons of supervised and unsupervised machine learning models. Remote Sens. 2020, 12, 502. [Google Scholar] [CrossRef]
- Xu, Q.B.; Chen, G.; He, J.F.; Gong, S.F.; Xiao, Z.B. Flexural performance experiment of connection joint for composite reinforcement concrete prefabricated square piles. J. Zhejiang Univ. Eng. Sci. 2017, 51, 1300–1308. [Google Scholar] [CrossRef]
- Zhou, J.W.; Wang, Y.F.; Gong, S.F.; Zhang, A.H.; Liu, C.B.; Fan, H. Study on flexural behavior of prestressed concrete square pile connection joint with resilient clamping. Build. Struct. 2020, 50, 121–127. [Google Scholar] [CrossRef]
- Qi, J.L.; Gong, S.F.; Zhou, Z.D.; Liu, Y.S. Study on flexural behavior of prestressed concrete special-shaped square pile connection joint with bolt-shackle. Build. Struct. 2021, 51, 105–109. [Google Scholar] [CrossRef]
- Wang, B.; Qi, L.; Yang, Y.D. Experimental study on bending resistance of new type joint of prestressed concrete pipe pile. Symmetry 2022, 14, 1920. [Google Scholar] [CrossRef]
- Huang, F.M.; Zhang, J.; Zhou, C.B.; Wang, Y.H.; Huang, J.S.; Zhu, L. A deep learning algorithm using a fully connected sparse autoencoder neural network for landslide susceptibility prediction. Landslides 2020, 17, 217–229. [Google Scholar] [CrossRef]
- Jiang, S.H.; Huang, J.S.; Huang, F.M.; Yang, J.H.; Yao, C.; Zhou, C.B. Modelling of spatial variability of soil undrained shear strength by conditional random fields for slope reliability analysis. Appl. Math. Model. 2018, 63, 374–389. [Google Scholar] [CrossRef]
- Tang, M.X.; Ling, Z.; Liu, C.L.; Hu, H.S. Influence of pile toe type on bearing capacity of rock-socketed DPC piles. Chin. J. Rock Mech. Eng. 2022, 41 (Suppl. S1), 3053–3062. [Google Scholar] [CrossRef]
- Chen, G.; Zhou, Q.H.; Xu, Q.B.; Gong, S.F.; Xiao, Z.B.; Liu, C.B. Study on flexural performance of prestressed steel strand reinforced ultra-high strength concrete pipe piles. J. Build. Struct. 2019, 40, 173–182. [Google Scholar] [CrossRef]
- Ling, Z.; Wu, J.B.; Wang, W.D. Numerical analysis of bearing behavior of the prebored precast pile with an enlarged base. Adv. Civ. Eng. 2021, 2021, 1505482. [Google Scholar] [CrossRef]
- Liu, H.L.; Kong, G.Q.; Ding, X.M.; Chen, Y.M. Performances of large-diameter cast-in-place concrete pipe piles and pile groups under lateral loads. J. Perform. Constr. Facil. 2013, 27, 191–202. [Google Scholar] [CrossRef]
- Wang, A.; Zhang, D.; Deng, Y. Lateral response of single piles in cement-improved soil: Numerical and theoretical investigation. Comput. Geotech. 2018, 102, 164–178. [Google Scholar] [CrossRef]
- Wei, D.M.; Wang, P. Investigation on corrosion of welded joint of prestressed high-strength concrete pipe piles. Procedia Eng. 2017, 210, 79–86. [Google Scholar] [CrossRef]
- Lu, L.; Han, S.; Chen, Z.; Wang, G.; Wang, Y.; Zhao, K. Study on bending performance of prefabricated square pile with socket and spigot joint. J. Build. Struct. 2018, 39, 153–161. [Google Scholar] [CrossRef]
- CBMF (China Building Materials Federation). GB 13476-2009 Pretensioned Spun Concrete Piles; Standards Press of China: Beijing, China, 2009. [Google Scholar]
- CABR (China Academy of Building Research). GB 50010-2010 Code for Design of Concrete Structures (2015 Edition); China Architecture & Building Press: Beijing, China, 2015. [Google Scholar]
- Sun, X.F.; Fang, X.S.; Guan, L.T. Mechanics of Materials, 5th ed.; Higher Education Press: Beijing, China, 2009. [Google Scholar]
- Wahalathantri, B.L.; Thambiratnam, D.P.; Chan, T.H.T.; Fawzia, S. A material model for flexural crack simulation in reinforced concrete elements using ABAQUS. In Proceedings of the First International Conference on Engineering, Designing and Developing the Built Environment for Sustainable Wellbeing, Queensland University of Technology, Brisbane, Australia, 28 April 2011; pp. 260–264. [Google Scholar]
- Liang, J.H.; Fan, Q.Y.; Qin, K. Influence of Karst Caves on the Pile’s Bearing Characteristics-A Numerical Study. Front. Earth Sci. 2022, 9, 754330. [Google Scholar] [CrossRef]
- Mercan, B.; Schultz, A.E.; Stolarski, H.K. Finite element modeling of prestressed concrete spandrel beams. Eng. Struct. 2010, 32, 2804–2813. [Google Scholar] [CrossRef]
- Nguyen, T.H.A.; Bui, T.Q.; Hirose, S. Smoothing gradient damage model with evolving anisotropic nonlocal interactions tailored to low-order finite elements. Comput. Methods Appl. Mech. Eng. 2018, 328, 498–541. [Google Scholar] [CrossRef]
- Yuen, T.Y.; Wen, T.-H.; Hung, C.-C.; Zhang, H.; Pham, P.A.H.; Deng, Y. An eigendecomposition-based and mesh-sensitivity reduced constitutive model for nonlinear analysis of concrete structures under non-proportional cyclic loading. J. Build. Eng. 2022, 47, 103875. [Google Scholar] [CrossRef]
- Lubliner, J.; Oliver, J.; Oller, S.; Onate, E. A plastic-damage model for concrete. Int. J. Solids Struct. 1989, 25, 299–326. [Google Scholar] [CrossRef]
- Lee, J.; Fenves, G.L. A plastic-damage model for cyclic loading of concrete structures. J. Eng. Mech. 1998, 124, 892–900. [Google Scholar] [CrossRef]
- Lee, J.; Fenves, G.L. A plastic-damage concrete model for earthquake analysis of dams. Earthq. Eng. Struct. Dyn. 1998, 27, 937–956. [Google Scholar] [CrossRef]
- Abaqus. Analysis User’s Manual, Version 6.14; Dassault Systemes Simulia Corp.: Providence, RI, USA, 2016. [Google Scholar]
- Yang, Z.J.; Lei, Y.Q. Finite element analysis of the shear behavior of prestressed high-strength concrete piles. Eng. Mech. 2020, 37 (Suppl. S1), 200–207. [Google Scholar] [CrossRef]
- Hu, R. Research on Shear and Flexural Behaviour of Prestressed Concrete Pipe Pile with Hybrid Reinforcement; Wuhan University: Hubei, China, 2018. [Google Scholar]
- He, L.; Wang, J.L. Method of equivalent load and temperature reduction on prestressing tendon for effective prestress simulation. J. Highw. Transp. Res. Dev. 2015, 32, 75–80. [Google Scholar] [CrossRef]
- Tang, G.Z.; Wang, Q.; Yu, Q. Discussion on calculating method of cracking resistance and ultimate bending moment of pretensioned spun concrete piles. Ind. Constr. 2004, 34, 57–59. [Google Scholar] [CrossRef]
- Huang, G.L.; Yan, R.H.; Zhou, F.; Mei, G.X. Experimental study and discussion on the flexural capacity of prestressed high strength concrete supporting piles. Build. Struct. 2012, 42, 113–116. [Google Scholar] [CrossRef]
- Huang, F.Y.; Wu, S.W.; Luo, X.Y.; Chen, B.C.; Lin, Y.W. Pseudo-static low cycle test on the mechanical behavior of PHC pipe piles with consideration of soil-pile interaction. Eng. Struct. 2018, 171, 992–1006. [Google Scholar] [CrossRef]
- Liu, Y.; Tan, Y.S. Studies on longitudinal reinforcement ratio of flexural RC member. Archit. Technol. 2006, 40, 389–390. [Google Scholar] [CrossRef]
- Yu, P. Experimental Study on the Influence of Hoop or Clamp Joint on the Mechanical Behavior of PRC Pipe Pil; Zhengzhou University: Zhengzhou, China, 2021. [Google Scholar]
- Guo, Y.B.; Gao, G.F.; Jing, L.; Shim, V.P.W. Response of high-strength concrete to dynamic compressive loading. Int. J. Impact Eng. 2017, 108, 114–135. [Google Scholar] [CrossRef]
- Minh, H.-L.; Khatir, S.; Wahab, M.A.; Cuong-Le, T. A concrete damage plasticity model for predicting the effects of compressive high-strength concrete under static and dynamic loads. J. Build. Eng. 2021, 44, 103239. [Google Scholar] [CrossRef]
- Ptuhina, I.; Alzhanova, R.; Akhatuly, A.; Maier, V. Comparative Analysis of Pile Joints. Adv. Mater. Res. 2014, 1082, 270–276. [Google Scholar] [CrossRef]
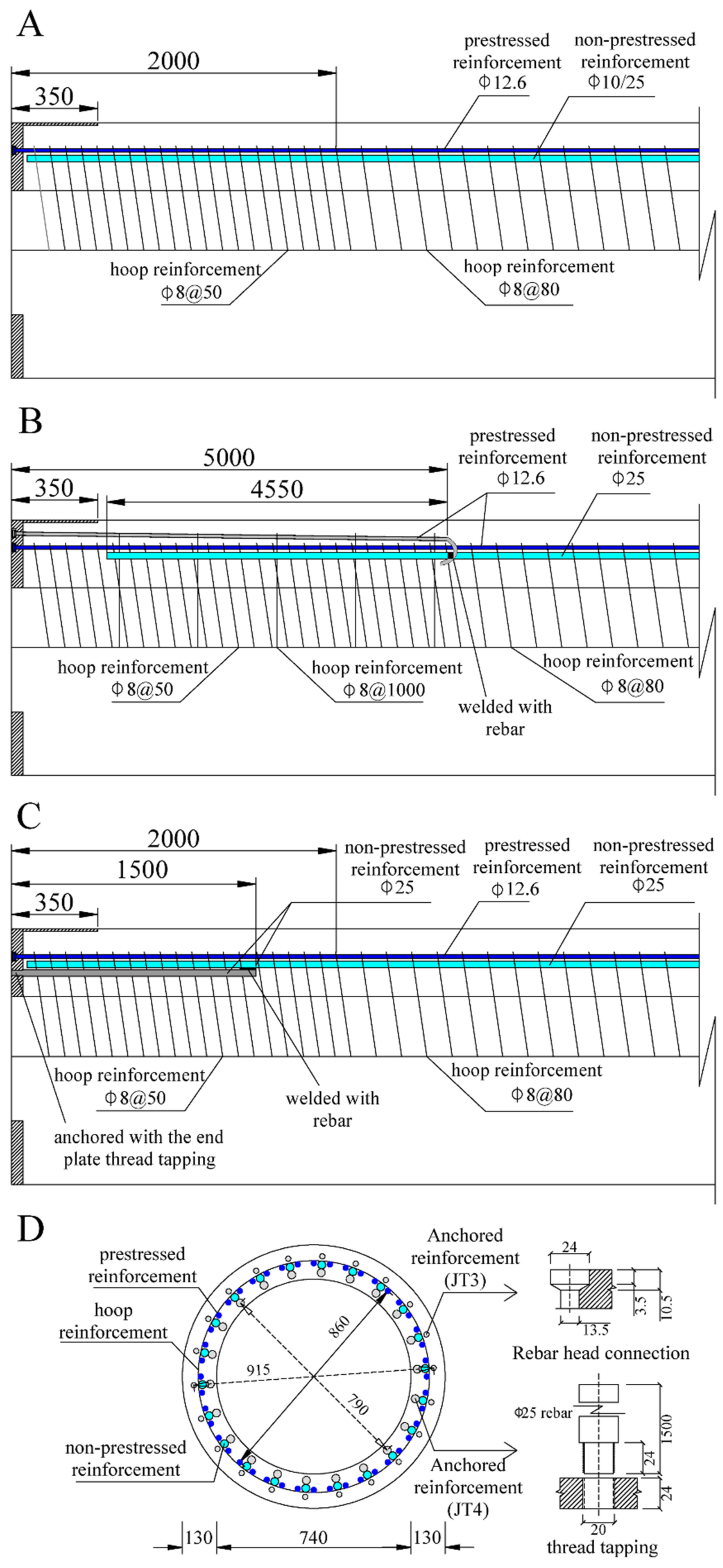

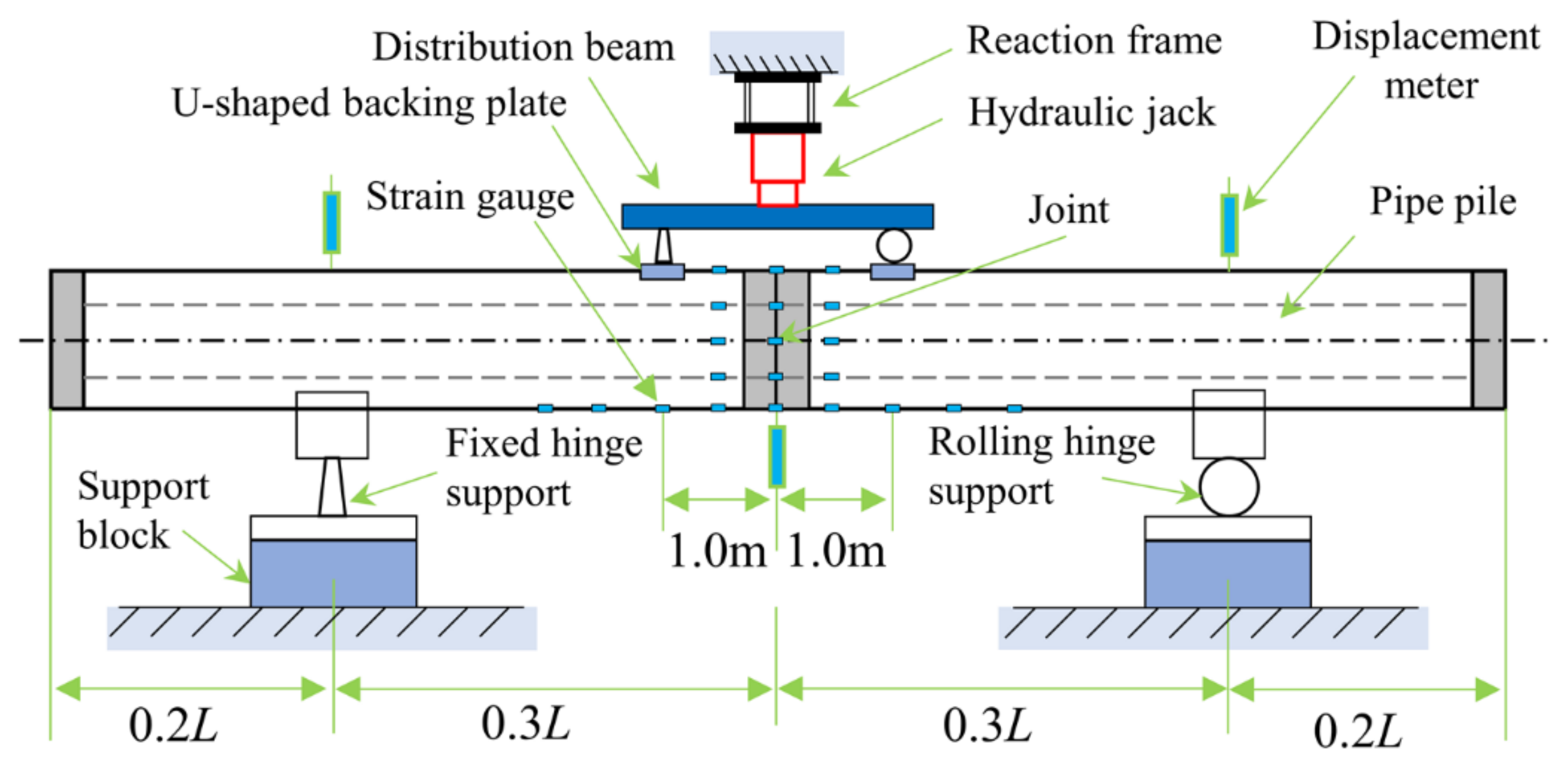
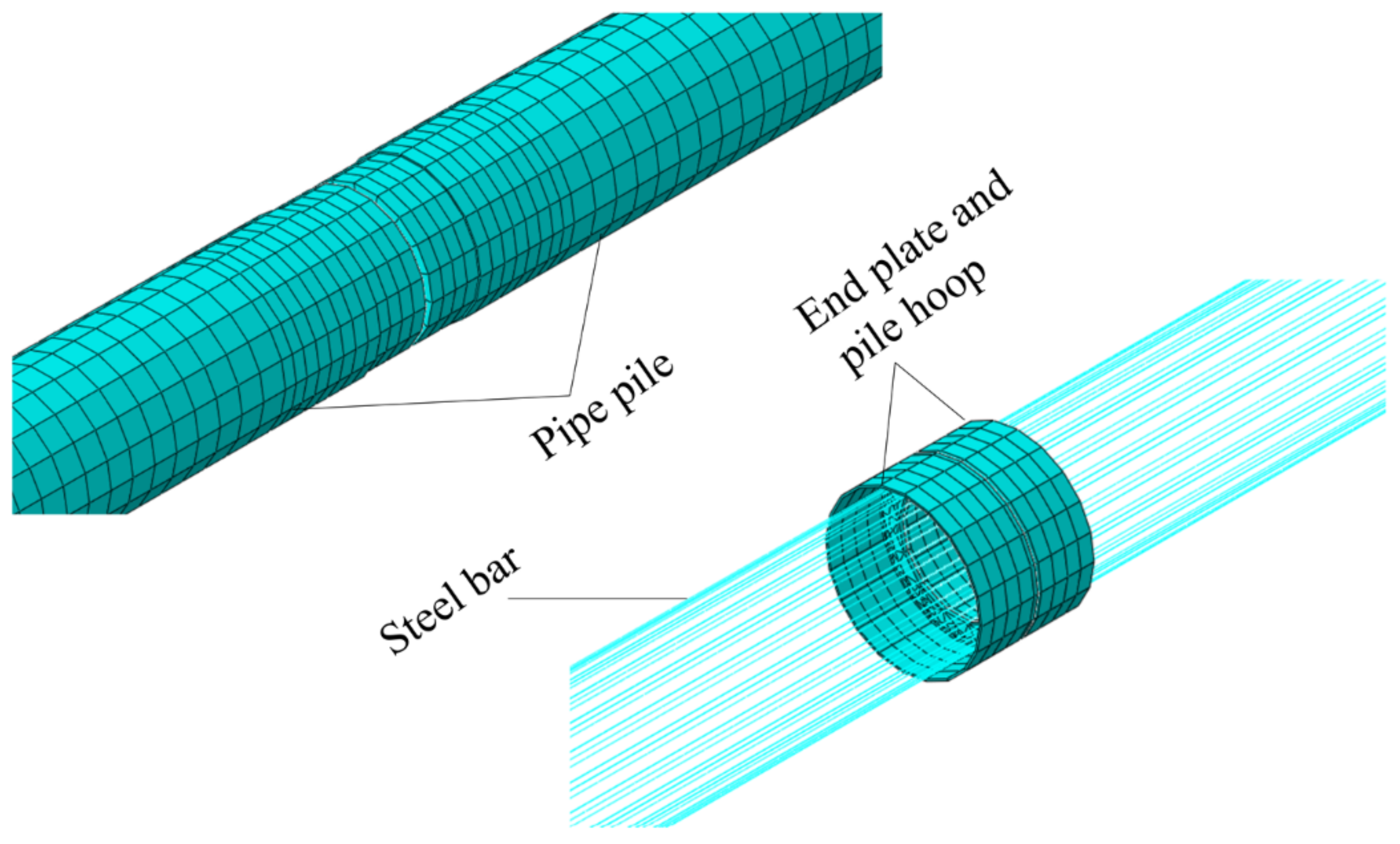
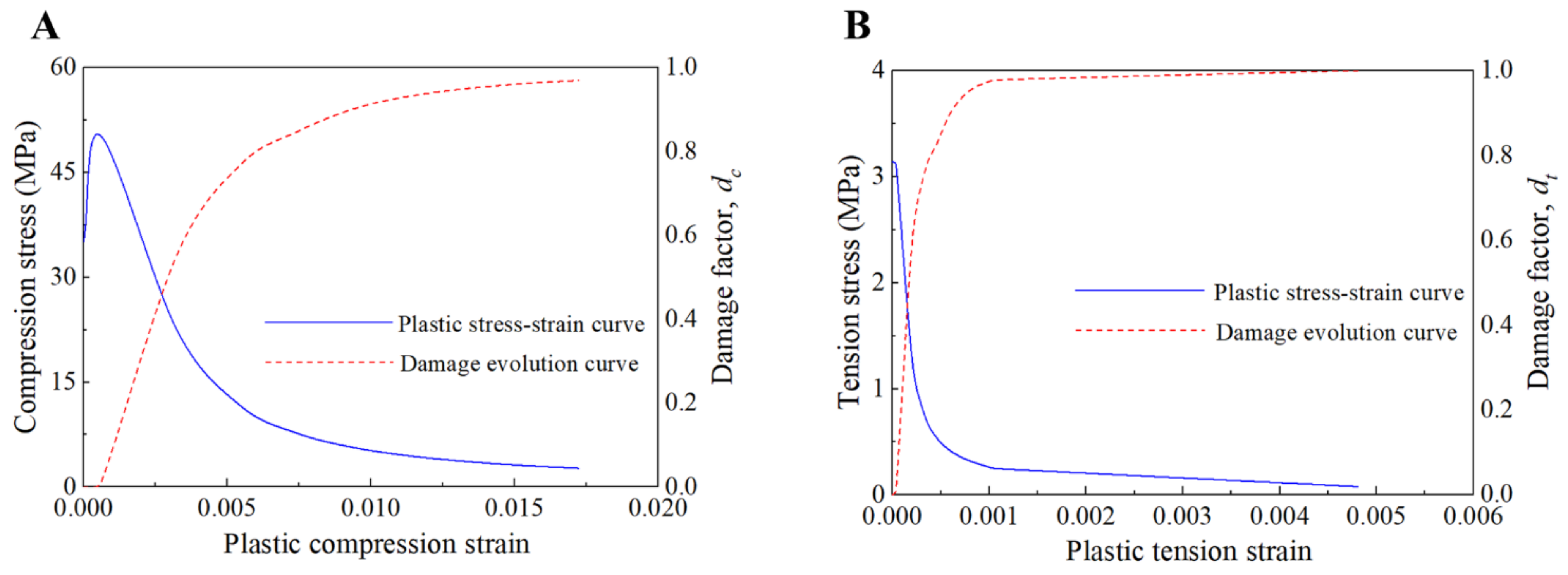



| Test Sample | Pile Length (m) | Prestressed Reinforcement | Non-Prestressed Reinforcement | Hoop Reinforcement | Anchored Reinforcement | (%) |
|---|---|---|---|---|---|---|
| ZS1 | 40 | 44Φ12.6 | 22Φ10 | Φ8 | / | 2.0 |
| JT1 | 26 + 26 | 44Φ12.6 | 22Φ10 | Φ8 | / | 2.0 |
| JT2 | 26 + 26 | 44Φ12.6 | 22Φ25 | Φ8 | / | 4.6 |
| JT3 | 20 + 20 | 44Φ12.6 | 22Φ25 | Φ8 | 22Φ12.6 | 2.3–5.4 |
| JT4 | 20 + 20 | 44Φ12.6 | 22Φ25 | Φ8 | 22Φ25 | 7.6 |
| Material | Density (kg/m3) | Elastic Modulus E (GPa) | Poisson’s Ratio | Yield Strength fy (MPa) | Compression Strength fc (MPa) | Tensile Strength ft (MPa) |
|---|---|---|---|---|---|---|
| Prestressed steel rods | 7800 | 200 | 0.30 | 1280 | / | 1420 |
| Non-prestressed reinforcements | 7800 | 200 | 0.30 | 400 | / | 540 |
| End plate | 7800 | 200 | 0.30 | 225 | / | 400 |
| Pile hoop | 7800 | 200 | 0.30 | 345 | / | 470 |
| C60 concrete | 2400 | 38 | 0.20 | / | 50.2 | 3.11 |
| C70 concrete | 2400 | 37 | 0.20 | / | 44.5 | 2.99 |
| C80 concrete | 2400 | 36 | 0.20 | / | 38.5 | 2.85 |
| Test Sample | Mcr (kN·m) | Mu (kN·m) | M’cr (kN·m) | M’u (kN·m) | M’cr/Mcr | M’u/Mcr | M’u/Mu |
|---|---|---|---|---|---|---|---|
| ZS1 | 1285 | 2760 | 1503 | 2958 | 1.17 | 2.30 | 1.07 |
| JT1 | 1285 | 2760 | 1478 | 2400 | 1.15 | 1.87 | 0.87 |
| JT2 | 1285 | 3640 | 1542 | 2298 | 1.20 | 1.79 | 0.63 |
| JT3 | 1285 | 3675 | 1565 | 2506 | 1.22 | 1.95 | 0.68 |
| JT4 | 1285 | 3640 | 1576 | 2093 | 1.23 | 1.63 | 0.58 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Tang, M.; Ling, Z.; Qi, Y. Bending Strength of Connection Joints of Prestressed Reinforced Concrete Pipe Piles. Buildings 2023, 13, 119. https://doi.org/10.3390/buildings13010119
Tang M, Ling Z, Qi Y. Bending Strength of Connection Joints of Prestressed Reinforced Concrete Pipe Piles. Buildings. 2023; 13(1):119. https://doi.org/10.3390/buildings13010119
Chicago/Turabian StyleTang, Mengxiong, Zao Ling, and Yuliang Qi. 2023. "Bending Strength of Connection Joints of Prestressed Reinforced Concrete Pipe Piles" Buildings 13, no. 1: 119. https://doi.org/10.3390/buildings13010119
APA StyleTang, M., Ling, Z., & Qi, Y. (2023). Bending Strength of Connection Joints of Prestressed Reinforced Concrete Pipe Piles. Buildings, 13(1), 119. https://doi.org/10.3390/buildings13010119






